Resolving the conundrum of inbreeding depression but no inbreeding avoidance: Estimating sex‐specific selection on inbreeding by song sparrows (Melospiza melodia)

Abstract

Inbreeding avoidance among interacting females and males is not always observed despite inbreeding depression in offspring fitness, creating an apparent “inbreeding paradox.” This paradox could be resolved if selection against inbreeding was in fact weak, despite inbreeding depression. However, the net magnitude and direction of selection on the degree to which females and males inbreed by pairing with relatives has not been explicitly estimated. We used long‐term pedigree data to estimate phenotypic selection gradients on the degree of inbreeding that female and male song sparrows (Melospiza melodia) expressed by forming socially persistent breeding pairs with relatives. Fitness was measured as the total numbers of offspring and grand offspring contributed to the population, and as corresponding expected numbers of identical‐by‐descent allele copies, thereby accounting for variation in offspring survival, reproduction, and relatedness associated with variation in parental inbreeding. Estimated selection gradients on the degree to which individuals paired with relatives were weakly positive in females, but negative in males that formed at least one socially persistent pairing. However, males that paired had higher mean fitness than males that remained socially unpaired. These analyses suggest that net selection against inbreeding may be weak in both sexes despite strong inbreeding depression, thereby resolving the “inbreeding paradox.”

Mating decisions enacted by individual organisms fundamentally shape the course of evolution because they shape social and reproductive interactions and influence the frequencies of alleles and genotypes contributed to subsequent generations, thereby driving and reinforcing social and sexual selection (e.g., Kirkpatrick and Barton 1996; Wolf et al. 1999; Kokko et al. 2003; Weir et al. 2011; Lyon and Montgomerie 2012). One influential mating decision is whether to inbreed, either by self‐fertilization (Jarne and Charlesworth 1993; Charlesworth 2006) or by mating with some non‐self relative (i.e., biparental inbreeding, Parker 2006; Szulkin et al. 2013). Inbreeding has pervasive short‐ and long‐term evolutionary consequences because it increases homozygosity of resulting offspring and can alter genetic (co)variances, responses to selection, speciation rates, and population persistence, and might facilitate evolution of social traits expressed among interacting relatives (Michod 1979; Charlesworth and Charlesworth 1987; Breden and Wade 1991; Wolf et al. 1999; Keller and Waller 2002; Charlesworth 2006; van Buskirk and Willi 2006; Charlesworth and Willis 2009; Wright et al. 2013; Wolak and Keller 2014). Comprehensive understanding of any resulting evolutionary dynamics therefore necessitates understanding the evolution of inbreeding itself (Michod 1979; Charlesworth and Charlesworth 1987; Goodwillie et al. 2005; Charlesworth 2006). This in turn requires the total net fitness consequence of inbreeding expressed through any particular form of mating, and hence the direction and magnitude of “selection on inbreeding,” to be quantified (Fig. 1A; Jarne and Charlesworth 1993; Goodwillie et al. 2005; Busch and Delph 2012; Sletvold et al. 2013; Stone et al. 2014).

Figure 1.

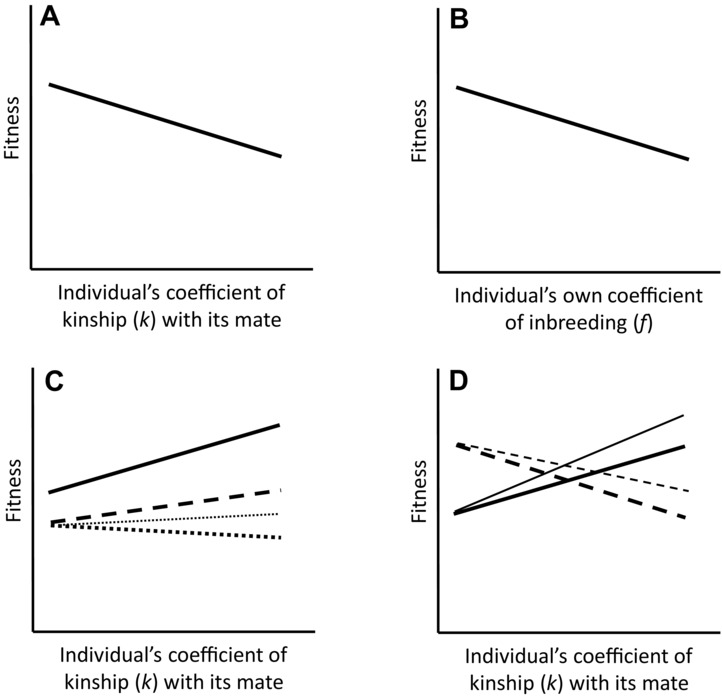
(A) “Selection on inbreeding”: individuals that pair with more closely related mates (i.e., higher pairwise coefficient of kinship k) are widely postulated to have lower fitness than individuals that pair with less closely related mates, driving evolution of inbreeding avoidance. (B) “Selection on being inbred,” typically termed “inbreeding depression”: individuals that are themselves inbred (i.e., have a higher coefficient of inbreeding f) and hence whose parents were closely related (i.e., high k) commonly have lower fitness than individuals that are less inbred. (C) An individual's initial fecundity could be positively correlated with the degree to which it inbreeds (solid line). Its resulting contribution of descendant organisms to subsequent generations could be positively correlated (thick dashed line) or only weakly negatively correlated (thick dotted line) with the degree to which it inbreeds, despite weak or strong inbreeding depression in offspring survival (causing the differences in slope between the thick solid, dashed, and dotted lines). However, due to the intrinsic transmission advantage of an allele that increases inbreeding, individuals that pair with closer relatives could still contribute more identical‐by‐descent allele copies to subsequent generations, even if they contribute fewer descendant organisms (thin vs. thick dotted lines). (D) The magnitude and direction of selection on inbreeding could potentially differ between males (solid lines) and females (dashed lines) measured in terms of numbers of descendant organisms (thick lines) or identical‐by‐descent allele copies (thin lines).

Evidence from numerous domesticated, experimental, and wild populations of animals and plants shows that inbreeding frequently causes inbreeding depression, defined as reduced fitness of inbred offspring produced through selfing or biparental inbreeding (Lynch and Walsh 1998; Keller and Waller 2002; Charlesworth and Willis 2009). Survival and reproductive success of inbred individuals can be substantially reduced, causing strong “selection against being inbred” (Fig. 1B, e.g., Willis 1993; Kruuk et al. 2002; Grueber et al. 2010; Wagenius et al. 2010; Sletvold et al. 2013; Benesh et al. 2014; Reid et al. 2014). It is widely assumed that the existence of “selection against being inbred” (i.e., inbreeding depression, Fig. 1B) will cause “selection against inbreeding” (Fig. 1A), and thereby drive evolution of inbreeding avoidance mechanisms, including choice among related and unrelated potential mates (Tregenza and Wedell 2000; Jennions et al. 2004; Hansson et al. 2007; Jamieson et al. 2009; Ala‐Honkola et al. 2011; Szulkin et al. 2013) as well as dispersal (Pusey and Wolf 1996; Lehmann and Perrin 2003; Szulkin and Sheldon 2008) and self‐incompatibility (Charlesworth and Charlesworth 1987; Goodwillie et al. 2005; Busch and Delph 2012). Consequently, numerous studies have tested for avoidance of biparental inbreeding through pre‐copulatory and/or post‐copulatory mate choice in wild and experimental populations of birds, mammals, fish, and insects. Although some studies have observed inbreeding avoidance (e.g., Pizzari et al. 2004; Firman and Simmons 2007; Bretman et al. 2009; Fitzpatrick and Evans 2014; Liu et al. 2014), other studies have not, even when strong inbreeding depression is evident (e.g., Keller and Arcese 1998; Jennions et al. 2004; Reid et al. 2006, 2015a,b; Hansson et al. 2007; Edvardsson et al. 2008; Jamieson et al. 2009; Rioux‐Paquette et al. 2010; Ala‐Honkola et al. 2011; Billing et al. 2012; Olson et al. 2012; Robinson et al. 2012; Tan et al. 2012; Ihle et al. 2013; Szulkin et al. 2013; Reynolds et al. 2014).

This apparent “inbreeding paradox” of inbreeding depression but no inbreeding avoidance through non‐random mating or fertilization might arise because the widely held assumption that inbreeding depression (e.g., Fig. 1B) will inevitably cause selection against biparental inbreeding (e.g., Fig. 1A), and hence drive evolution of mating strategies that reduce inbreeding, is simplistic (Kokko and Ots 2006; Olson et al. 2012; Szulkin et al. 2013). This assumption is immediately complicated because inbreeding and resulting inbreeding depression are expressed in different generations. Inbreeding occurs when two relatives mate, while inbreeding depression is defined as reduced fitness of resulting inbred offspring compared to outbred offspring (Charlesworth and Charlesworth 1987; Lynch and Walsh 1998; Charlesworth and Willis 2009). Selection on the degree to which individuals inbreed, and the consequent dynamics of alleles underlying inbreeding or inbreeding avoidance, will therefore depend on the lifetime numbers of inbred and outbred offspring that individuals produce, not only on the relative fitness of those offspring as affected by inbreeding depression. This complexity is explicitly recognized in the context of selfing (e.g., Porcher and Lande 2005; Busch and Delph 2012; Stone et al. 2014), but has been less widely considered in the context of biparental inbreeding (Keller and Arcese 1998; Jamieson et al. 2009; Olson et al. 2012). Here, there is no clear theoretical expectation that individuals that inbreed will necessarily conceive fewer offspring than individuals that outbreed; the occurrence of inbreeding depression in offspring fitness does not mean that parents’ initial fecundities will necessarily decrease with the degree to which they inbreed (e.g., Keller 1998; Kruuk et al. 2002; Firman and Simmons 2007; Schørring and Jäger 2007; Edvardsson et al. 2008; Grueber et al. 2010; Tan et al. 2012; Liu et al. 2014). Indeed, individuals that inbreed might potentially conceive or rear more offspring than individuals that outbreed (Fig. 1C), for example, if avoiding inbreeding imposes direct costs of time, energy, or failure to mate (Keller and Arcese 1998; Kokko and Ots 2006), if optimal reproductive timing or location are correlated across relatives leading to assortative pairing (Robinson et al. 2012; Reid et al. 2015b), or if inbreeding is associated with expression of beneficial social traits (Breden and Wade 1991; Schørring and Jäger 2007). It consequently cannot be assumed that individuals that inbreed will necessarily leave fewer long‐term descendants than individuals that outbreed, or hence that there will be selection against biparental inbreeding, even if inbred offspring have low fitness due to inbreeding depression (Fig. 1C).

Furthermore, the basic assumption that inbreeding depression in offspring fitness will necessarily drive evolution of inbreeding avoidance ignores the potential evolutionary advantage of an allele that increases the degree of biparental inbreeding (Waser et al. 1986; Kokko and Ots 2006; Parker 2006; Szulkin et al. 2013), which is analogous to the widely recognized evolutionary advantage of an allele that increases selfing (Lande and Schemske 1985; Goodwillie et al. 2005; Charlesworth 2006; Busch and Delph 2012; Stone et al. 2014). The potential advantage arises because inbred offspring can inherit an identical‐by‐descent copy of an allele that is present in a focal parent from the parent's related mate as well as from the focal parent itself, meaning that parents are more closely related to inbred offspring than to outbred offspring (Lynch and Walsh 1998, p. 136). Consequently, even if individuals that inbreed contribute fewer direct descendant organisms to future generations than individuals that outbreed, those descendants might still contribute more identical‐by‐descent copies of any allele carried by the focal individual, potentially increasing the frequency of alleles that increase biparental inbreeding (all else being equal, Waser et al. 1986; Parker 2006; Duthie and Reid 2015; Fig. 1C).

In addition, selection on biparental inbreeding is widely postulated to be sex‐specific, because costs of producing inbred offspring with low fitness might be greater for the resource‐limited sex (typically females) than for the mate‐limited sex (typically males, Lehmann and Perrin 2003; Pizzari et al. 2004; Kokko and Ots 2006; Parker 2006; Fig. 1D). Any evolutionary response to selection on inbreeding by one sex might then be constrained by divergent selection on inbreeding by the other sex. Overall, understanding the evolutionary dynamics of biparental inbreeding therefore requires quantification of the degree to which females and males that inbreed to greater or lesser degrees through any particular form of mating contribute more or fewer descendants or identical‐by‐descent allele copies to future generations (Fig. 1D). However, while numerous studies have estimated inbreeding depression in components of fitness in wild populations where biparental inbreeding occurs (thereby estimating “selection on being inbred,” Fig. 1B; e.g., Keller 1998; Keller and Waller 2002; Szulkin et al. 2007; Jamieson et al. 2009; Grueber et al. 2010; Wagenius et al. 2010; Billing et al. 2012; Reid et al. 2014), the overall magnitude and direction of sex‐specific selection on the degree to which individuals inbreed through any particular form of mating (i.e., “selection on inbreeding,” Fig. 1D) has not been explicitly estimated.

In reproductive systems where females and males form distinct socially persistent breeding pairs and provide substantial biparental care to dependent offspring, one key component of an individual's overall expression of inbreeding is its coefficient of kinship (k) with the mate with which it forms such a breeding pair (hereafter “social pairing,” Appendix S1). Some degree of extra‐pair reproduction commonly occurs in such systems, potentially allowing females to adjust the coefficient of inbreeding (f) of their offspring, and allowing males to accrue additional reproductive success (Reid et al. 2011b, 2015a). However, most females and males typically accrue most direct reproductive success by producing offspring with their socially paired mate (Webster et al. 1995; Griffith et al. 2002; Lebigre et al. 2012). Furthermore, the k between socially paired mates might shape the evolution and expression of social traits such as parental care (e.g., Michod 1979; Breden and Wade 1991; Wolf et al. 1999), and constrain or facilitate further reproduction by relatives (Waser et al. 1986; Duthie and Reid 2015). The “social pair” therefore constitutes one fundamental unit of social and genetic structure that arises through pre‐copulatory mate choice, and the degree to which individuals pair with more or less closely related mates could substantially affect an individual's fitness measured as the numbers of direct descendants and identical‐by‐descent allele copies contributed to subsequent generations.

Numbers of descendants and expected identical‐by‐descent allele copies contributed to any specific generation or timepoint through reproduction by any focal individual can be calculated from long‐term pedigree data. In general, fitness is often appropriately measured across one zygote‐to‐zygote generation (Wolf and Wade 2001). However, when phenotypic traits of interest are expressed by adults and early offspring survival depends largely on parental phenotype and hence genotype, fitness might be appropriately measured across one adult‐to‐adult generation (e.g., the number of adult offspring left by each adult, Wolf and Wade 2001). In addition, for traits pertaining to mating decisions and reproductive strategies expressed by adults where selection is hypothesized to stem from consequent variation in offspring survival or reproductive success (as for inbreeding by parents and consequent inbreeding depression in offspring), it can also be informative to measure fitness across two generations (i.e., adult to grand‐offspring), thereby explicitly incorporating variation in offspring fitness associated with expression of parental traits (Day and Otto 2001; Kokko et al. 2003; Hunt et al. 2004; Reid et al. 2005). In such circumstances, a useful overall approach is to measure fitness to multiple successive stages spanning one and two generations.

We used multi‐generational pedigree data from free‐living song sparrows (Melospiza melodia) to quantify phenotypic variation in female and male fitness in relation to individuals’ k with the mates with which they paired, and thereby estimate sex‐specific selection on the degree to which individuals formed socially persistent breeding pairs with relatives. We measured the fitness of individual adults as the relative numbers of genealogical descendants contributed across up to two complete generations. We additionally estimated the fitness of any allele carried by an individual adult as the number of identical‐by‐descent copies expected to be contributed through these descendants, by weighting each descendant by its k with the focal adult. We thereby consider the validity of the widely prevailing assumption that there will necessarily be “selection against inbreeding” (e.g., Fig. 1A) in systems where inbreeding depression (i.e., “selection against being inbred,” Fig. 1B) is observed, and consequent selection for mechanisms that reduce the degree of biparental inbreeding expressed through formation of socially persistent breeding pairs among relatives.

Methods

STUDY SYSTEM

Song sparrows form socially persistent breeding pairs, where both sexes contribute to territory defense and parental care. A resident song sparrow population inhabiting Mandarte island, BC, Canada, has been studied intensively since 1975 (Smith et al. 2006) and recently numbered 30 ± 12 SD breeding pairs. Previous analyses of long‐term pedigree data showed substantial inbreeding depression in embryo, juvenile and adult survival, and in reproductive success (Keller 1998; Keller et al. 2008; Reid et al. 2011b, 2014, 2015a). Individuals whose parents were closely related therefore have low fitness (e.g., Fig 1B). However, despite this inbreeding depression, there is little evidence of inbreeding avoidance expressed through non‐random social pairing (Keller and Arcese 1998; Reid et al. 2006, 2015b), or through non‐random extra‐pair reproduction by females (Reid et al. 2015a,b). These observations present an apparent “inbreeding paradox” (i.e., inbreeding depression but no inbreeding avoidance, Keller and Arcese 1998), as has also been noted in some other wild vertebrate populations (e.g., Hansson et al. 2007; Jamieson et al. 2009; Billing et al. 2012).

DATA COLLECTION

Each year, all nests on Mandarte were located and all offspring were marked with unique combinations of metal and color bands approximately six days post‐hatch. Mandarte, lies within a large natural song sparrow meta‐population, is surrounded by numerous other similarly small subpopulations, and regularly receives immigrants (1.1 per year on average, Smith et al. 2006). New immigrants were mist‐netted and color‐banded. All adults (i.e., ≥1year‐old) alive in each year were identified and all socially persistent pairings that formed and attempted to breed, and the outcomes of all breeding attempts, were documented (Smith et al. 2006; Reid et al. 2006, 2014, 2015b; Sardell et al. 2010). The relatively high local recruitment rates, and general absence of Mandarte‐banded individuals on surrounding islands, suggest that emigration from Mandarte is infrequent and hence that the fitness of resident adults can be accurately measured (Reid et al. 2005; Wilson and Arcese 2008).

Both sexes can first breed aged one year, and social pairings can rear up to three broods per year of up to four offspring per brood (Smith et al. 2006). Median adult life span is two–three years (maximum nine years, Lebigre et al. 2012), creating overlapping reproductive generations. Social pairings frequently persist across consecutive breeding attempts and years, but both sexes can repair following mortality of their previous mate, and sometimes divorce a surviving mate and repair both within and between years (Smith et al. 2006; Reid et al. 2015b). All adult females alive in each year formed at least one social pairing. However, because the adult sex ratio was often male‐biased, some adult males remained socially unpaired (3–67% per year, Sardell et al. 2010). These males occasionally sired extra‐pair offspring reared by other social pairings (Sardell et al. 2010; Lebigre et al. 2012, see Results). Neither socially paired nor socially unpaired males care for extra‐pair offspring that they sire, but socially paired males do care for extra‐pair offspring produced by their paired female (i.e., offspring that they did not sire) alongside within‐pair offspring that they did sire. Both sexes typically accrue most direct reproductive success through within‐pair offspring produced with their socially paired mates rather than through extra‐pair reproduction (Reid et al. 2011a,b; Lebigre et al. 2012).

PEDIGREE AND KINSHIP

To construct a pedigree from which k between paired females and males could be calculated, field observations were initially used to link all offspring banded during 1975–2012 to their socially paired parents (i.e., the female and male that provided care, Keller 1998; Reid et al. 2008, 2014). To identify true genetic sires and thereby minimize pedigree error, virtually all offspring banded during 1993–2012 and their potential parents were genotyped at 160 polymorphic microsatellite loci (Sardell et al. 2010; Reid et al. 2014, 2015a; Nietlisbach et al. 2015). Bayesian parentage analyses confirmed that all mothers were correctly identified from parental behavior, and assigned genetic sires to >99% of banded chicks with >99% individual‐level statistical confidence (Sardell et al. 2010; Reid et al. 2015a). Overall, 72% of chicks were assigned to the male that was socially paired to their mother (i.e., within‐pair paternity). All genetic paternity assignments were used to correct the pedigree for extra‐pair paternity that occurred during 1993–2012 (Reid et al. 2014). To further reduce remaining pedigree error, paternity of individuals hatched before 1993 that survived to breed was also genetically verified so far as available samples allowed (Reid et al. 2014).

Standard algorithms were used to calculate k between socially paired mates, thereby measuring the probability that two homologous alleles drawn from the two mates will be identical‐by‐descent relative to the pedigree baseline (Keller 1998; Lynch and Walsh 1998, p. 135). Each individual's own f, which measures the probability that two homologous alleles within the individual will be identical‐by‐descent (and equals k between the individual's genetic parents), was also calculated (Lynch and Walsh 1998, p. 135). Although the full pedigree presumably contains error stemming from unknown extra‐pair paternity prior to 1993, approximately 86% of pre‐1993 links (i.e., all maternal links and 72% of paternal links) will be correct assuming a similar extra‐pair paternity rate to that observed subsequently. Utilizing the full pedigree therefore provides more informative estimates of k among post‐1993 breeders than the alternative assumption that the 1993 breeders are all unrelated (Reid et al. 2011b). Effects of remaining pre‐1993 pedigree error on estimates of k and f among contemporary sparrows quickly become trivial with increasing depth of genetically verified pedigree (Reid et al. 2014, 2015a). The song sparrow dataset therefore permits relatively accurate estimation of k between contemporary Mandarte‐hatched females and males that formed socially persistent breeding pairs, and of these individuals’ f values, relative to the defined baseline. Values of k = 0, 0.0625, 0.125, and 0.25 equate to pairings between unrelated individuals and between outbred first‐cousins, half‐sibs, and full‐sibs (or equivalent relatives), respectively.

Inbreeding coefficients of immigrants are undefined relative to the pedigree baseline (Keller 1998; Reid et al. 2006). However, microsatellite genotypes suggest that immigrants are not closely related to existing Mandarte natives (Keller et al. 2001). Immigrant‐native pairings were therefore defined as outbreeding (k = 0, Reid et al. 2006, 2011b; Keller et al. 2008). Immigration is sufficient to prevent inbreeding from rapidly accumulating and to maintain variation in k, such that all non‐immigrant males and females had some opportunity to pair with a range of different relatives throughout their lives (Reid et al. 2015a,b).

LIFETIME DEGREE OF INBREEDING

We quantified the degree to which each individual participated in socially persistent breeding pairs with relatives as the mean k between each focal individual and the socially paired mate with which it made each breeding attempt (i.e., each nest in which eggs were laid) during its lifetime (hereafter ƙ_mate). The number of observations that contributed to ƙ_mate for each individual therefore increased with the number of breeding attempts made (Appendix S1). However, ƙ_mate is an unbiased metric of the lifetime degree of inbreeding that individuals expressed through social pairing, and does not simply regress more to the population mean k with increasing breeding attempts because social pairings frequently persisted across multiple successive breeding attempts and years rather than forming afresh for each attempt (Appendix S1).

LIFETIME REPRODUCTIVE SUCCESS AND ALLELIC FITNESS

Each adult female's fitness was measured as its lifetime reproductive success (LRS), counting its total number of (1) banded offspring, (2) adult offspring, (3) banded grand‐offspring, and (4) adult grand‐offspring. These four measures (hereafter four “generational timepoints”) hierarchically incorporate (1) a female's total fecundity; (2) variation in survival of a female's offspring to age one year, thereby capturing inbreeding depression in offspring survival, resulting from inbreeding expressed by the focal female through its total within‐pair and extra‐pair reproduction, and measuring fitness through one complete adult‐to‐adult life cycle; (3) the lifetime number of banded offspring produced by a female's offspring, thereby capturing inbreeding depression in offspring reproductive success, resulting from total inbreeding expressed by the focal female; and (4) survival of a female's grand‐offspring to age one year, thereby measuring fitness through two complete adult‐to‐adult life cycles. LRS measured to banded offspring might incorporate some variation in early offspring survival due to the offspring's own f rather than solely reflecting a female's own intrinsic fecundity (Reid et al. 2015a). However, early offspring survival depends substantially on parental care in passerine birds, and is therefore partly a parental trait.

Each adult male's fitness was measured as its LRS to the same four generational timepoints, counting genealogical offspring and grand‐offspring. Specifically, LRS was measured as the numbers of banded and adult offspring that each male sired (including extra‐pair offspring sired) not as offspring that he reared (i.e., excluding extra‐pair offspring produced by the male's socially paired female), and as banded and adult offspring of the sired offspring (i.e., each male's true grand‐offspring).

The “allelic value” of each offspring and grand‐offspring relative to each of its parents and grandparents was calculated as twice the parent‐offspring or grandparent‐grand‐offspring k, respectively (computed from the pedigree, Appendix S2). Allelic value therefore measures the number of copies of an autosomal allele that is present in a focal parent or grandparent that is expected to be present identical‐by‐descent in a particular offspring or grand‐offspring (assuming weak selection on any allele, Michod 1979). It increases as functions of the degrees to which focal parents inbreed and are themselves inbred (Appendix S2; Lynch and Walsh 1998, p. 136). For reference, allelic values of an outbred offspring and grand‐offspring relative to an outbred parent or grand‐parent are 0.5 and 0.25, respectively, with inbreeding in one or both generations causing higher values (Appendix S2; Lynch and Walsh 1998, p. 136).

Lifetime allelic fitness (LAF) was then calculated for each adult female and male as the sum of the allelic values of all their banded or adult offspring or grand‐offspring. Total LAF was divided by (1 + f _i), where f _i is the focal female or male's own f, thereby quantifying LAF per copy of any autosomal allele expected to be present identical‐by‐descent within each focal individual (hereafter “LAF_f,” Appendix S2).

In age‐structured populations with overlapping generations, it can be valuable to measure variation in individuals’ annual fitness rather than lifetime fitness (Engen et al. 2011), but the appropriate measure of “annual fitness” becomes unclear when one objective is to measure fitness in terms of grand‐offspring. However, we additionally explored whether overall relationships between LRS and LAF_f measured to banded offspring and ƙ_mate arose because females or males with higher ƙ_mate produced more banded offspring per breeding year and/or survived for more breeding years (Appendix S3).

STATISTICAL ANALYSES

To estimate sex‐specific “selection on inbreeding” (e.g., Fig. 1D), linear selection gradients (β) on the degree to which individuals formed socially persistent breeding pairs with relatives were calculated by regressing w‐standardized fitness (i.e., individual fitness divided by mean fitness) on ƙ_mate, with fitness measured as LRS and LAF_f to each of the four specified generational timepoints. Although our primary aim was not to re‐estimate inbreeding depression in fitness in the study population (see Keller 1998; Keller et al. 2008; Reid et al. 2011b, 2014), we also regressed w‐standardized fitness on individual f, thereby simultaneously estimating “selection on being inbred” (e.g., inbreeding depression, Fig. 1B) as well as “selection on inbreeding” (e.g., Fig. 1D) within a multiple regression.

We primarily present SD standardized selection gradients on ƙ_mate and f, calculated by regressing w‐standardized fitness on (ƙ_mate – μ_k)/σ_k and (f – μ_f)/σ_f, respectively, where μ_k, μ_f, σ_k, and σ_f are the means and SDs of ƙ_mate and f, respectively. However, because there may be no single best means of standardizing β that facilitates all comparative purposes, we also calculated mean‐standardized selection gradients by regressing w‐standardized fitness on (ƙ_mate – μ_k)/μ_k and (f – μ_f)/μ_f (Appendix S4, Lande and Arnold 1983; Hereford et al. 2004; Matsumura et al. 2012). Fitness, ƙ_mate and f were standardized within sexes, and within cohorts to account for among‐cohort variation (Smith et al. 2006; Reid et al. 2014; Appendix S5). Bootstrap confidence intervals were computed by resampling residuals 10,000 times.

Separate analyses were run for females and males to ensure independence of observations. Analyses were restricted to individuals hatched on Mandarte during 1993–2001 that survived to adulthood (i.e., age one year). All these individuals had genetically verified parents (and typically more distant relatives), ensuring accurate proximate pedigree. All their offspring had died by 2012, meaning that LRS and LAF_f to adult grand‐offspring were completely measured by 2013 with no censoring. LRS and LAF_f measured to banded offspring cannot contain any error due to offspring emigration. Furthermore, because emigration is thought to be infrequent, any error or bias in LRS and LAF_f measured to subsequent generational timepoints is likely to be small (see Reid et al. 2005). All adult females formed social pairings, meaning that ƙ_mate was observable. By contrast, ƙ_mate was unobservable and undefined for adult males that never socially paired (due to the male‐biased adult sex ratio). Selection on phenotypic ƙ_mate therefore cannot be directly estimated across all adult males, potentially biasing any subsequent evolutionary inference (e.g., Hadfield 2008; Mojica and Kelly 2010). However, to evaluate selection on pairing versus failing to pair, the LRS and LAF_f of permanently unpaired males (which might exceed zero if they sired extra‐pair offspring) were compared to those of males that socially paired for at least one breeding attempt. Male fitness was w‐standardized by calculating mean fitness across all males from each cohort that formed at least one social pairing, but conclusions remained similar when mean fitness was calculated across all males from each cohort that survived to adulthood.

Four immigrant females and one immigrant male were excluded from analyses as focal individuals because they were defined as unrelated to all existing population members at arrival and hence had no immediate opportunity to inbreed, and because f is undefined for immigrants relative to the pedigree baseline (Reid et al. 2006). However, immigrants were (implicitly) included as socially paired mates of focal opposite‐sex natives. Further models suggested that quadratic (nonlinear) selection gradients on ƙ_mate and f were small and did not differ significantly from zero. However, these gradients were estimated with substantial uncertainty, and are not reported. Analyses were run in R version 3.0.1 (R Core Team 2013). Raw means are presented as ±1 SD, and IQR is the interquartile range. Data are available from the Dryad Digital Repository: doi:10.5061/dryad.0015b.

Results

FEMALE KINSHIP, INBREEDING, AND FITNESS

In total, 99 female song sparrows that hatched on Mandarte during 1993–2001 survived to adulthood (i.e., age one year). These females made a mean of 5.2 ± 4.0 breeding attempts during their lifetimes (median 4, IQR 2–7, range 1–22), and socially paired with a mean of 1.9 ± 1.1 different males (median 2, IQR 1–2, range 1–5). Mean ƙ_mate was 0.073 ± 0.029 (median 0.074, IQR 0.054‐0.086, range 0.000–0.169, Appendix S1). Distributions of female LRS and LAF_f measured as banded and adult offspring and grand‐offspring are summarized in Table 1 and depicted in Appendix S6.

Table 1.

Descriptive statistics for female LRS and LAF_f measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring across 99 adult female song sparrows, and the SD‐standardized selection gradients (β, with 95% bootstrapped confidence intervals, 95% CI) of w‐standardized LRS and LAF_f on the mean coefficient of kinship with the socially paired males with which each female made its breeding attempts (ƙ_mate) and on the female's own coefficient of inbreeding (f)

				ƙmate	f
		Mean ± SD	Median, IQR, range	β (95% CI)	β (95% CI)
(A) Banded offspring	LRS	10.2 ± 8.3	7, 4–16, 0–50	0.19 (0.04 to 0.34)	−0.15 (−0.29 to −0.01)
	LAFf	5.8 ± 4.8	3.8, 2.3‐9.1, 0–29.2	0.21 (0.07 to 0.35)	−0.15 (−0.29 to −0.01)
(B) Adult offspring	LRS	2.0 ± 2.6	1, 0–3, 0–18	0.16 (−0.05 to 0.38)	−0.24 (−0.45 to −0.02)
	LAFf	1.1 ± 1.4	0.6, 0.0–1.7, 0–10.1	0.18 (−0.03 to 0.39)	−0.24 (−0.45 to −0.02)
(C) Banded grand‐offspring	LRS	16.2 ± 29.0	6, 0–19.5, 0–232	0.19 (−0.06 to 0.45)	−0.26 (−0.52 to −0.01)
	LAFf	5.8 ± 11.3	2.2, 0–7.4, 0–96.3	0.20 (−0.06 to 0.46)	−0.27 (−0.53 to −0.01)
(D) Adult grand‐offspring	LRS	2.8 ± 5.0	1, 0–4, 0–33	0.15 (−0.15 to 0.45)	−0.34 (−0.64 to −0.03)
	LAFf	1.0 ± 1.7	0.3, 0–1.2, 0–12.2	0.17 (−0.13 to 0.47)	−0.34 (−0.64 to −0.04)

IQR = Interquartile range is the interquartile rang. Bold signifies selection gradients whose 95% CIs did not overlap zero.

The estimated phenotypic selection gradients of relative female fitness on ƙ_mate were all positive (Table 1, Fig. 2), where positive gradients indicate that females that socially paired with more closely related males across their lifetimes had higher fitness. Bootstrapped 95% CIs estimated across banded offspring did not overlap zero, but 95% CIs estimated across adult offspring and banded and adult grand‐offspring were wide and overlapped zero (Table 1). Selection gradients estimated for LAF_f were more positive than those estimated for LRS at analogous generational timepoints (Table 1). However, the differences were small, especially relative to the 95% CIs (Table 1, Fig. 2). SD‐standardized ƙ_mate explained <5% of phenotypic variation in relative LRS and LAF_f. Additional analyses showed that the positive relationships between female LRS and LAF_f measured as banded offspring and ƙ_mate arose because females with higher ƙ_mate tended to have longer breeding life spans, and tended to produce more banded offspring per year (Appendix S3).

Figure 2.

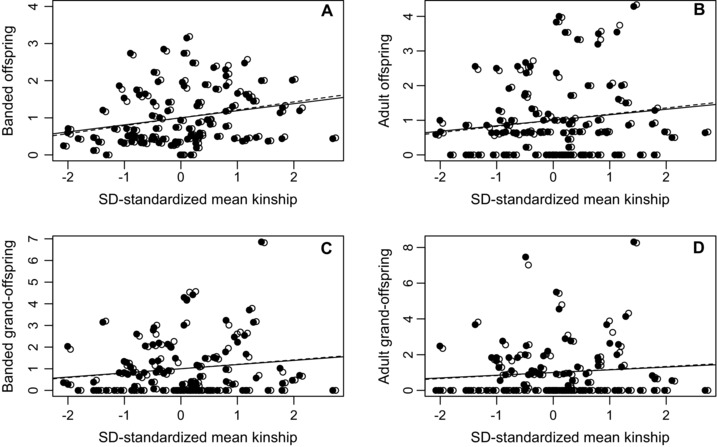
Relationships between w‐standardized female LRS (filled symbols) and LAF_f (open symbols) measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring and SD‐standardized mean coefficient of kinship with the socially paired males with which each female made its breeding attempts (ƙ_mate) across 99 female song sparrows. Slopes of regression lines equal SD‐standardized selection gradients for LRS (solid lines) and LAF_f (dashed lines), representing “selection on inbreeding.” Points for LAF_f are offset for presentation.

Across the 99 females, mean f was 0.064 ± 0.039 (median 0.066, IQR 0.035–0.084, range 0.000–0.211). SD‐standardized ƙ_mate and f were weakly positively correlated across these females (r ₉₇ = 0.15). The estimated phenotypic selection gradients of relative female fitness on f were all negative, showing that more inbred females had lower fitness (i.e., inbreeding depression, Table 1, Fig. 3). The 95% CIs did not overlap zero, and estimates became increasingly negative across successive generational timepoints (Table 1, Fig. 3). SD‐standardized f explained 4 – 8% of variation in relative LRS and LAF_f. Estimated phenotypic “selection on inbreeding” was therefore opposite in direction to the estimated “selection on being inbred” in females (Figs. 2 and 3).

Figure 3.

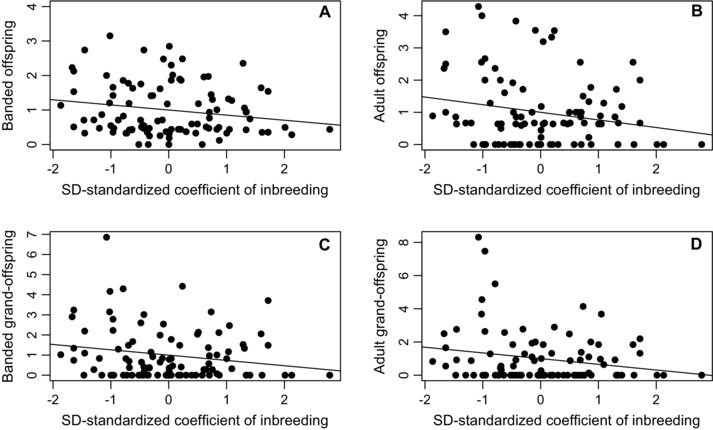
Relationships between w‐standardized female LRS measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring and SD‐standardized coefficient of inbreeding (f) across 99 female song sparrows. Slopes of regression lines equal SD‐standardized selection gradients for LRS, representing “selection on being inbred.” Selection gradients for LAF_f were virtually identical (Table 1).

MALE KINSHIP, INBREEDING, AND FITNESS

A total of 101 male song sparrows that hatched on Mandarte during 1993–2001 survived to adulthood and made at least one breeding attempt with a socially paired female (meaning that ƙ_mate was observable). A further 56 males that hatched during 1993–2001 survived to adulthood but never socially paired, meaning that ƙ_mate was unobservable and undefined. The 101 males that paired made a mean of 4.3 ± 3.4 breeding attempts during their lifetimes (median 3, IQR 2–6, range 1–14) and socially paired with a mean of 1.7 ± 1.0 different females (median 1, IQR 1–2, range 1–5). Mean ƙ_mate was 0.075 ± 0.043 (median 0.072, IQR 0.052–0.088, range 0.000–0.310, Appendix S1).

Distributions of LRS and LAF_f measured as banded and adult offspring and grand‐offspring for the 101 males that socially paired are summarized in Table 2 and depicted in Appendix S6. Across the 56 adult males that never socially paired, and hence for whom any direct reproductive success came solely through extra‐pair paternity, mean LRS and LAF_f were, respectively, 0.2 ± 0.7 (range 0–3) and 0.1 ± 0.4 (range 0–1.7) across banded offspring, 0.02 ± 0.1 (range 0–1) and 0.01 ± 0.1 (range 0–0.5) across adult offspring, and uniformly zero across banded and adult grand‐offspring. Males that did not socially pair therefore had zero grand‐offspring, and hence had zero direct fitness measured across two generations.

Table 2.

Descriptive statistics for male LRS and LAF_f measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring across 101 adult male song sparrows that formed at least one social pairing, and the SD‐standardized selection gradients (β, with 95% bootstrapped confidence intervals, 95% CI) of w‐standardized LRS and LAF_f on the mean coefficient of kinship with the socially paired females with which each male made its breeding attempts (ƙ_mate) and on the male's own coefficient of inbreeding (f)

				ƙmate	f
		Mean ± SD	Median, IQR, range	β (95% CI)	β (95% CI)
(A) Banded offspring	LRS	8.9 ± 8.3	6, 3–14, 0–37	0.02 (−0.16 to 0.20)	−0.27 (−0.46 to −0.09)
	LAFf	5.1 ± 4.7	3.5, 1.7–7.9, 0–20.5	0.06 (−0.12 to 0.24)	−0.27 (−0.45 to −0.09)
(B) Adult offspring	LRS	1.7 ± 2.4	1, 0–2, 0–15	−0.11 (−0.36 to 0.15)	−0.37 (−0.63 to −0.12)
	LAFf	1.0 ± 1.3	0.6, 0–1.2, 0–8.3	−0.08 (−0.33 to 0.17)	−0.37 (−0.63 to −0.12)
(C) Banded grand‐offspring	LRS	16.3 ± 28.3	3, 0–20, 0–147	−0.22 (−0.52 to 0.08)	−0.27 (−0.57 to 0.03)
	LAFf	5.6 ± 9.5	1.2, 0–7.5, 0–52.3	−0.19 (−0.50 to 0.12)	−0.26 (−0.57 to 0.04)
(D) Adult grand‐offspring	LRS	3.1 ± 5.3	1, 0–4, 0–25	−0.32 (−0.63 to −0.01)	−0.35 (−0.66 to −0.03)
	LAFf	1.0 ± 1.7	0, 0–1.3, 0–8.0	−0.30 (−0.61 to −0.001)	−0.33 (−0.64 to −0.02)

IQR = interquartile range. Bold signifies selection gradients whose 95% CIs did not overlap zero.

The estimated phenotypic selection gradients of relative male fitness on ƙ_mate were very weakly positive across banded offspring, but increasingly negative across adult offspring and banded and adult grand‐offspring (Table 2, Fig. 4), where negative gradients indicate that males that socially paired with more closely related females had lower fitness than males that socially paired with less closely related females. The 95% CIs for the selection gradients estimated across adult grand‐offspring did not overlap zero, but the other 95% CIs were wide and overlapped zero (Table 2). Selection gradients estimated for LAF_f were slightly less negative than those estimated for LRS at analogous generational timepoints, but these differences were again small, especially relative to the 95% CIs (Table 2, Fig. 4). SD‐standardized ƙ_mate explained <5% of variation in relative LRS and LAF_f. Additional analyses showed that males with higher ƙ_mate tended to sire more banded offspring per year, but tended to have slightly shorter breeding life spans (Appendix S3).

Figure 4.

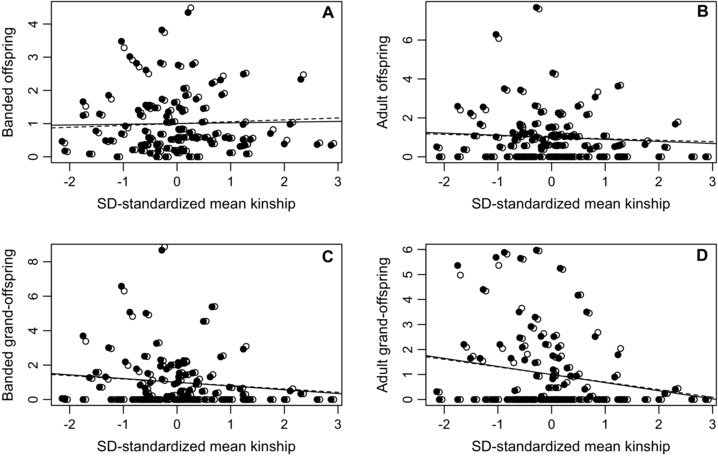
Relationships between w‐standardized male LRS (filled symbols) and LAF_f (open symbols) measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring and SD‐standardized mean coefficient of kinship with the socially paired females with which each male made its breeding attempts (ƙ_mate) across 101 male song sparrows that formed at least one social pairing. Slopes of regression lines equal SD‐standardized selection gradients for LRS (solid lines) and LAF_f (dashed lines), representing “selection on inbreeding.” Points for LAF_f are offset for presentation.

Across the 101 males, mean f was 0.061 ± 0.037 (median 0.060, IQR 0.042–0.077, range 0.000–0.257). SD‐standardized ƙ_mate and f were moderately positively correlated across these males (r ₉₉ = 0.27). The estimated phenotypic selection gradients of relative male fitness on f were consistently negative showing that, across males that formed at least one social pairing, more inbred males had lower fitness (i.e., inbreeding depression, Table 2, Fig. 5). The 95% CIs slightly overlapped zero when LRS and LAF_f were measured across banded grand‐offspring, but not otherwise (Table 2). SD‐standardized f explained 5–10% of variation in relative LRS and LAF_f. Selection gradients on f were similar when calculated across all 157 adult males, including those that never socially paired (Appendix S4). Estimated phenotypic “selection on inbreeding” therefore primarily operated in the same direction as the estimated “selection on being inbred” across males that formed at least one socially persistent breeding pair during their lifetimes (Figs. 4 and 5).

Figure 5.

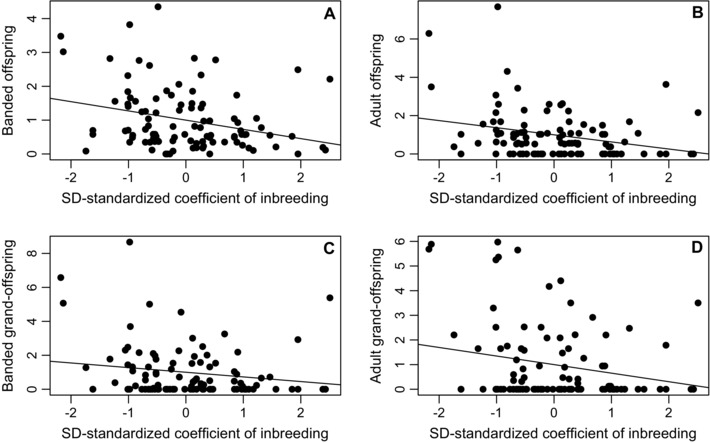
Relationships between w‐standardized male LRS measured across (A) banded offspring, (B) adult offspring, (C) banded grand‐offspring, and (D) adult grand‐offspring and SD‐standardized coefficient of inbreeding (f) across 101 male song sparrows that formed at least one social pairing. Slopes of regression lines equal SD‐standardized selection gradients for LRS, representing “selection on being inbred.” Selection gradients for LAF_f were virtually identical (Table 2).

Discussion

Inbreeding depression in the fitness of offspring produced by matings between relatives is widely postulated to cause selection against biparental inbreeding, thereby driving evolution of inbreeding avoidance through pre‐copulatory and/or post‐copulatory processes (Pusey and Wolf 1996; Tregenza and Wedell 2000; Jennions et al. 2004; Hansson et al. 2007; Jamieson et al. 2009; Ala‐Honkola et al. 2011). However, such inbreeding avoidance is not always observed, even when diverse relatives and non‐relatives are available as potential mates and inbreeding depression is severe (e.g., Keller and Arcese 1998; Hansson et al. 2007; Jamieson et al. 2009; Rioux‐Paquette et al. 2010; Billing et al. 2012; Olson et al. 2012; Reid et al. 2015b).

There are multiple possible explanations for this apparent “inbreeding paradox.” Inbreeding avoidance might not have evolved in species with historically large panmictic populations and correspondingly low probabilities of biparental inbreeding, even if severe inbreeding depression is expressed during experimental inbreeding or contemporary population bottlenecks (Jennions et al. 2004; Jamieson et al. 2009; Rioux‐Paquette et al. 2010; Ala‐Honkola et al. 2011). However, even when inbreeding regularly occurs, selection against inbreeding could be weakened or reversed by ecological or genetic benefits of mating with relatives, or by costs of inbreeding avoidance such as immediate or lifelong failure to find alternative mates (Keller and Arcese 1998; Lehmann and Perrin 2003; Kokko and Ots 2006; Jamieson et al. 2009; Olson et al. 2012). Comprehensive models predicting the net fitness consequence of inbreeding have been extensively analyzed and parameterized in the context of self‐fertilization versus outcrossing, incorporating effects of fertility assurance, reduced outcrossing (e.g., pollen discounting), and the intrinsic transmission advantage of alleles promoting selfing, as well as inbreeding depression in offspring fitness (e.g., Lande and Schemske 1985; Jarne and Charlesworth 1993; Willis 1993; Goodwillie et al. 2005; Porcher and Lande 2005; Charlesworth 2006; Busch and Delph 2012; Stone et al. 2014). However, empirical studies aiming to understand the evolution of biparental inbreeding have rarely considered similarly multifaceted components of selection (Kokko and Ots 2006; Jamieson et al. 2009; Szulkin et al. 2013). Selection on biparental inbreeding cannot necessarily be inferred from existing models or estimates of selection on selfing because these reproductive systems exhibit very different distributions of relatedness and opportunities for mating failure and sexual antagonism (Parker 2006; Szulkin et al. 2013).

Numerous studies have quantified inbreeding depression in wild populations where biparental inbreeding occurs by regressing some measure of an individual's fitness on its own coefficient of inbreeding (f) or multilocus heterozygosity, thereby implicitly measuring “selection on being inbred” (Keller and Waller 2002; Szulkin et al. 2007; Chapman et al. 2009; Jamieson et al. 2009; Billing et al. 2012; Reid et al. 2014). In contrast, no studies have quantified total sex‐specific selection on the degree to which an individual inbreeds through any form of mating (thereby measuring “selection on inbreeding”) by regressing an individual's fitness on its coefficient of k with its mates. Furthermore, no studies have accounted for the intrinsic transmission advantage of an allele that increases biparental inbreeding. Consequently, no studies have explicitly considered whether evolution of mechanisms that reduce biparental inbreeding should be expected. We used comprehensive pedigree data from free‐living song sparrows to simultaneously estimate selection on the degree to which females and males formed socially persistent breeding pairs with relatives, and selection on the degree to which females and males were themselves inbred, in relation to relative LRS and LAF_f measured over up to two complete generations of descendants.

ESTIMATED “SELECTION ON INBREEDING”

Perhaps unexpectedly, estimated phenotypic selection gradients on the degree to which female song sparrows paired with related males were positive across all four generational timepoints considered; females that paired with closer relatives tended to have higher fitness and contribute more descendants to the study population. However, ƙ_mate explained a small proportion of variation in female fitness, and confidence intervals around selection gradients estimated across adult offspring, and across banded and adult grand‐offspring, were wide and overlapped zero. Selection gradients for LAF_f were slightly more positive than those estimated for LRS to the same generational timepoints. This is expected because parents are more closely related to inbred offspring (and resulting grand‐offspring) than to outbred offspring (Lynch and Walsh 1998; Appendix S2), creating the potential transmission advantage of any allele that increases the degree of inbreeding (e.g., Waser et al. 1986; Parker 2006). However, these increments were small, reflecting the moderate degree of inbreeding occurring in song sparrows.

In contrast, estimated phenotypic selection gradients on the degree to which male song sparrows paired with related females became increasingly negative as LRS was measured across consecutive generational timepoints, and were strongly negative across adult grand‐offspring. Males that paired with closer relatives therefore contributed fewer descendants to the study population than males that paired with more distant relatives. The negative selection gradients were slightly ameliorated, but far from eliminated, by the transmission advantage of an allele that increases inbreeding as measured by LAF_f relative to LRS. Consequently, across males that formed at least one pairing and hence for whom ƙ_mate was observable, males that paired with more closely related females made smaller relative allelic contributions through adult grand‐offspring.

The increasingly negative selection gradients estimated across the four generational timepoints for males might be expected because the successive timepoints increasingly capture the low survival and reproductive success of inbred offspring (i.e., inbreeding depression, Keller 1998; Keller et al. 2008; Reid et al. 2014). Males that paired with more closely related females would therefore leave fewer grand‐offspring per within‐pair offspring sired than males that paired with more distantly related females. However, the estimated selection gradients for females did not decrease substantially across the four generational timepoints. This may be because extra‐pair reproduction means that inbreeding depression in a female's offspring is partly decoupled from her k with her socially paired mate (although 72% of females’ offspring were sired by socially paired males on average, Sardell et al. 2010). To understand the demographic mechanisms underlying the apparent sex‐specific selection on pairing among relatives, future analyses should partition sex‐specific variation in LRS and LAF_f in relation to ƙ_mate into components stemming from female and male within‐pair and extra‐pair reproduction. Although numerous studies have examined the degree to which females avoid inbreeding through extra‐pair reproduction (Reid et al. 2015a), the degree to which males alter offspring f through extra‐pair reproduction has not yet been examined.

The estimated sex‐specific selection gradients on the degree of inbreeding expressed through social pairing differed from each other to the degree that the 95% CIs for females mostly did not overlap the estimates for males measured to equivalent generational timepoints, and vice versa. Proximately, these patterns arose because females that paired with closer relatives tended to survive for more breeding years and hatched more offspring per year than females that paired with more distant relatives, but these relationships were less evident for males (Appendix S3). This apparent evidence that selection against pairing with a closer relative might be stronger in males than females contradicts the prevailing expectation that selection against inbreeding will be stronger in females (e.g., Pizzari et al. 2004; Parker 2006). However, estimates of overall selection on any trait, and consequent evolutionary predictions, can be biased by “invisible fractions” of individuals that do not express the focal phenotype and are consequently excluded from phenotypic selection analyses (e.g., Hadfield 2008; Mojica and Kelly 2010). Due to the study population's male‐biased adult sex ratio, 36% of adult male song sparrows never formed a socially persistent breeding pair and consequently did not express any degree of inbreeding through such pairing. These males cannot contribute to estimates of phenotypic selection because ƙ_mate is unobservable and undefined. Such socially unpaired males could potentially accrue some reproductive success by siring extra‐pair offspring of females that socially paired with other males. However in practice their success in siring banded offspring was low (see also Sardell et al. 2010; Lebigre et al. 2012) and their longer‐term fitness was zero; males that never socially paired contributed zero grand‐offspring to the study population. The most important component of male reproductive strategy in influencing fitness might therefore simply be to form a social pair irrespective of female relatedness rather than necessarily to choose among differently related females, especially if choice were to increase the probability of remaining unpaired.

ESTIMATED “SELECTION ON BEING INBRED”

Inbreeding depression in the fitness of offspring produced through biparental inbreeding is commonly measured as the slope of a regression of log‐fitness on f (thereby measuring “lethal equivalents,” assuming multiplicative effects of recessive alleles expressed across loci, Morton et al. 1956), and/or as the slope of a regression of raw fitness on f estimated within a statistically appropriate linear model (e.g., Keller 1998; Kruuk et al. 2002; Szulkin et al. 2007; Grueber et al. 2010; Reid et al. 2014). In contrast, inbreeding depression is not generally measured as the slope of a (multiple) regression of w‐standardized fitness on SD‐ or mean‐standardized f, thereby explicitly estimating phenotypic “selection on being inbred” on scales that facilitate quantitative comparison with other selection gradients, and allowing simultaneous estimation of selection on potentially correlated traits such as k (e.g., Lande and Arnold 1983; Hereford et al. 2004; Matsumura et al. 2012). Current analyses demonstrated strong selection against being inbred in female and male song sparrows, concurring with previous estimates of inbreeding depression in terms of lethal equivalents and other statistically appropriate regression slopes (Keller 1998; Keller et al. 2008; Reid et al. 2011b, 2014). Furthermore, in females, the magnitude of selection against being inbred estimated across adult grand‐offspring was twice that estimated across banded offspring, demonstrating that estimates of total inbreeding depression can increase substantially with the number of life‐history stages included in the measure of fitness (e.g., Szulkin et al. 2007; Grueber et al. 2010).

INTERPRETATION AND IMPLICATIONS

Our analyses imply that, despite strong inbreeding depression in fitness and consequent “selection against being inbred,” there might not be strong “selection against inbreeding” by female song sparrows in terms of forming socially persistent breeding pairs with relatives. Net selection against pairing with relatives might also be weak in males despite the negative selection gradients estimated across individuals that formed at least one social pairing, because individuals that never socially paired had zero direct long‐term fitness. Sexual conflict over pairing with relatives might therefore be weaker than initially indicated by the conflicting sex‐specific phenotypic selection gradients, and weaker than is commonly postulated (e.g., Pizzari et al. 2004; Parker 2006). If similar patterns have persisted over evolutionary time, they might explain why song sparrows do not avoid pairing with relatives (i.e., avoid inbreeding through one primary expression of pre‐copulatory mate choice, Keller and Arcese 1998; Reid et al. 2006, 2015b), thereby resolving the apparent “inbreeding paradox.” Indeed, even when inbreeding depression is strong, f typically explains little variance in fitness (Keller and Waller 2002; Kruuk et al. 2002). Consequently, there might commonly be substantial scope for variation in the magnitude and direction of net selection on the degree to which individuals pair with relatives, especially if females could also adjust offspring f and males could gain or lose fitness through extra‐pair reproduction. Social pairing between relatives also means that males are still somewhat related to extra‐pair offspring that they rear (i.e., extra‐pair offspring of their related socially paired female), potentially facilitating evolution of social traits such as parental care. Therefore, contrary to widely held expectations, an observation of strong inbreeding depression should not be assumed to imply that there will necessarily be selection against the formation of socially persistent breeding pairings among relatives, or consequent evolution of biparental inbreeding avoidance through pre‐copulatory mate choice.

However, any evolutionary inferences based on estimated phenotypic selection gradients are subject to multiple provisos. Primarily, they assume that focal phenotypic trait(s) directly and solely cause correlated variation in fitness (Rausher 1992; Kruuk et al. 2008; Morrissey et al. 2010). This might not be valid for the degree of inbreeding (or any other trait) when selection gradients are estimated from natural variation in inbreeding and fitness. Most obviously, variation in offspring f resulting from extra‐pair reproduction could also contribute to variation in individual fitness in reproductive systems characterized primarily by socially persistent breeding pairs. There is little evidence that female song sparrows actively or substantially alter offspring f through extra‐pair reproduction (Reid et al. 2015a,b). Future studies, on diverse systems, could usefully attempt to estimate selection on inbreeding expressed through extra‐pair mating or reproduction. Furthermore, the degree to which individuals inbreed is correlated with various traits and ecological circumstances in song sparrows and other species (Kruuk et al. 2002; Reid et al. 2008; Szulkin and Sheldon 2008; Herfindal et al. 2014). Phenotypic correlations between inbreeding and fitness might therefore arise indirectly rather than causally, due to correlated effects of other factors on both pairing and fitness. However, because song sparrows rarely paired with their own descendants, high individual fitness did not systematically cause high ƙ_mate (i.e., reversing the assumed direction of causality, Appendix S1).

Further major challenges in measuring selection on inbreeding, and predicting any evolutionary response, arise because the concept of “individual fitness” becomes complicated when mating decisions that affect inbreeding are made among numerous interacting relatives. The total fitness consequences of an individual's decision to pair with a relative (or not) cannot necessarily be quantified by summing an individual's direct reproductive success achieved with relatives and non‐relatives. This is because such summations do not incorporate inclusive fitness accrued through relatives with which a focal individual decides not to pair, but whose reproductive success might be influenced by that decision. For example, a focal individual's decision not to pair with a relative affects who that relative pairs with, and hence affects the fitness of the focal individual, and their rejected relative, and potentially of other relatives that the rejected individual subsequently pairs with (Duthie and Reid 2015). Comprehensive estimation of selection on inbreeding might therefore require simultaneous measurement of the fitness consequences of inbreeding that did not happen as well as inbreeding that did happen, which is not straightforward.

One useful future approach might be to directly estimate any evolutionary response to selection on inbreeding by estimating sex‐specific additive genetic covariances between the degree of inbreeding that individuals express and fitness. Given appropriate data and models, this explicit quantitative genetic approach could exclude environmental covariances, incorporate the fitness of individuals for whom phenotypic inbreeding cannot be observed (e.g., individuals that die before adulthood or never pair) and incorporate the relative fitness and degree of inbreeding expressed across numerous interacting relatives (e.g., Rausher 1992; Hadfield 2008; Morrissey et al. 2010; Reid 2012). Such analyses will require remaining conceptual and practical hurdles of appropriately measuring relatedness and fitness among numerous interacting relatives to be overcome.

DATA ARCHIVING

The doi for our data is doi: 10.5061/dryad.0015b.

Supporting information

Appendix S1. Distributions of coefficients of kinship.

Appendix S2. Expressions quantifying allelic value.

Appendix S3. Female and male lifespan and annual reproductive success.

Appendix S4. Additional selection gradients.

Appendix S5. Summary statistics for cohorts.

Appendix S6. Distributions of female and male fitness.