Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 Oct 31;7:13314. doi: 10.1038/ncomms13314

How EF-Tu can contribute to efficient proofreading of aa-tRNA by the ribosome

Jeffrey K Noel 1,2,3, Paul C Whitford 4,a
PMCID: PMC5095583  PMID: 27796304

Abstract

It has long been recognized that the thermodynamics of mRNA–tRNA base pairing is insufficient to explain the high fidelity and efficiency of aminoacyl-tRNA (aa-tRNA) selection by the ribosome. To rationalize this apparent inconsistency, Hopfield proposed that the ribosome may improve accuracy by utilizing a multi-step kinetic proofreading mechanism. While biochemical, structural and single-molecule studies have provided a detailed characterization of aa-tRNA selection, there is a limited understanding of how the physical–chemical properties of the ribosome enable proofreading. To this end, we probe the role of EF-Tu during aa-tRNA accommodation (the proofreading step) through the use of energy landscape principles, molecular dynamics simulations and kinetic models. We find that the steric composition of EF-Tu can reduce the free-energy barrier associated with the first step of accommodation: elbow accommodation. We interpret this effect within an extended kinetic model of accommodation and show how EF-Tu can contribute to efficient and accurate proofreading.

The translation of mRNA by the ribosome is governed by a series of large-scale conformational transitions. Here the authors use MD simulations to demonstrate how the rate of dissociation of elongation factor Tu affects the dynamics of tRNA accommodation and proofreading.

The ribosome is a large RNA-protein assembly (2–3 MDa) and it is the sole producer of proteins in the cell. The elongation process, in which messenger RNA (mRNA) is read and proteins are synthesized, involves multiple large-scale conformational transitions that result in individual amino acids being added to the growing protein chain1,2,3,4,5,6,7. Each round of elongation begins with the delivery of an aminoacyl-transfer RNA (aa-tRNA) molecule to the ribosome by elongation factor thermally unstable (EF-Tu in bacteria, or eEF1A in eukaryotes). During initial association of ternary complex (aa-tRNȦEF-Tu̇GTP) with the ribosome, codon–anticodon interactions are formed between aa-tRNA and mRNA. Based on differences in hydrogen bonding energies, a ternary complex that contains a cognate (correct) aa-tRNA molecule is only marginally more stable (several kBT) than one carrying a near-cognate aa-tRNA. Such small differences in stability can explain a preference for correct, over incorrect, tRNAs of approximately 100:1. However, in bacteria, the accuracy of translation is roughly 3,000:1, or greater (for review, see refs 1, 7). To account for this discrepancy, Hopfield showed that the fidelity of aa-tRNA selection could be amplified if it were composed of at least two steps that are separated by an irreversible chemical event8.

Decades of biochemical, structural and single-molecule measurements have provided a broad understanding of the tRNA selection process. The existence of proofreading was originally verified by biochemical data that implicated two distinct steps during tRNA selection (that is, initial selection and proofreading)9,10. During initial selection, aa-tRNA (in complex with EF-Tu·GTP) forms codon–anticodon interactions with mRNA that trigger GTPase activation and finally hydrolysis11,12,13. GTP hydrolysis is followed by Pi release, which signals a conformational change in EF-Tu (ref. 14) and aa-tRNA accommodation (the proofreading step). The observed level of proofreading is typically between 15 and 60 (refs 9, 10, 12, 13, 15, 16, 17, 18; though larger values have been reported19), where variations may arise from differences in experimental protocols and mismatch composition. From a structural perspective, cryoelectron microscopy (cryo-EM) and X-ray crystallography have elucidated the conformational properties of these biochemically identified states and have provided detailed insights into steps preceding and following accommodation20,21,22,23,24,25,26. To complement these static snapshots, single-molecule FRET (smFRET) studies have shed light on the dynamics of individual aa-tRNA molecules during initial selection27 and accommodation28. This body of work provides a strong structural and biochemical foundation, upon which our physical–chemical understanding may be refined.

Experimental studies have forcefully demonstrated that proofreading is associated with aa-tRNA accommodation, though the role of EF-Tu during this process is less clear. Accommodation involves a large conformational rearrangement (≈100 Å) that follows Pi release and precedes peptide bond formation, where kinetic partitioning at this step can account for observed levels of proofreading12,13. Consistent with a large rearrangement being necessary for accurate selection, subtle mutations to the aa-tRNA can perturb29 the A/T conformation (Fig. 1b) and impact the degree of proofreading30,31. With regards to the molecular factors that govern selectivity, biochemical studies have identified many important inter- and intra-molecular interactions17,32 and have shown that the same molecular features may be exploited during initial selection and proofreading15. However, the latter observation appears to be sensitive to the tRNA species, which suggests the possibility of multiple proofreading mechanisms19. Despite some remaining controversies (for review, see ref. 7), it is widely accepted that a delicate balance of kinetic steps is critical for accurate proofreading. In the prevailing kinetic model of proofreading, aa-tRNA accommodation and EF-Tu dissociation from the ribosome are treated as two independent and parallel steps1,12,13,15,17,18,19,30,32,33. Since they occur on comparable timescales14,33,34, the current study explores the physical relationship between these potentially inter-related events.

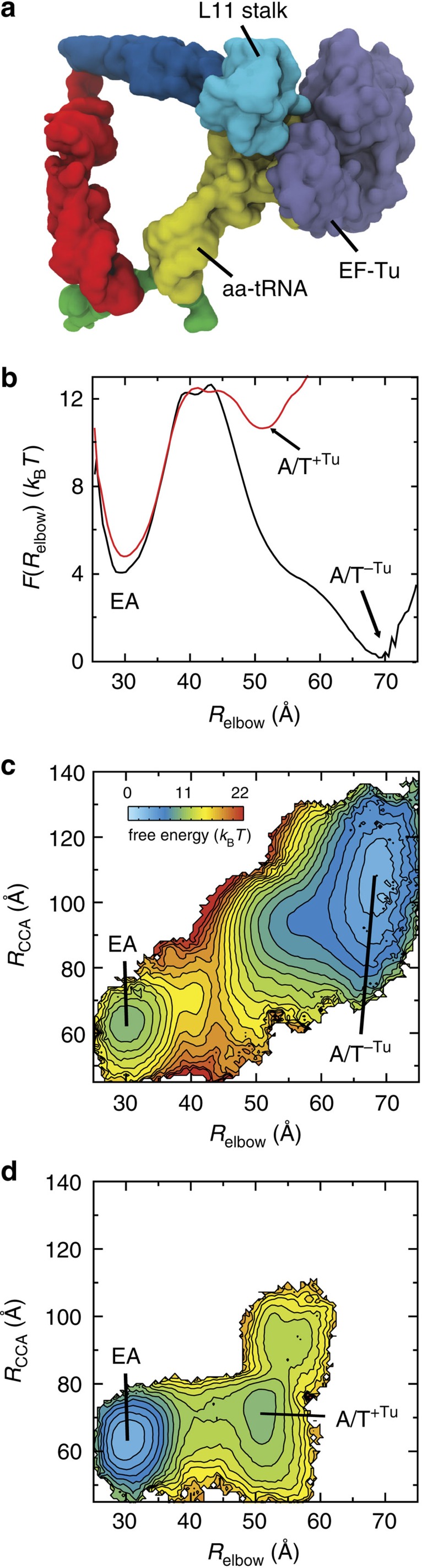
Figure 1. aa-tRNA elbow accommodation has a two-state-like free-energy landscape.

Figure 1

Open in a new tab

(a) Full 70S ribosome29 with 23S rRNA in grey, 16S rRNA in cyan and proteins in blue. The A/T-configured aa-tRNA is shown in yellow. P and E-site tRNAs are shown in red and orange. EF-Tu is shown in ice blue. The white/black box outlines the accommodation corridor35. (b) The first step of aa-tRNA accommodation involves elbow movement, where it transitions from the A/T to EA conformation and partially binds the ribosomal A-site. Relbow is the distance between the O3′ atoms of U8 in the P-site tRNA and U60 of aa-tRNA48. RCCA (separation of O3′ atoms of A76 in the aa-tRNA and P-site tRNA) monitors movement of the 3′-CCA end towards the peptidyl transferase centre. (c) With the employed model, the free energy as a function of Relbow possesses two dominant minima, which correspond to EA and A/T-like (labelled A/T−Tu) ensembles. The barrier arises from steric interactions between aa-tRNA and H89 (Supplementary Fig. 2). All structural depictions were prepared using VMD70.

Computational35,36 and experimental28,37,38 studies have implicated the following sequence of conformational rearrangements during aa-tRNA accommodation: (1) aa-tRNA moves from an A/T to an elbow-accommodated (EA) conformation, (2) the aa-tRNA arm accommodates into the A-site and (3) the 3′-CCA end enters the peptidyl transferase centre (PTC). The first simulations of factor-free accommodation employed targeting techniques and showed that sequential movement of the elbow, arm and 3′-CCA end is sterically accessible35. Unrestrained simulations that used an electrostatics-free model corroborated this overall ordering of events and predicted that the EA conformation represents an intermediate (that is, a local free-energy minimum)36. smFRET measurements have shown that the incoming aa-tRNA may reversibly sample EA-like and A/T-like ensembles before peptide bond formation occurs28, consistent with elbow accommodation preceding 3′-CCA accommodation. Other experiments have found that mischarging aa-tRNA (attaching the wrong amino acid) does not alter elbow dynamics37, which suggests elbow and 3′-CCA accommodation are separable events. Finally, a cryo-EM reconstruction of the ribosome in complex with the antibiotic HygA found the elbow to be in an accommodated position, while the 3′-CCA end was displaced from the A-site38. The same study also applied smFRET and reported the signature of an intermediate in which the elbow is nearly fully accommodated, which would appear to be consistent with the predicted EA intermediate36. Taken together, these theoretical and experimental studies implicate a multi-step accommodation process that begins with reversible elbow movement into the A-site and finishes with 3′-CCA end accommodation into the PTC.

Through the use of energy landscape principles39,40 and molecular dynamics simulations, we provide evidence for how the rate of EF-Tu dissociation can affect accommodation dynamics and proofreading. By employing a model in which all non-hydrogen atoms are represented, these simulations highlight the large influence steric interactions can have on the free-energy landscape of accommodation. Specifically, we find that the steric composition of EF-Tu allows it to increase the rate of elbow accommodation by several orders of magnitude. While we find a conformational change in EF-Tu can amplify this effect, it is not required. These observations show how EF-Tu may drive aa-tRNA into a partially accommodated conformation, which provides a molecular-level physical–chemical explanation for how EF-Tu may help facilitate efficient proofreading by the ribosome.

Results

An energetic model for aa-tRNA accomodation

There are many force fields available for the simulation of biomolecules, each suited to address different aspects of the dynamics. For example, explicit-solvent models provide a detailed description of RNA and protein energetics, which has enabled the simulation of folding of tetraloops41,42 and the calculation of free-energies for isolated regions of the ribosome43,44, as well as the evaluation of enthalpies45 and diffusion coefficients46 for fully-assembled ribosomes. While these models have proven to be effective when describing small-scale/narrowly defined conformational transitions, the vast phase space associated with accommodation makes it computationally intractable to calculate the free energy. In contrast, through the use of simpler models, one may evaluate and compare the free energy of accommodation for a variety of molecular constructs. In the current study, we adopt this approach and perform simulations using an all-atom structure-based model (SBM)47,48 constructed from a structural model of the ribosome in complex with aa-tRNAPhe (ref. 25). This allows us to identify how the shape of the ribosome and EF-Tu contribute to the free-energy landscape of elbow accommodation (the first substep of accommodation).

In the model used here, all non-hydrogen atoms are explicitly represented, and interatomic interactions specifically stabilize the A/A conformation (the endpoint of accommodation, Fig. 1b). This construction of the potential energy is referred to as a structure-based model (SBM) since the minimum is defined by a preassigned structure. In this class of models, electrostatic and solvent contributions are typically accounted for implicitly, where each stabilizing interaction describes the net effect of the myriad energetic factors that favour the experimentally obtained structure49. In addition to the purely structure-based interactions, we also include explicit short-range electrostatic (Debye-Hückel) interactions between the tRNA and accommodation corridor, as employed in protein-DNA50 and protein folding51 simulations. The rationale for including these non-specific interactions (that is, those not found in the A/A structure) is that the aa-tRNA molecule samples conformations far from A/A during accommodation. As discussed below, we find that the magnitude of the free-energy barrier depends on the electrostatic details. However, qualitative similarities between the free-energy profiles obtained with electrostatic and electrostatics-free models (Supplementary Fig. 1) suggest that biomolecular structure is a major determinant of the overall character of elbow accommodation.

Kinetics and free energy of factor-free accommodation

With the employed forcefield, the free-energy profile associated with aa-tRNA elbow accommodation has two clear minima that are separated by a significant free-energy barrier (Fig. 1c). The minima correspond to EA (Relbow∼30 Å) and extended (A/T-like, Relbow∼70 Å) ensembles. We will refer to the extended ensemble as A/T−Tu, since EF-Tu is not included in this calculation. In the A/T−Tu basin, aa-tRNA is base paired with mRNA, and it can adopt larger values of Relbow than found in A/T crystal structures24,29. For a more detailed description of the A/T ensemble, see ref. 36. Note that, since the current calculations only sampled the A/T to EA transition, and not 3′-CCA end accommodation, the EA free-energy minimum is shifted slightly from the A/A value of Relbow36.

To describe the free-energy barrier associated with elbow accommodation, one may inspect the structural properties of the transition state ensemble (TSE)52,53,54. In the current model, steric interactions lead to displacement of H89 in the TSE (Supplementary Fig. 2c), consistent with the transient formation of H89-tRNA interactions35,48 and previous predictions of H89 movement36. Experimentally, mutations in the vicinity of H89 have been found to affect accommodation55, which also suggests H89-tRNA interactions may be formed in the TSE. To verify that the barrier arises from H89 interactions, we repeated our calculations using a model in which H89-tRNA interactions are absent. Upon making this virtual deletion of H89, the free-energy barrier is largely attenuated (Supplementary Fig. 2a,b). These observations highlight how the ribosome can impose strict boundaries on the available aa-tRNA conformations, which lead to this sterically induced free-energy barrier.

To calculate rates from free-energy profiles, we describe elbow accommodation in terms of diffusive movement across a free-energy landscape39,52,56. Specifically, rates are calculated via the relation39,57:

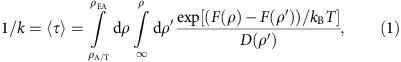graphic file with name ncomms13314-m1.jpg

where ρ is the reaction coordinate Relbow (the distance between U60 in aa-tRNA and U8 in the P-site tRNA) and D is the diffusion coefficient (estimated from explicit-solvent simulations46). While interatomic distances can provide intuitive descriptions of elbow movement, the full dynamics are multi-dimensional. To ensure that the essential characteristics of the process are not masked by the choice of coordinate58, we previously showed that Relbow can reliably separate the end points and accurately capture the TSE associated with elbow accommodation48. Further, motion along Relbow was observed to be diffusive in the neighbourhood of the TSE. Accordingly, one may use equation (1) to calculate rates from free-energy profiles. As an example, the barrier in Fig. 1c would correspond to a rate of ∼20 s−1 for EF-Tu-free elbow accommodation, which is similar to experimentally determined rates for full accommodation (∼40 s−1 (ref. 16)). The similarity of these numbers suggests that the theoretical free-energy barriers are within a biologically relevant kinetic regime, though the quantitative relationship between biochemically measured rates and the elbow accommodation substep has not been rigorously established.

EF-Tu can accelerate elbow accommodation

Inspired by the experimental observation that aa-tRNA accommodation and EF-Tu dissociation occur on comparable timescales14,33,34, we asked whether EF-Tu can directly influence the rate of accommodation after it releases the tRNA molecule. To this end, we used our simplified model to calculate the free energy of aa-tRNA elbow accommodation with EF-Tu bound to the ribosome in its post-hydrolysis, pre-Pi-release conformation21,29. Relative to the calculations described in Fig. 1, the only difference in this set of simulations is that EF-Tu is restrained to a ribosome-bound conformation29: TuA/T (Fig. 2a). We find that the presence of EF-Tu shifts the A/T basin by 15 Å, from Relbow≈70 Å to ≈55 Å (Fig. 2b). In this implementation of the model, EF-Tu and aa-tRNA only interact through non-specific electrostatic and excluded-volume interactions. Despite the fact that these interactions slightly favour A/T conformations (Supplementary Fig. 3), the A/T ensemble is destabilized by more than 10 kBT (6 kcal mol−1, Fig. 2b). One may interpret this as arising from a reduction in the number of conformations that are accessible to the tRNA molecule when EF-Tu is present. That is, many A/T-like conformations are sterically occluded by the presence of EF-Tu (Fig. 2d), where more extended conformations are only accessible when EF-Tu is absent (Fig. 2c). An interesting observation is that, while EF-Tu significantly affects the stability of the A/T ensemble, the location of the free-energy barrier (Relbow≈40 Å and RCCA≈70 Å) is consistent with that observed in the EF-Tu-free simulations. This indicates that, rather than change the dominant route of accommodation, EF-Tu helps aa-tRNA overcome this pre-existing barrier.

Figure 2. EF-Tu occludes access of aa-tRNA to A/T-like conformations.

Figure 2

Open in a new tab

(a) EF-Tu, in the form of ternary complex (EF-Tu̇GTṖaa-tRNA), binds the ribosome at the opening of the accommodation corridor20,21,22,23,24,26,29. (b) When EF-Tu is restrained to its GTP conformation on the ribosome, and a minimal affinity for aa-tRNA is included, the barrier to elbow accommodation is reduced by ∼10 kBT (red curve), relative to the barrier when EF-Tu is absent (black curve). (c,d) Two-dimensional free-energy profiles, calculated for the models that lack EF-Tu (c) and include EF-Tu (d). There is a drastic reduction in the range of accessible conformations of aa-tRNA when EF-Tu is bound to the ribosome. This leads to destabilization, and shift in position, of the A/T-like ensemble (A/T−Tu in c and A/T+Tu in d).

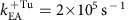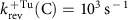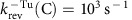The decrease in barrier height when EF-Tu is bound raises the possibility that elbow accommodation is accelerated by EF-Tu. In accordance with the discussion in the previous section, we used equation (1) to calculate the predicted rate of elbow accommodation in the presence of EF-Tu. The profiles in Fig. 2b would implicate the following rates for cognate aa-tRNA: Inline graphic, Inline graphic, Inline graphic and Inline graphic, where (C) indicates that a rate is associated with cognate aa-tRNA and the subscript rev denotes reverse-elbow accommodation. It is important to recognize that, while 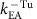Inline graphic is comparable to experimentally reported rates of full accommodation16, the scale of the barrier can be influenced by minor changes in the electrostatic model. Accordingly, the overall magnitude of the rates should not be interpreted as a precise prediction. Instead, the principal conclusion one should draw is that the rate of elbow accommodation can be increased significantly (>1,000-fold) if EF-Tu releases aa-tRNA before dissociating from the ribosome.

Robustness of EF-Tu-accelerated elbow accommodation

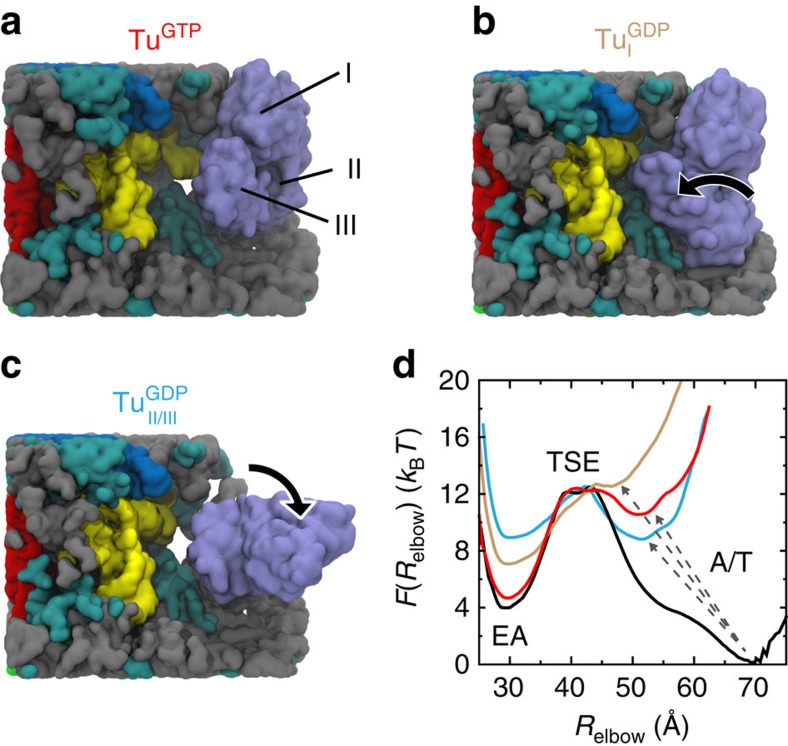
To further characterize the role of EF-Tu sterics on accommodation, we considered additional conformations that EF-Tu may adopt before it dissociates from the ribosome. While it is known that the formation of codon–anticodon interactions triggers GTP hydrolysis, which leads to Pi release and a conformational change in EF-Tu11,12,13, the precise role of this large-scale rearrangement is not fully understood. Accordingly, we constructed several structural models of potential post-Pi-release configurations, to determine whether EF-Tu-accelerated elbow accommodation is robust to the conformation of EF-Tu. In the pre-Pi-release conformation of ternary complex20,21,22,23,24, the 3′-CCA end of aa-tRNA binds to a crevice between domains I and II of EF-Tu. This interface is not formed in the GDP form of EF-Tu (ref. 59), consistent with its reduced affinity for aa-tRNA60. In addition, in the GDP form, there is a displacement of domain I and the II/III superdomain (Fig. 3a–c), relative to the GTP form. To study the effects of such a rearrangement on accommodation, we considered two possible orientations of EF-Tu·GDP59, where it was partially aligned (see the ‘Methods' section) to the ribosome-bound GTP conformation of EF-Tu (ref. 29). In the first scenario, interactions between domain I and the ribosome remain intact, while domains II/III are displaced (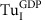Inline graphic, Fig. 3b). Alternately, the interface between domains II/III and the ribosome may remain formed, while domain I pivots (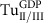Inline graphic, Fig. 3c).

Figure 3. The steric influence of EF-Tu on accommodation is robust.

Figure 3

Open in a new tab

(a) EF-Tu in a pre-Pi-release conformation, aligned to the simulated system. In this conformation, domain I of EF-Tu contacts the 50S subunit, while domains II/III contact protein S12 and the neighbouring RNA on the 30S subunit. Upon Pi release, EF-Tu undergoes a conformational transition while still associated with the ribosome33, where at least one point of contact is likely to be maintained. To probe the influence of EF-Tu·GDP on accommodation, we constructed hypothetical structural models, where EF-Tu·GDP is aligned to either (b) domain I 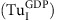Inline graphic, or (c) domains II/III Inline graphic of the EF-Tu·GTP structure. In panels a–c, RNA and proteins in the accommodation corridor are coloured gray and cyan, and the remaining molecules are coloured as in Fig. 2. The L11 stalk is not visualized, though it was included in all simulations. (d) For all models, the A/T basin is significantly destabilized (arrows) when EF-Tu is present. In contrast to the shift in position of the A/T-like basin, the locations of the EA basin and the TSE are insensitive to the conformation of EF-Tu.

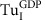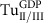To assess the kinetic consequences of a structural rearrangement in EF-Tu before dissociation from the ribosome, we recalculated the free-energy profiles for accommodation with EF-Tu in the Inline graphic, or Inline graphic, configuration (Fig. 3d). In the 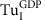Inline graphic configuration, domains II/III are displaced towards the ribosomal A-site (relative to TuA/T), in a manner that would resemble a power-stroke-like conformational change. This configuration of EF-Tu precludes sampling of the A/T-like ensemble and yields a free-energy profile that is ‘downhill' in the direction of accommodation (Fig. 3d, tan curve). In the 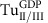Inline graphic configuration, domain I is displaced away from the ribosomal A-site. While this represents a markedly different orientation of EF-Tu, the 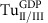Inline graphic configuration also significantly destabilizes the A/T ensemble (Fig. 3d, cyan curve). This demonstrates that the effect of EF-Tu on accommodation is robust to the precise sequence of rearrangements that may occur after EF-Tu releases the tRNA molecule. Specifically, given that EF-Tu remains nominally associated with the ribosome, the elbow accommodation barrier should be significantly reduced. While the precise value of the barrier depends on the conformation, we find that EF-Tu destabilizes the A/T basin by at least 8 kBT for all post-hydrolysis models, relative to when EF-Tu is absent. Since the rate is exponentially related to the barrier height, these results reinforce the finding that elbow accommodation may be accelerated by EF-Tu (by a factor of e8∼103). This sterics-based mode of acceleration is distinct from other assemblies that directly utilize chemical energy to overcome a rate-limiting barrier61,62. That is, these results implicate an indirect mode of coupling between hydrolysis on EF-Tu and accommodation dynamics, though a power stoke may amplify the effect.

An extended kinetic model for proofreading

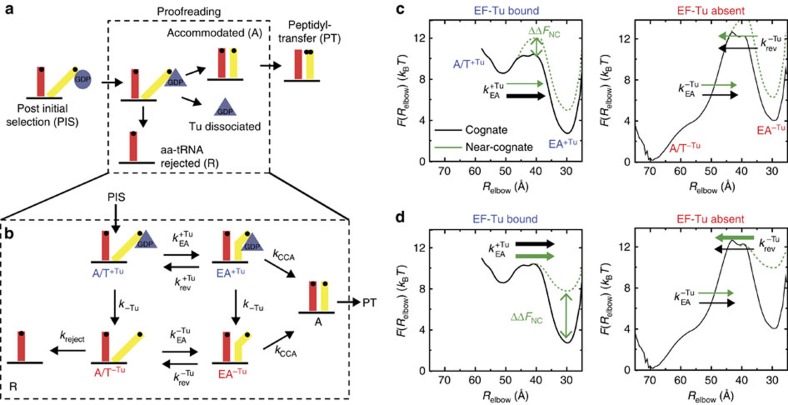
Biochemical analysis has provided an intricate kinetic description of the elongation process12,13,15,17,18,19,30,32,33,63. With the current calculations suggesting that EF-Tu may influence the rate of accommodation, we have extended the prevailing kinetic model for proofreading (Fig. 4a) to include substates that account for the relationship between EF-Tu and accommodation (Fig. 4b). The essential features of this extended model are: (1) significant free-energy barriers are associated with aa-tRNA elbow accommodation and 3′-CCA end accommodation36. (2) aa-tRNA can reversibly sample EA-like conformations28. (3) The rate of forward elbow accommodation is accelerated by EF-Tu̇GDP. (4) EF-Tu̇GDP dissociation from the ribosome is irreversible. One should note that allowing for re-association of EF-Tu̇GDP is kinetically equivalent to decreasing the effective rate of dissociation (k−Tu), which does not qualitatively change the presented analysis. (5) We assume that a rejected aa-tRNA follows the same path, but in the opposite direction, as an accepted aa-tRNA. As a consequence, aa-tRNA may only be rejected after EF-Tu dissociates from the ribosome. (6) 3′-CCA end accommodation is treated as irreversible. This assumption is supported by the fact that it is possible to crystallize the A/A conformation for both cognate and near-cognate tRNA64, indicating that the A/A conformation can be very stable. This set of conditions suggests a kinetic model for accommodation that consists of the following six states (Fig. 4b): aa-tRNA may adopt an A/T-like conformation (post initial selection) with EF-Tu bound (A/T+Tu) or without EF-Tu bound (A/T−Tu) to the ribosome; aa-tRNA may adopt an EA conformation in the presence of EF-Tu (EA+Tu), or in the absence of EF-Tu (EA−Tu); aa-tRNA may be fully accommodated (A) or rejected (R).

Figure 4. Simulated landscapes suggest a kinetic model for proofreading that includes the influence of EF-Tu on elbow accommodation.

Figure 4

Open in a new tab

(a) Schematic representation of the current model of proofreading12,13,15,17,18,19,30,32,33. After initial selection is completed (PIS), accommodation, EF-Tu dissociation and rejection are described as parallel kinetic steps. (b) An extended kinetic model for accommodation suggested by simulations. After initial selection, aa-tRNA will reach one of two states: accommodated (A) or rejected (R). The top branch (EF-Tu bound) transitions to the bottom branch (EF-Tu absent) at the rate of k−Tu. (c) The free-energy profiles describing the transition between A/T and EA conformations are shown for EF-Tu bound (left) and EF-Tu absent (right). An induced fit mechanism of accommodation would imply an increased barrier height for near-cognate tRNA (ΔΔFNC). 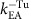In terms of the extended kinetic model, this would correspond to smaller values for Inline graphic and Inline graphic for near-cognate molecules, while leaving 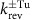Inline graphic unchanged. (d) An alternative potential effect of near-cognate codon interactions is that the EA ensemble is destabilized. In that scenario, the forward rates would be identical for all tRNA molecules, whereas the reverse rates would change. To explore the impact of EF-Tu dissociation on fidelity, the proofreading factor and efficiency were calculated for each of these energetic descriptions of near-cognate molecules.

The extended kinetic model (Fig. 4b) was used to evaluate the selectivity of proofreading, as well as the efficiency for a range of kinetic parameters. These calculations specifically focused on the proofreading factor Pf. Proofreading contributes to the total selectivity of translation according to I × Pf, where I is the selectivity of initial selection. To calculate Pf, we first derived expressions for the rates of rejection and accommodation under the steady-state approximation, which entails analysing the populations of states A and R, given a fixed population of the A/T+Tu state. This provides the probability that aa-tRNA will be accepted (A) or rejected (R) after successfully passing initial selection (labelled PIS in Fig. 4). Pf is defined as the ratio of cognate ([A]C) to near/non-cognate ([A]NC) tRNA that arrive at the fully accommodated state:

graphic file with name ncomms13314-m14.jpg

The efficiency E of proofreading is then defined as the number of cognate tRNA molecules that are accommodated, relative to the total number of cognate tRNA molecules that pass initial selection:

graphic file with name ncomms13314-m15.jpg

For a detailed discussion of the kinetic equations, see Supplementary Methods. Below, we consider two different ways in which the landscape may depend on cognate and near-cognate interactions. For both types of landscape effects, we calculated the corresponding proofreading factor and efficiency for a wide range of EF-Tu dissociation rates. This analysis suggests how previously measured levels of proofreading may depend, in part, on the precise timing of EF-Tu release from the ribosome.

EF-Tu and the efficiency of induced fit based proofreading

To explore the potential biological implications of EF-Tu-accelerated elbow accommodation, we used the extended kinetic model (Fig. 4b) to study an induced fit description of accommodation. Induced fit dynamics is consistent with bulk kinetic measurements that have found the rate of accommodation to be faster for cognate aa-tRNA, than for near-cognate12. These differences in rates may be schematically described as an increase in the free-energy barrier for near-cognate molecules (Fig. 4c). In terms of the kinetic model, we simply assigned the rate of elbow accommodation to be 50-fold slower for near-cognate molecules. We then calculated the proofreading factor and efficiency as functions of the EF-Tu dissociation rate k−Tu (Fig. 5a).

Figure 5. Rate of EF-Tu dissociation influences the proofreading factor Pf.

Figure 5

Open in a new tab

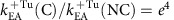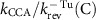(a) Pf is shown as a function of Inline graphic for the induced fit mechanism of proofreading (Fig. 4c). For this calculation, accommodation of cognate tRNA is modelled as being 50-fold faster than near-cognate: Inline graphic. Only Inline graphic =10 is shown since Pf is only sensitive to this ratio when it is <1. (b) For the EA-destabilized mode of proofreading (Fig. 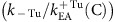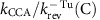4d), Pf depends on the relative rates of EF-Tu dissociation Inline graphic and 3′-CCA accommodation Inline graphic. Each curve is labelled by the value of the relative rate of 3′-CCA accommodation (Inline graphic). A representative value of ΔΔFNC=8 kBT was used to demonstrate the overall behaviour of Pf. In contrast to the induced fit description, proofreading through EA destabilization depends on the value of kCCA. This is because, when tRNA is in the EA basin, the reverse step for near-cognate tRNA, with a rate of 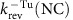Inline graphic, will be competitive with forward accommodation (rate of kCCA). In contrast, the reverse barrier is large for all molecules in the induced-fit framework. Common to both descriptions is that fidelity increases monotonically with the rate of EF-Tu dissociation k−Tu. Solid (dashed) lines distinguish between regions where efficiency is greater (less) than 0.9. As the rate of EF-Tu dissociation increases, the efficiency decreases. This suggests that EF-Tu dissociation should be sufficiently slow, such that it may accelerate elbow accommodation (increase efficiency), yet fast enough to allow for rejection (increase selectivity).

For codon–anticodon mismatches that obey induced fit dynamics, the extended kinetic model predicts the proofreading factor will depend monotonically on k−Tu. When EF-Tu dissociation is very slow, Pf approaches 1 (no proofreading), since EF-Tu is assumed to impede aa-tRNA dissociation from the ribosome, irrespective of the codon. Accordingly, the efficiency would be high (Fig. 5a, solid line) since all molecules would eventually reach the accommodated state A. Pf then increases with k−Tu, indicating that kinetic partitioning of cognate and near-cognate molecules is possible12. As k−Tu is increased, it also becomes increasingly likely that EF-Tu will dissociate before cognate elbow accommodation, which leads to population of the A/T−Tu state. When this occurs, the barrier encountered by aa-tRNA is expected to be significantly increased in magnitude (Fig. 2b). Thus, the probability of rejecting a cognate aa-tRNA will increase, and the efficiency will decrease (Fig. 5a, dashed line). This behaviour suggests a very dynamic picture of proofreading, where EF-Tu dissociation should be sufficiently slow that it can assist accommodation (increase efficiency), yet fast enough to allow for rejection (enable selectivity).

In addition to increasing the barrier height, near-cognate interactions may also destabilize the A/T ensemble, which could lead to codon-dependent value of kreject. In support of this, near-cognate tRNAs are more rapidly rejected than cognate molecules12. Since this will amplify the degree of proofreading, the values of Pf described here should be considered lower bounds.

Balancing EF-Tu dissociation and reverse accommodation

While the above analysis suggests that EF-Tu can play a pivotal role within an induced fit framework, we now address the relevance of EF-Tu-accelerated accommodation within an alternate kinetic description for proofreading. Specifically, we ask how EF-Tu-accelerated accommodation may contribute to proofreading if the EA ensemble were to be destabilized by near-cognate interactions. Recent structural models suggest that the steric characteristics of the decoding centre may lead to destabilization of the accommodated conformation for near-cognate aa-tRNA molecules64. That is, when aa-tRNA adopts the A/A conformation, the decoding centre (h18, h44, S12 and H69) sterically confines the first two bases, such that they are forced to adopt a cognate-like geometry. A near-cognate pair is then energetically penalized, relative to its relaxed state, by either breaking its hydrogen bonds or distorting the decoding centre. Free-energy calculations have estimated the penalty for a near-cognate codon to be ∼10–15 kBT (ref. 43), though it is not known how this is distributed between the A/T, transition state, EA and A/A ensembles. As discussed in the previous section, destabilization of the TSE (increasing the barrier height) would lead to induced fit dynamics and destabilization of the A/T ensemble would likely increase the rate of rejection kreject. To complement these landscape descriptions, we now considered the kinetic effects of EA destabilization (ΔΔFNC in Fig. 4d). If the EA ensemble were destabilized by near-cognate interactions, the reverse-elbow accommodation rearrangement could serve as a proofreading step, where codon-dependent destabilization would lead to tRNA-dependent rates of reverse elbow accommodation krev.

If near-cognate interactions destabilize the EA ensemble, the proofreading factor Pf will depend monotonically on the rate of EF-Tu dissociation (Fig. 5b). Again, the physical interpretation for this dependence is that EF-Tu dissociation should be sufficiently fast that near-cognate molecules may be rejected, yet slow enough to maintain efficient accommodation of cognate tRNA. An interesting qualitative distinction between our analysis of the induced fit framework and the EA-destabilized framework is that in the latter, Pf and E depend on the rate of 3′-CCA end accommodation. This is due to the fact that the elbow accommodation barrier is predicted to be very small for all tRNA molecules when EF-Tu is present, in the EA-destabilized framework. Thus, EF-Tu would load cognate and near-cognate tRNA molecules into the marginally stable EA intermediate. Smaller values of kCCA would be associated with a prolonged sampling of the EA−Tu state, which would increase the probability reaching the A/T−Tu state, for all tRNA molecules. By returning to the A/T−Tu state, the tRNA may be subsequently rejected, which would reduce the chance of incorporating a mistake, while also reducing the efficiency.

The unique influence of kCCA within the EA-destabilized proofreading approach provides an avenue for experimentally determining the extent to which this mechanism is employed. For example, it may be possible to introduce mutations near the PTC that specifically increase/decrease kCCA. A strong dependence of fidelity on these mutations would then be consistent with the utilization of a reverse-elbow proofreading mechanism.

Discussion

It is widely recognized that proofreading by the ribosome is accomplished during a conformational step related to accommodation1,4,12,13,15,17,18,19,20,21,22,23,24,25,27,28,30,32,33,63. In an effort to provide insights into the physical–chemical properties of proofreading, we have analysed aa-tRNA elbow accommodation through the lens of energy landscape theory. Our simulations predict that EF-Tu can drive aa-tRNA towards a partially accommodated intermediate. By integrating this result within a kinetic model, we have provided two explanations for how EF-Tu may facilitate efficient proofreading. The most striking result is that a balance between the rates of EF-Tu dissociation and aa-tRNA accommodation can heavily influence the level and efficiency of proofreading.

From a physical perspective, the current study demonstrates how the structure of the ribosome can shape the free-energy landscape that governs biological dynamics. In the context of accommodation, multiple free-energy barriers are introduced by steric features that impede aa-tRNA motion. Here, we have shown how EF-Tu can help aa-tRNA overcome one such steric obstacle, H89. Relative to general discussions pertaining to the stochasticity of elongation, the predicted action of EF-Tu is reminiscent of the Brownian ratchet model of elongation65, where the steric contributions of EF-Tu increase the probability of tRNA crossing a large free-energy barrier.

Taken together, the presented simulations and kinetic model reveal how the structure of the ribosome can contribute to efficient proofreading. However, with the critical role of gene expression, the ribosome has likely evolved redundant strategies19,44 to ensure that accurate selection is robust across aa-tRNA species and cellular conditions. As biophysical investigations continue to explore the many facets of ribosome dynamics, the current study illustrates how the combination of experimental observations, computational tools and theoretical modelling may be used to identify robust biomolecular relationships that underly function.

Methods

Structure-based model (SBM)

All simulations employed a structure-based model (SBM)47. The SBM was implemented as in ref. 48, with the exception that non-specific electrostatic interactions are also included here. In the SBM, the potential energy minimum is defined by the A/A conformation25. All non-hydrogen atoms are included and each atom is represented as a bead of unit mass. Bond lengths, bond angles, improper dihedrals and planar dihedrals are maintained by harmonic potentials. The dihedral interactions are defined such that each angle is at a minimum in the A/A conformation. Non-bonded atom pairs that are in contact in the A/A conformation are given attractive interactions. Since intermolecular arm and 3′-CCA contacts only form after elbow accommodation35,36, they were not included in the current model48. This modification was introduced so that the calculations could specifically focus on the elbow accommodation step, and not the subsequent rearrangements. Additionally, stabilizing interactions between the A-site tRNA and P-site tRNA (elbow contacts) were scaled by 0.5. This reduction in the strength of A/A-specific contacts is in accordance with each contact representing effective interatomic energetics49. That is, the intramolecular interactions that maintain the structural integrity of the ribosome and each tRNA molecule are effectively more stable than the transiently formed intermolecular interactions between tRNA molecules. All atom pairs that do not interact via bonded or contact terms were given repulsive interactions, which account for the atomic excluded volume. In the current implementation of the SBM, non-specific electrostatic interactions between aa-tRNA and the ribosome were also included. The functional form of the potential is given by:

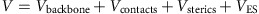graphic file with name ncomms13314-m16.jpg
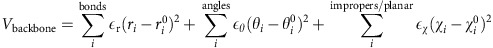graphic file with name ncomms13314-m17.jpg
graphic file with name ncomms13314-m18.jpg
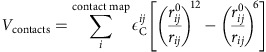graphic file with name ncomms13314-m19.jpg
graphic file with name ncomms13314-m20.jpg
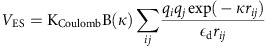graphic file with name ncomms13314-m21.jpg
graphic file with name ncomms13314-m22.jpg

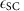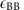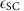Vcontacts describes short-range attractive interactions between atoms i and j that are in contact in the A/A conformation. A 4 Å cutoff was used to define the native contacts66. As described elsewhere67, three ratios are defined to assign values of Inline graphic, Inline graphic and Inline graphic. (1) Inline graphic and Inline graphic are scaled so that 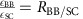Inline graphic. (2) The energetic weight of each dihedral and contact is also scaled, such that the ratio of total contact energy to total dihedral energy 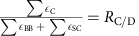Inline graphic, is satisfied. (3) The total stabilizing energy is set, such that 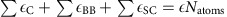Inline graphic, where Inline graphic is the reduced energy unit. RBB/SC=2 for protein, RBB/SC=1 for RNA and RC/D=2. 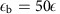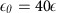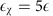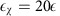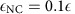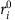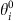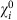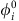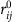Inline graphic, Inline graphic, Inline graphic for improper dihedrals, Inline graphic for planar dihedrals, and Inline graphic. Inline graphic, Inline graphic, Inline graphic, Inline graphic and Inline graphic are given the values found in the A/A crystal structure, and rNC=2.5 Å. Contacts between stacked RNA bases (bases adjacent in sequence) are scaled by 1/3 relative to all other contacts. Electrostatic interactions were modelled by a Debye-Hückel potential (VES), as in ref. 50. According to Debye–Hückel theory, the electrostatic interactions are screened by monovalent ions with a characteristic Debye screening length κ−1. For dilute solutions of monovalent ions at room temperature, B(κ)≈1 and 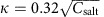Inline graphic. Here, an effective salt concentration of 0.05 M was used, corresponding to κ=1.4 nm, and a dielectric 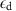Inline graphic of 80 rescaled into reduced units (see Supplementary Methods). Crystallographic magnesium ions were given a charge +2, the phosphorous atom of each nucleic acid was given a charge of −1, and Arg, Lys, Asp and Glu residues were charged ±1 (His were uncharged). 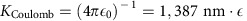Inline graphic.

Structural models

A crystallographic model of the A/A structure (PDB code: 3I8F)25 was used to define the EF-Tu-free (−Tu) SBM. To reduce the computational requirement of each simulation, only the atoms within a rectangular prism containing the accommodation corridor (23,888 of ∼150,000 atoms) were explicitly included in the simulations. This reduction in the number of atoms resulted in numerous boundary atoms having interactions removed from the model. To avoid introducing artifacts, the boundary atoms were subject to harmonic restraints centred at the crystallographic coordinates. The strength of each restraint was determined through an iterative fluctuation-matching protocol, as described previously48. There is excellent agreement between the fluctuations in the truncated system and the full ribosome, where the correlation coefficient of the atomic root mean squared fluctuations (RMSF) values is 0.98 (Supplementary Fig. 4).

The structural model containing EF-Tu in its GTP conformation (TuGTP) was generated by aligning an A/T structure of the ribosome with EF-Tu bound29 to the small ribosomal subunit of the −Tu model. The aligned coordinates of EF-Tu were then directly used to restrain EF-Tu during the simulations. The alignment process introduced no steric clashes between EF-Tu and the ribosomal corridor, where no atoms were closer than 3 Å, and 20 atom pairs were within 4 Å. 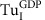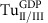The two models of possible post-Pi-release conformations of EF-Tu, Inline graphic and Inline graphic, were generated by fitting the GDP crystal structure of EF-Tu (ref. 59) to either domain I or the II/III superdomain of TuGTP. For both, the RMSD of the fitted atoms was <3 Å, showing that the conformational change in EF-Tu can be predominantly described as a relative displacement of domains.

Molecular dynamics simulations

Free-energy profiles were calculated from molecular dynamics simulations performed with GROMACS v4.5.3 (ref. 68). The input forcefield files were generated by the SMOG web server (http://smog-server.org)67. In simulations that included EF-Tu, each atom in EF-Tu was restrained to the assigned position. Umbrella sampling was applied by imposing restraints on the distance between the geometric centres of the tRNA elbows. About the TSE, an additional umbrella restraint roughly perpendicular to the elbow displacement was necessary to sample both the major and minor groove routes (see Supplementary Fig. 5 for details). The Weighted Histogram Analysis Method69 was used to obtain the free energy as a function of Relbow (distance between U8 in the P-site tRNA and U60 in the A-site tRNA, as defined as in ref. 48). The positions of individual umbrellas were sufficiently close that the full range of Relbow values were sampled (Supplementary Fig. 6). Umbrella simulations were performed twice, once after initializing the simulations by iteratively increasing Relbow and then again by decreasing Relbow (Supplementary Fig. 7). For a summary of all systems simulates, see Supplementary Table 1. See Detailed Methods in Supplementary Information for full simulation details.

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.

Additional information

How to cite this article: Noel, J. K. & Whitford, P. C. How EF-Tu can contribute to efficient proofreading of aa-tRNA by the ribosome. Nat. Commun. 7, 13314 doi: 10.1038/ncomms13314 (2016).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Material

Supplementary Information

Supplementary Figures 1-7, Supplementary Table, Supplementary Methods and Supplementary References.

ncomms13314-s1.pdf (1.2MB, pdf)

Acknowledgments

We thank Ryan Hayes for discussions on the kinetic model. We would also like to thank Kien Nguyen for his critical feedback on the manuscript. This work was supported in part by an NSF CAREER Award (Grant MCB-1350312) and the Center for Theoretical Biological Physics sponsored by the NSF (Grant PHY-1427654 and NSF-MCB-1214457). J.K.N. is a Humboldt Postdoctoral Fellow and has also been supported in part by the Welch Foundation (Grant C-1792). We acknowledge generous support provided by the Rice University Bluegene/P supercomputer, the Northeastern University Discovery Cluster and the C3DDB Cluster, as well as technical support from Northeastern ITS Research Computing. This research was supported in part by the National Science Foundation through XSEDE resources provided by the Texas Advanced Computing Center under Grant No. TG-MCB110021.

Footnotes

Author contributions J.K.N. and P.C.W. performed the simulations, analysed the data and prepared the manuscript.

References

  1. Rodnina M. V. & Wintermeyer W. Fidelity of aminoacyl-tRNA selection on the ribosome: Kinetic and structural mechanisms. Annu. Rev. Biochem. 70, 415–435 (2001). [DOI] [PubMed] [Google Scholar]
  2. Korostelev A., Ermolenko D. N. & Noller H. F. Structural dynamics of the ribosome. Curr. Opin. Chem. Biol. 12, 674–683 (2008). [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Frank J., Gao H., Sengupta J., Gao N. & Taylor D. J. The process of mRNA-tRNA translocation. Proc. Natl Acad. Sci. USA 104, 19671–19678 (2007). [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Johansson M., Lovmar M. & Ehrenberg M. Rate and accuracy of bacterial protein synthesis revisited. Curr. Opin. Microbiol. 11, 141–147 (2008). [DOI] [PubMed] [Google Scholar]
  5. Wekselman I. et al. Ribosome's mode of function: Myths, facts and recent results. J. Pept. Sci. 15, 122–130 (2009). [DOI] [PubMed] [Google Scholar]
  6. Schmeing T. M. & Ramakrishnan V. What recent ribosome structures have revealed about the mechanism of translation. Nature 461, 1234–1242 (2009). [DOI] [PubMed] [Google Scholar]
  7. Zaher H. S. & Green R. Fidelity at the molecular level: Lessons from protein synthesis. Cell 136, 746–762 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Hopfield J. J. Kinetic proofreading: a new mechanism for reducing errors in biosynthetic processes requiring high specificity. Proc. Natl Acad. Sci. USA 71, 4135–4139 (1974). [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Thompson R. C. & Stone P. J. Proofreading of the codon-anticodon interaction on ribosomes. Proc. Natl Acad. Sci. USA 74, 198–202 (1977). [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Ruusala T., Ehrenberg M. & Kurland C. G. Is there proofreading during polypeptide synthesis? EMBO J. 1, 741–745 (1982). [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Rodnina M. V., Fricke R., Kuhn L. & Wintermeyer W. Codon-dependent conformational change of elongation factor Tu preceding GTP hydrolysis on the ribosome. EMBO J. 14, 2613–2619 (1995). [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Pape T., Wintermeyer W. & Rodnina M. Induced fit in initial selection and proofreading of aminoacyl-tRNA on the ribosome. EMBO J. 18, 3800–3807 (1999). [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Gromadski K. B. & Rodnina M. V. Kinetic determinants of high-fidelity tRNA discrimination on the ribosome. Mol. Cell 13, 191–200 (2004). [DOI] [PubMed] [Google Scholar]
  14. Kothe U. & Rodnina M. V. Delayed release of inorganic phosphate from elongation factor Tu following GTP hydrolysis on the ribosome. Biochemistry 45, 12767–12774 (2006). [DOI] [PubMed] [Google Scholar]
  15. Daviter T., Gromadski K. B. & Rodnina M. V. The ribosome's response to codon-anticodon mismatches. Biochimie 88, 1001–1011 (2006). [DOI] [PubMed] [Google Scholar]
  16. Wohlgemuth I., Pohl C. & Rodnina M. V. Optimization of speed and accuracy of decoding in translation. EMBO. J. 29, 3701–3709 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  17. Zaher H. S. & Green R. Hyperaccurate and error-prone ribosomes exploit distinct mechanisms during tRNA selection. Mol. Cell 39, 110–120 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Johansson M., Zhang J. & Ehrenberg M. Genetic code translation displays a linear trade-off between efficiency and accuracy of tRNA selection. Proc. Natl Acad. Sci. USA 109, 131–136 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Zhang J., Ieong K.-W., Mellenius H. & Ehrenberg M. Proofreading neutralizes potential error hotspots in genetic code translation by transfer RNAs. RNA 22, 896–904 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Stark H. et al. Visualization of elongation factor Tu on the escherichia coli ribosome. Nature 389, 403–406 (1997). [DOI] [PubMed] [Google Scholar]
  21. Valle M. et al. Incorporation of aminoacyl-tRNA into the ribosome as seen by cryo-electron microscopy. Nat. Struct. Biol. 10, 899–906 (2003). [DOI] [PubMed] [Google Scholar]
  22. Voorhees R. M., Schmeing T. M., Kelley A. C. & Ramakrishnan V. The mechanism for activation of GTP hydrolysis on the ribosome. Science 330, 835–838 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  23. Agirrezabala X. et al. Structural insights into cognate versus near-cognate discrimination during decoding. EMBO J. 30, 1497–1507 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
  24. Schmeing T. M. et al. The crystal structure of the ribosome bound to EF-Tu and aminoacyl-tRNA. Science 326, 688–694 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  25. Jenner L. B., Demeshkina N., Yusupova G. & Yusupov M. Structural aspects of messenger RNA reading frame maintenance by the ribosome. Nat. Struct. Mol. Biol. 17, 555–560 (2010). [DOI] [PubMed] [Google Scholar]
  26. Fischer N. et al. Structure of the E. coli ribosome-EF-Tu complex at <3 angstrom resolution by Cs-corrected cryo-EM. Nature 520, 567–570 (2015). [DOI] [PubMed] [Google Scholar]
  27. Blanchard S. C., Gonzalez R. L., Kim H. D., Chu S. & Puglisi J. D. tRNA selection and kinetic proofreading in translation. Nat. Struct. Mol. Biol. 11, 1008–1014 (2004). [DOI] [PubMed] [Google Scholar]
  28. Geggier P. et al. Conformational sampling of aminoacyl-tRNA during selection on the bacterial ribosome. J. Mol. Biol. 399, 576–595 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  29. Schmeing T. M., Voorhees R. M., Kelley A. C. & Ramakrishnan V. How mutations in tRNA distant from the anticodon affect the fidelity of decoding. Nat. Struct. Mol. Biol. 18, 432–436 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
  30. Cochella L. & Green R. An active role for tRNA in decoding beyond codon:anticodon pairing. Science 308, 1178–1180 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
  31. Ledoux S., Olejniczak M. & Uhlenbeck O. C. A sequence element that tunes Escherichia coli tRNA(Ala)(GGC) to ensure accurate decoding. Nat. Struct. Mol. Biol. 16, 359–364 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  32. Cochella L., Brunelle J. L. & Green R. Mutational analysis reveals two independent molecular requirements during transfer RNA selection on the ribosome. Nat. Struct. Mol. Biol. 14, 30–36 (2007). [DOI] [PubMed] [Google Scholar]
  33. Pape T., Wintermeyer W. & Rodnina M. V. Complete kinetic mechanism of elongation factor Tu-dependent binding of aminoacyl-tRNA to the A site of the E. coli ribosome. EMBO J. 17, 7490–7497 (1998). [DOI] [PMC free article] [PubMed] [Google Scholar]
  34. Liu W. et al. EF-Tu dynamics during pre-translocation complex formation: EF-Tu-GDP exits the ribosome via two different pathways. Nucleic Acids Res. 43, 9519–9528 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  35. Sanbonmatsu K. Y., Joseph S. & Tung C.-S. Simulating movement of tRNA into the ribosome during decoding. Proc. Natl Acad. Sci. USA 102, 15854–15859 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
  36. Whitford P. C. et al. Accommodation of aminoacyl-tRNA into the ribosome involves reversible excursions along multiple pathways. RNA 16, 1196–1204 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  37. Effraim P. R. et al. Natural amino acids do not require their native tRNAs for efficient selection by the ribosome. Nat. Chem. Biol. 5, 947–953 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  38. Polikanov Y. S. et al. Distinct tRNA accommodation intermediates observed on the ribosome with the antibiotics hygromycin A and A201A. Mol. Cell 58, 832–844 (2015). [DOI] [PMC free article] [PubMed] [Google Scholar]
  39. Bryngelson J. D. & Wolynes P. G. Intermediates and barrier crossing in a random energy model (with applications to protein folding). J. Phys. Chem. 93, 6902–6915 (1989). [Google Scholar]
  40. Onuchic J. N., Luthey-Schulten Z. & Wolynes P. G. Theory of protein folding: The energy landscape perspective. Annu. Rev. Phys. Chem. 48, 545–600 (1997). [DOI] [PubMed] [Google Scholar]
  41. Garcia A. E. & Paschek D. Simulation of the pressure and temperature folding/unfolding equilibrium of a small RNA hairpin. J. Am. Chem. Soc. 130, 815–817 (2008). [DOI] [PubMed] [Google Scholar]
  42. Chen A. A. & Garcia A. E. High-resolution reversible folding of hyperstable RNA tetraloops using molecular dynamics simulations. Proc. Natl Acad. Sci. USA 110, 16820–16825 (2013). [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Satpati P. & Åqvist J. Why base tautomerization does not cause errors in mRNA decoding on the ribosome. Nucleic Acids Res. 42, 12876–12884 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  44. Satpati P., Sund J. & Aqvist J. Structure-based energetics of mRNA decoding on the ribosome. Biochemistry 53, 1714–1722 (2014). [DOI] [PubMed] [Google Scholar]
  45. Bock L. V. et al. Energy barriers and driving forces in tRNA translocation through the ribosome. Nat. Struct. Mol. Biol. 20, 1390–1396 (2013). [DOI] [PubMed] [Google Scholar]
  46. Whitford P. C., Onuchic J. N. & Sanbonmatsu K. Y. Connecting energy landscapes with experimental rates for aminoacyl-tRNA accommodation in the ribosome. J. Am. Chem. Soc. 132, 13170–13171 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
  47. Whitford P. C. et al. An all-atom structure-based potential for proteins: Bridging minimal models with all-atom empirical forcefields. Proteins 75, 430–441 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  48. Noel J. K., Chahine J., Leite V. B. P. & Whitford P. C. Capturing transition paths and transition states for conformational rearrangements in the ribosome. Biophys. J. 107, 2881–2890 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
  49. Chan H. S., Zhang Z., Wallin S. & Liu Z. Cooperativity, local-nonlocal coupling, and nonnative interactions: Principles of protein folding from coarse-grained models. Annu. Rev. Phys. Chem. 62, 301–326 (2011). [DOI] [PubMed] [Google Scholar]
  50. Givaty O. & Levy Y. Protein sliding along DNA: Dynamics and structural characterization. J. Mol. Biol. 385, 1087–1097 (2009). [DOI] [PubMed] [Google Scholar]
  51. Weinkam P., Pletneva E. V., Gray H. B., Winkler J. R. & Wolynes P. G. Electrostatic effects on funneled landscapes and structural diversity in denatured protein ensembles. Proc. Natl Acad. Sci. USA 106, 1796–1801 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  52. Socci N., Onuchic J. N. & Wolynes P. G. Diffusive dynamics of the reaction coordinate for protein folding funnels. J. Chem. Phys. 104, 5860–5868 (1996). [Google Scholar]
  53. Zhang Z. & Chan H. S. Transition paths, diffusive processes, and preequilibria of protein folding. Proc. Natl Acad. Sci. USA 109, 20919–20924 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  54. Best R. B., Hummer G. & Eaton W. A. Native contacts determine protein folding mechanisms in atomistic simulations. Proc. Natl Acad. Sci. USA 110, 17874–17879 (2013). [DOI] [PMC free article] [PubMed] [Google Scholar]
  55. Petrov A. N., Meskauskas A., Roshwalb S. C. & Dinman J. D. Yeast ribosomal protein l10 helps coordinate tRNA movement through the large subunit. Nucleic Acids Res. 36, 6187–6198 (2008). [DOI] [PMC free article] [PubMed] [Google Scholar]
  56. Hyeon C. & Thirumalai D. Capturing the essence of folding and functions of biomolecules using coarse-grained models. Nat. Commun. 2, 487 (2011). [DOI] [PubMed] [Google Scholar]
  57. Hänggi P., Talkner P. & Borkovec M. Reaction-rate theory: Fifty years after Kramers. Rev. Mod. Phys. 62, 251–341 (1990). [Google Scholar]
  58. Best R. & Hummer G. Reaction coordinates and rates from transition paths. Proc. Natl Acad. Sci. USA 102, 6732–6737 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
  59. Polekhina G. et al. Helix unwinding in the effector region of elongation factor EF-Tu-GDP. Structure 4, 1141–1151 (1996). [DOI] [PubMed] [Google Scholar]
  60. Dell V. A., Miller D. L. & Johnson A. E. Effects of nucleotide- and aurodox-induced changes in elongation factor Tu conformation upon its interactions with aminoacyl transfer RNA. A fluorescence study. Biochemistry 29, 1757–1763 (1990). [DOI] [PubMed] [Google Scholar]
  61. Thirumalai D. & Lorimer G. H. Chaperonin-mediated protein folding. Annu. Rev. Biophys. Biomol. Struct. 30, 245–269 (2001). [DOI] [PubMed] [Google Scholar]
  62. Hyeon C., Lorimer G. H. & Thirumalai D. Dynamics of allosteric transitions in GroEL. Proc. Natl Acad. Sci. USA 103, 18939–18944 (2006). [DOI] [PMC free article] [PubMed] [Google Scholar]
  63. Johansson M., Bouakaz E., Lovmar M. & Ehrenberg M. The kinetics of ribosomal peptidyl transfer revisited. Mol. Cell 30, 589–598 (2008). [DOI] [PubMed] [Google Scholar]
  64. Demeshkina N., Jenner L., Westhof E., Yusupov M. & Yusupova G. A new understanding of the decoding principle on the ribosome. Nature 484, 256–259 (2012). [DOI] [PubMed] [Google Scholar]
  65. Spirin A. S. The ribosome as a conveying thermal ratchet machine. J. Biol. Chem. 284, 21103–21119 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  66. Noel J. K., Whitford P. C. & Onuchic J. N. The Shadow map: A general contact definition for capturing the dynamics of biomolecular folding and function. J. Phys. Chem. B 116, 8692–8702 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
  67. Noel J. K. et al. SMOG 2: A versatile software package for generating structure-based models. PLoS Comp. Biol. 12, e1004794 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
  68. Hess B., Kutzner C., van der Spoel D. & Lindahl E. GROMACS 4: Algorithms for highly efficient, load-balanced, and scalable molecular simulation. J. Chem. Theory Comput. 4, 435–447 (2008). [DOI] [PubMed] [Google Scholar]
  69. Ferrenberg A. & Swendsen R. Optimized Monte Carlo data analysis. Phys. Rev. Lett. 63, 1195–1198 (1989). [DOI] [PubMed] [Google Scholar]
  70. Humphrey W., Dalke A. & Schulten K. VMD: Visual Molecular Dynamics. J. Mol. Graph. 14,, 33–38 (1996). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-7, Supplementary Table, Supplementary Methods and Supplementary References.

ncomms13314-s1.pdf (1.2MB, pdf)

Data Availability Statement

The data that support the findings of this study are available from the corresponding author upon reasonable request.

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES