Revisiting some chalcogenides for thermoelectricity

Abstract

Thermoelectric materials that are efficient well above ambient temperature are needed to convert waste-heat into electricity. Many thermoelectric oxides were investigated for this purpose, but their power factor (PF) values were too small (∼10⁻⁴ W m⁻¹ K⁻²) to yield a satisfactory figure of merit zT. Changing the anions from O²⁻ to S²⁻ and then to Se²⁻ is a way to increase the covalency. In this review, some examples of sulfides (binary Cr–S or derived from layered TiS₂) and an example of selenides, AgCrSe₂, have been selected to illustrate the characteristic features of their physical properties. The comparison of the only two semiconducting binary chromium sulfides and of a layered AgCrSe₂ selenide shows that the PF values are also in the same order of magnitude as those of transition metal oxides. In contrast, the PF values of the layered sulfides TiS₂ and Cu_0.1TiS₂ are higher, reaching ∼10⁻³ W m⁻¹ K⁻². Apparently the magnetism related to the Cr–S network is detrimental for the PF when compared to the d⁰ character of the Ti⁴⁺ based sulfides. Finally, the very low PF in AgCrSe₂ (PF = 2.25 × 10⁻⁴ W m¹ K⁻² at 700 K) is compensated by a very low thermal conductivity (κ = 0.2 W m⁻¹ K⁻¹ from the measured C_p) leading to the highest zT value among the reviewed compounds (zT_700K = 0.8). The existence of a glassy-like state for the Ag⁺ cations above 475 K is believed to be responsible for this result. This result demonstrates that the phonon engineering in open frameworks is a very interesting way to generate efficient thermoelectric materials.

Introduction

Increasing the figure of merit, z, for thermoelectric materials to convert waste-heat into electricity is a fascinating challenge for the solid state chemists and physicists. The product of figure of merit and temperature, T, is a dimensionless parameter defined as zT = (S²/ρκ)T, where S, ρ and κ are the Seebeck coefficient, electrical resistivity and thermal conductivity, respectively; z is also the power factor, PF = S²/ρ, divided by κ. Best zT values require a combination of semiconducting properties, as the highest PF values are typically found in degenerate semiconductors, together with low thermal conductivities that are dominated by the lattice contribution. This explains why Bi₂Te₃ is the only commercial thermoelectric (TE) material [1].

To harvest waste-heat at temperature beyond 600 K, other TE candidates have been intensively studied such as skutterudites derived from (Co,Fe)Sb₃; thermoelectric materials composed of tellurium, antimony, germanium and silver (TAGS); clathrates; and Zintl phases such as Yb₁₄MnSb₁₁ [2]. Transition metal oxides (TMO) provide another large class of potential TE materials [3]. Depending on the TM-O bonding, the 3d electrons in TMO can be localized or delocalized with electronic correlations that invalidate the degenerate semiconductor picture. However, the typical PF values for best TE oxides are ∼10⁻⁴ Wm⁻¹ K⁻², as for Na_xCoO₂ or ‘Ca₃Co₄O₉’ [4]. Increasing the PF requires systems with larger covalency, which can be achieved by changing the anions. Interesting candidates for TE materials can also be found among TM chalcogenides, but contrasting with the oxides, anion–anion bonding in chalcogenides can lead to molecular anion. Besides, the higher covalency in chalcogenides favors the metal–metal interactions [5]. Concerning the thermoelectric selenides, several recent reports illustrate this different chemistry for Bi₂Se₃ nanostructures [6], doped PbSe [7], quaternary tin selenide K_1−xSn_5−xBi_11+xSe₂₂ [8], p-type Cu_2+xZn_1−xGeSe₄ [9] and Ag₂Se [10]. Also zT = 0.6 at 1150 K for a (Cu/Fe)_xMo₆Se₈ Chevrel phase was reported [11]. In these molybdenum chalcogenides, the Mo ions are forming [Mo₆] octahedra surrounded by a distorted cube of anions. The Mo₆X₈ framework creates channels that are partly filled with rattling cations such as Cu/Fe in the aforementioned compound. These cations are believed to be responsible for unexpectedly low κ values in metallic compounds (ρ_300K ∼1 mΩ cm⁻¹), as low as κ ≈ 1.3 W m⁻¹ K⁻¹ at 1000 K in (Cu/Fe)₂Mo₆Se₈. Sulfides have covalency intermediate to those of oxides and selenides. They have been broadly investigated for their thermoelectric properties, as illustrated by the recent reports on BaVS₃ [12], TiS₂ [13], complex layered TiS₂-based compounds [14] and doped PbS [15]. In that respect, the review by Rao and Pishardy [5] is very instructive. All the transition metal sulfides (TMS) and disulfides (TMS₂) were described. Among the TM sulfides, the possibility of transition from semiconductors to metals in the range CrS to CrS_1.5 attracted our attention to the Cr–S binary system. The investigation of sulfides was also motivated by the report of a high zT value for the ternary compound, CuCrS₂, in the form of crystals flakes, reaching zT = 2 at 300 K [16]. As for the TMO, in which the magnetism was shown to play a role with the spin and degeneracy term β used in the modified Heikes formula [17], it is also important to report on the physical properties including magnetic and electronic properties as to evaluate these materials for thermoelectricity.

In this review, a limited number of chalcogenides was selected to illustrate some relationships between structure, magnetism and thermoelectric properties. Most of the Cr_1−xS binary compounds, including Cr₂S₃, crystallize in structures derived from NiAs type; the Cr analogue to the TiS₂ was not reported. Two examples were chosen, Cr₂S₃ and Cr₅S₆, a semiconductor and a metal. Furthermore, in contrast to all Cr/S binary compounds, including Cr₂S₃, which are antiferromagnetic, the magnetism of Cr₅S₆ is unique with a ‘ferromagnetic-like’ transition [18]. Then two layered ternary chalcogenides, Cu_xTiS₂ [19] and AgCrSe₂ [20], are reviewed to emphasize the important role of the CdI₂-type layers. Intercalation of the countercations between these layers can be used to reach low κ values.

Synthesis

As the measurements of thermoelectric properties require very dense (porosity ∼0%) materials, all the present chalcogenides have been prepared by a two-step method. In the first step, elements (Cr, Ti, Ag, S and Se) were mixed in stoichiometric amounts to prepare about 10 g of Cr₂S₃, Cr₅S₆, TiS₂ and AgCrSe₂ powders. After pressing, bars of typical dimensions 2 × 2 × 10 mm were set in silica tubes and sealed under primary vacuum. The ampoules were heated at temperatures T_h for durations t that are summarized in table 1. After cooling down to room temperature in ∼6 h, the reacted bars were crushed to obtain the powders used for the spark plasma sintering (SPS, the second step). The temperature used for the SPS (T_SPS) and the time at T_SPS (t_SPS) are also indicated in table 1. The typical time for cooling down from T_SPS to room temperature was 10 min. The density of all the sintered cylinders was higher than 95% of the theoretical density. In the case of Cu_xTiS₂, the stoichiometric amount x of metallic copper was added to the TiS₂ powder to obtain a homogeneous mixture prior to the SPS treatment.

Table 1.

Preparation conditions of the precursors (heating temperatures T_h and duration t) and the SPS samples (temperature T_SPS and cooling time t_SPS).

	Th(°C)	t (h)	TSPS (°C)	tSPS (min)
Cr2S3	900	12	1100	30
Cr5S6	900	12	900	10
CuxTiS2	650	12	900	30
AgCrSe2	900	12	700	30

Structural characterizations

Structural characterizations were performed by powder x-ray diffraction (XRD) measured at ambient temperature after the first synthesis step and then after the SPS process. For that purpose, we used a PANalytical Xpert Pro diffractometer with CuKα radiation (5° ⩽ 2ѳ ⩽ 120°). In some cases, transmission electron microscopy (TEM) was employed for electron diffraction, energy dispersive x-ray (EDX) analysis and high-resolution imaging.

Cr₂S₃

The as-prepared powder corresponds to the R 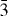space group [a = 5.9339(1) Å and c = 16.677 24(4) Å] with a Cr/S atomic ratio from EDX in good agreement with the value of 2/3 from the chemical formula [21]. Interestingly, the sample obtained by SPS crystallizes in the crystallographic form reported for trigonal Cr₂S₃, i.e. in a trigonal structure [SG: 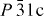; a = 5.9441(1) Å and c = 11.203 39 (2) Å]. Both sets of unit cell parameters are consistent with previous studies [22–24]. The different set found for the SPS-treated sample could be related to two factors. The first one is the deviation from the 2:3 Cr/S atomic ratio in the SPS sample, with a 2:2.8 ratio detected by EDX, and the second one is the rapid cooling used at the end of the SPS process. The second reason is consistent with previous reports in which quenching was necessary to stabilize the trigonal form. In the following, the SPS-prepared form is labeled ‘T-Cr₂S_2.8’ and the precursor ‘R-Cr₂S₃’.

Cr₅S₆

The powders of both as-prepared and SPS Cr₅S₆ were found to crystallize in the 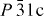trigonal space-group, with a = b ≈ 5.98 Å and c ≈ 11.509 Å. These values are close to those first reported in [24]. They are also close to that of the trigonal form of Cr₂S₃ showing that these structures all derive from the NiAs type. Small amounts (∼1% from x-ray data) of Cr₃S₄ impurity were found in the T-Cr₂S_2.8 sample, which could be related to the S departure. Similarly, a Cr₇S₈ impurity was detected in the SPS-prepared Cr₅S₆ phase.

Structural relationships

Cr_1−xS sulfides

The NiAs-type structure of CrS can be described by starting from the hexagonal packing of the S atoms (figure 1). In that network, octahedral voids are created that can be fully or partially occupied by the Cr cations. In R-Cr₂S₃, T-Cr₂S_2.8 and trigonal T-Cr₅S₆, the Cr vacancies are ordered in every alternate layer along the c-axis with a 1/3 occupation by Cr in Cr₂S₃ and 2/3 in the case of Cr₅S₆ (figure 1). In addition to their different atomic ratio, the difference between R-Cr₂S₃ and T-Cr₂S_2.8 originates also from the ordering and disordering, respectively, of the Cr species at the level of the Cr-deficient layer over three different crystallographic positions (figure 2). Thus, going from Cr₂S₃ to Cr₅S₆, one expects a more 3D Cr–S magnetic network in the latter, and for the Cr formal oxidation state (considering S²⁻), a decrease from υ_Cr = 3 to υ_Cr = 2.4, i.e. a larger electron concentration in Cr₅S₆, with an intermediate value υ_Cr = 2.8 for T-Cr₂S_2.8.

Figure 1.

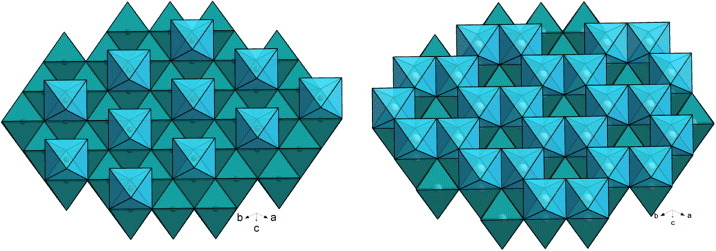
Structural models for Cr₂S₃ (left) and Cr₅S₆ (right). The stacking of S layers creates octahedral voids which are fully occupied to form a dense CrS layer of CrS₆ edge-shared octahedra which alternates with a partially filled layer, Cr_1/3S (left) and Cr_2/3S (right) for Cr₂S₃ and Cr₅S₆, respectively.

Figure 2.

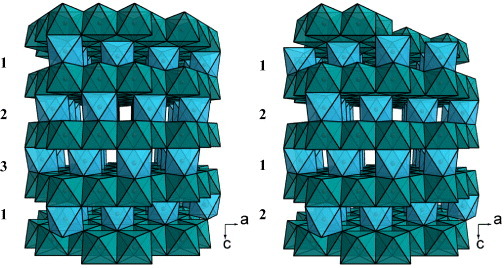
Structural models for Cr₂S₃. Two sequences, 1–2–3 and 1–2–1–2, are obtained along the stacking direction c, corresponding to the rhombohedral and trigonal forms, respectively.

TiS₂ and AgCrSe₂

TiS₂ crystallizes in the CdI₂ structure within the space group. The edge-shared layers of TiS₆ octahedra are interconnected through van der Waals forces, creating octahedral sites that can be occupied by intercalated species [25]. The as-prepared TiS₂ precursor can be indexed in the space group with parameters a = 3.4062 Å and c = 5.707 Å in good agreement with the values from the literature (a = 3.4073 Å and c = 5.6953 Å [26]). After SPS, the Cu intercalation with starting composition corresponding to Cu_xTiS₂ with x ⩽ 0.10 was confirmed by EDX and powder XRD. In particular, the c-axis parameter along the stacking direction of the TiS₂ slabs increased from 5.707 Å to 5.760 Å with x increasing from x = 0 to x = 0.10 [19].

In AgCrSe₂ [27], the CrSe₂ layers, also of the CdI₂ type, are stacked in a way that half tetrahedral sites are occupied by Ag⁺ in the interspace (figure 3). In fact, the network made by the tetrahedra centers forms a honeycomb network with only one type of tetrahedra occupied below 475 K (ordered form). At higher temperatures it evolves toward a disordered state where the two types of tetrahedra are randomly occupied. At 300 K, the unit cell parameters are fitted in the polar space group R3 m with a = 3.682 Å and c = 21.23 Å [27].

Figure 3.

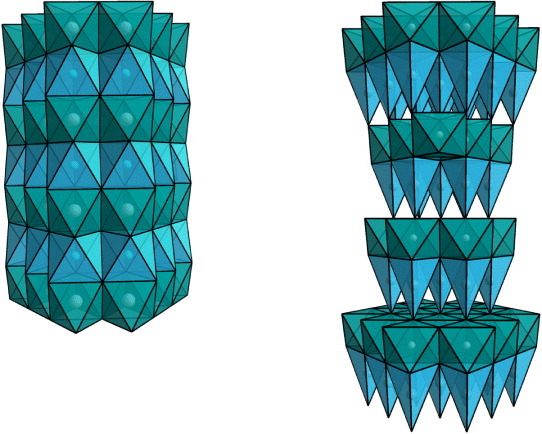
Structures of Cu_xTiS₂ (left) and AgCrSe₂ (right). Both structures can be described as a stacking of CdI₂-type layers, TiS₂ or CrSe₂ (Ti or Cr cations are at the centers of the green octahedra), with Cu cation in the van der Waals spacing (blue octahedra) or Ag in tetrahedral coordination (blue tetrahedra), respectively.

For the Cu_xTiS₂ and AgCrSe₂ layered compounds, as pressure is used during the SPS treatments, it tends to align parallel the largest faces (ab-plane) of the platelet-like microcrystals leading to a clear texturation [19]. For these materials, all the ρ, S and κ measurements have been performed along the directions lying in the conducting planes ab.

Magnetoresistance: role of the spins on the transport properties in Cr₂S₃ and Cr₅S₆

The comparison of the temperature-dependent magnetic susceptibilities (χ) of the Cr₂S₃ samples [21], measured with a SQUID magnetometer, shows that depending on the crystallographic form, either a ferromagnetic-like component develops for R-Cr₂S₃ below T_N = 120 K, the antiferromagnetic (AF) transition, or for T-Cr₂S_2.8 a pure AF transition occurs below 100 K (figure 4). The presence of a ferromagnet-like component in R-Cr₂S₃, in good consistency with the literature [28], creates some spin scattering above T_N, so that a clear decrease of the electrical resistivity (ρ) is seen below T_N (figure 5(a)). Accordingly, upon application of an external magnetic field, a negative magnetoresistance effect is observed in R-Cr₂S₃ but not in T-Cr₂S_2.8 (figure 5(a)). These data are supported by the isothermal curve collected at 100 K showing a negative magnetoresistance of −8% in 9 T for the R-Cr₂S₃ compound (figure 5(b)).

Figure 4.

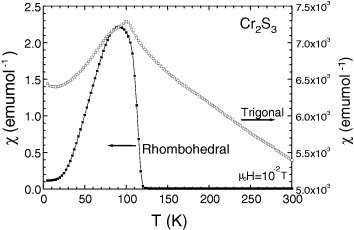
Temperature-dependent magnetic susceptibility (χ = M/H) for Cr₂S₃ before (rhombohedral R-Cr₂S₃, left y-axis) and after SPS (trigonal T-Cr₂S_2.8, right y-axis). The measurements were carried out in the zero-field cooling mode.

Figure 5.

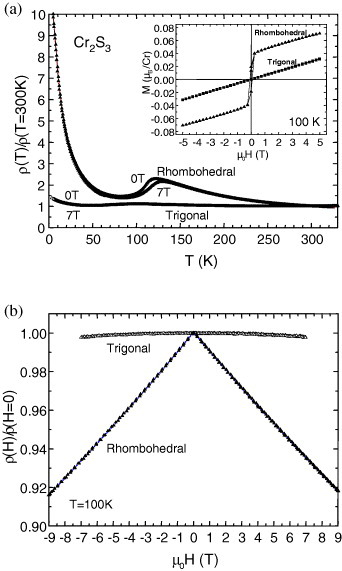
(a) Normalized electrical resistivity (ρ) as a function of temperature for both Cr₂S₃ samples, rhombohedral R-Cr₂S₃ and trigonal T-Cr₂S_2.8. The data collected upon cooling in H = 0 and 7 T reveal the existence of a negative magnetoresistance in the T_N region for the rhombohedral precursor R-Cr₂S₃. Inset: magnetic field driven magnetization (M) for both Cr₂S₃ samples (T = 100 K). (b) Magnetic field (H) driven normalized resistivity collected at 100 K for both Cr₂S₃ samples.

The magnetism of Cr₅S₆ is more complex as can be seen on the corresponding χ(T) curve (figure 6). A crenel shape curve is observed between two magnetic transitions at 160 and 300 K. The existence of ferrimagnetic (FI) state between these temperatures, followed by an AF one below 160 K, is well documented in the literature [29–31]. However, as mentioned in the first papers by Yuzuri and Nakamura [31], the FI component was found to be dependent on the quality of the vacancy ordering at the level of the Cr_2/3S layers of the structure. Increasing this disorder was supposed to create some breaking along the magnetic exchange directions. This is supported by the comparison between the curves of the Cr₅S₆ precursor prepared in a closed vessel (cooling in 6 h from 900 °C) with that of the final product sintered by SPS with a 10 min cooling. The χ′ values between T_C and T_N are decreased by at least one order of magnitude after SPS (figure 6). Thus, there is a parallel to be made with Cr₂S₃ which ferromagnetic-like component is suppressed after SPS, i.e. in the more disordered trigonal crystallographic form T-Cr₂S_2.8. Cr₅S₆ exhibits a more metallic behavior (figure 7) as compared to the Cr₂S₃ samples (figure 5(a)), which both show larger ρ values at 5 K than at 300 K. In Cr₅S₆ the dρ/dT coefficient is always positive (figure 7), with a slight change of slope at T_N as in Cr₂S₃. Despite that an FI-like component is observed on the χ(T) curves, the absolute values of magnetoresistance are limited, reaching a maximum at 150 K (near T_N) of only 2% in 7 T (figure 8). Interestingly, these magnetoresistance curves measured with current perpendicular to the magnetic field direction reveal the existence of a transition induced by the external magnetic field, hysteretic and with characteristic magnetic field that decreases as temperature increases from 140 K (below T_N) to T_N. This field-induced transition reflects the hysteretic-field-driven transition from AF to FI phase in both the precursor and SPS-prepared sample (figure 8). In 9 T, the maximum magnetization value corresponds to ∼0.08 μ_B/Cr in the sample against 0.13 μ_B/Cr in the precursor. They are similar to the value of 0.07 μ_B/Cr reached at 100 K (below T_N) in the R-Cr₂S₃ sample (inset of figure 5(a)). The specific heat measurements performed without magnetic field on the Cr₅S₆ sample (figure 9) confirm the existence of two magnetic transitions at 160 and 300 K.

Figure 6.

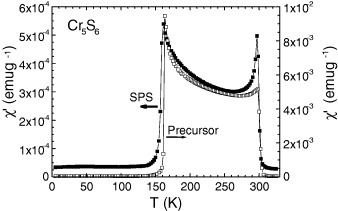
Temperature dependence of the ac magnetic susceptibility (χ′, real part) for Cr₅S₆, before and after SPS (μ₀H_ac = 10 Oe, f = 100 Hz).

Figure 7.

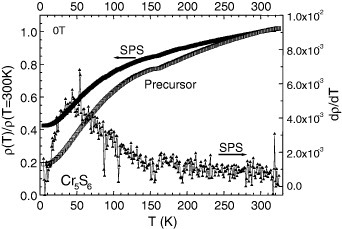
Normalized electrical resistivity for Cr₅S₆ (left y-axis). The corresponding dρ/dT = f(T) curve is also given for the SPS sample (right y-axis).

Figure 8.

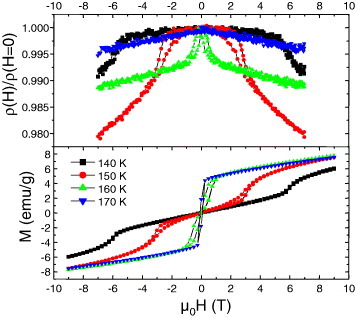
Top panel: magnetic field (H) driven resistance ratio for Cr₅S₆. The temperatures are indicated in the bottom panel where the corresponding M(H) curves are given. The measurements were performed with current perpendicular to H.

Figure 9.

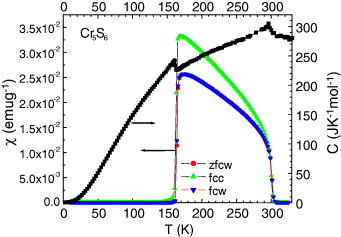
Temperature-dependent specific heat C of the Cr₅S₆ precursor measured upon warming (right y-axis) and corresponding χ(T) curves measured upon cooling (c) and warming (w) after zero-field-cooling (zfc) and field-cooling (fc) processes (left y-axis).

Finally, these measurements demonstrate that not only magnetic properties are sensitive to the preparation technique (i.e. to the structural disorder or/and S stoichiometry) but also the ρ values. This is illustrated for Cr₂S_3−x: densification by SPS allows to reduce its ρ by more than one order of magnitude, down to ρ _300k = 2.65 mΩ cm for T-Cr₂S_2.8 (table 2). This value is even slightly lower than that reported for a trigonal (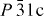) Cr₂S_2.84 crystal (ρ_300K = 5.0 mΩ cm [23]).

Table 2.

Physical parameters.

Sample	ρ300 K (Ω cm)	ρ700 K (Ω cm)	S700 k (μV K−1)	PF700 K (W m−1 K−2)	κ700 K (W m−1 K−1)	zT700 K
R-Cr2S3	0.045	–	–	–	–	–
T-Cr2S2.8	0.00265	0.0030	-100	3.4×10−4	2.0	0.12
Cr5S6	0.00062	0.00080	−40	2.0×10−4	2.8	0.05
CuxTiS2
x=0.00	0.0015	0.0070	-270	1.0×10−3	2.2	0.34
x=0.10	0.0002	0.0015	-130	1.1×10−3	2.0	0.39
AgCrSe2	0.12	0.04	300	2.25×10−4	0.2	0.8

Comparison of the power factors

Cr_1−xS sulfides

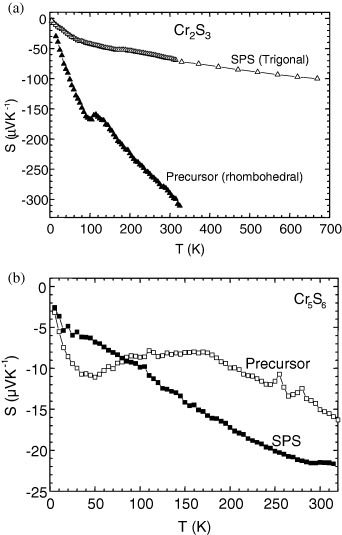
As mentioned in the introduction, the charge carriers in R-Cr₂S₃ and T-Cr₂S_2.8 samples can be anticipated from the chemical formula to be electrons created by the Cr²⁺ species, with carrier concentration increasing from R-Cr₂S₃ to T-Cr₂S_2.8 and being even larger in Cr₅S₆. The comparison of the S(T) curves (figures 10(a) and (b)) confirms that order, with S_300K values of −290, −70, −15 and −22 μV K⁻¹ for the samples R-Cr₂S₃, T-Cr₂S_2.8, Cr₅S₆ precursor and SPS-Cr₅S₆, respectively. For both Cr₂S_3−x samples, a slight change of slope is detected at T_N in good agreement with the change detected on the ρ(T) curves.

Figure 10.

Temperature-dependent Seebeck coefficient (S) for the precursor and SPS-treated Cr₂S₃ (a) and Cr₅S₆ (b).

Although the ρ(T) dependence differs for these Cr–S samples, all the S(T) curves show a metal-like behavior above the magnetic transition temperatures with an increase of 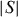(T) up to the highest temperature used for these measurements (∼700 K). This is illustrated in figure 11 for the SPS-prepared Cr₅S₆ sample. The calculated values of the power factor PF are given in figure 12, and all values at 700 K are summarized in table 2. Due to the lower ρ value for T-Cr₂S_2.8 after SPS of R-Cr₂S₃, the PF of T-Cr₂S_2.8 is found to be higher than that of the precursor, reaching PF = 3.4 × 10⁻⁴ W m⁻¹ K⁻¹ at 700 K (table 2). This value is comparable to that of the SPS-prepared Cr₅S₆ sample with PF = 2 × 10⁻⁴ W m⁻¹ K⁻¹ at 700 K (table 2 and figure 12).

Figure 11.

Temperature dependences of ρ and S for SPS-treated Cr₅S₆.

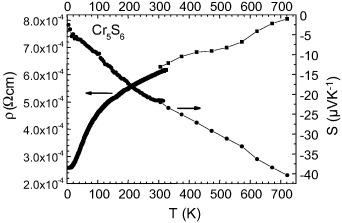
Figure12.

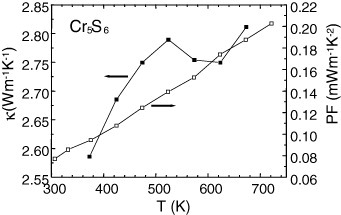
SPS-densified Cr₅S₆: PF and κ versus temperature.

Layered Cu_xTiS₂ and AgCrSe₂

The PF values of Cr₂S₃ and Cr₅S₆ are relatively small and similar to those reported in TE oxides. The values measured in the layered sulfides Cu_xTiS₂ for x ⩽ 0.1 are all around 1 mW m⁻¹ K⁻² at 700 K (figure 14 and table 2); ρ and Seebeck coefficient depend on the Cu content x which controls the amount of Ti³⁺ electrons in the Ti⁴⁺matrix, with both ρ and 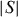decreasing with increasing x. The AgCrSe₂ compound exhibits a lower PF value of 2.25 × 10⁻⁴ W m⁻¹ K⁻² at 700 K (table 2, from the data of figure 15). To summarize that part, chromium sulfides and selenide, with either more 3D or 2D structure, all exhibit lower PF values than usually expected for efficient TE materials. Low thermal conductivity values are thus required to increase zT in these materials. The magnetism of Cr³⁺ (3d³) is absent as shown for Cu_xTiS₂, with no magnetic ordering at low temperature, and the PF values are the highest reaching ∼10⁻³ W m⁻¹ K⁻² at 700 K. It must be also pointed out that the TiS₂ compound exhibits a high power factor of 1.7 × 10⁻³ W m⁻¹ K⁻² at room temperature.

Figure 14.

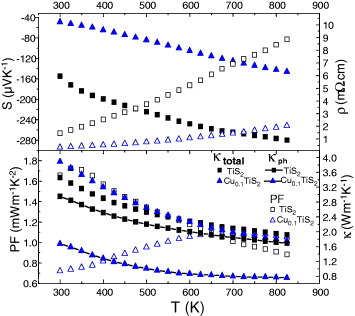
Cu_xTiS₂ (x = 0.00 and x = 0.10). Top panel: S(T) (solid symbols), ρ(T) (open symbols). Lower panel: PF(T), κ(T) [total and lattice part κ_ph].

Figure 15.

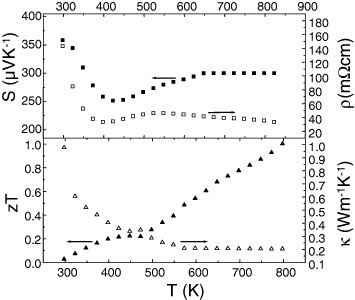
AgCrSe₂: ρ(T), S(T), zT(T) and κ(T).

Thermal conductivities

The measurements were carried out above 300 K by combining the thermal diffusivity from the laser flash technique (Netzsch457), with heat capacity (DCS404).

The κ(T) values for the dense Cr/S phase prepared by SPS are close to 2–3 W K⁻¹ m⁻¹ at 700 K (figures 12 and 13). Combining them with the PF_700k values yields zT of 0.05–0.1 (table 2).

Figure 13.

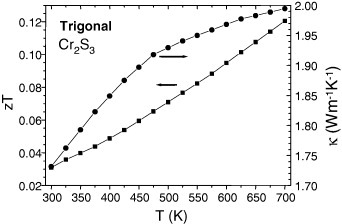
Trigonal densified Cr₂S₃ sample T-Cr₂S_2.8. Thermal conductivity (right y-axis), and zT (left y-axis) versus temperature.

In Cu_xTiS₂, the κ(T) curves of the SPS-prepared phases are evolving in the opposite direction with temperature, κ decreasing as T increases (figure 14). Although ρ decreases by a factor of 4 as x increases from 0 to 0.10, the values of κ are almost independent of x. This indicates that the phonon part of κ, κ_ph, is reduced as x increases (figure 14), i.e. as to compensate the electronic part of κ that increases. Such a behavior can be explained by considering the structural disorder generated by the local Cu intercalation between the TiS₂ layers. Best zT values are observed for Cu_0.10TiS₂ with zT = 0.39 at 700 K (figure 16). At high temperatures, the κ values are similar for Cu_0.10TiS₂, Cr₅S₆ and Cr₂S_2.8. The higher zT of Cu_0.10TiS₂ comes from its higher PF values.

Figure 16.

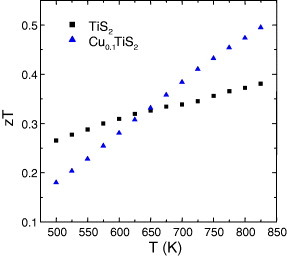
zT curves for Cu_xTiS₂ with x = 0 and 0.10.

In contrast, the measured κ values in AgCrSe₂ are much smaller at high temperatures, as low as κ = 0.2 W m⁻¹ K⁻¹ at 700 K (figure 15). This low κ could be related to the order–disorder transition in the Ag⁺ separating layers occurring at 475 K. Above this temperature, the Ag⁺ ions are randomly distributed over the two different crystallographic positions in AgCrSe₂, which is known to be a fast ionic conductor [32], and thus the disordered character of that layer is believed to be responsible for the glass-like values for κ. This could explain why κ is so low in that material, even lower than the κ = 0.5 W m⁻¹ K⁻¹ at 700 K value calculated by using the Dulong–Petit value for the specific heat (figure 17), which leads to zT = 0.32 instead of zT = 0.8. A similar behavior was recently reported in Cu₂Se [33], another superionic conductor with a liquid-like thermal conductivity, for which the specific heat at high temperatures decreases significantly below the 3Nk_B value of the Dulong–Petit model, where N is the Avogadro constant and k_B is the Boltzmann constant.

Figure 17.

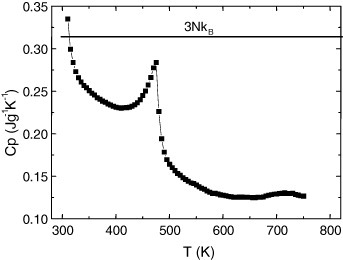
Temperature dependence of specific heat for AgCrSe₂. The horizontal line indicates the Dulong Petit value 3Nk_B.

Conclusions

The reported comparison of several chalcogenides demonstrates that these materials could provide good candidates for thermoelectric devices. In the case of the chromium chalcogenides with structures deriving from either NiAs or CdI₂ types, magnetically ordered states set in at low temperatures. They are related to the magnetism of Cr³⁺, a high-spin d³ cation. In contrast to oxides, for which the Seebeck coefficient of La_1−xSr_xCrO₃ orthochromites is explained at high temperature by considering the Heikes formula with an additional term of spin and orbital degeneracy [34], in the reviewed chromium chalcogenides, no S saturation is observed at high temperatures, the S(T) curves showing 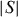αT metallic dependence. Although the physics differs from that of oxides, the power factor values are rather similar. The role of the spins can be evaluated by considering the higher PF value of the layered sulfide Cu_xTiS₂ containing a majority of the d⁰Ti⁴⁺ cations: at 300 K, the ρ value of Cu_0.1TiS₂ is lower by one order of magnitude than that of Cr₅S₆ despite 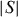of the former is higher. Thus, the spin scattering in the paramagnetic state could be detrimental to the PF. However, low PF values are not redhibitory as the thermal conductivity can be significantly reduced by cation disorder as in AgCrSe₂. In that respect, the compounds crystallizing in 2D structures, where disorder can be created between the conducting planes, are very promising. Finally, the SPS process enables the densification of chromium sulfides. Furthermore, this treatment favors a sulfur nonstoichiometry which is clearly evidenced in the case of ‘Cr₂S₃’ by SPS, the R-Cr₂S₃ precursor leads to a T-Cr₂S_2.8 dense ceramic. For this type of binary sulfides such as Cr₂S₃ and Cr₅S₆, SPS densification allows to achieve better thermoelectric properties as compared to the precursors.