Coordinating long-latency stretch responses across the shoulder, elbow, and wrist during goal-directed reaching

Here we examined the flexibility and utility of coordinating the long-latency stretch response across multiple joints during reaching. After elbow perturbations, long-latency stretch responses evoked in shoulder and wrist muscles counteracted intersegmental dynamics but did not appear to exploit kinematic redundancy.

Abstract

The long-latency stretch response (muscle activity 50–100 ms after a mechanical perturbation) can be coordinated across multiple joints to support goal-directed actions. Here we assessed the flexibility of such coordination and whether it serves to counteract intersegmental dynamics and exploit kinematic redundancy. In three experiments, participants made planar reaches to visual targets after elbow perturbations and we assessed the coordination of long-latency stretch responses across shoulder, elbow, and wrist muscles. Importantly, targets were placed such that elbow and wrist (but not shoulder) rotations could help transport the hand to the target—a simple form of kinematic redundancy. In experiment 1 we applied perturbations of different magnitudes to the elbow and found that long-latency stretch responses in shoulder, elbow, and wrist muscles scaled with perturbation magnitude. In experiment 2 we examined the trial-by-trial relationship between long-latency stretch responses at adjacent joints and found that the magnitudes of the responses in shoulder and elbow muscles, as well as elbow and wrist muscles, were positively correlated. In experiment 3 we explicitly instructed participants how to use their wrist to move their hand to the target after the perturbation. We found that long-latency stretch responses in wrist muscles were not sensitive to our instructions, despite the fact that participants incorporated these instructions into their voluntary behavior. Taken together, our results indicate that, during reaching, the coordination of long-latency stretch responses across multiple joints counteracts intersegmental dynamics but may not be able to exploit kinematic redundancy.

NEW & NOTEWORTHY

Here we examined the flexibility and utility of coordinating the long-latency stretch response across multiple joints during reaching. After elbow perturbations, long-latency stretch responses evoked in shoulder and wrist muscles counteracted intersegmental dynamics but did not appear to exploit kinematic redundancy.

our ability to rapidly and flexibly adjust our movements has led to the proposal that goal-directed actions rely on the rapid and flexible manipulation of sensory feedback (Scott 2004; Todorov 2004; Todorov and Jordan 2002). One line of evidence supporting this proposal is the finding that many factors known to modulate goal-directed reaching also modulate arm muscle activity 50–100 ms after a mechanical perturbation (i.e., the long-latency stretch response; for reviews see Pruszynski and Scott 2012; Shemmell et al. 2010). Such factors include an individual's volitional intent (Colebatch et al. 1979; Crago et al. 1976; Evarts and Granit 1976; Hammond 1956; Omrani et al. 2013; Pruszynski et al. 2008), movement decision-making processes (Nashed et al. 2014; Selen et al. 2012; Yang et al. 2011), the arm's mechanical properties (Crevecoeur et al. 2012, 2016; Crevecoeur and Scott 2013; Kurtzer et al. 2008, 2009; Soechting and Lacquaniti 1988), as well as general task demands (Dietz et al. 1994; Doemges and Rack 1992a, 1992b; Nashed et al. 2012; Weiler et al. 2015) and environmental dynamics (Ahmadi-Pajouh et al. 2012; Cluff and Scott 2013; Kimura et al. 2006; Krutky et al. 2010).

Another line of evidence supporting this proposal is the finding that the long-latency stretch response can manifest in muscles that were not mechanically stretched by the perturbation. For example, in the so-called “tea cup” experiment, Marsden and colleagues (1981) showed that, after a mechanical perturbation that pulled a participant's left arm, a long-latency stretch response could be recorded on either the right arm's biceps or triceps depending on whether the participant was holding a tea cup or bracing against a table, respectively. This flexible routing of the long-latency stretch response has also been demonstrated in situations in which people coordinate actions across the two arms (Dimitriou et al. 2012; Manning et al. 2012; Mutha and Sainburg 2009; Omrani et al. 2013) as well as different digits within the same hand (Cole et al. 1984; Ohki and Johansson 1999).

We have recently investigated this routing by examining how the long-latency stretch response is coordinated across shoulder, elbow, and wrist joints for goal-directed reaches after elbow perturbations (Weiler et al. 2015). Specifically, we designed an experiment such that movement at both the elbow and wrist joints (but not the shoulder joint) could help transport the hand toward the visual target—a simple form of kinematic redundancy (Bernstein 1967). We found that elbow perturbations elicited long-latency stretch responses across the shoulder, elbow, and wrist and that these responses were appropriately modulated for bringing the hand to the target. That is, long-latency stretch responses appeared to be modulated in elbow and wrist muscles to help move the hand toward the target and in shoulder muscles to counteract anticipated interaction torques caused by arm movement.

Here we present three experiments that further examine the coordination of long-latency stretch responses across the shoulder, elbow, and wrist joints and whether this coordination serves to counteract intersegmental dynamics and/or exploits kinematic redundancy. In the first experiment we examined the general flexibility of this coordination by testing whether long-latency stretch responses elicited in shoulder, elbow, and wrist muscles are sensitive to elbow perturbation magnitude or whether they merely reflect a preplanned movement triggered by the perturbation (see Ravichandran et al. 2013; Shemmell et al. 2009). We found that elbow perturbations elicited long-latency stretch responses across the shoulder, elbow, and wrist muscles and that these responses increased as a function of perturbation magnitude. In the second experiment we detailed the trial-by-trial relationship between the long-latency stretch responses of shoulder and elbow muscles as well as between elbow and wrist muscles. We predicted a positive correlation between muscles that flex the shoulder and elbow, which would be consistent with accounting for anticipated interaction torques, and a negative correlation between muscles that flex the elbow and wrist muscles, which would be consistent with exploiting kinematic redundancy (see Scott 2004; Todorov 2004; Todorov and Jordan 2002). The same predictions were made for pairs of muscles that both act as extensors. Interestingly, our predictions were not fully confirmed, as we found positive correlations for both muscle pairs. In the third experiment we followed up on this unexpected result by testing whether participants could modulate long-latency stretch responses routed to the wrist when we explicitly instructed them how to exploit kinematic redundancy between the elbow and wrist joints. We found that participants incorporated our instructions into their voluntary behavior but that long-latency stretch responses routed to wrist muscles were unaffected. Taken together, our results indicate that after elbow perturbations long-latency stretch responses are flexibly routed to elbow muscles to move the hand to the desired location and to shoulder and wrist muscles to counteract local torques that arise from rapid arm movement. Intriguingly, our results suggest that exploiting kinematic redundancy may be outside the functional capacity of the neural circuits that generate the long-latency stretch response.

METHODS

Participants

Forty-seven individuals participated in this work. From this group of participants, 20 (14 men, 6 women; mean age 20.9 yr), 15 (13 men, 2 women; mean age 23.4 yr), and 16 (14 men, 2 women; mean age 21.9 yr) volunteered for experiments 1, 2, and 3, respectively. Before data collection all participants reported having normal or corrected-to-normal vision and provided informed written consent. All experiments were approved by the Office of Research Ethics at Western University and were completed in accordance with the Declaration of Helsinki.

Apparatus

Participants grasped the handle of a three degree-of-freedom (shoulder, elbow, wrist) exoskeleton robot (Interactive Motion Technologies, Boston, MA) such that the wrist was in a neutral position (see Weiler et al. 2015 for depiction of exoskeleton). The exoskeleton permits hand movement in a horizontal plane via flexion/extension of the shoulder, elbow, and wrist joints and can apply independent or concurrent torques directly at the shoulder, elbow, and wrist joints. The device is equipped with encoders to measure kinematics at the shoulder, elbow, and wrist. Visual stimuli were projected downward with a 46-in. TV monitor (60 Hz, 1,920 × 1,080 pixels, Dynex DX-46L262A12, Richfield, MN) onto a semisilvered mirror that occluded vision of the participant's arm. Participants were provided with feedback of their hand position with a 1-cm-diameter turquoise circle, which was displayed at the coordinates of the exoskeleton's handle (i.e., hand feedback cursor). Participants were comfortably seated, and the lights in the experimental suite were extinguished for the duration of data collection.

General Procedure

We used similar procedures for all three experiments (see also Weiler et al. 2015). Participants moved the hand feedback cursor to a red circle (i.e., the home location: 2-cm diameter), which was located at the hand feedback cursor's position when the shoulder, elbow, and wrist were at 70°, 60°, and 10° of flexion, respectively (external angle coordinate system). After a 1,500-ms delay, the exoskeleton gradually applied a flexion or extension torque to the elbow over 2,000 ms, which plateaued at a constant torque of ±3 Nm (i.e., the preload; positive value reflects a torque that flexed the elbow). When the torque plateaued, the hand feedback cursor was removed and a large white target circle (20-cm diameter) was presented. The target circle could be placed 1) where the preload would displace the participant's hand toward the center of the target or 2) where the preload would displace the participant's hand away from the center of the target. Participants were required to counteract the preload and maintain their hand in the home location. After participants maintained hand position on the home location for a randomized foreperiod (1,000–2,500 ms), a step-torque (i.e., perturbation) was applied to the elbow. Perturbations that diametrically opposed the preload were delivered by turning off the exoskeleton's torque motors (see below). The perturbation direction (i.e., flexor or extension) was randomized on a trial-by-trial basis and could displace the participant's hand either 1) toward the center of the target (IN target condition) or 2) away from the center of the target (OUT target condition). Participants were instructed to move their hand into the target in <375 ms after perturbation onset. Movement feedback was provided after every trial. If the participant successfully moved his/her hand into the target in <375 ms the target circle changed from white to green; otherwise the target circle changed from white to red. Regardless of trial outcome, the step-torque was gradually removed 1,300 ms after perturbation onset. Trials in which the participant moved outside the home location before perturbation onset were aborted and rerun later in the experiment.

Participants completed practice trials before data collection until a success rate of ∼75% was reached. This process usually lasted ∼10 min. Rest breaks were given throughout each experiment approximately every 20 min or when requested.

Experiment-Specific Procedures

In experiment 1 the exoskeleton applied a ±4.5-Nm, ±3.0-Nm, or ±1.5-Nm perturbation following a ±3-Nm preload. These perturbations displaced the participant's hand into or away from the visual target. Participants completed 20 trials for each of the 24 experimental conditions (2 preloads; 2 target locations; 6 perturbations) in a randomized order for a total of 480 trials.

In experiment 2 the exoskeleton applied a ±3-Nm elbow perturbation following a ±3-Nm preload, which displaced the participant's hand into or away from the target. The primary purpose of this experiment was to understand the trial-by-trial relationship between EMG activity at shoulder and elbow muscles and between elbow and wrist muscles. To increase the reliability of these trial-by-trial analyses, participants completed 60 trials for each of the eight experimental conditions (2 preloads; 2 target locations; 2 perturbations) in a randomized order for a total of 480 trials.

In experiment 3 participants completed three blocks of trials. For each block the exoskeleton applied a ±3-Nm perturbation at the elbow following a ±3-Nm preload, which displaced the hand into or away from the target. In one block (No Instruct), participants were not given any explicit instructions on how to use their wrist in response to an elbow perturbation (as in experiments 1 and 2). In the other two blocks, participants were given specific instructions about how to use their wrist to transport their hand to the goal target after the elbow perturbation. In one of these blocks (Minimum Use), participants were told: “Use your wrist as little as possible following the elbow perturbation.” In the other block (Maximum Use), participants were told: “Use your wrist as much as possible following the elbow perturbation.” Participants completed 20 trials for each of the eight experimental conditions (2 preloads; 2 target locations; 2 perturbations) in a randomized order for each of the three blocks, for a total of 480 trials. Participants always completed the “No Instruct” block first to ensure that their natural wrist recruitment was not biased by explicit wrist instructions provided in the other two blocks. The order of the remaining two blocks (“Minimum Use,” “Maximum Use”) was counterbalanced across participants.

Muscle Activity

Participants' skin was cleansed with rubbing alcohol, and EMG surface electrode (Delsys Bagnoli-8 system with DE-3.1 sensors, Boston, MA) contacts were covered with conductive gel. EMG contacts were then placed over the belly of six muscles [pectoralis major clavicular head (PEC; shoulder flexor); posterior deltoid (DELT; shoulder extensor); biceps brachii long head (BI; shoulder and elbow flexor, wrist supinator); triceps brachii lateral head (TRI; elbow extensor); flexor carpi ulnaris (WF; wrist flexor); extensor carpi radialis (WE; wrist extensor)] at an orientation that runs parallel to the muscle fibers. We restricted analyses of the BI to its activity in relation to elbow movement (i.e., we treated it as an elbow flexor), although the BI acts to flex the shoulder and supinate the wrist. We also restricted analyses of the WF and WE to their activity in relation to wrist movement, although these muscles could, in principle, help flex or extend the elbow because they originate on the medial epicondyle and lateral supracondylar ridge of the humerus, respectively. We took great care in our placement of the electrodes, but because of the nature of surface EMG it is possible that a small portion of our measured signal may reflect activity from surrounding muscles. A reference electrode was placed over the participants' left clavicle. EMG signals were amplified (gain = 10³), band-pass filtered (20–450 Hz), and then digitally sampled at 2,000 Hz.

Data Reduction and Analysis

All data were aligned on perturbation onset. Angular positions of the shoulder, elbow, and wrist were sampled at 500 Hz. Participants' hand position was computed based on their measured arm segment lengths (i.e., upper arm, lower arm, and hand) and joint angles. Kinematic data were low-pass filtered (15 Hz, 2-pass, 2nd-order Butterworth). EMG data were band-pass filtered (25–250 Hz, 2-pass, 2nd-order Butterworth) and full-wave rectified. Flexor muscles (i.e., PEC, BI, WF) were normalized to their own mean activity over the 200-ms period before perturbation onset when the BI was loaded by the exoskeleton (i.e., elbow extension torque preload). Extensor muscles (i.e., DELT, TRI, WE) were normalized to their own mean activity over the 200-ms period before perturbation onset when the TRI was loaded by the exoskeleton (i.e., elbow flexion torque preload). Trials in which the mechanical perturbation shortened the preloaded elbow muscle were not analyzed. These trials comprised half of the conditions within each experiment and were included so participants could not predict in which direction the perturbation would displace their hand relative to the target. Those interested in how the long-latency stretch response is modulated when the preloaded muscle is shortened should see Nashed et al. (2015).

Mean hand displacement as well as shoulder, elbow, and wrist rotation were computed from −200 ms to 400 ms relative to perturbation onset for each participant. EMG activity from specific muscles was occasionally discarded because the robot dislodged the electrode during data collection. One BI sample from experiment 1 and one WF sample from experiment 3 were excluded for this reason. Mean EMG activity was computed for the remaining muscle samples from −200 ms to 400 ms relative to perturbation onset and binned into predefined epochs of muscle activity: preperturbation (PRE, −50 to 0 ms relative to perturbation onset), short-latency stretch response (SL, 25 to 50 ms), long-latency stretch response (LL, 50 to 100 ms), and voluntary response (VOL, 100 to 200 ms).

We focused our analyses of the PEC, BI, and WF on conditions in which the BI was preloaded and stretched by the mechanical perturbation. Similarly, we focused our analyses of the DELT, TRI, and WE on conditions in which the TRI was preloaded and stretched by the mechanical perturbation. We completed several different statistical tests (e.g., repeated-measures ANOVA, paired and single-sample t-tests, Spearman rank correlations) in each of the three experiments. Specific details of these procedures are provided in results. All experimental results were considered reliably different if P < 0.05.

RESULTS

Experiment 1

The primary objective of our first experiment was to examine whether long-latency stretch responses routed to shoulder, elbow, and wrist muscles are sensitive to elbow perturbation magnitude. Before detailing these findings we provide a description of participants' behavior.

Features of behavior.

Figure 1 shows the mean hand path trajectories in response to elbow extension (Fig. 1A) and flexion (Fig. 1B) perturbations for both IN and OUT targets. As expected, we found that hand trajectories for IN and OUT targets initially overlapped for a given perturbation magnitude and eventually diverged as participants moved toward the displayed target. We also found that movement time (MT: time from perturbation onset until the hand entered the target) was influenced by the magnitude of the perturbation and target position (Table 1).

Fig. 1.

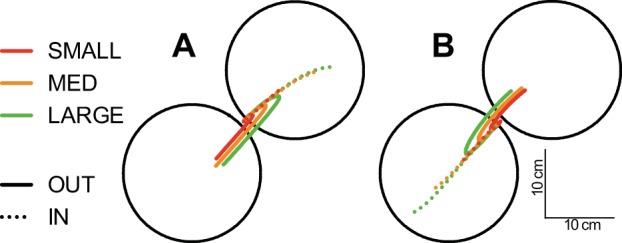
A: mean hand paths after small (1.5 Nm), medium (3.0 Nm), or large (4.5 Nm) extension perturbations applied at the elbow. Dotted and solid lines denote IN and OUT target conditions, respectively. Note that only 1 target is presented on any given trial. B: same format as A but for flexion loads.

Table 1.

Experiment 1 movement time for IN and OUT conditions as function of perturbation magnitude

	Flexion Perturbation			Extension Perturbation
	Small	Medium	Large	Small	Medium	Large
IN	93 (3)	48 (4)	17 (4)	99 (3)	50 (4)	17 (4)
OUT	307 (5)	326 (6)	349 (8)	311 (4)	338 (6)	369 (8)

Experiment 1 movement time values (ms) for IN and OUT conditions as a function of perturbation magnitude are shown. Values within parentheses reflect ±1 SE.

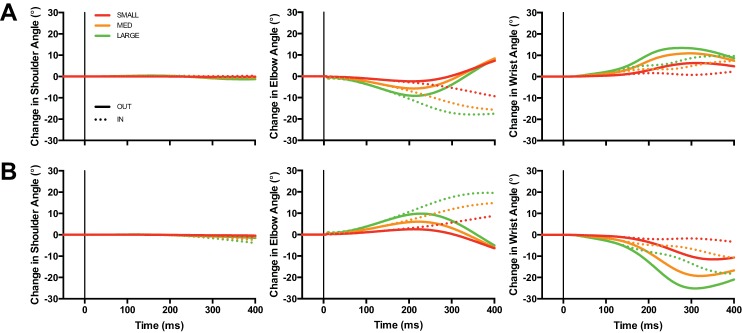
Figure 2 shows the mean change in shoulder, elbow, and wrist angles in response to elbow extension (Fig. 2A) and flexion (Fig. 2B) perturbations for both IN and OUT targets. As expected, we found that changes in elbow angle increased as a function of elbow perturbation magnitude. We also found that changes in elbow angle initially overlapped between IN and OUT targets for matched perturbation magnitudes and eventually diverged as participants moved toward the displayed target. There are three additional aspects of Fig. 2 worth highlighting. First, elbow perturbations did not generate substantial motion at the shoulder (Fig. 2, left). Second, elbow extension perturbations generated wrist flexion (Fig. 2A, right) and elbow flexion perturbations generated wrist extension (Fig. 2B, right). And third, similar to motion at the elbow, changes in wrist angle initially overlapped between IN and OUT conditions for matched perturbation magnitudes and diverged ∼150 ms after perturbation onset as participants moved toward the displayed target. This last finding indicates that participants used their wrist to help transport their hand to the target even though the mechanical perturbation was applied at the elbow.

Fig. 2.

A: change in shoulder (left), elbow (center), and wrist (right) angle after small (1.5 Nm), medium (3.0 Nm), and large (4.5 Nm) extension perturbations applied at the elbow. Dotted and solid lines denote IN and OUT target conditions, respectively. Data are aligned to perturbation onset. B: same format as A but for flexion loads.

Multimuscle coordination of long-latency stretch response.

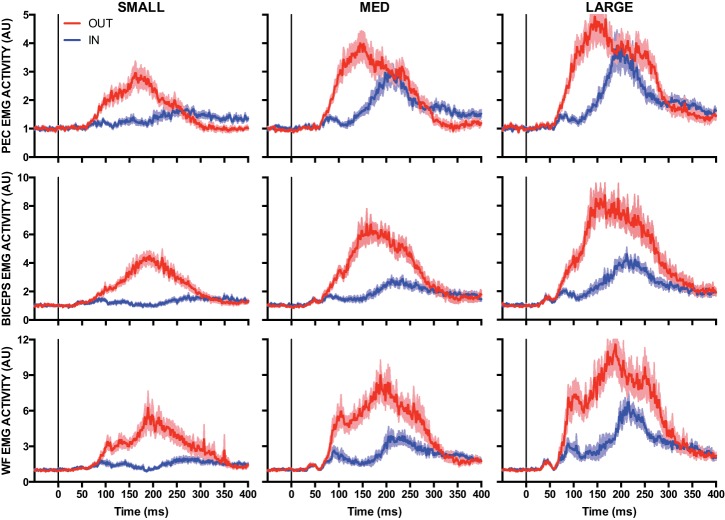
Figure 3 shows mean EMG activity of the PEC, BI, and WF for elbow extension perturbations. Critically, for all muscles, EMG activity for IN and OUT targets appeared to diverge within the long-latency epoch and the difference between these conditions increased as a function of perturbation magnitude. We quantified how the magnitude of the elbow perturbation and target position influenced muscle responses across multiple joints with two different ANOVA models. First, we submitted mean EMG of the BI and TRI to a 2 (Epoch: short latency, long latency) × 2 (Target: IN, OUT) × 3 (Perturbation Magnitude: small, medium, large) repeated-measures ANOVA. Second, we submitted mean EMG within the long-latency epoch for the remaining four muscles to a 2 (Target: IN, OUT) × 3 (Perturbation Magnitude: small, medium, large) repeated-measures ANOVA. Our BI and TRI analyses included epoch as a factor because these muscles were stretched by the mechanical perturbation and thus in a position to show a short-latency stretch response.

Fig. 3.

Mean EMG activity of the pectoralis (PEC, top), biceps (middle), and wrist flexor (WF, bottom) muscles after small (1.5 Nm, left), medium (3.0 Nm, center), and large (4.5 Nm, right) extension loads applied at the elbow. Blue and red traces denote IN and OUT conditions, respectively. Data are aligned to perturbation onset. Shading represents ±1 SE. AU, arbitrary units.

The analysis of BI and TRI yielded reliable three-way interactions between Epoch, Target, and Perturbation Magnitude [BI: F(2,36) = 14.28, P < 0.001, η_partial² = 0.44; TRI: F(2,38) = 24.03, P < 0.001, η_partial² = 0.56; see Table 2 for a full statistical summary]. We decomposed these interactions by submitting mean EMG activity within the short- and long-latency epochs to their own 2 (Target: IN, OUT) × 3 (Perturbation Magnitude: small, medium, large) repeated-measures ANOVA. Mean BI and TRI EMG activity within the short-latency epoch yielded simple main effects of Perturbation Magnitude [BI: F(2,36) = 21.21, P < 0.001, η_partial² = 0.54; TRI: F(2,38) = 28.61, P < 0.001, η_partial² = 0.60]. We used within-subject contrasts to determine whether these simple effects were best fit with a linear or quadratic function (see also below), and results of these contrasts indicated that both effects were best explained by a linear increase as a function of perturbation magnitude [BI: F ratio for linear fit (1,18) = 28.43, P < 0.001, η_partial² = 0.61, F ratio for quadratic fit (1,18) = 1.28, P = 0.27, η_partial² = 0.07; TRI: F ratio for linear fit (1,19) = 47.39, P < 0.001, η_partial² = 0.71, F ratio for quadratic fit (1,19) = 1.34, P = 0.26, η_partial² = 0.07].

Table 2.

Main effects and lower-order interactions for experiment 1

Muscle	Effect	DOF	F Ratio	P Value	ηpartial2
PEC	Target	1,19	40.21	<0.001	0.68
	Magnitude	2,38	40.78	<0.001	0.68
DELT	Target	1,19	44.65	<0.001	0.70
	Magnitude	2,38	43.26	<0.001	0.69
BI	Epoch	1,18	63.44	<0.001	0.78
	Target	1,18	16.07	<0.001	0.47
	Magnitude	2,36	46.75	<0.001	0.72
	Epoch × Target	1,18	38.60	<0.001	0.68
	Epoch × Magnitude	2,36	15.69	<0.001	0.47
	Target × Magnitude	2,36	10.00	<0.001	0.36
TRI	Epoch	1,19	75.71	<0.001	0.80
	Target	1,19	34.72	<0.001	0.65
	Magnitude	2,38	86.77	<0.001	0.82
	Epoch × Target	1,19	40.24	<0.001	0.68
	Epoch × Magnitude	2,38	46.59	<0.001	0.71
	Target × Magnitude	2,38	13.57	<0.001	0.42
WF	Target	1,18	17.92	<0.001	0.50
	Magnitude	2,36	24.12	<0.001	0.57
WE	Target	1,19	35.09	<0.001	0.65
	Magnitude	2,38	29.80	<0.001	0.61

Analyses of BI and TRI used a 2 (Epoch: short-, long-latency) × 2 (Target: IN, OUT) × 3 (Magnitude: small, medium, large) repeated-measures ANOVA. Analyses of PEC, DELT, WF, and WE used the same ANOVA model but excluded the factor of Epoch.

Analysis of mean BI and TRI activity within the long-latency epoch yielded reliable two-way interactions between Target and Perturbation Magnitude [BI: F(2,36) = 15.18, P < 0.001, η_partial² = 0.46; TRI: F(2,38) = 22.60, P < 0.001, η_partial² = 0.54]. We decomposed these interactions by computing within-participant goal-dependent activity (i.e., OUT minus IN EMG activity at matched time points; see Fig. 4, A and B) across the three different perturbation magnitudes. We then computed mean goal-dependent activity within the long-latency epoch for BI and TRI and submitted these values to their own one-way repeated-measures ANOVAs. The outcome of this analysis for both the BI and TRI revealed a simple main effect, and within-subject contrasts revealed that both effects were best explained by a linear increase in goal-dependent activity as a function of perturbation magnitude [BI: linear F(1,18) = 20.21, P < 0.001, η_partial² = 0.53; TRI: linear F(1,19) = 30.02, P < 0.001, η_partial² = 0.61; see Fig. 4, G and H].

Fig. 4.

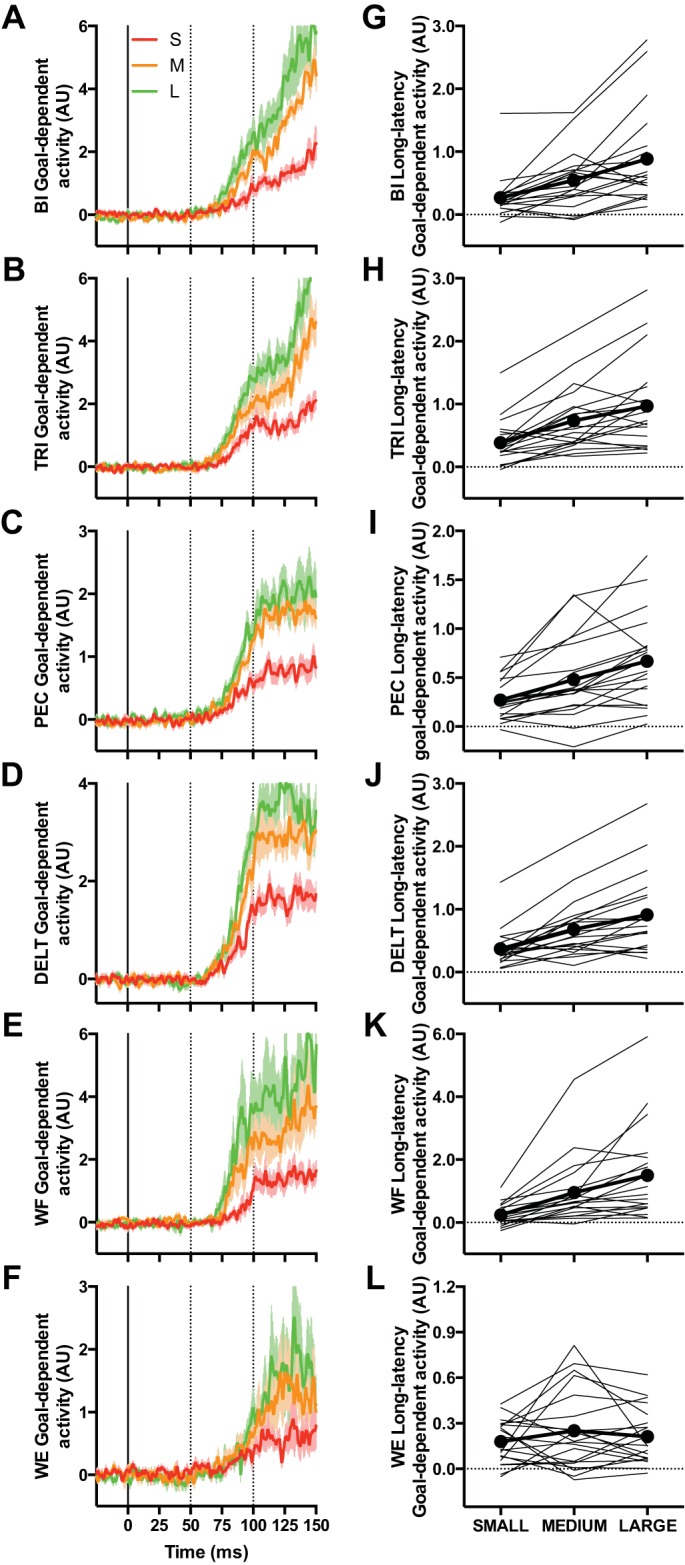
A–F: mean goal-dependent EMG activity (i.e., EMG of OUT − IN condition at matched time points) for biceps (BI), pectoralis (PEC), and wrist flexor (WF) after elbow extension perturbations as well as triceps (TRI), deltoid (DELT), and wrist extensor (WE) after elbow flexion perturbations. Red, orange, and green traces denote small (1.5 Nm), medium (3.0 Nm), and large (4.5 Nm) perturbation magnitudes, respectively. Shading represents ±1 SE. Data aligned to perturbation onset. Vertical dotted lines reflect the boundaries of the long-latency epoch (50–100 ms after perturbation onset). G–L: goal-dependent EMG activity within the long-latency epoch as a function of perturbation magnitude for BI, PEC, and WF after elbow extension perturbations and TRI, DELT, and WE after elbow flexion perturbations. Thin gray lines represent mean goal-dependent activity in the long-latency epoch from individual participants, and thick black lines represent the group mean. AU, arbitrary units.

Our analysis of the remaining muscles was restricted to the long-latency epoch and revealed reliable two-way interactions between Target and Perturbation Magnitude for the PEC [F(2,38) = 18.54, P < 0.001, η_partial² = 0.49], DELT [F(2,38) = 24.06, P < 0.001, η_partial² = 0.56], and WF [F(2,38) = 16.14, P < 0.001, η_partial² = 0.47]. We decomposed these interactions by computing mean goal-dependent activity (Fig. 4, C–F) within the long-latency epoch for each participant as a function of perturbation magnitude and then submitted these values to their own one-way repeated-measures ANOVA. Results yielded reliable simple effects for each muscle [PEC: F(2,38) = 18.54, P < 0.001, η_partial² = 0.49; DELT: F(2,38) = 24.06, P < 0.001, η_partial² = 0.56; WF: F(2,36) = 16.13, P < 0.001, η_partial² = 0.47], and within-subject contrasts revealed that all effects were best explained by a linear increase in goal-dependent activity as a function of increasing perturbation magnitude [PEC: linear F(1,19) = 28.54, P < 0.001, η_partial² = 0.60; DELT: linear F(1,19) = 27.78, P < 0.001, η_partial² = 0.56; WF: linear F(1,18) = 20.24, P < 0.001, η_partial² = 0.53; see Fig. 4, I–K]. Analysis of the WE activity did not yield a reliable two-way interaction (Fig. 4L). Instead, we found a main effect of Target [F(1,19) = 35.09, P < 0.001, η_partial² = 0.65], such that OUT target trials had larger EMG activity compared with their IN target counterparts, as well as a main effect of Perturbation Magnitude [F(2,38) = 29.80, P < 0.001, η_partial² = 0.61], which was best explained by a linear increase in EMG activity as a function of perturbation magnitude [linear F(1,19) = 43.75, P < 0.001, η_partial² = 0.70].

In summary, the BI and TRI mean activity within the short-latency epoch was not modulated by IN or OUT targets but did increase linearly as a function of perturbation magnitude. Furthermore, for five of the six muscles, mean activity within the long-latency epoch was larger for OUT compared with IN target trials, and this difference increased linearly as a function of perturbation magnitude.

Experiment 2

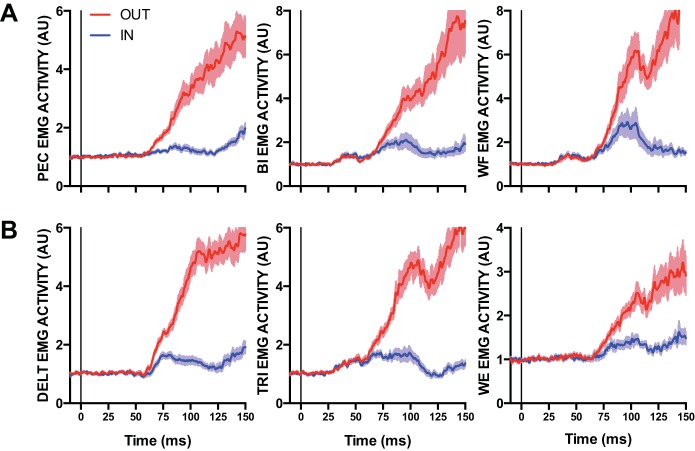
In our second experiment we examined whether and how long-latency stretch responses are coordinated between muscles on a trial-by-trial basis. To robustly estimate these relationships, participants completed the medium perturbation condition used in experiment 1 but with 60 trials per experimental condition instead of 20 trials per experimental condition. Average behavioral and muscle responses were consistent with experiment 1. Mean MT for the IN target was 29 ms (5 SE) for elbow flexion perturbations and 26 ms (5 SE) for extension perturbations. Mean MT for the OUT target was 316 ms (7 SE) for elbow flexion perturbations and 316 ms (8 SE) for elbow extension perturbations. Reliable goal-dependent activity within the long-latency epoch was present for all muscles [all t(14) > 5.08, all P < 0.001, all η_partial² > 0.65] (Fig. 5).

Fig. 5.

A: mean EMG activity of the pectoralis (PEC), biceps (BI), and wrist flexor (WF) after elbow extension perturbations. Blue and red traces denote IN and OUT conditions, respectively. Data are aligned to perturbation onset. Shading represents ±1 SE. B: same format as A but for the deltoid (DELT), triceps (TRI), and wrist extensor (WE) after elbow flexion perturbations. AU, arbitrary units.

Correlations between muscle responses and MT.

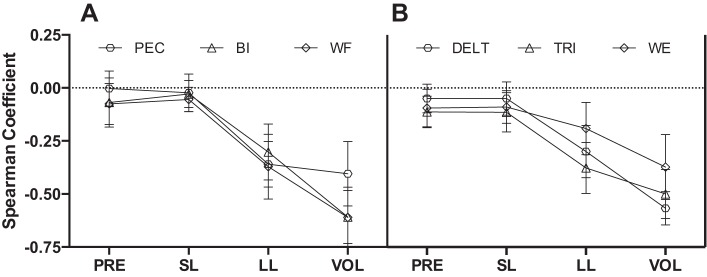
Before assessing whether long-latency stretch responses were coordinated between muscles on a trial-by-trial basis, we wanted to determine when muscle activity became correlated with MT. For each participant we computed Spearman rank correlations between trial-by-trial MT and trial-by-trial mean muscle activity for the OUT target within four epochs (i.e., preperturbation: −50 to 0 ms; short latency: 25 to 50 ms; long latency: 50 to 100 ms; voluntary: 100 to 200 ms) and compared the groupwise correlation coefficients to a theoretical value of zero with single-sample t-tests (Table 3). We found negligible, but occasionally reliable (i.e., P < 0.05), correlations within the preperturbation and short-latency epochs for all muscles (mean correlation coefficient range: 0.00 through −0.11; see PRE and SL in Fig. 6). In contrast, all muscles showed reliable negative correlations within the long-latency epoch and these negative relationships with MT generally strengthened further within the voluntary epoch. That is, larger EMG in the long-latency and voluntary epochs was associated with shorter MTs, and vice versa (see LL and VOL in Fig. 6).

Table 3.

Number of reliable correlations per participant for experiment 2

Muscles	PRE	SL	LL	VOL	Muscle Pairs	Two Com	Load Com	Goal Com
PEC	1/15	1/15	12/15	10/15	PEC/BI	10/15	6/15	10/15
DELT	1/15	3/15	10/15	14/15	BI/WF	9/15	13/15	12/15
BI	1/15	0/15	9/15	15/15	TRI/DELT	13/15	14/15	15/15
TRI	3/15	1/15	11/15	14/15	TRI/WE	7/15	6/15	10/15
WF	3/15	0/15	9/15	14/15
WE	2/15	2/15	7/15	8/15

Left: number of reliable (i.e., P < 0.05) correlations computed between movement time and muscle activity within the preperturbation (PRE), short-latency (SL), long-latency (LL), and voluntary (VOL) epochs. Right: number of the reliable correlations computed between pairs of muscles that used trials that combined (Two Com) or isolated the load-dependent (Load Com) and goal-dependent (Goal Com) components that contribute to the long-latency stretch response.

Fig. 6.

A: mean Spearman rank correlations computed between binned muscle activity (PEC, BI, and WF) relative to perturbation onset (PRE: −50 to 0 ms; SL: 25 to 50 ms; LL: 50 to 100 ms; VOL: 100 to 200 ms) and movement time on a trial-by-trial basis. Correlations were derived from trials in which the BI was preloaded and the mechanical perturbation extended the elbow and moved the hand away from the displayed target (i.e., OUT target). Error bars reflect 95% confidence intervals, and error bars that do not cross zero reflect reliable correlations (i.e., P < 0.05; see Cummings 2013). B: same format as A but for DELT, TRI, and WE. Correlations were computed from trials in which the TRI was preloaded and the mechanical perturbation flexed the elbow and moved the hand away from the displayed target (i.e., OUT target).

Correlations across muscles.

We tested for the coordination of long-latency stretch responses between muscles of adjacent joints during a single goal-directed action. For each participant, we computed mean activity within the long-latency epoch for all muscles from OUT target trials. We then correlated (Spearman rank) the mean activity during the long-latency epoch of the PEC with that of the BI, the BI with that of the WF, the DELT with that of the TRI, and the TRI with that of the WE, on a trial-by-trial basis.

Figure 7, A and B, show scatterplots from an exemplar participant. Each point on these plots reflects the mean activity within the long-latency epoch measured from two muscles during a single trial (PEC and BI, Fig. 7A; BI and WF, Fig. 7B), and the r values denote the correlation between the muscles computed across all these trials. The filled circles in Fig. 7C show the computed correlation coefficients for the four muscle pairings from all participants. That is, each filled circle represents the computed correlation coefficient from an individual participant. We compared these values to a theoretical value of zero with single-sample t-tests, which revealed that the trial-by-trial magnitude of the long-latency stretch responses was reliably positively correlated for all muscle pairs [PEC and BI: t(14) = 4.87, P < 0.001, η_partial² = 0.63; BI and WF: t(14) = 5.39, P < 0.001, η_partial² = 0.67; DELT and TRI: t(14) = 8.94, P < 0.001, η_partial² = 0.85; TRI and WE: t(14) = 4.81 P < 0.001, η_partial² = 0.62]. The same pattern of results was observed when a nonparametric test (i.e., Wilcoxon signed-rank test) was used for these analyses.

Fig. 7.

A: scatterplot of an exemplar participant's mean activity from the long-latency epoch between the PEC and BI. Open circles reflect individual trials in which the BI was preloaded and the mechanical perturbation extended the elbow and moved the hand away from the displayed target. r Value represents computed Spearman rank correlation coefficient. B: same format as A but for the BI and WF. C: black dots represent Spearman rank correlation coefficients computed for each participant from trial-by-trial mean muscle activity within the long-latency epoch between the PEC and BI, the BI and WF, the DELT and TRI, and the TRI and WE. Correlations involving the BI were derived from trials in which the BI was preloaded and the mechanical perturbation extended the elbow and moved the hand away from the target. Correlations involving the TRI were derived from trials in which the TRI was preloaded and the mechanical perturbation flexed the elbow and moved the hand away from the target. Red dots reflect the exemplar participant's correlation coefficient from A and B. Upward triangles reflect correlations computed from trials in which the elbow muscle was preloaded and the mechanical perturbation moved the hand into the target. Downward triangles reflect correlations computed from trials in which the elbow muscle was preunloaded and the mechanical perturbation moved the hand away from the target. AU, arbitrary units.

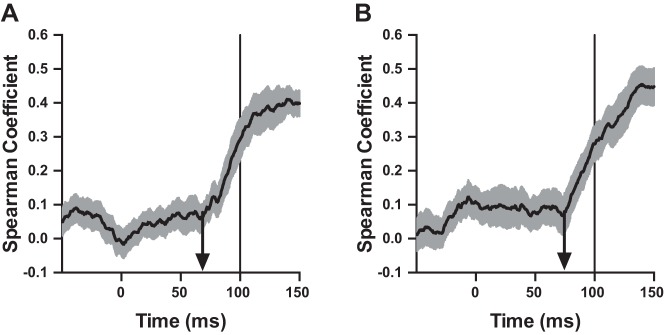
To illustrate how these positive trial-by-trial relationships develop over time, we computed the time series of correlations for each participant using a moving window of mean muscle activity for the four muscle pairs. The window was 50 ms wide and moved in 1-ms increments for each correlation. That is, the first computed correlation used mean EMG activity from −100 to −50 ms relative to perturbation onset, the second computed correlation used mean activity from −99 to −49 ms relative to perturbation onset, and so on through the whole data set. Figure 8 shows the resulting time series of mean Spearman rank correlations between PEC and BI (Fig. 8A) and between BI and WF (Fig. 8B). Note that although the positive correlational structure appears to increase ∼70 ms after perturbation onset (arrows in Fig. 8), the true onset of correlational structure may be up to 25 ms earlier because of lag induced by our moving window. A qualitatively similar pattern of results was observed between DELT and TRI and between TRI and WE.

Fig. 8.

A: causal moving average (50-ms bin size, 1-ms step) of Spearman rank correlation coefficient between PEC and BI muscle activity. Correlations were computed from trials in which the BI was preloaded and the mechanical perturbation extended the elbow and moved the hand away from the displayed target. Because of the 50-ms bin size of the moving average, correlation coefficients at 100 ms (vertical line) are computed from muscle activity 50–100 ms after perturbation onset (i.e., the long-latency epoch; see also Fig. 7C). Positive correlational structure appears to increase ∼70 ms after perturbation onset (arrow). Shading represents ±1 SE. B: same format as A but for BI and WF.

Multiple components of long-latency stretch responses.

Previous work has suggested that muscle activity within the long-latency epoch is comprised of (at least) two independent components: a load-dependent component that is sensitive to the excitability of the motoneuron pool and a goal-dependent component that is sensitive to target position (Kurtzer et al. 2014; Pruszynski et al. 2011a). We tested whether both these contributors structure long-latency stretch responses between muscles by computing trial-by-trial correlations under experimental conditions thought to isolate these components. We reasoned that the goal-dependent component may show negative correlations related to kinematic redundancy and that this structure may have been masked by positive correlations in the load-dependent component in our main analysis above, which included both contributors. For the load-dependent component, we computed Spearman rank correlations from trials in which the BI or TRI was preloaded and the mechanical perturbation moved the hand into the target (Fig. 7C, upward triangles). These trials are thought to isolate the load-dependent component of the long-latency stretch reflex because altering the excitability of the motoneuron pool can modulate long-latency stretch response activity independent of the target location. For the goal-dependent component, we used trials in which the BI or TRI was preunloaded and the mechanical perturbation moved the hand away from the target (Fig. 7C, downward triangles). These trials are thought to isolate the goal-dependent component of the long-latency stretch reflex because altering the target location can modulate long-latency stretch response activity independent of the excitability of the motoneuron pool (for further details on isolating these components see Pruszynski et al. 2011a). Single-sample t-tests of these coefficients were not consistent with our prediction. All muscle pairs showed reliable positive correlations for both the load- and goal-dependent components of the long-latency stretch response [PEC and BI: load dependent: t(14) = 6.23, P < 0.001, η_partial² = 0.74; goal dependent: t(14) = 7.49, P < 0.001, η_partial² = 0.80; BI and WF: t(14) = 7.08, P < 0.001, η_partial² = 0.78; t(14) = 6.91, P < 0.001, η_partial² = 0.77; DELT and TRI: t(14) = 8.62, P < 0.001, η_partial² = 0.84; t(14) = 17.85, η_partial² = 0.96, P < 0.001; TRI and WE: t(14) = 4.89, P < 0.001, η_partial² = 0.63; t(14) = 7.75, P < 0.001, η_partial² = 0.81]. The same pattern of results was observed when a nonparametric test (i.e., Wilcoxon signed-rank test) was used for these analyses.

Experiment 3

The results of our second experiment were counter to our original predictions. Specifically, we anticipated that long-latency stretch responses between elbow and wrist muscles would be negatively correlated because both muscles help to move the hand into the target. One reasonable explanation for the robust positive correlation we observed is that, rather than being modulated to exploit kinematic redundancy, modulation of long-latency stretch responses in wrist muscles was—like shoulder muscles—dominated by the need to counter local torques arising because of intersegmental dynamics. For example, after an elbow extension perturbation that moves that hand away from the target, the rapid elbow flexion required to complete our task generates extension torque at the wrist and countering this torque would require activity of WF muscles.

We examined whether long-latency stretch responses in wrist muscles are modulated to exploit kinematic redundancy or counteract expected intersegmental torques by having participants complete the same task as in experiment 2 while we manipulated how the wrist contributes to the voluntary movement. We did this by explicitly instructing participants how to respond with their wrist to help move their hand to the target (NO Instruct, MIN Instruct, MAX Instruct; see methods). If wrist long-latency stretch responses are modulated to exploit kinematic redundancy, then goal-dependent activity within these muscles should be altered by these different instructions. In contrast, if wrist long-latency stretch responses are modulated to counteract expected intersegmental torques, then the different instructions should have relatively little impact on goal-dependent activity. As described below, our results are consistent with the latter explanation.

Wrist behavior.

Figure 9 shows the mean change in elbow and wrist angles following elbow extension and flexion perturbations for both IN and OUT targets as a function of our three wrist instructions. Consistent with our previous work, we found that changes in elbow and wrist angle initially overlapped for IN and OUT targets and eventually diverged as participants moved toward the displayed target. Importantly, we found that the Maximum Use instruction resulted in the largest change in wrist angle between IN and OUT targets whereas the Minimum Use instruction resulted in the smallest change in wrist angle between IN and OUT targets. These results demonstrate that participants followed our instructions on how to use their wrist to help transport their hand to the target after a mechanical perturbation applied at the elbow.

Fig. 9.

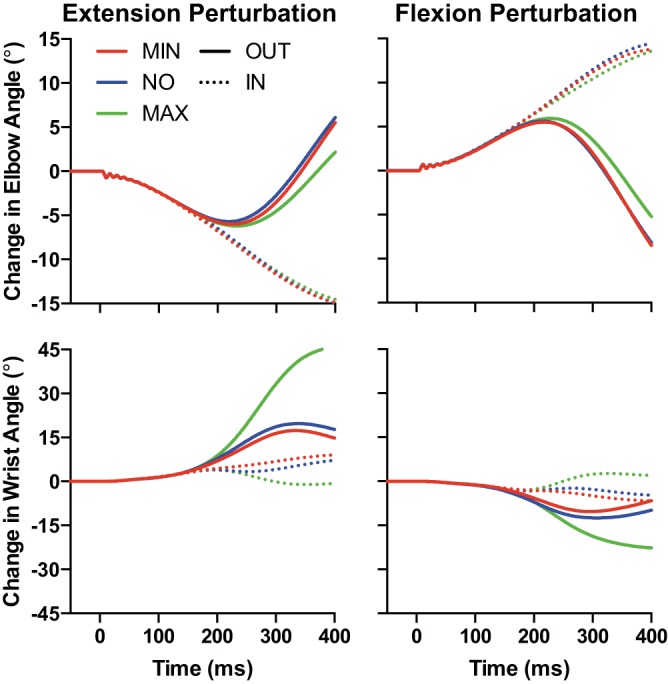
Change in elbow (top) and wrist (bottom) angle following elbow extension (left column) and flexion (right) perturbations. Dotted and solid lines denote IN and OUT target conditions, respectively. Red, blue, and green traces denote Minimum Use, No Instruction, and Maximum Use wrist instruction conditions, respectively. Data are aligned to perturbation onset.

Muscle activity as function of wrist instructions.

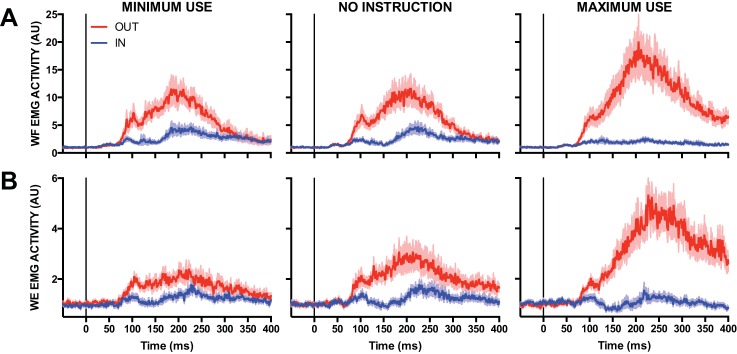
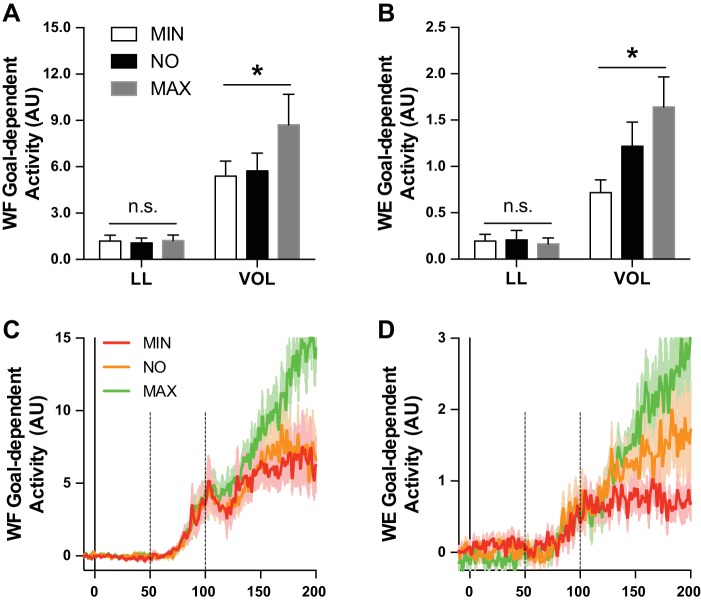
Figure 10 shows mean EMG for the WF (Fig. 10A) and WE (Fig. 10B) for the Minimal Use, No Instruction, and Maximum Use wrist use conditions. Each panel contrasts EMG activity between IN and OUT targets. We submitted mean EMG activity within the long-latency and voluntary epochs for the WF and WE to a 2 (Epoch: long latency, voluntary) × 2 (Target: IN, OUT) × 3 (Instruction: Minimum Use, No Instruction, Maximum Use) repeated-measures ANOVA. Both analyses yielded a reliable three-way interaction [WF: F(2,28) = 6.02, P < 0.01, η_partial² = 0.30; WE: F(2,30) = 6.32, P < 0.01, η_partial² = 0.30; see Table 4 for full statistical summary]. We decomposed these interactions by computing goal-dependent muscle activity (i.e., OUT-IN) within the long-latency and voluntary epochs as a function of the three wrist instruction conditions. We submitted these values to their own one-way repeated-measures ANOVA, which revealed that mean EMG activity within the long-latency epoch did not reliably differ as a function of the instruction set for the WF [F(2,28) = 0.35, P = 0.71, η_partial² = 0.02] or WE [F(2,30) = 0.12, P = 0.87, η_partial² = 0.01], whereas mean EMG with the voluntary epochs showed reliable differences as a function of instruction for both muscles [WF: F(2,28) = 5.93, P < 0.01, η_partial² = 0.30; WE: F(2,30) = 4.77, P < 0.05, η_partial² = 0.24; see Fig. 11].

Fig. 10.

A: mean EMG activity of the WF after elbow extension perturbations as a function of Minimum Use, No Instruction, and Maximum Use wrist instruction conditions. Blue and red traces denote IN and OUT conditions, respectively. Data are aligned to perturbation onset. Shading represents ±1 SE. B: same format as A but for the WE after elbow flexion perturbations. AU, arbitrary units.

Table 4.

Main effects and lower-order interactions for experiment 3

Muscle	Effect	DOF	F Ratio	P Value	ηpartial2
WF	Epoch	1,14	24.46	<0.001	0.64
	Target	1,14	26.25	<0.001	0.65
	Epoch × Instruction	2,28	5.523	<0.01	0.28
	Target × Instruction	2,28	9.07	<0.001	0.39
	Epoch × Target	1,14	26.40	<0.001	0.65
WE	Epoch	1,15	47.10	<0.001	0.76
	Target	1,15	38.41	<0.001	0.72
	Epoch × Target	1,15	29.47	<0.001	0.66

Analyses of WF and WE used a 2 (Epoch: long-latency, voluntary) × 2 (Target: IN, OUT) × 3 (Instruction: NO, MIN, MAX) repeated-measures ANOVA.

Fig. 11.

A: mean goal-dependent (EMG of OUT − IN conditions) activity for the WF within the long-latency (LL: 50–100 ms relative to perturbation onset) and voluntary (VOL: 100–200 ms relative to perturbation onset) epochs after elbow extension perturbations. Goal-dependent activity is plotted as a function of Minimum Use (MIN), No Instruction (NO), and Maximum Use (MAX) wrist instruction conditions. Error bars represent ±1 SE. Note that goal-dependent activity reliably differs between wrist instruction conditions (*P < 0.05) within the voluntary epoch but not within the long-latency epoch [not significant (n.s.)]. B: same format as A but for WE after elbow flexion perturbations. C: mean goal-dependent activity for the WF after elbow extension perturbation at matched time points relative to perturbation onset. Red, orange, and green traces reflect goal-dependent activity associated with the Maximum Use, No Instruction, and Maximum Use wrist instruction conditions. Shading represents ±1 SE, and vertical dashed lines reflect the long-latency epoch. D: same format as C but for WF after elbow flexion perturbation. AU, arbitrary units.

DISCUSSION

We have previously shown that long-latency stretch responses elicited by mechanically perturbing the elbow can be routed to shoulder, elbow, and wrist muscles to support goal-directed reaching (Weiler et al. 2015). Here we designed three experiments to further examine the flexibility and function of this coordination. Consistent with our predictions, we found that the magnitude of goal-dependent activity within the long-latency epoch increased as a function of elbow perturbation magnitude for muscles of the shoulder, elbow, and wrist (experiment 1) and that the magnitudes of long-latency stretch responses in shoulder and elbow muscles were positively correlated (experiment 2). However, we also found that the magnitudes of long-latency stretch responses in elbow and wrist muscles were also positively correlated—a finding inconsistent with our prediction that long-latency stretch responses are routed to wrist muscles to exploit kinematic redundancy (experiment 2). Rather, these results are consistent with the need to counteract interaction torques that will arise at the wrist from rapid arm movement. We then had participants complete the same task but with explicit instructions on how to use their wrist to help transport their hand to the target (experiment 3). We reasoned that if long-latency stretch responses were routed to the wrist to exploit kinematic redundancy, then goal-dependent activity within the long-latency epoch would scale with the amount that participants used their wrist to transport their hand to the target. We found that although participants clearly incorporated the instructions into their overt behavior and voluntary muscle activity, long-latency stretch responses in wrist muscles were not modulated by the instruction set. Taken together, the results of all three experiments indicate that, after elbow perturbations, long-latency stretch responses are flexibly routed to elbow muscles to move the hand to the desired location and to shoulder and wrist muscles to counteract local torques that will arise from rapid arm movement. Our results also suggest that exploiting kinematic redundancy may be outside the functional capacity of the neural circuits that generate the long-latency stretch response.

Flexible Routing and Modulation of Short-Latency Stretch Responses

Although we focused on how long-latency stretch responses are routed across muscles of the arm to support goal-directed reaches, it is important to note that the short-latency stretch reflex can, under some circumstances, also be routed to multiple muscles in a task-appropriate fashion. For example, cutaneous afferent input associated with stepping on a noxious stimulus (e.g., a nail) can excite α-motoneurons of leg flexor muscles of the stimulated foot and leg extensor muscles of the nonstimulated foot to rapidly shift body weight and avoid injury (Sherrington 1910). This crossed-extensor reflex mechanism elicits a motor response primarily governed by stimulus intensity and is largely independent of top-down contributions.

In our work, the short-latency stretch response also appeared to be dependent on the stimulus and was not influenced by top-down control. That is, we found that the magnitude of the BI and TRI short-latency stretch response increased as a function of perturbation magnitude but was not modulated by the participant's movement goal. Although we did not observe goal-dependent modulation of the short-latency stretch response in our task, there are several examples showing that, with extended training lasting days or weeks, the magnitude of this response (Christakos et al. 1983; Wolf and Segal 1996) or the Hoffmann reflex—an electrically induced analog of the short-latency stretch response (Carp et al. 2006; Chen et al. 2006; Thompson et al. 2009; Wolpaw 1987; Wolpaw et al. 1983)—can be intentionally up- or downregulated by direct reinforcement. These studies suggest that it may be possible to volitionally modulate the short-latency stretch response in our task with extensive practice and that this putative spinal plasticity may promote functional recovery for those with upper-limb sensorimotor dysfunction in a manner similar to what has been recently demonstrated for the lower limb (Chen et al. 2006).

Flexible Routing and Utility of Long-Latency Stretch Responses

Many studies have demonstrated that long-latency stretch responses can be flexibly routed to multiple muscles to support a diverse range of functions (Cole et al. 1984; Crevecoeur et al. 2016; Dimitriou et al. 2012; Gielen et al. 1988; Kurtzer et al. 2008, 2009; Manning et al. 2012; Marsden et al. 1981; Mutha and Sainburg 2009; Ohki and Johansson 1999; Omrani et al. 2013; Pruszynski et al. 2011b; Soechting and Lacquaniti 1988; Weiler et al. 2015). For example, Mutha and Sainburg (2009) demonstrated that the long-latency stretch response supports bimanual movements by having participants move a cursor to targets in which the cursor's position was yoked to the averaged position of both hands. On trials in which a mechanical perturbation was applied to one limb, long-latency stretch responses were routed to appropriate muscles of the nonperturbed limb to help correct for the cursor's displacement but only on trials when both limbs could contribute to task success (see also Diedrichsen 2007; Omrani et al. 2013). Dimitrou et al. (2012) also showed a similar flexible routing of long-latency stretch responses in an experiment that required participants to hold a virtual serving tray. In this task, mechanically perturbing one arm elicited long-latency stretch responses in appropriate muscles of the nonperturbed arm to rapidly adjust the tray to its desired orientation.

Long-latency stretch responses can also be flexibly routed to muscles to stabilize the upper limb. In an elegant experiment, Gielen et al. (1988) had participants supinate their wrist after a wrist pronation perturbation and demonstrated that although only the BI was stretched by the mechanical perturbation, long-latency stretch responses were elicited in both the BI (an elbow flexor and wrist supinator) and the TRI. Such routing stabilizes the upper limb because recruiting the BI to counteract the wrist pronation perturbation will also yield unwanted elbow flexion, and this unwanted elbow flexion is counteracted by TRI recruitment. Further evidence that long-latency stretch responses contribute to limb stabilization comes from experiments demonstrating that these feedback responses are routed to muscles to oppose underlying joint torques generated by the mechanical perturbation as opposed to the resulting local joint motion (Kurtzer et al. 2008, 2009; Pruszynski et al. 2011b; Soechting and Lacquaniti 1988) and that long-latency responses are larger when mechanical perturbations are delivered in compliant compared with stiff environments (Akazawa et al. 1983; Dietz et al. 1994; Perreault et al. 2008).

Here we show that long-latency stretch responses are flexibly routed to multiple muscles to serve specific functions required for goal-directed reaching. We found that, after elbow perturbations, long-latency stretch responses were evident in elbow muscles, presumably to help transport the hand to the target, and evident in shoulder and wrist muscles, presumably to counteract local torques that will arise from rapid arm movement. These results are consistent with Crevecoeur and colleagues (2016), who used a paradigm similar to our own but applied perturbations to the shoulder while participants grasped an object. They found that perturbations that displaced the hand away from the target concurrently elicited long-latency stretch responses in the shoulder muscle and in muscles of the fingers that were grasping the object. These authors argued that long-latency responses were routed to shoulder muscles to help move the hand to the target and to the fingers to ensure that the object would not slip out of the hand during rapid arm movement.

Long-Latency Stretch Responses Are Not Preplanned Responses

It has been suggested that modulation of muscle activity within the long-latency epoch reflects the release of a preplanned movement that is elicited by a mechanical perturbation (Ravichandran et al. 2013; Shemmell et al. 2009). Two aspects of our present work do not support this claim. First, flexion and extension perturbations were randomized across all trials, which precluded participants from knowing the appropriate movement before perturbation onset. Despite this, participants were still able to modulate long-latency stretch responses across their entire upper limb as a function of their ultimate movement goal. Second, we found that goal-dependent activity within the long-latency epoch scaled with the magnitude of the elbow perturbation. This would be unlikely to occur if long-latency stretch responses simply reflected the release of a preplanned action. Instead, our findings support the results of many other studies (see Pruszynski and Scott 2012), showing that somatosensory information arising after perturbation onset can be rapidly evaluated with respect to the movement goal and transformed to support the production of the desired goal-directed action.

Long-Latency Stretch Responses Are Positively Correlated Between Upper-Limb Joints

In these experiments we specifically placed the targets so that movement of the elbow and wrist could both contribute to task success. We reasoned that if one or more of the mechanisms that contribute to the long-latency stretch responses aim to optimize task success, they should exploit this kinematic redundancy to minimize overall muscle recruitment—and thus minimize motor noise and control effort (see minimum intervention principle; Todorov 2004; Todorov and Jordan 2002). That is, trials with relatively large long-latency stretch responses at the elbow should be paired with relatively small long-latency stretch responses at the wrist, and trials with relatively small long-latency stretch responses at the elbow should be paired with relatively large long-latency stretch responses at the wrist. We were surprised, therefore, that long-latency stretch responses elicited in elbow and wrist muscles were positively correlated on a trial-by-trial basis. To ensure this was not a spurious finding, we reran our trial-by-trial analysis using data from experimental conditions thought to isolate two independent components (i.e., load- and goal-dependent components: see Pruszynski et al. 2011a) of the long-latency stretch responses. We thought that the load-dependent (and putatively spinal) component of the long-latency stretch responses may have been positively correlated and obscured a negative correlation from the goal-dependent (and putatively cortical) component. This, however, was not the case, as both isolated components of the long-latency stretch responses showed positive correlations between elbow and wrist muscles.

One possible reason why we did not observe negative correlations between long-latency stretch responses of elbow and wrist muscles is because our task was not optimized to exploit kinematic redundancy. For example, our targets were relatively large, and participants may not have been pressured to minimize motor noise and thus optimize muscle recruitment. Another possibility is that changes in arousal (e.g., attention or motivation) throughout our experiment may have masked a negative trial-by-trial relationship between long-latency stretch responses from elbow and wrist muscles. Arousal has been linked with activity of the locus coeruleus (Carter et al. 2010; Usher et al. 1999), which is the nervous system's primary site for norepinephrine synthesis. Neurons that comprise this nucleus make diverse connections throughout the central nervous system to targeted regions that use norepinephrine as a neuromodulator (e.g., spinal cord, brain stem, cerebellum, cortex). Given the broad influence the locus coeruleus has on neural circuitry—including primary motor cortex, which is a key node in the transcortical pathway that contributes to muscle activity within the long-latency stretch response (Cheney and Fetz 1984; Evarts and Fromm 1977; Evarts and Tanji 1976; Omrani et al. 2014, 2016; Picard and Smith 1992; Pruszynski et al. 2011b, 2014; Wolpaw 1980)—it would not be surprising that general changes in arousal could up- or downregulate neural circuits that generate the long-latency stretch response.

We are currently testing this second possibility by examining participants' pupil size prior to a mechanical perturbation. Recent studies have shown that pupil size is correlated with BOLD activity within locus coeruleus (Alnæs et al. 2014; Murphy et al. 2014) and can be used as an index of attentional engagement (Alnæs et al. 2014; Unsworth and Robison 2016; Wierda et al. 2012). If the neural circuitry involved in generating the long-latency stretch response is modulated by output of the locus coeruleus, it may be possible to use pupil size as a covariate to factor out any influences of arousal and potentially reveal a negative correlation between elbow and wrist responses in our task.

Failure to Volitionally Modulate Long-Latency Stretch Responses

In our third experiment, we had participants explicitly exploit kinematic redundancy to different degrees by instructing them how to use their wrist to transport their hand to the target. Although participants clearly incorporated our instructions into their overt behavior, they did not modulate their long-latency stretch responses at the wrist. This finding runs counter to numerous studies showing that long-latency stretch responses can be modulated by a wide variety of experimental factors (see introduction), including how an individual intends to use the wrist when the wrist is mechanically perturbed (Lee and Tatton 1982; Manning et al. 2012; Weiler at al. 2015).

What explains this finding? Given its surprising nature, we are reluctant to make definitive claims. However, our data indicate that muscle activity reflects task goals (elbow muscles in Figs. 3–5, 10, 11) and accounts for intersegmental dynamics (shoulder and wrist muscles in same figures) ∼70 ms after perturbation onset, whereas muscle activity that exploits kinematic redundancy (wrist muscles in Fig. 11) occurs ∼40 ms later. As described above, one possible explanation is that our task was not optimized to exploit kinematic redundancy—an issue we are actively exploring by manipulating the size and position of the goal target as well as perturbation magnitude. A more intriguing explanation is that the neural computations required to exploit kinematic redundancy are more complex than the neural computations required for integrating task goals or accounting for intersegmental dynamics, and thus require additional processing time within the transcortical feedback pathway thought to underlie muscle activity within the long-latency epoch (see Matthews 1991; Pruszynski and Scott 2012). Such neural processing time is evident in primary motor cortex, which responds within ∼20 ms of perturbation onset but requires an additional ∼25 ms to reflect task goals (Evarts and Tanji 1976; Omrani et al. 2016; Pruszynski et al. 2014) and to account for intersegmental dynamics (Pruszynski et al. 2011b). The neural processing time associated with exploiting kinematic redundancy remains unknown, but our data suggest it may be substantially longer. More generally and more interestingly, it remains unknown whether the additional time associated with exploiting kinematic redundancy—or, for that matter, reflecting task goals or accounting for intersegmental dynamics—reflects further processing within primary motor cortex or is a consequence of delayed input from other neural structures.

GRANTS

J. Weiler was supported during this work through the Natural Sciences and Engineering Research Council of Canada (NSERC). P. L. Gribble was supported during this work through NSERC and the National Institutes of Health (NIH). J. A. Pruszynski was supported during this work through NSERC and the Canada Research Chairs Program.

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the author(s).

AUTHOR CONTRIBUTIONS

J.W. and J.A.P. conception and design of research; J.W. and J.S. performed experiments; J.W. and J.S. analyzed data; J.W., P.L.G., and J.A.P. interpreted results of experiments; J.W. prepared figures; J.W. drafted manuscript; J.W., J.S., P.L.G., and J.A.P. edited and revised manuscript; J.W., P.L.G., and J.A.P. approved final version of manuscript.