Experimental determination of excitonic band structures of single-walled carbon nanotubes using circular dichroism spectra

Abstract

Experimental band structure analyses of single-walled carbon nanotubes have not yet been reported, to the best of our knowledge, except for a limited number of reports using scanning tunnelling spectroscopy. Here we demonstrate the experimental determination of the excitonic band structures of single-chirality single-walled carbon nanotubes using their circular dichroism spectra. In this analysis, we use gel column chromatography combining overloading selective adsorption with stepwise elution to separate 12 different single-chirality enantiomers. Our samples show higher circular dichroism intensities than the highest values reported in previous works, indicating their high enantiomeric purity. Excitonic band structure analysis is performed by assigning all observed E_ii and E_ij optical transitions in the circular dichroism spectra. The results reproduce the asymmetric structures of the valence and conduction bands predicted by density functional theory. Finally, we demonstrate that an extended empirical formula can estimate E_ij optical transition energies for any (n,m) species.

The experimental determination of band structure of single wall carbon nanotubes (SWCNTs) is a challenging task, and often must be theoretically predicted. Here, the authors separate SWCNTs in high purity and experimentally determine their excitonic band structures using circular dichroism spectra.

For more than 20 years since the discovery of single-walled carbon nanotubes (SWCNTs), we have lacked accurate knowledge of their unique optical and physical properties due to variations in the allowed structures (n,m), which have been defined using chiral indices¹. It is known that E_ii optical transitions are allowed for the polarization of the electric field of light in parallel to the nanotube axis, whereas E_ij (i_v→j_c) transitions are allowed for cross polarization²,³,⁴,⁵. Usually, only the E_ii transitions can be observed from optical absorption and photoluminescence spectra because a strong local field induced by the applied field obscures the cross-polarized optical transitions³,⁴,⁵. Limited information about the E_ii energies alone is insufficient for constructing valence and conduction band structures that require E_ij values. Although Miyauchi et al³,⁴. succeeded in measuring the cross-polarized optical transitions using aligned SWCNTs, the results were limited. Previously, the detailed band structures of specific SWCNTs could only be obtained by density functional theory (DFT) calculations as predictions⁶ except in the case of experimental studies utilizing scanning tunnelling spectroscopy⁷,⁸. On the other hand, the DFT calculations suggest that all optically allowed transitions E_ii and E_ij are observable in circular dichroism (CD) spectra, even for randomly aligned chiral SWCNTs⁹,¹⁰,¹¹ due to the comparable signal intensities of both transitions. Although SWCNTs have one-dimensional electronic systems, an interaction between right- or left-handed SWCNTs and circularly polarized light should exist. The values of all E_ii and E_ij transitions allow us to analyse the detailed band structures of the SWCNTs with more precision than can be implemented by other optical measurements.

A mixed chirality sample cannot be used for the band structure analysis because numerous peaks are expected in the CD spectra. Furthermore, this analysis requires the preparation of not only high-purity single-chirality but also highly selected enantiomers because the CD intensity is proportional to the differential ratios between left- and right-handed SWCNTs. Peng et al.¹² reported the first enantiomer separation using a specially designed chiral dispersant molecule with different affinities for the left- and right-handed enantiomers¹³, and Ghosh et al.¹⁰ used a nonlinear density-gradient ultracentrifugation (DGU) to achieve enantiomer separation using a common chiral dispersant. However, the purities of these samples were not high enough for the band structure analysis because the observed CD peaks were difficult to assign to a specific chirality. Gel column chromatography with the overloading technique has also been used for the enantiomer separation, but the enantiomer purity was not high enough¹⁴. For the detailed analysis of the band structure, even very weak peaks must be recognized. Higher enantiomer purity is required for obtaining a high signal/noise ratio in a CD spectrum.

To improve both single-chirality purity and enantiomer purity, we developed a separation method that combines selective adsorption with the overloading technique and selective desorption¹⁵ with a stepwise elution gel chromatography. Using a dextran-based gel for the column medium and a mixed surfactant for the eluent, this method can simultaneously separate single-chirality SWCNTs and enantiomers based on the effects of the optically active dextran. Using this technique, here we successfully separate 12 distinct (n,m) single-chirality species of semiconducting SWCNTs and measure their CD spectra. The CD intensity, which is normalized by its optical absorbance, is higher than that of previous reports to the best of our knowledge, indicating that the highest enantiomer purity is obtained¹⁰,¹³,¹⁴. Excitonic band structure analysis is also performed by assigning all the CD peaks to allowed optical transitions. Our results are in excellent agreement with the DFT calculations, which include asymmetries of the valence and conduction bands⁶. Interestingly, a plot of the allowed E_ij transitions against the SWCNT diameters shows a typical ‘family behaviour', which suggest that our results are quite reasonable. Our challenge of excitonic band structure analysis is based on a simple analysis of the CD spectrum, but it originates from an interaction between a circular polarized photon and the chiral structure of the carbon network. The results presented here could be an important reference for further studies on the physical properties of SWCNTs. Moreover, the new separation method developed in this work may be important for possible biochemical and medical applications because the high-purity SWCNT enantiomers could be used for chiral separation and the synthesis of chiral molecules¹⁶.

Results

Single-chirality enantiomer separation and evaluation

In this work, higher-purity separation was achieved by combining the overloading technique for the C–C bond curvature-selective adsorption of semiconducting SWCNTs¹⁷ with diameter-selective desorption by stepwise elution chromatography¹⁵. In the first separation step, 80 ml of a SWCNT dispersion in 3% SDS solution was injected onto a multicolumn filled with 5.6 ml of the dextran-based gel to adsorb semiconducting SWCNTs with very narrow C–C bond curvature distributions. After sufficiently flushing the column, 1% sodium cholate (SC) solution was injected for elution. From the first separation step, we obtained a mixture of several species of SWCNTs in a 1% SC solution (Supplementary Fig. 1). Before the second separation step, the same amount of 1% SDS solution was added to the SWCNTs solution to create a 0.5% SC+0.5% SDS mixed solution. This SWCNT solution was injected onto the gel column, and then stepwise elution chromatography was performed using an aqueous solution of 0.5% SC+0.5% SDS+x% sodium deoxycholate (DOC). For the diameter-selective elution, the DOC concentration x was increased stepwise from 0.01 to 0.07%. In this process, single-chirality species with smaller diameters were eluted with lower DOC concentrations. The relationship between the separation order of (n,m) nanotubes and DOC concentration is consistent with our previous report of diameter-selective desorption¹⁵,¹⁸ (Supplementary Fig 2–4). Importantly, each chirality eluted at two different DOC concentrations, x₁ and x₂ (for example, (6,5) SWCNTs were eluted at 0.030 and 0.035% DOC, Supplementary Fig. 2d), which corresponded to two enantiomers with the same band structure but different affinities to the gel. Finally, we measured their CD spectra and confirmed enantiomer separation ((+)- or (−)-(n,m), described in detail below). This separation method of stepwise elution chromatography is effective not only for single-chirality separation but also simultaneous enantiomer separation. Compared with our previous work¹⁴, a higher resolution of single-chirality enantiomeric separation was achieved in this study, which is mainly contributed by the introduced mixed-surfactant system and the stepwise elution procedure¹⁵.

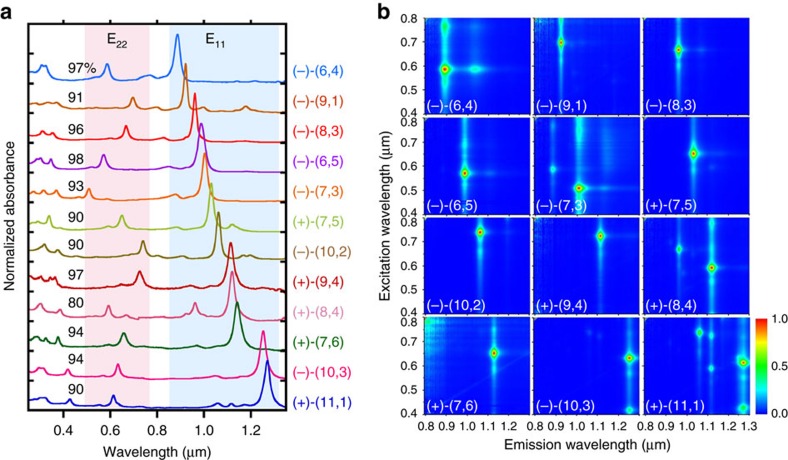
Figure 1a shows the optical absorption spectra of the 12 single-chirality enantiomers of semiconducting SWCNTs obtained in this work. The spectra of either (+)- or (−)-(n,m) enantiomers with higher purities are shown here. Each spectrum exhibits a series of sharp absorption peaks corresponding to the E₁₁, E₂₂, E₃₃ and E₄₄ transitions with very low baseline absorption, which is known as a typical feature of chirality-sorted SWCNTs. These species were identified as (6,4), (9,1), (8,3), (6,5) (7,3), (7,5), (10,2), (9,4), (8,4), (7,6), (10,3) and (11,1) from their E₁₁ and E₂₂ peak positions¹⁹,²⁰. Notably, the baseline absorption of each sample was very low, indicating that impurities such as nanotube aggregates, amorphous carbon, and catalyst particles, were successfully removed in the separation processes²¹,²². The purity of each (n,m) species was basically calculated as an integrated intensity ratio of the absorption peak of the target chirality to the sum of all other peaks using PeakFit software (Supplementary Fig. 5). Since the absorption cross section of each (n,m) species is different²³,²⁴, we have corrected the above purity using a theoretical formula of chirality-dependent oscillator strengths reported in ref. 23. The final purity of each species is indicated in Fig. 1a. Most of the samples were more than 90% pure except for species (8,4). Because the band structure analysis requires high-purity, we selected only high-purity species to maintain the reliability of the analysis. The purities of all species except (8,4) were the same or higher than those of previous reports, including studies using DGU¹⁰, DNA wrapping²⁵ and gel chromatography¹⁷,²⁶ (Supplementary Table 1). Figure 1b shows the photoluminescence (PL) excitation-emission mapping of 12 sorted species corresponding to Fig. 1a. In general, because the E₂₂ excitation - E₁₁ emission peak is dominant in the PL maps, each map had only one bright peak. In addition, the phonon sideband peak is well known to be observed in the excitation spectrum for the E₁₁ emission. In the cases of (10,3) and (11,1), the E₁₁ emission by E₃₃ excitation (∼420 nm) was also observed. Furthermore, we sometimes observe a PL peak from oxide states that were unexpectedly produced in the ultrasonic dispersion process²⁷,²⁸,²⁹. Because the PL intensity of the oxide state was much higher than the original peak, this peak was noticeably bright. Natural oxidation is diameter-dependent and is more frequently observed in small diameter SWCNTs with highly curved side walls, such as (6,4) at 1,050 nm. In general, most of the peaks on the PL map could be assigned to the original E₁₁ transitions, phonon side bands, and oxide states, indicating that high-purity single-chirality SWCNTs were present. In the PL map of (11,1), however, four additional peaks from (10,2), (10,3), (9,4) and (8,4) were observed, while very weak peaks were also observed in the absorption spectra. This suggested that the virtual PL efficiency of (11,1) was lower than that of the impurities, which was probably due to a self-absorption. After obtaining a high-purity single-chirality sample, we could estimate the PL efficiency by measuring the PL intensity, but this was not performed in this work. We assumed that the purity should not be determined by PL intensity, but instead by optical absorption.

Figure 1. Optical evaluation of the separated 12 (n,m) species.

(a,b) Optical absorption spectra (a) and photoluminescence contour maps (b) of the 12 sorted (n,m) semiconducting SWCNTs.

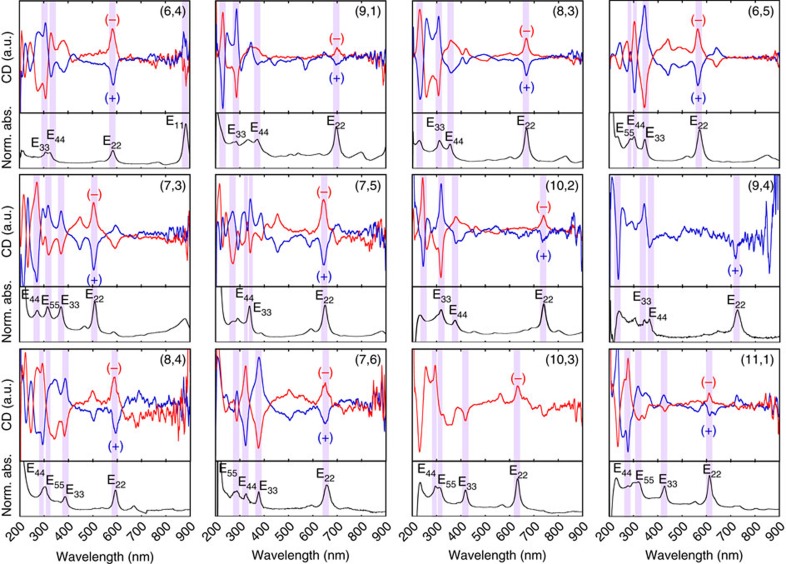
Figure 2 shows the CD spectra and optical absorption spectra for the 12 different (n,m) enantiomers. In the CD spectra, the SWCNTs that exhibited a positive E₂₂ CD peak were denoted as (−), whereas the SWCNTs that exhibited a negative E₂₂ CD peak were denoted as (+)¹⁰,³⁰. Here, (+) and (−) refer to enantiomers that showed plus and minus E₁₁ CD peaks, respectively. Both (+)- and (−)-(n,m) were obtained for all types except (9,4) and (10,3). The missing (9,4) and (10,3) enantiomers were mixed with the other chiralities and could not be recovered as a single chirality. By comparing the CD spectra with the optical absorptions, the CD peak positions of E₂₂, E₃₃ and E₄₄ were determined and were used for the assignments. A photograph of the separated single-chirality SWCNT enantiomers is shown in Supplementary Fig. 6, in which different colours for each chirality indicate different electronic structures; (+)- and (−)-enantiomers are shown in the same colour when the concentrations are same. For example, the same concentrations of (+)- and (−)-(6,5) enantiomers are shown in exactly the same colour (Supplementary Fig. 7).

Figure 2. CD spectra of the separated single-chirality enantiomers.

CD spectra and optical absorption spectra of the 12 different (n,m) species. The CD intensities were normalized for all 12 samples.

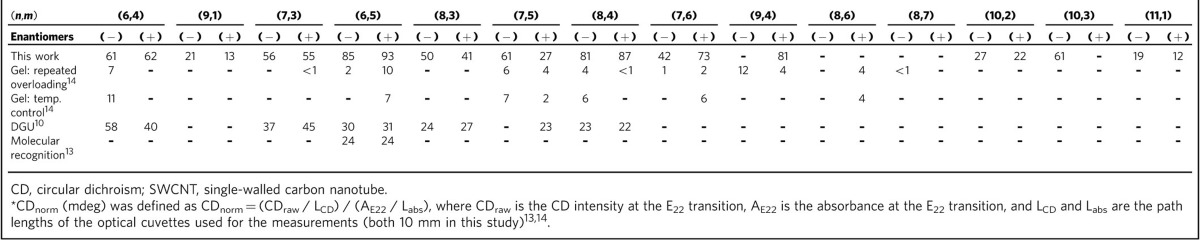
The relative enantiomeric purity of sorted enantiomers can be evaluated by a normalized CD intensity (CD_norm)¹³,¹⁴, instead of commonly used anisotropic factor that cannot be defined for SWCNT. Table 1 summarizes the CD_norm values of individual (n,m) enantiomers obtained in this work and in previous reports. All CD_norm values obtained in this study were higher than those obtained using the repeated overloading method¹⁴, temperature controlled gel chromatography¹⁴, DGU¹⁰ and molecular recognition¹³, indicating that the highest enantiomeric purity was obtained in the present study. The conventional enantiomeric purity of (6,5) enantiomers was roughly estimated to be higher than 90% for both (+)- and (−)-enantiomers. A detailed analysis should be discussed elsewhere.

Table 1. CD_norm^* values of sorted single-chirality SWCNT enantiomers.

Alternative behaviour of CD between Type 1 and Type 2

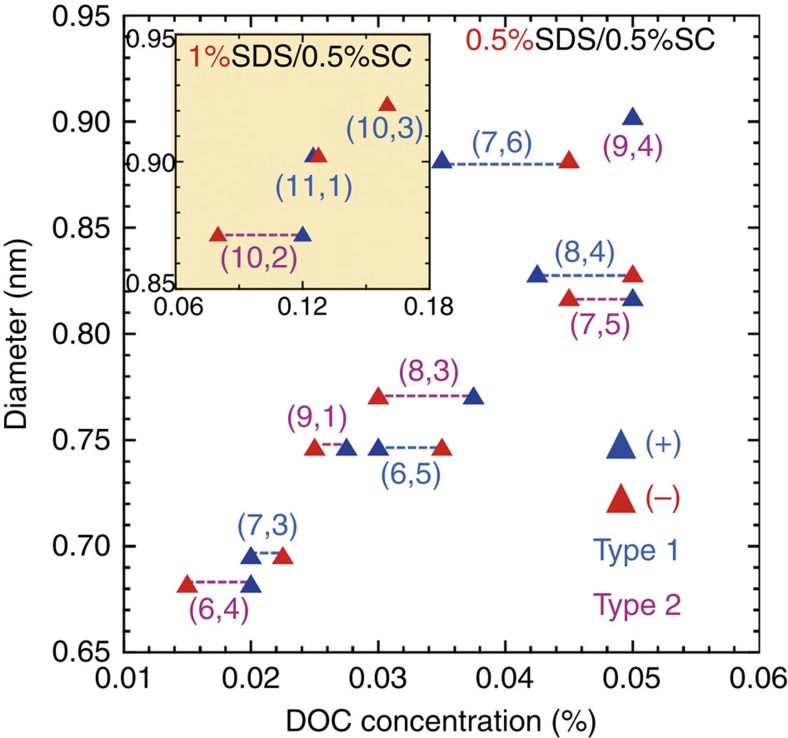
The order of the affinity between the SWCNT enantiomers and the dextran-based gel was scaled by the corresponding DOC concentrations of the stepwise elutions. Figure 3 shows the results of the stepwise elution for the 12 different single-chirality SWCNT enantiomers as a function of nanotube diameter. A clear relationship between the diameter and the DOC concentration can be seen; namely, the required DOC concentration was higher for SWCNTs with larger diameters. Interestingly, for the separation of Type 1 enantiomers (|n−m|=3a+1, where ‘a' is an integer¹⁹,²⁰,³¹; (7,3), (6,5) (7,6) and (8,4)), higher DOC concentrations were required for the (−)-enantiomers than that for the (+)-enantiomers, whereas lower DOC concentrations were required for the (−)-enantiomers than for the (+)-enantiomers for Type 2 (|n−m|=3a+2; (6,4), (9,1), (8,3) and (7,5)). A similar relationship was obtained in the separation of the (10,2), (11,1) and (10,3) enantiomers, which are large diameter enantiomers requiring the use of 0.5% SC+1% SDS as a solvent instead of 0.5% SC+0.5% SDS (Fig. 3, inset). The enantiomer separation was probably achieved due to the interaction between the optically active SWCNT and the optically active dextran-based gel. If this assumption is correct, the separation order of handedness should be the same for all single-chirality SWCNTs. However, our results showed that the separation order of (+)- and (−)-enantiomers depended on the type of SWCNT index. A possible explanation is that the CD signals of Type 1 and Type 2 SWCNTs with a specific handedness have opposite signs. This statement is supported by theoretical calculation of CD spectra of SWCNTs described below.

Figure 3. Relationship between DOC concentration and diameter.

The SWCNTs eluted at higher DOC concentrations have higher affinity toward the dextran-based gel. Inset: the relationships for (10,2), (11,1) and (10,3) SWCNTs separated in the solvent containing 0.5% SC and 1% SDS (see Supplementary Methods and Supplementary Fig. 4). Blue and red triangles denote (+)- and (–)-SWCNTs, respectively. Type 1 and Type 2 chirality (n,m) are denoted as blue and pink letters, respectively.

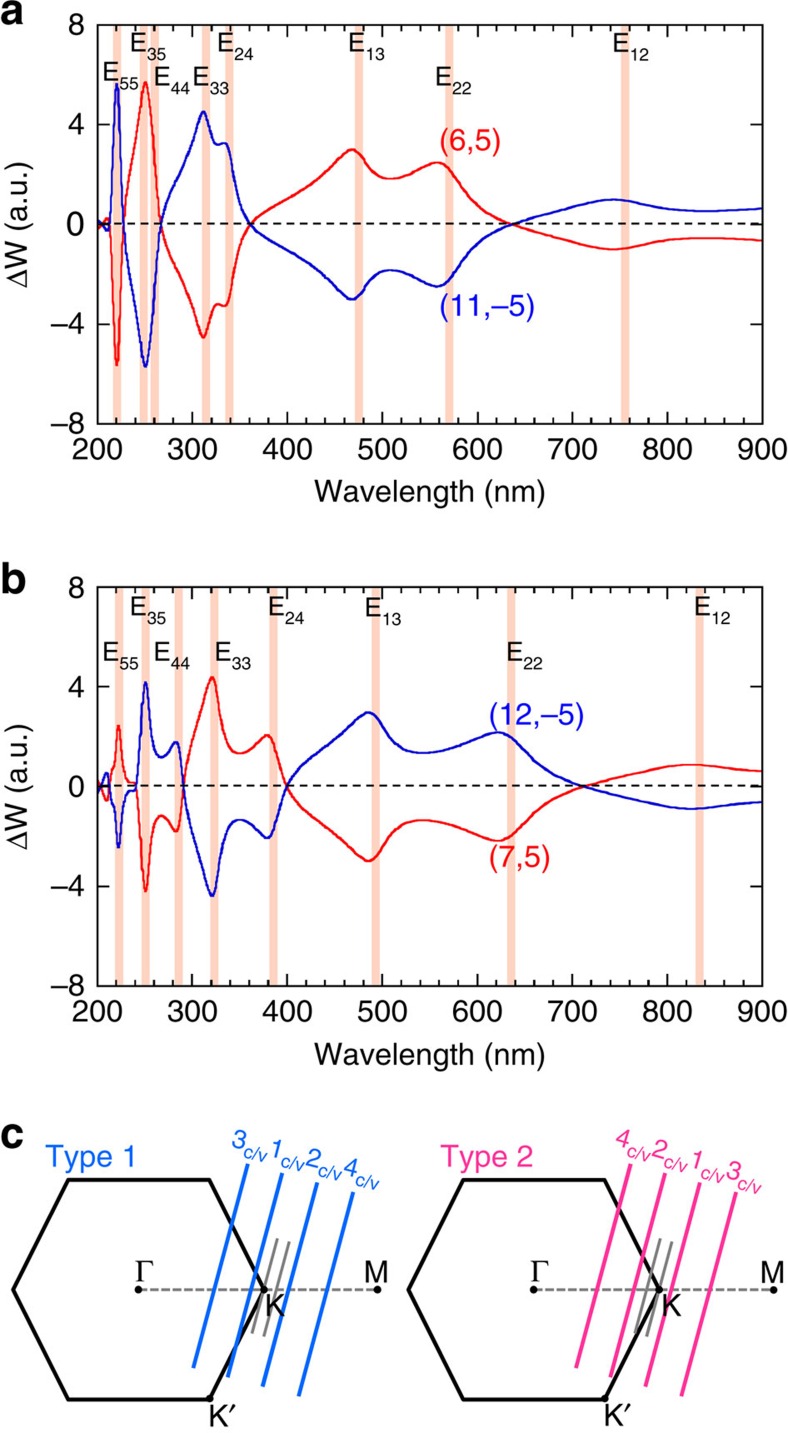
In Fig. 4a, we show the calculated CD signal ΔW as a function of the wavelength of the incident circular polarized lights in the direction perpendicular to the nanotube axis, for (6,5) (red line) and (11,−5) (blue line) SWCNTs in which (n,m) and (n+m,−m) SWCNTs are enantiomers to each other. In the numerical calculation, ΔW is defined by the difference of optical absorption probability of (6,5) (or (11,−5)) SWCNT for the left- and right-handed circular polarized lights within tight-binding method for optical transition of SWCNTs³². It is important to note that the optical absorption probability near the K point for the right-handed circular polarized light is identical to that near the K′ point for the left-handed circular polarized light because of the time reversal symmetry between the K and K′ points, if we adopt an approximation of the vertical optical transition in the k space. It means that we would get ΔW=0 if we adopted this approximation. Thus it is essential in the calculation of ΔW to consider the phase difference of the light for different carbon atom positions that is usually neglected in the calculation of the absolute values of the optical absorption. The detail of the calculation will be reported elsewhere.

Figure 4. Theoretical calculation of CD spectra of enantiomers.

(a,b) Calculated CD signal ΔW of enantiomers for (a) (6,5) (red) and (11,−5) (blue) and (b) (7,5) (red) and (12,−5) (blue), as a function of wavelength of incident light. Shaded lines correspond to E_ii and E_ij van Hove singularity of joint density of states. (c) Reciprocal space of the graphene and cutting lines corresponding to Type 1 (left of panel) and Type 2 (right of panel) SWCNTs.

The calculated results reproduce the experimental results in many aspects: (1) ΔW of (6,5) SWCNT changes the sign at E₂₂, E₃₃, and E₄₄ and (2) ΔW of (11,−5) SWCNTs gives the opposite sign to that of (6,5) for all the wavelength of the light. Further, when we change (n,m) from (6,5) (Type 1 SWCNT) to (7,5) (Type 2 SWCNT) as shown in Fig. 4b, we get the opposite sign of ΔW to that for (6,5) for each E_ii energy. This phenomenon can be explained by the difference in the reciprocal space of the graphene and cutting lines between Type 1 and Type 2 SWCNTs as shown in Fig. 4c. The optical absorption for E_ii van Hove singularity of Type 1 SWCNT occurs at the opposite k points measured from the K point compared with that of Type 2 SWCNT, whose transition optical dipole moment around the K point gives the opposite sign³³. The opposite behaviour between Type 1 and 2 SWCNTs in the calculation is also consistent with the experimental expectation based on their type-dependent affinities with gel. Thus, alternative behaviour of ΔW as a function of the wavelength comes from the change of the wavefunctions on one-dimensional cutting line near the K point³⁴,³⁵.

It should be mentioned that we measure CD of SWCNTs with random orientation in the experiment. For incident circular polarized light, there are two inequivalent geometries of SWCNTs: The propagating directions of the light are (1) perpendicular to the nanotube axis (the case of Fig. 4a,b) and (2) parallel to the nanotube axis (not shown). Even though we should integrate the CD for possible direction of the light, we did not show this for simplicity. We can imagine that the case of (2) gives a minor contribution after integration of azimuth angle of spherical coordinate. Further, we did not consider the effect of the exciton in this calculation in the first approximation. Since the wavefunction of an exciton is given by linear combination of the Bloch functions along one-dimensional Brillouin zone (or cutting line)³⁶,³⁷, we can say that the behaviours of CD do not change even after we consider the exciton effect. Thus the present calculated results are reasonable to simulate the experimental results.

Asymmetric band structure analysis

For the analysis of the CD peaks, we needed to know the selection rules for the CD signal and the related optical transitions for an individual SWCNT³⁸. The energy bands and the allowed optical transitions of a semiconducting SWCNT are shown in Supplementary Fig. 8. Optical transitions from the valence band (denoted as n_v) to the conduction band (denoted as n_c) in a semiconducting SWCNT are characterized by Δn, where Δn=n_c−n_v. When the electric field polarization of incident light is parallel to the SWCNT axis, optical transitions with Δn=0 (E₁₁, E₂₂, E₃₃…) are allowed, and when the polarization is perpendicular to the axis, optical transitions with Δn=±1 (E₁₂/E₂₁, E₁₃/E₃₁, E₂₄/E₄₂…) are allowed⁵,³⁸. Usually, only E_ii transitions can be clearly observed in optical absorption spectra for randomly aligned SWCNTs because the intensity of the E_ii transitions is much higher than the intensity of the E_ij transitions. However, it is known that both E_ii and E_ij can be observed comparably in the CD spectra. Furthermore, based on this alternating behaviour of E_ii CD signals of the (+)- and (−)-enantiomers, the E₁₁∼E₅₅ signals of (+)-enantiomers exhibit a (+,−,+,−,+) progression, whereas the signals of the (−)-enantiomers exhibit the inverted (−,+,−,+,−) progression. This rule helped us to assign the deep E_ii transitions, in which the trigonal warping effects tended to modify the E_ii energy orders⁹,¹⁰,³⁹. Actually, it has been reported that (6,4) has a higher E₃₃ value compared with its E₄₄ value⁹,³⁹. Optical absorption cannot detect this crossover, but the sign of the CD signal can. Similarly, in this work, three E_ii transition peaks were observed in the absorption spectrum of (8,3) SWCNTs, where the corresponding CD sign of the (–)-enantiomer exhibited a (+,+,–) progression from long to short wavelengths, as shown in Fig. 2. On the basis of the signs of the CD signals, the first peak at 668 nm could be assigned to the E₂₂ transition, and the following two peaks at 357 and 307 nm could be assigned to the E₄₄ and E₃₃ transitions, respectively. This crossover is consistent with the reported family pattern of higher order transitions³⁹.

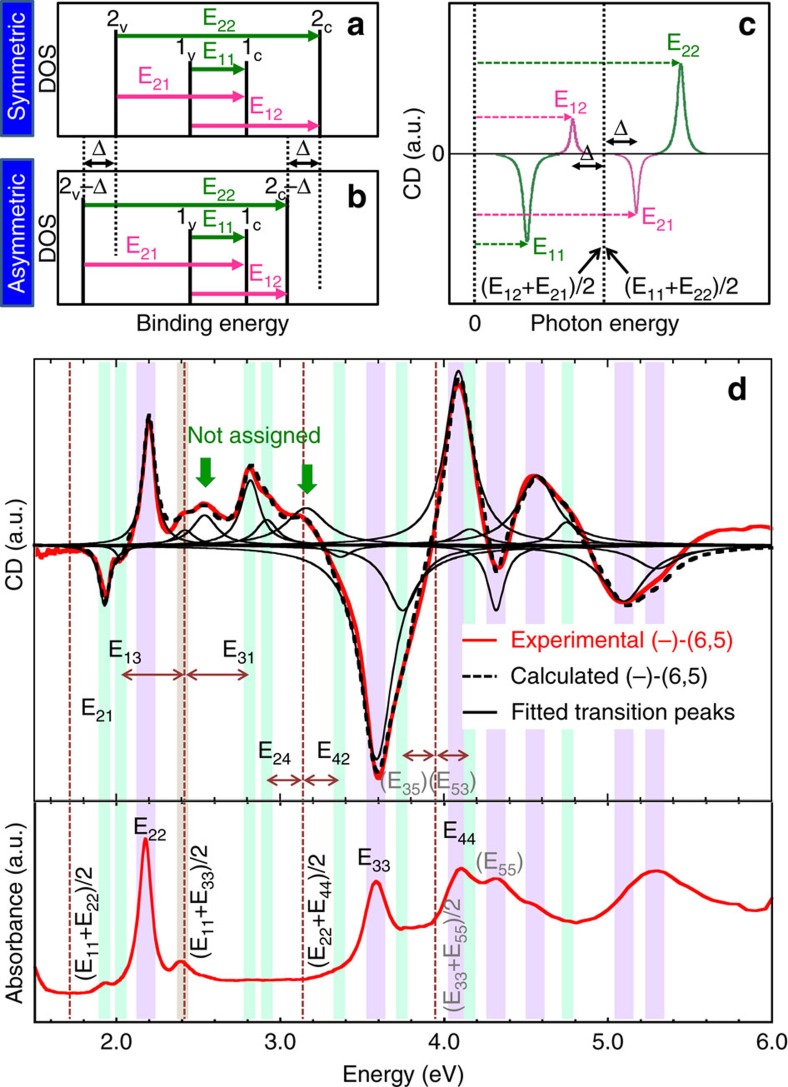
Because a CD spectrum is equally sensitive to optical transitions of parallel and perpendicular polarizations, we could deeply analyse the band structure of SWCNTs using CD spectra. Figure 5a illustrates a symmetric band structure, in which E₂₁=E₁₂, and Fig. 5b shows a more realistic asymmetric band structure model. For both band structures, the values of E₁₁ and E₂₂ were the same, but the 2_v and 2_c bands were shifted relatively deeper than the Δ value for the asymmetric band (Fig. 5b). Using this simple asymmetric band structure model, we analysed an asymmetric SWCNT band structure for which E₂₁−E₁₂=2Δ. For both the symmetric and asymmetric band structures, the same relationship of (E₁₁+E₂₂)/2=(E₁₂+E₂₁)/2 was satisfied, meaning that the centre position of the E₁₁ and E₂₂ peaks were the same as the centre of the E₁₂ and E₂₁ peaks. This result is shown in a schematic CD spectrum in Fig. 5c. In this spectrum, the E₁₂ and E₂₁ transition peaks are located at the lower (left of panel) and higher (right of panel) energy sides of the centre of E₁₁ and E₂₂ by a distance of Δ. Using this model, we found E₁₂ and E₂₁ peaks between the E₁₁ and E₂₂ peaks that were the same distance from the centre of the E₁₁ and E₂₂ peaks and had opposite signs⁹. Similarly, we found E₁₃ and E₃₁ between E₁₁ and E₃₃, E₂₄ and E₄₂ between E₂₂ and E₄₄, and so on. Here we did not consider the exciton binding energy difference in these optical transitions.

Figure 5. Analysis of CD and optical absorption spectra.

(a) Schematic diagrams of a symmetric band structure. (b) Schematic diagrams of an asymmetric band structure with a shift of Δ for 2_c and 2_v bands compared with a. (c) Schematic CD spectrum of a (−)-enantiomer with the asymmetric band structure shown in b. (d) CD spectra (upper panel) and absorption spectra (lower panel) of the sorted (−)-(6,5) SWCNTs. Four vertical dashed lines indicate the center positions of E₁₂/E₂₁, E₁₃/E₃₁, E₂₄/E₄₂, and E₃₅/E₅₃. The E_ii and E_ij transitions are depicted as purple and green strips, respectively. Individual single Lorenzian peaks were plotted using the energy values of all assigned transitions. The calculated CD spectrum (black dashed curve) is represented as the sum of all plotted peaks for comparing the experimental spectrum (red solid curve). Two unassigned peaks are labelled by green arrows.

Figure 5d shows the CD spectra (upper panel) and absorption spectra (lower panel) of the (−)-(6,5) SWCNTs separated in this work. Highly symmetric CD spectra of (6,5) enantiomers (shown in Fig. 2) indicated that our sample purity is high enough and we did not need to consider any impurities that might have affected the CD spectrum. All CD peaks could be assigned to (6,5) SWCNTs. First, we assigned E_ii transitions by comparing the CD spectra with the absorption spectra, which are depicted as purple strips. After the determination of the E_ii transition, the centre positions of E_ij transition pairs (E₁₂/E₂₁, E₁₃/E₃₁, E₂₄/E₄₂ and E₃₅/E₅₃) were determined and depicted as vertical dashed lines. Then, we looked for E_ij transitions on both sides of the centrelines at the same distance and found an appropriate set of E_ij transitions, which are depicted as green strips in Fig. 5d. Because the sensitivity of the detector in the low energy region (∼1.7 eV) was not high enough to see the detail of the spectrum structure, we could not find the E₁₂ peak. Thus, the assigned E₂₁ peak did not have a partner E₁₂ peak. Although the transitions higher than E₄₄ can be observed in high energy region, it is difficult to accurately assign them due to the complicated CD peaks. Using all assigned peak energies, the CD spectrum was reproduced by Lorentzian oscillator fitting (black solid curves). The calculated CD spectrum (black dashed curve) was highly consistent with the experimental spectrum (red solid curve), except for two unassigned CD peaks at 2.54 eV, 3.14 eV. These peaks were probably contributed by phonon-assisted excitonic recombination, which was resolved in the PL measurement⁴⁰. Under the same analysis rules, the CD peaks of the other 11 (n,m) species were assigned (Supplementary Fig. 9).

Using all the E_ii and E_ij transition energies, the valence and conduction band energy levels (n_v and n_c) were determined for the 12 different sorted (n,m) species. The experimental results based on CD spectra were compared with the electronic density of states (DOS) calculated by DFT using local density approximation (LDA)⁶ (see Supplementary Fig. 10). Generally, GW approximation gives more precise band structure including bandgap⁴¹. However, GW calculations were limited for very small diameter SWCNTs to date due to required huge resources. On the other hand, Saito et al. have already published DFT band structures for most of SWCNTs using LDA⁶. They also compared LDA and GW calculations for (7,0) SWCNT and found that well known bandgap underestimation in LDA can be corrected by simple bandgap enlargement⁴². Therefore, here we corrected DFT results by shifting n_c and n_v away from the Fermi level for +0.15 and −0.15 eV, respectively. On first observation, all SWCNTs appear to have asymmetric valence and conduction bands, which is roughly consistent with the DFT calculation. Since we modified LDA bandgap to fit the excitonic band gap, it is trivial that 1_v and 1_c levels show good agreement for most of species. Especially, the species (6,4), (8,3), and (10,2) showed good agreement in the second conduction and valence bands (2_c and 2_v). However, (6,5), (7,5), (9,4), (8,4), (7,6), (10,3) and (11,1) showed redshift of both 2_c and 2_v bands keeping good agreement for E₂₂. Since E₂₂ and E₂₁ CD peaks were used to estimate asymmetric 2_v and 2_c levels, the redshift in 2_v and 2_c levels from LDA band is probably caused by the difference in exciton binding energy between E₂₁ and E₂₂ transitions. On the other hand, the species (9,1), and (7,3) disagreed with E₂₂ value from DFT calculation. This suggests that the exciton binding energies of E₁₁ and E₂₂ are different in these species. Most of species showed rather large deviations in the higher order energy bands (n_c, n_v≥3) probably due to more complicated excitonic and environmental effects. As we mentioned above, we did not consider the exciton effects in our analysis. This means that the band structures obtained in our analysis included the excitonic effects because all optical transitions in carbon nanotubes are modified by the one-dimensional exciton effect. By contrast, the LDA calculations did not include the excitonic effects, and only bare band structures were obtained by the LDA calculations. Even if both band structures were perfectly correct, the deviation could be caused by excitonic effects. Another possible explanation is the band gap correction that we employed in the comparison. We shifted the LDA band by ±0.15 eV to correct for the underestimation of the bandgap, though this bandgap correction seems overly simple. In the future, the underestimation of the bandgap in LDA could be corrected by a heavier but more precise GW approximation. Precise calculations would provide a better comparison between the experimental band analysis and theoretical calculations. Furthermore, we must account for environment effects⁴³,⁴⁴. In our experiments, the SWCNTs were in water containing surfactants, while the SWCNTs in the DFT calculations were in a vacuum. Furthermore, the optical transition energies of SWCNT are highly modified by environmental effects, which were not considered in the DFT calculation or in our CD analysis. Although we must note the potential causes of these deviations, the band structures obtained by our CD analysis were far from those of the tight binding model and generally showed good agreement with the DFT bands. Though the pure experimental band analysis is the first challenge, the crossover of band energy observed in this study is helpful for understanding the DFT calculations because the calculated DOS does not explain the band index in the high energy region. We believe our simple analysis method may provide a tool for the experimental analysis of the unclear band structure of SWCNTs.

Discussion

Because the band structure of a SWCNT is basically determined by a projection from the two dimensional graphene band to the one-dimensional SWCNT band, the band structure of a SWCNT has a systematic trend depending on its chiral structure. Usually, only the family behaviour of E_ii transitions is discussed because the usual optical measurements can only give information regarding E_ii. However, the CD analysis allows for a discussion of the family behaviour of E_ij transitions. The experimental E_ii and E_ij transition energies of the 12 (n,m) species obtained in this work are plotted as a function of the diameter (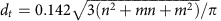; Supplementary Fig. 11). In agreement with the previous report³⁸, the transition energy crossover of E_ii (E₃₃>E₄₄) was observed in some of Type 2 species such as (6,4), (9,1), (8,3), (10,2) and (9,4). Interestingly, further crossover of the E₃₁ and E₄₂ (E₃₁>E₄₂) was observed in a few Type 2 species, (9,1), (8,3) and (10,2) for the first time. These crossovers are known to be caused by a trigonal warping effect⁹,¹⁰,³⁹. In the previous works, a simple scaling behaviour for the E_ii optical transitions have been studied using an empirical formula shown in the following⁴⁵,⁴⁶,⁴⁷,

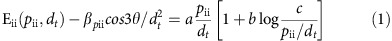where θ indicates a chiral angle, p_ii indicates the cutting line index for E_ii transition; p_ii=1, 2, 4 and 5 for E₁₁, E₂₂, E₃₃ and E₄₄, respectively. To examine our assigned E_ii and E_ij transitions, the similar analysis was performed. First, we confirmed the same nonlinear scaling behaviour of E_ii transitions obtained in this work (Supplementary Fig. 12a). Because resonance energies of Raman scattering was used for the fitting in the previous work, E_ii values are limited in the range of excitation laser. The E_ii values obtained in this work are in the different diameter range from them but the fitting parameters are almost same and all data are on the same curve. For the analysis of E_ij transitions, we tried to extend Eq. 1 as follows.

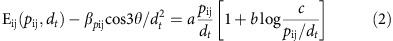Here, the cutting line index p_ij for E_ij transition is 1.33, 1.67, 2, 3, 3 and 4 for E₁₂, E₂₁ E₁₃, E₃₁, E₂₄ and E₄₂, respectively. After careful optimization, we found that E_ij transitions also exhibit a similar scaling behaviour with E_ii transitions although the higher transitions have rather large deviations (Supplementary Fig. 12b). This means that the empirical formula is also available for E_ij transitions.

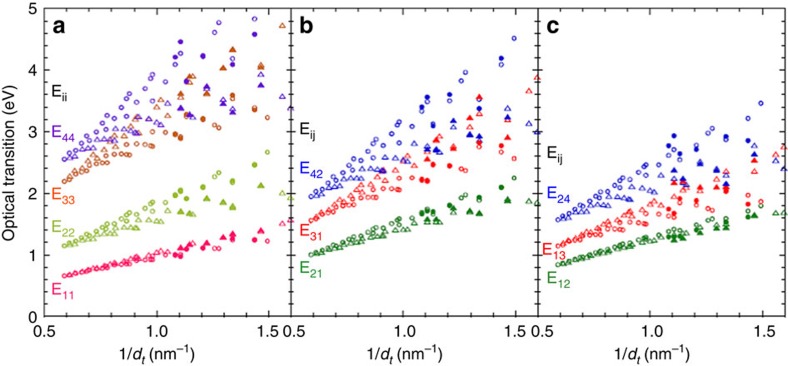
Figure 6a–c shows the E_ii and E_ij transition energies estimated from the CD analysis as a function of 1/d_t (denoted as solid circles and solid triangles). The transition energies calculated from Eq. 1 and Eq. 2 are also shown for comparison (denoted as open circles and open triangles). The empirical calculation well reproduced all experimental values including their family behaviours. For example, E₂₁ is always larger than E₁₂ due to large electron-hole asymmetry. Interestingly, chiral angle dependence of E₁₂ is very small compared with the other transitions. Type dependences are also observed not only in E_ii but also in E_ij. Even for higher transitions, we can expect the family behaviour. However, rather large deviations were observed especially for small diameter species. The largest error was 0.36 eV for E₂₄ transition of (7,3), which is probably due to the modified band structure by a large curvature effect. Because of the good agreement between the experimental and calculated values, E_ij values can be estimated for other species that are not measured. Although the exciton effects are not well known especially for higher lying transitions, our findings firstly reveal that the diameter, chiral angle, type play an important role in the electron-hole asymmetry of SWCNTs, which is very useful for deeper understanding of the physical properties of SWCNT.

Figure 6. E_ii and E_ij transition energies.

(a–c) Transition energy of E_ii and E_ij as a function of 1/d_t. Open and solid symbols (circles for Type 1 and triangles for Type 2 species) indicate the calculation and experimental results, respectively.

In conclusion, we developed an advanced method for separating single-chirality SWCNT enantiomers by column chromatography in combination with overloading selective adsorption and stepwise elution using a mixed surfactant. This separation afforded the highest purity of both single-chirality samples and enantiomeric SWCNTs obtained thus far. The high-purity single-chirality SWCNTs enantiomers enabled us to analyse their complicated CD spectra. We could assign E_ii and E_ij optical transitions to the CD peaks by considering the selection rules of optical transitions, the alternating CD signs for E_ii transitions, the crossovers of E_ii transitions, the relationships between E_ii and E_ij (for example, (E₁₁+E₂₂)/2=(E₁₂+E₂₁)/2), and other factors. Using the obtained E_ii and E_ij energies, the asymmetric excitonic band structures of the 12 different (n,m) SWCNTs were successfully determined. This is the first experimental determination of asymmetric band structures of an extensive set of (n,m) SWCNTs. Furthermore, based on the present experimental results and empirical formula, we demonstrated E_ij for many (n,m) species and revealed (n,m) dependent electron-hole asymmetry.

Methods

Preparation of SWCNT dispersion

SWCNTs produced by high-pressure catalytic CO decomposition (HiPco, Raw, 0.8∼1.2 nm in diameter, NanoIntegris, Skokie, Illinois, USA) were used as a starting material. A net weight of 100 mg of SWCNT powder was dispersed in 100 ml of 3% SDS (ultra-pure ≥99.0%, Sigma-Aldrich) aqueous solution in a glass bottle by an ultrasonic homogenizer (output 30%, Sonifier 250D, Branson) for 20 h (all surfactant concentrations were weight percentage). The bottle was immersed in a water bath at 20 °C. After ultrasonication, the solution was ultracentrifuged for 2 h to sediment bundles and impurities (S50A rotor, 210,000 × g, 25 °C, CS150GX, Hitachi Koki, Tokyo, Japan). The upper 80% of the supernatant was collected as the isolated SWCNT dispersion and was used for the following separation.

Chirality and enantiomer separation

The first separation utilized the overloading selective adsorption method. Five open columns filled with 5.6 ml of gel (Sephacryl S-200, GE Healthcare) were vertically connected. After equilibration with 3% SDS solution, 80 ml of the SWCNT dispersion was loaded into the top of the multicolumn. Because the amount of SWCNTs was much larger than the capacity of the gel, excess SWCNTs flowed through the bottom column (the unadsorbed fraction). The excess SWCNTs were recovered for the next round of overloading selective adsorption. After a wash with 3% SDS solution, the multicolumn was separated into its individual columns. The adsorbed SWCNTs were eluted with 1% SC (ultra-pure ≥99.0%, Sigma-Aldrich) solution, producing fractions from Col.1 to Col.5. Six rounds of the overloading process using the unadsorbed fraction were repeated, and fractions from Col.1 to Col.30 were obtained (Supplementary Fig. 1). Then, the second separation utilizing a stepwise elution with a mixed surfactant was conducted. The same amount of 1% SDS solution was added to the fractions (Col.1, 6, 11, 16 or 26). The resulting SWCNTs in 0.5% SC+0.5% SDS solution were injected into a column filled with 5.6 ml of the gel equilibrated with 0.5% SC+0.5% SDS solution, followed by washing with the same solution. The SWCNTs adsorbed onto the gel were eluted by a stepwise increase in the concentration of DOC (purity ≥96.0%, Wako Pure Chemical Industries, Osaka, Japan) in the 0.5% SC+0.5% SDS solution; the concentration of DOC was successively increased from 0.01 to 0.07% with 0.01 or 0.005% steps (Supplementary Fig. 2). Separation processes were performed at room temperature (23±0.5 °C). The detailed methods and results of the separation under different SDS concentrations in the first separation and of the separation of larger diameter enantiomers are given in the Supplementary Methods, Figs 3 and 4.

Optical measurements

Optical absorption spectra were measured from 1,400 to 200 nm in 2 nm increments using an ultraviolet–visible near-infrared spectrophotometer (UV-3600, Shimadzu, Kyoto, Japan). Photoluminescence spectra were measured using a spectrofluorometer (Nanolog, HORIBA, Kyoto, Japan) equipped with a liquid nitrogen-cooled InGaAs near-infrared array detector. The excitation wavelength was varied from 400 to 800 nm in 5 nm steps, and the emission wavelength was varied from 791 to 1304, nm in 1 nm increments. The spectral slit widths were 7 nm for both the excitations and emissions. All raw intensities were corrected with the intensities of the lamp spectrum. The CD spectra of the sorted single-chirality enantiomers were measured from 900 to 200 nm in 1 nm increments using a CD spectropolarimeter (J-820, JASCO, Tokyo, Japan).

Date availability

The data that support the findings of this study are available from the corresponding author on request.

Additional Information

How to cite this article: Wei, X. et al. Experimental determination of excitonic band structures of single-walled carbon nanotubes using circular dichroism spectra. Nat. Commun. 7, 12899 doi: 10.1038/ncomms12899 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-12, Supplementary Table 1, Supplementary Methods and Supplementary References