Carbon dioxide mediates Mn(II)-catalyzed decomposition of hydrogen peroxide and peroxidation reactions

Abstract

Mn(II) can catalyze the decomposition of H₂O₂ and, in the presence of H₂O₂, can catalyze the oxidation of NADH. Strikingly, these processes depend on the simultaneous presence of both CO₂ and 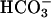. This explains the exponential dependence of the rates on [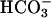], previously noted by other workers. These processes are inhibited by Mn-superoxide dismutase, establishing the generation of 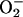and its role as an essential reactant. A scheme of reactions, consistent with the known properties of this system, is proposed. The large rate enhancements provided by 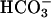+ CO₂, and the abundance of both of these species in vivo, suggest that similar reactions have relevance to the oxidative stress imposed by 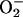and H₂O₂.

The Cu,Zn superoxide dismutase (SOD1) catalyzes the oxidation of a variety of substrates by H₂O₂ (1–8). This peroxidative activity was thought to depend on 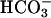(2–7), but was shown to actually require CO₂ (8). Carbonate radical (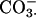) is proposed to be the strong oxidant that is responsible for the peroxidations observed (3–8). 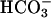-dependent peroxidations and H₂O₂ decomposition, catalyzed by Mn(II) and Fe(II), have been reported (9–17). The possibility that, in these reactions, CO₂ rather than 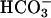might be required, needs to be considered. One puzzling aspect of the Mn(II) + 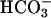-catalyzed peroxidations and H₂O₂ decompositions was the exponential dependence of rates on [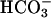] within a given range of [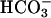]. Thus, Sychev et al. (9, 10) reported dependence of the rate on more than the square of [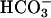], whereas the Stadtman group (13) reported a third-order dependence on [].

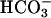In what follows, we demonstrate a dependence on CO₂ and an apparent synergism between CO₂ and 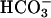, which largely explains the reported exponential responses to [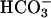]. The abundance of CO₂ and 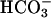in biological systems and their role in the peroxidations catalyzed by SOD1 and metal cations, such as Mn(II), could have relevance to the oxidative stress experienced by aerobic organisms.

Materials and Methods

NaHCO₃, MnCl₂, sodium pyrophosphate, and H₂O₂ were from Mallinckrodt; Tris was from Merck; catalase was from Roche Molecular Biochemicals; human MnSOD was from Biotechnology General (Rehovot, Israel); and NADH was from Sigma. Reaction mixtures usually contained 10 mM H₂O₂, 0.1 mM MnCl₂, and 20 mM NaHCO₃ in 100 mM Tris buffer at pH 7.4 and room temperature (23°C). Reactions were followed spectrophotometrically, and in cases where the addition of reactants entailed sudden changes in absorbance, due either to dilution or the absorbance of the reagent added, such changes were corrected in the data shown. When absorbance changes with time were too rapid to record, they are shown as dashed lines as in Fig. 3. CO₂ was added as ice-cold water saturated with CO₂ gas.

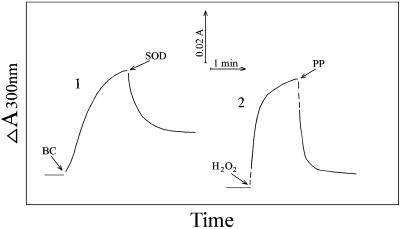
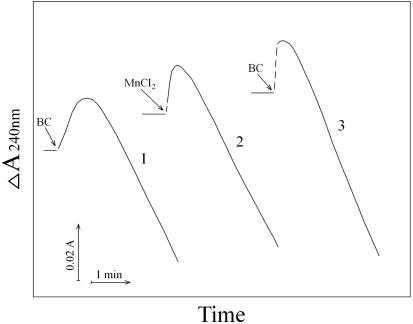
Fig. 3.

Time dependence of absorbance at 300 nm. Reaction conditions were as in Fig. 1. Line 1, 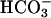(BC) added at the first arrow and MnSOD to 24 μg/ml at the second arrow. Line 2, H₂O₂ added at the first arrow and pyrophosphate (PP) to 0.5 mM at the second arrow.

Results

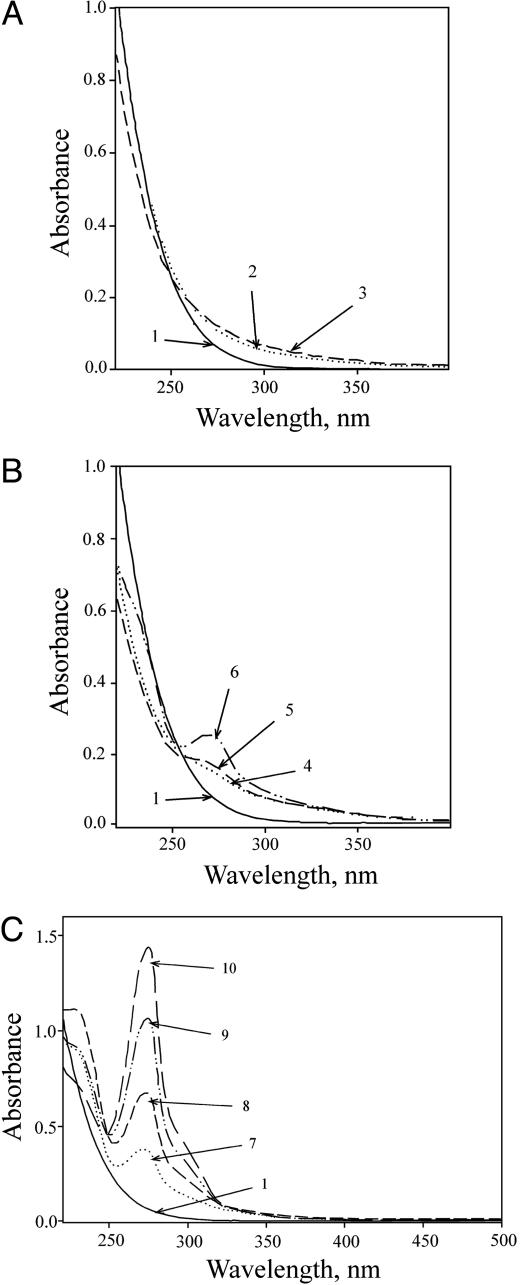
Absorption Spectra. Addition of the third component (H₂O₂ or MnCl₂, or NaHCO₃), to Tris-buffered solutions of the other two, initiated the changes in absorption spectrum shown in Fig. 1. It should be noted that several buffering species were explored, including phosphate, cacodylate, and Hepes, but only Tris supported the changes observed. Fig. 1 A shows that the most rapid (0–3 min) was an increase in absorbance in the range of 250–350 nm and a decrease at wavelengths <250 nm. At longer reaction times (0–20 min), absorbance appeared at ≈270 nm, whereas the absorbance <250 nm first decreased and then increased, as shown in Fig. 1B. At still longer times (Fig. 1C), the band at ≈270 nm became more pronounced, a shoulder developed at ≈300 nm, and absorbance <250 nm decreased.

Fig. 1.

Absorption spectra during the Mn + H₂O₂ + 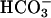reaction. Reactions were started by adding NaHCO₃ to 20 mM to 10 mM H₂O₂, 0.1 mM MnCl₂, and 100 mM Tris chloride at pH 7.4. (A) Spectra taken at 0, 1, and 3 min (lines 1, 2, and 3). (B) Spectra taken at 0, 6, 10, and 20 min (lines 1, 4, 5, and 6). (C) Spectra taken at 0, 30, 50, 80, and 120 min (lines 1, 7, 8, 9, and 10). The zero time spectrum was taken before the addition of .

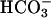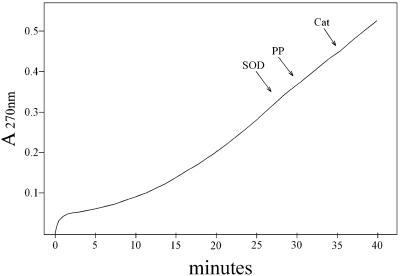
The species responsible for the absorbance centered at 270 nm is some stable product of Tris oxidation, as similar absorbance is seen in Tris buffer that has aged for many months. The kinetics of the absorbance change at 270 nm is shown in Fig. 2. Starting the reaction by adding NaHCO₃ caused a small but rapid increase at 270 nm, which was followed by a slower increase that was not interrupted by late addition of 48 μg/ml MnSOD, 0.5 mM pyrophosphate, or 120 units/ml catalase, any of which inhibited if present at the outset. The initial rapid increase at 270 nm was due to the rapidly formed species absorbing in the 250- to 350-nm region. Evidently, the stable A₂₇₀ species is produced from a preformed precursor and does not depend on the continued presence of H₂O₂, 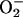, or Mn(III). The latter deduction is based on the abilities of catalase to remove H₂O₂, MnSOD to remove 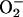, and pyrophosphate to complex and thus trap Mn(III).

Fig. 2.

Time dependence of absorbance at 270 nm. Reaction mixtures were as in Fig. 1, with the reaction started by the addition of 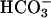. At the arrows, MnSOD to 48 μg/ml, pyrophosphate (PP) to 0.5 mM, or catalase (Cat) to 120 units/ml was added.

The time course of the broad band followed at ≈300 nm is illustrated in Fig. 3. Addition of NaHCO₃ to the buffered solution of Mn(II) + H₂O₂ caused a rapid increase in absorbance that approached a plateau. This plateau evidently represented a dynamic steady state, as adding MnSOD (Fig. 3, line 1) or pyrophosphate (Fig. 3, line 2) caused a swift decline. The species followed at 300 nm could not have been Mn(III), because H₂O₂ was seen to instantly bleach the absorbance of Mn(III) acetate. These results indicate that both 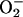and higher valent states of Mn(II) are needed for the formation of the A₃₀₀ species. The rate of appearance of the A₃₀₀ species was slower when NaHCO₃ was the last component added than it was when H₂O₂ was added last (Fig. 3). This could be explained by the relative slowness of the formation of CO₂ after the addition of the alkaline NaHCO₃ to the reaction mixture buffered at pH 7.4. This view is supported by the fact that carbonic anhydrase at 50 μg/ml, when present in the reaction mixture, sharply increased the rate seen when NaHCO₃ was added last and made it indistinguishable from line 2 in Fig. 3.

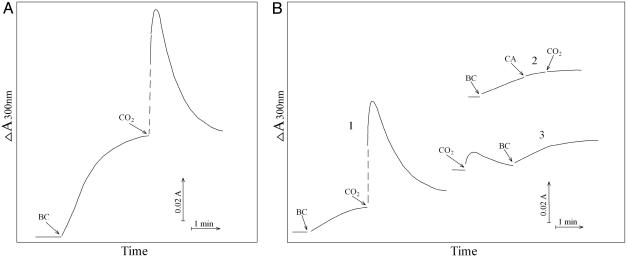
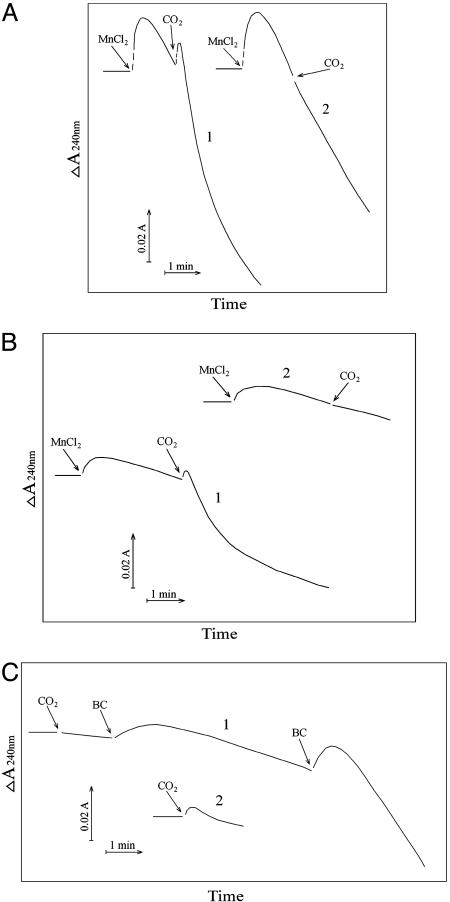
CO₂ Is a Reactant. The addition of NaHCO₃ initiated a rapid increase in A₃₀₀ that approached a plateau within 3 min, as seen in Fig. 3. When CO₂ was added, it caused a marked increase followed by a decline to the plateau that would have been reached in the absence of the addition of CO₂, as seen in Fig. 4A. MnSOD (24 μg/ml) or pyrophosphate (0.5 mM) present at the outset prevented the increase in A₃₀₀ (data not shown). The effect of added CO₂ was even more striking when the amount of 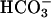used to start the reaction was halved. Thus, as shown in Fig. 4B, the increase in A₃₀₀ was decreased by ≈5-fold when [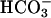] was halved, in agreement with an exponential dependence of rate on [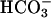]. Subsequent addition of CO₂ again caused an abrupt but temporary increase in A₃₀₀ (Fig. 4B, line 1). The presence of carbonic anhydrase eliminated the effect of added CO₂ (Fig. 4B, line 2). When CO₂ was added before, rather than after, 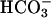, a very small and transient increase in A₃₀₀ was seen, and the subsequent addition of 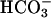gave the modest and limiting increase in A₃₀₀ that would have been observed in the absence of the CO₂ addition (Fig. 4B, line 3). Evidently, CO₂ greatly increases A₃₀₀ only when 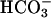is also present. Clearly, CO₂ and 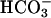both are needed to support the reaction and behave synergistically.

Fig. 4.

Effect of CO₂ on absorbance at 300 nm. (A) Conditions were as in Fig. 1. Reaction was started by addition of 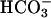at the first arrow and 0.15 ml of ice water saturated with CO₂ added to the total volume of 3.0 ml at the second arrow. (B) Conditions were as in Fig. 1 except that 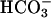was added to 10 mM. Line 1, 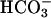added at the first arrow. Line 2, carbonic anhydrase (CA) was added to 50 μg/ml at the second arrow and CO₂ added at the third arrow. Line 3, CO₂ added at the first arrow and 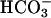added at the second arrow.

The apparent difference between the effects of MnSOD and pyrophosphate, when added after the reaction was underway, as compared to their effects when added at the outset, is easily explained. Thus, there is overlap between the spectra of the A₂₇₀ and A₃₀₀ species. Thus, by the time the MnSOD or pyrophosphate had been added (Fig. 3), some of the stable A₂₇₀ product had accumulated, and its absorbance at 300 nm prevented the complete ablation of the A₃₀₀. In contrast, when MnSOD or pyrophosphate was present at the outset, they prevented production of both the A₃₀₀ and A₂₇₀ species.

It thus appears that the rapidly forming A₃₀₀ species is needed for production of the A₂₇₀ species.

Consumption of H₂O₂. Absorbance at 240 nm reflects both H₂O₂ and the rapidly forming A₃₀₀ species. Hence, the reaction between Mn(II), H₂O₂, 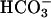, and CO₂ produces a transient increase at 240 nm followed by a linear decrease (Fig. 5). Starting the reaction by adding NaHCO₃ last gave a slower increase in A₂₄₀ than did adding MnCl₂ last (Fig. 5, compare lines 1 and 2). This is explained by the slowness of the uncatalyzed dehydration of H₂CO₃ to CO₂, as shown by the effect of carbonic anhydrase (Fig. 5, line 3). The linear decrease in A₂₄₀ that followed the transient increase reflects consumption of H₂O₂, which was ≈0.5 mM/min, of H₂O₂, and it was not influenced by carbonic anhydrase (Fig. 5, compare lines 1 and 3). Ethanol at 1% did not inhibit, but MnSOD or pyrophosphate did when added after the linear rate had been achieved, after a lag of 5–10 s (data not shown).

Fig. 5.

Kinetics of absorbance changes at 240 nm. Conditions were as in Fig. 1. Line 1, reaction was started by the addition of 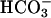. Line 2, reaction was started by the addition of Mn(II). Line 3, carbonic anhydrase was present at 50 μg/ml, and reaction was started by the addition of .

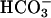Addition of CO₂ increased the consumption of H₂O₂ as illustrated by line 1 in Fig. 6A. Prior addition of carbonic anhydrase eliminated this effect of CO₂, as shown by Fig. 6A, line 2. The effect of CO₂ was even more striking when the concentration of 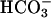was decreased from 20 to 10 mM. Thus, as shown in Fig. 6B, the rate of H₂O₂ consumption was then much slower, ≈0.1 mM/min, and the effect of added CO₂ was more obvious. The presence of carbonic anhydrase eliminated the effect of added CO₂, as shown by Fig. 6A, line 2. CO₂ was able to enhance the rate of H₂O₂ decomposition only if 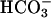was present. Thus, as shown by line 1 in Fig. 6C, addition of CO₂ to the Tris-buffered mixture of Mn(II) plus H₂O₂ was without effect, and subsequent additions of 10 mM 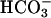gave the rates previously seen at these levels of 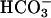in the absence of CO₂ addition. Doubling the amount of CO₂ added, in the absence of 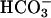(Fig. 6C, line 2), had only a small effect, undoubtedly due to some conversion to 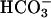. These results again bespeak a synergism between CO₂ and 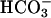, due to the requirement for both to support the decomposition of H₂O₂.

Fig. 6.

Effects of CO₂ on absorbance changes at 240 nm. (A) Conditions were as in Fig. 1. Line 1, reaction was started by addition of Mn(II) at the first arrow, and then CO₂ was added at the second arrow. Line 2 was as in line 1, but carbonic anhydrase was present at 50 μg/ml. (B) Conditions were as in A except that 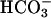was present at 10 mM. (C) Line 1, CO₂ was added to Tris-buffered solution containing 10 mM H₂O₂ and 0.1 mM Mn(II) at the first arrow, and then 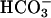to 10 mM was added at the second arrow, and at the third arrow 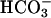was raised to 20 mM. Line 2, 0.3 ml of CO₂ solution was added to the H₂O₂ + Mn(II) reaction mixture at the arrow.

Oxidation of NADH. Previous workers (11, 12, 14, 17) demonstrated that Mn(II) + H₂O₂ + 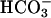could cause the oxidation of diverse substrates, such as amino acids. We chose NADH because it absorbs at 340 nm, where the rapidly formed A₃₀₀ species does not absorb significantly. Moreover, 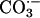, considered a likely oxidant in this reaction system, is known to oxidize NADH with a rate constant of ≈10⁹ M^–1·s^–1 at 25°C (18). It should also be noted that HO• attacks NADH at sites that do not yield NAD⁺, whereas 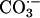does so (18). Line 1 in Fig. 7A shows that addition of 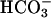to 20 mM to the Tris-buffered mixture of H₂O₂ + Mn(II) initiated oxidation of NADH at a rate of ≈0.02 mM/min after a brief lag. Halving the amount of 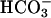added decreased the rate by 5.3-fold, and subsequent addition of CO₂ greatly increased the rate (Fig. 7A, line 2). MnSOD inhibited the oxidation of NADH, and this inhibition was not overcome by subsequent addition of CO₂ (Fig. 7A, line 3). The lags seen when 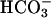was used to start the reaction were not as evident when Mn(II) or H₂O₂ was the last component added, or when carbonic anhydrase was present at the time 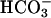was added (Fig. 7B). It follows that the lags seen in Fig. 7A were due to the time required for conversion of some of the 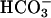to CO₂. Ethanol added to 1% did not inhibit NADH oxidation (Fig. 7B, line 1), whereas MnSOD did so (Fig. 7B, line 2). Thus, HO• is not an intermediate in this process, but 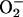is. In full accord with the deductions so far, adding CO₂ after 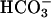caused a marked increase in the rate of NADH oxidation (Fig. 7C, line 1), and this effect of CO₂ was eliminated if carbonic anhydrase was present (Fig. 7C, line 2). Addition of CO₂ in the absence of 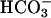transiently increased the rate due undoubtedly to the generation of some 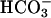, the second component needed.

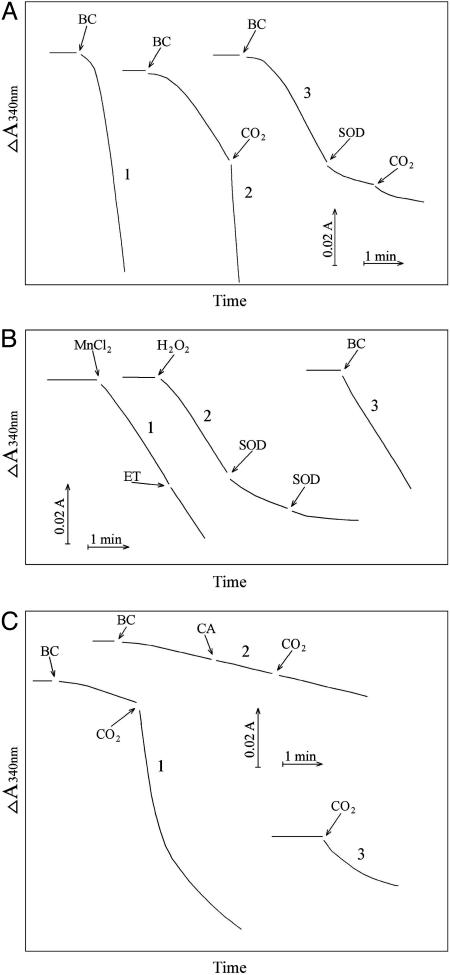
Fig. 7.

Oxidation of NADH. NADH was present at 0.1 mM, and oxidation was followed at 340 nm. (A) Line 1, conditions were as in Fig. 1 except as noted below, and 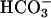was added to 20 mM at the arrow. Line 2, 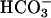was added to 10 mM at the first arrow, and 0.15 ml of CO₂ solution was added at the second arrow. Line 3, 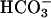was added to 10 mM at the first arrow, MnSOD was added to 30 μg/ml at the second arrow, and 0.15 ml of CO₂ solution was added at the third arrow. (B) Mn(II) was added at 0.1 mM, H₂O₂ was added at 10 mM, and 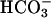was added at 10 mM. Line 1, Mn(II) was added at the first arrow, and ethanol to 1% was added at the second arrow. Line 2, reaction was started with H₂O₂ at the first arrow, and then MnSOD was added to 6 μg/ml at the second arrow and to 18 μg/ml at the third arrow. Line 3, as in lines 2 and 3in A but carbonic anhydrase was present at 50 μg/ml. (C) Mn(II) was added at 0.1 mM, H₂O₂ at 10 mM, and 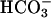, when present, at 5 mM. Line 1, 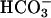was added at the first arrow, and 0.15 ml of CO₂ solution was added at the second arrow. Line 2, 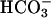was added at the first arrow, carbonic anhydrase to 50 μg/ml was added at the second arrow, and 0.15 ml of CO₂ solution was added at the third arrow. Line 3, 0.15 ml of CO₂ solution was added at the arrow, but 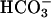was not present.

Discussion

One aspect of the toxicity of 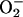is thought to involve the univalent oxidation of the [4Fe–4S] clusters of dehydrases, such as aconitase. This destabilizes the clusters causing release of Fe(II), and that released iron can then generate potent oxidants by reacting with hydroperoxides, in what is called Fenton chemistry (19). Iron is not the only metal cation that can participate in Fenton chemistry. Thus, Mn(II), Co(II), Cr(II), and Cu(II) can do likewise, and 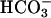or CO₂ has been shown to enhance the oxidations caused by several of these metals in the presence of H₂O₂ (11, 12, 14, 16, 20). In one of these cases, Xiao et al. (20) showed that CO₂ enhanced the luminescence of luminol caused by Co(II) + H₂O₂. H₂O₂ decomposition and oxidations, catalyzed by Mn(II) + H₂O₂, in the presence of 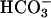, have been studied by a number of workers, such as Sychev et al. (9–12) and the Stadtman group (13–15); the exponential dependence of rate on [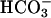] has been noted, and mechanisms have been proposed.

Our present demonstration that both 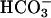and CO₂ are required for this process explains the exponential dependence on [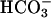], as elevating [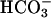] also raises the [CO₂] in equilibrium with it. Any valid mechanism must explain the dual requirement for 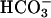and CO₂ and also the involvement of 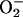. Moreover, analogy with the Cu,ZnSOD + H₂O₂ system indicates that 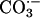is a likely participant, whereas HO• is not (1, 3–8). The role of 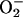could not be the reduction of Mn(III) to Mn(II), as suggested by the Stadtman group (13), because H₂O₂ itself rapidly accomplishes this reduction. On the other hand, the oxidation of Mn(II) to Mn(III) is also not likely to be the role of 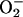, as H₂O₂ would then be produced from the 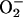, yet rapid H₂O₂ consumption is a hallmark of this process.

The following scheme of reactions is in accord with the known properties of the system. It is proposed in the realization that it is an oversimplification that others may want to elaborate.

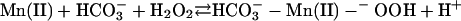[1]

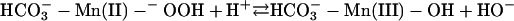[2]

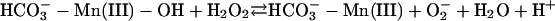[3]

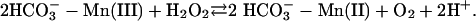[4]

In reactions 1–4, H₂O₂ has been decomposed to H₂O + O₂, and 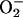has been generated, in what may be viewed as the initiation phase, setting the stage for the chain reaction depicted by the following reactions. In what follows, the manganese may or may not be participating in the form of an 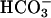complex.

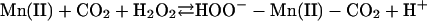[5]

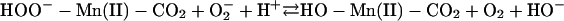[6]

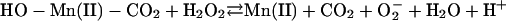[7]

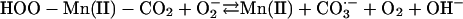[8]

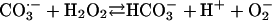[9]

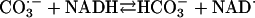[10]

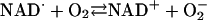[11]

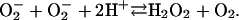[12]

The 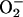generated in reaction 3 is essential for reactions 6 and 8, and it may be viewed as the initiating radical. This is in accord with the observation by Sychev et al. (10) that tetranitromethane, which reacts with 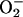at ≈10⁹ M^–1·s^–1, strongly inhibited the decomposition of H₂O₂ and that the simultaneous addition of tetranitromethane and N,N′-dimethyl-p-nitrosoaniline prevented the oxidation of the N,N′-dimethyl-p-nitrosoaniline until all of the tetranitromethane was consumed. In our case, we used MnSOD, which scavenges 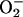as efficiently as does tetranitromethane, but which acts catalytically rather than stoichiometrically. The product of reaction 6 may itself act as the oxidizing species, or carbonate radical, made as in reaction 8, may be the oxidant that can oxidize H₂O₂ (reaction 9) or NADH (reaction 10). Reactions 6 and 7 or 8 and 9 may be alternative or simultaneous pathways. Note the similarity of pathways 6 and 7 as well as 8 and 9 to the classical Haber–Weiss chemistry (21). Because 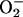is merely acting as a reductant, other reductants in the cell could possibly trigger these potentially damaging processes. Reaction 12 is a termination reaction, and it explains the inhibition by MnSOD. The product of reaction 2 contains trivalent manganese, and it could be the site of inhibition by pyrophosphate. Oxidation of Tris by one or more of the oxidants generated in this scheme would account for the slowly forming and stable species followed at 270 nm. Sychev et al. (9, 10) worked without buffer and maintained pH by titration. Hence, it cannot be the case that Tris is needed for the process under study. We must rather conclude that Tris did not significantly interfere whereas phosphate, Hepes, and cacodylate did so.

The biological significance of Fenton chemistry has been questioned in part because the rate constants for the reactions of reduced metals, and their complexes, with H₂O₂ are not rapid, and their in vivo metal ligands are unknown (22). The present observations of the synergistic rate accelerations by 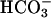+ CO₂ plus the work of Sychev et al. (9–12) and the Stadtman group (13–16) overcome this bottleneck and may well be of importance in the imposition of oxidative stress in biological systems. In this regard, the observation of Stadtman and Berlett (16) that 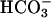greatly enhances the ability of Fe(II) plus H₂O₂ to cause the oxidation of amino acids is also very interesting. The exponential dependence of rates on [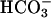] can have two components. Thus, the second-order dependence reported by Sychev et al. (10) is explained by the dual need for 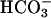and CO₂. To this can be added the effect of 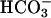in decreasing the amount of free hexaquo Mn(II) available for consumption of 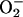by the reaction Mn(II) + 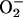+ 2H⁺ ⇄ Mn(III) + H₂O₂. This, together with the need for CO₂ + 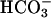, could explain the third-order dependence noted by the Stadtman group (13).