Biconcave shape of human red-blood-cell ghosts relies on density differences between the rim and dimple of the ghost's plasma membrane

Significance

The shape of the human red blood cell (RBC) is known to be a biconcave disc. The experiments in this paper identify some of the underlying determinates. RBC ghosts were made such that they were spheres in hypo-osmotic solutions and biconcave discs in iso-osmotic solutions. The spherical ghosts were centrifuged onto coverslips. When these spheres became biconcave discs by flushing with an iso-osmotic solution, the ghosts laid flat on the coverslip. This indicates that, during centrifugation, the spherical ghosts were oriented by a dense band in their equatorial planes. This unique orientation appears to be the first evidence that differences in the densities between the rim and the dimple regions of RBCs and their ghosts underlie their biconcave shape.

Abstract

The shape of the human red blood cell is known to be a biconcave disk. It is evident from a variety of theoretical work that known physical properties of the membrane, such as its bending energy and elasticity, can explain the red-blood-cell biconcave shape as well as other shapes that red blood cells assume. But these analyses do not provide information on the underlying molecular causes. This paper describes experiments that attempt to identify some of the underlying determinates of cell shape. To this end, red-blood-cell ghosts were made by hypotonic hemolysis and then reconstituted such that they were smooth spheres in hypo-osmotic solutions and smooth biconcave discs in iso-osmotic solutions. The spherical ghosts were centrifuged onto a coated coverslip upon which they adhered. When the attached spheres were changed to biconcave discs by flushing with an iso-osmotic solution, the ghosts were observed to be mainly oriented in a flat alignment on the coverslip. This was interpreted to mean that, during centrifugation, the spherical ghosts were oriented by a dense band in its equatorial plane, parallel to the centrifugal field. This appears to be evidence that the difference in the densities between the rim and the dimple regions of red blood cells and their ghosts may be responsible for their biconcave shape.

This paper is concerned with identifying a possible determinant responsible for the biconcave shape of human red blood cells (RBCs). Although RBCs (“globules”) were first discovered in the latter part of the 17th century (1), it was not until 1827 that they were definitively shown by Hodgkin and Lister (2) to be biconcave discs. Since that time much has been learned about RBC composition (3), in particular identification of its membrane/cytoskeletal (M/CS) elements and structure (4). Our particular interest focuses on whether the symmetry of the M/CS is the same or different between the cell’s dimple and rim regions. If different, such an observation might provide insight into the structural basis for the cell’s biconcave shape. The approach used was to centrifuge hypotonically sphered red-blood-cell ghosts onto a coverslip upon which they adhered. The question was, what was the orientation of the stuck ghosts when they were made into biconcave discs upon exposure to an isotonic solution?

It is important to mention that others have used a quite different approach to understand the basis for the red blood cell’s biconcave shape. These types of studies have used solutions of theoretical models that are mainly based on the red blood cell’s known physical parameters, such as the membrane’s bending energy and elastic properties (e.g., 5–11) in addition to lateral inhomogeneities in the M/CS (12). These analyses have remarkably generated not only the cell’s biconcave shape but also many other types of shapes that the cells are known to assume. Thus, they provide insight into and understanding of the types of forces that characterize and modulate red-blood-cell shape. A known and essential limitation of these models is that the molecular identities and arrangements of the elements responsible for the biconcave shape, which presumably comprise the M/CS complex, are not completely clear. The present work provides an experimental (and beginning) approach to defining a molecular basis for structures responsible for the cell’s biconcave shape.

Results and Discussion

For this study we used resealed human RBC ghosts. The ghosts so prepared were observed to be biconcave discs in isotonic solutions and spheres in hypotonic solutions. This fact justifies the use of ghosts to help access a basis for the red blood cell’s biconcave shape. The procedure used to make ghosts was principally that described by Bodemann and Passow (13) and Lepke and Passow (14). Details of the procedures used are given in Materials and Methods. It was known before (15) by their cation permeability characteristics that the population of ghosts prepared by hypotonic hemolysis resulted in three types of ghosts: type I ghosts were those that spontaneously resealed, type II ghosts were those that could be resealed, and type III ghosts were those that remained leaky. By carrying out hemolysis at pH 6.0 it was shown (14) that the quantity of type II ghosts could be notably increased and therefore were chosen for use. It had been shown by Nakao et al. (16) that ATP helped preserve RBC shape so this was incorporated into the ghosts at the time of hemolysis. It is important to note that hemoglobin (Hb) has been shown to go to diffusion equilibrium at the time of hemolysis (17). Thus, because the RBCs were hemolyzed as one part cells to 40 parts of medium, they resulted in pink ghosts that contained no more than 2% Hb. This was thought to minimize the masking of any density differences of the M/CS complex by intracellular Hb. Although it is not known what the ghosts retain of their original cytoskeletal components, it is clear that they still harbor the constituents responsible for their biconcave shape.

The main reason for using RBC ghosts in this work was to avoid the possible masking by the high concentration of Hb in the cytoplasm of any differences in density that might exist in the M/CS complex between the rim and dimple regions of the intact biconcave cell. To this end, a procedure was devised to test a possible basis for the red blood cell’s biconcave shape. The overall procedure used is presented in Fig. 1. The details are specified in Materials and Methods. Here it is evident that washed intact red blood cells were hemolyzed and then reversed to replenish their intracellular ionic composition and return the medium to isotonicity before resealing the membranes to recover from the injury incurred at hemolysis. The resulting ghosts were split and washed with either hypotonic solution B or isotonic solution A. The ghosts in both solutions were, respectively, observed microscopically to be either smooth spheres or smooth biconcave discs as they tumbled in the fluid microcurrents on a slide. The presence of bovine serum albumin (BSA) protected the cells from undergoing shape changes due to disk-sphere transformation of constant volume (18). The sphered ghosts in solution B were then suspended on the top of a hypotonic solution C that contained sucrose. It was independently observed that the ghosts suspended in solution C retained their smooth spherical shape. The addition of sucrose, in increasing the density of the hypotonic solution C, was to buffer the speed of ghost sedimentation during their centrifugation. The density of solution C was based on the assumption that the density of the ghosts was ∼1–3% of that of intact cells estimated to be 1.097 (18). After temperature equilibration, the ghosts were centrifuged, which resulted in the ghosts adhering to a coverslip coated with poly-d-lysine (PDL). The coverslip together with solution C was then transferred to a shallow dish for microscopic examination. The shape of the ghosts adhered to the coverslip was first observed in hypotonic solution and then after the chamber was flushed with an isotonic solution. The question, as depicted in Fig. 1, was, what was the shape distribution of the biconcave ghosts attached to the coverslip? Was their orientation mainly vertical, random, or flat?

Fig. 1.

A simplified overview of the experimental protocol used in this study. See text and Materials and Methods for details and explanation.

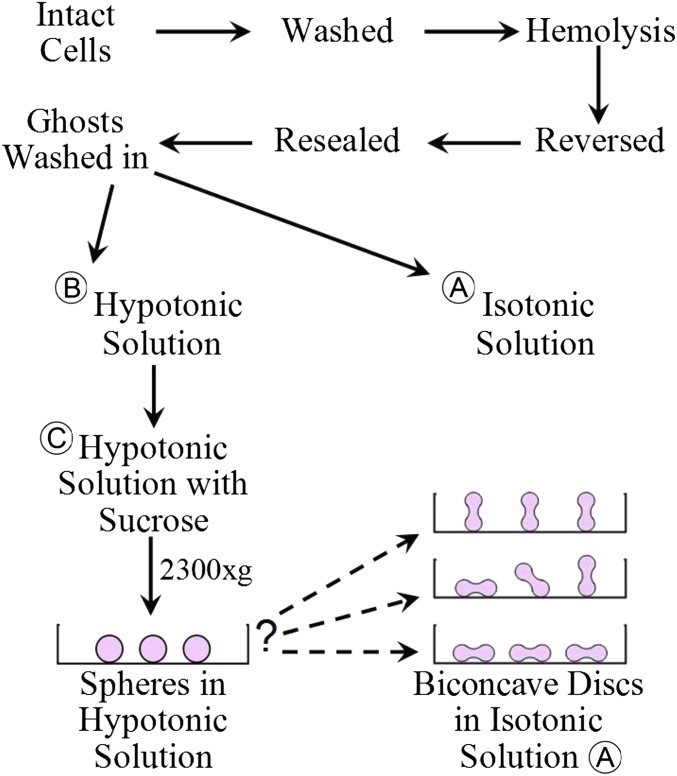
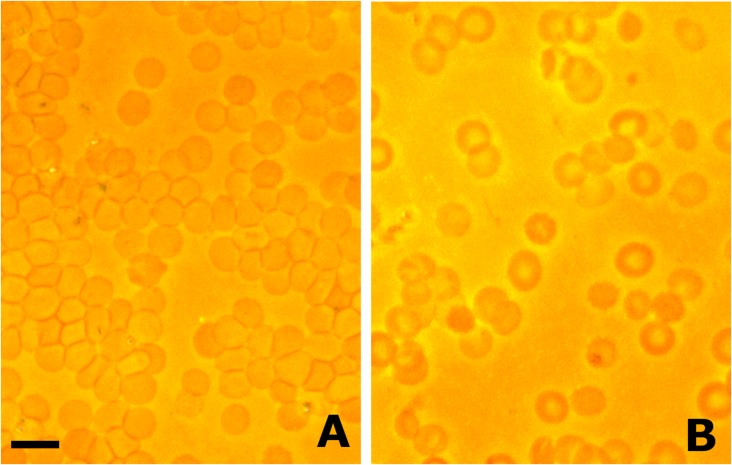
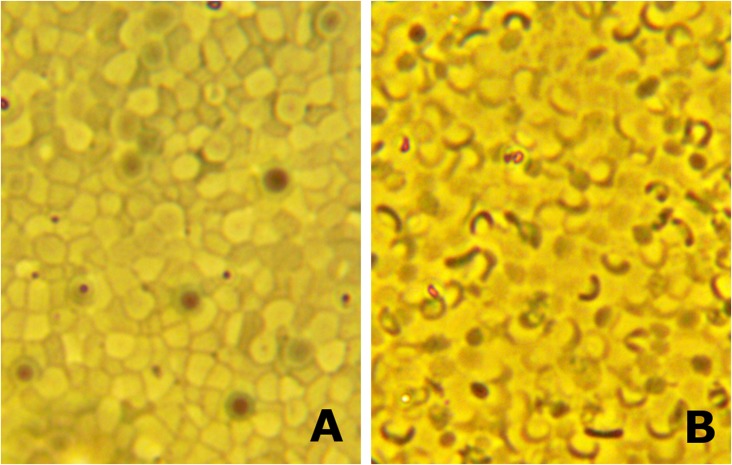
The results are shown in Figs. 2–4, in which Fig. 2 A and B are from the same coverslip in one experiment; Fig. 3 A and B and Fig. 4 A and B are taken from another experiment but from separate coverslips in the same experiment. Figs. 2A, 3A, and 4A all show the distribution and appearance of spherical ghosts in hypotonic media. Figs. 2B, 3B, and 4B all show the change in ghost shape to biconcave discs when the chamber was flushed with isotonic media. For technical reasons it was not possible to show the results of the same field after flushing. Although, as stated before, there was unavoidable heterogeneity of ghost shape in the population, it is clear that upon close scrutiny that the majority of ghosts in each photograph are either smooth spheres in the A-labeled Figs. 2–4 or smooth discs in the B-labeled Figs. 2–4. It is also clear that in each case the resultant transition of the spherical ghosts to biconcave discs is that the latter’s orientation is predominantly flat. It should also be mentioned that the shape changes were reversible in that the flat biconcave ghosts became spheres again when the chamber was flushed with hypotonic media. It is also clear that the clusters of packed spherical ghosts, seen particularly in Figs. 3A and 4A, prevent any rollover before or during exposure to the isotonic solution. On the other hand, because the diameter of the biconcave ghosts is larger than the spheres from which they were derived, there is necessarily a forced rearrangement that occurs. This presumably accounts for parts of some of the biconcave ghosts being out of the plane of focus in Figs. 3B and 4B.

Fig. 2.

The results of one experiment with resealed human red-blood-cell ghosts that have been centrifuged onto a glass coverslip coated with PDL to enhance attachment. The ghosts were first washed and sphered in hypotonic solution B. A portion of the ghosts was then layered onto the top of a slightly more dense solution C and then centrifuged as detailed in the text. The coverslip was then transferred to a small chamber for microscopic observation. (A) The sphered ghosts adhering to the coverslip. (B) The transformation of the shape of the ghosts from spheres to biconcave discs when the chamber was flushed with isotonic solution A. It is important to note that the orientation of the biconcave ghosts on the coverslip was flat. (Scale bar in A, which also applies to B and to Figs. 3 and 4: 10 μm.) See text for discussion.

Fig. 4.

The ghosts as depicted are from the same experiment as those of Fig. 3 A and B, but from a coverslip taken from a paired centrifuge tube. (A) Sphered ghosts in hypotonic solution attached to the coverslip. (B) The transformation of the ghosts to biconcave discs lying flat after flushing the chamber with isotonic solution A. See text for discussion.

Fig. 3.

The ghosts in this figure were from an experiment different from those depicted in Fig. 2. (A and B) Ghosts treated in the same fashion as that described in Fig. 2. (A) Attached spherical ghosts. (B) The transformation of the ghosts to biconcave discs lying flat when the chamber was flushed with isotonic solution A. See text for discussion.

The presumed interpretation of these results is that, during the centrifugation of the spherical ghosts, there was preferential orientation due to a denser band, i.e., the membrane’s rim, around the ghosts’ equatorial plane. The orientation of this more dense band was horizontal to the centrifugal field. This would explain the fact that the orientation of the ghosts was flat when they were transformed into biconcave discs on the coverslip. These results are interpreted to indicate that there is a difference in density of the M/CS complex between the ghost’s rim and dimple areas.

It is, of course, not known what molecular/structural organization of the M/CS complex could account for the difference in density between the rim and the dimple regions of the ghosts. Nor is a theoretical basis known for why the ghosts have any inferred orientation of a dense band horizontal to the centrifugal field. Understanding of these aspects must necessarily remain for future work (as mentioned in ref. 19). It should be mentioned that previous measurements found no difference between the “stiffness” of the dimple versus the rim regions of intact biconcave human red blood cells (20). These measurements were made by sucking a portion of the membrane into a glass pipette. It is, of course, not known if the glass interaction with the membrane alters the latter’s properties (e.g., 21, p.150); nor is it known what the relationship is between membrane stiffness and density.

It is reasonable to assume that the density differences between the rim and dimple regions of red-blood-cell ghosts exist in the intact biconcave red blood cell. That the intact cell membrane displays a type of memory or hysteresis has been indicated in previous studies and is perhaps consistent with the differences in density. Ponder (18) describes observations in which a particle attached to the dimple region of a cell returns to its same position after repeated cycles of disk/sphere shape changes induced by alterations in the osmotic pressure of the medium. Others have shown in studies on the “tank-treading” of intact biconcave red blood cells that different types of membrane memory exist. Tank-treading is a phenomenon in which the cell’s membrane has been shown to circulate around the cell in shear flow with the cell remaining a biconcave disk (22). Fischer (23) has observed that a particle attached to a known region of the membrane returns to its identical location with tank-treading. He then found that, if the tank-treading was stopped before the particle reached its original position, it would in time return to that position. In a different type of study in which the cells were distorted under varying conditions during tank-treading, they returned to their original shapes when the shearing forces were halted (24). Another type of memory is perhaps associated with a study by Paul LaCelle that has been quoted before (in ref. 25). LaCelle reported that, when a biconcave disk drawn into a cigar shape in a pipette was then split (without hemolysis) into two fragments, each fragment formed a biconcave disk upon extrusion from the pipette. These types of observations may reflect differences in the density of the cell’s M/CS complex in terms of its molecular makeup or architecture. Future work will be necessary to discern the structural elements of the M/CS complex responsible for the density differences between the dimple and the rim regions. These differences may be able to be resolved by selective labeling of the M/CS complex with imaging by superresolution microscopy.

Materials and Methods

Normal human blood was taken (as approved by Yale's Human Investigation Committee) from an anticubical vein, heparinized, and suspended in an isotonic solution [310 milliosmoles (mOsm), iced at 4 °C] that contained 150 mM NaCl, 20 mM Tris–Cl, and 0.3% BSA (pH 7.2 at 23 °C). After centrifugation, the cells were washed twice (with white cells removed after the second wash) by centrifugation at 4 °C in a HB-4 Sorval horizontal rotor for 5 min at 10,000 × g. The cells were diluted to an ∼50% hematocrit and put at −1.0 °C for use as described below.

In the preparation of red-blood-cell ghosts, all vessels that were used in this work were first washed with 2 M nitric acid to minimize contamination with traces of detergent, and all solutions used were filtered through 0.22μ Millex GV filters to reduce contamination in the pictures of the centrifuged ghosts.

After testing various procedures that we previously used for the preparation of ghosts, we settled on those described by Bodemann and Passow (13) and Lepke and Passow (14). These methods, with modifications, provided ghosts the shapes of which were best preserved and could be manipulated as desired as described in the text. Thus, 1 mL of red blood cells was hemolyzed with 20 parts of solution at −1.0 °C at pH 6.0. The magnetically stirred hemolysis solution contained 2 mM sodium phosphate, 2 mM Na₂ATP, 4 mM MgSO₄, and 0.2 mM EDTA with pH adjustment made with acetic acid. After 3–5 min, the stirred hemolysis solution at −1.0 °C was brought to pH 7.2 with 2 M Tris⋅Base and immediately reversed, again at −1.0 °C, with 1.1 mL of solution that contained 0.3 M NaCl + 2.7 M KCl. The ghosts were then resealed (26) by incubation, with gentle shaking, of the hemolysis solution for 40 min at 37 °C. Reversal and its attendant resealing is known to result in a repletion of the ghost cation content with a marked reduction in ghost leakiness. This suspension was then centrifuged in a Sorval horizontal rotor at 13,000 × g for 5 min. The ghosts were washed twice with solution A, with the button removed after the first wash. One-half of the ghosts were again washed with solution A; the other half was washed with hypotonic solution B, which contained 75 mM NaCl, 20 mM Tris–Cl, and 0.3% BSA (170 mOsm).

Ghosts washed in iced (4 °C) hypotonic solution B were diluted with the same solution such that various numbers of ghosts in the different experiments were pipetted onto the top of the 3 mL of iced solution C contained in Beckmann polycarbonate thin-walled centrifuge tubes (13 mm × 52 mm). These tubes each had a flat sylgard gel button (∼5 mm) on the bottom onto which was placed a piece of a PDL-coated coverslip (Corning BioCoat). Solution C was a modified solution B such that sucrose was added to make the density of the solution equal to 1.003 with the NaCl content reduced to compensate for the sucrose addition to maintain the same osmolality. The centrifuge tubes were loaded into a preice-cooled SW-55 Ti Beckman (horizontal) rotor with the rotor put in the centrifuge at 4 °C for 60 min for temperature equilibration. The ghosts were then centrifuged for 1 h at 5,000 × g (2,300 × g) in a LE-80K Beckman ultracentrifuge. The PDL-coated coverslips were then removed and put into a shallow dish (Delta-T), the bottom of which was a 0.17-mm coverslip. They were kept in the same solution C or flushed with solution B or with solution A.

By using a 40× objective and a 10× or 20× eyepiece, darkfield microscopic observations were made of the ghost shapes attached to the PDL-coated coverslips. Ghosts in solution B or C were generally spherical-shaped whereas ghosts in solution A were mostly biconcave discs lying flat on the coverslip as described. In no case were the ghosts observed to be crenated, spread, or disfigured after attachment to the PDL substrate. Fig. 1 presents the general protocol used. Photographs were taken with an OMax 3.2 MP digital USB microscope eyepiece camera connected to a Mac-Pro computer for monitoring and storage. The photographs of the ghosts used in the figures were slightly modified in brightness and in contrast to make the results clearer; original color is artificial.