Accuracy of eye position for saccades and smooth pursuit

Abstract

In this study, we address the question of whether a target is foveated during smooth pursuit. Specifically, we examine whether smooth pursuit eye movements land near the center-of-mass of the target, as is the case for saccades. To that end, we instructed eight untrained, healthy participants to follow moving targets, presented monocularly in a scanning laser ophthalmoscope. Stimuli moved either in a modified step-ramp (smooth pursuit), or made a single step (saccade), stepping 6° from the center. Targets were ring-shaped and either 0.6° or 1.7° in diameter. In an additional set of experiments, two participants collected more extensive data on smooth pursuit and saccades for a larger range of target sizes (0.6°, 1.7°, or 4.3°). During pursuit, eyes were rarely placed at target center, even when participants' fixational stability was taken into account. Furthermore, there was a clear tendency for distance from target center to increase with target size. This outcome was in contrast to saccades, where there was no effect of target size across participants. The difference in foveal placement between the two types of eye movements is consistent with their different purposes: closer inspection of the target for saccades versus maintenance of the target in the visual field for smooth pursuit.

Introduction

There is continuing debate as to whether smooth pursuit relies on the foveation of the moving target. On the one hand, smooth pursuit is thought to be driven by a velocity signal, and to be largely insensitive to position (Rashbass, 1961). Furthermore, smooth pursuit can occur in the periphery (Shanidze, Fusco, Potapchuk, Heinen, & Verghese, 2016; Winterson & Steinman, 1978), albeit with lower gains. On the other hand, smooth pursuit has long been viewed as a complement to saccades to help primates keep objects on the fovea (for reviews, see de Xivry & Lefevre, 2007; Krauzlis, 2004). Moreover, recent studies have provided evidence that smooth pursuit velocity can be modulated by a position signal (Blohm, Missal, & Lefèvre, 2005), although position error alone generally cannot produce a smooth pursuit response (de Xivry & Lefevre, 2007). The close relationship between saccades and smooth pursuit is borne out by the fact that smooth pursuit has been recorded only in foveate animals.

The foveal nature of smooth pursuit is also interesting in the context of its relationship to saccades. There is a volume of anatomical and physiological evidence that suggests that these two types of eye movements share many of the same or similar pathways (reviewed in Krauzlis, 2004) and that both can be modulated by velocity and position inputs (reviewed in de Xivry & Lefevre, 2007). The purpose of saccades is to direct the fovea towards a stationary object of interest. When the target is large, or made up of a group of objects, previous studies have shown that the fovea is directed towards the center of mass of the target (Coren & Hoenig, 1972; He & Kowler, 1989; Kowler & Blaser, 1995; Melcher & Kowler, 1999). Recent work by Heinen et al. (2016a) suggests that smooth pursuit characteristics differ for small targets that fit within the fovea and larger stimuli that may not require foveation. In their work, the authors find that small spot stimuli elicited frequent catch-up saccades and lower pursuit accelerations than larger objects. These findings suggest a greater reliance on the position signal for small targets, but the question of the exact foveation strategy still remains. Additionally, the authors did not examine changes in eye position with target size—an important aspect of our study.

Here, we test the hypothesis that discrete targets are foveated during smooth pursuit and that the fovea is placed at, or close to, the center of mass of the target, analogous to the tendency reported previously for saccades. We tested participants using the step-ramp paradigm (Rashbass, 1961) and the scanning laser ophthalmoscope (SLO), so we could directly visualize the location of the target on the retina. We define foveation as the centering of gaze on the target within the limits of the fixational stability of the individual. All participants were tested using ring-shaped targets of two sizes: small (0.6° diameter) and large (1.7° diameter) to determine the effect of target size on pursuit. Given that all targets were circles with the same center of mass, regardless of size, we predicted that eye position relative to target center would not change between target sizes. Smooth pursuit outcomes were compared with those of analogous saccade experiments. The same target sizes were used and participants were asked to make saccades from a central target to one that appeared at a 6° eccentricity, in one of eight radial directions. To address our hypothesis, we measured the distance of the fovea from the center of the target during smooth pursuit and saccades, and determined how this metric was affected by target size and task. Previous studies have reported considerable across–subject variability in eye placement during saccades (Kowler & Blaser, 1995) and variability in response to a position signal during smooth pursuit (Blohm et al., 2005). To address this potential difficulty, we included a larger number of participants (n = 8) that allowed us to look at a breadth of responses.

Our results suggest that foveation is not necessary for smooth pursuit, with eye position during pursuit falling as far as 3°–4° from the target boundary, even when accounting for fixational jitter. Additionally, unlike saccades where the eyes tend toward the center of mass of the target, the distance of the eye from the center increases with target size in smooth pursuit.

These results are consistent with the distinct purposes of the two types of eye movements. In the case of saccades, the goal is to bring the area of highest visual acuity to the object of interest. Placing the fovea close to the center of mass of the target (He & Kowler, 1989) is consistent with this objective. Furthermore, such placement improves the resolution of nearby objects of interest. Thus, foveating the center of mass is an optimal strategy from an information gathering perspective (Renninger, Verghese, & Coughlan, 2007). Although smooth pursuit can be used to visualize the details of a moving target, its fundamental purpose may be to follow a moving target and to maintain it in the visual field.

Methods

Participants

All research was performed in accordance with the Declaration of Helsinki and was approved by the Institutional Review Board of The Smith-Kettlewell Eye Research Institute. We recruited eight untrained participants (age: 25–35, three males) with normal vision. One participant (P7) did not return for the saccade portion of the study. Participants P1 and P3 were authors NS and SG, respectively.

Equipment

For all eye movement experiments, participants were tested in a confocal SLO (SLO 101, Rodenstock, Munich, Germany). Each participant was typically tested in two sessions: smooth pursuit and saccades. Participants were seated with their chins and foreheads resting in the SLO head support, without glasses (with SLO correction). The field size used was 40°, at a resolution of 17.7 pixels/°. Participants were tested monocularly (with their dominant eye), with the nontested eye occluded.

Fixation

Fixation stability data were measured monocularly with an Optos OCT/SLO, with a patch over the nonviewing eye. Participants viewed a white cross, subtending 2° × 2° visual angle, on a black background. Data were collected for 10 s in a dark room (Crossland & Rubin, 2002). Horizontal and vertical eye position data were saved for offline analysis. To estimate fixation stability, we calculated the bivariate contour ellipse area (BCEA) using an in-house algorithm (Ghahghaei & Walker, 2016) that incorporates Steinman's (1965) method for estimating the variability of eye position on a given trial (Steinman, 1965). The algorithm calculates the area, the length of the major and minor axes, and the angle of the ellipse with respect to the horizontal.

Smooth pursuit

All participants were tested with their dominant eye for all trials. Participants were instructed to “follow the moving ring as closely as possible for the whole trial.” Each trial started with the fixation cross in the center of the screen. Once the experimenter determined that fixation was acquired (eye position became stable), participants were asked “Are you ready?,” and the trial was initiated after an affirmative response. The fixation cross was then replaced by a ring, indicating the start of the trial to the participants. Two ring sizes were used (0.6° and 1.7°, Figure 1). The same ring size was used within a block of trials, with the direction of motion of the stimulus selected randomly on each trial. The order of small versus large target trials was determined at random for each participant.

Figure 1.

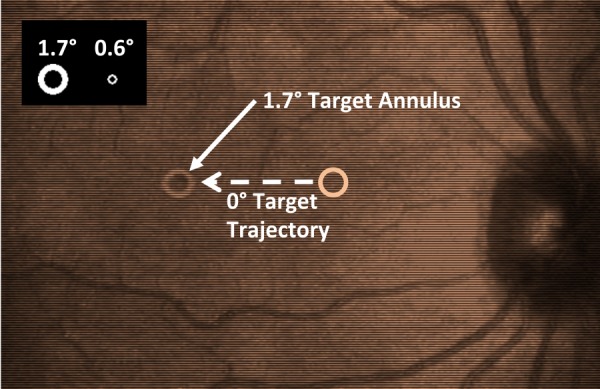
Stimulus and recording apparatus. SLO image of a participant's retina (optic disc included for reference) with the 1.7° target visible at the fovea. Dashed line represents an example target trajectory (0°) for smooth pursuit. Inset: Targets as they appear at the large (1.7°) and small (0.6°) size: The annulus width relative to target size remained constant as the overall target size was changed.

All stimuli were shown at 100% contrast. Participants fixated the ring target for 1 s. The target then jumped 6° in one of eight possible directions (0°, 45°, 90°, 135°, 180°, 225°, 270°, or 315°) and then moved at a constant velocity (between 5°/s and 6 °/s) back through fixation in a “step-ramp” fashion (Rashbass, 1961). The trials were approximately 3 s in duration, and the targets traversed approximately 12° of visual angle. The test was repeated for each of the eight directions at least once. Data was sampled at 60 frames/s and recorded in video and text formats for further analysis (Shanidze et al., 2016).

Saccades

In the second session, participants fixated a central target and were asked to make a saccade to the target that was displaced at the 1-s mark, using the same set of instructions as for smooth pursuit. The target was displaced in the same manner as for the pursuit trials (6° displacements in one of eight radial directions) and remained visible at the new location for 1 s, after which the screen was blanked until the appearance of the fixation cross signaling the next trial.

Target size and shape effects

Participants P1 and P3 participated in additional data collection sessions for saccade and smooth pursuit trials. For these sessions, only the two horizontal directions (0°, 180°) and three ring target sizes (0.6°, 1.7°, 4.3°) were used. For all experiments target sizes were defined as: small (0.6°), large (1.7°) and largest (4.3°). An additional set of blocks was collected with a 1.7°, solid disk stimulus. Each participant completed an average of 40 trials for each target size, type, and condition (smooth pursuit versus saccades). Target directions were randomized (between left and right) for each trial in the smooth pursuit and saccade blocks.

Data analysis

Target and eye positions were analyzed using the same method as described in Shanidze et al. (2016). Briefly, to provide a reference for eye position, a retinal feature, such as the intersection of two blood vessels, was chosen in the first available video frame of each trial, and its position was tracked throughout the trial (MacKeben & Gofen, 2007). Foveal location was determined using the initial fixation period and was confirmed visually by the appearance of a dark patch, when available. The position difference between the fixation locus and the retinal feature above was then used throughout the trial to determine foveal position.

For smooth pursuit trials, horizontal and vertical eye positions were filtered (two-pole Butterworth filter with a 25 Hz cutoff), differentiated, and geometrically combined to derive eye velocity. Saccades were detected when eye velocity exceeded 40°/s, or its variance exceeded 150 (°/s)². All velocity traces were visually inspected for additional saccades. Saccades were excised only for the purpose of calculating smooth velocity, but were left in the records for the eye position analysis. Excised saccades were replaced with a line that interpolated eye velocity before and after the saccade (e.g., Heinen, Badler, & Ting, 2005).

For saccade and smooth pursuit trials, we computed BCEA metrics (center coordinates, area, major and minor axes, and angle) for the first 30 frames of the fixation period, the last 30 frames of the second fixation period (for saccade trials), and for the longest continuous period of smooth pursuit (during the longest continuous period of velocity within ±0.2 of the median velocity after pursuit onset). Values were computed using the algorithm described previously, using a 68% confidence interval.

During fixation, we defined the distance between eye and target as the distance between the center of the fixation locus (BCEA) and the center of the target. The fovea was considered to be on the center of mass of the target if the target center overlapped with the fixation region, given each participant's fixational stability (BCEA). Fixation was considered to be on any part of the target if the eye position fell within the BCEA of the target perimeter (Figure 3, inset). Effectively, the on-target region now included the ellipse defined by the sum of target radius and the axes of the 68% BCEA. For smooth pursuit, distances were computed on a frame-by-frame basis and also as an average of all frames during continuous pursuit. For saccades, a postsaccade fixation area was computed using the same BCEA analysis as already mentioned, and its center was used to compare with the target center location. These metrics were used for all comparisons—across/between subjects, target sizes, and tasks.

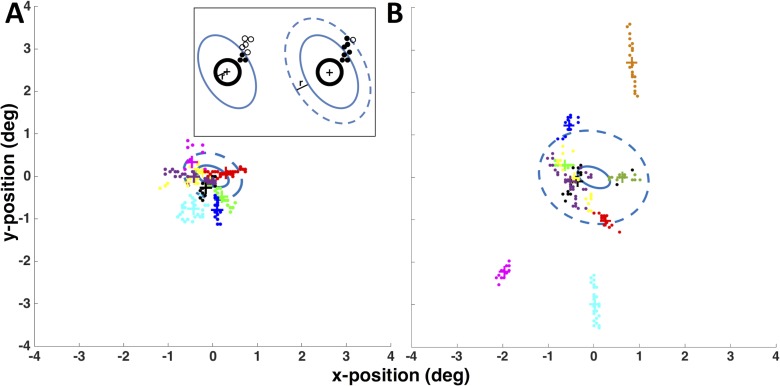
Figure 3.

Eye position for individual smooth pursuit trials relative to the center of a small, 0.6° (A) or big, 1.7° (B) target for participant P7. Solid blue ovals mark fixation +68% BCEAs calculated over 10 s of fixation and placed at target center for reference. Dashed ovals mark the region within the 68% BCEA of the target perimeter. Dot cluster of each color represents an individual smooth pursuit trial, and each dot is a single frame from that trial. Target is centered at (0, 0). Crosses are per-trial average eye positions. Inset: Criteria for eye–position location. Blue, solid oval marks the BCEA around the target center (+). Solid dots represent eye positions on the target center, (that fall within the BCEA), whereas open circles are outside the target center, even when fixation stability (BCEA) is taken into account. The dashed oval marks the locus of fixations within BCEA of the target perimeter and includes eye positions on any part of the target (see relative increase in the number of solid dots).

Results

Experiment 1: Smooth pursuit

Participants exhibited smooth pursuit gains that were within normal range (mean = 0.90 ± 0.22) but showed some variation from trial to trial and participant to participant. Figure 2 illustrates a typical smooth pursuit trial.

Figure 2.

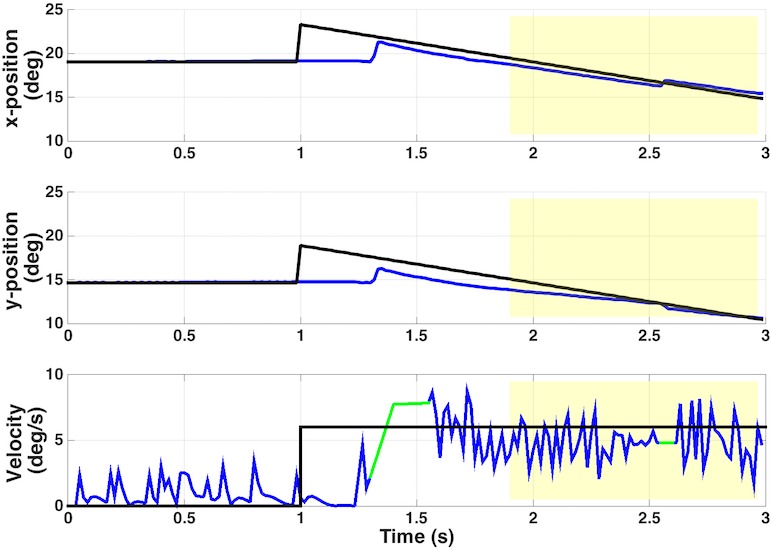
Typical smooth pursuit trial, participant P1. Top two panels illustrate target (black) and eye (blue) horizontal and vertical position for a 0.6° target moving in a 135° trajectory. Yellow shading represents the period of continuous velocity used to calculate gain for this trial. Bottom panel illustrates target and eye velocity for the same trial. Green intrusions are regions where a saccade was removed and velocity interpolated.

First, we looked at the position of the fovea (the center of the loci of fixations as defined by the BCEA) relative to the center of mass of the target during pursuit, across all participants (Figure 3).

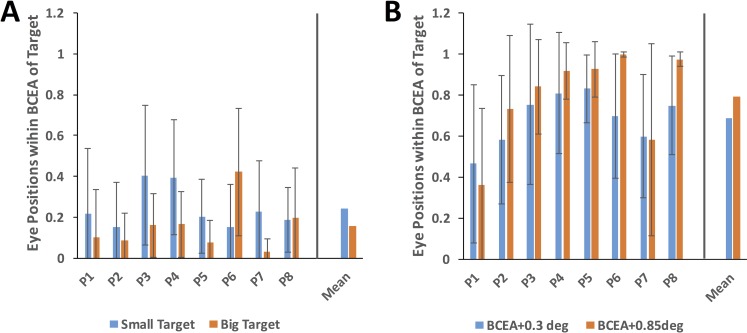
For each participant and each trial, we calculated the ratio of frames that the fovea (eye position defined by the BCEA) landed on the target center ([0,0] in Figure 3) for small (0.6°) and large (1.7°) targets. Across participants, the fixational BCEA rarely fell on the target center, for either small (ratio: 0.24 ± 0.10) or large (ratio: 0.16 ± 0.12) targets (Figure 4A). However, when we increased the area of interest to include the entire target (dashed oval in Figure 3), instances where the BCEA fell within this target area were substantially more common, as shown in Figure 4B (small: 0.69 ± 0.13; large: 0.79 ± 0.22, comparison of ratios on target center or on any part of the target: ANOVA with Bonferroni correction for multiple comparisons, F(3, 28) = 35.63, p < 0.0001). To ensure that these results were not an artifact of the fixation data being collected in a different SLO over a longer time interval, we also used the pretrial fixation periods to estimate BCEA parameters in the Rodenstock SLO. We found the 10-s BCEAs measured in the Optos OCT/SLO to be significantly larger than the majority of the prepursuit fixations for 0.6° and 1.7° targets (ANOVA adjusted for multiple comparison of group means, F(2, 21) = 29.29, p < 0.0001), indicating that the eye positions within BCEA of the target were counted appropriately. Furthermore, additional analysis using the prepursuit fixation BCEA showed very similar results to Figure 4 (see Supplementary Materials, Figure S1).

Figure 4.

Proportion of eye positions on (A) target center (within BCEA of center) and (B) on any part of target (within the BCEA of target perimeter) during smooth pursuit. Blue bars are data for small (0.6°) target trials, and orange bars are data for large (1.7°) target trials. Error bars are one standard deviation of the mean. Group means are shown as the rightmost bars for each plot.

Because pursuit gains showed some variation across trials, we considered the possibility that retinal slip could account for the participants' lack of foveation on some trials. To test this hypothesis, we analyzed only those trials where eye velocities were within 15% of target velocity and repeated the analysis above. The results did not change significantly (small: 0.25 ± 0.14 and 0.73 ± 0.19; large: 0.18 ± 0.15 and 0.81 ± 0.26 for eye position on target center and on any part of the target, respectively). To further confirm that the trend was not due to a possible increase in BCEA size during pursuit, we also analyzed the average eye position during continuous smooth pursuit for each trial, relative to the center of the target, and computed the ratio of trials where mean eye position fell within the BCEA relative to target center and within the BCEA of target perimeter, on average (small: 0.29 ± 0.23 and 0.77 ± 0.14; large: 0.51 ± 0.27 and 0.76 ± 0.28). Statistically, when comparing the three approaches (all trials, gains close to 1, and average eye position) there was no difference for either target size, with or without including target radius, except the average eye position for the large target was different from the other two approaches (ANOVA adjusted for multiple comparison of group means, F(2, 20) = 8.19, p = 0.0025).

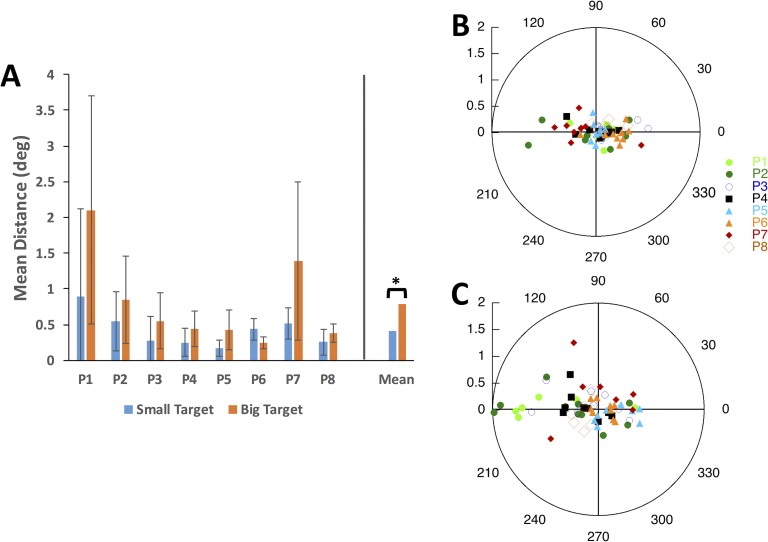
Two observations from the data provide evidence against a centering strategy of the eye on target. First, the eye was often placed on some noncentral portion of the target (compare Figures 4A vs. 4B: blue bars for 0.6° targets and orange bars for 1.7° targets) and second, there were a number of frames where eye position relative to target center was outside of the BCEA, even with target size taken into account (as far as 3°–4° from BCEA boundary, e.g., participant P7 in Figure 3B). Summarized another way, when we looked at the average eye position placement relative to the center of the target, participants tended to place their eyes further away from center for the large target than for the small (mean eye distance to center: 0.795 and 0.417 for 1.7° and 0.6° targets, respectively, paired-sample t test: t(7) = 2.403, p = 0.047, Figure 5). At an individual level, all but one participant (P6) showed an increase in eye distance to center with increased target size. Looking at the difference in eye–target placement, the absolute (Euclidean) distance of the mean eye position from the center, was significantly different for all participants and target sizes, except for one (participant P1, small target, t(8) = 2.186, p = 0.06, Figure 5A). We also examined if there was a preferred placement location of the eye relative to target across trials for a given participant, or across the entire group. We rotated all trials so that the target was at the origin moving leftwards and found a broad distribution of eye placements for both target sizes (Figure 5B, C). In particular, there is no systematic trend for the eyes to lag the target (to be on the right of the target). However, eye position is further from target center for the larger target (Figure 5C).

Figure 5.

Mean eye distance relative to the center of the target during smooth pursuit (A). Asterisk indicates a significant difference in eye–target distance for small (0.6°) and large (1.7°) targets (p = 0.047). Error bars are one standard deviation of the mean. Group means are the rightmost set of bars. Distribution of average eye positions relative to the target (crosses in Figure 3) during smooth pursuit for 0.6° (B) and 1.7° (C) targets. For all trials, eye positions have been rotated such that they lie along the horizontal axis, with target center at the origin, and the direction of motion leftward.

Experiment 2: Saccades

A sample of a saccade trial is illustrated in Figure S2 in Supplementary Materials. Fixation stability was measured at the initial and post-saccade positions for both target sizes using the BCEA approach described above (30 central fixation and 30 postsaccade eccentric fixation frames were used to compute the respective BCEAs). For all participants, fixation stability was reduced for the 6° eccentric, postsaccade targets (Wilcoxon signed-rank test, Z = −2.366, p = 0.018 for small and large targets).

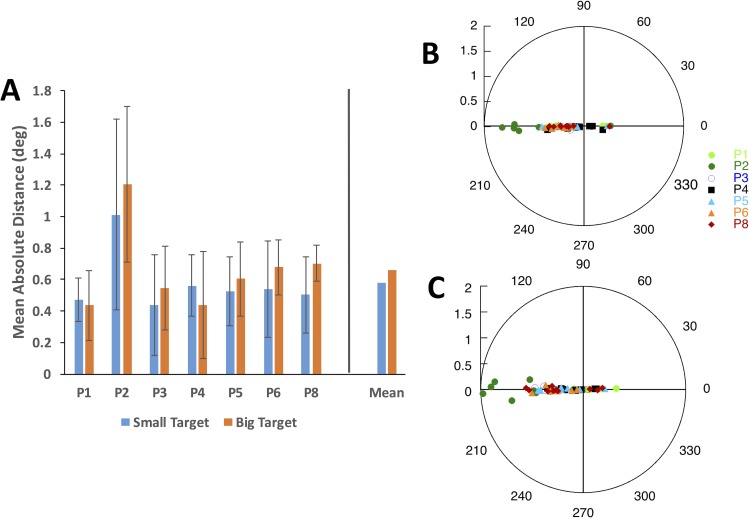
To confirm previous findings that eyes tend toward the center of mass of the target after a saccade, we compared BCEAs for large versus small targets at central fixation and postsaccade eccentric locations. Consistent with previous observations, BCEA values were not significantly different for 1.7° versus 0.6° targets at either viewing location (Wilcoxon signed-rank test, central: Z = −1.183, p = 0.237; eccentric: Z = −0.524, p = 0.600). Although this measure addresses the effects of target size on fixational stability, it does not directly address the eye centering characteristics. Therefore, we also compared the target center and BCEA center locations at the eccentric location. Unlike for the smooth pursuit case, average eye position distance from the center did not increase for larger target size (Table 1, Wilcoxon signed-rank test, Z = −1.521, p = 0.128; Figure 6).

Table 1.

Mean BCEAs for central and eccentric gaze fixations (units: deg² of visual angle).

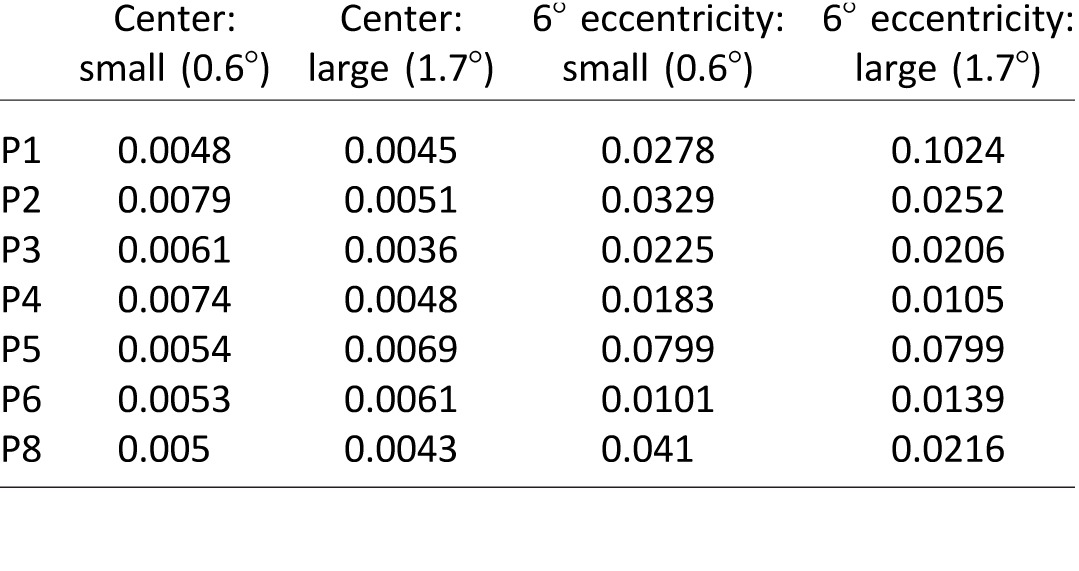
Figure 6.

Mean eye distance relative to the center of the target after a 6° saccade (A). Error bars are one standard deviation of the mean. Group means are the rightmost set of bars. Distribution of postsaccade eye positions relative to the target for 0.6° (B) and 1.7° (C) targets. All target and eye positions have been rotated to lie on the horizontal axis, with target center at the origin. Negative eye positions relative to the target indicate hypometric saccades.

To determine whether eye position was placed on the center of the target, we looked at the eye positions relative to the target in the direction of target motion (saccade). First, we examined the absolute distance between mean eye position and target center across trials, for each participant. There was a significant offset from the zero distance for all participants and target sizes (one-sample t test, p < 0.05; see Supplementary Materials, Table S1 for individual values). To determine whether the offset was in a particular direction relative to the target, all targets and eye positions were rotated to lie on the horizontal axis with the saccade target at the origin (Figure 6B, C). On the whole, saccades were hypometric but there was no significant difference between eye placements for 1.7° versus 0.6° targets (paired t test, t(6) = 1.07, p = 0.323).

Experiment 3: Target shape

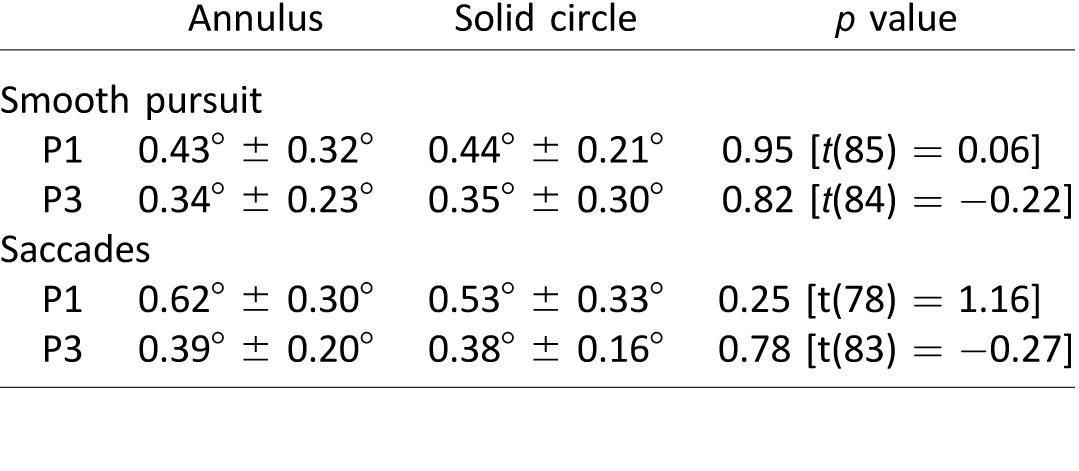
To test whether the annular shape of the target was responsible for the results reported previously, we repeated the smooth pursuit and saccade experiments in two participants with a 1.7° solid disk. Specifically, we wanted to determine whether the higher fraction of eye positions on the edge of the annular target was because participants were tracking a visible part of the annulus, rather than its virtual center. Due to trial-to-trial variability we observed in the data for many of the participants, we collected 40 saccade and 40 smooth pursuit trials per participant for the annular and solid targets, using a target that was randomly displaced 6° left or right and either stayed at the location (saccades) or moved in the opposite direction along the horizontal axis (smooth pursuit). We calculated eye–target distance as described for Figures 5 and 6A for smooth pursuit and saccades, respectively. For both participants (P1 & P3), we found no difference in eye placement relative to the target center between the two target shapes, for smooth pursuit or saccades (Table 2, p values are calculated using a two-sample t test). These results suggest that the presentation of a solid versus annular target shape does not alter the distribution of eye positions around the target.

Table 2.

Mean eye–target distances for 1.7° annular and solid targets.

Experiment 4: Relationship of eye position and target size

Our data from Experiments 1 and 2 suggest that target size affects eye placement during smooth pursuit, but not saccades. However, a previous systematic study of saccade accuracy showed a strong relationship between eye placement and target size for one of their two participants (Kowler & Blaser, 1995). In that study, the authors used a range of sizes for the saccade target, from a point stimulus to a 4° target that could appear either on the left or the right. To determine whether the pattern of results we obtained in Experiments 1 and 2 would hold for a larger range of target sizes, we repeated the saccade and smooth pursuit experiments using three target sizes (0.6°, 1.7°, and 4.3°) for participants P1 and P3. Akin to Kowler and Blaser's original experiment, we used a larger number of trials per condition (∼40 per target size and eye movement condition) and a reduced number of target directions (0° and 180°). Target directions were randomized within blocks. Across all target sizes, we did not see a difference in absolute eye–target distance between target directions for either participant for saccades (two-way ANOVA, P1: F(1, 108) = 0.096, p = 0.758; P3: F(1, 128) = 0.345, p = 0.558). For smooth pursuit, target direction did not affect relative eye-target placement for P3 (two-way ANOVA, F(1, 123) = 2.535, p = 0.114). However, mean eye–target distance was greater for the 180° than 0° direction for P1 (F(1, 126) = 7.799, p = 0.006). We plot the leftward and rightward data separately in the Supplementary Materials (Figure S3), but show the combined data across rightward and leftward trials in Figure 7.

Figure 7.

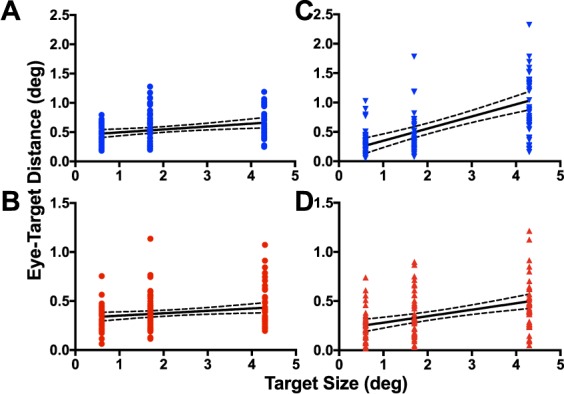
Distribution of eye–target distances for participants P1 (A & C) and P3 (B & D) for saccade (A & B) and smooth pursuit (C & D) trials. Each symbol represents an individual trial. Solid black lines are the linear regression fit. Dashed lines indicate the corresponding 95% confidence intervals for the regression.

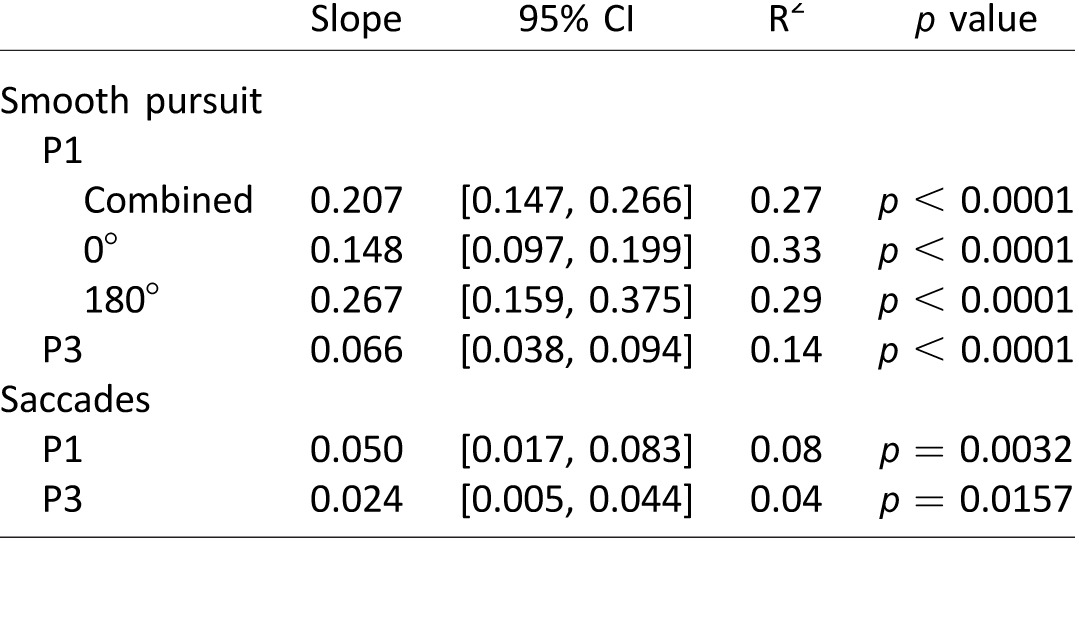
To determine the effect of size on eye placement relative to target we regressed eye−target distance versus target size for both smooth pursuit and saccades. For smooth pursuit, there was a strong relationship between eye distance from target center and target size. For saccades, the relationship was present, but substantially weaker (Table 3). We repeated the analysis for P1's smooth pursuit trials, separating the two target directions and found a significant positive slope for both directions (Table 3). There was no significant interaction between target size and target direction for these data (two-way ANOVA, F(2, 126) = 1.099, p = 0.336).

Table 3.

Linear regression fit parameters for eye–target distance as a function of target size.

Discussion

Foveation during smooth pursuit

This paper examines foveation during smooth pursuit and static pre- and postsaccade viewing in untrained observers. Participants' smooth pursuit gains were somewhat variable, but within normal range, consistent with previous results for naïve participants (Collewijn & Tamminga, 1984). Our results suggest that unlike for saccades, the fovea is not centered on the target during smooth pursuit. Previous studies have shown that peripheral smooth pursuit is possible (Winterson & Steinman, 1978) and may benefit from larger targets, meant to stimulate peripheral retina (Heinen, Potapchuk, & Watamaniuk, 2016a). The current study addresses the complementary question of whether foveation necessarily occurs during pursuit. The results suggest that the fovea might not track the center of the target, as is illustrated in Figure 3 (e.g., turquoise trial in Figure 3A or turquoise, magenta, orange, and blue trials in Figure 3B). Interestingly, these trials are not an indication of worse pursuit gain.

On a large number of pursuit trials, participants did foveate a portion of the target; however, eyes were rarely placed on the center of the target, with foveation of a target edge being much more common (24% vs. 69% of the analyzed pursuit time for the 0.6° and 16% vs. 29% for the 1.7° targets). Our data also show an increase in the average distance of eye position from target center for bigger (1.7°), as compared with small targets. This trend is not consistent with the center-of-mass tendency that has previously been reported for saccades (He & Kowler, 1989), and the invariance of saccadic eye position with target size in our experiment. When we repeated the pursuit experiment with two of the participants using a larger number of trials per condition and three target sizes, we confirmed a significant increase in the distance of eye placements relative to target center with increasing target size (Figure 7C, D). Our results for pursuit are consistent with previous work that argues against a center-of-mass strategy for smooth pursuit when a static target is flashed in the vicinity of a pursuit target (Blohm et al., 2005).

The variation between participants in their eye placement strategies is interesting to note, with some virtually never tracking the target center and others more frequently foveating the center (e.g., compare P2 and P3, small target in Figure 4B). Although all subjects had little to no prior experience with smooth pursuit tracking, other experiences may have played a role in this variability. For example, when we examined eye placement strategies in terms of average eye position, participants who had extensive video game or psychophysical experience (P3, P4, P5, and P8) seemed to exhibit, on average, a more central eye placement strategy for big targets. This result may be related to previous findings that suggest a wider attentional visual field and, therefore, reduced need for gaze shifting (reviewed in Spence & Feng, 2010) and improvement in saccade accuracy (West, Al-Aidroos, & Pratt, 2013) by experienced video game players.

Foveation during saccades

To compare this result to the center-of mass strategy previously reported for saccades, we modified the experiment so that observers made a single saccade to an eccentric target. Consistent with previous literature, we found similar eye placement relative to target center for large (1.7°) and small (0.6°) targets. When we examined the target size—eye placement relationship with three target sizes, we did find a small, but significant, increase in distance of eye placement from target, across the three sizes (Figure 7A, B). Although this outcome is similar to that reported for one of the participants in the Kowler and Blaser (1995) study, the size effect in that work was most evident for largest targets, whereas in our data, the greatest increase in eye–target distance occurs between the smallest (0.6°) and the intermediate (1.7°) target. It is important to note that Kowler and Blaser also showed variable effects of target size on eye placement between their two participants—with one having a clear increase in eye position offset with increasing target size, and the other only showing the effect for largest presented target (4°). Figure 6 demonstrates a similar variation between participants in our data, although to a lesser extent.

Eye placement accuracy

Eye placement accuracy may decrease simply due to the eccentricity of the target in the visual field. Examining the distribution of eye placements on the saccade target (BCEA), we found that fixation stability decreased for the 6° eccentric target, but did not differ for small (0.6°) and large (1.7°) targets. This finding is consistent with previous results that showed a decrease in fixation stability with increasing ocular eccentricity (Dang, Walker Renninger, & Fletcher, 2010; Stahl, 2001). Our results confirm that although BCEA may increase when viewing eccentric targets, its placement is consistent with a centering strategy for saccades.

Our findings provide another interesting insight. When we looked at the average distance of eye relative to target center, we found that for both saccades and smooth pursuit there was an offset in the absolute distance between eye position and target center. The saccade results are consistent with the findings of Kowler and Blaser (1995) that showed that saccade landing positions can be offset for both large and small targets. In that study, experiments were performed on two participants. Saccades were accurate, overshot, or undershot the target, depending on target size, the observer, and on the side (left, right) the target was presented. The authors in that study tested target eccentricities between 3.8° and 4.2°, smaller than those in our study. Previous studies with larger target eccentricities (5°) tended to have greater undershoots (e.g., Kapoula & Robinson, 1986). Participants in our study had saccades that were hypometric on average by 6% and 7% for small (0.6°) and large (1.7°) targets, respectively. Kowler and Blaser tested targets over a range of sizes from point targets to 4°, compared with our target sizes of 0.6° and 1.7° in Experiments 1 and 2. Their larger target sizes showed a tendency for saccade landing positions to be further from target center as target size increased, with the smaller target sizes showing a smaller dependence on target size. Our results across seven participants for saccades to small and large targets (0.6° and 1.7°, respectively) indicate that the eye position offset does not change regardless of target size (Figure 6). However, when we repeated the experiment with a target that matched the largest target size (4°) used in Kowler and Blaser (with targets on the left and right and a larger number of trials per direction) we did find a small, but significant increase in eye position offset across target sizes for the two participants tested. These results suggest that the dependence on target size for saccades is rather subtle and may be seen best for targets stepping horizontally.

Smooth pursuit versus saccades

Our finding for saccades is in contrast to our results for smooth pursuit, where mean eye distance to the target increased with target size across seven out of eight participants in Experiments 1 and 2 (Figure 5), and for the two participants tested with a larger range of sizes in Experiment 4. The data suggests that unlike saccades, eye placement in smooth pursuit is not simply a consistent offset, but is related to target size. This noncentering tendency is also consistent with a lack of foveation of the pursuit target on some of the trials.

These findings may reflect the everyday purpose of the two eye movement types. In the case of saccades, eye movements are initiated to bring the region of highest visual acuity onto a feature of interest in the environment for localization or identification. In the case of smooth pursuit, the main goal is to maintain an object in the visual field of the observer (Blohm et al., 2005; de Xivry & Lefevre, 2007), while the specific task at hand and the nature of the target, may or may not require the high acuity of the fovea. Given a specific task, the two types of eye movement can be highly synergistic with each other, consistent with the tightly linked underlying physiology subserving the two (de Xivry & Lefevre, 2007; Orban de Xivry, Bennett, Lefèvre, & Barnes, 2006). However, when the task does not require identification of a specific feature, foveation is likely unnecessary. Catch-up saccades may occur in cases where distinct features of interest need to be identified (Heinen et al., 2016a), if there is an attentional component (Heinen, Potapchuk, & Watamaniuk, 2016b), or if retinal slip causes the object to disappear from the observer's visual field. Furthermore, catch-up saccades may be required during pursuit of fast-moving or unpredictable targets, where the smooth pursuit system is unable to adjust eye velocity such that position error accumulates (de Xivry & Lefevre, 2007).

Limitations and future directions

There are several potential limitations to our data. First, we show that fixational stability decreases in postsaccadic eccentric viewing. One could argue that this outcome may explain the distribution of viewing locations away from the target center during smooth pursuit. However, there are several factors that make this explanation unlikely. First, for our smooth pursuit analysis, we use the standard 10-s fixational stability measurement, which yields BCEA values that are, on average, more than 10 times greater than those collected over the duration of each portion of the movement experiment; and are therefore a generous estimate of the dispersion of fixation for each participant. Second, due to smooth pursuit latency considerations, the eye position data during pursuit is rarely collected at the most eccentric, initial position of the target, with average stop and start times being 40%–70% into the ramp portion of the trial, respectively; a range that includes the time when the target crosses the center of the screen. It is important to note that these values did not vary between small (0.6°) and large (1.7°) targets, since there was no difference in smooth pursuit latency between target sizes (Small target: mean latency = 0.53 ± 0.36; Large target: mean latency = 0.52 ± 0.37). The variation in target position and frames used for analysis, therefore, make any effects of pure eccentric viewing unlikely.

Smooth pursuit of a spot stimulus is known to elicit catch-up saccades (e.g., Heinen et al., 2016a). However, saccades were very rare in our experiment, likely due to the short trial duration and low velocity of the target (although still within natural range, e.g., Carl & Gellman, 1987). The velocity and trajectory length were limited by the properties of the SLO and future experiments with a larger screen size and higher velocities could help further elucidate these results.

It is possible that participants' eye placement accuracy relative to the target center may have improved with a more predictable target motion, additional practice on the task, or longer stimulus duration. However, having only two directions and increasing the number of trials in each direction did not improve eye placement accuracy for pursuit for P1 and P3 in Experiment 3. Both participants continued to show a systematic offset from the target center that increased with target size. Furthermore, the lack of practice and predictability did not lead to a similar offset from target center for saccades.

For Experiments 1 and 2 we did not use a pure spot stimulus—instead, participants were asked to follow an annulus. To confirm that this choice of stimulus did not contribute to the participants' tendency to follow a noncentral portion of the target, we repeated our experiment with a solid disk of the same size as the annulus target (1.7°, Experiment 3). For both participants, we did not see a difference between target types in eye position offset for either smooth pursuit or saccade trials, suggesting that the off-center eye placement strategy we observe during smooth pursuit is independent of whether the target is an annulus or a solid disk.

Finally, a limitation of the SLO is that all viewing was done monocularly, with the dominant eye, and the other eye patched. It may be argued, for example, that fusional vergence movements could have introduced error into the eye positions reported here. However, in our view, two aspects of the data make this possibility unlikely. First, eye positions for pursuit tended to be evenly distributed around the center of the target. A fusional vergence signal would have likely introduced a nasal bias to the eye position, which was not evident for any of the participants (e.g., see Figure 3). Second, we expect the deviation due to any fusional influence to be similar regardless of target size, which is contrary to our findings of an increase in eye position spread between large and small targets (compare Figure 3A with Figure 3B).

Conclusion

The present study provides the first systematic comparison of eye placement strategies during smooth pursuit and saccades. The data suggest that foveation is not a given during smooth pursuit, even for single spot stimuli, as previously thought, and that eye placement relative to the target center differs significantly between the two tasks.