Quantifying Myocardial Contractility Changes Using Ultrasound Based Shear Wave Elastography

Abstract

BACKGROUND

Myocardial contractility, a significant determinant of cardiac function, is valuable for diagnosis and evaluation of treatment in cardiovascular disorders including heart failure. Shear Wave Elasticity Imaging (SWEI) is a newly developed ultrasound-based elastography technique that can directly assess the stiffness of cardiac tissue. The aim of the study was to verify the ability of technique to quantify contractility changes in the myocardium.

METHODS

In 12 isolated rabbit hearts we made SWEI measurements of systolic stiffness at 5 different coronary perfusion pressures from 0 to 92 mmHg. The changes in coronary perfusion were used to induce acute stepwise reversible changes in cardiac contractility via the Gregg effect. The Gregg effect is the dependency of contractility on coronary perfusion. In 4 of the hearts, we repeated the measurements after delivery of Gadolinium, which is known to block the Gregg effect.

RESULTS

Systolic stiffness measured by SWEI changed linearly with coronary perfusion pressure with a slope of 0.27 kPa/mmHg (mean of 95% CI, R²=0.73). As expected the change in contractility due to the Gregg effect was blocked by Gadolinium with a significant reduction of the slope to 0.08 kPa/mmHg.

CONCLUSION

SWEI measurements of systolic stiffness provide an index of contractility in the unloaded isolated rabbit heart. While this study was done under ideal imaging conditions and with non-physiological loading conditions, it reinforces the concept that this ultrasound technique has the potential to provide a direct and noninvasive index of cardiac contractility.

INTRODUCTION

Myocardial contractility or the inotropic state of the heart is one of the major indicators of cardiac function during systole. In many cardiovascular disorders such as systolic heart failure or cardiomyopathies, the contractility of the heart decreases and inotropic agents are used to raise the ejection force. Contractility is usually measured by methods such as dP/dt or by evaluating ejection fraction. On rare occasions, it is measured invasively using Pressure-Volume (PV) loop analysis. Recently, echocardiography-based tissue Doppler, tissue strain and strain rate imaging have been used clinically to assess contractility; however, these techniques do not provide a contractility measurement of the myocardium independent of factors such as intra-ventricular volume or cardiac motion ^1–3. It has been shown that transverse stiffness, measured using small high frequency indentations, is an index of contractility in beating canine inter-ventricular septum ⁴. However, none of the available clinical techniques measure systolic stiffness this directly.

Elastography is a newly developed image-based stiffness measurement technique that involves exciting the tissue mechanically and measuring the response. Imaging of the response has been done by both magnetic resonance and ultrasound imaging ^5–7. To use the ultrasound-based technique, an acoustic pulse is used to push the tissue and subsequent acoustic pulses are used to measure the mechanical response. As the tissue moves axially, a shear wave is launched in the transverse direction. The velocity of this wave is related to the stiffness modulus (shear modulus) of the tissue. This method is called Shear Wave Elasticity Imaging (SWEI). The technique is being used clinically to diagnose liver disorders by directly measuring liver elastance ⁷.

It has been shown that SWEI stiffness of the heart can be measured with intra-cardiac probes invasively ⁸. The acute change in tissue stiffness associated with cardiac ablation lesions has been characterized using this technique ⁹. Recently, the feasibility of transthoracic cardiac SWEI measurements in humans have been used to characterize diastolic stiffness ¹⁰. However, there are few papers that describe SWEI measurements to characterize inotropic changes in cardiac tissue. Kolipaka et al. assessed the effect of epinephrine on myocardial contractility using MR-based shear wave stiffness measurements ¹¹. A study by Pernot et al. showed that SWEI can be used as a contractility index in the isolated rat heart with a constant volume balloon in the left ventricle. They demonstrated that the SWEI measurement was relatively independent of preload volume when tested with a constant volume ventricle and that the SWEI determined modulus of elasticity, max dP/dt and peak systolic pressure changed in a dose dependent manner after administration of an inotropic agent (Isoproterenol) ¹².

In this study, we used the “Gregg Effect” phenomenon in a Langendorff perfused rabbit heart model to investigate the potential of SWEI to quantify changes in cardiac contractility in the absence of pre-load and after-load. Researchers have shown that coronary perfusion pressure affects myocardial contractility during systole; this is known as the “Gregg effect”^13,14. It has been shown that the circumferential stress caused by increased perfusion pressure causes a mechanical deformation in the membrane of cardiomyocytes. This deformation changes ion flux through stretch activated channels (SACs) resulting in increased Ca delivery to the myocytes and increased contractility ¹⁵. Further details about this effect can be found in the second and third paragraph of the discussion section. This effect can be blocked by Gadolinium, a stretch-activated ion channel blocker ¹⁵. The Gregg effect in combination with the control of perfusion afforded by the Langendorff preparation allowed us to induce stepwise and random changes in cardiac contractility. In addition, the contractility changes are acute and reversible unlike with most inotropic agents. The purpose of this study was to produce multiple reversible levels of contractility to verify the ability of SWEI elastography method to quantify the inotropic state of the heart in the unloaded condition.

METHODS

Imaging Setup

Ultrasound-based SWEI measurements were made using a Siemens Sonoline AntaresTM scanner (Siemens Healthcare, Ultrasound Business Unit, Mountain View, CA, USA). Imaging was performed using the VF10–5 linear probe. The acoustic radiation force pulse used to push the tissue was 300 cycles at 5.7 MHz. The focus was set at 16 mm and the tracking Pulse Repetition Frequency (PRF) was 4.3 kHz. Displacement estimation algorithms were used to calculate the shear wave velocity.

The long acoustic pulse produced a radiation force that displaced the tissue in the focal region by transferring momentum from the ultrasound wave to the tissue. This transfer of momentum is also a significant portion of the mechanism of attenuation. The push caused displacements on the order of microns in the direction of ultrasound propagation. The rapid ‘point’ displacement generated a shear wave that propagated perpendicular to the push. The velocity was calculated by tracking the displacements caused by the propagating wave using regular ultrasound at several lateral locations. For further information regarding the signal processing techniques please see the publications by Loupas et al. and Rouze et al. ^16,17. The intrinsic motion of the heart was subtracted using a quadratic motion filter before calculating the shear wave speed of propagation¹⁸. The following formula (assuming a linearly elastic isotropic medium) relates the shear modulus of stiffness to the shear wave velocity, C_t in (m/s):

$$C_t=\sqrt{\frac{\mu }{\rho }}=\sqrt{\frac{E}{2(1+\nu )\rho }}$$ (1)

Where μ (kPa) is shear modulus, E (kPa) is Young’s modulus, ν is Poisson’s ratio, and ρ (Kg/m³) is the density of the tissue. Stiffness measurements were made continuously throughout the cardiac cycle every 29 ms for 1.2 seconds. Acquisitions that included a premature ventricular contraction (PVC) were repeated.

Animal Model

An isolated heart preparation was created using hearts from New Zealand white rabbits (N = 12, Weight = 3.68 ± 0.64 kg). The study was performed according to the Institutional Animal Care and Use Committee (IACUC) at Duke University and conformed to the Guide for the Care and Use of Laboratory Animals. Rabbits were heparinized and anaesthetized by Xylazine and Ketamine IM and IV until the reflexes disappeared. A bilateral thoracotomy was performed and the heart removed and placed in Tyrode’s solution at 0–4°C. The aorta was cannulated and perfused with Tyrode’s solution in Langendorff mode. The Langendorff preparation keeps the heart alive and beating for several hours by retrograde aortic perfusion. The Langendorff apparatus was built using a combination of Radnoti glassware (Radnoti LLC Monrovia, CA, USA) and custom-built parts. Tyrode’s solution was made fresh on the day of the experiment and the concentration of Ca²⁺, Na⁺, K⁺ and pH were maintained in the normal range. P_o2 was maintained at greater than 300 mmHg. The isolated heart was submerged in a saline bath maintained at 37–38 °C.

The coronary arteries in an isolated heart can be perfused in two configurations: Langendorff or Working. In the Langendorff configuration, the aorta is perfused retrogradely and the aortic valve remains closed, the coronary arteries, located above the valve are perfused and the LV does not receive fluid through the patent aortic valve. In the Working configuration, the heart is perfused similarly to a normal beating heart in which the left atrium receives flow and the LV ejects fluid out through the aorta and into the coronary arteries. Our experiments were performed in the Langendorff configuration.

Experimental Design

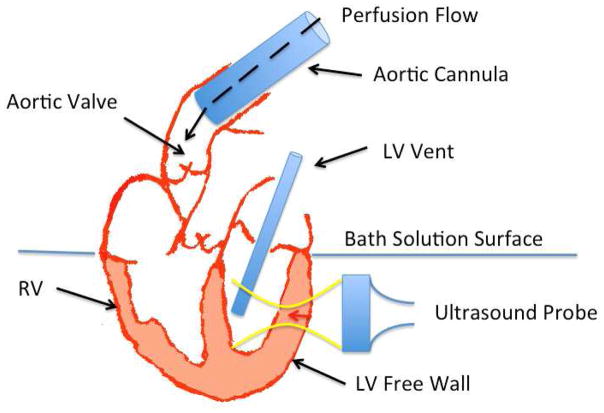
A schematic of the experimental setup is shown in figure 1. The preparation was designed to avoid fluid build up in the LV in order to minimize the load dependency of our measurements. This was done by retrogradely perfusing the aorta, keeping the atrium above the fluid level of the bath as much as possible, and by placing a vent through the mitral valve to keep it open and prevent a pressure build up in the LV. Thus, the preload and afterload in these hearts was near zero.

Figure 1.

The schematic diagram of the experimental setup is shown in this figure. The yellow lines show the focused ultrasound ARFI push and the red arrow shows the location the push pulse on the LV free wall for SWEI measurements. The black dashed arrow inside aorta shows the direction of the perfusate flow in the aorta. The flow keeps the aortic valve closed while perfusing the coronary arteries immediately above the valve. The LV vent keeps the mitral valve open at all times to empty any fluid leaking through the aortic valve.

Short axis images of the LV free wall were taken from a distance of 10 to 15 mm. Shear wave velocity was calculated by tracking the wave as it moved through 5 mm of myocardium laterally. To minimize the effects of anisotropy, displacements were averaged over the wall thickness axially (3–5 mm) before the velocity was calculated. Electrodes were placed in the bath and the ECG recorded using Labchart/Powerlab data acquisition system. The maximum shear wave velocity recorded through the cardiac cycle was identified and its systolic timing was confirmed by simultaneous ECG. Occasionally the algorithm could not determine an accurate shear velocity due to noise in the displacement measurement, in these cases (6 of 96 measurements), the data point following the noisy maximum point during that cardiac cycle was used. The noisy points were identified as points with a shear wave speed greater than 9 m/s or by a tissue displacement profile that did not follow a wave propagation pattern.

The Langendorff configuration can be perfused in one of two modes, constant pressure and constant flow, where perfusion of the aorta and the coronary arteries is achieved by maintaining a constant pressure or a constant flow respectively. In these experiments the hearts were perfused in constant pressure mode set by the height of the perfusion chamber connected to the aorta. The identical random sequence of perfusion steps was used in each heart: [75, 25, 75, 50, 75, 0, 75, 92] mmHg. Coronary perfusion pressure changes were achieved by adjusting the height of the perfusion fluid chamber. After each change, the coronary perfusion pressure was returned to normal (75 mmHg) and the measurements repeated. The normal perfusion pressure for Langendorff rabbit hearts has been reported to be between 60 and 100 mmHg in the literature. We chose 75 mmHg for all the hearts to maintain consistency for the measured perfusion pressure steps between the hearts. The total time required to obtain measurements for the entire sequence of pressures was under 30 minutes. In four of the hearts, after the initial measurements, Gadolinium (GdCl₃.6H₂O) at a concentration of 10 uM was added to the perfusate and the hearts perfused for 30 minutes. The SWEI measurements at each perfusion pressure step were then repeated.

Statistical Analysis

The statistical analysis was performed using R (R Foundation) and Matlab (The MathWorks, Natick, MA, USA) programs. A multivariate linear regression model was used with coronary perfusion pressure steps as an independent continuous variable and rabbit hearts as a categorical variable. Rabbits were treated as a categorical variable to account for between animal differences including imaging site and intrinsic myocardial characteristics.

RESULTS

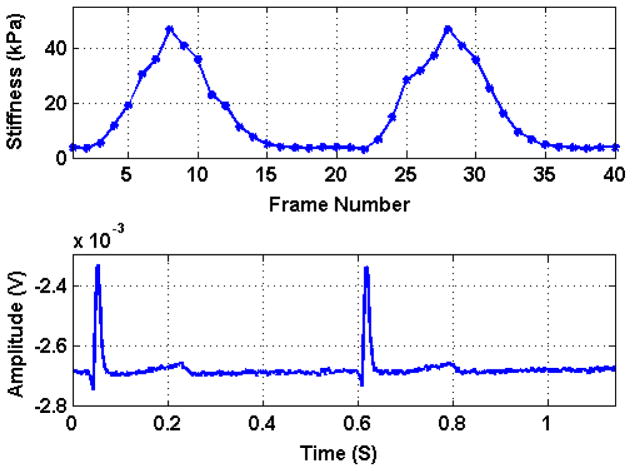
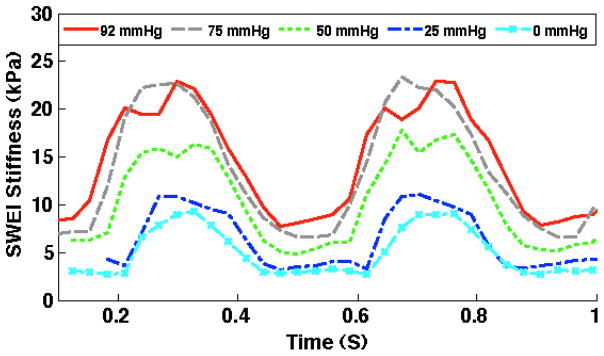
Figure 2 panel A shows the stiffness values corresponding to the shear velocity throughout the cardiac cycle. Panel B shows the simultaneous ECG indicating that stiffness changes synchronously with the cardiac cycle. The myocardial stiffness measurements during the cardiac cycle at different pressure steps are shown in figure 3 for one representative heart. As the coronary perfusion is increased the cardiac stiffness increases at all phases of the cardiac cycle. This increase of stiffness in systole is called the Gregg effect and in diastole is known as the garden hose effect ¹⁹

Figure 2.

Top panel shows the shear modulus of stiffness (index of contractility) values corresponding to the shear velocity throughout the cardiac cycle. The bottom panel shows the simultaneous ECG indicating that stiffness changes cyclically, as expected, during the cardiac cycle.

Figure 3.

The myocardial shear modulus of stiffness measurements by SWEI during the cardiac cycle at the different perfusion pressure steps are shown for one of the rabbits. As the coronary perfusion is increased the cardiac stiffness increases at all phases of the cardiac cycle, though it is more significant during systole corresponding to Gregg Effect phenomenon.

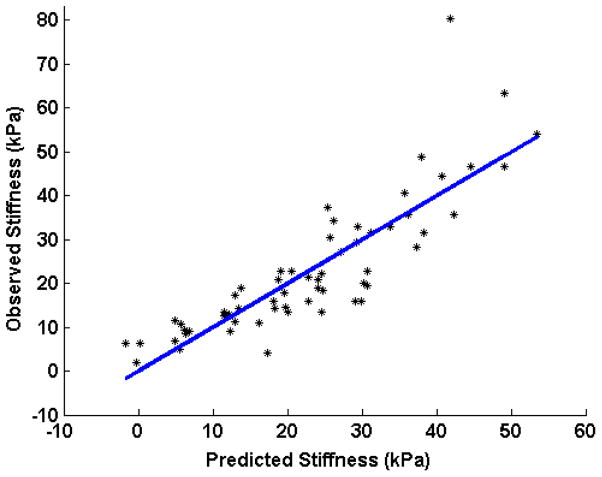
Table 1 lists the regression slope and R² values for the systolic stiffness (index of contractility) at different perfusion pressure steps in each of the hearts. The results of the statistical analysis are shown in figure 4. This figure shows the predicted values of the linear model based on perfusion pressure vs. the observed stiffness values. The 95% confidence interval for the slope of the regression line was found to be 0.02–0.04 m/s/mmHg (R²= 0.80). After converting the shear velocities to shear modulus values and performing the multivariate linear regression, the 95% confidence interval for the slope was found to be 0.20 – 0.34 kPa/mmHg (R²= 0.73).

Table 1.

Regression slope and R² values of the systolic shear modulus of stiffness (index of contractility) versus coronary perfusion pressure relationship in each of the hearts.

Animal	1	2	3	4	5	6	7	8	9	10	11	12
Slope	0.348	0.222	0.181	0.150	0.113	0.187	0.213	0.662	0.165	0.434	0.356	0.155
R2	0.97	0.67	0.57	0.86	0.94	0.86	0.43	0.70	0.78	0.78	0.77	0.94

Figure 4.

Linear regression model showing the shear modulus of stiffness during systole (index of contractility) as it changes with coronary perfusion pressure in all of the hearts. X-axis shows the predicted stiffness from the perfusion pressure considering the rabbits as independent variables and Y-axis shows the recorded shear modulus of stiffness during systole.

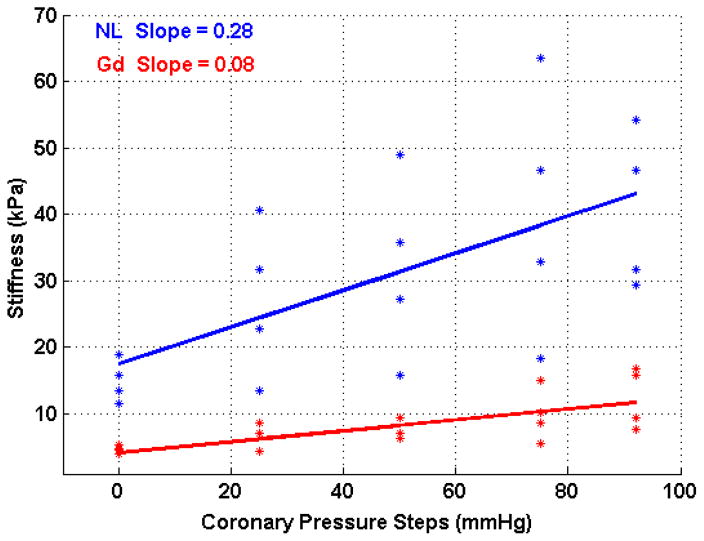
The results of the Gadolinium infusion are presented in tables 2 and 3 for systole and diastole respectively for four hearts. Figure 5 shows the effect of Gadolinium on the Gregg effect by the change in the slope of the linear regression model. The blue line shows the linear regression corresponding to the Gregg effect in normally perfused myocardium with a slope of 0.28 kPa/mmHg (R² = 0.39). In the Gadolinium perfused myocardium, the slope decreased to 0.08 kPa/mmHg (R² = 0.52). This change was determined to be significant (p<0.05) using a t-test. The change in slope during diastole, corresponding to a measurement of the passive properties of the myocardium, was not significant (table 3).

Table 2.

Regression slope and R² values of the systolic shear modulus of stiffness (index of contractility) versus coronary perfusion pressure in four hearts during normal and Gadolinium perfusion

Animal	1	2	3	4
Normal Slope	0.165	0.434	0.356	0.155
Normal R2	0.78	0.78	0.77	0.94
Gadolinium Slope	0.065	0.107	0.125	0.029
Gadolinium R2	0.96	0.83	0.96	0.66

Table 3.

Regression slope and R² values of the diastolic shear modulus of stiffness versus coronary perfusion pressure in four hearts during normal and Gadolinium perfusion

Animal	1	2	3	4
Normal Slope	0.020	0.048	0.025	0.013
Normal R2	0.39	0.25	0.83	0.21
Gadolinium Slope	0.024	0.049	0.043	0.016
Gadolinium R2	0.76	0.92	0.48	0.67

Figure 5.

The effect of Gadolinium on the Gregg Effect shown as the change in the slope of the perfusion pressure versus measured myocardial stiffness linear regression. The blue line shows the linear regression corresponding to the Gregg effect in normally perfused myocardium with a slope of 0.28 kPa/mmHg (R² = 0.39). The slope of the Gadolinium perfused myocardium, shown in red, decreased to 0.08 kPa/mmHg (R² = 0.52).

DISCUSSION

In this study, we show that ultrasound-based SWEI measurements of systolic stiffness can quantify changes in myocardial contractility in the unloaded rabbit heart. The preload and afterload in this experiment were both near zero. To our knowledge there has been no previous contractility measurement in a whole beating heart with near zero loading conditions. A phenomenon known as the Gregg Effect was utilized to verify this capability. The Gregg effect refers to the changes in myocardial contractility caused by acute changes in coronary perfusion pressure. Gadolinium perfusion was used as a stretch-activated ion channel blocker to demonstrate the blockade of the Gregg effect. Hearts perfused by Gadolinium showed a significant decrease in the Gregg effect phenomenon. The increase in contractility in normally perfused hearts and the block of the effect in the Gd³⁺ perfused hearts was shown using SWEI stiffness measurements.

In 1958, Gregg et al. described that an increase in coronary perfusion pressure increases cardiac muscle contraction and oxygen consumption. These changes known as the “Gregg Effect” have been shown using the indentation method, PV loops measurements and other techniques^13,20. As coronary perfusion increases, cardiac contractility increases due to an increase in Ca²⁺ delivery to the myocytes. Reviews of this effect can be found in Downey et al. and Westerhof et al. ^21,22. Livingston et al. showed that there is a linear relationship between the myocardial stiffness and the perfusion pressure corresponding to this effect ⁴. The Gregg effect can be small when auto-regulation is intact as the coronary circulation adjusts to maintain flow; however, it has been shown to be pronounced in the isolated heart preparation and during auto-regulation blockade ²³. Lamberts et al. in 2002 investigated the effect of different agents on the Gregg phenomenon and concluded that stretch-activated ion channels (SACs) are the major contributors to this effect. Using Gadolinium to block these channels, they showed that the developed force in myocardium in response to changes in coronary pressure was blocked in rats ¹⁵.

The coronary vascular space accounts for 10% to 15% of left ventricular wall volume. Changes in the passive myocardial stiffness during diastole due to increased perfusion of the coronary vasculature is called the ‘erectile’, or ‘turgor’ or ‘garden hose’ effect. This effect was characterized in a previous publication from our group. Consistent with the literature, the 95% CI for the garden hose effect on stiffness was found to be 0.04 kPa/mmHg with R² = 0.83 ^19,24. This slope is significantly lower than that of the Gregg effect relationship found in this study (95% CI is 0.20–0.34 kPa/mmHg). The garden hose effect is the passive effect of perfusion pressure on stiffness while the Gregg effect is an active effect. In other words, the difference between these two slopes demonstrates the inotropic effect of higher coronary pressure. According to our previous work in which most of the hearts (n=8) were the same as the hearts of this study; diastolic compliance can be written as:

$${\mathrm{\mu }}_{\mathrm{d}}=\mathrm{A}\ast \mathrm{P}+\mathrm{\alpha }=0.04\ast \mathrm{P}+1.7$$ (2)

where α is the intercept and A is the slope of the stiffness versus pressure regression in diastole. Our results show that we can write a similar linear formula for systolic stiffness:

$${\mathrm{\mu }}_{\mathrm{s}}=\mathrm{B}\ast \mathrm{P}+\mathrm{\beta }=0.27\ast \mathrm{P}+10$$ (3)

where β is the intercept and B is the slope of the regression in systole. The intercept in systole (10 kPa) is greater than diastole (1.7 kPa) and this difference could be related to a baseline contractility of the heart due to the Ca²⁺ reserve in sarcoplasmic reticulum. In addition, the diastolic slope (i.e. garden hose effect) shown in table 3 did not change significantly by blocking stretch activated Ca²⁺ channels, while the Gregg effect was significantly decreased by Gadolinium perfusion indicating a Ca²⁺ delivery phenomenon as the likely cause. The systolic slope of increase in stiffness after blocking of the Ca²⁺ channels for the Gadolinium perfused heart approached the diastolic slope corresponding to the passive effect.

As it was described in the method section, all efforts were made to make the measurements independent of LV intra-ventricular pressure. To measure the actual change in the LV pressure during a Langendorff experiment in one heart, we inserted a Millar pressure catheter into the LV and filled the left atrium to keep solution instead of air in the LV and the afterload column was closed. The measured LV pressure during the cardiac cycle was in the range of 2 to 9 mmHg. This is a minimal change compared to the normal LV pressure (10–70 mmHg). We believe that our measurements were acquired under a minimal preload and afterload conditions. Although Pernot et al. showed minimal effect of preload on stiffness measurements ¹², the contribution of load to the SWEI measurement of stiffness is important and calls for additional research. The results of this study show that SWEI can measure stiffness changes in the myocardium while the LV is under minimal loading conditions.

It is important to note that the ARFI push pulses are similar to those used in Power Doppler imaging with a difference in duration. For example an ARFI push is about 200–300 cycles compared to a 10-cycle wave in Doppler. An ARFI push sufficient for generating displacements in soft tissue on the order of micrometers is achievable within standard diagnostic limits (MI, TI, Ispta) ⁵. This technique is currently being used in the clinic for diagnosing liver and prostate disorders.

While our measurements were performed in an isolated heart setup, we think that the principles would translate to human measurements. This measurement is taken directly from myocardium and could be made noninvasively using ultrasound imaging ¹⁰. Song et al. have recently shown the feasibility of transthoracic diastolic stiffness measurements. Diastolic measurements have higher signal to noise ratio due to the increased displacement in the softened tissue. All transthoracic measurements are subject to image quality issues due to the availability of suitable windows and the presence of clutter and this measurement would be no less reliant on image quality. With additional imaging improvements, the technique may soon be feasible during systole. Other possible approaches include trans-esophageal and intra-cardiac echo (ICE) ²⁵. Any real time measure of contractility would be beneficial in clinical applications for the diagnosis of cardiovascular disorders and/or for assessing changes in the inotropic state of the heart. In addition, this experimental preparation combined with the SWEI velocity measure of contractility could provide a simpler environment for testing the effects of inotropic agents. A technique that demonstrates changes in contractility without the need for pressure-volume measurements could be valuable for this application.

LIMITATIONS

According to Apstein et al. hypo-perfusion for more than 30 minutes in rabbits would cause ischemia ²⁶. In our experiments we tried to keep the steps less than 10 minutes. In addition, to make sure that the procedures did not induce ischemia, the hearts in eight of the animals were stained with Tetrazolium at the end of the experiments. The average heart rate for all the steps in all the subjects was calculated to be 116 ± 8 with the normal perfusate and dropped to 57 ± 9 after the Gadolinium perfusion. This could be explained by the positive force frequency relationship or by the effect of Gadolinium on the ionic currents. The change in heart rate with Gd infusion is consistent with other studies using Gadolinium perfusion ²⁷. Figure 5 shows a reduction in the overall contractility as well as a reduced dependence of contractility on perfusion pressure. Its likely that the shift in the curve to reduced levels of contractility is due to the reduction in heart rate ^28,29; whereas, the change in the slope after Gd infusion is caused by the blocked Gregg effect ²⁷.

In this study, SWEI measurements of stiffness have been reported mainly in (kPa) units by squaring the shear velocity. We also reported the shear velocity values (m/s) for reference because they do not require assumptions of linearity, isotropy and elasticity that are needed to convert them to stiffness moduli.

It has been shown that anisotropy and fiber orientation of the myocardium could affect the SWEI measurements ³⁰. To reduce this variability, we kept the location of the ultrasound probe constant during each experiment. In addition, we averaged the propagating shear waves through the depth of myocardium. The variation in stiffness seen between subjects could be caused by multiple factors including the differences in fiber orientation relative to the imaging plane for each subject or by other local or global intrinsic factors related to each subject. To test the spatial repeatability of the slope measurement, we repeated the measurement of SWEI velocity for a second set of coronary perfusion pressure steps at a second location in the left ventricle of one heart. The two locations had slope values of 0.29 and 0.26 kPa/mmHg with R² value of 0.90 and 0.97 respectively. Although the measurement is local, they were repeatable across at least two locations in one heart. Characterizing the spatial and temporal repeatability of SWEI measurements will be an important component of future work.

Regarding the statistical analysis, we considered each subject as independent to allow for differences in fiber orientation and other intrinsic myocardial characteristics. Further studies are required to fully investigate the effect of these variables on SWEI measurements of stiffness.

CONCLUSION

The SWEI elastography technique provides an index of myocardial contractility or the inotropic state of the heart in the unloaded state. This measurement is taken directly from myocardium and could be measured transthoracically using ultrasound imaging ¹⁰. This measurement technique may be beneficial in clinical applications for the diagnosis of cardiovascular disorders and for assessing changes in the inotropic state of the heart following the administration of therapies. The technique may also find value in testing new inotropic agents and in understanding the contraction physiology of the heart.

Highlights.

• Improved measures of contractility would impact the diagnosis of heart failure

• Ultrasound SWEI can provide direct systolic measurement of myocardial stiffness

• Stepwise changes in cardiac contractility was achieved using the Gregg Effect

• SWEI measurements of stiffness linearly correlated with contractile state

ABBREVIATIONS AND ACRONYMS

EF

Ejection Fraction

IM

Intra-Muscular

IV

Intra-Venous

PV

Pressure-Volume

SACs

Stretch Activated Ion Channels

SWEI

Shear Wave Elasticity Imaging