Variation and Evolution of the Meiotic Requirement for Crossing Over in Mammals

Abstract

The segregation of homologous chromosomes at the first meiotic division is dependent on the presence of at least one well-positioned crossover per chromosome. In some mammalian species, however, the genomic distribution of crossovers is consistent with a more stringent baseline requirement of one crossover per chromosome arm. Given that the meiotic requirement for crossing over defines the minimum frequency of recombination necessary for the production of viable gametes, determining the chromosomal scale of this constraint is essential for defining crossover profiles predisposed to aneuploidy and understanding the parameters that shape patterns of recombination rate evolution across species. Here, I use cytogenetic methods for in situ imaging of crossovers in karyotypically diverse house mice (Mus musculus domesticus) and voles (genus Microtus) to test how chromosome number and configuration constrain the distribution of crossovers in a genome. I show that the global distribution of crossovers in house mice is thresholded by a minimum of one crossover per chromosome arm, whereas the crossover landscape in voles is defined by a more relaxed requirement of one crossover per chromosome. I extend these findings in an evolutionary metaanalysis of published recombination and karyotype data for 112 mammalian species and demonstrate that the physical scale of the genomic crossover distribution has undergone multiple independent shifts from one crossover per chromosome arm to one per chromosome during mammalian evolution. Together, these results indicate that the chromosomal scale constraint on crossover rates is itself a trait that evolves among species, a finding that casts light on an important source of crossover rate variation in mammals.

THE exchange of genetic material between homologous chromosomes via crossing over is a hallmark step of mammalian meiosis. Cytologically, crossovers can be visualized as chiasma that interlock homologous chromosomes. These physical ties serve to counteract the polarizing forces of the meiotic spindle and prevent premature segregation of homologous chromosomes at meiosis I (Page and Hawley 2003). In the absence of at least one well-positioned crossover, homologous chromosomes are susceptible to nondisjunction, an outcome associated with aneuploidy (Hassold and Hunt 2001). Both reduced crossover rates and abnormal patterning of crossovers have been directly associated with infertility in multiple organisms (Koehler et al. 1996).

In genetic data, crossovers result in the reciprocal exchange of heterozygous sites flanking the focal repair site. In this manner, crossovers shuffle alleles into new multilocus combinations and serve as the primary determinant of haplotype diversity within populations (Pritchard and Przeworski 2001). Moreover, by decoupling high fitness alleles from linked deleterious variants, crossing over can reduce selective interference between alleles to facilitate the action of natural selection (Hill and Robertson 1966). At the same time, the extent of the reduction in linked neutral diversity that accompanies the fixation (Maynard Smith and Haigh 1974) or loss (Charlesworth et al. 1993) of selected variants is a function of recombination rate. Recent molecular evidence even points to the possibility that the recombination mechanism itself is mutagenic (Arbeithuber et al. 2015) and may, therefore, directly contribute to fluctuations in DNA diversity across mammalian genomes.

The genetic and evolutionary functions of recombination impose strong constraints on crossover rate evolution (Coop and Przeworski 2007). At the upper extreme, the frequency of crossing over is likely held in check by the joint action of multiple biological forces, including the concentration of rate limiting enzymes involved in meiotic DNA repair, the strength of the mutagenic effect of recombination, and the rate at which recombination breaks up high fitness haplotypes. At the lower extreme, recombination rates are bounded by the essentiality of crossovers for chromosome segregation (Mather 1938).

Despite these dual constraints, crossover rates vary considerably in nature. Across mammals, genome-wide average recombination rates span an order of magnitude (Coop and Przeworski 2007). Even among closely related and interfertile subspecies of house mice, global crossover rates differ by 30% (Dumont and Payseur 2011a). Within populations, individuals display marked variation for crossover rate (Kong et al. 2004; Coop et al. 2008; Dumont et al. 2009), with especially pronounced differences between sexes in many species (Burt et al. 1991; Kong et al. 2010; Ma et al. 2015).

Given its fundamental significance to myriad aspects of biology, it is of considerable interest to identify factors that contribute to variation in recombination rate among organisms. Association analyses (Kong et al. 2004, 2008; Chowdhury et al. 2009; Hunter et al. 2016), genetic mapping in pedigrees and controlled crosses (Thomsen et al. 2001; Murdoch et al. 2010; Parvanov et al. 2010; Dumont and Payseur 2011b), and candidate gene-driven approaches (Baudat et al. 2010; Myers et al. 2010) have recently led to the discovery of numerous recombination-modifying loci in diverse species, including the identification of individual genes. Multiple environmental variables, such as exposure to xenobiotic compounds (Susiarjo et al. 2007; Vrooman et al. 2015) and various measures of stress (Plough 1917; Parsons 1988; Singh et al. 2015), have also been demonstrated to influence recombination rates.

Beyond genes and environment, evolutionary shifts in the biological bounds that constrain recombination rates provide an additional mechanism for recombination rate variation. For instance, genetic variation in components of the DNA repair machinery could alter the maximum capacity for recombination repair in different individuals. Indeed, polymorphisms in DNA repair genes have been associated with variation in the efficiency of DNA repair in mitotic contexts (Wei et al. 1993, 1996; Parshad et al. 1996), with important implications for cancer risk (Jeggo et al. 2016) and aging (Best 2009). Population genetic differences between organisms (Keightley and Otto 2006; Hartfield et al. 2010) and variation in life histories (Burt and Bell 1987; Sharp and Hayman 1988) could contribute to distinct limits on the rate of recombination in different taxa. A final and more trivial observation is that genomic rearrangements leading to changes in chromosome number between organisms will result in new demands for crossing over to ensure accurate homolog segregation at meiosis.

Even for a fixed diploid chromosome number, differences in chromosome architecture may impose unique meiotic recombination requirements for proper chromosome disjunction. A minimum of one crossover per chromosome is required for correct chromosome segregation, but evidence from some taxa suggests that the meiotic requirement may be stricter, necessitating at least one crossover per chromosome arm. For example, on marker dense human genetic linkage maps, all chromosome arms measure ≥50 cM (Broman et al. 1998; Kong et al. 2010), consistent with the presence of at least one exchange event per arm. In mammals, the correlation between chiasma number and chromosome arm number is higher than the corresponding correlation with chromosome number (Dutrillaux 1986), suggesting that the chromosomal constraint lies at the level of the chromosome arm (Pardo-Manuel De Villena and Sapienza 2001; Segura et al. 2013). In contrast, crossover events visualized as MLH1 foci on surface spread spermatocytes from voles, deer mice (Dumont and Payseur 2011a), shrews (Borodin et al. 2008), and rhesus macaques (Hassold et al. 2009) reveal a high frequency of achiasmate chromosome arms, suggesting that, at least in these taxa, there is only one crossover per chromosome. Similarly, marker dense linkage maps from pig (Ellegren et al. 1994; Vingborg et al. 2009), horse (Swinburne et al. 2006), and rat (Bihoreau et al. 2001) feature chromosome arms measuring <50 cM.

Although evidence suggests that the chromosomal requirement for recombination at meiosis may vary among species, this point has received limited recognition (see Borodin et al. 2008 for an exception). Instead, there is a common perception that all mammals require one crossover per chromosome arm for homolog disjunction (Dutrillaux 1986; Pardo-Manuel De Villena and Sapienza 2001; Dumas and Britton-Davidian 2002; Segura et al. 2013). Even as molecular details on the fine-scale control of recombination hotspots continue to accumulate (Ségurel et al. 2011; Baker et al. 2014, 2015), the simple, fundamental question of how many crossovers an organism needs to ensure the proper segregation of homologous chromosomes remains unsettled.

Here, I aim to address this open question through experimental and phylogenetic tests of the genomic crossover distribution at meiosis. Using a cytogenetic approach for in situ mapping of crossovers in two independent groups of closely related, karyotypically diverse mammals (house mice and North American gray voles), I demonstrate species variation in the chromosomal scale of the meiotic requirement for crossing over. By analyzing published recombination data from >100 mammalian taxa in an evolutionary framework, I provide evidence for multiple independent shifts from a minimum of one crossover per chromosome arm to one crossover per chromosome across the mammalian phylogeny. These findings point to species variation in the chromosomal constraint on recombination at meiosis and provide new insights into how karyotype evolution shapes recombination rate variation in mammals.

Materials and Methods

Animal husbandry and ethics statement

Inbred house mouse strains BALB/cByJ (2N = 40), WSB/EiJ (2N = 40), PERA/EiJ (2N = 40), CBy.RBF-Rb(8.12)5Bnr (2N = 38), RBF/DnJ (2N = 34), and ZALENDE/EiJ (2N = 26) were purchased from The Jackson Laboratory and housed in the North Carolina State University (NCSU) Biological Resources Facility according to an animal care protocol approved by the NCSU Institutional Animal Care and Use Committee. Mice were provided with food (PicoLab Mouse Diet 20 5058*) and water ad libitum. Adult males from each inbred strain were killed by exposure to CO₂ at 8–10 weeks of age.

Testis tissue for the prairie vole (Microtus ochrogaster) was kindly donated by Lisa McGraw at NCSU. MLH1 mapping data for the meadow vole, M. pennsylvanicus, have been previously published (Dumont and Payseur 2011a).

Mogollon (M. mogollonensis) and montane voles (M. montanus) were obtained in collaboration with Carol Chambers and Valerie Horncastle at Northern Arizona University (NAU). Adult males were live caught in the high-altitude White Mountains of Eastern Arizona and temporarily housed at the Biological Sciences Annex at NAU following protocols approved by the NAU Institutional Animal Care and Use Committee. Wild animals were then transported via courier service to the Yates Mill satellite animal facility operated by NCSU in accordance with protocols approved by the NCSU Institutional Animal Care and Use Committee. Animals were killed by CO₂.

Spermatocyte cell spreads and immunostaining

Spermatocyte cell spreads were prepared using the drying down method of Peters et al. (1997) and immunostained as previously described (Dumont et al. 2015). Briefly, slides were incubated with anti-mouse MLH1 (BD Biosciences, San Jose, CA), anti-human CREST (Antibodies, Davis, CA), and anti-goat SYCP3 (Santa Cruz Biotechnology, Santa Cruz, CA) antibodies diluted to a concentration of 1:100 in 1× ADB [10× ADB: 2.5 ml normal donkey serum (Jackson ImmunoResearch, West Grove, PA), 22.5 ml 1× PBS, 0.75 g bovine serum albumin (Fraction V; Fisher Scientific, Waltham, MA), and 12.5 μl Triton X-100]. Secondary antibodies (donkey anti-goat Rhodamine Red-X, donkey anti-mouse FITC, and donkey anti-human Coumarin AMCA; Jackson ImmunoResearch) were used at 1:200 dilution. Slides were washed three times in 1× PBS with constant agitation, rinsed briefly in distilled water, and mounted in several drops of ProLong Gold antifade media (Molecular Probes, Eugene, OR).

Slides were visualized with a Leica DM5500 B microscope equipped with a Photometrics CoolSNAP HQ2 CCD camera and a ×63 oil-immersion objective lens. Images were captured as RGB stacks in Leica Application Suite (v. 2.3.5) software and stored as high-resolution tiff files. Images were subsequently cropped and the fluorescent intensity was adjusted with the Fiji image analysis platform (Schindelin et al. 2012).

Over 80 late pachytene cells characterized by (i) the complete merger of SYCP3 signals from all autosomal homologs; (ii) a full complement of chromosomes; (iii) low background fluorescence; and (iv) bright, punctuate MLH1 signals were imaged for each inbred strain. Cells that were damaged during preparation or displayed bulbous chromosome termini (indicative of transition into diplotene) were not imaged. For each spermatocyte meeting these criteria, the total number of MLH1 foci and the number of achiasmate chromosome arms were recorded. MLH1 foci on the XY bivalent were excluded from all analyses, as the meiotic dynamics of the sex chromosomes are temporally decoupled from those of the autosomes (Kauppi et al. 2011).

The meiotic chromosome axes of ZALENDE/EiJ animals exhibited frequent end-to-end associations that yielded long, multichromosome chains (Supplemental Material, Figure S1). These structures made it challenging to resolve the boundaries between chromosomes. Consequently, I was unable to ascertain the number of MLH1 foci per chromosome arm on the majority of spermatocyte preparations from this strain. Instead, for most of the ZALENDE/EiJ spermatocytes examined (100 of 130; 77%), only the total autosomal crossover count per spermatocyte was scored.

Statistical analyses

All statistical analyses were carried out in R: A Language and Environment For Statistical Computing using base packages and functions (R Development Core Team 2013).

To accommodate the ordinal nature of MLH1 count data, nonparametric methods were preferentially used. However, to test for significant strain, species, and karyotype effects on mean MLH1 foci number, I adopted a one-way ANOVA framework. Although the use of count data violates the normality assumption of ANOVA, in practice this departure from model assumptions is not likely to affect qualitative conclusions.

Database collection and phylogenetics

A database of mammalian recombination and karyotype data was compiled using literature searches (Table S1). Recombination rate estimates derived from chiasma analysis, distribution of MLH1 foci on late pachytene spermatocytes and oocytes, and high-quality genetic linkage maps were included. Linkage map lengths were transformed into estimates of the average number of crossovers per meiosis by dividing the total map length in centimorgan units by 50 cM. Only animals with standard species karyotypes were included in this analysis.

An informal supertree of the 112 mammalian species with recombination data was assembled by manually splicing previously published phylogenetic trees (Sanderson et al. 1998; Bininda-Emonds 2004; Table S2). Species relationships that are not yet resolved, or for which there is conflicting or weak phylogenetic evidence, were collapsed to polytomies.

Data availability

The author states that all data necessary for confirming the conclusions presented in this article are represented fully within the article and its supplemental files.

Results and Discussion

Crossover rate variation among karyotypically diverse house mice

Western European house mice (Mus musculus domesticus) are a powerful, natural model system for testing whether the chromosome-scale constraint on meiotic recombination lies at the level of the chromosome arm or the whole chromosome. The genomes of these mice are characterized by exceptionally high rates of Robertsonian (Rb) translocation (Nachman and Searle 1995; Pialek et al. 2005; Garagna et al. 2014). These genomic rearrangements result in the fusion of two chromosomes at their centromeres, reducing the number of chromosomes in the karyotype while maintaining the number of chromosome arms [i.e., the fundamental number (FN)]. Multiple Rb races of house mice are commercially available as inbred strains, providing the opportunity to directly test the effects of karyotypic rearrangements on crossover rates in a controlled laboratory environment.

I used cytogenetic mapping of MLH1 foci to measure global crossover rates in multiple males from each of three karyotypically distinct Rb inbred strains of M. m. domesticus [CBy.RBF-Rb(8.12)5BnR/J, RBF/DnJ, and ZALENDE/EiJ], two wild-derived inbred strains of M. m. domesticus with the standard 2N = 40 house mouse karyotype (WSB/EiJ and PERA/EiJ), and a common laboratory strain (BALB/cByJ; 2N = 40). Collectively, the karyotypes of the inbred Rb strains capture a nested series of chromosome fusions (Table 1 and Figure S2). The CBy.RBF-Rb(8.12) strain has a single metacentric chromosome resulting from the fusion of chromosomes 8 and 12. RBF/DnJ has three metacentric chromosomes, including the chromosome 8 and chromosome 12 rearrangement. The three RBF metacentric chromosomes are also present in the highly rearranged ZALENDE karyotype (2N = 26), which is defined by seven Robertsonian fusion events.

Table 1. Variation in MLH1 number and distribution in male house mice with diverse karyotypes.

Strain	Animal	2N	No. cells	Fraction of chromosomes				Fraction of achiasmate arms	Average MLH1	SD MLH1
				0 MLH1 foci	1 MLH1 focus	2 MLH1 foci	3 MLH1 foci
PERA	1	40	52	0.0051	0.7065	0.2885	0.0000	0.0051	24.38	2.13
WSB	1	40	21	0.0251	0.6667	0.3058	0.0000	0.0251	24.29	2.00
WSB	2	40	41	0.0128	0.7291	0.2567	0.0013	0.0128	23.68	2.23
WSB	3	40	38	0.0125	0.6759	0.3102	0.0014	0.0125	24.71	2.14
Total			100	0.0153	0.6958	0.2874	0.0011	0.0153	24.20	2.18
BALBc	1	40	32	0.0082	0.6809	0.3109	0.0000	0.0082	24.75	2.31
BALBc	2	40	22	0.0048	0.6914	0.3038	0.0000	0.0048	24.68	1.73
BALBc	3	40	29	0.0054	0.6806	0.3085	0.0054	0.0054	24.97	2.37
Total			83	0.0063	0.6836	0.3082	0.0019	0.0063	24.81	2.17
CBy.RBF	1	38	27	0.0391	0.5679	0.3868	0.0062	0.0453	24.48	3.04
CBy.RBF	2	38	33	0.0168	0.6077	0.3653	0.0084	0.0139	24.55	1.99
CBy.RBF	3	38	28	0.0099	0.5700	0.4095	0.0079	0.0144	25.50	2.44
CBy.RBF	4	38	29	0.0192	0.6437	0.3333	0.0038	0.0192	23.79	1.86
CBy.RBF	5	38	38	0.0132	0.5614	0.4167	0.0088	0.0146	25.58	2.76
CBy.RBF	6	38	30	0.0082	0.5342	0.4316	0.0085	0.0099	25.90	3.73
CBy.RBF	7	38	32	0.0122	0.5573	0.4253	0.0052	0.0174	25.63	2.25
Total			217	0.0166	0.5778	0.3957	0.0070	0.0188	25.09	2.70
RBF	1	34	19	0.0221	0.7353	0.2426	0.0000	0.0486	19.53	1.22
RBF	2	34	6	0.0208	0.7500	0.2250	0.0000	0.0781	19.83	1.72
RBF	3	34	28	0.0231	0.6944	0.2731	0.0093	0.0486	20.32	1.83
RBF	4	34	38	0.0148	0.7303	0.2434	0.0115	0.0405	20.03	1.85
Total			91	0.0192	0.7213	0.2514	0.0079	0.0465	20.00	1.72
ZALENDE	1	26	78	0.0032	0.2560	0.5000	0.2262	0.0220	23.72	1.99
ZALENDE	2	26	52	0.0119	0.2833	0.5167	0.2000	0.0076	24.21	2.23
Total			130	0.0051	0.2632	0.5044	0.2193	0.0167	23.92	2.10

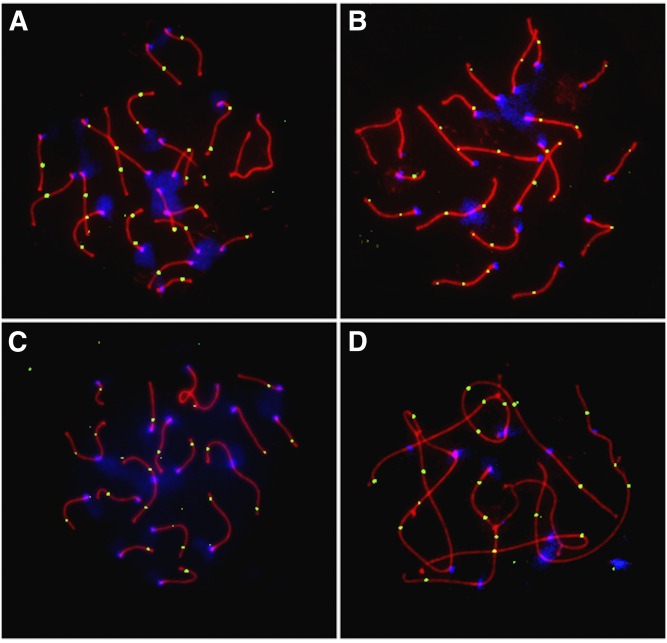
Representative images of surface spread spermatocytes immunostained for MLH1 and the synaptonemal complex protein SYCP3 are shown in Figure 1. I analyzed the total number of autosomal MLH1 foci in >80 late-pachytene-stage cells per strain, considering multiple biological replicates per strain (with the exception of PERA; Table 1). Although a subset of mammalian crossovers is resolved by an MLH1-independent pathway (Hollingsworth and Brill 2004; Holloway et al. 2008), prior studies have established the power and accuracy of this approach for estimating global crossover rates in mammals (Anderson et al. 1999; Koehler et al. 2002; Sun et al. 2006; Hassold et al. 2009).

Figure 1.

Representative images of pachytene spermatocytes from (A) BALB/cBy (2N = 40), (B) CBy.RBF(8.12) (2N = 38), (C) RBF/DnJ (2N = 34), and (D) ZALENDE/EiJ (2N = 26) males. Cells were immunostained for the mismatch repair protein MLH1 (green foci), which localizes to most sites of crossing over; the synaptonemal complex protein SYCP3 (red); and for centromeric proteins (CREST; blue). The cells displayed have 26, 27, 20, and 21 autosomal MLH1 foci, respectively. Foci on the sex bivalent were not considered in this study.

There is significant interstrain variation in mean MLH1 foci count among males from the six inbred strains surveyed (one-way ANOVA treating strain identity as a factor: F_5,667 = 67.75, P < 10⁻¹⁶). At the extremes, RBF spermatocytes have a mean of 20 MLH1 foci per spermatocyte, whereas CBy.RBF-Rb(8.12) males average 25% more MLH1 foci per meiosis (mean = 25.09; Table 1). The repeatability of MLH1 measurements across biological replicates within a strain indicates that much of this variation is genetic [one-way ANOVA P > 0.1 for all strains except CBy.RBF-Rb(8.12); P = 0.01673 for CBy.RBF-Rb(8.12)]. This apparent genetic influence on recombination could be due to modifiers of recombination rate that are segregating among strains and/or karyotypic differences between strains.

The chromosomal distribution of crossovers in house mice

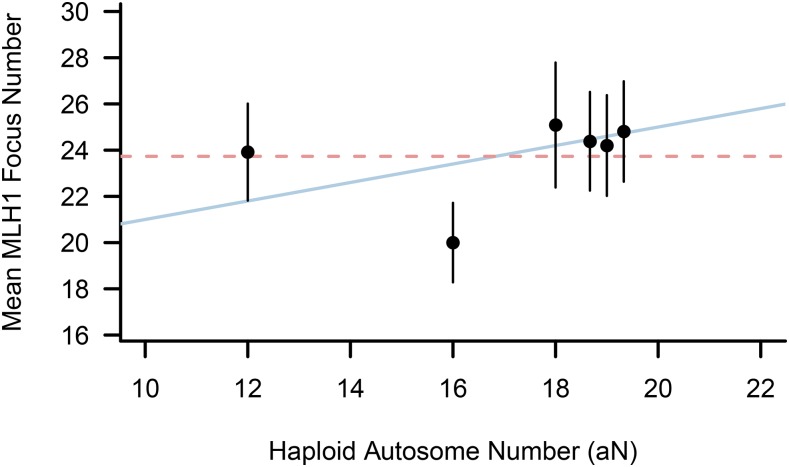
If crossover rates in house mice are constrained by a minimum biological requirement of one crossover per chromosome, mean MLH1 foci counts should decrease in proportion to the number of Rb fusions in a karyotype. In contrast, if the meiotic requirement for recombination necessitates at least one crossover per chromosome arm, mean MLH1 foci counts should be similar among house mouse strains, irrespective of the number of Rb rearrangements.

Using guidance from these predictions, MLH1 count data from karyotypically diverse male mice are most consistent with a requirement of one crossover per chromosome arm for accurate homolog disjunction (Figure 2). Although MLH1 counts are significantly reduced in RBF (2N = 34) males relative to mice with the standard karyotype (Mann–Whitney U-test P < 10⁻¹⁵ in all pairwise strain comparisons; Table 2), the two other Rb strains do not conform to this pattern. In fact, there is no difference in mean MLH1 foci count between ZALENDE (2N = 26) and either of the two 2N = 40 wild-derived inbred strains. Moreover, CBy.RBF-Rb(8.12) males have a significantly higher frequency of crossing over than either WSB or PERA (Table 2).

Figure 2.

Mean MLH1 foci count as a function of diploid chromosome number in standard 2N = 40 and Robertsonian inbred male mice. Error bars denote ± 1 SD. The dashed horizontal line corresponds to the expected distribution of MLH1 differences among strains if a single crossover per chromosome arm is needed for accurate disjunction. The solid sloped line approximates the expected pattern under the scenario where only a single crossover per chromosome is needed to ensure proper segregation. Points for the three standard karyotype strains analyzed are jiggered about the aN = 19 x-coordinate for visualization.

Table 2. Mann–Whitney U-test P-values comparing male MLH1 counts between strains.

	PERA	BALB/c	CBy.RBF	RBF	ZALENDE
WSB	0.603	0.092	4.41 × 10−4	2.01 × 10−25	0.313
PERA		0.379	0.031	1.35 × 10−18	0.168
BALB/c			0.138	6.92 × 10−26	0.007
CBy.RBF				3.38 × 10−35	7.57 × 10−7
RBF					8.00 × 10−27

The absence of a systematic reduction in crossover rate in Rb relative to 2N = 40 mice contrasts with the conclusions of several prior studies (Castiglia and Capanna 2002; Dumas and Britton-Davidian 2002; Merico et al. 2003). There are at least two potential explanations for this discrepancy. First, the trends in recombination rate summarized above are based on a small sample of three karyotypically diverse inbred strains that may not capture a representative snapshot of recombination rate variation in Rb animals. Second, any influence of karyotype on crossover rate variation in Rb vs. 2N = 40 strains may be overwhelmed by the independent effect of strain-specific recombination rate modifiers (see below).

Frequency of achiasmate chromosome arms in Rb mice

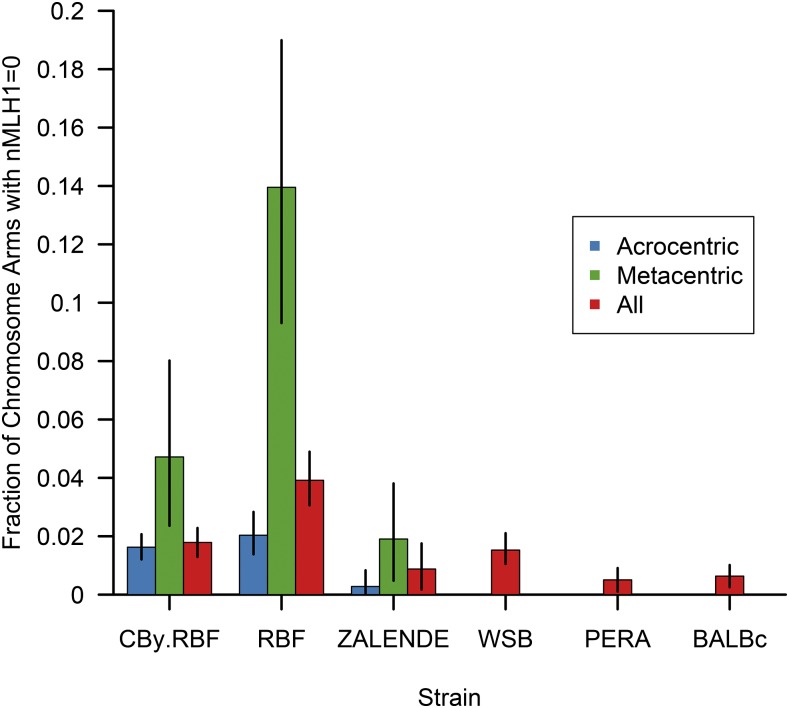
A complementary approach for discriminating between the two levels of chromosomal constraint on meiotic crossover rates is to contrast the frequency of achiasmate chromosome arms in Rb and 2N = 40 mice. If global recombination rates are biologically constrained by a minimum of one crossover per chromosome in this system, the frequency of achiasmate chromosome arms should be increased in Rb mice relative to animals with the 2N = 40 karyotype. Consistent with this prediction, there is a 2.3-fold increase in the frequency of achiasmate chromosome arms in the three Rb strains relative to animals with the standard karyotype (achiasmate arm frequency in Rb mice = 0.0227; achiasmate arm frequency in 2N = 40 mice = 0.0099; Mann–Whitney U-test P = 5.6 × 10⁻⁵; Figure 3). This trend is driven, in large part, by the exceptionally high frequency of chromosome arms lacking an MLH1 focus in RBF animals (frequency = 0.039; Figure 3). Nonetheless, even after excluding this strain, the frequency of non-MLH1-bearing chromosome arms is significantly higher in Rb mice relative to 2N = 40 strains (Mann–Whitney U-test P = 0.007). This increase is driven by the elevated fractions of both acrocentric and metacentric chromosomes lacking an MLH1 focus in Rb animals (Figure 3). This observation hints at potential genome-wide misregulation of crossover control in Rb mice or greater reliance on MLH1-independent mechanisms of DNA repair in these strains.

Figure 3.

Fraction of autosomal chromosome arms lacking an MLH1 focus in inbred male mice. For the three Robertsonian strains [Cby.RBF(8.12), RBF, and ZALENDE], the frequency of acrocentric chromosomes with no MLH1 foci and metacentric fusion chromosomes with at least one arm lacking a MLH1 focus are presented in blue and green, respectively. The overall frequency of chromosome arms with no MLH1 foci (metacentric and acrocentric chromosomes combined) is shown in red. For 2N = 40 strains (WSB, PERA, and BALBc) possessing karyotypes composed of only acrocentric chromosomes, reported fractions correspond to the frequency of chromosomes lacking an MLH1 focus. Error bars denote 95% confidence intervals determined by bootstrap sampling the observed data 1000 times.

Although the frequency of non-MLH1-bearing chromosome arms is increased on both acrocentric and Rb fusion chromosomes in Rb mice, arms on metacentric chromosomes are especially susceptible to the absence of an MLH1 focus (Figure 3). At the most extreme, nearly 14% of metacentric chromosomes in RBF lack an MLH1 focus on at least one chromosome arm. One interpretation for this pattern is that metacentric chromosomes experience a relaxation in the meiotic demands for recombination, consistent with the meiotic crossover constraint falling at the level of the whole chromosome in house mouse males. If valid, this interpretation would contradict the conclusion reached from the analysis of total crossover numbers presented above.

A critical point of recognition, however, is that MLH1 marks only the subset of crossovers subject to crossover interference. A second class of crossovers that are not subject to the rules of interference are resolved via non-MLH1-dependent mechanisms and cannot be detected by the assay used here (de los Santos et al. 2003; Hollingsworth and Brill 2004; Holloway et al. 2008). Strong positive interference prevents the formation of crossovers in close spatial proximity along a chromosome (Broman et al. 2002; de Boer et al. 2006) and extends uninterrupted across mammalian centromeres (Broman and Weber 2000). Thus, the absence of an MLH1 focus on one arm of metacentric chromosomes may not indicate a missing crossover on that arm, but rather the absence of an MLH1-dependent crossover on that arm. This important consideration complicates the interpretation of these data and motivates additional investigations of crossover rate variation in Rb mice using alternative experimental approaches for crossover detection. Net, MLH1 data from Rb house mice align most compellingly with the conclusion that a minimum of one crossover per chromosome arm is required for accurate meiotic chromosome segregation.

Limitations of Rb mice for testing the meiotic requirement for crossing over

A caveat to the use of Rb mice for understanding the meiotic constraints on crossing over is that, in addition to karyotypic differences, strains are also genetically distinct. There are striking differences in global MLH1 frequency between inbred house mouse strains (Koehler et al. 2002; Dumont and Payseur 2011a), and prior studies have established that this divergence carries a clear genetic basis (Murdoch et al. 2010; Dumont and Payseur 2011b; Liu et al. 2014). Ideally, to distinguish between the one-crossover-per-chromosome and one-crossover-per-chromosome-arm alternatives, one would rely on a nested series of Rb rearrangements on a common genetic background. Such a resource does not exist in mice or, to the best of my knowledge, in any species. However, in light of this limitation, the contrast between BALB/cByJ (2N = 40) and Cby.RBF-Rb(8.12) is especially informative. The Cby.RBF-Rb(8.12) strain was derived by backcrossing a Rb(8.12) fusion chromosome onto the BALB/cByJ background, affording the opportunity to test the effect of karyotype rearrangement on crossover frequency, largely excluding the influence of genetic effects. Notably, mean MLH1 foci counts are indistinguishable between these two closely related strains (Mann–Whitney U-test P = 0.1383), an observation that lends further support to the conclusion that a minimum of one crossover event per chromosome arm is required in mice.

A second limitation of this analysis is that it presumes that changes in crossover rate occur immediately (or nearly immediately) after an Rb fusion event. If the intrinsic factors regulating chromosome-scale recombination are predominately due to the action of genes rather than inherent properties of chromosomal architecture, the recombinational response to karyotypic changes could operate on a lag, the duration of which is determined by the waiting time for new mutations affecting the chromosomal distribution of crossovers. Thus, the observation that every chromosome arm carries a crossover in house mice may not accurately mirror the underlying biological constraints on the chromosome-scale crossover distribution, but instead reflect the recent acrocentric ancestry of both chromosomes arms on Rb metacentric chromosomes. One solution to address this limitation is to examine recombination patterns in more divergent organisms, including those with karyotype rearrangements borne by mechanisms other than Rb translocations.

Patterns of crossover rate variation in Microtus

Gray voles of the genus Microtus are a second exemplary system for dissecting the relationship between recombination rate and karyotype. North American voles radiated from a common ancestor ∼2 MYA (Jaarola et al. 2004). Despite this recent divergence, there are remarkable levels of karyotype diversity among species, with karyotypes ranging from 2N = 17/18 to 2N = 64 (Modi 1987a). Unlike house mice, however, vole karyotypes are not simple Rb-derived permutations on a common ancestral genome. Thus, contrasting crossover rates between closely related vole species provides an opportunity to assess the impact of large-scale genome rearrangements, including changes in FN, on recombination between more divergent organisms.

I used MLH1 mapping to measure the global crossover rate in four North American vole species: prairie voles (M. ochrogaster; n = 2 animals), montane voles (M. montanus; n = 1), meadow voles (M. pennsylvanicus; n = 3), and mogollon voles (M. mogollonensis, formerly M. mexicanus; n = 2). These species capture a diverse snapshot of karyotype variation within Microtus, both in terms of absolute chromosome number and FN (Table 3 and Figure S3). M. ochrogaster has the highest chromosome number (2N = 54), with a karyotype composed of acrocentric and small metacentric chromosomes. M. montanus has a significantly reduced karyotype (2N = 12) characterized by 11 biarmed autosomes (aFN = 46). The karyotypes of M. pennsylvanicus and M. mogollensis contain intermediate chromosome numbers (2N = 46 and 2N = −44, respectively) and are dominated by acrocentric chromosomes (corresponding to aFN = 50 and aFN = 54, respectively).

Table 3. Variation in MLH1 count and distribution in males from karotypically diverse vole species.

Species	Animal	2N	aFN	No. large autosome arms	No. cells	Fraction of chromosomes				Fraction of achiasmate arms	Average MLH1	SD MLH1
						0 MLH1 foci	1 MLH1 focus	2 MLH1 foci	3 MLH1 foci
M. montanus	1	24	44	38	54	0.0034	0.1515	0.7879	0.0556	0.1600	20.91	1.23
M. mogollonensis	1	44	54	50	18	0.0053	0.7937	0.2010	0	0.1376	25.11	1.28
M. mogollonensis	2	44	54	50	45	0.0053	0.8233	0.1714	0.0011	0.1510	24.56	1.18
Total					63	0.0053	0.815	0.1800	0.0008	0.1469	24.71	1.22
M. ochrogaster	1	54	64	52	12	0.0160	0.9231	0.0609	0	0.2273	27.17	1.80
M. ochrogaster	2	54	64	52	47	0.0033	0.9828	0.0147	0	0.2308	26.32	0.75
Total					59	0.0059	0.9707	0.0241	0	0.2300	26.49	1.09
M. pennsylvanicus	1	46	50	48	7	0.0130	0.9156	0.0714	0	NA	23.29	1.70
M. pennsylvanicus	2	46	50	48	23	0.0079	0.9012	0.0889	0.0020	NA	23.87	1.18
M. pennsylvanicus	3	46	50	48	20	0.0159	0.8910	0.0910	0.0023	NA	23.75	1.18
Total					50	0.0118	0.8991	0.0873	0.0018	NA	23.74	1.50

The number of MLH1 foci per meiosis differs significantly among males from these four vole species (one-way ANOVA treating species identity as a factor: F_3,222 = 193.04, P < 10⁻¹⁵). There is no evidence for polymorphism among individuals within a species (P > 0.1 for all pairwise Mann–Whitney U-tests), suggesting that observed levels of species variation are not likely misestimated on account of unsampled polymorphism in this phenotype. Observed variation in mean MLH1 counts is strongly correlated with species differences in both chromosome number (Spearman’s rho = 0.75, P < 10⁻¹⁵) and fundamental number (Spearman’s rho = 0.85, P < 10⁻¹⁵).

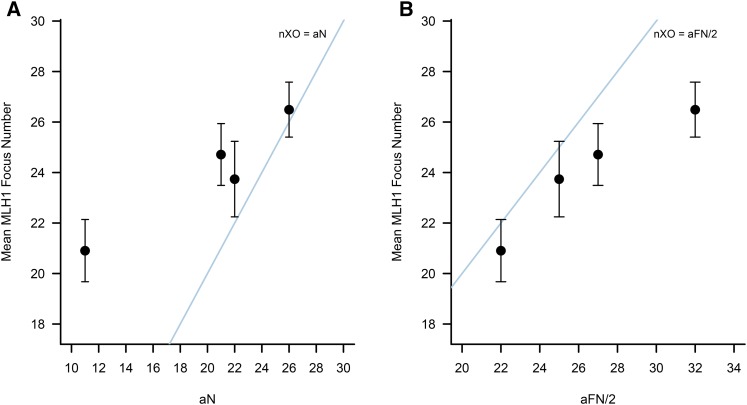
If the global rate of recombination in male voles is constrained by a minimum of one crossover per chromosome arm, as in house mice, mean MLH1 foci counts should be greater than or equal to the haploid number of autosomal chromosome arms (aFN/2) in each vole species. This expectation is not met (Figure 4). In each of the four vole species, >70% of spermatocytes have fewer MLH1 foci than expected if ≥1 crossover per chromosome arm is required for successful completion of the meiotic cell cycle.

Figure 4.

Distribution of mean (± 1 SD) MLH1 foci counts in male voles as a function of (A) the number of autosomes in a haploid genome and (B) the haploid number of autosomal chromosome arms. From left to right on both plots, points correspond to species values for M. montanus, M. mogollonensis, M. pennsylvanicus, and M. ochrogaster. (A) All species harbor more crossovers than autosomal bivalents, as demonstrated by the fact that all points fall above the y = x line corresponding to one crossover per chromosome. (B) In contrast, none of the examined vole species has, on average, more crossovers than chromosome arms, with all points falling below the nXO = aFN line.

On small metacentric and submetacentric chromosomes, one crossover may suffice to counter spindle tension, ensure bipolar alignment at the metaphase plate, and promote correct segregation at anaphase I. Indeed, I rarely observed two MLH1 foci on the small metacentric chromosomes of M. ochrogaster and M. mogollonensis and the short arms of submetacentric chromosomes frequently lacked an MLH1 focus. These arms are dominated by heterochromatin (Modi 1987b), and crossovers are strongly suppressed in heterochromatic regions (Stack 1984; Laurie and Hultén 1985; Froenicke et al. 2002; Segura et al. 2013). Even after conservatively excluding one arm from small metacentric chromosomes and the short arms of submetacentric chromosomes in each karyotype, a substantial fraction of spermatocytes (>33%) from M. mogollonensis and M. pennsylvanicus still possess fewer MLH1 foci than large chromosome arms.

As noted above, MLH1 only designates crossovers subject to interference. In house mice, this class of crossovers accounts for >90% of all crossovers (Holloway et al. 2008), indicating that the overwhelming majority are detected by MLH1 mapping in mammals. To determine whether the small subset of “missing” noninterferring crossovers could plausibly explain the high frequency of non-MLH1-bearing chromosome arms in voles, I artificially inflated the MLH1 count for each spermatocyte by 10%. Over 40% of spermatocytes from M. mogollonensis and M. ochrogaster still have fewer crossovers than chromosome arms. A more modest fraction of cells (<20%) from M. montanus and M. pennsylvanicus fail to meet this threshold.

Taken together, these findings suggest that crossover rates are limited by a meiotic requirement of one crossover per chromosome in voles.

Crossing over and karyotype variation in mammals

Motivated by the apparent difference in the chromosomal constraint on recombination between house mice and voles, I next sought to define the lower bound on meiotic recombination across a broader phylogenetic sample of mammals. Using literature searches, I compiled a database of published crossover counts from chiasma analysis, genetic linkage maps, and MLH1 foci mapping. For each species, I also recorded the number of chromosomes, the fundamental number, and the number of autosomal chromosomes arms (aFN) in the karyotype of each species. This resource includes 112 mammalian species, including representatives from 16 mammalian orders (Table S1).

A notable limitation of this dataset is that >80% of species have crossover rate estimates derived from males only (94 of 112 species, 83.9%; Table S1). This sex bias owes, in large part, to the difficulty of obtaining early meiotic tissue for cytogenetic analysis of female recombination (Handel and Eppig 1998; Morelli and Cohen 2005). Despite this challenge, it is well established that many mammalian species exhibit a strong sex dimorphism for crossover rate and distribution. In humans, for example, the frequency of crossing over in females is nearly twice that in males (Broman et al. 1998; Kong et al. 2010) and the distribution of crossovers is more strongly polarized to distal subtelomeric regions in males than in females. In contrast, in many marsupial species (Hayman et al. 1988) and domestic sheep (Jagiello et al. 1974; Logue 1977), males have higher crossover rates than females. Although species differences in crossover rate are often much larger than the sex differences observed within species, evolutionary insights from this dataset are, overwhelmingly, based on data from one sex.

Both the number of chromosomes and the number of chromosome arms are strongly positively correlated with crossover counts across mammals (Table 4). The magnitude of the correlation with chromosome number is nominally higher than that with the number of autosomal chromosome arms, a finding inconsistent with prior results (Dutrillaux 1986; Pardo-Manuel De Villena and Sapienza 2001; Segura et al. 2013). This discrepancy likely owes to the sensitivity of this correlation to both the method for estimating crossover frequency and taxonomic sampling. For example, karyotype is a weaker predictor of crossover frequency estimated from MLH1 foci than from chiasma counts, and the autosomal fundamental number explains a greater fraction of the variance in chiasma counts than does the number of chromosomes (Table 4). Repeated, random down-sampling of the full dataset to include just 45 species [comparable to the number of taxa analyzed by Pardo-Manuel De Villena and Sapienza (2001)] yields considerable variation in the magnitude of the correlations between crossover frequency and both 2N and FN. In addition, simulation replicates vary with regard to whether 2N or FN is the stronger predictor of crossover rate (Figure S4). For 37.3% of 1000 randomly down-sampled datasets, the correlation between crossover frequency and chromosome arm number is higher than the corresponding correlation with chromosome number, opposite the pattern seen with the complete dataset. Overall, the results of these simple simulations indicate that insights into the relationship between karyotype and the meiotic crossover constraint based on correlational analyses are sensitive to sampling.

Table 4. Spearman correlation coefficients between autosomal fundamental number and crossover counts measured using different experimental approaches.

	2N	aFN
All methods	0.79	0.78
n = 112 species	P < 2.2 × 10−16	P < 2.2 × 10−16
MLH1	0.65	0.55
n = 47 species	P = 9.8 × 10−7	P = 6.9 × 10−5
Chiasma	0.81	0.82
n = 77 species	P < 2.2 × 10−16	P < 2.2 × 10−16
Linkage maps	0.39	0.34
n = 16 species	P = 0.1386	P = 0.1945

Evolution of the meiotic constraint on crossing over

Although the number of chromosome arms in a karyotype is strongly predictive of crossover rate (Table 4), a critical observation is that many mammalian species (23% of the species analyzed here) have fewer crossovers than chromosome arms (Figure 5 and Figure S5). The appreciable fraction of species with nXO < aFN/2 cannot be readily explained by biases inherent to individual methods for quantifying crossover rates, as species with crossover rates measured via MLH1 mapping, chiasma analysis, and genetic linkage maps fall below the one-crossover-per-chromosome-arm threshold. In addition, for the 17 mammalian species with crossover rate estimates available from multiple experimental methods, there is strong consensus on the crossover-to-karyotype relationship across approaches (Table S1). Nonetheless, MLH1-based crossover counts are almost certainly underestimates of the true crossover rate (Hollingsworth and Brill 2004; Holloway et al. 2008).

Figure 5.

The phylogenetic distribution of the meiotic constraint on crossing over in mammals. An informal supertree was assembled to depict the evolutionary relationships among taxa for which crossover frequency estimates are available. Note that branch lengths on this cladogram are not proportional to evolutionary divergence and represent only the relations among taxa. Poorly resolved species relationships are represented as polytomies. The column to the right of each species name denotes whether available recombination estimates for that species derive from analysis of chiasma counts (red dot), MLH1 foci counts (blue dot), or genetic linkage maps (green dot). Lineages leading to species with fewer crossovers than chromosome arms are shown in red. The names of species with karyotypes composed of only acrocentric chromosomes are shown in blue.

To gain a window onto the evolution of the meiotic constraint on recombination, I investigated the phylogenetic relationships among species with variable chromosomal crossover distributions at meiosis (Figure 5). Several important findings emerge from analyzing these data in an evolutionary context. First, most mammalian species, including a majority of the most ancestral lineages, have mean crossover counts in excess of the number of autosomal chromosome arms in the haploid genome (aFN/2). These patterns suggest that the meiotic constraint on recombination in the mammalian common ancestor was likely at the level of the chromosome arm. By extension, most taxa with only a single crossover per homologous chromosome pair at meiosis have emerged recently in mammalian evolution.

A second key observation is that species with fewer crossovers than chromosome arms are nonrandomly distributed across the tree, with multiple independent transitions from one crossover per chromosome arm to one crossover per chromosome evident in mammals. For example, all members of the Cricetidae family (voles, hamsters, and lemmings) are characterized by nXO < aFN/2. Three of the four macaque species analyzed exhibit fewer crossovers per meiosis than chromosome arms, pointing to a possible relaxation of the chromosomal constraint on recombination in the common ancestor of Macaca. The cotton-top tamarin (Saguinus oedipus) also conforms to this trend, revealing multiple independent shifts in the chromosomal distribution of crossovers within the primate clade alone. Two of the four Caniformia species in this analysis (Mustela vison and Vulpes vulpes) also fall short of the one-crossover-per-chromosome-arm threshold. Interestingly, the karyotype of the other two species analyzed in this clade, the domestic dog and gray wolf, are composed exclusively of acrocentric chromosomes. These species may only appear to superficially meet the one-per-chromosome-arm criterion because the number of chromosome arms and chromosomes are equivalent. Thus, the common ancestor of Caniformia may have also experienced a relaxation in the chromosomal constraint on meiotic recombination.

It is noteworthy that two of the clades characterized by nXO < aFN/2—Cricetidae and Caniformia—harbor species with all acrocentric karyotypes [domestic dog and gray wolf in Caniformia and snow voles (genus Chionomys; recombination data not available) in Cricetidae]. In general, the emergence of acrocentric karyotypes in mammals appears to be preceded by a relaxation of the distributional constraint to one crossover per chromosome. A further hint at this trend is apparent in the murine rodents. The most basal species analyzed in this clade—the Norway rat (Rattus norvegicus)—has fewer crossovers than chromosome arms, suggesting that the minimum number of crossover events in the common ancestor of Murinae was defined by the haploid chromosome number. The remaining murine rodents analyzed have acrocentric karyotypes, erasing the distinction between the number of crossovers per chromosome arm and per chromosome. Rigorous tests of coevolution between the chromosomal distribution of crossovers and basic features of karyotype structure, including acrocentric status, will require the application of evolutionary modeling and phylogenetic comparative methods to the dataset compiled here. In the absence of DNA sequence datasets that can be used to derive branch lengths for these taxa, this objective lies outside the scope of the current analysis and represents an open area for future investigations.

Determinants of the chromosomal distribution of meiotic crossovers

Multiple independent evolutionary shifts in the chromosome-scale distribution of crossovers have occurred during mammalian evolution, raising the question of how changes from one crossover per chromosome arm to one per chromosome are rendered at a mechanistic level.

One very likely explanation is that the lower bound on meiotic recombination is tied to key properties of an organism’s karyotype, including chromosome number, size, and genomic composition. Among the 112 mammalian species in this dataset, diploid chromosome numbers range from 2N = 10 to 2N = 78 and display even greater variation in fundamental number (FN = 17–134). Despite order-of-magnitude differences in chromosome (arm) number, total genome size varies less than twofold among mammals (Bachmann 1972; Redi and Capanna 2012). Consequently, the karyotypes of mammalian species with high chromosome (arm) numbers are dominated by small chromosomes. A single crossover may suffice to ensure proper chromosome segregation on small biarmed chromosomes. Indeed, species with nXO < aFN/2 possess, on average, both higher chromosome and chromosome arm numbers than species with crossover counts in excess of the number of autosomal chromosome arms (Figure S6; Mann–Whitney U-test; haploid chromosome number P = 0.0154; aFN P = 2.25 × 10⁻⁶).

Changes in chromosome content due to the accumulation of heterochromatin may also influence the chromosomal scale of the meiotic crossover constraint. The expansion of heterochromatic regions is a major mechanism for karyotype evolution in mammals (Pathak et al. 1973; Baverstock et al. 1977), but the local chromatin environment of these regions is refractory to recombination (Beadle 1932; Stack 1984; Laurie and Hultén 1985; Choo 1998). Indeed, as noted above, chromosome arms dominated by heterochromatin are commonly achiasmate in North American voles, and several other mammalian species falling below the one-crossover-per-chromosome threshold also harbor large and rapidly evolving heterochromatic regions (Pathak et al. 1973; Popescu and DePaolo 1980; Patton and Sherwood 1982; Modi 1987b). Although the accumulation of heterochromatin-dense chromosome arms that escape crossing over may provide a mechanism for shifts in the chromosomal scale of the meiotic crossover constraint, this common mode of karyotype evolution has likely had limited influence on the overall frequency of recombination in mammalian genomes (Pardo-Manuel De Villena and Sapienza 2001). That is, the accumulation of recombination-inert heterochromatic sequence is not apt to promote additional crossovers in a genome, even though heterochromatin composition may determine whether the crossover distribution is defined by a minimum of one crossover per chromosome arm or one crossover per chromosome.

In addition to chromosome size and architecture, species differences in kinetochore structure could drive the evolution of the chromosomal requirement for crossing over. The kinetochore is a multiprotein complex that assembles on centromeres to link chromosomes to the spindle (Przewloka and Glover 2009). Differences in kinetochore size or protein makeup could alter the number or strength of microtubule attachments, translating into unique biomechanical requirements for chiasma number and distribution along chromosomes to counterbalance spindle tension and ensure stable alignment of homologs along the metaphase plate. Heterochromatic satellite sequences are known to serve as anchor points for the kinetochore protein scaffold (Vafa et al. 1999; Fukagawa and Earnshaw 2014), and large centromeres and/or adjacent blocks of heterochromatin may provide expansive terrain for the attachment of microtubule fibers. Although kinetochore sizes are known to differ between species (Cherry et al. 1989) and even between chromosomes (Cherry and Johnston 1987), it remains unknown whether this variation directly influences the crossover requirement for meiotic chromosome segregation.

Conclusions

I have shown that house mice and North American voles possess distinct chromosome-scale crossover distributions at meiosis. In a phylogenetic metaanalysis of recombination and karyotype data, I extended this conclusion to show that the number of crossovers has dropped below the one-per-chromosome-arm threshold multiple independent times during mammalian evolution. Although prior studies have suggested that mammalian crossover rates are constrained by a requirement of one crossover per chromosome arm (Pardo-Manuel De Villena and Sapienza 2001; Segura et al. 2013), the data presented here indicate that the broad-scale constraints on meiotic recombination are not so simply defined.

These findings add an additional layer of complexity to our growing understanding of the causes of recombination rate variation and the mechanisms contributing to their evolution. At the maximum, recombination rates are presumably held in check by the essential need to maintain genome integrity (Coop and Przeworski 2007). At the lower extreme, the minimum number of crossovers is constrained by their biological roles in chromosome segregation at meiosis (Mather 1938). Here, I have demonstrated that this lower bound is not a simple function of chromosome or chromosome arm number, but rather a trait that itself evolves among species. An intriguing yet unaddressed possibility is that this chromosomal constraint also varies among individuals, potentially rendering certain recombination profiles more liable to induce nondisjunction in different genetic backgrounds.