GsMTx4: Mechanism of Inhibiting Mechanosensitive Ion Channels

Abstract

GsMTx4 is a spider venom peptide that inhibits cationic mechanosensitive channels (MSCs). It has six lysine residues that have been proposed to affect membrane binding. We synthesized six analogs with single lysine-to-glutamate substitutions and tested them against Piezo1 channels in outside-out patches and independently measured lipid binding. Four analogs had ∼20% lower efficacy than the wild-type (WT) peptide. The equilibrium constants calculated from the rates of inhibition and washout did not correlate with the changes in inhibition. The lipid association strength of the WT GsMTx4 and the analogs was determined by tryptophan autofluorescence quenching and isothermal calorimetry with membrane vesicles and showed no significant differences in binding energy. Tryptophan fluorescence-quenching assays showed that both WT and analog peptides bound superficially near the lipid-water interface, although analogs penetrated deeper. Peptide-lipid association, as a function of lipid surface pressure, was investigated in Langmuir monolayers. The peptides occupied a large fraction of the expanded monolayer area, but that fraction was reduced by peptide expulsion as the pressure approached the monolayer-bilayer equivalence pressure. Analogs with compromised efficacy had pressure-area isotherms with steeper slopes in this region, suggesting tighter peptide association. The pressure-dependent redistribution of peptide between “deep” and “shallow” binding modes was supported by molecular dynamics (MD) simulations of the peptide-monolayer system under different area constraints. These data suggest a model placing GsMTx4 at the membrane surface, where it is stabilized by the lysines, and occupying a small fraction of the surface area in unstressed membranes. When applied tension reduces lateral pressure in the lipids, the peptides penetrate deeper acting as “area reservoirs” leading to partial relaxation of the outer monolayer, thereby reducing the effective magnitude of stimulus acting on the MSC gate.

Introduction

GsMTx4 is a gating modifier peptide from spider venom (1, 2), notable for its selective inhibition of cation-permeable mechanosensitive channels (MSCs) belonging to the Piezo (3) and TRP (4, 5) channel families. It has become an important pharmacological tool for identifying the role of these excitatory MSCs in normal physiology and pathology (6, 7, 8). GsMTx4 is similar to many other channel-active peptides isolated from spider venom, which are small (3–5 kD) amphipathic molecules built on a conserved inhibitory cysteine-knot (ICK) backbone (9). However, it is unique because 1) of its high potency for inhibiting mechanosensitive channels and 2) inhibition by GsMTx4 is not stereospecific, i.e., both its enantiomers (L- and D-form) inhibit MSCs (1), a feature not observed with other ICK peptides (10).

All ICK peptides are amphipathic, having a hydrophobic face that can promote interfacial adsorption to the lipid bilayer (10, 11). In the membrane-absorbed state, many of these peptides modify channel kinetics (1, 12) by directly binding to channel gating elements (13, 14, 15) rather than occluding the channel pore. GsMTx4’s lack of stereospecificity, but local effect on the channel (within a Debye length of the channel pore), suggests a different mechanism of inhibition than other ICK peptides. MSCs, like Piezo channels, appear to be activated by bilayer tension (16), and tension modulates bilayer density (17) and thickness (18). This prompted the current model of GsMTx4 inhibition, suggesting it acts by modulating local membrane tension near the MSCs. However, because all ICK peptides are amphipathic, we wanted to know why GsMTx4 is more potent at inhibiting MSCs.

GsMTx4 is highly positively charged (+5) (19) compared with other ICK peptides, primarily because of its six lysine residues. However, surprisingly, it only has a weak preference for anionic over zwitterionic lipids (11). Other ICK peptides, like GsMTx1 and SGTx1, with lower net positive charge (+3), show a strong preference for anionic lipids. Despite GsMTx4’s weak selectivity for anionic lipids, its partitioning energies were comparable with the peptides cited above (11, 20). GsMTx4’s high energy of partitioning into either lipid type may be associated with its relatively high hydrophobicity and lysine content compared with other ICK peptides; lysine plays an important role in peptide-lipid interactions (21, 22).

Partitioning energies are only one factor affecting inhibition of channels by ICK peptides. The depth of peptide penetration following absorption is an important modulator of interactions with both intramembrane and extracellular gating elements (23), and the depth of penetration is dependent on membrane tension (24). Based on molecular dynamics (MD) modeling, two binding modes have been suggested for how GsMTx4 is positioned in the bilayer. In one mode, there is an energy minima at the interfacial boundary (25, 26, 27, 28, 29). A second less-occupied mode was found where the peptides bound deeper and interacted with both monolayers simultaneously (27, 29, 30). Although GsMTx4 can occupy both of these modes, the simulations suggest it is less likely to occupy the deeper mode than other ICK peptides (25, 30). The relatively stronger interaction of GsMTx4 with the membrane interface may inhibit occupancy of the deeper mode in relaxed bilayers (25, 29, 30).

Although the hydrophobic face of ICK peptides is clearly important for membrane insertion, the role played by GsMTx4’s high lysine content could be important as well. MD simulations suggest the positive charge on GsMTx4 is critical in setting the depth of penetration and the lipid displacement (29). We experimentally investigated the role of charge by synthesizing six different lysine to glutamate (K-E) analogs, resulting in a net charge reduction to +3 compared with the +5 on the wild-type (WT). The functional differences among the K-E analogs were determined by the magnitude and kinetics of their inhibition of Piezo1 channels. Four of these analogs showed compromised steady-state inhibition that was not due to changes in the kinetics of inhibition or the lipid partitioning energies. However, using different lipid binding assays, we found that all peptides bound superficially to bilayers but penetrated deeper in expanded monolayers, occupying greater surface area and producing a “tension clamping” effect. The four compromised analogs showed reduced expulsion as the monolayer was compressed, thus reducing the area reservoir for tension clamping. To our knowledge, these results support a new model of peptide inhibition where 1) the distribution of charges influences GsMTx4’s orientation and depth in the relaxed membranes and 2) the equilibrium between shallow and more deeply bound modes is dependent on bilayer tension changes (17).

Materials and Methods

Peptide synthesis and folding

The WT GsMTx4 (synthetic L amino acid form) and lysine to glutamate substituted analogs were chemically synthesized and folded by CSBio (Menlo Park, CA) according to published procedures (19). Six analog peptides were generated, which we called K8E, K15E, K20E, K22E, K25E, and K28E.

Circular dichroism

Circular dichroism (CD) measurements were performed on peptides at 2 μM using an upgraded Jasco-720 spectropolarimeter (Japan Spectroscopic, Tokyo, Japan). Normally 80–100 scans were recorded to increase the signal-to-noise ratio at 200–260 nm using a 1 nm optical path. Spectra were corrected for background scattering by subtracting a vesicle-only spectra. Temperature was controlled by a Peltier unit.

Electrophysiology

Human embryonic kidney cell (HEK)293 cells were transfected with 0.5–1 μg of mouse Piezo1 cDNA expression vector that coexpressed Green Fluorescent Protein (GFP; gift from A. Patapoutian, Scripps Research Institute, La Jolla, CA) (3). Mechanically activated currents were recorded from outside-out patches with an Axopatch 200B amplifier (Axon Instruments, Sunnyvale, CA). An HSPC-1 pressure clamp (ALA Scientific Instruments, Farmingdale, NY) was used to apply the stimulus that consisted of a train of 500 ms positive pressure steps separated by 1500 ms relaxation intervals. We analyzed Piezo patch currents that showed consistent responses within 40–90 mmHg. This range showed the most consistent activation of currents despite some variability in factors such as patch diameter and extent of cytoskeletal disruption (31). Integrated current (i.e., the total charge transferred) was used because this measure tended to smooth the data between pressure steps compared with the large step-to-step variations observed in peak current measurements. Outside-out patch formation also caused labile Piezo inactivation so we did not analyze the inactivation kinetics. From observing more than 100 outside-out patch currents, none of the analogs showed an obvious effect on inactivation kinetics. Individual peptides were superfused onto the patches using an ALA MP285 perfusion system. Electrical recordings (data were acquired at 10 kHz and filtered online at 2 kHz), stimulus generation, and peptide applications were controlled by QuBio software, and analyzed using QuB Express (http://www.qub.buffalo.edu/wiki/index.php/QUB_Express) and Origin 8.5. For Piezo1 channels, the extracellular solution contained 145 mM NaCl, 5 mM KCl, 3 mM MgCl₂, 0.1 mM CaCl₂, and 10 mM HEPES (pH 7.4). The pipette solution contained 133 mM CsCl, 10 mM HEPES (pH 7.4). The inhibition produced by application of the peptides was followed by ∼60 s of washout, followed by application of WT GsMTx4 as a control. Peptide solutions were made fresh daily, and the peptide concentration emerging from the perfusion tip was sampled and quantified with an Agilent (Santa Clara, CA) 1100 series HPLC before use.

Kinetic rate constants and KD calculation

We modeled the current decay and recovery rates with either double or single exponential equations, respectively (see Fig. 2 B). These rates were used to calculate the association (k_a) and dissociation (k_d) constants (see Fig. 2 D). The k_a1 values shown in Fig. 2 D are calculated from the shorter time constants that represented >80% of the total reduction in current amplitude (both WT and analogs). There were no statistical differences in the k_a2 values calculated from the longer time constants (Fig. S5 in the Supporting Material). The ratio of k_a1/k_d for each peptide was used to calculate the effective equilibrium dissociation constant (K_D, see Fig. 2 E).

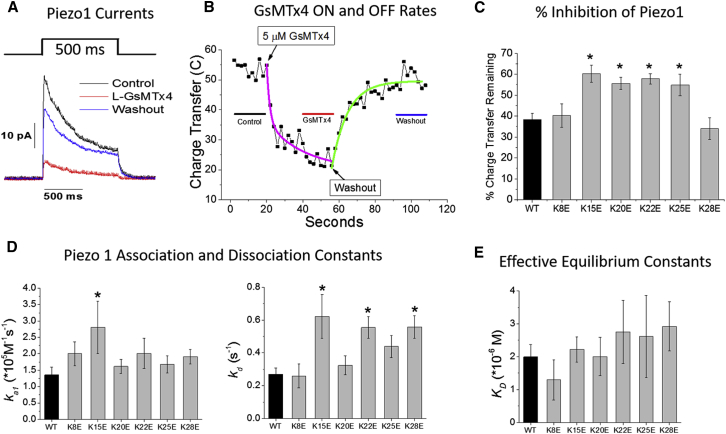
Figure 2.

(A) Typical averaged currents in an outside-out patch from a cell expressing Piezo1, before (black), during (red), and after (blue) application of 5 μM WT GsMTx4. Patch currents were recorded at −50 mV pipette potential. (B) A typical experiment (not the same as A) shows the integrated current (charge transfer) levels diminishing over time after GsMTx4 application and recovering after washout. The regions from which the charge transfer levels were determined are indicated by lines in (B) with colors coordinated to the traces in (A). The mean reduction of Piezo 1 charge transfer by the different peptides is shown in (C). The average charge transfer from the 500 ms steps before peptide application (control line in B) were set to 100% to normalize the inhibition of different patches. The percent values in (C) represent the average fractional reduction in steady-state charge-transfer determined from the region indicated by the red line in (B). For a summary of individual patch data, see Fig. S4. Asterisks represent significantly different means at α = 0.05. Time constants were determined by modeling the decay and recovery phases with exponential fits (magenta and green curves, respectively, in B). The net association (k_a) and dissociation (k_d) rate constants for inhibition of Piezo1 (D) were calculated from the first-order time constants derived from the exponential fits to the data (mean constant values ± SE). The number of patches analyzed to compute the mean k_as was WT = 38, K8E = 15, K15E = 14, K20E = 19, K22E = 16, K25E = 15, and K28E = 21; and for k_ds the numbers were WT = 28, K8E = 17, K15E = 16, K20E = 15, K22E = 18, K25E = 14, and K28E = 19. Control WT GsMTx4 constants are shown in black, analogs in gray. (E) The calculated effective equilibrium constants (K_D) ± SE are shown. Analogs that were significantly different (α = 0.05) from WT are denoted by an ^∗. To see this figure in color, go online.

Statistics

The goodness of the exponential fits to the decay and recovery phases for individual patches were assessed using the value-adjusted r-square in Origin 8.5 software, and time constants from fits with R² > 0.7 were used to calculate the association (k_a) and dissociation (k_d) constants. This generally included >90% of the patches tested. The k_a and k_d from multiple patches were averaged for each analog and statistically different values for analogs versus WT constants were evaluated by one-way ANOVA using both the Tukey and Bonferroni test methods (α = 0.05). We determined the 90% confidence intervals and calculated SE for the k_d/k_a ratio (the effective equilibrium constants (K_D)) using Fieller’s method (32) where the means for k_a and k_d approximated Gaussian distributions. Statistically significant differences between the percent channel inhibition for the WT and analog peptides was evaluated by one-way ANOVA (α = 0.05).

Fluorescence measurements of membrane penetration

The interaction between these tryptophan-containing peptides and lipid vesicles was studied as described in (33). Briefly, 2 μM peptide was dissolved in 10 mM NaPO₄ buffer (pH 7.4). Tryptophan (Trp) fluorescence was measured using a SPEX Flurolog FL 3-22 steady-state fluorescence spectrometer (Jobin Yvon, Edison, NJ) equipped with double-grating excitation and emission monochromators. Trp residues were excited at 280 nm and emission spectra were recorded between 290 and 500 nm using excitation and emission spectral slits of 2 and 4 nm, respectively. For acrylamide quenching, 295 nm excitation was used to reduce inner filter effects. Measurements were made at 25°C in 2 × 10 mm cuvettes oriented perpendicular to the excitation beam. Quenching of Trp emission was measured in the presence of large unilamellar vesicles (LUVs) composed of 1-palmitoyl-2-oleoyl-sn-glycero-3-phosphocholine (POPC) and 1-palmitoyl-2-oleoyl-sn-glycero-3-phosphoglycerol (POPG) (Avanti Polar Lipids, Alabaster, AL). All spectra were recorded after equilibration of the sample. Corrections for background and the fitting of spectra were performed as previously described (34). It should be noted that, due to peptide aggregation, a two-state model (transitions between soluble and membrane bound pools) is insufficient to explain the differences in the peak and absolute Trp fluorescence intensity. Shifts in the peak fluorescence and changes in I_max are likely a combination of transitioning among multimer, monomer, and membrane-associated pools, each with unique Trp fluorescence quenching properties. However, the mole fraction partition coefficient is not dependent on the absolute I_max value (33), so we were able to calculate the free energy of partitioning from the relative fluorescence intensity changes upon lipid titration.

For depth-dependent quenching we used a series of brominated lipids (Avanti Polar Lipids) at positions 6,7; 9,10; and 11,12 along one of the acyl chains (6,7; 9,10; and 11,12-brominated phosphatidylcholine (BrPCs)). Depth-dependent quenching profiles were generated by taking the ratio of tryptophan intensity F, to that in the absence of quenchers, F₀, as a function of the depth of the bromine. Because the maxima of the quenching profile are shallower than the depths of available bromolipids, we used a priori constraints to estimate the depth. We used the method of distribution analysis (35, 36) that approximates the quenching profile as Gaussian. Because the quenching profile is poorly defined, no unique solution exists when all three fitting parameters are varied. One can estimate the average position assuming a particular width of the distribution. Based on our previous experience with quenching of various peptides, we assumed a value of 5 Å for the dispersion of the Gaussian distribution (37). Note, that narrower distributions would be inconsistent with the presence of the two neighboring tryptophan residues whereas a wider distribution would have little effect on our conclusions.

Langmuir monolayer experiments

All experiments were conducted in a buffer made with 50 mM KCl, 10 mM KPi, titrated to pH 7.2. In both liposome and monolayer experiments, the lipids were composed of POPG-POPC (3:1 mol/mole). The peptides were dissolved into buffer to make stock solutions of 150 μM and then stored for no more than 2 days at 4°C; samples were never frozen. Langmuir monolayer experiments were done with a two-barrier, rectangular (22 × 6 cm) trough (Kibron MicroTrough XS; Helsinki, Finland) housed in an AirClean enclosure. A Dyneprobe (Kibron) metal alloy needle was used as the Wilhelmy plate. A POPG-POPC mixture (both from Avanti Polar Lipids) was dissolved in chloroform to a final concentration of 0.2 mg/ml. The lipids were deposited onto the subphase using a gastight 50 μL Hamilton syringe; the measurements were started after 10 min to allow the solvent to evaporate. Pressure/area (π-A) isotherms of monolayers were measured at room temperature (∼20°C) from 114 to 18 cm² at a barrier rate of 20 mm/min. For each analog, isotherms were measured at least three times. The inverse lateral compressibility moduli Cs⁻¹ = A(dπ/dA) were determined from the isotherms (38).

Isothermal titration calorimetry

POPG-POPC (3:1) liposomes were prepared by rehydrating 2 mg of dried lipid mixture in a round-bottom glass tube with buffer made with 50 mM KCl, 10 mM KPi, titrated to pH 7.2. They were then vortexed and sonicated in a Branson-type bath sonicator for 1 min. Isothermal calorimetry (ITC) experiments were carried out using a Microcal (Malvern Instruments, Malvern, UK) VP-ITC Microcalorimeter. The heat of the high peptide dilution predefined the titration configuration in which 10–30 μM peptide was in the cell and a 0.3–1 mM liposome suspension was in the syringe. Injections of 20 μL were spaced by 300 s intervals. For all peptides, interactions with POPG-POPC liposomes were exothermic. After minor baseline adjustments, the thermograms were fitted with single-site binding curves to determine the mean parameters (usually defined by the major low-affinity population of sites). Then, guided by this fit, we fit to a two-site model. The two-site fittings were significantly better suggesting the presence of a second, higher affinity population of sites.

Simulations of GsMTx4 interaction with monolayer

For the POPC monolayer simulations, the united-atom parameters GROMOS53A6 (39) for protein and GROMOS53A6_L (40) for POPC were used. The simulation programs, conditions and H₂O models were basically the same as those used in (41), except for the surface tension coupling used to control the pressure of the monolayer. The system was comprised of a monolayer of 40 POPC, one peptide (WT or K15E) and 1460 H₂O along with Cl ions that neutralized the positive charges of the peptides. The basic configuration of the system was similar to the previous reports (42, 43). Twenty different initial structures were prepared using the bilayer/peptide structures randomly sampled from our recent 400 ns trajectories with bilayers (41) and initially subjected to a 50 ns run with the surface tension (γ_s) coupling at 50 mN/m that brought the area per lipid to ∼0.64 nm² in the monolayer. These twenty runs for both WT and K15E were continued with 20 mN/m stepwise increases and decreases in the target value of γ_s, i.e., 70 and 90 mN/m, and 30, 10, 0, and −10 mN/m runs, respectively, with the time length being 40 ns for each except for the run with γ_s,= −10 mN/m, which was extended to 100 ns. This procedure controlled γ_s with precision of SD < 0.1 mN/m in all cases. The final 20 ns of each run were used to compute the monolayer area and the peptide height (z-position of the center of mass). Due to this relatively short equilibration period at each tension value, we acknowledge that our simulation data, shown in Fig. 7, should rather be regarded as a qualitative one, which needs to be reexamined using longer equilibration runs (e.g., ∼1 μs). For the height analysis, POPC phosphorus atoms were used as the references, but the phosphorus atoms located within 1 nm of any peptide atoms were not used due to local perturbation of lipids headgroups specific to the peptides that made the WT-versus-K15E comparison difficult.

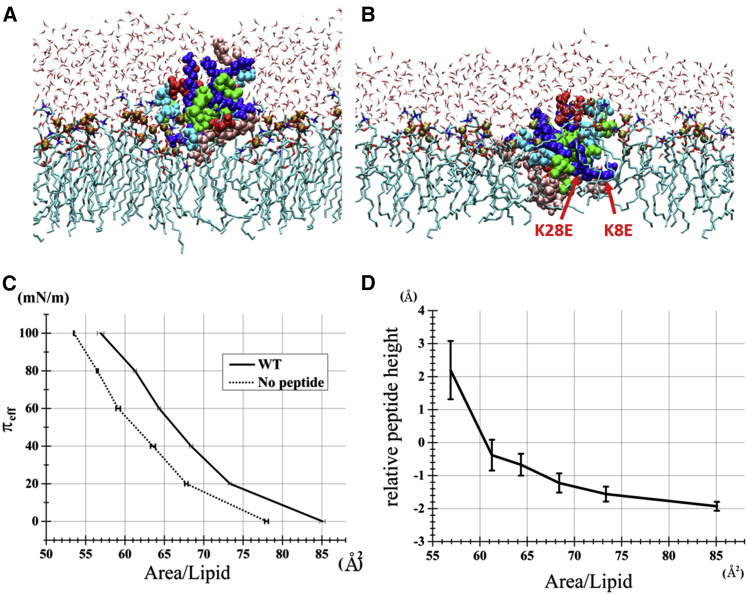
Figure 7.

Representative structures of the POPC monolayer simulations. (A) A snapshot from a trajectory with the π_eff of 100 mN/m (i.e., surface tension coupled to −10 mN/m). WT atoms are shown as Van der Waals spheres with the amino acid (single letter designation) residues colored in pink (W, F), green (A, C, L, P), blue (R, K), cyan (S, T, N, G), and red (D, E). POPC phosphorus atoms are highlighted in large ocher spheres. Other atoms/groups are presented as follows: lime licorice (POPC acyl chains), cyan, red, and blue licorice (carbon, oxygen, and nitrogen atoms of POPC headgroup), and thin licorice (water molecules). (B) A snapshot from the simulations with π_eff = 0 mN/m (i.e., surface tension coupled to 90 mN/m), with the same representation scheme as in (A). The two lysines that when changed to glutamates produced uncompromised analogs are indicated showing greater depth under higher tension. (C) The relationship between the π_eff and the membrane area in the WT-containing (and control) POPC monolayer simulations. Vertical axis shows the π_eff derived from the monolayer surface tension as described in Materials and Methods. The horizontal axis shows the total area of the POPC monolayer of the simulation box after simply being divided by the number of the POPC molecules (i.e., 40). The mean ± SE of the 20 trajectories that were subjected to the stepwise compression/expansions are plotted. (D) Position along the membrane normal (penetration depth) of the WT peptide as a function of the membrane area. Horizontal axis shows the total area of the POPC monolayer of the simulation box simply divided by the number of the POPC molecules (i.e., 40). Vertical axis shows the height of WT center of mass relative to the mean z-coordinate of phosphorus atoms of POPC calculated as described in Materials and Methods. The mean ± SE of the 20 trajectories are plotted. To see this figure in color, go online.

Of note, it is not straightforward to compare the computed surface tension γ_s with the absolute experimental π values. In theory, the following formula allows us to convert γ_s to π: π = γ_wv − γ_m and the γ_m = γ_s − γ_wv, where, γ_m is the monolayer/water interfacial tension and γ_wv is the surface tension at the water/vacuum interface (44). However, the simulated surface tensions at the water/vacuum interface actually differ considerably from the experimental values due to defects in the water models (43). To better compare the γ_s-area curve with the experimental π-area curve, we chose the method described in Baoukina et al. (43), with which correction to the γ_wv was introduced to obtain an effective γ_wv^∗ so that the two curves can be compared. We compared our computed γ_s-area curve with the experimental curve of a POPC monolayer (45) and chose γ_wv^∗ = 45 mN/m to convert γ_s to the effective monolayer pressure π_eff. This choice was close to the suggestion of 47 mN/m based on the dipalmitoylphosphatidylcholine (DPPC) simulations (43).

Results

Structural analysis of lysine analogs

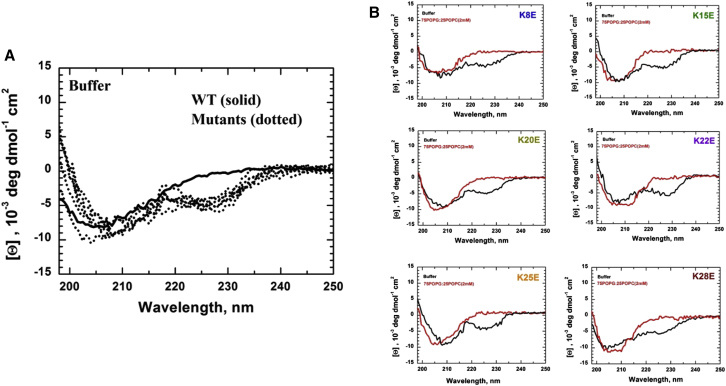
At a pH of 7.4, lysine residues are protonated and contribute substantially to the net positive charge on GsMTx4. MD simulations predict electrostatic interactions between the lysine residues and the carbonyl/phosphates of lipid head groups (25, 29). We made six single substitutions of lysine-to-glutamate generating analogs with a net charge of +3. Mass spectrometry confirmed the formation of peptides of expected mass containing three disulfide bonds. As an additional check of the secondary structure, we measured the CD spectra of the peptides. The L-GsMTx4 spectrum was relatively unchanged by the addition of either 100% POPC (zwitterionic) or 3:1 POPG:POPC (negatively charged) LUVs. A general increase in intensity was observed when bound to POPG:POPC, likely due to stabilization of the secondary structure (Fig. S1 A). The spectrum of D-GsMTx4, which is inverted with respect to that of L-GsMTx4, is also shown. The in-solution spectrum of the WT was similar at all wavelengths to that of the analogs except for a distinct negative peak at ∼228 nm for every analog (Fig. 1 A). However, the spectra of the WT and analogs in POPG:POPC LUVs were similar and lacked the 228 nm peak (Fig. 1 B). The peak reappeared when the analogs were added to POPC LUVs (Fig. S1 B). The 228 nm peak may arise from the formation of aggregates due to interpeptide hydrophobic interactions in solution or when weakly bound to membranes (see Figs. S2 and S3). If so, then a strong association with negatively charged membranes, such as the POPG:POPC LUVs, may dissociate aggregates.

Figure 1.

(A) Representative CD spectrum from all GsMTx4 peptides (2 μM) shows a distinct negative peak at 228 nm for all K-E analogs compared with WT. Other regions of the spectrum are similar to WT. (B) CD spectra before and after binding to negatively charged POPG-POPC lipid vesicles is shown. When bound, the 228 nm peak disappears and the spectrum more closely resembles that of the WT. To see this figure in color, go online.

Functional analysis of MSC inhibition

All of the peptides (5 μM) were tested on outside-out patches from HEK cells transfected with mouse Piezo1 cDNA (Fig. 2). We measured the charge transfer (integrated current over the entire pressure step) elicited by the pressure steps before, during perfusion with ∼5 μM peptide (until the charge transfer reached steady-state), and during washout (Fig. 2 B). Charge transfer recovery during washout did not return to 100% due to channel rundown, but the recovery level was similar for all analogs and consistent with control levels of rundown (Fig. S4, A and B). The potency of each peptide is represented by the percent charge transfer remaining (Fig. 2 C) measured at the steady-state level of inhibition during peptide application (Fig. 2 B). The summarized results from all patches are shown in Fig. S4 C. The WT peptide reduced Piezo1-mediated charge transfer to 38% of its initial levels (Fig. 2 C). K8E and K28E had the same potency as WT. However, four analogs (K15E, K20E, K22E, and K25E) inhibited Piezo1 less than the WT showing that the effect was not one of net peptide charge, but of the location of the charges. These four analogs reduced Piezo1 charge transfer by a similar amount (to 55–60% of initial levels), suggesting that these lysines share some functional similarity in inhibition.

To determine if differences in the binding kinetics underlie the losses in activities of the analogs, we calculated effective equilibrium dissociation constants (K_D) from the charge transfer decay and recovery rates as determined with either a double or single exponential model, respectively (Fig. 2, B, D, and E; see Materials and Methods). The K_D for the WT peptide determined by this method (2.0 ± 0.6 μM) was in good agreement with the K_D (2.0 ± 0.2 μM) calculated from a Hill equation fit to the concentration dependence of inhibition of Piezo1 (Fig. S6). The k_a values for the analogs were generally similar to WT, with the exception of K15E, which was significantly greater (shorter time constant). Three of the analogs had k_d values significantly greater than WT (longer unbinding time constants), though there was no strong correlation between this rate constant and compromised inhibition. Further, weaker K_D values were not correlated with the compromised analogs suggesting additional steps in the interaction kinetics that were not revealed in this analysis. It should be noted that the Hill fit from the concentration dependence curve in Fig. S6 predicts a Hill coefficient of 1.5 ± 0.2 suggesting some level of cooperativity. These data suggest that K_D represents more than a typical two-state (free/bound) binding reaction.

The generality of GsMTx4 effects on MSCs was determined by testing it on K⁺ selective TREK1 channels expressed in HEK cells. Instead of inhibition, we observed that these channels were potentiated by extracellular GsMTx4 (Fig S7). This suggests that GsMTx4 inhibitory activity is not common to all MSCs probably due to different channel gating mechanisms. Since it was difficult to evaluate the kinetics of potentiation due to the slow washout times and TREK channels being active in resting patches, the analogs were not tested on these channels.

Inhibition of Piezo1 is not influenced by peptide aggregation

Differences in peptide aggregation are another factor that could affect inhibition by modulating the effective concentration of the peptides. The peptides showed different degrees of aggregation, which were especially prevalent at higher concentrations (Fig. S2). Some analogs appear to form larger aggregate sizes than the WT (Figs. S2 and S3), although aggregation appeared to decrease substantially at concentrations used in the Piezo1 experiments (Fig. S3). In addition, the K28E analog, that has a potency equivalent to WT, showed similar aggregation properties to the compromised analog K15E (Fig. S3). These data suggest that, in general, larger multimeric peptide aggregates do not play a significant role in the kinetics or magnitude of Piezo1 inhibition.

Peptide-lipid affinity

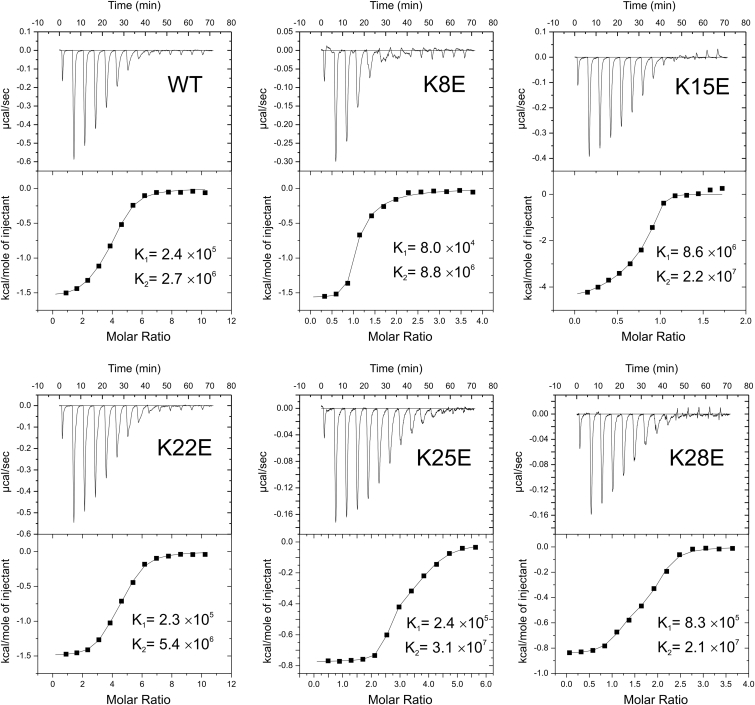
To isolate the peptide-lipid binding kinetics from the more complicated channel inhibition kinetics we performed two different lipid vesicle binding assays. The first method determined the binding energy of the peptides to POPG:POPC vesicles by ITC. The resulting isotherms were fitted with a two-site binding model. The fitted isotherms and their corresponding parameters for WT and five of the analogs are shown in Fig. 3 and Table S1. The interactions of the peptides with POPG-POPC liposomes were always exothermic, with the exception of some low-amplitude events at the end of titration for K15E and K28E. In most cases, the smaller of the two binding constants was between 8 × 10⁴ and 8 × 10⁵ M⁻¹ (Table S1), corresponding to interaction energies (ΔG) of −28 to −32 kJ/mol. However, curve-fitting identified the presence of a second higher affinity site(s) with constants in the range of 2 × 10⁶ to 3 × 10⁷ M⁻¹ of comparable density, as shown by N₁ and N₂. The tight ranges for the negative enthalpies (−0.9 to −1.5 kcal/mol) and positive entropies (20–30 kcal/molK) suggest similarities in the chemistries of binding to both types of sites. K15E had significantly higher binding constants (8.6 × 10⁶ M⁻¹ or −40 kJ/mol), a result that is consistent with the stronger association constant calculated from the inhibition data. It also had a positive ΔH₁ and high ΔS₁, meaning that the low-affinity binding mode is quite different for this analog. K25E had the highest affinity for the second site. The positive entropies probably signify disordering associated with partial desolvation. These experiments do not preclude a larger variety of sites, especially as we recall that the binding site is not chiral specific; however, the model with two sites is sufficient to fit the data.

Figure 3.

Titration isotherms for WT and K-E analogs with different inhibitory capacity suggesting more than one type of binding site in the vesicles. All titrations were done at 30°C with unilamellar POPG:POPC liposomes (n = 3). The peptide was present in the chamber at 20–30 μM and liposomes (0.5–1 mM of lipid) were injected (20 μl per step) from a syringe at 300 s intervals.

We also measured the free energy (ΔG) of peptide partitioning into bilayers by following the equilibrium quenching of the intrinsic tryptophan (Trp) fluorescence with titration by lipid vesicles in the presence of the aqueous quenching agent KI (11, 33) (Fig. S8). The fluorescence intensity of WT GsMTx4 peaked at 365 nm in solution. This emission peak did not change when bound to lipids (Fig. S8 A; Table S2), even though I_max for lipid binding showed a robust association (Fig. S8 B). The K-E analogs all showed a peak emission intensity that was blue-shifted ∼8 nm from WT in solution. This suggests a common effect of net charge on the Trp environment (Fig. S8 A), possibly due to differences in analog aggregation (Figs. S2 and S3) increasing shielding of Trp from the aqueous quencher. The K-E analogs also displayed robust association with lipid vesicles (Fig. S8 B), although, unlike WT, they were further blue-shifted upon binding (Fig. S8 A). This suggests the Trp residues in the analogs were exposed to a more hydrophobic environment, such as deeper membrane penetration than WT (see below).

To determine the affinity of the different peptides to lipids, we fit the vesicle titration curves with a two-state model (adequacy of model is explained in Materials and Methods) and calculated the binding energies from the fluorescence changes (Fig. S8 B; Table S2). As shown previously (11), the binding energy of the WT D and L enantiomers to LUVs is only weakly sensitive to the head group charge (Table S2; Fig. S8 A). The analogs showed a similar lack of sensitivity to lipid headgroup charge, and the corresponding interaction energies with either type of vesicle (27–34 kJ/mol) were consistent with the ITC data. The differences in interaction energies between WT and the analogs were small for the Trp quenching data. This was similar to the ITC data and patch association rates, supporting the idea that membrane binding differences do not significantly contribute differences in inhibition potency. The second higher affinity sites observed in ITC were not seen in the spectroscopic analysis.

Peptide penetration depth in LUV membranes

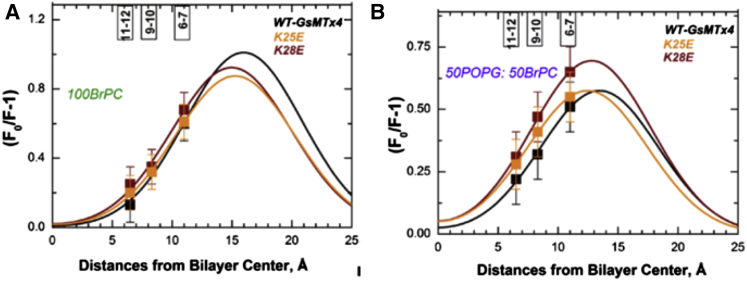
To determine the membrane penetration depth we measured Trp fluorescence quenching using vesicles containing brominated lipids. The bromine atoms were located at different positions on the aliphatic chains between carbons 6-7, 9-10, and 11-12, which were 11, 8, and 6 Å from the bilayer center, respectively. We compared WT GsMTx4 with the K25E and K28E analogs that had compromised and uncompromised activity respectively. Quenching was measured in the presence of either 100% BrPC (zwitterionic) or 50:50% POPG:BrPC (anionic) LUVs (Fig. 4, A and B, respectively). Shallower brominated lipids quenched tryptophan fluorescence better for all peptides, i.e., quenching at C6-7 > C9-10 > C11-12 (Fig. 4 A (100% BrPC) and B (50:50% POPG:BrPC)). These results suggest GsMTx4 WT and the analogs occupy relatively shallow positions in membranes compared with other peptides ((20, 46) and see Discussion). However, because of the shallow depth, no peak in the data was observed for the available brominated lipids. So the data were approximated with a Gaussian fit using a priori constraints based on previous experience with other peptides (see Materials and Methods). Although not significantly different, in anionic membranes, the analogs tended to penetrate deeper than WT.

Figure 4.

Distribution analysis of the depth-dependent fluorescence quenching profiles obtained for WT, K25E, and K28E GsMTx4 in bromolipid containing vesicles. Three bromo PC lipids (brominated at position 6-7, 9-10, and 11-12) were incorporated into LUVs. The quench ratio for the three peptides was plotted with respect to the bromolipid present for both 100BrPC (A) and 50POPG:50BrPC (B). Data were fitted with a Gaussian function (σ was fixed at 5.0 Å). In the presence of 100BrPC LUV, the Gaussian parameters were h_m = 16.0 ± 2.1 Å, s = 1.0 ± 0.1 for WT-GsMTx4; h_m = 15.2 ± 0.4 Å, s = 0.9 ± 0.1 for K25E; and h_m = 14.6 ± 0.9 Å, s = 0.9 ± 0.2 for K28E. In the presence of 50POPG:50BrPC LUV, the Gaussian parameters were h_m = 14.0 ± 0.7 Å, s = 0.6 ± 0.1 for WT-GsMTx4; h_m = 12.4 ± 0.1 Å, s = 0.6 ± 0.1 for K25E; and h_m = 12.7 ± 0.5 Å, s = 0.7 ± 0.1 for K28E. (h_m indicates the most probable location of the probe (here tryptophan) and s is proportional to quenchibility). All peptide tests have n = 3. To see this figure in color, go online.

A second method to assess peptide membrane penetration depth used the Trp fluorescence ratio of the peptides in the presence of aqueous (acrylamide) and membrane resident (10-DN) quenching agents (Fig. S9; Table S3). Although greater aggregation of the analogs tended to exaggerate the estimated depth differences, the calculated Stern-Volmer constants suggest that, in general, the K-E analogs bind deeper than the WT.

Surface pressure-dependent monolayer association

Because GsMTx4 modulates the gating of channels that are sensitive to membrane tension, we characterized membrane binding as a function of lipid packing density in a Langmuir trough. The control pressure-area (π-A) isotherms (Fig. 5, green line) showed no inflections, and rose smoothly with compression until the monolayer collapsed at ∼44 mN/m. The control curve passed 40 mN/m at a molecular area of 68 Å², which corresponds to the lipid density in pure POPC bilayers (68.3 Å²) (47). The surface pressure that packs lipids at this bilayer density is called the monolayer-bilayer equivalence pressure (πB). For many lipids πB is traditionally defined in the range of 30–35 mN/m (48), but according to Brockman (49) the πB for a similar SOPC lipid is near 40.5 mN/m, which is closer to the collapse pressure observed here.

Figure 5.

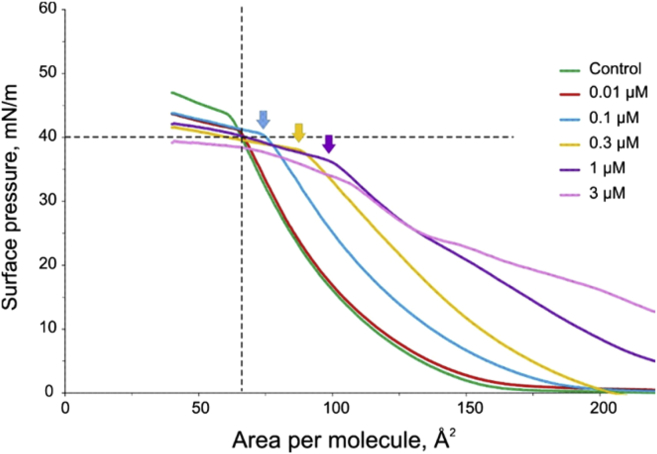
Representative pressure-area isotherms taken with different concentrations of WT GsMTx4 in the subphase. Qualitatively similar interactions of WT GsMTx4 with POPG-POPC monolayers at different concentrations are illustrated by compression isotherms. Visible monolayer “swelling” is observed at subphase peptide concentrations of 0.1 μM and above. The peptide tightly binds to the expanded monolayer. There is no obvious effect of gradual “squeezing-out” of the peptide from the monolayer at higher pressures. The monolayer instead has a tendency to collapse earlier (arrows) as the peptide concentration increases and pressure approaches 37–40 mN/m. In the control, the monolayer collapsed at 43–45 mN/m. The statistics are presented in Table 1. To see this figure in color, go online.

Addition of 0.1 μM WT peptide into the subphase visibly increased surface pressure over a wide range of areas. At 1 μM or above, GsMTx4 increased surface pressure immediately, i.e., without compression, signifying massive intercalation into the monolayer at low packing density. As seen from the family of curves (Fig. 5), the increase in surface pressure is concentration-dependent. Up to ∼37 mN/m, the compression curves lay above the control. However, above that pressure the GsMTx4 curves bent and intersected with the control. The bend could be due to repartitioning of the peptide from the film into the subphase or due to the peptide moving from a deeper penetration depth to the surface. The peptide pressure curves intersect the control curve in the vicinity of 40 mN/m, i.e., near πB. The flattening of the curves at this level probably marks the expulsion of the peptide that continues during a gradual collapse of the monolayer. The area, at which the transition (the break point) occurred, increased with peptide concentration, while the pressure at the bending point decreased. The untreated monolayer seems to withstand higher pressure before collapsing than it can in the presence of peptide. The shape of the curve gets more complicated at 3 μM, so we focused on 1 μM where an inflection occurred but the curves maintained a similar shape.

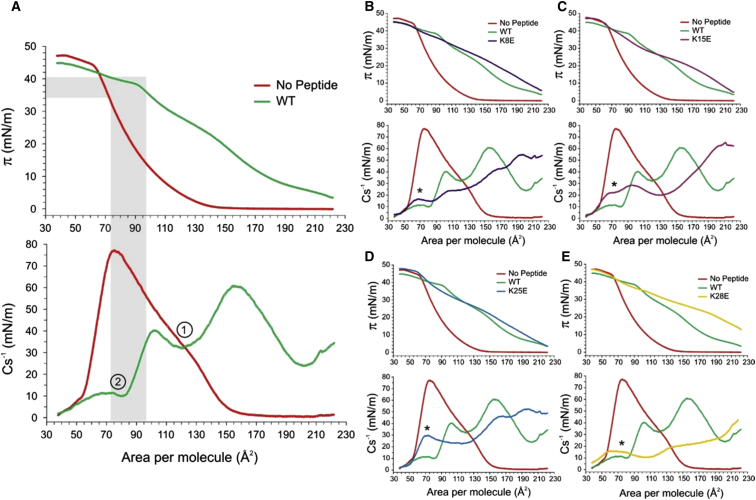
The bending of the isotherm near the monolayer-bilayer equivalence pressure (Fig. 5, arrows) suggests that the peptide-harboring film undergoes a phase transition. This is better illustrated in Fig. 6 A, in which the control monolayer (no peptide) π-A isotherm (top) and the corresponding inverse compressibility Cs⁻¹ plot (bottom), representing stiffness of the film, are compared with the traces measured in the presence of WT GsMTx4. The monolayer stiffness in peptide-free controls shows a monotonic growth with compression, reaching a maximum of ∼80 mN/m in the vicinity of monolayer-bilayer equivalence pressure (πB), followed by a sharp decrease signifying collapse. In contrast, the stiffness of the film in the presence of GsMTx4 exhibits several minima. The first reproducible minimum observed between 110 and 130 Å² coincides with inflection in the middle of the isotherm at π∼25–30 mN/m. This inflection may reflect some compaction, reorientation, or ordering of the peptide in the film. The second minimum observed toward the end of compression (near 80 Å² per molecule) most likely reflects a shift of the peptide from a deeper to a shallower position in the monolayer or to a peripherally absorbed state. This reversible partitioning of the peptide between the surface (see Discussion) and deeper positions suggests that, near the πB, changes in the area will not change lateral pressure/tension significantly, producing a pressure (or tension) clamp effect.

Figure 6.

Compression (π-A) and inverse compressibility modulus (Cs⁻¹ = A × (dπ/dA) isotherms of POPG-POPC monolayers in the presence of 1 μM WT GsMTx4 (A), K8E (B), K15E (C), K25E (D), and K28E (E). WT (green) and control (red) curves are repeated on (B–E) for comparison. The control monolayers, only lipids, compress smoothly with no phase transition. The monolayer collapses near 41.7 ± 2.9 mN/m (n = 3). At the πB taken as 40 mN/m (48), the molecular area per lipid is 66 ± 2.9 Å². Plots of Cs⁻¹ that reflect stiffness of the monolayer are aligned on the area scale with the compression isotherms. For WT GsMTx4 (A), the Cs⁻¹ plot exhibits two characteristic minima (designated 1 and 2). The first minim takes place in the middle of the compression isotherm, whereas the second minimum reached at 60–80 Å²/molecule occurs near the monolayer-bilayer equivalence pressure (πB). The gray shading highlights the area and pressure ranges where the presence of GsMTx4 may exert physiological effects. All analogs with normal activity exhibit low Cs⁻¹ values in that region indicating a facilitated pressure-dependent exchange of the peptide between “deep” and “superficial” states in the film. In the areas marked by the asterisk (B–E), the isotherms for the less-active peptides (K15E and K25E) are elevated higher above the control monolayer curves than K8E and K28E. Instead of a second minimum or low plateau as in WT (green curves), an additional peak in Cs⁻¹ is present for K15E and K25E indicating higher stiffness and no exchange. These traits suggest tighter association of the less-active peptides with the lipid; their position in the film is not as strongly perturbed by pressure. To see this figure in color, go online.

We compared the π-A and Cs⁻¹ isotherms of WT with four analogs having inhibitory activities that were either reduced (K15E and K25E) or unchanged (K8E and K28E) compared with WT (Fig. 6, B–E). The value of Cs₁⁻¹ corresponding to the first minimum (Table 1) is reproducibly higher for WT GsMTx4 than for other analogs suggesting that the peptide resides in a more stable conformation in the expanded monolayer. The compression isotherms for the WT and the analogs with WT level of activity intersect with the control curves near πB and the rigidity curves (Cs⁻¹) show clear second minimums of 13–14 mN/m in that region (see regions marked with asterisk in Fig. 6, B–E, and Table 1). For the compromised K15E, the second Cs⁻¹ minimum was considerably higher (∼22 mN/m), whereas for K25E it was completely absent, though Cs⁻¹ was clearly above ∼25 mN/m in this region. This behavior shows that the peptide residence in the lipid film in this region is strongly dependent on lateral pressure. WT peptide tends to “clamp” the tension near the monolayer-bilayer equivalence pressure. K8E and K28E, which have uncompromised activity, resemble the WT isotherm in that region. In contrast, the partially active peptides K15E and K25E exhibited a different behavior near πB (Fig. 6). Their π-A curves lay above and do not intersect with the control curve. The Cs₁⁻¹ parameter does not show a minimum, rather a local maximum (asterisk). This indicates that the peptides have greater stability in the lipid film near πB and never completely leave it. Based on the compression isotherms, the K15E and K25E analogs will not be able to clamp the pressure (tension) in the peptide exposed monolayer as effectively as WT.

Table 1.

Mechanical Parameters of 75POPG:25POPC Monolayers in the Presence of 1 μM of GsMTx4 and Several K-E Analogs

Analog	Breaking Point		2nd Minimum		1st Minimum		n
	A (Å2)	π (mN/m)	Cs−1 (mN/m)	π (mN/m)	Cs−1 (mN/m)	π (mN/m)
Control	66.0 ± 2.9	41.7 ± 2.9	–	–	–	–	9
WT	89.8 ± 4.1	38.7 ± 0.9	14.3 ± 2.6	39.5 ± 0.5	32.2 ± 4.0	26.1 ± 1.5	10
K8E	51.7 ± 3.2	43.7 ± 0.9	13.7 ± 2.3	36.9 ± 0.9	21.3 ± 2.2	30.8 ± 1.1	8
K15E	52.3 ± 2.2	43.7 ± 0.2	21.8 ± 1.2	40.5 ± 0.6	19.7 ± 1.0	26.4 ± 0.3	4
K25E	59.8 ± 1.8	47.1 ± 0.6	–	–	22.2 ± 1.5	29.3 ± 1.7	8
K28E	56.7 ± 7.2	43.8 ± 1.4	13.3 ± 2.5	35.5 ± 1.0	20.5 ± 1.4	28.8 ± 0.7	4

Breaking point of isotherms signifies the change of slope, which in case of WT shows the early onset of peptide expulsion. For all other analogs an obvious isotherm flattening was associated with collapse. The position of the second minimum of the inverse compressibility parameter Cs₂⁻¹ reflects “softening” of the monolayer associated with pressure-dependent redistribution of the peptide to a shallower position. The second minima are low for the active peptides, but are either higher for the compromised peptides (K15E), or may not even exist (K25E). The first Cs₁⁻¹ minimum presumably reflects compaction and ordering of the peptides within the still expanded monolayer and its position shows no direct correlation with activity. The number of experiments is shown in the last column (n).

To visualize the area occupied by the peptide we replotted the pressure-area curves in area-pressure coordinates and presented the area difference in log scale (Fig. S10). This shows that the logarithmic slope of the monolayer area decrease with pressure reflects the molecular area (a) of the expelled peptide. For WT GsMTx4, a is estimated as 3.3 nm² per molecule, whereas for the compromised K25E a is only 0.87 nm². The reduction of inhibitory activity in different analogs correlates with stable residence of peptides in the monolayer.

Modeling WT peptide association with monolayers supports tension-dependent depth changes

We visualized monolayer-peptide interactions by performing atomistic MD simulations in a peptide-containing POPC monolayer/water/vacuum system. The monolayer surface tension was varied over the range corresponding to effective membrane pressures (π_eff) between 0 and 100 mN/m (as described in Materials and Methods). The monolayer was laterally compressed at the π_eff = 100 mN/m and expanded and reached a rather disordered state at the π_eff = 0 as expected (Fig. 7, A and B). In the area/lipid versus pressure plot (Fig. 7 C), we see that the area/lipid is greater over the entire range of pressures when peptide is present, consistent with the experimental results shown in Fig. 6. Also, in agreement with the experimental data, the area/lipid difference between pure lipid and peptide containing monolayers decreases as pressure increases (7 Å² at 0 mN/m vs. 3.5 Å² at 100 mN/m) representing peptide expulsion and a corresponding loss of peptide area contribution. K15E produced a nearly identical π_eff-area curve to that of the WT-containing monolayer. This is more clearly illustrated when we plot the relation between pressure generated changes in lipid area and peptide depth (Fig. 7 D). WT was located at shallow positions relative to the POPC phosphorus atoms in condensed monolayers (areas/lipid of 54–60 Å²) under high pressures and moved to lower (deeper) positions in expanded monolayers (areas/lipid of 65–85 Å²) at low pressures. Analysis of acyl chains atoms that were in contact with WT supported these results; at high π_eff, the acyl chain atoms in contact with WT exhibited a skewed pattern, that is, there were far more frequent contacts of shallowly positioned atoms (i.e., C2 and C3) relative to C16–C18 atoms. In contrast, at low π_eff the pattern was less skewed. (Fig. S11). Although not statistically significant we observed a trend for K15E to remain deeper than WT peptide at high pressures (data not shown). Longer simulation times would likely produce results more closely aligned with the experimental data. Although the simulated pressure-area curves and corresponding depth changes do not precisely duplicate the experimental data, the results support the general mechanism and qualitatively illustrate the trend for peptide relocation between the deep and shallow positions depending on pressure.

Discussion

GsMTx4 tension-clamping model

The previous model of GsMTx4 inhibition of MSCs (1) postulated that static association of the peptide with a relaxed bilayer distorted the local membrane thickness/curvature near the channel so that greater membrane tension would be required to induce channel gating. However, MD simulations predict that GsMTx4 and other ICK peptides produce only minor local membrane perturbations (25, 29, 30). We showed that in relaxed membranes GsMTx4 is most stable in a shallow, surface-absorbed mode (Fig. 7) (29, 30). When the bilayer is stretched the lateral pressure decreases (17, 50) and GsMTx4 sinks deeper, acting as a local tension/area clamp. Our model suggests that residue changes that shift the binding equilibrium toward deeper penetration in relaxed membranes could lower the efficacy of the peptide as an area buffer when tension increases. Our findings reconcile well with other recent studies on the mechanism of inhibition of peptide toxins (23, 24) and atomistic simulations of GsMTx4 and the K-E analogs (41).

Effects on MSC gating

Eukaryotic MSCs fall into two categories: 1) Piezo- and TRP channels that cause depolarization and 2) the K⁺-selective channels of the 2P domain family that are hyperpolarizing. The crystal structure of 2P domain channels supports a model with the channel mechanosensory elements located on the cytoplasmic leaflet of the membrane (51). Recent cryoelectron microscopic structural data for the Piezo1 channels tentatively place the mechanosensory structures on the outer leaflet (52). Thus, it would be predicted that tension-dependent depth changes of GsMTx4 in the outer leaflet would have different effects on Piezo and 2P domain channels. Our data support this assertion as the external application of GsMTx4 inhibits Piezo but potentiates TREK-1 channels. This property occurs because the inner monolayer is coupled to the outer monolayer (53) due to interdigitation of acyl chains (50) so that tension changes in one monolayer are transferred to the other leaflet. Thus the GsMTx4 mediated area expansion of the outer leaflet could transfer tension to the fixed-area inner monolayer resulting in TREK potentiation. The mechanosensitive bacterial channels MscL and MscS are also sensitive to GsMTx4 in a concentration-dependent manner, showing inhibition at low concentrations and potentiation at high concentration (54). But these channels likely have a different gating mechanism from eukaryotic channels based on their sensitivity to membrane thickness (55, 56) and relatively high activation tensions (MscL and MscS require 7–14 mN/m half activation compared with 3–4 mN/m for Piezo).

The role of lysines in peptide binding

Lysine plays a key role in stabilizing proteins at the membrane interface (21, 22) through energetically favorable interactions with carbonyl and phosphate groups at the bilayer interface. The six lysines on GsMTx4 cover most of the hydrophilic surface and stabilize the shallow binding mode. Simulations also reveal a deep-binding mode where lysines 15, 20, 22, and 25 stabilize the peptide at the water-bilayer interface (25, 41), whereas lysines 8 and 28 interact with the opposing monolayer surface. All analogs with changes to lysines that stabilize the interaction with the outer leaflet (K15E, K20E, K22E, and K25E) showed a similar reduction in potency, suggesting equivalent roles. However, K8E and K28E analogs have uncompromised activity against Piezo1 supporting the model where amino acids that promote deeper insertion will reduce redistribution of the peptide under lateral tension and diminish its effectiveness as an “area reservoir.”

GsMTx4 association with relaxed membranes is strong and shallow, but channel inhibition is not simply a function of lipid binding strength

The energy of binding to lipids was largely unaffected by the K-E analogs revealing an independence from net charge. This supports our previous analysis showing that peptide-lipid dynamics do not reflect total charge and that GsMTx4’s effective charge (Z_eff) is low as estimated from the slope of ΔG versus surface potential (11). This weak contribution of charge to binding suggests that association is dominated by the positive entropic energy (energy change is negative) caused by desolvation. The negative ΔG of desolvating the hydrophobic face is relatively large compared with the electrostatic repulsion from the positive charge of the methylamines on POPC.

Estimates of binding energies for the peptides to POPG:POPC vesicles were in close agreement, primarily between 27–34 kJ/mol by both the tryptophan fluorescence quenching and ITC assays. These differences in binding energies were relatively small and there was no correlation between lower binding energies and decreased Piezo inhibition potency. This implies a more complicated process than a simple two-state binding reaction as we concluded from the effective equilibrium constants derived from the inhibition kinetics. This was also suggested by the presence of at least two binding sites for all peptides in the ITC isotherms. The first constant may represent the binding energy of monomers and/or multimers into the shallow position, whereas the second higher affinity site may represent a deeper bound mode, or the energy for dissolution of multimers (see below). The π-A isotherms from the monolayer experiments also suggest the existence of different binding modes. Absorption to the surface and subsequent deeper penetration has been seen for antimicrobial, viral, and signaling peptide binding (57), though the tension dependence of this property has never been tested. Other factors such as absorption versus partitioning, lateral diffusion to the channel and interaction with specific lipids may also contribute to the observance of multimode binding kinetics.

The binding energies to zwitterionic POPC membranes as measured by Trp quenching (25–26 kJ/mol), were in good agreement with the predicted binding energies from our companion MD study (18–27 kJ/mol) in the same lipid environment (41). These simulations suggest that GsMTx4 stably resides at the shallow binding depths compared with Hanatoxin (58) and HpTx2 (29). This is supported by the brominated lipids quenching experiments placing both WT and analog peptides at a shallow depth (>12 Å from the bilayer center) compared with toxins that interact with voltage-gated channel intramembrane gates like SGTx (9 Å (20)) and Hanatoxin (8 Å (46)). Both the brominated lipids and acrylamide/10-DN Trp quenching experiments suggest the K-E analogs penetrate deeper than WT in relaxed bilayers. However, K28E that had WT levels of inhibitory efficacy also showed deeper penetration. This may be due to the assays’ insensitivity to differences between changes in the peptide center of mass versus tilt. Both the tilt of the peptides with respect to the plane of the bilayer surface, and overall peptide binding depth, can influence Trp fluorescence and the surface area occupied by the peptide in a relaxed membrane. In simulations of the K-E peptides in bilayers, many of the analogs displayed significant (1–2 Å) tilt of the Trp residues compared with WT (41).

Tension-dependent insertion

As expected for amphipaths, GsMTx4 and its analogs showed strong partitioning into the water-air interface. As the monolayer approaches the bilayer equivalence pressure (25–37 mN/m) the WT isotherm transitions to a shallow compression slope corresponding to the first dip in compressibility, likely reflecting changes in tilt or depth of the peptides as they are compacted by the lipids (Fig. 6 A, top panel). Upon reducing the surface area further, the slope of the pressure increase becomes even shallower through πB and up to the point of collapse. This strongly contrasts with the progressive increase in pressure in control monolayers. We suspect this represents a massive expulsion of peptide at compressions approaching the monolayer-bilayer equivalence pressure (πB ∼37–40 mN/m). The structure of GsMTx4 (59) has dimensions of ∼2 × 2 nm, predicting that the maximal area that can be occupied by the peptide in the plane of the membrane is ∼4 nm². The steep slope transition in the WT compression curve (Cs⁻¹) predicts expulsion of peptides occupying an effective area of 3.3 nm² at a depth of ∼1.5 nm, just below πB. At 1 μM, this would represent the bulk of the peptide’s “area reservoir.” Thus, at πB the peptide would protrude and occupy less area giving it greater area buffering capacity. When tension is applied to a bilayer as in a patch, that tension is predominately in the outer monolayer that is constrained by the patch electrode surface (60, 61). External application of GsMTx4 allows for expansion of the outer monolayer with respect to the inner monolayer. Due to the coupling of the outer to the inner monolayer (53), the inner monolayer now takes a larger share of the total tension, so tension in the outer monolayer is reduced and Piezo gating inhibited.

All analogs had modified π-A isotherms compared with WT. However, the two compromised analogs (K15E and K25E) have significantly lower compressibility near πB and less than half the area change of WT (Fig. S10). The two uncompromised analogs, K8E and K28E, had area changes comparable with WT and the MD simulations predicted these lysines stabilize the deep binding mode (41).

Increasing bilayer surface tension in the simulations (varied between 60 and 75 mN/m) causes the WT peptide to sink deeper into the membrane by ∼1Å (41). In contrast, peptide/monolayer simulations showed the peptide sinking deeper when the area/lipid ratio is near πB. We also observed that higher pressures (∼100 mN/m for an area change from 57 to 85 Å²) are required in simulations than under experimental conditions (∼10mN/m) (Fig. 5). The analysis of expulsion areas has also suggested possible peptide dimerization (see dimensional changes predicted from the slope of the area-pressure plot in Fig. S10 and (41)), increasing the area buffering capacity.

The incremental cross-sectional areas may represent the expulsion of peptides, perhaps including peptides leaving the membrane with lipids attached. From the pressure midpoint of the last transition for WT (π = 40 mN/m), we estimate the energy of insertion ΔG = area × π = −79 kJ/mol, which translates into a very high affinity with K_D = 6.9 × 10¹³ M⁻¹. Thus, the estimated insertion energy must be steeply dependent on the lipid area displaced by the peptide. If we reduce the area by half (a = 1.65 nm²), then the insertion energy drops to ΔG = −39 kJ/mol, giving K_D = 8 × 10⁶ M⁻¹, which is much closer to the measured affinities. From this calculation we might infer that the peptides are expelled as dimers (see below) or with clusters of lipids. If the process of expulsion is concomitant with collapse, then the peptide may induce nonlamellar structures that are in equilibrium with the lamellar part, precisely at πB. This would create another peptide/lipid buffer that could “clamp” the tension in the monolayer.

Effects of aggregation on membrane-binding kinetics are minimal

Dynamic light scattering (DLS) showed that, in most cases, the reduced charge on the analogs increased aggregation, which may affect bilayer interactions and the effective peptide concentration. However, K15E (compromised) and K28E (uncompromised) show similar levels of aggregation as concentration decreases. It is also possible that aggregation is responsible for the appearance of the additional negative peak at 225–230 nm in CD spectra of analogs in solution. Aggregation can effect exciton splitting produced by B_b transition in tryptophan absorbance (62), which is known to affect CD spectra in peptides containing several Trp residues (63). Whatever the cause, this feature disappears upon binding to POPC:POPG LUV, indicating that there is no substantial difference in the fold of the WT and mutant peptides.

Although larger aggregate structures do not appear to affect analog activity, smaller multimeric assemblies may play a role in inhibition. Our MD study shows that dimers sometimes form when associated with the membrane that was stable for more than 300 ns, with one peptide inserted in the bilayer and the second peptide riding on top of the first (41). When tension was applied to the bilayer, the second stacked peptide rotates to partition into the bilayer. Experimental and MD simulations of antimicrobial cationic peptides show a similar mechanism of binding where peptide dimers are attracted to the membrane surface, followed by sinking of the hydrophobic cores into the bilayer (64). This might explain the Hill coefficient of 1.5 observed for the concentration dependence of inhibition. Thus, an alternative to the monomer-depth change model is partitioning of surface-absorbed multimers into the bilayer as tension changes.

Conclusions

The bilayer lateral pressure/tension profile, when altered by membrane stretching, works on MSCs, favoring states with larger in-plane areas (65, 66, 67, 68, 69). The data suggest that GsMTx4, acting as a “mobile reserve” of membrane material, incorporates into the membrane in a tension-dependent manner and distorts the distribution of tension near the channel, making the transfer of force from the bilayer to the channel less efficient. This is fully consistent with the earlier observation that GsMTx4 does not block the pore, but produces a rightward shift of the tension-activation curve (3). GsMTx4 primarily resides in a shallow mode that is in a tension-dependent equilibrium between a surface-adsorbed state (low surface area) and deeper insertion as the free volume increases (17). Changes to lysine residues that surround the hydrophobic face of the peptide and stabilize the shallow mode allow the peptide to penetrate deeper and possibly tilt in the relaxed membrane, reducing the peptide’s area reservoir and shifting lateral pressure that drives the partitioning between the shallow and deep states. The tension-dependent depth changes of GsMTx4 modulate the area of the outer monolayer inhibiting Piezo activation. These same principles likely apply to other amphipathic molecules (70). Although all amphipaths affect monolayer area, the magnitude of their effect on MSCs depends on the tension at the relevant depths, which appears to be a key difference between GsMTx4 and other ICK peptide inhibitors.

Author Contributions

R.G.—electrophysiological recordings of Piezo1 and TREK1 channels, testing sensitivity to GsMTx4 and analog peptides, native gel electrophoresis of peptide aggregation, data analysis, interpretation, and drafting and editing the manuscript; C.G.—tryptophan quenching and vesicle protection experiments, binding affinity determination, depth of penetration, CD spectrum of native structures, and editing of manuscript; A.Y.—tension-dependent peptide depth measurements, isothermal calorimetry, dynamic light scattering assessment of aggregation, data analysis, and manuscript editing; K.N.—molecular dynamics simulations, data analysis, and manuscript editing; F.S.—conception and design of the experiments, interpretation of data, and critically revising manuscript; A.S.L.—conception and design of the experiments, analysis and interpretation of data, and drafting and critically revising the manuscript; S.I.S.—conception and design of the experiments, analysis and interpretation of data, and drafting and critically revising the manuscript; and T.M.S.—conception and design of the experiments, analysis and interpretation of data, and drafting and critically revising the manuscript.

Editor: Paulo Almeida