Scaled vibratory feedback can bias muscle use in children with dystonia during a redundant, one-dimensional myocontrol task

Abstract

Vibratory feedback can be a useful tool for rehabilitation. We examined its use in children with dystonia to understand how it affects muscle activity in a population that does not respond well to standard rehabilitation. We predicted scaled vibration (i.e. vibration that was directly or inversely proportional to muscle activity) would increase use of the vibrated muscle because of task-relevant sensory information, while non-scaled vibration would not change muscle use. The study was conducted on 11 subjects with dystonia and 14 controls. Each subject underwent 4 different types of vibration on the more dystonic biceps muscle (or non-dominant arm in controls) in a one-dimensional, bimanual myocontrol task. Our results showed that only scaled vibratory feedback could bias muscle use without changing overall performance in children with dystonia. We believe there may be a role in rehabilitation for scaled vibratory feedback to retrain abnormal muscle patterns.

INTRODUCTION

Dystonia is a movement disorder characterized by involuntary sustained or intermittent muscle contraction, overflow of electromyography (EMG) activity, and co-contraction of antagonistic muscles, leading to repetitive movements and abnormal postures^1,2. Primary dystonia is mostly genetically-derived, and presents no structural brain abnormalities, while secondary dystonia is a result of degenerative processes or injury, such as seen in children with cerebral palsy (CP)³. While dystonia can occur in both adults and children, most of the research in this field has been conducted to understand how dystonia affects adults^4,5. Sensory deficits are common in secondary dystonia due to dyskinetic cerebral palsy⁶, and along with motor deficits, can result in reduced skill acquisition and poor motor performance. We believed these effects were even more pronounced in children since the inability to acquire new motor skills during early stages of development may further exacerbate their motor disability and limit their social development. Constraint-induced movement therapy and deep brain stimulation are tools that have been used to improve movement^2,7–8. Furthermore, transcranial direct current stimulation (tDCS) of motor cortex has been used with mixed results^9–11. While these treatments are helpful to reduce the symptoms of dystonia, there is an unmet clinical need for solutions that would promote sensorimotor learning. Therefore, it is worthwhile exploring whether augmented sensory information can ameliorate sensory deficits, and thus improve motor skill acquisition.

The efficacy of augmented sensory feedback for rehabilitation has been widely demonstrated, and many studies have been conducted using visual, auditory, and tactile modes of feedback to understand how they affect performance^12–14. Among different biofeedback modalities, the use of vibratory or tactile feedback in stroke rehabilitation, and its effects on muscle activation and cortical excitability have been studied extensively, mostly in adults^15–20. However, the effectiveness of augmented feedback on arm function in patients going through rehabilitation is not well-understood²¹.

Augmented sensory feedback in the form of vibration is able to direct attention to specific areas of the body, possibly resulting in a more efficient selection of sensory inputs, and causing an increase in behavioral impact²³. In this paper, we aimed to understand how (and in which situations) vibration affects motor behavior and muscle use in childhood dystonia. We hypothesized that scaled feedback (i.e. feedback that is directly or inversely proportional to EMG) could provide task-relevant information, thereby enabling changes in muscle activation based on how the added sensory information is utilized. In particular, we compared effects of scaled vs. non-scaled forms of vibratory feedback to evaluate our primary hypothesis that scaled vibration augments sensory awareness and would produce a wider range of behaviors in a multi-muscle task with numerous solutions. We did not expect non-scaled vibration (i.e. feedback that is either constant or random, and not correlated to EMG) to cause similar behaviors because it would not provide task-relevant sensory information. These types of vibration would act like background noise that the system would ignore. According to Brooks, when a previously relevant stimulus turns out to be irrelevant, neurons in the temporal cortex stop responding to it, resulting in subject habituation²⁴. We also expected scaled vibration to benefit children with dystonia more so than healthy controls because the latter group already has an intact sensorimotor system.

In order to test our hypotheses, we designed a one-dimensional bimanual myocontrol task that provided enough redundancy to allow for limited exploration, but also had a set of optimal (and efficient) solutions. We derived the basis for this task from previous work by Latash et al.^25,26. A myocontrol task was designed because it allowed us to measure the effects of vibratory feedback at the level of muscle activation. Task performance was measured using the speed-accuracy trade-off, formulated by Fitts’ Law^27–29. Such a paradigm provided the opportunity to study how well one could modulate muscle contraction (both amplitude and duration), before and after vibratory feedback. In this study, we measured task performance with four kinds of vibratory feedback: scaled (proportional, reverse) and non-scaled (constant and random).

MATERIALS AND METHODS

Subjects

We recruited eleven children (eight males, three females; age 16.7±3.0) affected by either primary or secondary dystonia in at least one of their upper limbs (see Table 1), and fourteen healthy control children (nine males, five females; age 15.5±3.2). The subjects with dystonia were recruited from Children’s Hospital of Los Angeles, and all had sufficient cognitive and verbal ability to understand the instructions. The upper extremity components of the Barry-Albright Dystonia (BAD) scale were used to assess level of motor skill and assess differences between arms³⁰. The more impaired arm was used for vibration (in control subjects, the non-dominant arm was used for vibration). The University of Southern California Institutional Review Board approved the study protocol. All children and their parents gave informed written assent/consent for participation. Authorization for analysis, storage, and publication of protected health information was obtained from parents according to the Health Information Portability and Accountability Act (HIPAA). This study was performed in accordance with the Declaration of Helsinki.

Table 1.

Subject characteristics. Vibration types were: P- proportional, C- constant, R- reverse, Ra- random). BAD (Barry-Albright Dystonia upper extremity motor scale) scores shown for left (L) and right (R) arms.

Subject	Age (years)	Gender	Diagnosis	BAD scores	Vibrated arm	Vibration type
P1	16	M	Secondary generalized dystonia; cerebral palsy	L: 3; R: 3	Right	P,C,R,Ra
P2	17	F	Primary generalized dystonia; non-DYT1	L: 1; R: 2	Left	P,C,R,Ra
P3	20	F	Secondary generalized dystonia; cerebral palsy	L: 3; R: 3	Left	P,C,R,Ra
P4	16	M	Secondary generalized dystonia; cerebral palsy	L: 2; R: 0	Left	P,C,R,Ra
P5	14	M	Secondary generalized dystonia; cerebral palsy	L: 1; R: 3	Right	P,C,R,Ra
P6	12	M	Secondary generalized dystonia; cerebral palsy	L: 3; R: 3	Left	P,C
P7	18	M	Secondary generalized dystonia Unknown cause	L: 1; R: 1	Left	P,C,R,Ra
P8	12	M	Secondary generalized dystonia; cerebral palsy	L: 0; R: 3	Right	P,C,R,Ra
P9	20	M	Secondary generalized dystonia; traumatic brain injury	L: 3; R: 0	Left	P,C,R,Ra
P10	20	F	Primary generalized dystonia; DYT1 mutation	L: 1; R: 1	Right	P,C,R
P11	19	M	Primary generalized dystonia; DYT1 mutation	L: 1; R: 0	Right	P,C,R,Ra

Experimental setting

We designed a bimanual myocontrol task where the activation and relaxation of the left and right biceps muscles, via elbow joint flexion, controlled the vertical position of a single red line on the computer screen. The modified sum of the EMG amplitudes from the two biceps muscles controlled the movement of the red line (i.e. the cursor position) as such:

$$\mathit{\text{Cursor position}}={[{{\mathit{EMG}}_{\mathit{\text{left biceps}}}}^{1/1.2}+{{\mathit{EMG}}_{\mathit{\text{right biceps}}}}^{1/1.2}]}^{1.2}$$ Equation 1

Position on the screen thus corresponded to muscle activity, and the position was scaled so that the top of the screen corresponded to 100% of maximal voluntary contraction (MVC). The exponents of 1.2 were chosen empirically so that any given line position is achieved most efficiently when both muscles are activated equally (Figure 1).

Figure 1.

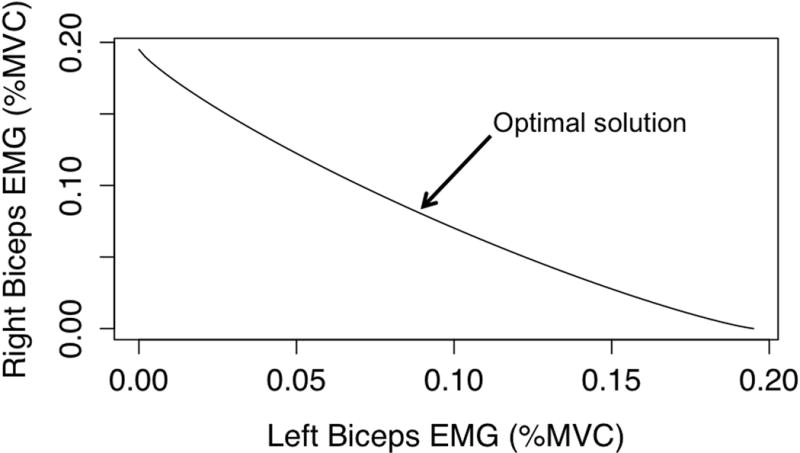
The possible solutions for each target can be represented as a function of how activation of each biceps muscle contributes to control of the line on computer screen. Here we show the solution space for one of the indices of difficulty (ID 4). An optimal solution always exists i.e. equal use of both biceps muscles.

Custom software was developed to create the interface for the task (Visual Studio 6.0, Microsoft, Redmond, WA, USA). According to the Fitts’ Law paradigm²⁷, we designed five virtual targets, represented by a blue bar with a specific width and vertical position. We used 3 bar widths (0.1, 0.2, 0.3% MVC), and 3 bar positions (vertical height) (0.25, 0.5, 0.75% MVC). Index of difficulty (ID) was calculated according to Fitts’ Law:

$$\mathit{\text{Index of Difficulty}}(\mathit{\text{ID}})={\log }_2\left(\frac{2-\mathit{\text{Height}}}{\mathit{\text{Width}}}\right)$$ Equation 2

The range of indices of difficulty was 1.32–3.32 bits.

The task was designed in a manner where the most energetically efficient strategy to reach each target was achieved by activating both of the biceps muscles equally, in the sense that this solution minimizes the sum of squared muscle activations. The specific exponent values in Eqn.1 were chosen to make the task difficult but not impossible, based on data collected during pilot experiments. This is explained in Figure 1, where it can be seen how modulation of the two muscles allows for task success (i.e. reaching the target). The energetically favorable solution was always to flex both muscles equally.

We placed surface EMG electrodes (DE-2.1 electrodes with Bagnoli-8 amplifier, Delsys Incorporated, Boston, MA, USA) with 20–450 Hz band-pass filter and 1000× amplification over the left and right biceps muscle bellies. The EMG signals were sampled at 1KHz (Power 1401, Cambridge Electronic Design Limited, Cambridge, UK) using custom data acquisition software. The EMG signals from each muscle were processed online in the following manner: a high-pass Butterworth filter (fourth order, 1 Hz cutoff) followed by a Bayesian filter³¹, and then a low-pass Butterworth filter (second order, 5 Hz cutoff). A round 2-inch gel ground electrode (PainRX Store, Fountain Valley, CA, USA) was placed on the right hip. Maximum voluntary contraction (MVC) was obtained at the beginning of each experiment set by asking subjects to flex each of their biceps muscles maximally during a period of 32 s. The EMG trace during this period was broken into segments of equal length, and the signal in each bin was averaged to obtain a value. The maximum of each of the bins was determined to be the MVC.

In order to provide vibration, we first attached a surface EMG sensor (Biometrics Ltd, Newport, UK) next to the electrode on the more dystonic/non-dominant arm. Input from the sensor was processed at 1 KHz by an electromyograph (DataLOG MWX8, Biometrics Ltd, Newport, UK) that then wirelessly sent the data to a program (on Visual Studio 6.0), which controlled the type and amount of vibration to be applied via a portable vibrating unit (designed and developed by TDS; patent number: US 8,311,623 B2). The vibrating unit sensor was placed directly on top of the electrode on the more dystonic/non-dominant arm that was used to control the red line. It must be noted that the vibrating unit had the functionality to both measure EMG signals and provide vibratory output scaled proportionally to EMG levels; however, we used the device on slave mode so that we could control the vibration pattern. The ground electrode for the DataLOG system was embedded in a cloth bracelet that we tightened around the non-vibrated arm’s wrist.

Task

Subjects were seated in front of a table with the computer screen placed at eye-level. They were asked to place their elbows and lower arms, with palms facing up, on the chair’s armrest. We strapped their wrists onto the armrests using wrist straps to ensure isometric muscle contractions during the elbow joints flexion. Subjects were asked to activate both the left and right biceps muscles in order to move the red line into the blue target bar on the screen (each target appeared on the screen for 3s per trial). They were asked to do this as fast as possible, using any combination of the two muscles as they saw fit. Task success was achieved when the color of the bar turned from blue to cyan (this occurred when a subject stabilized the red line within the target bar for at least 500ms), at which point they could relax their muscles in order to return the line back to the bottom of the screen (Figure 2). The experiment was divided into 4 blocks (AABA design), each containing 15 trials, with the 5 different IDs presented in a pseudorandom order within each trial. In block 3, one of the 4 modes of vibration was applied. We tested four types of vibration in a pseudorandom order: 1) Proportional-vibration was provided at a level proportional to the measured EMG, 2) Constant-vibration was provided at a constant level (50% of the power generated by the motor), 3) Random-vibration levels were generated, via a random number generator, between 0 and 100% of the power of the motor, 4) Reverse-vibration was provided at a level inversely proportional to the measured EMG. The vibration was applied to the more dystonic arm (assessed using BAD scale) in children with dystonia, and to the non-dominant arm in controls. Each block lasted approximately 8 minutes. Subjects came in on 4 separate days to complete the experiment for each of the four modes of vibration. Two of the 14 controls only completed proportional and constant types of vibration. Table 1 lists subject characteristics for children with dystonia.

Figure 2.

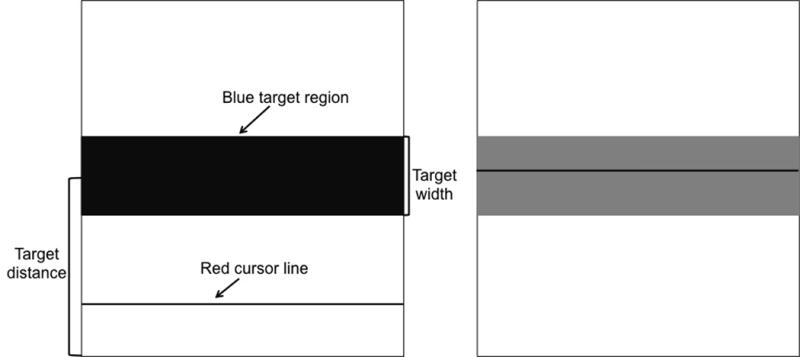
Each target appears on the computer screen as a blue bar (shown here in black) with a specific width and distance from bottom of the screen. The black line on the bottom of the screen is controlled via a specific combination of left and right biceps activation. Successful task completion was signified by the blue bar changing color to cyan (black to gray in this figure), as shown on the screen to the right.

Data analysis and statistics

Data were analyzed using Matlab^® R2013a software (Mathworks^® Inc., Natick, MA, USA). The movement time (MT) of the cursor was calculated as the time interval between appearance of the target and successful task completion. We analyzed performance by measuring overall throughput (TP) values before and after vibration was provided³². TP (bits/s) was calculated as:

$$TP=\frac{1}{N}{\displaystyle {\sum }_{i=1}^N{\mathit{ID}}_1/{\mathit{MT}}_i,}$$ Equation 3

where N=5 is the number of ID conditions.

In order to assess how vibration affected muscle use, we determined the average ratio of EMG in the vibrated muscle to non-vibrated muscle for each subject. We assessed how this ratio changed during vibration (Ratio_2,3) and post-vibration(Ratio_2,4) by comparing to the ratio in the pre-vibration phase (baseline). A positive ratio meant that the biceps of the vibrated arm had higher activation than that of the non-vibrated arm.

$$\begin{array}{l}R=\raisebox{1ex}{${\mathit{EMG}}_{\mathit{\text{vibrated arm}}}$}\!\left/ \!\raisebox{-1ex}{${\mathit{EGM}}_{\mathit{\text{non vibrated arm}}}$}\right.\\ \begin{array}{cc}{\mathit{\text{Ratio}}}_{2,3}=\frac{R_{\mathit{\text{Block}}3}-R_{\mathit{\text{Block}}2}}{R_{\mathit{\text{Block}}3}+R_{\mathit{\text{Block}}2}}& {\mathit{\text{Ratio}}}_{2,4}=\frac{R_{\mathit{\text{Block}}4}-R_{\mathit{\text{Block}}2}}{R_{\mathit{\text{Block}}4}+R_{\mathit{\text{Block}}2}}\end{array}\end{array}$$

These ratios were calculated for two stages of the task: the feedforward stage and the stabilization stage. We defined the feedforward stage to be the first 100ms post appearance of a bar, based on work by Milner & Franklin (2005). The stabilization stage was defined as the period in which the subject had to maintain the line inside a bar for 500ms in order to successfully complete the task. All EMG signals were normalized to the previously measured MVC values before analysis. Positive ratio values indicated increased use of the vibrated arm (with respect to block 2), while negative values showed the opposite.

In order to test Fitts’ Law, we performed linear regressions on average movement time across subjects within each type of vibration via the method of least squares. The correlation coefficient indicated the goodness of fit of movement time in successful trials as a function of ID³³.

Statistical analysis was performed using RStudio^® version 0.98.977 (RStudio Inc.^®, Boston, MA, USA). We used a linear mixed effects model (R-package lme4, version 1.1-7) to determine interactions and effects of four factors (ID, block, subject type, vibration type) on outcome measures i.e. the dependent variables. We created linear mixed effects models using maximum likelihood (R-package lme4, version 1.1-7) to analyze effects on a dependent variable. For the analysis of movement time, the variables ID (5 levels), block (4 levels), vibration type (4 levels), and subject type (2 levels) were set up as fixed effects, while the intercepts for subjects were random effects. This is the model for movement time:

$$\mathit{\text{Movement Time}}-\mathit{\text{Subject type}}+\mathit{\text{Vibration type}}+\mathit{\text{Block}}+\mathit{\text{ID}}+(1+\mathit{\text{ID}}|\mathit{\text{Subject}})$$

In order to analyze effects of block, vibration type and subject type on throughput, we created the following model:

$$\mathit{\text{Throughput}}-\mathit{\text{Subject type}}+\mathit{\text{Vibration type}}+\mathit{\text{Block}}+(1|\mathit{\text{Subject}})$$

For analyzing the effects on the vibrated arm, we created the following model:

$${\mathit{EMG}}_{\mathit{\text{vibrated arm}}}-\mathit{\text{Subject type}}+\mathit{\text{Vibration type}}+\mathit{\text{Block}}+\mathit{\text{ID}}+(1+\mathit{\text{ID}}|\mathit{\text{Subject}})$$

After creating these models, we tested the significance of each of the fixed effects on the dependent variable by comparing the model (full) against reduced models (null) in which one of the fixed effects was removed each time. Afterwards, we looked at the interaction between fixed effects by testing a model with interaction against one without it. In order to compare the significance between models and to find the model that best fit our data, we ran one-way ANOVA to obtain P values and the Akaike information criterion (AIC) values³⁴. A lower AIC value in the model with interaction, as well as P<0.05 indicated that a significant interaction between the tested factors existed. To determine significance of the different levels in a factor on the dependent variable, we ran post-hoc analyses on the data by running pairwise Tukey’s tests on reduced models.

RESULTS

Fitts’ Law

Both groups of subjects followed behavioral patterns described by Fitts’ Law during the experiments i.e. the movement times were higher for the higher IDs, as expected (Figure 3). Movement time showed a significant linear regression on ID for both subject groups in all 4 types of vibration. In patients, the Pearson’s correlation coefficients were 0.930 [t(18)=10.707, P<0.001], 0.911 [t(18)=9.369, P<0.001], 0.959 [t(18)=14.351, P<0.001], 0.962 [t(18)=15.024, P<0.001] for proportional, constant, random, and reverse types of vibration respectively. In controls, the correlation coefficients for the same four vibration types were: 0.964 [t(18)=15.394, P<0.001], 0.950 [t(18)=12.931, P<0.001], 0.898 [t(18)=8.671, P<0.001], 0.963 [t(18)=15.131, P<0.001]. In patients, the coefficient of determination varied from 0.816–0.989 across the different types of vibration; in controls, it varied between 0.662 and 0.976. There was no significant difference in the R-squared values (P=0.989) and the slopes of the linear fits (P=0.708) between patients and controls.

Figure 3.

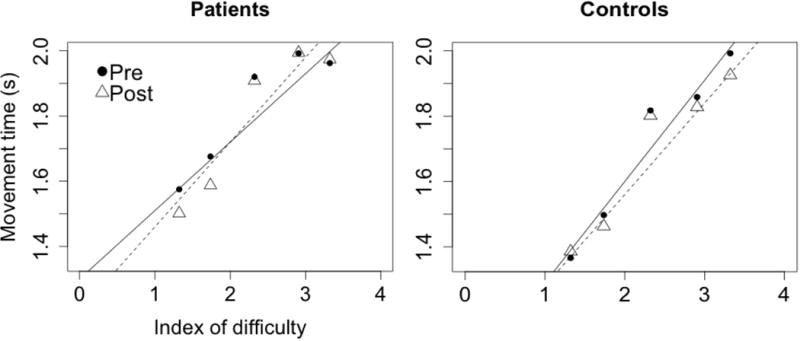
Subjects generally followed Fitts’ Law in the task both pre and post vibration. The graphs here show this relationship for proportional vibration (R² in patients: pre-0.816, post-0.898; R² in controls: pre-0.939, post-0.924). This relationship persists for all other types of vibration as well. The solid line represents regression in the pre vibration data, and dashed line represents the regression on post vibration data.

Linear mixed effects modeling showed that these factors had significant effects on movement time during the target stabilization period: subject type (AIC_full=−69.368; AIC_null=−56.449; P<0.001), vibration type (AIC_full=−69.368; AIC_null=−55.329; P<0.001), block (AIC_full=−69.368; AIC_null=−59.672; P<0.01), ID (AIC_full=−69.368; AIC_null=7.274; P<0.001). Movement time in subjects with dystonia was 0.173 ± 0.0406 seconds higher than in controls, as expected. Overall, across all subjects, movement time was lowest for random (1.699 ± 0.0267 s), followed by constant (1.701 ± 0.0261 s), then reverse (1.731 ± 0.0265 s), and finally proportional vibration (1.752 ± 0.0261 s).

We saw a significant interaction between subject and vibration type (AIC_full=−78.084; AIC_null=−69.368; P<0.01), implying that the effect of different kinds of vibration was different for the two subject groups. During constant, random, and reverse vibration, controls moved significantly faster: 0.162 ± 0.0446s (P=0.0163), 0.230 ± 0.0457s (P=0.0002) and 0.189 ± 0.0452s (P=0.0031) respectively. Movement time was lowest during the vibration block (mean: 1.698 ± 0.0262s) when compared to other blocks. However, it was not significantly lower than that in the block before it.

Throughput

We ran a linear mixed effects model to determine effects of subject type, vibration type and block on throughput (TP). We found subject type (AIC_full=1269.3; AIC_null=1276.8; P<0.01) and vibration type (AIC_full=1269.3; AIC_null=1274.3; P<0.05) to have significant effects on TP, while block (AIC_full=1269.7; AIC_null=1269.3; P=0.129) did not. There were also no significant interactions between any of the factors in the model. Using the Tukey test, we found that overall TP of subjects with dystonia was 0.127 ± 0.0391 bits/s (P=0.0031) lower than in controls, thus showing that control subjects performed significantly better on the task (as shown in Figure 4). With regards to effects of vibration type on TP, we found the only significant difference to be between proportional and random vibration, with TP being 0.0744 ± 0.0233 bits/s (P=0.0079) higher in the case of random vibration.

Figure 4.

Throughput does not significantly change post vibration in either patients (grey) or controls (black). However, there is a significant (P<0.01) difference in the throughput values across subject groups.

Muscle use during stabilization period

For the linear mixed effects model on EMG of vibrated arm, we found block (AIC_full=−6701.1; AIC_null=−6676.8; P<0.001) and ID (AIC_full=−7112.8; AIC_null=−6701.1; P<0.001) were the only factors that had significant effects, while subject type and vibration type did not. There were significant interactions between subject type and block, vibration type and block, and subject type and vibration type. The model that best fit (i.e. had the lowest AIC) the data included all factors, along with interaction between subject and vibration type (AIC_full=−7135.4; AIC_null=−7112.8; P<0.001). We saw significant (P<0.0001) decreases in EMG levels between blocks 1 and 3 (0.0105 ± 0.00213), and blocks 2 and 3 (0.0121 ± 0.00212), and a significant (P<0.0001) increase (0.0101 ± 0.00212) in EMG levels between blocks 3 and 4.

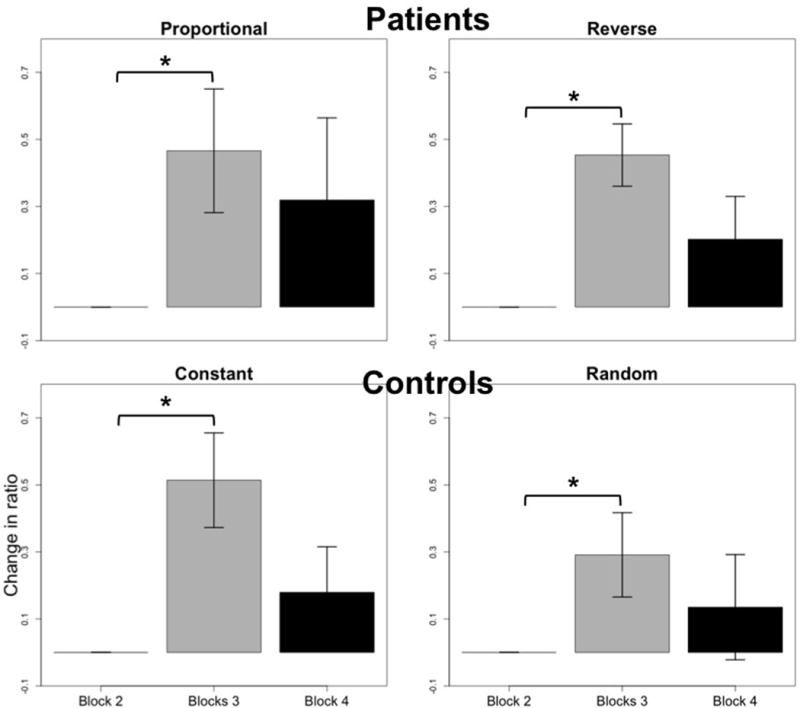
Figure 5 shows increase in Ratio_2,3 for patients during proportional and reverse vibration, and for controls during constant vibration. Patients were able to increase use of their vibrated arm 73% of the time during proportional vibration, and 90% of the time with reverse vibration. For the control subjects during constant vibration, their vibrated arm use increased for 72% of trials.

Figure 5.

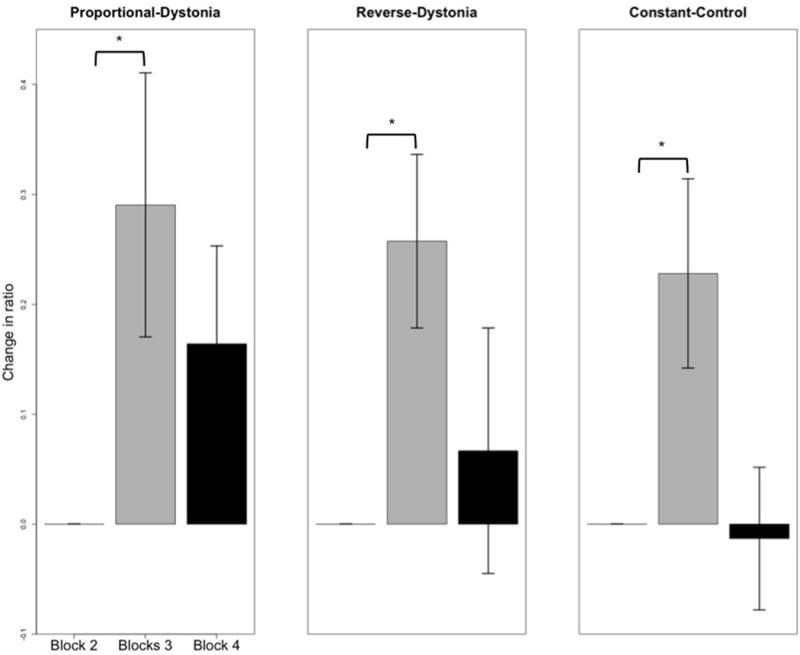
In patients, vibrated arm use increased significantly (P<0.05) when provided with proportional or reverse-scaled vibration (left and middle plots), as shown by the increase in the ratio from block 2 (no vibration) to block 3 (vibration provided). However, this significance did not persist post vibration. In controls, there was a significant increase (P<0.05) in vibrated arm use only during constant vibration. Standard error bars shown.

We found that Ratio_2,4, was not significant for any of the vibration types. However, in patients, the average of this ratio is positive only for the scaled forms of vibration; hence, increased vibrated arm use only persists when vibration is scaled to muscle activity, and not when patients are provided with either constant or random vibration. In addition, more than 50% of subjects reported they believed they utilized the vibrated arm more during vibration (as seen on the raw EMG traces). This signifies that subjects truly had an increased awareness of their body during that period.

Muscle use during feedforward stage

We conducted similar mixed effects modeling to analyze how the previously studied factors affected vibrated arm use during the feedforward phase (the first 100ms post appearance of a target)³⁵. We found these factors had significant effects on vibrated arm use during the feedforward stage: block (AIC_full=−8972.6; AIC_null=−8896.5 ; P<0.001), vibration type (AIC_full=−8896.5; AIC_null=−8891.7; P<0.05), and ID (AIC_full=−9013.4; AIC_null=−8972.6; P<0.001). Subject type was not significant. There were significant interactions between subject type and vibration type (AIC_full=−8931.9; AIC_null=−8896.5; P<0.001), subject type and block (AIC_full=−9018.7; AIC_null=−8967.5; P<0.001), and vibration type and block (AIC_full=−9029.6; AIC_null=−8972.5; P<0.001). There was a significant (P<0.0001) decrease in vibrated muscle use between blocks 2 and 3 and a significant (P<0.0001) increase in vibrated muscle use between blocks 3 and 4. We found subjects had significantly (P=0.042) higher vibrated muscle use during random vs. proportional vibration, and significantly (P=0.0148) higher muscle use during random vs. reverse vibration.

In patients, Ratio_2,3 was significantly higher than baseline during proportional and reverse vibration (Figure 6), similar to that which is seen during the target stabilization phase. These increases are more pronounced than in the stabilization phase i.e. the increases in vibrated arm use were seen 82% of the time during proportional vibration, and 100% of the time during reverse vibration. In controls, Ratio_2,3 showed significant increases in both constant and random vibration. This was seen 100% and 71% of the time, respectively. Generally, the ratio was unchanged in the scaled modes of vibration for controls.

Figure 6.

Muscle use in the feedforward phase. In patients, there was a significant increase (P<0.05) in use of vibrated arm during both proportional and reverse vibration, similar to what was seen during the stabilization phase. This is seen by the increase in the ratio from block 2 to block 3, in which vibration was provided. Control subjects showed significant increases in vibrated arm use during constant and random vibration. These effects did not persist post vibration for either group of subjects.

DISCUSSION

We have shown that in children with dystonia, scaled forms of vibratory feedback increased sensory awareness to task-relevant information, thus supporting our primary hypothesis. This increased sensory awareness was made apparent via changes in muscle use that were not accompanied by changes in overall performance. In a previous open-label clinical trial, we had found that long-term use of vibratory biofeedback (via a portable vibration unit) scaled to muscle activity improved specific motor skills in children with secondary dystonia²². Our present results showed changes in muscle activity, but without significant changes in task performance for both groups of subjects. This may have been due to the nature of the task and the shorter period of time in which the stimulus was given. It must be noted, however, that control subjects performed better overall and had lower movement times to reach targets than did patients, as expected in speed-accuracy trade-off tasks involving healthy controls and children with dystonia^28,29,37,38. Patients moved slower due to the inherently present muscle activity that is not correlated to the task.

The type of vibration was key to causing an unconscious bias in muscle use. We saw that only muscle activity-related vibration was able to cause significant changes in the pattern of muscle use during the stabilization period in subjects with dystonia. Thus, it is possible that in the dystonic group, scaled vibration provided useful sensory information to the system, while non-scaled vibration resulted in habituation to the stimulus, with no significant changes occurring as a result³⁶. These effects were seen during the feedforward stage as well, signifying that some sort of anticipatory behavioral adjustments took place in the presence of scaled types of vibration, prior to when feedback started to play a role. In control subjects, we saw a significant increase in muscle use only during constant vibration. This may have occurred because control subjects are already able to perform this task close to an energetically favorable manner, and providing them with scaled vibration is redundant to signals provided by the properly functioning sensory system. On the other hand, their behavior may have changed with non-scaled vibration since task-irrelevant information could have been distracting and induced a response involving more muscle activity. Only constant vibration caused significant changes in muscle activity after the feedforward stage, though, which was not as expected because we hypothesized random vibration would have also caused similar changes. Perhaps random vibration is more easily ignored in this type of task as compared to constant vibration. Further investigation should help understand this difference better.

We also showed that although there was always an energetically favorable solution for completing the task, scaled forms of vibration were able to bias away from this, and bring attention to the more dystonic limb (in subjects with dystonia). This is clinically relevant because we have shown that it is possible to selectively change muscle patterns in children with dystonia, thus potentially alleviating cramping and discomfort, and in the long-term, improving performance. The results are even more interesting since we did not give specific instructions to subjects to use one muscle more than the other, and they were thus able to change their actions subconsciously. It is possible that the mechanism of efficacy of vibration feedback is different in different disorders. This particular use of vibration could, however, be used for learning purposes when retraining muscle patterns in children with dystonia³⁹.

In this experiment, we were unable to account for subject expectation after the first visit because we followed the same block design, with a different type of vibration each time. However, expectations within trial were dealt with since the bar targets were presented in a pseudorandom order. Persistent effects are also not significant in this study, mostly because the vibration was only applied during one block i.e. approximately 8 minutes. We believe that a longer period of vibration could have caused a strong effect similar to what was seen during the vibration block, and multiple days of vibratory feedback could potentially cause long-term changes. It is possible that scaled vibratory feedback may strengthen the cortical representations associated with the movements within the task, thus Hebbian plasticity may be a mechanism involved^22,36. Further studies will have to be performed to test this hypothesis.

CONCLUSION

Vibratory biofeedback is a promising therapy for improving muscle patterns in children with dystonia. The ability to increase muscle awareness while maintaining overall performance is important since our goal is not to train someone to do a very specific task, but to enable a better understanding of limb manipulation. We believe this method of learning gives children with dystonia the ability to explore new solution spaces, potentially resulting in more efficient solutions. While we have demonstrated the utility of scaled vibratory feedback for these purposes, there is still much to be understood about its role in changing the sensorimotor dynamics of the limb. Further research in this area will hopefully provide additional insights that will help us develop relevant therapeutic tools.