Crystals creeping out of cracks

The formation of crystalline materials from liquids or vapors is important for many fundamental processes and applications in material sciences, biology, environmental sciences, and physics and chemistry. The type of crystalline polymorph as well as the number of crystals and their size and shape all depend upon the conditions under which they form. The emergence of a new macroscopic crystal usually requires the nucleation of a tiny crystal-like cluster that can grow subsequently to macroscopic size. In many processes the nucleation of such clusters is rate-limiting and is often catalyzed by some type of surface. What exactly makes a good catalyst for such heterogeneous crystal nucleation is a topic of intense research in many disciplines. Several prerequisites are discussed in the literature such as size, lattice match, surface chemistry, or surface defects (1, 2). It is not clear which of them, if any, is most important, because experimental studies of crystal formation often lack the resolution for detecting the initial clusters and the “active sites” (i.e., the location where they form). Campbell et al. (3) in PNAS show experimentally that a cavity can be an active site for crystal formation from water and organic vapors. They observed that condensates, either liquid or crystalline, formed via capillary condensation in a surface wedge of Muscovite mica, and subsequently crystals grew out of these wedges.

The formation of condensates can occur from pure vapor in a process termed homogeneous nucleation, or it may be catalyzed by a substrate onto which the condensates nucleate heterogeneously. Homogeneous nucleation of droplets from the vapor often requires very high saturation S_L, that is, a high vapor phase partial pressure P_G relative to the bulk phase liquid vapor pressure P_L(bulk) (4). This behavior is a result of the fact that the very small initial clusters that form during nucleation are in the nanometer size range and, thus, they have very large surface-to-volume ratios. As a consequence, such droplets exhibit much larger vapor pressures than a bulk liquid, and the logarithm of this vapor pressure ratio increases proportionally to the liquid/vapor interfacial energy more commonly known as surface tension (σ_L), and inversely proportional to the droplet radius, r:

$$\ln \left(\frac{P_{\mathrm{L}}\left(r\right)}{P_{\mathrm{L}}\left(\text{bulk}\right)}\right)\propto \frac{\hspace{0.17em}2{\mathrm{\sigma }}_{\mathrm{L}}}{r}.$$ [1]

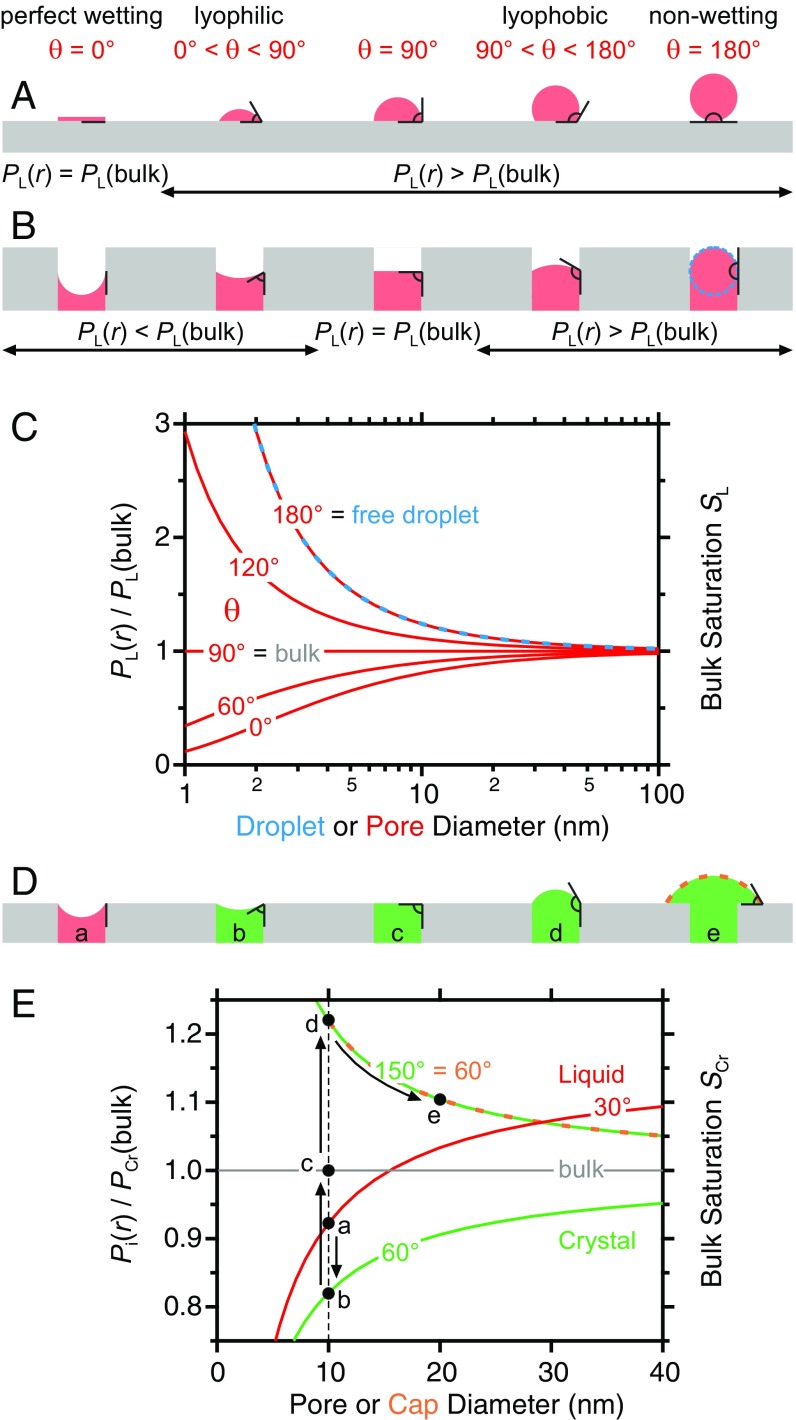
For example, at ambient temperature a water droplet with a 1-nm radius has a vapor pressure that is about a factor of 3 larger than that of a bulk water puddle (with formally infinite radius r_∞), and, correspondingly, water droplets nucleate homogeneously from the vapor at high saturation values of about S_L ≈ 3–4 (4). In contrast, heterogeneous nucleation of liquids on substrates occurs already at lower saturation, depending upon how well the substrate is wetted by the condensing liquid. One can distinguish several cases of how a liquid wets a smooth surface by studying the equilibrium contact angle θ of a droplet residing on the substrate surface as shown schematically in Fig. 1A: A surface may be perfectly wettable (θ = 0°; left), lyophilic (θ < 90°), lyophobic (θ > 90°), or perfectly nonwettable (θ = 180°). The contact angle is a result of the interplay of the different interfacial energies between the three phases: substrate, liquid, and vapor. Note that for the same volume of liquid the resulting radius of the droplets is smallest in the nonwetting case and becomes infinite for perfect wetting. Correspondingly, in all cases in which θ > 0° a larger-than-bulk vapor pressure results (Fig. 1A).

Fig. 1.

Behavior of condensates on smooth surfaces and within pores. The surface wettability determines the contact angle θ and the curvature of a liquid condensate on a smooth surface (A) and within a pore (B), where θ and the pore diameter strongly affect the liquid’s vapor pressure (C), here for the case of liquid water. Capillary condensation of a liquid (red) and subsequent crystal (green) nucleation and growth (D) for water and ice in a 10-nm pore (E).

The situation changes when instead of a smooth surface the substrate contains cavities into which the liquid can condense. The resulting geometry of the liquid within the cavity again depends upon the liquid/substrate contact angle (Fig. 1B). For cylindrical pores and contact angles of θ > 90° the condensed liquid exhibits a convex curvature, thus implying higher-than-bulk vapor pressure. For the nonwetting case (θ = 180°) the liquid curvature radius corresponds to that of a free droplet without surface interactions (compare the dashed blue circle). At θ = 90° no curvature results, and for θ < 90° the concave curvature implies a negative radius and a lower-than-bulk vapor pressure (Fig. 1C). These dependencies imply that a liquid can condense into pores of lyophilic materials (θ < 90°) already below bulk saturation levels, that is, at S_L < 1.

The behavior gets more complicated when the temperature is below the bulk melting point of the condensate (5). In that case, a competition between the condensation of the liquid and the deposition of the crystal may occur at smaller pore radii. Because the bulk crystal is stable below the melting point, the chemical potential and, hence, the vapor pressure of the bulk crystal is lower than that of the metastable liquid. Therefore, deposition requires a smaller saturation than does condensation. This behavior is modulated by pore geometry, the crystal versus liquid contact angle with the substrate (θ_Cr versus θ_L), and the crystal/gas and liquid/gas interfacial energies. In Fig. 1 D and E a scenario is depicted for water and ice at ‒15 °C with hypothetical values of θ_L = 30° and θ_Cr = 60°, respectively, when a cylindrical pore with a 10-nm diameter is subjected to a continuous increase in vapor saturation. Capillary condensation of the liquid may occur (a) before the condensed pore liquid crystallizes, which reduces the vapor pressure to that of the crystal at the appropriate contact angle θ_Cr (b). However, in order for the crystal to creep out of a cylindrical pore a maximum saturation is required for the formation of a spherical crystal cap with the diameter of the pore and contact angle θ_Cr (d). Thereafter, the crystal cap is at unstable equilibrium and thus grows toward the bulk (e). Note that for other pore geometries the above process may be more complex.

Campbell et al. (3) studied such processes by making use of acute wedges tens of nanometers wide that developed on the surface of Muscovite mica during cleavage. They exposed these surface pockets to vapors of different organics such as camphor or to water vapor and used optical microscopy and interferometry to observe the formation and growth of condensates in the pockets as a function of the vapor saturation. Because the investigated condensates partially wet mica they formed concave menisci in the acute wedge of the pockets. It turns out that in each case crystals finally grew out of the corners of the pockets. Although the authors were not able to observe the crystal nucleation step itself, these experiments convincingly show that the wedges were the spots where crystals always formed. Campbell

et al. (3) suggest that such surface cavities may be the elusive active sites for crystal formation during heterogeneous vapor nucleation.

The crystallization processes observed by Campbell et al. (3) will likely trigger future studies. For example, experiments with model surfaces with different pore topography, varying wettability, and with or without crystal/substrate lattice match may help to pin down the exact dependencies between these parameters and the crystal formation process. Such studies may help in the development of the coating of materials and of catalysts by way of vapor deposition methods of volatile precursors. They may also aid in the design of ice-nucleating materials for various purposes such as cloud seeding (6) or controlled ice nucleation in cryotechnology (7), or even by providing particularly active sites as sacrifice spots for ice nucleation at low saturation levels to protect more sensitive parts from becoming iced up. The experimental observations may also have implications for the development of anti-icing surfaces. Some anti-icing materials are produced by preparing a nanoporous surface or coating, often from relatively hydrophilic materials (8, 9). These are subsequently modified by a covalently bound monolayer or coating of hydrophobic molecules, leading to a superhydrophobic surface. When a supercooled droplet collides with such a surface it may rebounce even if it freezes upon impact, thus leaving the surface devoid of attached ice crystals. Such icephobic materials are discussed, for example, as surface materials to protect against wind turbine or aircraft icing. The experiments by Campbell et al. (3) imply, however, that if the hydrophobic molecular monolayer or coating is damaged the exposed porous hydrophilic substrate underneath may actually be a better nucleus than a smooth, nontreated surface, thus becoming a threat instead of protection from icing up.

The processes observed by Campbell et al. (3) are highly relevant for understanding deposition ice nucleation, which has recently been hypothesized to actually be pore condensation and freezing (10). Indeed, independent evidence for the formation and existence of ice in porous aerosol particles has been provided in recent experiments by Wagner et al. (11). After an initial formation of ice on porous particles at relatively high supersaturation the particles were brought to bulk subsaturation, which normally leads to evaporation of all ice. In some materials such as clay minerals and zeolites an apparent negative curvature of ice in 5- to 8-nm pores allowed for its survival even at bulk subsaturation and, thereafter, ice formed at lower supersaturation. Whether or not this preactivation can occur only with porous minerals or also with soluble materials remains to be studied in detail. It has been shown that ice freezing in water-soluble organic aerosols and subsequent aerosol freeze-drying leads to porous glassy organic aerosol particles, which may lead to enhanced ice nucleation (12, 13).

Ice crystal formation is an important and widespread environmental process. Often heterogeneous ice nucleation is the prevailing rate-limiting pathway, as is the case in atmospheric cloud formation. In clouds, heterogeneous nucleation is triggered by ice-nucleating particles, which consist predominantly of mineral dust particles and of biological particles. Experiments show that certain alkali feldspars such as microcline are particularly active ice nucleators at high temperature (14). More recently, it was shown that the (100) surface plane of microcline is the ice-nucleating crystal plane, which is a high-energy surface and, therefore, is usually hidden (15). It is, however, exposed in grooves or grain boundaries of microcline, from which ice crystals then nucleate. Hence, it is conceivable that first a liquid condenses into such grooves by capillary condensation, and that this liquid subsequently, probably immediately, nucleates heterogeneously at the (100) plane to ice, which then grows out of the groove or crack to form macroscopic ice crystals.

The experiments by Campbell et al. (3) challenge experimentalists and theoreticians alike: They call for comprehensive studies of crystal nucleation in cavities at high spatial resolution to visualize the nucleation step, and they also ask for molecular dynamic simulations of ice nucleation (16) in confined geometries, in particular because small clusters can have more complex wetting states than those implied in Fig. 1 (17).