Skip to main content
PMC home page
Proceedings of the National Academy of Sciences of the United States of America logoLink to Proceedings of the National Academy of Sciences of the United States of America
. 1991 Dec 15;88(24):10983–10987. doi: 10.1073/pnas.88.24.10983

Chemical implementation of neural networks and Turing machines.

A Hjelmfelt 1, E D Weinberger 1, J Ross 1
PMCID: PMC53057  PMID: 1763012

Abstract

We propose a reversible reaction mechanism with a single stationary state in which certain concentrations assume either high or low values dependent on the concentration of a catalyst. The properties of this mechanism are those of a McCulloch-Pitts neuron. We suggest a mechanism of interneuronal connections in which the stationary state of a chemical neuron is determined by the state of other neurons in a homogeneous chemical system and is thus a "hardware" chemical implementation of neural networks. Specific connections are determined for the construction of logic gates: AND, NOR, etc. Neural networks may be constructed in which the flow of time is continuous and computations are achieved by the attainment of a stationary state of the entire chemical reaction system, or in which the flow of time is discretized by an oscillatory reaction. In another article, we will give a chemical implementation of finite state machines and stack memories, with which in principle the construction of a universal Turing machine is possible.

Full text

PDF
10983

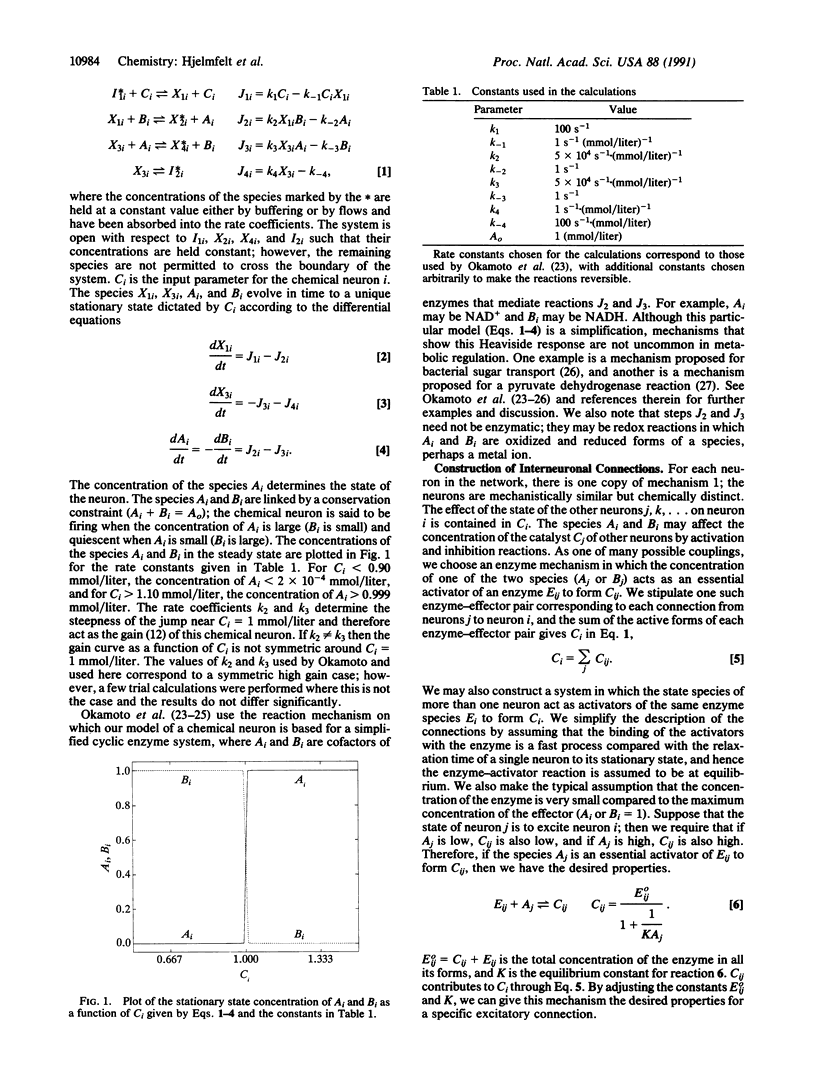
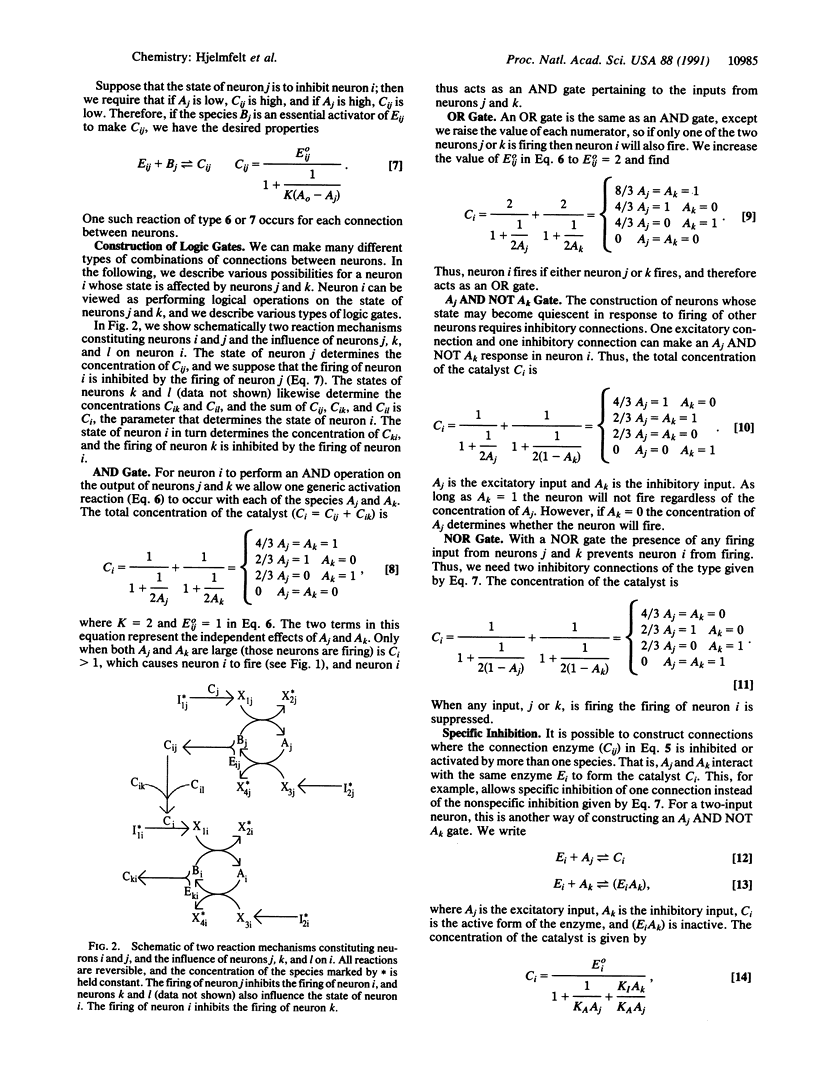
Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Hopfield J. J. Neural networks and physical systems with emergent collective computational abilities. Proc Natl Acad Sci U S A. 1982 Apr;79(8):2554–2558. doi: 10.1073/pnas.79.8.2554. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Hopfield J. J. Neurons with graded response have collective computational properties like those of two-state neurons. Proc Natl Acad Sci U S A. 1984 May;81(10):3088–3092. doi: 10.1073/pnas.81.10.3088. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Hopfield J. J., Tank D. W. Computing with neural circuits: a model. Science. 1986 Aug 8;233(4764):625–633. doi: 10.1126/science.3755256. [DOI] [PubMed] [Google Scholar]
  4. Moore C. Unpredictability and undecidability in dynamical systems. Phys Rev Lett. 1990 May 14;64(20):2354–2357. doi: 10.1103/PhysRevLett.64.2354. [DOI] [PubMed] [Google Scholar]
  5. Okamoto M., Hayashi K. Dynamic behavior of cyclic enzyme systems. J Theor Biol. 1983 Oct 21;104(4):591–598. doi: 10.1016/0022-5193(83)90247-3. [DOI] [PubMed] [Google Scholar]
  6. Okamoto M., Katsurayama A., Tsukiji M., Aso Y., Hayashi K. Dynamic behavior of enzymatic system realizing two-factor model. J Theor Biol. 1980 Mar 7;83(1):1–16. doi: 10.1016/0022-5193(80)90369-0. [DOI] [PubMed] [Google Scholar]
  7. Okamoto M., Sakai T., Hayashi K. Biochemical switching device realizing McCulloch-Pitts type equation. Biol Cybern. 1988;58(5):295–299. doi: 10.1007/BF00363938. [DOI] [PubMed] [Google Scholar]
  8. Okamoto M., Sakai T., Hayashi K. Switching mechanism of a cyclic enzyme system: role as a "chemical diode". Biosystems. 1987;21(1):1–11. doi: 10.1016/0303-2647(87)90002-5. [DOI] [PubMed] [Google Scholar]
  9. Stadtman E. R., Chock P. B. Superiority of interconvertible enzyme cascades in metabolic regulation: analysis of monocyclic systems. Proc Natl Acad Sci U S A. 1977 Jul;74(7):2761–2765. doi: 10.1073/pnas.74.7.2761. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Wolfram S. Undecidability and intractability in theoretical physics. Phys Rev Lett. 1985 Feb 25;54(8):735–738. doi: 10.1103/PhysRevLett.54.735. [DOI] [PubMed] [Google Scholar]

Articles from Proceedings of the National Academy of Sciences of the United States of America are provided here courtesy of National Academy of Sciences

RESOURCES