Abstract
The range over which thermodynamic temperatures have been realized by gas thermometry at the NBS has been extended to 730 K. The results are preserved by measuring the corresponding international practical temperatures. The difference between them is expressed as the following polynomial:
which is valid in the range 273 to 730 K.
The difference found and the estimated uncertainties at the three defining fixed points in the range covered are
| t(°C) | T/K–T68/K68 | Uncertainty
|
|
|---|---|---|---|
| Random (99% confidence limits) | Systematic | ||
|
| |||
| 100 | −0.0252 | ±0.0018 | ±0.00054 |
| 231.9681 | −0.0439 | ±0.0022 | ±0.0015 |
| 419.58 | −0.0658 | ±0.0028 | ±0.0028 |
Keywords: Gas thermometry, International Practical Temperature Scale of 1968, temperature scale differences, thermodynamic temperatures
1. Introduction
The National Bureau of Standards’ gas thermometer has been described in numerous papers [1–10]1 that give details of many of the important parts of the equipment and of some of the special measurements required. The last two of these papers also give the results of measurements with the gas thermometer from 0 to 142°C. A comparison of these results with most earlier work elsewhere shows there are systematic differences that we ascribe to our more complete elimination of the effects of sorption. It was stated in the last paper (and has been further confirmed by more recent observations) that our gas thermometer system and the helium filling gas were clean enough that any residual effect of sorption on these measurements would probably be negligibly small. Ultimately, it will be rewarding to remeasure these temperatures, when the benefits of further experiences with the gas thermometer can be realized. At present, it is more productive to extend the measurements to higher temperatures, both for their own intrinsic interest, and as a further step leading to the gold point. This paper gives the results of the initial measurements of the difference between thermodynamic temperatures and international practical temperatures, at temperatures in the range from 141 to 457 °C, and combines them with the earlier data [9, 10], reappraised in the light of further information.
2. Equipment
The essential elements of the gas thermometer have been described in earlier papers [9, 10]. Briefly, it is a constant volume type comprising a 432.5 cm3 bulb of platinum-rhodium alloy in the form of a right circular cylinder connected by small bore 90 percent platinum 10 percent rhodium tubing to a valve. The gas thermometer can be connected through the valve with a diaphragm pressure transducer, so that thereafter equality of the gas thermometer pressure with the pressure in the manometer can be established. The gas thermometer has a counter-pressure arrangement, a narrow annulus about 0.25 mm wide formed around the bulb by a heavy Inconel2 600 case surrounding the bulb.
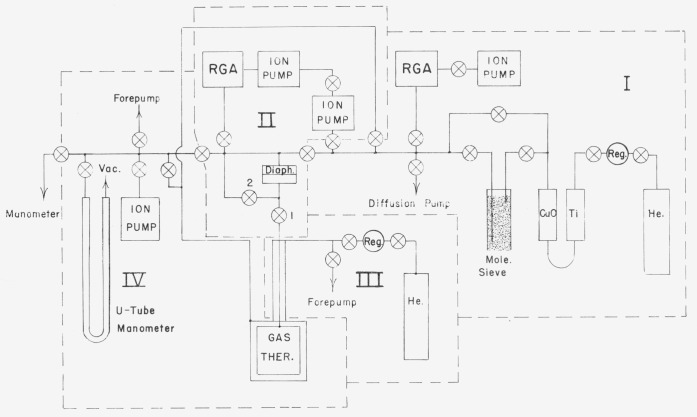
The gas thermometer has been improved by installing more thermocouples to measure the temperature distribution along the small bore tube, and by modifying the gas handling and vacuum equipment.3 The new arrangement of the vacuum system, shown in figure 1, allowed the system to be subdivided into four parts (labeled I, II, III, and IV) plus the manometer, so that all the critical sections could be pumped by ion pumps. The subsystems were arranged in such a way as to be able to attain the best vacuum in part II, which included the gas thermometer bulb in direct communication with the residual gas analyzer (RGA).
Figure 1. Schematic of vacuum system.
Boundaries of Parts I, II, III, and IV, which can be isolated for pumping, are shown by broken lines.
Two discrepancies from the earlier papers [9, 10] should be noted. The composition of the bulb is not all 80 percent Pt-20 percent Rh as stated in [9], nor all 88 percent Pt-12 percent Rh as stated in [10]; it is some of each. The sheet supplied for the top was found to have been 80 percent Pt-20 percent Rh, but the sheet for the sides and bottom was 88 percent Pt-12 percent Rh. However, the bulb is the same Bulb III referred to previously; the effect of this difference will be considered in the Discussion. Also, the volume of 454.8 cm3 given for Bulb III has been re-evaluated as 432.5 cm3. The effect of this difference on earlier results will be tabulated in table 4, section 8.
Table 4.
Gas thermometer results previously published [10]
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
| ||||||||||||||
| State | Ave bath t °C | 701 | 703 | 707 | Vault °C | π | Vτ mm3/K | Gage blocks in | Z cm | T′GT/KGT − T68/K68 | δtbulb K | δttherm K | δtimp -K | T/K− T68/K68 |
|
| ||||||||||||||
| 2.02 | 100.00097 | 0093 | 0065 | 0133 | 20.0101 | 8.63 | 0.723352 | 30.0496312 | 76.569444 | −0.0325 | + 0.0019 | 0.0001 | 0.0058 | −0.0247 |
| 1.03 | −.00468 | 464 | 485 | 455 | 20.0128 | 10.64 | .836102 | 22.0592231 | 56.053471 | Fiducial | ……. | ……. | ……. | ……. |
| 2.03 | 99.99979 | 9972 | 9995 | x002 | 20.0381 | 8.62 | .721825 | 30.0496312 | 76.568881 | −.0330 | .0019 | .0001 | .0058 | −.0252 |
| 1.04 | −.00460 | 464 | 477 | 441 | 20.0239 | 10.64 | .835937 | 22.0592231 | 56.053319 | Fiducial | ……. | ……. | ……. | ……. |
| 1.05 | −.00066 | 59 | 86 | 55 | 20.1096 | 10.64 | .835661 | 3.9999996 | 10.164256 | Fiducial | ……. | ……. | ……. | ……. |
| 2.04 | 100.00427 | 423 | 397 | 461 | 20.0166 | 8.64 | .724165 | 5.4888939 | 13.884388 | −.0319 | .0019 | .0041 | .0010 | −.0249 |
| 3.01 | 120.78497 | 8478 | 8464 | 8548 | 20.0204 | 8.30 | .701367 | 5.7488897 | 14.567342 | −.0406 | .0023 | .0050 | .0013 | −.0320 |
| 4.01 | 141.59376 | 9364 | 9334 | 9429 | 20.0146 | 8.03 | .685585 | 6.0488946 | 15.431416 | −.0473 | .0028 | .0057 | .0016 | −.0372 |
| 2.05 | 100.00536 | 515 | 511 | 581 | 19.9973 | 8.60 | .719052 | 5.4488939 | 13.884378 | −.0333 | .0019 | .0041 | .0010 | −.0263 |
| 2.06 | 10.000484 | 473 | 447 | 531 | 20.0091 | 8.59 | .718361 | 5.4489006 | 13.884336 | −.0290 | .0019 | .0041 | .0010 | −.0220 |
| 3.02 | 120.79043 | 935 | 9011 | 9084 | 20.0052 | 8.30 | .701608 | 5.7488981 | 14.657444 | −.0382 | .0023 | .0050 | .0013 | −.0296 |
| 4.02 | 141.59935 | 9925 | 9898 | 9983 | 20.0029 | 8.03 | .685669 | 6.0488977 | 15.431489 | −.0455 | .0028 | .0057 | .0016 | −.0354 |
| 2.07 | 100.00710 | 698 | 681 | 749 | 20.0080 | 8.59 | .717602 | 5.4489006 | 13.884351 | −.0305 | .0019 | .0041 | .0010 | −.0235 |
| 1.06 | .00254 | 252 | 243 | 269 | 19.9962 | 10.61 | .831801 | 4.0000047 | 10.164242 | Fiducial | ……. | ……. | ……. | ……. |
| 5.01 | 20.65967 | 5969 | 5955 | 5976 | 20.0006 | 10.10 | .804239 | 4.3000022 | 10.932764 | −.0040 | .0003 | .0010 | .0002 | −.0025 |
| 6.01 | 41.35574 | 5582 | 5556 | 5583 | 19.9952 | 9.64 | .779314 | 4.6002021 | 11.702679 | −.0094 | .0007 | .0018 | .0004 | −.0065 |
| 7.01 | 62.06274 | 6282 | 6257 | 6283 | 19.9905 | 9.21 | .753338 | 4.9001996 | 12.472959 | .0155 | .0010 | .0026 | .006 | −.0113 |
| 8.01 | 82.79370 | 9344 | 9333 | 9434 | 19.9716 | 8.90 | .738540 | 5.2000040 | 13.243896 | −.0275 | .0015 | .00035 | .0008 | −.0217 |
| 2.08 | 100.01211 | 1195 | 1186 | 1253 | 19.9975 | 8.59 | .718271 | 5.4889006 | 13.884349 | −.0344 | .0019 | .0041 | .0010 | −.0274 |
| 1.07 | .00580 | 574 | 571 | 595 | 19.9929 | 10.61 | .832181 | 4.0000047 | 10.164321 | Fiducial | ……. | ……. | ……. | ……. |
| 5.02 | 20.66189 | 6184 | 6190 | 6193 | 19.9816 | 10.10 | .804688 | 4.3000022 | 10.932783 | −.0045 | .0003 | .0010 | .0002 | −.0030 |
| 6.02 | 41.36032 | 6030 | 6016 | 6051 | 19.9789 | 9.67 | .783029 | 4.6002021 | 11.702738 | −.0111 | .0007 | .0018 | .0004 | −.0082 |
| 7.02 | 62.06529 | 6521 | 6519 | 6546 | 20.0144 | 9.24 | .756769 | 4.9001996 | 12.472967 | −.0166 | .0010 | .0026 | .0006 | −.0124 |
| 7.03 | 62.06526 | 6537 | 6521 | 6520 | 20.0145 | 9.24 | .756665 | 4.9001996 | 12.472965 | −.0167 | .0010 | .0026 | .0006 | −.0125 |
| 8.03 | 82.78611 | 8611 | 8600 | 8621 | 20.0119 | 8.86 | .733769 | 5.2000040 | 13.243744 | −.0236 | .0015 | .0035 | .0008 | −.0178 |
| 1.08 | .00497 | 500 | 479 | 514 | 19.9822 | 10.61 | .832388 | 4.0000047 | 10.164280 | Fiducial | ……. | ……. | ……. | ……. |
Identification of columns: Columns 1–6 as for table 2, 7 = relative pressure head effect, 8 = value for dead space ΣkVki/Tki, 9 = gage block height used in manometer, 10 = Z=augmented pressure expressed in cm of Hg, 11 = difference of values found from gas thermometer and PRT’s, 12 = correction required for the revised volume of the bulb, 13 = correction for the thermomolecular pressure effect, 14 = correction for gas imperfection, 15 = difference of thermodynamic and International Practical Kelvin temperatures at the temperature of t68, given in column 2.
The liquids in the stirred baths were changed from organic materials. The liquid for the bath at 0 °C was changed to water, to which potassium chromate was added as a rust inhibitor, and the liquid for the bath at temperatures from 142 to 457 °C was changed to a eutectic mixture of molten potassium nitrate, sodium nitrate and lithium nitrate. Despite the lesser viscosity of the new fluids, no important change in the performance of the thermostat was observed.
New equipment was installed for the electrical measurements. All resistances of the platinum resistance thermometers (PRT’s) were measured with improved speed and precision on an ac bridge [11]. All thermocouple emf’s were measured by a digital voltmeter.
For thermal expansion measurements, a new interferometer furnace, designed for use up to the gold point, was assembled, and it was operated from −25 to 550 °C so that the thermal expansion of samples of the bulb material could be measured. Details of this equipment and the results will be given in a separate paper.
The apparatus shown in figure 2 was constructed for the determination of thermomolecular pressure effects. It consisted of two tubes, an 0.8 mm i.d., 1.6 mm o.d. stainless steel tube running inside a 9.6 mm i.d. Inconel tube, connected across a diaphragm pressure transducer. The outer tube was chosen of such a size that it could be inserted into one of the PRT wells of the gas thermometer bulb case to thermostat it in the stirred molten-salt bath. The apparatus was attached to the gas handling system, so that it could be evacuated, and thereafter gas could be introduced. The overall pressure was read with sufficient accuracy on a simple U-tube mercury manometer. The valves in the system were arranged so that when valves 2 and 4 were closed and 1 was open, the null of the diaphragm could be read, and when 1 and 3 were closed and 2 and 4 were opened, the thermomolecular pressure difference could be read on the diaphragm gage.
Figure 2.
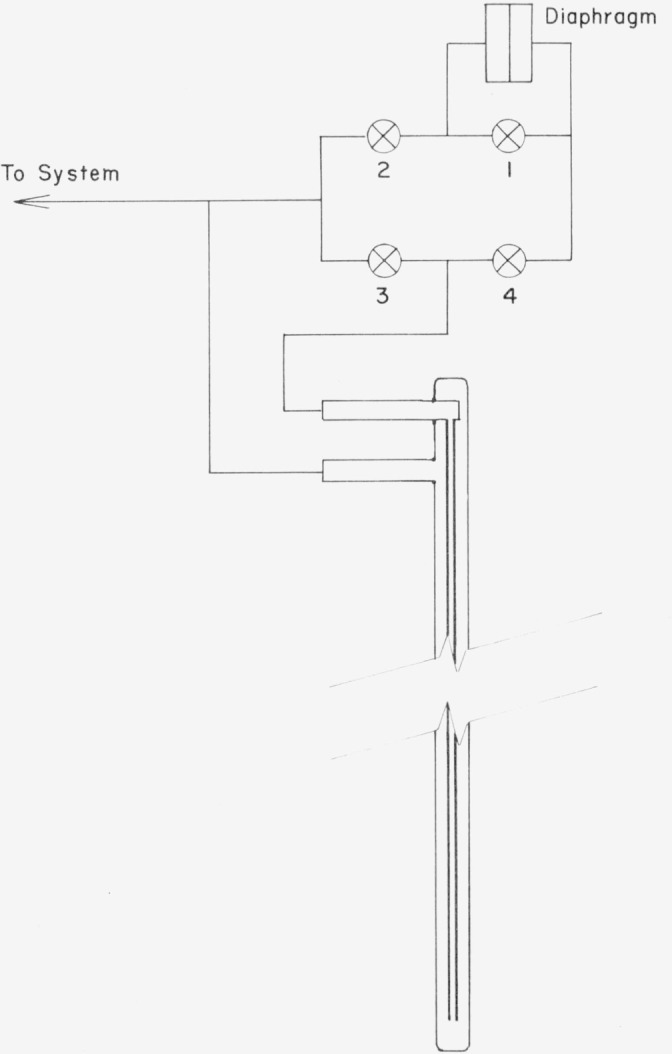
Apparatus for measuring thermomolecular pressure.
3. Preliminary Considerations
A study of the early results in the extended temperature range showed the need for modifying the experimental procedures. This section presents a discussion of some of those problems and their solutions.
During vacuum bakeout we observed the ubiquity and persistence of hydrogen that is a common experience, and for which there has been a common explanation: that the hydrogen was absorbed in the metal in great quantity and diffused out of it slowly. Perhaps it was a happy accident that we introduced some wet helium into the counter-pressure system at 700 °C, and to our astonishment observed a drastic rise of the hydrogen peak on the RGA scan of the gas thermometer contents within 20 s. Inasmuch as the helium peak did not change, the hydrogen must have diffused through the wall, made of 88 percent Pt-12 percent Rh metal. The diffusion of hydrogen through platinum is covered in the literature, which is ample, and is adequately summarized in Dushman [12]. There is considerable variation in the results, but a typical set of values at 750 °C is as follows:
Hydrogen diffuses through pure Pt at the rate of 0.13 Pa-liter per cm2 per min per mm thickness of the metal, with a hydrogen pressure of 105 Pa on one side and a vacuum on the other (the “permeation rate”). Platinum immersed in hydrogen at a pressure of 105 Pa absorbs 0.34 Pa-liters of the gas per cm2 per mm thickness.
Both the permeation rate and the solubility for hydrogen vary as P½, so their relative relationship remains the same at lower concentrations. The inescapable conclusion is that hydrogen initially dissolved in the platinum will quickly be exhausted, and conversely that persistent concentrations of hydrogen observed during vacuum bakeout must be maintained by diffusion through the platinum. The same range of values of diffusion and permeation can be expected for platinum-rhodium alloys. The concentration of free hydrogen in the atmosphere is very small; the only possible sources are water or other hydrogen bearing materials which decompose to release hydrogen. Therefore, we surmised that the problem might be resolved by control of the ambient atmosphere. The furnace was flushed with argon during the bakeout, and a slow flow maintained thereafter. Previously, the partial pressure of hydrogen in the gas thermometer bulb had never been lower than 70 μPa, but it declined to 20 μPa at 700 °C when the protective atmosphere was provided.
For the present temperature range up to 457 °C, we found it necessary to change our procedures in three other ways which will be described in the following paragraphs:
Modification of procedure to avoid creep of the gas thermometer bulb
Above 327 °C, the gas thermometer bulb is subject to creep (non-elastic deformation under small stress). It was discovered from the early results of measurements carried out in the same manner as for the 0–142 °C range. The manometer equipment by its nature permits the accurate realization of only one pressure a day, with one or more experimentally determined values of temperature of the gas thermometer necessary to balance that pressure. Overnight, between measurements, we have always pumped out those portions of the system immediately adjacent to the gas thermometer bulb (parts II and IV) in order to maintain the purity of the system. (The pumpout of section II is also desirable for one of the “integrity checks” described below.) The typical pressure in the gas thermometer bulb at 457 °C was 105 Pa; it was expected that it would deform elastically when the counter pressure annulus (in part IV) was evacuated. However, it was found that gas thermometer temperatures determined by measurements made in descending order agreed with those determined by measurements made in ascending order only up to 327 °C. Above 327 °C, the gas thermometer temperatures differed more as the temperature (and, to some extent, time) increased. We also observed some hysteresis of the gas thermometer bulb after the counter-pressure was restored. It was necessary, therefore, in all subsequent measurements to keep the pressure inside and outside the bulb nearly equal until the bulb temperature was below 300 °C. Because it was more convenient to equalize the pressure by reducing it in the counter-pressure system, all values reported in the paper were derived from runs starting at the highest temperature and proceeding by consecutive steps of about 33 °C to the lower limit.
Modification of Temperature Measurement of the Dead Space
The effect of the volume in the connecting tube of the gas thermometer, the “dead space,” expressed in kelvins, is about six times as large at the zinc point as at the steam point. The uncertainties of the dead space effect can best be evaluated by examining the combined equation for the dead-space correction, δtds. This is
| (1) |
where the summation is over the length of the connecting tube in mm increments, T1 is the fiducial or reference temperature, T2 is the measuring temperature, P1 and P2 are the corresponding pressures, V0 is the volume of the bulb at T1 Vki and Tki are the corresponding values of volume and temperature at the kth position for state i. The element of volume varies between the two states only because of thermal expansion, i.e., Vki=Vk(1 + βkiδtki), where βki is the coefficient of thermal expansion depending upon the position and the state, and δtki is the difference in temperature of the kth position in the ith state from the calibrating temperature of 23 °C. The determination of the volume is thought to be sufficiently accurate [7]; the measurements of the Tki’s on the other hand required improvement.
We note from the equation that for any region of the dead-space actually at the gas thermometer temperature in both states, the net contribution is zero. The accuracy is increased, then, by maintaining the level of the liquid in the bath as high as possible. Unfortunately, the molten salt, because of low viscosity and low surface tension, could not be confined as readily as the earlier bath fluid. Even at the highest temperature the bath had to be less completely filled or the salt would overflow, and it contracted so much over the range of temperature that the level was appreciably reduced at the lowest temperatures.
Previously the temperatures for the dead space calculations for the steam point were derived on the following assumptions:
That the lowest junction is at the temperature of the bath.
That the emf’s of the Pt-10 percent Rh/Pt thermocouples installed for dead space measurement follow the same temperature dependence as was determined for annealed thermocouples of the same spools of wire.
That the portion of the dead space above the uppermost junction was at the temperature of that junction.
That the values of the temperature can be adequately interpolated from least squares fits of the observed temperatures to polynomials chosen according to the complexity of the temperature profile.
The consequence of these assumptions is that one may measure all emf’s as differences from the bottom one and then the temperatures can be derived from the calibrations by calculating the equivalent emf of each couple with an ice junction.
Probably the steam point dead space calculation was adequately accurate because its contribution in kelvins was comparatively small (0.034 K), the height of the bath liquid was satisfactory, the gradients were not severe, and the actual thermocouple emf’s were reasonably close to standard table values. However, in this present temperature range, all these factors are less ideally fulfilled. The value of the dead space correction becomes large, partly because the bath liquid level had to be lower; the gradients, of course, were substantial, and as the temperature became high, the deviation of the actual emf’s versus temperature from the table values became important. It is certain moreover that the bottom thermocouple was not adequately immersed. All these factors required that a better measurement and calculation be employed.
Instead of referring the temperatures to the bottom thermocouple, if the temperature of the reference junctions of the dead space thermocouples were established, the error in the dead space calculation could be reduced. This is true because the temperature is then more accurately known than before at the lower temperatures, where the relative effect of a given volume of the dead space is larger; the same discrepancy in emf produces a smaller error in kelvins if it affects the higher temperatures where the thermoelectric power becomes twice as large as at room temperature; and the accuracy of the result then no longer relies upon the bottom couple being at bath temperature. The details of this change will be discussed in section 6.
Modification in the handling of the platinum resistance thermometers
We found the platinum resistance thermometers to be much more sensitive to shock in the upper range of the temperature investigated. The vibration of the stirred liquid baths caused a significant increase of the resistances of the PRT’s above 200 °C (not accountable by the effects reported by Berry [13], so that the procedures were changed to minimize the time during which the thermometers were kept in the bath. The measurements of the PRT resistances were made at the end of the time they were in the bath, therefore we used the values of the triple points that were found after each gas thermometer run to calculate their resistance ratios. With such precautions, the triple point values did not increase greatly, being subject to a change of <40 μΩ from work hardening during a measurement period, and being partially restored to their original values by annealing at 425 and 457 °C whenever those temperatures were measured.
4. Procedure
The gas thermometer was prepared for operation by vacuum bakeout at 750 °C. After an initial pumpdown to a system pressure of 0.01 Pa by the oil diffusion pump, it was connected with the RGA and isolated from all other parts of the system. It was then pumped only by the ion pump of the RGA, a procedure that gives both the highest concentration in the RGA for the detection of sorbable species, and very clean pumping for the gas thermometer. The entire bakeout furnace was isolated and a protective atmosphere of argon was introduced into it in order to exclude ambient air and prevent the diffusion of water vapor to the hot zone. The pumping was continued until the partial pressure of all contaminating gases was less than 0.1 mPa in the gas thermometer.
The measurements for this paper were made over a period of 15 months during which time the gas thermometer was loaded 10 times and measured at 123 different temperatures. Of the first 51 temperatures measured only 9 are free of creep effects, and of the remainder, the last 43 have the best measurements of the temperatures for the dead space.
The gas thermometer was loaded while being thermostated at the highest temperatures to be measured. The initial measurements were commenced on the following day. At the beginning of a measurement, parts I, II, and III of the vacuum system were isolated but each was being evacuated, part I by its diffusion pump, part II by both ion pumps and part III by its ion pump. The procedure for the measurement of a gas thermometer temperature was as follows:
Before loading, or at other times before introducing helium as a pressure transmitting gas, the diaphragm section was isolated by valves 1 and 2, and the rate of pressure buildup was determined over a period of a half hour or more.4 The rate varied between the limits of 2.5 to 5 mPa/min. With a total volume of about 100 mm3, the relative amount of gas accumulating in the diaphragm compared with the amount of gas in the gas thermometer (for a pressure of 105 Pa) varied between 6 × 10−12 min−1 and 1.2 × 10−11 min−1. The measurement is an “integrity check” to ascertain that the diaphragm is neither contaminated nor has a leak from the outside, and further that there is no significant leakage across the seat of valve 1. The same rate was observed whether the gas thermometer was evacuated or filled, hence it indicated no significant leakage occurred across the valve seat, and probably represented mostly, or totally, degassing.
At the same time, the heater for the Ti-CuO purification trap was turned on. Within 1 hr, the trap reached its operating temperature (700 °C), as confirmed by measurement with a Pt-10 percent Rh/Pt thermocouple referred to ice.
Two integrity checks of the ac bridge and triple point cell were made. First, the ratio of the standard 100 Ω resistor to a 100 Ω PRT was read to assure proper thermostating of the standard. Then the ratio of the resistance of a standard PRT immersed in a triple point cell to the standard resistance was determined on the ac bridge. This thermometer was treated with the utmost care, so that the ratio was reproducible within less than 1 part in 107 over a period of a month, a fact that tended to confirm that the system was functioning properly. Over longer periods, the observed small changes in the ratio could have occurred because of drift in the resistances of either the standard resistance or the thermometer.
The ratios of the resistances of our 3 measuring PRT’s to the standard resistor were determined at the temperature of the triple point of water.
The MacLeod gage, monitoring the vacuum in the upper cell of the manometer was read (typically 0.15 mPa or less) (an integrity check).
The ion pump currents were recorded (an integrity check). The RGA ion pump, open to part II, could be expected to have a current of 0.5 μa, equivalent to 0.2 μPa pressure.
The Dewar around the molecular sieve (#13) trap was filled with liquid nitrogen, and when the temperature of the Ti-CuO trap had reached 700 °C, part I of the vacuum system was isolated and helium was slowly passed through the traps into the lines.
After isolating the ion pumps, helium was admitted to sections 2 and 4, until the pressure as indicated on the U-tube manometer was higher than the pressure in the manometer lines. Invariably, the gas flow was controlled to proceed in the direction from I to II and IV so that no backflow of helium would occur from other parts of the gas thermometer. Finally the manometer gas line was opened and the system pressure adjusted. When it was nearly at the pressure needed, the motor of the valve allowing communication of mercury between the upper cell and the lower cells of the manometer was turned on. The length of time it was necessary to open the valve before flow commenced was recorded (an integrity check). Final adjustments of the amount of gas in the system were made, the mercury valve was opened fully and the level of mercury in the cells was checked. The mercury level was remarkably stable over periods of weeks (within about 2 μm), a fact that depends not only upon a stable temperature but also upon a stable contact angle with the wall. After any needed adjustment of mercury level, the gas pressure was controlled by “thermal injection”, governed by feedback from the capacitance bridge to maintain the pressure at the manometer setting.
After reading the diaphragm zero, we closed the by-pass valve and opened the valve that connected the gas thermometer valve with the diaphragm. The pressure in the gas thermometer was made equal to the pressure in the manometer by adjusting the temperature of the liquid thermostat. When the pressures were about equal, the gas thermometer valve was closed, the by-pass valve opened, and the null of the diaphragm reread. The valves were then reset to reconnect the diaphragm to the gas thermometer so that any small difference between the pressure in the gas thermometer and the pressure in the manometer system could be observed and recorded. Then the gas thermometer was again isolated and the null reread.
The resistances of the PRT’s in the gas thermometer bulb case were read, as well as that of the manometer reference PRT, then the 5 thermocouples of the manometer and the 16 thermocouples of the dead space.
Another set of null-pressure-pressure-null measurements was made with the diaphragm.
Data also included were the time, the values of the gage blocks used in the manometer, and the regulator bridge and control settings.
The resistances of the platinum resistance thermometers were measured at the temperature of the triple point of water.
5. Equations and Calculations
The calculations for the gas thermometer temperatures can be made “symmetric”, in that the equations can be devised so that the same calculation is performed for each measurement including that of the reference state. The details are given in [9]; the essentials are repeated here. We calculate a quantity designated Z that is an “augmented pressure”, expressed in cm of Hg at 20 °C, where the thermal expansion, the pressure head and the dead space effects are accounted for. It is
| (2) |
where i refers to the ith measurement, h0i is the height of the gage blocks used in the manometer, and
| (3) |
In the order of the terms in eq (3), the significance of the symbols is as follows:
| (3a) |
where M is the molecular weight of the gas, g is the acceleration due to gravity, R is the molar gas constant = 8.3137×106 cm3 Pa mol−1 K−1 Tki is the thermodynamic temperature for the kth element of length and ith measurement, and lk is the increment of length.
The volume thermal expansion coefficient of the bulb, β, is derived from
as determined from samples of material of the bulb of length l0 at 0 °C. The term πlβi is negligible.
The remainder of eq (3) is the deadspace term. Ti is the thermodynamic temperature of the bath, Bi is the second virial coefficient of the thermometric gas at Ti Pi is the pressure of the ith measurement, R is the molar gas constant, Vo is the volume of the gas thermometer bulb at 0 °C, Vk is the volume of the kth element of the connecting tube at 23 °C, βki is the thermal expansion coefficient of the volume of the tube at temperature tki, in the kth position during the ith measurement, and δtki=tki−23 °C. This same temperature tki, is expressed as an absolute thermodynamic temperature for Tki in the denominator. The quantity Zi is also given by the expression
| (4) |
where
| (4a) |
with n0 the number of moles of gas, ρ0 the density of mercury at 20 °C, and other quantities as defined before. δZi is a term for thermomolecular pressure, and any variation of the pressure head in the manometer line from the level of the gas thermometer in the colder thermostat. We have calculated an “approximate gas thermometer temperature”, T′GT, from the equation
| (5) |
Then the gas thermometer temperature, TGT was derived by correcting T′GT for the effects of thermomolecular pressures and differing pressure heads, so that
| (5a) |
We can substitute the expression for Zj0 and Zi0 from 4a into 5a, and then because ρ0, g, and V0 are constants, and n0 is maintained constant, we get
| (6) |
with additional, insignificant second and higher order terms. Thus the gas thermometer temperature is equal to the thermodynamic temperature plus a term for the effects of gas imperfection. Substantially
| (7) |
so that TGT/KGT=(Tj+aPj)/K. Thus by determining TGT/KGT at a constant pressure ratio but differing pressure ranges, Tj/K may be found as the intercept of the straight line of TGT/KGT versus Pj and the difference of the second virial coefficients can be found from the slope.
The calculation is made by computer in a series of programs:
The international temperatures of the gas thermometer and the manometer reference station are calculated by a program in Fortran IV named BRIDGE. This program is given in appendix I, with a file of the calibration constants A and B of the PRT’s (TCAL75). A calibration on the ac bridge at the zinc, tin and triple points was made in June 1974, and the last gas thermometer measurements included in this paper were made in September of the same year. (The exact time does not appear to be important. Starting before the measurements were commenced, and extending to a time one year afterward, 4 sets of the thermometer calibrations have been shown to give a reproducibility of values at the temperatures of the fixed points within a standard deviation of 0.45 mK.)
-
The effect of the pressure head is calculated by a program in Basic named PYE. This program is given in appendix 2, and the subprogram LSFIT1 is given in appendix 3. In this program, the the treatment of the temperature distribution is made as described in section 6. The international temperatures, t68, were determined from the emf’s of the thermocouples for the locations shown in figure 3. A correction was applied to t68 to give the thermodynamic temperature, tth, and this in turn was converted to a value of reciprocal absolute temperature, τ. The volume of the gas in the constant volume valve and the first 6 mm of tubing within the valve were at the temperature of the valve. It is expected that the good thermal contact provided by the vacuum seal between the tube and the top of the header, H, brought the temperature of the tube substantially into agreement with the temperature of the header at that point. Between the valve and the top of the header (33 mm), the values of r were interpolated linearly, and similarly between the top of the header and the position of the thermocouple A1 (19 mm). The length of the tube above the top of the header varied by an amount D2, which reflected the differential expansion between the connecting tube of the gas thermometer and its Inconel suspension. (Direct measurements confirmed the calculated value of D2 within experimental error.) The thermocouples A1 …, A12, and B1 on the other hand terminated close to the connecting tube but were independently suspended, being installed in a harness that was self-supporting and through which the tube could move. The expansion of the thermocouple harness was included in the thermal expansion term for the tube.
In order to interpolate the reciprocal temperature between the thermocouple locations, a quadratic equation, τ=B(1)+B(2) I+B(3) I2, was fitted by least squares to the first four pairs of values of τ versus position, I, for A1 … A4 (76 mm). A polynomial giving τ as
was fitted by least squares to the 9 pairs of reciprocal values of temperature versus position for A4…A12 (203 mm). In the interval from A12 to B1 (25 mm) τ was linearly interpolated. If the difference in emf between B1 and the calculated value for the temperature of the bath (the level of which was near or not more than 20 mm below B1) was sufficiently large (>0.1 mV), the difference of the corresponding reciprocal absolute temperatures was linearly interpolated over the next 136 units of length (126 mm), and if <0.1 mV, over 82 units of length (76 mm). The balance of the length of the connecting tube, and an additional length up to the center of the gas thermometer bulb, for a total length count of 688 (639 mm) was included in the calculation. The unit of length was 0.929 mm, which arose as a chart coordinate in measuring the tube diameter. The quantity πi is calculated as:
as given in eq (3a), with the added definition that αki is the linear expansion coefficient of the kth element for the ith. measurement, and Δtki=tki−23. The output of the program gives the notebook reference number (Sϕ is the notebook and page, S1 the assigned run number), the value of πi in ppm, and the displacement D2 in mm. The calculation of the deadspace effect depends upon the evaluation of ΣkVki/Tki and involves exactly the same temperature calculations. They are associated with values of the volumes over the lengths from k-1 to k. The calculation is performed by computer with a program in basic named DEDSPS, given in appendix 4. A file of chart readings for the diameter of the tube is a part of this program, that also required the entry of D2 from the PYE calculation. The thermal expansion of the tube and of the thermocouple locations is combined in the term (l+βkiΔtki). The output of the program gives the notebook and run reference numbers and the value of ΣkVki/Tki, designated V*TAU.
The value of Z is calculated by a program in Fortran IV named SUMMA, which is given in appendix 5. The calculation of the pressure is derived from eq (27) of [5], and is expressed in cm of mercury corrected to 20 °C. The output gives the notebook and run reference numbers and the value of Z in cm.
Figure 3. Thermocouple installation for measurement of the temperatures of the pressure head and dead space.
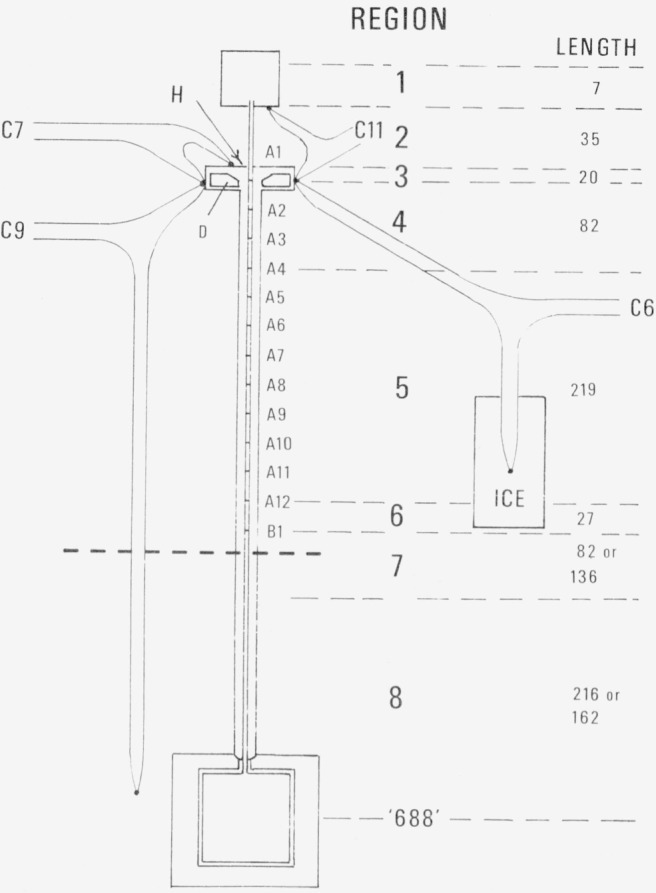
The unit of length=0.929 mm.
6. Determination of Parameters
Numerous calibrations and special measurements are involved in the gas thermometer calculations. Some of them have been mentioned in earlier sections, but they will be summarized here.
6.1. The Gas Thermometer Pressure
The value of pressure used in the gas thermometer equation is the sum of the pressure calculated by eq (27) of [5] plus the difference measured at the diaphragm. The height of the mercury column was determined by the length of the calibrated gage blocks. The density of the mercury was adjusted for its variation in temperature from 20 °C. We used the values of the thermal expansion of mercury published by Beattie et al. [14]. There is an offsetting effect of expansion of the gage blocks; the value in SUMMA reflects the difference between the volume expansion of mercury and the linear expansion of chromium carbide. The small values of the copper-constantan difference thermocouple readings for the manometer cells and for the main mercury column were converted to temperature differences by use of a “standard value” of the thermoelectric power, 40.5 μV/K [15], and the reference temperature was measured by a calibrated standard platinum resistance thermometer.
In order to calculate the pressure differences from the gage readings of the diaphragm, its sensitivity was determined from the pressure changes calculated to have resulted from imposed changes of gas thermometer temperature.
6.2. The Thermal Expansion of the Bulb
The term in the gas thermometer equation second in importance to the pressure ratio is the term for the thermal expansion of the bulb. The thermal expansion coefficients of both 80 percent Pt-20 percent Rh and 88 percent Pt-12 percent Rh have been carefully measured from −25 to 550 °C. The constants entered into SUMMA are those for the thermal expansion of 88 percent Pt-12 percent Rh where the length ratio is expressed as a polynomial,
| (8) |
The estimate of the standard deviation of a predicted point varies from 0.08 ppm in mid range to 0.14 ppm at either extreme. The estimate of the residual standard deviation of 0.14 ppm is consistent with our expectation of the average imprecision of a single measurement.
6.3. The Deadspace
The values used in the deadspace calculations were obtained from various sources. The volume of the bulb was calculated from its dimensions except for the last gas load. In the bakeout preceding the final set of measurements, the bulb was deformed by an external overpressure. When the bulb was removed from the apparatus, its changed volume was determined from the contained weight of water to be 4.2103×105 mm3 at 0 °C, and this value was used in the calculations for the final set.
To determine the volume of the tube, its diameter is required as a function of position. This was determined as described in [7] with an estimated total uncertainty that is insignificant in the final results.
Temperatures were derived from thermocouple readings for the calculation of π and Vτ. It may be useful to remark in advance that considerable error in the thermocouple values will not significantly affect the accuracy of the final results. The final arrangement illustrated in figure 3 was applicable to the last 30 states, where thermocouples were added about the header, H. The couples C6, C7, and C11 comprise copper-constantan legs (Type T), and C9 has Pt-10 percent Rh/Pt legs (Type S). The couple C6, with its reference junction at 0 °C, was used to measure the temperature of the side of the header, and inferentially of the reference junctions of A1 … A12, and B1, because it is expected that the temperature of the reference ring D is nearly the same as the side of the header. The difference couples, C7 and C11 were used to determine the increment of temperature between the top and side of the header and between the end of the tube at the constant volume valve and the side of the header, respectively. C9 was a difference couple running between the bath and the side of the header. It was not needed for the last 30 states, but was a necessary link with earlier measurements to evaluate the earlier data. All copper-constantan readings were converted to temperatures by use of a standard table [15]. The temperatures determined by all Pt-10 percent Rh/Pt thermocouples were evaluated from calibrations of similar couples which could be represented by a quartic,
| (9) |
with a standard deviation of the values at each interval of 25 °C up to 500 °C of 0.47 μV when B1 = 5.45846×10−3 μV/°C, B2 = 1.13497×10−6 μV/(°C)2, B3 = −1.52447×10−8 μV/(°C)3 and B4=9.06033×10−12 μV/(0C)4. When the emf of the Pt-10 percent Rh/Pt thermocouple B1 was measured at 400 °C (under special conditions where the depth of immersion was thought to be adequate), however, it was found to differ from the equation by −28 μV; the cause of the difference appeared to be work-hardening of the platinum leg during installation that was not subsequently relieved by annealing. This difference was assumed to be typical of all the deadspace couples and was taken into account in the gas thermometer calculations by multiplying all values of A1 … A12, and B1 by 1.00895.
In an earlier arrangment of the thermocouples applicable to the preceding 42 states, the C9 junction was located on the top of the header, and there was no C6, C7, nor C11 couple. Furthermore, C9 was calibrated at 400 °C and found to differ from the value calculated from eq (9) by −51 μV. Consequently, the measured emf’s for C9 were multiplied by a factor, 1.01644, to account for the difference.
To carry out the calculation described in the preceding paragraphs it was necessary to predict values of A1(5), C7(5), and C11(5), for the earlier measurements. This could be done from the information available from later measurements. In one switch position, the thermocouple emf’s could be read with respect to B1 as the reference junction (position 4) and were designated as A1(4), etc. In another switch position, the thermocouples were referenced to their own junctions with copper (position 5), and measurements with this reference were designated as A1(5), etc. Because the temperature of the reference ring is uniform, A1(5) – A1(4) = B1(5). Position 5 of the switch was used to read the thermocouples for the last 30 states. As shown in figure 4, the values of A1(5) and C7(5) are well-behaved functions of the bath temperatures as expressed by C9(5). As for C11(5), its values depend not only upon the temperature of the bath, but also upon the temperature of the room and of the plate directly below it which is cooled by tap water. For constant room and cooling water temperatures, C11 decreases linearly as the bath increases in temperature, over a range of about 40 μV. The cooling water temperature varies seasonally from less than 8 °C in the winter to over 20 °C in the summer; this explains the large differences in C6(5). The 40 μV range of C11(5) tends to be more negative as the cooling water temperature increases. The values of C11 (5) used in table 2 were derived on the basis of a linear variation from 40 or 41 μV at 0 °C to 0 μV at 457 °C. A uniform change of 20 μV in the values of C11(5) causes an error in the calculated gas thermometer temperature varying from 0.2 ppm at 140 °C, up to 0.5 ppm at 457 °C. Therefore high accuracy in predicting these C11(5) values is not a requirement.
Figure 4.
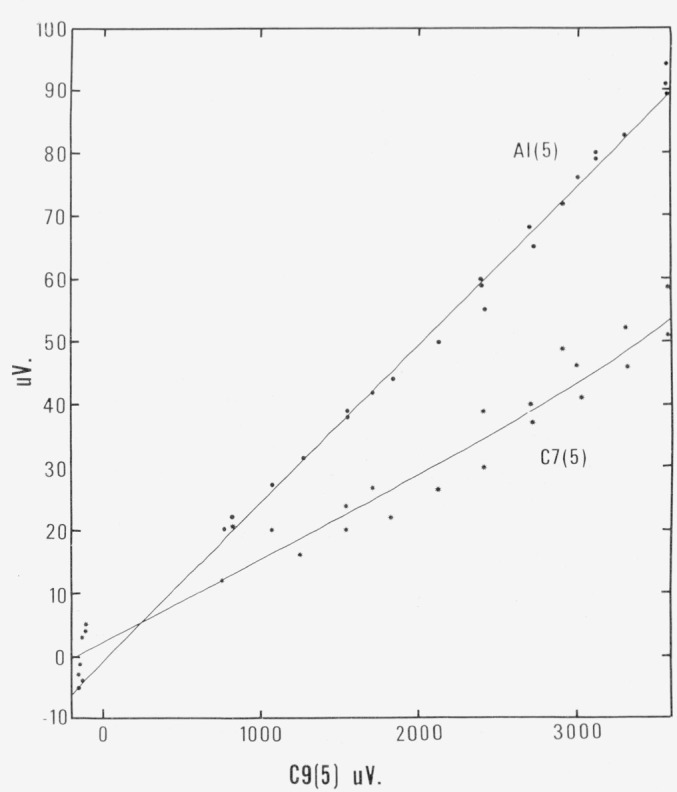
Values of A1(5) and C7(5) as a function of bath temperature, given by C9(5).
Table 2.
Gas thermometer results, sets A, B, C and D
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
| |||||||||||||||
| State | Ave bath t °C | 701 | 703 | 707 | Vault °C | C6 μV | C7 μV | C11 μV | π | Vτ mm3/K | Gage block in | Z cm | T′GT/KGT | Σ δt TGT/KGT − T68/K68 | T/K−T68/K68 |
|
| |||||||||||||||
| 2.04 | 199.57969 | 8035 | 7965 | 7907 | 19 9179 | 575 | 19 | 26 | 7.60 | 0 843234 | 19.6000184 | 50.098428 | −0.0468 | −0.0462 | −0.0390 |
| 11.01 | 167.81883 | 1936 | 1882 | 1832 | 19.9868 | 607 | 15 | 29 | 8.03 | .879305 | 18.3000156 | 46.732459 | −.0442 | −.0438 | −.0381 |
| 1.12 | .05212 | 5216 | 5187 | 5234 | 19.9569 | 611 | 0 | 41 | 10.95 | 1.143320 | 11.3926111 | 28.955979 | −.00002 | −.0002 | −.0002 |
| 1.13 | .00282 | 271 | 307 | 268 | 19.9797 | 611 | 0 | 41 | 10.96 | 1.144712 | 11.3906113 | 28.950756 | Fiducial | ………. | ………. |
| 6.03 | 327.25501 | 5619 | 5644 | 5239 | 20.0016 | 547 | 35 | 14 | 6.51 | .730605 | 24.8000249 | 63.628995 | −.0601 | −.0587 | −.0444 |
| 8.04 | 391.69635 | 9901 | 9732 | 9272 | 20 0017 | 559 | 45 | 7 | 6.00 | .677523 | 27.4080254 | 70.456473 | −.0836 | −.0821 | −.0634 |
| 10.02 | 457.23666 | 4089 | 3804 | 3107 | 20.0045 | 587 | 54 | 0 | 5.67 | .644306 | 30.0483289 | 77.400202 | −.1092 | −.1075 | − 0842 |
| 10.03 | 457.23976 | 4431 | 4036 | 3459 | 20.0285 | 575 | 54 | 0 | 5.67 | .644577 | 30.0483289 | 77.400044 | −.1138 | −.1121 | −.0888 |
| 10.04 | 457.24714 | 4748 | 5327 | 4066 | 20.0225 | 560 | 54 | 0 | 5.68 | .645384 | 30 0483289 | 77.400303 | −.1188 | −.1170 | −.0937 |
| 10.05 | 457.23765 | 3943 | 3835 | 3518 | 20.0272 | 560 | 54 | 0 | 5.67 | .644916 | 30.0483289 | 77 399842 | −.1136 | −1119 | −.886 |
| 10.06 | 457.23818 | 4196 | 4074 | 3185 | 20.0058 | 500 | 54 | 0 | 5.68 | .646497 | 30.0483989 | 77.400268 | −.1101 | −.1084 | −.0851 |
| 9.03 | 424.93184 | 3321 | 3360 | 2870 | 20.0049 | 500 | 48 | 3 | 5.88 | .667050 | 28.7480296 | 73.977531 | −.0976 | −.0960 | −.0751 |
| 9.04 | 424.93024 | 3153 | 3209 | 2709 | 20.0042 | 500 | 48 | 3 | 5.87 | .665542 | 28.7480296 | 73.977283 | −.0984 | −.0967 | −.0758 |
| 8.05 | 391.71112 | 1223 | 1222 | 891 | 20.0034 | 480 | 45 | 7 | 6.07 | .687159 | 27.4080254 | 70.457598 | −.0878 | −.0863 | −.0676 |
| 10.07 | 457.23859 | 4473 | 3692 | 3411 | 19.9553 | 667 | 51 | 0 | 5.65 | .642461 | 30.0483289 | 77.400091 | −.1009 | −.0992 | −.0759 |
| 9.05 | 424.92780 | 2889 | 2977 | 2473 | 19.9956 | 639 | 49 | 3 | 5.84 | .661441 | 28.7480296 | 73.976691 | −.0907 | −.0891 | −.0682 |
| 8.06 | 391.71202 | 1381 | 1263 | 961 | 19.9858 | 635 | 45 | 7 | 6.05 | .683365 | 27.4080254 | 70.457391 | −.0803 | −.0788 | −.0601 |
| 7.03 | 359.55953 | 6005 | 6244 | 5610 | 19.9158 | 635 | 39 | 10 | 6.26 | .704933 | 26.1080274 | 67.050862 | − 0692 | −.0679 | −.0514 |
| 6.04 | 327.27613 | 7628 | 7866 | 7344 | 19.9305 | 627 | 36 | 14 | 6.50 | .729316 | 24.8000949 | 63.629693 | −.0654 | −.0641 | −.0498 |
| 6.05 | 327.27642 | 7648 | 7867 | 7409 | 19.9298 | 627 | 36 | 14 | 6.50 | .729117 | 24.8000249 | 63.629769 | −.0649 | −.0637 | −.0494 |
| 5.06 | 295.24589 | 4676 | 4682 | 4409 | 19.9887 | 667 | 31 | 17 | 6.74 | .752268 | 23.5000240 | 60.235626 | −.0589 | −.0578 | −.0454 |
| 4.05 | 263.30187 | 295 | 171 | 94 | 19.9734 | 615 | 27 | 20 | 7.02 | .780777 | 22.2000212 | 56.850078 | −.0583 | −.0573 | −.0468 |
| 3.04 | 231.41455 | 1508 | 1502 | 1355 | 19.9860 | 579 | 23 | 23 | 7.33 | .811778 | 20.9000191 | 53.470746 | −.0558 | −.0549 | −.0461 |
| 1.14 | 2.40786 | 787 | 762 | 810 | 19.9939 | 500 | 0 | 40 | 10.91 | 1.14086 | 11.4900096 | 29.205208 | −.00001 | −.00001 | +.00003 |
| 1.15 | −.00855 | 851 | 874 | 841 | 20 0051 | 524 | 0 | 40 | 10.97 | 1.14563 | 11.3900086 | 28.949104 | Fiducial | ………. | ………. |
| 3.05 | 231.41455 | 1570 | 1449 | 1345 | 20.0092 | 599 | 22 | 23 | 7.35 | .814564 | 20.9000191 | 53.470813 | −.0551 | −0542 | −.0454 |
| 2.05 | 199.49531 | 9505 | 9614 | 9472 | 19.9819 | 587 | 18 | 26 | 7.72 | .851899 | 19.6000184 | 50.098911 | −.0506 | −.0500 | −.0423 |
| 11.02 | 167.82802 | 2895 | 2784 | 2728 | 19.9700 | 587 | 15 | 29 | 8.02 | .879258 | 18.3000156 | 46.723628 | −.0449 | −.0446 | −.0389 |
| 1.16 | .08860 | 8860 | 8849 | 8870 | 19.9863 | 555 | 0 | 40 | 10.10 | 1.14442 | 11.3940123 | 28.959419 | .00017 | .00017 | .00017 |
| 10.08 | 457.27365 | 7427 | 7405 | 7262 | 19.9872 | 647 | 54 | 0 | 5.67 | .644047 | 30.0483289 | 77.400303 | −.1050 | −.1033 | −.0800 |
| 10.09 | 457.27530 | 7767 | 7593 | 7229 | 19.9846 | 647 | 54 | 0 | 5 67 | .644099 | 30.0483289 | 77.400310 | −.1066 | −.1049 | −.0816 |
| 10.10 | 457.27417 | 7428 | 7685 | 7137 | 19.9864 | 639 | 54 | 0 | 5.67 | .644346 | 30.0483289 | 77.400339 | −.1052 | −.1034 | −.0801 |
| 10.11 | 457.27320 | 7314 | 7602 | 7043 | 19.9864 | 639 | 54 | 0 | 5.67 | .644343 | 30.0483289 | 77.400290 | −.1047 | −.1029 | −.0796 |
| 9.06 | 424.96377 | 6803 | 6335 | 5993 | 19.9716 | 627 | 49 | 3 | 5.85 | .663317 | 28.7480296 | 73.977511 | −.0913 | −.0896 | − 0687 |
| 8.07 | 391.74159 | 4258 | 4223 | 3998 | 19.9660 | 587 | 45 | 7 | 6.06 | .685320 | 27.4080254 | 70.457874 | −.0790 | −.0775 | −.0588 |
| 7.04 | 359.57478 | 7510 | 7576 | 7349 | 20.0132 | 579 | 40 | 10 | 6.28 | .706876 | 26.1080274 | 67.049888 | −.0686 | −.0672 | −.0507 |
| 6.06 | 327.29613 | 9601 | 9679 | 9559 | 19.9712 | 575 | 36 | 14 | 6.51 | .730882 | 24.8000249 | 63.629483 | −.0635 | −.0622 | −.0479 |
| 1.17 | .02188 | 2221 | 2182 | 2160 | 20.1028 | 500 | 0 | 40 | 10.98 | 1.14667 | 11.3910128 | 28.951180 | Fiducial | ……… | ………. |
| 12.01 | 400.06997 | 7221 | 6941 | 6827 | 20.0582 | 619 | 45 | 6 | 6.04 | .683636 | 36.0000341 | 92.567811 | −.0873 | −.0865 | −.0617 |
| 12.02 | 400.07089 | 7205 | 7250 | 6812 | 20.0345 | 635 | 45 | 6 | 6.02 | .681597 | 36.0000341 | 92.567881 | −.0877 | −.0869 | −.0621 |
| 13.01 | 323.87915 | 7933 | 8026 | 7785 | 20.0446 | 615 | 36 | 14 | 6.55 | .734768 | 32.0000308 | 82.093154 | −.0658 | −.0653 | −.0470 |
| 14.01 | 248.07564 | 7583 | 7615 | 7496 | 20.0355 | 619 | 27 | 22 | 7.17 | .796473 | 28.0000326 | 71.669921 | − 0578 | −.0571 | −.0444 |
| 15.01 | 172.63305 | 3289 | 3369 | 3256 | 20.0243 | 595 | 17 | 29 | 7.97 | .874040 | 24.0000336 | 61.296616 | −.0479 | − 0473 | −.0403 |
| 1.18 | .12744 | 2718 | 2756 | 2757 | 20.0106 | 575 | −1 | 36 | 10.95 | 1.14332 | 14.7860234 | 37.580545 | Fiducial | ………. | ………. |
Identification of columns: 1 = arbitrary designation of a gas thermometer measurement, 2 = average t68 determined by 3 PRT’s, 3, 4, 5 last 4 digits of t68 as determined by the thermometer designated by 701, 703, 706, or 707, 6 = vault temperature, 7, 8, 9 = thermocouple readings as discussed in text, 10 = π, relative pressure head effect, 11 = value for dead space ΣkVki/Tki, 12 =gage block values used in manometer, 13= Z, augmented pressure expressed in cm of Hg, 14=difference between values found from gas thermometer and PRT’s, 15 = difference of values in 14 corrected for a pressure head difference between baths, and the thermomolecular pressure effect, 16 = the difference of values from 15 further corrected for the effects of gas imperfection.
To summarize: For all the earlier data for which only values of C9(5) referenced to the top of the header are available, we find the temperature distribution along the tube by the following steps:
Read C7(5) and A1(5) from figure 4. Calculate 6711(5) by E−40 (457−tbath)/457 μV.
Find the emf of a standard couple referred to ice for the top of the header in μV for Pt−10 percent Rh/Pt from Vϕ (tbath)−Eϕ×1.01644, where Vϕ is calculated from the quartic equation and Eϕ is C9(5).
Deduct C7(5) × 0.125 μV to account for the difference in emf corresponding to the difference in temperature between the top of the header and the aluminum reference ring. The equivalent emf for C6(5) can be found if desired.
Add A1(5) × 1.00895 to give a synthesized value of emf referenced to ice.
Find tA1 from the derived emf. Similarly, the temperatures can be found for all other positions including B1.
6.4. Determination of Thermomolecular Pressures
Using the apparatus developed for this purpose, and described in section 2, we measured some thermomolecular pressure differences for helium at temperatures of 140, 260, 374, and 457 °C at pressures varying from 2.5×103 to >1×105 Pa, The results were correlated by an equation
| (10) |
based on Weber’s treatment [16], where the product of the average pressure and the pressure difference Δp is expressed in relation to T2, the upper temperature; T1, the lower temperature (296 K); and T0, the reference temperature (273.15 K). The reference temperature is involved only in that the exponent n is defined by the assumption that the temperature dependence of the viscosity of helium can be expressed by
| (11) |
For the temperature range 0 °C <t <460 °C, n was evaluated as 0.145. A least squares solution gave C1 = 3738 Pa2, with a standard deviation of 1.8 Pa2. The average deviation of the measured values from the equation is 1.8 percent, and the maximum deviation is 3.4 percent. Because C1 varies inversely as the square of the diameter of the tube, the measured value of C1 was modified to account for the smaller diameter of the gas thermometer tube by a factor (0.823/0.904)2, or C1=3098 Pa2.
7. Tabulation of Results
The measurements which will be presented in subsequent tables are subdivided according to experimental groupings labeled Set A to Set I. More information by group is given in table 1.
Table 1.
Designations of sets, with added comments
| Set | Period | Pressure range | Other comments |
|---|---|---|---|
|
| |||
| A | Nov. 15–Dec. 5, 1973. | Intermediate | Effects of creep. |
| B | Jan. 15–Feb.1, 1974 | Intermediate | |
| C | Feb. 6–Feb. 19, 1974 | Intermediate | |
| D | Feb. 20–Mar. 5, 1974 | High | |
|
|
|||
| Gas thermometer vacuum pumped at 457 °C May 10– May 15, 1974
|
|||
| E | May 16–June 6, 1974 | High | |
|
|
|||
| Resistance thermometers calibrated June 1–June 14, 1974
|
|||
| F | June 24–July 1, 1974 | Low | |
| G | July 6–Aug. 8, 1974 | Intermediate | Suspected contamination. |
|
|
|||
| Gas thermometer vacuum pumped at 457 °C August 9–August 12, 1974
|
|||
| H | Aug. 13–Aug. 30, 1974. | Intermediate | |
|
|
|||
| Gas thermometer vacuum Dumped at 920 °C August 31–September 11, 1974
|
|||
| I | Sept. 12–Sept. 13, 1974. | Intermediate | Change of bulb volume. |
The values of the calculated quantities for the states of the gas thermometer are tabulated in tables 2 and 3. The results have been divided into those in table 2, in which temperatures of the header were derived by calculation, and those in table 3 for which the thermocouple emf’s were experimentally observed. The deviations of international temperatures from gas thermometer temperatures were calculated from the equation
| (12) |
where Ti is the absolute temperature of the chosen reference state with the corresponding value of Z=Zi. The difference T′GT/K′GT − T68/K68 was further modified for the effect of the lower level of the gas thermometer bulb in the high temperature bath; the smaller pressure head requires a decrease in all the gas thermometer temperatures of 0.5 ppm except those measured in the low temperature bath. These changes are smaller than the thermomolecular pressure effects that were calculated by eq (10), and that were included in the results in column 15, headed TGT/KGT − T68/K68.
Table 3.
Gas thermometer results, sets E, F, G, H and I
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
| |||||||||||||||
| State | Ave Bath t °C | 701 | 703 | 707 | Vault °C | C6 μV | C7 μV | C11 μV | π | Vτ mm3/K | Gage blocks in | Z cm | T′GT/KGT−T68/K68 | TGT/KGT−T68/K68 | T/K−T68/K68 |
|
| |||||||||||||||
| 9.04 | 400.07781 | 7938 | 7913 | 7493 | 19.9122 | 1049 | 50 | 3 | 5.84 | 0.656597 | 36.0000180 | 92.566491 | −0.0863 | −0.0855 | −0.0607 |
| 9.05 | 400.07842 | 8163 | 7785 | 7577 | 19.9145 | 1041 | 50 | 14 | 5.84 | .656825 | 36.0000180 | 92.566547 | −.0882 | −.0874 | −.0626 |
| 8.01 | 323.88316 | 8329 | 8433 | 8185 | 19.9505 | 1007 | 39 | 27 | 6.37 | .710510 | 32.0000120 | 82.091827 | −.0620 | −.0614 | −.0431 |
| 7.01 | 248.08543 | 8546 | 8605 | 8478 | 19.9266 | 989 | 27 | 32 | 7.00 | .773604 | 28.0000080 | 71.669463 | −.0555 | −.0551 | −.0424 |
| 6.01 | 172.64554 | 4527 | 4590 | 4546 | 19.8996 | 921 | 20 | 45 | 7.82 | .853059 | 24.0000090 | 61.296727 | −.0458 | −.0455 | −.0380 |
| 1.01 | .21285 | 1298 | 1260 | 1298 | 19.4946 | 841 | 4 | 79 | 10.89 | 1.13583 | 14.7900210 | 37.591184 | −.00009 | −.00009 | −.00009 |
| 1.02 | .02653 | 2657 | 2662 | 2641 | 19.9315 | 853 | 5 | 82 | 10.90 | 1.13647 | 14.7800240 | 37.565563 | Fiducial | ………. | ………. |
| 5.01 | 140.68118 | 8090 | 8143 | 8121 | 19.9331 | 883 | 21 | 67 | 8.23 | .893283 | 22.3000130 | 56.902358 | −.0373 | −.0371 | −.0313 |
| 10.01 | 457.29908 | 9784 | x000 | 9939 | 19.9697 | 1153 | 60 | 2 | 5.49 | .620079 | 10.5510989 | 27.177196 | −.1042 | −.0872 | −.0790 |
| 10.02 | 457.29983 | 9816 | x333 | 9801 | 19.9738 | 1158 | 62 | −1 | 5.49 | .619983 | 10.5510989 | 27.177176 | −.1055 | −.0885 | −.0803 |
| 11.01 | 379.56253 | 6289 | 6353 | 6117 | 19.9944 | 1096 | 49 | 16 | 5.97 | .670790 | 9.4508962 | 24.285564 | −.0757 | −.0617 | −.0555 |
| 11.02 | 379.56304 | 6331 | 6412 | 6170 | 19.9939 | 1093 | 49 | 20 | 5.96 | .668803 | 9.4508962 | 24.285539 | −.0769 | −.0629 | −.0567 |
| 1.03 | .06066 | 6070 | 6050 | 6078 | 19.9752 | 914 | 3 | 62 | 10.88 | 1.13410 | 4.0000033 | 10.166566 | Fiducial | ……… | ………. |
| 10.03 | 457.29595 | 9467 | 9880 | 9437 | 20.0108 | 1318 | 57 | −25 | 5.47 | .617593 | 30.0483217 | 77.396673 | −.0960 | −.0889 | −.0656 |
| 10.04 | 457.29569 | 9559 | 9732 | 9417 | 20.0127 | 1318 | 58 | −19 | 5.46 | .616579 | 30.0483217 | 77.396573 | −.0913 | −.0896 | −.0663 |
| 10.05 | 457.29379 | 9446 | 9548 | 9143 | 20.0123 | 1321 | 55 | −46 | 5.47 | .617047 | 30.0483217 | 77.396510 | −.0900 | −.0883 | −.0660 |
| 12.01 | 424.97992 | 8277 | 8039 | 7662 | 20.0264 | 1302 | 52 | −49 | 5.66 | .636436 | 28.7480235 | 73.973390 | −.0785 | −.0769 | −.0560 |
| 13.01 | 391.76711 | 6662 | 6968 | 6503 | 20.0098 | 1231 | 46 | −26 | 5.86 | .657983 | 27.4080211 | 70.454443 | −.0723 | −.0708 | −.0521 |
| 14.01 | 359.61197 | 1100 | 1368 | 1122 | 19.9801 | 1202 | 40 | −5 | 6.08 | .679571 | 26.1080190 | 67.047804 | −.0640 | −.0626 | −.0460 |
| 15.01 | 327.32650 | 2646 | 2677 | 2627 | 19.9761 | 1228 | 39 | −16 | 6.30 | .701470 | 24.8000160 | 63.626934 | −.0596 | −.0584 | −.0441 |
| 16.01 | 231.46721 | 6677 | 6738 | 6747 | 19.9887 | 1128 | 24 | −13 | 7.15 | .786948 | 20.9000176 | 53.469426 | −.0519 | −.0510 | −.0422 |
| 16.02 | 231.46773 | 6751 | 6776 | 6792 | 19.9878 | 1129 | 24 | −1 | 7.15 | .786771 | 20.9000176 | 53.469488 | −.0518 | −.0509 | −.0421 |
| 17.01 | 136.16997 | 6928 | 7004 | 7058 | 19.9771 | 1085 | 12 | 12 | 8.26 | .893907 | 17.0000212 | 43.372362 | −.0358 | −.0355 | −.0311 |
| 1.04 | .02701 | 2679 | 2715 | 2709 | 19.9949 | 1040 | −1 | 24 | 10.85 | 1.12928 | 11.3900096 | 28.948912 | Fiducial | ……… | ………. |
| 10.06 | 457.29574 | 9756 | 9418 | 9549 | 19.9837 | 1165 | 51 | −11 | 5.51 | .623306 | 30.0483217 | 77.397914 | −.1017 | −.1000 | −.0767 |
| 10.07 | 457.29670 | 9884 | 9768 | 9358 | 19.9867 | 1176 | 52 | −12 | 5.51 | .622538 | 30.0483217 | 77.397748 | −.1043 | −.1025 | −.0792 |
| 10.08 | 457.29657 | 9844 | 9777 | 9350 | 19.9857 | 1179 | 52 | −11 | 5.50 | .622227 | 30.0483217 | 77.397772 | −.1039 | −.1022 | −.0789 |
| 12.02 | 424.97991 | 8042 | 8006 | 7926 | 19.9913 | 1213 | 46 | −27 | 5.68 | .639977 | 28.7480235 | 73.974725 | −.0879 | −.0863 | −.0654 |
| 13.02 | 391.76040 | 5917 | 6261 | 5943 | 19.9937 | 1190 | 41 | −28 | 5.88 | .660398 | 27.4080291 | 70.455153 | −.0798 | −.0783 | −.0596 |
| 14.02 | 359.60084 | 1 | 126 | 124 | 19.9980 | 1171 | 37 | −22 | 6.09 | .682020 | 26.1080270 | 67.048033 | −.0706 | −.0693 | −.0528 |
| 15.02 | 327.31830 | 1827 | 1782 | 1882 | 20.0035 | 1172 | 30 | −20 | 6.36 | .709625 | 24.8000240 | 63.627550 | −.0645 | −.0632 | −.0490 |
| 18.01 | 295.29733 | 9749 | 9761 | 9689 | 19.9961 | 1154 | 27 | −21 | 6.61 | .734450 | 23.5000305 | 60.234739 | −.0589 | −.0577 | −.0453 |
| 19.01 | 263.34529 | 4570 | 4505 | 4513 | 19.9825 | 1175 | 22 | −20 | 6.87 | .760698 | 22.2000277 | 56.848808 | −.0572 | −.0562 | −.0456 |
| 20.01 | 231.45545 | 5538 | 5528 | 5568 | 19.9956 | 1155 | 20 | −6 | 7.17 | .789847 | 20.9000256 | 53.469639 | −.0540 | −.0531 | −.0443 |
| 21.01 | 199.62867 | 2848 | 2866 | 2886 | 20.0017 | 1156 | 16 | −7 | 7.50 | .821595 | 19.6000301 | 50.097231 | −.0500 | −.0494 | −.0417 |
| 1.05 | .01667 | 1671 | 1654 | 1677 | 20.0060 | 1105 | −3 | 3 | 10.83 | 1.12683 | 11.3900096 | 28.948732 | Fiducial | ………. | ………. |
| 21.02 | 199.62796 | 2762 | 2790 | 2836 | 19.9792 | 1075 | 23 | −9 | 7.48 | .819122 | 19.6000301 | 50.098373 | −.0503 | −.0497 | −.0420 |
| 1.06 | .01724 | 1734 | 1727 | 1711 | 19.9817 | 990 | −3 | 37 | 10.86 | 1.13133 | 11.3900096 | 28.949522 | Fiducial | ……… | ……… |
8. Review of Previously Published Results
The results reported in our last paper [10] were calculated by programs similar to those used for the present data. The PYE and DEDSPS programs derived temperatures in the manner described as our “original method,” and the SUMMA program differed in the form of the equation and the constants used for the thermal expansion of the bulb, with a trivial effect on the results. Both of these differences can be expected to produce a difference less than 0.5 mK from the present approach. The original data and some of the quantities derived from intermediate calculations are given in table 4, columns (1) to (10), and the values reported previously are given in column (11). As stated in section 2, the actual volume of the bulb was found to be different from the value used in the original SUMMA program. The change necessitated by correcting the volume of the bulb is given in column (12). A further adjustment for the effects of thermomolecular pressure, as determined by eq (10), is given in column (13).
9. The Effect of Gas Imperfection
When the numerical differences, TGT/KGT−T68/K68, for the same temperature obtained at nearly constant pressure ratio but over different ranges of pressure are extrapolated vs the upper pressure to zero pressure, the intercept is T/K−T68/K68. Alternatively, if the equation of state for 1 mol of gas is considered in the form pV=RT+Bp, the thermodynamic temperature T is related to a gas thermometer temperature TGT by
| (13) |
where j’s refer to the measuring state, and i’s to the reference state, and R to the molar gas constant. Considered in terms of this equation, the accuracy with which the thermodynamic temperature can be found by extrapolation will be optimized when the range of pj’s is large, so long as the lowest pi is still large enough to be realized with the same relative accuracy as large values. However, at low pressures, thermomolecular pressure effects become important. For instance, two pressure ranges were used at 100 °C. For pj = 1×105 Pa, the combined thermomolecular pressure effects for pj and pi are 0.34 ppm, while for pj= 18374 Pa, the combined thermomolecular pressure effects are 10.6 ppm. For all values in Sets A, B, C, G, H, and I, all pj’s were above 5×104 Pa, so that the thermomolecular pressure affects the gas thermometer temperature by at most 2 mK.
Unfortunately, the number of measurements at varying pressure ranges was insufficient to permit precise extrapolation to zero pressure. An alternative to extrapolating is to calculate the effects of gas imperfection from independently determined second virial coefficients of helium. Our original decision to rely upon extrapolation involved our appraisal that the use of virials from the literature would not give as accurate thermodynamic temperatures. This remains our conclusion, provided there are sufficient gas thermometer measurements;6 in the interim we are compelled to use literature values of virials to derive thermodynamic temperatures, but with more uncertainty than we would expect when our own measurements are sufficiently complete.
Second virial coefficients calculated from compressibility measurements are probably more accurate than from other sources; the Burnett method [17] is probably the most accurate of the experimental compressibility techniques. We have chosen a set of virial coefficients based upon the values obtained at the NBS by the Burnett method for temperatures up to 150 °C [18]. The values at higher temperatures are those of Yntema and Schneider [19], who also used the Burnett method. The interpolation and smoothing were made consistent with acoustic results of Gammon and Douslin [20]. The results from these three sources differ from each other substantially more than their respective estimated uncertainties. The values used and the equation derived by a least squares fit to the data are given in table 5.
| (14) |
Table 5.
The second virial coefficient of Helium
| t (°C) | B (cm3/mol) |
|---|---|
|
| |
| 0 | 12. 00 |
| 25 | 11. 89 |
| 50 | 11. 77 |
| 75 | 11. 67 |
| 100 | 11. 56 |
| 125 | 11. 46 |
| 150 | 11. 36 |
| 300 | 10. 76 |
| 400 | 10. 45 |
| 500 | 10. 14 |
| 600 | 9. 82 |
The effects of gas imperfection increase with increasing temperature and, of course, with increasing pressure. It amounts to 0.0227 K for T2=730 K and p= 105 Pa, and only 0.0002 K for T2=293.81 K and p =14500 Pa. The multiplying factor on B1, f =pi/pj·Tj/Ti, is always greater than unity above 0 °C, therefore the sign of the difference of the virials B2−fB1, is always negative. Consequently, the thermodynamic temperature calculated from eq (13) is always greater than the gas thermometer temperature.
The results corrected for gas imperfection are given in the final column of tables 2, 3, and 4, and represent the difference between international and thermodynamic temperatures. An equation giving the deviation as
| (15) |
was fitted to all the values in tables 2, 3, and 4 by least squares. The constants found are given in table 6.
Table 6.
Constants for calculation of T/K−T68/K68 (eq (15))
| A1= −1.208877838 × 105K682 |
| A2= + 1.2135329499 × 103K68 |
| A3= −4.3159552 |
| A4= + 6.4407564676 × 10−3 K68−1 |
| A5=−3.5663884587 × 10−6 K68−2 |
The estimate of the standard deviation of the fit,
| (16) |
where N is the number of points and M is the number of constants, is 1.52 mK. The estimate of the standard deviation of a predicted point varies from 0.75 mK near the triple point, to 0.34 mK in the middle of the range, to 0.46 mK near the upper end. The values and the calculated curve are shown graphically in figure 5. The least squares computer program is given in appendix 6 and the computer printout of the least squares solution is given in appendix 7.
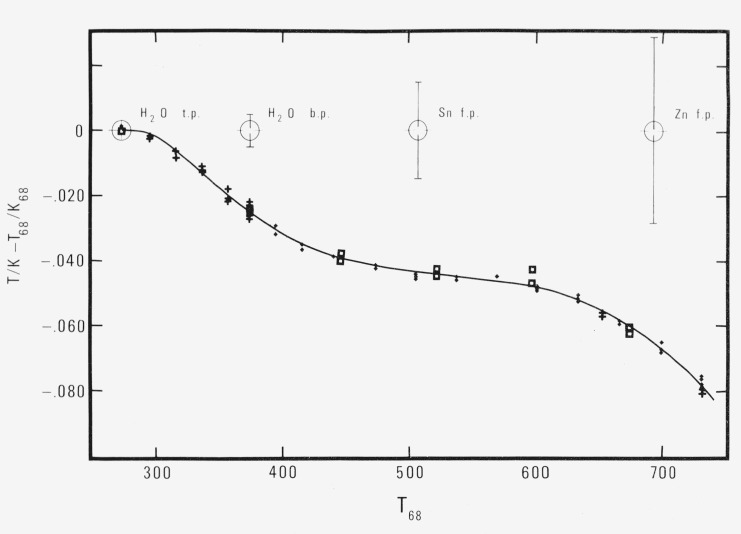
Figure 5. Deviation of International Practical Temperatures from thermodynamic temperatures in the range from 273 to 730 K.
The estimated uncertainties at the defining fixed points published in the original text of the IPTS-68 are shown by the error bars. Key: □ high pressure range, ♦ medium pressure range, + low pressure range.
10. Study of IPTS Realization Above 300 °C
Above 200 °C, the values of temperature determined from 3 standard PRT’s differed from one another at the same temperature by more than might be expected from the calibration results. The average deviation from the mean of their temperature values versus t68 was derived from columns 2, 3, 4, and 5 of tables 2, 3, and 4 and is shown in figure 6.
Figure 6.
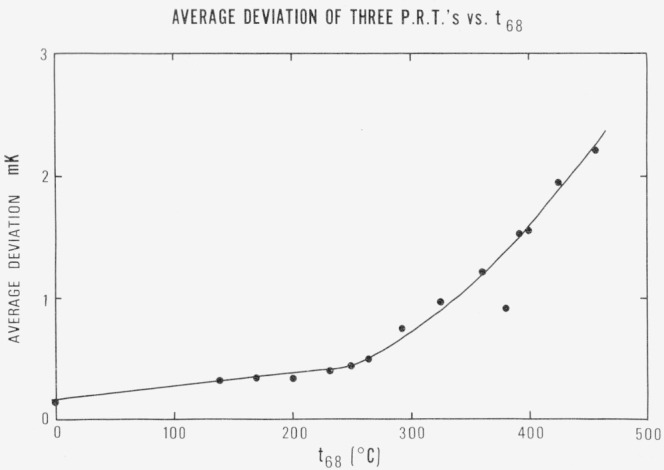
The average deviation of three PRT’s versus the average IPTS temperature.
Subsequent to all the reported gas thermometer measurements, we carried out special measurements at 408 and 414 °C to study this apparent contradiction. It has already been noted that the triple point resistances of the thermometers usually increased with time of immersion in the stirred liquid thermostat of the gas thermometer. But we have also observed that the triple point resistance, if already in substantial excess of its calibration (annealed) value, decreases initially when placed in the thermostat at 457 °C. We now interpret this fact as resulting from a balance between the rate of increase of the resistance from work hardening of the platinum by the mechanical acceleration from the stirring and the rate of decrease of the resistance by annealing of the platinum at 457 °C. At somewhat lower temperatures (350 to 420 °C) the rate of increase from work hardening remains high, but the annealing rate is substantially smaller, so that after the thermometers had been in the thermostat, a rise in the triple point resistance was observed. We found the triple point resistances to increase progressively with succeeding periods in the thermostat at 408 °C, and, although the average temperature remained nearly constant—both in fact and as calculated from the average of the values determined from the thermometers—the average deviation from the mean increased successively from 1.63 mK to 1.89 mK to 2.13 mK. When annealed before each measurement, the average deviation from the mean became 0.65 mK and remained essentially constant with further cycles. The precision of the values of t68 was the same after ½ h anneals as after 2½ h anneals. Although we also used different annealing temperatures, the best choice, on the basis of Berry’s isochronal step depression results [13], is probably 450 °C. Some conclusions one may draw:
Platinum resistance thermometers become very sensitive to mechanical shock at temperatures in excess of 350 °C; and up to 457 °C, the calculated temperature determined by them tends to drift in value with progressive work hardening. For relatively minimal lengths of exposure these increases were limited to steps of resistance corresponding to less than 0.30 mK.
The direction of change of the resistance of a PRT at the zinc point from its annealed value because of work hardening is unpredictable on an a priori basis. For the thermometers we happened to use, the average of the values of t68 calculated from the measurements were a good approximation (within 1 mK) of the true value of t68, whether determined from the annealed thermometers, or from thermometers of the same group which exhibited the effects of work hardening.
The values of t68 calculated for a given temperature as measured with a group of thermometers kept as closely as possible in an annealed condition, are in good agreement with one another. The best choice of annealing temperature is probably 450 °C, and at least for thermometers only slightly work hardened, a half-hour of annealing is sufficient. We believe under our conditions of measurement, the thermometers should be annealed each time before measurement is made, and, as has become our practice, the time during which the thermometers are subjected to mechanical acceleration should be kept as short as possible.
Other causes of measured temperature difference, investigated and found insignificant, were possible temperature gradients in the gas thermometer bulb case and possible imprecision of the thermostat temperature.
11. Estimation of Total Uncertainty
The stated total uncertainty consists of the limits, at 99 percent confidence level, for the random errors; and the systematic errors, estimated conservatively enough to warrant about the same confidence level. We discriminate between errors which fall into three categories:
Sources of random error which contribute to the imprecision observed in the results.
Sources of error which produce a constant bias in the results, but which are estimable in terms of random errors determined from other experiments.
Sources of “systematic” error which produce a constant bias in the results, but for which there is inadequate experiment and theory to permit their evaluation by accepted statistical techniques.
The estimates of the values of the random errors (A and B) are expressed initially as one standard deviation.
The error of the reported quantity, the deviation of international temperatures from thermodynamic temperatures, T/K−T68/K68, can be estimated by combining in quadrature the errors of realizing the international temperatures with the errors of realizing the thermodynamic temperatures. The errors for each depend upon the range of temperature, and for the thermodynamic temperatures, also upon the range of pressures. There are two values of temperature for which a larger number of values was determined—373 and 730 K. We propose to assess the errors at these two temperatures, being values at or near the extremes of the range, as well as to consider the statistical evaluation of the imprecision derived from fitting the curve in terms of the estimate of the standard deviation of a predicted point.
The errors in the realization of the international temperatures are those from:
- The calibrations. The systems of measuring instruments, fixed points and thermometers is sufficiently exact that for the four standard PRT’s used in the measurements, the constants A and B in the equation
determined in four successive calibrations over a period of two years defined the value of the temperature at the zinc point with an estimated standard deviation of the mean of the thermometers of 0.20 mK.(17) The values of t68 determined by three standard instruments which had the precision of calibration stated in (1) above.
The estimate of the standard deviation of the mean in realizing the international temperature can be evaluated by combination of the estimates of the standard deviations of the mean of the temperature imprecision of the 3 thermometers because of the imprecision of the constants of calibration, S1; and 3 factors involved in the measurement of a temperature: the imprecision of the thermostat bath regulation, S2; the imprecision of the measurement of the resistances, S3, and the uncertainties because of the effects of work hardening discussed in section 10, S4. These are given in table 7.
Table 7.
Errors of realization of t68 estimated standard deviation of the mean
| Element | t68 °C | Type of error
|
||
|---|---|---|---|---|
| A mK68 | B mK68 | Ca mK68 | ||
|
| ||||
| S1 (Calib.) | 457 | 0.45 | ||
| 100 | .30 | |||
| S2 (Thermo.) | 457 | 0.20 | ||
| 100 | .10 | |||
| S3 (Resis.) | 457 | .02 | 0.10b | |
| 100 | .02 | .05 | ||
| S4 (Hard.) | 457 | .33 | ||
| 100 | .07 | |||
| 457 | .21 | .45 | .34 | |
| 100 | .12 | .30 | .086 | |
These values are not to be added with A and B on the basis that their combination with random errors is not philosophically acceptable. They are stated at 1/3 the value we estimate, for easy comparison with the errors of Type A and B.
These are values, suggested by experiment, for the ac effects of shunting by dc leads, i.e., a lossy capacitor.
The approximate gas thermometer temperature was calculated from eqs (2) through (5a) and the thermodynamic temperature was then found by accounting for the effects of thermomolecular pressure and gas imperfection. The approximate gas thermometer temperature can be expressed in terms of the ratio in a way useful for error analysis as
| (18) |
The value of the second term is small relative to TGT, so that the relative errors of the products in the first term are directly reflected as relative errors in the gas thermometer temperature. In the order of the quantities in eq (18), the errors are
-
The relative uncertainty of the pressure ratio,
The error has two main components, an imprecision depending upon the regulation of the pressure and the variability of the diaphragm, and a fixed uncertainty that can be evaluated from the imprecisions in p, g and h. The pressure difference between the gas thermometer and the manometer was measured by the diaphragm transducer before and after the bath temperature readings. The estimates of the standard deviation of the mean were calculated from the deviations for the experimental pressures at 100 and 457 °C and also at the fiducial point.For 457 °C, the estimate of the standard deviation of the gas thermometer temperature from this source, for a pressure of 105 Pa is ±0.23 mKGT. To this should be added a possible random fluctuation of the regulated pressure, estimated in terms of a variance from the manometer setting. This is estimated to amount to ±0.033 Pa at 1 atm (101,325 Pa) and 0.02 Pa at 3.5×104 Pa. These effects combined in quadrature with the variability of the diaphragm are, for one standard deviation, 0.33 mKGT at 105 Pa and 0.52 mKGT at 3.5×104 Pa.
For 100 °C, the estimate of the standard deviation of the mean of the diaphragm readings affects the gas thermometer temperature by only 0.34 mKGT for 105 Pa, and 0.17 mKGT at 1.8×104 Pa. An estimate of random fluctuation in gas thermometer temperature, from the manometer setting at 105 Pa of 0.033 Pa is equivalent to 0.12 mKGT and at 1.8×104 Pa of 0.02 Pa is equivalent to 0.41 mKGT. These errors combined in quadrature are 0.12 mKGT for 105 Pa and 0.44 mKGT for 1.8×104 Pa.
The total uncertainty of the pressure ratio at the manometer is discussed in [5]. It was stated to be ±1.5 ppm at the 99 percent confidence level, or about 0.5 ppm for 1 standard deviation of the mean. The error can be evaluated from measured quantities, so is of a “B” type.
The contribution of the pressure head to the measured pressure in the present temperature range varies between 4.5 ppm and 11.5 ppm and is unlikely to be in error by more than 2 or 3 percent. Therefore, the error of the pressure head correction is relatively insignificant.
- The errors in the thermal expansion measurements used to calculate the thermal expansion of the bulb. It is believed that the systematic effects are relatively small. Therefore, the error can be evaluated from the imprecision of the measurements, and is of type “B”. The volume expansion is calculated from
where(19)
l0 is the length at 0 °C, and t68 is the temperature at which l was determined. As stated in section 6, a fifth order power series was fitted by least squares to the data. The estimate of the standard deviation of a predicted point varied from a minimum of 0.8 part in 107 from 0 to 54 °C, 1.9 part in 107 at 457 °C, and was 1 part in 107 at 100 °C. Therefore, the error contributed to the thermodynamic temperature, expressed as the estimate of a standard deviation is 0.38 ppm at 100 °C and 0.62 ppm at 457 °C.(20) - The uncertainty in the dead space calculation is primarily due to the uncertainty in the determination of temperatures along the connecting tube. These were measured by thermocouples, the measurement problems of which have been discussed earlier in this paper. The effect of the temperature uncertainty can be evaluated from an approximation for the value of Vτ, which is
where is the absolute temperature averaged between the temperatures of the room and the thermostat. We have evaluated the error in the gas thermometer temperatures resulting from a total uncertainty in of δt= ±0.4%×(t−23) °C. For instance at 457 °C, the average temperature, , is assumed uncertain by 1.74 °C for the measurements (corresponding to an uncertainty of about 2.5 °C at t itself). A summary of the error calculations, which become insignificant below 141 °C, is given in table 8.(21)
Table 8.
Estimate of the uncertainty, δtGT, in TGT from the assumed uncertainty, δt, in Thermocouple readings
|
T °C68 |
δt K68 |
K68 |
KGT |
δTGT KGT |
|
|---|---|---|---|---|---|
|
| |||||
| 457 | 1.74 | 515 | 0.0034 | 0.7583 | 0.0026 |
| 425 | 1.61 | 499 | .0032 | .7124 | .0023 |
| 392 | 1.48 | 482 | .0031 | .6670 | .0021 |
| 360 | 1.35 | 466 | .0029 | .6248 | .0018 |
| 327 | 1.22 | 450 | .0027 | .5864 | .0016 |
| 295 | 1.09 | 434 | .0025 | .5438 | .0014 |
| 263 | .96 | 418 | .0023 | .5027 | .0012 |
| 232 | .83 | 402 | .0021 | .4621 | .0010 |
| 200 | .71 | 386 | .0018 | .4224 | .0008 |
| 173 | .60 | 373 | .0016 | .3995 | .0006 |
| 141 | .48 | 357 | .0013 | .3522 | .0005 |
The value of one standard deviation of the mean, Sm, is about ⅓ of the total uncertainty given in the final column.
The remaining sources of error come from uncertainties in the evaluation of thermomolecular pressure and gas imperfection effects. The thermomolecular pressure correction was largest for states 10.01 and 10.02 at 457 °C, where it amounted to 16.4 mKGT. The only significant error in the correction derives from the imprecision of the measurements for determining thermomolecular pressure. The estimate of the standard deviation of the mean for the results at each temperature measurement was 1.1 percent at 140.5 °C, 0.68 percent at 260 °C, 1.1 percent at 374 °C and 0.92 percent at 457 °C. The error is of type B, and amounts to about 1 percent of the calculated correction, which in general is not significant.
The effects of gas imperfection were calculated from the virial coefficients for helium, selected as we described earlier. The largest correction for gas imperfection was made for states 9.04 and 9.05 at 400 °C, but it was almost as large for the numerous measurements at 457 °C with p = 105 Pa. We postulate that the instrument error in the measurements to evaluate Z=pV/RT=1+Bp/RT produces a total uncertainty in Z of at least ±5 ppm, and is that small only for exceptional work. On the basis of a constant error in the virial coefficient of ±0.11 cm3/mol, this in turn makes an error for the combination of B2 − B1 of ±0.16 cm3/mol, which is perhaps that small only in the range 0–150 °C. At least twice that total uncertainty should be assumed for the measurements at 400 °C (states 9.04 and 9.05), and for the measurements at 457 °C. These uncertainties amount to ±4.4 mK for states 9.04 and 9.05 and ±3.7 mK for the higher pressure measurements at 457 °C.
We can summarize the errors in the thermodynamic temperature as being
| (22) |
where Sm(GT) is the estimate of the standard deviation of the mean of the gas thermometer temperature, Sm (Thermp) is the estimate of the standard deviation of the mean of the thermomolecular pressure correction, and Sm (Imp) is the estimate of the standard deviation of the mean of the gas imperfection correction.
The estimate of the standard deviation of the mean of the gas thermometer temperature can in turn be broken up into
| (23) |
where Sm (PR) is the estimate of the standard deviation of the mean of the gas thermometer temperature produced by errors in the pressure ratio.7
Sm (TE) is the estimate of the standard deviation of the mean of the gas thermometer temperature produced by errors in the values of the thermal expansion of the bulb.
Sm (DS) is the estimate of the standard deviation of the mean of the gas thermometer temperature produced by errors in the evaluation of the correction for the deadspace.
The total can be found by addition in quadrature of the values given in table 9.
Table 9.
Standard deviations of the mean, and systematic errors in the realization of thermodynamic temperatures
| Class of error
|
||||
|---|---|---|---|---|
| A (mK) | B (mK) | Ca (mK) | ||
|
| ||||
| 0.33 | ||||
| .52 | 0.37 | |||
| Sm(PR) | .12 | |||
| .44 | .18 | |||
| Sm(TE) | 457 °C | .45 | ||
| 100 °C | .14 | |||
| Sm(DS) | 457 °C | 0.87 | ||
| 100 °C | .10 | |||
| Sm(Thermp) | Negligible | |||
| Sm(Imp) | 1.23 | |||
| .43 | ||||
| .65 | ||||
| .12 | ||||
| .33 | 1.51 | |||
| .52 | .58 | .97 | ||
| .12 | .66 | |||
| .44 | .23 | .16 | ||
The errors of Classes A and B are given as one standard deviation. The estimated values of Class C are divided by 3 in order to give a “common basis” of comparison with A and B.
Thus the estimate of the standard deviation of the mean of T/K−T68/K68 is the combination of A, B, and C from tables 7 and 9, as follows:
The observed imprecision should be consistent with the sum of “A” of table 10 and S4 of table 7 (the observed systematic deviation from work hardening of the PRT’s). These latter are, in quadrature, the following:
5.2×10−4 at 105 Pa and 457 °C
6.6×10−4 at 3.5×104 Pa and 457 °C
1.9×10−4 at 105 Pa and 100 °C
4.8×10−4 at 1.8×104 Pa and 100 °C.
The corresponding “observed imprecision”, the standard deviation of the mean of the experimental values of T/K−T68/K68, at each temperature is
6.6×10−4 for 105 Pa and 457 °C
-
1.02×10−3 for 104 Pa and 100 °C.
The large experimental value at 100 °C may indicate sources of random error that were not adequately appraised (as discussed in [8]). The observed imprecisions can also be evaluated more broadly in terms of the deviations of the 72 values of T/K−T68/K68 from the least-squares fit. The standard deviation of a predicted point for T/K−T68/K68 is 4.6×10−4 at 457 °C and 3.5×10−4 at 100 °C.
Table 10.
Estimate of the standard deviation of the mean and of systematic errors in the determination of T/K−T68/K68
| Class of error
|
||||
|---|---|---|---|---|
| A (mK) | B (mK) | C (mK) | ||
|
| ||||
| 457 °C | 105 Pa 3.5 × 104 Pa |
0.73 | 1.55 1.03 |
|
| 100 °C | 105 Pa 1.8 × 104 Pa |
.38 | .67 .18 |
|
The estimates of the total uncertainty can be derived by adding the estimates of type A and B in quadrature, and multiplying by 3 as the approximate factor from Student's Table for a 99 percent confidence level. The systematic errors, part C, are similarly increased, because they were arbitrarily stated at ⅓ of their estimated values in order to compare them with the values of Types A and B. The summed values for 457 and 100 °C are given in table 11.
Table 11.
Total uncertainty of T/K−T68/K68 at 730 and 373.15 K68
| 457 °C | 105 Pa | 2.7×10−3 random error 4.6×10−3 systematic error |
| 3.5×104 Pa | 2.8×10−3 random error 3.1×10−3 systematic error |
|
| 100 °C | 105 Pa 1.8×104 Pa |
1.2×10−3 random error 2.0×10−3 systematic error 1.8×10−3 random error .54×10−3 systematic error |
12. Discussion
All data, including those published previously, have been interpreted in the light of further information and improved understanding of the apparatus. The reevaluation of the volume of the bulb makes an appreciable difference in the dead space calculation for the data published previously (amounting to as much as 2.8 mK at 414.75 K). In contrast, the difference in composition of the top of the bulb from the remainder causes no net change in bulb volume with temperature (compared with an all 88 percent Pt–12 percent Rh composition) in excess of 1 ppm, because the shape of the distortion is compensating in the volume to the first order. The problem is minimized because the total thermal expansion of the two alloys becomes equal for t~360 °C. Inasmuch as we had used the values for 88 percent Pt–12 percent Rh in the previous calculations there is no error exceeding 1 ppm from this cause in any of the results, old or new.
All the evidence of system cleanliness—RGA scans, diaphragm, and gas thermometer stability, reproducibility before and after gas thermometer bakeouts—supports the proposition that the results are free of significant effects of sorption (except set G which was not included in the final results). Above 0.01 °C, gas thermometer temperatures are increased by the effects of sorption. It is not generally recognized (although it was mentioned in [9]), that our earliest measurements yielded “thermodynamic temperatures” in excess of 373.15 K at the normal boiling point of water; these values declined as the gas thermometer became cleaner. We believe we could adjust the purity of the gas thermometer to produce a selected value of the steam point over a range of about 0.1 K. In the less clean condition, we expect that there would be less precision and stability, but the gas thermometer would probably be deceptively stable even with enough sorption effect to give “Tth” = 373.15 K.
Operating in the higher temperature range emphasized certain problems, viz., the measurement and treatment of dead space temperatures and of thermomolecular pressures, the evaluation of the effects of gas imperfection, and the realization of international temperatures, all of which can be handled better in the future with higher resulting accuracy. We have installed a new gas thermometer that has a much improved thermocouple installation for measuring the dead space temperatures. Special annealing and testing equipment was built to assure that these couples were uniform and had emf’s in close agreement with accepted values. The effects of thermomolecular pressure and gas imperfection are both encountered in any of our gas thermometer measurements, but they can be evaluated with little loss of accuracy by a modification of the customary measuring techniques:
Independent measurement of thermomolecular pressures. The results of some measurements have been reported. Further work is planned where conditions are more exactly defined and more accurate values are obtained at lower average pressures.
Use of relatively small fiducial pressures. Our ability to determine the virial coefficients is limited by our ability to determine thermomolecular pressures. Therefore, the highest accuracy results when only one factor is involved. The measurement of thermomolecular pressures and the theoretical justification for the transfer of results to the gas thermometer will be the subject of a separate paper.
It has recently become possible to operate the gas thermometer with no loss of accuracy in a pressure range much lower than was the limit ten years ago. Of special benefit in this respect is the ten-fold improvement in the calibration of gage blocks. No new manometer errors become significant in a low range of pressure based on a fiducial value of 2.54 cm of Hg. The great advantage of this lower pressure range is that the correction for gas imperfection is sufficiently small that the uncertainty is less than 1 ppm at 457 °C for a pressure of 6.78 cm Hg, and the thermomolecular pressure correction, though large, is measurable with such accuracy that its uncertainty is about the same.
13. Summary and Conclusions
The deviation of international temperatures from thermodynamic temperatures has been evaluated by a constant volume helium gas thermometer between 0 and 457 °C. Previously reported values were modified for a correction in the dead space effect, and also in light of a reinterpretation of the effects of gas imperfection and thermomolecular pressure. The results are consistent with independent measurements of each of these effects. As examples, the total uncertainty, consisting of the estimate of the random errors at the 99 percent confidence limits and the systematic errors, intended to be conservatively enough stated to warrant about the same confidence, was calculated for each of two pressures at 100 and 457 °C. The estimate of the systematic error in each case was appreciably larger for the higher pressure range, but the results agree with those measured in a lower pressure range. Hence the uncertainties for the lower pressures are regarded as the proper ones, and are given following the value of the deviation:
| t | T/K–T68/K68 |
|---|---|
|
| |
| 457 °C | −0.0794 ±2.8 × 10−3 random error ±3.1 × 10−3 systematic error, and |
| 100 °C | −.0252 ± 1.8 × 10−3 random error ±.54 × 10−3 systematic error |
The errors at temperatures between 100 and 457 °C ought to be approximately proportional to the temperature so that one obtains:
| At the tin point, t68 = 231.9681 °C | |
|---|---|
|
| |
| T/K–T68/K68= −0.0439 | ±2.2×10−3 random error ±1.5×10−3 systematic error |
|
| |
| At the zinc point, t68 = 419.58 °C | |
|
| |
| T/K–T68/K68= −0.0658 | ±2.8×10−3 random error ±2.8×10−3 systematic error |
Improved equipment and improved procedures are expected to substantially lower the uncertainties in the next set of measurements, which have been commenced.
APPENDIX I
100 * FORTRAN IV PROGRAM FOR CALCULATION OF IPTS-68 VALUES 110 * FROM PRT RESISTANCE MEASUREMENTS, NAMED “BRIDGE” 120 DOUBLE PRECISION RT(5), R(5), W, A(10), B(10), T(5), T1, Z 130 DIMENSION N(5) 140 CALL DEFINE (1, 7HTCAL74,) 150 CALL DEFINE (2, 4HPRT,) 160 READ (1, 110)(A(J),E(J),J= 1, 10) 170 110 FORMAT(D15.8,2X,D15.8) 180 120 READ (2, 130) (S1, S2, (N(J),FT(J),R(J),J=1,4)) 190 130 FORMAT(2X,F6.2,X,F6.3,(4(/2X,I4,D15.8,D15.8))) 200 WRITE (9, 130)(S1, S2, (N(J), RT(J), R(J), J= 1, 4)) 210 WRITE (9, 150) S1, S2 220 150 FORMAT(/14X, ‘STATE’ F6.2,2X,F6.3,/) 230 DO 240 J= 1, 4 240 W=RT(J)/(CR(J)-0.996D-03) 250 I=N(J)-700 260 T(J) = (-A(I)+DSQRT(A(I)**2-4.*(1.-W)*B(I)))/(2.+ B(I)) 270 Z = 0.45D-03*T(J)*(T(J)/100.-1.)*(T(J)/419.58-1.)*(T(J)/630.74-1.) 280 T(J)=T(J)+Z 290 WRITE (9,230)N(J),T(J) 300 230 FORMAT(‘THERM.NO.’ I3, 3X, ‘ T(I NT.1968) =’ D17.10) 310 240 (ONTINUE 320 T1 = (T(2) + T(3) + T(4))/3 330 WRITE (9, 270) T1 340 270 FORMAT(‘AVE.T(INT.1968) = ’ D17.10,/,/) 350 GO TO 120 360 END TCAL74 0.39849575D-02 -0.58762415D-06 0.39850801D-02 -0.58772119D-06 0.39848143D-02 -0.53762185D-06 0.39849917D-02 -0.58769654D-06 0.39849663D-02 -0.58756733D-06 0.39847884D-02 -0.58757724D-06 0.39848898D-02 -0.58760947D-06 0.39850236D-02 -0.58778636D-06 0.39838174D-02 -0.58757363D-06 0.39855387D-02 -0.58755669D-06 PRT 10 9.01 10.036 12 710 0.27587830D 02 0.25557448D 02 14 701 0.63945402D 02 0.25577651D 02 16 703 0.63731295D 02 0.25492645D 02 18 707 0.63956557D 02 0.25582540D 02
APPENDIX II
100 REM PYE, IN XBASIC, 9.29.75 110 REM USING TWO FILES, DATA AND LSFIT1 115 DBL 120 READ C1, C2, C5, C6 130 DATA 8.74E-6,3E-9,13.0105E-6,.384333E-08 140 DIM Y(18), V(6, 18),G(18),E(18) 150READB0, B1, B2, B3, B4,N(1),N(2),N(3) 160 DATA 135,.545846E-02,.113497E-04,-.152447E-07,.906033 E-11 170DATA1, 4, 12 180FORI= 1TO 13 190PEADG(I) 200NEXTI 210 DATA 62.2,89.6, 116.9, 144.2, 171.6, 198.8,226.3 220 DATA 253.6, 280.9, 308.3, 335.6, 363.0, 390.3 230 FILES DATA,, DISP 240 READ # 1, S0, S1, T9, E0, E1, E2 242 IF END #1 THEM 1090 250 PRINT, 260, S0, S1, T9, E0, E1, E2 260 FMT F7.3, F7.2, F11.5, I6, I5, I5 270 FOR I= 1TO 13 280 READ # 1, E(I) 290 PRINT, 300, E(I), 300 FMT I5 310NEXTI 320 PRINT 330 D2= 0 340 W=0 350 V0=B1*T9 + B2*T9 † 2+B3*T9 † 3+B4*T9 † 4 360 FOR I = 1 TO 13 370 T1 = B0 380 E(I) = 113+(E0-787)*.147+E(I)* 1.00895 390 E(I) =E(I)* 1E-3 400 LET F=B1*T1-E(I)+B2*T1†2+B3*T1†3+E4*T1†4 410 LET G=B1 + 2*B2*T1 + 3*B3*T1†2+4*B4*T1†3 420 T1 = T1-F/G 430 IF ABS(F/G) >2E-4THEN400 440LETD1 = T1+(T1/100)*(-.006+(T1/100-1)*(.04106-7.363E-5*T1)) 450LETY (I)= 1/(D1+273, 15) 460NEXTI 470 CALL LSFIT1 480 FOR I=62 TO 144 490 LET X=B(1)+B(2)*I+B(3)*I †2 500 GOSUB 930 510 NEXT I 520 FOR I = 145TO363 530 LET X=B(4)/(I † 2)+B(5)/I + B(6)+B(7)*I + B(8)*I†2 540 GOSUB 930 550 NEXT I 560 FOR I = 364 TO 390 570 LET X=Y(12)+(Y(13)-Y(12))*(I-363)/27 580 GOSUB 930 590 NEXT I 600 IF V0-E(13)>.1 THEN 700 610 FOR I = 391 TO 472 620 LET X=Y(13) + (1/(T9 + 273.15)-Y(13))*(I-390)/82 630 GOSUB 930 640 NEXT I 650 FOR I=473 TO 688 660 LET X= 1/(T9+273.15) 670 GOSUB 930 680 NEXT I 690 GO TO 780 700 FOR I = 391 TO 526 710 LET X=Y(13) + (1/(T9 + 273.15)-Y(13))*(I-390)/136 720 GOSUB 930 730 NEXT I 740 FOR I=527 TO 688 750 LET X= 1/(T9 + 273.15) 760 GOSUB 930770 NEXT I 780 LET D2=D2-55*(C5-C1+(C6-C2)*T3)*T2 790 T(1)=(E0+E1-787)/40+20 800 T(2)=(E0+E2-787)/40+20 810 FOR I = 1TO2 820 T1=T(I) 830 LET D1=T1+(T1/100)*(-0.006+(T1/100-1)*(0.04106-7.363E-5*T1)) 840 T(I)=D1+273.15 850 NEXT I 860 W=W+ 13/T(2) + 2*(42-D2)/(T(2) + T(1))+30/(T(D+1/Y(D) 870 LET F=4*980.6/(82.07*1013250) 880 PRINT 890 PRINT“ BOOK STATE PI DISPL” 900 PRINT S0; S1; F*W*.0929, D2*.929 910 PRINT 911 WRITE # 3* D2*.929 920 GO TO 240 930 LET T2=1/X-293.15 940 LET T3 = T2+ 20 950 LET W=W+X* (1 + (C1 + C2*T3)*T2) 960 LET D2=D2+(C5-C1+(C6-C2)*T3)*T2 970 RETURN 980 DATA 10.036,9.05,400.07842, 1041, 50, 14 990 DATA 79,207, 488,849, 1266, 1721,2137,2458,2671 1000 DATA 2846, 2979, 3037, 3073 1090 STOP 1100 END
APPENDIX III
460LETA9 = 1 470FORA=2TO3 480FORI=N(A-1)TON(A) 490I FN (A) -N (A-1) = 3THEN 520 500LETN=5 510GOTO530 520LETM= 3 530FORK=1 TOM 5401 FM = 3THEN 570 550LETV(K, I)=G(I)†(K-3) 560GOTO 580 570LETV(K, I)=G(I)†(K-1) 580NEXTK 590NEXTI 600LETZ = N(A) 610GOSUB680 620FORL=1TOM 630LETB(A9)=-V(M+ 1,Z+L) 640LETA9=A9+ 1 650NEXTL 660NEXTA 670 RETURN 680FORI=N (A-1)TON(A) 690LETV(M+1, I)=Y(I) 700NEXTI 710FORI = N(A)+1 TON(A)+M 720FORK=1TOM+1 730LETV(K,I) = 0 740NEXTK 750LETV(I -Z, I)=1 760nexti 770fork= 1tom+ 1 780i fk=m+1 then 810 790lett= 1 800goto840 810lett=-1 820goto850 830lett=-t 840i fk= 1 then910 850forl=1tok-1 860lfts(l) = 0 870FORJ =N (A-1) TON(A) 880LETS(L) = S(L) + V(K, J) * V(L, J) 890NEXTJ 900NEXTL 910FORI = N(A-1)TON(A)+M 920I FK= 1THEN 960 930FORL=1TOK-1 940LETV(K,I)=V(K, I) -S(L)*V(L, I) 950NEXTL 960NEXTI 970IFT< 0THEN 1070 980LETD(K)=0 990FORI =N(A-1) TON(A) 1000LETD(K) = D(K) + V(K,I) *V(K, I) 1010NEXTI 1020LETD= SQR(D(K)) 1030FORI =N(A-1)TON(A)+M 1040LETV(K,I) = V (K, I)/D 1050NEXTI 10601 FT>0THEN 830 1070NEXTK 1080RETURN DATA 10 10.018, 10.08, 457.27365, 647, 54, 0 12 86, 223, 511, 915, 1377, 1900, 2392, 2772, 3017, 3243, 3421, 3548, 3665 14 10.018, 10.09, 457.27530, 647, 54, 0 16 83, 219, 507, 916, 1376, 1903, 2401, 2787, 3033, 3257, 3431, 3545, 3654 18 10.018, 10.10, 457.27417, 639, 54, 0 20 90, 230, 519, 924, 1376, 1890, 2373, 2753, 2998, 3228, 3410, 3531, 3649 22 10.018, 10.11, 457.27320, 639, 54, 0 24 88, 227, 512, 919, 1371, 1894, 2387, 2772, 3019, 3244, 3421, 3535, 3646 26 10.018, 9.06, 424.96377, 627, 49, 3 28 78, 200, 455, 829, 1227, 1698, 2145, 2498, 2733, 2944, 3115, 3221, 3314 30 10.018, 8.07, 391.74159, 587, 45, 7 32 71, 180, 405, 728, 1078, 1491, 1889, 2209, 2433, 2635, 2805, 2897, 2990 34 10.018, 7.04, 359.57478, 579, 40, 10 36 63, 160, 359, 640, 948, 1312, 1662, 1950, 2161, 2350, 2513, 2607, 2684
APPENDIX IV
100 REM DEDSPS, IN XBASIC,9.29.75 110 REM WITH THREE FILES: “DATA, ” GIVING S0, S1, T9, E0, E1,E2 AND 120 REM A1 THROUGH B1; “DATA2, ” LISTING VALUES OF “A” FOR I.D. 130 REM OF TUBE, AND LSFIT1 FOR LEAST SQUARES. 140 DBL 150 READ C1, C2, C3, C4 160 DATA 8.7252E-6, 2.5279E-9, 3.00786E-12, 2.67373E-15 170 DIM Y(18),V(6, 18),G(18),H(18),E(18),P(390) 180READB0, B1, B2, B3, B4/N(1), N(2)/N(3) 190 DATA 135,.545846E-02,.113497E-04,-.152447E-07,.906033E-11 200 DATA1, 4, 12 210 FOR I = 1TO 13 220 READ H(I) 230NEXTI 240 DATA 62.2,89.6,116.9,144.2,171.6,198.8,226.3 250 DATA 253.6,280.9, 308.3, 335.6, 363,390.3 260 FILES DATA, DATA2, DISP 270 FOR I = 1TO 390 280 READ #2,A 290 P(I) = 0.90123+2.748E-4*A 300NEXTI 310 READ # 1,S0,S1,T9,E0,E1,E2 320 IF END #1 THEN 1210 330 READ # 3,Z2 340 PRINT Z2 350 PRINT, 360,S0,S1,T9,E0,E1,E2 360 FMT F7.3,F7.2,F11.5,I6,I5,I5 370FORI=1 TO 13 380 G(I)=H(I)-Z2 390NEXTI 400 H2= 0 410 IF Z2<.5 THEN 450 420 H2=H2+1 430 Z2=Z2-1 440 GOTO410 450 PRINT 460 FOR I = 1 TO 13 470 READ # 1, E(I) 480 PRINT,490,E(I), 490 FMT I5 500NEXTI 510 PRINT 520 V0=B1* T9 + B2* T9 † 2+B3*T9 † 3+B4*T9† 4 530FORI=1 TO 13 540 E(I) = (113+ (E0-787)*.147+E(I)* 1.00895)/1000 550 H1 = E(I) 560 GO SUB 1140 570 Y(I) = 1/(D1 + 273.15) 580NEXTI 590 CALL LSFIT1 600 D1=(E0-787)/40+20 610 D3=E2/40 620 D4 = E1/40 630 T6 = D1 + D3 640 D2=T6-23 650 X7=(1+ (C1 + C2*T6+C3*T6† 2+C4*T6† 3)* D2) †3 660 V5=5.367*X7/(T6+273.15) 670 FOR I= 1TO 7 680 GO SUB 1100 690NEXTI 700 FOR I=8TO42-H2 710 T6=D1+D4+(D3-D4)*(42-H2-I)/(42-H2-8) 720 GO SUB 1100 730NEXTI 740 FOR I=43-H2TO62-H2 750 T6= 1/Y(1) + (D1 + D4+273.15-1/Y(1))*(62-H2-I)/20-273.15 760 GO SUE 1100 770NEXTI 780PRINT 790 FOR I = 63-H2TO 144-H2 800 T6= 1/(B(1)+B(2)*I+B(3)*(I†2))-273.15 810 GO SUB 1100 S20NEXTI 830 FOR I=145-H2TO363-H2 840 T6= 1/(B(4)/(I † 2) +B(5)/I + B(6) + B(7) * I + B(8) *(I † 2))-273.15 850 GO SUB 1100 860NEXTI 870 FOR I = 364-H2TO390-H2 880 T6=L/((Y(13)-Y(12))*(I -(363-H2))/27+Y(12))-273.15 890 GO SUE 1100 900NEXTI 910 I = 390 920 IF V0-E(13) >.1 THEN 1020 930 FOR J= 391-H2 TO 472 940 T6=1/(Y(13) + (1/(T9 + 273.15)-Y(13))*(J-(390-H2))/(82+H2))-273.15 950 GOSUB 1100 960NEXTJ 970 FOR J=473 TO 526 980 T6=T9 990 GOSUB 1100 1000NEXTJ 1010 GO TO 1060 1020 FOR J = 391-H2 TO 526 1030 T6=1/(Y(13) + (1/(T9 + 273.15)-Y(13))*(J-(390-H2))/(136+H2))-273.15 1040 GO SUE 1100 1050NEXTJ 1060 PPIMT“ BOOK STATE SUM V*TAU” 1070 PPINT S0;S1;V5 1080 PPINT 1090 GO TO 310 1100 D2=T6-23 1110 X7=(1+(C1+C2*T6+C3*T6† 2+C4*T6† 3)*D2) †3 1120 V5=V5+.735395*P(I)†2*.929*X7/(T6+273.15) 1130RETURN 1140 T1 = B0 1150 F=B1*T1-H1 + B2*T1 † 2+B3*T1† 3 + B4*T1† 4 1160 LET G=B1 + 2*B2*T1 + 3*B3*T1†2+4*B4*T1†3 1170 T1=T1-F/G 1180 IF ABS(F/G)>2E-4 THEM 1150 1190 D1 = T1 + (T1/100)* (-.006+(T1/100-1)*(0.04106-7.363E-5*T1)) 1200RETURN 1210 STOP 1220 END
APPENDIX V
100 * PROGRM “SUMMA”, REVISED APRIL 21, 1975 110 * FORTRAN IV DOUBLE PRECISION, BULE IIIB, DIAPHRAGM IA 120 DOUBLE PRECISION C, C1,C2,C3,C4, V, T, E1, E2, E3, E4 130 DOUELE PRECISION H, H1, T9, PI, V9, D 1, C5, C6 140 DOUBLE PRECISION T1, T2, T3, T4, P, Y, Z, W 150 DATA C,V/0.17255D-03, 0.4325D 06/ 160 DATA C1,C2/0.8750894565D-05, 0.2278241267D-08/ 170 DATA C3,C4/-0.2476697519D- 11, 0.3461903664D-14/ 175 DATA C5/-0.1911694941D-17/ 180 (ALL DEFINE (1,6HDATA3,) 190 60 READ(1,65) S0,S1,E1,E2,E3,E4 200 65 FORMAT(4X,F6.3,X,F6.2,X,D9.2,X,D9.2,X,D9.2,X,D9.2) 210 WRITE (9,65) S0,S1,E1,E2,E3,E4 220 READ (1,75) T,H,H 1 230 75 FORMAT (4X,D13.6,X,D13.6,X,D10.3) 240 WRITE (9, 75) T,H,H 1 250 READ (1,85) T9,PI,V9,D1 260 85 FORMAT (4X, D15.8,X, D13.6,X, D13.6, X, D9.2) 270 WRITE (9,85) T9, PI, V9, D1 280 T1 = T+E1/0.4858D 03 290 T2=T+E2/0.1012D 04 300 T3=T+E3/0.4048D 03 310 T4=T+E4,0.4858D 03 320 H=(H + H1* lD-06)*0.254D 01 330 P=-C*H*(T2-0.2D 02)+0.227D-07*H*H-0.1D-03*H 340 H=H+P+0.6085D-03*((T3+T4),0.2D 01-T1) + 0.1108D-04* (T 1-0.2D 02) 350 H=H+ 0.1289D-03+0.1324503D-05*D1 355 C6= C1 + C2*T9 + C3*T9**2+C4*T9**3+C5*T9**4 360 Y=((0,1D 01+C6*T9)**3)-0.1D 01 370 W= PI+Y+ PI*Y+(T9 + 0.27315D 03+0.133053D-02*H)*V9/V 380 Z=H+H*W 390 WRITE (9, 240) S0,S1,Z 400 240 FORMAT (‘ BOOK ’ 4X, ‘ STATE’ 18X, ‘Z’,F6.3,X,F6.2,4X,D17* 10) 410 GO TO 60 420 END DATA3 10 10.03615.01 -0.47D 02 -0.20D 01 -0.15D 02 -0.24D 02 12 0.199761D 020.248000D 020.160D 02 14 0.32732650D 030.629742D-050.701470D 000.18D 02 16 10.03616.01 -0.50D 02 -0.40D 01 -0.16D 02 -0.25D 02 18 0.199887D 020.209000D 020.176D 02 20 0.23146721D 030.714875D-050.736947D 00 -0.50D 01 22 10.03616.02 -0.50D 02 -0.60D 01 -0.15D 02 -0.25D 02 24 0.199878D 020.209000D 020.176D 02 26 0.23146773D 030.714704D-050.786771D 000.29D 02 28 10.03617.01 -0.51D 02 -0.20D 01 -0.16D 02 -0.27D 02 30 0.199772D 020.170000D 020.212D 02 32 0.13616997D 030.825908D-050.893907D 00 -0.20D 01 34 10.0361.04 -0.53D 020.00D 00 -0.16D 02 -0.27D 02 36 0.199949D 020.113900D 020.960D 01 38 0.27010000D-010.108452D-040.112928D 01 -0.10D 01
APPENDIX VI
5 DIM Y(90), V(6, 90) 6 DIM B(90), W(90) 7 FILES L5DAT 10 PPINT “INPUT: NO.OF CONSTANTS” 20 INPUT M 110 I = 1 111 GO TO 115 112 I=I+1 115 READ # l,B(I),Y(I) 116 IF END #1 THEN 260 117 B(I)=B(I) + 273.15 120 W(I)= 1 130 V(1, I)=1/B(I)† 2 140 V(2, I) = 1/B(I) 150 V(3,I) = 1 160 V(4, I) =B(I) 170 V(5, I) = B(I) † 2 180 V(6, I) = B(I) † 3 250 GO TO 112 260 N=I- 1 261 PRINT 262 PRINT “N =” N 263 PPINT 270 GOSUE 313 280STOP 313LETN 1 = 0 315FORI=1TON 316LETV(M+1,1)=Y(I) 319LETM 1=N 1+ 1 321NEXTI 333FORI =N+1TON + M 344FORK=1TOM+ 1 355LETV(K,I)=0 366NEXTK 377LETV(I-N,I)=1 399NEXTI 411FORK=1TOM+ 1 4121 FK=M+1THEN417 415LETT= 1 416GOTO422 417LETT=-1 413GOTO433 420LETT=-T 422I FK= 1THEN511 433FORL=1TOK- 1 444LETS(L) = 0 455FORJ=1TON 466 S(L)=S(L)+V(K,J)*V(L,J)*W(J) 477NEXTJ 488NEXTL 511FORI=1TON+M 533IFK=1THEN577 544FORL= 1TOK- 1 555LETV(K,I)=V(K,I)-S(L)*V(L,I) 566NEXTL 577NEXTI 600IFT< 0THEN699 611LETD(K) = 0 622FORI=1 TON 633 D(K) = D(K)+V(K, I)*V(K, I)*W(I) 644NEXTI 655LETD=SQR(D(K)) 666FORI=1TON+M 677LETV(K, I)=V(K, I)/D 688NEXTI 693IFT> 0THEN420 699NEXTK 922LETS= 0 933FORI=1TON 944 S=S+V(M+ 1, I) * V(M+ 1, I)*W(I) 955NEXTI 966LETS=SQR(S/(N1-M)) 1111PRINT“TERM”, “COEFFICIENT”, “STD.DEV.” 1122PRINT 1133FORL= 1 TOM 1144 LET C(L) =0 1155 FORK=LTOM 1166LETC(L)=C(L)+V(K, N+L)*V(K,N+L) 1177NEXTK 1188LETC(L)=S*SQR(C(L)) 1199PRINTL,-V(M+1,N+L>, C(L) 1211NEXTL 1222PRINT 1233PRINT 1244PRINT“STANDARD DEVIATION=”S 1255PRINT 1266PRINT 1277 PRINT“B(I) ”, “Y (I) ”, “Y”, “DEV.” 1288PRINT 1299FORI =1 TON 1311 PRINT B(I);Y(I) ;Y(I)-V(M+ 1, I) ; V(M+ 1, I) 1313 X=X+V(M+ 1, I) 1322NEXTI 1323 PRINT 1324 PRINT “SUM OF RESIDUALS =” X 1333 RETURN 6000 END 1400 457.29, .0790, 457.29, .0803., 457.29, .0800, 457.29, .0801 1410 457.29, .0767, 457.29, .0792, 457.29, .0789, 457.29, .0759 1420 457.29, .0816, 457.29, .0796 1430 424.98, .0654, 424.98, .0687, 424.98, .0632 1440 400.08, .0607, 400.08, .0626, 400.08, .0617, 400.08, .0621 1450 391.76, .0596, 391.76, .0588, 391.76, .0601 1460 379.56, .0555, 379.56, .0567 1470 359.56, .0514, 359.56, .0507, 359.56, .0528 1480 327.32, .0490, 327.32, .0493, 327.32, .0494, 327.32, .0479 1490 323.88, .0431, 323-88, .0470 1500 295.30, .0453, 295.30, .0454 1510 263.35, .0457, 263.35, .0468 1520 248.09, .0424, 248.09, .0447 1530 231.45, .0443, 231.45, .0461, 231.45, .0454 1540 199.63, .0417, 199.63, .0420, 199.63, .0423 1550 172.65, .0380, 172.65, .0403 1560 167.83, .0389 1570 141.59, .0372, 141.59, .0354 1580 120.78, .0320, 120.73, .0296 1590 100, .0247, 100, .0252, 100, .0249, 100, .0263, 100, .0220, 100, .0235 1600 100, .0274 1610 82.79, .0217, 82.79, .0220, 82.79, .0178 1620 62.06, .0113, 62.06, .0124, 62.06, .0125 1630 41.36, .0065, 41.36, .0082 1640 20.66, .0025, 20.66, .0030 1650 2.4079, -.00003 1660 .0886, -.00017 1670 .2129, .00009 1680 .0521, .00002 1690 0.0100, 0
APPENDIX VII
| TEPM | COEFFICIENT | STD.DEV. |
|---|---|---|
| 1 | 120887.7838 | 6691.4096838 |
| 2 | −1213.5329499 | 62.65908179 |
| 3 | 4.3159552 | .21163095 |
| 4 | −.64407564676E-02 | .30556699962E-03 |
| 5 | .35663884587E-05 | .15950626366E-06 |
STANDAFD DEVIATION =.15230847863E-02
| B(I) | Y (I) | Y | DEV. |
|---|---|---|---|
| 730.44 | .79000000000E-01 | .79392362821E-01 | −.39236282133E-03 |
| 730.44 | .80300000000E-01 | .79392362821E-01 | .90763717880E-03 |
| 730.44 | .80000000000E-01 | .79392362821E-01 | .60763717865E-03 |
| 730.44 | .80100000000E-01 | .79392362821E-01 | .70763717862E-03 |
| 730.44 | .76700000000E-01 | .79392362821E-01 | −.26923628212E-02 |
| 730.44 | .79200000000E-01 | .79392362821E-01 | −.19236282138E-03 |
| 730.44 | .78900000000E-01 | .79392362821E-01 | −.49236282130E-03 |
| 730.44 | .75900000000E-01 | .79392362821E-01 | −.34923628212E-02 |
| 730.44 | .81600000000E-01 | .79392362821E-01 | .22076371787E-02 |
| 730.44 | .79600000000E-01 | .79392362821E-01 | .20763717875E-03 |
| 698.13 | .65400000000E-01 | .67446957072E-01 | −.20469570721E-02 |
| 698.13 | .68700000000E-01 | .67446957072E-01 | .12530429280E-02 |
| 698.13 | .68200000000E-01 | .67446957072E-01 | .75304292793E-03 |
| 673.23 | .60700000000E-01 | .60436414255E-01 | .26358574537E-03 |
| 673.23 | .62600000000E-01 | .60436414255E-01 | .21635857454E-02 |
| 673.23 | .61700000000E-01 | .60436414255E-01 | .12635357453E-02 |
| 673.23 | .62100000000E-01 | .60436414255E-01 | .16635857454E-02 |
| 664.91 | .59600000000E-01 | .58479128545E-01 | .11208714551E-02 |
| 664.91 | .58800000000E-01 | .58479128545E-01 | .32037145507E-03 |
| 664.91 | .60100000000E-01 | .58479128545E-01 | .16208714551E-02 |
| 652.71 | .55500000000E-01 | .55930437313E-01 | −.43043731304E-03 |
| 652.71 | .56700000000E-01 | .55930437313E-01 | .76956268638E-03 |
| 632.71 | .51400000000E-01 | .52511784289E-01 | −.11117842889E-02 |
| 632.71 | .50700000000E-01 | .52511784289E-01 | −.18117842888E-02 |
| 632.71 | .52800000000E-01 | .52511784289E-01 | .28821571121E-03 |
| 600.47 | .49000000000E-01 | .48688352293E-01 | .31164770716E-03 |
| 600.47 | .49800000000E-01 | .48688352293E-01 | .11116477072E-02 |
| 600.47 | .49400000000E-01 | .48688352293E-01 | .71164770717E-03 |
| 600.47 | .47900000000E-01 | .48688352293E-01 | −.78835229279E-03 |
| 597.03 | .43100000000E-01 | .48383358275E-01 | −.52833582746E-02 |
| 597.03 | .47000000000E-01 | .48383358275E-01 | −.13833582745E-02 |
| 568.45 | .45300000000E-01 | .46431954863E-01 | −.11319548632E-02 |
| 568.45 | .45400000000E-01 | .46431954863E-01 | −.10319548632E-02 |
| 536.5 | .45700000000E-01 | .45060865144E-01 | .63913485577E-03 |
| 536.5 | .46800000000E-01 | .45060865144E-01 | .17391348557E-02 |
| 521.24 | .42400000000E-01 | .44511634947E-01 | −.21116349468E-02 |
| 521.24 | .44700000000E-01 | .44511634947E-01 | .18836505315E-03 |
| 504.6 | .44300000000E-01 | .43862096791E-01 | .43790320916E-03 |
| 504.6 | .46100000000E-01 | .43862096791E-01 | .22379032092E-02 |
| 504.6 | .45400000000E-01 | .43862096791E-01 | .15379032091E-02 |
| 472.78 | .41700000000E-01 | .42088479685E-01 | −.38847968548E-03 |
| 472.78 | .42000000000E-01 | .42088479685E-01 | −.88479685445E-04 |
| 472.78 | .42300000000E-01 | .42088479685E-01 | .21152031448E-03 |
| 445.8 | .38000000000E-01 | .39573254893E-01 | −.15732548930E-02 |
| 445.8 | .40300000000E-01 | .39573254893E-01 | .72674510700E-03 |
| 440.98 | .38900000000E-01 | .38990228025E-01 | −.90228024703E-04 |
| 414.74 | .37200000000E-01 | .34956871926E-01 | .22431280741E-02 |
| 414.74 | .35400000000E-01 | .34956871926E-01 | .44312807410E-03 |
| 393.93 | .32000000000E-01 | .30615283123E-01 | .13847168776E-02 |
| 393.93 | .29600000000E-01 | .30615283123E-01 | −.10152831225E-02 |
| 373.15 | .24700000000E-01 | .25233918747E-01 | −.53391874735E-03 |
| 373.15 | .25200000000E-01 | .25233918747E-01 | −.33918747361E-04 |
| 373.15 | .24900000000E-01 | .25233918747E-01 | −.33391874734E-03 |
| 373.15 | .26300000000E-01 | .25233918747E-01 | .10660812526E-02 |
| 373.15 | .22000000000E-01 | .25233918747E-01 | −.32339187474E-02 |
| 373.15 | .23500000000E-01 | .25233918747E-01 | −.17339187473E-02 |
| 373.15 | .27400000000E-01 | .25233918747E-01 | .21660812527E-02 |
| 355.94 | .21700000000E-01 | .20071511472E-01 | .16284885281E-02 |
| 355.94 | .22000000000E-01 | .20071511472E-01 | .19284885280E-02 |
| 355.94 | .17800000000E-01 | .20071511472E-01 | −.22715114719E-02 |
| 335.21 | .11300000000E-01 | .13314065430E-01 | −.20140654299E-02 |
| 335.21 | .12400000000E-01 | .13314065430E-01 | −.91406542990E-03 |
| 335.21 | .12500000000E-01 | .13314065430E-01 | −.81406542992E-03 |
| 314.51 | .65000000000E-02 | .66790537379E-02 | −.17905373787E-03 |
| 314.51 | .82000000000E-02 | .66790537378E-02 | .15209462622E-02 |
| 293.81 | .25000000000E-02 | .15210989612E-02 | .97890103884E-03 |
| 293.81 | .30000000000E-02 | .15210989612E-02 | .14789010388E-02 |
| 275.5579 | −.30000000000E-04 | .94616373689E-04 | −.12461637369E-03 |
| 273.2386 | −.17000000000E-03 | .25398657250E-03 | −.42398657250E-03 |
| 273.3629 | .90000000000E-04 | .24301654224E-03 | −.15301654224E-03 |
| 273.2021 | .20000000000E-04 | .25726150310E-03 | −.23726150310E-03 |
| 273.16 | 0 | .26106913775E-03 | −.26106913775E-03 |
SUM OF RESIDUALS =−.47748471843E-11 The least squares solution presented does not give a calculated value of zero for T68/K68 − T/K at T68 = 273.16 K68, but amounts to 0.000261. The equation has a minimum at 278.57 K68, where the value is less than 0.000023. However, the variation at 273.16 K68 is within experimental error, and can be ignored for any scale adjustments.
Footnotes
Figures in brackets indicate the literature references at the end of this paper.
The use of commercial product names is for convenience and exactness and does not imply endorsement by the National Bureau of Standards.
A new oil diffusion pump has been installed. It is stated by the manufacturer to have a backstreaming rate of “almost zero—less than 5×10−5 mg per cm2 per min.”
The subsequent discussion assumes the gas thermometer was already loaded.
Scale comparisons will be given as pure numbers. All temperature scale quantities are therefore divided by their corresponding unit.
However, another approach using low pressures corrected by carefully determined thermomolecular pressures is probably better. It is discussed in the final section of this paper.
The error of the pressure head is estimated to be insignificant.
14. References
- 1.Guildner LA. Temperature, Its Measurement and Control in Science and Industry. Vol. 3. Reinhold Publishing Corp.; New York: 1962. Part I, Chapter 18. [Google Scholar]
- 2.Guildner LA, Stimson HF. Rev Sci Instr. 1963;34:658. [Google Scholar]
- 3.Guildner LA, Edsinger RE. J Res Nat Bur Stand (US) 1965 Jan-Mar;69C(1):13–18. Eng. and Instr. [Google Scholar]
- 4.Anderson RL, Guildner LA. Rev Sci Instr. 1965;36:615. [Google Scholar]
- 5.Guildner LA, Stimson HF, Edsinger RE, Anderson RL. Metrologia. 1970;6:1. [Google Scholar]
- 6.Anderson RL, Guildner LA, Edsinger RE. Rev Sci Instr. 1970;41:1076. [Google Scholar]
- 7.Edsinger RE, Guildner LA, Anderson RL. Rev Sci Instr. 1971;42:7. [Google Scholar]
- 8.Guildner LA, Anderson RL, Edsinger RE. J Res Nat Bur Stand (US) 1973 Sep-Oct;77A(5):667–670. doi: 10.6028/jres.077A.038. Phys. and Chem. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 9.Guildner LA, Anderson RL, Edsinger RE. Temperature, Its Measurement and Control in Science and Industry. Vol. 4. Instrument Society of America; Pittsburgh: 1972. Part 1, Chapter 29. [Google Scholar]
- 10.Guildner LA, Edsinger RE. J Res Nat Bur Stand (US) 1973 Jul-Aug;77A(4):383–389. doi: 10.6028/jres.077A.026. Phys. and Chem. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 11.Cutkosky RD. J Res Nat Bur Stand (US) 1970 Jan-Jun;74C(Nos. 1 & 2):15–18. Eng. and Instr. [Google Scholar]
- 12.Dushman S, Lafferty JM. Scientific Foundations of Vacuum Technique. Second ed. John Wiley & Sons, Inc; New York: 1962. pp. 535–573. [Google Scholar]
- 13.Berry RJ. Metrologia. 1974;10:145. [Google Scholar]
- 14.Beattie JA, Blaisdell BE, Kaye J, Gerry HT, Johnson CA. Proc Am Acad Arts Sci. 1941;74:371. [Google Scholar]
- 15.Powell RL, Hall WJ, Hyink CH, Jr, Sparks LL, Burns GW, Scroger MG, Plumb HH. Thermocouple reference tables based on the IPTS–68. Nat. Bur. Stand. (U.S.); Mar, 1974. p. 410. (Monogr. 125). [Google Scholar]
- 16.Weber S, Keesom WH, Schmidt G. Comm No 246a of the Kamerlingh Onnes Laboratory. Vol. 22. Univ. of Leiden; 1936–38. [Google Scholar]
- 17.Burnett ES. J Appl Mech. 1936;3:A136. [Google Scholar]
- 18.Waxman, M., private communication.
- 19.Yntema YL, Schneider WG. J Chem Phys. 1950;18:641. [Google Scholar]
- 20.Gammon BE, Douslin DR. Proc. Fifth Symp. Thermophys. Props.; New York: Am. Soc. Mech. Engr.; 1970. p. 107. [Google Scholar]