Zero-gap semiconductor to excitonic insulator transition in Ta₂NiSe₅

Abstract

The excitonic insulator is a long conjectured correlated electron phase of narrow-gap semiconductors and semimetals, driven by weakly screened electron–hole interactions. Having been proposed more than 50 years ago, conclusive experimental evidence for its existence remains elusive. Ta₂NiSe₅ is a narrow-gap semiconductor with a small one-electron bandgap E_G of <50 meV. Below T_C=326 K, a putative excitonic insulator is stabilized. Here we report an optical excitation gap E_op ∼0.16 eV below T_C comparable to the estimated exciton binding energy E_B. Specific heat measurements show the entropy associated with the transition being consistent with a primarily electronic origin. To further explore this physics, we map the T_C–E_G phase diagram tuning E_G via chemical and physical pressure. The dome-like behaviour around E_G∼0 combined with our transport, thermodynamic and optical results are fully consistent with an excitonic insulator phase in Ta₂NiSe₅.

The nature of an insulating phase in Ta₂NiSe₅ is an open question. Here, Lu et al. report transport, thermodynamic and optical evidences being fully consistent with an excitonic insulator phase in this material.

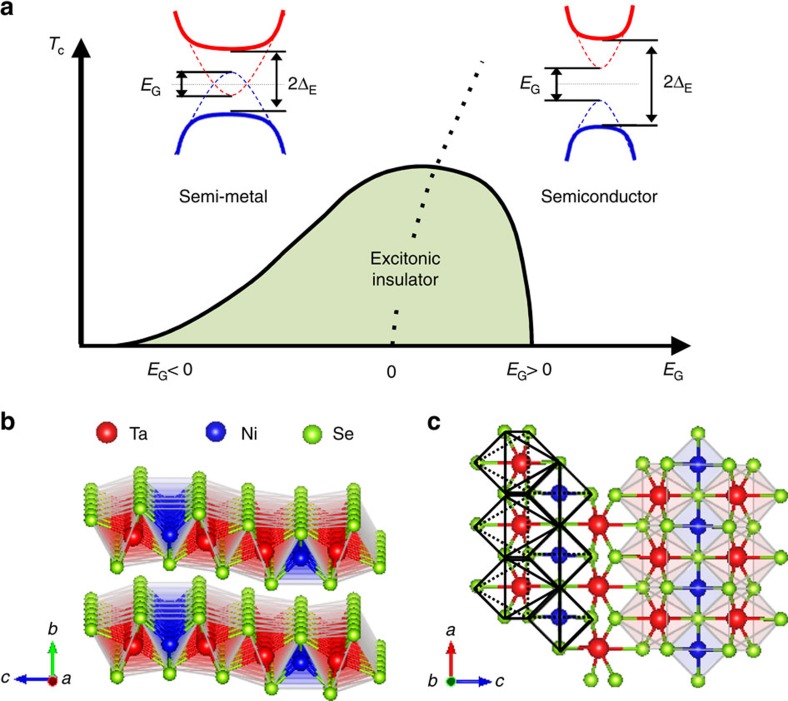
Formation of exotic electronic phases driven by electron correlations is among the most intriguing phenomena in condensed matter physics. One such many-body ground state is the excitonic insulator being stabilized in materials where the conduction and valence band are either separated by a small energy gap (semiconductors) or are overlapping in energy by a small amount (semimetals). In narrow-gap semiconductors and semimetals, the Coulomb interaction between electrons and holes may lead to a spontaneous formation of electron–hole pairs, namely, excitons¹,²,³,⁴. These charge neutral pairs can in analogy to superconductivity give rise to an unconventional insulating ground state—the excitonic insulator—which is characterized by a many-body gap 2Δ_E opening in the single particle excitation spectrum (Fig. 1a). 2Δ_E mirrors the exciton binding energy E_B.

Figure 1. Excitonic insulator and Ta₂NiSe₅ structure.

(a) Schematic phase diagram of excitonic gap formation as a function of E_G. The dotted line indicates the crossover from semimetallic to semiconducting behaviour. The insets show the relation between E_G and Δ_E for the metallic and insulating limits. E_G is the one-electron bandgap between conduction (red) and valence band (blue). The Coulomb interaction between electron- and hole-like excitations can lead to electron–hole pairing (exciton formation) and the opening of a new many-body excitation gap Δ_E in the excitonic insulator phase. (b) The orthorhombic phase of Ta₂NiSe₅ shown in two orientations emphasizing the layered nature in the a–c plane, and (c) the TaSe₆ and NiSe₄ chains along the a direction (right).

The excitonic state is thermodynamically most stable for a zero-gap semiconductor E_G=0. Reducing E_G to a negative value (the semimetallic region) increases the electron/hole density in the one-electron band structure due to band overlap. The increased carrier density screens the effective Coulomb interaction between electrons and holes, and consequently suppresses the stability of excitonic pairs and therefore T_C. This is in strong contrast to a simple hybridization gap, which also exists for a large band overlap. For positive E_G (the semiconducting phase), the spontaneous formation of excitons is supressed with increasing E_G and the excitonic phase becomes unstable against the semiconducting ground state for E_G∼E_B (refs ^{2, 4}). In the semimetallic negative E_G region where interactions are well screened and weak, the transition may be described by BCS mean-field theory due to the analogy with superconductivity²,⁴,⁵,⁶. On going to the positive E_G region, one crosses over to a BEC-like limit as a consequence of strong coupling. This BCS–BEC crossover might be reflected in 2Δ_E/k_BT_C being strongly enhanced over the BCS weak coupling value. In Fig. 1a, we schematically show the generally predicted phase diagram with its characteristic dependence of the excitonic transition temperature T_C on the one electron bandgap E_G (refs ^{2, 4}).

Candidate materials previously discussed include TmSe_0.45Te_0.55 (refs ^{7, 8, 9}) and 1T-TiSe₂ (refs ^{10, 11}). In both cases, transitions to possible excitonic insulator phases were observed. These transitions are however accompanied by strong finite q lattice distortions as expected in the excitonic scenario for such indirect-gap semiconductors. Analysis of the entropy changes involved based on specific heat showed that the transitions in these materials are driven not only by the small number of electrons and holes but are strongly influenced by lattice and spin degrees of freedom¹²,¹³. This is in contrast to the excitonic insulator limit, where lattice deformations play a secondary role¹,²,³,⁴,⁵,⁶,¹⁴. Alternative scenarios such as a charge density wave or a band Jahn–Teller effect, which were put forward especially for 1T-TiSe₂ (refs ^{15, 16}), can therefore not be excluded to describe the relevant physics of these materials, leaving room for question on the existence of a canonical excitonic insulator phase.

Ta₂NiSe₅, first reported by Sunshine et al.¹⁷ and Di Salvo et al.¹⁸, is a layered compound stacked by van der Waals interactions and crystallizing in an orthorhombic Cmcm structure above T_C, as illustrated in Fig. 1b,c (ref. ¹⁸). Each layer is composed of parallel chains of edge-shared TaSe₆ octahedra and corner-shared NiSe₄ tetrahedra, running along the crystallographic a axis.

Band structure calculations in the orthorhombic high-temperature phase of Ta₂NiSe₅ show that the conduction band minimum and the valence band maximum are almost degenerate at the Γ point¹⁴,¹⁹ implying a very small direct gap E_G. The valence band is composed of Ni 3d orbitals with a Se 4p admixture while Ta 5d orbitals dominate the conduction bands. Note that the valence and the conduction bands in the orthorhombic phase belong to different irreducible representations of the crystal structure¹⁴,¹⁹. The formation of a single particle hybridization gap is therefore forbidden by crystal symmetry.

The small direct gap semiconductor realised in Ta₂NiSe₅ above T_C is an ideal situation for the formation of an excitonic insulator phase. A semiconductor-to-insulator transition is indeed observed at T_C≈326 K (ref. ¹⁸). This is accompanied by a very weak q=0 structural phase transition to monoclinic C2/c with the unit cell size remaining the same across the transition and whose small magnitude cannot account for the observed electronic changes¹⁴. This excludes a lattice distortion-mediated charge density wave mechanism driving the phase formation, as discussed in the case of Mo bronze²⁰, suggesting it to be electronic in origin¹⁴. Recent X-ray photoemission spectroscopy (XPS) and angle-resolved photoemission spectroscopy (ARPES) measurements revealed a flattening of the valence band below T_C, which was interpreted as the formation of an additional gap²¹. Based on these observations, the low-temperature phase was proposed to be an excitonic insulator. Ta₂NiSe₅ is distinct from other excitonic insulator candidates. First, due to the direct gap, the excitonic states do not require a finite q super-lattice modulation and can be accessed easily by optical spectroscopy. Second, electrons in Ta chains and holes in Ni chains are spatially separated in real space, which could stabilize the excitonic pair and hence the excitonic insulator.

Recognizing its uniqueness as a candidate excitonic insulator, electric transport, optical and specific heat measurements as well as physical and chemical pressure experiments were conducted on Ta₂NiSe₅ single crystals to test the validity of the excitonic insulator scenario. We will demonstrate that Ta₂NiSe₅ is a nearly zero-gap semiconductor at high temperatures with an optical gap E_op∼0.16 eV, comparable to the expected excitonic binding energy E_B, developing below T_C=326 K. The majority of entropy change at the transition appears to originate from thermally excited electrons and holes consistent with the thermodynamics of phase formation to be dominated by electronic degrees of freedom. The physical and chemical pressure experiments indicate the suppression of T_C both by moving towards negative (semimetal) and positive (semiconductor) bandgap E_G resulting in a dome-shaped T_C versus E_G curve around E_G=0. All these results are consistent with those expected for a canonical excitonic insulator.

Results

Zero-gap semimetal-to-semiconductor transition

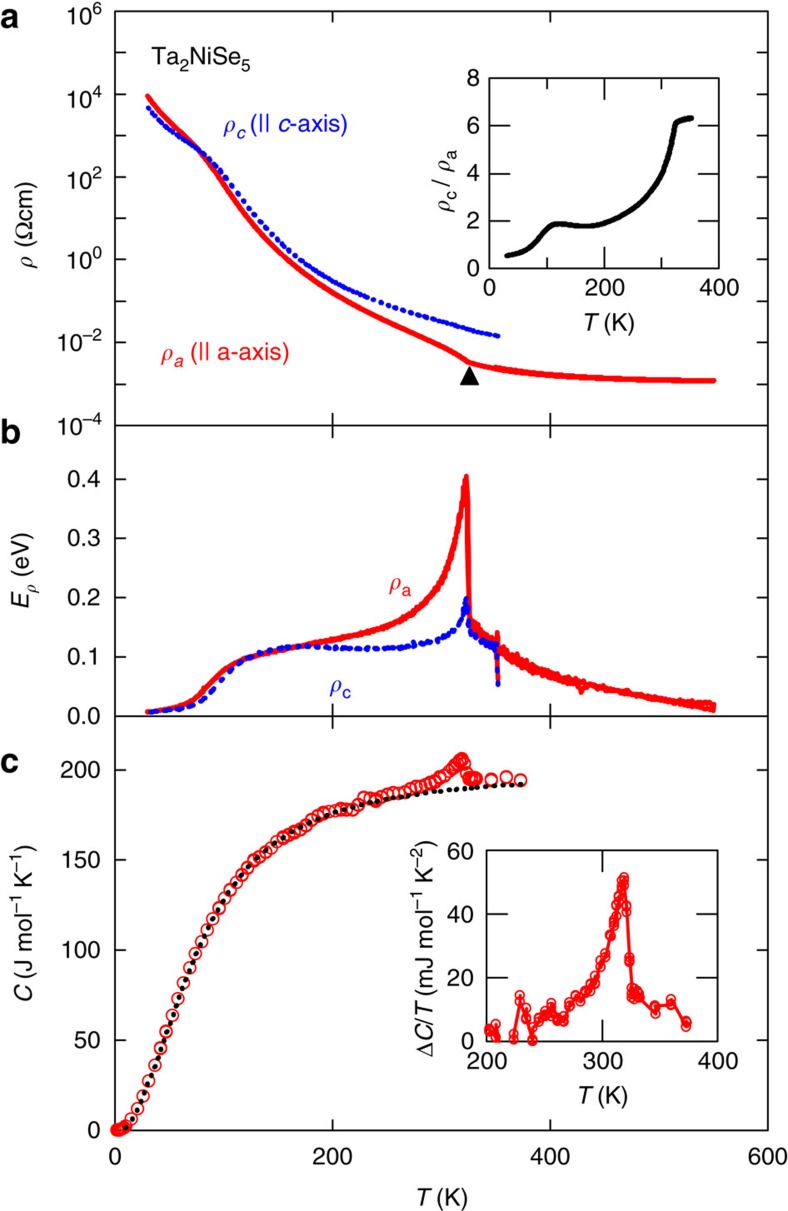
The transition in Ta₂NiSe₅ crystals is most readily seen in transport as shown in Fig. 2a. Here we present the resistivity ρ_a parallel to the Ta and Ni chains (I || a axis) as a function of temperature. A weak but well-defined kink is observed at T_C=326 K. This agrees well with previous reports¹⁸. At high temperatures, the resistivity is essentially temperature independent over a wide range from 400 to 550 K. This means the transport gap is at most of the order of ∼0.05 eV, suggestive of an almost zero energy gap above T_C. Right below T_C, the resistivity increases rapidly, suggesting the opening of an additional gap. It is instructive for an excitonic insulator transition to present the data in form of an activation energy plot showing E_ρ=−k_BT²(‖lnρ/‖T) as a function of temperature (Fig. 2b; Supplementary Fig. 1). E_ρ reflects an activation energy E_A if the temperature dependence at a given temperature is approximated by a thermally activated behaviour ρ=ρ₀exp(−E_A/k_BT) and therefore mirrors the energy scale of thermal excitation of charge carriers. E_ρ shows a clear jump at T_C consistent with theoretical calculations⁴,²², predicting a step-like increase of E_ρ at T_C. E_ρ below the jump is 0.1−0.2 eV, which suggests a gap of the order of a few tenths of an eV opening below T_C. At lower temperature below 100 K, we observe a suppressed activation energy E_ρ. In this temperature regime, the conduction due to impurity states, with much lower energy scale of charge excitations, very likely dominates the temperature dependence.

Figure 2. Ta₂NiSe₅ transport and specific heat.

(a) Temperature dependence of the in-plane resistivity of Ta₂NiSe₅ single crystal ρ_a (red) along the a axis parallel to the Ta and Ni chains, and ρ_c (blue) along the c axis perpendicular to the chains. An anomaly is observed at T_C=326 K (emphasized by black triangle). The inset shows the temperature dependence of the resistivity ratio, ρ_c/ρ_a as an indicator of the in-plane anisotropy. (b) The temperature dependence of the activation energy E_ρ of the resistivity data in a given by E_ρ=−k_BT²(‖lnρ/‖T). (c) Temperature dependence of heat capacity C of a Ta₂NiSe₅ single crystal. An anomaly in C at T_C is clearly visible. The phonon contribution (black dashed line) is roughly estimated from a Debye fit to the low-temperature data below T_C. The inset shows the excess specific heat ΔC/T around T_C obtained from the raw heat capacity by subtracting the lattice contribution.

The resistivity perpendicular to the layer is orders of magnitude higher than the in-plane resistivity and we were not able to obtain a reliable estimate of the out-of-plane resistivity. Reflecting the presence of quasi-one-dimensional Ta and Ni chains, a moderate in-plane anisotropy is observed. As shown in Fig. 2a, above T_C, the resistivity perpendicular to the chains (I || c axis), ρ_c, is larger than ρ_a along the chains by a factor of 6, indicating weak but appreciable electronic quasi-one-dimensionality. Below T_C, this quasi-one-dimensionality in transport appears to be washed out, suggesting that the excitonic gap formation makes charge transport isotropic. This isotropy might be related to the flattening of the one-dimensional dispersion. We note that such temperature dependence in the ρ_c/ρ_a plot is absent in the family compound Ta₂NiS₅, which does not show any signature of an excitonic-like transition (Supplementary Fig. 2).

Gap formation below T _C

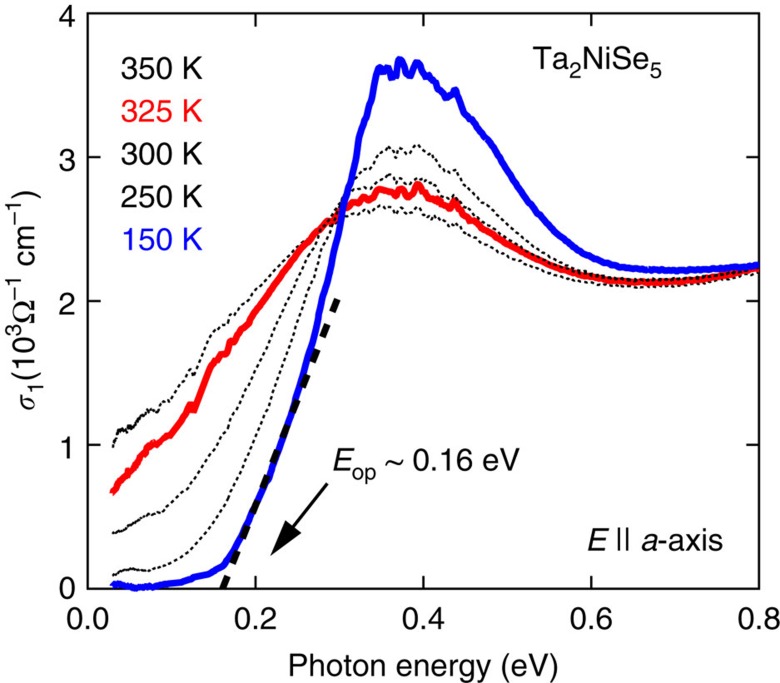
An additional many-body excitation gap 2Δ_E should open at T_C due to the excitonic transition. While ARPES²¹ measurements are consistent with such a gap opening, a complete picture of the gap formation has not been unveiled yet due to the limitation of the measurement to occupied states below the Fermi level and the restricted temperatures at which the experiments were carried out. The formation of an excitation gap at T_C is much more clearly evidenced by the optical conductivity spectrum with photon polarization along the chain direction a shown in Fig. 3. At high temperatures above T_C, a broad continuum is observed with a hump-like structure around 0.3–0.4 eV, which is consistent with Ta₂NiSe₅ being an almost zero-gap semiconductor. On cooling below T_C, the spectral weight is transferred from below an isosbestic point of E*∼0.3 eV to higher energies with a full gap of E_op∼0.16 eV forming. At 150 K, a gap structure with an absorption peak at 0.4 eV is well established. The opening of a gap is further demonstrated by the temperature evolution of the conductivity at an energy well below the gap which we show in the Supplementary Fig. 3. According to the previous ARPES study, the band maximum relative to the Fermi energy—the effective valence band gap—was estimated to be 0.17 eV, which is roughly consistent with the characteristic energy for spectral weight transfer (isosbetic point) E*∼0.3 eV and the optical gap E_op∼0.16 eV in the optical conductivity.

Figure 3. Optical conductivity.

Optical conductivity spectra of Ta₂NiSe₅ single crystal at various temperatures, showing the opening of a charge excitation gap associated with the transition at T_C=326 K. The gap of E_op≈0.16 eV is estimated from the usual extrapolation of the linear regime of conductivity (black dashed line). The electric polarization was parallel to the a axis.

Thermodynamic signature of phase transition

A clear anomaly in the specific heat C(T) can be identified at T_C (Fig. 2c), with the shape being reminiscent of a BCS-type superconducting transition. This constitutes thermodynamic evidence for the opening of a gap in the electronic excitation spectrum at this transition. The entropy associated with the transition is roughly ΔS∼0.3 J mol⁻¹ K⁻¹. The density of states D at the quasi-one-dimensional band edge is of the order of 1 state per eV per unit formula¹⁴, yielding a rough estimate of density of electrons and holes at T_C=326 K of D × k_BT_C∼0.03 per unit formula in the zero-gap case. This is not inconsistent with the Hall coefficient +R_H∼10⁻² cm³ C⁻¹ (hole like) measured at T_C (Supplementary Fig. 4), neglecting the ambiguity arising from the coexistence of electrons and holes. The quenched entropy of 3k_B for 0.03 carriers corresponds to an entropy change ΔS∼0.03 × 3 R=0.7 J mol⁻¹ K⁻¹, agreeing with the experimental value within a factor of 2. This rough estimate implies that the majority of entropy change associated with the transition and therefore the thermodynamically relevant degrees of freedom originate from the electronic entropy indicating an electronically driven phase transition.

Phase diagram as a function of energy gap

The isovalent substitution of Se with Te and S was conducted to control the bandgap E_G. Ta₂NiS₅ has compared with the isostructural Ta₂NiSe₅ a larger transport activation energy of E_ρ∼0.2 eV in resistivity (inset, Fig. 5), indicative of the presence of a much larger bandgap E_G than in Ta₂NiSe₅. As discussed, band calculations indicate that the valence band of Ta₂NiSe₅ is composed of Ni 3d and Se 4p orbitals¹⁴. With increased S-substitution, the hybridization between Ni 3d and Se 4p (S 3p) orbitals are weakened. This lowers the energy of the states at the top of the valence band while the conduction band is less affected, resulting in an overall enhancement of the bandgap E_G compared to Ta₂NiSe₅. Changes in the Ta-chalcogen hybridization further enhance the band gap with increasing S-substitution but are predominantly affecting the energetically higher lying Ta-d bands above 1 eV (ref. ²³). Tellurium doping, on the other hand, pushes the system in the opposite direction, likely bringing the nearly zero gap of Ta₂NiSe₅ to a negative region. Single crystals of the isovalent series Ta₂Ni(Se_1−xS_x)₅ and Ta₂Ni(Se_1−xTe_x)₅ were grown. Te doping was limited up to x=0.2 due to the solubility limit likely due to the much larger ionic radius of Te.

Figure 4. Chemical and physical pressure.

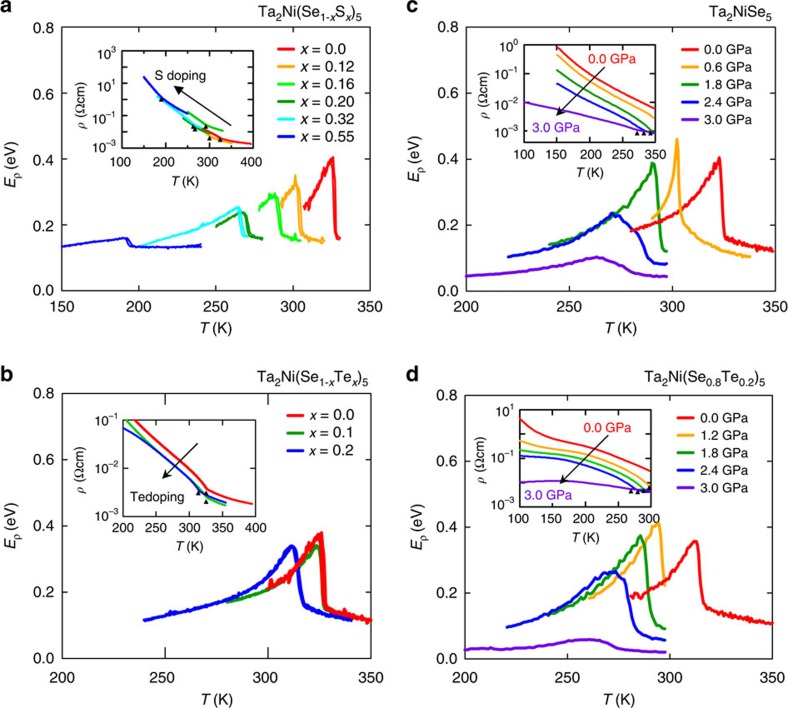
(a) The activation energy plot E_ρ=−k_BT²(‖lnρ/‖T) for Ta₂Ni(Se_1−xS_x)₅ that clearly shows the systematic shift of T_C with S doping. The inset shows resistivity curves (I || a axis) as a function of temperature. Triangles mark the transition temperatures for each sample. Variations in absolute values are due to uncertainties in, for example, exact sample dimensions. In b, we show the equivalent data for the Ta₂Ni(Se_1−xTe_x)₅ series. (c) The activation energy plot as a function of temperature for a single high-pressure experiment showing both the systematic reduction of E_ρ with pressure as well as the suppression of T_C. Additional data from a high-temperature run are also included. The inset shows the equivalent resistivity data with emphasis of T_C by triangles. (d) Equivalent data to c for Ta₂Ni(Se_0.8Te_0.2)₅.

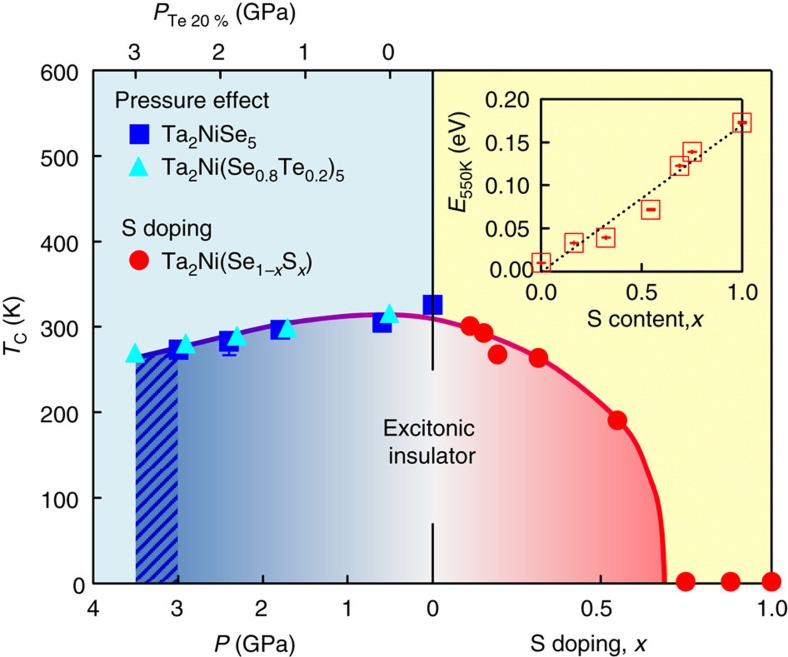
Figure 5. Phase diagram.

Phase diagram of the excitonic insulator phase in Ta₂NiSe₅ given by the transition temperature T_C as a function of S doping (right) and pressure and Te doping (left), corresponding to the semiconducting and the semimetallic regions in the predicted phase diagram respectively. The pressure effect on Ta₂Ni(Se_0.8Te_0.2)₅ is also shown in the left region with an offset of chemical pressure of 0.5 GPa (see text). The shaded area in the left region indicates the region for phase separation behaviour at high pressures (see text), likely originating from a first-order transition. The inset shows the S doping x dependence of the transport activation energy of resistivity at high temperatures (500–550 K), E_550 K. E_550 K gives a measure of the one electron gap E_G and indicates that E_G increases monotonically as a function of x from almost zero for x=0 to at least a few tenths of an eV for x=1.0. The sulfur substitution level x and pressure P may therefore be mapped onto the single band gap E_G in the high-temperature phase.

We show the activation energy plot of resistivity for Ta₂Ni(Se_1−xS_x)₅ and Ta₂Ni(Se_1−xTe_x)₅ in Fig. 4a,b, respectively. It is clear that both by increasing and by decreasing the band gap T_C is suppressed, meaning T_C is peaked for Ta₂NiSe₅ with a nearly zero bandgap. The inset of Fig. 5 shows the evolution of the activation energy E_550K for Ta₂Ni(Se_1−xS_x)₅ estimated from high-temperature data in the temperature range of 500–550 K well above T_C (Supplementary Fig. 1), which should be closely related to the bandgap E_G. It is monotonically increasing and can be described by a linear correlation to leading order as shown, implying the continuous and almost linear increase of the bandgap E_G upon S-substitution. As E_550K is systematically enhanced with increasing S-substitution, T_C is suppressed to lower temperatures and eventually, for x>0.55, no phase transition was observed in the temperature dependent resistivity down to 2 K. For Ta₂Ni(Se_1−xTe_x)₅, the decrease of resistivity at 350 K by ∼70% for x=0.1 and 0.2, respectively, indicates the continuous suppression of the energy gap (Fig. 4b). Although the range of substitution is limited, the plot of E_ρ indicates a small but well resolved decrease of T_C.

To confirm the suppression of T_C by decreasing E_G towards the negative side, namely by Te-substitution, physical pressure was applied to Ta₂NiSe₅. As can be seen from the activation energy plot in Fig. 4c, a clear decrease of T_C is observed with increasing pressure. At 2.5 GPa, T_C is decreased from 326 to 280 K, corresponding to a T_C suppression rate of ∼20 K GPa⁻¹, which can be reasonably ascribed to the decrease of the energy gap in the negative gap region. With 20% of Te-substitution, T_C is reduced by 10 K, which is equivalent to 0.5 GPa of pressure. We performed the same pressure experiment also on 20% substituted Ta₂Ni(Se_0.8Te_0.2)₅ and observed again the suppression of T_C with a rate of ∼20 K per GPa (Fig. 4d). Therefore, once we offset an effective chemical pressure equivalent to 0.5 GPa, the two T_C (P) curves for pure and 20% Te substitute crystals fall onto a universal curve, strongly suggesting that the phase transition is controlled by a single parameter E_G. In the pressure range above 2.4 GPa, we observe that upon increasing pressure further the transition temperature does not shift significantly, while the magnitude of the anomaly is substantially supressed (Fig. 4c,d) and conductivity in the low-temperature limit is significantly enhanced, suggestive of a first-order phase transition and the resultant phase coexistence. A recent pressure study up to 10 GPa on Ta₂NiSe₅ indeed indicates that there is a first-order structural phase transition at P_C∼2.5 GPa, and the system becomes metallic above P_C²⁴. A reduction of resistivity under pressure is also measured for Ta₂NiS₅ but no phase transition was observed up to 3 GPa.

The control of T_C by changing E_G via chemical substitution and physical pressure effects can be summarized in the phase diagram shown in Fig. 5, where the pressure is a measure of decreasing E_G on the negative E_G side and the S-substitution is the measure of increasing E_G on the positive side. Te-substitution was converted to equivalent pressure on the pressure axis. We clearly see the expected dome-shaped dependence of T_C around E_G∼0 (Ta₂NiSe₅).

Discussion

The high-temperature phase of Ta₂NiSe₅ appears to be very close to a zero-gap semiconductor making it an ideal candidate system for the stabilization of an excitonic insulator phase. Our optical data indeed show that below the phase transition temperature of T_C=326 K, a gap E_op∼0.16 eV develops in the excitation spectrum consistent with the enhanced activation energy observed in our transport data. Significant spectral weight shifts occur from below the isosbestic point of ∼0.3 eV to higher energy scales of up to ∼0.6 eV. These energy scales should reflect the characteristic energy scales of the excitonic insulator and in particular experimentally constrains the scale of the exciton binding energy E_B to be of the order of a few tenths of an eV. Expressed in terms of an effective temperature (0.1 eV corresponding to ≈1,000 K) E_B is significantly larger than the experimental transition temperature to the excitonic insulator phase at 326 K, enabling the formation of this phase in Ta₂NiSe₅.

The exciton binding energy E_B of the order of a few tenths of an eV is orders of magnitude larger as compared with those of conventional three-dimensional semiconductors. However, we argue that the unusual properties of Ta₂NiSe₅ explain at least in part the enhanced E_B. The excitonic binding energies can be captured by E_B=−13.6 eVμ/m₀ɛ². In this Rydberg formula, μ is a material specific effective mass and ɛ an effective permittivity²⁵. First of all the d-band character of conduction (Ta 5d) and valence bands (Ni 3d) result in an effective reduced mass μ of the order of m₀ as can be determined from ARPES data²¹ and band structure calculations¹⁴. This reduced mass is up to two orders of magnitude larger than in conventional direct gap semiconductors²⁶. Furthermore the effective low dimensionality of the band structure and resulting physical properties are known to generally lead to a strong increase of the exciton binding energy²⁶. Taken together, these effects can be expected to lead to a large enhancement of the exciton binding energy to a value consistent with tenths of an eV.

We now turn to the evolution of these properties under pressure and chemical substitution. The emerging phase diagram based on our transport measurements of T_C as a function of the bandgap E_G in the high-temperature phase shown in Fig. 5 is consistent with the theoretical expectation for the T_C–E_G dependence⁴, further supporting the excitonic state. T_C is optimized for the nearly zero-gap semiconductor (E_G∼0) Ta₂NiSe₅. On increasing E_G in the solid solution Ta₂Ni(Se_1−xS_x)₅, the excitonic state should be suppressed and switch into a normal semiconductor when E_G∼E_B. For Ta₂NiS₅ located outside the dome in the phase diagram, the high-temperature (500–550 K) transport activation energy of ∼0.2 eV, shown in the inset in Fig. 5, implies an energy gap E_G of ∼0.4 eV. Recent optical measurements show a similar trend with a gap of ∼0.25 eV at 350 K measured in Ta₂NiS₅ (ref. ²³) compared with the effective zero gap in Ta₂NiSe₅ (Fig. 3). These results imply that, in contrast to Ta₂NiSe₅, E_G in Ta₂NiS₅ is larger than E_B, which has a weaker dependence upon sulfur substitution²³. These findings are fully consistent with the absence of an excitonic transition in Ta₂NiS₅. In between these limits around x=0.5, the energy scale of the bandgap E_G in the high-temperature phase can therefore be expected to become comparable with the exciton binding energy E_B when crossing over from several hundreds of meV in Ta₂NiS₅ to effectively zero in Ta₂NiSe₅. This accounts for the stabilization of the excitonic insulator phase around that doping level.

We now turn our attention to the left hand part of the phase diagram. With going to the negative E_G side with pressure, T_C goes down with decreasing E_G. This is consistent with the suppression of the excitonic binding due to the increased screening. The system is approaching the weak coupling BCS regime within the excitonic insulator scenario. Consequently the near-zero-gap Ta₂NiSe₅ with the highest T_C is in the BCS–BEC crossover regime and a relatively strong coupling is anticipated. Indeed, the experimentally obtained E_op/k_BT_C∼6.5, a measure of coupling strength, for Ta₂NiSe₅ is much larger than 3.52 for weak coupling BCS, pointing to a moderately strong coupling.

Finally, we are not aware of any other electronically driven mechanism that could give rise to the observed behaviour. Since there is no evidence for magnetic-order scenarios such as Slater- or Mott-insulating phases can be readily excluded. Neither is there any Fermi surface nesting possible that could give rise to a partial gapping of the band structure. Indeed, the excitonic insulator scenario is the only theoretical proposal we are aware of that is consistent with all the observed experimental facts. In particular, the theoretically conjectured dome-shaped phase diagram we observe in this system is the cleanest among all excitonic insulator candidates and therefore represents a key advance in the search for this phase.

In summary, due to the relatively large effective mass of electrons and holes, and the reduced effective dimensionality, the layered direct gap semimetal Ta₂NiSe₅ can have a large exciton binding energy of a few tenths of an eV. The separation of electrons and holes confined within the quasi-one-dimensional chains may further help stabilizing the formation of excitons. The optical, transport and specific heat measurements on Ta₂NiSe₅ single crystals indicate that the system has an almost zero energy gap at high temperature but, below an electronically driven transition at T_C=326 K, an excitation gap of E_op∼0.16 eV develops. The excitation gap is comparable to the expected exciton binding energy and is reasonably ascribed to the excitonic gap. The electronic phase diagram, T_C as a function of the energy gap shows a dome-shaped behaviour centred at E_G=0 expected for an excitonic insulator. Furthermore, the excitonic phase indeed disappears on increasing the energy gap E_G when E_G becomes comparable to the exciton binding energy, as expected. Based on this analysis, we conclude that an excitonic state is realized below T_C=326 K in the nearly zero-gap semiconductor Ta₂NiSe₅.

Methods

Sample preparation

Single crystals of Ta₂NiCh₅ (Ch=S, Se and Te) were synthesized by chemical vapour transport. Elemental powders of tantalum, nickel and chalcogens (S, Se and Te) were mixed with a stoichiometric ratio and sealed into an evacuated quartz tube (∼1 × 10⁻³ Pa) with a small amount of I₂ as transport agent. The mixture was sintered under a temperature gradient of 950/850 °C. After sintering for 1 week, needle-like single crystals were grown at the cold end of the tube.

Physical properties measurements

Electric resistivity and heat capacity measurements were carried out by using a PPMS (Quantum Design). Pressure experiments were carried out using a HPC-33 pressure cell by ElectroLab. High-temperature electronic resistivity was measured by using a bespoke measurement device. Optical parameter were determined using spectroscopic ellipsometry²³.

Data availability

All relevant data are available from the corresponding authors upon request.

Additional information

How to cite this article: Lu, Y. F. et al. Zero-gap semiconductor to excitonic insulator transition in Ta₂NiSe₅. Nat. Commun. 8, 14408 doi: 10.1038/ncomms14408 (2017).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Material

Supplementary Information

Supplementary Figures 1-5