Skip to main content
PMC home page
Proceedings of the National Academy of Sciences of the United States of America logoLink to Proceedings of the National Academy of Sciences of the United States of America
. 1990 Jan;87(2):723–727. doi: 10.1073/pnas.87.2.723

Application of entropy measures derived from the ergodic theory of dynamical systems to rat locomotor behavior.

M P Paulus 1, M A Geyer 1, L H Gold 1, A J Mandell 1
PMCID: PMC53338  PMID: 1967830

Abstract

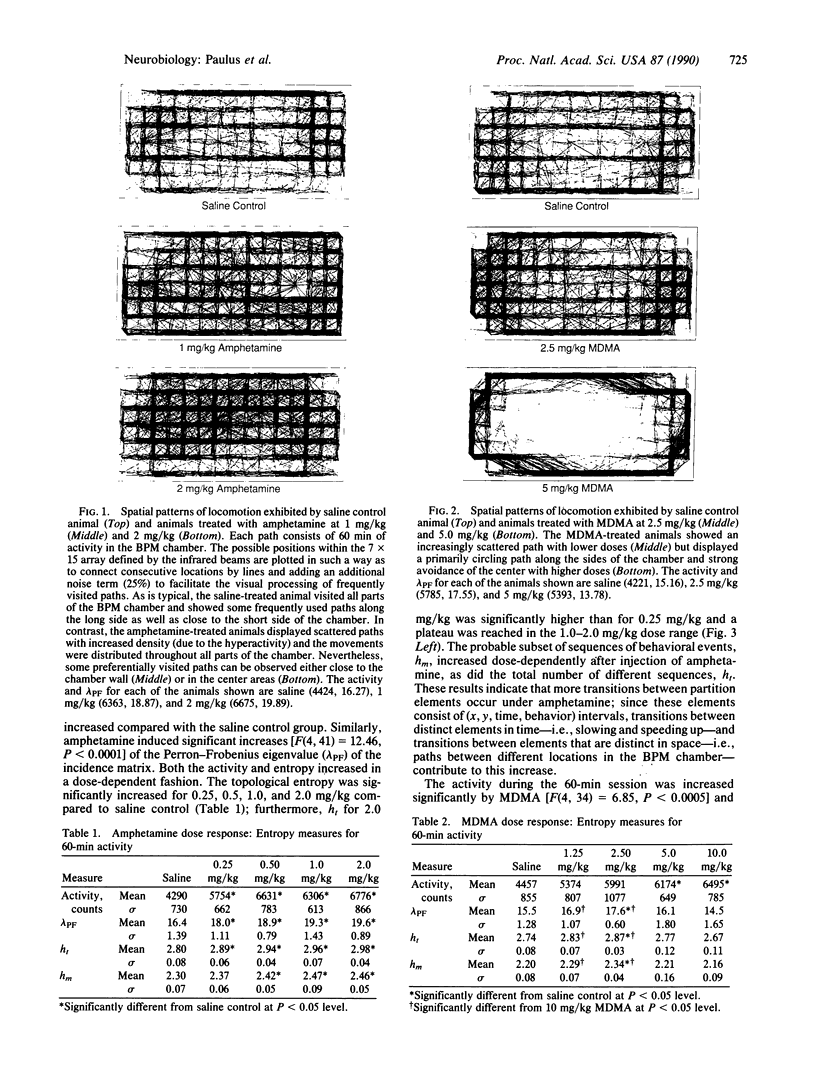
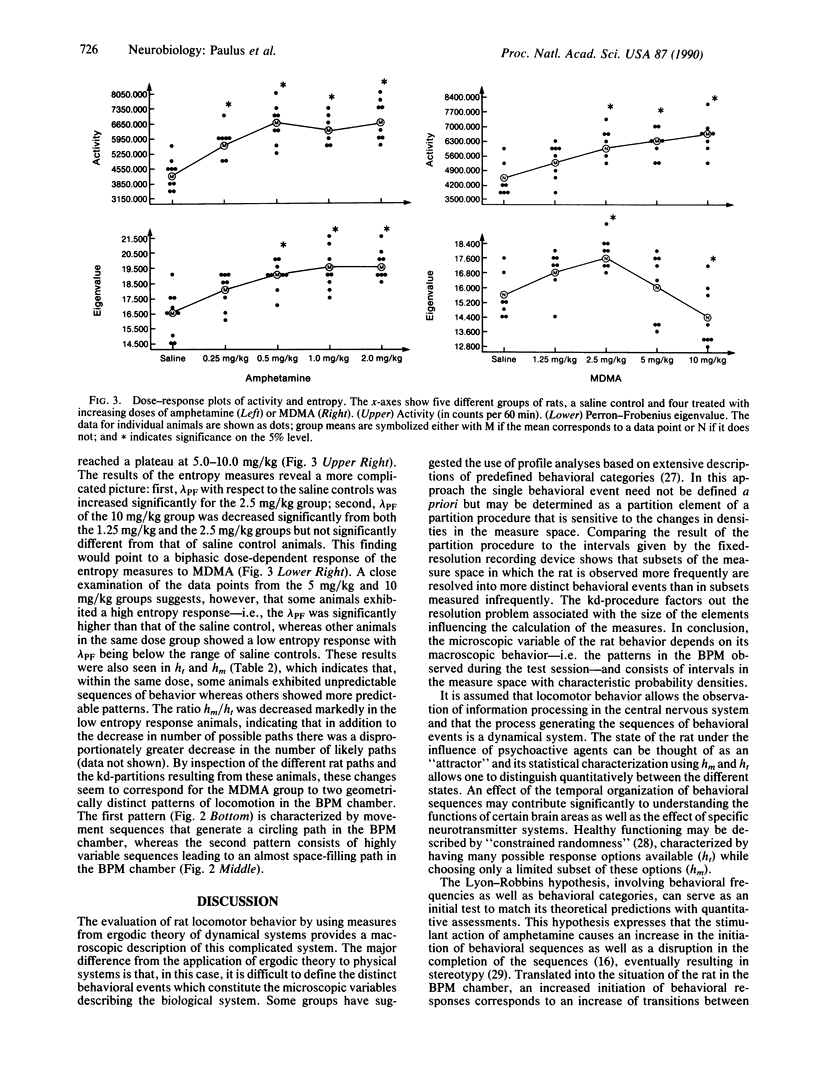
Measures of complexity derived from ergodic theory of dynamical systems were developed and applied to an exemplary data set describing locomotor movements of rats in a bounded space. A symbolic dynamical system was obtained by partitioning the event space into equally probable partition elements, using a k-dimensional tree. The measures calculated from the symbolic sequences included the topological entropy (ht)--i.e., the rate of increase of all possible sequences with increasing sequence length--and the metric entropy (hm)--i.e., the rate of increase of all likely sequences with increasing sequence length. These measures were used to assess changes in rat locomotor behavior as recorded in the behavioral pattern monitor (BPM) that were induced by amphetamine (0.25, 0.50, 1.0, or 2.0 mg/kg) and 3,4-methylenedioxymethamphetamine (MDMA; 1.25, 2.5, 5.0, or 10.0 mg/kg). Amphetamine increased the mean activity, ht, and hm. MDMA resulted in a monotonic dose-response curve for activity but exhibited a biphasic dose response in ht and hm. In particular, some animals in the higher dose groups showed a ht in the range of the saline controls, whereas other animals exhibited a significantly reduced ht and a greater decrease in hm, suggesting that two different behavioral reactions coexist within the same higher dose range of MDMA. An important implication of our method is that, in applied ergodic measure-theoretic approaches, the partition that determines the elements of the symbolic dynamical system should not be specified a priori on abstract mathematical grounds but should be chosen relative to its significance with respect to the data set in question. Here, the animal constructs its own spatiotemporal partition in behavioral phase space.

Full text

PDF
723

Images in this article

725Image
on p.725
725Image
on p.725
725Image
on p.725
725Image
on p.725
725Image
on p.725
725Image
on p.725

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Abrams R. Clinical prediction of ECT response in depressed patients. Psychopharmacol Bull. 1982 Apr;18(2):48–50. [PubMed] [Google Scholar]
  2. Cohen A, Procaccia I., I Computing the Kolmogorov entropy from time signals of dissipative and conservative dynamical systems. Phys Rev A Gen Phys. 1985 Mar;31(3):1872–1882. doi: 10.1103/physreva.31.1872. [DOI] [PubMed] [Google Scholar]
  3. DEWS P. B. Studies on behavior. IV. Stimulant actions of methamphetamine. J Pharmacol Exp Ther. 1958 Jan;122(1):137–147. [PubMed] [Google Scholar]
  4. Geyer M. A., Russo P. V., Masten V. L. Multivariate assessment of locomotor behavior: pharmacological and behavioral analyses. Pharmacol Biochem Behav. 1986 Jul;25(1):277–288. doi: 10.1016/0091-3057(86)90266-2. [DOI] [PubMed] [Google Scholar]
  5. Geyer M. A., Russo P. V., Segal D. S., Kuczenski R. Effects of apomorphine and amphetamine on patterns of locomotor and investigatory behavior in rats. Pharmacol Biochem Behav. 1987 Nov;28(3):393–399. doi: 10.1016/0091-3057(87)90460-6. [DOI] [PubMed] [Google Scholar]
  6. Gold L. H., Koob G. F., Geyer M. A. Stimulant and hallucinogenic behavioral profiles of 3,4-methylenedioxymethamphetamine and N-ethyl-3,4-methylenedioxyamphetamine in rats. J Pharmacol Exp Ther. 1988 Nov;247(2):547–555. [PubMed] [Google Scholar]
  7. Halsey TC, Jensen MH, Kadanoff LP, Procaccia I, I, Shraiman BI. Fractal measures and their singularities: The characterization of strange sets. Phys Rev A Gen Phys. 1986 Feb;33(2):1141–1151. doi: 10.1103/physreva.33.1141. [DOI] [PubMed] [Google Scholar]
  8. Jensen MH, Kadanoff LP, Procaccia I., I Scaling structure and thermodynamics of strange sets. Phys Rev A Gen Phys. 1987 Aug 1;36(3):1409–1420. doi: 10.1103/physreva.36.1409. [DOI] [PubMed] [Google Scholar]
  9. Ljungberg T., Enquist M. Disruptive effects of low doses of d-amphetamine on the ability of rats to organize behaviour into functional sequences. Psychopharmacology (Berl) 1987;93(2):146–151. doi: 10.1007/BF00179924. [DOI] [PubMed] [Google Scholar]
  10. May R. M. Simple mathematical models with very complicated dynamics. Nature. 1976 Jun 10;261(5560):459–467. doi: 10.1038/261459a0. [DOI] [PubMed] [Google Scholar]
  11. Nickolson V. J. Detailed analysis of the effects of apomorphine and d-amphetamine on spontaneous locomotor behaviour of rats as measured in a TV-based, automated open-field system. Eur J Pharmacol. 1981 Jun 10;72(1):45–56. doi: 10.1016/0014-2999(81)90295-8. [DOI] [PubMed] [Google Scholar]
  12. Schmidt C. J., Levin J. A., Lovenberg W. In vitro and in vivo neurochemical effects of methylenedioxymethamphetamine on striatal monoaminergic systems in the rat brain. Biochem Pharmacol. 1987 Mar 1;36(5):747–755. doi: 10.1016/0006-2952(87)90729-5. [DOI] [PubMed] [Google Scholar]
  13. Segal D. S., Mandell A. J. Long-term administration of d-amphetamine: progressive augmentation of motor activity and stereotypy. Pharmacol Biochem Behav. 1974 Mar-Apr;2(2):249–255. doi: 10.1016/0091-3057(74)90060-4. [DOI] [PubMed] [Google Scholar]

Articles from Proceedings of the National Academy of Sciences of the United States of America are provided here courtesy of National Academy of Sciences

RESOURCES