Abstract
The greatest merit of in vivo magnetic resonance spectroscopy (MRS) methodology used in biomedical research is its ability for noninvasively measuring a variety of metabolites inside a living organ. It, therefore, provides an invaluable tool for determining metabolites, chemical reaction rates and bioenergetics, as well as their dynamic changes in the human and animal. The capability of in vivo MRS is further enhanced at higher magnetic fields because of significant gain in detection sensitivity and improvement in the spectral resolution. Recent progress of in vivo MRS technology has further demonstrated its great potential in many biomedical research areas, particularly in brain research. Here, we provide a review of new developments for in vivo heteronuclear 31P and 17O MRS approaches and their applications in determining the cerebral metabolic rates of oxygen and ATP inside the mitochondria, in both animal and human brains.
Keywords: In vivo 31P MRS, in vivo 17O MRS, in vivo heteronuclear MRS, brain, magnetic field, cerebral bioenergetics, brain metabolism, brain function, MRI
1. Introduction
1.1. Cerebral Bioenergetics and Brain Function
The brain is an extraordinary organ; with its unique structure and complex functions, it distinguishes itself from other organs in many aspects. Unlike cardiac and skeletal muscles, the brain does not perform mechanical work. The major cellular functions in the brain include excitation and conduction resulting in unceasing electrophysiological activities in normal brain, even at rest. These integrated neuronal activities are essential for performing brain functions. Nevertheless, to sustain these activities and functionalities, the brain has a high energy demand which is mainly fulfilled by the energy metabolism at the cellular level through adenosine triphosphate (ATP) production and utilization (1–8).
The vast majority of ATP formation relies on the oxidative phosphorylation occurring inside the mitochondria. The activity of cytochrome oxidase, a key mitochondrial enzyme of oxidative energy metabolism, is regulated by the cell functional activities and their energy demand (9, 10). At normal condition, the cerebral oxidative phosphorylation is expected to be tightly coupled and supported by the oxygen metabolism.
Figure 15.1 illustrates a simplified schema showing the major metabolic processes and ATP energy transportation occurring at various subcellular compartments and the associated hemodynamic processes for supporting normal brain function. Oxygen and glucose are the major chemical substrates for brain metabolism and they are continuously supplied by the circulating blood in the capillary bed. Glucose is first converted to two pyruvate molecules in the cytosol, then the pyruvate enters the mitochondria and is metabolized oxidatively with diffused oxygen molecules through the mitochondrial respiratory chain and the cytochrome oxidase enzyme resulting in substantial oxygen utilization and final products of water and CO2 (i.e., Reaction 1 or R1 in Fig. 15.1). This oxygen metabolism is coupled with the oxidative phosphorylation for producing ATP from inorganic phosphate (Pi) and adenosine diphosphate (ADP) through the enzyme of F1F0-ATPase inside the mitochondria (i.e., Reaction 2 or R2 in Fig. 15.1). In general, the mitochondrial oxidative phosphorylation dominates up to 90% of ATP production (11). In contrast, ATP utilization mainly occurs in the cytosol space resulting in ADP and Pi products (i.e., Reaction 5 or R5 in Fig. 15.1), ultimately, providing chemical energy for supporting various cellular activities and brain functions, in which a significant amount of ATP energy is used for neuronal signaling. This ATP utilization is particularly essential for maintaining and restoring the Na+/K+ ion gradients across the cellular membranes through the enzyme of Na+/K+-ATPase as well as for supporting the signaling process (e.g., neuronal transmission and cycling) at resting and activated brain states (11–14).
Fig. 15.1.
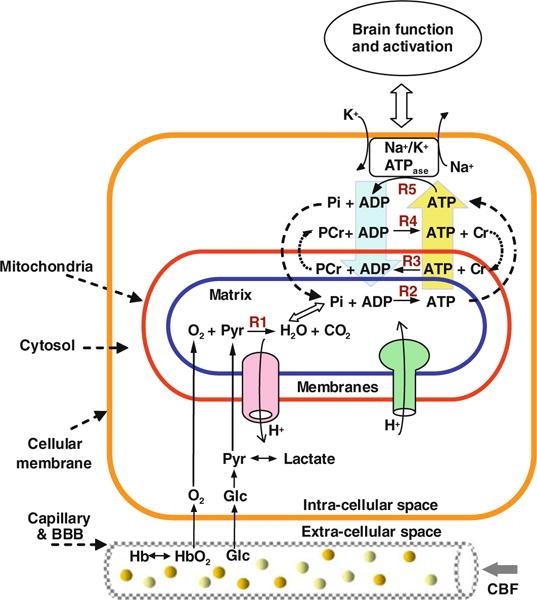
Schematic diagram of simplified major brain network involving metabolisms and hemodynamics occurring in the capillary, subcellular compartments including the mitochondria and cytosol spaces. Oxygen dissociates from hemoglobin (Hb) and enters mitochondria and it is metabolized with pyruvate (Pyr) which is converted from glucose (Glc). This oxygen utilization via Reaction 1 (R1) is tightly coupled with four ATP reactions for generating ATP (R2), consuming ATP (R5), transporting and buffering ATP energy via the paired CK reactions (R3 vs. V4). These four ATP reactions constitute a chemical exchange network PCr↔ATP↔Pi. This neurovascular-metabolic coupled network is essential for brain function.
The high energy demand in the brain causes extremely fast chemical cycling among ATP, ADP and Pi, which requires rapid transportation of these phosphate components between the cytosolic and mitochondrial compartments. This energy transportation could be partially accomplished by phosphocreatine (PCr) through the reversible creatine kinase (CK) reaction, thus, maintaining a stable ATP level in the brain cells (15–17). There are at least two apparently coupled CK reactions: one occurring in the mitochondrial intermembrane space (i.e., Reaction 3 or R3 in Fig. 15.1) and another one occurring in the cytosol space (i.e., Reaction 4 or R4 in Fig. 15.1) although, in reality, these coupled CK reactions likely exist of these subcellular spaces. They play an important role in carrying the ATP molecules generated in the mitochondria into the cytosol for energy utilization, and then to bring the products of ADP and Pi back to mitochondria for sustaining ATP production. Therefore, PCr serves a vital role for energy transportation among subcellular compartments. Four ATP-related reactions (two reactions each for ATPase and CK) constitute a complex ATP metabolic process. They are tightly coupled and integrated with the oxygen metabolism and the hemodynamic process as depicted in Figure 15.1 for controlling the dynamics of ATP production and utilization, all of which play a central role in the cerebral bioenergetics and function in normal and diseased brains. Development of in vivo tools being able to noninvasively detect and quantify the cerebral metabolic rate of oxygen utilization (CMRO2) and ATP formation (CMRATP), therefore, become extremely important for studying and understanding the relation between ATP metabolism and cerebral bioenergetics and its impact on brain function.
1.2. In Vivo Heteronuclear MRS Approaches for Studying Brain Bioenergetics and Function
Although there are a number of techniques capable of studying brain metabolism, in vivo heteronuclear magnetic resonance spectroscopy (MRS) allows for noninvasively determining the physiological parameters and their changes that can be linked to brain metabolism, chemical kinetics and cerebral bioenergetics. The most commonly used in vivo heteronuclear MRS approaches for studying brain bioenergetics are in vivo 31P, 13C and 17O MRS. Here, we will focus on 31P and 17O MRS in vivo.
In vivo 31P MRS allows noninvasive assessment of numerous fundamental biochemical, physiological and metabolic events occurring inside a living brain (e.g., (17–23)). The primary information provided by in vivo 31P MRS includes: intracellular pH, intracellular free magnesium concentration ([Mg2+]) and high-energy phosphate (HEP) metabolites such as ATP, ADP and PCr. All these phosphate metabolites (except ADP) and Pi can be directly observed in an in vivo 31P MR spectrum as demonstrated in Fig. 15.2A which was acquired from the human occipital-lobe at high field (7 tesla) (24). The pH value can be calculated from the chemical shift difference (δi) between the Pi and PCr resonance peaks according to the following equation: (25)
| (15.1) |
and [ADP] can be calculated by the following equation:
| (15.2) |
where Keq is the equilibrium constant of the creatine kinase reaction and [Cr] is the total creatine concentration given by the summation of PCr and non-phosphorylated Cr.
Fig. 15.2.
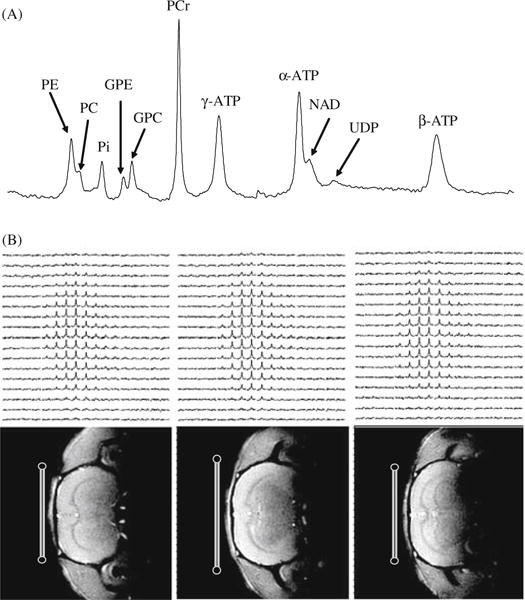
(A) A typical 31P MR spectra acquired from the human occipital lobe at 7T with a total sampling time of 6.4 minutes. The spectrum is characterized by excellent spectral resolution and NMR sensitivity, and a large number of well-resolved resonance peaks from phosphoethanolamine (PE); phosphocholine (PC); inorganic phosphate (Pi); glycerophosphoethanolamine (GPE); glycerophosphocholine (GPC); phosphocreatine (PCr); adenosine triphosphate (ATP); nicotinamide adenine dinucleotides (NAD) and uridine diphospho sugar (UDP). Adapted from Lei et al. of Reference 24. (B) 3D 17O MRSI of natural abundance H2 17O (top row) and corresponding 1H anatomical images (bottom row) of rat brain acquired at 9.4T. The 17O surface RF coil positions and cross sections are indicated in the images.
Besides the aforementioned phosphate compounds and physiological parameters, other detectable phosphate compounds in the human brain 31P MRS include: uridine diphospho (UDP) sugar (an important precursor for glycogen metabolism), diphospho nicotinamide adenine dinucleotides (NAD) involving oxidative respiratory chains, and four resolved phosphate compounds of glycerophosphoethanolamine (GPE), glycerophosphocholine (GPC), phosphoethanolamine (PE) and phosphocholine (PC), which are actively involved in the membrane phospholipid metabolism through phospholipid biosynthetic enzymes (26–29). In vivo 31P MRS has been widely applied to study both normal and diseased brains and has led to a large number of publications (e.g., (19, 21–24, 26–28, 30–42). The abnormality in the HEP metabolites has been frequently observed in the diseased brains using in vivo 31P MRS, and these changes or the change of HEP concentration ratios have been commonly applied in many clinical studies.
Although the steady-state concentrations of cellular HEP metabolites are closely linked to the ATP metabolism, they are relatively stable under normal physiological conditions. This could be attributed to the rigorous regulation of the ATP metabolic reactions and their kinetics (see Fig. 15.1) for maintaining stable chemical energy supply of ATP in the brain. This is likely the case for the brain activation caused by brain stimulation and task performance. Thus, the measurements of the kinetics of ATP metabolism (i.e. reaction rate constants and fluxes) should logically be more sensitive and meaningful for quantifying the cerebral bioenergetics and its change under varied brain activity states as compared to the measurement of steady-state ATP concentrations. In vivo 31P MRS, in combination with the magnetization transfer (MT) approach (18, 43–48), is capable of noninvasively determining the chemical reaction fluxes related to the ATP metabolism and enzyme activity; and thus becomes an important tool for studying the brain ATP metabolisms and cerebral bioenergetics. One example is to apply this approach for measuring the metabolic flux of oxidative phosphorylation in the brain (20,49,50). This useful in vivo approach and its new development will be described here.
Another alternative in vivo heteronuclear MRS approach which can directly and noninvasively assess the fluxes of oxidative metabolism occurring in the mitochondria (i.e., R1 in Fig. 15.1) is the use of in vivo 17O MRS approach for imaging CMRO2 (e.g., (51–54) and a recent review article (55) and the references cited therein). The basic idea underlying this approach is to apply in vivo 17O MRS for detecting the dynamic change of the 17O-labeled metabolic water (H2 17O) produced through the metabolism of inhaled 17O2 gas, and ultimately for determining and imaging CMRO2. Recent progress, especially at ultrahigh fields, has advanced the in vivo 17O MRS methodology for imaging CMRO2 and great promise has been demonstrated in the animal applications at high fields. This topic is also discussed here in some detail.
Although the metabolic fluxes measured by in vivo 31P MRS and in vivo 17O MRS reflect different metabolic reactions and pathways, these two MRS approaches are quite complementary. They are vital for investigating the coupling between the oxygen utilization and oxidative phosphorylation in the brain mitochondria under normal and pathological conditions.
2. Benefit of In Vivo 31P and 17O MRS at Ultrahigh Magnetic Field
2.1. Challenges of In Vivo Heteronuclear MRS
In practice, in vivo heteronuclear MRS faces many technical challenges due to its unfavorable sensitivity. For instance, the phosphate metabolites detected by in vivo 31P MRS and the metabolic H2 17O detected by in vivo 17O MRS are in a range of few millimolar concentrations, which are approximately 5000 times lower than the tissue water concentration, resulting in a much lower detection sensitivity. Thus, a high number of signal averaging is required to achieve reasonable spectral quality. This low sensitivity is even more manifest for the nuclear spins (e.g., 31P and 17O) with a relatively low magnetogyric ratio (γ) compared to in vivo 1H MRS. This significantly limits the reliability, applicability, spatial and temporal resolutions of in vivo 31P and 17O MRS for general application, as well as clinical studies. One common trade off is to reduce the spatial and/or temporal resolution of in vivo MRS, thereby gaining detection sensitivity. However, in order to determine the nonuniform distribution of cerebral metabolites and chemical reaction fluxes in different brain regions, there is a high demand on improving the spatial resolution of in vivo MRS. Moreover, the dynamic changes caused by physiological perturbation (e.g., sensory stimulation) may occur in a relatively short time and the magnitudes of the changes are usually subtle compared to those observed under pathological states. Therefore, both reasonably high spatial and temporal resolutions are desired and they rely heavily on the achievable detection sensitivity, which poses the major challenge for in vivo heteronuclear MRS. One way to overcome this challenge is the use of high/ultrahigh field MRS. Besides the sensitivity gain at high fields, the spectral resolution of in vivo MRS is also significantly improved and many overlapped resonance peaks from different metabolites as observed at low fields become resolvable at ultrahigh fields as demonstrated in Fig. 15.2A.
2.2. Sensitivity Improvement of In Vivo Heteronuclear MRS at High Field
One of the most important advantages at ultrahigh fields is the potential gain in detection sensitivity. This is particularly crucial for the low γ nuclei MRS such as in vivo 17O and 31P MRS. However, it is not so straightforward to evaluate and compare detection sensitivity (or signal-to-noise ratio, SNR) at different magnetic field strengths (B0). The apparent SNR achievable at a given field strength relies not only on the B0 but also on other B0-dependent parameters, such as the longitudinal relaxation time (T1), the apparent transverse relaxation time (T2*) and the repetition time (TR) for acquiring MRS signal. It is, in general, more useful to determine and evaluate the SNR of signal detected in a given unit sampling time under optimal acquisition condition, which means that the excitation flip angle (α) satisfies the Ernst equation of cos(αopt) = exp(−TR/T1) (56). For a simple case with the single pulse and data acquisition scheme, such an averaged SNR can be quantified by Eq. (15.3) (56–60),
| (15.3) |
where
| (15.4) |
C is the concentration of the nuclear spin under observation; at is the spectrum acquisition time; Q is the radio frequency (RF) coil quality factor. C and γ are B0-independent and can be treated as constants.
Equation (15.3) accounts for most B0-dependent parameters that have influences in the apparent SNR, such as T2* signal loss and the partial saturation effect on signal due to a relatively short TR of ≪ 5T1. For in vivo 31P MRS applications, the term of approximates to 1.0 (60), thus Eq. (15.5) is a simplification
| (15.5) |
or
| (15.6a) |
if one applies the approximation of resulting β* = β+1/4 (57,60). The values for β* and β based on the theoretical predictions are 1.75 and 1.5 respectively (57).
For precisely quantifying and understanding the sensitivity of in vivo 31P MRS as a function of magnetic field strength, it is necessary to quantify all B0-dependent parameters used in Eq. (15.6A).
2.2.1. Field Dependence of in vivo 31P MRS Sensitivity
It has been demonstrated that both SNR and the quality of in vivo 31P MRS acquired in human brain are improved at higher fields (24,61–63). Recently, we have quantitatively measured and compared the values of T1, T2* and SNR of PCr resonance peak acquired from the human occipitallobe at 4 tesla and 7 tesla (60). We found that not only T2* of PCr, but also T1 of PCr (i.e. T1,PCr) were shortened at 7 tesla compared to 4 tesla. This observation indicates that the field dependence of T1,PCr is likely determined by two competing relaxation mechanisms, i.e. the chemical shift anisotropy (CSA) and the dipolar interaction that simultaneously influence the 31P longitudinal relaxation time (60,64,65). In the case with dominant CSA contribution, the T1 value decreases with increasing field strength following the relation of ; in contrast, the T1 value increases with increasing field strength when the dipolar interaction mechanism dominates. The opposite trends between these two relaxation mechanisms lead to a decrease in T1,PCr at higher field and offer an advantage for in vivo 31P MRS as a shorter repetition time can be employed which allows more signal averaging within the same sampling time; as a result, improving SNR and leading to a total SNR gain of 56% at 7T as compare to 4T. Moreover, the β value derived from the experimental measurements and Eq. (15.5) in this study was 1.4 (60). This value is in an excellent agreement with the theoretically predicted β value of 1.5 as described in the literature (57).
Another interesting observation of this study is that the linewidth broadening of the phosphate resonance peak with increased field strength is not linearly correlated with the field strength and, thus, at higher field strength, there is a small increase in the line width of phosphate signals (60). Moreover, higher field strength increases the chemical shift dispersion (i.e., in Hz unit) of in vivo 31P MRS linearly with field strength. The combination of these two factors (i.e., large increase in chemical shift dispersion and relatively small increase in linewidth broadening) improves the 31P spectral resolution at 7T (see Fig. 15.2A). This improvement makes it possible to resolve many adjacent phosphate metabolite resonance peaks (e.g., α-ATP versus NAD) and it is especially helpful for resolving the Pi peak from other adjacent resonance peaks. The well-resolved Pi peak is crucial for measuring the ATP production flux and will be discussed later.
2.2.2. Field Dependence of in vivo 17O MRS Sensitivity
17O is a stable oxygen isotope with a spin quantum number of 5/2 and detectable by 17O MRS. It possesses an electric quadrupolar moment and its natural abundance is only 0.037%, which is almost 30 times lower than that of 13C and 2700 times lower than that of 1H. Moreover, the γ value of 17O is 7.4 times lower than that of 1H. These factors attribute to the low inherent 17O sensitivity that might be the major obstacle for the development and application of in vivo 17O MRS approaches.
The relaxation mechanism of 17O spin is distinct from 31P spin. The 17O quadrupolar moment can interact with local electric field gradients and the temporal fluctuations of this interaction induced by molecular motion dominate the 17O relaxation processes and determine both T1 and T2 of H2 17O (66), where T2 is the transverse relaxation time. Theoretically, the T1, T2 and T2* values of 17O water spins are expected to be insensitive to B0 (55, 66). We have experimentally investigated and compared the 17O relaxation times of the natural abundance H2 17O in rat brain at both 4.7 and 9.4 tesla. We confirmed that the 17O relaxation times are B0-independent (67). Recent experimental evidence has indicated that the B0-independence of 17O relaxivity can further extend to a much higher magnetic field, such as 17.6 tesla (68). The field independence of 17O relaxivity reveals that 17O sensitivity gain at higher fields is not compromised by the relaxation times, and this could lead to a substantial sensitivity gain when the field strength increases according to Eq. (15.6B) if the same acquisition parameters (e.g., TR and at) are applied at different field strengths,
| (15.6b) |
Our experimental results showed approximately a four-fold SNR gain in the in vivo 17O MRS signal measured in the rat brain at 9.4 tesla compared to 4.7 tesla (67). Moreover, the β* value deduced from these experimental measurements and Eq. (15.6B) was ∼2 (67), which is close to the theoretically predicted β* value of 7/4 (57). The significant sensitivity gain provides adequate SNR for acquiring the 3 dimensional 17O MRS imaging of the natural abundance H217O from the small brain of rat with a temporal resolution of ∼10 seconds at 9.4T (see one example shown in Fig. 15.2B).
Clearly, the increase of B0 should benefit all of the in vivo multinuclear MRS approaches. In vivo 17O MRS probably benefits the most from the substantial sensitivity gain at ultrahigh fields. On the other hand, in vivo 31P MRS can benefit from both the moderate sensitivity gain and the significant spectral resolution improvement, which is crucial for resolving many overlapping resonance peaks of different metabolites. All these benefits provided by high field strength are essential for improving the reliability of in vivo 17O and 31P MRS and for accurately determining cerebral metabolic fluxes.
3. How to Apply In Vivo 17O MRS for Imaging CMRO2
In vivo 17O MRS methodology has two major applications for studying brain function and cerebral bioenergetics through imaging either cerebral blood flow (CBF) or CMRO2 (51–55, 67, 69–87). Both applications rely upon the measurement of brain H2 17O content and its dynamic change using in vivo 17O MRS. The CBF measurement is based on monitoring the washout rates of inert H2 17O tracer in the brain tissue following an intravascular bolus injection of 17O-labeled water. The CMRO2 measurement is based on monitoring the dynamic changes of metabolically generated H2 17O in the brain tissue from inhaled 17O-labeled oxygen gas (55). There are two types of magnetic resonance approaches for monitoring brain H2 17O in vivo: a direct approach by using 17O MRS detection, and an indirect approach by using 1H magnetic resonance imaging (MRI) to measure the changes in T2- or T1ρ-weighted proton signals caused by the 17O-1H scalar coupling and proton chemical exchange (see recent review article of (55) and the references cited therein). Both direct and indirect approaches are suitable for CBF measurements. However, recent studies indicated that the in vivo 17O MRS approach at ultrahigh fields seems to have more advantages for quantifying and imaging CMRO2, which perhaps is the most important application of in vivo 17O MRS. Here, the in vivo 17O MRS approach for imaging CMRO2 is described in detail.
3.1. Theory and Quantification of CMRO2 Based on In Vivo 17O MRS Approach
In general, there is a similarity between in vivo 17O MRS approach and positron emission tomography (PET) approach (88–91) for measuring CMRO2 through the use of isotropic labeled oxygen gas (17O2 for MRS and 15O2 for PET). After gas exchange in the lung, the inhaled and labeled O2 molecules quickly bind to hemoglobin (Hb) in the blood, forming O2-hemoglobin complexes (HbO2) (see Fig. 15.1). Through the feeding arteries and arterioles, the HbO2 complex enters the brain capillaries. The O2 molecules are dissociated from hemoglobin in the capillaries, then cross the brain blood barrier (BBB) in the form of free gas, diffuse into the brain tissue (intra- and extracellular space), and finally enter mitochondria where they are metabolized and produce the water with the isotope label following the chemical reaction (3):
| (15.7) |
According to this reaction (equivalent to R1 in Fig. 15.1), one oxygen molecule produces two isotopic labeled water molecules (H2 17O for 17O MRS and H215O for PET), which can move out of the mitochondria (traversing the opposite pathway as O2 entry) and finally be washed out from the brain through venules and veins. The dynamic change of the isotope labeled water in the brain is tightly linked to the cerebral oxygen utilization rate. It provides the vital signal source for determining CMRO2 for both 15O PET and 17O MRS.
Though the principle underlying the 17O MRS approach for measuring CMRO2 was historically adopted from the well-established 15O PET approach, there is significant distinction between these two neuroimaging approaches. For instance, one complication in PET is that it is unable to distinguish the 15O signal contribution from 15O2 molecules versus the metabolically generated H215O molecules. This limits the robustness of PET technique for imaging CMRO2 (55, 90). In contrast, the 17O MRS approach specifically detects the metabolically generated H217O without confounding signals from 17O2 molecules because of the “invisibility” of 17O2 in an in vivo 17O spectrum (55). This unique magnetic resonance specificity leads to a great advantage which significantly simplifies the in vivo 17O MRS methodology for measuring and quantifying CMRO2 (55, 87). Nevertheless, the dynamic change of the metabolically generated H217O concentration in the brain during an 17O2 inhalation is interplayed by three parallel processes (see Fig. 15.3): (i) Cerebral oxygen utilization for generating the metabolic H2 17O in the brain tissue, (ii) Cerebral blood perfusion resulting in H2 17O washout from the brain, and (iii) Blood recirculation bringing the metabolically generated H2 17O in the entire body back to the brain. All contributions from these three processes have to be considered for quantifying CMRO2. Based on the Kety – Schmidt theory (92–94), the mass balance of the isotope labeled H217O in the brain tissue during an 17O2 gas inhalation can be derived as (52,54,55,87):
| (15.8) |
where Ca(t), Cb(t) and Cv(t) are the metabolic H217O concentrations in excess of the natural abundance of H217O concentration in the arterial blood, brain tissue and venous blood respectively, as a function of 17O2 inhalation time (t, unit = minute); α is the 17O enrichment fraction of the oxygen atoms in the inhaled 17O2 gas; λ is the brain/blood partition coefficient (95). The factor of two accounts for the fact that one O2 converts to two H2O molecules through oxidative metabolism according to Eq. (15.7); f1 and f2 are two unit conversion factors (54, 87). The correction factor m is used in Eq. (15.8) to account for the water permeability restriction across the brain blood barrier (96), and n is another correction factor that accounts for the permeability restriction occurring when H2 17O molecules which are metabolically generated inside the mitochondria across the mitochondrial membranes (54,87). Both m and n depend on CBF (54,87). The function of Ca(t) (or artery input function) is determined by the total metabolic H217O generated in all aerobic organs of a living body. It can approximate as a linear function of 17O2 inhalation time (i.e., Ca(t)=At, A is a constant) (52, 54, 78, 87). Under this approximation, the solution for solving the differential equation of Eq. (15.8) is:
| (15.9) |
According to this equation, the CMRO2 value at each data point measured at different inhalation time (t) can be calculated by using the experimentally measured CBF, A, n, Cb(t) values and other known constants (f1, f2, m, α and λ). The quantification approach based on Eq. (15.9) is a complete model which accounts for all required parameters for precisely determining CMRO2 (54,55,87).
Fig. 15.3.
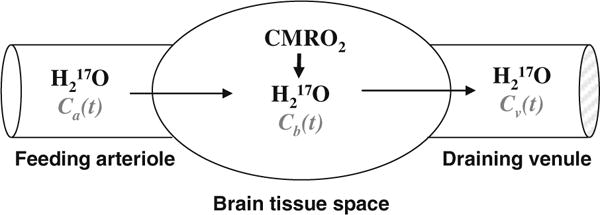
Schematic illustration of a “complete model” describing three parallel processes of the 17O -labeled metabolic water (H2 17O) occurring in the brain when the 17O-labeled oxygen gas molecules are introduced via an inhalation. In this model, only the metabolic H2 17O is considered because the 17O-labeled O2 is invisible by in vivo 17O NMR. Ca(t), Cb(t) and Cv(t) stand for the H2 17O concentration in arteriole, brain tissue and venule, respectively, as a function of the 17O2 inhalation time.
3.2. Measurement and Imaging of CMRO2 using In Vivo 17OMRS Approach
The complete model as described by Eq. (15.9) requires multiple experimental measurements of four variables (A, n and Cb(t) and CBF) in order to calculate CMRO2. Figure 15.4 illustrates the procedures for performing these in vivo 17O MRS measurements and the results of a rat brain study with α-chloralose anesthesia at 9.4 T (54,55).
Fig. 15.4.
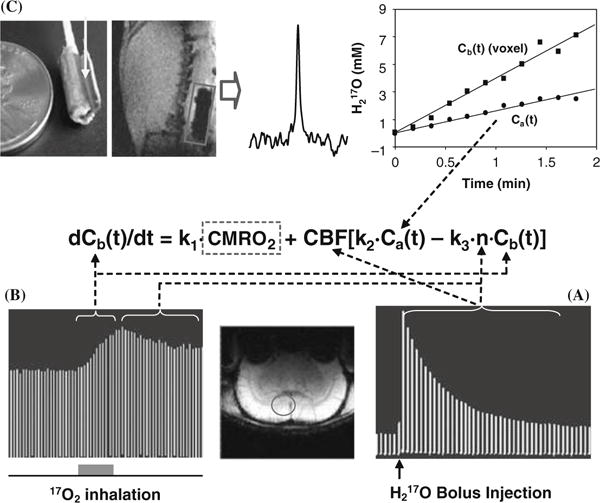
Schematic diagram showing the multiple in vivo 17O measurements for determining CMRO2 using the complete model according to the mass balance equation of Equation (15.8) which links Cb(t), Ca(t), CBF and n with CMRO2. To simplify the equation, three known constants of 2α1, mf2 and m/λ used in Equation (15.8) are replaced by k1, k2 and k3, respectively. (A) Stacked plot of the 17O spectra of cerebral H2 17O tracer from one representative rat brain voxel as indicated by the circle in the anatomical image (low center insert). The spectra were acquired before and after a bolus injection of H2 17O for CBF measurements. (B) Stacked plot of the 17O spectra of the metabolic H2 17O from the same representative voxel acquired before (natural abundance), during (as indicated by the gray bar under the stacked plot) and after a two-minute 17O2 inhalation. (C) Measurement of Ca(t) by using an implanted 17O RF coil (the left insert). The middle insert illustrates a 17O spectrum of natural abundance H2 17O obtained from the rat carotid artery blood by using the implanted RF coil before H217O inhalation. The right insert shows the time course of Ca(t) (circle symbol) and Cb(t) from a representative 3D 17O CSI voxel (square symbol) in the same rat during a two-minute inhalation of 17O2. Finally, the ratio between the 17O signal decay detected after a bolus injection of H2 17O (see Fig. 15.4A) versus the 17O signal decay detected after the cessation of 17O2 inhalation (see Fig. 15.4B) gives the constant of n. Adapted from Zhu et al. of Ref (54).
3.2.1. Imaging CBF
The CBF measurement was performed via bolus injection of a small amount of 17O-enriched water into one internal carotid artery and monitoring the washout process of the H2 17O tracer in the brain using 3 dimensional 17O chemical shift imaging (CSI) (67). Figure 15.4A demonstrates the stacked plots of H2 17O spectra acquired from a single voxel (as indicated in the brain anatomy image) of 3 dimensional 17O CSI data set in a representative rat before and after the H2 17O bolus injection. The peak height of the H2 17O spectra shows an exponential decay and its decay rate determines the CBF value in the CSI voxel (54,55,67).
3.2.2. Imaging Cb(t)
The crucial step for CMRO2 measurements is to monitor and image the dynamic changes of the metabolic H2 17O content in the brain (i.e., Cb(t)) during an inhalation of 17O2 gas. Figure 15.4B illustrates the stacked plots of 17O spectra of cerebral H2 17O from the CSI voxel acquired before, during and after a 2-minute inhalation of 17O2 (54). It indicates excellent 17O sensitivity for detecting the cerebral H2 17O signal and its change during the inhalation; and the approximately linear increase of H2 17O during a short 17O2 inhalation is evident, and the slope is tightly coupled to CMRO2.
One practical challenge for most in vivo MRS approaches is the difficulty for measuring the absolute concentrations of metabolites of interest. Nevertheless, the natural abundance H2 17O signal which can be accurately measured in the brain before the introduction of 17O-labeled oxygen gas (see Fig. 15.4B) provides an excellent internal reference for quantifying the absolute concentration of Cb(t) for each CSI voxel (54, 87). This self-calibration method is independent of the 17O detection sensitivity. This is particularly valuable when a surface RF coil with nonuniform spatial distribution of detection sensitivity is applied.
3.2.3. Other Measurements
The arterial input function of Ca(t) was measured in vivo by an implanted 17O RF coil (97) wrapped around a carotid artery (in the rat). Figure 15.4C illustrates the implanted 17O RF coil, the natural abundance H2 17O signal detected from the rat carotid blood and Ca(t) measured during a two-minute inhalation of 17O2 (54). The experimental result showed an approximately linear relation between the arterial H2 17O concentration and the 17O2 inhalation time, and the linear fitting of Ca(t) gave the value of constant A required by Eq. (15.9). Note that the Ca(t) (see Fig. 15.4C) and Cb(t) (see Fig. 15.4B) measurements could be conducted simultaneously with the configuration of dual 17O RF coils and receivers (97).
Finally, the ratio between the decay rates of H2 17O signal measured after the cessation of 17O2 inhalation (see Fig. 15.4B) versus that after a H2 17O bolus injection (see Fig. 15.4A) gives the constant of n, reflecting the H2 17O permeability restriction across the mitochondrial membranes (54,87).
3.2.4. Imaging CMRO2 in Rat Brain
The values of Cb(t), CBF and n measured from each 17O MRS imaging (MRSI) voxel and the value of A measured from each 17O inhalation measurement in the same animal as demonstrated in Fig. 15.4 can be used to calculate the absolute CMRO2 value as a function of inhalation time according to Eq. 15.9. Figure 15.5A shows one example of CMRO2 time course from a representative 17O MRSI voxel with a temporal resolution of 11 seconds (54). It is evident that the CMRO2 values are independent of 17O2 inhalation time if the first two CMRO2 values characterized with relatively large fluctuations are excluded. These CMRO2 values were averaged for improving measurement accuracy. The same procedure and calculation can be applied to all 17O MRSI voxels for generating 3D CMRO2 images in the rat brain (54, 77). Figure 15.5B demonstrates three adjacent CMRO2 images in the coronal orientation from a representative rat brain. The averaged CMRO2 and CBF values in the rat brains anesthetized with α-chloralose were 2.19 ± 0.14 μmol/g/min and 0.53 ± 0.07 ml/g/min (n=7), respectively (54). The CMRO2 value is consistent with the reported values in the literature, which were measured by other independent techniques under similar physiological condition (98–100).
Fig. 15.5.
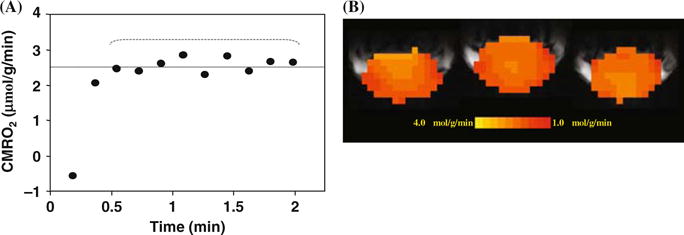
(A) Plot of the calculated CMRO2 values using the complete model as described by Equation (15.9) as a function of 17O2 inhalation time. (B) Three-dimensional coronal CMRO2 images of rat brain measured by in vivo 17O MRS approach during a two-minute 17O2 inhalation. Adapted from Zhu et al of Ref. (s). (See Color Plate)
3.3. Establishing a Robust and Noninvasive 17O MRS Approach for Imaging CMRO2
3.3.1. Noninvasive Approach
The major technical limitation of the complete model for determining CMRO2 using in vivo 17O MRS is the requirement of invasive measurements (e.g., CBF, Ca(t), n). This could significantly limit the potential of this in vivo approach for broad biomedical applications, especially in humans. Thus, it is crucial to examine the feasibility of developing a completely noninvasive 17O approach for imaging CMRO2. Attempts have been made to simplify the experimental procedures and the models for determining CMRO2 based on a number of approximations (52, 53, 73, 79, 81, 87). We discuss one of these models, in which the invasive measurements could be eliminated by using the simplified model based on the Taylor’s and Polynomial Theorems (87). Briefly, the Cb(t) time course can be expressed by a polynomial expansion
| (15.10) |
In this expansion, the first-order (or linear) coefficient of a1 is directly proportional to CMRO2 according to the following equation (87)
| (15.11) |
where α and f1 are known constants (see above). Thus, using this simplified model, only the time course of Cb(t) measured noninvasively by in vivo 17O CSI approach is needed and it can be fitted to the polynomial function given by Eq. (15.10) to calculate the linear coefficient of a1, and ultimately determining CMRO2 according to Eq. (15.11). For practical applications, a quadratic polynomial function provides a good approximation for fitting the time course of Cb(t) with moderate measurement fluctuation (101). We have demonstrated that the CMRO2 value obtained based on the complete model with invasive procedures has no statistical difference from that based on the simplified model and quadratic function fitting where only a single noninvasive measurement of Cb(t) is required (87). Moreover, our results also validated that the linear fitting of Cb(t) could provide a good approximation for determining CMRO2 in the rat brain when the 17O2 inhalation time is relatively short (e.g., 2 minutes) (87). This is consistent with the prediction based on either Eq. (15.8) or (15.10). During the initial 17O2 inhalation period, both Ca(t) and Cb(t) have not built up significantly resulting in near zero value of the second term on the right side of Eq. (15.8). Therefore, Eq. (15.8) can be approximated as a linear differential equation, and Cb(t) becomes a linear function of the inhalation time. This is also evident in Eq. (15.10) in which the high-order terms become negligible when t is short. Therefore, it is possible to use the simplified model for determining and imaging CMRO2 based on a single and completely noninvasive 17O spectroscopic imaging measurement of Cb(t) (55,86,87).
Recently, we have further examined the simplified model for determining CMRO2 under varied physiological conditions (86). In this study, we compared the CMRO2 results obtained with the complete model versus the simplified model using linear fitting of Cb(t) under normothermia (37°C) condition and hypothermia (32°C) condition, which is a well known factor leading to significant suppression of both CBF and CMRO2. Figure 15.6 demonstrates an excellent consistency of the CMRO2 results between the complete and simplified models for either the voxel-wised comparison (Fig. 15.6A) or the averaged CMRO2 comparison (Fig. 15.6B) at both brain temperatures (86). The comparison results reveal the validity of the simplified 17O approach for imaging CMRO2 applicable at a wide physiological range. Additional technical development which further advances the in vivo 17O methodology could ultimately provide the simplest and completely noninvasively 17O neuroimaging approach for imaging CMRO2 (in humans).
Fig. 15.6.
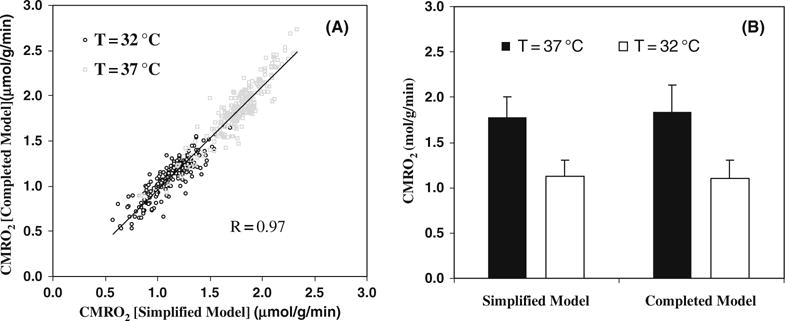
(A) Voxel based CMRO2 calculation and comparison using the completed and simplified models from a representative rat (total voxel number used was 224 for 32°C and 254 for 37°C, voxel size = 75μ). (B) Averaged CMRO2 values in the same rat brain at normothermia (37°C) and hypothermia (32°C) conditions, calculated with simplified and completed model, respectively. Adapted from Zhu et al of Ref. (86).
3.3.2. Robustness and Reliability
Another merit of the 17O CMRO2 imaging approach is its ability for performing repeated CMRO2 imaging measurements with a short interval between two measurements. This is due to the fact that the cerebral H2 17O concentration can quickly reach a new and steady level within a few minutes after the cessation of 17O2 inhalation, which allows repeated CMRO2 measurements in the same subject and experimental session (see Fig. 15.7A). Figure 15.7B shows the excellent reproducibility of repeated CMRO2 measurements in five rats (1st and 2nd measured CMRO2 values were 2.26 ± 0.18 and 2.20 ± 0.14 μmol/g/min giving a ratio of 1.03 ± 0.05 between the consecutive measurements) (86). The results demonstrate the robustness and reliability of the simplified in vivo 17O approach for noninvasively and rapidly imaging CMRO2 repeatedly in a small brain of rat. This capability is particularly valuable for studies aiming at CMRO2 changes induced by physiological or pathological perturbations in which multiple measurements are required under different conditions (e.g., control versus stimulation for brain function study). Therefore, the combination of the simplified model and ultrahigh field in vivo 17O MRS may potentially provide an alternative neuroimaging modality for studying the central role of oxidative metabolism in brain function and neurological diseases (55,77).
Fig. 15.7.
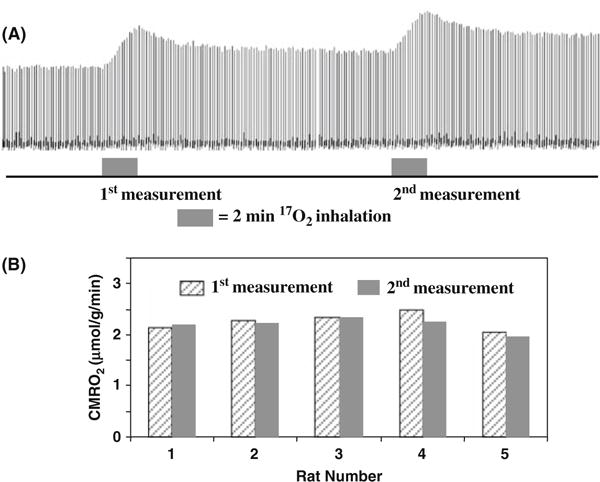
(A) Stacked plots of H217O spectra from a representative voxel of 3D 17O MRSI data acquired before, during and after two consecutive two-min 17O2 inhalations in a rat brain. (B) The comparison results between two repeated CMRO2 measurements in five rat brains. Adapted from Zhu et al of Ref. (86).
3.3.3. Demonstration of in vivo 17O MRS Application for Studying Brain Bioenergetics
It is well documented that the basal CMRO2 is sensitive to the brain temperature (see (3, 102) and the references cited therein). However, most studies reported in the literature were based on the global CMRO2 measurements of entire brain using the Kety-Schmidt method (92, 93), and were limited by the lack of spatial information regarding regional CMRO2. We have conducted a CMRO2 imaging study using 3D in vivo 17O CSI combined with the simplified model at 9.4 tesla for quantifying absolute CMRO2 values in the rat brain at normal brain temperature (37°C) (i.e., normothermia) and mild hypothermia (32°C) conditions (86). Figure 15.8A illustrates an example showing three representative slices of 3D CMRO2 maps from a rat brain under normothermic and hypothermic conditions. These images clearly show significant reduction of CMRO2 crossing the entire brain induced by lowering brain temperature several degrees. This metabolic suppression occurring at hypothermia was consistently observed in all five rats studied (Fig. 15.8B), resulting in an average of 45% CMRO2 reduction as compared to normothermic condition (86). These results indicate that the established in vivo 17O MRS approach is sensitive to determine the dynamic CMRO2 change and its spatial distribution due to physiological perturbation. The measured CMRO2 can be quantitatively correlated to other associated physiological parameter changes. Figure 15.9 illustrates one example showing the quantitative relation between CMRO2 and CBF: both of them were measured by in vivo 17O MRS in the α-chloralose anesthetized rat under a wide range of physiological conditions from normothermia to hypothermia (86). It shows a strong correlation between CBF and CMRO2 with a linear correlation coefficient of R = 0.97 indicating a tight vascular-metabolic coupling in the rat brain.
Fig. 15.8.
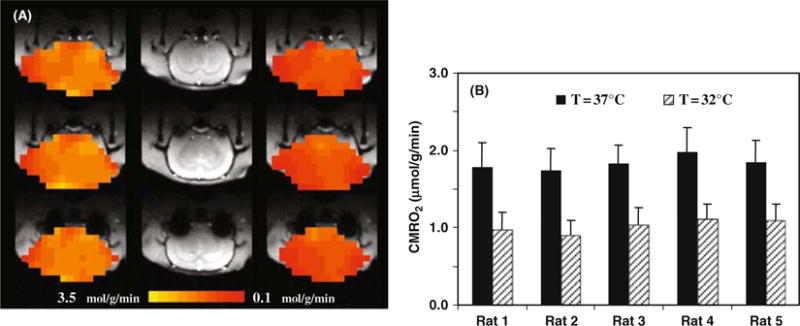
(A) 3D CMRO2 maps of a representative rat brain obtained at normothermia (left column) and hypothermia (right column), and their corresponding anatomic images (middle column). (B) Summary of CMRO2 results measured at normothermia and hypothermia conditions (n = 5). Adapted from Zhu et al of Ref. (86). (See Color Plate)
Fig. 15.9.
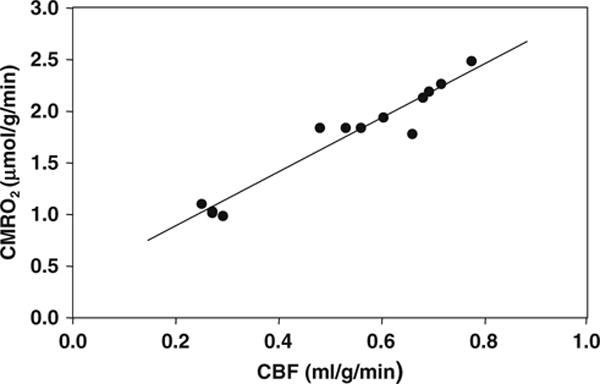
Correlation of CBF and CMRO2 values in the rat brains anesthetized with α-chloralose at brain temperature range of 32–37°C. The linear correlation coefficient (R) was 0.97. Adapted from Zhu et al. of Ref. (86).
4. How to Apply In Vivo 31P MRS for Studying Cerebral ATP Metabolic Fluxes and Bioenergetics
The ATP metabolism for regulating both ATP production and utilization plays a fundamental role in cerebral bioenergetics, brain function and neurodegenerative diseases. Two important chemical reactions that contribute to the brain ATP metabolism are the ATPase and CK reactions. They are coupled together and constitute a three-31P-spin chemical exchange system involving ATP, PCr and Pi (i.e., PCr↔ATP↔Pi). One vital function of this exchange system is to maintain a stable cellular ATP concentration ensuring continuous energy supply for sustaining electro-physiological activity in the brain. Logically, the measures of ATP metabolic fluxes should be more sensitive to the brain activity and energy state and their change than that of steady-state ATP and other HEP concentration. Therefore, they should provide a useful index reflecting cerebral bioenergetics under various brain states. It would be, thus, essential to find a noninvasive and reliable technique being able to assess the cellular exchange rates (or fluxes) of PCr↔ATP↔Pi in brain in situ. The sole approach for serving this purpose is the use of in vivo 31P MRS combined with magnetization transfer method (20,43–47,50,103). However, to completely determine the kinetics and fluxes involved in the PCr↔ATP↔Pi exchange requires extensive measurements and information including three steady-state phosphate metabolite concentrations (i.e., [ATP], [PCr] and [Pi]) and their intrinsic T1 values, and four pseudo first-order chemical reaction rate constants (forward and reverse rate constants for the CK reaction and the ATPase reaction, respectively) (50). The products of the rate constants and their related phosphate concentrations can provide four ATP metabolic fluxes along both forward and reverse reaction directions in the PCr↔ATP↔Pi exchange system (see Fig. 15.10).
Several in vivo 31P MT methods have been developed such as conventional two-spin magnetization saturation transfer (CST), inversion recovery transfer (IT) and two-dimensional chemical exchange spectroscopy (2D-EXSY) (44, 45, 49, 104). They have been applied to physiological studies of ATP metabolism in a variety of organs, from hearts to brains (20, 24, 45, 46, 49, 50, 103, 105–111). Among these methods, the CST method is most commonly used in biomedical research, perhaps due to its methodological simplicity and high efficiency. This method is particularly useful in measuring the forward rate constants and fluxes in the chemical exchange system of PCr↔ATP↔Pi when a frequency-selective RF saturation pulse train is applied to fully saturate the γ-ATP spin. Under this circumstance, the three-spin chemical exchange system of PCr↔ATP↔Pi can be treated as two independent two-spin chemical exchange systems (i.e., PCr↔ATP and ATP↔Pi); consequently, the forward rate constants and fluxes for both the CK reaction (i.e., R4 in Figs. 15.1 and 15.13) and ATPase reaction (i.e., R2 in Figs. 15.1 and 15.13) can be explicitly determined. These forward metabolic fluxes reflect the ATP synthesis or production rates catalyzed by ATPase and CK, respectively. However, the measurements of the reverse CK flux (ATP→PCr) using CST (i.e., by saturating PCr) resulted in an inequality between the forward and reverse CK fluxes (112–114), which is paradoxical, in the sense that the CK fluxes into and out of the PCr pool must be equal when the CK reaction is under chemical equilibrium condition. One possible explanation is that the ATP metabolism involves other chemical exchange reactions besides the CK reaction, for example the ATP hydrolysis reaction (115). Consequently, neglecting ATP hydrolysis may lead to an error in estimating the reverse CK flux. Therefore, it is necessary to consider PCr→ATP↔Pi as a three-spin chemical exchange system in order to accurately determine all kinetic parameters; in particular, the reverse rate constants and fluxes (45,46,50,115,116).
Fig. 15.13.
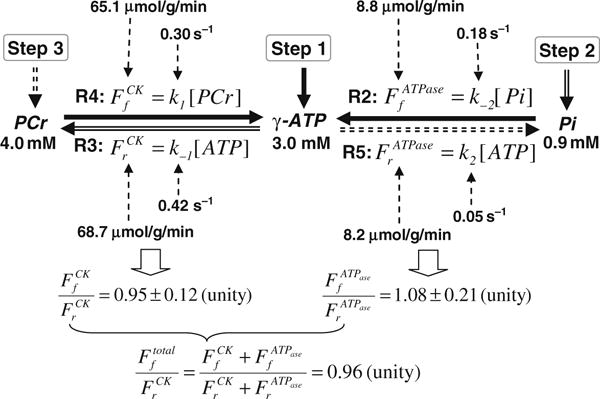
Chart showing the three-step measurements of in vivo 31P MSS MT approach for determining the entire ATP kinetic network and associated metabolic rate constants and fluxes, and the measurement results from the human occipital lobe. Step 1 measures two forward reactions (R2 and R4) along the solid arrows. Step 2 measures the indirectly coupled reverse reaction (R3) along the double-line arrows. Step 3 measures another reverse reaction (R5) along the dotted-line arrows. All results point to the fact that flux ratios satisfy the chemical equilibrium condition.
We will discuss a newly introduced in vivo 31P MT approach being able to noninvasively study the PCr↔ATP↔Pi exchange in the brain explicitly through the in vivo measurements of the following rate constants and fluxes associated with four coupled reactions in different subcellular compartments (50):
The forward flux of ATPase reaction (i.e., R2 in Figs. 15.1 and 15.13) occurring inside the mitochondria;
The forward flux of CK reaction (i.e., R4 in Figs. 15.1 and 15.13),
The reverse flux of ATPase reaction (i.e., R5 in Figs. 15.1 and 15.13) occurring in the cytosol space;
The reverse flux of CK reaction (i.e., R3 in Figs. 15.1 and 15.13).
4.1. In vivo 31P MT Approach for Determining Entire PCr↔ATP↔Pi Exchange
A newly introduced in vivo 31P MT approach for determining all kinetic parameters of the PCr↔ATP↔Pi exchange is called the Multiple Single-site Saturation (MSS) MT approach (50). The MSS approach requires a total of four in vivo 31P spectra: one control spectrum in the absence of RF saturation plus three single-site, RF-saturated spectra with the saturation frequency on PCr, γ-ATP and Pi, respectively (50). The quantification of the ATP metabolic fluxes relies on solving three coupled Bloch equations based on the three-spin chemical exchange model. For simplifying the mathematical derivation, the symbols of a, b and c were used which stand for PCr, ATP and Pi, respectively. The Bloch equations describing the magnetizations of a, b and c and their changes as a function of time are given by (43),
| (15.12a) |
| (15.12b) |
| (15.12c) |
where Ma, Mb and Mc are the magnetizations of PCr, γ-ATP and Pi; , and are the magnetizations at Boltzmann thermal equilibrium; k1 and k−2 are the pseudo first-order forward rate constants involving ATP production through the CK reaction and the ATPase reaction, respectively; k−1 and k2 are the pseudo first-order reverse rate constants involving ATP utilization through the CK reaction and the ATPase reaction, respectively; T1a, T1b and T1c are the intrinsic spin-lattice relaxation times of PCr, γ-ATP and Pi, which are B0 dependent. Four rate constants involving the PCr↔ATP↔Pi exchange and their four associated ATP metabolic fluxes can be determined by the following three-step measurements with frequency-selective RF saturation on the γ-ATP (Step 1), Pi (Step 2) and PCr (Step 3) resonance peak, respectively.
Step 1: Progressive saturation of γ-ATP for determining intrinsic spin-lattice relaxation times of PCr and Pi, forward rate constants and fluxes
The first step of the 31P MT measurements is to apply a frequency-selective RF pulse train for completely saturating the γ-ATP resonance peak with varied saturation time (t) (i.e., the progressive saturation experiment commonly used in the CST approach). For this case, the three-spin chemical exchange system of PCr↔ATP↔Pi can be treated as two independent two-spin chemical exchange systems (i.e., PCr↔ATP and ATP↔Pi) Solving Eqs. (15.12a) and (15.12c) with the boundary condition of Mb = 0 results in Eqs. (15.13a) and (15.13b) (47),
| (15.13a) |
| (15.13b) |
with
Therefore, the parameters of apparent spin-lattice relaxation rates (αa and αc) and intrinsic spin-lattice relaxation times (T1a and T1c) at a given B0 can be determined via regressions as a function of t, where both k1 and k−2 are replaced by their apparent spin-lattice relaxation rate and intrinsic spin-lattice relaxation time (see Eqs. (15.13a) and (15.13b)). Then, the forward rate constants (k1 and k−2) can be calculated by their relations with the apparent spin-lattice relaxation rate and intrinsic spin-lattice relaxation time as depicted above.
When a steady-state condition is approached with complete saturation of γ-ATP (i.e., dMa/dt = 0 and dMc/dt = 0), Eqs. (15.13a) and (15.13b) can be further simplified to the following formulae:
| (15.13c) |
| (15.13d) |
where and are the steady-state magnetizations of a and c when b is fully saturated. Therefore, the forward rate constants of k1 and k−2 can be calculated by using Eqs. (15.13c) and (15.13d). For this case, only two steady-state spectra acquired in the presence and absence of saturating b are needed to determine both k1 and k−2, if T1a and T1c have already been determined at a given B0 through the progressive saturation measurement accordingly to Eqs. (15.13a) and (15.13b). Finally, the forward fluxes for the CK and ATPase reactions can be determined by the following relations,
| (15.13e) |
Step 2: Steady-state saturation of Pi for determining CK reverse rate constant and flux
The second step of the in vivo 31P MSS MT measurements is to apply a frequency-selective RF pulse train for completely saturating the Pi resonance peak with a sufficiently long saturation time resulting in steady-state magnetizations of a and b. For this case, Mc = 0, dMa/dt = 0, dMb/dt = 0, and Eqs. (15.12a) and (15.12b) yield
| (15.14a) |
| (15.14b) |
with
where and are the steady-state magnetizations of a and b when Pi is fully saturated. Only Eq. (15.14a) is needed in the MSS MT approach to determine the CK reverse rate constant (k−1) by using the measured magnetizations (i.e., , and ) and other relaxation parameters (i.e., αa and T1a), which are determined by the measurement in Step 1. The reverse flux of CK reaction can be calculated by the following relation:
| (15.14c) |
Step 3: Steady-state saturation of PCr for determining ATPase reverse rate constant and flux
The third step of the in vivo 31P MSS MT measurements is to apply a frequency-selective RF pulse train for completely saturating the PCr resonance peak with a sufficiently long saturation time resulting in steady-state magnetizations of b and c. For this case, Ma=0, dMb/dt=
| (15.15a) |
| (15.15b) |
where and are the steady-state magnetizations of b and c when PCr is fully saturated. Only Eq. (15.15a) is needed in the MSS MT approach to determine the ATPase forward rate constant (k2) by using the measured magnetizations (i.e., , and ) and other relaxation parameters (i.e., αc and T1c), which are determined by the measurement in Step 1. The reverse flux of the ATPase reaction can be calculated via following relation:
| (15.15c) |
Therefore, by combining these three measurement steps, one is able to measure all kinetic parameters which determine the entire exchange of PCr↔ATP↔Pi including all four ATP metabolic fluxes for both forward and reverse reaction directions as schematically summarized in Figs. 15.1 and 15.13. In total, a minimal number of three selective saturated in vivo 31P spectra plus one control in vivo 31P spectrum (for quantifying [ATP], [Pi], [PCr], i.e., , and ) are required by the MSS MT approach for the measurements (50).
4.2. Application of in vivo 31P MSS MT Approach in Human Brain at 7T
The in vivo 31P MT measurements can significantly benefit from the high-field advantages of improved MR detection sensitivity and spectral resolution. We have conducted extensive measurements at 7T for validating the in vivo 31P MSS MT approach, and ultimately, determining the entire chemical exchange of PCr↔ATP↔Pi in the human occipital lobe (20,50).
4.2.1. Progressive Saturation for Measuring Intrinsic T1, Forward Rate Constants And Fluxes
Figure 15.10A illustrates the progressive saturation transfer measurements and related in vivo 31P spectra when the γ-ATP resonance peak was completely saturated (i.e., Step 1 measurement used in the in vivo 31P MSS MT approach). It shows a gradual decrease of Pi signal when the γ-ATP saturation time increases because of the chemical exchange between γ-ATP and Pi (i.e., ATP↔Pi) and the increased magnetization transfer effect (20). This MT effect was observed on the chemical-exchangeable Pi resonance but not for those adjacent and non-chemical-exchangeable phosphate metabolites such as PDE and PME groups. Moreover, the MT effect on Pi disappeared when the RF saturation frequency was moved to the opposite side of the in vivo 31P spectrum with the same chemical shift difference as shown in Figure 15.10B. Figure 15.10C plots the quantitative relation between the normalized Pi signal intensity (i.e., in Eq. (15.13a)) under the γ-ATP saturation with the saturation time of t. This plot can be used to calculate the intrinsic T1 of Pi (i.e., T1a) and the forward ATPase reaction rate constant (k1) by the regression fitting according to Eq. (15.13a). Then, the forward ATPase reaction flux can be determined according to Eq. (15.13e). The same in vivo 31P MT spectral data can be applied to quantify the MT effect on the PCr resonance as a function of the γ-ATP saturation time, ultimately, to determine the intrinsic T1 of PCr (i.e., T1c) and the forward CK reaction rate constant and flux according to Eqs. (15.13b) and (15.13e).
Fig. 15.10.
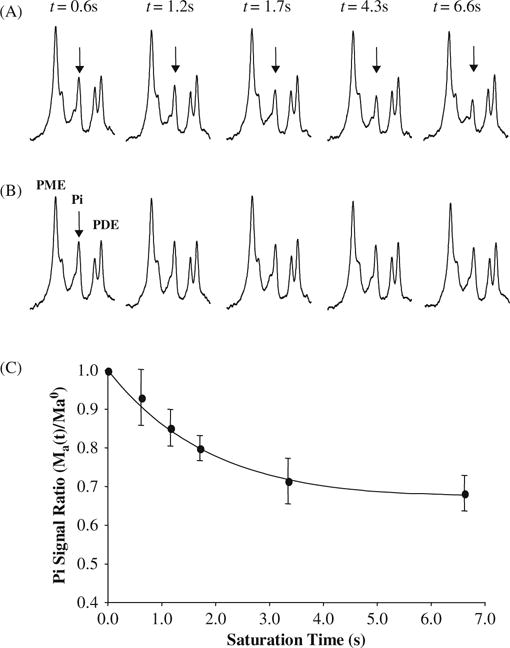
(A) Progressive saturation measurements and averaged in vivo 31P spectra (partially displayed) as a function of γ-ATP saturation time (t), and (B) their corresponding control 31P spectra. The arrows point to the Pi resonance peak. (C) Normalized Pi signal change as a function of t and regression fitting (solid line) according to Eq. (15.13a). Adapted from Lei et al. of Ref. (20).
4.2.2. Steady-State Saturation for Measuring Forward Rate Constants And Fluxes
One technical limitation of progressive saturation approach is the requirement of a number of saturated in vivo 31P spectra with varied saturation time (e.g., Fig. 15.10). One alternative but more robust approach is the use of steady-state saturation if the intrinsic T1 values have been determined at a given B0 (see details in the discussion of Step 1 measurement above). Figure 15.11 demonstrates such a measurement (i.e., Step 1) and the in vivo 31P MT spectra acquired in the absence (control spectrum; Fig. 15.11A) and presence (saturated spectrum; Fig. 15.11B) of sufficiently long RF saturation for ensuring complete saturation of the γ-ATP resonance peak and approaching steady-state magnetizations for both Pi and PCr. Figure 15.11C shows the difference spectrum by subtracting the control and γ-ATP saturated spectra (20, 50). The relative Pi signal reduction can be used to calculate the forward rate constant and flux for the ATPase reaction according to Eqs. (15.13d) and (15.13e). Similarly, the relative PCr signal reduction can be used to calculate the forward rate constant and flux for the CK reaction according to Eqs. (15.13c) and (15.13e).
Fig. 15.11.
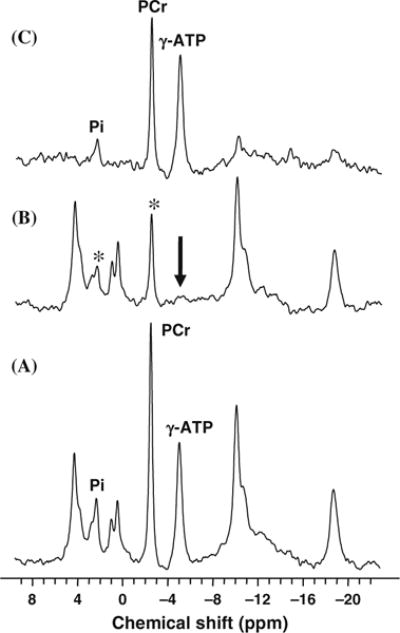
In vivo 31P spectra acquired from a health human occipital lobe in the absence (A) and presence (B) of complete γ-ATP saturation, and the difference spectrum (C) between the two. Only the Pi and PCr resonance peaks show the magnetization transfer effect due to the saturation. The intensity reduction of Pi can be used to determine the forward rate constant and flux for the ATPase reaction, and the intensity reduction of PCr can be used to determine the forward rate constant and flux for the CK reaction. Adapted from Lei et al. of Ref. (20).
4.2.3. In vivo 31P MSS MT Measurements for Determining all ATP Metabolic Fluxes Involving PCr↔ATP↔Pi Exchange in Human Occipital Lobe
Figure 15.12 illustrates one example of in vivo 31P MSS MT measurements in the human occipital lobe (50). A total of four in vivo 31P spectra were collected in the absence (Fig. 15.12A) and presence of complete RF saturation on the resonance peak of Pi (Step 2; Fig. 15.12B), PCr (Step 3; Fig. 15.12C) and γ-ATP (Step 1; Fig. 15.12D), respectively. It reveals that single-site saturation on one phosphate spin can lead to significant magnetization reductions in the other two coupled phosphate spins through the three-spin chemical exchange system of PCr↔ATP↔Pi. For instance, the PCr saturation results in a significant signal reduction for both γ-ATP and Pi as shown in Fig. 15.12C. All required steady-state saturated magnetizations can be determined by using the three saturated in vivo 31P spectra as shown in Fig. 15.12B–D, and all equilibrium magnetizations can be determined by using the control in vivo 31P spectrum as shown Fig. 15.12A. The values of these magnetizations were used to determine four rate constants (k1, k2, k−1 and k−2) and four fluxes ( , , and associated with the PCr↔ATP↔Pi exchange in the human brain. These measured results and the ratios between the forward and reverse fluxes are summarized in Fig. 15.13 indicating several important observations. First, there is no statistical difference between the forward and reverse exchange fluxes for both the CK reaction (i.e., versus ; p = 0.38) and the ATPase reaction (i.e., versus ; p = 0.46) (50). Second, the ratios between the forward and reverse fluxes for the CK reaction and the ATPase reaction are close to unity. In general, the CK fluxes reflect the nonoxidative phosphorylation and the ATPase fluxes, in contrast, reflect oxidative phosphorylation. Both of these phosphorylation pathways can contribute to the total ATP production flux (i.e., ) and the total ATP utilization flux (i.e., ). The ratio between and is again close to unity (=0.96) indicating that the total ATP production flux equals the total ATP utilization flux. These results lead us to conclude that the fluxes measured by the in vivo 31P MSS MT approach satisfy the chemical equilibrium conditions for the CK and ATPase reactions in the human brain, and indicate that the MSS MT approach is able to explicitly determine four rate constants in the PCr↔ATP↔Pi kinetic process and their associated ATP metabolic fluxes in the brain noninvasively. Nevertheless, such equal forward and reverse flux relations are unable to be obtained for the saturation transfer measurement if the three-spin PCr↔ATP↔Pi exchange system was treated as two independent two-spin change systems (i.e., PCr↔ATP and ATP↔Pi) even when the same experimental MSS MT data were analyzed (50). These findings clearly indicate that the chemical exchange system of PCr↔ATP↔Pi has to be treated as a three-spin exchange system in order to accurately determine the reverse rate constants and fluxes for both the CK and ATPase reactions.
Fig. 15.12.
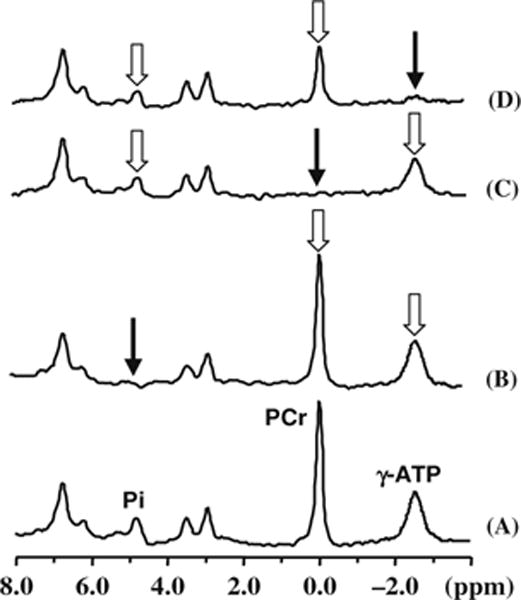
In vivo 31P MSS MT measurements and four spectra acquired from a representative human occipital lobe in the absence (A) and presence of complete RF saturation on the resonance peak of (B) Pi (Step 2), (C) PCr (Step 3) and (D) γ-ATP (Step 1), respectively. The narrow arrows indicate the saturation sites and the wide arrows indicate the signal reductions due to the magnetization transfer. Adapted from Du et al. of Ref. (50).
The PCr↔ATP↔Pi exchange system in a normal brain likely reaches chemical equilibrium (or near equilibrium) under most circumstances. As long as the equilibrium condition is satisfied, one can further simplify the in vivo 31P MT measurement procedure by using the chemical equilibrium relation (50). In this case, only two in vivo 31P spectra (one control and another with full saturation of γ-ATP as illustrated in Fig. 15.11) are practically required to determine the entire PCr↔ATP↔Pi exchange process (50). Thus, this simplification can make the in vivo 31P MT measurement more robust for rapidly determining the ATP metabolic flux changes induced by either physiological or pathological perturbations.
5. Perspective and Discussion
Despite its low detection sensitivity compared to 1H MRS, researchers in the past three decades have revealed the great merits of in vivo heteronuclear MRS for a broad range of biomedical applications. Two of them (i.e., 31P and 17O) have been discussed here in great detail and each method provides a unique tool for noninvasively studying specific metabolic pathways in the brain through the measurements of metabolite concentrations and biochemical reaction fluxes. The combination of different in vivo multinuclear MRS approaches may significantly enhance the MR capability to perform comprehensive studies concerning brain physiology, neurochemistry, bioenergetics and their relations to brain function. This point is addressed by answering the following questions:
5.1. Why is in vivo 31P MT Approach Attractive and Promising for Studying Brain Bioenergetics?
A large amount of research efforts have been spent since early 1980s for exploring the possibility of determining the ATP metabolic fluxes using a variety of in vivo 31P MRS MT approaches and great progress has been made (20, 44–46, 49, 50, 103–110, 115, 117). Nevertheless, the course for advancing the in vivo 31P MRS MT methodology and its clinical application has been significantly slowed down particularly in brain studies during the last decade. One of the major causes is probably in regard to two controversial observations in this research field. The first one is the inconsistency in the results of the measured reverse ATP fluxes in the literature (e.g., (112–114)). In our opinion, this inconsistency is likely caused by the lack of comprehensive spin-exchange models used in the early research work. And this controversy can be resolved by using more sophisticated three-spin exchange models for accurately determining the reverse ATP fluxes for both the CK and ATPase reactions (45,46,50,115,116).
Another surprising observation has raised question regarding whether the measured forward ATPase reaction flux truly reflects the oxidative phosphorylation in the mitochondria in a living organ. The ATP synthesis reaction (i.e., R2 in Figs. 15.1 and 15.13) catalyzed by the coupled activities of glycolytic enzymes glyceraldehyde-3-phosphate dehydrogenase (GAPDH) and phosphoglycerate kinase (PGK) have been shown to be a major contributor to the Pi → ATP reaction flux measured by the 31P MT approaches in E. coli (118), yeast (119), liver (120), and the myocardium (121). For instant, in the perfused rat heart, GAPDH/PGK mediated exchange dominates the Pi → ATP flux measured by the 31P MT approach in the myocardium (121). Under these circumstances, only if the GAPDH/PGK effect was eliminated either directly using exogenous inhibitor iodoacetate or indirectly by eliminating all exogenous and endogenous sources of glucose, the myocardium Pi → ATP flux determined by the in vivo 31P MRS MT measurement was found to be the same as the net rate of oxidative ATP synthesis calculated as the product of the cardiac oxygen consumption rate and the P:O ratio, and then the correlation between the measured flux and oxygen consumption rate became evident (121). These observations and findings reveal the complexity of biological systems and potential limitation of in vivo 31P MRS MT approaches for directly measuring the ATP metabolic flux related to oxidative phosphorylation in some organs such as heart and liver. Interestingly, in contrast to the observations in the myocardium, and analogous observations in yeast, E. coli and liver, the Pi → ATP flux measured by in vivo 31P MRS MT in the human brain is similar to the net oxidative ATP synthesis rate which can be estimated by the available CMRO2 data and the P:O ratio based on a tight link between the cerebral oxidative phosphorylation and oxygen utilization under normal physiological condition (20, 50). The CMRO2 value in the human occipital lobe has been measured to be 1.71 μmol/g/min by PET (91). The ATP synthesis rate attributed by oxidative phosphorylation can be calculated by multiplying this CMRO2 value by the P:O ratio of ∼2.5 (122) and factor of 2, resulting in an estimated value of 8.6 μmol/g/min. This rate is almost identical to the cerebral ATP synthesis rate of , which was directly measured by in vivo 31P MRS MT (50). A similar relation was also evident in the animal studies showing a tight correlation between the measured Pi → ATP flux and the estimated oxidative phosphor rate (49,123). These comparisons of results, thus, lead us to conclude that in the brain, the Pi→ATP flux measured by the in vivo 31P MT approach equals the net oxidative ATP synthesis rate linking to the cerebral oxidative phosphorylation, and this flux should provide a vital index reflecting the major bioenergetics for supporting brain activity and function (7, 12, 20, 123). This conclusion makes in vivo 31P MT attractive for studying the central role of the cerebral bioenergetics associated with oxidative phosphorylation and brain activity, though the underlying mechanism for explaining the discrepancy in the 31P MT measurements between the brain and other organs (e.g., heart and liver) remains to be explored (20).
5.2. What Is the Possible Role that in vivo 31P and 17O MRS can Play for Studying Brain Activation and Function?
As mentioned earlier, both in vivo 31P and 17O MRS methods can provide noninvasive tools for studying brain metabolism and bioenergetics. Each of these methods has unique features for probing the metabolic functions in mitochondria. Specifically, the in vivo 17O MRS imaging approach is useful for mapping the absolute CMRO2 within a relatively short 17O2 inhalation time. The CMRO2 images should reflect the total metabolic rate of oxygen utilization (i.e., R1 in Fig. 15.1) and its spatial distribution in the brain. In contrast, the in vivo 31P MT approach is powerful for measuring all the cerebral ATP metabolic fluxes associated with four ATP reactions (i.e., R2 to R5 in Fig. 15.1) occurring in different subcellular compartments. All of these measured physiological parameters can be linked to brain ATP energy and ATP transportation. Therefore, the combination of in vivo 31P and 17O MRS methods may provide an essential in vivo MR tool being able to studying the crucial roles of both oxygen and ATP metabolisms in cerebral bioenergetics and brain functions.
One relevant question in the brain function and neuroimaging research fields is how much extra brain energy is needed during the brain stimulation and/or task performance. This topic is still in intense debate, especially regarding how much CMRO2 is induced by brain stimulation (7, 8, 91, 124–136). A more important question, perhaps, is how many more ATP molecules are consumed during brain activation as compared to the resting brain because of a close relation between the ATP utilization and energy consumption (3, 7, 8). The answers to these questions are central for understanding the mechanisms underlying most modern neuroimaging techniques including two most popular methods of the functional MRI (fMRI) based on the blood oxygenation level dependent (BOLD) contrast (137–142) and PET (7,8,143). One major hurdle for addressing the questions is the lack of sophisticated and robust neuroimaging modalities for directly assessing the absolute CMRO2 and CMRATP, which is equivalent to in this article, and their changes elevated by brain activation. Therefore, the in vivo 31P and 17O MRS approaches as described here could fill this methodological gap and make significant contribution in understanding the relation between brain bioenergetics and function. This notion is convincingly supported by several lines of evidence provided by recent research progresses. One example of these progresses was to apply the in vivo 17O MRS imaging approach for successfully mapping CMRO2 images in the cat brain under resting and visual stimulation conditions (144). A significant CMRO2 increase was observed in the activated cat visual cortex. This initial observation indicates the possible central role of oxygen metabolism for supporting the elevated brain activity during activation.
Another significant progress is related to the in vivo 31P MT application in the rat brain for studying the quantitative correlation between the CMRATP and the varied brain activity from mild anesthesia state to the isoelectric state (123). In this study, we observed a strong correlation between the Pi→ATP flux measured by the in vivo 31P MT approach and the brain activity quantified by EEG signal (123). This result indicates a tight correlation between the ATP synthesis rate and brain bioenergetic demand under varied brain activity states. Therefore, the measurement of Pi→ATP flux should provide a sensitive energy index for quantifying the brain bioenergetics. In contrast, the steady-state HEP concentrations (e.g., [ATP], [PCr] and [Pi]) and pH are relatively stable in a wide range of brain activity level (123). These results support the view that one of the major functions of the PCr↔ATP↔Pi exchange process is to sustain the balance between the ATP supply and demand in the brain resulting in a stable ATP concentration under the normal physiological condition. Such a balance is likely maintained by the rapid adjustments of both forward and reverse metabolic fluxes involved in the PCr↔ATP↔Pi exchange according to the change of brain energy. We conclude that the ATP metabolic flux measurements using the in vivo 31P MT approach should be more useful for studying the brain bioenergetic change in response to brain activation as compared to the steady-state HEP concentration measurements using conventional in vivo 31P MRS. This is evident from two high-field in vivo 31P MRS studies showing a few percents of decrease in the PCr signal in contrast to a >30% increase in the CK forward flux in the human visual cortex during visual stimulation (108, 145). It is conceivable that the in vivo 31P MT approach, as a sole in vivo tool being able to directly and quantitatively measure the cerebral ATP metabolic fluxes, will play a vital role for studying the brain bioenergetics associated with brain function and activation. One particularly interesting question possibly to be addressed by this in vivo 31P MT approach is whether more ATP utilization is required by the elevated neuronal activity, and if yes, how much more is needed? The answers should advance our understanding regarding the central roles of cerebral oxidative phosphorylation and mitochondria in supporting brain function.
5.3. What are the Potential Applications of in vivo 31P and 17O MRS for Clinical Research and Diagnosis?
In vivo MRS methodology can provide detailed metabolite fingerprints in the living organs, and it is perhaps one of the most classic molecular imaging modalities. Many metabolites and their abnormal changes, detectable by in vivo MRS, have been proved to be tightly linked to various brain diseases and disease progression.
Both of the in vivo 17O MRS and 31P MRS MT approaches as discussed here are especially useful for studying the brain oxidative metabolism and the mitochondrial metabolic function. They would provide opportunities for a variety of clinical brain research and potentially for clinical diagnosis because of the obvious role of oxidative metabolism in the pathology associated with many brain disorders and neurodegenerative diseases such as schizophrenia, Alzheimer’s disease, Huntington’s disease, Parkinson’s disease, mitochondrial dysfunction and aging problems (e.g., (146–150)). One line of evidence is the histopathological findings indicating that the activity of cytochrome oxidase, the key mitochondrial enzyme that catalyzes the reduction of oxygen to form water, is significantly impaired in schizophrenic (146) and Alzheimer’s patients (147, 148). Another line of evidence is from the studies of diseases caused by mitochondrial DNA mutations suggesting that a variety of degenerative processes in Parkinson’s disease and Alzheimer’s disease may be associated with defects in mitochondrial oxidative phosphorylation (151, 152). Thus, the in vivo 17O MRS and 31P MT approaches may play vital roles for investigating the neurodegenerative diseases associated with the mitochondrial abnormality and metabolic syndrome with great potential in clinical diagnosis and monitoring the brain functional recovery after medical treatment.
6. Conclusion
Although, in reality, in vivo heteronuclear MRS has not been widely applied compared to 1H MRI/MRS due to its lower detection sensitivity, the currently available ultrahigh MRI/MRS scanners, technology and their associated high field merits have undoubtedly stimulated the in vivo heteronuclear MRS research and methodological development (153). Significant progresses have been made for advancing in vivo MRS in brain research. One of the unique in vivo MRS utilities is to noninvasively measure the cerebral metabolic fluxes and their dynamic changes, which may lead to many important applications in medical research. Two interesting and exciting applications of the in vivo 17O MRS approach and/or 31P MRS MT approach are: (i) to study the dynamic changes in oxygen utilization and oxidative ATP metabolism during activation in normal brains; (ii) to explore the possibility for detecting abnormality in the oxidative metabolic fluxes during the progression of brain diseases prior to showing metabolite concentration abnormality. The outcomes from these applications will be essential for better understanding of brain function and dysfunction. Finally, the in vivo 17O and 31P MRS can be readily combined with many other MRI utilities for imaging brain anatomy, perfusion, diffusion and BOLD etc within the same scanning session. This combined MRS/MRI strategy can make it particularly powerful in neuroscience research.
Acknowledgments
The authors would like to thank Drs. Hellmut Merkle, Run-Xia Tian, Peter Andersen, Gregor Adriany, Pete Thelwall and Mr. John Strupp for their technical assistance, support and scientific discussion. Part of the reviewed work was supported by NIH grants of NS41262, EB02632, NS39043, EB00329, P30NS057091 and P41 RR08079, the W.M. Keck Foundation and the MIND Institute.
References
- 1.Boyer PD. What makes ATP synthase spin? Nature. 1999;402(6759):247, 249. doi: 10.1038/46193. [DOI] [PubMed] [Google Scholar]
- 2.Stock D, Leslie AG, Walker JE. Molecular architecture of the rotary motor in ATP synthase. Science. 1999;286(5445):1700–1705. doi: 10.1126/science.286.5445.1700. [DOI] [PubMed] [Google Scholar]
- 3.Siesjo BK. Brain energy metabolism. New York: Wiley; 1978. pp. 101–110. [Google Scholar]
- 4.Raichle ME. Circulatory and metabolic correlates of brain function in normal humans. In: Mountcastle VB, Plum F, Geiger SR, editors. Handbook of Physiology-The Nervous System. Bethesda: American Physiological Society; 1987. pp. 643–674. [Google Scholar]
- 5.Clarke DD, Sokoloff L. Circulation and energy metabolism of the brain. In: Siegel GJ, et al., editors. Basic Neruochemistry: Molecular, Cellular and Medical Aspects. Philadephia: Lippincott-Raven Publishers; 1999. pp. 633–669. [Google Scholar]
- 6.Shulman RG, Rothman DL, Behar KL, Hyder F. Energetic basis of brain activity: Implications for neuroimaging. Trends Neurosci. 2004;27(8):489–495. doi: 10.1016/j.tins.2004.06.005. [DOI] [PubMed] [Google Scholar]
- 7.Hyder F, Patel AB, Gjedde A, Rothman DL, Behar KL, Shulman RG. Neuronal-glial glucose oxidation and glutamatergic-GABAergic function. J Cereb Blood Flow Metab. 2006;26(7):865–877. doi: 10.1038/sj.jcbfm.9600263. [DOI] [PubMed] [Google Scholar]
- 8.Raichle ME, Mintun MA. Brain work and brain imaging. Annu Rev Neurosci. 2006;29:449–476. doi: 10.1146/annurev.neuro.29.051605.112819. [DOI] [PubMed] [Google Scholar]
- 9.Hevner RF, Wong-Riley MT. Regulation of cytochrome oxidase protein levels by functional activity in the macaque monkey visual system. J Neurosci. 1990;10(4):1331–1340. doi: 10.1523/JNEUROSCI.10-04-01331.1990. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 10.Hevner RF, Duff RS, Wong-Riley MT. Coordination of ATP production and consumption in brain: Parallel regulation of cytochrome oxidase and Na+, K+-ATPase. Neurosci Lett. 1992;138(1):188–192. doi: 10.1016/0304-3940(92)90502-x. [DOI] [PubMed] [Google Scholar]
- 11.Rolfe DF, Brown GC. Cellular energy utilization and molecular origin of standard metabolic rate in mammals. Physiol Rev. 1997;77(3):731–758. doi: 10.1152/physrev.1997.77.3.731. [DOI] [PubMed] [Google Scholar]
- 12.Attwell D, Laughlin SB. An energy budget for signaling in the grey matter of the brain. J Cereb Blood Flow Metab. 2001;21(10):1133–1145. doi: 10.1097/00004647-200110000-00001. [DOI] [PubMed] [Google Scholar]
- 13.Shulman RG, Rothman DL, Hyder F. Stimulated changes in localized cerebral energy consumption under anesthesia. Proc Natl Acad Sci USA. 1999;96(6):3245–3250. doi: 10.1073/pnas.96.6.3245. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Magistretti PJ, Pellerin L, Rothman DL, Shulman RG. Energy on demand. Science. 1999;283(5401):496–497. doi: 10.1126/science.283.5401.496. [DOI] [PubMed] [Google Scholar]
- 15.Wallimann T, Hemmer W. Creatine kinase in non-muscle tissues and cells. Mol Cell Biochem. 1994;133–134:193–220. doi: 10.1007/BF01267955. [DOI] [PubMed] [Google Scholar]
- 16.Saks VA, Ventura-Clapier R, Aliev MK. Metabolic control and metabolic capacity: Two aspects of creatine kinase functioning in the cells. Biochim Biophys Acta. 1996;1274(3):81–88. doi: 10.1016/0005-2728(96)00011-4. [DOI] [PubMed] [Google Scholar]
- 17.Kemp GJ. Non-invasive methods for studying brain energy metabolism: what they show and what it means. Dev Neurosci. 2000;22(5–6):418–428. doi: 10.1159/000017471. [DOI] [PubMed] [Google Scholar]
- 18.Shulman RG, Brown TR, Ugurbil K, Ogawa S, Cohen SM, den Hollander JA. Cellular applications of 31P and 13C nuclear magnetic resonance. Science. 1979;205(4402):160–166. doi: 10.1126/science.36664. [DOI] [PubMed] [Google Scholar]
- 19.Ackerman JJH, Grove TH, Wong GG, Gadian DG, Radda GK. Mapping of metabolites in whole animals by 31P NMR using surface coils. Nature. 1980;283:167–170. doi: 10.1038/283167a0. [DOI] [PubMed] [Google Scholar]
- 20.Lei H, Ugurbil K, Chen W. Measurement of unidirectional Pi to ATP flux in human visual cortex at 7 Tesla using in vivo 31P magnetic resonance spectroscopy. Proc Natl Acad Sci U S A. 2003;100:14409–14414. doi: 10.1073/pnas.2332656100. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Gadian DG, Williams SR, Bates TE, Kauppinen RA. NMR spectroscopy: current status and future possibilities. Acta Neurochir Suppl (Wien) 1993;57:1–8. doi: 10.1007/978-3-7091-9266-5_1. [DOI] [PubMed] [Google Scholar]
- 22.Weiner MW. NMR spectroscopy for clinical medicine. Animal models and clinical examples. Ann N Y Acad Sci. 1987;508:287–299. doi: 10.1111/j.1749-6632.1987.tb32911.x. [DOI] [PubMed] [Google Scholar]
- 23.Hossmann KA. Studies of experimental cerebral ischemia with NMR spectroscopy. Arzneimittelforschung. 1991;41(3A):292–298. [PubMed] [Google Scholar]
- 24.Lei H, Zhu XH, Zhang XL, Ugurbil K, Chen W. In vivo 31P magnetic resonance spectroscopy of human brain at 7 T: An initial experience. Magn Reson Med. 2003;49:199–205. doi: 10.1002/mrm.10379. [DOI] [PubMed] [Google Scholar]
- 25.Petroff OA, Prichard JW, Behar KL, Alger JR, den Hollander JA, Shulman RG. Cerebral intracellular pH by 31P nuclear magnetic resonance spectroscopy. Neurology. 1985;35(6):781–788. doi: 10.1212/wnl.35.6.781. [DOI] [PubMed] [Google Scholar]
- 26.Burt CT, Ribolow HJ. A hypothesis: Noncyclic phosphodiesters may play a role in membrane control. Biochem Med. 1984;31(1):21–30. doi: 10.1016/0006-2944(84)90054-1. [DOI] [PubMed] [Google Scholar]
- 27.Burt CT, Ribolow H. Glycerol phosphorylcholine (GPC) and serine ethanolamine phosphodiester (SEP): Evolutionary mirrored metabolites and their potential metabolic roles. Comp Biochem Physiol Biochem Mol Biol. 1994;108(1):11–20. doi: 10.1016/0305-0491(94)90158-9. [DOI] [PubMed] [Google Scholar]
- 28.Ross BM, Moszczynska A, Blusztajn JK, Sherwin A, Lozano A, Kish SJ. Phospholipid biosynthetic enzymes in human brain. Lipids. 1997;32(4):351–358. doi: 10.1007/s11745-997-0044-x. [DOI] [PubMed] [Google Scholar]
- 29.Podo F. Tumour phospholipid metabolism. NMR Biomed. 1999;12(7):413–439. doi: 10.1002/(sici)1099-1492(199911)12:7<413::aid-nbm587>3.0.co;2-u. [DOI] [PubMed] [Google Scholar]
- 30.Young RS, Chen B, Petroff OA, Gore JC, Cowan BE, Novotny EJ, Jr, Wong M, Zuckerman K. The effect of diazepam on neonatal seizure: In vivo 31P and 1H NMR study. Pediatr Res. 1989;25(1):27–31. doi: 10.1203/00006450-198901000-00006. [DOI] [PubMed] [Google Scholar]
- 31.Welch KM, Levine SR, Martin G, Ordidge R, Vande Linde AM, Helpern JA. Magnetic resonance spectroscopy in cerebral ischemia. Neurol Clin. 1992;10(1):1–29. [PubMed] [Google Scholar]
- 32.Duncan JS. Imaging and epilepsy. Brain. 1997;120(Pt 2):339–377. doi: 10.1093/brain/120.2.339. [DOI] [PubMed] [Google Scholar]
- 33.Hetherington HP, Pan JW, Spencer DD. 1H and 31P spectroscopy and bioenergetics in the lateralization of seizures in temporal lobe epilepsy. J Magn Reson Imaging. 2002;16(4):477–483. doi: 10.1002/jmri.10177. [DOI] [PubMed] [Google Scholar]
- 34.Brown GG, Levine SR, Gorell JM, Pettegrew JW, Gdowski JW, Bueri JA, Helpern JA, Welch KM. In vivo 31P NMR profiles of Alzheimer’s disease and multiple subcortical infarct dementia. Neurology. 1989;39(11):1423–1427. doi: 10.1212/wnl.39.11.1423. [DOI] [PubMed] [Google Scholar]
- 35.Pettegrew JW, Klunk WE, Panchalingam K, McClure RJ, Stanley JA. Magnetic resonance spectroscopic changes in Alzheimer’s disease. Ann N Y Acad Sci. 1997;826:282–306. doi: 10.1111/j.1749-6632.1997.tb48480.x. [DOI] [PubMed] [Google Scholar]
- 36.Riehemann S, Volz HP, Smesny S, Hubner G, Wenda B, Rossger G, Sauer H. Phosphorus 31 magnetic resonance spectroscopy in schizophrenia research. Pathophysiology of cerebral metabolism of high-energy phosphate and membrane phospholipids. Nervenarzt. 2000;71(5):354–363. doi: 10.1007/s001150050569. [DOI] [PubMed] [Google Scholar]
- 37.Jensen JE, Al-Semaan YM, Williamson PC, Neufeld RW, Menon RS, Schaeffer B, Dens-more M, Drost DJ. Region-specific changes in phospholipid metabolism in chronic, medicated schizophrenia: 31P-MRS study at 4.0 Tesla. Br J Psychiatry. 2002;180:39–44. doi: 10.1192/bjp.180.1.39. [DOI] [PubMed] [Google Scholar]
- 38.Ramadan NM, Halvorson H, Vande-Linde A, Levine SR, Helpern JA, Welch KM. Low brain magnesium in migraine. Headache. 1989;29(9):590–593. doi: 10.1111/j.1526-4610.1989.hed2909590.x. [DOI] [PubMed] [Google Scholar]
- 39.Altura BM, Altura BT. Role of magnesium and calcium in alcohol-induced hypertension and strokes as probed by in vivo television microscopy, digital image microscopy, optical spectroscopy, 31P-NMR, spectroscopy and a unique magnesium ion-selective electrode. Alcohol Clin Exp Res. 1994;18(5):1057–1068. doi: 10.1111/j.1530-0277.1994.tb00082.x. [DOI] [PubMed] [Google Scholar]
- 40.Nioka S, Zaman A, Yoshioka H, Masumura M, Miyake H, Lockard S, Chance B. 31P magnetic resonance spectroscopy study of cerebral metabolism in developing dog brain and its relationship to neuronal function. Dev Neurosci. 1991;13(2):61–68. doi: 10.1159/000112142. [DOI] [PubMed] [Google Scholar]
- 41.Nioka S, Smith DS, Mayevsky A, Dobson GP, Veech RL, Subramanian H, Chance B. Age dependence of steady state mitochondrial oxidative metabolism in the in vivo hypoxic dog brain. Neurol Res. 1991;13(1):25–32. doi: 10.1080/01616412.1991.11739961. [DOI] [PubMed] [Google Scholar]
- 42.Chance B, Leigh JS, Jr, Nioka S, Sinwell T, Younkin D, Smith DS. An approach to the problem of metabolic heterogeneity in brain: Ischemia and reflow after ischemia. Ann N Y Acad Sci. 1987;508:309–320. doi: 10.1111/j.1749-6632.1987.tb32913.x. [DOI] [PubMed] [Google Scholar]
- 43.Frosen S, Hoffman RA. Study of moderately rapid chemical exchange by means of nuclear magnetic double resonance. J Chem Phys. 1963;39:2892–2901. [Google Scholar]
- 44.Alger JR, Shulman RG. NMR Studies of enzymatic rates in vitro and in vivo by magnetization transfer. Quart Rev Biophys. 1984;17:83–124. doi: 10.1017/s0033583500005266. [DOI] [PubMed] [Google Scholar]
- 45.Ugurbil K. Magnetization transfer measurements of individual rate constants in the presence of multiple reactions. J Magn Reson. 1985;64:207–219. [Google Scholar]
- 46.Ugurbil K. Magnetization transfer measurements of creatine kinase and ATPase rates in intact hearts. Circulation. 1985;72:IV94–IV96. [PubMed] [Google Scholar]
- 47.Degani H, Laughlin M, Campbell S, Shulman RG. Kinetics of creatine kinase in heart: a 31P NMR saturation- and inversion-transfer study. Biochemistry. 1985;24(20):5510–5516. doi: 10.1021/bi00341a035. [DOI] [PubMed] [Google Scholar]
- 48.Bottomley PA, Ouwerkerk R, Lee RF, Weiss RG. Four-angle saturation transfer (FAST) method for measuring creatine kinase reaction rates in vivo. Magn Reson Med. 2002;47(5):850–863. doi: 10.1002/mrm.10130. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49.Shoubridge EA, Briggs RW, Radda GK. 31P NMR saturation transfer measurements of the steady state rates of creatine kinase and ATP synthetase in the rat brain. FEBS Lett. 1982;140(2):289–292. doi: 10.1016/0014-5793(82)80916-2. [DOI] [PubMed] [Google Scholar]
- 50.Du F, Zhu XH, Qiao H, Zhang X, Chen W. Efficient in vivo 31P magnetization transfer approach for noninvasively determining multiple kinetic parameters and metabolic fluxes of ATP metabolism in the human brain. Magn Reson Med. 2006;57(1):103–114. doi: 10.1002/mrm.21107. [DOI] [PubMed] [Google Scholar]
- 51.Mateescu GD, Yvars GM, Dular T. Water, Ions and O-17 Magnetic Resonance Imaging. In: Lauger P, Packer L, Vasilescu V, editors. Water and Ions in Biological Systems. Basel-Boston-Berlin: Birkhauser Verlag; 1988. pp. 239–250. [Google Scholar]
- 52.Pekar J, Ligeti L, Ruttner Z, Lyon RC, Sinnwell TM, van Gelderen P, Fiat D, Moonen CT, McLaughlin AC. In vivo measurement of cerebral oxygen consumption and blood flow using 17O magnetic resonance imaging. Magn Reson Med. 1991;21(2):313–319. doi: 10.1002/mrm.1910210217. [DOI] [PubMed] [Google Scholar]
- 53.Fiat D, Ligeti L, Lyon RC, Ruttner Z, Pekar J, Moonen CT, McLaughlin AC. In vivo 17O NMR study of rat brain during 17O2 inhalation. Magn Reson Med. 1992;24(2):370–374. doi: 10.1002/mrm.1910240218. [DOI] [PubMed] [Google Scholar]
- 54.Zhu XH, Zhang Y, Tian RX, Lei H, Zhang N, Zhang X, Merkle H, Ugurbil K, Chen W. Development of 17O NMR approach for fast imaging of cerebral metabolic rate of oxygen in rat brain at high field. Proc Natl Acad Sci U S A. 2002;99(20):13194–13199. doi: 10.1073/pnas.202471399. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 55.Zhu XH, Zhang N, Zhang Y, Zhang X, Ugurbil K, Chen W. In vivo 17O NMR approaches for brain study at high field. NMR Biomed. 2005;18(2):83–103. doi: 10.1002/nbm.930. [DOI] [PubMed] [Google Scholar]
- 56.Ernst RR, Bodenhausen G, Wokaun A. Principles of Nuclear Magnetic Resonance in One and Two Dimensions. New York: Oxford University Press; 1991. [Google Scholar]
- 57.Hoult DI, Richards RE. The signal-to-noise ratio of nuclear magnetic resonance experiment. J Magn Reson. 1976;24:71–85. doi: 10.1016/j.jmr.2011.09.018. [DOI] [PubMed] [Google Scholar]
- 58.Wen H, Chesnick AS, Balaban RS. The design and test of a new volume coil for high field imaging. Magn Reson Med. 1994;32:492–498. doi: 10.1002/mrm.1910320411. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 59.Wang Z, Wang DJ, Noyszewski EA, Bogdan AR, Haselgrove JC, Reddy R, Zimmerman RA, Leigh JS. Sensitivity of in vivo MRS of the N-δ proton in proximal histidine of deoxymyoglobin. Magn Reson Med. 1992;27(2):362–367. doi: 10.1002/mrm.1910270217. [DOI] [PubMed] [Google Scholar]
- 60.Qiao H, Zhang X, Zhu XH, Du F, Chen W. In vivo 31P MRS of human brain at high/ultrahigh fields: A quantitative comparison of NMR detection sensitivity and spectral resolution between 4 T and 7 T. Magn Reson Imaging. 2006;24(10):1281–1286. doi: 10.1016/j.mri.2006.08.002. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 61.Boska MD, Hubesch B, Meyerhoff DJ, Twieg DB, Karczmar GS, Matson GB, Weiner MW. Comparison of 31P MRS and 1H MRI at 1.5 and 2.0 T. Magn Reson Med. 1990;13(2):228–238. doi: 10.1002/mrm.1910130206. [DOI] [PubMed] [Google Scholar]
- 62.Hardy CJ, Bottomley PA, Roemer PB, Redington RW. Rapid 31P spectroscopy on a 4-T whole-body system. Magn Reson Med. 1988;8(1):104–109. doi: 10.1002/mrm.1910080113. [DOI] [PubMed] [Google Scholar]
- 63.Hetherington HP, Spencer DD, Vaughan JT, Pan JW. Quantitative 31P spectroscopic imaging of human brain at 4 Tesla: assessment of gray and white matter differences of phosphocreatine and ATP. Magn Reson Med. 2001;45:46–52. doi: 10.1002/1522-2594(200101)45:1<46::aid-mrm1008>3.0.co;2-n. [DOI] [PubMed] [Google Scholar]
- 64.Evelhoch JL, Ewy CS, Siegfried BA, Ackerman JJ, Rice DW, Briggs RW. 31P spin-lattice relaxation times and resonance linewidths of rat tissue in vivo: Dependence upon the static magnetic field strength. Magn Reson Med. 1985;2(4):410–417. doi: 10.1002/mrm.1910020409. [DOI] [PubMed] [Google Scholar]
- 65.Mathur-De Vre R, Maerschalk C, Delporte C. Spin-lattice relaxation times and nuclear Overhauser enhancement effect for 31P metabolites in model solutions at two frequencies: Implications for in vivo spectroscopy. Magn Reson Imaging. 1990;8(6):691–698. doi: 10.1016/0730-725x(90)90003-k. [DOI] [PubMed] [Google Scholar]
- 66.Abragam A. In: The Principles of Nuclear Magnetism. Mott NF, Bullard EC, Wilkinson DH, editors. London: Oxford University Press; 1961. [Google Scholar]
- 67.Zhu XH, Merkle H, Kwag JH, Ugurbil K, Chen W. 17O relaxation time and NMR sensitivity of cerebral water and their field dependence. Magn Reson Med. 2001;45(4):543–549. doi: 10.1002/mrm.1073. [DOI] [PubMed] [Google Scholar]
- 68.Thelwall PE, Blackband SJ, Chen W. Proc Intl Soc Mag Reson Med (ISMRM) Toronto: 2003. Field dependence of 17O T1, T2 and SNR – in vitro and in vivo studies at 4.7, 11 and 17.6 Tesla; p. 504. [Google Scholar]
- 69.Mateescu GD, Yvars G, Pazara DI, Alldridge NA, LaManna JC, Lust DW, Mattingly M, Kuhn W. 17O-1H magnetic resonance imaging in plants, animals, and materials. In: Baillie TA, Jones JR, editors. Synthesis and Application of Isotopically Labeled Compounds. Amsterdam: Elsevier; 1989. pp. 499–508. [Google Scholar]
- 70.Hopkins AL, Barr RG. Oxygen-17 compounds as potential NMR T2 contrast agents: Enrichment effects of H2 17O on protein solutions and living tissues. Magn Reson Med. 1987;4(4):399–403. doi: 10.1002/mrm.1910040413. [DOI] [PubMed] [Google Scholar]
- 71.Kwong KK, Hopkins AL, Belliveau JW, Chesler DA, Porkka LM, McKinstry RC, Finelli DA, Hunter GJ, Moore JB, Barr RG, Rosen BR. Proton NMR imaging of cerebral blood flow using H2 17O. Magn Reson Med. 1991;22(1):154–158. doi: 10.1002/mrm.1910220116. [DOI] [PubMed] [Google Scholar]
- 72.Arai T, Nakao S, Mori K, Ishimori K, Morishima I, Miyazawa T, Fritz-Zieroth B. Cerebral oxygen utilization analyzed by the use of oxygen-17 and its nuclear magnetic resonance. Biochem Biophys Res Commun. 1990;169(1):153–158. doi: 10.1016/0006-291x(90)91447-z. [DOI] [PubMed] [Google Scholar]
- 73.Pekar J, Sinnwell T, Ligeti L, Chesnick AS, Frank JA, McLaughlin AC. Simultaneous measurement of cerebral oxygen consumption and blood flow using 17O and 19F magnetic resonance imaging. J Cereb Blood Flow Metab. 1995;15(2):312–320. doi: 10.1038/jcbfm.1995.36. [DOI] [PubMed] [Google Scholar]
- 74.Ronen I, Navon G. A new method for proton detection of H2 17O with potential applications for functional MRI. Magn Reson Med. 1994;32(6):789–793. doi: 10.1002/mrm.1910320616. [DOI] [PubMed] [Google Scholar]
- 75.Ronen I, Merkle H, Ugurbil K, Navon G. Imaging of H2 17 O distribution in the brain of a live rat by using proton-detected 17O MRI. Proc Natl Acad Sci U S A. 1998;95(22):12934–12939. doi: 10.1073/pnas.95.22.12934. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 76.Reddy R, Stolpen AH, Leigh JS. Detection of 17O by proton T1 rho dispersion imaging. J Magn Reson B. 1995;108(3):276–279. doi: 10.1006/jmrb.1995.1133. [DOI] [PubMed] [Google Scholar]
- 77.Chen W, Zhu XH, Ugurbil K. Imaging cerebral metabolic rate of oxygen consumption (CMRO2) using 17O NMR approach at ultra-high field. In: Shulman RG, Rothman DL, editors. Brain Energetics and Neuronal Activity. New York: John Wiley & Sons Ltd; 2004. pp. 125–146. [Google Scholar]
- 78.Arai T, Mori K, Nakao S, Watanabe K, Kito K, Aoki M, Mori H, Morikawa S, Inubushi T. In vivo oxygen-17 nuclear magnetic resonance for the estimation of cerebral blood flow and oxygen consumption. Biochem Biophys Res Commun. 1991;179(2):954–961. doi: 10.1016/0006-291x(91)91911-u. [DOI] [PubMed] [Google Scholar]
- 79.Fiat D, Kang S. Determination of the rate of cerebral oxygen consumption and regional cerebral blood flow by non-invasive 17O in vivo NMR spectroscopy and magnetic resonance imaging: Part 1. Theory and data analysis methods. Neurol Res. 1992;14(4):303–311. doi: 10.1080/01616412.1992.11740074. [DOI] [PubMed] [Google Scholar]
- 80.Fiat D, Dolinsek J, Hankiewicz J, Dujovny M, Ausman J. Determination of regional cerebral oxygen consumption in the human: 17O natural abundance cerebral magnetic resonance imaging and spectroscopy in a whole body system. Neurol Res. 1993;15(4):237–248. doi: 10.1080/01616412.1993.11740143. [DOI] [PubMed] [Google Scholar]
- 81.Fiat D, Kang S. Determination of the rate of cerebral oxygen consumption and regional cerebral blood flow by non-invasive 17O in vivo NMR spectroscopy and magnetic resonance imaging. Part 2. Determination of CMRO2 for the rat by 17O NMR, and CMRO2, rCBF and the partition coefficient for the cat by 17O MRI. Neurol Res. 1993;15(1):7–22. doi: 10.1080/01616412.1993.11740100. [DOI] [PubMed] [Google Scholar]
- 82.Mateescu GD. Functional oxygen-17 magnetic resonance imaging and localized spectroscopy. Adv Exp Med Biol. 2003;510:213–218. doi: 10.1007/978-1-4615-0205-0_35. [DOI] [PubMed] [Google Scholar]
- 83.Stolpen AH, Reddy R, Leigh JS. 17O-decoupled proton MR spectroscopy and imaging in a tissue model. J Magn Reson. 1997;125(1):1–7. doi: 10.1006/jmre.1996.1071. [DOI] [PubMed] [Google Scholar]
- 84.Reddy R, Stolpen AH, Charagundla SR, Insko EK, Leigh JS. 17O-decoupled 1H detection using a double-tuned coil. Magn Reson Imaging. 1996;14(9):1073–1078. doi: 10.1016/s0730-725x(96)00227-5. [DOI] [PubMed] [Google Scholar]
- 85.de Crespigny AJ, D’Arceuil HE, Engel-horn T, Moseley ME. MRI of focal cerebral ischemia using 17O-labeled water. Magn Reson Med. 2000;43(6):876–883. doi: 10.1002/1522-2594(200006)43:6<876::aid-mrm14>3.0.co;2-y. [DOI] [PubMed] [Google Scholar]
- 86.Zhu XH, Zhang Y, Zhang N, Ugurbil K, Chen W. Noninvasive and three-dimensional imaging of CMRO2 in rats at 9.4 T: Reproducibility test and normothermia/hypothermia comparison study. J Cereb Blood Flow Metab. 2007;27(6):1225–1234. doi: 10.1038/sj.jcbfm.9600421. [DOI] [PubMed] [Google Scholar]
- 87.Zhang N, Zhu XH, Lei H, Ugurbil K, Chen W. Simplified methods for calculating cerebral metabolic rate of oxygen based on 17O magnetic resonance spectroscopic imaging measurement during a short 17O2 inhalation. J Cereb Blood Flow Metab. 2004;24(8):840–848. doi: 10.1097/01.WCB.0000125885.54676.82. [DOI] [PubMed] [Google Scholar]
- 88.Ter-Pogossian MM, Eichling JO, Davis DO, Welch MJ. The measure in vivo of regional cerebral oxygen utilization by means of oxyhemoglobin labeled with radioactive oxygen-15. J Clin Invest. 1970;49:381–391. doi: 10.1172/JCI106247. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 89.Lenzi GL, Jones T, Frackowiak RS. Positron emission tomography: state of the art in neurology. Prog Nucl Med. 1981;7:118–137. [PubMed] [Google Scholar]
- 90.Mintun MA, Raichle ME, Martin WR, Herscovitch P. Brain oxygen utilization measured with O-15 radiotracers and positron emission tomography. J Nucl Med. 1984;25(2):177–187. [PubMed] [Google Scholar]
- 91.Fox PT, Raichle ME, Mintun MA, Dence C. Nonoxidative glucose consumption during focal physiologic neural activity. Science. 1988;241:462–464. doi: 10.1126/science.3260686. [DOI] [PubMed] [Google Scholar]
- 92.Kety SS, Schmidt CF. The determination of cerebral blood flow in man by the use of nitrous oxide in low concentrations. Am J Physiol. 1945;143:53–66. [Google Scholar]
- 93.Kety SS, Schmidt CF. Nitrous oxide method for the quantitative determination of cerebral blood flow in man: theory, procedure and normal values. J Clin Invest. 1948;27:476–483. doi: 10.1172/JCI101994. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 94.Kety SS, Schmidt CF. Effects of altered arterial tensions of carbon dioxide and oxygen on cerebral blood flow and cerebral oxygen consumption of normal young men. J Clin Invest. 1948;27:484–492. doi: 10.1172/JCI101995. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 95.Herscovitch A, Raichle ME. What is the correct value for the brain-blood partition coefficient for water? J Cereb Blood Flow Metab. 1985;5:65–69. doi: 10.1038/jcbfm.1985.9. [DOI] [PubMed] [Google Scholar]
- 96.Herscovitch P, Raichle ME, Kilbourn MR, Welch MJ. Positron emission tomographic measurement of cerebral blood flow and permeability-surface area product of water using [15O]water and [11C]butanol. J Cereb Blood Flow Metab. 1987;7(5):527–542. doi: 10.1038/jcbfm.1987.102. [DOI] [PubMed] [Google Scholar]
- 97.Zhang X, Zhu XH, Tian R, Zhang Y, Merkle H, Chen W. Measurement of arterial input function of 17O water tracer in rat carotid artery by using a region-defined (REDE) implanted vascular RF coil. Magma. 2003;16(2):77–85. doi: 10.1007/s10334-003-0013-9. [DOI] [PubMed] [Google Scholar]
- 98.Nakao Y, Itoh Y, Kuang TY, Cook M, Jehle J, Sokoloff L. Effects of anesthesia on functional activation of cerebral blood flow and metabolism. Proc Natl Acad Sci U S A. 2001;98(13):7593–7598. doi: 10.1073/pnas.121179898. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 99.Hyder F, Kennan RP, Kida I, Mason GF, Behar KL, Rothman D. Dependence of oxygen delivery on blood flow in rat brain: A 7 tesla nuclear magnetic resonance study. J Cereb Blood Flow Metab. 2000;20(3):485–498. doi: 10.1097/00004647-200003000-00007. [DOI] [PubMed] [Google Scholar]
- 100.Yee SH, Lee K, Jerabek PA, Fox PT. Quantitative measurement of oxygen metabolic rate in the rat brain using microPET imaging of briefly inhaled 15O-labelled oxygen gas. Nucl Med Commun. 2006;27(7):573–581. doi: 10.1097/01.mnm.0000220586.02591.fd. [DOI] [PubMed] [Google Scholar]
- 101.Zhang NY, Zhu XH, Chen W. Proc Intl Soc Mag Reson Med (ISMRM) Seattle: 2006. Evaluation of the simplified method for mapping cerebral metabolic rate of oxygen based on 17O NMR approach; p. 952. [Google Scholar]
- 102.Erecinska M, Thoresen M, Silver IA. Effects of hypothermia on energy metabolism in Mammalian central nervous system. J Cereb Blood Flow Metab. 2003;23(5):513–530. doi: 10.1097/01.WCB.0000066287.21705.21. [DOI] [PubMed] [Google Scholar]
- 103.Weiss RG, Gerstenblith G, Bottomley PA. ATP flux through creatine kinase in the normal, stressed, and failing human heart. Proc Natl Acad Sci U S A. 2005;102(3):808–813. doi: 10.1073/pnas.0408962102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 104.Balaban RS, Kantor HL, Ferretti JA. In vivo flux between phosphocreatine and adenosine triphosphate determined by two-dimensional phosphorous NMR. J Biol Chem. 1983;258(21):12787–12789. [PubMed] [Google Scholar]
- 105.Degani H, Alger JR, Shulman RG, Petroff OA, Prichard JW. 31P magnetization transfer studies of creatine kinase kinetics in living rabbit brain. Magn Reson Med. 1987;5(1):1–12. doi: 10.1002/mrm.1910050102. [DOI] [PubMed] [Google Scholar]
- 106.Bottomley PA, Hardy CJ. Mapping creatine kinase reaction rates in human brain and heart with 4 Tesla saturation transfer 31P NMR. J Magn Reson. 1992;99:443–448. [Google Scholar]
- 107.Sauter A, Rudin M. Determination of creatine kinase parameters in rat brain by NMR magnetization transfer. J Biological Chem. 1993;268:13166–13171. [PubMed] [Google Scholar]
- 108.Chen W, Zhu X-H, Adriany G, Ugurbil K. Increase of creatine kinase activity in the visual cortex of human brain during visual stimulation: A 31P NMR magnetization transfer study. Magn Reson Med. 1997;38:551–557. doi: 10.1002/mrm.1910380408. [DOI] [PubMed] [Google Scholar]
- 109.Braunova Z, Kasparova S, Mlynarik V, Mierisova S, Liptaj T, Tkac I, Gvozdjakova A. Metabolic changes in rat brain after prolonged ethanol consumption measured by 1H and 31P MRS experiments. Cell Mol Neurobiol. 2000;20(6):703–715. doi: 10.1023/A:1007002925592. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 110.Mlynarik v ZS, Brehm A, Bischof M, Roden M. An optimized protocol for measuring rate constant of creatine kinase reaction in human brain by 31P NMR saturation transfer. 13th Proc Intl Soc Mag Reson Med. 2005:2767. [Google Scholar]
- 111.Joubert F, Mateo P, Gillet B, Beloeil JC, Mazet JL, Hoerter JA. CK flux or direct ATP transfer: Versatility of energy transfer pathways evidenced by NMR in the perfused heart. Mol Cell Biochem. 2004;256–257(1–2):43–58. doi: 10.1023/b:mcbi.0000009858.41434.fc. [DOI] [PubMed] [Google Scholar]
- 112.Matthews PM, Bland JL, Gadian DG, Radda GK. A 31P-NMR saturation transfer study of the regulation of creatine kinase in the rat heart. Biochim Biophys Acta. 1982;721(3):312–320. doi: 10.1016/0167-4889(82)90084-2. [DOI] [PubMed] [Google Scholar]
- 113.Mora BN, Narasimhan PT, Ross BD. 31P magnetization transfer studies in the monkey brain. Magn Reson Med. 1992;26(1):100–115. doi: 10.1002/mrm.1910260111. [DOI] [PubMed] [Google Scholar]
- 114.Bittl JA, DeLayre J, Ingwall JS. Rate equation for creatine kinase predicts the in vivo reaction velocity: 31P NMR surface coil studies in brain, heart, and skeletal muscle of the living rat. Biochemistry. 1987;26:6083–6090. doi: 10.1021/bi00393a021. [DOI] [PubMed] [Google Scholar]
- 115.Ugurbil K, Petein M, Maiden R, Michurski S, From A. Measurement of an individual rate constant in the presence of multiple exchanges: Application to myocardial creatine kinase rates. Biochemistry. 1986;25:100–108. doi: 10.1021/bi00349a015. [DOI] [PubMed] [Google Scholar]
- 116.Spencer RG, Balschi JA, Leigh JS, Jr, Ingwall JS. ATP synthesis and degradation rates in the perfused rat heart. 31P-nuclear magnetic resonance double saturation transfer measurements. Biophys J. 1988;54(5):921–929. doi: 10.1016/S0006-3495(88)83028-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 117.Joubert F, Mazet JL, Mateo P, Hoerter JA. 31P NMR detection of subcellular creatine kinase fluxes in the perfused rat heart: Contractility modifies energy transfer pathways. J Biol Chem. 2002;277(21):18469–18476. doi: 10.1074/jbc.M200792200. [DOI] [PubMed] [Google Scholar]
- 118.Mitsumori F, Rees D, Brindle KM, Radda GK, Campbell ID. 31P-NMR saturation transfer studies of aerobic Escherichia coli cells. Biochimica et Biophysica Acta. 1988;969(2):185–193. doi: 10.1016/0167-4889(88)90074-2. [DOI] [PubMed] [Google Scholar]
- 119.Campbell SL, Jones KA, Shulman RG. 31P NMR saturation-transfer measurements in Saccharomyces cerevisiae: Characterization of phosphate exchange reactions by iodoacetate and antimycin A inhibition. Biochemistry. 1987;26(23):7483–7492. doi: 10.1021/bi00397a043. [DOI] [PubMed] [Google Scholar]
- 120.Thoma WJ, Ugurbil K. Saturation-transfer studies of ATP-Pi exchange in isolated perfused rat liver. Biochimica et Biophysica Acta. 1987;893(2):225–231. doi: 10.1016/0005-2728(87)90043-0. [DOI] [PubMed] [Google Scholar]
- 121.Kingsley-Hickman PB, Sako EY, Mohanakrishnan P, Robitaille PM, From AH, Foker JE, Ugurbil K. 31P NMR studies of ATP synthesis and hydrolysis kinetics in the intact myocardium. Biochemistry. 1987;26(23):7501–7510. doi: 10.1021/bi00397a045. [DOI] [PubMed] [Google Scholar]
- 122.Hinkle PC. P/O ratios of mitochondrial oxidative phosphorylation. Biochim Biophys Acta. 2005;1706(1–2):1–11. doi: 10.1016/j.bbabio.2004.09.004. [DOI] [PubMed] [Google Scholar]
- 123.Du F, Zhang Y, Friedman M, Zhu XH, Ugurbil K, Chen W. Proc Intl Soc Mag Reson Med (ISMRM) Seattle: 2006. Study of correlation between brain activity and ATP metabolic rates by means of 31P magnetization transfer and electroencephalograph measurement; p. 2125. [Google Scholar]
- 124.Fox PT, Raichle ME. Focal physiological uncoupling of cerebral blood flow and oxidative metabolism during somatosensory stimulation in human subjects. Proc Natl Acad Sci USA. 1986;83:1140–1144. doi: 10.1073/pnas.83.4.1140. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 125.Ribeiro L, Kuwabara H, Meyer E, Fujita H, Marrett S, Evans A, Gjedde A. Cerebral blood flow and metabolism during nonspecific bilateral visual stimulation in normal subjects. In: Uemura K, editor. Quantification of Brain Function in Tracer Kinetics and Image Analysis in Brain PET. New York: Elsevier Science; 1993. pp. 229–236. [Google Scholar]
- 126.Roland PE, Ericksson L, Stone-Elander S, Widen L. Does mental activity change the oxidative metabolism of the brain? J Neurosci. 1987;7:2373–2389. [PMC free article] [PubMed] [Google Scholar]
- 127.Marrett S, Fujita H, Meyer E, Ribeiro L, Evans A, Kuwabara H, Gjedde A. Stimulus specific increase of oxidative metabolism in human visual cortex. In: Uemura K, editor. Quantification of Brain Function in Tracer Kinetics and Image Analysis in Brain PET. New York: Elsevier Science; 1993. pp. 217–228. [Google Scholar]
- 128.Prichard J, Rothman D, Novotny E, Petroff O, Kuwabara T, Avison M, Howseman A, Hanstock C, Shulman RG. Lactate rise detected by 1H NMR in human visual cortex during physiologic stimulation. Proc Natl Acad Sci (USA) 1992;88:5829–5831. doi: 10.1073/pnas.88.13.5829. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 129.Shulman RG, Hyder F, Rothman DL. Cerebral energetics and the glycogen shunt: neurochemical basis of functional imaging. Proc Natl Acad Sci U S A. 2001;98(11):6417–6422. doi: 10.1073/pnas.101129298. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 130.Vafaee MS, Meyer E, Marrett S, Paus T, Evans AC, Gjedde A. Frequency-dependent changes in cerebral metabolic rate of oxygen during activation of human visual cortex. J Cereb Blood Flow Metab. 1999;19(3):272–277. doi: 10.1097/00004647-199903000-00005. [DOI] [PubMed] [Google Scholar]
- 131.Davis TL, Kwong KK, Weisskoff RM, Rosen BR. Calibrated functional MRI: Mapping the dynamic of oxidative metabolism. Proc Natl Acad Sci USA. 1998;95:1834–1839. doi: 10.1073/pnas.95.4.1834. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 132.Hoge RD, Atkinson J, Gill B, Crelier GR, Marrett S, Pike GB. Investigation of BOLD signal dependence on cerebral blood flow and oxygen consumption: The deoxyhemoglobin dilution model [In Process Citation] Magn Reson Med. 1999;42(5):849–863. doi: 10.1002/(sici)1522-2594(199911)42:5<849::aid-mrm4>3.0.co;2-z. [DOI] [PubMed] [Google Scholar]
- 133.Hyder F, Chase JR, Behar KL, Mason GF, Siddeek M, Rothman DL, Shulman RG. Increase tricarboxylic acid cycle flux in rat brain during forepaw stimulation detected with 1H-{13C} NMR. Proc Natl Acad Sci USA. 1996;93:7612–7617. doi: 10.1073/pnas.93.15.7612. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 134.Kim SG, Rostrup E, Larsson HB, Ogawa S, Paulson OB. Determination of relative CMRO2 from CBF and BOLD changes: Significant increase of oxygen consumption rate during visual stimulation. Magn Reson Med. 1999;41(6):1152–1161. doi: 10.1002/(sici)1522-2594(199906)41:6<1152::aid-mrm11>3.0.co;2-t. [DOI] [PubMed] [Google Scholar]
- 135.Chen W, Zhu XH, Gruetter R, Seaquist ER, Ugurbil K. Study of oxygen utilization changes of human visual cortex during hemifield stimulation using 1H-{13C} MRS and fMRI. Magn Reson Med. 2001;45:349–355. doi: 10.1002/1522-2594(200103)45:3<349::aid-mrm1045>3.0.co;2-8. [DOI] [PubMed] [Google Scholar]
- 136.Kasischke KA, Vishwasrao HD, Fisher PJ, Zipfel WR, Webb WW. Neural activity triggers neuronal oxidative metabolism followed by astrocytic glycolysis. Science. 2004;305(5680):99–103. doi: 10.1126/science.1096485. [DOI] [PubMed] [Google Scholar]
- 137.Ogawa S, Lee T-M, Kay AR, Tank DW. Brain magnetic resonance imaging with contrast dependent on blood oxygenation. Proc Natl Acad Sci USA. 1990;87:9868–9872. doi: 10.1073/pnas.87.24.9868. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 138.Ogawa S, Tank DW, Menon R, Ellermann JM, Kim S-G, Merkle H, Ugurbil K. Intrinsic signal changes accompanying sensory stimulation: Functional brain mapping with magnetic resonance imaging. Proc Natl Acad Sci USA. 1992;89:5951–5955. doi: 10.1073/pnas.89.13.5951. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 139.Kwong KK, Belliveau JW, Chesler DA, Goldberg IE, Weisskoff RM, Poncelet BP, Kennedy DN, Hoppel BE, Cohen MS, Turner R, Cheng HM, Brady TJ, Rosen BR. Dynamic magnetic resonance imaging of human brain activity during primary sensory stimulation. Proc Natl Acad Sci USA. 1992;89:5675–5679. doi: 10.1073/pnas.89.12.5675. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 140.Bandettini PA, Wong EC, Hinks RS, Tikofsky RS, Hyde JS. Time course EPI of human brain function during task activation. Magn Reson Med. 1992;25:390–397. doi: 10.1002/mrm.1910250220. [DOI] [PubMed] [Google Scholar]
- 141.Frahm J, Bruhn H, Merboldt KD, Han-icke W. Dynamic MR imaging of human brain oxygenation during rest and photic stimulation. J Magn Reson Imag. 1992;2(5):501–505. doi: 10.1002/jmri.1880020505. [DOI] [PubMed] [Google Scholar]
- 142.Blamire AM, Ogawa S, Ugurbil K, Rothman D, McCarthy G, Ellermann JM, Hyder F, Rattner Z, Shulman RG. Dynamic mapping of the human visual cortex by high-speed magnetic resonance imaging. Proc Natl Acad Sci USA. 1992;89:11069–11073. doi: 10.1073/pnas.89.22.11069. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 143.Barinaga M. What makes brain neurons run. Science. 1997;276:196–198. doi: 10.1126/science.276.5310.196. [DOI] [PubMed] [Google Scholar]
- 144.Zhu XH, Zhang Y, Zhang NY, Zhang XL, Ugurbil K, Chen W. Proc Intl Soc Mag Reson Med (ISMRM) Seattle: 2006. Direct imaging of CMRO2 in cat visual cortex at rest and visual stimulation; p. 457. [Google Scholar]
- 145.Lei H, Zhu XH, Li YX, Ugurbil K, Chen W. Proc Intl Soc Mag Reson Med (ISMRM) Kyoto, Japan: 2004. Changes of cerebral metabolism in human primary visual cortex during functional activation observed by in vivo 31P MRS at 7T; p. 2361. [Google Scholar]
- 146.Maurer I, Zierz S, Moller H. Evidence for a mitochondrial oxidative phosphorylation defect in brains from patients with schizophrenia. Schizophr Res. 2001;48(1):125–136. doi: 10.1016/s0920-9964(00)00075-x. [DOI] [PubMed] [Google Scholar]
- 147.Wong-Riley M, Antuono P, Ho KC, Egan R, Hevner R, Liebl W, Huang Z, Rachel R, Jones J. Cytochrome oxidase in Alzheimer’s disease: Biochemical, histochemical, and immunohistochemical analyses of the visual and other systems. Vision Res. 1997;37(24):3593–3608. doi: 10.1016/S0042-6989(96)00210-6. [DOI] [PubMed] [Google Scholar]
- 148.Maurer I, Zierz S, Moller HJ. A selective defect of cytochrome c oxidase is present in brain of Alzheimer disease patients. Neurobiol Aging. 2000;21(3):455–462. doi: 10.1016/s0197-4580(00)00112-3. [DOI] [PubMed] [Google Scholar]
- 149.Frackowiak RS, Herold S, Petty RK, Morgan-Hughes JA. The cerebral metabolism of glucose and oxygen measured with positron tomography in patients with mitochondrial diseases. Brain. 1988;111(Pt 5):1009–1024. doi: 10.1093/brain/111.5.1009. [DOI] [PubMed] [Google Scholar]
- 150.Beal MF. Does impairment of energy metabolism result in excitotoxic neuronal death in neurodegenerative illnesses? Ann Neurol. 1992;31(2):119–130. doi: 10.1002/ana.410310202. [DOI] [PubMed] [Google Scholar]
- 151.Wallace DC. Mitochondrial genetics: A paradigm for aging and degenerative diseases? Science. 1992;256(5057):628–632. doi: 10.1126/science.1533953. [DOI] [PubMed] [Google Scholar]
- 152.Wallace DC. Mitochondrial diseases in man and mouse. Science. 1999;283(5407):1482–1488. doi: 10.1126/science.283.5407.1482. [DOI] [PubMed] [Google Scholar]
- 153.Ugurbil K, Adriany G, Akgün C, Andersen P, Chen W, Garwood M, Gruetter R, Henry P-G, Marjanska M, Moeller S, Van de Moortele P-F, Prüssmann K, Tkac I, Vaughan JT, Wiesinger F, Yacoub E, Zhu X-H. High Magnetic Fields for Imaging Cerebral Morphology, Function and Biochemistry. In: Robitaille PMLaB LJ., editor. Biological Magnetic Resonance: Ultra High Field Magnetic Resonance Imaging Volume 26. New York: Springer; 2006. pp. 285–342. [Google Scholar]


