Electron transfer pathways in a multiheme cytochrome MtrF

Significance

Cellular respiration process in dissimilatory metal-reducing bacteria is coupled to electron transfer. In Shewanella species, a decaheme cytochrome MtrF transfers electrons to extracellular insoluble substrates, such as Fe(III) and Mn(III/IV). Using the atomic coordinates of the MtrF crystal structure and analyzing interactions with the protein environments, we calculated the redox potential (E_m) values of the 10 hemes in MtrF. The E_m profiles show how the electron transfer pathways proceed in MtrF. We showed that, when MtrF is reduced, the direction of the ET pathway switches, and bound flavin becomes the terminal electron acceptor.

Abstract

In MtrF, an outer-membrane multiheme cytochrome, the 10 heme groups are arranged in heme binding domains II and IV along the pseudo-C₂ axis, forming the electron transfer (ET) pathways. Previous reports based on molecular dynamics simulations showed that the redox potential (E_m) values for the heme pairs located in symmetrical positions in domains II and IV were similar, forming bidirectional ET pathways [Breuer M, Zarzycki P, Blumberger J, Rosso KM (2012) J Am Chem Soc 134(24):9868–9871]. Here, we present the E_m values of the 10 hemes in MtrF, solving the linear Poisson–Boltzmann equation and considering the protonation states of all titratable residues and heme propionic groups. In contrast to previous studies, the E_m values indicated that the ET is more likely to be downhill from domain IV to II because of localization of acidic residues in domain IV. Reduction of hemes in MtrF lowered the E_m values, resulting in switching to alternative downhill ET pathways that extended to the flavin binding sites. These findings present an explanation of how MtrF serves as an electron donor to extracellular substrates.

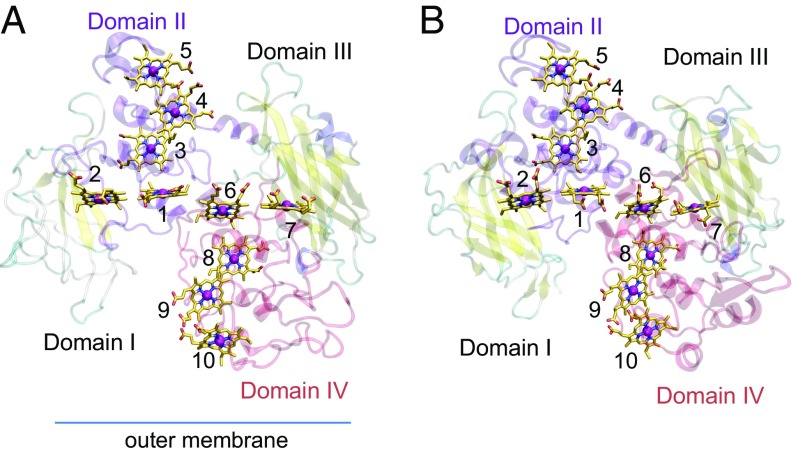
During cellular respiration in many Gram-negative bacteria, ATP synthesis on the inner membrane is coupled to electron transfer (ET). In anaerobic conditions, dissimilatory metal-reducing bacteria can use metal oxides, such as Fe(III) and Mn(III/IV), as the final electron acceptor (1). Because these metal oxides are membrane-impermeant, in Shewanella species, multiheme cytochromes transfer electrons to the metal oxides [e.g., a soluble decaheme cytochrome on the periplasmic side (MtrA), a hypothetical β-barrel porin in the transmembrane region (MtrB), and a multiheme cytochrome on the outer membrane (MtrC) (2)]. The 1.8-Å resolution crystal structure of MtrC shows that domains I and III are both β-barrel domains and structurally similar (Fig. 1) (3). These domains with the extended Greek key split-barrel structures are possible binding sites of flavin mononucleotide (FMN). The crystal structure of the extracellular decaheme cytochrome OmcA shows similar structural features (4).

Fig. 1.

Overview of multiheme cytochromes. (A) The crystal structure of MtrF (5). Hemes 2 and 7 have been proposed to be located near the FMN binding site (5, 6). The orientation of MtrF with respect to the outer membrane was proposed by Clarke et al. (5). α-Helices and β-strands are depicted as purple or red coils and yellow ribbons, respectively. (B) The crystal structure of MtrC (3).

MtrF is a homolog of MtrC as confirmed by the crystal structure of MtrF at 3.2-Å resolution (5), and domains I and III flank the heme binding domains (3, 5, 6). However, domain I contains only two β-strands, whereas domain III is a β-barrel domain (Fig. 1) (figures in ref. 5), a striking difference from the other decaheme cytochromes MtrC and OmcA. In the crystal structure of MtrF, the 10 hemes are located in domains II and IV, forming ET pathways (Fig. 1). Using the protein–protein interface server, Clarke et al. (5) proposed that heme 10 is on the periplasmic side and that heme 5 is solvent-exposed. They also proposed that the two ET pathways that are terminated by heme 2 or 7 may function in reduction of FMN at the binding site (3, 7), whereas the ET pathway that is terminated by heme 5 may be used for direct reduction of extracellular insoluble substrates [e.g., Fe(III)] (5). Insoluble substrates may also be reduced by FMN at the binding site (7, 8). In reduced MtrC, FMN showed pronounced binding affinity compared with oxidized MtrC (3). The absence of the atomic coordinates of FMN in the MtrF crystal structure (5) implies that the crystal is in the oxidized state. In contrast, in the living system, hemes are likely to be in the reduced states because of continuously supplied electrons (9), which lead to pronounced FMN binding affinity and enhance the extracellular ET (10).

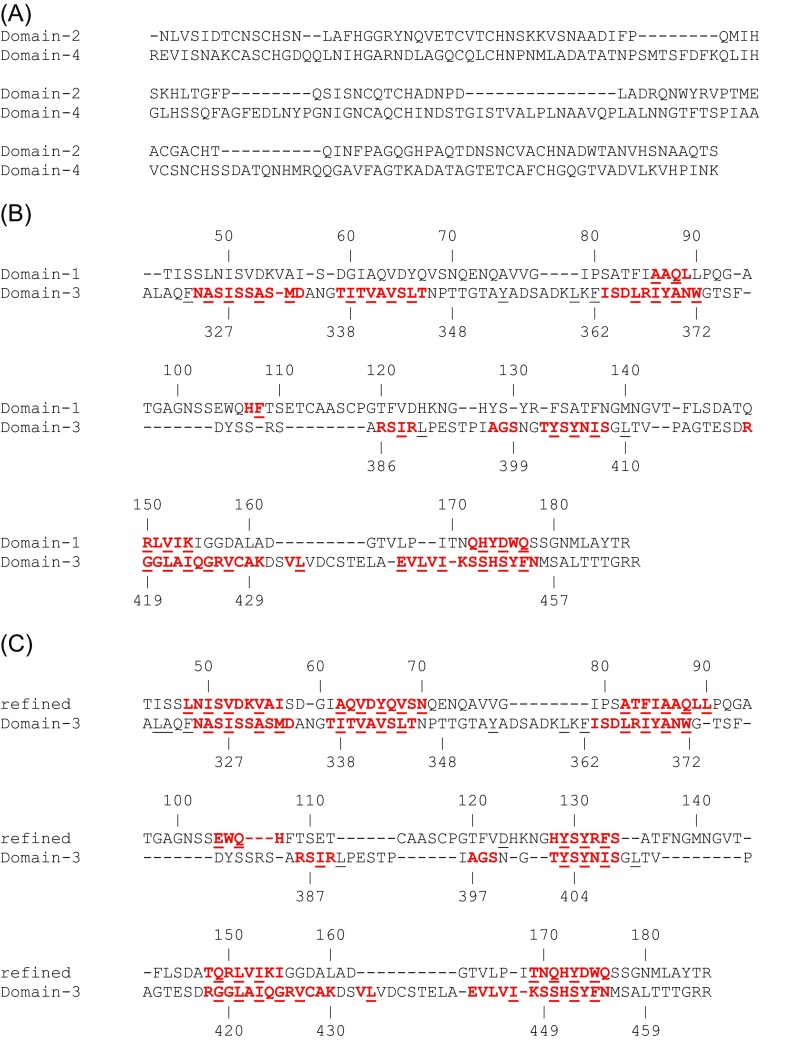
To understand the mechanism of ET in MtrF, the redox potential (E_m) values of the 10 heme groups must be determined. Although protein film voltammetry showed that the E_m values of the 10 hemes in MtrF range from −44 to −312 mV (5), specific values were not assigned to individual hemes. Breuer et al. (11) calculated the E_m values using a thermodynamic integration (TI) approach based on molecular dynamics (MD) simulations. Breuer et al. (11) uniformly added the constant C = −1,567 mV to reproduce the E_m range from −44 to −312 mV (5) reported for the hemes in MtrF by protein film voltammetry. Hemes 1 (−41 mV) and 6 (−51 mV) in the middle of the ET chain had the highest E_m values, whereas hemes 4 (−266 mV) and 9 (−279 mV) had the lowest E_m values, resulting in an ET chain energy profile that was essentially symmetrical (11). However, neither the amino acid sequences nor the locations of charged residues are highly conserved between domains II and IV (5) (Fig. S1).

Fig. S1.

Amino acid sequence alignment using ClustalW. (A) Domains II and IV in MtrF. Sequential alignments of domains I and III of (B) the MtrF crystal structure and (C) the refined structure. Residues oriented toward the inner hydrophobic core are underlined. Residues that form β-strands are shown in red. Only 17 residues in domain I (and 71 residues in domain III) form β-strands in the original MtrF crystal structure, whereas 55 residues in domain I form β-strands in the refined MtrF structure.

Here, we present the E_m values of the 10 hemes in MtrF by solving the linear Poisson–Boltzmann equation and considering the protonation states of all titratable residues and heme propionic groups, in which the protonation states change in response to the heme redox states.

Results

Structural Disorder in Domain I of the MtrF Crystal Structure.

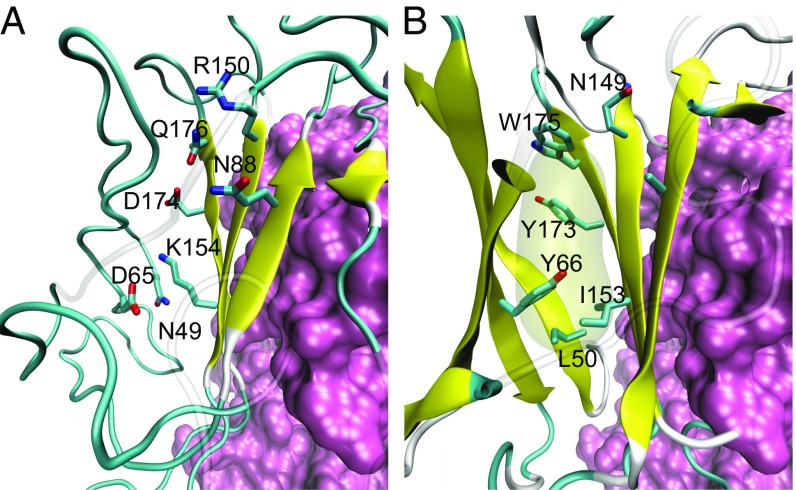
In a split-barrel environment, the nonpolar and polar residues are likely to alternate along the β-strands, with the nonpolar residues oriented inward, forming the hydrophobic core, whereas the polar residues are exposed to the bulk solvent (Fig. S2). Indeed, domain I of MtrF shows the alternating polar/nonpolar pattern as do other decaheme cytochromes. However, we found that, in domain I of the MtrF crystal structure, the hydrophobic residues are oriented toward the bulk solvent (e.g., Leu50, Tyr66, Ile153, Tyr173, and Trp175), whereas the charged and polar residues are oriented toward the protein interior (e.g., Asp65, Asn88, Arg150, Lys154, Asp174, and Gln176) (Fig. 3A and Fig. S1) (5). The MtrF crystal structure (5) shows few interstrand H bonds in the β-barrel domain, a striking difference from the crystal structures of other decaheme cytochromes [e.g., MtrC (3) and OmcA (4)]. To evaluate the structural stability of the MtrF crystal structure, MD simulations were performed before calculating the E_m for the hemes in MtrF. The MD simulations, performed using the original atomic coordinates of the MtrF crystal structure (5), suggested significant structural disorder specifically in domain I (Fig. 2).

Fig. S2.

(A) Typical alternating orientations of side chains in a β-strand and (B) hydrogen bonded pairs in the MtrC crystal structure.

Fig. 3.

Orientation of the side chains in domain I of MtrF. (A) The original MtrF crystal structure (PDB ID code 3PMQ), in which charged and polar residues are oriented toward the inner core and hydrophobic residues are exposed to the bulk (5). (B) The refined structure used for E_m calculations (solving the linear Poisson–Boltzmann equation).

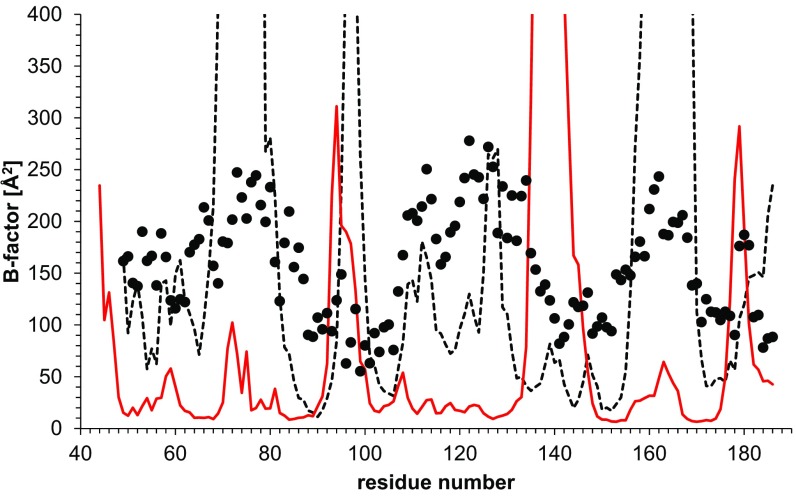
Fig. 2.

Calculated B factors of the backbone Cα atoms in domain I (residues 44–186) corresponding to the original MtrF crystal structure (black dotted line) or the (domain I replaced) refined structure (red solid line). For comparison, the B factors stated in the PDB file (PDB ID code 3PMQ) are also shown (black circles).

Next, we performed homology modeling as follows. (i) We constructed a sequential alignment with other decaheme cytochromes to reproduce the proper orientations of the polar and nonpolar residues and the location of the interstrand H bond in domain I of MtrF (Fig. S1); (ii) we determined the atomic coordinates of domain I of MtrF using domain I of the MtrC crystal structure at a resolution of 1.8 Å as a template (3). Using the resulting homology model, we conducted MD simulations for structural refinement and verification. We found that the structural disorder of domain I, specifically that of the β-strands, was significantly decreased (Fig. 2) and that the β-barrel structure of domain I was stable (Fig. 3B and Fig. S3) during MD simulations. These results suggest that domain I of MtrF is highly likely to contain a β-barrel structure as identified in the MtrC crystal structure (Fig. 1B). Thus, we replaced domain I of the MtrF crystal structure with the one obtained by 1.0-μs MD simulation and used the structure for the following quantum mechanical/molecular mechanical (QM/MM) calculations.

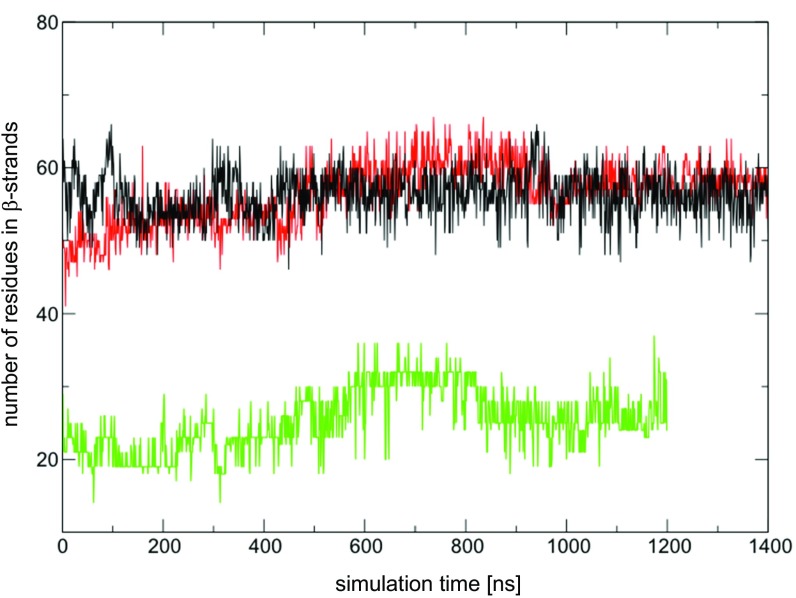
Fig. S3.

The number of residues that form β-strands in domain I in MD simulations using the original MtrF crystal structure (green lines) or the MtrF crystal structure, where the side chain orientations were corrected in domain I (black lines). For comparison, the number of residues that form β-strands in domain III in MD simulations using the original MtrF crystal structure is also shown (red lines).

In the MtrF crystal structure, His ligands [e.g., hemes 6 and 10 (Fig. S4)] seemingly cause steric repulsion. The QM/MM calculations showed changes in the geometries of other heme groups in the MtrF. We replaced all 10 bis-histidine ligated c-type heme groups with the QM/MM-optimized geometry (“refined MtrF structure”).

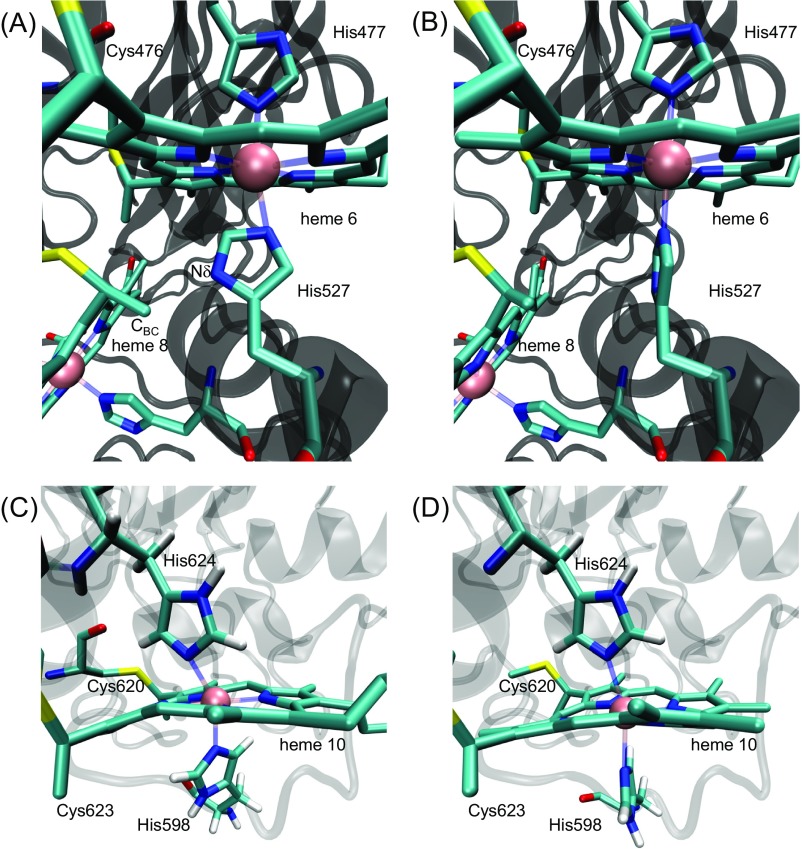
Fig. S4.

Orientation of the His ligands with respect to the heme planes. (A and B) Orientation of the His527 ligand with respect to the heme 6 plane. The Nδ atom of His527 (B-factor values ∼100 Ų), an axial ligand of heme 6, is only 2.7 Å distant from a carbon atom (C_BC) of heme 8, seemingly causing steric repulsion. Indeed, QM/MM calculations showed an ∼90° twist of the His527 imidazole ring along the Fe_{heme 8}…Nε_His527 axis. (A) The original MtrF crystal structure (PDB ID code 3PMQ), in which the Nδ atom of His527 is only 2.7 Å distant from the C_BC atom of heme 8. (B) The QM/MM-optimized geometry used for E_m calculations in this study. (C and D) Orientations of the His598 ligand with respect to the heme 10 plane. (C) The original MtrF crystal structure (PDB ID code 3PMQ). (D) The QM/MM-optimized geometry used for E_m calculations in this study: QM/MM calculations resulted in the ∼90° twist of the His598 imidazole ring along the Fe_{heme 10}…Nε_His598 axis.

Effect of Structural Modifications on the E_m.

The E_m values obtained by solving the linear Poisson–Boltzmann equation using the refined MtrF structure were almost the same as those for the MtrF crystal structure (Table S1) (5). Most modifications in the refined structure are in domain I, whereas domains II and IV remain unchanged, except for the bis-histidine ligated c-type heme regions. These results suggest that domain I, which is not the heme binding domain, did not significantly affect the calculated E_m values. Below, we refer to the E_m values calculated for the refined structure unless otherwise specified (atomic coordinates are in Dataset S1).

Table S1.

E_m values obtained using the post-MD structure and the original MtrF crystal structure for oxidized and reduced MtrF (in millivolts)

Heme	Post-MD structure		Crystal structure
	Oxidized	Reduced	Oxidized	Reduced
1	−112	−231	−113	−231
2	−94	−176	−64	−176
3	−47	−246	−5	−246
4	−141	−290	−132	−290
5	−178	−252	−177	−252
6	−137	−274	−135	−272
7	−169	−185	−169	−185
8	−214	−322	−210	−320
9	−336	−392	−332	−390
10	−296	−277	−297	−277

E_m Values.

Breuer et al. (11) calculated the E_m for hemes in oxidized MtrF, where E_m for the focusing heme was obtained in the presence of the other nine hemes being in the oxidized states (i.e., oxidized MtrF). In this study, we calculated the E_m values for both oxidized and reduced MtrF. The E_m values obtained solving the linear Poisson–Boltzmann equation were −47 to −336 mV for oxidized MtrF and −176 to −392 mV for reduced MtrF (Table 1). The calculated E_m shifts on changes in the MtrF redox state are consistent with the E_m shifts observed in electrochemical analysis (9). These values are in the E_m range reported for MtrF based on protein film voltammetry [−44 to −312 mV (5)] or that reported for the MtrCAB complex [0 to −450 mV (12)].

Table 1.

Calculated E_m values and factors that determine the E_m values for oxidized and reduced MtrF (in millivolts)

Em				Contribution to Em
				[Protein volume]	[Charge]
Domain	Heme	Oxidized	Reduced		Oxidized	Reduced
II	5	−178	−252	60	−18	−92
II	4	−141	−290	51	28	−121
II	3	−47	−246	117	56	−143
II	2	−94	−176	49	77	−5
II	1	−112	−231	68	40	−79
IV	6	−137	−274	79	4	−133
IV	7	−169	−185	55	−4	−20
IV	8	−214	−322	94	−88	−196
IV	9	−336	−392	34	−150	−206
IV	10	−296	−277	30	−106	−87

Contribution of the protein (dielectric) volume corresponds to the E_m shift caused by loss of solvation of heme in the protein environment.

Discussion

ET Pathways.

In contrast to the symmetric E_m profile reported by Breuer et al. (11), we obtained an E_m profile that indicated that the ET is more likely to be downhill from domain IV to II (Fig. 4). In particular, among the heme pairs in domains II and IV, which are located at symmetrical positions with respect to the pseudo-C₂ axis, the (heme 4, heme 9) pair has the largest E_m difference (ΔE_m = 195 mV) (Table 1), and the (heme 3, heme 8) pair has the second largest E_m difference (ΔE_m = 167 mV). These E_m differences (i.e., low E_m values for hemes 9 and 8 in domain IV with respect to hemes 3 and 1 in domain II) are mainly caused by the acidic residues at Asp631, Asp518, Asp490 (in domain IV), and Asp377 (in domain III), specifically localized in domain IV (Tables 2 and 3) [e.g., Asp631 decreases the E_m for heme 9 by −136 mV (Table S2)]. These acidic residues are not present in the corresponding regions of domain II. Although Breuer et al. (11) also reported that Asp631 decreased the E_m for heme 9, the contribution was −1,362 mV, which is unusually large (as discussed later).

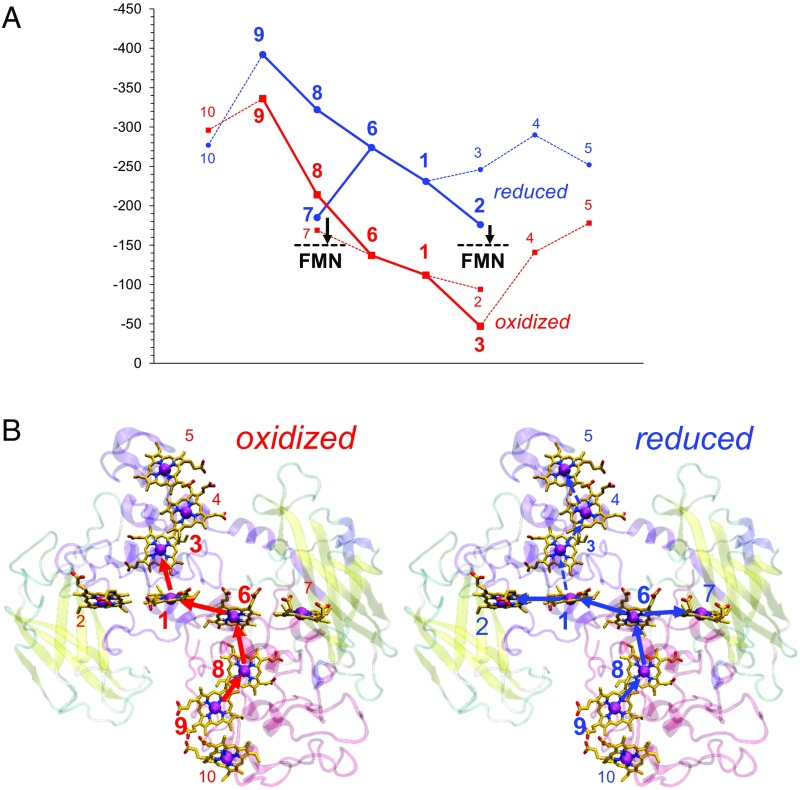
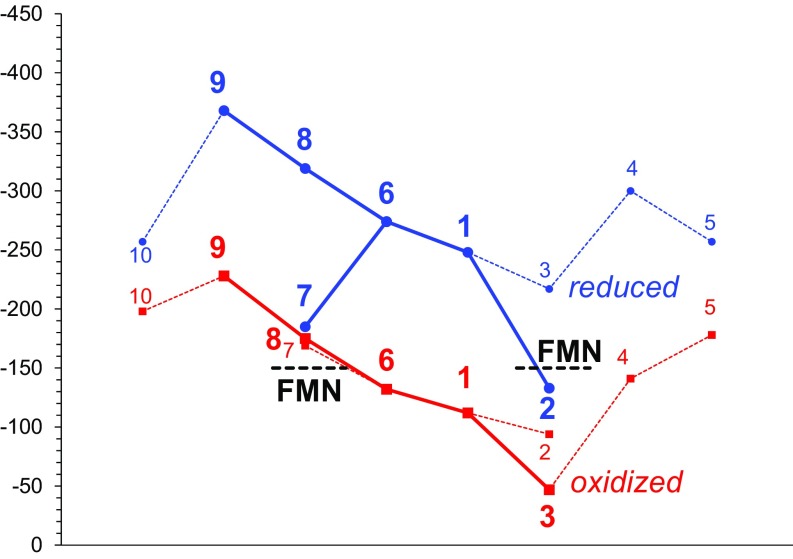
Fig. 4.

(A) E_m profiles of oxidized (red) and reduced (blue) MtrF. Table 1 shows the E_m values. Black dotted lines indicate the E_m values for bound FMN (8, 16). (B) Geometry of the ET pathways for (Left) oxidized and (Right) reduced MtrF. Thick solid arrows indicate the main downhill pathways; [hemes 9 → 8 → 6 → 1 → 3] may be more pronounced in reduced MtrF than in oxidized MtrF (dotted arrows).

Table 2.

Residue pairs in domains II and IV that increase E_m(heme 4) − E_m(heme 9) in oxidized MtrF (in millivolts)

Residue pairs		Contribution to Em(heme 4) − Em(heme 9)
Domain II	Domain IV
Trp306	Asp631	124
Ser247	Gly544	29
Ile229	Asp518	28

Table 3.

Residue pairs in domains II and IV that increase E_m(heme 3) − E_m(heme 8) in oxidized MtrF (in millivolts)

Residue pairs		Contribution to Em(heme 3) − Em(heme 8)
Domain II	Domain IV
Arg262	Ile557	88
Ile229	Asp518	74
Asn264	Ser558	46
Trp306	Asp631	43
Gln210	Asp490	28

The residue pair Glu102 (Domain I)/Asp377 (Domain IV) also contributes to an increase of 68 mV in E_m(heme 3) – E_m(heme 8).

Table S2.

Contributions of groups, including titrated propionic groups (-COO⁻), to the E_m reported in this work and that reported by Breuer et al. (11) (in millivolts)

Heme and groups	Contribution to Em
	This work	Breuer et al. (11)
1
Lys237	61	1,547
Asp192	−23	−1,113
2
Asp228	−61	−2,280
Lys237	70	1,478
Arg207	39	1,466
Lys222	116	1,312
Heme 1	42	−1,071
-COO−	−23	n.d.
-COO−	−1	n.d.
3
Arg262	99	1,446
Arg267	20	1,234
Arg186	97	1,189
Glu272	−28	−1,155
Asp261	−21	−1,098
Glu212	−30	−1,080
4
Heme 5	82	−1,685
-COO−	−56	n.d.
-COO−	−18	n.d.
Asp305	−8	−1,002
5
Asp294	−21	−1,135
6
Lys472	12	1,118
Asp479	−15	−1,001
7
Arg488	139	1,927
Asp518	−64	−1,240
Arg381	3	1,211
Asp490	−68	−1,194
heme 6	0	−1,122
-COO−	−26	n.d.
-COO−	11	n.d.
8
Lys520	164	1,387
Asp518	−80	−1,317
Lys472	28	1,271
Arg464	95	1,261
Arg465	71	1,257
Asp377	−84	−1,123
Glu466	−8	−1,035
9
Heme 10	64	−1,515
-COO−	−27	n.d.
-COO−	−19	n.d.
Asp631	−136	−1,362
Asp593	−4	−1,211
Lys634	9	1,003
10
Arg600	26	1,484
Asp631	−111	−1,410
Glu618	−9	−1,114
Asp593	−5	−1,009

n.d., not determined.

The MtrF crystal structure shows that the Asp631 side chain is oriented toward heme 9 (∼4 Å), which significantly decreases the E_m for heme 9 (Table 2). The E_m profile along the ET pathways remained downhill, even when titrated in the presence of protonated Asp631 (Fig. S5). This result suggests that the ET pathways could still be downhill, even if the orientation of Asp631 was disordered in the geometry of the MtrF crystal structure and protonated. The symmetric E_m profile along the ET pathways proposed by Breuer et al. (11) might be supported if the amino acid sequences of the heme binding domains II and IV were similar. However, the amino acid sequence identity between domains II and IV of MtrF is low (23% using ClustalW) (13) (Fig. S1). Thus, each symmetrical pair of hemes is more likely to have different E_m values (Table 1) because of the contributions of different types of residues as shown in Tables 2 and 3.

Fig. S5.

E_m profiles titrated in the presence of protonated Asp631 for oxidized (red) and reduced (blue) MtrF.

MtrF, MtrD, and MtrE are homologs of MtrC, MtrA, and MtrB, respectively. In the MtrCAB complex, MtrC has been reported to have higher E_m values than MtrA based on electrochemical analysis using cyclic voltammetry (12) [i.e., the ET pathway from MtrA to MtrC is downhill, whereas the ET pathway (Mtr pathway) can also mediate reversible ET (14)]. Considering the analogy between the MtrCAB and MtrFDE complexes (5), the corresponding ET pathway may proceed from MtrD to MtrF. It seems plausible that the ET pathway from domain IV to II is downhill in terms of the location and function of MtrF, which is at the terminus of the intermolecular ET chain via the MtrFDE complex and directly reduces extracellular substrates via hemes 2, 5, or 7 (5, 6). It should also be noted that ET occurs in the uphill ET pathway [e.g., the cytochrome c subunit of photosynthetic reaction centers from Blastochloris viridis (15)]; this fact also suggests that a completely symmetric E_m profile (11) is not necessarily required to facilitate the reversible ET (14) in the Mtr conduit.

Switching the ET Pathway in Response to the MtrF Redox State.

In oxidized MtrF, the E_m values for hemes increase along the chain of hemes 9, 8, 6 (domain IV), 1, and 3 (domain II), resulting in a downhill ET pathway [hemes 9 → 8 → 6 → 1 → 3] (Fig. 4). In reduced MtrF, the E_m values were significantly lower, switching the ET pathway to [hemes 9 → 8 → 6 → 1 → 2] or [hemes 9 → 8 → 6 → 7] (Fig. 4).

Intriguingly, (i) in reduced MtrC, FMN showed pronounced binding affinity compared with oxidized MtrC (3). (ii) Hemes 2 and 7 have been proposed to be located near the FMN binding site (5, 6). (iii) The E_m of bounded FMN is not known for MtrF, but for MtrC, it is reported to be ∼–150 mV using differential pulse voltammetry (8, 16). If this E_m value holds true for MtrF, bound FMN can serve as an electron acceptor for both hemes 2 and 7 at the terminus of the entire ET pathway (–176 and –185 mV, respectively) (Table 1) when MtrF is reduced (Fig. 4). Notably, in oxidized MtrF, the ET from heme 2 (–94 mV) (Table 1) to FMN [∼–150 mV (8, 16)] is uphill. (iv) Okamoto et al. (8) showed that binding of FMN at the decaheme cytochrome leads to significant enhancement of ET. This finding (i.e., activating the ET pathways [hemes 9 → 8 → 6 → 1 → 2 → FMN] and [hemes 9 → 8 → 6 → 7 → FMN]) fits well with involvement of bound FMN as an electron acceptor (3, 7, 8) when MtrF is reduced in terms of both the E_m values and the location of the ET pathway (Fig. 4). MtrF may alter its function by switching the ET pathway in response to the redox environment. Among the 10 hemes, heme 3, which is surrounded by hemes 1, 2, and 4, shows the largest change in E_m, –199 mV, in the transition from oxidized MtrF to reduced MtrF (Table 1); this E_m shift results in a less uphill, more isoenergetic ET pathway toward heme 5 (Fig. 4). Intriguingly, heme 5 has been proposed to serve as a site that can directly reduce extracellular insoluble substrates (5, 6). Fig. 4 shows that the ET pathway [hemes 9 → 8 → 6 → 1 → 3] may be more pronounced in reduced MtrF than in oxidized MtrF.

E_m Reported by Breuer et al.

Electrostatic influence of residues.

In the present study, the ET pathways are downhill along domains IV and II for both reduced and oxidized MtrF, which is caused by the different contributions of the electrostatic influences of domains II and IV to the hemes (Tables 2 and 3). The influence of the protein dielectric volume (SI Discussion) on E_m, which decreases the solvation of the heme group and lowers the E_m value, is similar in the domain (II, IV) heme pairs [e.g., (heme 4, heme 9) and (heme 3, heme 8)] (Table 1). Thus, the electrostatic influence of residues is the main factor that differentiates the E_m values of hemes in domains II and IV.

In the results reported by Breuer et al. (11), residues make unusually large contributions to E_m values (e.g., Asp228 decreased the E_m for heme 2 by –2,280 mV), whereas it decreased the E_m for heme 2 by −61 mV in this study (Table S2). Breuer et al. (11) also listed a number of residues that contributed more than 1,000 mV to the E_m shift, which suggests that their E_m values for hemes were determined using overestimated electrostatic influences.

E_m values obtained using a TI approach.

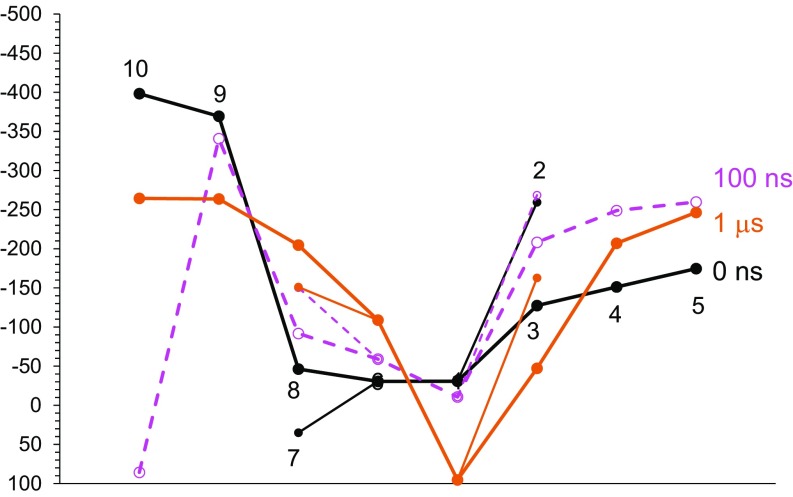
Breuer et al. (11) calculated E_m values using a TI approach based on MD simulations. To understand how they determined the E_m of MtrF, we also calculated the E_m using a TI approach based on the original MtrF crystal structure (not the refined MtrF structure). Notably, our total time for sampling simulation is comparable with that reported by Breuer et al. (11) (Computational Procedures). The E_m profiles obtained after equilibrating for 100 ns and 1 μs differ significantly from those reported by Breuer et al. (11) (Fig. 5). In addition, the different E_m profiles obtained after equilibrating for 100 ns and 1 μs indicate that the MtrF structure can change even after equilibrating for 100 ns. Breuer et al. (11) equilibrated for only 5 ns. The three different E_m profiles obtained using three different equilibration times indicates that the TI approach is not applicable under the conditions used in the previous studies, and either the equilibrating or sampling simulation times must be insufficient. The difficulty in reproducing the E_m profiles reported in the previous studies, even with a longer equilibration time, argues against the quality of their calculated E_m values.

Fig. 5.

E_m profiles obtained for oxidized MtrF using a TI approach based on MD simulations, in which the initial structures were obtained after equilibrating for 0 ns (black), 100 ns (pink), and 1 μs (orange). It should be noted that Breuer et al. (11) uniformly added the constant C = −1,567 mV to reproduce the E_m range of −44 to −312 mV (5) reported for the hemes in MtrF. The corresponding constants were 453, 434, and 511 mV after equilibrating for 0 ns, 100 ns, and 1 μs, respectively.

Breuer et al. (11) seem to have used the original atomic coordinates of the MtrF crystal structure, in which domain I contains marked structural disorder (Fig. 2) because of the orientation of the side chains that prevents formation of the β-strands (Figs. 1, 2, and 3). Using the unstable structure may be crucial when calculating the E_m using an MD-based TI approach and may contribute to the uncertainty of their calculated E_m values.

Breuer et al. (11) fixed the protonation states of the heme propionic groups as permanently ionized, even in the presence of reduced heme groups. Fixation of the protonation states of titratable residues can also be a fundamental problem when using an MD-based TI approach to calculate the E_m value, in particular for heme proteins, because the protein structure changes with respect to the original atomic coordinates of the crystal structure to reproduce the initially considered single-protonation pattern of the titratable residues. In addition, the protonation state of the heme propionic group is strongly coupled with the redox state of the heme ring (Table S3) and affects the E_m value, which can often explain the pH dependence of the E_m for heme (17–19). Fixation of the protonation states of the heme propionic groups (to be ionized) should also overstabilize the oxidized state of heme and lower the E_m values. Thus, for E_m calculations of heme proteins, it is a prerequisite to reproduce the Henderson–Hasselbalch curve for titratable residues near the heme ring (20), which can be achieved only when the partial protonation state of the heme propionic groups is appropriately considered as shown in a number of electrostatic approaches (17–19).

Table S3.

Protonation probabilities (protonated = 1 and deprotonated = 0) of heme propionic groups (acid A and acid D) in reduced MtrF

Heme and redox state	Protonation states
	Acid A	Acid D
1
Oxidized	0.00	0.96
Reduced	0.00	1.00
2
Oxidized	0.07	0.51
Reduced	0.38	0.58
3
Oxidized	0.00	0.00
Reduced	0.20	0.00
4
Oxidized	0.76	0.28
Reduced	0.75	0.62
5
Oxidized	0.00	0.11
Reduced	0.46	0.38
6
Oxidized	0.25	0.98
Reduced	0.84	1.00
7
Oxidized	0.27	0.00
Reduced	0.80	0.17
8
Oxidized	0.00	0.00
Reduced	0.00	0.00
9
Oxidized	0.97	0.24
Reduced	0.98	0.47
10
Oxidized	0.31	0.18
Reduced	0.65	0.20

Although the focusing heme was titrated (i.e., fully oxidized or reduced), the other hemes were in the reduced states.

SI Discussion

The protein dielectric volume (i.e., the space obtained by merging the volumes of the van der Waals spheres of all protein atoms) is a main contributor of “protein hydrophobicity” to the hemes: the protein dielectric volume prevents water molecule access to the hemes and thus, decreases the availability of solvation energy. In particular, the loss of solvation energy significantly destabilizes the oxidized state, increasing the E_m.

Conclusions

The E_m values for the 10 hemes in MtrF were calculated by solving the linear Poisson–Boltzmann equation and considering the protonation states of all titratable residues and heme propionic groups. The E_m profiles calculated show that the ET pathway proceeds downhill from domain IV to II. When MtrF is reduced, the direction of the ET pathway switches, and FMN becomes the terminal electron acceptor. These findings (i.e., switching of the ET pathways to [hemes 9 → 8 → 6 → 1 → 2 → FMN] or [hemes 9 → 8 → 6 → 7→ FMN]) are concordant with FMN acting as the bound electron acceptor (3, 7, 8) when MtrF is reduced.

Computational Procedures

Initial Coordinates and Atomic Partial Charges.

The atomic coordinates of MtrF were taken from the X-ray structure of MtrF of Shewanella oneidensis at a resolution of 3.2 Å [Protein Data Bank (PDB) ID code 3PMQ] (5). H atoms were generated and energetically optimized with CHARMM36 (21), and all titratable groups were kept in their standard protonation states. Atomic partial charges of the amino acids were adopted from the all-atom CHARMM36 (21) parameter set. The atomic charges of the low-spin c-type heme, including histidine and cysteine ligands, were determined by fitting the electrostatic potential in the neighborhood of these molecules by using the restrained electrostatic potential (RESP) procedure (22). The electronic wave functions were calculated after geometry optimization with the unrestricted density functional theory (DFT) method with the B3LYP functional and LACVP* basis sets with the JAGUAR program (23).

Homology Modeling of Domain I in MtrF.

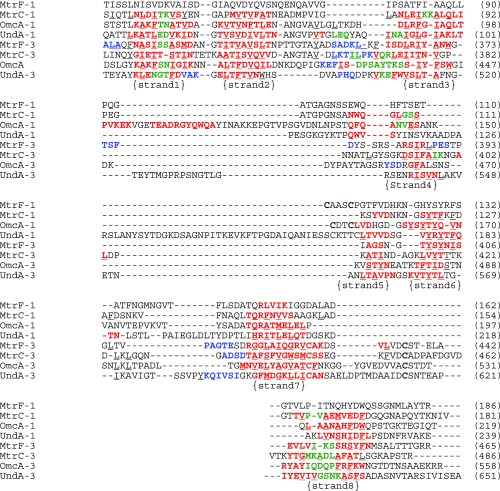
To understand the absence and presence of the β-barrel structure in domain I of the MtrF (5) and MtrC (3) crystal structures, respectively (Fig. 2), we prepared the atomic coordinates where the domain I region in the MtrF crystal structure was reconstructed using a homology modeling approach with the SWISS-MODEL web interface (24). The crystal structure of MtrC from S. oneidensis at a resolution of 1.8 Å (PDB ID code 4LM8) was used as the main structural template of domain I for reconstruction of MtrF (Fig. S1) (3), except for the Lys86 and Lys87 region of MtrC, because the two positively charged residues KK in MtrC are replaced with the single nonpolar residue I− in MtrF and G− in OmcA (Fig. S6). The crystal structure of OmcA at a resolution of 2.7 Å (PDB ID code 4LMH) (4) was used as the main structural template for the corresponding region. The atomic coordinates obtained were used as the initial structure for subsequent MD simulations (Results).

Fig. S6.

Amino acid sequence alignment of domains I and III in MtrF (PDB ID code 3PMQ), MtrC (PDB ID code 4LM8), OmcA (PDB ID code 4LMH), and UndA (PDB ID code 3UFK). Residues that orient toward the inner hydrophobic core in β-strands are underlined. Residues in α-helices (blue), β-strands (red), and disordered strands (green) are also shown.

MD Simulations.

MD simulations were performed for the following two purposes (1): for E_m calculations using the linear Poisson–Boltzmann approach [i.e., equilibrating the reconstructed homology model (see above) and obtaining the refined MtrF structure (Results)] (2) and for E_m calculations using a TI approach [i.e., equilibrating the unmodified original MtrF crystal structure, as used by Breuer et al. (11), and calculating the E_m values using a TI approach]. In both cases, the following procedures were used; for comparison, the protonation states of the titratable residues were identical to those used by Breuer et al. (11). The 10 hemes were oxidized; the acidic groups, including the heme propionic groups, were negatively charged. The basic residues were positively charged, and the histidine residues (except His451) were treated as electrostatically neutral; His451 was positively charged. The respective models were processed as follows: (i) model arrangement in a periodic boundary box, (ii) solvation with TIP3P water models (25), (iii) structural optimization with positional restraints on heavy atoms using the initial structure as a reference, (iv) MD simulation for 1.0 ns with positional restraints with the Berendsen thermostat at 300 K and the barostat at 1.0 bar (26), (v) MD simulation with gradual releasing restraints over 1.0 ns under identical thermostat and barostat conditions, and (vi) production MD run over 1.0 μs with the Nose–Hoover thermostat (27, 28) at 300 K with t_t = 0.5 ps and the Parrinnello–Rahman barostat (29) at 1.0 bar with t_p = 5.0 ps. All MD simulations above were conducted with an MD engine GROMACS 5.0.7 (30–32) with an adopted CHARMM36 force field (33).

QM/MM Calculations.

We used the Qsite (34) program code. We used the unrestricted DFT method with the B3LYP functional and LACVP* basis sets.

E_m Calculation I: Solving the Linear Poisson–Boltzmann Equation.

To obtain the absolute E_m values for the protein, we calculated the electrostatic energy difference between the two redox states in a reference model system by solving the linear Poisson–Boltzmann equation with the MEAD program (35) and using a known experimentally measured E_m value for bis-histidine ligated heme [−220 mV in water (36)]. The difference in the E_m value of the protein relative to the reference system was added to the known E_m value. The ensemble of the protonation patterns was sampled by Monte Carlo method with Karlsberg (37). The linear Poisson–Boltzmann equation was solved using a three-step grid-focusing procedure at resolutions of 2.5, 1.0, and 0.3 Å. Monte Carlo sampling yielded the probabilities [A_ox] and [A_red] of the two redox states of molecule A. The E_m was evaluated using the Nernst equation. A bias potential was applied to obtain an equal amount of both redox states ([A_ox] = [A_red]), thereby yielding the redox midpoint potential as the resulting bias potential. To facilitate direct comparisons with previous computational results (19, 38, 39), identical computational conditions and parameters were used. All computations were performed at 300 K, pH 7.0, and an ionic strength of 100 mM (ref. 40 discusses the influence of the ionic strength on the calculated E_m values); the dielectric constants were set to 4 for the protein interior (ε_p) and 80 for water (ε_w). The size of the ε_p value depends on what is not included explicitly in the protein model used. Lower ε_p values (e.g., ε_p = 1) may be used when all factors that describe electrostatic interactions (e.g., flexibility of the protein structure and flexibility of the protonation states of the protein titratable residues) are considered explicitly (41, 42). Because we have consistently used ε_p = 4 and ε_w = 80 and reproduced the experimentally measured E_m and pK_a values in many redox active proteins [e.g., heme (19) and flavin (38, 39)], ε_p = 4 seems to be optimal in our computational models.

E_m Calculation II: Using TI.

We also calculated the E_m using a TI approach as used by Breuer et al. (11). After restraint-releasing simulations, the initial structures for TI simulations were obtained after equilibration for (i) 0 ns, (ii) 100 ns, and (iii) 1 μs. TI simulations were conducted over 10 ns with an MD time step of 2.0 fs, namely Δλ = 2.0 × 10⁻⁷, by reducing a focusing oxidized heme and fixing the protonation states of the other titratable groups. In these simulations, oxidized heme (Fe³⁺) was gradually reduced (to Fe²⁺) over 10 ns. The total sampling simulation time used in the previous study by Breuer et al. (11) is comparable with the present study.