Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2017 Mar 22;8:14842. doi: 10.1038/ncomms14842

Constructive quantum interference in a bis-copper six-porphyrin nanoring

Sabine Richert 1,*, Jonathan Cremers 2,*, Ilya Kuprov 3, Martin D Peeks 2, Harry L Anderson 2,a, Christiane R Timmel 1,b
PMCID: PMC5364408  PMID: 28327654

Abstract

The exchange interaction, J, between two spin centres is a convenient measure of through bond electronic communication. Here, we investigate quantum interference phenomena in a bis-copper six-porphyrin nanoring by electron paramagnetic resonance spectroscopy via measurement of the exchange coupling between the copper centres. Using an analytical expression accounting for both dipolar and exchange coupling to simulate the time traces obtained in a double electron electron resonance experiment, we demonstrate that J can be quantified to high precision even in the presence of significant through-space coupling. We show that the exchange coupling between two spin centres is increased by a factor of 4.5 in the ring structure with two parallel coupling paths as compared to an otherwise identical system with just one coupling path, which is a clear signature of constructive quantum interference.

Quantum interference in charge transport is attracting interest with applications in nanoelectronics and quantum computing. Here, the authors present a method for quantifying electronic transmission through molecules, and demonstrate constructive quantum interference in a molecule with two identical, parallel coupling paths.

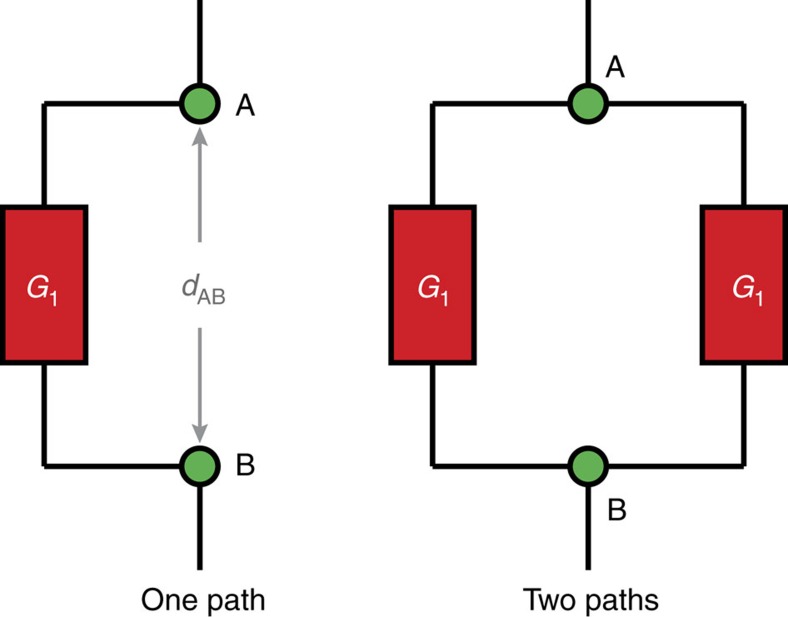
When electrons tunnel through a molecule via more than one pathway, the wavefunctions corresponding to different routes may be in-phase or out-of-phase with each other, leading to constructive or destructive interference. Quantum interference effects of this type are thought to control electron transfer through proteins1,2,3. Quantum interference also provides many opportunities for enhancing performance in single-molecule electronic devices, by creating sharp transport resonances4,5. When the distance of the transmission exceeds a certain threshold, interactions with vibrational degrees of freedom result in a change of mechanism from phase-coherent single-step tunnelling to incoherent multistep hopping, erasing interference effects6,7. It is difficult to measure coherence lengths in molecular structures and the size limit for quantum mechanical behaviour is not well established. The simplest test for quantum coherence is to compare transmission between two points, A and B, through two identical channels in parallel, each of conductance G1, with that through just one isolated channel as shown in Fig. 1.

Figure 1. Schematic illustration of transmission through one or two paths.

Figure 1

Open in a new tab

Quantum coherence can be tested by comparison of the transmission properties. Assuming two identical parallel paths connecting A and B with the total conductance referred to as GAB, coherent transmission is expected to yield 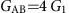Inline graphic, whereas non-coherent transport should result in 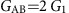Inline graphic. The transmission G is closely related to the exchange coupling J.

A classical description predicts that the total conductance for the two channels will be given by Kirchhoff's circuit law as 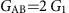Inline graphic; this result is expected when the distance dAB is greater than the coherence length. On the other hand, if the system behaves coherently, constructive quantum interference is expected to give a total conductance of 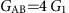Inline graphic (refs 4, 8).

Recently, this scenario has been tested experimentally for charge transport through single molecules. STM break-junction measurements on a thioether-linked cyclophane (dAB=0.7 nm) gave the result 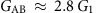Inline graphic, providing evidence for quantum interference9. Similar experiments compared the conductance of a carbobenzene macrocycle (dAB=0.8 nm) with a single-path reference molecule to give 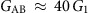Inline graphic, although in this case the higher conductance of the two-path system is partly a consequence of its greater conformational rigidity10. These results highlight the challenges involved in using single-molecule charge transport measurements to probe quantum coherence.

Despite many advances in methodology11,12,13, it is difficult to measure molecular conductances accurately enough to detect the predicted fourfold increase in GAB bestowed by constructive interference, particularly in large molecules, which are expected to be on the threshold between coherent and incoherent transport, and which have very low conductances. Furthermore, it is difficult to synthesize pairs of molecules that have identical conformations and differ only in the number of available tunnelling channels.

Here we present solutions to both these problems: We implement the scheme shown in Fig. 1 by testing the through-bond exchange coupling, J, between two paramagnetic centres, which can be measured accurately by electron paramagnetic resonance (EPR), and we lock the molecular wire into a well-defined conformation by using supramolecular assembly on a radial template. Comparison of the mean value of the exchange coupling between copper(II) centres in compounds P2||P2, with two paths, and P2||X, with one path gives 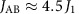Inline graphic for a through-bond tunnelling distance of dAB=3.9 nm, demonstrating constructive quantum interference over a remarkably long distance.

Results

Chemical systems

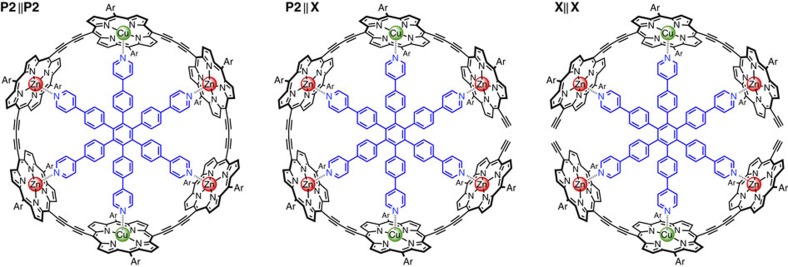
Figure 2 shows the chemical structures of the three systems investigated in this work. The cyclic porphyrin hexamer complex on the left exhibits D2h symmetry with the two copper porphyrin units arranged in opposing positions, connected to each other on either side via identical zinc porphyrin dimer (P2) bridges. The highly conjugated structure is locked into a well-defined conformation by a hexapyridyl template which coordinates to the central metals of the porphyrin units. The ring represents a molecular analogue of the two-path model in Fig. 1 in which transmission between the two copper spin centres is possible through two identical, parallel pathways. We refer to this structure as P2||P2.

Figure 2. Chemical structures of the three compounds.

Figure 2

Open in a new tab

The structures differ in the number of pathways between the two copper centres: left—P2||P2, two paths; centre—P2||X, one path; right—X||X, no path. The porphyrin side group ‘Ar' represents an aryl substituent, 3,5-di-tert-butylphenyl in the case of P2||P2 and 3,5-bis(trihexylsilyl)phenyl for P2||X and X||X, which provides high solubility and prevents aggregation.

Interruption of the conjugation in one path of an otherwise unaltered ring assembly leads to formation of a molecular structure resembling the ‘one-path' model and referred to as P2||X, see Fig. 2 (centre). This complex consists of a bis-copper linear porphyrin hexamer coordinated to the radial hexapyridyl template; it is extremely stable with a formation constant of more than 108 M−1 in toluene at 298 K. In the third structure, the bonds between both sets of neighbouring Zn porphyrin units have been broken and we consequently refer to this structure as X||X. This is the 2:1 complex formed from two equivalents of a linear porphyrin trimer and the hexapyridyl template. UV–visible–near-infrared (UV–vis–NIR) titrations show that each porphyrin trimer coordinates the template with a binding constant of 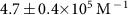Inline graphic, which implies that the mole fraction of the 2:1 complex is about 0.8 in toluene at 298 K (0.2 mM). All relevant details on the synthesis, binding studies and sample preparation are given in Supplementary Figs 1–8 and Supplementary Notes 1 and 2 for P2||X and X||X. The synthesis of P2||P2 has been reported elsewhere14.

Methodology

The main focus of this work is the quantification of the interspin exchange coupling, J, between the two copper centres which reports on wavefunction overlap and interference phenomena. Large exchange couplings (>>1 cm−1) can conveniently be measured by SQUID15,16, whereas EPR can be used to determine J values in the sub-cm−1 regime, as we shall demonstrate below. The unpaired electrons also couple through space via the dipolar interaction, D. If EPR experiments can be conducted in the fast tumbling regime, the effects of the anisotropic dipolar coupling vanish, allowing an isolation of the isotropic exchange coupling. Such an approach was not possible here as these large molecules tumble too slowly even at room temperature.

The dipolar interaction is defined as

graphic file with name ncomms14842-m7.jpg

where r is the interspin distance, θ is the angle between the spin-spin vector and the dipolar Z axis, ge the electronic g-factor, βe the Bohr magneton and μ0 the vacuum permeability. D is thus identical for all three structures whose architectures (and therefore r) are controlled by the same rigid template. Here we demonstrate that, with this information in hand, we can employ the technique of double electron electron resonance (DEER) to quantify and compare the exchange interaction in structures P2||P2 and P2||X.

DEER is usually employed to elucidate molecular structures and their flexibility via the determination of interspin distances and their distributions. The latter are extracted from modulations in the DEER traces presumed to be solely due to the dipolar interaction, which, for the typically addressed interspin distances (1.5−8 nm), much exceeds the magnitude of the exchange interaction17,18,19. However, in highly π-conjugated systems, such as ours, the two couplings might be of comparable magnitude and their respective contributions to the DEER time trace need to be disentangled.

For conjugated nitroxide biradicals such a separation was successfully demonstrated for cases where both singularities of the Pake pattern could be detected after Fourier transformation of the DEER traces20,21,22,23. However, generally, the separation of the two contributions is difficult, especially if D and J are of the same order of magnitude.

To determine J from the experimental DEER data, we derived the analytical expression given in equation (2), describing the dependence of the dipolar evolution (DEER) time trace on both D and J in the limit when the difference between the pump and probe frequencies in the absence of coupling are much larger than the pseudosecular part of the coupling Hamiltonian (weak coupling approximation).

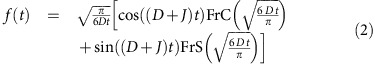graphic file with name ncomms14842-m8.jpg

In this equation, f(t) is the reduced form factor, FrS and FrC stand for the Fresnel sine and cosine integrals, respectively, and all other parameters have their usual meanings. A full derivation of the expression is given in Supplementary Note 4. The use of this equation requires orientation selective effects on the modulation frequency to be negligible. Its applicability in the present case is justified in Supplementary Note 4.

As can be seen from the analytic result, the DEER time trace in the presence of through-bond exchange coupling has a complicated dependence on D and J; to a first approximation, however, the experimental signal is expected to oscillate with a frequency roughly corresponding to D+J.

Determination of the exchange interaction

Equipped with an analytical expression for the DEER trace including D and J, the first objective is the determination of the former in the absence of the latter. To this end, we quantify the dipolar coupling between the copper centres by conducting DEER on X||X, the ring structure devoid of any exchange coupling paths between the copper centres.

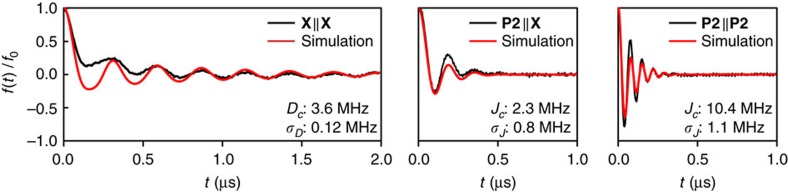
Figure 3 (left) shows the experimental background-corrected DEER time trace recorded for X||X. The frequency of the pronounced dipolar modulation corresponds to a distance of roughly 2.5 nm (at g=2.05) as determined by Fourier transformation of the time trace (cf. Supplementary Fig. 10). This result is in good agreement with published X-ray crystallography and density functional theory (DFT) data for a similar template-bound six-membered porphyrin ring for which a ring diameter of 2.5 nm was determined14,24.

Figure 3. Experimental DEER data and simulations.

Figure 3

Open in a new tab

Black: Experimental background-corrected DEER traces of X||X, P2||X and P2||P2. Red: Simulations of the individual experimental traces using the analytic expression given in equation (2). The simulation parameters are indicated in the graphs.

A first inspection of the DEER trace for the two exchange coupled systems, P2||X and P2||P2 in Fig. 3 (centre and right, respectively) reveals immediately that introduction of through-bond coupling paths leads to a significant increase in the modulation frequency. Fourier transformation of the traces further establishes the dominant frequency components for P2||X as 5.2 MHz and for P2||P2 as 13 MHz, providing first proof that the exchange interaction has pronounced but distinct effects in the two coupled systems. To demonstrate that the observed frequency is indeed due to intramolecular coupling rather than nuclear electron spin echo envelope modulation effects or intermolecular interactions, data for P2||P2 were also recorded at different spectrometer frequencies and sample concentrations. The results are shown in Supplementary Fig. 9 and confirm that the high-frequency modulation indeed arises from through-bond exchange coupling since the measured modulation frequency is found to be the same in all cases.

To quantify the magnitude of J, the background-corrected experimental data were simulated using equation (2) assuming Gaussian distributions in the frequency domain for D and J.

The results of the simulations are compared with the corresponding experimental data in Fig. 3. Although the relative modulation amplitudes cannot be reproduced exactly, partially due to uncertainties in the DEER background correction, the simulations can be considered satisfactory since the modulation frequencies and the dampening of the oscillations, which contain all the relevant information for our purposes, can be well reproduced and determined with high precision. The background signal in the DEER traces can be attributed to incomplete complex formation, as discussed in Supplementary Note 5 and Supplementary Figs 13–15. The conclusions of the discussion of the background signal are also supported by electron-nuclear double resonance measurements shown in Supplementary Fig. 11 and described in Supplementary Note 3.

The centres and widths of the frequency distributions in J and D, obtained from the simulations, are indicated in the corresponding graphs in Fig. 3. First, the distribution in D was determined using the X||X sample with two breaks in the conjugation. The resulting distribution then served as an input into the simulations of the P2||X and X||X DEER traces in which, consequently, only the centre and width of the distribution in J were adapted. The distribution in D was found to be relatively narrow corresponding to a width of 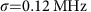Inline graphic, whereas the distributions in J are generally found to be much wider. The centre frequency of 3.6 MHz determined for D corresponds to a Cu⋯Cu distance of 2.47 nm at g=2.05, in excellent agreement with expectations from available experimental data and DFT calculations14,24.

From equation (2) it appears that only the relative sign of D and J can in principle be determined by a simulation of the experimental data using this relation. However, it has been shown that the frequency distribution of combined dipole–dipole and exchange coupling does depend on the sign of the exchange coupling17, which enables us to determine the absolute value of J as also confirmed in Supplementary Fig. 12 and discussed in Supplementary Note 5. The sign of J could also be confirmed by DFT calculations as described in Supplementary Note 6.

For P2||P2, an exchange coupling of 10.4 MHz (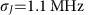Inline graphic) could be determined from the simulation. Since equation (2) was derived for an exchange coupling Hamiltonian of 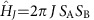Inline graphic, a positive J value indicates antiferromagnetic coupling (ES<ET). The magnitude of the exchange interaction between the two copper centres is remarkable given the large through-bond interspin distance of 3.9 nm, certainly a consequence of the high degree of conjugation between the paramagnetic centres25.

The most crucial finding, however, is that interruption of one coupling path in P2||X leads to attenuation of J to just 2.3 MHz.

Discussion

We observe an approximately fourfold increase in the size of the exchange coupling in the two-path structure as compared to the one-path case. This result is in excellent agreement with the prediction of a fourfold increase in the transmission of a closed system with two parallel branches as compared to a single linear chain under conditions of constructive quantum interference4. Although quantum interference has been observed in two-path systems by conductance measurements9,10, this is the first time that it has been detected via exchange coupling.

The major advantage of employing DEER is that the data allow the direct quantification of the exchange coupling between the spins alleviating the need for any connecting electrodes. Our study does not report on conductance phenomena directly but rather addresses quantum interference via the exchange interaction between the two paramagnetic centres. However, it has been shown previously that conductance and antiferromagnetic coupling exhibit strongly correlated trends26,27 so that the fourfold increase in the exchange coupling by introduction of a second, parallel path is consistent with the prediction based on transmission considerations.

These results provide an unambiguous demonstration of constructive quantum interference in a closed model system with two identical parallel paths. They illustrate the applicability of DEER for measuring long-range exchange couplings in molecular wires and indicate that efficient long-range electronic communication is favoured by a highly rigid molecular framework.

The nanorings studied here provide a rich playground for further work. The effects of inequivalent pathways (arranging the copper centres in ortho or meta positions), ring size, connecting geometry and linkers are presently being tested in our laboratory.

Methods

Sample preparation

The synthesis of the compounds used in this study is presented in Supplementary Note 1. UV–vis–NIR titrations shown in Supplementary Figs 5–8 and discussed in Supplementary Note 2 demonstrate formation of the complexes shown in Fig. 2. For the preparation of the EPR samples, the porphyrin oligomers were first dissolved in CHCl3. The hexapyridyl template was added in stoichiometric amounts for complex formation (0.5 eq. for X||X, 1 eq. for P2||X) and complex formation was verified by UV–vis–NIR spectroscopy. The solvent was then removed under high vacuum conditions and the complex subsequently redissolved in deuterated toluene to yield a solution with a concentration of about 0.2 mM. After verification of complex formation in toluene, the solutions were degassed by the freeze–pump–thaw method and backfilled with argon. The deoxygenated solutions were then transferred to an EPR tube and the EPR tube closed with a subaseal. For storage in liquid nitrogen the subaseal was removed. The frozen samples were directly inserted into the EPR resonator for the measurements at 15 K.

Details of the EPR measurements

DEER experiments were performed at 15 K and Q-band frequencies on a Bruker ELEXSYS E580 spectrometer equipped with a Bruker EN 5107D2 resonator and a liquid helium flow cryostat using the sequence π/2−τ1−π−τ1−τ2−π−τ2–echo at the detection frequency νdet while applying a single π pulse at the pump frequency νpump during the interval τ1−τ2. The pump π pulse position was varied step-wise starting at a time t0<τ1 after the first detection π pulse up to a time t<τ1+τ2, shortly before the second π pulse at νdet.

A pulse length of 12 ns was chosen for the pump pulse, whereas all detection pulses had a length of 16 ns. A 16-step phase cycle needed to be employed to remove unwanted echos (arbitrary waveform generator (AWG), coherent source). Deuterium nuclear modulations were averaged by increasing τ1 in eight steps of 16 ns, typically starting at τ1=400 ns. To record the traces shown in the text, the pump frequency was set to the maximum of the field-swept EPR spectrum (xy) and the detection frequency was 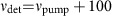Inline graphic MHz. The pump pulse position was varied in steps of either 4 or 8 ns and data were collected at a repetition rate of 2 μs using τ2 values between 2 and 3 μs.

Data availability

The data that support the findings of this study are available from the corresponding authors on reasonable request.

Additional information

How to cite this article: Richert, S. et al. Constructive quantum interference in a bis-copper six-porphyrin nanoring. Nat. Commun. 8, 14842 doi: 10.1038/ncomms14842 (2017).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Material

Supplementary Information

Supplementary Figures, Supplementary Notes and Supplementary References

ncomms14842-s1.pdf (2.2MB, pdf)
Peer Review File
ncomms14842-s2.pdf (406.2KB, pdf)

Acknowledgments

Financial support from the EPSRC (EPL011972/1, EP/M016110/1 and EP/J015067/1) and the ERC (Grant 320969) is gratefully acknowledged. We thank the EPSRC UK National Mass Spectrometry Facility at Swansea University for mass spectra. We would like to acknowledge the use of the University of Oxford Advanced Research Computing (ARC) facility in carrying out this work (http://dx.doi.org/10.5281/zenodo.22558).

Footnotes

The authors declare no competing financial interests.

Author contributions J.C. synthesized (BE) the compounds and demonstrated formation of the supramolecular complexes. S.R. performed the EPR experiments and analysed the data. S.R. and I.K. performed the simulations. M.D.P. carried out DFT calculations. C.R.T. and H.L.A. coordinated the study. S.R., H.L.A. and C.R.T. wrote the manuscript. All authors discussed the results and commented on the manuscript.

References

  1. Balabin I. A. & Onuchic J. N. Dynamically controlled protein tunneling paths in photosynthetic reaction centers. Science 290, 114–117 (2000). [DOI] [PubMed] [Google Scholar]
  2. Beratan D. N. et al. Steering electrons on moving pathways. Acc. Chem. Res. 42, 1669–1678 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. de la Lande A. et al. Quantum effects in biological electron transfer. Phys. Chem. Chem. Phys. 14, 5902–5918 (2012). [DOI] [PubMed] [Google Scholar]
  4. Lambert C. J. Basic concepts of quantum interference and electron transport in single-molecule electronics. Chem. Soc. Rev. 44, 875–888 (2015). [DOI] [PubMed] [Google Scholar]
  5. Hansen T. & Solomon G. C. When conductance is less than the sum of its parts: exploring interference in multiconnected molecules. J. Phys. Chem. C 120, 6295–6301 (2016). [Google Scholar]
  6. Goldsmith R. H., Wasielewski M. R. & Ratner M. A. Electron transfer in multiply bridged donor–acceptor molecules: dephasing and quantum coherence. J. Phys. Chem. B 110, 20258–20262 (2006). [DOI] [PubMed] [Google Scholar]
  7. Kocherzhenko A. A., Grozema F. C. & Siebbeles L. D. A. Single molecule charge transport: from a quantum mechanical to a classical description. Phys. Chem. Chem. Phys. 13, 2096–2110 (2011). [DOI] [PubMed] [Google Scholar]
  8. Magoga M. & Joachim C. Conductance of molecular wires connected or bonded in parallel. Phys. Rev. B 59, 16011–16021 (1999). [Google Scholar]
  9. Vazquez H. et al. Probing the conductance superposition law in single-molecule circuits with parallel paths. Nat. Nanotechnol. 7, 663–667 (2012). [DOI] [PubMed] [Google Scholar]
  10. Li Z. et al. Towards graphyne molecular electronics. Nat. Commun. 6, 6321 (2015). [DOI] [PubMed] [Google Scholar]
  11. Xiang D., Wang X., Jia C., Lee T. & Guo X. Molecular-scale electronics: from concept to function. Chem. Rev. 116, 4318–4440 (2016). [DOI] [PubMed] [Google Scholar]
  12. Marqués-González S. & Low P. J. Molecular electronics: history and fundamentals. Aust. J. Chem. 69, 244–253 (2016). [Google Scholar]
  13. Aradhya S. V. & Venkataraman L. Single-molecule junctions beyond electronic transport. Nat. Nanotechnol. 8, 399–410 (2013). [DOI] [PubMed] [Google Scholar]
  14. Cremers J. et al. Nanorings with copper(II) and zinc(II) centers: forcing copper porphyrins to bind axial ligands in heterometallated oligomers. Chem. Sci. 7, 6961–6968 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
  15. Pardo E. et al. Long-range magnetic coupling through extended π-conjugated aromatic bridges in dinuclear copper(II) metallacyclophanes. J. Am. Chem. Soc. 125, 10770–10771 (2003). [DOI] [PubMed] [Google Scholar]
  16. Castellano M. et al. Dicopper(II) metallacyclophanes as multifunctional magnetic devices: a joint experimental and computational study. Acc. Chem. Res. 48, 510–520 (2015). [DOI] [PubMed] [Google Scholar]
  17. Jeschke G. Determination of the nanostructure of polymer materials by electron paramagnetic resonance spectroscopy. Macromol. Rapid. Commun. 23, 227–246 (2002). [Google Scholar]
  18. Jeschke G. & Polyhach Y. Distance measurements on spin-labelled biomacromolecules by pulsed electron paramagnetic resonance. Phys. Chem. Chem. Phys. 9, 1895–1910 (2007). [DOI] [PubMed] [Google Scholar]
  19. Jeschke G. DEER distance measurements on proteins. Annu. Rev. Phys. Chem. 63, 419–446 (2012). [DOI] [PubMed] [Google Scholar]
  20. Bode B. E., Plackmeyer J., Prisner T. F. & Schiemann O. PELDOR measurements on a nitroxide-labeled Cu(II) porphyrin: orientation selection, spin-density distribution, and conformational flexibility. J. Phys. Chem. A 112, 5064–5073 (2008). [DOI] [PubMed] [Google Scholar]
  21. Margraf D., Cekan P., Prisner T. F., Sigurdsson S. T. & Schiemann O. Ferro- and antiferromagnetic exchange coupling constants in PELDOR spectra. Phys. Chem. Chem. Phys. 11, 6708–6714 (2009). [DOI] [PubMed] [Google Scholar]
  22. Weber A., Schiemann O., Bode B. & Prisner T. F. PELDOR at S- and X-band frequencies and the separation of exchange coupling from dipolar coupling. J. Magn. Reson. 157, 277–285 (2002). [DOI] [PubMed] [Google Scholar]
  23. Reginsson G. W. et al. W-band PELDOR with 1 kW microwave power: molecular geometry, flexibility and exchange coupling. J. Magn. Reson. 216, 175–182 (2012). [DOI] [PubMed] [Google Scholar]
  24. Sprafke J. K. et al. Belt-shaped π-systems: relating geometry to electronic structure in a six-porphyrin nanoring. J. Am. Chem. Soc. 133, 17262–17273 (2011). [DOI] [PubMed] [Google Scholar]
  25. Wautelet P., Bieber A., Turek P., Le Moigne J. & André J.-J. Magnetic properties of iminonitroxide and nitronylnitroxide based diradicals. Mol. Cryst. Liq. Cryst. 305, 55–67 (1997). [Google Scholar]
  26. Nishizawa S., Hasegawa J. & Matsuda K. Theoretical investigation of the β-value of the π-conjugated molecular wires by evaluating exchange interaction between organic radicals. J. Phys. Chem. C 117, 26280–26286 (2013). [Google Scholar]
  27. Herrmann C. & Elmisz J. Electronic communication through molecular bridges. Chem. Commun. 49, 10456–10458 (2013). [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures, Supplementary Notes and Supplementary References

ncomms14842-s1.pdf (2.2MB, pdf)
Peer Review File
ncomms14842-s2.pdf (406.2KB, pdf)

Data Availability Statement

The data that support the findings of this study are available from the corresponding authors on reasonable request.

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES