Evolution of the early Antarctic ice ages

Significance

The Antarctic ice cap waxed and waned on astronomical time scales throughout the Oligo-Miocene time interval. We quantify geometries of Antarctic ice age cycles, as expressed in a new climate record from the South Atlantic Ocean, to track changing dynamics of the unipolar icehouse climate state. We document numerous ∼110-thousand-year-long oscillations between a near-fully glaciated and deglaciated Antarctica that transitioned from being symmetric in the Oligocene to asymmetric in the Miocene. We infer that distinctly asymmetric ice age cycles are not unique to the Late Pleistocene or to extremely large continental ice sheets. The patterns of long-term change in Antarctic climate interpreted from this record are not readily reconciled with existing CO₂ records.

Abstract

Understanding the stability of the early Antarctic ice cap in the geological past is of societal interest because present-day atmospheric CO₂ concentrations have reached values comparable to those estimated for the Oligocene and the Early Miocene epochs. Here we analyze a new high-resolution deep-sea oxygen isotope (δ¹⁸O) record from the South Atlantic Ocean spanning an interval between 30.1 My and 17.1 My ago. The record displays major oscillations in deep-sea temperature and Antarctic ice volume in response to the ∼110-ky eccentricity modulation of precession. Conservative minimum ice volume estimates show that waxing and waning of at least ∼85 to 110% of the volume of the present East Antarctic Ice Sheet is required to explain many of the ∼110-ky cycles. Antarctic ice sheets were typically largest during repeated glacial cycles of the mid-Oligocene (∼28.0 My to ∼26.3 My ago) and across the Oligocene−Miocene Transition (∼23.0 My ago). However, the high-amplitude glacial−interglacial cycles of the mid-Oligocene are highly symmetrical, indicating a more direct response to eccentricity modulation of precession than their Early Miocene counterparts, which are distinctly asymmetrical—indicative of prolonged ice buildup and delayed, but rapid, glacial terminations. We hypothesize that the long-term transition to a warmer climate state with sawtooth-shaped glacial cycles in the Early Miocene was brought about by subsidence and glacial erosion in West Antarctica during the Late Oligocene and/or a change in the variability of atmospheric CO₂ levels on astronomical time scales that is not yet captured in existing proxy reconstructions.

The early icehouse world of the Oligocene and Early Miocene epochs (hereafter referred to as Oligo-Miocene) is bracketed by two major climate events: the Eocene−Oligocene Climate Transition (∼34 My ago, EOT) and the onset of the Middle Miocene Climatic Optimum (∼17 My ago) (1). Deep-sea proxy records and sedimentological evidence from the Antarctic continental shelves indicate the expansion of continental-size ice sheets on Antarctica at the EOT (2, 3), and sedimentary records from the western Ross Sea on the East Antarctic margin document large subsequent oscillations in ice sheet extent on astronomical time scales during the Oligo-Miocene (4). In contrast, large ice sheets did not develop in the high northern latitudes until the Late Pliocene (5). Thus, the Oligo-Miocene presents an opportunity to study the dynamics of a unipolar (Antarctic) icehouse climate state without the overprint of Northern Hemisphere ice sheets on benthic foraminiferal δ¹⁸O records. Published proxy records of atmospheric CO₂ concentration show a decline from the Oligocene to the Miocene (6, 7) that is broadly contemporaneous with a strong minimum in the ∼2.4-My eccentricity cycle ∼24 My ago (8), which would promote continental ice sheet expansion if radiative forcing was the dominant control on ice volume. Previous studies using drill core records from the deep ocean demonstrate a climatic response to astronomical forcing for the Oligocene (9, 10) and parts of the Miocene (11–13). However, to improve understanding of the behavior of the climate/cryosphere system, we need longer high-resolution records from strategic locations that capture the changing response of the high latitudes to the combined effects of CO₂, astronomical forcing, and tectonic boundary conditions.

Walvis Ridge Ocean Drilling Program Site 1264

To shed light on southern high-latitude climate variability through the Oligo-Miocene, we analyze a new high-resolution benthic foraminiferal δ¹⁸O record from Walvis Ridge, located in the southeastern Atlantic Ocean [Ocean Drilling Program Site 1264; 2,505-m water depth; 2,000- to 2,200-m paleowater depth; 28.53°S, 2.85°E, Fig. 1 (14, 15)]. An astrochronology for Site 1264 was developed by tuning CaCO₃ estimates to the stable eccentricity solution independently of the benthic δ¹⁸O record (15). On the eccentricity-tuned age model, the Site 1264 record spans a 13-My time window between 30.1 My and 17.1 My ago and ranges between 405-ky Eccentricity Cycles 74 to 43 and ∼2.4-My Eccentricity Cycles 13 to 8 (Fig. 1) (15), representing the first continuous record from a single site spanning the mid-Oligocene to Early Miocene. Five distinct time intervals with clear multimillion-year climatic trends are identified in this new δ¹⁸O dataset from Walvis Ridge: (i) an Early Oligocene time interval of climate deterioration (∼30.1 My to 28.0 My ago); (ii) a generally cold but highly unstable mid-Oligocene time interval (∼28.0 My to 26.3 My ago), which we refer to as the Mid-Oligocene Glacial Interval (MOGI); (iii) a Late Oligocene time interval characterized by low-amplitude climate variability and stepwise climatic amelioration (∼26.3 My to 23.7 My ago), confirming that this warming trend is a real feature of Cenozoic climate history (9) rather than an artifact of composite records from multiple sites in different ocean basins; (iv) a time interval of persistently high-amplitude climate variability spanning the Oligocene−Miocene Transition (OMT) and the earliest Miocene (∼23.7 My to 20.4 My ago); and (v) a time interval of moderate-amplitude climate variability during the latter part of the Early Miocene (∼20.4 My to 17.1 My ago).

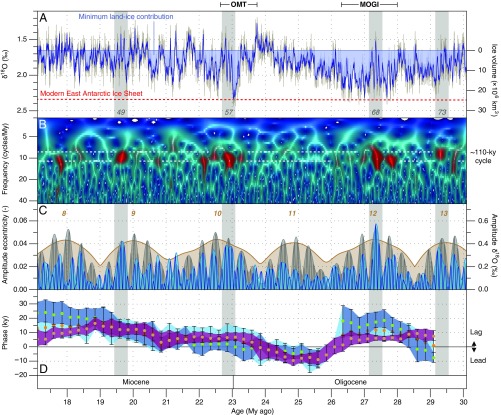
Fig. 1.

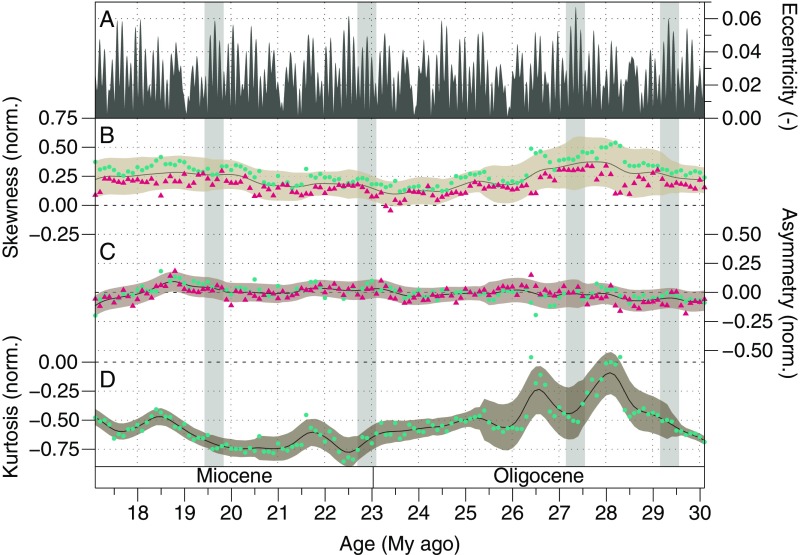
High-latitude climate−cryosphere evolution during the Oligo-Miocene and sinusoidal glacial−interglacial cycle properties. (A) Benthic foraminiferal (Cibicides mundulus) δ¹⁸O record from ODP Site 1264 (gray line) (15) and SiZer smooth (blue line; SI Methods). Minimum ice volume contribution (lilac area, right axis) to the benthic δ¹⁸O record is calculated relative to all values exceeding 1.65‰ (left axis; SI Methods). Dashed red line represents the contribution to benthic δ¹⁸O of a present-day-sized East Antarctic Ice Sheet (δ¹⁸O_ice = −42‰). (B–D) Sinusoidal glacial−interglacial cycle properties. (B) Wavelet analysis of the Site 1264 benthic δ¹⁸O record. White dashed lines represent the ∼95- and ∼125-ky eccentricity periodicities. (C) Filter of the Site 1264 benthic δ¹⁸O record centered around the ∼110-ky periodicity (dark blue line) and its amplitude modulation (light blue line and area), compared with those of eccentricity (gray lines and area). The filter values are proportional to the eccentricity (left axis) and the VPDB (Vienna Pee Dee Belemnite) scale (right axis). In the background (light brown line and area), the ∼2.4-My component of Earth’s orbital eccentricity is shown (+0.02 to aid visibility) and marked by brown bold italic cycle numbers. (D) Phase evolution of the ∼125-ky (dark blue area, green dots), ∼95-ky (purple area, brown dots), and combined (including intermediate frequencies) ∼110-ky (light blue area, orange dots) cycles to eccentricity, which are independant from one other. Vertical gray bars represent 405-ky Eccentricity Cycles 49, 57, 68, and 73 (dark gray italic numbers), characterized by exceptionally strong ∼110-ky responses in benthic δ¹⁸O (Fig. 3 B–E) (15).

Following the MOGI, the Late Oligocene warming phase proceeded in a series of three distinct steps (∼26.3, ∼25.5, and ∼24.2 My ago), with the peak warming/lowest ice volume confined to a ∼500-ky period (∼24.2 My to 23.7 My ago). This climate state was terminated by the OMT (∼23.7 My to 22.7 My ago), which consists of two rapid ∼0.5‰ increases in benthic δ¹⁸O that are separated by an interval (405-ky eccentricity cycle long) of partial δ¹⁸O recovery (15). The onset of the OMT is thereby comparable in structure to the EOT (3). A 405-ky-long overall decrease in benthic δ¹⁸O marks the recovery phase of the OMT.

Ice Volume Estimates

To better understand the significance of the documented δ¹⁸O variability on long-term change in the high-latitude climate system, we make a conservative estimate of the minimum contribution of continental ice volume to the Site 1264 benthic δ¹⁸O signal by assuming that Oligo-Miocene bottom-water temperatures at Site 1264 were never colder than the current temperature of 2.5 °C and applying an average δ¹⁸O composition of Oligo-Miocene ice sheets (δ¹⁸O_ice) of −42‰ Vienna standard mean ocean water (VSMOW) (SI Methods) (16). These minimum ice volume estimates (Fig. 1) do not fully account for the changing relative contributions of ice volume and deep-sea temperature to the benthic δ¹⁸O signal over glacial−interglacial cycles. However, they are largely consistent with estimates of glacioeustatic sea level change from the New Jersey shelf (17) and those generated by inverse models of (multisite composite) δ¹⁸O records (12, 18). These ice volume estimates and sea level reconstructions strongly suggest that a very large part of the benthic δ¹⁸O signal is linked to large ice volume changes on Antarctica.

Three major results stand out in the minimum ice volume calculations on the Site 1264 benthic δ¹⁸O record (Fig. 1A). First, excluding the OMT interval, the Oligocene glacials are characterized by larger continental ice sheet volumes than those of the Early Miocene, particularly during the MOGI. Second, across the OMT, Antarctica transitioned from a climate state that was near-fully deglaciated to one characterized by an ice sheet as large as the present East Antarctic Ice Sheet and back into a near-fully deglaciated state in less than 1 My. Third, many glacial−interglacial cycles in the benthic δ¹⁸O record are associated with a δ¹⁸O_sw change of at least ∼0.60 to 0.75‰, requiring the waxing and waning of ∼21−26 × 10⁶ km³ of ice, or ∼85 to 110% of present East Antarctic ice volume, on timescales of ≤110 ky.

SI Methods

All data reported in this paper are available online. Go to www.pangaea.de and search for ref. 15, or follow the link https://doi.pangaea.de/10.1594/PANGAEA.862589.

Ice Volume Calculations.

To obtain conservative minimum estimates of continental ice volume (36) across the Oligo-Miocene study interval, we calculated a Cibicides δ¹⁸O (δ¹⁸O_Cib) value from equation 9 of ref. 37 using (i) the modern Site 1264 bottom-water temperatures of ∼2.5 °C (38) and (ii) an ice-free seawater δ¹⁸O (δ¹⁸O_sw) value of −1.05‰ VSMOW (39, 40). This gives a δ¹⁸O_Cib value of 1.65‰ VPDB (Vienna Pee Dee Belemnite), indicating that values of ≥1.65‰ reflect a change in δ¹⁸O_sw and, hence, a contribution from land ice to the δ¹⁸O_Cib signal, presuming that Oligo-Miocene deep-water temperatures at Site 1264 never cooled below modern-day temperatures. To estimate the minimum Oligo-Miocene land ice volumes, we applied an ice-free ocean volume of ∼1.3574 × 10⁹ km³ (34, 41, 42) and used a modeled average δ¹⁸O value of Oligo-Miocene ice sheets of −42‰ VSMOW (16), which yields ∼3.8 million km³ of ice per 0.1‰ change in seawater δ¹⁸O. This approach does not account for decreasing δ¹⁸O_ice through the glacial cycle as the ice sheet becomes larger and higher in elevation. However, state-of-the-art ice sheet models show that, once a large Antarctic ice sheet is established, the average δ¹⁸O_ice must have been quite low [−39 to −48‰ (25)]. If we assume that δ¹⁸O_ice was higher than −40‰, calculated ice volumes are unrealistically large. These ice volume estimates are calculated to show that the ice volume component of the benthic δ¹⁸O record must have been large and that the sinusoidal and nonsinusoidal cycle properties that we quantify are most likely related to Antarctic ice sheet dynamics. We note that the cycle properties, or their long-term evolution, may partially reflect changing relative contributions of temperature and ice volume to benthic δ¹⁸O. Both the Gaussian and the non-Gaussian statistics are applied to the benthic δ¹⁸O record and are thus independent from the exact ice volume contribution to benthic δ¹⁸O.

Sinusoidal Cycle Properties.

All data were resampled at 2.5 ky and, for CaCO₃ estimates (est.) and δ¹⁸O, periodicities of >1 My were removed using a notch filter (frequency = 0.0 cycles per million years, bandwidth = 1.0 cycle per million years) before statistical analyses (43). To calculate phases and nonsinusoidal cycle shapes between 18.1 My and 17.1 My ago, the CaCO₃ est. and δ¹⁸O data from ref. 44 between 17.1 My and 16.1 My ago were used, as 2-My windows were required to perform these statistical analyses. The time-frequency transforms of δ¹⁸O were computed using an adaptation of a wavelet script (Fig. 1B and Figs. S1 and S8D) (15), and, for the wavelet analysis only, periodicities greater than 200 ky were removed from the δ¹⁸O record using a notch filter (43). Gaussian filtering of the ∼110-ky component of the δ¹⁸O record and a Hilbert transform of the filtered data were calculated to compute the ∼110-ky amplitude modulation (frequencies between 6.4 cycles per million years and 12.4 cycles per million years for Fig. 1C; frequency = 9.4 cycles per million years, bandwidth = 3.0 cycles per million years for Fig. S8E). Phase calculations of CaCO₃ est. and δ¹⁸O relative to eccentricity were calculated across 2-My windows with 250-ky time steps through Blackman−Tukey cross-spectral analysis (Fig. 1 and Figs. S2 and S3) (43). Linear trends were removed, the data were prewhitened and normalized, and 95% confidence levels on the error bars were computed. This procedure resulted in a frequency resolution of 0.1 cycles per million years. The frequency bandwidths of 2.2 to 2.7, 7.4 to 10.8, 7.4 to 8.3, and 9.8 to 10.8 cycles per million years were used to compute phases for the 405-, ∼110-, ∼125-, and ∼95-ky components, respectively. When coherent, the maximum, average, and minimum values were selected within these frequency bandwidths to yield phases and their 95% error estimates. Phase estimates are not depicted if none of the frequencies within a bandwidth was coherent. Smooths were taken of the benthic δ¹⁸O record, the atmospheric CO₂ data (6, 7), and the nonsinusoidal cycle properties using SiZer (significant zero crossings of derivatives), a statistical method that extracts the structures in curves (Figs. 1 and 3 and Figs. S6–S8) (45).

Fig. S1.

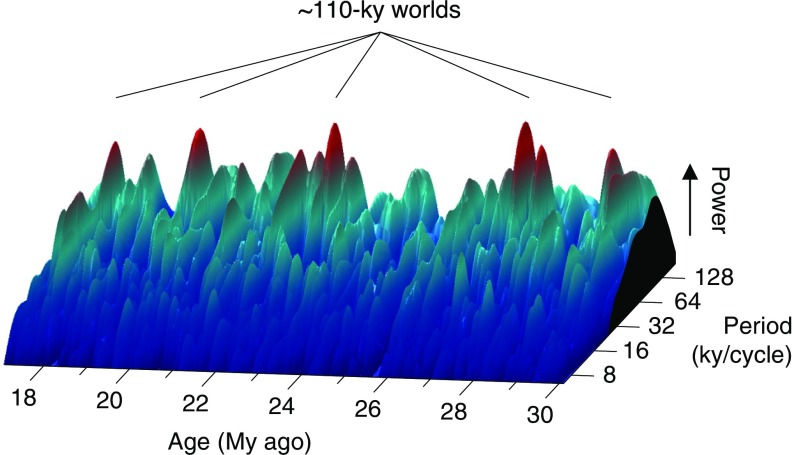
A 3D wavelet of δ¹⁸O. Wavelet analysis of the Site 1264 benthic δ¹⁸O record (21). Frequencies lower than five cycles per million years (i.e., periodicities higher than 200 ky per cycle) have been removed to emphasize the power on the ∼10 cycles per million years frequency (∼110-ky periodicity).

Fig. S8.

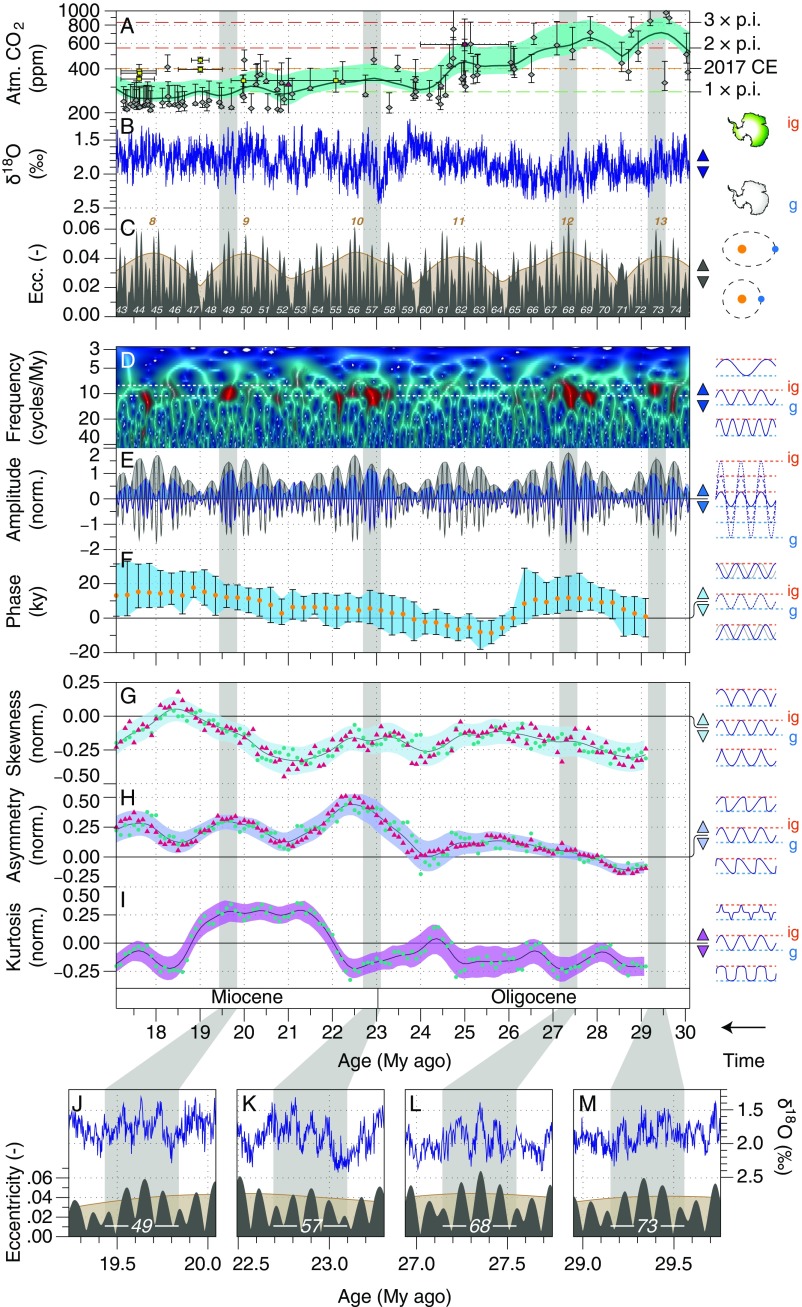
Sinusoidal and nonsinusoidal cycle properties of benthic δ¹⁸O. (A) Atmospheric CO₂ proxy estimates for the Oligo-Miocene and their long-term smooths (turquoise line and area; see SI Methods) through the reconstructed values and their maximum and minimum error estimates (black error bars). Gray diamonds represent phytoplankton CO₂ estimates, yellow squares are based on stomata, and purple-red triangles represent CO₂ estimates based on paleosols (6, 7). Multiplication factors on the right refer to preindustrial (p.i.) CO₂ concentrations of 278 ppm. CE, Common Era. (B) Benthic foraminiferal δ¹⁸O record from Site 1264, Walvis Ridge (15). (C) Earth’s orbital eccentricity (8) and its ∼2.4-My component (+0.02 to aid visibility) marked by brown bold italic cycle numbers. (D–F) Sinusoidal cycle properties. (D) Wavelet analysis of the Site 1264 benthic δ¹⁸O record. Frequencies lower than five cycles per million years (i.e., periodicities higher than 200 ky per cycle) have been removed to emphasize the power on the ∼10 cycles per million years frequency (∼110-ky periodicity). (E) Gaussian filters (lines) and amplitude modulations (areas) of the combined ∼95 ky and ∼125 ky periodicities (centered around ∼110 ky) of the eccentricity solution (gray) and detrended benthic δ¹⁸O data (blue). (F) Blackman−Tukey phase calculations across a 2-My sliding window (step size 0.25 My). The 95% significance estimates are indicated. (G–I) Nonsinusoidal cycle properties. (G) Skewness, (H) asymmetry, and (I) kurtosis, calculated using standard (turquoise circles) and bispectral methods (purple-pink triangles). Corresponding cycle shapes are indicated on the right; ig, interglacial; g, glacial. Background areas indicate the 2σ upper and lower ranges of these nonsinusoidal cycle properties. (J−M) Four recurrent intervals during the Oligo-Miocene characterized by high-amplitude ∼110-ky cyclicity in benthic δ¹⁸O (dark blue lines), compared with eccentricity (gray areas) and its ∼2.4-My component (light brown areas). (J) The Early Oligocene, contemporaneous with 405-ky Eccentricity Cycle 73. (K) The mid-Oligocene, contemporaneous with Cycle 68. (L) The Oligo-Miocene transition, contemporaneous with Cycle 57. (M) The Early Miocene, contemporaneous with Cycle 49. White numbers correspond to 405-ky eccentricity cycles. To the right of B−I, the Antarctic ice sheet (freevectormaps.com) and eccentricity conditions are suggested, and corresponding cycle shapes are depicted. Arrow indicates the direction of time. Vertical gray bars are as in Fig. 1.

Fig. S2.

Phase evolution of CaCO₃ est. with respect to eccentricity. (A) Phase evolution of the 405-ky cycle in the Site 1264 CaCO₃ est. record to that of eccentricity. (B) Phase evolution of the ∼110-ky cycle to eccentricity. (C) Phase evolutions of the ∼125- and ∼95-ky cycles to eccentricity. The ∼110-ky cycle of CaCO₃ est. (B) is continuously coherent and in phase within the 95% confidence level (i.e., ± 5 ky of in phase) with eccentricity, consistent with the tuning assumptions that have been used (15). All further phase calculations (A and C and Fig. S3) are derived from this phase assumption. Error bars represent the 95% Blackman−Tukey cross-spectral analysis confidence intervals. Phase calculations are only shown when coherent. Vertical gray bars are as in Fig. 1.

Fig. S3.

Phase evolution of δ¹⁸O with respect to eccentricity. (A) Phase evolution of the 405-ky cycle in the Site 1264 benthic δ¹⁸O record to that of eccentricity. (B) Phase evolution of the ∼110-ky cycle to eccentricity. (C) Phase evolutions of the ∼125- and ∼95-ky cycles to eccentricity, which show independent evolutions. Error bars represent the 95% Blackman−Tukey cross-spectral analysis confidence intervals. Phase calculations are only shown when coherent. Vertical gray bars are as in Fig. 1.

Fig. 3.

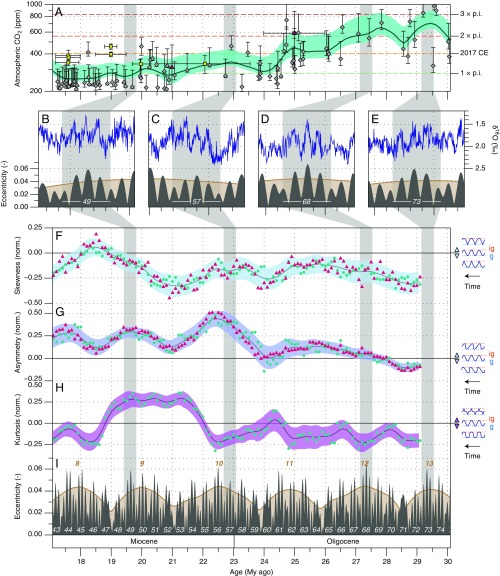
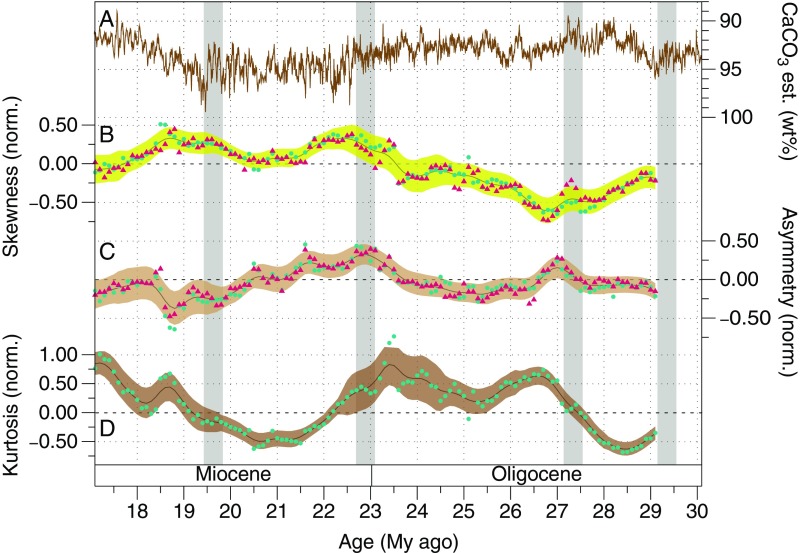
Nonsinusoidal glacial−interglacial cycle properties. (A) Atmospheric CO₂ proxy estimates for the Oligo-Miocene and their long-term smooths (turquoise line and area; SI Methods) through the reconstructed values and their maximum and minimum error estimates (black error bars). Gray diamonds represent phytoplankton CO₂ estimates, yellow squares are based on stomata, and purple-red triangles represent CO₂ estimates based on paleosols (6, 7). Multiplication factors on the right refer to preindustrial (p.i.) CO₂ concentrations of 278 ppm. CE, Common Era. (B−E) Four 405-ky-long intervals with exceptionally strong ∼110-ky cycles in benthic δ¹⁸O, plotted against eccentricity and its ∼2.4-My component (+0.02 to aid visibility). These intervals occur during (B) the Early Miocene, contemporaneous with 405-ky Eccentricity Cycle 49; (C) the Oligo-Miocene transition, Cycle 57; (D) the mid-Oligocene, Cycle 68; and (E) the Early Oligocene, Cycle 73 (white italic numbers). For B−E only, long ticks on the age axis indicate 500-ky steps, and short ticks indicate 100-ky steps. (F–H) Nonsinusoidal glacial−interglacial cycle properties. (F) Skewness, (G) asymmetry, and (H) kurtosis of the Site 1264 benthic δ¹⁸O record quantified over a 2-My-long sliding window using standard (turquoise circles) and bispectral (purple-pink triangles) methods (SI Methods). The colored areas indicate the 2σ upper and lower ranges of the cycle geometries. (I) Earth’s orbital eccentricity (8) and its ∼2.4-My component (+0.02 to aid visibility) marked by brown bold italic cycle numbers. Vertical gray bars are as in Fig. 1. To the right of F−H, the corresponding cycle shapes are depicted, and the direction of time is indicated; ig, interglacial; g, glacial.

Fig. S6.

Nonsinusoidal cycle properties of eccentricity. (A) Orbital eccentricity (8), and (B) its skewness, (C) asymmetry, and (D) kurtosis, calculated across a 2-My sliding window using standard (turquoise circles) and bispectral methods (purple-pink triangles). An unexplained, small offset in skewness (A) is observed between values calculated using the standard and bispectral methods. Vertical gray bars are as in Fig. 1.

Bispectral Analysis.

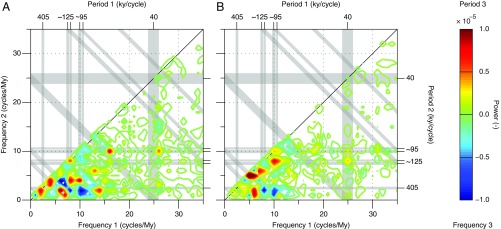
The bispectrum assesses coupling and energy transfers between frequencies within a single time series. The bispectrum is defined (26) as B_{f1, f2} = E[A_f1 A_f2 A*_{f1 + f2}], where E[] is the ensemble average of the triple product of complex Fourier coefficients A at the frequencies f₁, f₂, and their sum f₁ + f₂, and the asterisk indicates complex conjugation. The imaginary part of the bispectrum is linked to energy transfers (46) and is therefore shown in Fig. 2 and Fig. S4. Oligo-Miocene bispectral settings were as follows: resampling resolution = 2.5 ky, window length = 2 My, step-size = 0.1 My, blocks = 8, block length = 1 My, degrees of freedom = 16, and frequency resolution = 0.001 cycles per kiloyear (Fig. 2 and Figs. S4 and S6–S8). Plio-Pleistocene bispectra were calculated to extract geometries, applying the following settings (after refs. 27 and 28): resampling resolution = 2.5 ky, window length = 1 My, step size = 0.1 My, no blocks, degrees of freedom = 2, and frequency resolution = 0.001 cycles per kiloyear (Fig. S5). The colors in the bispectral plots (Fig. 2 and Fig. S4) range from 1 × 10⁻⁵ to −1 × 10⁻⁵. Rare values exceeding this range were set to match these maximum and minimum values to scale the color gradient to the part of the bispectrum where dominant interactions occur. In addition to couplings near eccentricity frequencies (Bispectral Analysis in the main text; f_ecc. = 2.5 and ∼10.0 cycles per million years), we observe some couplings between eccentricity and obliquity (f_obl. = 25.0 cycles per million years), which are indicated by, for example, the positive interactions at B(25.0, 8.0) cycles per million years in the mid-Oligocene and B(25.0, 10.5) cycles per million years in the OMT interval, where energy is transferred to f₃ = 33.0 and 35.5 cycles per million years (∼29 ky per cycle), respectively. Precession (f_prec. ≈ 50.0 cycles per million years) and obliquity are poorly expressed in the benthic δ¹⁸O record of Site 1264 (15), which may explain their weaker definition in interactions (Fig. S4).

Fig. 2.

Bispectra assessing phase coupling and energy transfers between frequencies in the δ¹⁸O data. Bispectral analyses on benthic δ¹⁸O across two, 2-My-long windows with strong ∼110-ky cycles (see also Fig. S4). (A) Bispectrum across the OMT interval, during ∼2.4-My Eccentricity Cycle 10 (23.54 My to 21.54 My ago). (B) Bispectrum across the MOGI, during ∼2.4-My Eccentricity Cycle 12 (28.30 My to 26.30 My ago). The colors of the bispectrum show the direction of the energy transfers. The intensity of the colors is indicative of the magnitude of energy transfers (SI Methods). Red indicates a transfer of spectral power from two frequencies, f₁ (see x axes) and f₂ (see y axes), to frequency f₃ (f₁ + f₂ = f₃). In contrast, blue represents a gain of spectral power at frequencies f₁ and f₂ from frequency f₃. Gray lines reflect the main astronomical frequencies of eccentricity, obliquity, and precession.

Fig. S4.

Bispectra assessing phase coupling and energy transfers between frequencies in the δ¹⁸O data. Bispectral results over three 2-My-long intervals that correspond to (A) ∼2.4-My Eccentricity Cycle 9 (21.10 My to 19.10 My ago, (B) Cycle 10 (23.54 My to 21.54 My ago), and (C) Cycle 12 (28.30 My to 26.30 My ago; see SI Methods). Gray lines reflect the main astronomical frequencies of eccentricity, obliquity, and precession. The two panels of Fig. 2 are reproduced here (B and C) and expanded to include the interactions with the precession frequencies.

Fig. S5.

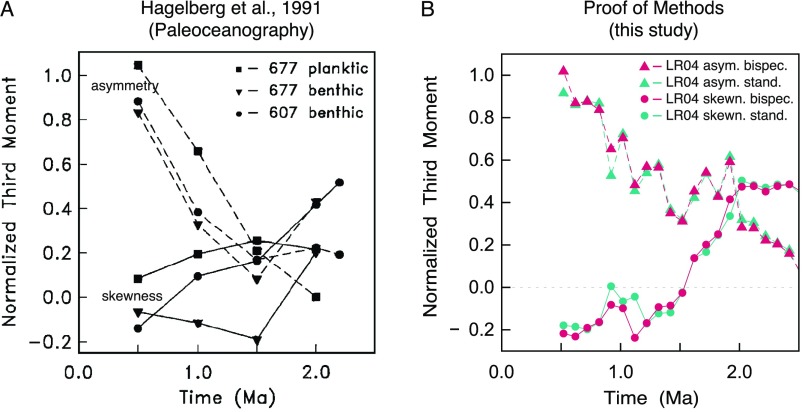
Proof of methods in quantifying nonsinusoidal cycle properties. (A) Original skewness and asymmetry calculations on Plio-Pleistocene benthic and planktic δ¹⁸O records. Reprinted from ref. 27. (B) Reproducing the results of ref. 27 on the Plio-Pleistocene LR04 benthic δ¹⁸O stack (54). Comparable results have been obtained using a different method (32). Triangles show asymmetry, and circles show skewness. Turquoise indicates the standard method, and purple-pink represents the bispectral method. Time (Ma) equates to Age (My ago).

Nonsinusoidal Cycle Properties.

Quantifying deviations from sinusoidality provides an objective way to describe cycle shapes (27, 28) or waveforms (47). Walvis Ridge Site 1264 was tuned using one tie point every ∼125 ky on average (15), and the cycle shapes of individual ∼110-ky cycles are therefore unaffected by the tuning approach. We calculate skewness, asymmetry, and kurtosis of eccentricity, CaCO₃ est., and benthic δ¹⁸O cycles across a 2-My sliding window (step size 0.1 My) to track the evolving geometry of the cycles with the highest variance (i.e., the ∼110-ky cycles). This 2-My sliding window smooths the signal and may explain the gradual onset of asymmetry already at 24 My ago. We note that nonsinusoidal cycle properties are not frequency-specific, as harmonics between multiple frequencies are needed to distort a sine-shaped cycle. They can, however, be attributed to frequency bandwidths (48). Skewness and asymmetry are quantified using both standard and bispectral methods (47), to ascertain the reproducibility of the outcome. Kurtosis is quantified using the standard method only, as no trispectra were calculated. The 2σ upper and lower boundary error ranges, calculated using a 2-My sliding window, were added to the long-term SiZer smooths of the (combined) geometry quantifications. Skewness is determined (49) as $Sk\left(x\right)=\langle {\left(x-\overline{x}\right)}^3\rangle /{\langle {\left(x-\overline{x}\right)}^2\rangle }^{3/2}$, where the overbar indicates the mean value, and where < > is the time averaging operator. Asymmetry is determined (50) as $As\left(x\right)=\langle H^3\left(x-\overline{x}\right)\rangle /{\langle {\left(x-\overline{x}\right)}^2\rangle }^{3/2}$, where H is the Hilbert transform. Kurtosis is defined (51) as $k\left(x\right)=\left[\langle {\left(x-\overline{x}\right)}^4\rangle /{\langle {\left(x-\overline{x}\right)}^2\rangle }^2\right]-3$. We extract skewness and asymmetry from the bispectrum following equation 3 of ref. 47, $Sk\left(x\right)+iAs\left(x\right)=\hspace{0.17em}\left[12{\displaystyle {\sum }_n{\displaystyle {\sum }_{l}B\left(f_n{f}_l\right)}}+6{\displaystyle {\sum }_{p=1}^{\frac{N}{2}}B\left(f_p,f_p\right)}\right]/E{\langle {\left(x-\overline{x}\right)}^2\rangle }^{3/2}$, where n and l range from 1 to N (at the Nyquist frequency), with n > l and n+l $\le N$. We note that not many studies since the pioneering work of refs. 27 and 28 on the Late Pleistocene records, more recently reproduced using different statistical methods (32), have quantified nonsinusoidal glacial−interglacial cycle properties. Most cyclostratigraphic studies have not commented on the nonsinusoidality of climate cycles or described these properties qualitatively.

Sinusoidal Glacial−Interglacial Cycle Properties

The 13 My-long Oligo-Miocene benthic δ¹⁸O record from Site 1264 shows distinct cyclicity on astronomical time scales. Wavelet analysis reveals (Fig. 1 and Fig. S1) (15) that the amplitude of variability at the ∼110-ky eccentricity periodicity is particularly pronounced (≥1.0‰ across the larger δ¹⁸O cycles). The amplitude of the 40-ky obliquity periodicity is subdued in comparison with published records from other sites, presumably because of the higher sedimentation rates at those sites (13, 19). Four relatively short (405 ky long) intervals with particularly strong ∼110-ky-paced δ¹⁸O variability are also identified in the record (vertical gray bars, Fig. 1), demonstrating a pronounced climate−cryosphere response to eccentricity-modulated precession of Earth’s spin axis (15). These intervals are contemporaneous with 405-ky eccentricity maxima during ∼2.4-My eccentricity maxima, specifically 405-ky Cycles 73, 68, 57, and 49. Thus, although the OMT deserves its status as a major transient Cenozoic event (1, 20) because it is a prominent but transient glacial episode that abruptly terminates Late Oligocene warming, the amplitude of ice age cycles observed as the climate system emerges from peak glacial OMT conditions is not unique in the Oligo-Miocene. In fact, this recovery phase of the OMT is one of four Oligo-Miocene intervals characterized by particularly high-amplitude ∼110-ky oscillations between glacial and interglacial Antarctic conditions (Fig. 1A). The record from Site 1264 is the first to unequivocally show that the ∼2.4-My eccentricity cycle paces recurrent episodes of high-amplitude ∼110-ky variability in benthic δ¹⁸O (9, 19) and provides a new global climatic context in which to understand Oligo-Miocene glacial history, carbon cycling (9, 21), midlatitude terrestrial water balance (22), and mammal turnover rates (23) that show similar pacing. The intervals with particularly strong ∼110-ky cycles are separated by prolonged periods of attenuated ∼110-ky cycle amplitude, indicating that not all ∼2.4-My and 405-ky eccentricity maxima trigger similar cryospheric responses (Fig. 1). Specifically, ∼2.4-My Eccentricity Cycle 11 in the Late Oligocene is not characterized by high-amplitude ∼110-ky cycles (Fig. 1). Furthermore, no consistent relationship is found between strong ∼110-ky cycles in benthic δ¹⁸O and the ∼1.2-My amplitude modulation of obliquity (15). This inconsistency suggests that some other factor or combination of factors is responsible for the changing response of the climate system to astronomical forcing on ∼110-ky time scales over the Oligo-Miocene.

We assess the phase relationships of the tuned δ¹⁸O data with respect to the main frequencies of orbital eccentricity to track the response times of the Oligo-Miocene climate system (Fig. 1 and Figs. S2 and S3). The benthic δ¹⁸O record from Site 1264 displays a marked multimillion-year evolution in the phasing of the ∼110-ky cycle relative to eccentricity, starting with a ∼10-ky phase lag during the mid-Oligocene, followed by an unstable phase relation at ∼26 My ago and a steady increase in phase that culminates in a 10- to 15-ky lag at ∼19.0 My ago (Fig. S3). The ∼95- and ∼125-ky frequencies show largely independent phase evolutions. On the basis of these data alone, we cannot rule out the possibility that part of the observed structure in the long-term phase evolution arises from changes in the proportional contribution of temperature and ice volume to benthic δ¹⁸O (24). However, the observed changes in phase are so large (approximately –10 ky to +15 ky) that changes in the response time of Antarctic ice sheets are most likely responsible; large continental ice sheets are the slowest-responding physical component of Earth’s climate system and the only mechanism capable of inducing phase lags in deep-sea benthic δ¹⁸O records of ∼10 ky to 15 ky (25). Analysis of phasing suggests that, over full glacial−interglacial cycles, the high-latitude climate–Antarctic ice sheet system responded more slowly to astronomical pacing during the MOGI (∼28.0 My to 26.3 My ago) and Early Miocene (≲23 My ago) than during either the Early Oligocene (∼30.1 My to 28.0 My ago) or Late Oligocene (∼26.3 My to 23.7 My ago).

Bispectral Analysis

To investigate phase coupling between (astronomical) cycles embedded in the Site 1264 benthic δ¹⁸O record, we apply bispectral techniques (26–28). A bispectrum identifies phase couplings between three frequencies: f₁, f₂, and their sum frequency f₁ + f₂ = f₃. When phase-coupled, energy transfers nonlinearly between these frequencies and is redistributed over the spectrum. This transfer of spectral energy results in lower and higher harmonics and in the formation of skewed and/or asymmetric cycle geometries such as those observed in the δ¹⁸O record. We compare bispectra for two selected time intervals with strong ∼110-ky cyclicity (Fig. 2): a mid-Oligocene interval (including the MOGI), during ∼2.4-My Eccentricity Cycle 12 (28.30 My to 26.30 My ago), and an OMT-spanning interval, during ∼2.4-My Eccentricity Cycle 10 (23.54 My to 21.54 My ago). A third, Early Miocene example is considered in Fig. S4. The bispectra show that, during both the MOGI and the OMT, numerous phase couplings occur with frequencies that include, but are not limited to, astronomical cycles. Most interactions occur between cycles with periodicities close to those of eccentricity (periods of 405, ∼125, and ∼95 ky per cycle, equal to frequencies of 2.5, 8.0, and 10.5 cycles per million years, respectively) that exchange energy among themselves and also with higher frequencies. The close proximity of both positive and negative interactions around eccentricity frequencies (Fig. 2 and Fig. S4) suggests that these frequencies redistribute energy by broadening spectral peaks in δ¹⁸O. This process may explain the observed ∼200-ky cycle (15). The main difference between the two selected time intervals is that the OMT bispectrum reveals many more nonlinear interactions (Fig. 2), both positive and negative, which indicates that the climate−cryosphere system responded in a more complex and indirect manner to insolation forcing across the OMT than during the MOGI. This observation may point to the activation of heightened positive feedback mechanisms across the OMT related to continental ice sheet growth and decay (13, 29), possibly involving the carbon cycle (30) or Antarctic sea ice (31).

Nonsinusoidal Glacial−Interglacial Cycle Properties

To further understand the nonlinearity in the climate system documented by the bispectra, we assess nonsinusoidal (i.e., non-Gaussian) cycle properties (Fig. 3 and Figs. S5–S8; see also SI Exploring Potential Cycle Shape Distortion). Nonlinearity in climate cycles can be quantified in terms of skewness, asymmetry, and kurtosis using standard and higher-order spectral analyses to elucidate the rapidity of climatic transitions (SI Methods). The remarkably consistent negative skewness in the δ¹⁸O record (mean −0.18; Fig. 3 and Fig. S8) indicates that Oligo-Miocene glacials were longer in duration than interglacials—a result that is consistent with the Late Pleistocene record (Fig. S5) (27, 28, 32). To assess the time spent per cycle in full glacial and full interglacial conditions (in contrast to skewness which records the duration of glacials versus interglacials), we also calculate the evolution of cycle kurtosis through the benthic δ¹⁸O record. Square-waved (platykurtic) glacial−interglacial cycles are more evident in the Site 1264 record than thin-peaked (leptokurtic) ones, apart from an Early Miocene interval between ∼21.5 My and 19.0 My ago when leptokurtic cycles prevail (Fig. 3 and Fig. S8). This observation indicates that the Oligo-Miocene climate system generally favored full glacial and full interglacial conditions and transitioned rapidly between those two climate states. We attribute this finding to the operation of well-documented strong positive feedbacks on ice sheet growth and decay (25, 29).

To understand the relative rates of ice sheet growth versus decay, we quantify cycle asymmetry. Although the Site 1264 record shows consistently skewed Oligo-Miocene ∼110-ky glacial−interglacial cycles, we document a major change over time in the symmetry of those cycles that is marked by a transition to more asymmetric cycles that began ∼23 My ago at the OMT. This change represents a shift to a new climatic state characterized by a ∼2.4-My pacing of glacial−interglacial asymmetry and is associated with lower atmospheric CO₂ levels (Fig. 3) (6, 7). Asymmetry in the data series is particularly pronounced during 405-ky Eccentricity Cycles 57 and 49 (at ∼22.7 and 19.5 My ago), which are characterized by distinctly sawtooth-shaped ∼110-ky cycles, suggesting a causal link between cycle amplitude and asymmetry during the Early Miocene, but not during the MOGI. The distinctly asymmetric cycles suggest that the Early Miocene Antarctic ice sheets periodically underwent intervals of growth that were prolonged relative to astronomical forcing and then underwent subsequent rapid retreat in a manner akin to the glacial terminations of the Late Pleistocene glaciations, in which the large ice sheets of the Northern Hemisphere were major participants (27, 28, 32). The highly asymmetric (sawtooth) nature of Late Pleistocene glacial−interglacial cycles is thought to originate from a positive ice mass balance that persists through several precession- and obliquity-paced summer insolation maxima. This results in decreasing ice sheet stability and more rapid terminations every ∼110 ky, once the ablation of the Northern Hemisphere ice sheets increases dramatically in response to the next insolation maximum. The increase in ablation is caused by lowered surface elevation of the ice sheets resulting from crustal sinking and delayed isostatic rebound (33). Similar mechanisms are implied for the large Antarctic ice sheets of the OMT (∼22.5 My ago) but it is less clear why the smaller ice sheets of the Early Miocene (∼19.5 My ago) would exhibit this distinctly sawtooth-shaped pattern of growth and decay (Fig. 3).

Climate–Cryosphere Evolution

Analysis of the new δ¹⁸O record from Site 1264 raises two important questions: (i) Why did Antarctic ice sheets decrease in size after the OMT? (ii) Why was hysteresis (i.e., glacial−interglacial asymmetry) apparently stronger for both the large OMT and the smaller Early Miocene ice sheets than for the large ice sheets of the Oligocene? One explanation for the long-term change in ice volume is that the large glacial ice volumes of the MOGI were possible because of higher topography in West Antarctica (34) that permitted formation of a large terrestrial ice sheet that also buttressed growth of ice sheets on East Antarctica (25, 35). In this interpretation, tectonic subsidence and glacial erosion during the Late Oligocene caused a shift to a smaller marine-based ice sheet in West Antarctica (25, 35), which limited the maximum size of the Early Miocene Antarctic ice sheets during peak glacial intervals.

The Early Miocene ice sheets may have been less responsive to astronomically paced changes in radiative forcing because of colder polar temperatures under lower CO₂ conditions from ∼24 My ago onward (7) or restriction of ice sheets to regions of East Antarctica above sea level following the Late Oligocene subsidence of West Antarctica (25, 35). Another possibility is that the large ice sheets that characterized the peak glacials of the MOGI underwent rapid major growth and decay because of higher-amplitude glacial−interglacial CO₂ changes than during the Early Miocene. Such hypothesized high-amplitude changes in CO₂ would have had a direct effect on radiative forcing, which, in turn, would have caused faster feedbacks and a more linear response to eccentricity modulation of precession. Given that larger ice volumes are to be expected in a climatic state that is characterized by high cycle asymmetry and low atmospheric CO₂ concentration, a third possibility is that the conservative calculations substantially underestimate true ice volumes for the Early Miocene. Each of these hypotheses can be tested through a combination of scientific drilling on the West Antarctic shelf margin and development of high-resolution CO₂ and marine temperature proxy records with astronomical age control. We predict that strong eccentricity-driven CO₂ cycles (∼110, 405, and ∼2,400 ky) that are closely in step with ice volume changes will emerge in proxy CO₂ reconstructions for the Oligo-Miocene time interval. Assuming that changes in partitioning of the benthic δ¹⁸O signal between temperature and ice volume are modest throughout the Oligo-Miocene, the deep-sea δ¹⁸O record from Site 1264 suggests a clear long-term shift from a more glacial Oligocene to a less glacial Early Miocene climate state—a pattern of change not readily reconciled with the long-term decrease in published CO₂ records.

SI Exploring Potential Cycle Shape Distortion

A number of processes may act to distort the geometry of a glacial−interglacial signal recorded in marine sediments (52). To test for cycle shape distortion in the stratigraphic domain caused by, e.g., coring disturbances and/or (cyclic) changes in sedimentation rates, we computed the nonsinusoidal cycle properties of the X-ray fluorescence core scanning-derived CaCO₃ est. tuning signal curve (15) and compared these results to the nonsinusoidal cycle properties of the benthic δ¹⁸O record to evaluate whether the geometries in each of these records are independent from each other. Both the CaCO₃ est. and δ¹⁸O records reveal strong cyclicity on eccentricity periodicities, and therefore we also compare their geometries to those of the eccentricity tuning target curve (8).

We note that the eccentricity solution, analyzed over a 2-My sliding window, has positive skewness, no asymmetry, and (overall) strong negative kurtosis (Fig. S6). Positive skewness for eccentricity over this window length is a counterintuitive result because individual ∼110-ky cycles are characterized by clear negative skewness. The CaCO₃ est. record shows an interval between ∼24 My and 18 My ago with positive skewness, which is preceded and followed by intervals between ∼30 My to 24 My ago and 18 My to 17 My ago with negative skewness (Fig. S7). Asymmetry of CaCO₃ est. does not show significant trends or offsets from zero. Kurtosis of CaCO₃ est. indicates mostly leptokurtic cycle shapes. The benthic δ¹⁸O record from Site 1264, also analyzed over 2-My long windows, has very comparable skewness to eccentricity (Fig. S8). However, asymmetry and kurtosis show long-term trends independent from eccentricity. Leptokurtic cycles in CaCO₃ strongly contrast the platykurtic cycles found in eccentricity and (generally) in benthic δ¹⁸O. Overall, geometries of eccentricity (tuning target), CaCO₃ est. (tuning signal, lithological proxy record), and benthic δ¹⁸O (climate proxy record) are largely independent from one other.

Fig. S7.

Nonsinusoidal cycle properties of CaCO₃ estimate record. (A) CaCO₃ est. from Site 1264 (15), and (B) its skewness, (C) asymmetry, and (D) kurtosis, calculated across a 2-My sliding window using standard (turquoise circles) and bispectral methods (purple-pink triangles). Seven prominent, decimeter-thick chalk layers are removed from the Oligocene part of the record before the quantification of nonsinusoidal cycle properties, as these layers distort the background cyclicity. Vertical gray bars are as in Fig. 1.

The inverse and (assumed) in-phase relationship between the CaCO₃ est. record and eccentricity (15) (where CaCO₃ maxima correspond to eccentricity minima) and the evolution of skewness suggest (52) that the sediments at Site 1264 result from a productivity-dominated oceanographic setting, despite long-term trends in absolute values that may reflect a secondary influence of dissolution. Further evidence that the control of dissolution on CaCO₃ cycle shape during the Oligocene was smaller than that of productivity comes from the continuously high CaCO₃ values, and from the fact that Site 1264, at 2,000- to 2,200-m paleowater depth, was positioned well above the calcite compensation depth and lysocline throughout the entire Oligo-Miocene (14). We consider the dilution component by terrestrial input to be of a lesser influence on the preserved cycle shapes at Site 1264, as it is positioned far away from land. Physical, grain-size-dependent, diffusion-like processes and bioturbation smooth the higher-frequency paleoclimate signals in the natural archive (53). However, this smoothing probably did not affect cycle geometry in a preferential direction. Similarity in patterns between X-ray fluorescence core scanning records of overlapping intervals from both drill holes (15) also rules out a significant effect of drilling disturbances on the deformation of specific intervals.