Distinct effects of nuclear volume fraction and cell diameter on high b-value diffusion MRI contrast in tumors

Abstract

Purpose

While many recent studies have demonstrated improved detection and characterization of malignant lesions using high b-value diffusion imaging techniques, little is known about the underlying physical characteristics of tumor cells that modulate the restricted water signal at high b on clinical scanners.

Methods

Monte Carlo simulations of diffusion in a synthetic tumor cell environment were used to study the specific effects of tumor cell diameter and nuclear volume fraction (ν) on high b diffusion contrast.

Results

Results indicate that clinical pulsed-gradient spin-echo diffusion-weighted signals measured at high b (~ 4000 s/mm²), long diffusion time (Δ ~ 40-60 ms) and long echo time (TE ~ 60-140 ms) are generally insensitive to tumor cell diameter, but increase exponentially with ν. Moreover, these results are predicted by a simple analytic expression for the intracellular restricted water signal with elevated T2 for the intra-nuclear versus cytosolic compartment.

Conclusion

Nuclear volume fraction is an important characteristic of cancer cells that modulates the apparent restriction of water at high b on clinical scanners. This model offers a possible explanation for the apparent unreliable correlation between tumor cell density (cellularity) and traditional ADC.

INTRODUCTION

Noninvasive cancer imaging biomarkers are important tools for the identification and characterization of neoplastic lesions in vivo, as well as for monitoring tumor response to treatment. Owing to the unique sensitivity of proton magnetic resonance signals to the molecular self-diffusion of water at microscopic length scales, diffusion-weighted imaging (DWI) is a powerful tool for probing microstructural and physiological properties of cancer in vivo at the cellular and sub-cellular level. While the field continues to progress at a rapid pace, the application of DWI as a clinical tool will surely benefit from a deeper understanding of the underlying biological mechanism producing diffusion contrast in both healthy and neoplastic tissue.

In diagnostic oncology, a hallmark radiologic feature of many malignant cancers is elevated DWI signal caused by reduced apparent diffusion coefficient (ADC) of water in the tumor microenvironment (1). While it is generally accepted that reduced ADC in tumors is caused by high packing density of cancer cells in these lesions, the physical mechanism through which this happens remains unclear. In principle, greater cell packing density can reduce ADC through two physical mechanisms. The first is decreased extracellular space volume fraction and increased tortuosity of the extracellular space matrix, which leads to greater diffusion hindrance and reduced mobility of extracellular water molecules (2–4). The second is increased intracellular space volume fraction (i.e. cell density) resulting in a greater number of water molecules that are restricted or trapped within the cells themselves (3,4). At long diffusion times (Δ ~ 30–60 ms) and high diffusion-weighting factors (b > 3000 s/mm²) signal from the hindered yet relatively fast extracellular water compartment is largely attenuated and DWI contrast is dominated by the slow intracellular restricted water signal (4). Building on this phenomenon, many recent studies have demonstrated improved tumor contrast-to-noise (i.e. conspicuity) utilizing DWI data at high b through reduced sensitivity to extracellular edema and necrosis (5–8). However, the restricted water signal magnitude remains highly variable both within and across different tumor types in a manner that can’t be explained by cell density alone. Case in point is lack of restricted signal on DWI in some cell rich cancers such as oligoastrocytoma. This suggests that the intrinsic properties of cancers cells themselves must also be modulating the apparent restriction of water in tumors, but it is presently unclear what properties of the cancer cells are at play and how these properties modulate diffusion contrast at high b.

Two distinguishing morphological characteristics of malignant cancer cells are their large size variations and elevated nuclear volume fractions compared with healthy cells (9). The goal of this study, therefore, is to investigate the specific role of these two parameters on the restricted intracellular signal as measured with high b clinical DWI protocols. To do so, we employ Monte Carlo simulations of water diffusion in a synthetic tumor cell to study the signal dependence on cell diameter and nuclear volume fraction under a variety of experimental conditions.

METHODS

The tumor microenvironment is a complex milieu that includes not only cancer cells but also stromal cells, inflammatory cells, and healthy cells. However, for the purpose of this study we model the tumor microenvironment to consist of only cancer cells and extracellular space. As such, the total signal can be written as the sum of signals from the extracellular and intracellular (cancer cell) compartment weighted by their respective volume fractions:

$${\mathrm{S}}_{\mathrm{total}}\approx f\cdot {\mathrm{S}}_{\mathrm{intra}}+\left(1-f\right)\cdot {\mathrm{S}}_{\mathrm{extra}},$$ [1]

where f is the volume fraction of cancer cells in the measured voxel. The main objective of this study was to investigate the nature of the intracellular restricted water signal S_intra obtained using clinically-relevant experimental DWI parameters at high b. High b DWI offers greater sensitivity and specificity to intracellular restricted water through intrinsic suppression (filtering) of the fast extracellular water fraction S_extra in Eq. [1]. This greatly simplifies the diffusion model and simulation requirements as the effects of edema, necrosis, and extracellular space tortuosity can largely be ignored. In the first paragraph of the Results and Discussion section we provide a demonstration of the attenuation of S_extra at high b over a range of tortuosity factors.

Cancer cell simulation model

Our cancer cell simulation model consisted a single three-dimensional (3D) spherical cell with a concentric spherical nucleus whose cellular (r_cell) and nuclear (r_n) radii were allowed to vary. (see Fig. 1). Each of the three compartments (intranuclear, cytoplasmic, and extracellular) was ascribed its own intrinsic diffusivity and transverse relaxation rate and separated by either an impermeable or semipermeable plasma membrane or highly permeable nuclear envelope. Specifically, for these initial simulations, we ascribed a plasma membrane permeability of either P_ce = 0 (impermeable) or P_ce = 0.003 m/ms. The later value was derived from prior experimental measurements of the intracellular/extracellular exchange rate for neural cells in intact functioning mammalian brains (10) using the permeability equation 1/P_ce = τ_ce*(S/V) with τ_ce = 550 ms and S/V corresponding to the surface-to-volume ratio of a 10 μm diameter spherical cell. The nuclear pore complex on the other hand allows for nearly free passage of water molecules between the nucleus and cytosol resulting in an effective exchange rate that is much higher than the plasma membrane (11,12). In order to emphasis the rapid exchange of water between the cytosol and nucleus, the nuclear membrane permeability was initially modeled as P_nc = 0.5 μm/ms, which corresponds to an average intranuclear residence time of approximately τ_nc = 1.65 ms for a 5 μm diameter nucleus. However, it should be noted that so long as τ_nc remains short relative to the experimental diffusion time (i.e. fast exchange regime, τ_nc ≪ Δ), the synthesized signal is relatively insensitive to the value for P_nc. To confirm this, we verified that the simulation results were nearly identical using P_nc = 0.5 μm/ms and the case of completely free exchange between the nucleus and cytosol, as others have modeled (13,14).

Figure 1.

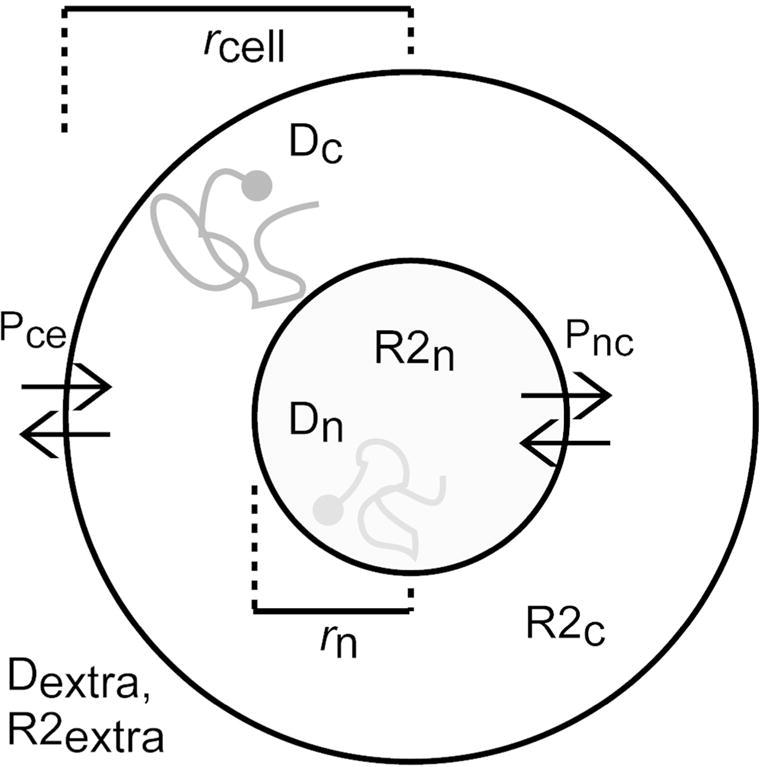
Cancer cell simulation model. r_cell = cell radius (varying), r_n = nuclear radius (varying), D_c = cytosol diffusivity (fixed), D_n = nuclear diffusivity (fixed), R2_c = cytosol transverse relaxation rate (fixed), R2_n = nuclear transverse relaxation rate (fixed), D_extra = extracellular diffusivity (fixed), R2_extra = extracellular transverse relaxation rate (fixed), P_nc = nuclear permeability (fixed), P_ce = plasma membrane permeability (varying).

The lack of available experimental data makes it difficult to precisely model the intrinsic diffusion constants and relaxation rates for the nucleus and cytosol. Therefore, for this initial study, the following previously published parameters were used (13–16): nuclear diffusivity D_n = 1.31 μm²/ms, cytoplasmic diffusivity D_c = 0.48 μm²/ms, extracellular water diffusivity D_extra = 1.82 μm²/ms, nuclear transverse relaxation T2_n = 1/R2_n = 63.29 ms, cytoplasmic transverse relaxation T2_c = 1/R2_c = 23.87 ms, and extracellular water relaxation T2_extra = 1/R2_extra = 150 ms. It should be noted that these parameters were measured in large ~0.5 mm single neurons isolated from Aplysia californica and therefore may not translate directly to tumor cells in vivo. To address this concern we verified that the simulation results were robust to a broader range of parameter settings provided their relative values remained similar according to: D_extra ≥ D_n > D_c, T2_extra ≥ T2_n > T2_c, and τ_nc ≪≪ τ_ce.

Intracellular signal model

At ultra short diffusion times, the diffusion length scales probed by intracellular spins are infinitesimal and the effects of restriction and exchange are negligible as the overwhelming majority of intracellular spins do not come in contact with the plasma or nuclear membrane. Under these experimental conditions the cytosolic and nuclear compartment are not exchanging and the total intracellular signal for a single cancer cell is proportional to the superposition of signals from the two compartments:

$${\mathrm{S}}_{\mathrm{intra},\text{short}\mathrm{\Delta }}\propto \left(1-v\right)·\underset{S_{\text{cytosol}}}{\underbrace{\exp \left(-\mathrm{TE}\cdot R{2}_c\right)·\exp \left(-b\cdot {\mathrm{D}}_c\right)}}+v\underset{S_{\text{nucleus}}}{\underbrace{·\exp \left(-\mathrm{TE}\cdot R{2}_n\right)·\exp \left(-b\cdot {\mathrm{D}}_n\right)}},$$ [2]

where $b:={\left(\gamma \delta G\right)}^2(\frac{\delta }{3}-\mathrm{\Delta })$ is the b-value of the experiment, γ is the gyromagnetic ratio for protons, G is the amplitude the bipolar diffusion gradients with duration δ and separation Δ, respectively, and TE is the echo time, and where v ≡ (r_n⁄r_cell)³ is the “cell-wise” nuclear volume fraction, as proportion of total intracellular volume. Note that in this study, we use “cell-wise” nuclear volume fraction ν as opposed to nuclear-to-cytoplasm ratio (N/C ratio), as the later is traditionally used to refer to the ratio of total nuclear to cytoplasmic (and extracellular) area using hematoxalin and eosin (H&E) counterstaining.

On clinical scanners, however, the diffusion time is typically long relative to the average residence time of water molecules within the nucleus (Δ ≫ τ_nc) and the nucleus and cytosol are in fast exchange (fully mixed condition). To model this regime, we replace the intrinsic diffusion constants and relaxation rates in Eq. [1] by an apparent diffusion coefficient (ADC_intra) and apparent relaxation rate (AR2_intra). The intracellular diffusion signal for a tumor cell in the long diffusion time fast exchange regime then becomes:

$${\mathrm{S}}_{\text{intra},\text{long}\mathrm{\Delta }}\propto \exp \left(-\mathrm{TE}\cdot {\mathrm{AR}2}_{\mathrm{intra}}\right)\cdot \exp \left(-b\cdot {\mathrm{ADC}}_{\mathrm{intra}}\right).$$ [3]

AD_Cintra in Eq. [3] reflects the net displacement of intracellular water molecules during the diffusion time and is therefore a complex function of the plasma membrane permeability (exchange with the extracellular compartment) and the geometry of the intracellular compartment. AR2_intra, on the other hand, depends on the intrinsic instantaneous transverse relaxation rates of the intracellular fluid pools and the cumulative time spent by each spin in their respective compartments. Noting that in the fast exchange regime the cumulative time spent in each compartment is proportional to the volume fraction, the AR2_intra in Eq. [3] then becomes a simple function of the compartment specific relaxation rates weighted by ν:

$$AR{2}_{\mathrm{intra}}=v\cdot R{2}_n+\left(1-v\right)\cdot R{2}_c.$$ [4]

Simulation details

Monte Carlo simulations (17–19) were used to evaluate the behavior of diffusing water molecules in our simplified cancer cell model and to generate synthetic intracellular diffusion signals under various experiment parameters. For each simulation run, 4 × 10⁵ spins were assigned to random starting locations within the cell (initial x, y, z coordinates within the nucleus and cytosol) and then allowed to diffuse following a random walk model using their respective diffusivities and a temporal step size of 0.001 ms. At each time step, the spin phase vector in the transverse plane was updated according to the applied gradient field and the position of each spin within the field. To model restrictions and exchange, spins were reflected elastically off the contacted barrier (nuclear or plasma membrane) or allowed to pass through depending on the permeability constant. Intracellular spins that were selected to exchange through the plasma membrane had their phase replaced with those of randomly drawn simulated spins from the extracellular compartment. The extracellular spin phases were generated using separate simulation runs with identical experimental parameters, but no cells present. For all simulations, the gradient duration was set equal to the gradient separation (δ = Δ) to mimic the clinical setting. Furthermore, to ensure the simulated signals were independent of starting location and the path history of each spin, each simulation run was repeated several times and plots were verified to be essentially identical. All simulations were performed in house using programs written in Matlab (Mathworks, Inc) running on a Dell workstation with a quad-core 2.67GHz Intel® Xeon® X5550 Processor with 48 GB of RAM.

RESULTS

Attenuation of the extracellular water signal at high b

Figure 2a plots the relative sensitivity of S_total to S_extra in terms of the ratio S_extra/S_intra as a function of b. For these plots S_extra was generated analytically using S_extra – exp(−TE · AR2_extra) · exp(−b · ADC_extra) with ADC_extra = 1.0 μm²/ms and AR2_extra = 1/150 ms, while S_intra was simulated with the following parameters: ν = 0.8, cell diameter = 10 μm, P_ce = 0.003 μm/ms TE = 140 ms, Δ = 60 ms. As demonstrated in the figure, the contribution of S_extra to the total signal decays rapidly as a function of b and is negligible at b = 4000 s/mm². This is true even when taking into account the predicted changes in ADC_extra due to changing extracellular space tortuosity λ with volume fraction α. Note that the high and low α regime plotted in Fig. 2a was derived from Figure 7 of Chen and Nicholson (20) (reprinted in Fig. 2b) which plots empirical and theoretical values for λ as a function of α under sever osmotic stress conditions. Specifically, for the high and low α regime we modulated ADC_extra by a factor of 1.13 and 0.88, respectively, which was derived from the ratio of λ² values in Fig. 2a. As a reference, the case of vasogenic edema in the tumor extracellular space is also plotted in Fig. 2a with elevated ADC_extra = 2.7 μm²/ms and reduced AR2_extra = 1/500 ms.

Figure 2.

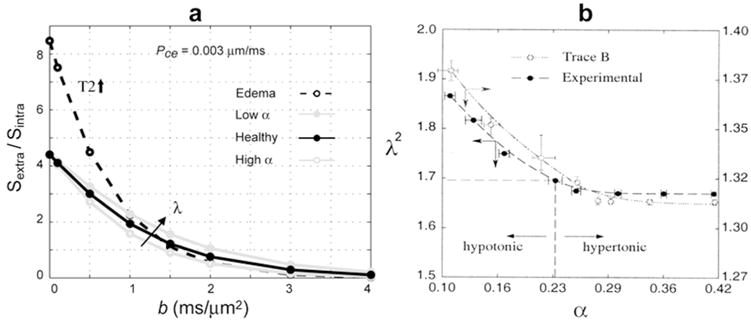
Reduced sensitivity to extracellular water at high b. (a) Ratio of S_extra/S_intra as a function of b demonstrating negligible contribution of the extracellular water signal to the total signal at b = 4000 s/mm². This is true even when taking into account the predicted change in ADC_extra due to changing extracellular space tortuosity λ with volume fraction α. (b) Theoretical and experimental λ vs. α plots (Reprinted with permission from Ref (20) Chen KC, and Nicholson C, PNAS 2000;97:8306-8311.) from which the high and low α curves were derived and plotted in (a).

Effects of cell diameter on S_intra

Figure 3 demonstrates the change in the intracellular restricted water signal S_intra with cell diameter at b = 4000 s/mm² over a range of diffusion times Δ and with nuclear volume fraction fixed. Also shown are the corresponding estimated values of AR2_intra and ADC_intra fit to the simulated signal according to Eq. [3]. Note the change in S_intra with cell diameter is more pronounced at short diffusion times, but at long (clinically-relevant) diffusion times the signal dependence on cell diameter is minimal (Fig. 3A). Also note that the cause of the slight decrease in S_intra with increasing cell diameter at long diffusion times is an increase in ADC_intra for larger cells (Fig. 3C black lines), while AR2_intra remains constant (Fig. 3B) as expected being only a function of ν (see Eq. [4]). Figure 3D demonstrates that a similar dependence in S_intra with cell diameter is observed when allowing for exchange with the extracellular compartment.

Figure 3.

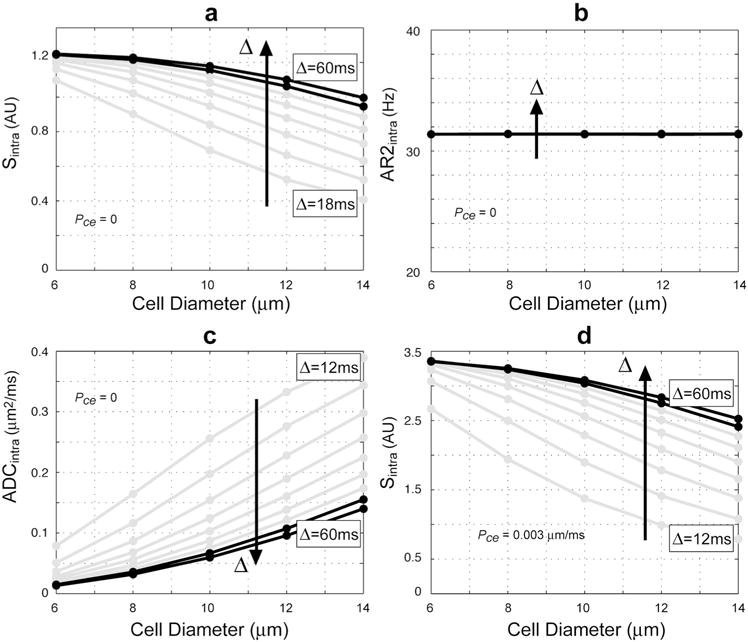
Simulated S_intra as a function of cell diameter (i.e., 2·r_cell). (a) S_intra with no exchange (P_ce = 0) and corresponding fitted values for (b) AR2_intra, and (c) ADC_intra. (d) S_intra allowing for exchange with the extracellular compartment P_ce = 0.003 μm/ms. Simulations parameters: ν = 0.4, b = 4000 s/mm², TE = 140 ms, δ = Δ = [12,18,24,30,36,42,48,54,60] ms. Black lines indicate clinically relevant parameters.

Effects of nuclear volume fraction on S_intra

Figure 4 demonstrates the change in the intracellular restricted water signal S_intra with nuclear volume fraction ν at b = 4000 s/mm² over a range of diffusion times Δ and with cell diameter fixed. Also shown are the corresponding estimated values of AR2_intra and ADC_intra fit to the simulated signal according to Eq. [3] Notice here that the change in S_intra is exponential in ν and is maximal at long (clinically-relevant) diffusion times. Also, notice the cause of the exponential increase in signal with ν is a corresponding linear decrease in AR2_intra (Fig. 4B) as expected from Eq. [4], while ADC_intra remains relatively constant (Fig. 4C). Note that the slight decrease in ADC_intra with ν at long diffusion time may be explained by the increased probability of intracellular spins reflecting off the nuclear membrane thereby reducing the effective pore size experience by each spin. Notice also that a similar exponential increase in signal with ν is observed when allowing for exchange with the extracellular compartment (Fig. 4D).

Figure 4.

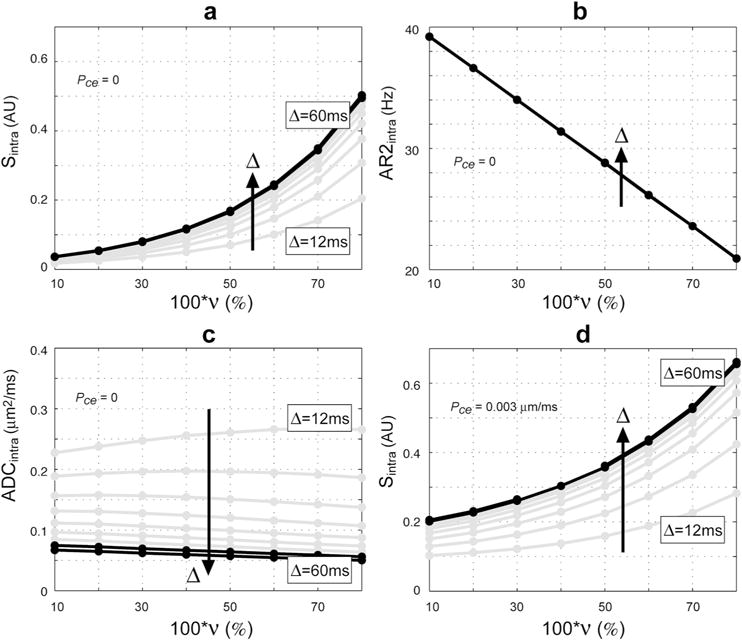
Simulated S_intra as a function of ν. (a) S_intra with no exchange (P_ce = 0) and corresponding fitted values for (b) AR2_intra, and (c) ADC_intra. (d) S_intra allowing for exchange with the extracellular compartment P_ce = 0.003 μm/ms. Simulations parameters: cell diameter = 10 μm, b = 4000 s/mm², TE = 140 ms, δ = Δ = [12,18,24,30,36,42,48,54,60] ms. Black lines indicate clinically relevant parameters.

DISCUSSION

The goal of this study was to investigate the specific effect of cell diameter and nuclear volume fraction on clinical DWI contrast of tumors at high b. We found that traditional pulsed-gradient spin echo diffusion-weighted signals at high b, long diffusion time, and long echo time are relatively insensitive to cell diameter over a physiologically relevant range, but increased exponentially with the nuclear volume fraction of individual tumor cells.

At first, our results demonstrating a strong dependency of high b clinical DWI contrast to cell-wise nuclear volume fraction (ν) seems at odds with the findings of Xu et al. (14) who observed limited or no sensitivity to ν by traditional pulsed-gradient diffusion measures and greater sensitivity to ν when sampling over a range of short diffusion times using oscillating gradients. However, it should be noted that although the Xu et al. study used identical diffusion parameters to the current study (i.e. D_n = 1.31 μm²/ms, cytoplasm D_c = 0.48 μm²/ms) they assumed the intracellular T2 was homogenous everywhere (i.e. R2_n = R2_c), which obviously removes the T2 effect. Furthermore, the comparisons in the Xu et al. study were performed at low b (b = 1 ms/μm²) and short echo time (TE = 40 ms), which also reduces sensitivity to T2 differences of intracellular water. Taken together, the results of this study suggest that while oscillating-gradient measures at low b, short TE, and short diffusion time appear sensitive to changes in ν through compartmental differences in diffusivity, but not T2, clinical pulsed-gradient diffusion measurements at high b, long TE, and long diffusion time appear sensitive to changes in ν through compartmental differences in T2, but not diffusivity.

Compartmental T2 filtering and cellular conspicuity factor

It is important to recall that diffusion signals are intrinsically dual filtered by both the b-value and echo time of the experiment and that although traditional ADC removes the effect of bulk tissue T2 on the diffusion estimate by normalizing with respect to the signal at b = 0, compartmental differences in T2 still cause a differential weighting of the respective water pools to the measured signal and ADC at long echo time. We demonstrate in this study that when the nucleus and cytosol are in fast exchange, the effective T2 for the intracellular compartment increases linearly with nuclear volume fraction causing cells with large ν to be weighted more to the measured signal and ADC compared with cells with small ν (Fig 4). Moreover, the weighting is exponential with ν can be quantified by substituting Eq. [4] into Eq. [3] resulting in:

$${\mathrm{S}}_{\mathrm{intra}}\propto \exp \left(-b\cdot {\mathrm{ADC}}_{\mathrm{intra}}\right)\cdot \kappa \left(v\right),$$ [5]

where

$$\kappa \left(v\right)=\exp \left(-\mathrm{TE}\cdot \left[v\cdot \mathrm{R}{2}_{\mathrm{n}}+\left(1-v\right)\cdot \mathrm{R}{2}_{\mathrm{c}}\right]\right)$$ [6]

We refer to κ = in Eq. [6] as the “cellular conspicuity factor” as it describes the relative contribution of individual cells (or collections of cells with similar properties) to the measured intracellular signal at high b. Note that Eq. [5] can be simplified further by noting that ADC_intra is independent of both diameter and ν and low at long diffusion time due to restriction effects with the plasma membrane (Fig 4), resulting in

$${\mathrm{S}}_{\mathrm{intra}}\propto \kappa \left(v\right).$$ [7]

as exp(−b · ADC_intra ~1. The concept of dual filtering with b and TE together with plots of the cellular conspicuity factor κ is provided in Fig 5.

Figure 5.

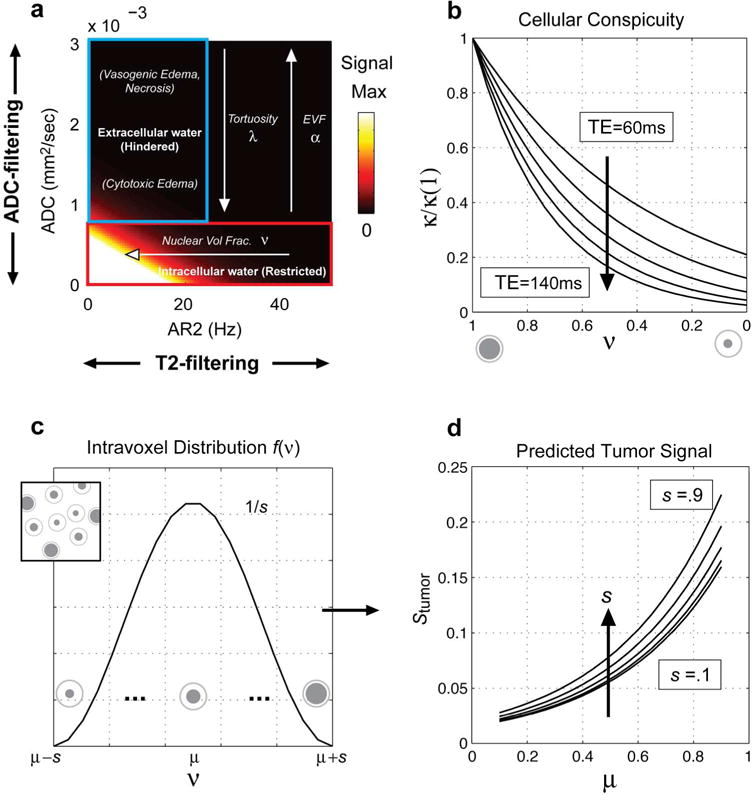
(a) DWI data are dual filtered along ADC and AR2 depending on the b-value and echo time, respectively. At high b and long Δ, water from the fast extracellular compartment is attenuated providing intrinsic selection of slow intracellular restricted water. Similarly, at long TE, cell populations with low T2 (high AR2) are attenuated providing intrinsic selection of cells with high T2 (low AR2). (b) Plots of the cellular conspicuity factor κ at different TEs [60,80,100,120,140 ms] quantifying the exponential T2 filtering of cells with decreasing ν (see Eq. [6]). (c) A raised cosign function for the intra-voxel nuclear volume fraction distribution f(ν) leads to (d) an analytic expression for the predicted voxel-level signal in tumors, which depends on both the mean μ and dispersion s [.1,.3,.5,.7,.9 shown] of the nuclear volume fraction distribution (see Eqs. [8–10]).

Predicted voxel-level signal

Note that the majority of this study focused on understanding and characterizing signals from individual cancer cells or collections of cells with identical properties. However, it is fairly strait forward to extrapolate to the case of a tumor voxel containing a distribution of cancer cells with varying nuclear volume fractions. To do so, we need to integrate Eq. [7] over a distribution of ν such that the predicted tumor signal at high b and long Δ becomes

$${\mathrm{S}}_{\text{tumor}}\propto {\displaystyle \int \kappa \left(v\right)f\left(v\right)dv},$$ [8]

where f(ν) describes the proportion of total volume taken up by cells with a given ν. While Eq. [8] applies to any arbitrary distribution of nuclear volume fractions, lets assume for the moment that f(ν) follows a raised cosign function with finite support over the interval [a = μ − s, b = μ + s] such that:

$$f\left(x;\mu ,s\right)=\frac{1}{2s}\left[1+\cos \left(\frac{x-\mu }{s}\pi \right)\right].$$ [9]

Then the solution to the integral equation for the predicted voxel-level signal in tumor is given by the following closed form expression:

$$S_{\text{tumor}}\propto -\frac{{\pi }^2}{2}\left[\frac{e^{TE\cdot \left\{R{2}_c\left(a-1\right)-R{2}_n\left(a\right)\right\}}-e^{TE\cdot \left\{R{2}_c\left(b-1\right)-R{2}_n\left(b\right)\right\}}}{\left(R{2}_c-R{2}_n\right)\cdot s\cdot TE\cdot ({\pi }^2+{(R{2}_c-R{2}_n)}^2\cdot s^2\cdot T{E}^2)}\right].$$ [10]

Note that the signal increases monotonically with both the mean μ and dispersion s of the intra-voxel distribution of nuclear volume fraction ν.

Clinical relevance: Revisiting the ADC/cellularity relation at low b

In clinic practice, tumor ADC values are traditionally calculated using monoexponential analysis of the diffusion-weighted signal decay over a relatively low b value range of around 1000 s/mm². For the purpose of this discussion we refer to the traditional low b value ADC as ADC_total to distinguish this quantity from ADC_intra calculated using Eq. [3] at high b values (~ 4000 s/mm²) and presented in Figs 3 and 4 in this study. Several studies have documented a negative correlation between tumor cell density (cellularity) and ADC_total (21–25), yet this relationship does not generalize across all tumor categories. For example, schwannomas and oligoastrocytomas are tumors with relatively high cell density, which would imply elevated restriction diffusion and, accordingly, low ADC_total; however, they exhibit comparatively high diffusion coefficients (1,26). While heterogeneity of tissue and partial voluming of vasogeneic edema may explain in part the high diffusion coefficient of these tumors, our results suggest another factor could be at play that has not been previously considered. Specifically, the results of this study suggest that high ADC_total measured in these tumors may result from the abundance of cells with relatively low nuclear volume fractions. As the tumor cell nuclei decrease in size relative to their respective diameters, the effective T2 of intracellular water decreases (Fig. 4b) causing a decrease in the relative weighting of the slow intracellular restricted water fraction (low ADC_intra, Fig. 4c) on the measured diffusion-weighted signal at long echo time (Fig. 4a). Accordingly, ADC_total will be elevated due to a stronger relative weighting of the fast extracellular hindered fraction (ADC_extra) in these tumors. On the other hand, the low ADC_total of tumors composed of cells with large nuclear volume fractions (e.g. glioblastomas and lymphomas) may result from a stronger relative weighting of the slow intracellular restricted pool versus the fast extracellular hindered pool. Interestingly, the study of Gauvain et al (25), demonstrated a stronger correlation of ADC_total with total nuclear area of tumors compared with total cellular area, which is consistent with this result. Moreover, we have noticed in our own work using a technique called Restriction Spectrum Imaging (RSI) (6,27–29) that the magnitude of the intracellular restricted signal at high b values appears to be modulated by both cell density and nuclear volume fraction of individual cells, although further work is required to validate this observation using quantitative stereology techniques.

Future directions

Many advanced DWI techniques are emerging to better characterize and model water compartmentalization and complex diffusion in tissues on clinical scanners such as diffusion kurtosis imaging (30), and RSI (29). Our results here suggest that by manipulating echo time and b-value, it may be possible to further decompose the diffusion into proportions of water that can be attributed to cells with small vs. large nuclear volume fractions. Moreover, the high intrinsic contrast to cells with large nuclei at high b and long echo time should in principle enable improved detection of even small populations of highly malignant tumor cells. Finally, given that a characteristic feature of many stem cells is high nuclear volume fractions, these techniques may also hold promise for non-invasive stem cell tracking applications without the need for extrinsic contrast agent, although the concentration limits and scan time requirements required for sufficient diffusion contrast remains to be determined and compared with established techniques.

CONCLUSION

In this study, we derive a simple analytic expression for the diffusion signal within tumors capturing the effect of tumor cell density and cell-wise nuclear volume fraction (ν) on clinical DWI data. We show how the intracellular restricted water signal measured with traditional pulsed-gradient spin-echo acquisitions at high b and with long diffusion times and long echo times, is relatively insensitive to the size of individual tumor cells, but increases exponentially with ν. Moreover, we provide a mathematic framework to validate the MRI-derived histological parameters directly against empirically derived distributions of nuclear volume fractions of individual cells, which may be obtained using stereological methods applied to histological data that stain for both plasma and nuclear membranes. The model, which is based on compartmental differences in T2 between the nucleus and cytosol, may help explain the additional variation in DWI signal and traditional ADC at low b (~1000 s/mm2) within and across different tumor types that can’t be explained on the basis of cell density alone. Specifically, our model predicts that at long echo time a component of reduced ADC in some tumors may be due to a greater weighting on the intracellular restricted water fraction for cells with larger nuclei due to the reduced apparent R2 (higher T2) for intracellular water in these cells. Conversely, the elevated ADC in some cell rich tumors may be due to a reduced weighting on the intracellular restricted water fraction for cells with smaller nuclei and a greater relative weighting of the fast extracellular water fraction in these tumors.