Wide bandgap BaSnO₃ films with room temperature conductivity exceeding 10⁴ S cm⁻¹

Abstract

Wide bandgap perovskite oxides with high room temperature conductivities and structural compatibility with a diverse family of organic/inorganic perovskite materials are of significant interest as transparent conductors and as active components in power electronics. Such materials must also possess high room temperature mobility to minimize power consumption and to enable high-frequency applications. Here, we report n-type BaSnO₃ films grown using hybrid molecular beam epitaxy with room temperature conductivity exceeding 10⁴ S cm⁻¹. Significantly, these films show room temperature mobilities up to 120 cm² V⁻¹ s⁻¹ even at carrier concentrations above 3 × 10²⁰ cm⁻³ together with a wide bandgap (3 eV). We examine the mobility-limiting scattering mechanisms by calculating temperature-dependent mobility, and Seebeck coefficient using the Boltzmann transport framework and ab-initio calculations. These results place perovskite oxide semiconductors for the first time on par with the highly successful III–N system, thereby bringing all-transparent, high-power oxide electronics operating at room temperature a step closer to reality.

With impressive electronic transport properties, wide bandgap perovskite oxides are promising transparent conductors. Prakash et al. report n-type BaSnO₃ films with room temperature conductivity exceeding 10⁴ S cm⁻¹ and investigate factors limiting carrier mobility.

Wide bandgap ternary oxides with perovskite structure have generated considerable excitement due to their impressive multi-functionality and the promising route they provide to potentially disruptive technologies for logic and resistive memory, utilizing both electronic and spintronic concepts¹,²,³,⁴. Rapid progress with perovskite oxide heterostructures has also been made, including demonstration of strain-stabilized non-equilibrium electronic and magnetic properties, two-dimensional electron gas formation, quantum oscillation effects, and exotic magnetism and superconductivity⁵. However, the realization of this staggering range of functionalities at room temperature remains a grand challenge in the field. Carrier mobility in semiconducting perovskite oxides provides an ideal, and very technologically important, example⁶.

The best room temperature values of the mobility and conductivity in the model perovskite oxide semiconductor SrTiO₃ have remained below 10 cm² V⁻¹ s⁻¹ and 500 S cm⁻¹, respectively for over 50 years⁶. Well-publicized advances with interfaces such as LaAlO₃/SrTiO₃ have similarly been restricted to low temperatures⁵. Very recently, bulk BaSnO₃ (BSO) has shown significantly higher room temperature mobilities and corresponding conductivities. Luo et al.⁷, first discovered a room temperature mobility of 103 cm² V⁻¹ s⁻¹ at a carrier concentration of 8 × 10¹⁹ cm⁻³ in bulk doped BSO crystals followed by the work of Kim et al.⁸,⁹ showing a much higher mobility of 320 cm² V⁻¹ s⁻¹ and a conductivity value of 4 × 10³ S cm⁻¹ at a carrier concentration of 8 × 10¹⁹ cm⁻³ (refs ^{8, 9}). Owing to these behaviours, doped BSO has gained significant interest as a high-performance transparent conductor⁷,⁸,⁹,¹⁰,¹¹,¹². A favourable conduction band offset of BSO with structurally similar oxides such as SrTiO₃ (STO) and LaAlO₃ (LAO) further makes it a promising candidate for high mobility channel material in oxide heterostructures for both power electronic applications and fundamental physics study⁶,¹³,¹⁴,¹⁵.

However, thin films of BSO have shown significantly lower mobilities. The highest reported mobility in thin films grown using molecular beam epitaxy (MBE) is 150 cm² V⁻¹ s⁻¹ on PrScO₃ (110) at a carrier concentration of 7.2 × 10¹⁹ cm⁻³, decreasing to 80–120 cm² V⁻¹ s⁻¹ for films on STO (001) between 6 × 10¹⁹–1 × 10²⁰ cm⁻³, which results in an overall conductivity of 2 × 10³–3 × 10³ S cm⁻¹ (ref. ¹⁶). The difference in mobility between bulk single crystals and thin films of BSO has been largely attributed to the substrate-induced misfit/threading dislocations¹⁶. Dislocations can introduce charged defects leading to compensation of carriers, and an overall reduced mobility. For instance, threading dislocation cores in GaN have been shown to consist of Ga-vacancy (V_Ga) based defect complexes¹⁷, which form deep compensating acceptors¹⁸,¹⁹ together with space charge regions around the dislocation lines, resulting in enhanced electron scattering and thus reduced mobility²⁰.

A similar effect can be expected from non-stoichiometry²¹, which also introduces charged point defects. Evidence that this effect is often at play in BSO is the observation of different mobility values for films grown via different synthesis routes despite having similar substrates and doping levels⁷,⁹,²²,²³. Even homoepitaxial films²⁴ show much lower mobility compared to bulk single crystals suggesting an important role of point defects. Furthermore, films grown by MBE show a large variation in the mobility values despite having identical carrier concentration¹⁶. These results raise the question of how non-stoichiometry and dislocations in films influence electronic transport. It further begs the question as to what scattering mechanisms limit the electron mobility and therefore the conductivity in BSO films and what can be the ultimate mobility in BSO if defects are minimized. It is also noteworthy that there are no transport studies in BSO below a doping level of 10¹⁹ cm⁻³ raising further questions regarding the identity of the compensating defects.

To understand the role of dislocations, non-stoichiometry, and chemical dopants on electronic transport, and to examine mobility-limiting scattering mechanisms in doped BSO, we combine experiments and modelling using the Boltzmann transport and density functional theory (DFT) calculations. First, we investigate the influence of dislocations on the carrier concentration and mobility in doped BSO by tailoring the dislocation density by varying undoped buffer layer thickness. Second, we investigate the role of charge compensation on the critical density for the metal-to-insulator transition, carrier concentration, mobility and the Seebeck coefficient. Finally, we calculate the band structure, mobility and Seebeck coefficient to shed light on the scattering mechanisms limiting the mobility at different carrier concentrations and temperatures and provide the upper limits for mobility in a dislocation-free material.

Results

Thickness optimization of buffer and active layers

All BSO/STO heterostructures were prepared using hybrid MBE. Growth conditions were chosen to yield phase pure, epitaxial and stoichiometric films on STO substrates²¹,²⁵. (See Supplementary Figs 1 and 2 for X-ray diffraction pattern and atomic force micrograph). The lattice mismatch of BSO is −5.12% (compressive) with the STO(001) substrate, resulting in the formation of misfit dislocations and strain relaxation for films with thickness above 1 nm (ref. ²⁵). The density of misfit dislocations is largest near the film/substrate interface, which reduces the carrier mobility and also affects defect densities. Inserting a thick insulating (undoped) buffer layer between the substrate and the doped (active) layer may reduce the defect density in doped layers grown on top of it²⁶. It must, however, be noted that dislocations remain present in doped layers as threading dislocations²⁵, which are inevitable without a lattice-matched substrate.

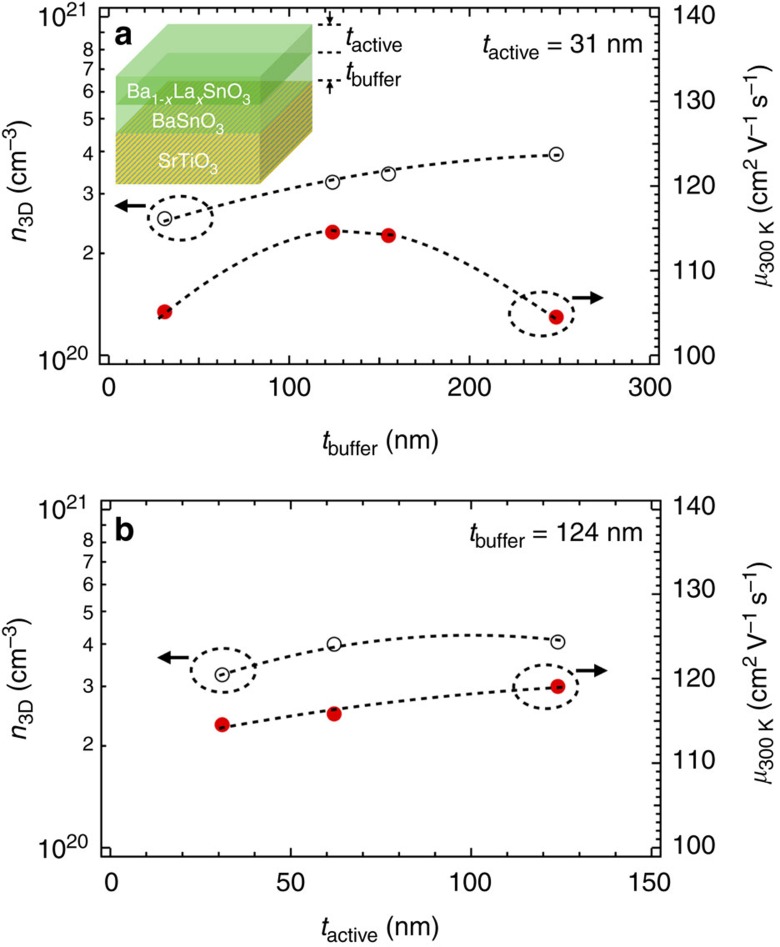
To investigate the role of dislocations on the electronic transport, we grew a series of 31 nm La-doped BSO/t_buffer BSO/STO(001) films, as illustrated in the inset of Fig. 1a. Here, we fix the thickness of the active layer for which the transport will be evaluated while varying t_buffer between 30 and 250 nm. Dopant density in the active layer was kept constant by fixing the La-cell temperature (T_La) at 1230 °C. Figure 1a shows room temperature carrier concentration (n_3D) and mobility (μ_300 K) as a function of t_buffer. n_3D increases monotonically between 2.5 × 10²⁰ cm⁻³ and 4 × 10²⁰ cm⁻³ whereas μ_300 K first increases to 115 cm² V⁻¹ s⁻¹ at t_buffer=124 nm and then decreases. The fact that n_3D increases with increasing t_buffer, which in turn decreases dislocation density in active layer (See Supplementary Fig. 3) suggests charge compensation being operative, and that threading dislocations act as acceptor-like defects. We interpret the decrease in μ_300 K for t_buffer>124 nm is due to scattering from increased surface roughness (See Supplementary Fig. 4)²⁷. Having determined the optimal buffer layer thickness which is similar to what has been reported by Park et al.²⁷, we then grew a series of t_active La-doped BSO/124 nm BSO/STO(001) films keeping the dopant density (T_La=1230 °C) fixed (Fig. 1b). No significant change in n_3D and μ_300 K was observed indicating no surface depletion effect or enhanced scattering at the buffer/active interface. That is, the active layer appears to be spatially uniform from a transport point of view. For rest of the study, we have chosen t_buffer=124 nm and t_active=124 nm unless otherwise stated.

Figure 1. Effect of buffer and active layer thicknesses on room temperature carrier density and mobility.

Variation of n_3D (black open circles) and μ_300 K (red solid circles) in doped BaSnO₃ films as a function of (a) t_buffer for fixed t_active=31 nm, and (b) t_active for fixed t_buffer=124 nm. Inset shows a schematic of the structure.

Metal-insulator transition and charge compensation in doped BaSnO3 films

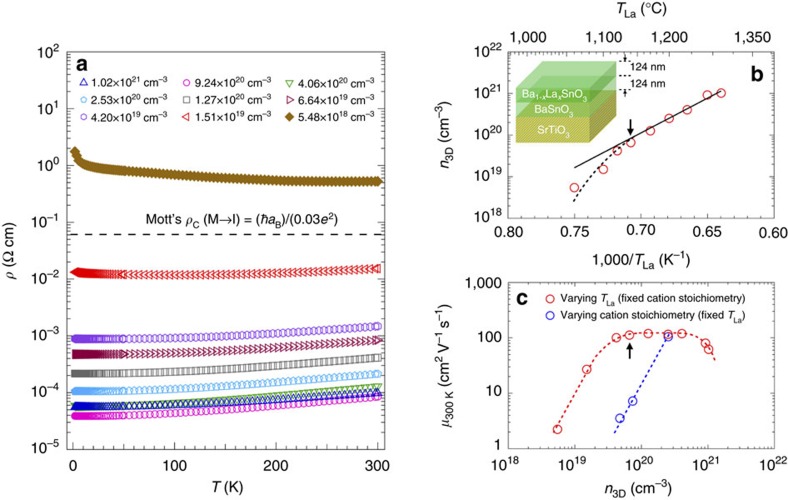
To systematically investigate the effect of charge compensation due to dislocations on electronic transport, we prepared a doping series of 124 nm Ba_1−xLa_xSnO₃/124 nm BSO/STO(001) heterostructures. The value of x, and thereby n_3D was controlled by varying T_La at a fixed Ba/Sn beam equivalent pressure (BEP) ratio. The oxygen flux was also kept fixed. Figure 2a shows the resistivity (ρ) versus T as a function of n_3D. Dashed line in Fig. 2a at 0.06 Ω-cm corresponds to the calculated values of ρ for metal-to-insulator transition based on Mott's minimum metallic conductivity (σ_min) criterion for a degenerately doped semiconductor²⁸:

Figure 2. Temperature dependence of resistivity and the influence of charge compensation on room temperature carrier density and mobility.

(a) Resistivity (ρ) of doped BaSnO₃ films as a function of temperature (T) for different free carrier concentration measured at 300 K. (b) Free carrier concentration (n_3D) at 300 K as a function of La cell temperature (T_La) representing dopant concentration. Inset shows the schematic of the structure used, and (c) μ_300 K versus n_3D for stoichiometric (red open circles) and Ba-deficient films (blue open circles). Non-stoichiometric films had 31 nm of buffer and active layer thicknesses. Black arrows mark the onset of charge compensation.

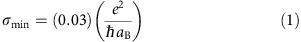where a_B is the Bohr radius with its value between 2.5 × 10⁻⁷ cm and 5.0 × 10⁻⁷ cm for BSO assuming an effective mass (m*) of 0.2 m_e–0.4 m_e (refs ^{22, 29}), and a dielectric constant of 20 (ref. ³⁰). A small upturn in ρ(T) was observed at low-temperatures and attributed to weakly localized transport. ρ(T) increases with decreasing n_3D leading to a metal-to-insulator transition between 5.48 × 10¹⁸ cm⁻³<n_3D<1.51 × 10¹⁹ cm⁻³. The theoretical critical carrier density (n_C) for the Mott metal-to-insulator transition in an uncompensated, degenerately doped semiconductor is shown in ref. 28:

Using equation 2, we calculate n_C for metal-to-insulator transition between 1 × 10¹⁷ cm⁻³ to 1.0 × 10¹⁸ cm⁻³, which is an order of magnitude smaller than our experimental value. This result suggests the existence of charge compensation in agreement with the presence of dislocations, as also observed in doped compensated semiconductors³¹,³².

Next, we discuss the influence of charge compensation on n_3D and μ_300 K. Figure 2b shows a semi-log plot of room temperature n_3D as a function of inverse of T_La. It is noted that 1/T_La is directly related to the dopant density (N_dopant) as 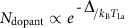, where Δ and k_B are activation energy of evaporation of La, and the Boltzmann constant respectively. For low 1/T_La (high N_dopant), n_3D first decreases linearly on a logarithmic scale, as one would expect with increasing 1/T_La if La is the source of electron and if it is fully-activated (See Supplementary Fig. 5). For high 1/T_La (low N_dopant), n_3D however decreases faster, that is, deviates from linearity, indicating electrons are being trapped at the charged dislocations present in the film resulting in lower carrier concentration. This trend is in agreement with the charge compensation being operative below n_3D≤6.64 × 10¹⁹ cm⁻³ (marked by an arrow) and is remarkably similar to the behaviour observed in doped III–N systems with dislocations¹⁷,¹⁸,¹⁹,²⁰,³³. Fig. 2c shows μ_300 K versus n_3D for two series of samples: doped Ba_1−xLa_xSnO₃ with a fixed cation stoichiometry but different x (red circle); and doped Ba_1−x−yLa_xSnO₃ with a fixed x but different y≥0 (blue circle). It is noted that y=0 corresponds to the cation stoichiometric composition with n_3D=2.53 × 10²⁰ and μ_300 K=105 cm² V⁻¹ s⁻¹. The value of y, and thereby Ba vacancies 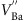was controlled by varying the Ba/Sn BEP at a fixed Ba BEP. We first discuss the stoichiometric samples (red circle) with different n_3D. We observe with decreasing carrier density, 4 × 10²⁰ cm⁻³<n_3D<1 × 10²¹ cm⁻³, mobility first increases; then remain unchanged between 6.64 × 10¹⁹ and 4.06 × 10²⁰ cm⁻³ followed by a steeper decrease at n_3D≤6.64 × 10¹⁹ cm⁻³ (marked by an arrow). Remarkably, the carrier density at which μ_300 K begins to decrease is identical to the density at which non-linearity occurs and the compensation kicks-in, as illustrated in Fig. 2b. This result suggests the decrease in mobility for low n_3D is due to scattering and compensation from charged defects largely owing to dislocations in the film, and is remarkably similar to the prior results obtained from GaN thin films¹⁹.

To further reveal scattering due to charged defects in low doping regime, we now turn to the second series of samples, Ba_1−x−yLa_xSnO₃ where x was kept constant, and y (thereby 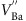) was varied (blue circle in Fig. 2c). We observe a reduction of n_3D accompanied by a decrease in mobility with increasing 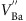, in agreement with the theoretical prediction of Ba-vacancies being an acceptor-like defect³⁴. Most importantly, we observe that the functional dependence of μ_300 K versus n_3D is similar for both sets of samples in the low doping regime. Besides indicating a similar scattering potential around dislocation cores and Ba-vacancies, this result also suggests that the dislocation cores in nominally stoichiometric films may consist of Ba-vacancies. Future experimental and theoretical studies should clearly focus on studying the composition of dislocation cores and electrostatic scattering potentials around them.

Mobility-limiting scattering mechanisms

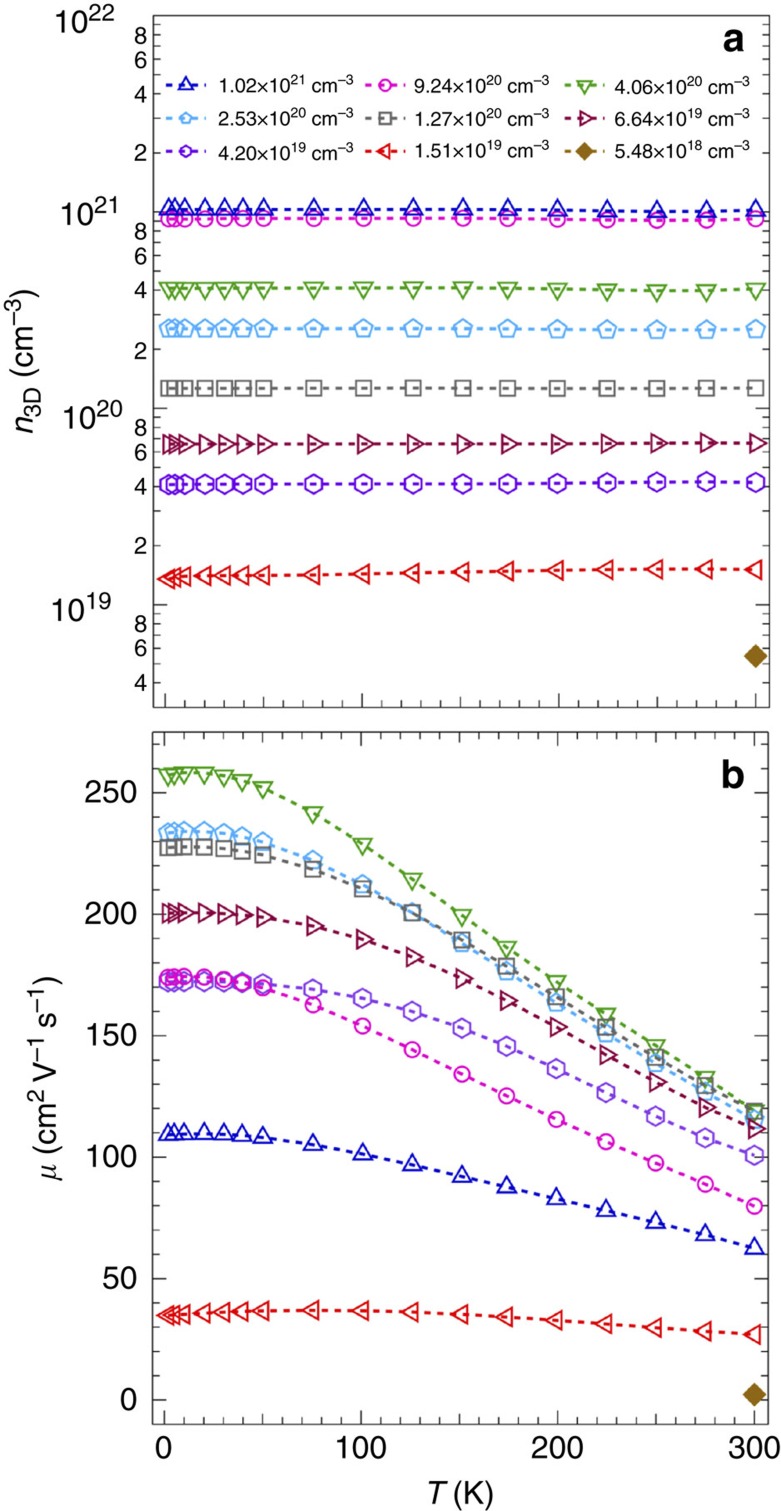
To get more insight into the transport in BSO films, we measured the temperature dependence of n_3D and μ for different dopant concentrations, as shown in Fig. 3. No carrier freeze-out was found down to 1.8 K for all measured electron concentrations (Fig. 3a), indicating that La-doped BSO forms a degenerate semiconductor. No T-dependence of the sample with electron concentration of 5.48 × 10¹⁸ cm⁻³ is shown due to the non-ohmic contacts at T<300 K. A strong T-dependence of μ was observed for all films (Fig. 3b) indicating different scattering mechanisms at play.

Figure 3. Temperature dependence of carrier concentration and mobility.

(a) n_3D, and (b) μ as a function of temperature (T) for doped BSO films with different n_3D.

To examine the role of individual carrier scattering mechanisms, we calculated μ as a function of T. For this purpose, we used AMSET: ab initio model for calculating the mobility and Seebeck coefficient using the Boltzmann transport equation (BTE)³⁵,³⁶. AMSET has proven reliable for the calculation of mobility and Seebeck coefficient of several other semiconductors, including ZnS (ref. ³⁷), GaAs and InN (ref. ³⁵), whose transport properties are also governed by a single conduction band, like BSO. The model setup and parameters used are summarized in the method section, with additional details found in the literature³⁵. In this model, the DFT band structure is used to calculate the group velocities of the carriers, which in turn are used to compute various carrier-scattering rates that enable us to explicitly solve the BTE; we then calculate the perturbation to the electronic distribution in the presence of low-electric field or thermal gradient, which gives us the mobility or Seebeck coefficient, respectively, of BSO. We considered both inelastic scattering (longitudinal polar optical phonon, (LO)) and elastic scattering (ionized impurity (IMP), acoustic phonon deformation potential (AC), transverse optical phonon (TO), piezoelectric (PE) and charged dislocation (DIS)) mechanisms. In our model, charged threading dislocations are treated as acceptor-like (negative charge) defects. We note that it is a reasonable assumption owing to its influence on the carrier density³⁸. Moreover, it is also natural to think that in the presence of charged dislocations (negative charge), ionized dopants (positive charge) and electronic carrier (negative charge) in the doped BSO film, there must also exist other types of donor-like (positive charge) defects to maintain charge neutrality. We therefore can write a charge neutrality condition³⁵:

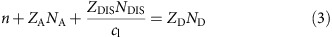where Z represents the charge; A stands for acceptor (− charge) and D for donor (+ charge); c_l is the lattice constant of BaSnO₃. Note the donor (N_D) here represents both charged dopants and other donor-like defects to compensate for charged dislocations. For n-doped BSO films, N_D≫N_A and thus acceptor term Z_AN_A can be neglected. Using the formulation from refs ^{35, 39}, we define an overall ionized impurity concentration, N_IMP in our films as (assuming N_A=0 as discussed above):

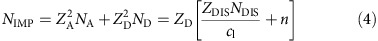where n is the electron concentration of the samples and Z_D is the charge state of the donor. Equation 4 shows how N_IMP depends not only on the electron concentration, n, but also on the concentration of charged threading dislocations, N_DIS. As seen in equation 5 (in method section), IMP scattering rate is proportional to N_IMP.

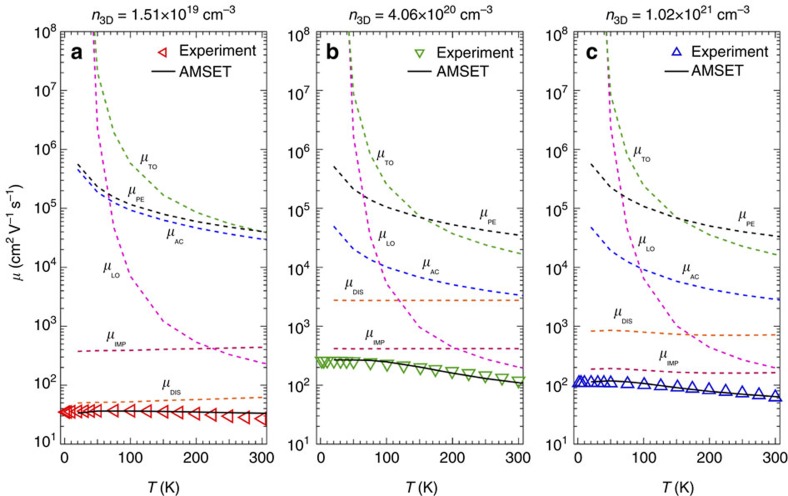
Figure 4 shows experimental (symbols) and calculated values (solid line) of μ versus T for three representative samples with n_3D belonging to low, intermediate and high density regimes of Fig. 2c. We also show individual contributions to the overall mobility due to different scattering mechanisms (dashed lines). We only show calculated mobility down to 20 K because below this temperature, the Fermi–Dirac distribution becomes step-like and the numerical integration of the distribution and density of state to calculate n_3D becomes unreliable. As N_DIS, Z_D and Z_DIS are not known for these samples, we use them as a fitting parameter for calculating μ, which yields Z_DZ_DISN_DIS between 7 × 10¹¹ and 3 × 10¹² cm⁻². Ionized impurities and dislocations both are charged defects; the overall functional dependence of μ on T thus remains same for the two scattering mechanisms. LO phonon scattering has a weak dependence on n_3D, and therefore remains nearly same for all three films. AC scattering, TO phonon scattering, and PE scattering, on the other hand, are dependent on n_3D. However, their dependence on n_3D becomes weaker at higher carrier concentrations.

Figure 4. Temperature dependence of mobility and individual contributions to the mobility from different scattering mechanisms.

μ versus T for doped BaSnO₃ films, (a) n_3D=1.51 × 10¹⁹ cm⁻³, (b) n_3D=4.06 × 10²⁰ cm⁻³, and (c) n_3D=1.02 × 10²¹ cm⁻³. Contributions from different carrier scattering mechanisms are shown with dotted curves. Dislocation density as calculated from the model were—(a) Z_DZ_DISN_DIS=2.3 × 10¹² cm⁻², (b) Z_DZ_DISN_DIS=7.0 × 10¹¹ cm⁻², and (c) Z_DZ_DISN_DIS=2.8 × 10¹² cm⁻² respectively for the three samples, where Z_D and Z_DIS are the overall charge states of the donors and charged dislocations respectively. See method section for more details.

Looking at the dominant scattering processes for films with low doping (n_3D=1.51 × 10¹⁹ cm⁻³) and high doping (n_3D=1.02 × 10²¹ cm⁻³), dislocation scattering and ionized impurity scattering limit the mobility respectively at all temperatures (Fig. 4a,c). However, for films with intermediate doping (n_3D=4.06 × 10²⁰ cm⁻³), mobility is mostly limited by ionized impurity scattering below 200 K (Fig. 4b), while at higher temperatures mobility is governed by LO phonon scattering. Moreover, from Fig. 4a–c, dependence of μ_IMP on the concentration decreases for n_3D=1.02 × 10²¹ cm⁻³, whereas it remained nearly unchanged between n_3D=1.51 × 10¹⁹ cm⁻³ and n_3D=4.06 × 10²⁰ cm⁻³. This seems counter intuitive as one would naively think IMP scattering should increase with increasing n and therefore μ_IMP should decrease proportionately. We interpret this behaviour owing to a combined effect of N_IMP and the charge screening on IMP scattering with different doping density. For instance, using equation 4, the values of N_IMP are 7 × 10¹⁹ cm⁻³ and 4.33 × 10²⁰ cm⁻³ for samples presented in Fig. 4a,b respectively (assuming Z_D=1) suggesting higher μ_IMP in Fig. 4a if N_IMP is the only mobility-limiting factor. However on the other hand, the calculated inverse charge screening lengths, β for samples presented in Fig. 4a–c are about 0.57 nm⁻¹, 0.98 nm⁻¹, 0.98 nm⁻¹ respectively at 300 K implying increased IMP scattering rates (See method section) and lower μ_IMP for the sample in Fig. 4a. Using the same argument, lower μ_IMP in Fig. 4c can also be understood owing to increased N_IMP. See the method section for more information on charge screening.

Figure 4a–c further revealed n-dependence of μ_DIS increases from n_3D=1.51 × 10¹⁹ cm⁻³ to 4.06 × 10²⁰ cm⁻³ and then decreases for n_3D=1.02 × 10²¹ cm⁻³. Using β, we calculate the DIS scattering rate, which is larger for sample with n_3D=1.51 × 10¹⁹ cm⁻³; and almost similar for other two samples. In addition, we found Z_DZ_DISN_DIS of 2.3 × 10¹², 7.0 × 10¹¹ and 2.8 × 10¹² cm⁻² for samples in Fig. 4a–c, respectively. Combining the effect of N_DIS and the charge screening on DIS scattering, μ_DIS is thus expected to possess the lowest value for sample with n_3D=1.51 × 10¹⁹ cm⁻³, increasing for n_3D=1.02 × 10²¹ cm⁻³ and being largest for n_3D=4.06 × 10²⁰ cm⁻³, as illustrated in Fig. 4.

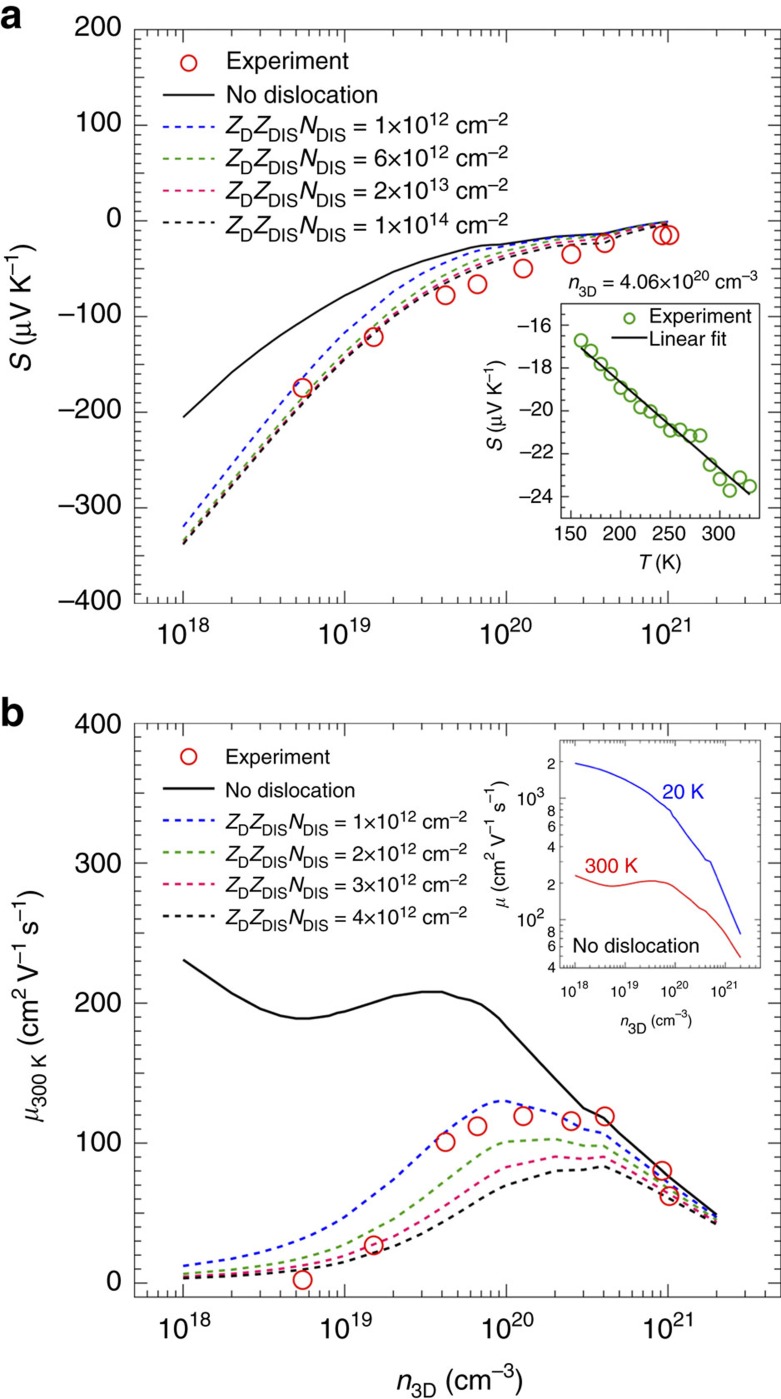
As a further check of our model, we perform measurements and calculations of the Seebeck coefficients (S) as a function of n_3D (Fig. 5a). Inset shows T-dependent Seebeck coefficient for a representative sample with n_3D=4.06 × 10²⁰ cm⁻³, indicating negative S consistent with n-type carriers. Calculations of S were performed accounting for all the scattering mechanisms as described above for calculating μ, with and without N_DIS. Significantly, overall trend of experimental value of S versus n_3D is similar to the calculated values with dislocations, in agreement with our transport results. The calculation further reveals that the Seebeck coefficient is independent of N_DIS in the high doping regime whereas increasing N_DIS seems to enhance and then saturate the Seebeck coefficient in the low doping regime.

Figure 5. Comparison of experimental and calculated values of Seebeck coefficient and mobility as a function of carrier concentration with and without dislocations.

(a) Seebeck coefficients (S) of doped BaSnO₃ films at 300K as a function of n_3D. Experimental data are shown as red circles and calculated values are represented using solid line for no dislocation, and using dotted lines for different Z_DZ_DISN_DIS. Inset shows the T-dependent S for n_3D=4.06 × 10²⁰ cm⁻³, consistent with n-type conduction. (b) Room-temperature mobility (μ_300 K) as a function of n_3D. Experimental data are shown in red circles while calculated values are represented with solid (without dislocation) and dotted lines (with varying dislocation density). Inset shows the variation of μ at two different temperatures (300 K and 20 K) for films with no dislocation.

To further examine the self-consistency of AMSET model, we plot in Fig. 5b the calculated dependence of μ_300 K versus n_3D for different N_DIS. μ_300 K is also calculated for the case, when no dislocations are present. We overlay experimental values of μ_300 K (red circles) on the same plot for comparison. The calculated values are again in good agreement with the experiment. The result suggests that our films may have different N_DIS despite of identical t_buffer, which may be due to different doping levels. We note that the calculated mobilities using AMSET are sometimes underestimated as the record room temperature mobility in bulk BSO has been 320 cm² V⁻¹ s⁻¹ at n_3D=8 × 10¹⁹ cm⁻³, whereas the calculated value in Fig. 5b is 195 cm² V⁻¹ s⁻¹ at a similar doping level, so these values should be treated as lower bounds. Most importantly, in addition to providing numerous insights into electronic transport behaviour of BaSnO₃, our calculations also suggest directions for future experiments. For instance, calculations reveal the overall trend of μ_300 K versus n_3D if there were no dislocations in the film. It tells that with decreasing n_3D, μ_300 K with no dislocation will increase owing to reduced impurity scattering. For 5 × 10¹⁸ cm⁻³<n_3D<4 × 10¹⁹ cm⁻³, μ_300 K is expected to decrease due to stronger interaction between low density electrons (that is, lower Fermi level) and optical phonons until electron-phonon interactions become much weaker (that is, reduced LO phonon scattering) resulting again in an increase of μ_300 K for n_3D<5 × 10¹⁸ cm⁻³. In contrast, mobility at low temperature (20 K) is expected to show a monotonically increasing behaviour with decreasing n_3D, reaching towards a value of 2,000 cm² V⁻¹ s⁻¹.

New outlook and opportunities

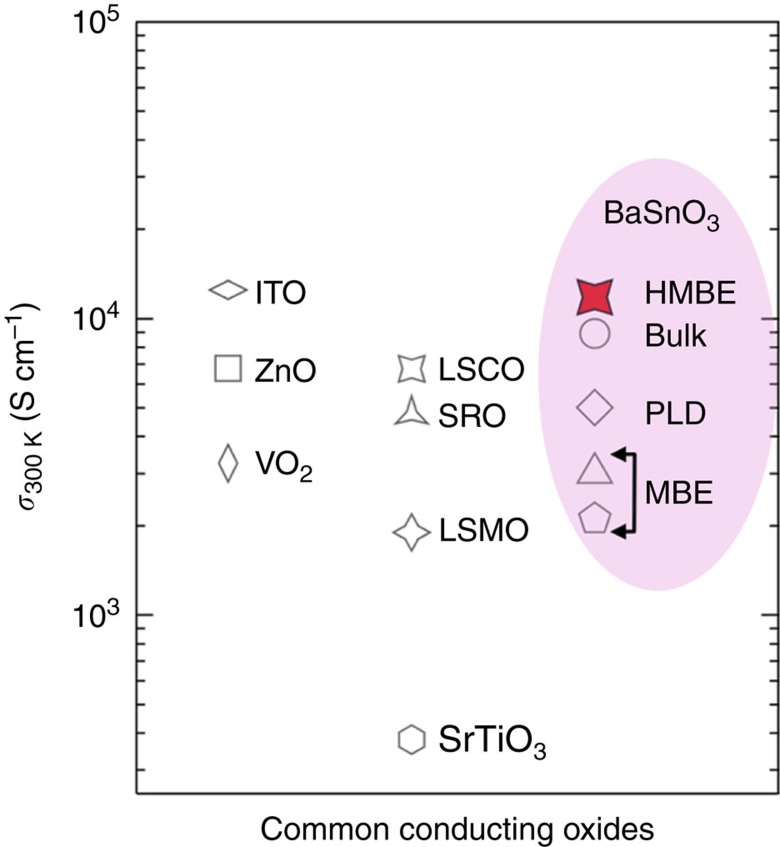
To benchmark our films with respect to the best wide gap oxide conductors, we show in Fig. 6 the highest reported value of room temperature conductivity σ (300 K) for different oxides, which are often used as metallic electrodes and TCOs in electronic applications. We also show the highest value of σ (300 K) of bulk BSO single crystals and thin films grown using different routes. Remarkably, the film grown using hybrid MBE possesses higher conductivity than those reported to-date both in the bulk BSO crystal and films, and is comparable to that of ITO, an industry standard for TCOs. We note that although high conductivity is achieved owing to high doping density and mobility, the ability of BSO to afford high conductivity without any phase segregation is very promising for fundamental study and application. For example, conductive BSO could be an ideal electrode material in oxide electronics providing not only electrode function but also transparency, which could be vital for applications such as solar cells or display technologies. In addition, high conductivity of BSO could also be important for fundamental study and applications at high frequencies. High-frequency measurements and devices require low resistance contacts, where epitaxially-grown highly conductive BSO can play a significant role. Moreover, high conductivity of BSO films can also be beneficial for the investigation of ultra-fast phase dynamics of multiferroics without potential issues of charging effect. The major advantage of using conductive BSO as opposed to metals is its structural compatibility with these materials. Finally, the ability of BSO to accommodate high density of electrons and mobility along with wide bandgap makes it a suitable material candidate for ultra-high density quantum wells for plasmonic and power electronics applications.

Figure 6. Comparison of conductivity values for common conducting oxides.

Compilation of highest room temperature conductivity σ_300 K of BaSnO₃ bulk single crystal and films grown by various deposition techniques (taken from refs ⁹, ¹⁶, ²²) including hybrid molecular beam epitaxy. The highest known σ_300 K for common transparent conducting oxides (ITO=indium tin oxide, ZnO) and other metallic oxides as conductive electrodes for high frequency applications (VO₂, LSCO=La_0.5Sr_0.5CoO₃, SrTiO₃, LSMO=La_0.7Sr_0.3MnO₃, SRO=SrRuO₃) are shown for comparison (refs ⁴⁹, ⁵⁰, ⁵¹, ⁵², ⁵³, ⁵⁴, ⁵⁵).

Discussion

In summary, we have demonstrated the hybrid MBE approach for reproducible and controlled doping of epitaxial BSO on STO(001) with La. We establish a correlation between dislocation, , carrier density, mobility and metal-insulator transition in BSO. The combination of controlled doping, detailed electronic characterization, with modelling using the Boltzmann transport equations and an ab initio band structure calculations allow us to understand, tune and predict the transport behaviour and mobility limiting mechanisms in BSO. An important outcome of the study is that room temperature mobility in BSO films is limited by both defects, and electron-phonon scattering. The results place doped-BSO on par with highly successful III-Nitrides in terms of mobilities but with the added benefit of having high carrier concentrations for high-power oxide electronics operating at room temperature.

Methods

Growth and characterization of BaSnO₃ films

Details of our hybrid MBE approach for stoichiometric BSO heteroepitaxy are discussed elsewhere²¹,²⁵; a brief description will be provided here. BSO films were grown via hybrid molecular beam epitaxy (hybrid MBE). Hexamethylditin was used as the metal-organic chemical precursor for Sn. Ba and La were evaporated using effusion cells, and oxygen was supplied using an RF plasma source. The oxygen pressure and substrate temperature were fixed at 5 × 10⁻⁶ torr and 900 °C respectively for all growths. A 350-nm-thick Ta layer was deposited on the back of the substrates to improve heat transfer between the film and the substrate heater. Substrates were cleaned in oxygen plasma for 20 min before film growth ensuring no carbon contamination was present at the surface. Dopant density in the films was controlled by varying La-cell temperature (1060 °C≤T_La ≤1290 °C). Films were annealed after growth in oxygen using rapid thermal annealer at 800 °C for 2 min to ensure oxygen stoichiometry. All substrates after film growth and undoped films were confirmed to be insulating (ρ>10⁵ Ω cm). Electronic transport measurements were performed in a Quantum Design Physical Property Measurement System (DynaCool) using indium as ohmic contacts in a van der Pauw geometry. Thermopower measurements were performed with a home built system described elsewhere⁴⁰.

AMSET and DFT calculation details

The AMSET model employs materials parameters that were obtained from the experimental literature, as listed in Table 1. In this work, the primary purpose of the AMSET model is to explain the observed experimental phenomena, so it is appropriate to use experimental parameters even though the same values can be calculated ab initio with only minor deviations to the resulting mobility and Seebeck coefficient. For instance, deformation potential (E_D) is calculated ab initio. We, however, emphasize that the choice of deformation potential in our calculations, even if an order of magnitude different than the value reported in Table 1 does not influence our interpretation of mobility data. It is noted that the mobility due to AC scattering, which uses E_D is not limiting at any temperature or concentration. The same holds true for the values of the elastic constants that were extracted from DFT generalized gradient approximation of Perdew, Burke and Ernzerhof (GGA-PBE) calculations⁴¹; these have essentially no effect on the overall mobility, as the mobility due to AC scattering is always orders of magnitude higher than the actual limiting mechanisms.

Table 1. Input parameters to our AMSET model using ab initio calculations and using experimental values.

Model parameters	Ab initio	Experiments56
Polar optical phonon frequency, ωPO (THz)	20.36	12.23
Low-frequency dielectric constant, ɛ0	5.2	17±2
High-frequency dielectric constant, ɛ∞	3.4	3.3
Deformation potential, ED (eV)	6.19	–

The bold numbers indicate parameters that are used in our calculations.

To calculate the electronic band structure of BSO, the unit cell was optimized using Kohn–Sham density functional theory (KS-DFT)⁴²,⁴³ as implemented in the Vienna ab initio Simulation Package⁴⁴,⁴⁵,⁴⁶,⁴⁷. We used the generalized gradient approximation of Perdew, Burke and Ernzerhof⁴⁸ (GGA-PBE) to express the exchange-correlation potential and Projector Augmented Wave potentials to represent the wave functions. On geometry optimization, the lattice constant (0.419 nm) was increased by 1.76% compared to the experimental value (0.412 nm). This is a common behaviour when using a GGA-PBE functional, due to delocalization of electrons. We used a k-point mesh of 6 × 6 × 6 for self-consistent calculations, as adding more k-points results in <0.01 eV difference in total energy. The energy cutoff for the plane wave basis set was set to 520 eV in all calculations.

For electronic properties calculations, we chose the k-points closest to the conduction band minimum (CBM), which for BaSnO₃ is at the Γ-point. An adaptive k-points mesh was used, which is denser close to the CBM. We used the AMSET code³⁵ to calculate the mobility and the Seebeck coefficient, which uses the DFT band structures to solve the BTE. The explicit solution to BTE is particularly important for the inelastic LO-phonon scattering mechanism as it is the major limiting mechanism for the sample with n_3D=4.06 × 10²⁰ cm⁻³, for T>200 K (Fig. 4b). More information on the LO-scattering can be found elsewhere³⁵,³⁹. On the other hand, two other major elastic scattering mechanisms in BSO are ionized impurity (IMP) and charged dislocation scattering (DIS). We note that it is the threading dislocations in the active layer, which limit the mobility. Misfit dislocations on the other hand are pinned at the film/substrate interface making themselves electrically separated from the active layer where electronic transport is measured. We write the expressions used to model these scattering phenomena from refs ^{35, 39} to clarify how these scattering rates and necessary parameters are calculated. The IMP and DIS scattering rates, ν, are expressed via the following equations:

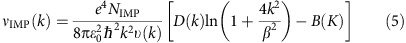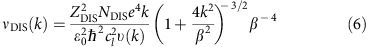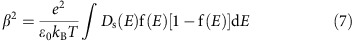where k is the magnitude of the wave vector, E is the energy, β is the inverse screening length due to charge defects, ɛ₀ is the static dielectric constant, e is the charge of an electron, c_l is the lattice parameter of BSO, υ(k) is the group velocity of electron which is directly derived from the DFT band structure, Z_DIS is the charge state of dislocations, B(K) and D(K) are parameters describing the non-parabolicity of the conduction band, which are directly derived from the DFT band structure, D_s is the density of states and k_B is the Boltzmann constant. We further note that to calculate the mobility values, Z_DZ_DISN_DIS is used as a fitting parameter instead of N_DIS as the charge states of the donor (defined as charged dopants and other donor-like defects to compensate for charged dislocations) and dislocations are not known. It is interesting to note that the screening lengths are the same in Fig. 4b,c both of which have very high concentrations indicating a highly degenerate semiconductor or essentially a metal. In fact, the calculated Fermi level for Fig. 4a is 0.15 eV inside the conduction band (above the CBM) while that is 0.94 and 1.03 eV for samples with higher n_3D. At such high concentrations, the departure from uniformity of the free-electron concentration in presence of a potential surrounding an impurity, δn, becomes insensitive to the concentration itself. δn is directly related to charge screening. See ref. 38 for more details.

Data availability

The data that support the main findings of this study are available from the corresponding authors on request.

Additional information

How to cite this article: Prakash, A. et al. Wide bandgap BaSnO₃ films with room temperature conductivity exceeding 10⁴ S cm⁻¹. Nat. Commun. 8, 15167 doi: 10.1038/ncomms15167 (2017).

Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Supplementary Material

Supplementary Information

Supplementary Figures and Supplementary Reference