Abstract
The hydrophobic interaction drives nonpolar solutes to aggregate in aqueous solution, and hence plays a critical role in many fundamental processes in nature. An important property intrinsic to hydrophobic interaction is its cooperative nature, which is originated from the collective motions of water hydrogen bond networks surrounding hydrophobic solutes. This property is widely believed to enhance the formation of hydrophobic core in proteins. However, cooperativity in hydrophobic interactions has not been successfully characterized by experiments. Here, we quantify cooperativity in hydrophobic interactions by real-time monitoring the aggregation of hydrophobic solute (hexaphenylsilole, HPS) in a microfluidic mixer. We show that association of a HPS molecule to its aggregate in water occurs at sub-microsecond, and the free energy change is −5.8 to −13.6 kcal mol−1. Most strikingly, we discover that cooperativity constitutes up to 40% of this free energy. Our results provide quantitative evidence for the critical role of cooperativity in hydrophobic interactions.
Hydrophobic interactions occur between nonpolar molecules in water and their experimental quantification can help the understanding of biological self-assembly. Here Jiang et al. examine the kinetics and thermodynamics of hydrophobic aggregation in a bulk environment and characterize its cooperativity.
Hydrophobic interactions drive nonpolar solutes to aggregate in aqueous solution1,2, and hence play an important role in many fundamental processes in nature. Molecular theories3,4,5,6,7,8 and computer simulations9,10 of hydrophobicity suggest that water molecules need to break some of their hydrogen bonds to accommodate large nonpolar solutes (radius larger than 1 nm). This loss of water–water hydrogen bonds will induce fluctuations and depletion of water density near large hydrophobic solutes10,11,12,13, and further lead them to collapse14,15. Therefore, hydrophobic interactions are originated from the collective motions of water hydrogen bond networks surrounding hydrophobic solutes16,17,18,19; hence, they are naturally cooperative20,21,22. This is in contrast to fundamental intermolecular interactions that are often treated as pairwise additive, such as ionic interactions, dipolar interactions and dispersion forces.
Cooperativity is a phenomenon whereby the overall interaction for a system containing multiple molecules is stronger than the summation of individual pairwise interactions. Such cooperative feature of hydrophobic interactions is widely believed to accelerate, stabilize and enhance the formation of hydrophobic core in proteins, the aggregation of misfolded proteins and the formation of lipid vesicles and micelles. However, the extent of contributions from cooperativity to these processes still remains unclear. Addressing this issue requires, first and foremost, quantitative measurements of hydrophobic interactions, which are very challenging as the size, chemistry and topography of solutes can affect the strength of hydrophobic interactions3,23,24,25,26,27.
Microfluidic mixing techniques have been widely applied to investigate many important chemical and biological processes, including protein and RNA folding28,29,30,31,32, enzyme activities33,34 and vesicle formations35,36. In this study, we quantified the thermodynamics and kinetics of hydrophobic interactions in bulk solution by real-time monitoring of fluorescence induced by the aggregation of hexaphenylsilole37 (HPS, C40H30Si) in a state-of-the-art microfluidic mixer at microsecond timescale. Using this technique, we show that in the attachment of a HPS molecule to its aggregate, the free energy change is −5.8 to −13.6 kcal mol−1, the timescale is sub-microsecond, and the cooperativity constitutes up to 40% of the free energy change.
Results
Microfluidic experiment and model fitting
We employed HPS molecules (Fig. 1a left) to study hydrophobic interactions in bulk solutions using a microfluidic mixer as depicted in Fig. 1b (Supplementary Note 1). The aggregation of HPS molecules is mainly driven by solvent-induced hydrophobic interactions, as six aromatic rings render its hydrophobic property and direct π−π stacking interactions between HPS molecules are negligible38. Importantly, HPS molecules emit strong fluorescence upon aggregation37. This unique feature allows us to track the progress of HPS aggregation in the microfluidic mixer (Fig. 2a), where the molecular aggregation occurs in a sample stream that was hydrodynamically sheathed to tens of nanometres in width within a few microseconds upon rapid solvent exchange. We can determine the time course of HPS aggregation via dividing the travelling distance of mixture solution along the exit microchannel by its flow velocity. The measured fluorescence intensities (Fig. 2b,c, symbol points) were linearly correlated with the total amount of aggregated HPS. This linear correlation was validated by quantum mechanics/molecular mechanics calculations39, fluorescence AFM experiments and spectrophotometer experiments (Supplementary Figs 1–4, 13, 19 and 20; Supplementary Notes 2 and 6).
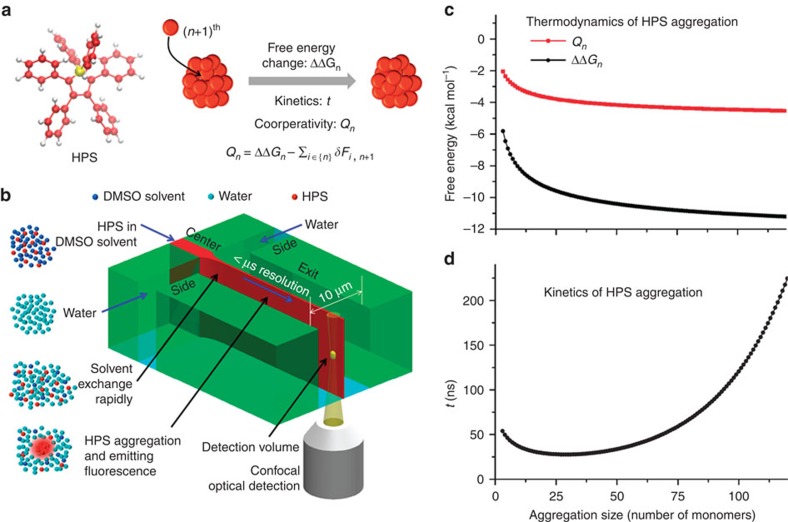
Figure 1. Quantifying cooperativity in hydrophobic interactions by monitoring HPS aggregation.
(a, left) The chemical structure of a HPS molecule. (right) A scheme of HPS aggregation thermodynamics and kinetics. Cooperativity (Qn) is defined as the difference between the associated free energy of attaching a HPS monomer to the aggregate (ΔΔGn) and the summarization of the two-body potential of mean force 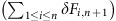 upon the attachment of the (n+1)th monomer to the aggregate. (b) The principle of real-time monitoring of HPS aggregation in the microfluidic mixer. HPS (red dots) dissolved in DMSO (blue dots) is continuously pumped into the centre microchannel, and then squeezed by two side water (cyan dots) streams to form an extremely narrow stream with tenths of nanometres in width. Thus, rapid solvents exchange occurs in a pure diffusion manner, and HPS molecules aggregate in downstream with strong fluorescence emission under confocal optical microscopy. Blue arrows indicate the directions of continuous fluid flow. Black arrows indicate specific locations. (c,d) Thermodynamics (ΔΔG) (c), cooperativity (Qn) (c) and kinetics (t) (d) of attaching a HPS monomer to aggregates in pure water.
upon the attachment of the (n+1)th monomer to the aggregate. (b) The principle of real-time monitoring of HPS aggregation in the microfluidic mixer. HPS (red dots) dissolved in DMSO (blue dots) is continuously pumped into the centre microchannel, and then squeezed by two side water (cyan dots) streams to form an extremely narrow stream with tenths of nanometres in width. Thus, rapid solvents exchange occurs in a pure diffusion manner, and HPS molecules aggregate in downstream with strong fluorescence emission under confocal optical microscopy. Blue arrows indicate the directions of continuous fluid flow. Black arrows indicate specific locations. (c,d) Thermodynamics (ΔΔG) (c), cooperativity (Qn) (c) and kinetics (t) (d) of attaching a HPS monomer to aggregates in pure water.
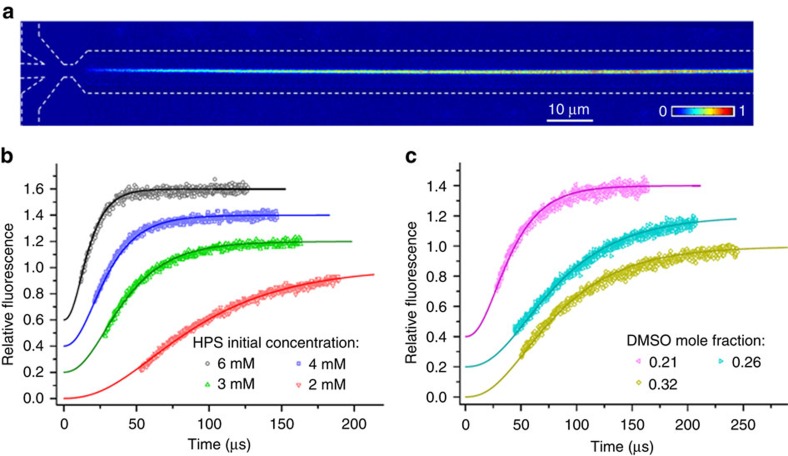
Figure 2. Time evolution of fluorescence intensity measured by experiment and fitted by theory.
(a) A representative fluorescence image of HPS aggregation in the microfluidic mixer (with subtraction of background fluorescence). White dashed lines indicate the outline of microfluidic mixer. (b) Kinetic profiles of HPS aggregation at various initial HPS concentrations (the solvent condition: DMSO mole fraction of 0.16). The solute–solvent surface tension (γsl) through theoretical fitting (solid lines) of experimental data (symbol points) are 20.9 (±0.6), 20.7 (±0.6), 20.5 (±0.6), and 20.3 (±0.6) cal mol−1 Å−2 at initial HPS concentration of 6, 4, 3 and 2 mM, respectively. (c) Kinetic profiles of HPS aggregation in various solvent conditions (initial HPS concentration of 6 mM). For clear illustrations, the relative fluorescence curves in part (b) corresponding to HPS concentrations of 3, 4 and 6 mM are shifted along y axis by 0.2, 0.4 and 0.6, respectively. Similarly, relative fluorescence curves in part (c) corresponding to DMSO mole fractions of 0.26 and 0.21 are shifted by 0.2 and 0.4, respectively. The solute–solvent surface tension (γsl) through theoretical fitting (solid lines) of experimental data (symbol points) are 19.5 (±0.6), 18.5 (±0.6) and 17.0 (±0.6) cal mol−1 Å−2 in the solvent condition with a DMSO mole fraction of 0.21, 0.26 and 0.32, respectively. The Pearson correlation coefficients for all fitted curves are larger than 0.98 (Supplementary Fig. 11).
Next, we fitted the measured fluorescence data to the classical nucleation-growth model40 (Fig. 3a; Supplementary Note 3). At each aggregation time point in this model, new nuclei are being formed and simultaneously existing aggregates are growing. Once new nuclei are formed, they continue to grow as long as the solution is supersaturated. Accordingly, the total amount of aggregated HPS per unit volume at time t is the integration of the product of nuclei generated at a previous time s and its size growing during the remaining time τ
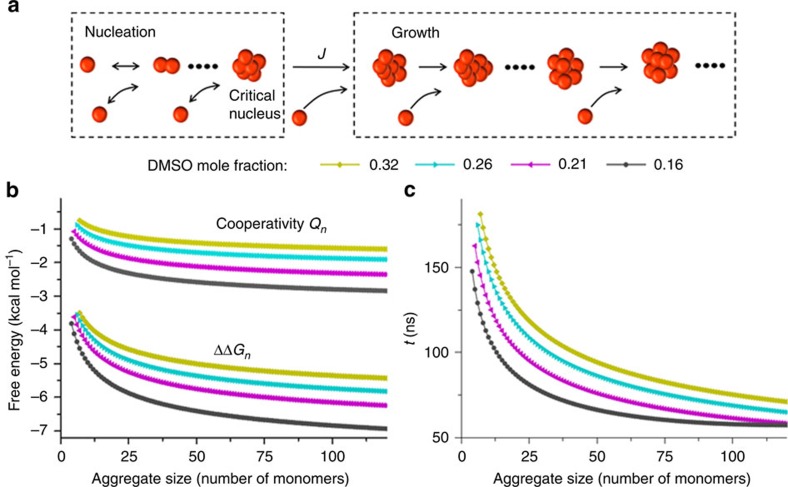
Figure 3. Thermodynamics and kinetics as well as cooperativity of HPS aggregation.
(a) A schematic illustration of the nucleation-growth model for HPS aggregation. At each aggregation time point in this model, new nuclei are being formed at a rate of J determined by the classical nucleation theory, and then are growing in size in a barrier-free and diffusion-controlled manner. (b) The free energy change and cooperativity associated with attaching a HPS monomer to aggregates in various DMSO/water solvent mixtures. (c) The time taken for aggregates to grow by one HPS molecule in various solvent mixtures at initial HPS concentration of 6 mM.
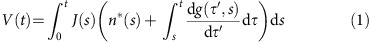 |
where J(s)is the number of nucleus generated in unit volume per time, n*(s) is the critical nucleus size, and 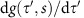 is the growth rate of the nucleus that formed at time s. By fitting the measured fluorescence to the model (Fig. 2b,c, solid lines), we obtained the free energy change associated with attaching a HPS monomer to its aggregate and resolved the kinetics of HPS aggregation in various solvent mixtures of dimethyl sulfoxide (DMSO) and water. The results were then extrapolated to the pure water condition (Supplementary Fig. 5).
is the growth rate of the nucleus that formed at time s. By fitting the measured fluorescence to the model (Fig. 2b,c, solid lines), we obtained the free energy change associated with attaching a HPS monomer to its aggregate and resolved the kinetics of HPS aggregation in various solvent mixtures of dimethyl sulfoxide (DMSO) and water. The results were then extrapolated to the pure water condition (Supplementary Fig. 5).
Free energy change associated with hydrophobic aggregation
We determined that the strength of hydrophobic interaction associated with attaching a HPS monomer to an existing aggregate (for example, 5.8–13.6 kcal mol−1 in pure water, Fig. 1c) is comparable to that of several water–water hydrogen bonds41. As shown in Figs 1c and 3b, hydrophobic interaction increases rapidly with aggregate size and decreases with the addition of DMSO solvent. For instance, the free energy of hydrophobic interaction by attaching a HPS monomer to an aggregate containing 10 molecules (∼29 Å diameter) is −8.2 kcal mol−1 in pure water, while it is only −3.8 kcal mol−1 in the solvent mixture with a DMSO mole fraction of 0.32. As the aggregate grows in size, the hydrophobic interactions become stronger; when the aggregate is infinitely large, they reach the asymptotic values of −13.6 and −6.7 kcal mol−1 in pure water and in the solvent mixture, respectively. In addition, the hydrophobic free energy per solvent accessible surface area (SASA) of a HPS molecule (SASA: 750 Å2) in pure water was calculated to be 18.1 cal mol−1 Å−2, a value close to the estimated one (∼20 cal mol−1 Å−2) for aromatic hydrocarbons from previous experiment42 and the predicted one (∼16 cal mol−1 Å−2) for benzene from MD simulations43. Interestingly, Chandler and co-workers predicted that there should exist a crossover in the length-scale for the hydrophobic effect at around 1 nm in solute radius1,3. Our experiment demonstrates the existence of this crossover by showing a kink during the transition from volume-based hydration free energy for monomer (smaller than 1 nm in radius) to area-based hydration free energy of aggregates (larger than 1 nm in radius, see Supplementary Note 7 and Supplementary Fig. 21 for details).
Cooperativity of hydrophobic interactions
We found that cooperativity constitutes up to 40% of the free energy for the hydrophobic association in aggregate formation. To compute the magnitude of cooperativity, we followed Wang et al.21 to define it as the excess multibody free energy upon attaching a monomer to the aggregate (Fig. 3b). Figures 1c and 3b show the dependence of cooperativity on the size of hydrophobic aggregate in different solvent conditions. The strength of cooperativity in attaching a HPS monomer to aggregates increases from 2.6 kcal mol−1 for aggregate containing five molecules (∼23 Å diameter) to 4.2 kcal mol−1for aggregate containing 50 molecules (∼45 Å diameter) in pure water, whereas the free energy change associated with these processes are –6.9 and –10.4 kcal mol−1, respectively. To evaluate the total amount of cooperativity in the formation of HPS aggregate in pure water, we integrated the contribution of cooperativity in Fig. 1c and compared this accumulated cooperativity with the aggregate formation free energy (that is the integrated hydrophobic interactions in Fig. 1c). As shown in Supplementary Fig. 6, both the aggregate formation free energy and the accumulated cooperativity increase linearly with aggregate size. Interestingly, our reported hydrophobic free energy at large n limit 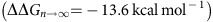 becomes equivalent to the free energy of transferring a HPS molecule from water to the HPS phase. Importantly, cooperativity constitutes up to 40% of the formation free energy in the aggregation process. This finding demonstrates a critical role of cooperativity in hydrophobic aggregation.
becomes equivalent to the free energy of transferring a HPS molecule from water to the HPS phase. Importantly, cooperativity constitutes up to 40% of the formation free energy in the aggregation process. This finding demonstrates a critical role of cooperativity in hydrophobic aggregation.
Kinetics of hydrophobic aggregation
Through our kinetics analysis, the attachment of a HPS molecule to an aggregate was estimated to occur at tens to hundreds of nanoseconds. We investigated the kinetics of HPS aggregation by tracking the growth of the first nucleus formed in the theoretical model (Supplementary Fig. 7). At the initial stage of aggregation when aggregate size is smaller than 10 molecules, the time required for attaching a monomer to aggregates ranges from 30 to 200 ns (Figs 1d and 3c). When the aggregate grows in size, the kinetics of hydrophobic aggregation first accelerate with increased surface area available for attaching, and then gradually slow down due to the depletion of HPS monomers. Also, the time required for HPS molecules in pure water to form an aggregate containing 50 molecules (∼45 Å diameter) is 1.9 μs (Supplementary Fig. 7). In addition, the initial HPS concentration also influences the aggregation kinetics (Supplementary Fig. 8). For instance, at an initial HPS concentration of 2 mM in the solvent with 0.16 DMSO mole fraction, the time required for monomers to form an aggregate containing 50 molecules is 11.8 μs (Supplementary Fig. 7). Our observation of microsecond timescale of HPS aggregation is comparable to the timescale of early stage protein folding (see the Methods section for details), during which nonspecific hydrophobic interactions among hydrophobic residues induce the initial collapse of polypeptide chain at microsecond timescale44.
Discussions
Organic molecules, such as proteins and lipid, bury their hydrophobic components to form stable cores. Hydrophobic interaction plays a crucial role in facilitating the collapse of protein chains into a globular shape25,45,46,47. The faster kinetics of hydrophobic aggregations (at microsecond), in contrast to protein folding (at millisecond or longer), suggest that the formation of protein cores by the aggregation of hydrophobic side-chains occurs at the early stage in the process of globular protein folding. Most importantly, we show that hydrophobic interaction, which is an interaction induced by collective behaviours of many water molecules, is strongly cooperative, and thus substantially enhance its strength during the aggregation of dispersed hydrophobic molecules in solution. We anticipate that our findings have profound implications in protein folding, as the protein core formation involves the collapse of hydrophobic side-chains. We acknowledge that these two processes are different in many aspects. For instance, flexible protein chains contain numerous conformations, while HPS molecules are relatively rigid. In addition, multiple sources may contribute to cooperativity in protein folding, such as the cooperative helix melting process due to hydrogen bonding48. Furthermore, even the extent of contributions by hydrophobic interactions to protein folding remains elusive49. In spite of these differences, our findings highlight the important role of hydrophobic cooperativity (as large as 40%) in the initial collapse of protein chain to form into a globular shape. We expect that our experimental platform will have promising applications in studying hydrophobic interactions of a wide range of organic molecules with aggregation-induced emission50, and in investigating the impact of important factors such as temperature on hydrophobic effect.
Methods
Microfluidic experiments
A solution of HPS molecules dissolved in DMSO was continuously pumped into the centre microchannel. This central stream was hydrodynamically squeezed by two side water streams to form an extremely narrow stream with tenths of nanometres in width. Hence, rapid solvents exchange occurred in a pure diffusion manner, and the immediate environment for HPS aggregation was reached within a few microseconds. In the mixer, the time course of HPS aggregation was determined by dividing the travelling distance of mixture solution along the exit microchannel by its flow velocity. Thus, the progress of HPS aggregation in downstream was monitored by the integrated confocal system at sub-microsecond temporal resolution. Fluorescence images were captured with spatial resolutions of 1 and 0.5 μm in vertical (depth) and horizontal (width) directions, respectively. A diode laser at 405 nm was employed as the excitation source. The excitation beam was focused into the centre layer of microchannels by an oil immersion objective lens (× 63/1.4 NA). Then, fluorescence was collected by the same objective and detected by a photomultiplier tube detector at wavelength of 420–600 nm. Pleases refer to Supplementary Note 1 for more details of the microfluidic mixing experimental setup.
The classical nucleation-growth theory
In this paper, we employed the classical nucleation-growth theory40 to model the time evolution of the total amount of aggregated HPS in solution. At each aggregation time point in this model, new nuclei are being formed and the rate of nuclei formation is determined by the classical nucleation theory (CNT). At the same time, existing aggregates are growing in size in a barrier-free and diffusion-controlled manner. Once new nuclei are formed, they continue to grow as long as the solution is supersaturated.
The nucleation work W(n) to form an aggregate containing n molecules, the critical nucleus size n* and the nucleation rate J(t), which is the number rate of new nuclei generated per volume per time, are obtained from the CNT40 with the assumption that HPS monomer and HPS aggregates are spherical as suggested by previous studies51:
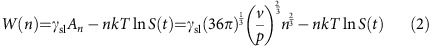 |
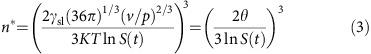 |
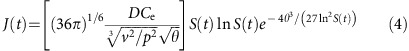 |
where γsl is the surface tension of HPS aggregates in the solvent mixture,  is the surface area of an aggregate containing n molecules, k is the Boltzmann constant, T is the temperature,
is the surface area of an aggregate containing n molecules, k is the Boltzmann constant, T is the temperature, 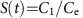 is the supersaturation ratio of HPS monomer in the solvent mixture changing with time t, v is the molecular volume of HPS monomer, p is the packing density of aggregates, which is the fraction of the molecular volume over the average volume that is occupied by a molecule in the aggregate, D is the diffusion coefficient of HPS molecule, Ce is the solubility of HPS in the solvent mixture and θ=γsl(36π)1/3(v/p)2/3/kT is related to the surface energy of aggregates. We adopted the same solute–solvent surface tension γsl for aggregates in the same solvent mixture.
is the supersaturation ratio of HPS monomer in the solvent mixture changing with time t, v is the molecular volume of HPS monomer, p is the packing density of aggregates, which is the fraction of the molecular volume over the average volume that is occupied by a molecule in the aggregate, D is the diffusion coefficient of HPS molecule, Ce is the solubility of HPS in the solvent mixture and θ=γsl(36π)1/3(v/p)2/3/kT is related to the surface energy of aggregates. We adopted the same solute–solvent surface tension γsl for aggregates in the same solvent mixture.
The HPS molecular volume, the packing density and the diffusion constant of HPS molecule were obtained as follows: by assuming that HPS molecules are spherical, the volume of HPS molecule can be calculated as 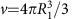 , where R1 is the molecular radius. For spherical aggregate, the packing density was chosen to be p=0.7 as suggested by previous studies43. The diffusion constant of HPS monomer D was calculated from the Einstein–Stokes relation,
, where R1 is the molecular radius. For spherical aggregate, the packing density was chosen to be p=0.7 as suggested by previous studies43. The diffusion constant of HPS monomer D was calculated from the Einstein–Stokes relation, 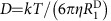 , where η is the viscosity of solvent mixtures obtained from the reported data52, and
, where η is the viscosity of solvent mixtures obtained from the reported data52, and 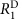 is the diffusive radius of HPS monomer. The molecular radius R1 and the diffusive radius
is the diffusive radius of HPS monomer. The molecular radius R1 and the diffusive radius 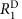 of HPS molecule were computed from the surface area of the HPS molecule with
of HPS molecule were computed from the surface area of the HPS molecule with 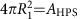 and
and 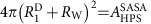 . Here RW=1.4 Å is the radius of water molecule, AHPS is the surface area of HPS molecule, and
. Here RW=1.4 Å is the radius of water molecule, AHPS is the surface area of HPS molecule, and 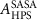 is the SASA of HPS molecule. With DFT calculations using the B3LYP functional53,54,55 and 6–31 g basis set56, we optimized the structure of HPS molecule (Fig. 1a, left), and obtained AHPS=437 Å2 and
is the SASA of HPS molecule. With DFT calculations using the B3LYP functional53,54,55 and 6–31 g basis set56, we optimized the structure of HPS molecule (Fig. 1a, left), and obtained AHPS=437 Å2 and 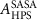 =750 Å2. These values give the radius of the HPS molecule R1=5.9 Å and the diffusive radius
=750 Å2. These values give the radius of the HPS molecule R1=5.9 Å and the diffusive radius 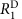 =6.3 Å, respectively. The calculated diffusion constants of HPS monomer in different solvent mixtures are listed in Supplementary Table 1.
=6.3 Å, respectively. The calculated diffusion constants of HPS monomer in different solvent mixtures are listed in Supplementary Table 1.
For the growth of HPS aggregates, we adopted a diffusion-controlled kinetic model in the absence of activation free energy barrier for HPS molecules attaching to an existing aggregate, and this model has been adopted by previous studies57. In our model, the monomer attachment frequency fn is the product of two terms: the flux of incoming molecules to encounter the surface of the existing aggregate containing n molecules, and the surface area of the aggregate:
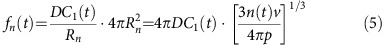 |
where D is the monomer diffusion constant, C1(t) is the monomer concentration at time t, Rn is the radius of the aggregate containing n molecules and n(t) is the size of the aggregate at time t. The molecular detachment frequency is independent of monomer concentration58, and is approximated to the attachment frequency at equilibrium 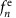 . Hence, the growth rate of an aggregate containing n molecules can be written as
. Hence, the growth rate of an aggregate containing n molecules can be written as
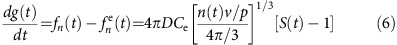 |
Fitting fluorescence data to the classical nucleation-growth model
We fitted the experimental measured fluorescence data to a theoretical model, which was built upon the classical nucleation-growth theory (equations (2)–(5), , , ). In our model, new nuclei are being formed at each aggregation time point, and simultaneously existing aggregates are growing. Once new nuclei are formed, they continue to grow as long as the solution is supersaturated. Accordingly, the total amount of aggregated HPS (V(t)) per unit volume at time t is the integration of the product of nuclei generated at a previous time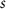 and its size growing during the remaining time τ. Meanwhile, the total amount of aggregated HPS (V(t)) can be independently calculated from supersaturation ratio (S(t), see Supplementary Note 3; Supplementary equation (8) for details). On the basis of these relations at a given initial concentration (C0), the solution of V(t) can be obtained numerically. As we showed that the total volume of aggregated HPS is proportional to the total fluorescence intensity, the measured dynamics of normalized fluorescence I(t)/IT should directly correlate to the HPS aggregation kinetics V(t)/VT. Therefore, we used the normalized total aggregates volume
and its size growing during the remaining time τ. Meanwhile, the total amount of aggregated HPS (V(t)) can be independently calculated from supersaturation ratio (S(t), see Supplementary Note 3; Supplementary equation (8) for details). On the basis of these relations at a given initial concentration (C0), the solution of V(t) can be obtained numerically. As we showed that the total volume of aggregated HPS is proportional to the total fluorescence intensity, the measured dynamics of normalized fluorescence I(t)/IT should directly correlate to the HPS aggregation kinetics V(t)/VT. Therefore, we used the normalized total aggregates volume 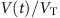 to directly fit the measured normalized fluorescence intensity. In our model, γsl, the surface tension of HPS aggregates in the solvent mixture, is the only fitting parameter. As our quantitative analysis heavily relies on the CNT, we have examined an alternative theory: a non-CNT59,60 involving stable prenucleation clusters (Supplementary Note 4). In non-CNT, the existence of stable prenucleation clusters will introduce a second free energy barrier, ɛ (Supplementary Note 4), in comparison with CNT containing only a single barrier. Applying non-CNT to our system, we show that ɛ has to be within 1.2kT (comparable with thermal fluctuations) in order to obtain reasonable fitting to experimental fluorescence (Supplementary Figs 14 and 15). Not surprisingly, with these small values of ɛ, the non-CNT theory provides consistent results with our CNT theory in both ΔΔG and cooperativity (Supplementary Fig. 16). These results suggest that the formation of stable prenulceation clusters is not substantial in the aggregation of HPS molecules, and thus CNT is well applicable to our system. In our CNT model, we treat the DMSO concentration as a constant, while the DMSO concentration profiles display noticeable drift along the microfluidic tube (see Supplementary Fig. 9d). Our further analysis shows that considering the DMSO concentration gradient does not have significant impact on the results (Supplementary Figs 22 and 23 and Supplementary Note 8).
to directly fit the measured normalized fluorescence intensity. In our model, γsl, the surface tension of HPS aggregates in the solvent mixture, is the only fitting parameter. As our quantitative analysis heavily relies on the CNT, we have examined an alternative theory: a non-CNT59,60 involving stable prenucleation clusters (Supplementary Note 4). In non-CNT, the existence of stable prenucleation clusters will introduce a second free energy barrier, ɛ (Supplementary Note 4), in comparison with CNT containing only a single barrier. Applying non-CNT to our system, we show that ɛ has to be within 1.2kT (comparable with thermal fluctuations) in order to obtain reasonable fitting to experimental fluorescence (Supplementary Figs 14 and 15). Not surprisingly, with these small values of ɛ, the non-CNT theory provides consistent results with our CNT theory in both ΔΔG and cooperativity (Supplementary Fig. 16). These results suggest that the formation of stable prenulceation clusters is not substantial in the aggregation of HPS molecules, and thus CNT is well applicable to our system. In our CNT model, we treat the DMSO concentration as a constant, while the DMSO concentration profiles display noticeable drift along the microfluidic tube (see Supplementary Fig. 9d). Our further analysis shows that considering the DMSO concentration gradient does not have significant impact on the results (Supplementary Figs 22 and 23 and Supplementary Note 8).
Free energy change of attaching a HPS monomer to an existing aggregate
The free energy change associated with attaching a HPS monomer to an existing spherical aggregate containing n molecules is
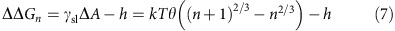 |
where the first term γslΔA corresponds to the free energy cost associated with creating additional solute–solvent interface when the size of aggregate increases from n to (n+1). θ=γsl(36π)1/3(v/p)2/3/kT. The second term, h, refers to the free energy of transferring a HPS molecule from the infinite-sized aggregate phase to the solution, where no additional interface between the aggregate and solution needs to be created. We followed ref. 61 to compute h:
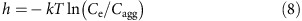 |
where Ce is the solubility of HPS monomer in the solvent mixture and Cagg=p/(vNa)=1.352 M is the HPS molar concentration in aggregates. Here, v is the molecular volume of a HPS monomer, p is the packing density and Na is the Avogadro's constant. As the solute–solvent surface tension γsl have already been obtained previously, we can then calculate the values of θ in various solvent mixtures (see Supplementary Table 2). As the solubility Ce was measured in our experiments (see Supplementary Fig. 10 for details), we can also obtain the values of h in various solvent mixtures (see Supplementary Table 2). Thus, free energy changes associated with attaching a HPS monomer to an existing aggregate in various DMSO/water solvent mixtures can be computed based on equation (7) and the results were shown in Fig. 3b.
We further determined the free energy associated with attaching a HPS monomer to aggregates in pure water (Fig. 1c). To achieve this, we obtained h and solute–solvent surface tension γsl in pure water as follows: first, by plotting the transferring free energy h against the surface tension of solvent γs at 20 °C (ref. 62; Supplementary Fig. 5a and Supplementary Table 1), we identified the relationship between h and γs to be linear (R2 of 0.98). We then extrapolated this fitted linear curve (from solvent mixture condition) to obtain h to be 13.6 kcal mol−1 in pure water, yielding a HPS solubility of 9.6 × 10−5 μM. Next, we plotted the solute–solvent surface tension of HPS aggregates γsl against the surface tension of the solvent (γs) (Supplementary Fig. 5b), and a linear relationship was obtained (R2 of 0.99). By extrapolating the fitted cure to the pure water condition, we obtained a solute–solvent surface tension of 31.8 cal mol−1 Å−2 for HPS aggregates in pure water. These two linear relationships (h versus γs, and γsl versus γs) were also shown in previous studies63,64.
Cooperativity in hydrophobic aggregation
We followed Wang et al.21 to define the cooperativity in hydrophobic interactions as the excess multibody free energy upon attaching a HPS monomer to an aggregate. For example, the cooperativity in a trimer formation is defined as the difference between the associated free energy to form a trimer and the summarized two-body potential of mean forces20,22. For systems containing more than three molecules, the free energy of an aggregate containing n molecules, Wn, can be decomposed into the single-body term 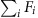 , the pairwise term
, the pairwise term 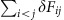 and the multibody term
and the multibody term 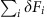 :
:
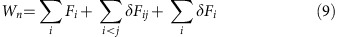 |
Then, the free energy of the aggregate containing (n+1) molecules, Wn+1, can be decomposed into the single-body term 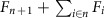 , the pairwise term
, the pairwise term 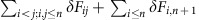 and the multibody term
and the multibody term 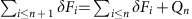 , where Qn is the excess multibody term, which is the quantity of the cooperativity in our definition. Therefore, we have
, where Qn is the excess multibody term, which is the quantity of the cooperativity in our definition. Therefore, we have
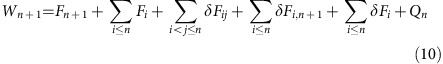 |
After simple rearrangement, we obtained,
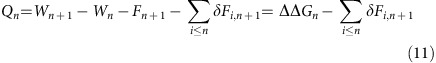 |
where 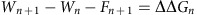 is the free energy change associated with attaching a HPS monomer to an aggregate containing n molecules, and
is the free energy change associated with attaching a HPS monomer to an aggregate containing n molecules, and 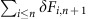 is the summarization of the two-body potential of mean force between the (n+1)th molecule and the ith molecule in the aggregate. The cooperativity Qn defined by equation (11) can be zero (additive), negative (cooperative) or positive (anti-cooperative).
is the summarization of the two-body potential of mean force between the (n+1)th molecule and the ith molecule in the aggregate. The cooperativity Qn defined by equation (11) can be zero (additive), negative (cooperative) or positive (anti-cooperative).
To compute the hydrophobic cooperativity as defined in equation (11), we need to obtain both 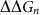 and
and 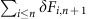 . The first term has been obtained from our experiment (Figs 1c and 3b). To compute the second term, we need to consider the potential of mean force to bring together the (n+1)th molecule and the ith molecule in the aggregate from infinite distance away to a particular distance determined by the location of ith molecule in the aggregate. If the (n+1)th molecule is in contact with the ith molecule in the newly formed aggregate containing (n+1) molecules, the magnitude of δFi,n+1 is significant due to the hydrophobic interactions associated with the desolvation between these two molecules. However, if the (n+1)th molecule is separated from the ith molecule by other HPS molecules, their centre of mass distance is at least two nanometres (∼2 diameters of HPS). In this case, no desolvation is needed and δFi,n+1 is thus negligible. Therefore, we only consider the contributions of these contact pairs to
. The first term has been obtained from our experiment (Figs 1c and 3b). To compute the second term, we need to consider the potential of mean force to bring together the (n+1)th molecule and the ith molecule in the aggregate from infinite distance away to a particular distance determined by the location of ith molecule in the aggregate. If the (n+1)th molecule is in contact with the ith molecule in the newly formed aggregate containing (n+1) molecules, the magnitude of δFi,n+1 is significant due to the hydrophobic interactions associated with the desolvation between these two molecules. However, if the (n+1)th molecule is separated from the ith molecule by other HPS molecules, their centre of mass distance is at least two nanometres (∼2 diameters of HPS). In this case, no desolvation is needed and δFi,n+1 is thus negligible. Therefore, we only consider the contributions of these contact pairs to 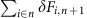 , and then need to estimate the number of contact pairs in the aggregate. Bezdek and Reid65 proved that the number of contact pairs in an arbitrary lattice packing of n unit balls in three-dimensional Euclidian space is always greater than (6n−7.862n2/3), but less than (6n−3.665n2/3). To make the best estimation of contact pairs in a spherical aggregate, we proved that the number of contact pairs in the aggregate containing n molecules is (see Supplementary Note 9 and Supplementary Fig. 24 for details):
, and then need to estimate the number of contact pairs in the aggregate. Bezdek and Reid65 proved that the number of contact pairs in an arbitrary lattice packing of n unit balls in three-dimensional Euclidian space is always greater than (6n−7.862n2/3), but less than (6n−3.665n2/3). To make the best estimation of contact pairs in a spherical aggregate, we proved that the number of contact pairs in the aggregate containing n molecules is (see Supplementary Note 9 and Supplementary Fig. 24 for details):
 |
The estimated contact pairs are shown in Supplementary Fig. 12. Next, we need to obtain the two-body potential of mean force (δF) of a contact pair. δF can be obtained from directly computing the free energy difference by bringing a pair of monomers from infinite distance to be in contact as suggested by Wang et al.21. In our study, it is challenging to directly measure δF in this way, because the smallest stable aggregate (that is the critical size plus one, according to the CNT) in experiment contains more than two molecules (see Supplementary Table 2 for details). Therefore, we estimated δF by approximating it to the pair strength in the smallest stable aggregate in a given solvent:
 |
In particular, the smallest stable aggregate (n*+1) contains 3–7 molecules in the solvent with a DMSO mole fraction of 0, 0.16, 0.21, 0.26 and 0.32, respectively. ΔΔG can be obtained from equation (7). Thus, the summarization of the two-body potential of mean force between the (n+1)th molecule and the ith molecule in the aggregate is obtained as follows:
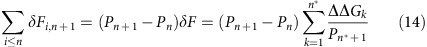 |
Substituting equations (7) and (14) into equation (11), we determined the hydrophobic cooperativity (Figs 1c and 3b). We note that the data reported in Figs 1c and 3b,c start from the smallest stable aggregate in the corresponding experimental condition. As our reported hydrophobic free energies at large n limit (ΔΔGn→∞) become equivalent to the free energy of transferring a HPS molecule from water to the HPS phase, this makes it possible to directly estimate the cooperativity even without performing microfluidics experiments, provided that one could also obtain the pair potential of mean force (for example, from MD simulations discussed in Supplementary Note 5 and Supplementary Figs 17 and 18).
Kinetics of the hydrophobic aggregation
To resolve the kinetics of HPS aggregation, we have tracked the growth of the first nucleus formed. Based on the theoretical model obtained previously, we derived the equation for nucleus growth as follows:
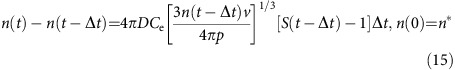 |
where n(t−Δt) is the size of the aggregate at time t−Δt. On the basis of equation (15), we obtained the nucleus growth curve as shown in Supplementary Fig. 7. Then, we defined the time for attaching a HPS monomer to the aggregate as the time required for the aggregate to grow by one molecule in size, and the results are shown in Figs 1d and 3c. The nucleation-growth curve (Supplementary Fig. 7) suggests the microsecond timescale of HPS aggregation, which is comparable to the timescale of early stage protein folding. This comparison is made between similar concentration of HPS in our experiment and local concentration of residues on a protein chain. We estimated local concentrations of protein residues by computing number of residues within a distance of the protein chain length (for example, a protein domain containing 89–100 residues has a chain length of 28–30 nm). The resulting local concentration of protein residues (1.3–1.8 mM) is comparable with our initial HPS concentration in the microfluidics tube: 2.0–6.0 mM.
Data availability
The data that support the findings of this study as well as the computer code for the CNT and non-CNT theories are available from the corresponding authors upon reasonable request.
Additional information
How to cite this article: Jiang, L. et al. Real-time monitoring of hydrophobic aggregation reveals a critical role of cooperativity in hydrophobic effect. Nat. Commun. 8, 15639 doi: 10.1038/ncomms15639 (2017).
Publisher's note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Supplementary Material
Supplementary Figures, Supplementary Tables, Supplementary Notes and Supplementary References
Acknowledgments
We thank Dr Lingle Wang for his critical reading of our manuscript. We acknowledge supports from National Basic Research Program of China (973 program, Grant No. 2013CB834703), Hong Kong Research Grants Council (Grant Nos. HKUST C6009-15G, AoE/P-705/16, ECS 60981, 621113, 16304215, F-HKUST605/15), and Innovation and Technology Commission (ITC-CNERC14SC01). We thank the Nanoelectronic Fabrication Facility, Biosciences Central Research Facility at HKUST and Dr Baoling Huang for providing technique support for microfluidics experiments.
Footnotes
The authors declare no competing financial interests.
Author contributions L.J. and S.Y. designed and performed the experiments. S.C., L.J. and X.H. established the classical nucleation and growth model for theoretical fitting and analysed fitting data. C.W.T.L. and B.Z.T. synthesized HPS and helped to perform solubility measurements. All authors discussed the results and contributed to the writing of the manuscript.
References
- Chandler D. Interfaces and the driving force of hydrophobic assembly. Nature 437, 640–647 (2005). [DOI] [PubMed] [Google Scholar]
- Whitesides G. M. & Grzybowski B. Self-assembly at all scales. Science 295, 2418–2421 (2002). [DOI] [PubMed] [Google Scholar]
- Lum K., Chandler D. & Weeks J. D. Hydrophobicity at small and large length scales. J. Phys. Chem. B 103, 4570–4577 (1999). [Google Scholar]
- Hummer G., Garde S., García A. E., Pohorille A. & Pratt L. R. An information theory model of hydrophobic interactions. Proc. Natl Acad. Sci. USA 93, 8951–8955 (1996). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pratt L. R. Molecular theory of hydrophobic effects: ‘She is too mean to have her name repeated.'. Annu. Rev. Phys. Chem. 53, 409–436 (2002). [DOI] [PubMed] [Google Scholar]
- Southall N. T., Dill K. A. & Haymet A. A view of the hydrophobic effect. J. Phys. Chem. B 106, 521–533 (2002). [Google Scholar]
- Berne B. J., Weeks J. D. & Zhou R. Dewetting and hydrophobic interaction in physical and biological systems. Annu. Rev. Phys. Chem. 60, 85 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Stillinger F. H. Structure in aqueous solutions of nonpolar solutes from the standpoint of scaled-particle theory. J. Solution Chem. 2, 141–158 (1973). [Google Scholar]
- Wallqvist A. & Berne B. Molecular dynamics study of the dependence of water solvation free energy on solute curvature and surface area. J. Phys. Chem. 99, 2885–2892 (1995). [Google Scholar]
- Wallqvist A. & Berne B. Computer simulation of hydrophobic hydration forces on stacked plates at short range. J. Phys. Chem. 99, 2893–2899 (1995). [Google Scholar]
- Huang X., Margulis C. & Berne B. Dewetting-induced collapse of hydrophobic particles. Proc. Natl Acad. Sci. USA 100, 11953–11958 (2003). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Acharya H., Vembanur S., Jamadagni S. N. & Garde S. Mapping hydrophobicity at the nanoscale: applications to heterogeneous surfaces and proteins. Faraday Discuss. 146, 353–365 (2010). [DOI] [PubMed] [Google Scholar]
- Patel A. J. et al. Extended surfaces modulate hydrophobic interactions of neighboring solutes. Proc. Natl Acad. Sci. USA 108, 17678–17683 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
- ten Wolde P. R. & Chandler D. Drying-induced hydrophobic polymer collapse. Proc. Natl Acad. Sci. USA 99, 6539–6543 (2002). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Liu P., Huang X., Zhou R. & Berne B. J. Observation of a dewetting transition in the collapse of the melittin tetramer. Nature 437, 159–162 (2005). [DOI] [PubMed] [Google Scholar]
- Chandler D. Hydrophobicity: two faces of water. Nature 417, 491–491 (2002). [DOI] [PubMed] [Google Scholar]
- Ball P. Chemical physics: how to keep dry in water. Nature 423, 25–26 (2003). [DOI] [PubMed] [Google Scholar]
- Davis J. G., Gierszal K. P., Wang P. & Ben-Amotz D. Water structural transformation at molecular hydrophobic interfaces. Nature 491, 582–585 (2012). [DOI] [PubMed] [Google Scholar]
- Ball P. Biophysics: more than a bystander. Nature 478, 467–468 (2011). [DOI] [PubMed] [Google Scholar]
- Czaplewski C. et al. Molecular simulation study of cooperativity in hydrophobic association. Protein Sci. 9, 1235–1245 (2000). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wang L., Friesner R. A. & Berne B. J. Hydrophobic interactions in model enclosures from small to large length scales: non-additivity in explicit and implicit solvent models. Faraday Discuss. 146, 247–262 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Shimizu S. & Chan H. S. Anti-cooperativity and cooperativity in hydrophobic interactions: Three-body free energy landscapes and comparison with implicit-solvent potential functions for proteins. Proteins 48, 15–30 (2002). [DOI] [PubMed] [Google Scholar]
- Rajamani S., Truskett T. M. & Garde S. Hydrophobic hydration from small to large lengthscales: understanding and manipulating the crossover. Proc. Natl Acad. Sci. USA 102, 9475–9480 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Giovambattista N., Lopez C. F., Rossky P. J. & Debenedetti P. G. Hydrophobicity of protein surfaces: separating geometry from chemistry. Proc. Natl Acad. Sci. USA 105, 2274–2279 (2008). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris R. C. & Pettitt B. M. Effects of geometry and chemistry on hydrophobic solvation. Proc. Natl Acad. Sci. USA 111, 14681–14686 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ma C. D., Wang C., Acevedo-Vélez C., Gellman S. H. & Abbott N. L. Modulation of hydrophobic interactions by proximally immobilized ions. Nature 517, 347–350 (2015). [DOI] [PubMed] [Google Scholar]
- Cheng Y.-K. & Rossky P. J. Surface topography dependence of biomolecular hydrophobic hydration. Nature 392, 696–699 (1998). [DOI] [PubMed] [Google Scholar]
- Lipman E. A., Schuler B., Bakajin O. & Eaton W. A. Single-molecule measurement of protein folding kinetics. Science 301, 1233–1235 (2003). [DOI] [PubMed] [Google Scholar]
- Voelz V. A. et al. Slow unfolded-state structuring in acyl-CoA binding protein folding revealed by simulation and experiment. J. Am. Chem. Soc. 134, 12565–12577 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Wunderlich B. et al. Microfluidic mixer designed for performing single-molecule kinetics with confocal detection on timescales from milliseconds to minutes. Nat. Protoc. 8, 1459–1474 (2013). [DOI] [PubMed] [Google Scholar]
- Jiang L. G. et al. Microsecond protein folding events revealed by time-resolved fluorescence resonance energy transfer in a microfluidic mixer. Anal. Chem. 87, 5589–5595 (2015). [DOI] [PubMed] [Google Scholar]
- Russell R. et al. Rapid compaction during RNA folding. Proc. Natl Acad. Sci. USA 99, 4266–4271 (2002). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Seong G. H., Heo J. & Crooks R. M. Measurement of enzyme kinetics using a continuous-flow microfluidic system. Anal. Chem. 75, 3161–3167 (2003). [DOI] [PubMed] [Google Scholar]
- Huebner A. et al. Development of quantitative cell-based enzyme assays in microdroplets. Anal. Chem. 80, 3890–3896 (2008). [DOI] [PubMed] [Google Scholar]
- Jahn A., Vreeland W. N., Gaitan M. & Locascio L. E. Controlled vesicle self-assembly in microfluidic channels with hydrodynamic focusing. J. Am. Chem. Soc. 126, 2674–2675 (2004). [DOI] [PubMed] [Google Scholar]
- Jahn A. et al. Microfluidic mixing and the formation of nanoscale lipid vesicles. ACS Nano 4, 2077–2087 (2010). [DOI] [PubMed] [Google Scholar]
- Chen J. et al. Synthesis, light emission, nanoaggregation, and restricted intramolecular rotation of 1, 1-substituted 2, 3, 4, 5-tetraphenylsiloles. Chem. Mater. 15, 1535–1546 (2003). [Google Scholar]
- Hong Y., Lam J. W. & Tang B. Z. Aggregation-induced emission: phenomenon, mechanism and applications. Chem. Commun. (Camb.) 4332–4353 (2009). [DOI] [PubMed] [Google Scholar]
- Zheng X. et al. Unraveling the aggregation effect on amorphous phase AIE luminogens: a computational study. Nanoscale 8, 15173–15180 (2016). [DOI] [PubMed] [Google Scholar]
- Kashchiev D. & Van Rosmalen G. Review: nucleation in solutions revisited. Cryst. Res. Technol. 38, 555–574 (2003). [Google Scholar]
- Jeffrey G. A. & Jeffrey G. A. An Introduction to Hydrogen Bonding Vol. 12, (Oxford University Press, 1997). [Google Scholar]
- Reynolds J. A., Gilbert D. B. & Tanford C. Empirical correlation between hydrophobic free energy and aqueous cavity surface area. Proc. Natl Acad. Sci. USA 71, 2925–2927 (1974). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Raschke T. M., Tsai J. & Levitt M. Quantification of the hydrophobic interaction by simulations of the aggregation of small hydrophobic solutes in water. Proc. Natl Acad. Sci. USA 98, 5965–5969 (2001). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Lapidus L. J. et al. Protein hydrophobic collapse and early folding steps observed in a microfluidic mixer. Biophys. J. 93, 218–224 (2007). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Duan Y. & Kollman P. A. Pathways to a protein folding intermediate observed in a 1-microsecond simulation in aqueous solution. Science 282, 740–744 (1998). [DOI] [PubMed] [Google Scholar]
- Zhou R., Huang X., Margulis C. J. & Berne B. J. Hydrophobic collapse in multidomain protein folding. Science 305, 1605–1609 (2004). [DOI] [PubMed] [Google Scholar]
- Dill K. A. Dominant forces in protein folding. Biochemistry 29, 7133–7155 (1990). [DOI] [PubMed] [Google Scholar]
- Ghosh K. & Dill K. A. Theory for Protein Folding Cooperativity: Helix Bundles. J. Am. Chem. Soc. 131, 2306–2312 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Harris R. C. & Pettitt B. M. Reconciling the understanding of ‘hydrophobicity' with physics-based models of proteins. J. Phys. Condens. Matter 28, 083003 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Mei J., Leung N. L. C., Kwok R. T. K., Lam J. W. Y. & Tang B. Z. Aggregation-induced emission: together we shine, united we soar!. Chem. Rev. 115, 11718–11940 (2015). [DOI] [PubMed] [Google Scholar]
- He G. et al. A novel picric acid film sensor via combination of the surface enrichment effect of chitosan films and the aggregation-induced emission effect of siloles. J. Mater. Chem. 19, 7347–7353 (2009). [Google Scholar]
- Catalan J., Diaz C. & Garcia-Blanco F. Characterization of binary solvent mixtures of DMSO with water and other cosolvents. J. Org. Chem. 66, 5846–5852 (2001). [DOI] [PubMed] [Google Scholar]
- Lee C., Yang W. & Parr R. G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 37, 785 (1988). [DOI] [PubMed] [Google Scholar]
- Becke A. D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 98, 5648–5652 (1993). [Google Scholar]
- Stephens P., Devlin F., Chabalowski C. & Frisch M. J. Ab initio calculation of vibrational absorption and circular dichroism spectra using density functional force fields. J. Chem. Phys. 98, 11623–11627 (1994). [Google Scholar]
- Hariharan P. C. & Pople J. A. The influence of polarization functions on molecular orbital hydrogenation energies. Theor. Chim. Acta 28, 213–222 (1973). [Google Scholar]
- Straub J. E. & Thirumalai D. Toward a molecular theory of early and late events in monomer to amyloid fibril formation. Annu. Rev. Phys. Chem 62, 437–463 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
- Oxtoby D. W. Homogeneous nucleation: theory and experiment. J. Phys. Condens. Matter 4, 7627 (1992). [Google Scholar]
- Prestipino S., Laio A. & Tosatti E. Systematic improvement of classical nucleation theory. Phys. Rev. Lett. 108, 225701 (2012). [DOI] [PubMed] [Google Scholar]
- Gebauer D. & Colfen H. Prenucleation clusters and non-classical nucleation. Nano Today 6, 564–584 (2011). [Google Scholar]
- Thompson J. D., Cramer C. J. & Truhlar D. G. Predicting aqueous solubilities from aqueous free energies of solvation and experimental or calculated vapor pressures of pure substances. J. Chem. Phys. 119, 1661–1670 (2003). [Google Scholar]
- Markarian S. A. & Terzyan A. M. Surface tension and refractive index of dialkylsulfoxide+ water mixtures at several temperatures. J. Chem. Eng. Data 52, 1704–1709 (2007). [Google Scholar]
- Yasuda S., Suzuki I., Shinohara K.-I. & Shigekawa H. Single molecular anatomy of solvophobic effects in host-guest interactions based on surface tension using atomic force microscopy. Phys. Rev. Lett. 96, 228303 (2006). [DOI] [PubMed] [Google Scholar]
- Li I. T. & Walker G. C. Interfacial free energy governs single polystyrene chain collapse in water and aqueous solutions. J. Am. Chem. Soc. 132, 6530–6540 (2010). [DOI] [PubMed] [Google Scholar]
- Bezdek K. & Reid S. Contact graphs of unit sphere packings revisited. J. Geom. 104, 57–83 (2013). [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Supplementary Figures, Supplementary Tables, Supplementary Notes and Supplementary References
Data Availability Statement
The data that support the findings of this study as well as the computer code for the CNT and non-CNT theories are available from the corresponding authors upon reasonable request.