Skip to main content
PMC home page
Bosnian Journal of Basic Medical Sciences logoLink to Bosnian Journal of Basic Medical Sciences
. 2017 May;17(2):164–171. doi: 10.17305/bjbms.2017.1765

Knee stiffness and viscosity: New implementation and perspectives in prosthesis development

Klemen Bohinc 1,*, Nejc Vantur 1, Drago Torkar 2, Tomaž Lampe 1, Marija Hribernik 3, Miroljub Jakovljević 4
PMCID: PMC5474111  PMID: 28422623

Abstract

The pendulum test is a method applied to measure passive resistance of the knee. A new and simple pendulum test with instrumentation based on infrared camera was used to evaluate knee stiffness and viscosity on a female human cadaver. The stiffness and viscosity were calculated based on the kinetic data. During the measurements, the periarticular and intraarticular soft tissue of the knee was gradually removed to determine the stiffness and viscosity as a function of the tissue removal rate. The measurements showed that the removal of tissue around the joint reduces the damping of leg oscillation, and therefore decreases the stiffness and viscosity. The contribution to knee joint damping was 10% for the skin, 20% for ligaments, and 40% for muscles and tendons. Tissue removal has a very large impact on the knee stiffness and viscosity.

KEY WORDS: Infrared camera, knee viscosity and stiffness, prosthesis, pendulum test

INTRODUCTION

The knee joint consists of articulations between the femur and tibia (tibiofemoral joint) including medial and lateral meniscus, and the femur and patella (patellofemoral joint). The tibiofemoral joint allows flexion, extension, and some medial and lateral rotation. The patellofemoral joint allows the patella to glide over the distal femur. Both articulations are within a common joint capsule. The stability of the knee joint depends on the surrounding muscles and their tendons (musculus [m.] quadriceps femoris, m. semimembranosus, m. semitendinosus, m. biceps femoris, and m. gastrocnemius) and on the extra and intracapsular ligaments. The extracapsular ligaments support the capsule on all four sides and include the patellar ligament with medial and lateral retinaculum on the anterior side, oblique and arcuate popliteal ligaments on the posterior side, and medial and lateral collateral ligaments. The patellar ligament and retinacula stabilize the patella, oblique and arcuate popliteal ligaments reinforce the joint capsule, and medial and lateral collateral ligaments stabilize the knee in extension and resist valgus and varus forces, respectively. The strong intracapsular anterior and posterior cruciate ligaments prevent forward and backward displacement of the tibia.

The joint resistance to movement is due to the cartilage properties and shapes of the contacting articular surfaces [1]. Ligaments, joint capsules, as well as muscles contain fibrous connective tissue that generates resistive forces when stretched. Displacement of a joint is immediately resisted by the forces produced within and over the joint. Physiologic changes that occur during a lifespan depend primarily on biophysical characteristics of fibrous connective tissue [2,3]. These factors include age [3-5], sex [4-7], muscle mass [6], muscle strength [8], hereditary connective tissue disease [4], circadian rhythm [9,10], temperature of joint [4,9], joint torque [11-13], joint position sense [5,13], contraction and co-contraction of muscle groups [14-17], and local [4,18] and systemic pathological changes [4,19-21].

The loss of elasticity in soft-tissue matrices increases muscle and joint stiffness with increased amounts of fibrous connective tissue [22]. All the structures located within and over the joint (muscles, tendons, skin, subcutaneous tissue, fascia, ligaments, joint capsule, and cartilage) contribute to joint stiffness [23-25]. Biomechanically, joint stiffness is regarded as the passive tissue property. It describes how external forces applied to the skeletal system are absorbed or transmitted by the articular tissues [26,27]. From a technical point of view, the stiffness is determined in terms of friction, elasticity, viscosity, inertia, and plasticity [4,23-25].

The pendulum test is a method applied to measure passive properties of the knee. In the pendulum test, the leg is dropped from an extended position under the influence of gravitation and then allowed to damp oscillate [28,29]. The pendulum test can be performed with no special equipment. The oscillatory movements of the lower leg can be recorded with electrogoniometers [20], uniplanar video-based methods, three-dimensional (3D) motion analysis systems, ultrasonic 3D position recording device [30], and isokinetic dynamometer [31]. However, all of these methods have some disadvantages. For example, video recording equipment is expensive and time-consuming. The isokinetic dynamometer can limit excessive knee flexion. The electrogoniometer attached directly to the knee joint can produce varying results because of spatial twist [32].

In this study, we developed a new instrumentation for the pendulum test, which overcomes the disadvantages of the other methods. Our goal was to determine the contributions of peri and intraarticular soft tissues of the knee to the passive knee stiffness and viscosity. Namely, the stiffness and viscosity represent passive resistance to joint motion, which is associated with the properties of the knee joint tissue, muscles, and tendons. According to our results, the pendulum test (Figure 1) can be a practical tool in measuring changes within the knee joint.

FIGURE 1.

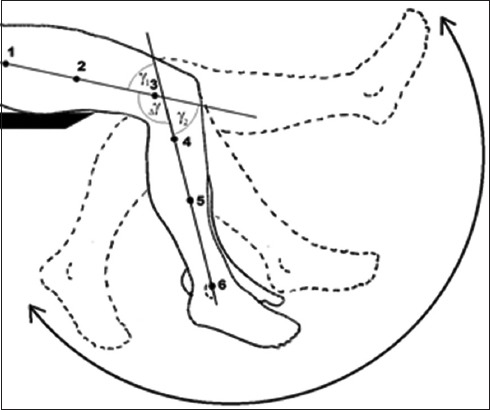
FIGURE 1

Open in a new tab

Schematic presentation of a pendulum test. The markers are attached to a thigh and to a lower leg in a way they form two virtual lines (123 and 456). During the oscillation of the lower leg, the angle between these lines is traced versus time.

MATERIALS AND METHODS

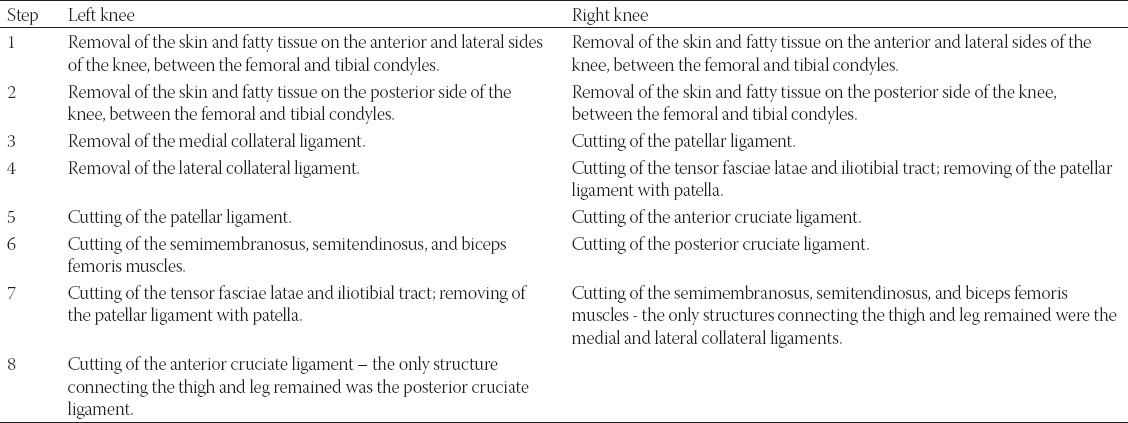
The pendulum test was repeatedly performed on female cadaver knees (age of 73 years). The cadaver was frozen at −18°C. The thawing took place one day before the experiment. Pre-freezing and thawing should not influence the mechanical characteristics of soft tissue, which was confirmed in animals [33] and later on human ligaments and muscular tendons [34]. The infra-red (IR) markers were attached to the leg by adhesive tape (Figure 2). The peri and intraarticular soft tissue was gradually removed between test repetitions. The step-by-step removal of the tissue is described in Table 1.

FIGURE 2.

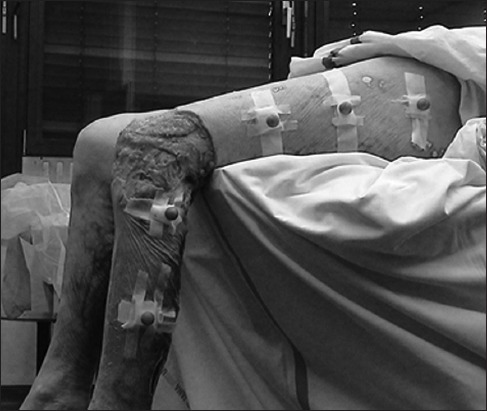
FIGURE 2

Open in a new tab

Cadaveric left leg with attached infrared markers. Parts of the knee tissue had already been removed.

TABLE 1.

Gradual removal of knee tissue

graphic file with name BJBMS-17-164-g003.jpg

Open in a new tab

Data collection

The data were collected by a machine vision system able to grab, analyze, and save images from a fast 1 MB complementary metal-oxide-semiconductor camera (75 fps, global shutter) in real time. The cadaver leg was equipped with 6 passive IR markers, spherically shaped, reflecting the incoming IR light back to its source. The IR light came from bright IR light emitting diodes with maximum emission at 850 nm, positioned on a ring around the camera lenses equipped with IR filter with 780 nm cut-on frequency. The IR markers were divided into two groups of three: Above (thigh) and below the knee (lower leg), each forming a virtual line. During the pendulum test, an angle between the two virtual lines was traced versus the time, reflecting the lower limb oscillation (Figure 2).

Pendulum test

The pendulum test consisted of the following steps: (1) grab sequence, (2) an image processing sequence, (3) saving results to disk, and (4) result analysis.

Just before the test, the leg was held in a near-horizontal position for a few moments to assure the starting angle was near 180°. In this phase, the visibility and position of the IR markers were checked on a computer monitor. Then, the camera image grabbing was started and the leg was released. During the period of the oscillation of the leg (approximately up to 15 seconds) full frame images obtained with the camera were stored in the computer memory, with frequency of 15 frames per second (fps). The frame rate was adjustable but limited with memory (higher frame rate requires more memory). The frame rate of 15 fps resulted in over 200 images as well as angle values, per one test. This amount of data was sufficient for the purpose of the study. Next, in the image processing phase, each image was analyzed in the following way. First, a binary threshold was applied to the filtered IR image resulting in a pure binary image. This simple and quick procedure was sufficient due to the use of IR filter blocking of the visible light reflections. Then, the blob detection was applied using the OpenCV library (opencv.org) followed by filtering out of very small and very big blobs with areas significantly deviating from the average blob area. This step was required due to occasional IR reflections from the background metallic parts. For each blob, its center of mass was calculated and then used to represent the whole blob. Because we need at least two points to define a line, the number of the remaining blobs must have been at least 4 out of 6. If this was not the case, the procedure was stopped and the next image was considered. There are several reasons why not all markers are present in all images, among which a temporal occlusion is the most frequent one. When the number of detected markers was <6, several possible scenarios were available (Table 2).

TABLE 2.

Number of detected blobs and their possible association with each line segment. Each line segment is defined by maximum 3 blobs

graphic file with name BJBMS-17-164-g004.jpg

Open in a new tab

In the case of N = 6 the combination 3-3 was assumed, relying on a successful removal of the artefacts in the previous steps. If N = 5, the following algorithm was implemented to separate the blob centers belonging to each line. First, the blob centers were sorted by their v (vertical) coordinate and then regarding the number of detected blobs each combination from Table 2 was tested using least-square line fitting algorithm through blob centers and comparing the correlation coefficients r,

graphic file with name BJBMS-17-164-g005.jpg

where,

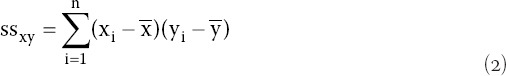
graphic file with name BJBMS-17-164-g006.jpg

graphic file with name BJBMS-17-164-g007.jpg

graphic file with name BJBMS-17-164-g008.jpg

and and are mean x̄ and ȳ values, respectively.

The combination having a larger sum of correlation coefficients was considered as the correct one. This procedure may give wrong results when the blobs are collinear (stretched knee), but this does not affect the calculated angle (Figure 3) between the lines, which in this case is near 180°. For N = 4, we first checked if the last two possibilities (3-1 and 1-3) exist (Table 2). They are irregular since the line cannot be defined by a single point. The line was fitted first through the first three blobs and then through the last three blobs. If both r were very close to 1, the blobs were collinear with α = 180°. If one r was close to 1 and the other was not, the procedure advanced to the next image. Only if both r were not close to 1 then a combination 2-2 was assumed and the lines were fitted accordingly. For each image, the traced knee angle was saved to a file together with the precise time when the image was grabbed from the camera. Then, the damped oscillation equation was applied to these data (Equation 5),

FIGURE 3.

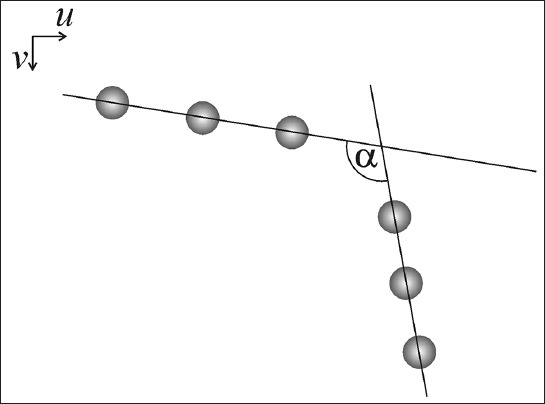
FIGURE 3

Open in a new tab

In a digital image, the infrared (IR) markers attached to a thigh and lower leg are represented by the two virtual lines intersecting at the angle a.

graphic file with name BJBMS-17-164-g010.jpg

where, A0 and A are the constants, β is the damping factor, ω is angular frequency, and δ is a phase angle.

From the measured kinematics, we obtained the knee angle as a function of time from which the damping ratio ζ and the angular frequency ω can be determined. The viscosity B and the stiffness K are obtained from [30,35],

graphic file with name BJBMS-17-164-g011.jpg

graphic file with name BJBMS-17-164-g012.jpg

where, J is the moment of inertia, l is the distance between the leg-foot center of gravity and the knee axis, m is the mass of the leg and K’=J ω2. The mass of the leg was measured and the moment of inertia was estimated.

RESULTS

Figure 4 shows the time dependence of the knee angle for two different cases. In the beginning (t = 0), the amplitude was approximately 170°, which corresponded to near full extension. The frequency of the oscillation was approximately 1 s−1. Removing tissue parts of the knee slightly decreased the frequency. The amplitude of pendulum oscillations decreased exponentially with time, meaning that the oscillation was damped. A heavily damped oscillating motion was observed for the intact knee, whereas the knee with removed muscles showed quasi-ideal oscillation with very weak damping.

FIGURE 4.

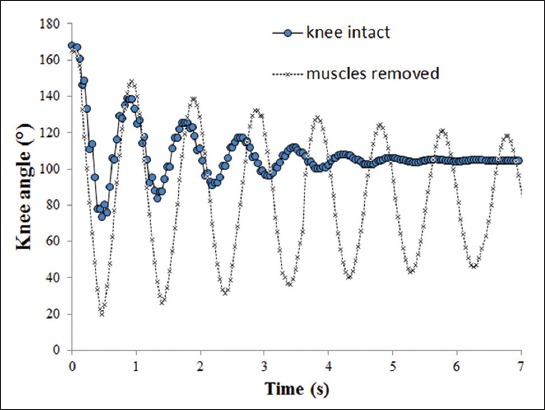
FIGURE 4

Open in a new tab

The knee angle as a function of time in two different cases. The full line corresponds to the intact knee, whereas the dashed line corresponds to the knee with all muscles removed.

The kinematic data of the leg oscillations were used to determine the knee stiffness and viscosity (Equations 6 and 7). With the following equation, relative changes in the viscosity were determined:

graphic file with name BJBMS-17-164-g014.jpg

where, Bi is the viscosity in the i-th step. The changes in the stiffness were defined similarly.

Figure 5 shows the relative changes in the stiffness and viscosity as a function of gradual tissue removal. For each removal step, we made two to three measurements from which we determined the stiffness and viscosity. For the intact left knee, the stiffness was 1.4 kg m2/s2 and viscosity was 0.023 kg m2/s, whereas for the intact right knee the stiffness was 0.85 kg m2/s2 and viscosity was 0.023 kg m2/s.

FIGURE 5.

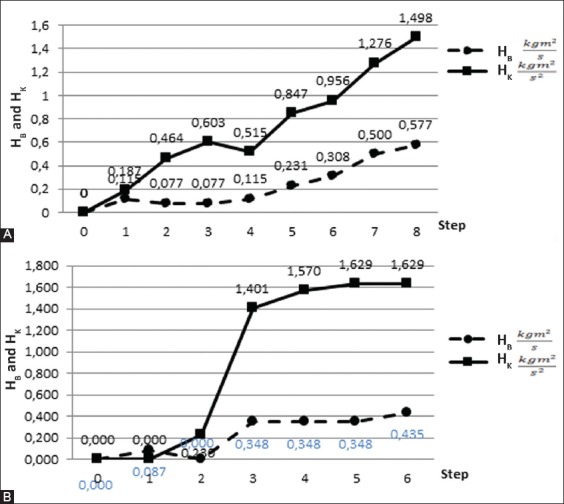
FIGURE 5

Open in a new tab

(A and B) Relative changes in knee stiffness and viscosity as a function of different tissue removal steps. Right leg (top) and left leg (bottom). In each removal step, different part of the knee tissue was removed.

The step-by-step removal of the tissue was different for the left and right knee (Table 1). This is the main reason why changes in the rigidity and viscosity as a function of the tissue removal were different between the two legs. As shown in Figure 5, the largest drop in the rigidity and viscosity for the right leg occurred in the fourth removal step, where the viscosity dropped by 0.008 kg m2/s and stiffness by 1.357 kg m2/s2. In the fourth, fifth, and sixth removal steps, the viscosity remained 0.015 kg m2/s for the right knee. The largest drop in the rigidity was in the fifth step, by 0.568 kg m2/s2. Correspondingly, the viscosity dropped by 0.008 kg m2/s. The largest discrepancy in the viscosity and stiffness between the left and right knee was observed between the third and fourth step. Namely, in this transition step, in the right knee, the patella and patellar ligament were entirely removed, whereas in the left knee, the patellar ligament was cut, but not removed.

Overall, different relative changes in the viscosity and stiffness were observed at different steps of gradual tissue removal, meaning that each tissue layer contributed differently to these changes (Figure 5). For example, the skin made a contribution of 10% to the joint damping. The ligaments contributed 20%, whereas the contribution of tendons was 40%.

DISCUSSION

In this case study, we performed a pendulum test and determined how gradual removal of the soft tissue structures affects the knee stiffness and viscosity. Our results showed that the tissue removal has a large impact on the leg oscillation. As shown in Figure 4, the oscillation of the leg with the intact knee was heavily damped whereas the oscillation of the knee with all muscles removed was very close to an ideal pendulum (i.e., not damped). The knee stiffness and viscosity decreased with the increasing rate of tissue removal (Figure 5). However, it should be emphasized that our results were obtained for only one human cadaver and they rather show a hypothetical performance of the pendulum test.

Our measurement results for the viscosity and stiffness of the intact knee are in a qualitative agreement with previous measurements made by Lin and Rymer [36]. The removal of tissue around the joint reduced the damping of leg oscillation and therefore the stiffness and viscosity decreased. The removal of tissue changed the spring and contractile elements of the muscle (Figure 6). Namely, the springs with stiffness Ki represent the elastic part, whereas damping (contractile) elements with the friction coefficient Bi represent the viscous part. The step-by-step tissue removal is described in Table 1. The contribution of a particular knee element to the viscosity and stiffness can be seen from the results given in Figure 5. For example, the removal of the patellar ligament and patella corresponded to a change in the viscosity by 0.008 kg m2/s2 and to a change in the stiffness by 1.4 kg m2/s. Based on these findings, we can also estimate the contribution of the patellar ligament and patella to the viscosity and stiffness.

FIGURE 6.

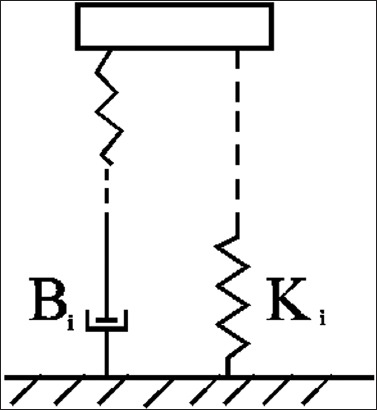
FIGURE 6

Open in a new tab

Schematic representation of a simple muscle model with spring and damping (contractile) elements. Ki is the spring constant of the i-th element, whereas Bi is the friction coefficient of the i-th element.

Our study results could be useful in orthotics and prosthetics, because currently, the primary measurement system is based on a gait analysis of the whole-body movements, and not on the analysis of individual body parts. For instance, in the field of technical aids, the pendulum test could be used for measuring the passive motion of the knee joint to determine the rigidity when various aids are placed around the joint. In general, orthoses are used to support or correct deformed body parts as well as to prevent injuries and improve the function of movable body parts [37]. In orthotics, there are numerous aids, i.e., orthoses that can be placed around the knee joint following the accepted therapeutic guidelines. They can be made of various materials, including steel, aluminum, titanium, carbon fibers, plastic, neoprene, canvas, and various composite materials. Some also include joints with different mechanical characteristics. Single-axis joints enable a simple way of permitting motion at the knee during gait. However, the anatomical knee joint possesses a moving axis of rotation which we cannot track with a single-axis joint (in orthotics). Thus, for active patients, polycentric knee joints provide a better approximation of natural knee motion and a better fit at all points in the knee flexion range [38]. In general, it would be beneficial to establish the impact of orthotic aids on the swing in relation to different parameters (i.e., materials, joints, the level of aid and weight) and to define restrictions and improvements in the movement capability, using healthy patients as a reference. Ultimately, these information would help in determining the right orthotic treatment.

Below-knee prosthetics covers different amputation levels of the tibia, achieved with techniques that are based on different muscle cuts [39]. Each type of amputation has an individual impact on the movement in the knee joint and depends on the length of the muscle that was cut or on a group of muscles.

Prosthesis is an aid that functionally and esthetically replaces the amputated limb or a part of it. The part of the prosthesis directly connected to the patient’s body is called a socket. It ensures optimal grip and enables the transfer of force to the other parts of the prosthesis, with the aim of replacing the function of the amputated limb. The sockets are made by various methods and from various materials. The components vary and depend on the individual needs of a patient [40]. In the case of transtibial amputation, it would be beneficial to apply the pendulum test at various levels of amputation without the prosthesis, to define the impact of different kinds of amputation on the function of the knee joint. The same measurement would also be helpful in different types of below-knee prosthetics, to determine the impact of various types of sockets, components, and materials on lower leg swing.

In the first half of the 20th century, metal refining enabled the production of different materials which were introduced in bone and joint surgery for internal use, especially in fracture fixation [41]. Further developments in metal processing and refining gradually led to the production of improved alloy material. According to the composition, these materials can be grouped into three alloy systems: The austenitic stainless steels (iron, chromium, and molybdenum), cobalt-chromium alloys, and titanium alloys [42]. More recently, different types of ceramics have been introduced, i.e., calcium phosphates, which were synthesized exclusively for the use in orthopedic surgery, and poly(methyl methacrylate), also known as acrylic cement [43]. At present, a large number of composite materials are utilized for internal application, and the use of these materials must comply with the relevant requirements and standards [44]. Over the last decade, a significant breakthrough was achieved in the field of tissue engineering, which offers a wide range of opportunities for the use of tissue materials [45]. Regenerative medicine enables regeneration, maintenance, and improvement of the function of damaged tissue. In tissue engineering, three different approaches have been adopted: (A) injection of functional and stem cells into damaged tissue, (B) the use of various synthetic or natural materials which help in the reconstruction of damaged tissue, and (C) the use of scaffolds with implanted tissue-specific cells. These artificial structures are capable of supporting 3D tissue formation and promoting survival and growth [46].

Advancements in these areas enable the fixation of joints as well as production of various artificial joint replacements, surrounding tissues, and bone parts. The use of composite and biological materials opens up new possibilities in this field and the impact of various materials on kinematics of joints should be investigated. In addition, the focus should be on the correlation between natural and biological materials implanted in joints or the surrounding tissues. The correlation could be tested by comparing the performance of a healthy joint and a joint with implanted artificial materials. Suggestions for further research also include the impact of various surgical procedures or treatment methods on pendulum test results.

CONCLUSION

In this study, we used a new pendulum test to measure knee motion on one human cadaver. It is important to emphasize here that this case study can only be used to illustrate the idea. New instrumentation for the pendulum test was developed, and passive stiffness and viscosity were determined using human cadaver limbs. Our results showed that removal of the knee tissue reduced the damping of leg oscillation. The removal of the tissue had a large impact on the knee viscosity and stiffness.

DECLARATION OF INTERESTS

The authors declare no conflict of interests.

REFERENCES

  • 1.Fung YC. Biomechanics: Mechanical properties of living tissues. 2nd ed. New York: Springer-Verlag; 1993. pp. 254–62. 525-35. https://doi.org/10.1007/978-1-4757-2257-4. [Google Scholar]
  • 2.Anthony CP, Kolthoff NJ. Textbook of anatomy and physiology. 9th ed. St. Louis: Mosby; 1975. [Google Scholar]
  • 3.Buckwalter JA, Woo SL, Goldberg VM, Hadley EC, Booth F, Oegema TR, et al. Soft-tissue aging and musculoskeletal function. J Bone Joint Surg Am. 1993;75(10):1533–48. doi: 10.2106/00004623-199310000-00015. https://doi.org/10.2106/00004623-199310000-00015. [DOI] [PubMed] [Google Scholar]
  • 4.Wright V, Johns RJ. Quantitative and qualitative analysis of joint stiffness in normal subjects and in patients with connective tissue diseases. Ann Rheum Dis. 1961;20(1):36–46. doi: 10.1136/ard.20.1.36. https://doi.org/10.1136/ard.20.1.36. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 5.Oatis CA. The use of a mechanical model to describe the stiffness and damping characteristics of the knee joint in healthy adults. Phys Ther. 1993;73(11):740–9. doi: 10.1093/ptj/73.11.740. [DOI] [PubMed] [Google Scholar]
  • 6.Blackburn JT, Riemann BL, Padua DA, Guskiewicz KM. Sex comparison of extensibility, passive, and active stiffness of the knee flexors. Clin Biomech (Bristol, Avon) 2004;19(1):36–43. doi: 10.1016/j.clinbiomech.2003.09.003. https://doi.org/10.1016/j.clinbiomech.2003.09.003. [DOI] [PubMed] [Google Scholar]
  • 7.Lin CC, Ju MS, Huang HW. Gender and age effects on elbow joint stiffness in healthy subjects. Arch Phys Med Rehabil. 2005;86(1):82–5. doi: 10.1016/j.apmr.2003.12.027. https://doi.org/10.1016/j.apmr.2003.12.027. [DOI] [PubMed] [Google Scholar]
  • 8.Ocarino JM, Fonseca ST, Silva PL, Mancini MC, Gonçalves GG. Alterations of stiffness and resting position of the elbow joint following flexors resistance training. Man Ther. 2008;13(5):411–8. doi: 10.1016/j.math.2007.03.009. https://doi.org/10.1016/j.math.2007.03.009. [DOI] [PubMed] [Google Scholar]
  • 9.Dick F. Sports training principles. London: A&C Black; 1997. [Google Scholar]
  • 10.Cutolo M, Masi AT. Circadian rhythms and arthritis. Rheum Dis Clin North Am. 2005;31(1):115–29. doi: 10.1016/j.rdc.2004.09.005. ix-x. https://doi.org/10.1016/j.rdc.2004.09.005. [DOI] [PubMed] [Google Scholar]
  • 11.Cannon SC, Zahalak GI. The mechanical behavior of active human skeletal muscle in small oscillations. J Biomech. 1982;15(2):111–21. doi: 10.1016/0021-9290(82)90043-4. https://doi.org/10.1016/0021-9290(82)90043-4. [DOI] [PubMed] [Google Scholar]
  • 12.Hunter IW, Kearney RE. Dynamics of human ankle stiffness: Variation with mean ankle torque. J Biomech. 1982;15(10):747–52. doi: 10.1016/0021-9290(82)90089-6. https://doi.org/10.1016/0021-9290(82)90089-6. [DOI] [PubMed] [Google Scholar]
  • 13.Tai C, Robinson CJ. Knee elasticity influenced by joint angle and perturbation intensity. IEEE Trans Rehabil Eng. 1999;7(1):111–5. doi: 10.1109/86.750561. https://doi.org/10.1109/86.750561. [DOI] [PubMed] [Google Scholar]
  • 14.Akazawa K, Milner TE, Stein RB. Modulation of reflex EMG and stiffness in response to stretch of human finger muscle. J Neurophysiol. 1983;49(1):16–27. doi: 10.1152/jn.1983.49.1.16. [DOI] [PubMed] [Google Scholar]
  • 15.Pousson M, Van Hoecke J, Goubel F. Changes in elastic characteristics of human muscle induced by eccentric exercise. J Biomech. 1990;23(4):343–8. doi: 10.1016/0021-9290(90)90062-8. https://doi.org/10.1016/0021-9290(90)90062-8. [DOI] [PubMed] [Google Scholar]
  • 16.De Serres SJ, Milner TE. Wrist muscle activation patterns and stiffness associated with stable and unstable mechanical loads. Exp Brain Res. 1991;86(2):451–8. doi: 10.1007/BF00228972. https://doi.org/10.1007/BF00228972. [DOI] [PubMed] [Google Scholar]
  • 17.Milner TE, Cloutier C, Leger AB, Franklin DW. Inability to activate muscles maximally during cocontraction and the effect on joint stiffness. Exp Brain Res. 1995;107(2):293–305. doi: 10.1007/BF00230049. https://doi.org/10.1007/BF00230049. [DOI] [PubMed] [Google Scholar]
  • 18.Oatis CA, Wolff EF, Lennon SK. Knee joint stiffness in individuals with and without knee osteoarthritis: A preliminary study. J Orthop Sports Phys Ther. 2006;36(12):935–41. doi: 10.2519/jospt.2006.2320. https://doi.org/10.2519/jospt.2006.2320. [DOI] [PubMed] [Google Scholar]
  • 19.Tiselius P. Joint stiffness determinations in rheumatoid arthritis. Acta Rheum Scad Suppl. 1969;14:5249. doi: 10.3109/rhe1.1969.14.suppl-14.01. [DOI] [PubMed] [Google Scholar]
  • 20.Bajd T, Vodovnik L. Pendulum testing of spasticity. J Biomed Eng. 1984;6(1):9–16. doi: 10.1016/0141-5425(84)90003-7. https://doi.org/10.1016/0141-5425(84)90003-7. [DOI] [PubMed] [Google Scholar]
  • 21.Katz RT, Rovai GP, Brait C, Rymer WZ. Objective quantification of spastic hypertonia: Correlation with clinical findings. Arch Phys Med Rehabil. 1992;73(4):339–47. doi: 10.1016/0003-9993(92)90007-j. https://doi.org/10.1016/0003-9993(92)90007-J. [DOI] [PubMed] [Google Scholar]
  • 22.Timiras PS, Navazio FM. The skeleton, joints, and skeletal and cardiac muscles. In: Timiras PS, editor. The physiological basis for aging and geriatrics. 4th ed. New York: Informa Healthcare USA; 2007. pp. 329–43. https://doi.org/10.3109/9781420007091. [Google Scholar]
  • 23.Riemann BL, Lephart SM. The sensorimotor system, part I: The physiologic basis of functional joint stability. J Athl Train. 2002;37(1):71–9. [PMC free article] [PubMed] [Google Scholar]
  • 24.Helliwell PS. Joint stiffness. In: Wright V, Radin EL, editors. Mechanics of joints: Physiology, pathophysiology and treatment. New York: Marcel Dekker; 1993. pp. 203–18. [Google Scholar]
  • 25.Wright V. Stiffness: A review of its measurement and physiological importance. Physiotherapy. 1973;59(4):107–11. [PubMed] [Google Scholar]
  • 26.Blanpied P, Smidt GL. Human plantarflexor stiffness to multiple single-stretch trials. J Biomech. 1992;25(1):29–39. doi: 10.1016/0021-9290(92)90243-t. https://doi.org/10.1016/0021-9290(92)90243-T. [DOI] [PubMed] [Google Scholar]
  • 27.Wilson GJ, Wood GA, Elliott BC. The relationship between stiffness of the musculature and static flexibility: An alternative explanation for the occurrence of muscular injury. Int J Sports Med. 1991;12(4):403–7. doi: 10.1055/s-2007-1024702. https://doi.org/10.1055/s-2007-1024702. [DOI] [PubMed] [Google Scholar]
  • 28.Wartenberg R. Pendulousness of the legs as a diagnostic test. Neurology. 1951;1(1):18–24. doi: 10.1212/wnl.1.1.18. https://doi.org/10.1212/WNL.1.1.18. [DOI] [PubMed] [Google Scholar]
  • 29.Brown RA, Lawson DA, Leslie GC, MacArthur A, MacLennan WJ, McMurdo ME, et al. Does the Wartenberg pendulum test differentiate quantitatively between spasticity and rigidity? A study in elderly stroke and Parkinsonian patients. J Neurol Neurosurg Psychiatry. 1988;51(9):1178–86. doi: 10.1136/jnnp.51.9.1178. https://doi.org/10.1136/jnnp.51.9.1178. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 30.Valle MS, Casabona A, Sgarlata R, Garozzo R, Vinci M, Cioni M. The pendulum test as a tool to evaluate passive knee stiffness and viscosity of patients with rheumatoid arthritis. BMC Musculoskelet Disord. 2006;7:89. doi: 10.1186/1471-2474-7-89. https://doi.org/10.1186/1471-2474-7-89. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 31.Bohannon RW. Variability and reliability of the pendulum test for spasticity using a Cybex II isokinetic dynamometer. Phys Ther. 1987;67(5):659–61. doi: 10.1093/ptj/67.5.659. [DOI] [PubMed] [Google Scholar]
  • 32.Syczewska M, Lebiedowska MK, Pandyan AD. Quantifying repeatability of the Wartenberg pendulum test parameters in children with spasticity. J Neurosci Methods. 2009;178(2):340–4. doi: 10.1016/j.jneumeth.2008.12.031. https://doi.org/10.1016/j.jneumeth.2008.12.031. [DOI] [PubMed] [Google Scholar]
  • 33.Matthews LS, Ellis D. Viscoelastic properties of cat tendon: Effects of time after death and preservation by freezing. J Biomech. 1968;1(2):65–71. doi: 10.1016/0021-9290(68)90008-0. https://doi.org/10.1016/0021-9290(68)90008-0. [DOI] [PubMed] [Google Scholar]
  • 34.Clavert P, Kempf JF, Bonnomet F, Boutemy P, Marcelin L, Kahn JL. Effects of freezing/thawing on the biomechanical properties of human tendons. Surg Radiol Anat. 2001;23(4):259–62. doi: 10.1007/s00276-001-0259-8. https://doi.org/10.1007/s00276-001-0259-8. [DOI] [PubMed] [Google Scholar]
  • 35.Bajd T, Bowman B. Testing and modelling of spasticity. J Biomed Eng. 1982;4(2):90–6. doi: 10.1016/0141-5425(82)90067-x. https://doi.org/10.1016/0141-5425(82)90067-X. [DOI] [PubMed] [Google Scholar]
  • 36.Lin DC, Rymer WZ. A quantitative analysis of pendular motion of the lower leg in spastic human subjects. IEEE Trans Biomed Eng. 1991;38(9):906–18. doi: 10.1109/10.83611. https://doi.org/10.1109/10.83611. [DOI] [PubMed] [Google Scholar]
  • 37.Knecht JF, Wetherbee E. Orthotic options for knee instability and pain. In: Lusardi MM, Nielsen CC, editors. Orthotics and prosthetics in rehabilitation. 2nd ed. St. Louis: Saunders; 2007. pp. 333–4. [Google Scholar]
  • 38.Edelstein JE, Bruckne J. Orthotics: A comprehensive clinical approach. Thorofare: Slack Inc; 2002. pp. 59–62. [Google Scholar]
  • 39.Bowker JH. Transtibial amputation: Surgical management. In: Smith DG, Michael JW, Bowker JH, editors. Atlas of amputations and limb deficiencies. 3rd ed. Rosemont: Decade; 2004. pp. 487–90. [Google Scholar]
  • 40.Kapp SL, Fergason JR. Transtibial amputation: Prosthetic management. In: Smith DG, Michael JW, Bowker JH, editors. Atlas of amputations and limb deficiencies. 3rd ed. Rosemont: Decade; 2004. pp. 510–1. [Google Scholar]
  • 41.Bechtol CO, Ferguson AB, Laing PG. Bone and joint surgery. Baltimore: Williams & Wilkins; 1959. Metals and engineering; p. 1370. [Google Scholar]
  • 42.Smith GK. Orthopaedic biomaterials. In: Newton DC, Nunamaker DM, editors. Textbook of small animal orthopaedics. Philadelphia, PA: JB Lippincott; 1985. p. 13. [Google Scholar]
  • 43.Lu J. Orthopedic bone cement. In: Poitout DG, editor. Biomechanics and biomaterials in orthopaedics. London, UK: Springer; 2004. pp. 87–8. https://doi.org/10.1007/978-1-4471-3774-0_8. [Google Scholar]
  • 44.Black J. Biological performance of materials: Fundamentals of biocompatibility. 4th ed. USA: Taylor & Francis Group; 2006. pp. 406–22. [Google Scholar]
  • 45.Berthiaume F, Yarmush ML. Fundamentals of tissue engineering. In: Palsson B, Hubbell JA, Plonsey R, Bronzino JD, editors. Tissue engineering. Boca Raton, FL: CRC Press; 2003. pp. 118–20. [Google Scholar]
  • 46.Jerman UD, Kreft ME. From tissue engineering to regenerative medicine - A modern approach to the reconstruction of the urinary tract. Zdravniški Vest. 2012;81(10):735–44. [Google Scholar]

Articles from Bosnian Journal of Basic Medical Sciences are provided here courtesy of Association of Basic Medical Sciences of Federation of Bosnia and Herzegovina

RESOURCES