Response time in economic games reflects different types of decision conflict for prosocial and proself individuals

Significance

Prosocial and proself predispositions dictate economic game players’ fast decisions but exert limited influence when game players take a longer time in making decisions. Prosocially predisposed individuals use the extended time to contemplate the risk of being exploited. Selfishly predisposed individuals are suggested to behave according to the immediate incentives of the games when they make decisions quickly and become behaviorally less selfish as they spend more time assessing the long-term strategic implications of always behaving according to immediate incentives. Different strategies are needed to promote prosocial behavior and to design institutions depending on the constitution of the population.

Abstract

Behavioral and neuroscientific studies explore two pathways through which internalized social norms promote prosocial behavior. One pathway involves internal control of impulsive selfishness, and the other involves emotion-based prosocial preferences that are translated into behavior when they evade cognitive control for pursuing self-interest. We measured 443 participants’ overall prosocial behavior in four economic games. Participants’ predispositions [social value orientation (SVO)] were more strongly reflected in their overall game behavior when they made decisions quickly than when they spent a longer time. Prosocially (or selfishly) predisposed participants behaved less prosocially (or less selfishly) when they spent more time in decision making, such that their SVO prosociality yielded limited effects in actual behavior in their slow decisions. The increase (or decrease) in slower decision makers was prominent among consistent prosocials (or proselfs) whose strong preference for prosocial (or proself) goals would make it less likely to experience conflict between prosocial and proself goals. The strong effect of RT on behavior in consistent prosocials (or proselfs) suggests that conflict between prosocial and selfish goals alone is not responsible for slow decisions. Specifically, we found that contemplation of the risk of being exploited by others (social risk aversion) was partly responsible for making consistent prosocials (but not consistent proselfs) spend longer time in decision making and behave less prosocially. Conflict between means rather than between goals (immediate versus strategic pursuit of self-interest) was suggested to be responsible for the time-related increase in consistent proselfs’ prosocial behavior. The findings of this study are generally in favor of the intuitive cooperation model of prosocial behavior.

The question of how humans succeeded in creating and maintaining large-scale cooperation has occupied social and biological scientists for the past few centuries (1–8). Social scientists traditionally sought answers to this question by focusing on the critical role of internalized social norms that constitute the internal norm enforcement system (1–3). Recent neuroscientific and behavioral studies extended this traditional norm-enforcement view of human prosociality in two directions. The first direction is to identify the neural mechanisms exerting cognitive control of the selfish drive. Neuroscientists have found structural and neural correlates of prosocial and norm-enforcing choices in economic games. Experimental evidence shows that prosocial choices in economic games are positively related to cortical gray matter volume, cortical thickness, and activation of the brain areas that exert control over selfish impulsive drives, such as the anterior cingulate cortex (9, 10), dorsolateral prefrontal cortex (DLPFC) (11–13), and temporoparietal junction (14). Impairment of the DLPFC prevents economic game players from engaging in alleged fairness-seeking behaviors (15–17). The second direction emphasizes the intuitive nature of prosocial behavior and challenges the view that emphasizes cognitive norm enforcement. The intuitive prosociality (18) or heuristic cooperation (19–21) model is supported by the findings that prosocial choices in economic games are made faster than selfish choices (19) and are promoted when an individual is under time pressure (19, 20, 22) or making decisions under cognitive load (23–25). Furthermore, a negative relationship was found between activity in the lateral prefrontal cortex and prosocial (26) and fairness-seeking (27) behavior in economic games. According to the heuristic prosociality model (19–21), selection favors humans who are predisposed to cooperate in social exchange situations. When this predisposition is overridden by deliberative reasoning mobilized to secure self-interest, people fail to behave in a prosocial manner. According to the dual-process decision model of human cooperation (28), people cooperate by default and scrutinize the immediate incentive structure surrounding economic games when the default choice of cooperation is not consistent with the immediate incentives, such as in anonymously played one-shot games. A recent metaanalytical study of the effects of manipulating intuitive cooperation shows that manipulations to enhance intuitive decision making promote nonstrategic cooperation but do not promote strategic cooperation (29), and provides further support for this model. Another study using the dictator game (DG) and the ultimatum game (UG) also provides support for this prediction in a different context by showing that the thickness of the DLPFC and psychological measures of deliberative thinking are negatively related to the proportion of giving in the DG, but not in the UG (30). This finding implies that players of economic games use deliberation to assess the incentives (it is better for immediate self-interest not to give any portion of the monetary endowment to the recipient in the DG) rather than to read the respondent’s reaction to secure their self-interest (according to which deliberative players with a thicker DLPFC who are capable of anticipating rejection of unfair offers by the respondent should make fairer offers than less deliberative players in the UG, but not in the DG).

Whether cooperation or defection is an intuitive choice in economic games reflects the social context in which game players are socialized and functioning (31–33). In this study, we focus on the relationship between response time (RT) and behavior in prosocially and selfishly predisposed individuals. The RT of prosocial and proself decisions is not necessarily an indicator of the intuitive versus deliberative nature of the decision (34, 35). In general, the conflict level between two opposing choices causes the more strongly preferred choice to be made more quickly than the weakly preferred choice. Specifically, in the context of prosocial and proself choices in economic games, the level of conflict is expected to be low and the RT is short either when game players’ strong prosocial preferences far exceed their desire for self-interest or when the level of prosocial preferences is too weak to resist their desire for self-interest. Players with intermediate levels of prosocial preferences face a strong conflict between prosocial and selfish goals, which are in balance with each other; their decisions are somewhere in-between extremely prosocial and extremely selfish, and it takes longer for them to reach the intermediate level of behavioral decision. These differential levels of conflict will yield an inverted U-shape relationship between behavioral prosociality and the RT: An initial drop in the players’ preference from extremely to mildly prosocial is accompanied by a drop in prosocial choices in economic games as well as an increase in RT, and a further drop in their preference from mildly proself to extremely proself is accompanied by a further drop in behavioral prosociality and a drop in RT (34, 35). We found exactly this pattern in our data on 443 nonstudent healthy adult (age range: 20–59 y) participants’ overall prosocial behavior and the RT in four economic games, including the DG, prisoner’s dilemma game (PDG), four-person social dilemma game (SDG), and trust game (TG) in which the participants played the trustee’s role. We then classified participants according to three different methods of measuring their social value orientation (SVO) that are commonly used to identify individual differences in prosocial and selfish preferences independent of actual behavioral choices in economic games (36–39). Furthermore, we measured the cortical thickness of the game players and their social preferences, and show the time–behavior relationship is modulated by different neuropsychological processes for prosocially and selfishly disposed players.

We first found the previously found negative relationship between DLPFC (left and right middle frontal gyrus) thickness and prosocial behavior to be stronger in fast decision makers; that is, the reduction in prosocial behavior through scrutiny of the game incentives by individuals with a thicker DLPFC (30) is more pronounced among fast rather than slow decision makers. The pronounced effect of the DLPFC among quick decision makers suggests that prosocial choices by fast decision makers result from a lack of cognitive calculation. We further found that the RT strongly correlated with the overall game behavior among prosocially and selfishly predisposed participants as they were measured by SVO, albeit in different directions. The RT of the consistent SVO prosocials positively correlated with social risk aversion. They are participants who have revealed their prosocial preferences across three different measures of SVO. They are also likely individuals whose prosocial preferences consistently exceed their selfish preferences. Thus, conflict between the prosocial and selfish preferences is not likely to emerge and prolong their RT in economic games. Thus, the RT and behavior correlation even among consistent SVO prosocials suggests that their prolonged decision time is not due to conflict between prosocial and selfish preferences; rather, it is suggested that prosocials who were afraid of the risk of being exploited by others became less cooperative after contemplating this risk. This correlation between social risk aversion and RT was not observed among consistent SVO proselfs. However, consistent SVO proselfs’ levels of overall prosocial game behavior increased with RT despite the fact that their selfish preferences far exceeded their prosocial preferences, suggesting a lack of conflict between the two preferences responsible for a prolonged RT. The time–behavior relationship of the proselfs is speculated to reflect their strategic calculations rather than conflict between prosocial and proself goals.

Results

Cognitive Intervention Reduces Prosocial Behavior When Decisions Are Made Fast.

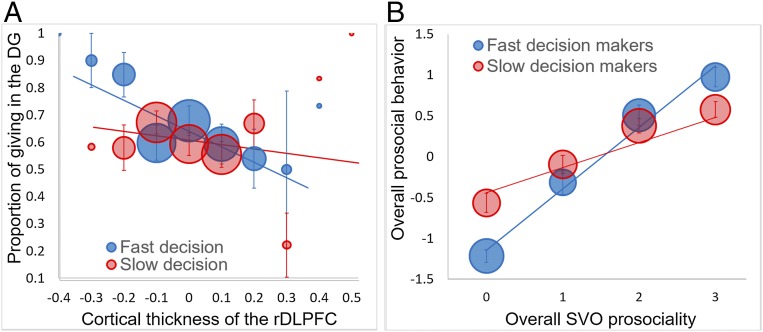
Dataset S1, which we used for analysis, included the same DLPFC and behavioral data that were used in an earlier study (30). This earlier study demonstrated a negative relationship between cortical thickness and prosocial giving behavior in a DG, but not in an UG, and suggested that deliberative scrutiny of the incentives of the DG, rather than reading the partner’s responses in the UG, induces players with a thick DLPFC to behave in a selfish manner. In this analysis, we further found that the negative effect of DLPFC thickness on prosocial giving in the DG interacted with RT in a regression analysis of prosocial giving, including the interaction term (interaction, right: β = 0.355, t = 2.58, P = 0.010; interaction, left: β = 0.238, t = 1.75, P = 0.081). Because the RT in the DG correlated with non–game-related time (r = 0.272, P < 0.0001), we used the RT adjusted for the predicted RT from the non–game-related time to eliminate the confounding effect of general agility in cognitive tasks. Table S1 displays the results with the original nonadjusted time, of which conclusions are mostly consistent with the ones reported in the main text. The DLPFC thickness was negatively correlated with DG prosocial behavior among the participants whose RT was in the faster half (right: r = −0.163, P = 0.022; left: r = −0.126, P = 0.076), but the effect was nonsignificant among the slower half of the participants (right: r = −0.066, P = 0.353; left: r = −0.125, P = 0.080) (Fig. 1A).

Table S1.

List of statistical analyses with the adjusted and nonadjusted RT

Adjusted RT	Nonadjusted RT
We further found that the negative effect of DLPFC thickness on prosocial giving in the DG interacted with the RT in a regression analysis of prosocial giving, including the interaction term (interaction: β = 0.355, t = 2.58, P = 0.010 for the right DLPFC; interaction: β = 0.238, t = 1.75, P = 0.081 for the left DLPFC)	Interaction: β = 0.324, t = 2.33, P = 0.020 for the right DLPFC; interaction β = 0.284, t = 2.10, P = 0.036 for the left DLPFC
The DLPFC thickness was negatively correlated with behavioral prosociality among the participants in the faster half (right: r = −0.163, P = 0.022; left: r = −0.126, P = 0.076), but the effect was nonsignificant among the slower half of participants (right: r = −0.066, P = 0.353; left: r = −0.125, P = 0.080)	Right: r = −0.180, P = 0.009; left: r = −0.148, P = 0.031; right: r = −0.022, P = 0.762; left: r = −0.090, P = 0.225
Cortical thickness of the DLPFC interacted with the overall RT (interaction, right: β = 0.824, t = 2.22, P = 0.027; interaction, left: β = 0.676, t = 1.95, P = 0.052)	Interaction: β = 0.693, t = 1.82, P = 0.069 for the right DLPFC; interaction: β = 0.622, t = 1.80, P = 0.073 for the left DLPFC
The DLPFC thickness negatively correlated with the overall prosocial behavior among the participants whose overall RT was in the faster half (right: r = −0.145, P = 0.041; left: r = −0.148, P = 0.037), but the effect was not significant among the slower half of the players (right: r = −0.079, P = 0.271; left: r = −0.087, P = 0.225)	Right: r = −0.119, P = 0.082; left: r = −0.131, P = 0.054; right: r = −0.068, P = 0.363; left: r = −0.100, P = 0.184
This negative effect of cognitive reappraisal of emotion on prosocial behavior… interacted with the overall RT (interaction: β = 0.132, t = 2.45, P = 0.015); specifically, the negative effect was significant among the faster half of the participants (r = −0.205, P = 0.002), whereas it was not significant among the slower half (r = −0.093, P = 0.172)	Interaction: β = 0.111, t = 2.07, P = 0.039; r = −0.236, P < 0.001; r = −0.029, P = 0.682
The effect of SVO prosociality on behavior interacted with the overall RT (interaction: β = −0.609, t = 5.72, P < 0.0001) (Fig. 1B). Although the effect of SVO prosociality was significant even among the slower half of participants (r = 0.533, P < 0.0001), it was much stronger among faster participants (r = 0.726, P < 0.0001)	Interaction: β = −0.548, t = 5.05, P < 0.0001; r = 0.546, P < 0.0001; r = 0.713, P < 0.0001
The consistent prosocials behaved less prosocially as they spent more time (r = −0.399, P < 0.001), and the consistent proselfs (r = 0.400, P < 0.0001) and the weak proselfs (r = 0.240, P = 0.038) behaved more prosocially as they spent more time; no significant time–behavior relationship was observed among the weak prosocials (r = −0.011, P = 0.909)	r = −0.366, P < 0.001; r = 0.366, P < 0.001; r = 0.210, P = 0.071; r = 0.025, P = 0.797
It should be noted that the RT–behavior relationship is much weaker in the weak prosocials and weak proselfs than in the consistent prosocials and consistent proselfs despite the equivalence in behavior variance [Levene’s test: F(3, 373) = 0.51, P = 0.676] and RT variance [F(3, 373) = 0.67, P = 0.568] in the four levels of SVO	RT variance [F(3, 373) = 1.07, P = 0.360] in the four levels of SVO
We first found that the effect of RT on social risk aversion interacted statistically marginally with SVO (β = 0.679, t = 1.72, P = 0.087) in such a way that consistent prosocials who were high on social risk aversion spent more time than consistent prosocials who were low on social risk aversion (r = 0.295, P = 0.007); on the other hand, the level of social risk aversion was not related to the consistent proselfs’ RT (r = 0.039, P = 0.706)	β = 0.605, t = 1.49, P = 0.137; r = 0.275, P = 0.011; r = 0.085, P = 0.408
Correlation among consistent prosocials… was not significant in these two games (r = −0.014, P = 0.901 in the DG; r = 0.060, P = 0.587 in the TG); in the other two games (the PDG and the SDG)… correlation was significant and strong both in the PDG (r = 0.308, P = 0.005) and the SDG (r = 0.350, P = 0.001); among the consistent proselfs, the correlation was negative and did not differ much between the two types of games (r = 0.007, P = 0.950 in the DG; r = −0.081, P = 0.436 in the TG; r = 0.030, P = 0.771 in the PDG; r = 0.117, P = 0.258 in the SDG)	r = −0.022, P = 0.846 in the DG; r = 0.053, P = 0.635 in the TG; r = 0.290, P = 0.008 in the PDG; r = 0.351, P = 0.001 in the SDG; r = 0.047, P = 0.648 in the DG; r = −0.018, P = 0.861 in the TG; r = 0.033, P = 0.747 in the PDG; r = 0.169, P = 0.099 in the SDG

Fig. 1.

Relationship between prosocial behavior and the correlates for the fast and the slow decision makers. (A) Cortical thickness of the right DLPFC (rDLPFC) and the proportion of giving in the DG. (B) SVO measure of prosociality and overall prosocial behavior. The circle size represents the sample size for each 0.1-mm interval of cortical thickness (A) or for each level of SVO prosociality (B). Error bars indicate SEs for the circles, of which n > 3. Regression lines are based on individual scores.

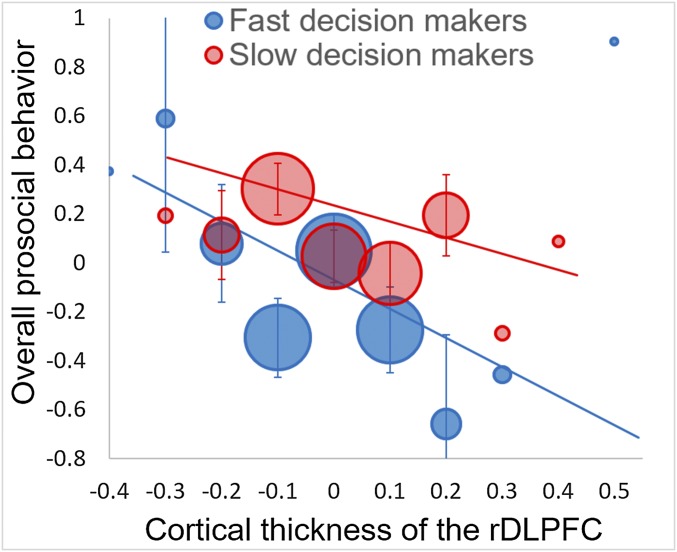
We further demonstrated that the interaction effect of DLPFC cortical thickness and RT was present in general prosocial behavior across the four economic games. For this purpose, we constructed an overall measure of prosocial behavior based on the participants’ behavior in four economic games (including the DG, the PDG, the four-person SDG, and the TG in which the participant played the responder’s role; these games are discussed in SI Materials and Methods, and the factor structure is displayed in Table S2). We also constructed an overall measure of the participants’ RT in those games adjusted for the RT in non–game-related tasks (the results with the original nonadjusted overall RT are discussed in SI Analysis). The cortical thickness of the DLPFC interacted with the overall RT (interaction, right: β = 0.824, t = 2.22, P = 0.027; interaction, left: β = 0.676, t = 1.95, P = 0.052). The DLPFC thickness negatively correlated with the overall prosocial behavior among the participants whose overall RT was in the faster half (right: r = −0.145, P = 0.041; left: r = −0.148, P = 0.037), but the effect was not significant among the slower half of the players (right: r = −0.079, P = 0.271; left: r = −0.087, P = 0.225) (Fig. S1). These results suggest that when deliberative scrutiny of the game incentives is made fast (possibly while participants read the instructions), it prevents prosocial preferences from being actualized in fast prosocial behavior.

Table S2.

Factor structure of the game behaviors, including and not including the second SDG (principal factor method with repeated communality estimation)

Game behavior	Loadings
	Five games	Four games
DG	0.657	0.698
Return behavior in the TG	0.758	0.802
PDG	0.796	0.796
SDG I	0.793	0.724
SDG II	0.733	—
Eigenvalue	2.804	2.289

Fig. S1.

Relationship between the overall prosocial behavior and the cortical thickness of the right DLPFC (rDLPFC). The circle size represents the sample size for each 0.1-mm interval of cortical thickness. Error bars indicate SEs for the circles, of which n > 3. Regression lines are based on individual scores.

The modulation of the relationship between DLPFC thickness and prosocial behavior was corroborated by a similar analysis of the relationship between emotional reappraisal and prosocial behavior. Participants’ responses to the emotional reappraisal scale (40), measuring the responder’s general tendency for cognitively construing an emotion-eliciting situation in a way that alters its emotional and behavioral consequences, negatively correlated with their overall prosocial behavior (r = −0.152, P = 0.001). This negative effect of cognitive reappraisal of emotion on prosocial behavior, which implies control of emotionally driven prosocial behavior, interacted with the overall RT (interaction: β = 0.132, t = 2.45, P = 0.015). Specifically, the negative effect was significant among the faster half of the participants (r = −0.205, P = 0.002), whereas it was not significant among the slower half (r = −0.093, P = 0.172).

SVO Modulates the RT–Behavior Relationship.

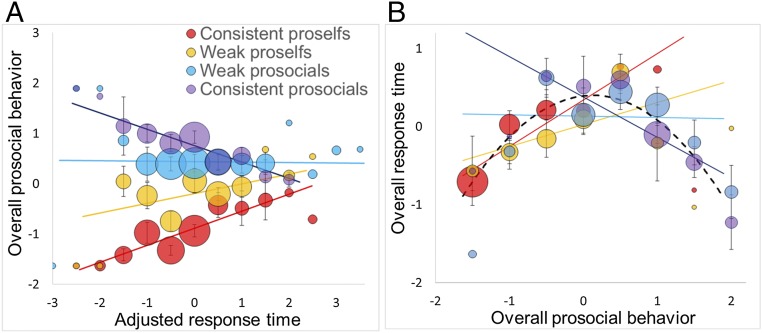
We next found that SVO, which is used in neuroscientific and social scientific studies to measure prosocial and selfish preferences (36–39), is a stronger predictor of the actual game behavior when the decision is made fast rather than slow. For this analysis, the participants were classified into four levels of SVO prosociality based on three classification methods [the slider method (36), the ring method (37), and the triple-dominance method (38)]: Participants identified as proselfs in all three methods (consistent proselfs, n = 98), participants identified as proselfs in two of the three methods (weak proselfs, n = 77), participants identified as prosocials in two of the three methods (weak prosocials, n = 115), and participants identified as prosocials in all three methods (consistent prosocials, n = 87). The levels of SVO prosociality (number of times the participant was classified as a prosocial) strongly correlated with overall prosocial behavior (r = 0.644, P < 0.0001). The effect of SVO prosociality on the overall game behavior interacted with the overall RT (interaction: β = −0.609, t = 5.72, P < 0.0001) (Fig. 1B). Although the effect of SVO prosociality was significant even among the slower half of participants (r = 0.533, P < 0.0001), it was much stronger among faster participants (r = 0.726, P < 0.0001). The interaction between RT and SVO also indicates that the time–behavior relationship was modulated by SVO prosociality (Fig. 2A). The consistent SVO prosocials behaved less prosocially as they spent more time in economic games (r = −0.399, P < 0.001), and the consistent proselfs (r = 0.400, P < 0.0001) and the weak proselfs (r = 0.240, P = 0.038) behaved more prosocially as they spent more time. No significant time–behavior relationship was observed among the weak prosocials (r = −0.011, P = 0.909). When the vertical and horizontal axes of Fig. 2A are transposed, the same interaction effect indicates an inverted U-shaped pattern (Fig. 2B) found in an earlier study (34). It should be noted that the RT–behavior relationship is much weaker in the weak prosocials and weak proselfs than in the consistent prosocials and consistent proselfs despite the equivalence in behavior variance [Levene’s test: F(3, 373) = 0.51, P = 0.676] and RT variance [F(3, 373) = 0.67, P = 0.568] in the four levels of SVO. See SI Analysis and Figs. S2 and S3 for within-individual RT–behavior relationship.

Fig. 2.

Relationship between the overall prosocial behavior and common log-transformed overall RT for each level of the SVO prosociality. (A) Overall prosocial behavior as a function of RT. (B) RT as a function of overall prosocial behavior. The circle size represents the sample size for each 0.5 interval of the common log-transformed RT (A) or 0.5 interval of the overall prosocial behavior (B). Error bars indicate SEs for the circles, of which n > 3. Regression lines are based on individual scores. The dotted line represents the curve linear relationship, including all four levels of SVO prosociality.

Fig. S2.

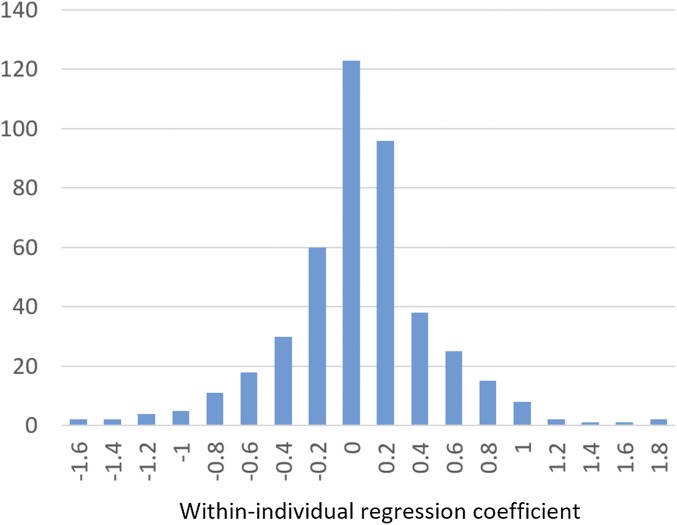
Frequency distribution of the within-individual regression coefficients (common log-transformed on the absolute value). The vertical axis represents the frequency of participants who fell within ±0.2 of the value on the horizontal axis.

Fig. S3.

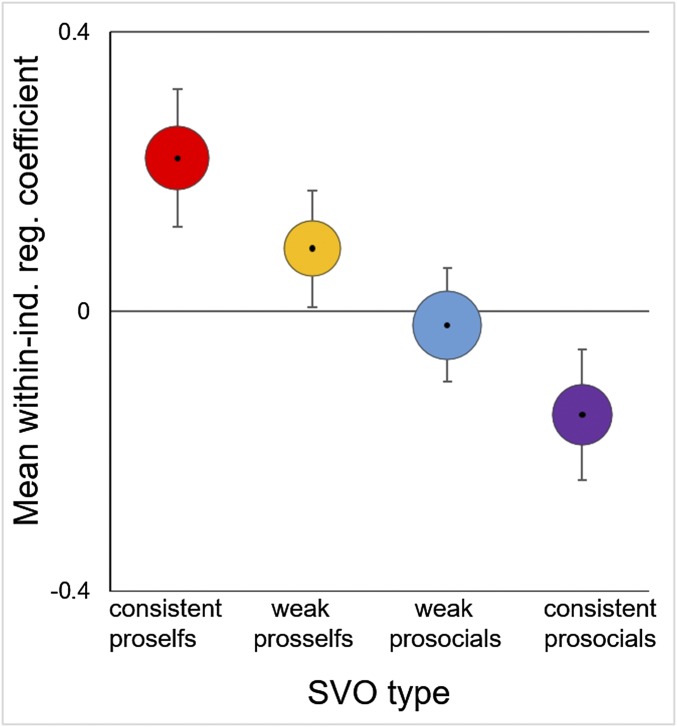
Within-individual regression coefficients as a function of SVO prosociality. Each circle represents the mean regression (reg.) coefficients (common log-transformed) and the number of participants in each type of SVO. Error bars indicate 95% confidence interval.

The Pure Preference Games and Games Vulnerable to Other Players’ Choices.

The decline over time in prosocial choices among prosocials is expected based on the assumption that they are dual-process decision makers (28) who make prosocial decisions by default but sometimes use deliberation to defect when the one-shot nature of the situation is clear. A psychological interpretation of prosocials as dual-process decision makers is that they are “nervous cooperators” who are willing to act in a prosocial way but are reluctant to do so unless they are convinced that they will not be exploited by noncooperators (41); they become reluctant to behave prosocially when they think about the possibility of being exploited by an interaction partner. In the measurement of SVO, respondents face hypothetical situations in which they unilaterally determine the outcome distribution; thus, SVO represents pure preference in the absence of possible exploitation by others. Facing such a choice, nervous prosocials will not hesitate to choose the prosocial alternative in the SVO measurement. On the other hand, some of them may become more hesitant when they face a real social exchange situation in which they could be exploited by others, especially when they figure out the incentives involved in the economic games that encourage proself behavior by the partner. To test this psychological interpretation, we compared levels of social risk aversion (the measurement of social risk aversion is discussed in SI Materials and Methods) between the consistent prosocials and proselfs whose respective preferences are consistent and strong, and who are thus less likely to face the time–behavior relationship within each level of SVO. We found that the effect of RT on social risk aversion interacted statistically marginally with SVO (β = 0.679, t = 1.72, P = 0.087) in such a way that consistent prosocials who were high on social risk aversion spent more time than consistent prosocials who were low on social risk aversion (r = 0.295, P = 0.007). On the other hand, the level of social risk aversion was not related to the consistent proselfs’ RT (r = 0.039, P = 0.706).

To examine further the possibility that nervous prosocials (41) who are willing to cooperate but are afraid of being exploited by others spend time reflecting on this possibility, the relationship between social risk aversion and the RT among consistent prosocials for each of the four constituent games was examined. In both the DG and the TG (trustee), players have the final say on the outcome, and thus need not worry about the negative consequence of their goodwill being exploited. The correlation among consistent prosocials (controlling for non–game-related RT) was not significant in these two games (r = −0.014, P = 0.901 in the DG; r = 0.060, P = 0.587 in the TG). In the other two games (the PDG and the SDG), the players’ final outcome depends on the other players’ choices as well as their own, and thus the players’ goodwill can be exploited. The correlation was significant and strong both in the PDG (r = 0.308, P = 0.005) and the SDG (r = 0.350, P = 0.001). Among the consistent proselfs, the correlation was negative and did not differ much between the two types of games (r = 0.007, P = 0.950 in the DG; r = −0.081, P = 0.436 in the TG; r = −0.030, P = 0.771 in the PDG; and r = 0.117, P = 0.258 in the SDG).

SI Materials and Methods

Sample.

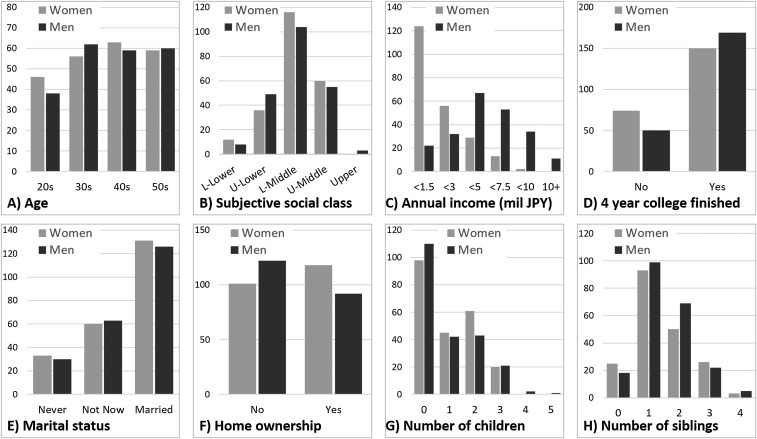
Six hundred nonstudent residents living in Machida, a suburban city of Tokyo, were selected from a list of about 1,670 applicants who responded to a brochure distributed to about 180,000 households. These 600 individuals, ranging in age between 20 and 59 y, consisted of 75 men and 75 women in each 10-y age group. Of the 600 individuals, 564 actually participated in the initial wave of this study (May–July 2012) in which demographic data were collected. One participant’s responses to the demographic items showed obvious inconsistencies; thus, this participant was excluded from the later waves of the study. Major demographic characteristics of the participants used for analysis (n = 443) are presented in Fig. S4.

Fig. S4.

Major demographic characteristics of the participants used for analysis. The number of participants in some panels is less than 443 due to missing responses. Gender and age (A), subjective social class (B), annual income in million (mil) JPY (C), number of participants with a 4-y college degree (D), marital status (E), home ownership (n = 433) (F), number of children (G), and number of siblings (n = 410, including deceased) (H) are shown.

Until the seventh wave of the research project, 16 economic games were conducted: the repeated one-shot PDG with within-participant manipulation of the stake-size (PDG1), the one-shot PDG with continuous choices (PDG2), the DG, the faith game, the TG, the risky DG, the first SDG, the second SDG, the trucking game, the stag-hunt game, the UG, the impunity game, the second-party punishment game, the third-party punishment game, the SDG game with punishment, and the preemptive strike game. To construct the overall measure of behavioral prosociality to be used in this study, we first eliminated games in which punishment was involved, because prosocial behavior in these games can be a purely strategic choice to avoid possible punishment rather than expression of the players’ prosociality. We also eliminated coordination and trust-related games because behaviors in these games are heavily influenced by the players’ beliefs about other players’ choices in addition to their prosociality. Finally, we eliminated the PDG1 in which decision time was not measured. Applying these criteria left five games (PDGs, two SDGs, the DG, and responder behavior in a TG).

The results of a factor analysis (n = 390 participants who participated in all five games; principal factor method with repeated communality estimation) shown in Table S2 indicate that these five game behaviors form a single factor. The eigenvalue of the first factor (2.804) far exceeded the eigenvalue of the second factor (0.259). Because the number of participants who played the second SDG about 3.5 y after the first wave was smaller (n = 424), we decided to exclude behaviors from this game in the following analysis. Another factor analysis excluding this game (n = 443) produced a similar result and is shown in Table S2. The correlation between the four-game version and the five-game version was high (r = 0.983, P < 0.0001). Therefore, we decided to use the remaining four game behaviors to construct the behavioral measure of prosociality. For the analysis, we used 443 participants who participated in all four economic games.

The triple-dominance measure of SVO (38) was administered in the third wave (n = 460), the slider measure (36) was administered in the fifth wave (n = 470), and the ring measure (37) was administered in the sixth wave (n = 433).

Settings Common to All Game Experiments.

Four to 10 people participated in one session, although they did not know how many others were actually in the laboratory, which consisted of 10 compartments. Participants were placed in individual compartments, where they were isolated from the other participants. The instructions were displayed on each participant’s computer screen, accompanied by vocalization of all instructions. The participant listened to the vocalized instruction, and could spend more time for reading the instructions at his/her own pace. The instructions were written with animated cartoons to facilitate the intuitive understanding of the game.

PDG.

The one-shot PDG with the simultaneous protocol was used. The participants were endowed with Japanese yen (JPY) 1,000 and decided how much of the endowment they were going to provide to their partner in increments of JPY 100. When the endowment was provided, the partner received twice the amount of the endowment. For example, when the participant provided JPY 300, the partner received JPY 600. Similarly, the participant received JPY 1,200 if the partner provided JPY 600. The portion of the endowment the participant did not provide was his/hers to keep. We used the proportion of the endowment the participant provided to his/her partner as an indicator of behavioral prosociality.

DG.

All of the participants first played a one-shot DG as the first players who decided how to divide the endowment money, expecting that half of them would be assigned the role of recipients after all of them acted as dictators. Each participant was given an endowment of JPY 1,000 and decided how much of the endowment to provide to his/her partner (the recipient). Participants were actually paid once as a dictator and once as a recipient. Following the initial DG, the participants played similar games six times as a dictator, with a different recipient each time. The size of the endowment varied each time, ranging from JPY 300 to JPY 1,300 (JPY 300, JPY 400, JPY 600, JPY 700, JPY 1,200, and JPY 1,300). Participants were told that they would play the game several times but were not told how many times they would play the game. All participants made allocation decisions as dictator in each game, expecting that two trials would be selected for actual payment. They were further informed that in one of the two games, they would receive the money they allocated to themselves as dictator, and that in the other game, they would receive the money that the matched participant allocated to them. They were actually paid according to this scheme. We used twice the mean proportion that the participant allocated to his/her partners as an indicator of behavioral prosociality in the DG. When twice the mean proportion exceeded 1, we set the participant’s prosociality indicator in the DG at 1, based on the assumption that giving one-half of the endowment is mostly prosocial. The additional analysis with the original score, rather than the truncated score, did not affect the conclusions. Although the previous study (30) included the first game in the analysis, we excluded the first game from the analysis because the RT was not measured in the first game.

SDG.

Participants were told that they would play the game in a group of which the actual size was not conveyed. The instruction was written for a 10-person group, but the participants were told that the actual group size could vary. The game was played once, and participants were paid based on their earnings in the game. Each participant was given an endowment of JPY 1,000 and decided how much of it to provide for the production of a public good in increments of JPY 100. The sum of the provided money for the public good was doubled and equally allocated to all members regardless of their provision level. We used the proportion of the endowment that the participant provided as an indicator of behavioral prosociality in the SDG.

TG.

The TG was played between two randomly matched participants: a truster and a trustee. The truster was provided with JPY 1,000 by the experimenter and decided how much of it to transfer to the trustee in increments of JPY 100. The transferred money was then tripled and provided to the trustee. The trustee received three times the transferred money and then decided how much of it to transfer back to the truster. The endowment money of JPY 1,000 was provided only to the truster and not to the trustee, which differs from the standard version in which both players receive the same amount of endowment money. We introduced this feature to help the older nonstudent participants understand clearly that the back-transfer of half of what they had received as a trustee resulted in a fair outcome when they were fully trusted by the truster. (Note that such is not the case in the standard TG or investment game). When the trustee returns half of the transferred money after it has been transferred, for example, when the truster transfers JPY 1,000 and the trustee returns JPY 1,500 (half of 3 × 1,000), the truster receives JPY 1,500 and the trustee receives JPY 1,500 + endowment of JPY 1,000 = JPY 2,500. In the standard TG, fairness depends on whether players focus only on the transferred money or include the original amount given to the trustee. All participants were told that they would play the game twice, each time with a different partner, and that their role would change. After they had read the instructions, they were all assigned the role of truster and decided how much of the JPY 1,000 to transfer to the trustee. The first game ended when all participants made their decisions as trusters. Then, they were told that they would play the game again, this time with a different partner and in the role of the trustee. In the second game, participants made decisions as trustees using the strategy method; that is, they were asked to indicate the amount of money they would transfer back to the truster in increments of 10% of the tripled money for each of the possible decisions of the matched truster (when they transferred JPY 100, JPY 200, JPY 300, JPY 400, JPY 500, JPY 600, JPY 700, JPY 800, JPY 900, and JPY 1,000). Finally, pairs of participants were formed randomly, and one of each pair was randomly chosen as a truster and the other as a trustee. Each participant’s earnings as a truster depended on the money he/she had provided in the first game and the money that the randomly matched trustee chose in response to the corresponding condition in the second game. We used twice the mean return proportion when the first player transferred more than half of the endowment as an indicator of behavioral prosociality in the TG. When twice the mean return proportion exceeded 1, we set the participant’s prosociality indicator in the TG at 1, based on the assumption that returning one-half of the endowment is mostly prosocial. The additional analysis with the original score rather than the truncated score did not affect the conclusions.

Non–Game-Related Decision Time.

The non–game-related decision time was the mean of the standardized decision time (log-transformed) in the following decision-making tasks: reading the mind in the eyes test (49), choosing a pen task (50), social mindfulness task (51), face recognition task, simplified beauty contest task, and the Machiavellian game (30) that measured the respondent’s strategic thinking. The decision time in each game was common log-transformed and standardized, and the mean of the transformed means was then calculated as the overall non–game-related decision time. The non–game-related time was positively correlated with the overall RT in the four games (r = 0.440, P < 0.0001).

Rock-Paper-Scissors Game Measure of Social Risk Aversion.

Participants were asked to play a hypothetical rock-paper-scissors game with another participant, in which one player shows his/her choice first (rock, paper, or scissors) and the other player makes his/her choice. The second player is thus assured of being a winner. The winner gets JPY 1,000, and the loser gets nothing. If the game is a draw, each player gets JPY 800. The first player has an option of not playing the game. If he/she chooses this option, each player gets JPY 300. The participant is then asked to assume that he/she has been randomly assigned the role of the first player, and is asked if he/she chooses to play the game as a first player. After this initial choice, he/she is again asked to assume a situation in which the second player is randomly chosen from a group of 10 people. These 10 people have been asked to indicate whether they would choose to win (the second player gets JPY 1,000, and the first player gets JPY 0) or to draw (each player gets JPY 800). The participant is asked to indicate whether or not to play the game in each of nine games with different combinations of second players (one draw and nine wins, …, nine draws and one win). In the analysis, we used the total number of choices not to play as a measure of social risk aversion.

SI Analysis

Within-Individual Regression Coefficients.

The pattern of the differential relationship between decision time and behavioral prosociality was also found in a within-individual analysis. For this analysis, we regressed standardized behavioral prosociality in the four games on the standardized decision time for each participant to obtain the within-participant regression coefficient. This within-individual regression coefficient was common log-transformed on the absolute value on both sides of 0 (the distribution is shown in Fig. S2). This coefficient was negatively correlated with the participant’s SVO prosociality (r = −0.291, P < 0.0001). The consistent prosocials decreased the level of behavioral prosociality when they took more time in decision making [i.e., the mean within-individual regression coefficient was negative and significantly different from 0; M = −0.147, t(86) = 3.17, P = 0.002] (Fig. S3). The mean within-individual regression coefficient for the consistent proselfs was positive [M = 0.229, t(97) = 4.56, P < 0.0001]. The mean within-individual regression coefficient was also significantly positive for the weak proselfs [M = 0.098, t(76) = 2.30, P = 0.024], whereas it did not significantly differ from 0 for the weak prosocials [M = −0.011, t(114) = 0.26, P = 0.793].

Analyses with the Original Measure and with the Adjusted Measure of the RT.

As shown in Table S1, the use of nonadjusted time did not substantially change the major conclusions.

Discussion

In this study, we first found additional evidence to support the intuitive prosociality model in general. An earlier study found a negative relationship between prosocial behavior and cortical thickness of the DLPFC in the DG, and we further found that this relationship is shared by other nonstrategic games and is unique to fast decision makers. Our finding that the negative relationship between DLPFC thickness is unique to fast decision makers indicates that prosocial behavior by the fast decision makers with a thinner DLPFC is a straightforward reflection of their predispositions unmitigated by cognitive intervention. This result is corroborated by another finding that emotional reappraisal reduced prosocial behavior only when the decision was made fast, suggesting that fast prosocial choices are straightforward reflections of the participants’ predispositions untamed by cognitive control of the emotional drive to prosociality. These results provide support for the view that the participants’ default choice that escapes cognitive control is a direct reflection of their prosocial preference.

We next compared the prosocials and the proselfs on their behavior–RT relationships to see if the strength of their social preferences similarly modulates the behavior–RT relationship as expected by the strength of preference account of the relationship, and found that the RT–behavior relationship was differentially mediated by factors other than strength of preferences. The time to reach a choice between two alternatives is, in general, a function of the strength of the preferences for the two alternatives. Upon closer examination, however, we found that the RT in economic games is not simply a reflection of the differential preferences for the prosocial and selfish goals. This conclusion derives from strong RT–behavior relationships in the opposite directions in consistent SVO prosocials and consistent proselfs, with each group made up of participants about equal in their levels of prosocial or proself preferences. It is difficult to attribute the RT–behavior relationships to the strength of prosocial or proself preferences within each level of SVO, because the strength of the preferences does not vary much within each SVO level. An alternative explanation is that participants’ preferences strongly affect their fast decisions but not their slow decisions (Fig. 2A). Granting that the conflict between alternative preferences makes the decision slower, it is not the only factor that induces decision makers to take longer to reach a decision. Other factors, such as fear of being exploited or strategic calculations on long-term consequences (i.e., conflict in the means to attain the goal rather than conflict between goals), may also prolong the decision making.

Given that fast decisions are straightforward reflections of the players’ preferences, the results of the current study, on their surface, support both the norm enforcement model and the intuitive cooperation model of the evolution of cooperation, such that the norm enforcement model explains the proselfs’ behavior and the intuitive cooperation model explains the prosocials’ behavior. Prosocials behaved highly prosocially when they made decisions fast, and as they spent more time, they became behaviorally less prosocial. This negative relationship between RT and prosocial behavior supports the view that heuristic prosocials are dual-process decision makers who behave prosocially by default but sometimes stop behaving in a prosocial manner as they scrutinize the incentives (28). Similarly, the proselfs behaved highly selfishly when they made decisions fast, and as they spent more time, they became behaviorally less selfish. This positive relationship between RT and prosocial behavior, on the surface, supports the view that the proselfs behave prosocially when their selfish impulses are suppressed by deliberative control by internalized social norms.

Rather than submitting ourselves to this rather superficial interpretation, we propose an alternative interpretation according to which the decrease in prosocial behavior among prosocials and the increase in prosocial behavior among proselfs may involve different psychological processes. The prosocials’ RT was positively related with the level of social risk aversion, and suggests another reason for the negative relationship between RT and prosocial behavior among prosocials, that is, the possibility that they behaved as “nervous cooperators” (41) who are happy to act prosocially but are reluctant to do so when they notice the possibility of being exploited. We found some evidence to support this interpretation. In two of the four economic games we analyzed (PDG and SDG), the player was not certain how much he/she would earn because his/her final outcome depended on other players’ choices as well as his/her own. In these games, in which prosocial choices may lead to the worst outcome for the player when game partners behave selfishly, the consistent prosocials’ levels of social risk aversion were positively related to their RT. On the other hand, in the other two games [DG and TG (trustee)], the player was assured of the final outcome when he/she made the choice and need not worry about the possibility of being exploited. In these games, the players’ levels of social risk aversion were not correlated with RT.

The correlation between social risk aversion and RT and the differences in correlations between the two types of games were not observed among the proselfs. Given that the consistent proselfs share a similar strong SVO preference for selfishness, it is unlikely that their levels of conflict between prosocial and proself goals are responsible for the strong positive RT–behavior relationship observed among consistent proselfs. A possible explanation may be in the conflict between the means to achieve the selfish goal; that is, the slow proselfs may be strategic rather than impulsive pursuers of self-interest. Compared with fast-deciding proselfs, the proselfs who spend more time may be those individuals who are committed to long-term gains in a wider context through establishing a good reputation and cooperative relationships with others. Although it is logically true that reputational concerns are irrelevant in anonymously played one-shot games, it is possible that selfishly predisposed participants spend extra time thinking about risk management strategies in social exchange (42), which balances the benefit of responding to immediate incentives and the possibility of making errors of missing unforeseen chances of monitoring even in apparently anonymous and one-shot encounters. The proselfs may spend time deliberating on how much weight one should assign to the risk of such unforeseen monitoring and avoid the risk accordingly. According to this speculation, fast and proself behavior of selfishly predisposed participants is not of an emotionally driven nature but, instead, is based on their understanding of the immediate incentives that occur before the decision session starts in the economic game; that is, both slower and faster proselfs clearly understand the immediate incentives while they read the instructions. The slower proselfs use deliberation “wisely” as suggested by a recent study of the positive relationship between wise deliberation and cooperative behavior in an economic game (43).

Our finding that proselfs behaved more prosocially as they spent more time is inconsistent with the earlier finding of no relationship among proselfs (44), although we replicated the negative RT–behavior relationship among prosocials. Based on the finding that the negative relationship is unique only to prosocials, Mischkowski and Glöckner (44) concluded that the heuristic cooperation model applies only to the prosocial type of individuals. If the relationship is negative (more time, less prosocial) among prosocials and does not exist among proselfs (44), the overall relationship, including both prosocial and proself participants, should be weakly negative. This prediction of an overall weak negative relationship is inconsistent with other findings (45). On the other hand, if the relationship is positive among proselfs, as reported in this study, the overall relationship could go in any direction depending on the relative proportion of prosocials and proselfs in the specific study sample, as well as the nature of the task (35). The differential constitution of the sample potentially explains the earlier inconsistencies in the overall relationship.

By definition, it is true that prosocials are individuals whose default choice is prosocial because they give high weight to others’ welfare compared with proselfs (36–38). From the conflict level approach, they are individuals for whom game decisions involve low conflict; thus, their decisions are quick and prosocial. Accordingly, the negative RT–behavior relationship among prosocials can be explained by the generally high level of default prosocial behavior (35). One important contribution of this study is the finding that either consistent prosocials or consistent proselfs, who share about the same level of extremely high or low levels of SVO prosociality within each category, and thus are not likely to face a conflict of goals (i.e., selfish goals and prosocial goals supported by the internalized social norm), show a strong RT–behavior relationship, albeit in different directions. These findings suggest the possibility that the conflict that slowed participants’ decisions was not limited to their goals. In the case of the consistent prosocials, the conflict was more likely between the preference for prosocial goals and aversion to being exploited by others. In the case of consistent proselfs, the conflict could be between the means to achieve the selfish goal rather than between goals. Seen this way, the proselfs’ behavioral pattern can be compatible with the intuitive prosociality model. The adaptive advantage of having a prosocial or proself predisposition depends on the institutions in which individuals are raised and functioning (31–33). When individuals are raised and embedded in collectivistic institutions in which maintaining a good reputation as a decent member of the group is indispensable for social success, an emotionally based drive to behave in a prosocial manner toward group members will function as an adaptive strategy (31, 42). Individuals who are raised and embedded in an individualistic institution that freely allows people to leave the group providing mutual protection and control under the rule of law (46) are likely to be trained to scrutinize the immediate incentives surrounding new encounters so that they can quickly identify the implications of the immediate environment to their self-interest. For the individuals who are embedded in such institutions, default selfish choices of proselfs are not necessarily reflections of their emotionally based intuition. Rather, they are likely to be between means of achieving the selfish goal. If the positive time–prosociality relationship among selfishly predisposed individuals reflects the level of conflict between the means to achieve the selfish goal rather than conflict between the prosocial and proself goals, it is not inconsistent with the intuitive prosociality model. Even proselfs may share the emotional drive for prosociality with prosocials, but their intuitive drive for prosociality can be chronically controlled by their realization of the incentives. If such is actually the case, time pressure or cognitive load may undermine the effort to scrutinize the incentives, and thus promote prosocial behavior even in selfishly predisposed shrewd inspectors of immediate incentives (19, 29). Such a test will enhance the power of the intuitive prosociality model.

Materials and Methods

The study protocol was approved by the Ethics Committee of the Brain Science Institute at Tamagawa University, where the study was conducted. It met the requirements of the Declaration of Helsinki and was executed according to the approved protocol. An informed consent form was signed by each participant after the nature and possible consequences of the study were explained.

Sample.

The study was conducted as part of a research project that started in the spring of 2012, and the ninth wave is under way as of April 2017. Participants were residents of a suburban city of Tokyo and its surroundings. From the original list of 600 registered participants, 564 attended in the first wave of the study. For the analysis, we used 443 participants who participated in all four economic games (the demographic composition of the final sample is shown in Fig. S4).

The Behavioral Measure of Prosociality.

Participants played four economic games: the PDG, DG, n-person SDG, and TG. In each game, participants were provided with some money from the experimenter and decided whether or not and how much of it to provide to another participant (details are provided in SI Materials and Methods, and the distribution of proportional provision of the endowment money in each game is shown in Fig. S5). Participants’ prosocial choice (provision of money) was first standardized within each game, and the overall measure of behavioral prosociality was then constructed by taking the mean of the standardized scores of prosocial behavior in the four games (Cronbach’s α = 0.841; the factor structure is displayed in Table S2). To facilitate interpretation of the findings, we standardized the overall measure of behavioral prosociality with a mean of 0 and SD of 1. The distribution of this overall behavioral prosociality is shown in Fig. S6.

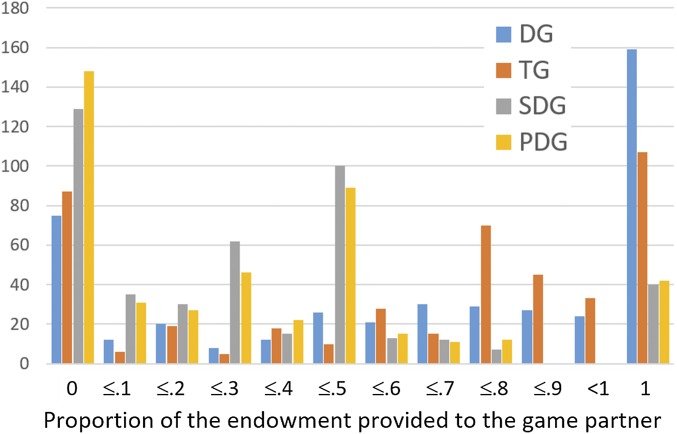
Fig. S5.

Frequency distribution of the proportional provision of the endowment in each of the four games.

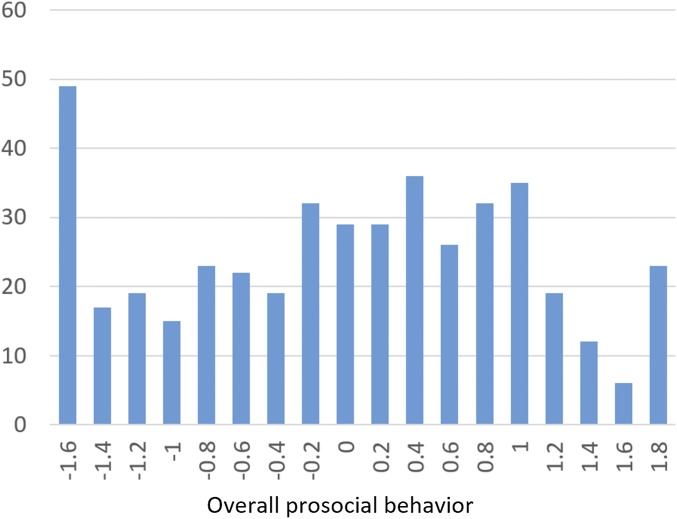
Fig. S6.

Frequency distribution of the overall behavioral prosociality. The vertical axis represents the frequency of participants who fell within ±0.2 of the value on the horizontal axis.

Measurement of RT.

In the four economic games used to measure RT, information was displayed on the screen and the player’s decisions were entered by clicking a relevant button on the screen. In each game, players first received instructions and then clicked the “ready” button when they understood the instructions. After clicking the ready button, a new screen for the decision phase appeared. A few seconds after the screen changed to the decision phase, the decision buttons appeared and the decision time count started. The decision time was measured in milliseconds by the clock function provided by Visual Studio 2010/2012 and was the duration between the appearance of the decision buttons and the player’s click of the relevant decision button. The overall decision time was calculated by the mean common log-transformed decision time in each game, which was standardized before being aggregated. The overall decision time was standardized after aggregation (the distribution is shown in Fig. S7).

Fig. S7.

Frequency distribution of the overall RT adjusted for non–game-related RT. The vertical axis represents the frequency of participants who fell within ±0.25 of the value on the horizontal axis.

The SVO Measures.

Participants’ SVO measure of prosocial preferences was assessed three times, using a different method each time. We combined these three measures to construct the overall measure of prosocial preference. Specifically, participants were first categorized into prosocials and proselfs according to each of the three measures of SVO [the slider measure (36), the ring measure (37), and the triple-dominance measure (38)], and the number of instances they were categorized as prosocials was used as the overall measure of SVO prosociality. Participants who did not complete all three methods were excluded from the analysis, leaving 377 participants for analysis involving SVO. The validity of our data was confirmed by the high correlation of r = 0.644 between overall behavioral prosociality and the overall SVO measure of prosociality, which is much higher than the correlation of around r = 0.30 between SVO measured by a single method and actual game behavior in a single game (47). The high correlation in the current study supports the advantage of the method we used to measure the participants’ behavioral prosociality by using their behaviors in several games and measuring the SVO prosociality with three different methods.

MRI Data Acquisition and Analysis.

Magnetic resonance images were recorded on a 3-T Siemens Trio A Tim MRI scanner. High-resolution anatomical images were acquired using a T1-weighted, 3D, magnetization prepared rapid acquisition gradient echo sequence (repetition time = 2,000 ms, echo time = 1.98 ms, field of view = 256 × 256 mm, number of slices = 192, voxel size = 1 × 1 × 1 mm, average = three times). The gray matter thickness of the regions labeled as the middle frontal gyrus was extracted as the volume of the DLPFC. Gray matter thickness values were estimated for the DLPFC of both hemispheres that was the focus of analysis in the earlier study (30) using the FreeSurfer package (version 5.1.0 for Linux CentOS 4; surfer.nmr.mgh.harvard.edu). Three T1-weighted magnetic resonance images were registered and averaged for each participant. The mean images were submitted to a fully automated procedure that reconstructed 3D models of the pial surface and the boundary between the gray and white matter. The initial part of the reconstruction procedure included registration to a common stereotactic space, image intensity correction for magnetic field inhomogeneity, and skull stripping. The boundary between the gray and white matter for each hemisphere was segmented, tessellated, and corrected for topological errors. The resulting surface models of the boundary were aligned to a surface template by matching the gyral and sulcal patterns to the template. We computed the cortical thickness of the regions of interest using cortical parcellation based on the atlas of Destrieux et al. (48), which divides each cortical hemisphere into 74 regions. Gray matter thickness was calculated as the closest distance between the gray/white matter boundary and the pial surface. We used the atlas of Destrieux et al. (48) to match the DLPFC with the same region used in the earlier study (30). Specifically, the DLPFC was matched with regions 15 (middle frontal gyrus) in the atlas of Destrieux et al. (48).

Statistical Analysis.

The number of participants varied across analyses because some participants took some measures but not others. The numbers of valid participants for each of the variables used in the analysis are as follows: n = 443 for age, gender, the emotion reappraisal scale, the behavior and the RT in the DG, the overall prosocial behavior, and the overall RT; n = 437 for the rock-paper-scissors measure of social risk aversion and n = 377 for SVO prosociality, including only the participants who responded to all three measures of SVO. All RTs reported in the analysis are adjusted for the non–game-related RT (SI Materials and Methods). Age and gender were controlled in all regression and correlation analyses (i.e., the reported r and β values are given after controlling for these variables), and intracranial volume was additionally controlled in the analysis involving the cortical thickness of the DLPFC.