Skip to main content
PMC home page
Nature Communications logoLink to Nature Communications
. 2016 Oct 10;7:13040. doi: 10.1038/ncomms13040

Isotope analysis in the transmission electron microscope

Toma Susi 1,a, Christoph Hofer 1, Giacomo Argentero 1, Gregor T Leuthner 1, Timothy J Pennycook 1, Clemens Mangler 1, Jannik C Meyer 1, Jani Kotakoski 1,b
PMCID: PMC5476802  PMID: 27721420

Abstract

The Ångström-sized probe of the scanning transmission electron microscope can visualize and collect spectra from single atoms. This can unambiguously resolve the chemical structure of materials, but not their isotopic composition. Here we differentiate between two isotopes of the same element by quantifying how likely the energetic imaging electrons are to eject atoms. First, we measure the displacement probability in graphene grown from either 12C or 13C and describe the process using a quantum mechanical model of lattice vibrations coupled with density functional theory simulations. We then test our spatial resolution in a mixed sample by ejecting individual atoms from nanoscale areas spanning an interface region that is far from atomically sharp, mapping the isotope concentration with a precision better than 20%. Although we use a scanning instrument, our method may be applicable to any atomic resolution transmission electron microscope and to other low-dimensional materials.

Inline graphicElectron microscopy can reveal a material’s chemical structure down to the atomic level, but has so far been blind to isotopic differences. Here the authors are able to map isotope concentrations in graphene by measuring the probability of ejecting atoms, demonstrating a ‘mass spectrometer in the microscope’.

Spectroscopy and microscopy are two fundamental pillars of materials science. By overcoming the diffraction limit of light, electron microscopy has emerged as a particularly powerful tool for studying low-dimensional materials such as graphene1, in which each atom can be distinguished. Through advances in aberration-corrected scanning transmission electron microscopy2,3 (STEM) and electron energy loss spectroscopy4,5, the vision of a ‘synchrotron in a microscope’6 has now been realized. Spectroscopy of single atoms, including their spin state7, has together with Z-contrast imaging3 allowed the identity and bonding of individual atoms to be unambiguously determined4,8,9,10. However, discerning the isotopes of a particular element has not been possible—a technique that might be called ‘mass spectrometer in a microscope’.

Here we show how the quantum mechanical description of lattice vibrations lets us accurately model the stochastic ejection of single atoms11,12 from graphene consisting of either of the two stable carbon isotopes. Our technique rests on a crucial difference between electrons and photons when used as a microscopy probe: due to their finite mass, electrons can transfer significant amounts of momentum. When a highly energetic electron is scattered by the electrostatic potential of an atomic nucleus, a maximal amount of kinetic energy (inversely proportional to the mass of the nucleus, ∝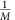Inline graphic) can be transferred when the electron backscatters. When this energy is comparable to the energy required to eject an atom from the material, defined as the displacement threshold energy Td—for instance, when probing pristine11 or doped13 single-layer graphene with 60–100 keV electrons—atomic vibrations become important in activating otherwise energetically prohibited processes due to the motion of the nucleus in the direction of the electron beam. The intrinsic capability of STEM for imaging further allows us to map the isotope concentration in selected nanoscale areas of a mixed sample, demonstrating the spatial resolution of our technique. The ability to do mass analysis in the transmission electron microscope thus expands the possibilities for studying materials on the atomic scale.

Results

Quantum description of vibrations

The velocities of atoms in a solid are distributed based on a temperature-dependent velocity distribution, defined by the vibrational modes of the material. Due to the geometry of a typical transmission electron microscopy (TEM) study of a two-dimensional material, the out-of-plane velocity vz, whose distribution is characterized by the mean square velocity 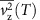Inline graphic, is here of particular interest. In an earlier study11 this was estimated using a Debye approximation for the out-of-plane phonon density of states14 (DOS) gz(ω), where ω is the phonon frequency. A better justified estimate can be achieved by calculating the kinetic energy of the atoms via the thermodynamic internal energy, evaluated using the full phonon DOS.

As a starting point, we calculate the partition function Z=Tr{e−H/(kT)}, where Tr denotes the trace operation and k is the Boltzmann constant and T the absolute temperature. We evaluate this trace for the second-quantized Hamiltonian H describing harmonic lattice vibrations15:

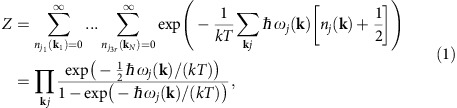graphic file with name ncomms13040-m3.jpg

where ħ is the reduced Planck constant, k the phonon wave vector, j the phonon branch index running to 3r (r being the number of atoms in the unit cell), ωj(k) the eigenvalue of the jth mode at k, and nj(k) the number of phonons with frequency ωj(k).

After computing the internal energy 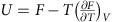Inline graphic from the partition function via the Helmholtz free energy F=−kT lnZ, we obtain the Planck distribution function describing the occupation of the phonon bands (Methods). We must then explicitly separate the energy into the in-plane Up and out-of-plane Uz components, and take into account that half the thermal energy equals the kinetic energy of the atoms. This gives the out-of-plane mean square velocity of a single atom in a two-atom unit cell as

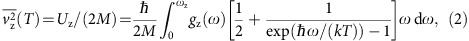graphic file with name ncomms13040-m5.jpg

where M is the mass of the vibrating atom, ωz is the highest out-of-plane mode frequency, and the correct normalization of the number of modes 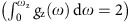Inline graphic is included in the DOS.

Phonon dispersion

To estimate the phonon DOS, we calculated through density functional theory (DFT; GPAW package16,17) the graphene phonon band structure18,19 via the dynamical matrix using the ‘frozen phonon method’ (Methods; Supplementary Fig. 1). Taking the density of the components corresponding to the out-of-plane acoustic (ZA) and optical (ZO) phonon modes (Supplementary Data 1) and solving equation 2 numerically, we obtain a mean square velocity 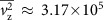Inline graphic m2s−2 for a 12C atom in normal graphene. This description can be extended to ‘heavy graphene’ (consisting of 13C instead of a natural isotope mixture). A heavier atomic mass affects the velocity through two effects: the phonon band structure is scaled by the square root of the mass ratio (from the mass prefactor of the dynamical matrix), and the squared velocity is scaled by the mass ratio itself (equation 2). At room temperature, the first correction reduces the velocity by 3% in fully 13C graphene compared with normal graphene, and the second one reduces it by an additional 10%, resulting in 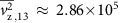Inline graphic m2s−2.

Electron microscopy

In our experiments, we recorded time series at room temperature using the Nion UltraSTEM100 microscope, where each atom, or its loss, was visible in every frame. We chose small fields of view (∼1 × 1 nm2) and short dwell times (8 μs) to avoid missing the refilling of vacancies (an example is shown in Fig. 1; likely this vacancy only appears to be unreconstructed due to the scanning probe). In addition to commercial monolayer graphene samples (Quantifoil R 2/4, Graphenea), we used samples of 13C graphene synthesized by chemical vapour deposition (CVD) on Cu foils using 13C-substituted CH4 as carbon precursor, subsequently transferred onto Quantifoil TEM grids. An additional sample consisted of grains of 12C and 13C graphene on the same grid, synthesized by switching the precursor during growth (Methods).

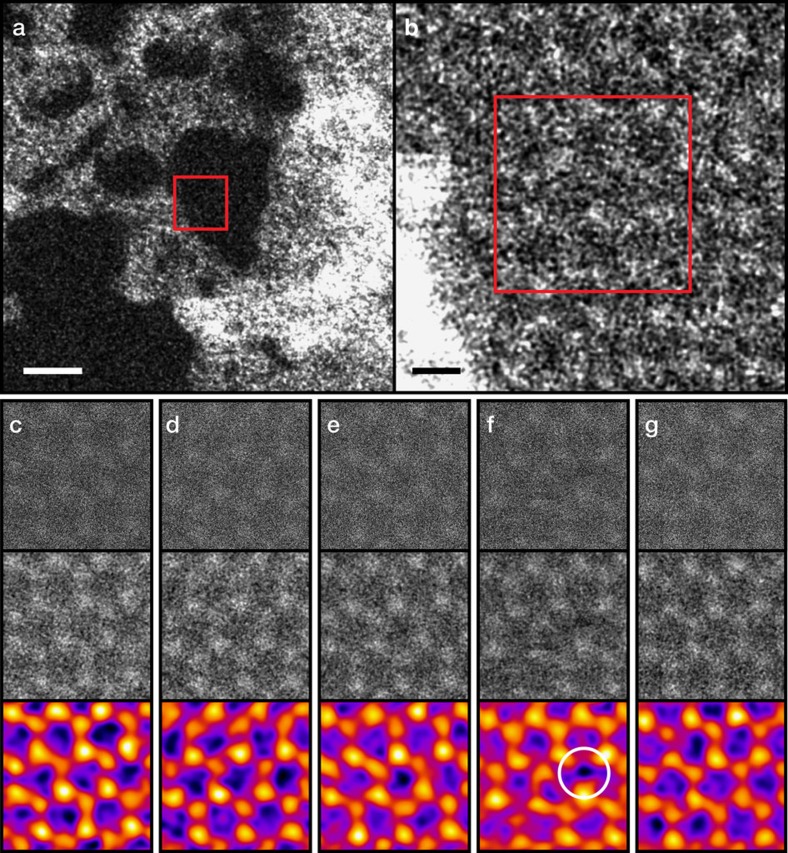
Figure 1. Example of the STEM displacement measurements.

Figure 1

Open in a new tab

The micrographs are medium angle annular dark field detector images recorded at 95 kV. (a) A spot on the graphene membrane, containing clean monolayer graphene areas (dark) and overlying contamination (bright). Scale bar, 2 nm. (b) A closer view of the area marked by the red rectangle in (a), with the irradiated area of the following panels similarly denoted. Scale bar, 2 Å. (c–g) Five consecutive STEM frames (∼1 × 1 nm2, 512 × 512 pixels (px), 2.2 s per frame) recorded at a clean monolayer area of graphene. A single carbon atom has been ejected in the fourth frame (f, white circle), but the vacancy is filled already in the next frame (g). The top row of (c–g) contains the unprocessed images, the middle row has been treated by a Gaussian blur with a radius of 2 px, and the coloured bottom row has been filtered with a double Gaussian procedure3 (σ1=5 px, σ2=2 px, weight=0.16).

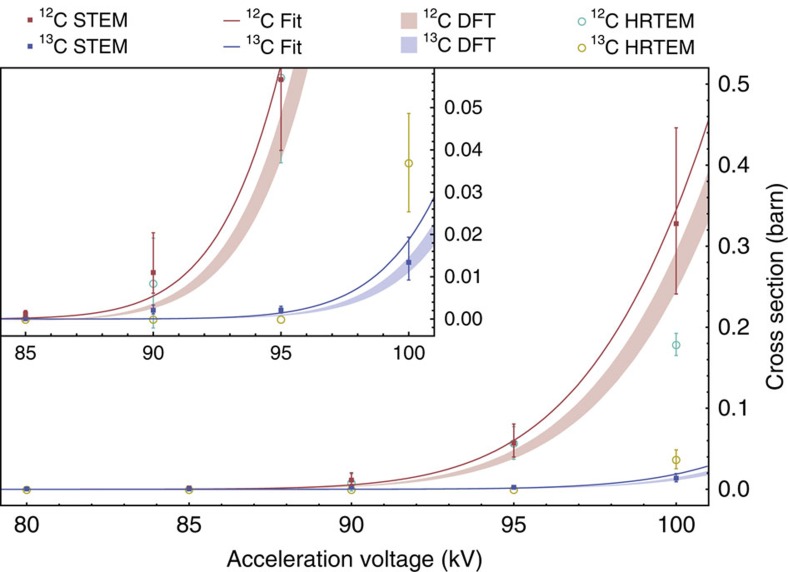
From each experimental dataset (full STEM data available20) within which a clear displacement was observed, we calculated the accumulated electron dose until the frame where the defect appeared (or a fraction of the frame if it appeared in the first one). The distribution of doses corresponds to a Poisson process12 whose expected value was found by log-likelihood minimization (Methods; Supplementary Fig. 2), directly yielding the probability of creating a vacancy (the dose data and statistical analyses are included in Supplementary Data 2). Figure 2 displays the corresponding displacement cross sections measured at voltages between 80 and 100 kV for normal (1.109% 13C) and heavy graphene (∼99% 13C), alongside values measured earlier11 using high-resolution TEM (HRTEM). For low-probability processes, the cross section is highly sensitive to both the atomic velocities and the displacement threshold energy. Since heavier atoms do not vibrate with as great a velocity, they receive less of a boost to the momentum transfer from an impinging electron. Thus, fewer ejections are observed for 13C graphene.

Figure 2. Displacement cross sections of 12C and 13C measured at different acceleration voltages.

Figure 2

Open in a new tab

The STEM data is marked with squares, and earlier HRTEM data11 with circles. The error bars correspond to the 95% confidence intervals of the Poisson means (STEM data) or to previously reported estimates of statistical variation (HRTEM data11). The solid curves are derived from our theoretical model with an error-weighted least-squares best-fit displacement threshold energy of 21.14 eV. The shaded areas correspond to the same model using the lowest DFT threshold Td∈[21.25, 21.375] eV. The inset is a closer view of the low cross section region.

Comparing theory with experiment

The theoretical total cross sections σd(T, Ee) are plotted in Fig. 2 for each voltage (Methods; Supplementary Table 1, Supplementary Data 2). The motion of the nuclei was included via a Gaussian distribution of atomic out-of-plane velocities P(vz, T) characterized by the DFT-calculated 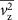Inline graphic, otherwise similar to the approach of ref. 11. A common displacement threshold energy was fitted to the data set by minimizing the variance-weighted mean square error (the 100 kV HRTEM point was omitted from the fitting, since it was underestimated probably due to the undetected refilling of vacancies, also seen in Fig. 1). The optimal Td value was found to be 21.14 eV, resulting in a good description of all the measured cross sections. Notably, this is 0.8 eV lower than the earlier value calculated by DFT, and 2.29 eV lower than the earlier fit to HRTEM data11. Different exchange correlation functionals we tested all overestimate the experimental value (by <1 eV), with the estimate Td∈[21.25, 21.375] closest to experiment resulting from the C09 van der Waals functional21 (Methods).

Despite DFT overestimating the displacement threshold energy, we see from the good fit to the normal and heavy graphene data sets that our theory accurately describes the contribution of vibrations. Further, the HRTEM data and the STEM data are equally well described by the theory despite having several orders of magnitude different irradiation dose rates. This can be understood in terms of the very short lifetimes of electronic and phononic excitations in a metallic system22 compared with the average time between impacts. Even a very high dose rate of 108 e−Å−2s−1 corresponds to a single electron passing through a 1 nm2 area every 10−10 s, whereas valence band holes are filled23 in <10−15 s and core holes24 in <10−14 s, while plasmons are damped25 within ∼10−13 s and phonons26 in ∼10−12 s. Our results thus show that multiple excitations do not contribute to the knock-on damage in graphene, warranting another explanation (such as chemical etching11) for the evidence linking a highly focused HRTEM beam to defect creation27. Each impact is, effectively, an individual perturbation of the equilibrium state.

Local mapping of isotope concentration

Finally, to test the spatial resolution of our method, we studied a sample consisting of joined grains of 12C and 13C graphene. Isotope labelling combined with Raman spectroscopy mapping is a powerful tool for studying CVD growth of graphene28, which is of considerable technological interest. Earlier studies have revealed the importance of carbon solubility into different catalyst substrates to control the growth process29. However, the spatial resolution of Raman spectroscopy is limited, making it impossible to obtain atomic-scale information of the transition region between grains of different isotopes.

The local isotope analysis is based on fitting the mean of the locally measured electron doses with a linear combination of doses generated by Poisson processes corresponding to 12C and 13C graphene using the theoretical cross section values. Although each dose results from a stochastic process, the expected doses for 12C and 13C are sufficiently different that measuring several displacements decreases the errors of their means well below the expected separation (Fig. 3c). To estimate the expected statistical variation for a certain number of measured doses, we generated a large number of sets of n Poisson doses, and calculated their means and standard errors as a function of the number of doses in each set. The calculated relative errors scale as 1/n and correspond to the precision of our measurement, which is better than 20% for as few as five measured doses in the ideal case. Although our accuracy is difficult to gauge precisely, by comparing the errors of the cross sections measured for isotopically pure samples to the fitted curve (Fig. 2), an estimate of roughly 5% can be inferred.

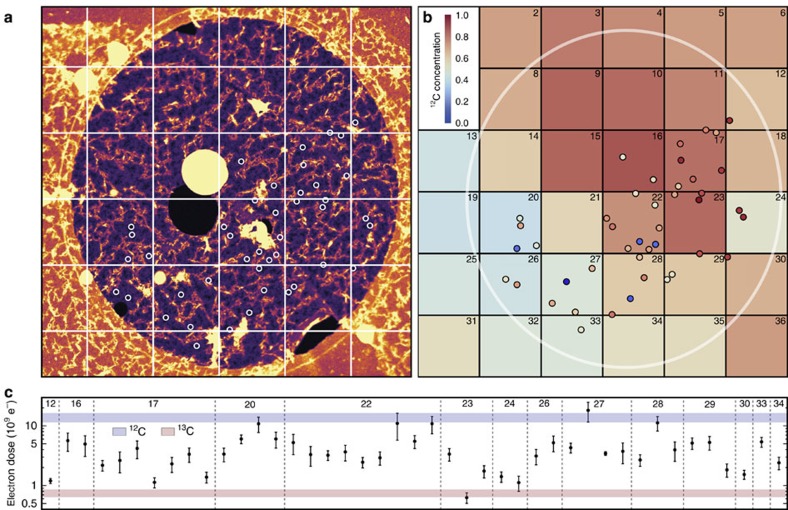
Figure 3. Local isotope analysis.

Figure 3

Open in a new tab

(a) A STEM micrograph of a hole in the carbon support film (1.3 μm in diameter), covered by a monolayer of graphene. In each of the overlaid spots, 4–15 fields of view were irradiated. The dimensions of the overlaid grid correspond to the pixels of a Raman map recorded over this area. (b) Isotope concentration map where the colours of the grid squares denote 12C concentration based on the fitting of the Raman 2D band response (Methods; Supplementary Fig. 3). The overlaid spots correspond to (a), with colours denoting the concentration of 12C estimated from the mean of the measured doses. (c) Locally measured mean doses and their standard errors plotted on a log scale for each grid square. The horizontal coloured areas show the means±s.e. of doses simulated for the theoretical 12C and 13C cross sections. Note that a greater variation in the experimental doses is expected for areas containing a mix of both carbon isotopes.

Working at 100 kV, we selected spots containing areas of clean graphene (43 in total) each only a few tens of nanometers in size (Fig. 1a), irradiating 4–15 (mean 7.8) fields of view 1 × 1 nm2 in size until the first displacement occurred (Fig. 1f). Comparing the mean of the measured doses to the generated data, we can estimate the isotope concentration responsible for such a dose. This assignment was corroborated by Raman mapping over the same area, allowing the two isotopes to be distinguished by their differing Raman shift. A general trend from 12C-rich to 13C-rich regions is captured by both methods (Fig. 3b), but a significant local variation in the measured doses is detectable (Fig. 3c). This variation indicates that the interfaces formed in a sequential CVD growth process may be far from atomically sharp30, instead spanning a region of hundreds of nanometers, within which the carbon isotopes from the two precursors are mixed together.

Discussion

It is interesting to compare our method to established mass analysis techniques. In isotope ratio mass spectrometry precisions of 0.01% and accuracies of 1% have been reported31. However, these measurements are not spatially resolved. For spatially resolved techniques, one of the most widely used is time-of-flight secondary ion mass spectroscopy (ToF-SIMS). It has a lateral resolution typically of several micrometers, which can be reduced to around 100 nm by finely focusing the ion beam32. In the case of ToF-SIMS, separation of the 13C signal from 12C1H is problematic, resulting in a reported33 precision of 20% and an accuracy of ∼11%. The state-of-the-art performance in local mass analysis can be achieved with atom-probe tomography34 (APT), which can record images with sub-nanometer spatial resolution in all three dimensions. A recent APT study of the 13C/12C ratio in detonation nanodiamonds reported a precision of 5%, but biases in the detection of differently charged ions limited accuracy to ∼25% compared to the natural isotope abundances35.

A limitation of ToF-SIMS is its inability to discriminate between the analyte and contaminants and that it requires uniform isotope concentrations over the beam area for accurate results. APT requires the preparation of specialized needle-like sample geometries, a laborious reconstruction process to analyse its results36, and its detection efficiency is rather limited37. In our case, we are only able to resolve relative mass differences between isotopes of the same element in the same chemical environment. While we do not need to resolve mass differences between different elements, since these differ in their scattering contrast, we do need to detect the ejection of single atoms, limiting the technique to atomically thin materials. However, our method captures the isotope information concurrently with atomic resolution imaging in a general-purpose electron microscope, without the need for additional detectors.

We have shown how the Ångström-sized electron probe of a scanning transmission electron microscope can be used to estimate isotope concentrations via the displacement of single atoms. Although these results were achieved with graphene, our technique should work for any low-dimensional material, including hexagonal boron nitride and transition metal dichalcogenides such as MoS2. This could potentially extend to van der Waals heterostructures38 of a few layers or other thin crystalline materials, provided a difference in the displacement probability of an atomic species can be uniquely determined. Neither is the technique limited to STEM: a parallel illumination TEM with atomic resolution would also work, although scanning has the advantage of not averaging the image contrast over the field of view. The areas we sampled were in total less than 340 nm2 in size, containing ∼6,600 carbon atoms of which 337 were ejected. Thus, while the nominal mass required for our complete analysis was already extremely small (131 zg), the displacement of only five atoms is required to distinguish a concentration difference of less than twenty per cent. Future developments in instrumentation may allow the mass-dependent energy transfer to be directly measured from high-angle scattering39,40, further enhancing the capabilities of STEM for isotope analysis.

Methods

Quantum model of vibrations

The out-of-plane mean square velocity 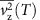Inline graphic can be estimated by calculating the kinetic energy via the thermodynamic internal energy using the out-of-plane phonon DOS gz(ω), where ω is the phonon frequency. In the second quantization formalism, the Hamiltonian for harmonic lattice vibrations is ref. 15

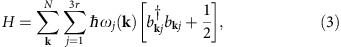graphic file with name ncomms13040-m11.jpg

where k is the phonon wave vector, j is the phonon branch index running to 3r (r being the number of atoms in the unit cell), ωj(k) the eigenvalue of the jth mode at k, and 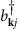Inline graphic and bkj are the phonon creation and annihilation operators, respectively.

Using the partition function Z=Tr{e−H/(kT)}, where Tr denotes the trace operation and k is the Boltzmann constant and T the absolute temperature, and evaluating the trace using this Hamiltonian, we have

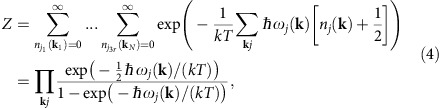graphic file with name ncomms13040-m13.jpg

where 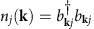Inline graphic is the number of phonons with frequency ωj(k).

The Helmholtz free energy is thus

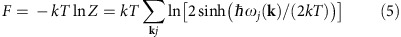graphic file with name ncomms13040-m15.jpg

and the internal energy of a single unit cell, therefore, becomes15

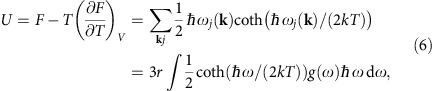graphic file with name ncomms13040-m16.jpg

where in the last step the sum is expressed as an average over the phonon DOS. Using the identity 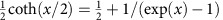Inline graphic yields the Planck distribution function describing the occupation of the phonon bands, and explicitly dividing the energy into the in-plane Up and out-of-plane Uz components, we can rewrite this as

graphic file with name ncomms13040-m18.jpg

where the number of modes is included in the normalization of the DOSes, that is, 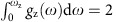Inline graphic, corresponding to the out-of-plane acoustic (ZA) and optical (ZO) modes (the in-plane DOS gp(ω) being correspondingly normalized to 4), and ωd is the highest frequency of the highest phonon mode.

Since half of the thermal energy equals the average kinetic energy of the atoms, and the graphene unit cell has two atoms, the out-of-plane kinetic energy of a single atom is

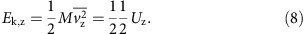graphic file with name ncomms13040-m20.jpg

Thus, the out-of-plane mean square velocity of an atom becomes

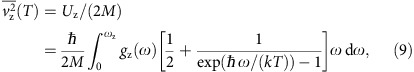graphic file with name ncomms13040-m21.jpg

where ωz is now the highest out-of-plane mode frequency. This can be solved numerically for a known gz(ω).

For the in-plane vibrations, we would equivalently get

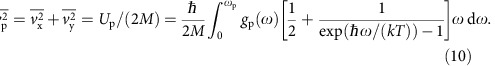graphic file with name ncomms13040-m22.jpg

Frozen phonon calculation

To estimate the phonon DOS, we calculated the graphene phonon band structure via the dynamical matrix, which was computed by displacing each of the two primitive cell atoms by a small displacement (0.06 Å) and calculating the forces on all other atoms in a 7 × 7 supercell (‘frozen phonon method’; the cell size is large enough so that the forces on the atoms at its edges are negligible) using DFT as implemented in the grid-based projector-augmented wave code (GPAW) package17. Exchange and correlation were described by the local density approximation41, and a Γ-centered Monkhorst-Pack k-point mesh of 42 × 42 × 1 was used to sample the Brillouin zone. A fine computational grid spacing of 0.14 Å was used alongside strict convergence criteria for the structural relaxation (forces <10−5 eVÅ−1 per atom) and the self-consistency cycle (change in eigenstates <10−13 eV2 per electron). The resulting phonon dispersion (Supplementary Fig. 1) describes well the quadratic dispersion of the ZA mode near Γ, and is in excellent agreement with earlier studies18,19. Supplementary Data 1 contains the out-of-plane phonon DOS.

Graphene synthesis and transfer

In addition to commercial monolayer graphene (Graphenea QUANTIFOIL R 2/4), our graphene samples were synthesized by CVD in a furnace equipped with two separate gas inlets that allow for independent control over the two isotope precursors29 (that is, either ∼99% 12CH4 or ∼99% 13CH4 methane). The as-received 25 μm thick 99.999% pure Cu foil was annealed for ∼1 h at 960 °C in a 1:20 hydrogen/argon mixture with a pressure of ∼10 mbar. The growth of graphene was achieved by flowing 50 cm3 min−1 of CH4 over the annealed substrate while keeping the Ar/H2 flow, temperature and pressure constant. For the isotopically mixed sample with separated domains, the annealing and growth temperature was increased to 1,045 °C and the flow rate decreased to 2 cm3 min−1. After introducing 12CH4 for 2 min the carbon precursor flow was stopped for 10 s, and the other isotope precursor subsequently introduced into the chamber for another 2 min. This procedure was repeated with a flow time of 1 min. After the growth, the CH4 flow was interrupted and the heating turned off, while the Ar/H2 flow was kept unchanged until the substrate reached room temperature. The graphene was subsequently transferred onto a holey amorphous carbon film supported by a TEM grid using a direct transfer method without using polymer42.

Scanning transmission electron microscopy

Electron microscopy experiments were conducted using a Nion UltraSTEM100 scanning transmission electron microscope, operated between 80 and 100 kV in near-ultrahigh vacuum (2 × 10−7 Pa). The instrument was aligned for each voltage so that atomic resolution was achieved in all of the experiments. The beam current during the experiments varied between 8 and 80 pA depending on the voltage, corresponding to dose rates of ∼5–50 × 107 e−Å−2s−1. The beam convergence semiangle was 30 mrad and the semi-angular range of the medium-angle annular-dark-field detector was 60–200 mrad.

Poisson analysis

Assuming the displacement data are stochastic, the waiting times (or, equivalently, the doses) should arise from a Poisson process with mean λ. Thus the probability to find k events in a given time interval follows the Poisson distribution

graphic file with name ncomms13040-m23.jpg

To estimate the Poisson expectation value for each sample and voltage, the cumulative doses of each data set were divided into bins of width w (using one-level recursive approximate Wand binning43), and the number of bins with 0, 1, 2... occurrences were counted. The goodness of the fits was estimated by calculating the Cash C-statistic44 (in the asymptotically-χ2 formulation45) between a fitted Poisson distribution and the data:

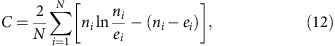graphic file with name ncomms13040-m24.jpg

where N is the number of occurence bins, ni is the number of events in bin i, and ei is the expected number of events in bin i from a Poisson process with mean λ.

An error estimate for the mean was calculated using the approximate confidence interval proposed for Poisson processes with small means and small sample sizes by Khamkong46:

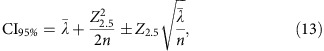graphic file with name ncomms13040-m25.jpg

where 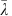Inline graphic is the estimated mean and Z2.5 is the normal distribution single tail cumulative probability corresponding to a confidence level of (100−α)=95%, equal to 1.96.

The statistical analyses were conducted using the Wolfram Mathematica software (version 10.5), and the Mathematica notebook is included as Supplementary Data 2. Outputs of the Poisson analyses for the main data sets of normal and heavy graphene as a function of voltage are additionally shown as Supplementary Fig. 2.

Displacement cross section

The energy transferred to an atomic nucleus from a fast electron as a function of the electron scattering angle θ is ref. 47

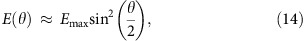graphic file with name ncomms13040-m27.jpg

which is valid also for a moving target nucleus for electron energies >10 keV as noted by Meyer and co-workers11. For purely elastic collisions (where the total kinetic energy is conserved), the maximum transferred energy Emax corresponds to electron backscattering, that is, θ=π. However, when the impacted atom is moving, Emax will also depend on its speed.

To calculate the cross section, we use the approximation of McKinley and Feshbach48 of the original series solution of Mott to the Dirac equation, which is very accurate for low-Z elements and sub-MeV beams. This gives the cross section as a function of the electron scattering angle as

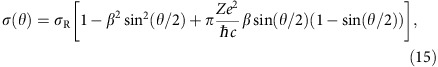graphic file with name ncomms13040-m28.jpg

where β=v/c is the ratio of electron speed to the speed of light (0.446225 for 60 keV electrons) and σR is the classical Rutherford scattering cross section

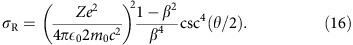graphic file with name ncomms13040-m29.jpg

Using equation 14 this can be rewritten as a function of the transferred energy49 as

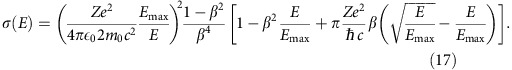graphic file with name ncomms13040-m30.jpg

Distribution of atomic vibrations

The maximum energy (in eV) that an electron with mass me and energy Ee=eU (corresponding to acceleration voltage U) can transfer to a nucleus of mass M that is moving with velocity v is

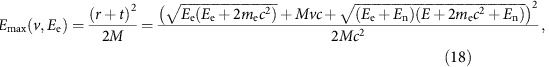graphic file with name ncomms13040-m31.jpg

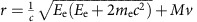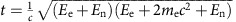where Inline graphic and Inline graphic are the relativistic energies of the electron and the nucleus, and En=Mv2/2 the initial kinetic energy of the nucleus in the direction of the electron beam.

The probability distribution of velocities of the target atoms in the direction parallel to the electron beam follows the normal distribution with a standard deviation equal to the temperature-dependent mean square velocity 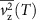Inline graphic,

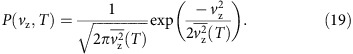graphic file with name ncomms13040-m35.jpg

Total cross section with vibrations

The cross section is calculated by numerically integrating equation 17 multiplied by the Gaussian velocity distribution (equation 19) over all velocities v where the maximum transferred energy (equation 18) exceeds the displacement threshold energy Td:

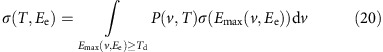graphic file with name ncomms13040-m36.jpg
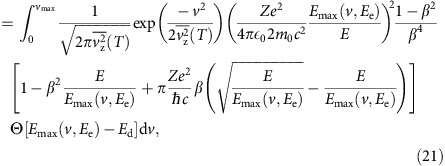graphic file with name ncomms13040-m37.jpg

where Emax(v, Ee) is given by equation 18, the term Θ[Emax(v, Ee)−Ed] is the Heaviside step function, T is the temperature and Ee is the electron kinetic energy. The upper limit for the numerical integration vmax=8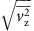Inline graphic was chosen so that the velocity distribution is fully sampled.

Displacement threshold simulation

For estimating the displacement threshold energy, we used DFT molecular dynamics as established in our previous studies12,13,50,51. The threshold was obtained by increasing the initial kinetic energy of a target atom until it escaped the structure during the molecular dynamics run. The calculations were performed using the grid-based projector-augmented wave code (GPAW), with the computational grid spacing set to 0.18 Å. The molecular dynamics calculations employed a double zeta linear combination of atomic orbitals basis52 for a 8 × 6 unit cell of 96 atoms, with a 5 × 5 × 1 Monkhorst-Pack k-point grid53 used to sample the Brillouin zone. A timestep of 0.1 fs was used for the Velocity-Verlet dynamics54, and the velocities of the atoms initialized by a Maxwell–Boltzmann distribution at 50 K, equilibrated for 20 timesteps before the simulated impact.

To describe exchange and correlation, we used the local density approximation41, and the Perdew-Burke-Ernzerhof (PBE)55, Perdew-Wang 1991 (PW91, ref. 41), RPBE56 and revPBE57 functionals, yielding displacement threshold energies of 23.13, 21.88, 21.87, 21.63 and 21.44 eV (these values are the means of the highest simulated kinetic energies that did not lead to an ejection and the lowest that did, respectively). Additionally, we tested the C09 (ref. 21) functional to see whether inclusion of the van der Waals interaction would affect the results. This does bring the calculated threshold energy down to [21.25, 21.375] eV, in better agreement with the experimental fit. However, a more precise algorithm for the numerical integration of the equations of motion, more advanced theoretical models for the interaction, or time-dependent DFT may be required to improve the accuracy of the simulations further.

Varying mean square velocity with concentration

Since the phonon dispersion of isotopically mixed graphene gives a slightly different out-of-plane mean square velocity for the atomic vibrations, for calculating the cross section for each concentration, we assumed the velocity of mixed concentration areas to be linearly proportional to the concentration

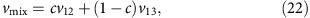graphic file with name ncomms13040-m39.jpg

where c is the concentration of 12C and v12/13 are the atomic velocities for normal and heavy graphene, respectively.

Raman spectroscopy

A Raman spectrometer (NT MDT Ntegra Spectra) equipped with a 532 nm excitation laser was used for Raman measurements. A computer-controlled stage allowed recording a Raman spectrum map over the precise hole on which the electron microscopy measurements were conducted, which was clearly identifiable from neighboring spot contamination and broken film holes.

The frequencies ω of the optical phonon modes vary with the atomic mass M as ω∝M−1/2 due to the mass prefactor of the dynamical matrix. This makes the Raman shifts of 13C graphene (12/13)−1/2 times smaller, allowing the mapping and localization of 12C and 13C domains28 with a spatial resolution limited by the size of the laser spot (nominally ∼400 nm). The shifts of the G and 2D bands compared with a corresponding normal graphene sample are given by 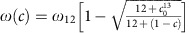Inline graphic, where ω12 is the G (2D) line frequency of the normal sample, c013=0.01109 is the natural abundance of 13C, and c is the unknown concentration of 12C in the measured spot.

Due to background signal arising from the carbon support film of the TEM grid, we analyzed the shift of the 2D band, where two peaks were in most locations present in the spectrum. However, in many spectra these did not correspond to either fully 12C or 13C graphene58, indicating isotope mixing within the Raman coherence length. To assign a single value to the 12C concentration for the overlay of Fig. 3, we took into account both the shifts of the peaks (to estimate the nominal concentration for each signal) and their areas (to estimate their relative abundances) as follows:

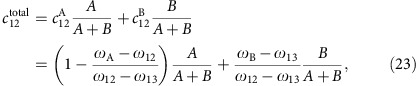graphic file with name ncomms13040-m41.jpg

where 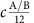Inline graphic are the nominal concentrations of 12C determined from the measured higher and lower 2D Raman shift peak positions, ωA/B are the measured peak centers of the higher and lower 2D signals, and A and B are their integrated intensities. The peak positions of fully 12C and 13C graphene were taken from the highest and lowest peak positions in the entire mapped area (covering several dozen Quantifoil holes), giving ω12=2,690 cm−1 and ω13=2,600 cm−1. The fitted 2D spectra, arranged in the same 6 × 6 grid as the overlay, can be found as Supplementary Fig. 3

Data availability

The full STEM time series data on which the determination of the 12C and 13C displacement cross sections (Fig. 2) are based are available on figshare with the identifier http://dx.doi.org/10.6084/m9.figshare.c.3311946 (ref. 20). The STEM data of Fig. 3 are available upon request. All other data are contained within the article and its Supplementary Information files.

Additional information

How to cite this article: Susi, T. et al. Isotope analysis in the transmission electron microscope. Nat. Commun. 7, 13040 doi: 10.1038/ncomms13040 (2016).

Supplementary Material

Supplementary Information

Supplementary Figures 1-3 and Supplementary Table 1

ncomms13040-s1.pdf (2.2MB, pdf)
Supplementary Data 1

The out-of-plane components of the graphene phonon density of states.

ncomms13040-s2.txt (195.3KB, txt)
Supplementary Data 2

A Wolfram Mathematica (version 10.5) notebook containing the dose data and its statistical analyses.

ncomms13040-s3.txt (940.5KB, txt)
Peer review file
ncomms13040-s4.pdf (487.8KB, pdf)

Acknowledgments

T.S. acknowledges funding from the Austrian Science Fund (FWF) via project P 28322-N36, and the computational resources of the Vienna Scientific Cluster. J.K. acknowledges funding from the Wiener Wissenschafts-, Forschungs- und Technologiefonds (WWTF) via project MA14-009. C.H., G.A., C.M. and J.C.M. acknowledge funding by the European Research Council Grant No. 336453-PICOMAT. T.J.P. was supported by the European Union’s Horizon 2020 research and innovation programme under the Marie Skłodowska-Curie grant agreement No. 655760-DIGIPHASE. We further thank Ondrej Krivanek for useful feedback.

T.S., J.C.M. and J.K. are named on a patent application relating to this method of isotope analysis (application number EP16183371). All other authors declare no competing financial interests.

Author contributions T.S. performed theoretical and statistical analyses and DFT simulations, participated in STEM experiments and their analysis, and drafted the manuscript. C.H. performed sample synthesis, and participated in STEM experiments, their analysis, and the Raman analysis. G.A. participated in sample synthesis and the Raman analysis. G.T.L. participated in STEM experiments and their analysis. C.M. and T.J.P. prepared special alignments for the STEM instrument with J.K., who supervised the theoretical and statistical analyses and STEM experiments. J.K. and J.C.M conceived and supervised the study.

08/30/2017

A correction has been published and is appended to both the HTML and PDF versions of this paper. The error has not been fixed in the paper.

References

  1. Geim A. K. & Novoselov K. S. The rise of graphene. Nat. Mater. 6, 183–191 (2007). [DOI] [PubMed] [Google Scholar]
  2. Nellist P. D. et al. Direct sub-Angstrom imaging of a crystal lattice. Science 305, 1741 (2004). [DOI] [PubMed] [Google Scholar]
  3. Krivanek O. L. et al. Atom-by-atom structural and chemical analysis by annular dark-field electron microscopy. Nature 464, 571–574 (2010). [DOI] [PubMed] [Google Scholar]
  4. Suenaga K. & Koshino M. Atom-by-atom spectroscopy at graphene edge. Nature 468, 1088–1090 (2010). [DOI] [PubMed] [Google Scholar]
  5. Krivanek O. L. et al. Vibrational spectroscopy in the electron microscope. Nature 514, 209–212 (2014). [DOI] [PubMed] [Google Scholar]
  6. Brown L. M. Proceedings of the institute of physics electron microscopy and analysis group conference, Cavendish Laboratory, University of Cambridge, 2–5 September 1997. In Rodenburg J. M. (ed.) Electron Microscopy and Analysis 1997., Vol. 191 of Conference Series Number 153 17–22 Institute of Physics Publishing Blackwell Science Ltd (1998). [Google Scholar]
  7. Lin Y.-C., Teng P.-Y., Chiu P.-W. & Suenaga K. Exploring the single atom spin state by electron spectroscopy. Phys. Rev. Lett. 115, 206803 (2015). [DOI] [PubMed] [Google Scholar]
  8. Zhou W. et al. Direct determination of the chemical bonding of individual impurities in graphene. Phys. Rev. Lett. 109, 206803 (2012). [DOI] [PubMed] [Google Scholar]
  9. Ramasse Q. M. et al. Probing the bonding and electronic structure of single atom dopants in graphene with electron energy loss spectroscopy. Nano Lett. 13, 4989–4995 (2013). [DOI] [PubMed] [Google Scholar]
  10. Kepaptsoglou D. et al. Electronic structure modification of ion implanted graphene: the spectroscopic signatures of p- and n-type doping. ACS Nano 9, 11398–11407 (2015). [DOI] [PubMed] [Google Scholar]
  11. Meyer J. C. et al. Accurate measurement of electron beam induced displacement cross sections for single-layer graphene. Phys. Rev. Lett. 108, 196102 (2012). [DOI] [PubMed] [Google Scholar]
  12. Susi T. et al. Silicon-carbon bond inversions driven by 60-keV electrons in graphene. Phys. Rev. Lett. 113, 115501 (2014). [DOI] [PubMed] [Google Scholar]
  13. Susi T. et al. Atomistic description of electron beam damage in nitrogen-doped graphene and single-walled carbon nanotubes. ACS Nano 6, 8837–8846 (2012). [DOI] [PubMed] [Google Scholar]
  14. Tewary V. K. & Yang B. Singular behavior of the Debye-Waller factor of graphene. Phys. Rev. B 79, 125416 (2009). [Google Scholar]
  15. Böttger H. Principles of the Theory of Lattice Dynamics Physica-Verlag Weinheim (1983). [Google Scholar]
  16. Mortensen J., Hansen L. & Jacobsen K. Real-space grid implementation of the projector augmented wave method. Phys. Rev. B 71, 035109 (2005). [Google Scholar]
  17. Enkovaara J. et al. Electronic structure calculations with GPAW: a real-space implementation of the projector augmented-wave method. J. Phys. Condens. Matter 22, 253202 (2010). [DOI] [PubMed] [Google Scholar]
  18. Wirtz L. & Rubio A. The phonon dispersion of graphite revisited. Solid State Commun. 131, 141–152 (2004). [Google Scholar]
  19. Mohr M. et al. Phonon dispersion of graphite by inelastic x-ray scattering. Phys. Rev. B 76, 035439 (2007). [Google Scholar]
  20. Susi T. et al. Atomic resolution electron irradiation time series of isotopically labeled monolayer graphene. figshare http://dx.doi.org/10.6084/m9.figshare.c.3311946 (2016).
  21. Cooper V. R. Van der waals density functional: an appropriate exchange functional. Phys. Rev. B 81, 161104 (2010). [Google Scholar]
  22. Egerton R., McLeod R., Wang F. & Malac M. Basic questions related to electron-induced sputtering in the TEM. Ultramicroscopy 110, 991–997 (2010). [Google Scholar]
  23. Egerton R. Control of radiation damage in the TEM. Ultramicroscopy 127, 100–108 (2013). [DOI] [PubMed] [Google Scholar]
  24. Brühwiler P. A. et al. π* and σ* excitons in C 1s absorption of graphite. Phys. Rev. Lett. 74, 614–617 (1995). [DOI] [PubMed] [Google Scholar]
  25. Yan H. et al. Damping pathways of mid-infrared plasmons in graphene nanostructures. Nat. Photon. 7, 394–399 (2013). [Google Scholar]
  26. Kang K., Abdula D., Cahill D. G. & Shim M. Lifetimes of optical phonons in graphene and graphite by time-resolved incoherent anti-Stokes Raman scattering. Phys. Rev. B 81, 165405 (2010). [Google Scholar]
  27. Robertson A. W. et al. Spatial control of defect creation in graphene at the nanoscale. Nat. Commun. 3, 1144 (2012). [DOI] [PubMed] [Google Scholar]
  28. Frank O., Kavan L. & Kalbac M. Carbon isotope labelling in graphene research. Nanoscale 6, 6363–6370 (2014). [DOI] [PubMed] [Google Scholar]
  29. Li X., Cai W., Colombo L. & Ruoff R. S. Evolution of graphene growth on Ni and Cu by carbon isotope labeling. Nano Lett. 9, 4268–4272 (2009). [DOI] [PubMed] [Google Scholar]
  30. Liu L. et al. Heteroepitaxial growth of two-dimensional hexagonal boron nitride templated by graphene edges. Science 343, 163–167 (2014). [DOI] [PubMed] [Google Scholar]
  31. Muccio Z. & Jackson G. P. Isotope ratio mass spectrometry. Analyst 134, 213–222 (2009). [DOI] [PubMed] [Google Scholar]
  32. Benninghoven A. Chemical analysis of inorganic and organic surfaces and thin films by static time-of-flight secondary ion mass spectrometry (TOF-SIMS). Angew. Chem. Int. Ed. (English) 33, 1023–1043 (1994). [Google Scholar]
  33. Stephan T. TOF-SIMS in cosmochemistry. Planet. Space Sci. 49, 859–906 (2001). [Google Scholar]
  34. Gault B., Moody M. P., Cairney J. M. & Ringer S. P. Atom Probe Microscopy Vol. 160, Springer Science & Business Media (2012). [Google Scholar]
  35. Lewis J. B., Isheim D., Floss C. & Seidman D. N. 12C/12C-ratio determination in nanodiamonds by atom-probe tomography. Ultramicroscopy 159, 248–254 (2015). [DOI] [PubMed] [Google Scholar]
  36. Baik S.-I. et al. An atomistic tomographic study of oxygen and hydrogen atoms and their molecules in CVD grown graphene. Small 11, 5968–5974 (2015). [DOI] [PubMed] [Google Scholar]
  37. Kelly T. F. & Miller M. K. Atom probe tomography. Rev. Sci. Instrum. 78, 031101 (2007). [DOI] [PubMed] [Google Scholar]
  38. Geim A. K. & Grigorieva I. V. Van der Waals heterostructures. Nature 499, 419–425 (2013). [DOI] [PubMed] [Google Scholar]
  39. Lovejoy T. et al. Energy-filtered high-angle dark field mapping of ultra-light elements. Microsc. Microanal. 20, 558–559 (2014). [Google Scholar]
  40. Argentero G., Mangler C., Kotakoski J., Eder F. & Meyer J. Towards weighing individual atoms by high-angle scattering of electrons. Ultramicroscopy 151, 23–30 (2015). [DOI] [PubMed] [Google Scholar]
  41. Perdew J. P. & Wang Y. Accurate and simple analytic representation of the electron-gas correlation energy. Phys. Rev. B 45, 13244–13249 (1992). [DOI] [PubMed] [Google Scholar]
  42. Regan W. et al. A direct transfer of layer-area graphene. Appl. Phys. Lett. 96, 113102 (2010). [Google Scholar]
  43. Wand M. P. Data-based choice of histogram bin width. Am. Statistician 51, 59–64 (1997). [Google Scholar]
  44. Cash W. Parameter estimation in astronomy through application of the likelihood ratio. Astrophys. J. 228, 939–947 (1979). [Google Scholar]
  45. Aschwanden M. J. & Schmahl E. RHESSI Team. Reconstruction of RHESSI solar flare images with a forward fitting method. In Lin R. P., Dennis B. R., Benz A. O. (eds) The Reuven Ramaty High-Energy Solar Spectroscopic Imager (RHESSI) 193–211Springer (2003). [Google Scholar]
  46. Khamkong M. Approximate confidence interval for the mean of poisson distribution. Open J. Stat. 02, 204–207 (2012). [Google Scholar]
  47. Mott N. F. & Massey H. The Theory of Atomic Collisions 3rd edn Clarendon Press (1965). [Google Scholar]
  48. McKinley W. A. Jr & Feshbach H. The Coulomb scattering of relativistic electrons by nuclei. Phys. Rev. 74, 1759–1763 (1948). [Google Scholar]
  49. Zobelli A., Gloter A., Ewels C. P., Seifert G. & Colliex C. Electron knock-on cross section of carbon and boron nitride nanotubes. Phys. Rev. B 75, 245402 (2007). [Google Scholar]
  50. Kotakoski J. et al. Stone-wales-type transformations in carbon nanostructures driven by electron irradiation. Phys. Rev. B 83, 245420 (2011). [Google Scholar]
  51. Kotakoski J., Santos-Cottin D. & Krasheninnikov A. V. Stability of graphene edges under electron beam: equilibrium energetics versus dynamic effects. ACS Nano 6, 671–676 (2012). [DOI] [PubMed] [Google Scholar]
  52. Larsen A. H., Vanin M., Mortensen J. J., Thygesen K. S. & Jacobsen K. W. Localized atomic basis set in the projector augmented wave method. Phys. Rev. B 80, 195112 (2009). [Google Scholar]
  53. Monkhorst H. J. & Pack J. D. Special points for Brillouin-zone integrations. Phys. Rev. B 13, 5188–5192 (1976). [Google Scholar]
  54. Swope W. C., Andersen H. C., Berens P. H. & Wilson K. R. A computer simulation method for the calculation of equilibrium constants for the formation of physical clusters of molecules: application to small water clusters. J. Chem. Phys. 76, 637–649 (1982). [Google Scholar]
  55. Perdew J. P., Burke K. & Ernzerhof M. Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996). [DOI] [PubMed] [Google Scholar]
  56. Hammer B., Hansen L. & Nørskov J. Improved adsorption energetics within density-functional theory using revised Perdew-Burke-Ernzerhof functionals. Phys. Rev. B 59, 7413 (1999). [Google Scholar]
  57. Zhang Y. & Yang W. Comment on ‘Generalized gradient approximation made simple’. Phys. Rev. Lett. 80, 890–890 (1998). [Google Scholar]
  58. Carvalho B. R. et al. Probing carbon isotope effects on the raman spectra of graphene with different 13C concentrations. Phys. Rev. B 92, 125406 (2015). [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Supplementary Information

Supplementary Figures 1-3 and Supplementary Table 1

ncomms13040-s1.pdf (2.2MB, pdf)
Supplementary Data 1

The out-of-plane components of the graphene phonon density of states.

ncomms13040-s2.txt (195.3KB, txt)
Supplementary Data 2

A Wolfram Mathematica (version 10.5) notebook containing the dose data and its statistical analyses.

ncomms13040-s3.txt (940.5KB, txt)
Peer review file
ncomms13040-s4.pdf (487.8KB, pdf)

Data Availability Statement

The full STEM time series data on which the determination of the 12C and 13C displacement cross sections (Fig. 2) are based are available on figshare with the identifier http://dx.doi.org/10.6084/m9.figshare.c.3311946 (ref. 20). The STEM data of Fig. 3 are available upon request. All other data are contained within the article and its Supplementary Information files.

Articles from Nature Communications are provided here courtesy of Nature Publishing Group

RESOURCES