Terahertz saturable absorbers from liquid phase exfoliation of graphite

Abstract

Saturable absorbers (SA) operating at terahertz (THz) frequencies can open new frontiers in the development of passively mode-locked THz micro-sources. Here we report the fabrication of THz SAs by transfer coating and inkjet printing single and few-layer graphene films prepared by liquid phase exfoliation of graphite. Open-aperture z-scan measurements with a 3.5 THz quantum cascade laser show a transparency modulation ∼80%, almost one order of magnitude larger than that reported to date at THz frequencies. Fourier-transform infrared spectroscopy provides evidence of intraband-controlled absorption bleaching. These results pave the way to the integration of graphene-based SA with electrically pumped THz semiconductor micro-sources, with prospects for applications where excitation of specific transitions on short time scales is essential, such as time-of-flight tomography, coherent manipulation of quantum systems, time-resolved spectroscopy of gases, complex molecules and cold samples and ultra-high speed communications, providing unprecedented compactness and resolution.

Graphene shows promise for saturable absorption, a key property for ultrafast lasing, yet graphene saturable absorbers operating in the terahertz region suffer from low absorption modulation. Here, the authors report terahertz saturable absorbers based on inkjet printed graphene with 80% transparency modulation.

Ultra-short laser pulses in the terahertz (THz) frequency range have great potential for information and communication technologies¹, security and spectroscopy applications², and as tools to probe light–matter interaction phenomena³ across the engineering, physical and biological sciences. Existing technologies for ultra-short THz pulse generation include the near-infrared ultrafast excitation of photoconductive switches⁴ and nonlinear crystals⁴, which provide broadband emission albeit with poor spectral bandwidth control and low output powers (∼μW)⁴, free electron lasers⁴ and large table-top optical ultrafast lasers⁴, which can provide intense (1 MV cm⁻¹ electric field) picosecond-pulses with controlled frequencies, but at the expense of a large footprint which hinders practical applications⁵. Quantum cascade lasers (QCLs) are solid state semiconductor sources with superior performances, in terms of compactness and spectral purity, with respect to any other technology for light generation at THz frequencies⁶, but presently do not operate passively in the ultra-short pulse regime. In addition to their Watt-range power⁷ and small intrinsic linewidth (100 Hz)⁸, their ‘bandstructure-by-design’⁶ allows one to tailor their frequency, bandwidth and pulse width independently⁶. It would thus be beneficial to develop techniques for generating ultra-short pulses directly from THz QCLs, such as passive mode-locking. This requires the development of appropriate saturable absorbers (SAs).

SAs operating in either transmission or reflection are routinely used to mode-lock lasers⁹, enabling a train of short pulses to be derived from continuous-wave operation. The key SA parameters include operation wavelength range⁹, dynamic response (recovery time)⁹ and saturation fluence (i.e., the pulse energy density required to achieve saturation)⁹.

Semiconductors can be used as SAs over a wide frequency range (from visible to mid-infrared, MIR)¹⁰,¹¹. Their recovery time and saturation fluence can be tuned by altering the growth parameters and/or the device geometry¹¹. However, due to the limited tuning range/bandwidth in reflection (tens nm)⁹ and the inherent free-carrier absorption losses in transmission¹², they are poorly suited for applications in the 1–10 THz range, hindering the corresponding development of passively mode-locked semiconductor lasers. In the THz range, the photon energy (4–50 meV) is smaller than any semiconductor band-gap and the free-carrier absorption, α_fr, significantly increases as a function of wavelength, following a square law wavelength-dependence (α_fr∼λ²) (ref. ⁶). Several n-doped semiconductors, such as GaAs, GaP and Ge, have been used as THz SAs, at electric fields of tens of kVcm⁻¹ (ref. ¹²). However, intra-cavity integration in the sub-wavelength (∼10–14 μm deep) cavities of available THz semiconductor lasers cannot be achieved without a significant increase of intra-cavity losses¹³. Although active mode-locking of THz QCLs has been achieved by exploiting injection seeding¹⁴, phase synchronization¹⁵, and by modulating the QCL driving current with an external RF synthesizer¹⁶, enabling the generation of laser pulses of a few ps duration¹⁴, passive mode-locking has not been achieved, due to the absence of suitable SAs in the THz region. As a further constraint, the inherently strong⁶,⁷,⁸ electron–phonon interaction in the QCL polar semiconductor gain medium leads to population inversion relaxation times as short as a few ps (refs ^{6, 14}).

Graphene¹⁷,¹⁸ and carbon nanotubes¹⁹ are potential SAs for ultrafast lasers. Carbon nanotubes can deliver broadband operation by exploiting a distribution of diameters¹⁹, while broadband operation is intrinsic to graphene¹⁷. This, along with its ultrafast recovery time²⁰, low saturation fluence¹⁷, and ease of fabrication²¹ and integration²²,²³, makes graphene a useful SA. Mode-locked lasers exploiting graphene SAs (GSAs) have been demonstrated at frequencies from the visible to the IR²⁴,²⁵,²⁶,²⁷,²⁸,²⁹. To the best of our knowledge, the only reported THz SA using multi-layer graphene grown on the carbon-face of silicon carbide³⁰ showed a maximum absorption modulation∼10%, too low to alter the intra-cavity field of existing THz QCLs³⁰. Furthermore, its intracavity integration within the 10–14 μm active region of THz semiconductor sources is hindered by the device architecture/material geometry⁶. It would, therefore, be desirable to develop a THz SA with a much higher absorption modulation, as well as one that can be deposited, as required, on any substrate, including on small (etched) trenches.

Liquid phase exfoliation (LPE) of graphite in a water/surfactant solution¹⁸,³¹ and organic solvents³¹,³²,³³ (for example, n-methylpyrrolidone; n-dimethylformamide; ortho-dichlorobenzene) is ideally suited for the mass-production of graphene. Graphene from LPE can be mixed or combined in dry or liquid form with a host polymer matrix, and can be used to produce both SAs¹⁸,³³ and printable inks³⁴,³⁵. Inkjet printing then allows for the deposition of films at low temperatures (<60 °C), with 1200 dpi resolution³⁶. GSA films operating at 1 μm have been made by vacuum filtration²⁶, while polymer composite GSAs have been demonstrated at 875 nm (ref. ²⁴), 1.5 μm (ref. ²³) and 2 μm (ref. ²⁷). Both inkjet printing and vacuum filtration are promising techniques to fabricate GSAs for QCLs, because they can be used to deposit films on a wide range of materials, ranging from Si wafers to flexible plastic substrates³⁴,³⁵. Inkjet printing also enables the deposition of films conforming to the substrate features³⁴.

To date, water-based inks prepared by LPE of graphite require post-processing to remove the surfactant/polymer³⁷, while the high boiling point of the resulting inks in n-methylpyrrolidone, n-dimethylformamide and ortho-dichlorobenzene hinders solvent removal by evaporation after the coating/printing process³⁸. Surfactants (for example, sodium deoxycholate, sodium cholate, Triton X-100) and solvents can have absorption coefficients larger than a few cm⁻¹ in the THz region³⁹, and may adversely affect the absorption of THz radiation in GSAs⁴⁰. Thus, surfactant-free and low boiling point (<80 °C) printable inks must be engineered in order to enable low-temperature (<100 °C) solvent removal.

Here, we use LPE of graphite to formulate both a water-based ink and also a surfactant-free, low boiling point, ethanol-based ink, and demonstrate THz saturable absorption from films produced by both vacuum filtration and inkjet printing. Through the combination of open-aperture z-scan experiments, transport analysis of field effect transistors (FETs) embedding these inks and Fourier transform infrared (FTIR) spectroscopy, we demonstrate 80% transparency modulation. This paves the way for the integration of graphene with existing sources to realize ultrafast, mode-locked lasers and passive ultrafast components across the THz frequency range.

Results

Materials and characterization techniques

The viscosity, η[mPa s], surface tension, γ[mJ m⁻²] and density, ρ[g cm⁻³] influence the jetting of individual drops from a nozzle⁴¹. A figure of merit, Z=(γρa)^1/2/η, can be used to characterize the drop formation⁴², and assess the jettability of an ink from a nozzle of diameter a (ref. ⁴¹). A range of 2<Z<24 has been identified as generally suitable for inkjet printing³³,⁴². Here we use a small 21 μm nozzle (Fujifilm DMC-11610), and formulate an ink with Z∼12. Low boiling point inks prepared from LPE of graphite previously exploited a two-solvent formulation⁴³,⁴⁴, where the mixture of water–isopropyl alcohol⁴³ or water-ethanol⁴⁴ is tuned to improve the affinity of the solvent to the exfoliated flakes. However, the different evaporation rate of the two solvents results in instabilities of η, γ and ρ (ref. ⁴⁵). Our approach exploits functionalized flakes in low boiling point solvents to formulate stable, surfactant-free, inkjet-printable inks. We print on Si/SiO₂ with a roughness Rq<0.3 nm and a water contact angle θ_c∼62°. To obtain films on a substrate with uniform flake distribution and morphology, we select a solvent with low contact angle (<40°) on SiO₂, such as ethanol. We also prepare a water-based ink³¹ for vacuum filtration.

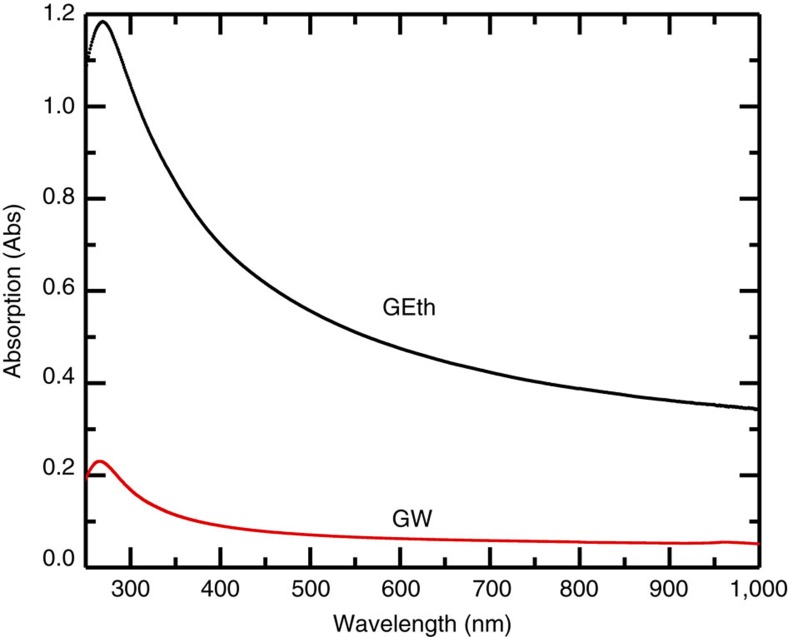
The water-based ink (GW) is prepared by ultrasonicating (Fisherbrand FB15069, Max power 800W) the graphite flakes (Sigma Aldrich Graphite) for 9 h in deionized water with sodium deoxycholate (SDC, 9 mg ml⁻¹)³¹. The dispersion is then ultracentrifuged (Sorvall WX100 mounting a TH-641 swinging bucket rotor) at 10 k r.p.m. for 1 h to remove thick (>10 nm) flakes. After ultracentrifugation, the top 70% of the dispersion is extracted and used for vacuum filtration and film transfer. We also prepare ethanol-based inks (GEth) by ultrasonicating (1 h) 5 mg ml⁻¹ flakes (Cambridge Nanosystems, GR1) produced by cracking methane and carbon dioxide in a plasma torch. The dispersion is then ultracentrifuged (Beckman Coulter Proteomelab XL-A, with a SW 32 Ti swinging bucket rotor) at 10 k r.p.m. for 1 h and the top 70% is collected for further characterization. Rheological measurements (viscosity, surface tension, density) give η_GW∼1.3 mPa s, γ_GW∼48 mN m⁻¹, ρ_GW∼0.8 g cm⁻³; η_GEth∼2.2 mPa s, γ_GEth∼30.7 mN m⁻¹, ρ_GEth∼0.98 g cm⁻³, consistent with previous reports³³,³⁴,³⁵,³⁶,³⁷,³⁸. Optical absorption spectroscopy is used to estimate the flakes concentration¹⁸,³¹,³² via the Beer–Lambert law, which correlates the absorbance A=αcl, with the beam path length l [m], the concentration c [g l⁻¹] and the absorption coefficient α [l g⁻¹ m⁻¹]. Figure 1 plots the absorption spectrum of GW and GEth inks diluted to 1:20 with water and ethanol respectively, to avoid possible scattering losses at higher concentrations. Using α∼1.390 l g⁻¹ m⁻¹ (ref. ³¹) and α∼2.460 l g⁻¹ m⁻¹ (ref. ¹⁸) at 660 nm for GW and GEth, respectively, we obtain c_GW∼0.1 mg ml⁻¹ and c_GEth∼0.36 mg ml⁻¹.

Figure 1. Absorption spectra.

Absorption of water-based (GW) and ethanol-based (GEth) inks from the visible to near-infrared range.

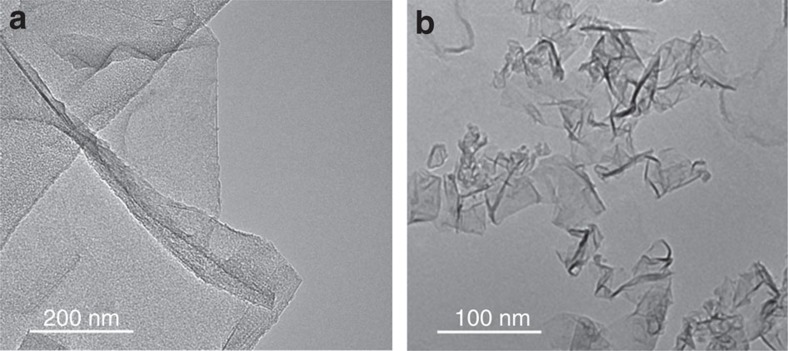
Figures 2a,b show high-resolution transmission electron microscopy (HRTEM) micrographs of a single-layer (SLG) and a few-layer graphene (FLG) flake from the GEth and GW inks, respectively. The associated HRTEM statistics³¹ from the GW ink shows ∼26% SLG, ∼22% bi- and ∼18% tri-layers with 150-300 nm average size. HRTEM statistics on the GEth flakes show ∼12% SLG, ∼30% bi- and ∼58% multi-layers, with ∼1 μm average size.

Figure 2. Transmission electron microscopy.

Transmission electron microscopy images of (a) single-layer graphene flakes from ethanol based (GEth) and (b) few-layer graphene flakes from water based inks (GW).

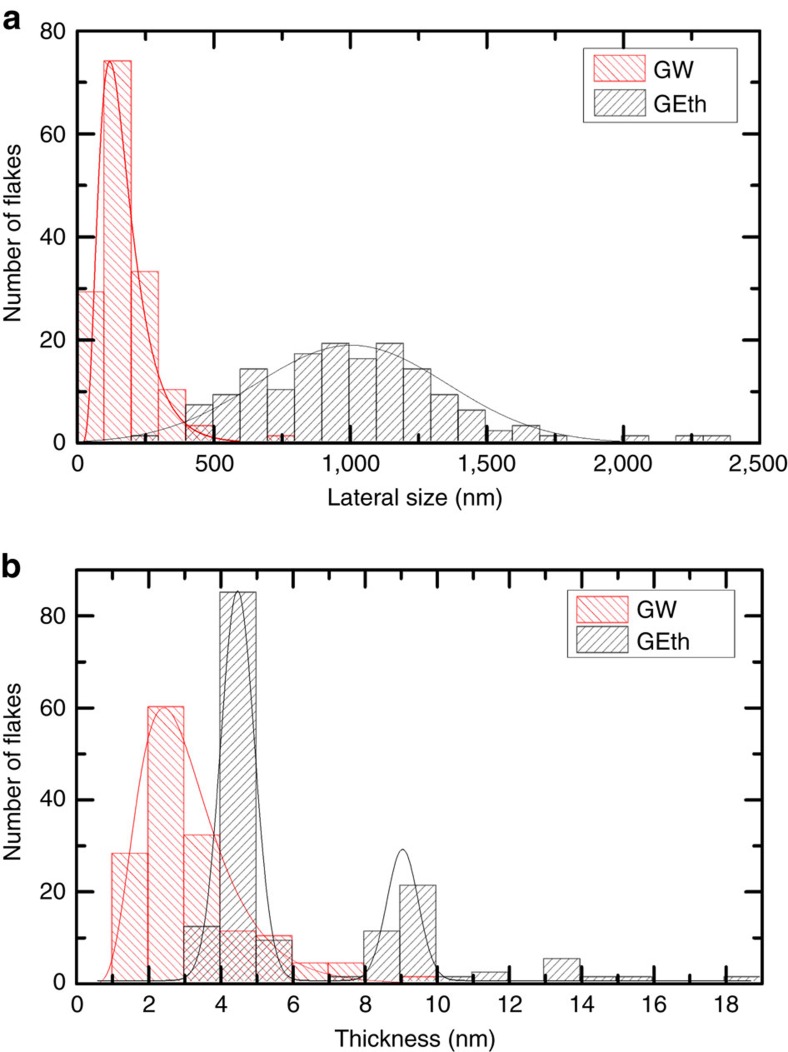
The average lateral size and thickness of the GW and GEth flakes are also estimated by atomic force microscopy (AFM). AFM statistics on the lateral size (Fig. 3a) show a log-normal distribution for the GW flakes peaked at 120 nm with a mean size ∼150 nm and a Gaussian distribution for GEth with a mean size ∼1.04 μm. The mean GW flake thickness is 2.8 nm (peaked at 2.5 nm) indicating that these are FLGs (<5 layers), while ∼57% of the GEth flakes are 4–5 nm thick (Fig. 3b) and ∼20% have higher thickness ∼9 nm.

Figure 3. Flakes distribution.

(a) Flake lateral size distribution and (b) thickness, for water-based (GW) and ethanol-based (GEth) inks.

GSAs are prepared as follows. The first, W-GSA, is made with the GW ink. Approximately 250 μl is vacuum filtered using 100 nm pore-size nitrocellulose filters. This blocks the flakes, while water passes through, leading to a film on the surface of the filter. This is then placed on an intrinsic Si/SiO₂ wafer and annealed at ∼80 °C for 2 h, to improve adhesion, followed by dissolution of the filter in acetone overnight. The resulting film is ∼65 nm thick, as determined by AFM. Following the procedure reported in ref. 27, we estimate a density∼0.72 g cm⁻³ for the W-GSA, derived from the weight (measured with a microbalance) of the filter before and after film deposition. This is ∼1/3 of the density of bulk graphite. Since the graphene flakes²⁷ in W-GSA films are, on average, stacked with the direction parallel to the SiO₂ surface, we can assume an interflake distance ∼3 times larger than that of graphite (0.33 nm), which results in ∼1 nm; thus the film thickness corresponds to an equivalent number of layers N∼65.

The second, Eth-GSA, is prepared by inkjet-printing (Fujifilm Dimatix, DMP-2800) the GEth ink on intrinsic Si/SiO₂ at an inter-drop spacing of 50 μm for 100 printing passes. The resulting thickness is ∼25 nm. In this case, once the droplets land on the substrate, they spread according to Young’s equation⁴⁶,⁴⁷ γ_SV−γ_SL−γ_LVcosθ_c=0, where γ_SV [mJ m⁻²] is the solid–vapour surface energy, γ_SL is the solid–liquid interfacial tension, and γ_LV is the liquid–vapour surface tension⁴⁸. When inkjet printing the GW ink, the high surface tension (γ_W∼47.8 mN m⁻¹) results in de-wetting (that is, isolated droplets caused by high θ_c). On the other hand, the lower surface tension of the GEth ink (γ_GEth∼30.7 mN m⁻¹) offers a lower θ_c, which causes the droplets to spread and join, enabling morphologically uniform films on Si/SiO₂. The boiling point of GEth (∼78 °C) and low surface tension (30.7 mN m⁻¹) minimize the effect of the cohesion forces responsible for the transport of material inside and around a coffee ring⁴⁹,⁵⁰.

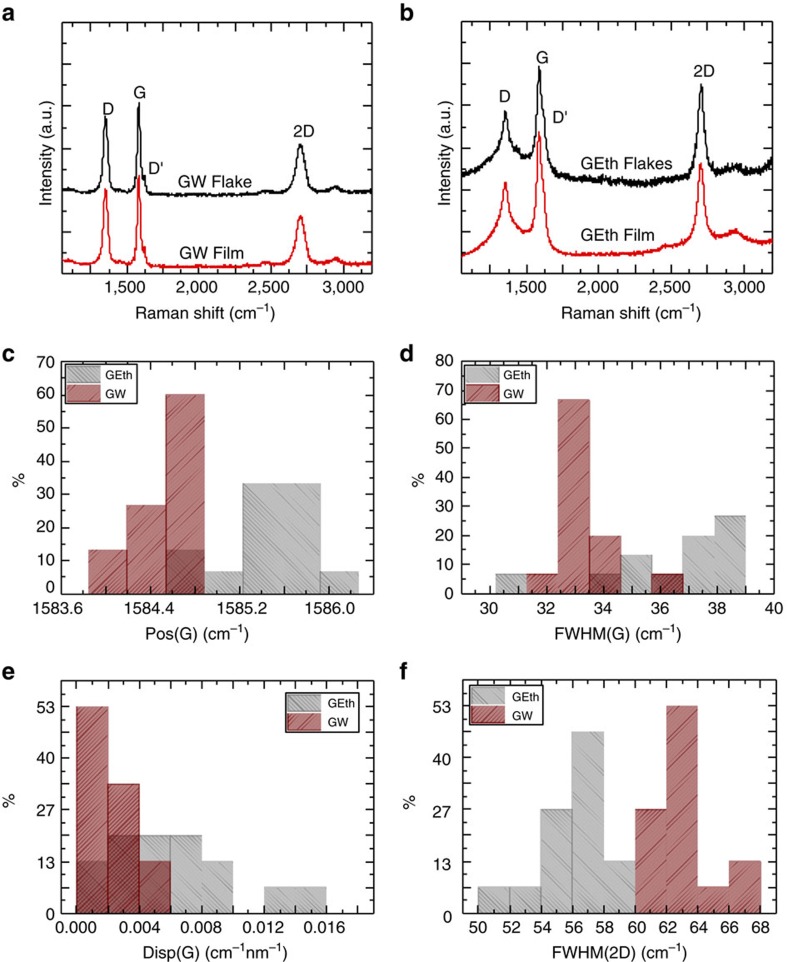
Raman spectroscopy is used to monitor the quality of the flakes at each step of the GSA preparation process for both inks (Fig. 4a,b). Raman spectra are acquired at 457, 514.5 and 633 nm with a Renishaw InVia with a × 50 objective (N.A.=0.85). The power on the sample is kept below 1 mW to avoid possible thermal damage. About 20 measurements are taken in different positions on each sample for each excitation wavelength. The G peak corresponds to the high frequency E_2g phonon at Γ, while the D peak, due to the breathing modes of six-atom rings, requires a defect for its activation⁵¹,⁵²,⁵³. This arises from the transverse optical phonons around the Brillouin zone edge of the K point⁵¹,⁵³, it is activated by a double resonance (DR) process⁵⁴, and is dispersive with the energy of excitation owing to a Kohn anomaly at the K point⁵⁵. Double resonance can also occur through an intra-valley process, that is, connecting two points belonging to the same cone around K or K′, which gives rise to the D′ peak. The 2D peak and the 2D′ peak are the second-order resonances of the D and D′ peaks, respectively. 2D and 2D′ are generated from the momentum conservation by two phonons having opposite wave vectors, meaning that for their activation no defects are required, and thus these modes are always active⁵⁶. The 2D peak is a single Lorentzian in SLG, whereas it splits into several components as the number of layers increases, reflecting the evolution of the electronic band structure⁵⁶.

Figure 4. Raman spectroscopy.

Raman spectra acquired at 514.5 nm of (a) GW flakes (black) and a GW film deposited on SiO₂/Si (red) and of (b) GEth flakes (black) and a GEth film (red). Statistics of (c) Pos(G), (d) FWHM(G), (e) Disp(G) and (f) FWHM(2D) for GEth (grey) and GW (red) films.

In disordered carbon, the position of the G peak, Pos(G) increases when the excitation wavelength λ_L decreases from the IR to UV⁵². Furthermore, the dispersion of the G peak, Disp(G)=ΔPos(G)/Δλ_L and the full-width-at-half-maximum of the G peak, FWHM(G) both increase with disorder⁵². The ratio of intensity of the D and G peaks, I(D)/I(G), analysed in combination with FWHM(G) and Disp(G), allows one to discriminate between disorder at the edges of the flakes and in the bulk, where a higher I(D)/I(G) implies a higher FWHM(G) and Disp(G). Figures 4a,b show that the film preparation process does not change the structural properties of the flakes significantly. On the other hand, Figs 4c–f indicate a difference between the GW-based and the GEth-based films, respectively. The GEth shows a more pronounced and wider D peak, as well as a wider G peak. This implies a more defective nature of these flakes. Furthermore, the higher Disp(G) and FWHM(G) indicate that, unlike the GW flakes, the defects are also spread within the flakes themselves. FWHM(2D) in Fig. 4f confirms that the GEth flakes mostly consist of multilayers. The quality of the GW flakes allows us to qualitatively estimate the Fermi energy (E_F≤250 meV) and, correspondingly, the doping (≤4 × 10¹² cm⁻²), by combining Pos(G) with the ratio of the 2D and G integrated areas, A(2D)/A(G) (refs ^{57, 58, 59}).

Optical experiments at THz frequencies

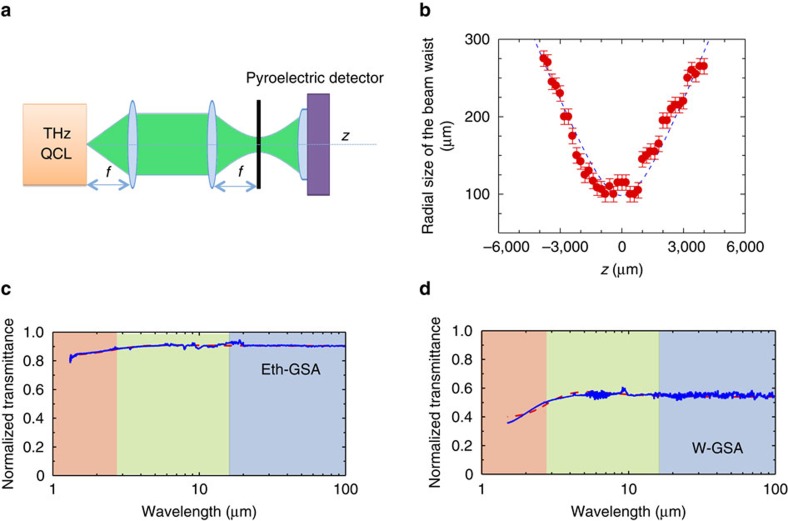
To investigate the THz-induced non-linear absorption properties of GSAs we use the open-aperture z-scan technique³⁰. The 3.5 THz radiation generated by a QCL (single-plasmon waveguide)⁷ is focused at normal incidence, by using two closely positioned convergent lenses with 3 cm focal length f (Fig. 5a).

Figure 5. Z-scan and FTIR measurements.

(a) Schematic diagram showing the open aperture z-scan setup; (b) beam waist (radial spot size) profile measured at different positions along the optical axis z, of the single-plasmon 3.5 THz QCL operating in pulsed-mode with a pulse width of 2 μs, a modulation frequency of 200 kHz, under an applied field of 7.6 kV cm⁻¹. The error bars represent the uncertainty associated with the measurement of the radius of the beam spot. The fitting function 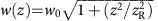allows to retrieve a radial spot size w₀∼95±6 mm. (c,d) FTIR transmittance of (c) Eth-GSA and (d) W-GSA normalized to the transmittance of the SiO₂/Si substrate, in the NIR, MIR and THz spectral ranges. The spectra collected separately in the three spectral ranges are plotted together. A white light source is employed in all cases combined with a PbSe detector (NIR) DTGS KBr detector (MIR) and DTGS-polyethylene detector (THz).

The samples are placed on a purpose-designed holder and translated along the optical axis (z) using a micrometric stage. A pyroelectric detector with a 7 mm² sensitive area is positioned at a fixed distance from the laser facet, behind the sample holder, to collect the transmitted radiation. The substrate transmittance is also measured and used to normalize the transmittance data. The QCL is operated in pulsed-mode, under different pulsed regimes. For W-GSA, we use a pulse width ∼2 μs and a modulation frequency 100 kHz, corresponding to a 20% duty cycle. For Eth-GSA, we repeat the experiment twice: under identical conditions as for W-GSA (20% duty cycle); and then by reducing the duty cycle, that is, with a pulse width of 1 μs and a modulation frequency 100 kHz, corresponding to 10% duty cycle, to investigate the sample behaviour under shorter pulses. In all cases, a superimposed additional square function modulation at 33 Hz and with 50% duty cycle is added to match the response of the pyroelectric detector.

To determine the QCL beam profile, we perform knife-edge⁶⁰ measurements at the focal point (red dots in Fig. 5b). We record the total power with the pyroelectric detector as the knife-edge is translated through the beam. From these experiments we extract the beam spot size, which has a characteristic Gaussian shape (fitting function, dashed line in Fig. 5b).

Taking into account the nearly circular Gaussian (x–y) spatial profile and the quasi-continuous wave driving regime of the QCL (so that the beam intensity can be time-independent), the dependence of the absorption coefficient on the pump intensity is expressed as:

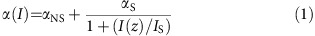where α_NS and α_S represent the non-saturable and saturable components of the linear absorption α₀=α(I=0)=α_NS+α_S, respectively, I(z) is the beam intensity along the optical axis, and I_S is the saturation intensity (that is, the intensity at which the saturable absorption is reduced by 50%). I(z) can be written as a function of the beam intensity at the focal point I₀, and of the Rayleigh length, z_R, which expresses the distance, along the propagation direction of a beam, from the waist to the place where the area of the cross section is doubled:

From the measurement of the beam profile (Fig. 5b), we extract a radial spot size w₀=95±6 μm, corresponding to z_R∼330 μm. We also measure at the focal point the average values I₀∼1.3 W cm⁻² for W-GSA, and I₀∼0.7 W cm⁻² and ∼1.3 W cm⁻² for Eth-GSA, due to the different pulsed operating regimes used for Eth-GSA.

FTIR spectroscopy can be used to determine the linear absorption α₀ in the THz, MIR and near-IR (NIR) range⁶¹. Spectra are acquired with deuterated triglycine sulfate (DTGS)-polyethylene, DTGS-KBr and PbSe detectors, optimized for THz, MIR and visible frequencies, respectively⁶.

The FTIR transmission spectra of Eth-GSA and W-GSA in the 3–230 THz range, normalized to the SiO₂/Si transmittance, are shown in Fig. 5c,d. These allow us to extract E_F, N and to evaluate the carrier scattering dynamics⁶¹.

The optical absorption can be written as a function of the sum of the interband conductivity σ_inter and the intraband conductivity σ_intra⁶¹:

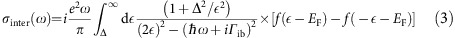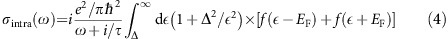where ω=2πν, ν is the frequency, f is the Fermi distribution, Г_ib describes the broadening of the interband transitions, and τ is the momentum relaxation time due to carrier intraband scattering. 2Δ represents the material bandgap and is set to zero⁶¹.

The optical transmission through N SLG layers on Si/SiO₂ normalized to the transmission through the reference Si/SiO₂ can be written as⁶¹:

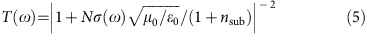where the Si/SiO₂ substrate refractive index, n_sub, is calculated by using the effective medium theory⁶² with a volume ratio (SiO₂ over Si) of 10⁻³, and using the values reported in literature for silica glass⁶³ and silicon in the 1–100 μm range.

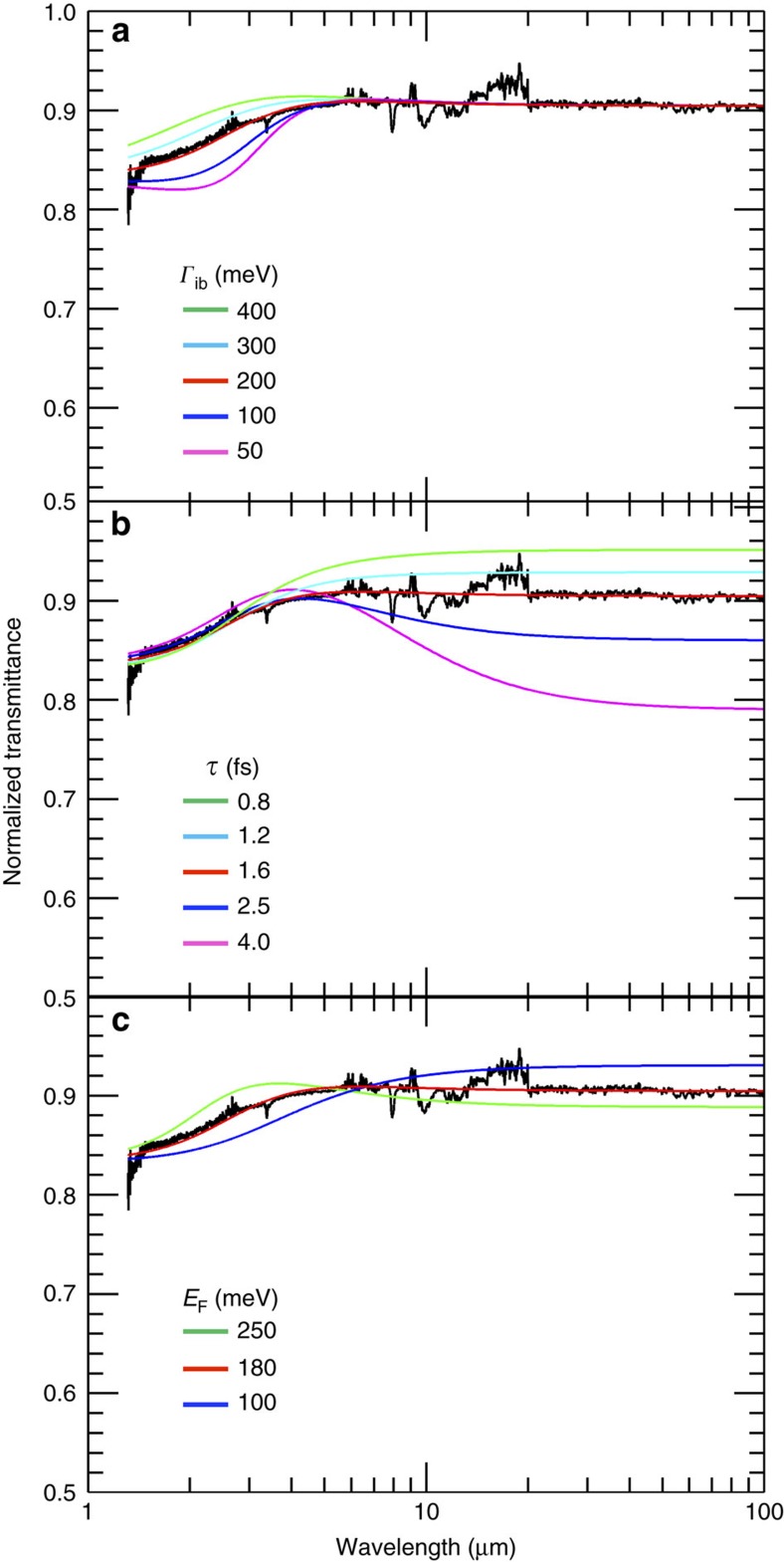
By combining equations (3)–(5), , and assuming that both interband and intraband processes are present, we get the dependence of the optical transmission on N, E_F, τ and Г_ib (ref. ⁶¹). Eth-GSA (Fig. 5c) shows an almost flat IR transmittance, which allows us to qualitatively evaluate N∼20 and E_F ∼180 meV via a set of iterative fitting procedures (see Methods and Fig. 6a–c).

Figure 6. Analysis of the FTIR spectra of ethanol-based graphene saturable absorber (Eth-GSA).

(a) Dependence on Γ_ib. FTIR transmission spectrum superimposed to fit function (Γ_ib=200 meV, red line) and a set of functions obtained by varying Γ_ib in the range 50–400 meV. (b) Dependence on τ. FTIR transmission spectrum superimposed to fit function (τ=1.64 fs, red line) and a set of functions obtained by varying τ in the range 0.8–4.0 fs. (c) Dependence on E_F. FTIR transmission spectrum superimposed to the fit function (E_F=181 meV, red line) and a set of functions obtained by varying E_F in the range 100–200 meV.

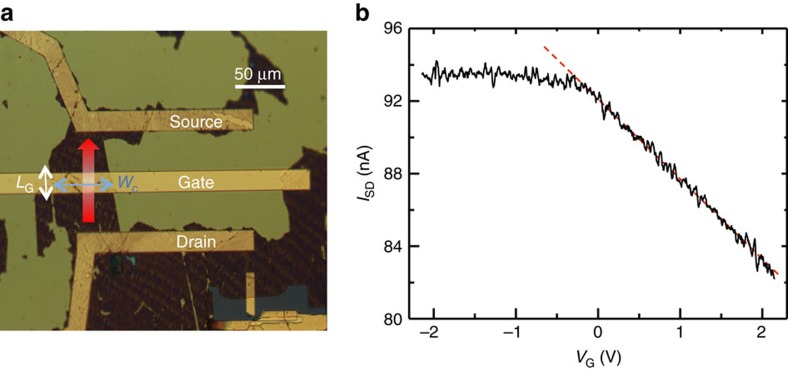
Conversely, the visible absorption cut-off in the transmission spectra of W-GSA (Fig. 5d) allows us to retrieve the above parameters via a more detailed procedure. First we estimate the density of free carriers in W-GSA by fabricating a top-gate FET having as active channel the W-GSA film (Fig. 7a) (see Methods). The source-drain distance is set to 100 μm, and the channel width W_C=60 μm, with the top-gate length L_G∼20 μm (Fig. 7a). We then perform room-temperature transport measurement and investigate the dependence of the source-to-drain current (I_SD) from the bias applied to the gate electrode (V_G), while keeping the source-to-drain bias (V_SD) equal to 1 mV. The extrapolated sample resistance is ∼11 kΩ at V_G=0 V. By increasing V_G, I_SD decreases linearly, unveiling a characteristic p-type FET behaviour, meaning that the density of majority carriers (holes) decreases (Fig. 7b). From the linear fit to the data (Fig. 7b, dashed line), we extrapolate the transconductance (g_m=4.4 nA V⁻¹) and the expected threshold voltage (V_th=20.5 V) for which the linear fit to the data intersects the source-drain current axis. The extrapolated V_th allows us to estimate the density of free carriers (n) at V_G=0 V, via the relation⁶⁴:

Figure 7. Field effect transistor and transport data.

(a) Optical microscope image of a top gate FET with a channel made by printing the water-based ink (W-GSA sample). (b) Dependence of the source-drain FET current (I_DS) from the gate bias V_G in the range from −2.2 V to +2.2 V, measured while a source-drain voltage V_SD=1 mV is applied. The dashed line represents the fit to the data in the linear region and gives a transconductance∼4.4 nA V⁻¹.

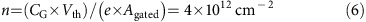where C_G=2.1 pF is the gate-to-channel capacitance (see Methods), e is the electric charge and A_gated is the gated area, calculated assuming that the full transistor channel is influenced by the gate potential. The extrapolated n approximately corresponds to E_F∼250 meV, which is consistent with the E_F estimated from Raman spectroscopy⁵⁶,⁵⁹. We then fit the transmission data of Fig. 5d by using equations (3)–(5), , and assuming E_F=250 meV as initial value for the iterative fitting procedure. The fitting curve, shown as a dashed line in Fig. 5d, gives N∼70 in good agreement with the AFM measurement, E_F∼210 meV, Г_ib∼130 meV and τ∼2 fs.

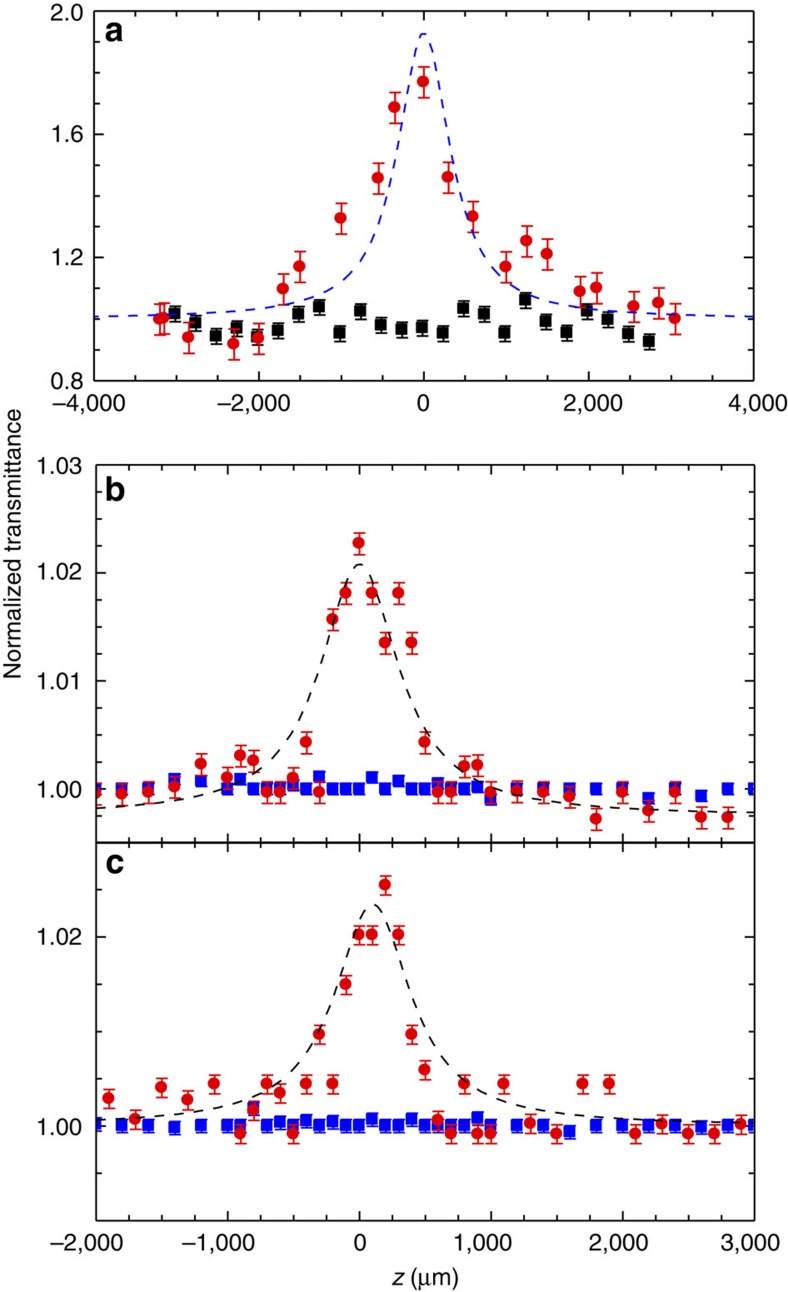
Figure 8a–c plots the z-scan transmission experiments. We detect an increase in transmission in all samples at z=0, where the z-scan trace is maximum, representing a SA signature. The transmission enhancement is significantly larger (80%) in W-GSA, compared with 2.5% in Eth-GSA. The transmission enhancement is constant (within 5%) in all investigated W-GSA and Eth-GSA samples. On the other hand, the Si/SiO₂ substrate shows no changes in transmission along the z-axis, confirming that the absorption bleaching is due to the films.

Figure 8. Transparency modulation.

(a–c) z-scan normalized transmittance traces of (a) water-based graphene saturable absorber (W-GSA) (dots) and (b,c) ethanol-based graphene saturable absorber (Eth-GSA) probed with a quantum cascade laser operating at (b) 10% duty cycle, and (c) 20% duty cycle, compared with the z-scan normalized transmittance traces of the Si/SiO₂ substrate (squares). The error bars correspond to the uncertainty interval on the measured normalized transmittance. The dashed lines are the fits with equation (7).

The normalized transmittance in the z-scan measurements can be written as⁶⁵:

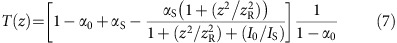By fitting the data in Fig. 8, we extract the saturable and non-saturable absorption coefficients, and the saturation intensity. For W-GSA, α_NS∼0.17±0.02, α_S∼0.68±0.1 and I_S=6.7±1 W cm⁻². For Eth-GSA: α_NS∼0.08±0.01, α_S∼0.17±0.02 when the QCL operates at a 10% duty cycle, and α_NS∼0.07±0.01, α_S=0.19±0.02 for a 40% duty cycle, showing the negligible role of the device operating conditions. In both cases, the extracted lower saturable absorption values for Eth-GSA reflect the different material quality, in agreement with Raman measurements. The corresponding saturation intensities for Eth-GSA are I_S=2.9±0.7 W cm⁻² (10% QCL duty cycle) and I_S=3.3±0.7 W cm⁻² (20% QCL duty cycle). In both W-GSA and Eth-GSA the saturation fluence reaches several μJ cm⁻². The maximum α_S in W-GSA is 68%, while α_NS<17% on average. The α_S coefficient is the largest reported to date for any spectral range¹⁷,²⁵,²⁸. The saturation intensity values are in agreement with what achieved in the far-IR³⁰ in presence of optical pulses much longer (2 μs) than the demarcation time scale set by the carrier-phonon scattering time (∼150 fs as derived by pump-probe)²⁰,⁶⁶. Under the above experimental conditions charge carrier distribution develops into quasi-equilibrium within the optical pulse duration.

Typically, non-saturable losses originate from scattering at the interfaces between the layers⁶⁷, free carrier absorption³⁰ or defects, which depend on the quality of the fabrication techniques. At visible wavelengths, the dominant non-saturable loss is due to scattering losses at the layer interfaces⁶⁷, unavoidable even in case of defect-free graphene⁶⁷. Alternative loss dynamics may, however, dominate under specific experimental conditions. For THz excitation, owing to the long wavelength, the multi-interface effect makes only a minor contribution. Additionally, when graphene is doped, non-saturable absorption can be linked to either free carrier absorption or to the reflectivity from the sheets, since in this regime the optical conductivity is well described by the Drude model. Here, at low pumping photon energies (THz frequencies), the optical absorption in graphene is dominated by intraband transitions⁶⁸. Thus, the dynamics of our THz GSA is governed by ultrafast intraband dynamic.

Discussion

The versatility offered by printable graphene films acting as fast SAs paves the way for new classes of photonic devices in the THz range, with specifically designed device functionalities. The 80% transparency modulation, combined with the flexibility offered by graphene films and inks, can allow, for example, the intra-cavity embedding of SAs in existing THz sources, providing a unique capability for mode-locked and compact THz sources. It will also enable the implementation of ultrafast modulators, filters and mirrors with impacts in the THz frequency range.

Methods

Rheological characterization

The surface tension is measured using the pendent drop method (First Ten Angstroms FTA1000B). The shape of the drop results from the relation between surface tension and gravity. The surface tension is then calculated from the shadow image of a pendant drop using drop shape analysis. The contact angle is measured by dispensing 1 μl of ultrapure DI water on the substrates and measuring the angle at which the ink interface meets the solid surface. A parallel plate rotational rheometer (DHR rheometer TA instruments (GW ink) and Bohlin C-VOR Rheometer (GEth ink)) is used to evaluate the viscosity as a function of shear rate, the infinite-rate viscosity is found for both the GEth and GW inks.

Transmission electron microscopy

Drops of both inks are dispensed on holey carbon TEM grids for HRTEM analysis, using a Tecnai T20 high-resolution electron microscope with an acceleration voltage of 200 kV operating in Bright Field mode.

Atomic force microscopy

A Bruker Dimension Icon working in peakforce mode is used. From the centrifuged dispersion four samples are collected and, after 20 times dilution, they are drop cast onto pre-cleaned (with acetone and isopropanol) Si/SiO₂ substrate. Each sample is scanned across three different areas. For each material, 150 flakes are counted.

Vacuum filtration transfer

∼1 ml GW ink is diluted with DI water at a ratio of 1:9 and is passed through a nitrocellulose membrane (100 nm pore size), hastened with the use of a Büchner flask attached to a vacuum pump. The filtered film is then washed with DI water in order to remove residual surfactant. The flakes on the membrane are then rinsed in DI water for 5 min and transferred onto an intrinsic Si/SiO₂ substrate. After oven annealing (∼80 °C) the sample is placed in an acetone bath to dissolve the nitrocellulose membrane.

Transistor fabrication

A top-gate FET is fabricated using W-GSA. Source and drain electrodes, shaped as stripes 100 μm distant from each other, are defined via a combination of electron beam lithography and thermal evaporation of a 5/100 nm sequence of Cr/Au. This geometry defines a channel having a100 μm length and an average width of 60 μm, set by the shape of the selected portion of graphene.

A high-k dielectric (HfO₂, ε_r=13–19)⁶⁹ oxide layer is then placed over the whole transistor plane via atomic layer deposition (ALD). In order to guarantee a good electrical isolation a thickness of 100 nm is chosen for this layer.

The gate electrode is designed as a rectangular stripe having a width W_G=20 μm. It is lithographically patterned across the central part of the FET channel with a length (120 μm) suitable to cover the whole channel. The gate to channel capacitance is with a finite element method (COMSOL Multiphysics). We get C_G∼2.0–3.0 pF.

Fourier infrared spectroscopy analysis of Eth-GSA samples

Figure 6a plots the FTIR transmission spectrum superimposed to the fit achieved by keeping N, E_F and τ as fixed parameters and by varying Γ_ib in the 50–400 meV range. The fitting curve (red curve in Fig. 6a) that well matches the experimental data corresponds to Г_ib=200 meV and gives: N∼19, E_F∼181 meV, corresponding to n∼1.5 × 10¹² cm⁻², τ∼1.6 fs. Figure 6b interpolates the data by exploiting the same fit function in which N, E_F and Г_ib are fixed parameters. τ is varied in the range 0.8–4.0 fs. The fitting curve (red curve in Fig. 6b) corresponds to τ=1.6 fs and gives: N∼19, E_F∼181 meV and Г_ib∼200 meV. Finally we investigate the influence of E_F on the fit. We keep N, τ and Г_ib as fixed parameters and vary E_F in the 100–200 meV range, obtaining: N∼19, Г_ib∼200 meV and τ∼1.6 fs with E_F=180 meV.

Data availability

The data that support the findings of this study are available from the corresponding author on request.

Additional information

How to cite this article: Bianchi, V. et al. Terahertz saturable absorbers from liquid phase exfoliation of graphite. Nat. Commun. 8, 15763 doi: 10.1038/ncomms15763 (2017).

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.