4-Aminoquinaldine monohydrate polymorphism: Prediction and impurity aided discovery of a difficult to access stable form

Abstract

Crystal structure prediction studies indicated the existence of an unknown high density monohydrate structure (Hy1_B°) as global energy minimum for 4-aminoquinaldine (4-AQ). We thus performed an interdisciplinary experimental and computational study elucidating the crystal structures, solid form inter-relationships, kinetic and thermodynamic stabilities of the stable anhydrate (AH I°), the kinetic monohydrate (Hy1_A) and this novel monohydrate polymorph (Hy1_B°) of 4-AQ. The crystal structure of Hy1_B° was determined by combining laboratory powder X-ray diffraction data and ab initio calculations. Dehydration studies with differential scanning calorimetry and solubility measurements confirmed the result of the lattice energy calculations, which identified Hy1_B° as the thermodynamically most stable hydrate form. At 25 °C the equilibrium of the 4-AQ hydrate/anhydrate system was observed at an a_w (water activity) of 0.14. The finding of Hy1_B° was complicated by the fact that the metastable but kinetically stable Hy1_A shows a higher nucleation and growth rate. The presence of an impurity in an available 4-AQ sample facilitated the nucleation of Hy1_B°, whose crystallisation is favored under hydrothermal conditions. The value of combining experimental with theoretical studies in hydrate screening and characterisation, as well as the reasons for hydrate formation in 4-AQ, are discussed.

1. Introduction

The objective of solid form screenings of biologically active substances (drugs, agrochemicals), that are manufactured to solid dosage forms, is to find as many solid forms as possible (polymorphs, hydrates, solvates, amorphous phase) and to identify the most suitable form(s) for further development.^1,2 However, no matter how extensive a screening programme may be, utilising either high throughput methodologies^3–6 or other widely used (manual) screening strategies,^7–10 there is no guarantee that all possible forms are found. The most critical and key parameters that influence the occurrence of solid forms are temperature, pressure, moisture, the nature of the solvent of crystallisation (including water activity) and supersaturation. Generally, a polymorph screen is performed by crystallising the compound from solvents/solvent mixtures¹¹ and by applying as many different conditions as possible, e.g. varying the rate of cooling, crystallisation temperature, (reverse) anti-solvent addition, solvent evaporation or liquid assisted grinding experiments. Furthermore, crystallisation from the melt,^12,13 sublimation,¹⁴ moisture sorption/desorption experiments, systematic desolvation (dehydration) studies^15,16 and pressure crystallisation^17–19 are successful ways to new crystal forms. Another tool for expanding polymorphism screens is the use of seeds or additives to induce the formation of new crystalline forms by heterogeneous nucleation (e.g. templating, isomorphic seeding).^20–22

Tailor made additives of structurally related impurities can significantly modify the nucleation/growth process of crystal forms.^23–25 An additive can inhibit nucleation by being incorporated into pronuclear clusters and aggregates or by binding to nuclei/crystals. While one polymorph may be affected by the additives, another polymorph may remain unaffected.²⁶ Similarly, the addition of an additive can inhibit the crystal growth by attaching to certain face(s) of crystals. Such an inhibition may block/reduce the formation of one polymorph but favour another form, whose growth rate is less or not affected by the additive.^27,28,29 Examples for successfully stabilising metastable forms due to (by-product) impurities have been demonstrated for substances such as sulfathiazole,³⁰ L-glutamic acid,²⁵ aspirin,³¹ and progesterone²².

As it is impossible to cover the entire experimental space that may lead to different solid forms computational methods ensuring that the most relevant forms have been found are in high demand. As yet, several multi-disciplinary solid form screening studies have been performed successfully, complementing experimental screens with crystal structure prediction (CSP) studies.^4,5,16,32,33 These studies provide a deeper understanding of the solid form landscape of each compound at the molecular level. Computationally generated crystal energy landscapes of creatine³⁴ and carbamazepine²¹ even directed experimental investigations to the finding of new polymorphs.

However, many fine chemicals, in particular pharmaceuticals, exist in both hydrated and anhydrous forms. The stability and behaviour of hydrates can vary significantly,^35,36 and the transformation routes between hydrate and anhydrate forms have to be studied extensively^37–44 in order to avoid problems during manufacturing and storage and to ensure the high quality standards of drug products. The dehydration and rehydration processes of a hydrate forming system can be rather complex^37,41,43, in particular when the compound forms multiple phases. A hydrate can be the most physically stable form under relevant production and storage conditions whereby the vapour pressure of water in the ambience (relative humidity, RH) is a main key parameter beside temperature and pressure. It is well known that solvent adducts, including hydrates, often crystallise more easily than solvent-free forms, because water/small solvent molecules, as the hydrogen bonding sites of an organic molecule are frequently better satisfied in the presence of a water molecule, which often compensates the lack of hydrogen bond donors or acceptors of a molecular compound.⁴⁵ However, the formation and stability of hydrates is still somewhat unpredictable, despite increasing computational efforts in this field, including CSP.

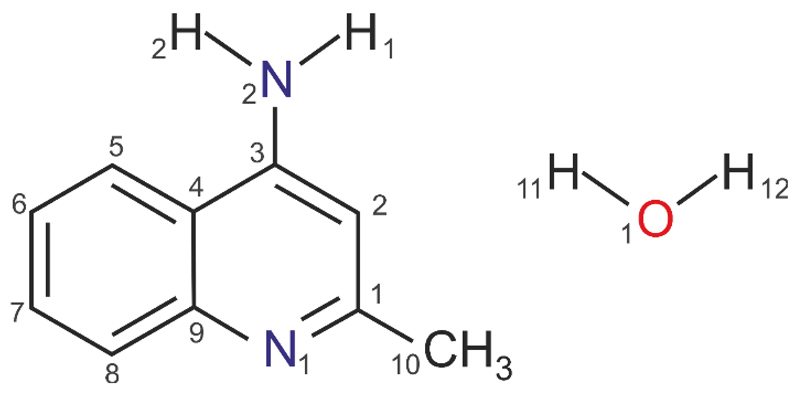
In seeking to improve our understanding of hydrate formation phenomena in organic (drug) compounds we are expanding our set of organic model hydrate systems^{34,42,46–48} with 4-aminoquinaldine (4-amino-2-methylquinoline, 4-AQ, Fig. 1). We previously investigated the solid form landscape of this compound and its intriguing structural features, ranging from void structure with and without solvent to closed packed structures.⁴⁹ The experimental screen had resulted in three polymorphs (AH I°, II and III), the monohydrate Hy1_A (Cambridge Structural Database⁵⁰ Refcode: LOBSOL⁵¹) and a carbon tetrachloride solvate. The computationally generated anhydrate crystal energy landscape allowed us to unravel the complex interplay between close packing and number of strong (hydrogen bonding) interactions in the solid state of 4-AQ. This was found to be in contrast to the known monohydrate (Hy1_A), which adopts a dense crystal packing arrangement and exhibits the maximum number of strong hydrogen bonding interactions.

Fig. 1.

Molecular diagram of 4-amino-2-methylquinoline (4-aminoquinaldine, 4-AQ) monohydrate.

In the present study we complement the 4-AQ solid state picture⁴⁹ with the computationally generated monohydrate crystal energy landscape. Surprisingly, the crystal energy landscape indicates that Hy1_A is not the global energy minimum hydrate but another monohydrate, which was not observed in the polymorph screen. Thus, we decided to expand our previous state-of-the art manual polymorphism screen. Guided by the CSP results we not only found the thermodynamically most stable monohydrate polymorph, but also developed a consistent picture of the structural, kinetic and thermodynamic features and inter-relationships of 4-AQ anhydrates and monohydrates. This recognition required a broad range of analytical techniques, including thermal analysis, X-ray diffraction, gravimetric moisture sorption/desorption studies, spectroscopic methods, water activity and solubility measurements, in combination with computational chemistry.

2. Experimental Section

2.1. Computational Generation of the Monohydrate Crystal Energy Landscape

The planar conformational energy minimum of 4-AQ was used in the CSP searches. 300,000 Z'=1 monohydrate structures were generated using CrystalPredictor2.0^52–54 in 48 common space groups for organic molecules (ESI† Section 1.1). The molecules were held rigid and the lattice energy was evaluated by an exp-6 potential with atomic charges derived using the CHELPG scheme⁵⁵ and minimized. All crystallographically distinct low energy crystal structures were used as starting points for optimising the intermolecular lattice energy (U_inter), with an improved model for the intermolecular forces. This was calculated using the FIT⁵⁶ exp-6 potential parameters and the distributed m+ultipoles⁵⁷ derived from the PBE0/6-31G(d,p) charge density using GDMA2.⁵⁸

The optimal proton positions of the amino group (i.e. pyramidal, deviation from planarity) and methyl group, in all crystal structures within 15 kJ mol^–1 of the global minimum (40 structures), were determined using the CrystalOptimizer database method.⁵⁹ This was done by minimising the lattice energy (E_latt), calculated as the sum of the intermolecular contribution (U_inter) and the conformational energy penalty paid for distortion of the molecular geometry to improve the hydrogen bonding geometries. Conformational energy penalties (∆E_intra, with respect to the pyramidal global conformational energy minimum) and isolated molecule charge densities were computed at the PBE0/6-31G(d,p) level, for each conformation considered in the minimisation of E_latt. All isolated-molecule wave function calculations were performed using GAUSSIAN09⁶⁰ and intermolecular lattice energies using DMACRYS.⁶¹

The 12 most stable structures (within 12 kJ mol^–1 of the global minimum) were used as starting points for periodic electronic structure calculations. The DFT-D calculations were carried out with the CASTEP plane wave code⁶² using the Perdew-Burke-Ernzerhof (PBE) generalized gradient approximation (GGA) exchange-correlation density functional⁶³ and ultrasoft pseudopotentials,⁶⁴ with the addition of a semi-empirical dispersion correction, either the Tkatchenko and Scheffler (TS)⁶⁵ or Grimme06 (G06)⁶⁶ model. For more details see ESI† Section 1.2.

PIXEL calculations^67–69 were also performed on the 12 lowest energy structures to estimate the repulsive (E_R), dispersion (E_D), electrostatic (Coulombic, E_C) and polarisation (also called induction, E_P) contributions from individual pairs of molecules within a crystal. The charge density for the crystal was constructed from the MP2/6-31G(d,p) ab initio charge density of the isolated molecule as extracted from the computed PBE-TS crystal structures. The electron density was described using medium cube settings and a step size of 0.08 Å, with the pixels condensed into superpixels with a condensation level n=4.

2.2. Materials and Solid Form Screen

Two commercial 4-AQ samples were used for the investigations, purchased from Aldrich (Lot# STBD1705V, purity 98%) and Fluka (Lot# 248847, purity 98%). The samples consist of AH I° (Aldrich) and Hy1_A (Fluka). The 29 solvents used for the polymorphism screen were all of analytical quality and all organic solvents were purchased from Aldrich or Fluka.

The solid form screen repeated the experiments presented in ref. 49 using as educts the commercial samples without purification. Solvent evaporation, cooling crystallisation, anti-solvent addition and liquid assisted grinding experiments were employed for the solvent screening and are detailed in ESI† Sections 2.2 and 2.3.

2.3. Powder X-ray Diffractometry

PXRD patterns were obtained using an X’Pert PRO diffractometer (PANalytical, Almelo, NL) equipped with a theta/theta coupled goniometer in transmission geometry, programmable XYZ stage with well plate holder, Cu-Kα_1,2 radiation source with a focussing mirror, a 0.5° divergence slit, a 0.02° Soller slit collimator on the incident beam side, a 2 mm antiscattering slit, a 0.02° Soller slit collimator on the diffracted beam side and a solid state PIXcel detector. The patterns were recorded at a tube voltage of 40 kV and tube current of 40 mA, applying a step size of 2θ= 0.013° with 80s per step in the 2θ range between 2° and 40°. For non-ambient RH measurements a VGI stage (VGI 2000M, Middlesex, UK) was used.

Powder X-ray diffraction data, used for determining the Hy1_B° structure, were collected on a STOE STADI MP diffractometer using strictly monochromatic Cu-Kα₁ radiation (λ = 1.54056 Å) from a focusing Ge(111) primary beam monochromator and a Mythen1k detector with 11° detection range. Measurements were taken in bisecting transmission geometry at ambient conditions, with a sample of 2 mm diameter placed between two zero-scattering foils. Data were collected in the range from 5 to 120° 2θ with a step size of 0.009°.

The model was refined, using the computationally generated global minimum structure as starting point, by the Rietveld method employing the FULLPROF.2000 program⁷⁰ and soft constraints. The background was modeled by a set of consecutive points with refineable intensities. The final refinement included a total of 61 parameters (16 profile, 4 cell, 1 scale, 1 isotropic temperature factor, 39 (non H) positions (using 24 soft distance constrains, 13 soft angle constrains) yielding a final R_wp= 5.84 (Fig. 2). For further details see §.

Fig. 2.

Rietveld refinement from PXRD data. Experimental intensities (points), calculated intensities (line), difference plot (line at the bottom). Green vertical bars indicate the positions of the Bragg peaks.

The resulting structure from the Rietveld refinement was further scrutinized by allowing all fractional coordinates to refine freely (61 parameters, R_wp = 4.64). As expected, the improvement in reliability factors came at the expense of some chemical sense (e.g. bond lengths), but otherwise, the geometry of the molecule was well preserved, confirming the correctness of restrained refined crystal structure.§

2.4. Thermal Analysis

For hot-stage thermomicroscopic (HSM) investigations a Reichert Thermovar polarisation microscope, equipped with a Kofler hot-stage (Reichert, A), was used. Photographs were taken with an Olympus DP71 digital camera (Olympus, D).

Differential Scanning Calorimetry (DSC) thermograms were recorded on a DSC 7 (Perkin-Elmer Norwalk, Ct., USA) controlled by the Pyris 2.0 software. Using a UM3 ultramicrobalance (Mettler, Greifensee, Switzerland), samples of approximately 2 - 20 mg were weighed into perforated or sealed aluminium pans or high pressure capsules. The samples were heated using rates in between 2 and 20 °C min^–1 and cooled using rates in between 0.1 to 5 °C min^–1 with dry nitrogen as the purge gas (purge: 20 mL min^–1). The instrument was calibrated for temperature with pure benzophenone (mp 48.0 °C) and caffeine (236.2 °C), and the energy calibration was performed with indium (mp 156.6 °C, heat of fusion 28.45 J g^–1). The errors on the stated temperatures (extrapolated onset temperatures) and enthalpy values were calculated at the 95% confidence intervals (CI) and are based on at least five measurements.

Thermogravimetric Analysis (TGA) was carried out with a TGA7 system (Perkin-Elmer, Norwalk, CT, USA) using the Pyris 2.0 Software. Approximately 2-5 mg of sample was weighed into a platinum pan. Two-point calibration of the temperature was performed with ferromagnetic materials (Alumel and Ni, Curie-point standards, Perkin-Elmer). A heating rate of 5 °C min^–1 was applied and dry nitrogen was used as a purge gas (sample purge: 20 mL min^–1, balance purge: 40 mL min^–1).

2.5. Karl Fischer Titration

The water content in 4-AQ samples was determined using a Karl Fischer coulometric titrator C20 instrument (Mettler Toledo, CH).

2.6. Gravimetric Moisture Sorption/Desorption Experiments

Moisture sorption and desorption studies were performed with the automatic multisample gravimetric moisture sorption analyser SPS23-10µ (ProUmid, Ulm, D). Approximately 150 - 200 mg of sample was used for each analysis. The measurement cycles were started at 40% with an initial stepwise desorption (decreasing humidity) to 0%, followed by a sorption cycle (increasing humidity) up to 90% and back to 0% relative humidity (RH). RH changes were set to 10% for all cycles. Between 0 and 10% RH an additional step at 5% RH was added. The equilibrium condition for each step was set to a mass constancy of ± 0.001 % over 60 minutes and a maximum time limit of 48 hours per step.

2.7. Water Activity Measurements

Excess of 4-AQ AH I°⁴⁹ was stirred (500 r.p.m.) in 1.5 – 2.5 mL of each methanol and water mixture (each containing a different mole fraction of water corresponding to a defined water activity^45,46 (ESI† Section 2.5) at 25.0 ± 0.1 °C for 14 days. Samples were withdrawn, filtered and the resulting phase was determined using PXRD and TGA.

2.8. Determination of Solubility

The Crystal16 crystallisation system (Avantium, NL) was used to determine the kinetic solubilities of Hy1_A and Hy1_B° in water. The temperature at the point the suspension becomes a clear solution upon heating or “clear point” (at 0.1 °C per minute) was taken as the saturation temperature of the measured sample with known concentration. To make sure that solvent-mediated transformations had not occurred during the measurements excess solid was stirred under the same conditions and PXRD patterns of the residual solid were recorded after reaching the highest clear point temperature derived from the solubility experiments.

2.9. Gas Chromatography-Mass Spectrometry (GC-MS)

The GC-MS system consisted of a HP7890 GC device with a HP5975C inert XL mass-selective detector (Agilent Technologies, Palo Alto, CA, USA). A DB-XLB column (30 m x 0.25 mm i.d. x 0.25 µm film thickness, J&W Scientific, Folsom, CA) was used for chromatographic separation. Carrier gas was helium with a flow rate of 1.0 mL min^–1. 1.0 µg mL^–1 solutions of SA and F in methanol were used as samples. Injection volume was 2 µL (splitless), injection temperature was 250 °C. The temperature program was as follows: 50 °C, hold 1 min; increase to 150 °C with 25 °C min^–1, to 320 °C with 10 °C min^–1, hold for 8 min and to 330 °C in 20 °C min^–1, hold for 7.5 min. MS was done in electron impact mode (70 eV) scanning from 50 to 800. Mass spectral data were recorded on a personal computer with the HP MS ChemStation software G1034C version D01.00 (Agilent Technologies).

3. Results

3.1. Computational Screening for 4-AQ Monohydrates

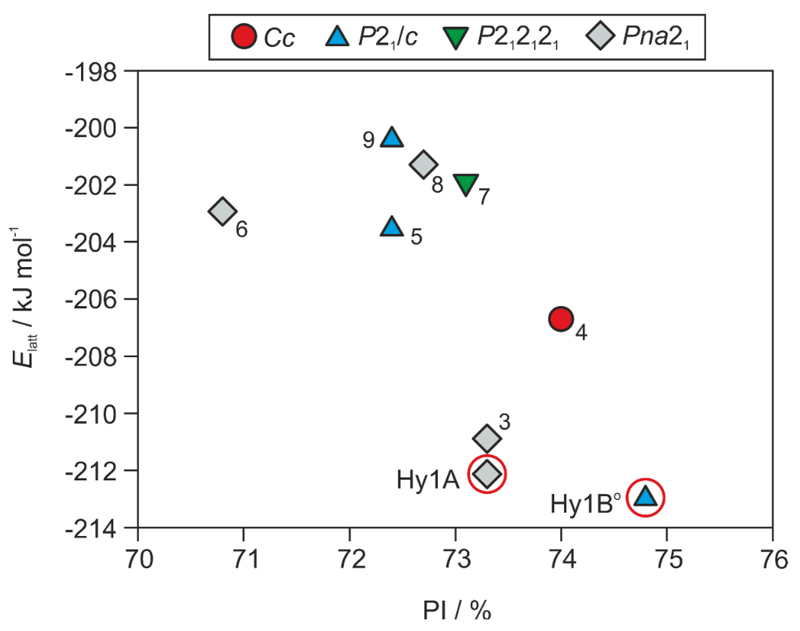
The computationally generated 4-AQ monohydrate crystal energy landscape (Fig. 3) has several thermodynamically feasible structures within the energy range expected for polymorphic forms.⁷¹ Three structures were found within three kJ mol^–1 of the global monohydrate minimum and six within the ten kJ mol^–1 range. The structure of the known monohydrate (LOBSOL,⁵¹ Hy1_A) corresponds to the second most stable structure in Fig. 3 and was estimated to be 0.7 kJ mol^–1 less stable than the PBE-G06 global minimum. The computational results suggest that Hy1_A may not be the only 4-AQ monohydrate and that a more stable hydrate phase could exist. Thus, the previously performed experimental solid form screen⁴⁹ may not have been exhaustive enough, and did not include conditions, which facilitate the formation of one or more further hydrate(s). We therefore started with analysing the computed lowest-energy structures in more depth, contrasting the packings and hydrogen bonding motifs to the experimental forms and expanding the experimental search space for 4-AQ solid forms (Section 3.2).

Fig. 3.

Crystal energy landscape for 4-AQ monohydrates, classified by space group. PI - Kitaigorodskii type of packing index, calculated using PLATON.⁷² Each symbol denotes a crystal structure. Experimental structures are encircled and labelled as Hy1_A (LOBSOL⁵¹) and Hy1_B°. Arabic numbers are used for the most stable hypothetical structures and the numbers correspond to their stability rank (ESI† Table S2).

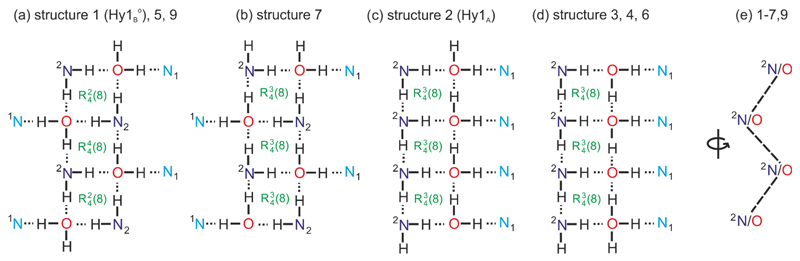
The most stable computed monohydrate structures are all densely packed, the packing indices range 70.8% to 74.8%. All 4-AQ molecules are essentially planar, with the amino group adopting a (slightly) pyramidal conformation. The polar protons deviate from the plane formed by the aromatic ring system optimising the geometry of the hydrogen bonding interactions. In all of the most stable computationally generated hydrate structures (Fig. 3), except structure 8, the maximal number of possible strong hydrogen bonds are formed. All four hydrogen bonding donor groups are used, forming N–H⋯O, N–H⋯N, O–H⋯N or O–H⋯O interactions (Fig. 4). The amino protons were found to interact either with the water oxygen atom or with the amino nitrogen atom (N2, Fig. 1). One water proton hydrogen bonds to the pyridine nitrogen (N1) and the second to either the amino or the water oxygen atom. The eight structures schematically depicted in Fig. 4 form tetrameric ring motifs with the graph set⁷³ notations: ${\mathbf{\text{R}}}_{\mathbf{4}}^{\mathbf{2}}(\mathbf{8})$, ${\mathbf{\text{R}}}_{\mathbf{4}}^{\mathbf{4}}(\mathbf{8})$ and ${\mathbf{\text{R}}}_{\mathbf{4}}^{\mathbf{3}}(\mathbf{8})$. The ring motifs form one dimensional chains propagating in a zig-zag fashion (Fig. 4e) along the shortest cell axis (ESI† Table S2). The O–H⋯N1 hydrogen bonds link the molecules into three-dimensional network structures. In addition to the strong hydrogen bonds also π⋯π stacking of the aromatic rings was found to contribute significantly to the stability of the eight structures (ESI† Section 1.5).

Fig. 4.

Hydrogen bonding motifs observed in the lowest computationally generated monohydrate structures (Fig. 3). Structure numbers correspond to energy rank. Nitrogen atoms are labelled according to Fig. 1.

The hydrogen bonding motifs shown in Fig.4a & b, as well as Fig. 4c & d, differ solely in the position/connectivity of proton atoms. Structures Hy1_A and 3 were found to be isostructural apart from hydrogen atom positions. Thus, the two structures might either “coexist” as a proton disorder structure or due to energetic preference structure 3 might convert to Hy1_A through proton transfer. The latter appears more likely as the Hy1_A structure solution gave no evidence for proton disorder.

The lowest energy structure forming only three strong hydrogen bonds is structure 8 and was calculated to be 11.5 kJ mol^–1 less stable than the global minimum. The global minimum structure is denser packed, more stable (at 0K) and structurally distinct from the known monohydrate. Therefore this structure must be regarded as an alternative monohydrate polymorph that is likely to be producible experimentally. The predicted structure 8 lacks similarity to the packing arrangements of the other hydrates, and since the calculated energy is high, we assumed that it is rather unlikely to produce this phase experimentally.

3.2. Extended Experimental Screen for 4-AQ (Mono)Hydrate Polymorphs

3.2.1. Moisture Dependent Hydration Studies

The initial experimental screening,⁴⁹ performed with purified 4-AQ, was extended with gravimetric moisture sorption/desorption studies using different 4-AQ batches as obtained from the supplier and the purified material. This included an over 20 years old sample (Fluka, Hy1_A, purity ~98%) and a second, recently purchased sample (Sigma Aldrich, AH I°, purity ˜98%). The Fluka sample (F, hereafter) had been stored under ambient conditions in a light proofed container and was yellowish in colour, whereas the Sigma Aldrich sample (SA) was a white powder, with a similar appearance as batches of 4-AQ that have been purified by solvent crystallisation (ESI† Section 2.1.).

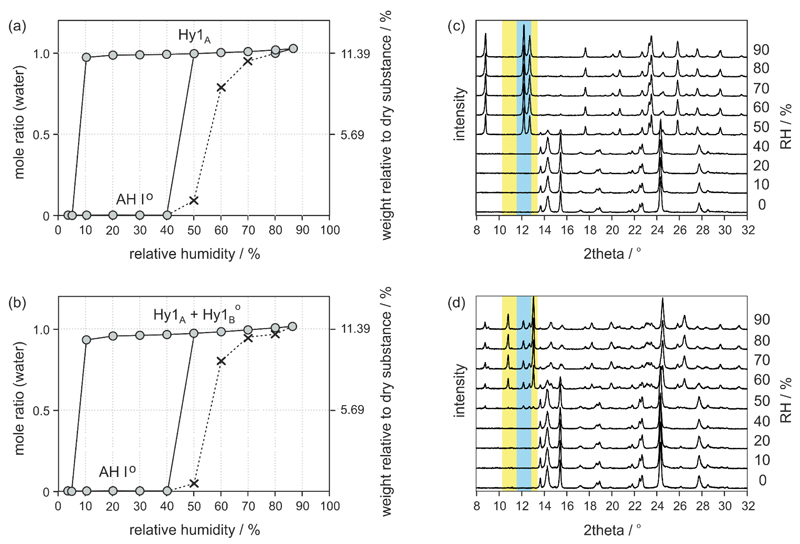
Moisture sorption/desorption studies using the SA and F samples are given in Fig. 5a&b. The equilibrium isotherms show distinct steps with plateaus and also a marked hysteresis between the sorption and the desorption cycle, which is a clear indication for a pronounced structural difference between the hydrated and anhydrous phase(s) and for the categorisation of 4-AQ as stoichiometric hydrate system. The sorption isotherms of the two investigated samples show that AH I° is stable (no water uptake) at and below 40% RH. At moisture conditions above 40% RH the samples take up water forming a hydrate with one mole water per mole 4-AQ. Above 40% RH the SA sample as well as its recrystallisation product (moisture sorption isotherm not shown) resulted in phase pure Hy1_A, whereas for the F sample a mixture of Hy1_A and a second phase was identified with PXRD and IR spectroscopy. The new phase was expected to be a second monohydrate polymorph, finally named Hy1_B°§§.

Fig. 5.

(a,b) Gravimetric moisture sorption and desorption curves of 4-AQ at 25 °C: (a) starting with sample SA and (b) starting with sample F (both AH I°). The grey circles represent data points reaching the equilibrium (constant mass) within the pre-set maximal equilibration time (see experimental section), whereas crosses and dashed lines mark data points where the sample did not reach the equilibrium moisture content within the allowed time limit of 48 h. (c) Moisture dependent PXRD measurements showing the hydration process of 4-AQ sample SA (AH I° to Hy1_A) and (d) sample F (AH I° to a mixture of Hy1_A and Hy1_B°). The angular range of characteristic reflections of Hy1_A are marked in blue whereas a yellow colour highlights the range of distinct Hy1_B° reflections. Due to different equilibration times and other parameters such as sample amount, dynamics of the atmosphere etc. the hydration kinetics in the gravimetric moisture chamber (SPS) is different to kinetics in the moisture stage (VGI) used for the PXRD recordings.

The desorption cycles show that the transformation of the monohydrate(s) to AH I° occurs only at very dry conditions (5% RH and below), indicating that the two hydrates are stable under conventional storage conditions. In contrast AH I° (thermodynamically most stable anhydrate polymorph in the entire temperature range⁴⁹) transforms to the monohydrate(s) already under usual indoor climate conditions (25°C, 40 – 60 % RH), suggesting that the hydrated forms should be used if (some) moisture cannot be avoided.

The structural changes upon hydration were monitored with moisture controlled PXRD experiments (Fig. 5C, D). To minimise particle size depending effects on hydration kinetics the samples were sieved (150 μm mesh). The two AH I° samples did not show any changes upon increasing the RH from 0 to 40% RH (Fig. 5C, D). On exceeding this moisture condition sample SA Transforms to Hy1_A whereas sample F shows reflections of Hy1_A and the second monohydrate Hy1_B°.

3.2.2. Solvent Screen using Different 4-AQ Batches/Purities

Driven by the finding of the new Hy1_B° We repeated the experimental screen for solid forms⁴⁹ using the two 4-AQ batches (SA and F) as obtained from the supplier.

Evaporation, cooling crystallisation, precipitation or liquid assisted grinding experiments with the original (un-purified) SA sample (ESI† Section 2.2) did not result in other forms than those observed in a previous screening programme.⁴⁹ This is also true for the F sample except for a few crystallisations, which resulted in a mixture of Hy1_A and Hy1_B° (ESI† Tables S9): fast and slow cooling crystallisation experiments from n-propanol or methyl acetate, whereas slow crystallisation experiments led to higher proportions of the new phase compared to fast crystallisation. Hy1_B° was also formed in slow cooling crystallisation experiments from acetonitrile or dichloromethane, concomitantly with Hy1_A and AH I°. TGA and coulometric Karl Fischer titration experiments of the n-propanol or methyl acetate samples indicated, despite being a mixture, the stoichiometry of a monohydrate. Differences in optical appearance of the purified or original SA (white powder) and F batches (yellowish powder) as well as the fact that the new phase was exclusively obtained with the F sample suggested that the impurity profiles of SA and F must be different and have an influence on the crystallisation/nucleation process. GC-MS (Fig. 6) and ESI-MS (ESI† Section 2.1.2) confirmed that the SA and F samples contain different chemical impurities in various quantities. Two 4-AQ derivatives could be identified as impurities: impurity 1 was identified as chloro-substituted 4-AQ (M_r = 192) and impurity 2 is presumably a carboxaldehyde-derivative of 4-AQ or N-(2-methyl-4-quinolinyl)-formamide (M_r = 186). For more information see ESI† Section 2.1. Further investigations focussing on the impact of the identified impurities on the nucleation of 4-AQ forms are planned.

Fig. 6.

GC-MS impurity screening in 4-AQ samples SA and F.

3.2.3. Crystallisation under Hydrostatic Conditions

CSP indicated that Hy1_B° is the most stable monohydrate structure at 0K with the highest packing index (Fig. 3). This result suggests that elevated pressure may favour the formation of this hydrate. To verify this suggestion, AH I° (sample SA) was suspended in an excess of water (about 15 mg in roughly 7 mg of water) and heated to 125 °C in an hermetically sealed (high pressure) DSC pan. Subsequently the sample was slowly cooled to 25 °C (0.1 K min^–1, in the sealed pan) and the phase identity was tested with PXRD. This hydrothermal method resulted in Hy1_B° confirming our suggestion. The crystallisation of the hydrate on cooling occurred in the temperature range between 82 and 86 °C. Increasing the water ratio lowers the crystallisation temperature range (77 to 82 °C) and more importantly results in the metastable hydrate Hy1_A. Thus, it is obvious that pressure favours the formation of the stable monohydrate Hy1_B° besides certain impurities which obviously induce the nucleation of this hydrate during the hydration process of the anhydrate at elevated moisture conditions.

3.3. Crystal Structures of the Monohydrates

The structure of the metastable Hy1_A, determined by Tai et al. (LOBSOL),⁵¹ is contrasted to the new polymorph of this monohydrate which was solved form laboratory PXRD data.

3.3.1. Monohydrate Hy1_A

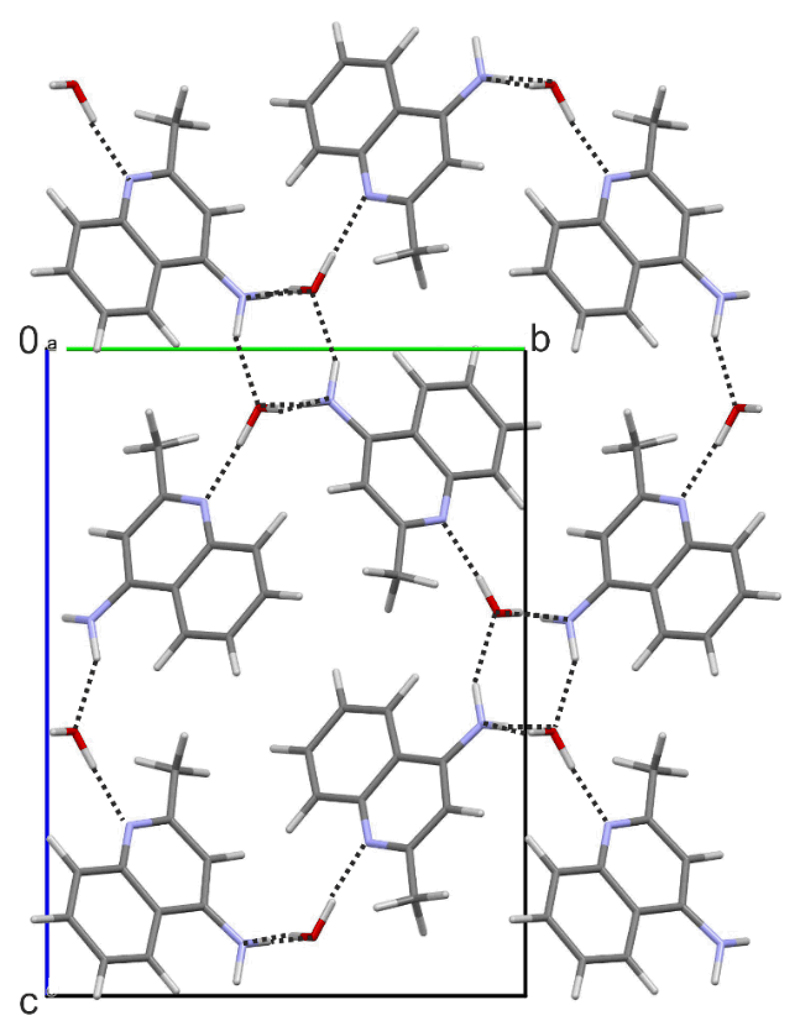
The hydrate crystallises in the orthorhombic space group Pna2₁ with each one 4-AQ and one water molecule in the asymmetric unit. The planar 4-AQ molecule forms three hydrogen bonding interactions (Fig. 4a), one N2–H1⋯N2, involving an adjacent 4-AQ molecule, and two 4-AQ⋯water interactions (N2–H2⋯Ow and Ow–H⋯N1, respectively). A forth strong H-bond is formed between water molecules, leading to a chain of water molecules propagating along [100]. A further characteristics of the structure are π⋯π stacks and C–H⋯π interactions of 4-AQ molecules. The water molecules are located in channels surrounded by the polar groups of the 4-AQ molecule and show a tetragonal coordination (Fig. 7). Assumable, the dehydration mechanism starts with the removal of the water through the [100] channels (ESI† Section 1.6).

Fig. 7.

Packing diagram of Hy1_A, viewed along the crystallographic [100] axis. Hydrogen bonding interactions are indicated with dotted lines.

3.3.2. Monohydrate Hy1_B°

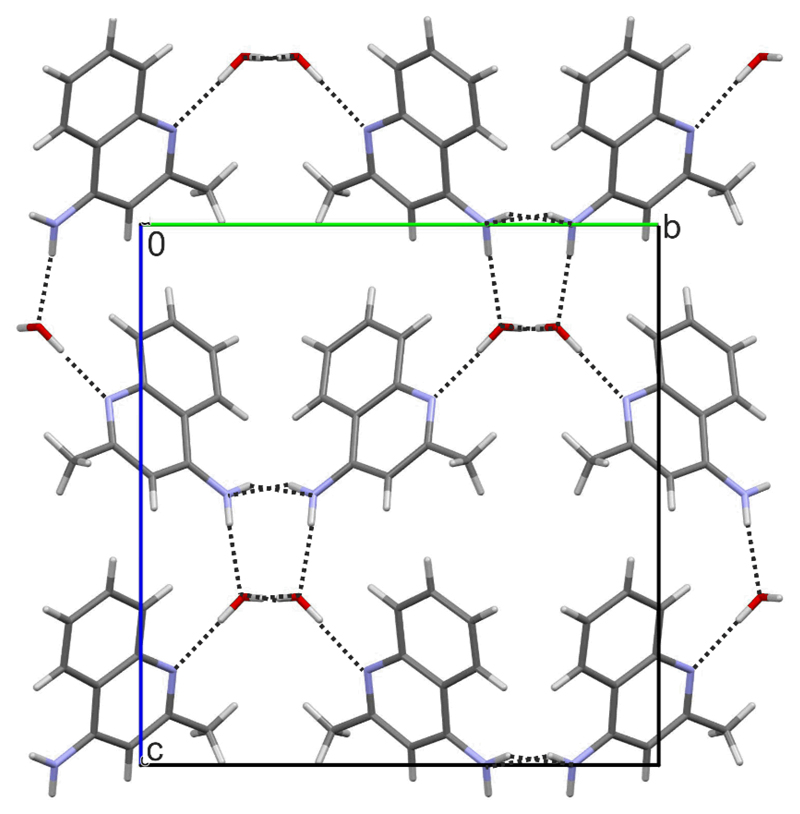
The second 4-AQ monohydrate structure has P2₁/c space group symmetry, with one 4-AQ and one water molecule in the asymmetric unit. All four hydrogen bonding donor groups are used and form four strong 4-AQ⋯water intermolecular interactions, i.e. N2–H1/2⋯Ow and Ow–H⋯N1/2 (Fig. 8). As in Hy1_A, the Hy1_B° structure forms tetrameric hydrogen bonded ring motifs, arranged in zig-zag fashion along the crystallographic [100] axis (Fig 4a) involving the amino groups and water molecules. The water molecules in the structure of Hy1_B° are strongly bound, tetragonally coordinated (alike in Hy1_A) but are isolated from each other and thus lack strong water⋯water hydrogen bonding interactions. Similar as in Hy1_A, the Hy1_B° packing exhibits polar and apolar regions and a channel-like arrangement of the water molecules in direction of the crystallographic [100] axis. Both structures are three-dimensional network structures. Dehydration of Hy1_B° may also be expected to start with the removal of the water through the channels, along the crystallographic [100] axis.

Fig. 8.

Packing diagram of Hy1_B°, viewed along the crystallographic [100] axis. Hydrogen bonding interactions are indicated with dotted lines.

The two monohydrate structures share the same type of 1D stacked 4-AQ molecules, i.e. π⋯π stacks along [100]. However, the two polymorphs differ substantially in hydrogen bonding interactions (involved groups, Fig. 4) and the overall packing arrangement (ESI† Section 2.7). Thus, a transformation between the two hydrates requires breaking of strong intermolecular interactions (see Section 4.1) and a considerable rearrangement of the molecules explaining the high kinetic stability of the metastable monohydrate Hy1_A and the fact that a transformation of this hydrate to the stable monohydrate polymorph Hy1_B° was not observed in slurry experiments (see Section 3.4).

3.4. Thermodynamic and Kinetic Stability of the 4-AQ Monohydrates and AH I°

3.4.1. RH Stability

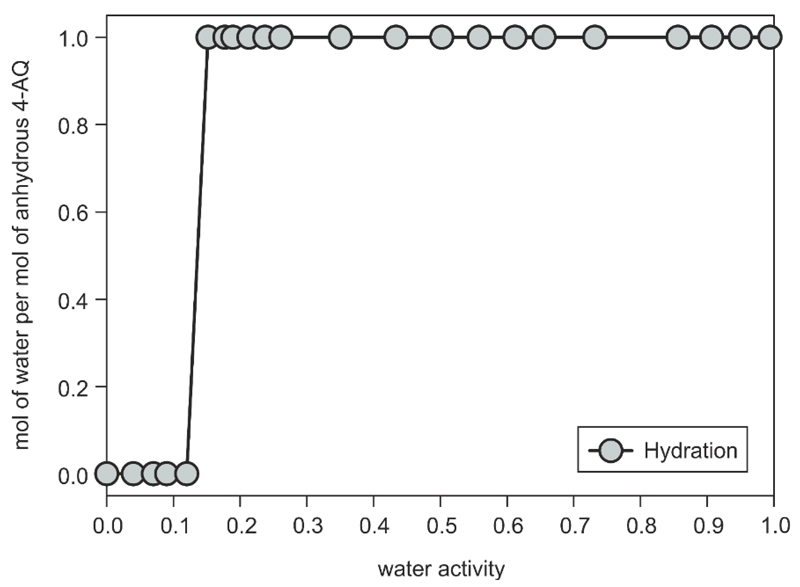
In order to overcome the kinetic control governing the gravimetric moisture sorption/desorption studies, the water activity where the anhydrate transforms to a hydrate was determined in methanol/water mixtures. Samples of 4-AQ AH I° (recrystallised from ethanol and dried at 100 °C) were added to methanol/water mixtures of various compositions (ESI† Section 2.5) representing different water activities (a_w: 0 to 1) and the suspensions were stirred for two weeks at 25° C to reach an equilibrium state. At a water activity (a_w) < 0.15, the isolated solid phase contained only AH I° (Fig. 9) whereas at a_w ≥ 0.15 Hy1_A was identified, suggesting that the equilibrium between the anhydrate and the monohydrate Hy1_A lies at an a_w of ˜ 0.14 at 25 °C. Repeating the water activity slurry experiments using the dried F sample resulted in the same thermodynamic stability ranges for anhydrous and hydrated 4-AQ.

Fig. 9.

Phase diagram of 4-AQ at different water activities in methanol/water mixtures at 25 °C. The AH I° was used as starting phase, the residual phase, after stirring the suspensions for two weeks, was determined with PXRD.

The results of this a_w study illustrates that the thermodynamic equilibrium between a hydrate and an anhydrate may be situated on one side of the hysteresis range observed in the moisture sorption/desorption experiments and not in the centre (see Fig. 5a,b). This suggests that the kinetic mechanisms and activation barriers of the two reversible processes (hydration ↔ dehydration) may vary considerably. The kinetic control of the anhydrate (AH I°) to hydrate (Hy1_A) transformation (hydration) is significantly stronger than in the reversible process (dehydration). This is indicated by the fact that the hydration occurs at an atmospheric moisture condition of a_w ≈ 0.5 (50% RH) and thus far above the true equilibrium a_w = 0.14 (Δa_w ≈ 0.3). In contrast, dehydration of Hy1_A occurs at an atmospheric moisture condition of a_w ≈ 0.05, which is very close to the equilibrium state (Δa_w ≈ 0.1) and indicates a distinctly weaker kinetic control for this dehydration process compared to hydration.

Hy1_B° was neither formed in any of the methanol/water slurries nor in liquid assisted grinding experiments, independent whether AH I°, Hy1_A of a mixture of these forms with Hy1_B° was used as starting material (time frame: two weeks). Since both hydrates were stable in any test involving solvents of sufficiently high water activity (a_w > 0.14) it was not possible to establish the thermodynamic stability order of the two polymorphic monohydrates based on the performed experiments.

3.4.2. Thermal Stability

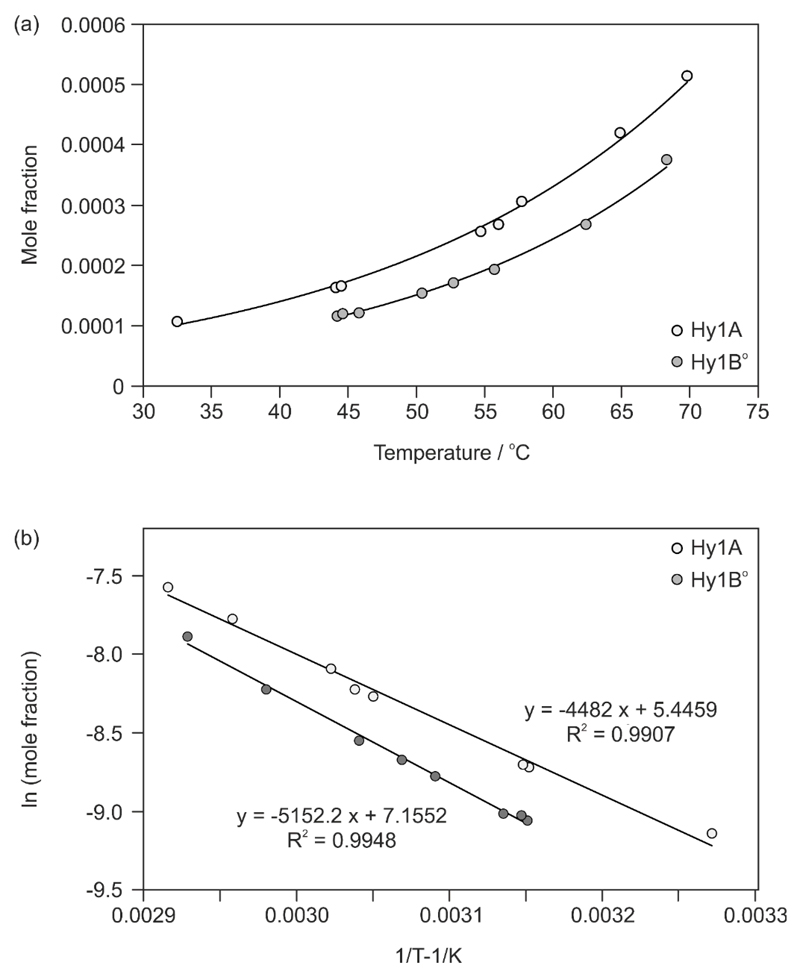
Since we failed to transform one of the polymorphic monohydrates in slurry experiments and by liquid assisted grinding we decided to determine the order of their thermodynamic stability via temperature dependent solubility experiments using a Crystal16 parallel reactor system and pure water as the solvent. As shown in Fig. 10 the water solubility of Hy1_B° is lower than that of Hy1_A which suggests that Hy1_B° is the thermodynamically stable 4-AQ monohydrate in the investigated temperature range (30 to 70 °C).

Fig. 10.

(a) Solubility (mole fraction) of 4-AQ monohydrates in water (a_w = 1.0) as a function of temperature. (b) Van’t Hoff plot of the molar solubility as a function of temperature.

Based on the extrapolated intersection of the van’t Hoff plots (Fig. 10b) the transition point between the two hydrate phases lies around 119 °C, which is only a very rough figure as such an extrapolation far beyond the experimental range is very error-prone. As shown later, the extrapolated transition point lies above the melting points of the two hydrates, suggesting a monotropic relationship. Therefore, the solubility data suggest that Hy1_B° is also the thermodynamically stable hydrate below RT (down to 0K), which is in agreement with the computational results (Fig. 3). Thermodynamic parameters, the enthalpy of solution (ΔH_sol) and entropy of solution (ΔS_sol) were estimated from the slope and constant of the regression line of the van’t Hoff plot, respectively (Table 1). The enthalpies and entropies of solution are positive for the two hydrates and greater for Hy1_B°.

Table 1. Values of the thermodynamic parameters obtained from solubility and thermal measurements and lattice energy calculations.

	Hy1A	Hy1B°	Δ (Hy1A – Hy1B°)
Solubility Measurements (30 – 70 °C, water)
ΔHsol, kJ mol–1 a	37.3	42.8	–5.6
ΔSsol, J mol–1 K–1 b	45.3	59.5	–14.2
Thermal Measurements (55– 100 °C)
Tfus (°C)c	111.1 ± 0.2	113.1 ± 0.2	–2.0 ± 0.4
ΔdehyHHy1x-I°d	53.5 ± 0.3	57.1 ± 0.2	–3.6 ± 0.5
ΔtrsHHy1x-I°e	11.9 ± 0.2	15.4 ± 0.3	–3.6 ± 0.4
Lattice Energy Calculations (0 K)
–Elatt (kJ mol–1), CryOpt (PBE0/6-31G(d,p))	172.65	176.66	–4.01
–Elatt (kJ mol–1), PBE-TS	234.45	232.48	–1.97
–Elatt (kJ mol–1), PBE-G06	212.13	212.80	–0.73
ΔdehyUHy1x-I°,d PBE- G06	79.95	80.62
ΔtrsUHy1x-I°,e PBE- G06	20.95	21.62

^aEnthalpy of solution, from the slope of van’t Hoff plot (slope = –ΔH_sol/R).

^bEntropy of solution, from the constant of the regression line (constant = ΔS_sol/R).

^cMelting point (onset temperature).

^dEnthalpy of dehydration.

^eEnthalpy of hydrate to AH I° phase transformation.

Based on the solubility difference of the two hydrate polymorphs shown in Fig. 10 we were able to produce Hy1_B° from Hy1_A/Hy1_B° mixtures. The fact that not only water, but also organic solvents such as n-propanol or methyl acetate preferentially dissolved Hy1_A indicates that also at lower a_w Hy1_B° is more stable than Hy1_A.

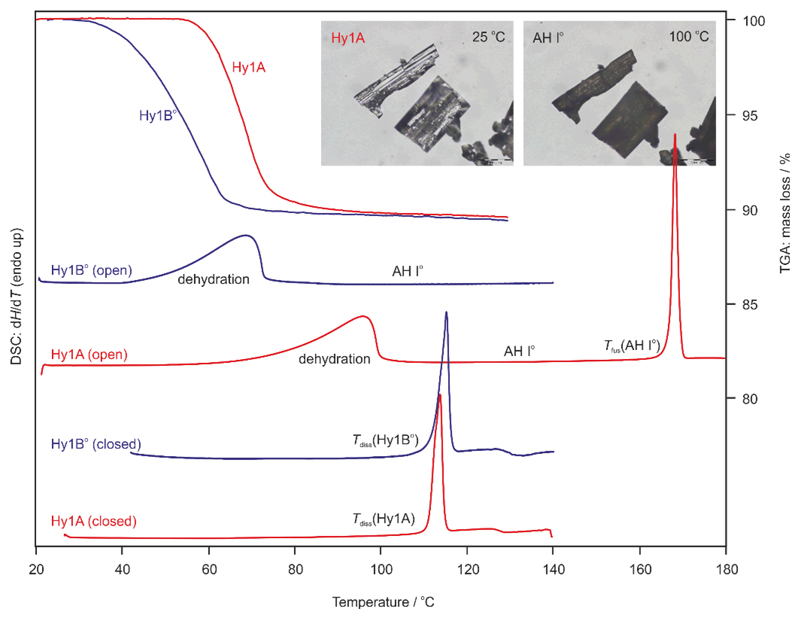
In addition to the solubility experiments HSM, TGA and DSC were employed to determine the thermal stability of the hydrates. Dehydration of Hy1_B° started at 40 °C and dehydration of Hy1_A at 60 °C (Fig. 11, TGA and “open” DSC runs). The hydrate crystals maintain their original shape but split into polycrystalline aggregates (exemplary shown for Hy1_A in Fig. 11, photomicrographs). This behaviour, termed ‘pseudomorphosis’,⁷⁴ is characteristic for stoichiometric solvates and is indicative for a pronounced reorganisation of the structure upon desolvation. Thermal dehydration of the two hydrate phases resulted exclusively in AH I°. Upon further heating sublimation of AH I° starts at a temperature above 130 °C in agreement with previous observations.⁴⁹ It has to be noted that the Hy1_B° batch consisted of very small crystals (powder) whereas the used Hy1_A sample contains larger crystals. Therefore, the onset temperature of the dehydration reaction does not allow conclusions about the thermal stability order of the two polymorphs, as crystal size influences the onset temperature of dehydration.⁷⁵

Fig. 11.

TGA curves of Hy1_A and Hy1_B° recorded at a heating rate of 5 °C min^–1. DSC thermograms of the two hydrate polymorphs were recorded in five pin-holed pans (open) at a heating rate of 5 °C min^–1 and in sealed pans (closed) at a heating rate of 10 °C min^–1. T_fus – melting point. Photomicrographs show exemplarily the pseudomorphosis of Hy1_A upon dehydration.

The TGA curves of the pure hydrates show a one-step mass loss. The mass loss observed in the TGA experiments corresponds for each of the hydrates to exactly one mol of water per mol 4-AQ.

The DSC measurements of Hy1_A and Hy1_B° were carried out either in pin-holed or sealed pans and at different heating Structures showing low packing efficiency and an unused hydrogen bond donor rates to investigate the influence of atmospheric conditions on the dehydration reaction. A broad desolvation endotherm is observed when samples are heated in pans with a five pin holed lid (Fig. 11, DSC open). The heats of dehydration were determined to be 53.5 ± 0.3 kJ mol^–1 (∆_dehyH_Hy1A-I°, T_max = 95 °C) and 57.1 ± 0.2 kJ mol^–1 (∆_dehyH_Hy1B-I°, T_max = 70 °C) for the Hy1_A and Hy1_B° to AH I° transformations, respectively. Congruent melting of the hydrate crystals can be determined at 111.1 ± 0.2 °C for Hy1_A and 113.1 ± 0.2 °C for Hy1_B°, if the hydrate sample is heated in sealed DSC pans or embedded in high viscosity silicon oil in hot-stage microscopic investigations. Based on the measured melting temperatures and ∆_dehyH enthalpies it can be concluded that the two hydrate forms are monotropically related, with Hy1_B° being the thermodynamically stable hydrate phase in the entire temperature range. Hy1_B° shows a higher melting temperature and higher enthalpy of dehydration to AH I° compared to Hy1_A. The DSC results are in agreement with the hydrate solubilities measured in water.

3.4.3. Enthalpy of Hydrate/Anhydrate and Hydrate/Hydrate Transformations

The enthalpies of the hydrate to anhydrate phase transformations (∆_trsH_Hy1-I°) were estimated by subtracting the heat of vaporisation of one mol of water, ∆_vapH, from ∆_dehyH_Hy1-I° at the dehydration temperature⁷⁶ (eq.1).

$${\Delta }_{tr}{H}_{Hy1–I°}={\Delta }_{dehy}{H}_{Hy1–I°}–{\Delta }_{vap}{H}_{H2O}$$ (1)

This resulted in ∆_trsH_Hy1A-I° of 11.9 ± 0.2 kj mol^–1 for the Hy1_A to AH I° transformation and ∆_trsH_Hy1B-I° of 15.4 ± 0.3 kJ mol^–1 for the Hy1_B° to AH I° transformation, using an average ∆_vapH°_H2O value of 41.858 kJ mol^–1 (80 °C). The Hy1_A phase was estimated to be 3.6 ± 0.4 kJ mol^–1 less stable than Hy1_B° (Table 1), in agreement with the stability order derived from the solubility data.

By comparing the lattice energies of the hydrates, the dehydration product AH I° and ice (59 kJ mol^–1)^77,78 a simple estimation of ∆_dehyU_Hy-AH and ∆_trsU_Hy-AH can be made according to eqs. (2) and (3).

$${\Delta }_{dehy}{U}_{{\text{Hy1-I}}^{\circ }}=-E_{latt(Hy)}-(-E_{latt(AH)})$$ (2)

$${\Delta }_{trs}{U}_{{\text{Hy1-I}}^{\circ }}\approx {\Delta }_{dehy}{U}_{{\text{Hy1-I}}^{\text{o}}}-n(-E_{latt(ice)})$$ (3)

∆_trsU_Hy1A-I° was calculated to be 20.95 kJ mol^–1 for the Hy1_A to AH I° and 21.62 kJ mol^–1 for the Hy1_B° to I° phase transformation. These results not only confirm that hydrate formation is driven by a greater potential energy of the hydrates but also that the monohydrates are stable phases (phase transition values of ca. 20 kJ mol^–1).

The energy differences of 0.7 kJ to 4.0 kJ mol^–1 (Table 1) between the monohydrate polymorphs, derived from static 0K lattice energy calculations, are of comparable magnitude to the experimentally measured data. Thus, the computational method not only correctly predicted the structures but also gave a reasonable energy estimation of the hydrate/anhydrate and hydrate/hydrate transformation energies.

3.4.4. Hy1_A to Hy1_B° Phase Transformation

The experiments presented in Section 3.4.1 were complemented with long term storage experiments to investigate the Hy1_A to Hy1_B° phase transformation. Phase pure and mixed hydrate samples were stored at ambient conditions, as well as at higher RH values (61%, 75%, 84% and ˜100%), for one year. A slow, but partial phase transformation from Hy1_A to Hy1_B° was observed for mixed hydrate samples stored at RH values in the range between 61% and 75% RH, in agreement with the monotropic relationship of the two hydrate polymorphs. Surprisingly, no change in phase composition was observed if mixtures were stored at high RH values (84% and 100% RH) after one year. At present, we do not have a plausible explanation for this behaviour. The pure hydrate samples did not transform within the investigated time period of one year. The fact that the 20-years old F sample did not transform to Hy1_B° indicates that metastable Hy1_A shows a very high kinetic stability.

4. Discussion

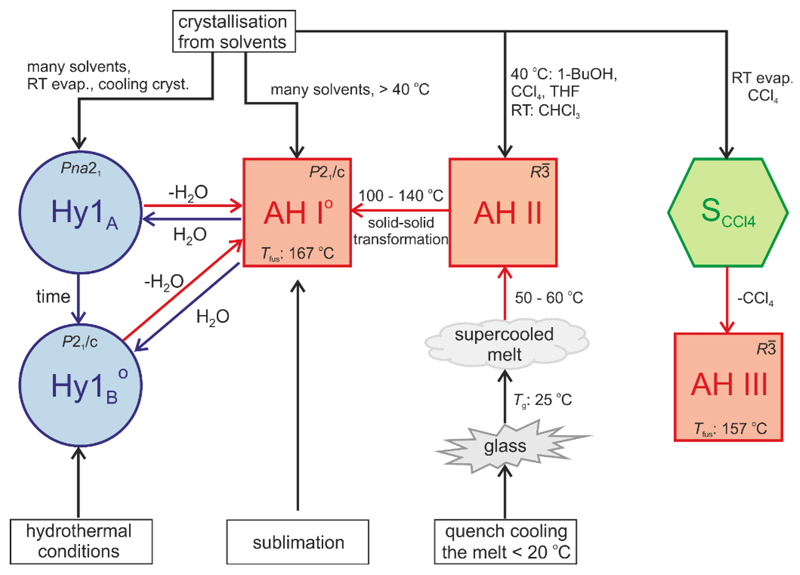
4-AQ shows a diverse solid form landscape (Fig. 12) with intriguing structural and stability phenomena. The combination of computational chemistry with experimental screening and solid form characterisation techniques allowed us to unravel the complex kinetic and thermodynamic stability relationship between the hydrates and AH I°. The results of the study complement and extend the recently established structural and thermodynamic relationships between the anhydrate polymorphs and the carbon tetrachloride solvate of 4-AQ. We now have achieved a thorough understanding of the solid state behaviour of the small organic drug-like model compound. The novel phase, Hy1_B°, is the thermodynamically most stable phase and monotropically related to the kinetic Hy1_A polymorph. Only at low RH values (< 14% RH) water free 4-AQ, AH I°, becomes more stable than the hydrates. The finding of the most stable phase, Hy1_B°, was complicated by the fact that the metastable but kinetically stabilised hydrate nucleates/grows more easily than Hy1_B°.

Fig. 12.

Preparation and transition pathways of the 4-AQ solid forms at different temperatures, relative humidities and hydrothermal conditions. AH I°, II and III – anhydrous forms, Hy1_A and Hy1_B°– monohydrate polymorphs, S_CCl4 – carbon tetrachloride solvate. Superscript “o” indicates the thermodynamically stable anhydrate/monohydrate form.

4.1. Why does 4-AQ form Hydrates?

In the anhydrous state 4-AQ was found to appear to some extent frustrated with respect to its packing efficiency and hydrogen bonding ability. Structures showing low packing efficiency (67.4%) and an unused hydrogen bonding donor group were characteristic of the computed anhydrate crystal energy landscape.⁴⁹ This is in contrast to Fig. 3 which has densely packed and with respect to hydrogen bonding interactions saturated structures (Fig. 4) as lowest energy structures.

The mismatch of hydrogen bond donor and acceptor groups in AH I°, only one of the two donor groups is involved in a strong intermolecular interaction, can be overcome by addition of water which acts as a strong hydrogen bonding donor and acceptor in Hy1_A and Hy1_B°. Pair-wise intermolecular energy calculations (U_inter), derived from PIXEL energy calculations (Table 2) showed that the strongest interactions in the hydrate structures are formed between the water and 4-AQ molecules. The water⋯4-AQ interactions per se are weaker than the strongest 4-AQ⋯4AQ AH I° hydrogen bond, however, this is compensated for by the fact that the water molecule forms four of the strongest intermolecular interactions in the hydrates (Table 2). The strongest 4-AQ⋯4-AQ pair-wise interaction observed in a hydrate, Hy1_A, was estimated to be less than half of the strength of the 4-AQ⋯4-AQ AH I° N2–H2⋯N1 hydrogen bond.

Table 2. PIXEL energy calculations on 4-AQ AH I° and monohydrate structures using the PBE–TS structure models. Only the strongest intermolecular interactions for pairs of molecules are listed.

Phase	Interactiona	ECb	EPc	EDd	ERe	Uinterf
		kJ mol–1
AH I°	N2–H2∙∙∙N1	–62.5	–29.4	–31.4	76.4	–46.9
	π∙∙∙π	–0.2	–3.7	–32.5	19.0	–17.4
	C–H∙∙∙π	–9.7	–4.8	–19.7	17.6	–16.6
Hy1A	Ow–H∙∙∙N1	–87.6	–49.7	–22.8	133.2	–27.0
	N2–H1∙∙∙Ow	–41.9	–14.4	–9.3	40.9	–24.7
	N2–H2∙∙∙N2 and C–H∙∙∙π	–16.9	–9.3	–27.7	31.2	–22.7
	π∙∙∙π	–2.7	–5.8	–43.0	31.6	–19.9
	Ow–H∙∙∙Ow	–59.3	–25.4	–8.6	77.0	–16.4
Hy1B	N2–H1∙∙∙Ow	–36.0	–11.5	–10.1	30.1	–27.4
	N2–H2∙∙∙Ow	–43.9	–16.5	–12.4	48.9	–24.0
	π∙∙∙π	–5.6	–5.7	–41.8	30.3	–22.9
	Ow–H∙∙∙N1	–87.6	–52.6	–21.4	138.9	–22.6
	Ow–H∙∙∙N2	–36.6	–18.5	–11.9	49.0	–18.0

^aPIXEL energies are for a pair of molecules. The pairs of molecules are defined by the strongest intermolecular interaction;

^belectrostatic (Coulombic) energy;

^cpolarisation energy;

^ddispersion energy;

^erepulsion energy;

^ftotal intermolecular energy: U_inter = E_C + E_P + E_D + E_R. The non-additivity of E_P is not included.

The water molecules in Hy1_A and Hy1_B° are well accommodated and strongly bound in the framework of the hydrates and account for more than half of the intermolecular energy (U_inter). Pairwise 4-AQ⋯4-AQ interactions were estimated to account for 47% and 42% of the Hy1_A and Hy1_B° U_inter, respectively. Water⋯4-AQ interactions for 40% in Hy1_A and for 55% in Hy1_B°. And finally water⋯water interactions account for the remaining 13% and 3% in Hy1_A and Hy1_B°, respectively. This may rationalise why upon dehydration a structural collapse to a distinct crystal packing, AH I°, is observed and not the formation of an isostructural dehydrate.⁷⁹ Water egress is likely to occur along the [100] axis in each of the two monohydrates, but this process is accompanied with a disruption of the 3D-network structures. Overall, the formation of additional and strong intermolecular interactions in the hydrate structures seems to be the driving force for hydrate formation.

The PIXEL U_inter calculations not only indicate water as the key pillar to the hydrate structure stabilities, but also the kinetic stability of the AH I° structure with respect to the stable hydrates. Breaking the strong N2–H2⋯N1 intermolecular interaction will require an appreciable activation energy. This may indicate why the sorption step in Fig. 5 is shifted to higher RH values (> 40% RH) relative to the estimated critical water activity of ˜0.14.

4.2. Value of CSP and Lattice Energy Calculations in Solid Form Screening and Characterisation

The computationally generated monohydrate crystal energy landscape (Fig. 3) unambiguously indicated the possibility of another more stable polymorph, which corresponds to Hy1_B°. 4-AQ is a prime example where CSP can be seen as an invaluable tool for revealing whether a more stable phase may exist and affirming whether an experimental screening should be extended or not. Thus, CSP despite being computationally time consuming, in particular for multi-component,⁸⁰ flexible and bigger systems,^81,82 has to be seen as an alternative and complementary screening approach.

The lattice energy calculations, using different methods, based on ab initio electronic calculations on either the crystal (DFT-D) or single molecule (CrystalOptimizer), solubility and thermal measurements give the same qualitative result that the new hydrate, Hy1_B°, is more stable than Hy1_A. The experimental energy differences between the two hydrates were found to be 3.6 ± 0.4 kJ mol^–1 (Table 1) when calculated from the dehydration enthalpies and ˜ 5.6 kJ mol^–1 when derived from Crystal16 solubility experiments. The lattice energy differences give estimates ranging from 0.7 to 4.0 kJ mol^–1 depending on the applied method (Table 1). The computationally derived energy differences are in good agreement with the experimental values considering that the calculations neglect zero-point and thermal effects. Computing the energy difference between a hydrate and anhydrate is more challenging than between polymorphs. The applied functional, PBE, is well known to overestimate water interactions,⁸³ which may explain why the calculations overestimate the hydrate to anhydrate transition energies (Table 1). The measured and computed energy differences were found to be in the range of other hydrate/anhydrate systems showing a stable hydrate phase, e.g. phloroglucinol dihdyrate⁴⁶ and creatine momohydrate.³⁴ Thus, the formation of hydrates, its structures and that the hydrates are stable with respect to the anhydrates could have been predicted.

4.3. Why was Hy1_B° not Found Earlier?

Firstly, the metastable Hy1_A shows a very high kinetic stability as evidenced from long time storage experiments of the F sample. Even after 20 years the Hy1_A did not transform to the thermodynamically stable Hy1_B° polymorph. Slurry experiments of Hy1_A or mixtures of Hy1_A/Hy1_B° at RT conditions (20 – 30 °C and a_w > 0.14) did not show any change in phase composition. Only lengthy storage experiments of a Hy1_A/Hy1_B° mixture in the RH range in between 61% and 75 % showed, after a year, that Hy1_A transforms very slowly to Hy1_B°. The reason for the slow transformation kinetics can be seen in an high activation energy barrier for the polymorphic phase transformation, as indicated by the different crystal packings, a phase transformation requires considerable rearrangement of the 4-AQ and water molecules, and the strong pair-wise intermolecular interactions present in Hy1_A (PIXEL energy calculations). Thus, phase pure Hy1_A is storage stable and can be handled without risk of inducing any phase transformations provided low RH values and higher temperatures are avoided.

Secondly, crystallisation of Hy1_B° at ambient pressure was found to be tied to a phase impurity, a chloro-4-aminoquinaldine derivate with a M_r of 186 (ESI† Section 2.1.3). Hy1_B° crystals obtained at the same conditions as Hy1_A crystals were smaller, indicating a slower growth rate of Hy1_B° compared to Hy1_A in the solvents that allowed the nucleation of Hy1_B°. Only specific crystallisations of impure 4-AQ from n-propanol, methyl acetate, acetonitrile or dichloromethane or exposure of impure anhydrous 4-AQ to high but not extreme moisture conditions yielded Hy1_B°. The fact that neither crystallisation, lyophilisation nor slurry experiments using water yielded Hy1_B° may be indicative that water favours the nucleation/growth of the metastable hydrate. This may be attributed to the fact that in Hy1_A direct water⋯water interactions are observed (as in solution), but water molecules are isolated in Hy1_B° which would require breaking the water⋯water interactions if water is incorporated from solution. The impurity is likely to inhibit the nucleation/growth of the kinetic form and thus facilitates the nucleation/growth of the thermodynamic form. Using a purified 4-AQ batch for the initial polymorph screen prevented us from finding the stable monohydrate form. This highlights the importance of additives as another variable in polymorphism screens as also demonstrated by the steadily growing list of examples where attempts to form co-crystals failed, but instead resulted in new polymorphs of a co-former (e.g., isonicotinamide⁸⁴, gallic acid monohydrates⁸⁵ and brucine dihydrate⁸⁶).

Thirdly, only by applying hydrothermal conditions (pressure) we were able to obtain Hy1_B° from chemically pure anhydrous 4-AQ. The variable pressure has been reported to be successful in producing solvates and polymorphic forms.^87,88 The hydrothermal crystallisation experiments were suggested by the results of CSP (Fig. 3) which indicated a high density structure as the global energy minimum. Thus, the computationally generated crystal energy landscape not only indicated that a more stable 4-AQ monohydrate should exist but also suggested a possible route for preparing the novel polymorph.

5. Conclusions

4-Aminoquinaldine shows both anhydrate and monohydrate polymorphism, with the hydrates being the stable forms under conditions of relative humidity (RH) typically found on production and storage. The metastable hydrate polymorph, Hy1_A, shows an appreciable kinetic stability and if phase pure, no conversion to the monotropically related, thermodynamically stable Hy1_B° polymorph was observed during storing the metastable phase for over 20 years at ambient conditions. Crystal structures and lattice energy calculations rationalise the stability behaviour. The introduction of water molecules generates a hydrogen bonding network of higher dimension as there is a better match between the numbers of hydrogen bond donor and acceptor groups than in the anhydrates, explaining the high tendency towards hydrate formation of 4-AQ.

It should be stressed that the discovery of the most stable solid form of 4-AQ in the present study occurred 80 years after its first synthesis.⁸⁹ It is particularly notable that this finding was guided by the computationally generated crystal energy landscape, which indicated the existence of a second more stable monohydrate with a higher density than the known monohydrate form. Crystallising Hy1_B° was complicated by the fact that the high density form shows a slow nucleation and growth rate, in contrast to Hy1_A. Therefore, a comprehensive classical polymorph screen only yielded the metastable monohydrate Hy1_A. Thanks to the availability of an impure sample of 4-AQ the stable monohydrate Hy1_B° could be experimentally produced, highlighting the remarkable impact of specific impurities on the nucleation process. Nevertheless, it was still difficult to produce phase pure Hy1_B° because of its very slow crystal growth rate why conventional strategies such as solvent mediated transformation experiments did not result in Hy1_B°. After having identified specific crystallisation conditions (hydrothermal) it was possible to obtain the thermodynamically most stable form in reasonable time.

Hence, this study highlights a serious problem in solid form screening and demonstrates clearly that finding the most stable solid form is not straightforward. Since it is practically impossible to exploit all experimental conditions that may lead to different solid forms⁸ the discovery of specific (say “practically useful”) crystal forms may be very difficult and requires some serendipity and/or high experimental demands. Theoretical methods may not only give assurance that relevant forms have been found, but may also give important indication of the existence of a “hidden” low energy form. The use of CSP as a complementary method to experimental solid form screening is therefore highly beneficial for identifying practical crystal forms. However, more experience is needed to identify the thermodynamically plausible structures and eliminate computed structures that are unlikely to be observed.^71,90 This is in particular true for more complex (flexible and drug-like), Z’>1 and charged molecules (e.g. salts), for which currently considerable computing time is required and may not give reliable results.

Finally, it should be indicated that creatine is a related case, where the thermodynamically stable anhydrate form has been predicted and experimentally verified (independently by us³⁴ and Arlin et al.⁹¹) 180 years after the discovery of the compound. However, in the case of creatine the formation and high stability of the monohydrate hampered the experimental access to the stable anhydrates.

Supplementary Material

† DFT-D calculations (details on methodology); computationally generated low energy monohydrate structures; representation of the experimental structures; PIXEL energies; void analysis; GC-MS, ESI-MS of commercial 4-AQ samples; conditions and outcomes of the manual solvent crystallization screen; water activity measurements; FT-IR and FT-Raman spectra; structure comparison. Hy1_B° crystallographic information files is available from the Cambridge Crystallographic Data Center (CCDC) upon request (http://www.ccdc.cam.ac.uk, CCDC deposition number 1419538)