Abstract
The robustness and plasticity of working memory were investigated in honey bees by using a delayed matching-to-sample (DMTS) paradigm. The findings are summarized as follows: first, performance in the DMTS task decreases as the duration between the presentation of the sample stimulus and the presentation of the comparison stimuli is increased. This decrease is well approximated by an exponential decay function. Performance is significantly better than random-choice level even at delays as long as 5 sec and is reduced to random-choice levels at an average delay time of 8.68 ± 0.06 sec. Second, when the DMTS task involves two samples (one relevant, the other irrelevant), bees can be trained to learn to use the relevant sample to perform the task if (i) the relevant sample is always at a fixed position, or (ii) the relevant sample always has the same place in the sequence of presentation (always first or always second). Bees that have learned to use the relevant sample and to ignore the irrelevant sample can generalize this learning, and apply it to novel sets of sample and comparison stimuli that they have never previously encountered. The findings point to a remarkably robust, and yet plastic, working memory in the honey bee.
Keywords: honey bee learning, matching-to-sample, maze, tunnel
Over the past decade, work on the honey bee has provided growing evidence that insects are not simple, reflexive creatures. The brains of honey bees are very small, but their ability to learn and memorize tasks is impressive. Bees display perceptual and “cognitive” capacities that are surprisingly rich, complex, and flexible (1–10). Bees can abstract general features of a stimulus, such as orientation or symmetry, and apply them to distinguish between other stimuli that they have never previously encountered (11–15). They can also extract several features and integrate them in a global representation that can be used to distinguish novel stimuli that present the learned layout from those not presenting it (16).
Bees can be taught to use new cues to detect camouflaged objects (17). They can learn to use symbolic rules for navigating through complex mazes and to apply these rules in flexible ways (18–19). Like monkeys, pigeons, and other vertebrates (20–22), recent studies have shown that even honey bees can learn delayed matching-to-sample tasks (DMTS) and can apply the learned concept of “sameness” or “difference” to solve a novel DMTS task (23–24). All of the above findings suggest that higher cognitive functions are not an exclusive privilege of vertebrates with much more complex nervous systems. The honey bee is an attractive model system for the investigation of learning and memory, because it demonstrates that even a creature with a relatively simple nervous system can display the essential elements of many complex behaviors.
Menzel and colleagues (25) have addressed the problem of memory dynamics of honey bees in a foraging context, in which the authors studied the foraging behavior of bees working on an artificial patch of flowers and characterized the different memory traces that underlie decision making at a longer or shorter delay. Working memory could be measured in this context. Later, Chittka et al. (26) verified the findings of Greggers and Menzel in the case of bumblebees and also characterized the duration and dynamics of working memory. Brown et al. (27, 28) examined the spatial working memory of honey bees and concluded that bees could choose location based on previous visits, either being attracted to or repelled from locations recently depleted of forage.
The DMTS experimental paradigm, introduced by Blough (20), is commonly used to study remembering over relatively short time intervals in nonhuman animals. Here, the animal is required to learn to match a sample stimulus with one of two or more subsequently presented comparison stimuli. Because of the delay between the presentation of the sample and the presentation of the comparison stimuli, the sample stimulus is no longer present when the subject makes a choice. Therefore, accurate performance at the end of the retention interval requires the animal to retain information about the identity of the sample. Because the sample alternates randomly from trial to trial, this information presumably is maintained in working memory (29). To fulfil the DMTS task, animals additionally have to remember and use the rules to make choices; these rules are believed to be stored in long-term memory. Here, we investigate the properties of working memory by using the DMTS paradigm.
Materials and Methods
General. The experiments were carried out in an All Weather Bee Flight Facility at the Australian National University's Research School of Biological Sciences. The facility consists of a modified glasshouse in which the internal temperature is regulated by a computer to maintain 24 ± 5°C during the day and 17 ± 3°C at night. A beehive with two entrances was mounted on the wall of the facility. One entrance allowed bees access to the inside of the facility and the other allowed bees to forage outside. Bees foraging indoors obtained sugar water from feeders in the facility. For each experiment, ≈15 bees were marked individually and trained to visit an experimental apparatus. Bees were trained to fly through a tunnel and then into a decision chamber (see below). We could control the numbers of bees flying into the apparatus by briefly blocking the entrance to enable each bee to make her choice individually. During training, the feeder was initially placed at the entrance of the tunnel and then moved step-by-step through the tunnel (advancing ≈20 cm every 10 min). Training was carried out by reinforcement: Bees that had flown through the tunnel and made a correct choice in the decision chamber obtained access to a reward of sugar solution. The trained bees flew regularly between the hive and the apparatus, averaging about three visits every 10 min.
Apparatus. The apparatus consisted of a tunnel and a decision chamber attached to the far end. The tunnel was composed of several concatenated sections, each 2.4 m long, 22.5 cm wide, and 21.5 cm high. This setup allowed the length of the tunnel to be varied. The sample pattern was presented at a point in the tunnel at a distance d1 from the tunnel entrance and a distance d2 from the entrance of the decision chamber. [The length of the tunnel was d1 + d2 (Fig. 1a).] The far end of the tunnel led to three vertically oriented cylinders, each with a diameter 22.5 cm and height 25 cm, covered by a sheet of transparent Perspex. The first cylinder (the decision chamber) had three holes with a 4-cm diameter. The holes were positioned halfway up the wall of the cylinder, i.e., 12.5 cm above the floor. One hole was the entrance to the cylinder and the other two were exits. Each exit carried one of the comparison stimuli, a square visual pattern of 18 cm surrounding the hole (Figs. 1a, 2a, and 3a).
Fig. 1.
Results of experimental series I, which examined retention of working memory. (a) Schematic illustration of apparatus used in the experiments of series I, which consists of a tunnel and a decision chamber attached to the far end. (b) Relationship between measured flight time (delay) and the distance between the sample and the decision chamber. (c) Variation of matching accuracy with time delay. This forgetting function is approximated rather well by an exponential decay function with parameters as shown. Details are in the text.
Fig. 2.
Results of experimental series II. (a) Schematic illustration of apparatus used in the experiments of series II. IP1 denotes the position of the incorrect pattern when presented at a distance of 170 cm, and IP2 denotes the position of the incorrect pattern when presented at a distance 50 cm from the entrance to the decision chamber. (b) The results indicate that the trained bees always treat the sample at the training distance as the correct sample and the other sample as the incorrect one. Asterisks denote statistically significant differences from the random choice level of 50%. ***, P < 0.001; ○, no significant difference from 50%. Details are in the text.
Fig. 3.
Results of experimental series III. (a) Schematic illustration of apparatus used in the experiments of series III. Bees were trained with two patterns (Sample 1 and Sample 2) in the tunnel, 50 cm apart. The results indicate that bees can learn whether it is the first stimulus (b) or the second stimulus (c) that is the one to be matched in the decision chamber. Furthermore, they can apply this learned rule to novel pairs of patterns never previously encountered. Details are in the text.
Training and Testing Procedures. The bees were trained to match the sample pattern with one of the two patterns in the decision chamber. That is, in each trial, the bee would receive a reward only if it chose a pattern that was the same as the sample pattern in that trial. This experiment was therefore a DMTS task. If a bee made a correct choice, it arrived in a subsequent cylinder (a reward cylinder) that contained a feeder with sugar solution. The reward cylinder had only one entrance hole. The bees were released from this cylinder after they had fed to repletion. Bees that made an incorrect choice entered an empty cylinder. They were released and allowed to reenter the tunnel to make another choice in the decision chamber.
During training and testing, the sample patterns were presented alternately in the tunnel, in 20-min training blocks (six visits per bee in each block of 20 min, on average). Each sample pattern was identical to one of the two comparison patterns in the decision chamber. The positions of the two comparison patterns (left or right) in the decision chamber were swapped every 10 min, that is, halfway through each block. The bees were trained to choose the comparison pattern that had the same orientation as the sample in each trial. Frequent swapping of the positions of the two comparison stimuli minimized the effects of possible biases caused by asymmetrical lighting or external landmarks.
Three series of experiments were carried out. In the first series, we examined how long the sample could be retained in working memory. This experiment was done by training bees to match a sample pattern with one of two comparison patterns that they encountered subsequently in a decision chamber. Retention of the sample was investigated by varying the distance between the sample pattern and the decision chamber, which varied the delay between the exposures to the sample pattern and the comparison patterns. In the second series, bees were trained with the sample pattern placed at a fixed distance from the decision chamber but were tested by confronting them with two sample patterns in the tunnel. One pattern representing the “correct” sample (the sample to be matched) was placed at the training distance, whereas the other one, representing the “incorrect” sample (the sample not to be matched) was placed at a different distance. The aim was to examine whether the trained bees would use the sample at the training position to make the correct choice in the decision chamber and disregard the other sample. In the third series, we trained the bees by using two sample patterns, placed one behind the other in the tunnel. We investigated whether bees could learn to match the comparison stimuli by using just one of the two samples: either the first sample (the sample encountered earlier, as the bees flew toward the decision chamber) or the second sample. Details of the experimental procedure for each series are described below. Each series was carried out by training a fresh group of bees.
Series I: How Long Can Bees Retain the Sample in Working Memory? During training, the sample pattern was a blue grating oriented at either 45° or 135°. In this series, the tunnel was 4.8 m long in all tests except the last two, where the length was increased to 7.2 m (Fig. 1a). During training, the sample pattern was positioned at 25 cm from the entrance of the decision chamber. Thus, the bees traveled a distance of 455 cm in the tunnel before encountering the sample pattern. They had to learn to fly through a hole at the center of the sample pattern, continue to fly a distance of 25 cm, and then enter the decision chamber, which presented two comparison patterns. Here, the bees had to choose the pattern that matched the sample encountered earlier in the tunnel (Movie 1, which is published as supporting information on the PNAS web site). If they made the correct choice, they arrived at the reward cylinder. The delay time is defined as the duration between the trained bees passing the sample and entering the decision chamber. This guideline provides a measurable but conservative estimate of the retention time of working memory, because the decision of the bees is actually made some time after entering the decision chamber.
Training lasted ≈2 days. The trained bees were tested after their performance in making the correct decision attained a plateau. In the tests, the position of the sample stimulus in the tunnel was varied systematically. That is, the distance d2 between the sample pattern and the entrance to the decision chamber was varied, thus varying the delay interval before a choice could be made. Before the trained bees were tested at each new distance, the bees were trained again at the training distance of 25 cm for two blocks. The bees were tested with d2 set at 25, 75, 125, 175, 375, 475, and 575 cm.
Series II: Can Bees Trained on a DMTS Task Continue to Perform Correctly When the Sample Pattern as Well as an Incorrect Pattern Are Presented in the Tunnel? The bees were trained as before, in a DMTS task, with a single sample pattern now located 120 cm from the entrance of the decision chamber (Fig. 2a). Two patterns were used in the test, one representing the sample pattern (the pattern to be matched) and the other pattern representing the incorrect pattern (the pattern that was not to be matched). In the tests, the sample pattern was always presented at the training distance (120 cm from the entrance to the decision chamber). The incorrect pattern, on the other hand, was presented at a distance of either 170 cm (IP1 in Fig. 2a) or 50 cm (IP2 in Fig. 2a) from the entrance to the decision chamber. To perform correctly, the bees had to use only the correct sample pattern as the cue (the pattern that was placed at the training distance of 120 cm). They had to ignore the other pattern, which was placed at a position different from the training distance.
Series III: Can Bees Learn Which of Two Sequentially Encountered Patterns in the Tunnel Is the Pattern to Be Matched in the Decision Cylinder? In this series, bees were trained with two sample patterns in the tunnel, 50 cm apart (Fig. 3a). The sample and choice patterns were blue/white gratings oriented at 45° or 135° to the horizontal. One pattern was the correct sample pattern (this pattern was the one to be matched in the decision chamber), whereas the other pattern was the incorrect sample pattern. During training, the positions of the two samples were changed regularly and simultaneously, but the distance between the two samples was always kept at 50 cm. Moreover, the sequential order of the correct and incorrect samples was always preserved. The tunnel was 4.8 m long.
In one training experiment, the correct sample pattern was always the first pattern that the bees encountered in the tunnel as they flew toward the decision chamber. Here, the bees had to learn to ignore the second pattern (or learn that it represented the pattern not to be chosen in the decision chamber). In another experiment, the correct sample pattern was always the second pattern. In this case, the bees had to learn to ignore the first one, or treat it as an indicator of the wrong comparison pattern.
Bees trained in this way were subsequently subjected to a series of learning tests to evaluate their performance in choosing the correct pattern in the decision chamber. The trained bees were also tested with a pair of novel patterns, namely, a ring pattern and a sector pattern. These tests examined whether bees could generalize the rule they had learned (about using the first or the second pattern as the matching stimulus, depending on the experiment) and apply this rule to deal with novel stimuli, which they had not previously experienced.
In the tests, the positions of the sample patterns were varied randomly from one test to the next, but their separation was always 50 cm. The results of tests conducted at various positions were pooled to obtain a grand average.
Stimuli. Visual patterns (18 cm × 18 cm) were printed on a color laser printer (Phaser 780 Graphics, Tektronix, Beaverton, OR).
Blue/white gratings, with a period of 4 cm and oriented at 45° and 135° to the horizontal, were used in the training and tests for the experiments of series I and II. A pair of center-symmetrical patterns, namely a radial pattern with six blue/white sectors and a ring pattern with three blue/white rings was used in one of the transfer tests of series III. A pair of blue/white gratings with a period of 4 cm, oriented at 0° and 90°, was used in another transfer test of series III.
Data Collection and Analysis. During training and testing, the sample patterns were presented alternately in the tunnel in 20 min training blocks. In the decision chamber, the positions of the two comparison patterns (left or right) were swapped every 10 min, that is, halfway through each block. Frequent swapping of the positions of the two comparison stimuli minimized the effects of possible biases caused by asymmetrical lighting or external landmarks.
We used two methods to analyze the choices of the bees. In the first method, we analyzed only the first choice for each visit of the bee from the hive. In the second method, we analyzed all choices for each visit of the bee from the hive. The results obtained by these two methods were quite similar. The results reported here were obtained by the second method.
The choices of the bees were recorded in each block during the training and in the tests. For each experimental series, 4–8 blocks of tests were carried out. We performed ANOVA for checking the homogeneity of data across all blocks and for individual bees for a given type of test by using the statistical software systat (Systat Software, Richmond, CA). Based on these tests, the performance of each bee was evaluated separately by pooling its correct choices and visits over all blocks and calculating the ratio of the number of correct choices to the number of visits. The average performance for a given type of test was obtained by averaging choice frequencies across bees. The sample size (n) was taken to be the number of bees, rather than the number of individual choices. This choice ensured that the samples were truly statistically independent. The data were analyzed to obtain mean values of choice frequency, standard deviations, and standard errors of means. In the text and in the figures, performance is indicated by the mean choice frequency (± SE). The Student t test was used to determine whether choice performance was significantly better than random choice.
In the experiments of series I, the time delay between encountering the sample pattern and entering the decision chamber was measured by using a stopwatch. This delay was taken as a measure of the time between the encountering of the sample and the making of a choice.
Control Tests. At the end of each experimental series, two kinds of control tests were run. One kind of test was used to check whether the trained bees had developed any side bias in their choices (i.e., whether they showed a tendency to prefer the left-hand or the right-hand pattern in the decision chamber). In this test, the choice performance of the bees was measured after removing the feeder and making both comparison patterns identical with the sample pattern. A preference for one side or the other would indicate a side bias.
Another type of control test was used to check whether the trained bees were making their choices based on odor cues that might have emanated from the feeder. The odors could have arisen from the material from which the feeder was constructed (Perspex), pheromones deposited on the feeder by the visiting bees, or the sugar solution itself. In this test, the two comparison stimuli were again identical with the sample stimulus, and the feeder was placed behind one of the comparison patterns. A preference for the comparison pattern that was in front of the feeder would indicate that the choices of the bees were influenced by scents from the feeder.
Results
Series I: How Long Can Bees Retain the Sample in Working Memory? Experiments began with pretraining, which included training the bees to fly in the tunnel, pass the sample pattern, and then make a choice in the decision chamber and find the feeder. Learning tests were commenced on the second day of training. In the learning tests, the sample pattern was positioned 25 cm from the entrance to the decision chamber (475 cm from the tunnel entrance). Performance was measured over a total of 286 visits by 16 bees. The ANOVA tests revealed that the data collected across all blocks and across all bees in series I were homogeneous (P > 0.05; see Table 1, which is published as supporting information on the PNAS web site). For each sample, the bees showed a strong and statistically significant preference for the matching comparison pattern. The average choice frequency in favor of the matching pattern was 0.75 ± 0.03, which is significantly greater than the random choice level of 50% (P < 0.001) (Fig. 1c). Thus, the bees learned this task well. The average delay between passing the sample pattern and entering the decision chamber was 1.24 ± 0.10 sec.
The performance of the trained bees was then tested by varying the distance d2 between the sample pattern and the entrance to the decision chamber. The distances tested were 75, 125, 175, 375, 475, and 575 cm, which led to a corresponding increase in the delay interval. The flight duration between passing the sample and entering the decision chamber was measured for each of these distances. The results, shown in Fig. 1b, reveal that the time delay between passing the sample and entering the decision chamber is almost linearly proportional to flight distance. The correlation coefficient between these two variables is 0.985. Thus, the speed of flight through the tunnel is approximately constant. The accuracy with which the bees matched the sample pattern decreased as the distance (and therefore the delay) increased (Fig. 1c). Performance was significantly better than random choice even at a distance of 375 cm, which corresponded to an average delay of 6.53 ± 0.86 sec. Performance fell to a random choice level at a distance of 475 cm, corresponding to an average delay of 8.86 ± 1.23 sec. The decline in performance with increasing delay can be approximated by an exponential decay function (see Fig. 1c). The significance of this will be explored in Discussion.
Series II: Can Bees Trained on a DMTS Task Continue to Perform Correctly When the Sample Pattern as Well as the Incorrect Sample Pattern Are Presented in the Tunnel? Bees were first trained with a single sample pattern placed 120 cm in front of the decision chamber entrance. Their matching performance was then measured in learning tests. Subsequently, the bees were tested by presenting them with two sample patterns, the correct one positioned at the training distance and the incorrect one placed at a different distance, either behind or in front of the correct pattern.
ANOVA tests showed that the data collected across all blocks and all bees in series II were homogeneous (P > 0.05; see Table 2, which is published as supporting information on the PNAS web site). In the learning tests, the trained bees used the sample pattern (which was always placed at a distance of 120 cm in front of the entrance to the decision chamber) to choose the matching pattern in the decision chamber at a rate that was significantly better than random choice (0.64 ± 0.03, n = 9, P < 0.001; see learning test 1 in Fig. 2b).
In one set of transfer tests, the trained bees were confronted with two sample patterns in the tunnel. The correct sample pattern was placed at the training distance (120 cm from the entrance to the decision chamber), whereas the incorrect sample pattern was placed either in front of or behind this pattern. When the incorrect sample pattern was presented behind the correct one (at a distance of 50 cm from the entrance of the decision chamber), the bees continued to choose the matching pattern in the decision chamber at a rate that was significantly better than random choice (0.64 ± 0.03, n = 7, P < 0.001, transfer test 1 in Fig. 2b). When the incorrect sample pattern was presented in front of the correct one (at a distance of 170 cm from the decision chamber entrance), the bees again performed significantly better than random choice (0.69 ± 0.04, n = 7, P < 0.001, transfer test 2 in Fig. 2b).
The bees were trained further for six blocks before conducting a second bout of learning tests and a third set of transfer tests. The learning tests showed that the trained bees continued to choose the matching pattern in the decision chamber at a rate that was significantly better than random choice (0.64 ± 0.03, n = 7, P < 0.001; see learning test 2 in Fig. 2b). In the third set of transfer tests, the correct sample pattern was placed 50 cm before the decision chamber entrance, and the incorrect sample pattern at a distance of 170 cm. In this case, neither sample was at the training distance of 120 cm. The results of this test show that the trained bees were totally confused. Performance was reduced to random choice level: the choice frequency for the correct comparison stimulus was now 0.50 ± 0.06, (n = 6, P > 0.90, transfer test 3 in Fig. 2b). Thus, the trained bees were distinguishing between the correct and incorrect sample patterns on the basis of their positions in the tunnel: the correct sample pattern was always taken to be the one that was positioned at the training distance of 120 cm.
Series III: Can Bees Learn Which of Two Sequentially Encountered Patterns in the Tunnel Is the Pattern to Be Matched in the Decision Cylinder? Here, bees were trained with two sample patterns in the tunnel. One represented the correct pattern, the other the incorrect one. In one experiment, the bees were trained with the correct sample placed in front of the incorrect one. In another experiment, the incorrect sample was placed in front of the correct one. The details are described in Materials and Methods. The question being addressed was: can bees perform the matching task by learning, in each experiment, the right sequence of correct and incorrect patterns?
ANOVA tests showed that the data collected across all blocks and all bees in series III were homogeneous (P > 0.05; see Table 3, which is published as supporting information on the PNAS web site). The results of the first experiment are shown in Fig. 3b. Learning test 1 (Fig. 3b) shows that the trained bees were able to learn to use the grating that was encountered first in the tunnel, to make a correct choice in the decision chamber. The choice frequency for the correct pattern in the decision chamber was 0.73 ± 0.03, (n = 23, P < 0.001, learning test in Fig. 3b). In the transfer tests, the bees were confronted with unfamiliar sets of sample and comparison patterns, to check whether the trained bees could carry out the matching task by using the first sample that was encountered even under these novel conditions. The bees performed well with novel patterns comprising rings and radial sectors: the choice frequency for the matching comparison pattern was 0.66 ± 0.04 (n = 18, P < 0.001, transfer test 1 in Fig. 3b). Performance was also good with a different set of novel patterns, comprising gratings at 90° and 0°. In this case, the choice frequency for the matching comparison pattern was 0.65 ± 0.02 (n = 19, P < 0.001, transfer test 2 in Fig. 3b).
In the second experiment, a fresh group of bees was trained with the first sample in the tunnel representing the incorrect pattern and the second sample the correct one. The results are shown in Fig. 3c. Here again, the trained bees performed well in the learning tests: the choice frequency in favor of the matching pattern in the decision chamber was 0.64 ± 0.03 (n = 15, P < 0.001, learning test in Fig. 3c). Performance was also good in a transfer test involving a novel set of patterns, namely rings and radial sectors: here, the bees chose the matching pattern in the decision chamber with a choice frequency of 0.67 ± 0.04 (n = 11, P < 0.001, transfer test 1 in Fig. 3c).
In another transfer test, the trained bees were tested by increasing the distance between two training samples to 100 cm. Here, the first (incorrect) sample was at a distance of 150 cm from the entrance to the decision chamber, whereas the second (correct) sample was at 50 cm from the entrance. Here again, the trained bees performed well in the tests: the choice frequency in favor of the matching pattern in the decision chamber was 0.60 ± 0.03 (n = 10, P < 0.001, transfer test 2 in Fig. 3c).
This series of experiments demonstrates that bees can indeed learn which of two sequentially encountered patterns is the pattern to be matched in a subsequent pattern-discrimination task.
Control Tests. In the first type of control test (see Materials and Methods), the choice frequency for one of the two (identical) comparison stimuli was 0.48, which was not significantly different from the random choice level of 0.50 (n = 31, P > 0.90). This result indicates that the bees did not have any significant side preference. In the second type of control test (see Materials and Methods), the choice frequency for the comparison stimulus that was associated with the feeder was 0.45, which was not significantly different from random choice (n = 90, P > 0.3). This finding indicates that olfactory cues had a negligible effect on the choice performance of bees in the experiments. This conclusion is further supported by the finding that the performance of trained bees in series I drops to random-choice levels at distances >475 cm (or delays >8.9 sec). If the bees were using olfactory cues from the feeder to choose the correct comparison stimulus, performance would never have dropped to random choice levels in this experiment.
Discussion
In general, our findings indicate that working memory in the honey bee, as revealed by performance in a variety of delayed-match-to-sample tasks, is robust as well as flexible.
The results of experimental series I show that information about a sample pattern can be held in working memory for a period as long as 5 sec before being used to make a choice between two test patterns in a decision chamber. The performance accuracy of the bees in choosing the correct test pattern diminishes as the time delay (Fig. 1c) between the sample and the decision chamber is increased. The decay in performance is well approximated by an exponential decay function as follows:
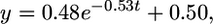 |
[1] |
where t denotes time delay in seconds. Thus, working memory decays with a time constant of 1/0.53 ≈ 1.9 sec.
The regression coefficient for the curve in Fig. 1c is 0.999, demonstrating that the data are approximated well by an exponential function. Thus, it appears that the information retained in working memory decays exponentially as a function of time (or of distance flown). A quantity that decays exponentially is one that decreases at a rate proportional to its current value. This finding is consistent with those from most DMTS experiments, where there is a decrement in accuracy as the time delay increases. The decay function has also been called the “forgetting function” (or retention function) (30–32).
Chittka and colleagues (26, 33) conducted a field study of bee foraging behavior to examine the implications of memory dynamics for flower choice in bumble bees. They inferred that the dynamics of working memory might explain the accuracy of flower constancy when visiting natural flowers. They found that the flight constancy was excellent at intervisit intervals of up to 2 sec, dropped to half-maximum accuracy at 3–4 sec, and had decayed completely at 9 sec. These numbers are surprisingly similar to those obtained in the present study, where we have measured the performance of working memory by directly manipulating delay time in our apparatus. One difference, however, is that in our study, the bee never received a reward at the sample, but only after it had chosen the matching comparison stimulus.
The results of experimental series II indicate that when bees are trained with a single sample stimulus at a fixed distance from the point where they have to make a decision, they not only learn the matching rule, but also acquire information about the distance of the sample from the decision point. Thus, when the trained bees are confronted with two sample stimuli, one at the training distance and the other at a different (unfamiliar) distance, they treat the stimulus at the familiar distance as the “true” sample stimulus and deem the other stimulus to be irrelevant. Recent work has shown that bees estimate distance flown in terms of the image motion that they experience en route (34–39). In our experiments, however, the walls and floor of the tunnel were devoid of any visual texture, thus precluding accurate measurement of image motion. How, then, were the bees estimating the position of the true sample stimulus? One possibility is that they were using visual landmarks external to the tunnel as reference cues.
Recently, researchers have shown that rhesus monkeys can learn a sequence of arbitrary images and subsequently apply that knowledge to answer new questions about that sequence (40). The experiments of series III demonstrate that honey bees possess a similar capability but in a simpler form. These experiments show that bees can learn which of two sequentially encountered patterns is the pattern to be matched in a subsequent pattern-discrimination task. They can also apply this learned rule to novel sets of sample and comparison patterns. The bees could not have solved this task merely by learning the position of the relevant sample. This conclusion is because, although the two samples in the tunnel were always separated by a fixed distance, their positions along the length of the tunnel were varied constantly, during the training as well as in the tests.
It is possible that, in this experimental series, the bees were not only learning that the relevant sample indicates which comparison pattern is the correct one but also that the irrelevant sample indicated which comparison pattern was not to be chosen. In other words, the bees could have been simultaneously learning a matching and a nonmatching task. Indeed, earlier work has shown that bees can be trained to perform matching as well as nonmatching tasks (23). Further study is required to explore whether bees can learn to perform similarly with sequences of more than two (e.g., three) sample stimuli. The question there would be whether bees can learn to treat the first, second, or the third sample stimulus as the relevant one, depending on the specific training paradigm.
Supplementary Material
Acknowledgments
We thank Hong Zhu for invaluable assistance with all of the experiments. This research was supported partly by Australian Research Council Grant DP-0450535 (to S.Z. and M.V.S.) and U.S. Defense Advanced Research Projects Agency Grant N00014-99-1-0506 (to M.V.S.). The Alexander von Humboldt Foundation supported the research of S.Z. in Germany, and the Bavarian Ministry of Agriculture supported F.B. and J.T.
Author contributions: S.Z., J.T., and M.V.S. designed research; S.Z., F.B., A.S., J.T., and M.V.S. performed research; S.Z. and F.B. analyzed data; A.S. assisted preparation of the manuscript; S.Z. wrote the paper; and M.V.S. assisted revising the manuscript.
Abbreviation: DMTS, delayed matching-to-sample.
References
- 1.Lindauer, M. (1969) in Processing of Optical Data by Organisms and by Machines, ed. Reichardt, W. (Academic, New York), pp. 527–543.
- 2.Menzel, R. (1990) in Neurobiology of Comparative Cognition, eds. Kesner, R. P. & Olton, D. S. (Lawrence Erlbaum Associates, Hillsdale, NJ), pp. 237–292.
- 3.Srinivasan, M. V. (1994) J. Insect Physiol. 40, 183–194. [Google Scholar]
- 4.Menzel, R. & Müller, U. (1996) Annu. Rev. Neurosci. 19, 379–404. [DOI] [PubMed] [Google Scholar]
- 5.Wehner, R. (1997) in The Origin and Evolution of Intelligence, eds. Scheibel, A. B., Schopf, J. W. Scheibel, A. B. & Schopf, J. W. (Jones and Bartlett, Boston), pp. 1–26.
- 6.Srinivasan, M. V. & Zhang, S. W. (1998) Zoology 101, 246–259. [Google Scholar]
- 7.Menzel, R. & Giurfa, M. (2001) Trends Cogn. Sci. (Regul. Ed.) 5, 61–71. [DOI] [PubMed] [Google Scholar]
- 8.Collett, T. S. & Collett, M. (2002) Nat. Rev. Neurosci. 3, 542–552. [DOI] [PubMed] [Google Scholar]
- 9.Giurfa, M. (2003) Curr. Opin. Neurobiol. 13, 726–735. [DOI] [PubMed] [Google Scholar]
- 10.Zhang, S. W. & Srinivasan, M. V. (2004) in The Visual Neurosciences, eds. Chalupa, L. & Werner, J. S. (MIT Press, Cambridge, MA), pp. 1501–1513.
- 11.Lindauer, M. (1969) in Processing of Optical Data by Organisms and by Machines, ed. Reichardt, W. (Academic, New York), pp. 510–526.
- 12.Wehner, R. (1971) J. Insect. Physiol. 17, 1579–1591. [Google Scholar]
- 13.van Hateren, J. H., Srinivasan, M. V. & Wait, P. B. (1990) J. Comp. Physiol. A 167, 649–654. [Google Scholar]
- 14.Horridge, G. A. & Zhang, S. W. (1995) J. Insect. Physiol. 41, 681–688. [Google Scholar]
- 15.Giurfa, M., Eichmann, B. & Menzel, R. (1996) Nature 382, 458–461. [DOI] [PubMed] [Google Scholar]
- 16.Stach1, S., Benard, J. & Giurfa, M. (2004) Nature 429, 758–761. [DOI] [PubMed] [Google Scholar]
- 17.Zhang, S. W. & Srinivasan, M. V. (1994) Nature 368, 330–333. [Google Scholar]
- 18.Zhang, S. W., Bartsch, K. & Srinivasan, M. V. (1996) Neurobiol. Learn. Mem. 66, 267–282. [DOI] [PubMed] [Google Scholar]
- 19.Zhang, S. W., Lehrer, M. & Srinivasan, M. V. (1999) Learn. Mem. 72, 180–201. [DOI] [PubMed] [Google Scholar]
- 20.Blough, D. S. (1959) J. Exper. Anal. Behav. 2, 151–160. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 21.Herman, L. M. & Gordon, J. A. (1974) J. Exp. Anal. Behav. 21, 19–26. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 22.D'Amato, M. R., Salmon, D. P. & Colombo, M. (1985) J. Exp. Psychol. Anim. Behav. Process 11, 35–51. [DOI] [PubMed] [Google Scholar]
- 23.Giurfa, M., Zhang, S. W., Jenett, A., Menzel, R. & Srinivasan, M. V. (2001) Nature 410, 930–933. [DOI] [PubMed] [Google Scholar]
- 24.Brown, M. F., Mckeon, D., Curley, T., Weston, B., Lambert, C. & Lebowitz, B. (1998) Anim. Learn. Behav. 26, 264–271. [Google Scholar]
- 25.Greggers, U. & Menzel, R. (1993) Behav. Ecol. Sociobiol. 32, 17–29. [Google Scholar]
- 26.Chittka, L., Gumbert, A. & Kunze, J. (1997) Behav. Ecol. 8, 239–249. [Google Scholar]
- 27.Brown, M. F. & Demas, G. E. (1994) J. Comp. Psychol. 108, 344–352. [DOI] [PubMed] [Google Scholar]
- 28.Brown, M. F., Moore, J. A., Brown, C. H. & Langheld, K. D. (1997) Anim. Learn. Behav. 25, 473–484. [Google Scholar]
- 29.Roitblat, H. L. (1987) in Introduction to Comparative Cognition (W.H. Freeman, New York), pp. 146–189.
- 30.White, K. G. & Wixted, J. T. (1999) J. Exper. Anal. Behav. 71, 91–113. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 31.Wixted, J. T. (1989) J. Exper. Anal. Behav. 52, 409–426. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Ziegler, P. E. & Wehner, R. (1997) J. Comp. Physiol. A 181, 13–20. [Google Scholar]
- 33.Chittka, L., Thomson, J. D. & Waser, N. M. (1999) Naturwissenschaften 86, 361–377. [Google Scholar]
- 34.Esch, H. & Burns, J. (1996) J. Exp. Biol. 199, 155–162. [DOI] [PubMed] [Google Scholar]
- 35.Srinivasan, M. V., Zhang, S. W., Lehrer, M. & Collett, T. S. (1996) J. Exp. Biol. 199, 237–244. [DOI] [PubMed] [Google Scholar]
- 36.Srinivasan, M. V., Zhang, S. W., Altwein, M. & Tautz, J. (2000) Science 287, 281–283. [DOI] [PubMed] [Google Scholar]
- 37.Esch, H. E., Zhang, S. W., Srinivasan, M. V. & Tautz, J. (2001) Nature 411, 581–583. [DOI] [PubMed] [Google Scholar]
- 38.Si, A., Srinivasan, M. V. & Zhang, S. W. (2003) J. Exp. Biol. 206, 1265–1273. [DOI] [PubMed] [Google Scholar]
- 39.Tautz, J., Zhang, S. W., Spaethe, J., Brockmann, A., Si, A. & Srinivasan, M. V. (2004) PLoS Biol. 2, 915–923. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Terrace, H. S., Son, L. K. & Brannon, E. M. (2003) Psychol. Sci. 14, 66–73. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.





