NMR at Low and Ultra-Low Temperatures

Conspectus

Solid state nuclear magnetic resonance (NMR) measurements at low temperatures have been common in physical sciences for many years, and are becoming increasingly important in studies of biomolecular systems. This article reviews a diverse set of projects from my laboratory, dating back to the early 1990s, that illustrate the motivations for low-temperature solid state NMR, the types of information that are available from the measurements, and likely directions for future research. These projects include NMR studies of both physical and biological systems, performed at low (cooled with nitrogen, down to 77 K) and very low (cooled with helium, below 77 K) temperatures, and performed with and without magic-angle spinning (MAS).

In NMR studies of physical systems, the main motivation is to study phenomena that occur only at low temperatures. Two examples from my laboratory are studies of molecular rotation and an orientational ordering in solid C₆₀ at low temperatures and studies of unusual electronic states, called skyrmions, in two-dimensionally confined electron systems within semiconductor quantum wells. NMR measurements on quantum wells were facilitated by optical pumping of nuclear spin polarizations, a signal enhancement phenomenon that exists at very low temperatures.

In studies of biomolecular systems, motivations for low-temperature NMR include suppression of molecular tumbling (thereby permitting solid state NMR measurements on soluble proteins), suppression of conformational exchange (thereby permitting quantitation of conformational distributions), and trapping of transient intermediate states in a non-equilibrium kinetic process (by rapid freeze-quenching). Solid state NMR measurements on AIDS-related peptide/antibody complexes, chemically denatured states of the model protein HP35, and a transient intermediate in the rapid folding pathway of HP35 illustrate these motivations.

NMR sensitivity generally increases with decreasing sample temperature. It is therefore advantageous to go as cold as possible, particularly in studies of biomolecular systems in frozen solutions. However, solid state NMR studies of biomolecular systems generally require rapid MAS. A novel MAS NMR probe design that uses nitrogen gas for sample spinning and cold helium only for sample cooling allows a wide variety of solid state NMR measurements to be performed on biomolecular systems at 20-25 K, where signals are enhanced by factors of 12-15 relative to measurements at room temperature.

MAS NMR at very low temperatures also facilitates dynamic nuclear polarization (DNP), allowing sizeable additional signal enhancements and large absolute NMR signal amplitudes to be achieved with relatively low microwave powers. Current research in my laboratory seeks to develop and exploit DNP-enhanced MAS NMR at very low temperatures, for example in studies of transient intermediates in protein folding and aggregation processes and studies of peptide/protein complexes that can be prepared only at low concentrations.

Introduction

There are several motivations for performing nuclear magnetic resonance (NMR) measurements at low temperatures, including: (1) to study phenomena that occur only at low temperatures; (2) to immobilize molecules in frozen solutions, thereby permitting solid state NMR measurements on soluble systems; (3) to trap transient states that would quickly disappear at higher temperatures; (4) to enhance the signal-to-noise of the NMR measurements; (5) to facilitate dynamic nuclear polarization and other hyperpolarization methods. This article reviews research projects in my laboratory that have involved both low temperatures (defined as sample temperatures that can be achieved with liquid nitrogen) and ultra-low temperatures (defined as sample temperatures that require liquid helium) and that illustrate these five motivations. Of course, many other laboratories have pursued low-temperature NMR measurements for various purposes and continue to do so. This article does not include a review of work from other laboratories, although results from other laboratories are mentioned at appropriate points.

Low-temperature NMR to study temperature-dependent phenomena

Molecular motions in solid C₆₀

Since biological processes do not ordinarily occur at low temperatures, the phenomena that are studied by low-temperature NMR (and other low-temperature techniques) are generally drawn from the fields of physics, physical chemistry, and materials science. In physics, phenomena such as superconductivity,¹ antiferromagnetism,² and charge density waves³ have been the subjects of numerous low-temperature NMR studies.

When simple methods for producing macroscopic quantities of the all-carbon molecules called fullerenes were invented in 1990,⁴ my colleagues at AT&T Bell Laboratories and I became interested in the dynamics of fullerenes in the solid state, originally with the idea that the soccer-ball shape proposed for the “buckminsterfullerene” C₆₀ molecule might lead to isotropic molecular rotation within solid C₆₀. This idea was born out by the natural-abundance ¹³C NMR spectra of C₆₀ powder shown in Fig. 1a, which show the broad chemical shift anisotropy (CSA) powder pattern lineshape expected for static molecules at temperatures below 140 K, but a single line at the isotropic chemical shift position at higher temperatures.⁵ The temperature-dependent change in the ¹³C NMR spectrum is due to averaging of the CSA to zero at the higher temperatures by rapid molecular reorientiation, together with the fact that all carbon sites in C₆₀ are related by rotational symmetry, making their isotropic chemical shifts equal.

Figure 1.

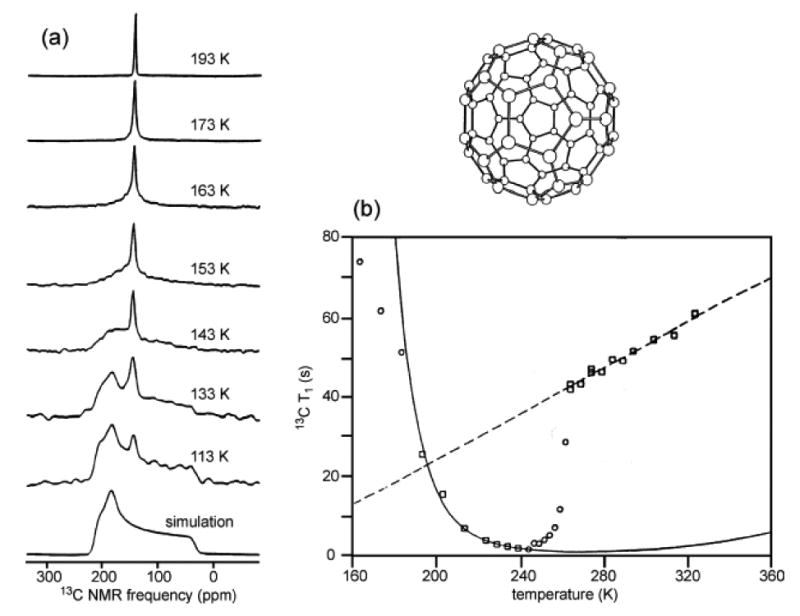
(a) ¹³C NMR spectra of polycrystalline C₆₀ at the indicated temperatures and 9.39 T, compared with the simulated chemical shift anisotropy powder pattern line shape for immobilized molecules. (b) Experimental temperature dependence of the spin-lattice relaxation time (squares and circles) and best-fit theoretical curves for low-temperature and high-temperature phases (solid and dashed lines, respectively) with different activation energies for molecular reorientation. [reprinted from Phys. Rev. Lett. 67, 1886 (1991)].

On the other hand, diffraction measurements on C₆₀ powder by Heiney et al.⁶ seemed to show that molecular motion stopped at an orientational order-disorder phase transition at T_c ≈ 250 K. More precisely, C₆₀ molecules appeared as nearly featureless spheres in diffraction data above T_c, but as objects with the expected icosahedral symmetry below T_c. Yet the solid state NMR spectra in Fig. 1a showed that rotational motion on the microsecond time scale continued down to 150 K.

The apparent contradiction between NMR and diffraction results for solid C₆₀ was resolved by the temperature-dependent ¹³C spin-lattice relaxation time (T₁) measurements shown in Fig. 1b. These measurements show that the nature of molecular motions changes qualitatively at the phase transition identified by diffraction, from reorientation on the ∼20 ps time scale immediately above T_c (which we found to be 260 K) to reorientation on the ∼3 ns time scale immediately below T_c.⁵ Above the phase transition, C₆₀ molecules reorient by rotational diffusion, assuming all possible orientations with nearly equal probabilities and therefore appearing as spheres in diffraction data. Below the phase transition, C₆₀ molecules reorient by thermally activated jumps among symmetry-related orientations and therefore appear as icosahedrally symmetry objects in diffraction data.

Skyrmions in two-dimensional electron systems

Technologically important devices often depend on thin-film materials, which are difficult to study by NMR due to the low sensitivity of conventional NMR measurements. For this reason, while still at Bell Labs, Sean Barrett and I became interested in optical pumping of nuclear spin polarizations in III-V semiconductors, a phenomenon in which excitation of electron-hole pairs by light tuned near the semiconductor band gap energy leads to the creation of large nuclear spin polarizations through hyperfine couplings between photo-excited electrons and nuclei.⁷ Optical pumping can generate nuclear spin polarizations on the order of 10%,⁸ but requires low temperatures where electron spin-lattice relaxation times (T_1e) and excited-state lifetimes are relatively long.

We decided to study GaAs/AlGaAs quantum well samples, i.e., samples produced by molecular beam epitaxy in which thin GaAs layers were separated by thicker AlGaAs layers. Due to the lower band gap energy in GaAs than in AlGaAs, the GaAs layers act as potential energy wells for conduction electrons, confining the conduction electrons to states that are essentially two-dimensional (2D) at sufficiently low temperatures.⁹ Strong optical pumping effects in GaAs had already been demonstrated,^10,11 interesting phenomena such as the integral and fractional quantum Hall effects were known to occur in GaAs/AlGaAs quantum wells,¹² and our colleagues Loren Pfeiffer and Ken West were willing to supply us with high-quality samples. Initially, we did not know precisely what we would learn from optically pumped NMR measurements on quantum wells. However, we thought we might find something new, simply because similar measurements on similar samples had not been attempted before.

Fig. 2a shows ⁷¹Ga NMR spectra of a quantum well sample at 1.6 K after various optical pumping times.^13,14 At short times, the spectrum contains one relatively broad line, arising from the GaAs layers where optical pumping occurs. At longer times, a second, sharper line grows in due to ⁷¹Ga-⁷¹Ga spin diffusion that transports optically pumped nuclear spin polarization to the AlGaAs layers. The frequency shift K between GaAs and AlGaAs lines is due to contact hyperfine couplings between ⁷¹Ga nuclei and electrons in the wells.

Figure 2.

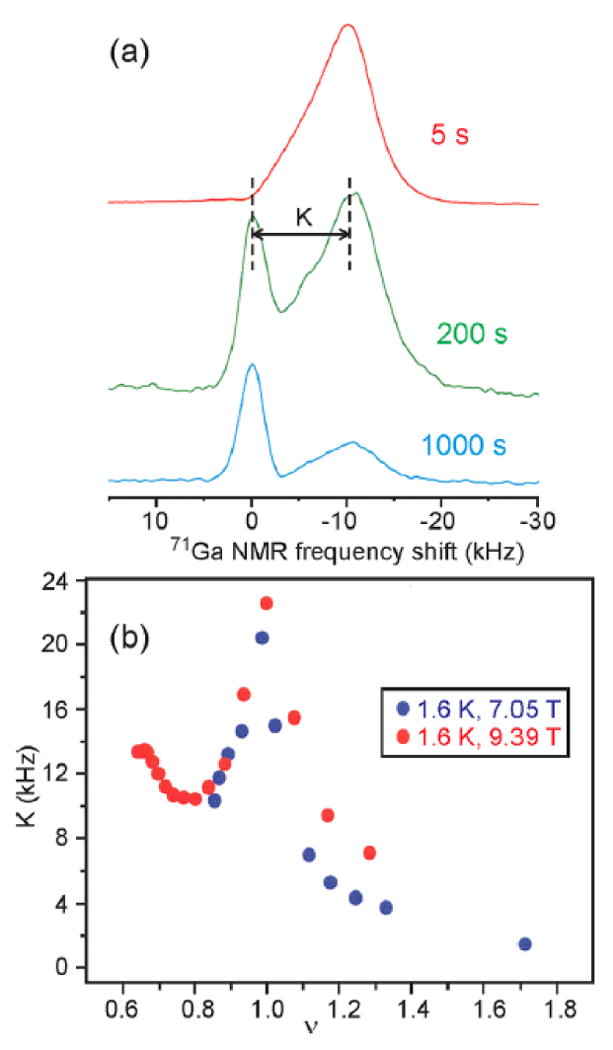
(a) ⁷¹Ga NMR spectra of a GaAs/AlGaAs quantum well (40 n-doped GaAs layers, 30 nm thick, separated by 180 nm AlGaAs layers, ∼0.3 cm² area) at 1.6 K at 7.05 T, with optical pumping at 806 nm wavelength for the indicated times after saturation of ⁷¹Ga spin polarization. (b) Dependence of the hyperfine shift K between GaAs and AlGaAs signals on Landau-level filling factor ν, varied by tilting the sample relative to the external magnetic field, at 7.05 T and 9.39 T.

Fig. 2b shows that K has a surprising dependence on a quantity called the Landau-level filling factor, or ν, which is the ratio between the actual number of electrons per unit area in a quantum well and the maximum number per unit area that can accommodated in a single “particle-in-a-box” state with the same spin direction.⁹ At ν = 1, K has its maximum value, indicating that all 2D electrons are fully polarized (i.e., have the same spin direction). But as ν becomes either slightly greater than or slightly less than one, K drops rapidly, indicating that many electrons flip their spins when a single extra electron is added or subtracted from the 2D electron system. In effect, each extra electron carries a spin substantially greater than 1/2. These unusual electron states arise from electron-electron interactions within the 2D electron system, and are called “skyrmions”. Data in Fig. 2b constitute the first experimental evidence that skyrmions exist in 2D electron systems at low temperatures and in strong magnetic fields.^13,14 These data stimulated a surge of subsequent experimental and theoretical studies of skyrmions in the quantum well research community.^15-17

Low-temperature NMR to immobilize proteins

In studies of proteins and other biopolymers, multidimensional solution NMR measurements constitute a powerful approach to full structure determination and detailed dynamical characterization of proteins and other biopolymers. However, these measurements become less informative as molecular weight increases and rotational diffusion slows down. In contrast, solid state NMR methods are applicable to biopolymers of arbitrarily high molecular weight (provided that some form of selective isotopic labeling is used, that natural-abundance ¹³C signals are not overwhelming, and that sufficiently high concentrations can be achieved). Thus, even for soluble proteins, it can be advantageous to freeze the sample and apply solid state NMR methods.

Fig. 3 shows one example, taken from our efforts to determine the conformations of peptides derived from the third variable (V3) loop of the HIV-1 gp120 protein when bound to anti-gp120 antibodies.^18,19 The V3 loop is of particular interest both because it is a primary target of antibodies that neutralize HIV-1 and because it interacts with chemokine receptors on host cell surfaces during the infection process. In Fig. 3a, the 2D ¹³C-¹³C spectrum of a free V3 peptide, uniformly ¹⁵N,¹³C-labeled at seven successive residues, shows relatively broad crosspeak signals in frozen glycerol/water at 153 K under magic-angle spinning (MAS), indicating substantial conformational disorder. In Fig. 3b, the 2D spectrum of the antibody-bound peptide shows much sharper signals, about 1.8 ppm wide (full width at half maximum), indicating a well-defined conformation in the peptide/antibody complex. From ¹³C chemical shifts and ¹³C-¹³C distance measurements, it was possible to develop a structural model for the epitope region of the antibody-bound V3 loop.¹⁹

Figure 3.

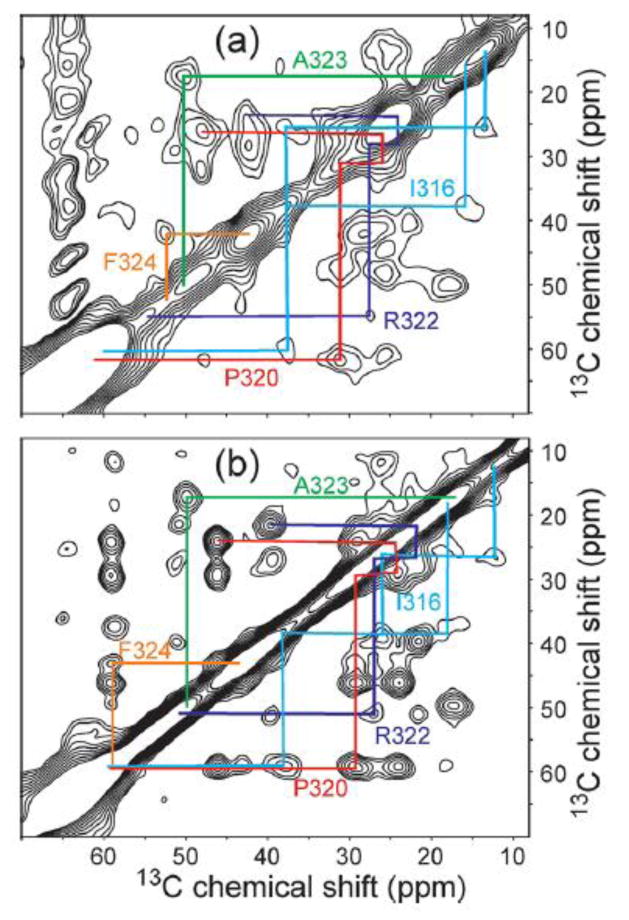
Aliphatic regions of 2D ¹³C-¹³C NMR spectra of a 20-residue peptide from the V3 loop of HIV-1 gp120 in the free (a) and antibody-bound (b) states, recorded in frozen solutions at 153 K and 9.39 T with MAS. The peptide is uniformly ¹⁵N,¹³C-labeled in seven consecutive residues, including the five non-glycine residues whose crosspeak signals are indicated by color-coded assignment paths.

Measurements in Fig. 3 were performed at 9.39 T, with 6 mm diameter MAS rotors and a 240 μl sample volume. The sample was cooled with cold N₂ gas, supplied to the sample space within the MAS module from the top of the magnet. To reduce the ¹H T₁ value to ∼1 s and thereby accelerate data acquisition, paramagnetic Cu²⁺ (20 mM CuNa₂EDTA) was added to the frozen solutions, as originally demonstrated by Weliky et al.¹⁸ With a 1.0 mM peptide/antibody concentration, the 2D spectrum in Fig. 3b was acquired in about 90 hr.

Similar measurement conditions were used to acquire the data in Fig. 4. In these experiments on the 35-residue villin headpiece subdomain (HP35), a popular model protein for folding studies,^20-23 we investigated the dependence of site-specific conformational distributions on the extent of unfolding induced by the denaturant guanidine hydrochloride (GdnHCl). In its fully folded state, HP35 is a three-helix bundle.²⁴ For solid state NMR measurements, HP35 samples were ¹³C-labeled at sequential pairs of backbone carbonyl sites; quantitative structural techniques developed in my laboratory^25-27 were used to place constraints on the backbone φ and ψ torsion angles between the labeled sites. The solid state NMR data revealed the specific conformational pathway shown in Fig. 4, in which helical segments present at [GdnHCl] = 0 convert first to mixtures of helical and extended conformations near the unfolding midpoint at [GdnHCl] = 4.5 M, then to mixtures of extended and polyproline II conformations in the fully unfolded state at [GdnHCl] = 7.0 M.²⁸ Identification of this conformational pathway was purely a consequence of the solid state NMR data, with no reliance on structural databases, prior expectations, or other measurements.

Figure 4.

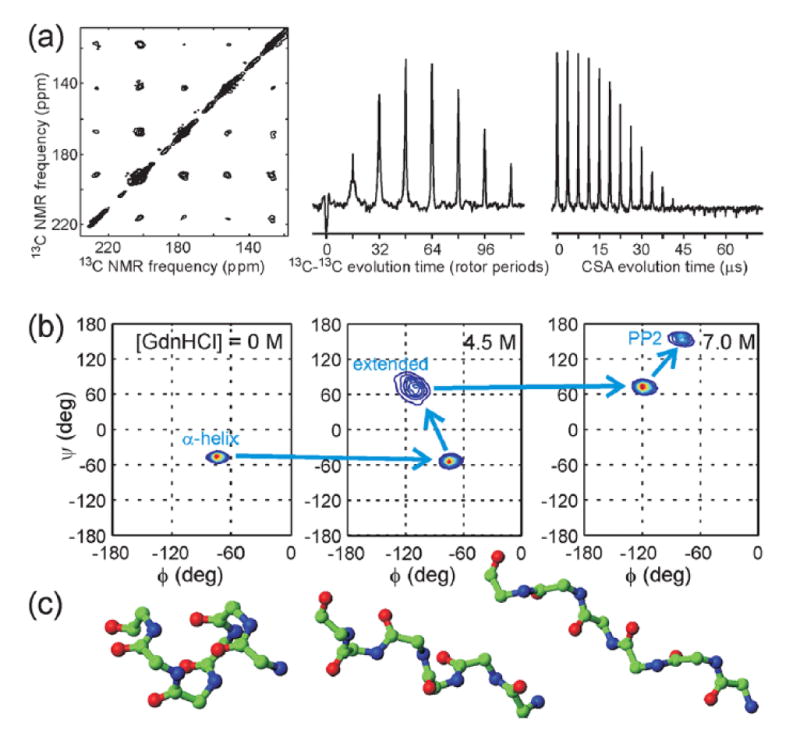
(a) 2D MAS exchange spectrum (left), constant-time double-quantum-filtered dipolar evolution data (center), and double-quantum CSA data (right) for HP35 in glycerol/water at 153 K. The protein is ¹³C-labeled at carbonyl sites of A49 and V50; the data place constraints on the backbone ϕ and ψ angles of V50. (b) Backbone conformational distributions at V50 for HP35 in frozen solution with indicated denaturant concentrations, derived from data as in panel a. (c) Models of a polypeptide backbone with the α-helical, extended, and polyproline II (PP2) conformations that are predominant components of the distributions in panel b.

These experiments on HP35 show how detailed structural information about unfolded states of proteins can be obtained from solid state NMR, information that is difficult to obtain from other experimental techniques. These experiments also illustrate how low-temperature NMR can be used to suppress conformational exchange in unfolded states (in addition to rotational diffusion), allowing conformational distributions to be characterized at a quantitative and site-specific level.

Low-temperature NMR to trap transient states

Partially and fully unfolded states created by addition of a chemical denaturant are equilibrium states. Transient, non-equilibrium states can also be created if solvent conditions, such as temperature, pH, ionic strength, or denaturant concentration, are changed rapidly from conditions that favor the unfolded state to conditions that favor the folded state. If a protein solution can be frozen on a time scale less than the structural equilibration time, then solid state NMR measurements can be used to characterize the protein structure in a transient intermediate state.

Fig. 5 shows examples of data from our initial demonstration of solid state NMR as a structural probe of transient states in a non-equilibrium protein folding process.²⁹ In these experiments, a glycerol/water solution of HP35 was heated to 363 K, creating a thermally unfolded state, then frozen by spraying a fine jet of the solution into a bath of isopentane at 128 K. Under our conditions, the time to cool from above 80° C to 0° C was 10-20 μs. Comparison of 2D ¹³C-¹³C NMR spectra of HP35 solutions that were frozen slowly from room temperature (Fig. 5a) with spectra of solutions that were frozen rapidly from 363 K (Fig. 5b) showed that the 10-20 μs freeze-quenching time was sufficient to trap an intermediate state. Distributions of crosspeak intensity in the 2D spectra showed that this intermediate state has about 60% of the helical secondary structure but not the full tertiary structure (i.e., ordered packing of the hydrophobic core) of fully folded HP35. In fact, no fully folded HP35 molecules were detected in spectra of the freeze-quenched intermediate state. This result is particularly interesting because previous studies of HP35 folding by optical spectroscopy²⁰ and recent computational studies²³ suggest a time scale less than 5 μs for folding (although under somewhat different conditions).

Figure 5.

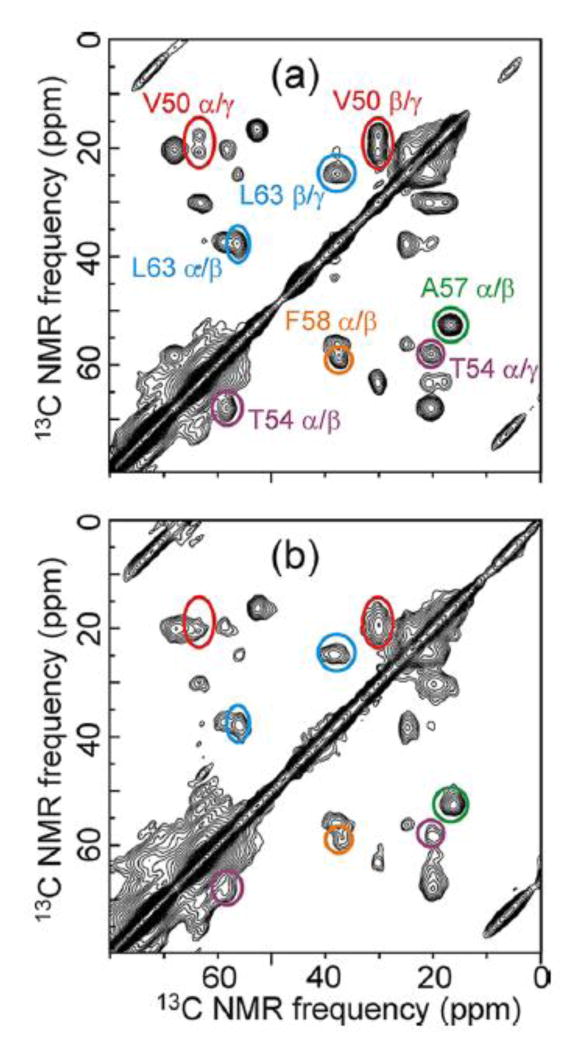
Aliphatic regions of 2D ¹³C-¹³C NMR spectra of HP35 in fully folded (a) and freeze-trapped intermediate (b) states, recorded in glycerol/water at 153 K and 9.39 T with MAS. Color-coded lines indicate signals that are unchanged (solid lines) or significantly shifted or broadened (dashed lines) in the partially folded intermediate state, for the uniformly ¹³C-labeled residues V50 (red), T54 (purple), A57 (green), F58 (yellow), and L63 (blue).

Even with Cu⁺² doping, a 200 μl sample volume, a 153 K sample temperature, and a 4 mM protein concentration, 2D spectra as in Fig. 5b required 100-240 hr of data acquisition. To make experiments of this sort more practical, further sensitivity enhancements are clearly needed.

Low-temperature NMR to enhance sensitivity

Nuclear spin polarizations at thermal equilibrium are proportional to 1/T, where T is the sample temperature. The noise amplitude from radio-frequency (rf) circuitry in the NMR probe is proportional to T^-1/2, if all circuit elements are at temperature T. The quality factor (Q) of the rf circuit generally increases with decreasing temperature. Therefore, the signal-to-noise ratio in an NMR spectrum, acquired with a given number of scans and a given sample size, is expected to increase by a factor of at least 15-60 if T is reduced from 300 K to 20 K. The main difficulty is that cooling to 20 K requires liquid helium, and most studies of biomolecular systems require MAS. MAS probe designs in which helium is used both to cool the sample and to supply gas to the MAS drive and bearing components suffer from high liquid helium consumption rates and relatively low MAS frequencies³⁰ unless MAS rotor volumes are small.³¹ We therefore chose to pursue an ultra-low-temperature MAS design in which nitrogen is used as the MAS drive and bearing gas, while helium is used only for sample cooling.

Fig. 6a shows the MAS system.³² We use zirconia MAS rotors with 4 mm outer diameter, 45.7 mm length, and a maximum sample volume of 82 μl. The low thermal conductivity of zirconia and the large rotor length allow the sample to be cooled to 20-25 K with helium, while the bearing and drive areas are well above 77 K. A teflon insert within the MAS module limits the helium-cooled volume to a minimal space around the rf coil. Cold helium from a liquid helium transfer line enters the MAS module from above and exits the sample area by flowing toward the bearings through the narrow gap between the rotor sleeve and the teflon insert. Vent holes near the bearings ensure that nitrogen bearing gas does not enter the helium-cooled volume. With this design, stable spinning at MAS frequencies up to 7.0 kHz is achieved with 20-25 K sample temperatures and liquid helium consumption rates of 2-4 l/hr. Rf performance is quite comparable to conventional MAS NMR probes. Rf arcing is not a serious problem, even during measurements that involve cross-polarization and ¹H decoupling at high powers, provided that helium gas is not allowed to escape from the sample space along the leads of the rf coil.

Figure 6.

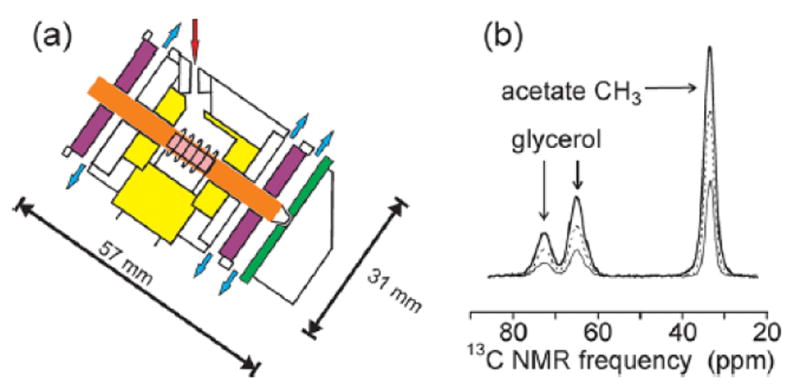
(a) Diagram of MAS module for ultra-low-temperature solid state NMR experiments. The rotor (orange) is supported by bearings (purple) and spun by a stator (green) that are driven by nitrogen gas, which exits as indicated by blue arrows. Cold helium (red arrow) enters the space around the rf coil and sample (pink) that is defined by the teflon insert (yellow) and exits along the outer surface of the rotor, toward the bearings. (b) ¹³C MAS spectra of ¹³CH₃-labeled sodium acetate in glycerol/water at 25 K (heavy solid line), 40 K (dashed line), and 79 K (thin solid line), recorded at 9.39 T with 7.00 kHz MAS.

Fig. 6b shows ¹³C MAS NMR spectra obtained at temperatures between 40 K and 79 K, demonstrating the 1/T dependence of signal amplitudes on sample temperature.³² In practice, only minor reductions in noise and increases in Q with decreasing temperature are observed, because only the rf coil (and not the rest of the rf circuitry) is cooled in our current probe design. Sample temperatures in Fig. 6b were determined from measurements of the T₁ of ⁷⁹Br in KBr powder, which can be measured quickly in a probe that tunes near the ¹³C NMR frequency and which decreases monotonically from 140 s to 2.6 s in the temperature range from 20 K to 60 K.³³ A less accurate, but nonetheless useful, assessment of sample temperature can be obtained from a fiber optic temperature sensor that is inserted into the helium-cooled volume within the MAS module.

¹H T₁ values for biomolecular samples near 20 K can be substantially greater than 10 s, especially for soluble, monomeric proteins in frozen glycerol/water solutions, where the effective ¹H T₁ is dominated by the T₁ of the solvent. Slow ¹H spin-lattice relaxation at low temperatures limits the rate at which data can be acquired, reducing the sensitivity advantage of low-temperature measurements. Near 20 K, the T_1e of Cu²⁺ becomes too long for Cu²⁺ to be an effective paramagnetic relaxation agent. As an alternative, we have found that Dy³⁺ in the form of DyH-EDTA at concentrations near 0.2 mM works well as a relaxation agent for monomeric proteins.³² On the other hand, even without paramagnetic doping, certain macromolecular assemblies such as amyloid fibrils may exhibit ¹H T₁ values of approximately 5 s near 20 K, presumably due to a large local concentration of methyl groups (or perhaps other chemical groups) with motional correlation times around 1 ns.

Dynamic nuclear polarization at ultra-low temperatures

Dynamic nuclear polarization is a phenomenon in which irradiation of electron paramagnetic resonance (EPR) transitions at microwave frequencies leads to enhancements of nuclear spin polarizations, and hence NMR signals, through cross-relaxation processes driven by electron-nucleus hyperfine couplings.^34,35 While my laboratory was developing the ultra-low-temperature MAS probe design described above, the M.I.T. group led by R.G. Griffin began to obtain quite promising results from their efforts to apply DNP to biomolecular solid state NMR with MAS at high magnetic fields.³⁶ Among their many contributions to DNP methods, the M.I.T. group showed that nitroxide radicals could be used as paramagnetic dopants in frozen solutions, leading to large enhancements of ¹H spin polarizations relative to thermal equilibrium values (i.e., DNP enhancement factors, ε_DNP) through the “cross effect” mechanism, especially when pairs of nitroxide moieties were combined within biradical dopants.^37,38 In addition, they showed that continuous-wave gyrotrons are effective sources of high-power microwaves at the EPR frequencies in high-field NMR magnets.³⁹

DNP generally requires that electron spin polarizations be perturbed from thermal equilibrium (i.e., partially saturated) by the applied microwaves, implying that the microwave fields must be strong enough to produce electron spin Rabi frequencies comparable to electron spin-lattice relaxation rates (1/T_1e). DNP experiments with MAS at M.I.T. were performed at 80 K or higher temperatures, where T_1e values for nitroxides are 0.1-1 ms.^40,41 Since we had constructed a MAS NMR probe that could operate in the 20-25 K range, we decided to explore the possibility of performing DNP experiments at ultra-low temperatures, where nitroxide T_1e values can be much greater than 1 ms.⁴² With longer T_1e, lower-power microwave sources could be used. In addition, intrinsic nuclear T₁ values (i.e., T₁ values in the absence of paramagnetic doping) are expected to be longer, at least in certain classes of samples, possibly leading to larger values of ε_DNP in cases where intrinsic nuclear spin-lattice relaxation competes with DNP processes. Finally, since ε_DNP represents polarization enhancement relative to thermal equilibrium, a given value of ε_DNP corresponds to larger NMR signals at 20-25 K than at 80-100 K.

Our initial DNP experiments were performed without MAS, at 9.39 T and temperatures in the 7-80 K range, using a tunable microwave source with a 30 mW maximum output.⁴³ Measurements on frozen glycerol/water solutions of various nitroxide compounds yielded values of ε_DNP for ¹H spins as large as 80 at 7 K. The largest values of ε_DNP and shortest DNP build-up times (T_DNP) were obtained with a tri-radical compound, called DOTOPA-TEMPO.⁴³ In our hands, the absolute DNP-enhanced ¹H NMR sensitivity at 20 K with 30 mW microwave power was comparable to the absolute sensitivity expected hypothetically at 80 K with ε_DNP ≈ 130 and T_DNP ≈ 1.4 s. Similar enhancements of cross-polarized ¹³C NMR sensitivity were observed in double-resonance DNP experiments at ultra-low temperatures.⁴⁴ These results encouraged us to construct a version of the ultra-low-temperature MAS probe that includes provisions for microwave irradiation for DNP.

Fig. 7 shows ¹³C MAS NMR spectra obtained recently with DNP at temperatures in the 20-25 K range, again at 9.39 T with a 30 mW microwave source.⁴⁵ One-dimensional spectra of ¹³C₃-L-alanine, dissolved in glycerol/water and doped with DOTOPA-TEMPO, show ε_DNP ≈ ±25 at 20 K (Fig. 7a). As predicted by theory and numerical calculations of cross-effect DNP under MAS,⁴⁶ the sign of the DNP-enhanced signal depends on the microwave frequency. The 2D ¹³C-¹³C spectrum of a model peptide (Fig. 7b) shows that high-quality 2D data can be obtained in about 2 hr from 82 μl of isotopically labeled peptides or proteins at 1.9 mM concentration.⁴⁵ Compared with the experimental conditions in Fig. 5, this represents a six-fold reduction in quantity of labeled sample and a 50-to-120-fold reduction in measurement time.

Figure 7.

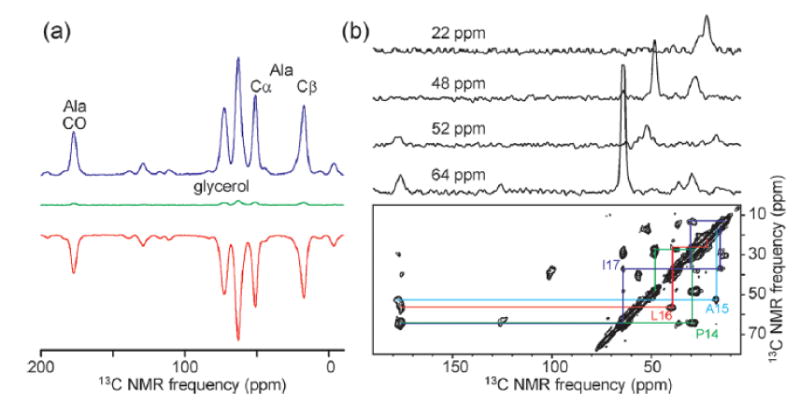
(a) ¹³C NMR spectra of 50 mM uniformly ¹³C-labeled L-alanine in partially deuterated glycerol/water with 10 mM DOTOPA-TEMPO, recorded at 20 K and 9.39 T with 6.7 kHz MAS. Spectra are shown with 30 mW microwave irradiation at 264.05 GHz (blue) and 264.65 GHz (red), and without microwave irradiation (green). (b) 2D ¹³C-¹³C spectrum of the 26-residue peptide melittin in partially deuterated glycerol/water with 9.9 mM DOTOPA-TEMPO, recorded at 25 K and 9.39 T with 6.4 kHz MAS. The peptide is uniformly ¹³C-labeled at P14, A15, L16, and I17. The peptide concentration is 1.9 mM, the sample volume is 82 μl, the recycle delay is 10 s, and the total measurement time is 2.2 hr.

Concluding remarks

Experimental examples discussed above illustrate some of the important motivations for pursuing NMR measurements at low and ultra-low temperatures, some of the techniques and technology that are associated with low-temperature experiments, and some of the likely directions for future research. In my laboratory, we are currently planning to apply ultra-low-temperature DNP with MAS to several structural problems in biomolecular systems, including freeze-trapped intermediate states in protein folding and peptide aggregation processes and membrane-associated peptide/protein complexes. The success of these specific efforts may depend on factors that are now under investigation, such as intrinsic ¹H T₁ values and ¹³C NMR linewidths under ultra-low-temperature DNP conditions. Further optimization of paramagnetic dopants and other aspects of sample preparation may be required. Modifications of our DNP apparatus to increase the available microwave power and to reach lower sample temperatures while reducing liquid helium consumption are also in progress. Through this combination of exploratory applications to biomolecular systems and ongoing technological developments, it seems likely that ultra-low-temperature NMR measurements will soon make important contributions to our understanding of systems that are of widespread interest outside the community of solid state NMR spectroscopists.