Effect of water activity on rates of serpentinization of olivine

Abstract

The hydrothermal alteration of mantle rocks (referred to as serpentinization) occurs in submarine environments extending from mid-ocean ridges to subduction zones. Serpentinization affects the physical and chemical properties of oceanic lithosphere, represents one of the major mechanisms driving mass exchange between the mantle and the Earth’s surface, and is central to current origin of life hypotheses as well as the search for microbial life on the icy moons of Jupiter and Saturn. In spite of increasing interest in the serpentinization process by researchers in diverse fields, the rates of serpentinization and the controlling factors are poorly understood. Here we use a novel in situ experimental method involving olivine micro-reactors and show that the rate of serpentinization is strongly controlled by the salinity (water activity) of the reacting fluid and demonstrate that the rate of serpentinization of olivine slows down as salinity increases and H₂O activity decreases.

Serpentinization of mantle rocks occurs in a variety of tectonic settings, but the controls on the rates of serpentinization are poorly constrained. Here, the authors developed an in situ experimental method to show that the rate of serpentinization is strongly controlled by the salinity of the reacting fluid.

Serpentinization encompasses a series of hydration reactions that occur when ultramafic rocks are exposed to circulating aqueous fluids at temperatures lower than ∼400 °C, leading to the formation of serpentine phases (lizardite and chrysotile)±brucite±talc±magnetite, among other minerals¹. Serpentinization affects the chemical composition, rheology, magnetic properties, seismic structure and habitability of the shallow lithosphere at slow- and ultraslow-spreading mid-ocean ridges, continental margins and forearc settings of subduction zones²,³,⁴,⁵,⁶,⁷,⁸. Serpentinization also influences subduction related processes⁹ and the geochemical cycling of volatile species (that is, H₂O, CO₂ and H₂S) and fluid-mobile elements¹⁰,¹¹,¹²,¹³. Recent findings suggest that serpentinization of olivine-rich lithologies also takes place on other planetary bodies, such as the icy moons of Jupiter and Saturn, which in turn, has important implications concerning their habitability¹⁴,¹⁵.

Despite the pivotal role that serpentinization plays in a number of geological and biological processes and its central role in current origin of life hypotheses¹⁶,¹⁷,¹⁸,¹⁹, few experimental studies have attempted to determine the rates of serpentinization reactions and the rates that have been reported diverge widely²⁰,²¹,²²,²³,²⁴,²⁵. Furthermore, the environmental factors that affect the reaction rates are incompletely constrained. In the present work, we used synthetic fluid inclusions (SFIs)²⁶,²⁷ as micro-reactors in olivine crystals to monitor the serpentinization reaction with time. We trapped fluid with different initial salinities and followed reaction progress at serpentinization conditions (280 °C). The results show that the rates of olivine serpentinization are strongly influenced by the aqueous fluid salinity. The micro-reactor technique presents several advantages and permits monitoring mineral precipitation and water activity in situ and in real time.

Results

SFI preparation

We trapped SFIs²⁶,²⁷ in gem quality (inclusion-free) natural olivine at 650 °C and 5.5 kbar, conditions where olivine and H₂O coexist stably (Fig. 1). The starting H₂O-NaCl-MgCl₂ fluids in each experiment had a Na/Mg molar ratio similar to that of seawater (8/1) and total salinities of 1, 3.5, 6 and 10 wt%. The capsules were sealed with an arc welder and then placed into cold-seal pressure vessels and held at the desired trapping conditions (650 °C and 5.5 kbar) for 30 days, which allowed the olivine host to anneal and trap some of the fluid as SFIs. The P–T conditions of trapping of the SFI were chosen such that the trapped fluid would have a density ∼0.8 g cm⁻³. Following inclusion synthesis, doubly polished wafers were prepared and individual wafers were placed into a furnace at 280 °C and ambient external pressure (∼1 bar)—at this temperature, olivine is no longer stable and will react with the aqueous solution to produce serpentinization. At a temperature of 280 °C and constant volume of the SFI, the calculated pressure inside the SFI is ∼500 bar.

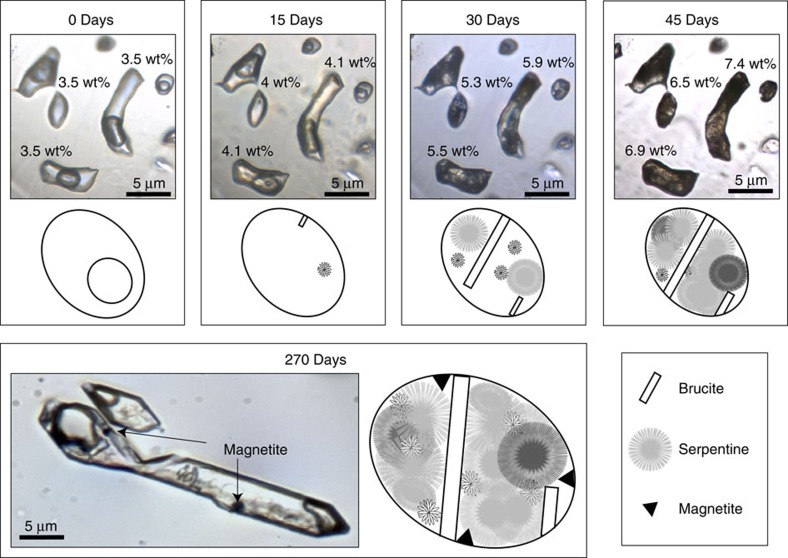
Figure 1. Photomicrographs and schematic illustrations of the evolution of the micro-reactors through time.

Photomicrographs of SFI taken before the serpentinization reactions were initiated (0 days) and after 15, 30, 45 and 120 days of reaction time at 280 °C. The images show the same group of SFIs that had an initial salinity of 3.5 wt%. The numbers next to individual SFIs in the 15-, 30- and 45-day images represent the measured salinity in wt%. The image for 120 days shows magnetite in an SFI that had an initial salinity of 1 wt%. The reaction progress is shown schematically below the photomicrographs. Before reaction the fluid inclusions only contain an aqueous solution and a vapor phase. After 15 days at 280 °C, small crystals of brucite and serpentine appear. After 30 days, more crystals have formed. After 45 days, the fluid inclusion is nearly filled with crystals. After 120 days, magnetite crystals appear.

The SFIs can be envisioned as micro-reactors, a reaction vessel in which material can only be exchanged between the aqueous solution and the surrounding host olivine. A major advantage of this approach over most other existing experimental techniques is that changes in mineralogy and fluid composition can be monitored simultaneously in real time as the reaction progresses.

Serpentinization experiments

Following the formation of the SFI in olivine, samples were heated to 280 °C and held at this temperature for 30 to 270 days. For each fluid composition and salinity, several SFI in each olivine wafer were selected to monitor serpentinization reaction progress (Table 1). Each inclusion was photographed and mapped, and each was regularly monitored optically, by microthermometry and by Raman spectroscopy. The polished olivine wafers were briefly (2–5 h) removed from the furnace every 5 days, examined petrographically and analysed by Raman spectroscopy, and then returned to the furnace. Figure 1 (0 days) shows a typical SFI trapped in olivine immediately before starting the heating experiments to initiate the serpentinization reaction.

Table 1. Overview of experimental conditions and alteration mineralogy.

Run	Fluid composition	S	Experiment type	T (°C)	t (days)	Alteration mineral assemblage
1	H2O-NaCl-MgCl2	1	Furnace	280 (±5)	120	S+B
2	H2O-NaCl-MgCl2	1	Chaixmeca	280 (±8)	120	S+B+M
3	H2O-NaCl-MgCl2	3.5	Furnace	280 (±5)	30	S+B
4	H2O-NaCl-MgCl2	3.5	Furnace	280 (±5)	60	S+B
5	H2O-NaCl-MgCl2	3.5	Furnace	280 (±5)	90	S+B
6	H2O-NaCl-MgCl2	3.5	Chaixmeca	280 (±8)	120	S+B+M
7	H2O-NaCl-MgCl2	6	Furnace	280 (±5)	120	S+B
8	H2O-NaCl-MgCl2	10	Furnace	280 (±5)	270	S+B+M

S, salinity in wt%.

Furnace, experiments in which sample was heated in a furnace and removed from the furnace and quenched to room temperature at various times for Raman and microthermometric analysis. As such, the sample was cycled from room temperature to 280 °C numerous times during the experiment.

Chaixmeca, experiments in a microscope heating stage whereby the SFI were kept at a constant temperature of 280 °C and the heating stage was placed onto the Raman microprobe at various times for analysis.

Mineral assemblages: B, brucite; M, magnetite; S, serpentine.

Reaction progress

After a few days of reaction time, small clusters of secondary minerals are observed in the SFI (Fig. 1, 15 days) and the size and number of individual crystals in the SFI progressively increased with time until the inclusions were nearly filled with solids, rendering them opaque (Fig. 1, 45 days).

The onset of the serpentinization reaction varied with the salinity of the starting fluid. Reaction products were first observed after 5 days in ∼50% of the SFIs with a starting salinity of 1 wt%. The proportion of the SFI showing evidence of reaction after 5 days decreased to ∼20, ∼5 and 0% in the SFI with starting salinities of 3.5, 6 and 10 wt%, respectively. At low salinities the proportion of SFI showing reaction products increased rapidly with time until they were ubiquitous in the SFI. For the highest salinity experiments (10 wt%), the first evidence of reaction product formation was observed only after >120 days.

In addition to the experiments in which samples were regularly removed from the furnace for observation and analysis, two samples containing SFI with salinities of 1 and 3.5 wt% were maintained at constant temperature using a Chaixmeca microscope heating stage²⁸. For these samples, the SFI could be monitored without having to cool the sample from 280 °C to room temperature. These experiments were conducted to confirm that repeatedly cycling the samples from 280 °C to room temperature and back to 280 °C did not significantly affect the progression of the experiments. No differences were observed between the thermal cycling experiments and the constant-temperature Chaixmeca experiments.

Mineral characterization

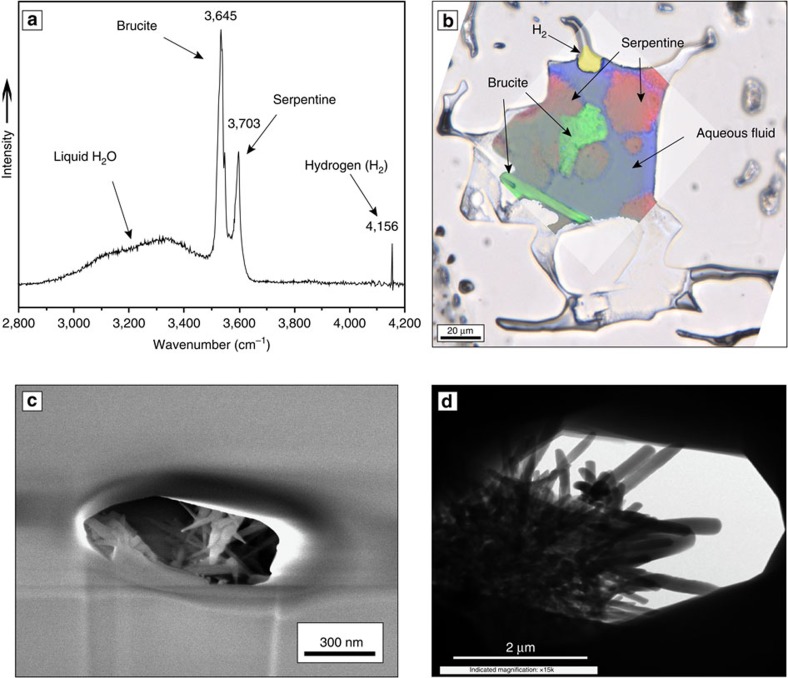
Raman spectroscopic analyses of the SFI (Fig. 2a,b) confirmed that the reaction products consist of serpentine minerals (Srp, lizardite and chrysotile) and brucite (Brc) produced according to the simplified reaction (note that Fe can substitute for Mg in all three mineral phases, but the reaction below is written in terms of the Mg endmembers):

Figure 2. Characterization of the reaction products.

(a) Raman spectrum showing characteristic O–H bands of serpentine (lizardite) and brucite, the broad band for liquid H₂O and H₂ in the vapour bubble. (b) Raman map of the SFI: serpentine shown in red, brucite in green, aqueous solution in blue and H₂ in yellow. (c) Reaction products attached to the wall of an SFI imaged after the SFI was excavated by FIB. (d) TEM image of an SFI showing the fibrous and conical shapes of serpentine (chrysotile) crystals.

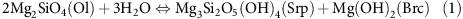Magnetite is a minor but common byproduct of serpentinization reactions. It forms when the Fe²⁺ released from olivine is oxidized in the fluid and precipitates magnetite and generates H₂:

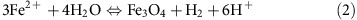In our experiments, magnetite was present in detectable amounts only after >120 days of reaction time. Small magnetite crystals, formed via reaction (2) (but not detectable by optical microscopy) should begin to precipitate inside the micro-reactors soon after reaction (3) starts and the solubility of magnetite in the solution is exceeded. However, we interpret the lack of observable magnetite in the inclusions early in the experiments to reflect the fact that the crystals are not optically resolvable (∼0.5 μm) by the methods used here (including scanning electron microscopy (SEM) and transmission electron microscopy (TEM) in Fig. 2c,d). In order for magnetite to become sufficiently large that they can be recognized either optically or during microanalysis, hydrogen must be lost from the SFI. The relatively long reaction time before magnetite is recognizable with optical microscopy in the SFI is attributed to its relatively sluggish precipitation, which may be further limited by competition for iron by serpentine and brucite, or by the slow rate at which hydrogen diffuses out of the inclusions²⁹ when (or if) the inclusion approaches equilibrium.

Mineral composition

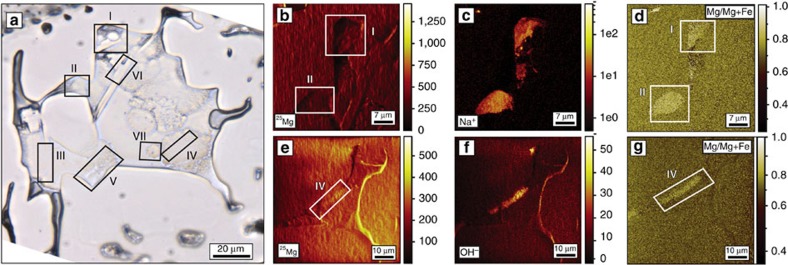
The chemical composition of the reaction products was inferred from elemental maps obtained from time-of-flight secondary ion mass spectrometry (TOF-SIMS) analyses of the SFI (Fig. 3 and Supplementary Tables 1 and 2). The ratio of Mg to Fe, defined as the Mg number (Mg#=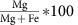), of brucite is 87–88, whereas serpentine phases have an Mg# ranging from 90 to 94. These values are similar to those of secondary minerals produced during serpentinization of olivine reported from other experimental studies²⁵.

Figure 3. TOF-SIMS elemental maps of the SFI from Fig. 2b.

(a) Photomicrograph of the SFI. (b–g) Elemental maps of different regions of the SFI, labelled I to VII, to show the ROI (regions of interest) where the TOF–SIMS analyses were performed (Supplementary Table 2). (b–d) ²⁵Mg⁺, Na⁺ and ²⁵Mg/(²⁵Mg+Fe) maps showing compositional differences between the host and the reaction products, labeled as ROI I and II. The ²⁵Mg/(²⁵Mg+Fe) map is obtained from the secondary ion yield map ²⁵Mg⁺/(²⁵Mg⁺+Fe⁺) by using a multiplication factor that ensures the olivine background yields a ratio of 0.8. (e–g) ²⁵Mg⁺, OH⁻ and ²⁵Mg/(²⁵Mg+Fe) maps showing compositional differences between the host and the reaction products labeled as ROI IV. The Na⁺ is contained in the frozen aqueous phase in the SFI. (f) OH⁻ maps showing the presence of serpentine and H₂O ice. (g) ²⁵Mg/(²⁵Mg+Fe) map of ROI IV.

Discussion

The serpentinization reaction, even in its simplest form (equation (1)), is the result of several simultaneous reactions that can be summarized as follows. First, olivine dissolves:

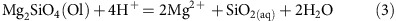When the concentrations of dissolved species become sufficiently high, serpentine and brucite nucleate and precipitate according to:

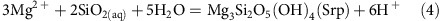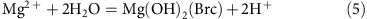Reactions (4) and (5) consume water and generate hydrogen ions (H⁺) that in turn promote forsterite dissolution (reaction 3). Eventually, the overall process reaches a steady state whereby the rates of Mg and SiO_2(aq) released from olivine dissolution (reaction 3) are balanced by their rates of consumption by serpentine (reaction 4) and brucite (reaction 5) formation.

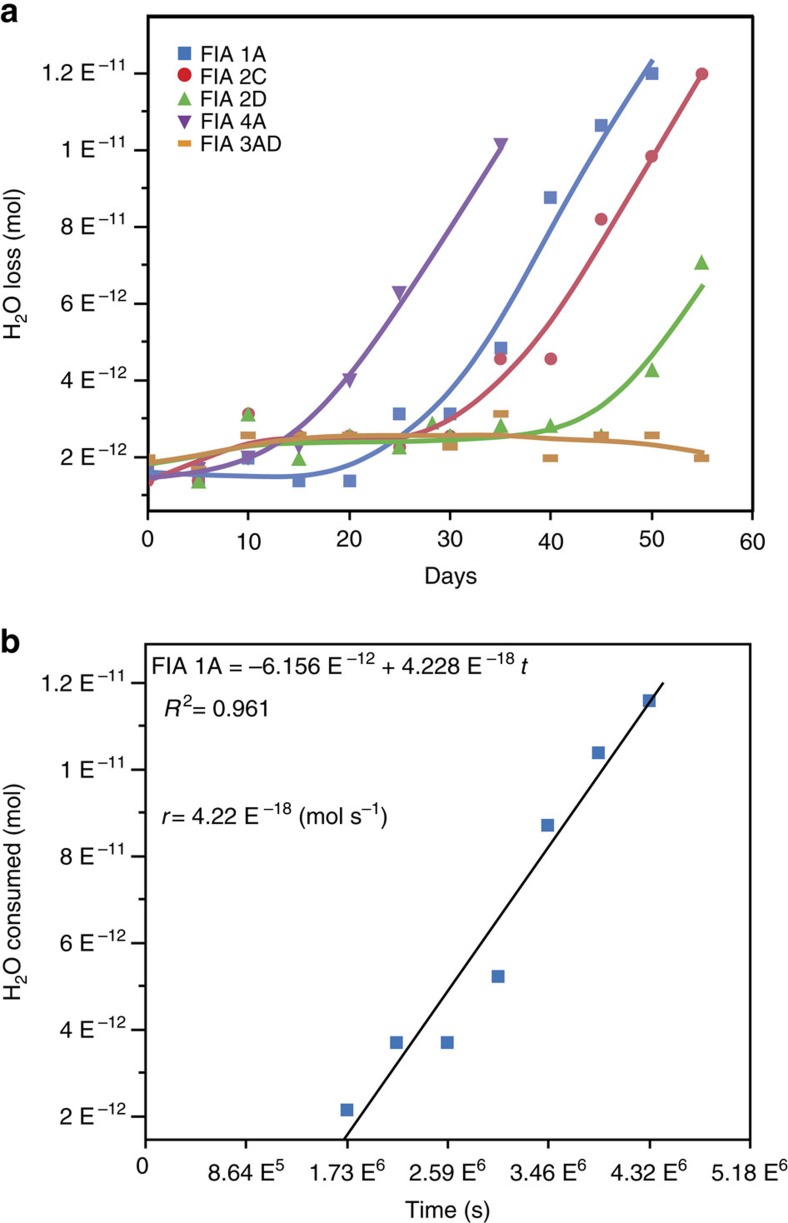
As the formation of serpentine and brucite consumes H₂O, the salinity of the aqueous solution in SFI increases as the reaction proceeds. We used the increase in salinity as a proxy for H₂O consumption and reaction progress, to quantify the reaction rates (mol m⁻² s⁻¹) (Fig. 4a,b, Supplementary Table 3 and Supplementary Data 1 and 2). The reaction progress is expressed as the extent of reaction (ξ), defined as the number of moles (n) of reactant consumed or product generated per unit time and normalized by the stoichiometric coefficient (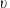) for the phase being consumed or produced. Therefore, the reaction rate of a species 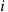is defined as the time (t) derivative of the extent of reaction:

Figure 4. Reaction progress as a function of H₂O consumed through time.

(a) Example showing the moles of H₂O consumed as a function of time for five SFI micro-reactors from Run 4 (3.5 wt% H₂O-NaCl-MgCl₂ starting salinity). Solid lines are drawn as references to identify data from individual SFI. (b) Moles of H₂O consumed versus time for SFI FIA1A from run 4. The initial rate method was applied for each SFI starting from the time at which the reaction started. The slope of the fitted line represents the rate of reaction (r).

As such, the reaction rate can be defined in terms of the rate of change in the amount of any of the species that participate in the reaction, that is,

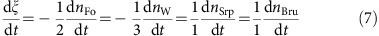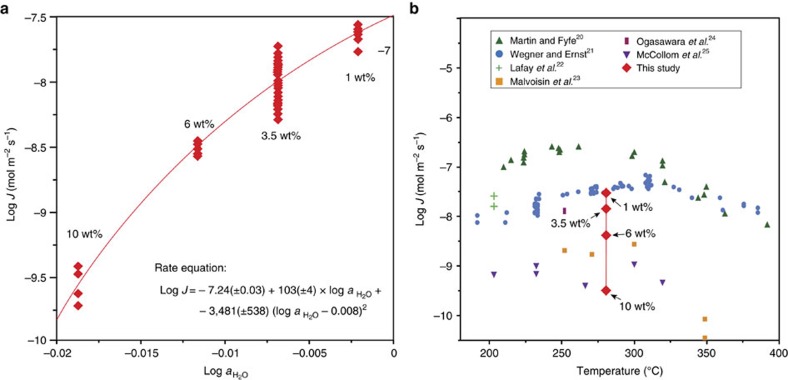
where the subscripts Fo, W, Srp and Bru correspond to the phases forsterite (olivine), water, serpentine and brucite, respectively. Figure 5a shows the rate of serpentinization as a function of the activity of water. The average rates of reaction were determined to be 2.71 × 10⁻⁸, 1.17 × 10⁻⁸, 3.37 × 10⁻⁹ and 2.86 × 10⁻¹⁰ mol m⁻² s⁻¹ for the SFIs with starting salinities of 1, 3.5, 6 and 10 wt%, respectively (Supplementary Table 3). The results show that serpentinization rates decrease rapidly with increasing salinity (or decreasing activity of water), and agree with previous studies that have reported that dissolution rates of forsterite at laboratory conditions (25 °C and 1 bar) decrease as 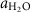decreases³⁰. A rate equation was generated by fitting the log of the reaction rates as a function of log 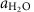with a second-order polynomial:

Figure 5. Experimentally determined serpentinization rates.

(a) Logarithm of the serpentinization rate (red diamonds) as a function of the logarithm of the activity of water. Each red diamond represents the result obtained from an individual SFI. The rate equation predicts a log rate constant of −7.24 and an apparent reaction order of 103. (b) Comparison of results of this study at 280 °C with experimentally determined reaction rates from the literature²⁰,²¹,²²,²³,²⁴ plotted as a function of temperature. Most of the previous experimental studies have used pure water²⁰,²¹,²³,²⁴ and a few others used different fluid compositions²²,²³,²⁵.

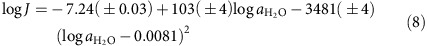where −7.24 is the log of the rate constant at 280 °C and the coefficient 103 represents the apparent reaction order of H₂O. The serpentinization rates obtained here agree with the rates of reaction from Wegner and Ernst²¹ after extrapolating the results to unit activity (pure water) with a log J of −7.51 (Fig. 5b).

These results document the dramatic effect of salinity, as a proxy for water activity 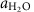, on the rate of serpentinization of olivine and are consistent with previous studies that have suggested that salinity can have a significant effect on dissolution kinetics³¹,³²,³³ including the dissolution of forsterite at far from equilibrium conditions³⁰,³⁴. The effect of water activity 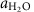on the reaction rate is consistent with the generalized serpentinization reaction given by equation (1), in which the driving force for production of serpentine and brucite is inversely proportional to the cube of the activity of H₂O and is also consistent with the dissolution of olivine (equation (3)) being the rate controlling step of the reaction²³.

These results provide a geochemical basis to support the previously recognized notion that pervasive serpentinization of oceanic lithosphere (which is dominated by the mineral olivine) requires open-system behaviour, as imprinted by the seawater-dominated isotope signature commonly observed in serpentinized peridotites³⁵,³⁶,³⁷,³⁸. In particular, this requires continual influx of a lower salinity aqueous fluid (seawater) to dilute the serpentinization fluid and allow serpentinization of olivine to continue³⁹. Moreover, salinity may be a major rate-limiting factor where fluid influx is restricted due to the absence of open fractures and fluid migration proceeds along grain boundaries or within nanoscale porosity⁴⁰,⁴¹,⁴². Furthermore, the effect of salinity on the serpentinization process places constraints on the environments in which serpentinization is likely to occur on Earth and possibly on other planetary bodies where high salinity fluids are believed to exist⁴³,⁴⁴,⁴⁵.

The micro-reactor technique used in this study provides a novel and promising tool to monitor fluid-rock reactions in situ and in real time and can be applied to a wide variety of host minerals, reaction products, temperatures and different starting fluid compositions (for example, CaCl₂, CO₂, SO₄ and Al₂O₃).

Methods

SFI preparation

SFIs were trapped in gem quality (inclusion free) natural olivine crystals (Fo85) at 650 °C and 5.5 kbar following procedures described by Sterner and Bodnar²⁶, and similar to Bali et al.²⁷. The crystals were thermally fractured by heating to 700 °C in an oven at atmospheric pressure and then quenching by dropping the crystals into cold distilled water. The crystals were dried overnight in a vacuum oven at 110 °C. Next, one or two 3–5 mm in diameter crystals of olivine were loaded into a platinum capsule (2.5 × 0.5 mm) together with ∼100 μl of an H₂O-NaCl-MgCl₂ fluid (Na/Mg weight ratio of 8/1) with salinities of either 1, 3.5, 6 or 10 wt%. The capsules were sealed with an arc welder. Platinum capsules were placed into cold-seal pressure vessels and held at 650 °C and 5.5 kbar for 30 days, which allowed the olivine host to anneal and trap some of the fluid it was in contact with as synthetic secondary fluid inclusions that were then used as micro-reactors in this study. After the fluid inclusion synthesis, the capsules were cooled to room temperature and the crystals removed from the capsules and mounted in thermal cement for cutting (∼100 to 200 μm wafers) and polishing. The sizes of the SFI varied between ∼1 and 40 μm, with most being ∼10 μm in diameter.

Raman characterization

Raman analyses were performed using a JY Horiba LabRam HR (800 mm) spectrometer, 600 grooves per mm grating, slit width of 150 μm and the confocal aperture was set at 100 μm. A 514.529 nm (green) Laser Physics 100S-514 Ar+ laser was used with a 50 mW output power at the source and ∼10 mW at the sample, focused through either a × 40 or × 100 objective, and an electronically cooled open electrode 1,024 × 256 pixel CCD (charge-coupled device) detector. Raman X–Y mapping was conducted using a JY Horiba LabRam HR confocal Raman spectrometer with a 473 nm laser source and a 600 grooves per mm grating and a 100 μm confocal aperture and × 100 objective.

Electron microprobe analyses

The composition of the olivine host crystal (Supplementary Table 1) used in the experiments was determined using a Cameca SX-50 electron microprobe (EMPA). Accelerating potential was 15 kV, 20 nA current and 1 μm beam size, using natural and synthetic mineral standards for calibration.

Mineral and chemical characterization

To expose the reaction products in the SFI to obtain SEM images, we used a Helios 600 NanoLab FIB-SEM. Chemical analyses of the contents of the SFI after the serpentinization experiments were conducted using TOF-SIMS with a TOF.SIMS 5 IONTOF instrument⁴⁶,⁴⁷,⁴⁸. At each step during the analysis, the ion beam consisting of Bi₁⁺ ion pulses (30 keV ion energy) was first set in the high current bunched mode (20 ns pulse duration, ∼3.7 pA measured sample current, ∼1 μm lateral resolution) to determine the masses of interest with high mass resolution. The beam was typically raster-scanned over areas of 100 × 100 μm or 200 × 200 μm depending on the region of interest. Then, the beam was set in the burst alignment mode (100 ns pulse duration, ∼0.03 pA measured sample current, ∼200 nm lateral resolution) to produce high spatial resolution maps of the selected regions for all masses of interest. To expose the SFI in the olivine hosts at various depths we used an O₂⁺ sputtering ion beam (1 keV ion energy, ∼300 nA measured sample current) that was raster-scanned over an area of 500 × 500 μm and centred over the region of interest. The resulting sputtering rate was calculated at 0.3 nm s⁻¹ by using a Wyko NT 9100 optical profilometer to estimate the sputtered crater depth. The sample was maintained at a base temperature of −100 °C at all times during the analysis by inductive liquid nitrogen cooling to prevent the water from desorbing from the exposed SFI. A constant current (21 eV electron energy) electron beam was shot on the sample during data acquisition to reduce sample charging. The mass resolution was >3,000 and >100 (m dm⁻¹) for the high current bunched and burst alignment modes, respectively. The TOF-SIMS provided ratios of the number of counts for one mass (isotope) to the number of counts for some other isotope and these counts were converted into a concentration ratio. To do this, the count ratio for Mg⁺ and Fe⁺ were converted into concentration ratios using the known Mg to Fe concentration ratio of the olivine host obtained from EMPA and the count ratio obtained by TOF-SIMS analyses for this same area of the crystal (see Supplementary Table 2).

TEM analyses

The sample was polished to a thickness of 30 μm using an Allied Multiprep with 3M Imperial diamond lapping papers of 30, 15, 6, 3 and 1 μm grit sizes. After polishing, the sample was mounted on a copper aperture grid with epoxy. Then the sample was milled in a Fischione 1,010 low-angle argon ion mill at ∼10 μm h⁻¹ for several hours until the SFI were exposed. Areas immediately adjacent to the hole became thin enough to be electron transparent. The sample was placed in a JEOL single tilt holder. TEM images were collected at Virginia Tech on a JEOL 2100 TEM operated at 200 kV, using a Gatan Orius CCD camera.

Monitoring reaction progress

The salinity of the SFIs was monitored by measuring the freezing point depression (FPD) of the aqueous phase (temperature of melting of the last ice crystal, T_m) using a Linkam THMSG 600 °C heating and freezing stage. During the serpentinization reaction H₂O was removed from the solution and incorporated into the reaction products, whereas Na⁺ and Cl⁻ remained in the solution. Trace amounts of Cl⁻ can be incorporated into serpentine, substituting for OH⁻ (0.1 to 0.4 wt%), but this is considered to have a negligible effect on salinity in the experiments. Each measurement was corrected for analytical error by measuring the T_m of a pure H₂O SFI. The correction factor ranged from 0.8 to 1.0 °C and the precision of the FPD measurements is ∼±0.1 °C. The salinity (wt% eq. NaCl) as a function of the FPD was calculated using FPD salinity equation⁴⁹. The triple point of pure H₂O occurs at 0.01 °C and 0.006 bars, where liquid H₂O, vapour H₂O and solid H₂O (in this case, the Ice I polymorph) occur in equilibrium. With increasing salt concentration, the triple point migrates to lower temperature and this effect is referred to as the FPD of the solution. FPD is a colligative property that depends only on the ratio of the number of moles of the solute (NaCl–MgCl₂ in our experiments) to that of the solvent H₂O. Thus, as H₂O was transferred from the aqueous solution into product phases, the ratio of moles of solute to moles of solvent (H₂O) increased and the temperature of the triple point decreased. The relationship between the salinity (moles of solute in the solution) and FPD has been determined using data for the ternary system H₂O-NaCl-MgCl₂ (refs ^{49, 50}).

In our experimental method, we quantify the rates of serpentinization based on the amount of water removed from the aqueous solution and incorporated into hydrous phases. We use the FPD of the aqueous solution as a proxy for the amount of water removed from solution and the precision of our FPD measurement is ±0.1 °C. However, some amount of H₂O must be removed from the solution and incorporated into hydrous phases before the salinity is increased by an amount sufficient to produce a 0.1 °C lowering of the FPD. The actual amount of H₂O that must be removed from solution to produce a 0.1 °C lowering of the FPD is also a function of the starting salinity. Thus, for example, if the initial salinity is 1 wt% NaCl (FPD=−0.6 °C), ∼15% of the H₂O in the initial fluid must be consumed by hydration reactions to drive the FPD lower by 0.1 °C to −0.7 °C (corresponding to a salinity of ∼1.2 wt% NaCl). However, the same 0.1 °C lowering of the FPD for a starting salinity of 3.5 wt% NaCl requires the consumption of 4.7% of the H₂O in the initial fluid, 6 wt% NaCl initial salinity requires a consumption of 2.6% of H₂O and 10 wt% NaCl initial salinity requires consumption of only 1.4% of the initial H₂O, as the FPD is lowered from −6.6 °C (10 wt% NaCl) to −6.7 °C (10.1 wt% NaCl). Thus, until a sufficient amount of reaction has occurred to consume enough H₂O to lower the FPD by ≥0.1 °C, in the absence of observed reaction products in the inclusions we have no way of knowing a priori that the reaction has started and, depending on the starting salinity, the amount of H₂O consumed (and, therefore, the amount of reaction that must occur) could require from ∼1 to 15% of the initial H₂O to be removed from solution. All of the microthermometric data (FPD, salinity and H₂O moles consumed) are provided in Supplementary Data 1.

Salinity changes and H₂O consumed during serpentinization

To calculate the mass of H₂O consumed by the serpentinization reaction as a function of the FPD, we constructed a simple mass balance model that relates the mass of H₂O (moles) removed from the aqueous fluid during the reaction to the FPD. The mass of H₂O (g) contained in a 10 μm diameter SFI was calculated for 0.1 °C increments of FPD as follows. The total mass of solution in the SFI is given by:

where V_SFI is the volume of an ideal spherical fluid inclusion (5.24·10⁻¹⁰ cm³) and ρ_SFI is the density of the solution in the SFI (in g cm⁻³). The density of the aqueous solution in the SFI was calculated using the HOKIEFLINCS H2O_NaCl programme⁵¹ and assuming that the system H₂O-NaCl provides a reasonable approximation for the relationship between salinity and density for the fluids in the SFI. Accordingly, the densities at 650 °C and 5.5 kbar for salinities of 1, 3.5, 6 and 10 wt% NaCl are ∼0.84, ∼0.87, ∼0.88 and ∼0.90 g cm⁻³, respectively. The masses of NaCl and H₂O in the SFI are given by:

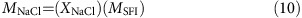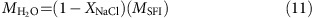where M_Nacl and 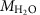are the masses of NaCl and H₂O, respectively, in the SFI in grams and X_Nacl is the mass fraction of NaCl (wt% NaCl/100) in the solution.

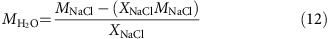where 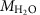is the mass of H₂O (g), M_Nacl is the mass of NaCl (g) and X_Nacl is salinity of the fluid in terms of weight fraction of NaCl.

The amount of H₂O consumed by the reaction is estimated from the measured FPD as follows. First, the mass of H₂O in the SFI corresponding to the initial salinity and measured FPD is calculated according to equation (12). Then, the amount of H₂O in the SFI after some amount of reaction has occurred to lower the FPD by some measureable amount (corresponding to an increase in salinity) is calculated. The loss of H₂O from the solution to the product phases corresponding to a given change in salinity was then calculated:

where, M_w,0 is the mass of H₂O in grams at t=0 and M_w is the mass of H₂O obtained from equation (9). The number of moles of H₂O consumed n_w was then calculated as:

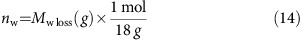For every initial salinity (1, 3.5, 6 and 10 wt%), the number of moles of H₂O consumed was fit as a polynomial expression to determine the number of moles of H₂O consumed as a function of the salinity of the SFI. For every FPD measurement in an individual SFI, the number of moles of H₂O leaving the system was calculated. All calculations and procedures are provided in Supplementary Data 2.

Rates of reaction and rate equation

Reaction progress was followed in several dozen SFI by monitoring the change in the FPD through time. To follow the reaction progress it was necessary to observe the changes inside the SFI. As reaction progressed, the amount of reaction products increased rendering the inclusion opaque and challenging our ability to follow the reaction progress at high extents of reaction. Moreover, as the H₂O inside the SFI is consumed by reaction (1), the internal pressure and volume, as well as the concentrations and compositions inside the inclusion, will be modified from the initial conditions. To avoid the problem of visibility and the effects of changing of the fluid compositions and pressure inside the SFI micro-reactors, our observations where analysed using the initial rate method⁵². Batch experiments usually require data collection that includes large extents of reaction to circumvent issues related to modifications of the initial conditions (that is, composition, pH, pressure and so on)⁵³. Alternatively, the initial rate method uses numerous short-term experiments with a small number of concentration measurements at equally spaced times. Then, the concentration data versus time data for each experiment are fitted to a function and the slope of that function (rate) is extrapolated to time=0. In our experiments, at time zero little or no brucite+serpentine had formed and no significant water was consumed. The small volume and pressure changes associated with this small extent of reaction did not affect the result. As such, in our experiments, the number of moles of H₂O consumed by the serpentinization reaction with time was found from the change in n_w with time:

where r is the rate of H₂O consumption in mol s⁻¹, Δn_w is the number of moles of H₂O consumed by the transformation reaction and Δt is the time in seconds. The number of moles of H₂O removed from solution and incorporated into hydrous phases as a function of time (t) for every SFI was fit by a linear regression and the rate of reaction was taken as the slope of the regression line⁵³,⁵⁴ (see Fig. 4).

As the reaction occurs at an interface that separates two phases (solid and liquid), the rate expresses how fast a component is transferred to or from that interface and the area of the interface must be taken into account as follows:

where J is the flux of H₂O from the liquid phase into the product phases, defined as the rate of H₂O consumption per unit surface area (mol m⁻² s⁻¹), 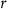is the rate of H₂O consumption in mol s⁻¹ and A is the surface area of a 10 μm spherical fluid inclusion (1.26 × 10⁻⁹ m⁻²) (Supplementary Data 2). To generate rate equations, rates obtained as described above were converted into rate equations that summarize the effects of solution compositions⁵². These experimental rates were fit to an equation that relates the rate to the concentration (a, activity) of H₂O, i, raised to a power (n).

This equation was transformed to a second order polynomial form:

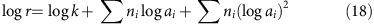The data were fit to an equation of this form using a polynomial function to determine the rate constant (k) and the apparent reaction order (n). The activities (a_i) used in this study were calculated with the software code EQ3/6 (ref. ⁵⁵) using the thermodynamic database for 50 MPa as described in Klein et al.⁵⁶. Data for the rates, fluxes, activities and extent of reaction of all the experiments are provided in Supplementary Table 3.

Serpentinization rates from the literature

To compare the serpentinization rates obtained in this study with the rate data available in the literature, we converted the published data²⁰,²¹,²²,²³,²⁴,²⁵,⁵⁷ into fluxes (mol m⁻² s⁻¹) by applying the Shrinking Particle Model⁵²,⁵⁸. This model assumes that all particles are spheres and the rate of dissolution equals the rate of conversion. As such, the conversion rates (olivine to serpentine and brucite, per day from the literature) were converted into fluxes (mol m⁻² s⁻¹) by determining the rate constant following expressions:

where α is the conversion rate fraction, k_p is the rate constant and t is time (s).

where k₊ is the flux (mol m⁻² s⁻¹), R₀ is the particle size radius at time zero and V_m is the molar volume of olivine. R₀ was obtained by using the averaging method of Tester et al.⁵²,⁵⁹, which finds the effective diameter of the grain sizes by the following method:

where D_e is the effective diameter (m), D_max is the maximum grain size and D_min is the minimum grain size diameter.

Data availability

The data that support the findings of this study are available from the corresponding author upon reasonable request.

Additional information

How to cite this article: Lamadrid, H. M. et al. Effect of water activity on rates of serpentinization of olivine. Nat. Commun. 8, 16107 doi: 10.1038/ncomms16107 (2017).

Publisher’s note: Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.