Abstract
We describe a first-and-second-diffractive-order intraocular lens ((1st,2nd)DIOL) within the class of hybrid refractive-diffractive designs for intraocular lenses (IOLs) and analyse its properties of focus extension and compensation of longitudinal chromatic aberration (LCA), particularly for lenses with low addition. Power, energy efficiency and their wavelength dependence are extended from monofocal IOL and conventional bifocal zeroth-and-first-diffractive-order IOL ((0th,1st)DIOL) to (1st,2nd)DIOL of low addition. Compensation of LCA is experimentally assessed in optical bench through the through-focus energy efficiency of three Tecnis IOLs with red, green and blue illuminations: ZA9003 (monofocal), ZKB00 (bifocal (0th,1st)DIOL with + 2.75 D add) and Symfony ZXR00. We prove Tecnis Symfony ZXR00 IOL can be considered an example of (1st,2nd)DIOL design of low addition, with LCA compensation in both the distance and intermediate foci, whereas the bifocal (0th,1st)DIOL does not compensate in the distance focus. However, the energy efficiency of (1st,2nd)DIOL for wavelengths other than the design wavelength is markedly more asymmetric.
OCIS codes: (260.0260) Physical optics; (330.7335) Visual optics, refractive surgery; (170.4460) Ophthalmic optics and devices; (050.1965) Diffractive lenses; (220.0220) Optical design and fabrication; (220.1010) Aberrations (global); (220.1000) Aberration compensation; (120.3620) Lens system design; (120.4800) Optical standards and testing; (120.4820) Optical systems
1. Introduction
In cataract surgery, an intraocular lens (IOL) replaces the natural crystalline lens. IOL power calculations of monofocal implants commonly target distinct distance vision. However, visual requirements at closer distances cannot be satisfied by monofocals and, similarly to the case of presbyopia, patients become spectacle dependent to perform their ordinary activity. Multifocal IOLs offer one (bifocals) or two (trifocals) additional focusing distances for near and intermediate vision. Diffractive multifocal IOLs (more precisely, refractive-diffractive multifocal IOLs) are based on the simultaneous image forming principle for which an in-focus image formed by one focus is overlaid by one or more secondary out-of-focus images formed by the other foci. This effect has an impact on the image contrast and is among the causes of dysphotopsia (such as halo and glare) reported by implanted patients. Recently, IOL designs based on extending the range of focus have been proposed to address the issue. Ideally, an extended-depth-of-focus (EDOF) lens produces a long and narrow focal segment in the image domain. An IOL of such a design would allow imaging in a continuous range of vision. The assessment of the IOL optical quality is conducted using optical and clinical methods. Optical bench testing of IOLs is objective, patient independent, and complementary to clinical assessment. The study and interpretation of both sorts of outcomes lead to clarify the characteristics of IOL designs with higher impact on vision and, hence, offer useful information to surgeons for implant selection and to designers for IOL optimization.
Diffractive bifocal intraocular lenses have a hybrid diffractive-refractive design that consists of a high-power refractive lens (carrier or base lens) and an additional (add) low-power diffractive profile engraved on either the front or the back surface of the IOL following a sawtooth pattern of echelettes. The optical path difference (OPD) for rays travelling through each zone, between the inner and outer step edges, is commonly expressed in terms of the design wavelength (λ0) or, equivalently, of the phase shift () introduced in a light wavefront. So far, most of the diffractive profiles mainly utilize the zeroth and first diffraction orders to make the intraocular lens ((0th,1st)DIOL) form two images: distance and near images, respectively [1,2]. For the distance image, the 0th order energy goes to the image formed by the high-power refractive carrier lens, whereas the 1st order energy goes to the near image formed by the combined carrier plus add powers. The distribution of the energy between these diffraction orders - and hence, between the distance and near images- can be properly adjusted by setting the step height of the profile. For example, if the diffractive steps are π-phase shift, meaning and , light will distribute ideally balanced between the two images (with theoretically 40.5% of energy efficiency each). Phase steps in the range benefit the 0th order at the expense of the 1st order whereas phase steps in the range have the opposite effect. Moreover, apodized IOLs use gradual reduction of the step height to continuously modulate the phase shift from center to periphery. As a result, the energy distribution between the 0th and 1st order foci becomes pupil dependent [3]. These properties have been exploited in a variety of bifocal diffractive IOL designs studied elsewhere (see, for instance, studies using computational and/or on optical bench techniques [2,4,5], clinical studies assessing the impact on visual function [6] or both [7,8]).
Recently, chromatic aberration has drawn increasing attention in IOL design and visual optics research (see, for instance, Refs. 9–12). A diffractive profile with maximum phase modulation – m being an integer number- operates at higher harmonic orders [13–15] and has proved to show interesting properties for achieving an effective reduction of the chromatic aberration that would otherwise affect severely common 1st-diffractive order lenses. Harmonic diffractive lenses with phase shift being an odd multiple of π (or, equivalently, OPD being an odd multiple of ) also direct light between two neighbor diffraction order images (with 40.5% energy efficiency each). Thus, for instance, a 3π-phase-shift () diffractive lens distributes light between the 1st and 2nd diffraction orders with balanced energy [16]. If such a diffractive pattern is cut onto the base curve of a refractive carrier lens, the combined optical element will become a bifocal lens. If this combined element is meant to be an IOL, the carrier refractive and the diffractive powers must be carefully calculated to provide a range for distinct vision. A low addition diffractive bifocal IOL –typically with diffractive add power < 2 D- would provide distinct vision at far and intermediate distances. Its near focus would actually provide distinct intermediate vision and for this reason we call it intermediate focus (instead of near focus) hereafter.
In this work, we describe analytically a hybrid refractive-diffractive IOL design named (1st,2nd)DIOL that operates with the 1st and 2nd diffractive orders, and prove experimentally its imaging properties on optical bench. An example of (1st,2nd)DIOL implementation can be found in the Tecnis Symfony ZXR00 IOL [Abbott Medical Optics, Abbott Park, IL]. This lens, henceforth the Symfony lens, has been introduced on the market with a patented novelty [17], as mentioned in Ref.18. As far as we know, this is the first time that the properties of a (1st,2nd)DIOL model are used to explain mathematically the chromatic performance of the Symfony lens. The verification of this hypothesis, both analytical and experimentally, constitutes an important objective of this work. The explanation is fully supported by the results of the experiment conducted to measure the red (R), green (G) and blue (B) through-focus energy efficiency (TF-EE) and the longitudinal chromatic aberration (LCA) of the foci.
For the sake of comparison, the experimental results obtained with a Symfony IOL are shown along with others corresponding to a monofocal (Tecnis ZA9003) IOL and a bifocal (0th,1st)DIOL (Tecnis ZKB00) of the same distance power. We review in this paper the mathematics concerning (0th,1st)- and (1st,2nd)- DIOLs. This leads us to discuss on bifocal design, Symfony’s lens EDOF design, and on the compensation of chromatic aberration. Such results and discussions provide an additional context to the modulation transfer function based results recently reported by related research [18–21].
2. Materials and methods
2.1 Optical power and energy efficiency of a hybrid refractive-diffractive IOL
We assume a model for a hybrid refractive-diffractive IOL, according to which a thin carrier lens of refractive index with a diffractive lens profile cut on one side [2] is immersed in a medium of refractive index , which represents both the aqueous and vitreous humors. The optical power P of such hybrid refractive-diffractive IOL, at a specific design wavelength , results from the addition of two terms, the refractive and the diffractive powers,
| (1) |
We assume in Eq. (1) that the lens thickness is negligible in comparison with the focal lengths involved. The power of the base refractive lens, with front and back radii, is
| (2) |
The diffractive profile, designed at wavelength , induces a phase shift () at every step edge that is proportional to its height (h), according to the expression
| (3) |
The diffractive lens contributes with some of its m diffracted order powers
| (4) |
to the total IOL power (Eq. (1)). It is worth noticing that, according to Eq. (4), the 0th diffraction order (m = 0) has null power. Let us assume that the step height of the diffractive lens produces an OPD of , with , equivalent to a phase shift of rad. When (p = 1) a widely known result is obtained: the diffractive lens, illuminated with the design wavelength, reaches maximum efficiency, ideally 100%, in its positive 1st order while the other orders vanish. The power of the diffractive lens is with denoting the radius of the first zone or central echelette.
For an illumination wavelength λ the diffraction energy efficiency of the m order is
| (5) |
where and α is defined as the fraction of 2π phase shift introduced for illumination wavelengths other than . In the context of the eye, α is given by
| (6) |
In Eq. (6), the factor in brackets accounts for the little influence of material dispersion and hence, Eq. (6) is frequently approximated by , with material dispersion neglected (see, for instance, Refs. 5,13). At wavelengths away from the design , the power of each diffractive order m follows the equation
| (7) |
but their diffracted energy efficiency –given by Eq. (5)- falls quickly (except for the harmonic wavelengths, that is, those that satisfy , which come to a common focus with the design wavelength and with same high efficiency [13,14]).
2.2 Longitudinal chromatic aberration in a 1st and 2nd diffractive order IOL
For non-integer values of p, let us say (with m integer), light mainly distributes between the two adjacent m and m + 1 diffraction orders. This is, among other examples, the mentioned case of p = 0.5, with light split and mostly directed to the 0th and 1st orders, and also the case of p = 1.5, with light mostly directed to the 1st and 2nd orders [2,14,16].
At any focus of a hybrid refractive-diffractive IOL, the optical power variation with illuminating wavelength λ -and hence, LCA- results from the addition of two terms that account for: one, the dispersive nature of the optical materials through their refractive indexes and two, the inherent spectral properties of the diffraction orders involved. Typically, LCA measures optical power variation along the optical axis in the image space (although it can be equivalently translated to the object space). Thus, each focus of a hybrid refractive-diffractive IOL, which is formed by the joint contribution of the base refractive lens power and a specific m-diffraction order, shows a LCA that can be written as
| (8) |
where subindexes r, d stand for refractive and diffractive contributions, respectively. The refractive power variation of the carrier lens is usually referred to {FdC} set of wavelengths, with , , and for which the coefficient of refractive dispersion named Abbe number is defined as The refractive power variation can be calculated using the mathematical expression [12]
| (9) |
where the refractive power of the lens for wavelength λ is given by Eq. (2).
The diffractive lens power change with wavelength can be obtained from Eq. (7), so that
| (10) |
with Δλ commonly limited to the visible spectral range. The negative sign in Eq. (10) accounts for a power variation in the opposite direction to that produced by the refractive lens (Eq. (9)). The step height of the profile plays an essential role in the contribution of the diffractive lens to the LCA of the IOL. To illustrate this effect with an example, let us consider a (0th,1st)DIOL, that is, a hybrid refractive-diffractive IOL with step height producing a π-phase shift in the design wavelength . As mentioned, this lens performs bifocally with balanced efficiency at that wavelength between the 0th and 1st diffraction orders, which are associated to the distance and near IOL powers, respectively. Since the 0th order has null power (Eq. (4)), the LCA at the distance focus is computed using Eq. (8) with m = 0,
| (11) |
which means that the chromatic aberration in the distance focus depends entirely on the refractive base lens. To obtain LCA in the near focus, we take Eq. (8) with m = 1, which involves the contribution of the diffractive lens operating in the 1st order
| (12) |
and as derived from Eq. (10) for the 1st diffraction order. We recall that Eq. (12) will be also valid for an intermediate focus provided the diffractive power value is sufficiently low.
Let us compare the conventional (0th,1st)DIOL with a lens designed to operate with the 1st and 2nd diffraction orders to provide the distance and near (intermediate) foci. We will show that this design leads to significant differences regarding power and spectral performance. For the sake of simplicity, we keep the term “near” along the explanation, but it could be replaced by “intermediate” depending on the value of the diffractive power. According to Eqs. (1), (2) and Eqs. (4), (5), the distance and near powers of such (1st,2nd)DIOL for the design wavelength are
| (13) |
| (14) |
and both have balanced efficiency of 40.5% at . According to Eqs. (8), (10), their LCAs are
| (15) |
| (16) |
which means that the distance and the near powers, as well as the LCAs in both foci, depend on both the carrier refractive lens and the diffractive lens characteristics. Since the LCAs of the refractive and diffractive contributions have opposite sign, it implies that some compensation of the chromatic aberration can be expected for both the distance and the near foci. This fact clearly differs from the (0th,1st)DIOL design (see Eqs. (11), (12)), for which, such compensation is only possible in the near focus but not in the distance focus [12]. We recall that Eqs. (11), (12), (15), (16) refer, exclusively, to the LCA introduced by the IOL and do not take into account the contributions of the cornea and other ocular media to the LCA of the eye.
Since the LCA produced by diffractive components depends linearly on the wavelength difference and the diffractive order (Eq. (10)), some care must be taken regarding the near focus in a (1st,2nd)DIOL. As Eqs. (15), (16) reveal, the compensation of chromatic aberration in the near focus doubles the compensation in the distance focus. For example, if the refractive power variation were fully compensated in the distance focus, it would be overcompensated in the near focus, thus producing an undesired chromatic aberration in it. Fortunately, this effect can be mitigated by three factors. First, the diffraction efficiency, governed by Eq. (5); second, the add power of the diffractive lens, , which is generally much lower than the power of the base refractive lens (about 10% in diopters), and third, the possibility of apodization. Step height reduction from center to periphery, benefits 1st order efficiency, as pupil size increases, at expenses of the 2nd. A good design must reach a trade-off with all these factors.
2.3 Intraocular lenses
Three Tecnis (Abbott Medical Optics, Abbott Park, IL) IOLs were used in the experiment: a Symfony ZXR00 (Fig. 1), a bifocal ZKB00, and a monofocal ZA9003. All three IOLs shared the same material and had the same aspheric design of the refractive base lens. They had similar power for distance vision (30D). The first two were hybrid refractive-diffractive designs. The monofocal IOL, with purely refractive design, was included as a reference in the study. Further IOL specifications and features are listed in Table 1. The diffractive design of Tecnis ZKB00, with + 2.75 D add power, is intended to produce a balanced distribution of energy between the distance and the near foci independently of the pupil diameter.
Fig. 1.
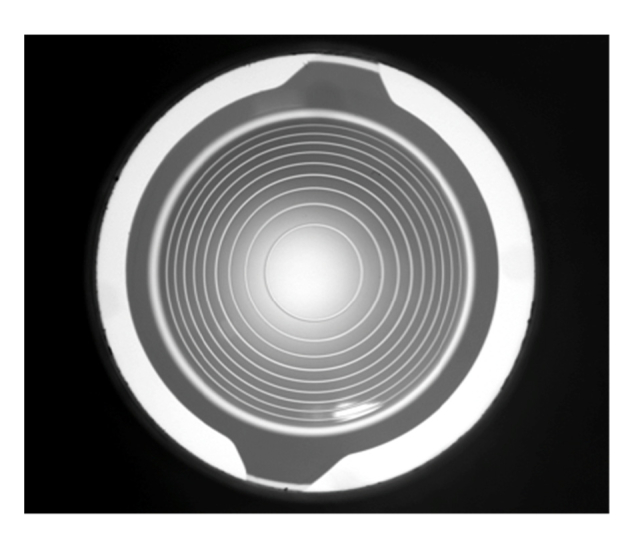
Symfony IOL tested in the work. Measured values for ring diameter and step height are listed in Table 2.
Table 1. Optical data of Tecnis (Abbott Medical Optics) intraocular lensesa.
| Symfony ZXR00 | ZKB00 | ZA9003 | |
|---|---|---|---|
| Material | Hydrophobic Acrylic | ||
| Refractive index n | 1.47 | 1.47 | 1.47 |
| Abbe value V | 55 | 55 | 55 |
| Color filter | UV-blocking | UV-blocking | UV-blocking |
| Optic Zone diameter (mm) | 6 | 6 | 6 |
| Aspheric surfaceb SA = c[4,0] (μm)c |
Anterior −0,27 |
Anterior −0,27 |
Anterior −0,27 |
| Diffractive design | Pupil-dependent Posterior |
Full-aperture Posterior |
NAd |
| Base Power (D) | 30 | 30 | 30 |
| Add Power (D) at IOL plane | + 1.75 | + 2.75 | NAd |
Specifications provided by the manufacturer for wavelength of 555 nm and 37°C [22].
Data for a 6mm pupil;
SA, spherical aberration;
NA, not applicable.
The Symfony ZXR00 IOL is claimed to be designed with a proprietary method based on a combination of refractive and diffractive technologies for providing EDOF and, therefore, extended range of vision with combined correction of spherical and chromatic aberration [21] for contrast sensitivity enhancement [10]. Valuable information is provided in Ref.21 concerning the principles used in design of the IOL diffractive profile: embedded echelettes for offset phase correction with respect to the baseline of the refractive lens [2] and multiple echelettes for EDOF with chromatic correction, but the authors do not mention the specific diffractive orders used for the lens design. Although further details describing the patented technology can be found out in Ref.17, it is not straightforward from this information to assign a specific diffractive design to the Symfony lens and hence, to fully understand the physical mechanisms of its performance.
We have measured ring diameter and step height of the central zones of the diffractive profile of the Symfony lens used in our study (Fig. 1) with confocal microscopy. For the sake of comparison, Table 2 contains these measurements along with the values corresponding to an exemplary diffractive profile, according to an embodiment of the invention described in Ref. 17. Let us remark the good agreement between the design and measured values. The exemplary profile includes OPD in terms of the design wavelength λ0 and the intended light distribution between the 1st and 2nd diffraction orders. Some parameters considered in the exemplary profile calculation were the refractive index of the aqueous of the eye (1.336), the refractive index (1.47) of the ophthalmic lens material and the design wavelength (550 nm approximately) which is within the range 546 ± 10nm recommended by ISO 11979-2:2014 [23]. According to the description, the diffractive profile consists of two main regions: central and peripheral. The central zone includes echelettes of 3π-phase shift steps (OPD = 1.5λ0) that ideally deviate similar amount of energy (40.5%) to the 1st and 2nd diffraction orders for balanced 50:50 energy distribution. The peripheral zone includes echelettes of 2.732π-phase shift steps (OPD = 1.366λ0) that ideally deviate 63% of energy to the 1st diffraction order and 21% to the 2nd one for 75:25 energy distribution. The base refractive power plus the 1st diffraction order direct a fraction of the incoming light to the distance focus while another fraction is directed to another focus, to which the base refractive power and the 2nd diffractive order contribute. The latter is called intermediate focus because of its low addition ( + 1.75 D) [17].
Table 2. Data of the Tecnis Symfony ZXR00 IOL (30D).
| Echelette/Ring | Step Height (μm) | Outer diameter (mm) | OPD (λ0 units) | |||
|---|---|---|---|---|---|---|
| Designa | Experimentalb ( ± 0.2) | Designa | Experimentalc ( ± 0.01) | Designa | ||
| 1 | 6.2 | NA | 1.60 | 1.57 | 1.5 | |
| 2 | 6.2 | 6.3 | 2.20 | 2.21 | 1.5 | |
| 3 | 6.2 | 6.2 | 2.75 | 2.72 | 1.5 | |
| 4 | 5.6 | 5.5 | 3.17 | 3.14 | 1.366 | |
| 5 | 5.6 | 5.7 | 3.55 | 3.53 | 1.366 | |
| 6 | 5.6 | 5.8 | 3.88 | 3.86 | 1.366 | |
| 7 | 5.6 | NAd | 4.20 | 4.19 | 1.366 | |
| 8 | 5.6 | NA | 4.48 | 4.46 | 1.366 | |
| 9 | 5.6 | NA | 4.76 | 4.74 | 1.366 | |
From exemplary diffractive profile, according to an embodiment of the invention described in Ref.17;
Measured with confocal microscope (PLμ Sensofar®) and 100x objective;
Measured from the digital image captured by the microscope and camera of the setup;
NA, not available.
The 0th order would not be used for imaging purposes and about 16% to 18% of the incoming light would be diverted to spurious diffractive orders [17]. The OPD increase introduced by central echelettes -from 0.5λ0 in many conventional bifocals to the current 1.5λ0 in the Symfony lens- would be specifically intended to compensate for chromatic aberration. For pupils within the central region, EDOF effect would be produced by central echelettes with balanced light distribution between the distance and intermediate foci; for larger pupils covering the central and part of the peripheral regions, peripheral echelettes would benefit the distance focus.
Taking into account the diameter and step height measurements as well as other parameters of the Symfony lens contained in Tables 1 and 2, we reasonably postulate that Symfony IOL may represent an example of (1st,2nd)DIOL. The verification of this hypothesis, both analytical and experimentally, constitutes an important objective of this work.
2.4 Experimental setup and metrics
The experimental setup was arranged on optical bench. Three LEDs with emission in the blue (B), green (G) and red (R) spectral bands (Table 3) illuminated the setup sequentially. We measured the R, G, B through-focus energy efficiency (TF-EE) of each IOL. The focus powers and their corresponding LCA were experimentally obtained in all lenses from the R, G, B TF-EE curves. For comparison, mathematical estimations of these magnitudes have been calculated using Eq. (5) and Eqs. (11) to (16). Each IOL was introduced in a wet cell with parallel windows. The optical setup was similar to that used by the authors in related works and described in detail elsewhere (Ref. 12 and, except for the artificial cornea, Ref. 4).
Table 3. Spectral data of LEDsa.
The TF-EE is the quality metrics we have used in this work to test the IOLs under R, G, B illumination. It is worth remarking that the energy efficiency (EE) values of the distance and near foci were straightforwardly obtained from dense sampling the through-focus scan, particularly in the axial neighborhood of focal planes. The same test was repeated for the reference monofocal IOL, but referred to its single (distance) focus.
The method used to measure the EE in the image space has been reported in detail in former works for one [4] or more wavelengths [12]. It includes a pinhole object at infinity and a microscope plus a camera for digital image acquisition. The method firstly applies an edge detection algorithm to segment the central core of the pinhole image at the focus plane of the lens. It secondly quantifies the amount of light intensity in the core (Icore) relative to the intensity in the full image that comprises the core and the background (Itotal = Icore + Ibackground). The EE is given by the ratio Icore /Itotal, which is easy to compute in the experimental practice and approaches the so-called light-in-the-bucket metric [25]. From definition, the light-in-the bucket metric captures the diffraction energy efficiency of IOL as well as image blur caused by aberrations and scattering. It quantifies the amount of light in the central core of the point spread function (PSF) relative to that in a monofocal diffraction-limited PSF for the same wavelength and pupil size [5]. The implementation of this metric in the experimental practice, for which the ideal point source is replaced by a pinhole of certain size, has been described and justified in a former work [12].
During the through-focus test, the core contour determined in the best focus plane was applied with no change to the defocus images obtained by scanning neighbor planes. Axial scanning was stretched to cover the segment of interest in case of multifocal or EDOF IOLs, including the distance and near (intermediate) foci.
A TF-EE curve was experimentally obtained for each IOL under study and every R, G, and B light. Through-focus measurements were taken in the IOL image space and covered about 7 D in 0.2 D steps. For more precision, double density sampling was used when testing the Symfony lens with 2.2mm pupil. LCA experimental values were obtained from the power difference between the furthest EE peaks (corresponding to R and B) at each focus plane.
The experimental work of this study has been done using the setup described elsewhere [12] and summarized here for the sake of conciseness. A 200-micron pinhole test object was placed at the front focal plane of a collimating lens of 200-mm focal length. The collimated beam illuminated the wet cell where the IOL was inserted and thus, one or two aerial images of the pinhole object, or a focal segment, were formed behind the wet cell depending on the lens design. A diaphragm, placed in front of the wet cell and used as entrance pupil, limited the IOL aperture to 3.5 mm diameter during most part of the experiment. With this pupil the impact of the negative spherical aberration of all tested IOLs on their images was still relatively low, which helped us to compute the light-in-the bucket metric. The Symfony IOL was additionally tested with an entrance pupil of 2.2mm that limited its aperture to two central echelettes (Table 2). Behind the wet cell an infinite corrected microscope focused the aerial image of interest and magnified it onto a monochrome 8-bit CCD camera for digital image acquisition. The set of microscope and camera was mounted on a translation holder for axial scanning and moved away along the bench axis with spatial resolution of ± 1 micron. For every IOL and R,G,B light, the intensity of the LED source and the time integration of image acquisition were adjusted to obtain a linear response of the camera in the intensity range of the aerial images (from distance to near images) with no saturation of the camera sensor.
3. Results
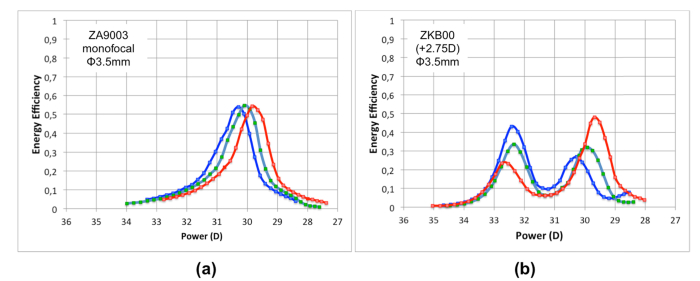
Figure 2 shows the experimental TF-EE curves measured for the monofocal (a) and bifocal (b) IOLs with a pupil size of 3.5mm under R, G, and B LED lights. Power and energy efficiency peak values, and LCA are listed in Table 4. A first explanation of these results can be found in a former work [12].
Fig. 2.
Experimental TF-EE curves of 30D Tecnis monofocal ZA9003 and bifocal ZKB00 ( + 2.75D) IOLs obtained in-vitro under R (solid red line), G (solid green line) and B (solid blue line) LED lights. Small squares represent experimental measurements.
Table 4. Power, energy efficiency and LCA measured for Tecnis ZA9003 and ZKB00 ( + 2.75 D) IOLsa.
| Tecnis Monofocal ZA9003 | Tecnis Bifocal ZKB00 + 2.75 (with OPD = 0.5λ0) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| λ | Power (D) | Energy efficiency | Power (D) |
Energy efficiency |
||||||
| Distance focus |
Near focus |
Distance focus |
Near focus |
Distance/Near Ratio | ||||||
| λ 0 | 550 | (30.0) | (30.00) | (32.75) | (0.41) | (0.41) | (1) | |||
| B | 455 | 30.29 | 0.54 | 30.26 | 32.42 |
0.27 (0.25) |
0.43 (0.58) |
0.62 (0.43) |
||
| G | 530 | 30.10 | 0.55 | 30.10 | 32.34 |
0.32 (0.38) |
0.34 (0.44) |
0.95 (0.86) |
||
| R | 625 | 29.83 | 0.54 | 29.68 | 32.74 |
0.48 (0.51) |
0.24 (0.31) |
2.04 (1.62) |
||
| LCA | 0.46 | 0.58 |
-0.32 (−0.27) |
|||||||
Nominal specifications and theoretical estimations of LCA and diffraction energy efficiency η are included in brackets, below the experimental values, for comparison.
The monofocal IOL (Fig. 2(a)) shows a single focus for distance vision and hence, only one efficiency peak under each R, G and B illumination. The RGB TF-EE curves of the monofocal IOL are very similar and close to each other, with the G peak centered at about 30 D, which is the nominal power for the design wavelength (550nm). The B and R peaks are separated away LCA = 0.46D (Table 4) and placed at either side of the G peak. The bifocal ZKB00( + 2.75D) IOL (Fig. 2(b)) has hybrid refractive-diffractive design with p = 0.5 and hence, it shows two foci, for distance and near vision, that correspond to the 0th and 1st diffraction orders, respectively. With G illumination, the closest to the design wavelength, the TF-EE curve shows quite balanced energy distribution between distance and near foci, with peak EE values of 0.32 (distance) and 0.34 (near) that are slightly below the estimated theoretically diffraction efficiency (0.38 for distance and 0.44 for near) (Table 4). The B and R curves of the bifocal show two peaks as well, but with opposite variations in EE as expected from the wavelength dependence of the diffraction efficiency (Eq. (5)). The LCA in the distance focus of the bifocal (0.58D) has the refractive contribution of the carrier lens (Eq. (11)) and, consequently, is very similar to the LCA obtained with the monofocal IOL (0.46D). The LCA in the near focus, however, is compensated in part by the contribution, with opposite sign, of the diffractive profile operating with the 1st diffraction order (Eq. (12)). Again, the LCA predicted theoretically for the near focus (−0.27D) is very close to the experimental value (). This interpretation, supported by the good agreement between the numerical estimations and the experimental results (Table 4), validates the (0th,1st)DIOL model developed for p = 0.5 and orders m = {0,1}in Section 2 (see also Ref. 12).
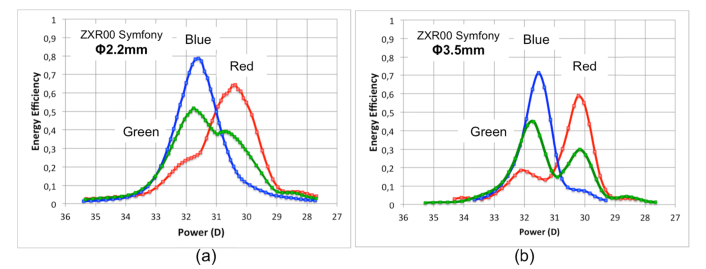
Figure 3 shows the experimental TF-EE curves measured for the Symfony lens for (a) 2.2 mm and (b) 3.5mm pupils under R, G, and B LED illumination. These curves differ quite significantly from those analyzed so far in this work. The B TF-EE curve has a single peak centered at about 31.5 D for both pupil sizes, being the peak obtained with the small 2.2mm aperture of larger full width at half maximum (FWHM) -about 50% larger- than the obtained with the 3.5mm aperture. The G TF-EE curve exhibits two separate peaks of different EE with 3.5mm pupil (Fig. 3(b)). The lowest, centered at about 30D, would correspond to the distance focus whereas the highest, centered at a power slightly below 32D, would correspond to the intermediate focus. Peak asymmetry is markedly prominent in the R TF-EE curve with 3.5mm pupil, but in the opposite direction to that shown by the G TF-EE curve. With 2.2mm pupil, however, the R and G TF-EE curves exhibit a single asymmetric wide peak with . The interpretation of these figures is not straightforward and, certainly, not possible in terms of the conventional bifocal (0th,1st)DIOL design.
Fig. 3.
Experimental TF-EE curves of 30D Tecnis Symfony ZXR00 IOL obtained in-vitro with (a) 2.2 mm pupil and (b) 3.5 mm pupil, under R (solid red line), G (solid green line) and B (solid blue line) LED lights. Small squares represent experimental measurements.
4. Discussion
The (1st, 2nd)DIOL design, as introduced in Section 2, is still a bifocal lens that may feature good properties in chromatic compensation and depth of focus extension when it uses low addition power. Other solutions for depth of focus extension have been proposed and described elsewhere (see, for instance, a review in Ref. 26).
Let us interpret the results shown in Fig. 3 for the Symfony IOL in the light of an optical design based on a 1st and 2nd diffractive order profile, with the term “near” replaced by “intermediate” to account for the relatively low add value ( + 1.75 D). Table 5 contains the results of the calculations obtained using Eqs. (1)–(16) with λ0 = 550nm, step height factors p = {1.5,1.336}, diffractive orders m = {1,2}, and phase delay fractions for the R, G, B lights approached by (Table 3) with material dispersion neglected. The first row of Table 5 shows the design values for the (1st, 2nd)DIOL: distance and intermediate powers (Pdistance = 30D, Pinterm = 31.75D), add power under λ0 illumination. From Eq. (13), the design refractive power of the carrier lens can be inferred, resulting The design intermediate power would add the 2nd diffraction order, which is , to the refractive power. Thus, using Eq. (14), it turns out , which satisfies the nominal power for intermediate focus.
Table 5. Theoretical estimations for (1st,2nd)DIOL.
| Power (D) | Diffraction Energy Efficiency | ||||||||
|---|---|---|---|---|---|---|---|---|---|
|
|
|||||||||
| Refract carrier | 1st order | 2nd order | Center (OPD = 1.5λ0) |
Peripheral (OPD = 1.366λ0) |
|||||
| Distance focus |
Interm. focus |
Distance focus |
Interm. focus |
||||||
| λ | |||||||||
| λ 0 | 28.25 | 1.75 | 3.50 | 30.00 | 31.75 | 0.41 | 0.41 | 0.63 | 0.21 |
| B | 28.49 | 1.45 | 2.90 | 29.93 | 31.38 | 0.05 | 0.89 | 0.19 | 0.66 |
| G | 28.25 | 1.69 | 3.37 | 29.94 | 31.62 | 0.32 | 0.50 | 0.54 | 0.28 |
| R | 27.97 | 1.99 | 3.98 | 29.96 | 31.94 | 0.71 | 0.16 | 0.87 | 0.06 |
| ΔP{RGB} | + 0.52 | −0.54 | −1.08 | ||||||
| LCA | -0.02 | -0.56 | |||||||
For the illumination with the R, G, B LEDs some assumptions can be made concerning the carrier refractive lens. First, the refractive power for the G light can be reasonably approximated by the design refractive power (i.e. ). Second, taking into account the design characteristics that the refractive carrier lens of the hybrid refractive-diffractive IOLs and the monofocal IOL of this work would have in common (see Tables 1 and 4) and although the refractive carrier of the Symfony lens would have a refractive power (28.25 D) somewhat lower than the other two (30 D), we assume that all three refractive designs would contribute similarly to LCA. Therefore, we assume 0.52 D for the chromatic power variation of the Symfony’s refractive carrier, which averages the values measured in the distance focus of the bifocal (0.58 D) and the single focus of the monofocal (0.46 D) (Table 4). We scaled the refractive powers of the Symfony’s carrier for B and R with respect to G light so as to produce a refractive contribution of 0.52 D to LCA. The resulting estimated values are and (Table 5). The 1st and 2nd order powers, and , for R, G, and B lights, have been calculated using Eq. (7), and the distance and intermediate powers with Eqs. (13), (14). The diffraction energy efficiency has been calculated using Eq. (5) for all wavelengths. The row contains the RGB power variations of the refractive carrier lens () and the diffractive profile (). They contribute to the LCA by compensating each other in the distance and the intermediate foci. As a result, LCA would be almost suppressed in the distance focus (LCAdistance = −0.02D) and would keep low (LCAinterm = −0.56D) in the intermediate focus.
When the step height factor is p = 1.5, it is worth mentioning the low efficiency of the 2nd diffraction order for R light (0.16) and the very low 1st diffraction order for B light (0.05), thus predicting a shortage of these color lights in either the intermediate or the distance focus to which they contribute. Conversely, the 1st diffraction order with R light reaches high energy efficiency (0.71) and so does the 2nd diffraction order with B light, reaching even a higher value (0.89), thus predicting the importance of these color lights in the specific focus to which they contribute.
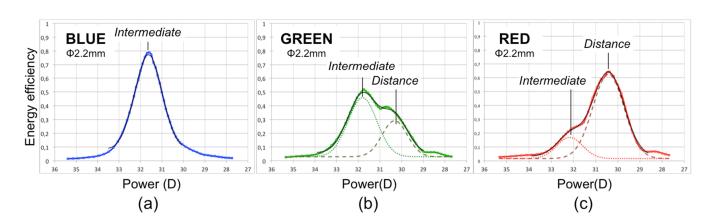
The estimations contained in Table 5 must be compared with the values experimentally obtained using the Symfony lens with 2.2mm aperture (Fig. 3(a)). According to the exemplary embodiment described in Ref. 17 and the outer diameters of the Symfony’s echelettes (Table 2), the 2.2mm aperture contains the two central echelettes, with p = 1.5 (OPD = 1.5λ0) and diffractive orders m = {1,2}. At a glance, only the B TF-EE curve seems to agree with the estimations (Table 5), but the R and G TF-EE curves appear to differ significantly. However, if we analyze them in detail –as we are going to do- we will find that they are in very good agreement too. Certainly, due to the relative proximity of the distance and the intermediate foci –only 1.75D apart- and the effect of a small 2.2mm pupil, which involves inherent depth of focus extension, we may consider that both foci overlap on the optical axis and, as a result, the TF-EE curve becomes the envelope of the addition of the two distance and intermediate EE peaks. We illustrate this effect in Fig. 4, more specifically in Figs. 4(b) and 4(c). For simplicity, we have considered that the energy efficiency curve in the vicinity of a focus can be approached by a Gaussian function. We have fit the experimental R and G TF-EE curves to the addition (in solid black line) of two Gaussian functions (in dashed and dotted lines) that minimize the accumulated square error with respect to the experimental curve over the range of interest (solid black line’s domain). The results in Figs. 4(b) and 4(c) show two Gaussian functions with different height, centered at the distance and intermediate foci. Their addition (solid black lines) fits the experimental curve with remarkable accuracy in either case. The B TF-EE curve was approached by a single Gaussian function corresponding to the intermediate focus. The central position and the maximum value of the obtained Gaussian functions correspond to the experimental power and energy efficiency of the R, G, and B distance and intermediate foci of the Symfony lens with 2.2mm pupil. In Table 6 we compare these values with the theoretical predictions taken from Table 5. The excellent agreement between theoretical estimations and experimental results for 2.2mm pupil (Table 6) confirms the validity of our analysis and interpretation of the RGB TF-EE curves of Fig. 3(a). Moreover, they allow us to experimentally prove that the central zone of the Symfony lens (two central echelettes within 2.2mm pupil) performs as (1st, 2nd)DIOL with low + 1.75D add power.
Fig. 4.
Decomposition of (a) B, (b) G, and (c) R experimental TF-EE curves of Tecnis Symfony ZXR00 IOL (Fig. 3a) in terms of addition of Gaussian functions centered at the distance and intermediate foci: Experimental curve (thick solid line), fit function (black solid line) resulting from the addition of Gaussian functions centered at distance focus (dark dashed line) and intermediate focus (dotted line) that minimizes the cumulative square error over the range.
Table 6. Tecnis Symfony ZXR00 IOL (30 D)a.
|
POWER (D)
|
|||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
Distance focus
|
Intermediate focus
|
Cumulative Square Error |
|||||||||||
| Theor estimation | Experimental |
Theor estimation | Experimental |
|
|||||||||
| λ | ∅b 2.2 | ∅ 3.5 | ∅ 2.2 | ∅ 3.5 | ∅ 2.2 | ∅ 3.5 | |||||||
| λ 0 | 30.00 | 31.75 | |||||||||||
| B | 29.93 | – | 30.13 | 31.38 | 31.63 | 31.54 | 0.0029 | 0.0004 | |||||
| G | 29.94 | 30.32 | 30.17 | 31.62 | 31.81 | 31.78 | 0.0027 | 0.0005 | |||||
| R | 29.96 | 30.42 | 30.19 | 31.94 | 32.17 | 31.91 | 0.0034 | 0.0026 | |||||
| LCA | -0.02 | Not eval. | −0.07 | -0.56 | −0.53 | −0.37 | |||||||
|
| |||||||||||||
| ENERGY EFFICIENCY | |||||||||||||
|
| |||||||||||||
|
Distance focus |
Intermediate focus |
Distance/Intermediate Ratio |
|||||||||||
| ∅ 2.2 | ∅ 3.5 | ∅ 2.2 | ∅ 3.5 | ∅ 2.2 | ∅ 3.5 | ||||||||
|
|
|
|
|||||||||||
| λ | Theor η | Exper | Theor η | Exper | Theor η | Exper | Theor η | Exper | Theor η | Exper | Theor η | Exper | |
|
| |||||||||||||
| λ 0 | 0.41 | 0.38 | 0.41 | 0.29 | 1 | 1.29 | |||||||
| B | 0.05 | Negl. | 0.07 | 0.07 | 0.89 | 0.77 | 0.68 | 0.70 | 0.05 | Negl. | 0.10 | 0.10 | |
| G | 0.32 | 0.29 | 0.31 | 0.29 | 0.50 | 0.46 | 0.36 | 0.44 | 0.63 | 0.64 | 0.84 | 0.66 | |
| R | 0.71 | 0.62 | 0.61 | 0.57 | 0.16 | 0.17 | 0.11 | 0.16 | 4.52 | 3.69 | 5.68 | 3.43 | |
| Cumulative Square Error (∅ 2.2): 0.023 Cumulative Square Error (∅ 3.5): 0.012 |
|||||||||||||
We have repeated this exercise for the experimental RGB TF-EE curves obtained with a 3.5mm pupil (Fig. 3(b)). In this case, the larger aperture of the pupil reduces the natural depth of focus, and hence, the peak mutual overlapping. The B TF-EE curve (Fig. 3(b)) shows a single intermediate focus with a very low plateau at the expected position of the distance focus. The R and G TF-EE curves show two asymmetric foci, placed at the distance and intermediate focus positions. Gaussian decompositions, similar to those presented in Fig. 4, have been calculated for the experimental RGB TF-EE measured with 3.5mm pupil although they are not plotted here for brevity. The corresponding center and maximum values appear in Table 6 to represent experimental power and energy efficiency values, respectively.
To further interpret the energy efficiency values obtained with a pupil of 3.5mm (Fig. 3(b)), we take into account that, according to the exemplary design described in Ref. 17 and the dimensions of the Symfony’s echelettes (Table 2), two more echelettes (the 3rd and 4th ones) are also included when using a 3.5mm pupil. We recall that the 4th echelette lies in the peripheral region of the lens aperture, with p = 1.366 (OPD = 1.366λ0). With 3.5mm pupil, the IOL was operating with 9.62 mm2 aperture area, of which, 5.94mm2 (61.7%) were corresponding to the first three echelettes, (with p = 1.5) and 1.95mm2 (20.3%) to the peripheral region (4th echelette, with p = 1.366). Table 5 contains the diffraction energy efficiencies of the 1st and 2nd diffraction orders calculated for the peripheral echelettes with p = 1.366, under the illumination of λ0, R, G, and B lights. The area covered by either the three central echelettes (61.7%) or the fourth echelette (20.3%) has been taken as weight to estimate the theoretical diffraction energy efficiencies of the RGB distance and intermediate foci, as well as the distance to intermediate efficiency ratio. The resulting theoretical estimations (Table 6) are in very good agreement with the corresponding experimental power and energy efficiencies computed from the Gaussian decomposition of the RGB TF-EE curves obtained with 3.5mm pupil.
We were dubious about considering the contribution of the 5th echelette because it was not fully covered by the 3.5mm pupil and could be also affected by the influence of small misalignments. We recalculated the RGB energy efficiencies of the distance and intermediate foci assuming the further contribution of the 5th echelette, but we obtained an accumulated square error of 0.068 with respect to the experimental values. Because this square error is higher than 0.012 (Table 6), obtained when assuming the first four echelettes, we concluded that the contribution of the 5th echelette could be discarded.
Let us highlight the excellent and very consistent agreement between the experimental values and the theoretical estimations for the distance and intermediate power values with both pupils (Table 6). The experimental results additionally confirm that LCA is negligible at the distance focus and very low (and negative) at the intermediate focus, as expected.
Finally, although LCA is almost extinguished in a (1st, 2nd)DIOL of low addition we point out the very strong asymmetry –much stronger than the already reported in conventional bifocal (0th, 1st)DIOL [12]- between the energy efficiency peaks of B and R lights. For instance, a (1st, 2nd)DIOL such as the Symfony lens, with 3.5mm pupil (Table 6), has an experimental distance/intermediate EE ratio of 3.43 for R light versus a ratio of 0.10 for B light, away by far from the ideal 1.29 for the design wavelength. A bifocal (0th, 1st)DIOL such as ZKB00 ( + 2.75), however, with the same 3.5mm pupil (Table 4), has an experimental distance/intermediate EE ratio of 2.04 for R light versus a ratio of 0.62 for B light, still far but in lesser amount from the balanced 1.0 ideal value for the design wavelength. This increase in both the R and B TF-EE asymmetry could have an impact on the color image quality that deserves further evaluation in future work.
Although the results analyzed in this work were obtained for 30 D IOLs (Table 1), they are translatable to IOLs of different power (a 20-24 D range is common) and can be estimated using the equations provided in Sections 2.1 and 2.2.
5. Conclusions
The imaging properties of two hybrid refractive-diffractive IOLs with different diffractive designs have been analyzed within the common context of a refractive carrier combined with a diffractive lens that operates with two adjacent diffraction orders, either (0th,1st) or (1st,2nd).
We have reviewed the mathematics for the distance and intermediate/near powers, the energy efficiency, their variation for a wavelength other than λ0, and LCA in each focus. An example of a 1st and 2nd order hybrid refractive-diffractive IOL design has been found in the Tecnis Symfony ZXR00 IOL and this pattern matching has been reported here for the first time. We have proved the imaging properties of such IOL design by numerical simulation and also experimentally by measuring in-vitro the TF-EE curves of the Symfony IOL for R, G, and B illuminations. Analogous TF-EE measurements, obtained for the Tecnis monofocal ZA9003 and bifocal ZKB00 ( + 2.75D) counterparts, have allowed us to compare the optical imaging properties of power and energy efficiency of all three lenses, not only between them but also with respect to theoretical predictions. An excellent agreement was obtained in all cases, thus giving a sound support to the analysis carried out and the IOL models considered.
The low add power ( + 1.75 D) of the tested (1st, 2nd)DIOL provides some focus extension from distance to intermediate vision with remarkable chromatic compensation. This chromatic compensation is almost complete in the distance focus and very relevant in the intermediate focus. This fact, along with the aspheric design of the refractive carrier lens, should contribute to enhance the imaging properties of the lens.
The relative energy efficiency between the distance and the intermediate foci is modulated by changing the echelette step height in two regions of the Symfony lens aperture: a central region that directs the design wavelength to both foci with balanced energy, and a peripheral region that benefits the distance focus.
Some limitations must be mentioned though. The add power ( + 1.75D) can be insufficient in some instances. Higher add powers could be considered as well but at the price of sacrificing some of the current properties of the lens, for instance, part of the chromatic compensation. The energy efficiency of the lens for wavelengths other than λ0 is markedly asymmetric. In particular, the energy efficiency for the blue light concentrates on the intermediate focus and is negligible in the distance focus. The opposite effect occurs with the red light: its energy efficiency in the intermediate focus is very low. These effects must be taken into consideration when assessing LCA compensation. Their impact on color image quality must be further investigated.
Disclosures
The authors declare that there are no conflicts of interest related to this article.
Acknowledgements
To Franco Rigato from INDO Horizons for assistance with the confocal microscope and Rosalba García Millán for assistance in the mathematical fit of experimental data.
Funding
Spanish Ministerio de Economía y Competitividad y Fondos FEDER (DPI2016-76019-R).
References and links
- 1.Cohen A. L., “Practical design of a bifocal hologram contact lens or intraocular lens,” Appl. Opt. 31(19), 3750–3754 (1992). 10.1364/AO.31.003750 [DOI] [PubMed] [Google Scholar]
- 2.Cohen A. L., “Diffractive bifocal lens designs,” Optom. Vis. Sci. 70(6), 461–468 (1993). 10.1097/00006324-199306000-00003 [DOI] [PubMed] [Google Scholar]
- 3.Davison J. A., Simpson M. J., “History and development of the apodized diffractive intraocular lens,” J. Cataract Refract. Surg. 32(5), 849–858 (2006). 10.1016/j.jcrs.2006.02.006 [DOI] [PubMed] [Google Scholar]
- 4.Vega F., Alba-Bueno F., Millán M. S., “Energy distribution between distance and near images in apodized diffractive multifocal intraocular lenses,” Invest. Ophthalmol. Vis. Sci. 52(8), 5695–5701 (2011). 10.1167/iovs.10-7123 [DOI] [PubMed] [Google Scholar]
- 5.Ravikumar S., Bradley A., Thibos L. N., “Chromatic aberration and polychromatic image quality with diffractive multifocal intraocular lenses,” J. Cataract Refract. Surg. 40(7), 1192–1204 (2014). 10.1016/j.jcrs.2013.11.035 [DOI] [PubMed] [Google Scholar]
- 6.Alfonso J. F., Fernández-Vega L., Baamonde M. B., Montés-Micó R., “Prospective visual evaluation of apodized diffractive intraocular lenses,” J. Cataract Refract. Surg. 33(7), 1235–1243 (2007). 10.1016/j.jcrs.2007.03.034 [DOI] [PubMed] [Google Scholar]
- 7.Alarcon A., Canovas C., Rosen R., Weeber H., Tsai L., Hileman K., Piers P., “Preclinical metrics to predict through-focus visual acuity for pseudophakic patients,” Biomed. Opt. Express 7(5), 1877–1888 (2016). 10.1364/BOE.7.001877 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 8.G. Cardona, F. Vega, M. A. Gil, C. Varón, J. A. Buil, and M. S. Millán, “Visual acuity and image quality in 5 diffractive intraocular lenses,” Eur J Ophthalmol. Jun 26:0 [Epub ahead of print] (2017). [DOI] [PubMed]
- 9.López-Gil N., Montés-Micó R., “New intraocular lens for achromatizing the human eye,” J. Cataract Refract. Surg. 33(7), 1296–1302 (2007). 10.1016/j.jcrs.2007.03.041 [DOI] [PubMed] [Google Scholar]
- 10.Weeber H. A., Piers P. A., “Theoretical performance of intraocular lenses correcting both spherical and chromatic aberration,” J. Refract. Surg. 28(1), 48–52 (2012). 10.3928/1081597X-20111103-01 [DOI] [PubMed] [Google Scholar]
- 11.Vinas M., Dorronsoro C., Cortes D., Pascual D., Marcos S., “Longitudinal chromatic aberration of the human eye in the visible and near infrared from wavefront sensing, double-pass and psychophysics,” Biomed. Opt. Express 6(3), 948–962 (2015). 10.1364/BOE.6.000948 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Millán M. S., Vega F., Ríos-López I., “Polychromatic image performance of diffractive bifocal intraocular lenses: longitudinal chromatic aberration and energy efficiency,” Invest. Ophthalmol. Vis. Sci. 57(4), 2021–2028 (2016). 10.1167/iovs.15-18861 [DOI] [PubMed] [Google Scholar]
- 13.Faklis D., Morris G. M., “Spectral properties of multiorder diffractive lenses,” Appl. Opt. 34(14), 2462–2468 (1995). 10.1364/AO.34.002462 [DOI] [PubMed] [Google Scholar]
- 14.Sweeney D. W., Sommargren G. E., “Harmonic diffractive lenses,” Appl. Opt. 34(14), 2469–2475 (1995). 10.1364/AO.34.002469 [DOI] [PubMed] [Google Scholar]
- 15.Calero V., García-Martínez P., Albero J., Sánchez-López M. M., Moreno I., “Liquid crystal spatial light modulator with very large phase modulation operating in high harmonic orders,” Opt. Lett. 38(22), 4663–4666 (2013). 10.1364/OL.38.004663 [DOI] [PubMed] [Google Scholar]
- 16.Albero J., García-Martínez P., Martínez J. L., Moreno I., “Second order diffractive optical elements in a spatial light modulator with large phase dynamic range,” Opt. Lasers Eng. 51(2), 111–115 (2013). 10.1016/j.optlaseng.2012.09.004 [DOI] [Google Scholar]
- 17.H. A. Weeber, “Multi-ring lens, systems and methods for extended depth of focus,” US Patent No. 2014/0168602 A1. Pub. Date: Jun 19, 2014.
- 18.Gatinel D., Loicq J., “Clinically Relevant Optical Properties of Bifocal, Trifocal, and Extended Depth of Focus Intraocular Lenses,” J. Refract. Surg. 32(4), 273–280 (2016). 10.3928/1081597X-20160121-07 [DOI] [PubMed] [Google Scholar]
- 19.Esteve-Taboada J. J., Domínguez-Vicent A., Del Águila-Carrasco A. J., Ferrer-Blasco T., Montés-Micó R., “Effect of large apertures on the optical quality of three multifocal lenses,” J. Refract. Surg. 31(10), 666–676 (2015). 10.3928/1081597X-20150928-01 [DOI] [PubMed] [Google Scholar]
- 20.Domínguez-Vicent A., Esteve-Taboada J. J., Del Águila-Carrasco A. J., Ferrer-Blasco T., Montés-Micó R., “In vitro optical quality comparison between the Mini WELL Ready progressive multifocal and the TECNIS Symfony,” Graefes Arch. Clin. Exp. Ophthalmol. 254(7), 1387–1397 (2016). 10.1007/s00417-015-3240-7 [DOI] [PubMed] [Google Scholar]
- 21.Weeber H. A., Meijer S. T., Piers P. A., “Extending the range of vision using diffractive intraocular lens technology,” J. Cataract Refract. Surg. 41(12), 2746–2754 (2015). 10.1016/j.jcrs.2015.07.034 [DOI] [PubMed] [Google Scholar]
- 22.Abbott Medical Optics, https://www.vision.abbott.
- 23.International Organization for Standardization, ISO 11979–2:2014 “Ophthalmic Implants - Intraocular Lenses - Part 2: Optical Properties and Test Methods.” Geneva; ISO; 2014. [Google Scholar]
- 24.Thorlabs, Inc. , https://www.thorlabs.com.
- 25.Thibos L. N., Hong X., Bradley A., Applegate R. A., “Accuracy and precision of objective refraction from wavefront aberrations,” J. Vis. 4(4), 329–351 (2004). 10.1167/4.4.9 [DOI] [PubMed] [Google Scholar]
- 26.Z. Zalevsky, “Extended depth of focus imaging: a review,” J. of Photonics for Energy 018001 (2010).