Development and Validation of Correction Formulas for Self-Reported Height and Weight to Estimate BMI in Adolescents. Results from the KiGGS Study

Abstract

Objective

The use of reported instead of measured height and weight induces a bias in prevalence rates for overweight and obesity. Therefore, correction formulas are necessary.

Methods

Self-reported and measured height and weight were available from the German Health Interview and Examination Survey for Children and Adolescents (KiGGS) baseline study (2003-2006) from 3,468 adolescents aged 11-17 years. With regression analyses, correction formulas for height and weight were developed. Cross-validation was conducted in order to validate and compare the formulas. Corrected BMI was calculated, and corrected prevalence rates were estimated. Sensitivity, specificity, and predictive values for overweight and obesity were calculated.

Results

Through the correction procedure, the mean differences between reported and measured height and weight become remarkably smaller and thus the estimated prevalence rates more accurate. The corrected proportions for overweight and obesity are less under-reported, while the corrected proportions for underweight are less over-reported. Sensitivity for overweight and obesity increased after correction. Specificity remained high.

Conclusion

The validation process showed that the correction formulas are an appropriate tool to correct self-reports on an individual level in order to estimate corrected prevalence rates of overweight and obesity in adolescents for studies which have collected self-reports only.

Introduction

Continuous monitoring of overweight and obesity in children and adolescents needs to be established since obesity has become a global epidemic [1]. The use of self-reported or proxy-reported height and weight is a cost-effective way to estimate prevalence rates for overweight and obesity based on BMI derived from reported height and weight. However, various studies have found that the use of reported instead of measured height and weight induces a bias in prevalence rates for overweight and obesity [2,3,4,5,6,7,8,9,10,11,12,13]. Based on data of the German Health Interview and Examination Survey for Children and Adolescents (KiGGS), the authors have previously compared adolescents’ self-reported height and weight with measured data and showed that in most cases height was overestimated while weight was underestimated. As a result, relying on self-reports leads to a lower prevalence of overweight and obese adolescents. Age, gender, and body perception were identified as the main predictors of the difference between reported and measured values [3].

Due to the result that the exclusive use of self-reported height and weight is not recommended, some studies have developed correction formulas for adolescents’ self-reported data in order to obtain more accurate estimates for prevalence rates of overweight and obesity [9,10,13,14]. Jansen et al. [9] generated two different formulas, based on a multiple linear regression, to correct BMI derived from self-reports of 12- to 13-year-old adolescents in the Netherlands. One included sociodemographic characteristics (country of origin and level of education) alone, the second one additionally contained body perception. The correction resulted in a higher prevalence of overweight, an increased sensitivity, and a decreased specificity [9]. Landsberg et al. [10] developed simple correction formulas for self-reported height and weight of 12- to 17-year-olds from a regional study in Germany, separately for boys and girls, based on a linear regression with no additional explanatory variables. Additional adjustment for age, educational background, and nationality showed no improvement of explained variance of the model. Formulas were estimated with a random sample and tested with a second random sample. The correction procedure led to an approximation of the proportions of overweight and obesity to measured prevalence rates and an increased sensitivity [10]. Kurth and Ellert [14] generated a formula to directly correct the prevalence rates derived from the same data analyzed here. Based on conditional probabilities, correction formulas were estimated taking gender and body perception into account [14]. The formulas of Kurth and Ellert have already been applied to other samples [12,15,16] and have been shown to be an appropriate tool for estimating more accurate prevalence rates for weight status derived from self-reports.

The Kurth and Ellert formulas provide corrected prevalence rates, but they cannot be used to derive corrected BMI values on an individual level, which are necessary for further analyses, e.g., analyses of association or analyses with BMI as a continuous variable. Thus, the aim of the present study is to develop and validate formulas to correct self-reported height and weight on an individual level in a sample of 11- to 17-year-old adolescents from the nationwide German KiGGS study considering the associated factors gender, age, and body perception. These formulas might be useful for KiGGS follow-up waves and other studies where only self-reported height and weight are available.

Material and Methods

Study Design and Study Population

The KiGGS study is part of the health monitoring system of the Robert Koch Institute (RKI). KiGGS is a nationwide survey with the aim to collect regularly comprehensive data on the health of children and adolescents aged between 0 and 17 years in Germany. The KiGGS baseline study was conducted as an interview and examination survey from May 2003 to May 2006 [17]. The first follow-up (KiGGS Wave 1) was carried out as a telephone-based survey from June 2009 to June 2012 [18]. The KiGGS study was approved by the Federal Office for Data Protection and by the ethics committee of Charité/Universitätsmedizin Berlin [17,18].

Object of investigation here are data of the KiGGS baseline study which examined a total of 17,641 boys and girls from 167 study locations (response rate: 66.6%). Amongst other instruments, data collection was carried out by self-administered questionnaires filled in by parents, parallel questionnaires for adolescents aged 11 years or older, and a physical examination [17]. Self-reported height and weight were collected face-to-face and only in the second half of the survey (starting November 2004). Participants with implausible or missing values for measured or reported height and weight were excluded from the analysis which led to a total sample size of 3,468 adolescents (1,792 boys and 1,676 girls) aged 11 to 17 years.

Anthropometric Measurements and Self-Reported Height and Weight

Anthropometric measurements were taken by trained staff using standardized methods. Body height was measured, without wearing shoes, with an accuracy of 0.1 cm, using a portable Harpenden stadiometer (Holtain Ltd., UK). Body weight was measured to the nearest 0.1 kg, wearing underwear, with a calibrated electronic scale (SECA, Ltd., Germany) [17]. Prior to the standardized measurement, adolescents were asked face-to-face to report their height and weight with an accuracy of 1 cm and 1 kg, respectively. Information about the study procedure was available in the internet beforehand.

BMI in kg/m² was calculated from self-reported and measured data. Weight status was classified according to age and gender into underweight (<10th percentile), normal weight (≥10th percentile to ≤90th percentile), overweight (>90th percentile) and obesity (>97th percentile) based on the national German reference [19]. Throughout this paper, the category ‘overweight’ includes obese adolescents.

Body Perception

Body perception was examined by asking the following question in the self-administered questionnaire: ‘Do you think you are …’ 1) ‘far too thin’, 2) ‘slightly too thin’, 3) ‘exactly the right weight’, 4) ‘slightly too fat’, or 5) ‘far too fat’ [20]?

Statistical Methods

Developing the Correction Formulas

Analyses were performed with SAS release 9.2 (SAS institute Inc., Cary, NC, USA). Extreme reports with a difference between reported and measured values greater than 3 standard deviations were excluded from the sample to calculate the correction formula. In order to develop formulas to estimate corrected height (height_c) and weight (weight_c), regression analyses were conducted with the GLMSELECT procedure with measured height or weight as dependent variables. In previous analyses, potential predictors like age, socioeconomic status, migration background, and parental overweight had been tested. Since age, reported data, and body perception emerged as the main predictors [3], they were used to derive the correction formulas. Five different models with the predictors exact age (2 decimal points), reported height (height_r) or weight (weight_r), and body perception (reference category: ‘exactly the right weight’) were built. Exact age, reported height and weight were additionally entered as fractional polynomials ((var)^-2, (var)^-1, (var)^-0.5, log(var), (var)^0.5, (var)², (var)³) [21]. Body perception was also entered as a three-category version with the combined categories: 1) ‘too thin’, 2) ‘right weight’, and 3) ‘too fat’. Five different correction formulas (Model A – E) were estimated by building models from the following candidate variables through stepwise selection based on the Schwarz Bayesian Criterion (SBC) [22]:

Model A: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (five categories)

Model B: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (three categories)

Model C: exact age, height_r or weight_r, body perception (five categories)

Model D: exact age, height_r or weight_r, body perception (three categories)

Model E: exact age, height_r or weight_r.

Validation of the Formulas

Cross-validation was conducted in order to validate and compare the five correction formulas derived from Model A to Model E. The correction formulas were calculated with 90% of the study population (training sample). The formulas were tested with the remaining 10% (test sample) by calculating height_c and weight_c as well as corrected BMI (BMI_c) derived from height_c and weight_c. The differences between height_c, weight_c and BMI_c with measured values were calculated. BMI_c was classified into weight status categories, and corrected prevalence rates were estimated. Sensitivity, specificity, and predictive values for overweight and obesity were calculated by comparing measured and corrected prevalence rates. The difference between corrected and measured prevalence rates for weight status was calculated. For each of the five models, the cross-validation process (including the exclusion of outliers and the whole model selection process) was repeated 400 times, and average values were calculated [23].

The correction formula of Kurth and Ellert [14], which directly corrects the prevalence rates, ran through the same cross-validation process (90% training sample with re-calculation of the correction formula; 10% test sample) with 400 repetitions. The formula including body perception was used [14,15]. The results of the cross-validation of the formula of Kurth and Ellert are here described as Model F. For comparison with the results of the correction formulas which correct on an individual level (Model A to Model E), the difference between corrected and measured prevalence rates for weight status was calculated for Model F as well. Since the method of Kurth und Ellert directly corrects the prevalence rates and does not operate on an individual level, it is not possible to calculate sensitivity, specificity, and predictive values for Model F.

Receiver Operating Characteristic Curves

As an adjunct measure for evaluation of the different models (Model A to Model E), receiver operating characteristic (ROC) curves and the area under the curves (AUCs) were calculated. These analyses were conducted without cross-validation. Height and weight were corrected with every formula, and BMI_c was calculated. Overweight (Yes / No) and obesity (Yes / No) based on BMI_c were determined and used as outcome variables. Age- and gender-specific BMI z-scores based on the KiGGS reference population [24] were used as continuous variables. Sensitivity and specificity were determined for each BMI z-score with logistic regression models. For each model, ROC curves (sensitivity versus 1 – specificity) and AUCs were calculated separately for boys and girls. With the help of AUC, the correction formula with the closest approximation of corrected self-reports to measured values regarding the definition of overweight and obesity was identified.

Results

The formulas for estimating height_c and weight_c derived from Model A are shown below:

Boys:

Height_c (cm) = −3.8930 + 1.0323 height_r (cm) + 364649.0 height_r ^-2 (cm) − 0.0015 age³ − 2044.6426 age^-2(1)

R² = 0.9474; RMSE = 2.9973

Weight_c (kg) = 7.6725 + 0.8407 weight_r (kg) + 0.0009 weight_r ² (kg) − 267.7952 age⁻² + x(2)

R² = 0.9676; RMSE = 2.9557

with x = −0.9662 far too thin or −0.9125 slightly too thin or 1.2018 slightly too fat or 2.058 far too fat.

Girls:

Height_c (cm) = 112.2058 + 0.000011 height_r ³ (cm)(3)

R² = 0.9166; RMSE = 2.4096.

Weight_c (kg) = −0.0664 + 0.9917 weight_r (kg) + 194.8001 age^-2 + x(4)

R² = 0.9739; RMSE = 2.0633

with x = −0.6247 far too thin or −0.2552 slightly too thin or 0.5886 slightly too fat or 0.8382 far too fat.

Since body perception was not selected for estimating height_c, the equations for height_c estimated with Model B are the same as the ones estimated with Model A. From Model B, we derived the following correction formulas for weight_c:

Boys:

Weight_c (kg) = 7.5204 + 0.8380 weight_r (kg) + 0.0009 weight_r ² (kg) − 241.3989 age⁻² + x(5)

R² = 0.9675; RMSE = 2.9599

with x = −0.9003 too thin or 1.2934 too fat.

Girls:

Weight_c (kg) = −0.2962 + 0.9949 weight_r (kg) + 208.9497 age^-2 + x(6)

R² = 0.9738; RMSE = 2.0637

with x = −0.3007 too thin or 0.5774 too fat.

The formulas for height_c and weight_c derived from Model C are displayed below:

Boys:

Height_c (cm) = 12.8626 + 0.8825 height_r (cm) + 0.4524 age(7)

R² = 0.9444; RMSE = 3.0816.

Weight_c (kg) = 2.0308 + 0.9700 weight_r (kg) + x(8)

R² = 0.9672; RMSE = 2.9759

with x = −0.6858 far too thin or −0.7486 slightly too thin or 1.369 slightly too fat or 1.9756 far too fat.

Girls:

Height_c (cm) = 19.2692 + 0.8774 height_r (cm)(9)

R² = 0.9114; RMSE = 2.4834.

Weight_c (kg) = 2.8733 + 0.9902 weight_r (kg) − 0.1293 age + x(10)

R² = 0.9738; RMSE = 2.0650

with x = −0.6383 far too thin or −0.2729 slightly too thin or 0.5658 slightly too fat or 0.8583 far too fat.

Since body perception was not selected for estimating height_c, the equations for height_c estimated with Model D and Model E are the same as the ones estimated with Model C. From Model D, we derived the following correction formulas for weight_c:

Boys:

Weight_c (kg) = 1.9262+ 0.9720 weight (kg) + x(11)

R² = 0.9670; RMSE = 2.9810

with x = −0.7357 too thin or 1.1592 too fat.

Girls:

Weight_c (kg) = 2.8646 + 0.9934 weight_r (kg) − 0.1400 age + x(12)

R² = 0.9738; RMSE = 2.0655

with x = −0.3178 too thin or 0.5858 too fat.

And with Model E the correction formulas for weight_c are as follows:

Boys:

Weight_c (kg) = 2.8077 + 1.0011 weight_r (kg) − 0.1601 age(13)

R² = 0.9656; RMSE = 3.0436.

Girls:

Weight_c (kg) = 2.6699 + 1.0081 weight_r (kg) − 0.1616 age(14)

R² = 0.9733; RMSE = 2.0846.

R squared (R²) and the estimated root mean square (RMSE) do not substantially distinguish between the different models.

Table 1 displays the difference between self-reports and cross-validated corrected self-reports to measured height, weight, and BMI. After the correction procedure, the mean difference between corrected self-reports and measured values were remarkably smaller. For example, the mean difference for self-reported to measured weight before correction was −0.6 and −0.8 kg for boys and girls, respectively. After the correction procedure, the mean difference between corrected self-report and measured weight was far below ±0.1 kg for both genders. The higher mean differences between reported and measured values seen in girls disappeared, and the mean differences in girls even got smaller in some cases, compared to boys. In summary, the five approaches of correction are very much alike according to the mean difference. After correction, the standard deviation is smaller, however still high.

Table 1.

Mean difference and standard deviation (SD) for height, weight, and BMI of self-reported/corrected values and measured data, from cross-validation

	Boys		Girls
	mean	SD	mean	SD
Reported – measured
Height, cm	0.144	4.42	0.523	3.83
Weight, kg	−0.568	3.98	−0.826	3.26
BMI, kg/m2	−0.227	1.84	−0.451	1.62
Corrected Model A – measured
Height, cm	−0.006	2.97	−0.050	2.57
Weight, kg	0.033	2.96	−0.030	2.06
BMI, kg/m2	0.024	1.24	−0.004	1.04
Corrected Model B – measured
Height, cm	0.004	2.97	−0.009	2.40
Weight, kg	0.027	2.97	0.001	2.06
BMI, kg/m2	0.021	1.24	−0.004	0.99
Corrected Model C – measured
Height, cm	−0.001	3.06	−0.006	2.49
Weight, kg	0.045	2.97	0.013	2.06
BMI, kg/m2	0.024	1.26	−0.003	1.01
Corrected Model D – measured
Height, cm	−0.003	3.05	−0.002	2.49
Weight, kg	0.034	3.00	0.008	2.05
BMI, kg/m2	0.019	1.27	−0.006	1.01
Corrected Model E – measured
Height, cm	−0.008	3.04	−0.008	2.56
Weight, kg	0.061	3.09	−0.048	2.07
BMI, kg/m2	0.031	1.30	−0.025	1.04

Model A: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (five categories).

Model B: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (three categories).

Model C: age and height_r or weight_r, body perception (five categories).

Model D: age and height_r or weight_r, body perception (three categories).

Model E: age and height_r or weigh_t.

Table 2 shows the mean values of sensitivity, specificity as well as positive and negative predictive values for overweight and obesity by gender after cross-validation and, for comparison, the corresponding values based on the uncorrected self-reports. Sensitivity for overweight and obesity increased after correction. Specificity remained high, except for overweight girls, where the specificity slightly decreased. Lower positive predictive values after correction were seen in overweight girls, whereas positive predictive values increased for overweight in boys and obesity in both genders. After correction, the negative predictive values were higher for all models in comparison to the values based on uncorrected self-reports.

Table 2.

Mean and 95% CI for sensitivity, specificity and predictive values for overweight and obesity for reported values compared to corrected values with the formulas derived from Model A to Model E, from cross-validation

	Boys				Girls
	overweight		obesity		overweight		obesity
	%	95% CI	%	95% CI	%	95% CI	%	95% CI
Sensitivity
Reported	75.8		64.9		73.7		68.8
Model A	84.1	83.4–84.8	78.4	77.2–79.5	81.0	80.3–81.6	81.5	80.4–82.5
Model B	84.1	83.4–84.8	77.7	76.5–78.8	85.6	84.9–86.2	83.8	82.7–85.0
Model C	84.2	83.6–84.8	78.4	77.2–79.6	84.9	84.2–85.5	84.8	83.8–85.9
Model D	84.1	83.4–84.7	75.9	74.7–77.1	84.5	83.8–85.2	84.9	83.8–86.0
Model E	83.6	82.9–84.2	76.5	75.3–77.7	80.9	80.3–81.5	82.4	81.3–83–4
Specificity
Reported	97.0		98.4		98.5		99.0
Model A	97.2	97.1–97.3	98.8	98.7–98.8	97.6	97.5–97.7	99.3	99.3–99.4
Model B	97.1	97.0–97.2	98.8	98.7–98.9	97.7	97.6–97.8	99.1	99.0–99.2
Model C	97.4	97.3–97.5	98.6	98.6–98.7	97.8	97.6–97.9	99.2	99.2–99.3
Model D	97.1	97.0–97.3	98.7	98.6–98.8	97.6	97.5–97.7	99.1	99.0–99.1
Model E	97.3	97.2–97.5	98.8	98.7–98.9	97.4	97.3–97.5	99.5	99.4–99.5
Positive predictive value
Reported	84.6		76.3		91.3		85.4
Model A	86.1	85.5–86.7	83.1	82.1–84.2	88.2	87.7–88.8	90.6	89.8–91.5
Model B	85.8	85.2–86.4	83.1	82.1–84.2	88.6	88.0–89.1	86.9	85.9–87.9
Model C	87.0	86.4–87.5	81.7	80.6–82.8	88.8	88.2–89.3	88.6	87.7–89.6
Model D	85.8	85.2–86.4	82.1	81.0–83.2	88.1	87.5–88.6	87.0	86.1–88.0
Model E	86.5	85.9–87.2	83.1	82.0–84.1	87.2	86.7–87.8	92.5	91.7–93.3
Negative predictive value
Reported	94.9		97.2		94.6		97.5
Model A	96.7	96.6–96.9	98.4	98.3–98.5	95.9	95.7–96.1	98.6	98.5–98.7
Model B	96.7	96.6–96.9	98.3	98.2–98.4	97.0	96.4–97.1	98.8	98.7–98.9
Model C	96.8	96.6–96.9	98.4	98.3–98.5	96.9	96.7–97.0	98.9	98.8–99.0
Model D	96.7	96.6–96.8	98.2	98.1–98.3	96.8	96.6–96.9	98.9	98.8–99.0
Model E	96.7	96.5–96.8	98.2	98.1–98.3	95.9	95.7–96.0	98.6	98.5–98.7

Reported values are based on reported height_r and weight_r.

Model A: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (five categories).

Model B: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (three categories).

Model C: age and height_r or weight_r, body perception (five categories).

Model D: age and height_r or weight_r, body perception (three categories).

Model E: age and height_r or weight_r.

In table 3 the mean differences in prevalence rates for corrected and measured weight status from the cross-validation are shown. Considering the results from Model A to Model E for boys, there is no favorite model seen. Correction with formulas derived from Model A, B and E led to a slight overestimation of the prevalence for normal weight, whereas Model C and D showed the smallest difference for normal weight. However, with Model C and D the differences for underweight / strong underweight or overweight/obesity were larger in comparison to the other models. Across all models, the corrected prevalence rates for overweight and obesity in girls led to a smaller underestimation in comparison to the uncorrected prevalence rate, whereas the prevalence of normal weight in girls was more strongly overestimated than it was before the correction. In girls, Model B showed the smallest differences in prevalence rates, with the difference for underweight / strong underweight being negligible. Overall, Model F, i.e. the formula developed by Kurth and Ellert which directly corrects the prevalence rates, showed the smallest differences between corrected and measured prevalence rates in comparison to Model A to Model E.

Table 3.

Mean differences with SD for prevalence rates (%) of weight status between corrected and measured data

Weight status	Uncorrected 1	Model A	Model B	Model C	Model D	Model E	Model F
Boys
Strong underweight	1.40	0.23 ± 1.19	0.21 ± 1.10	0.41 ± 1.08	0.46 ± 1.10	0.23 ± 1.18	0.03 ± 1.07
Underweight	0.50	0.31 ± 1.71	0.39 ± 1.82	0.55 ± 1.86	0.48 ± 1.78	0.19 ± 1.96	−0.10 ± 1.68
Normal weight	−0.06	0.39 ± 2.28	0.28 ± 2.40	0.10 ± 2.42	−0.06 ± 2.56	0.76 ± 2.46	0.02 ± 2.47
Overweight	−0.73	−0.32 ± 1.99	−0.21 ± 2.02	−0.58 ± 1.93	−0.15 ± 2.01	−0.31 ± 1.99	0.01 ± 2.14
Obese	−1.12	−0.60 ± 1.32	−0.67 ± 1.36	−0.49 ± 1.30	−0.73 ± 1.36	−0.88 ± 1.25	−0.02 ± 1.32
Girls
Strong underweight	0.84	−0.37 ± 0.73	−0.07 ± 0.77	−0.35 ± 0.72	−0.04 ± 0.71	−0.56 ± 0.73	−0.06 ± 0.72
Underweight	1.79	0.07 ± 1.53	0.07 ± 1.43	0.04 ± 1.06	−0.35 ± 1.55	0.07 ± 1.55	0.02 ± 1.54
Normal weight	0.78	2.45 ± 2.35	1.39 ± 2.40	1.88 ± 2.30	1.89 ± 2.35	2.45 ± 2.28	0.03 ± 2.33
Overweight	−1.91	−0.77 ± 1.87	−0.39 ± 2.09	−0.52 ± 2.00	−0.59 ± 2.00	−0.51 ± 1.84	−0.15 ± 1.89
Obese	−1.49	−1.39 ± 1.18	−1.00 ± 1.26	−1.05 ± 1.21	−0.91 ± 1.25	−1.46 ± 1.25	0.06 ± 1.21

¹Uncorrected difference (prevalence rates of weight status based on self-reports minus prevalence rates based on measured data).

Model A: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (five categories).

Model B: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (three categories).

Model C: age and height_r or weight_r, body perception (five categories).

Model D: age and height_r or weight_r, body perception (three categories).

Model E: age and height_r or weight_r.

Model F: application of the formula of Kurth and Ellert [14].

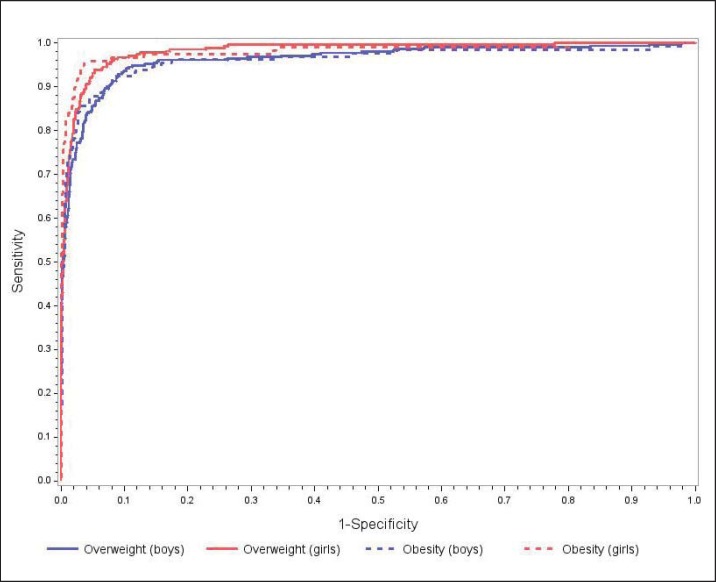
Table 4 shows the AUCs for overweight and obesity. With values close to 1, the accuracy of the prediction is high. Model A and Model B have the highest AUC values. Girls have higher AUC values than boys. For girls also Model A and Model B seems to have the best prediction of the prevalence of overweight and obesity. For boys in all models the AUCs are similar, having the highest values in Model A and Model B. For Model E, AUC was lowest. Figure 1 displays the ROC curves for Model B for overweight and obesity separately by gender.

Table 4.

Areas under ROC curves for Model A to Model E

	Overweight		Obesity
	boys	girls	boys	girls
Model A	0.963	0.982	0.960	0.982
Model B	0.963	0.982	0.961	0.982
Model C	0.960	0.978	0.960	0.972
Model D	0.959	0.976	0.961	0.973
Model E	0.954	0.975	0.959	0.972

Model A: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (five categories).

Model B: linear terms and fractional polynomials of exact age and height_r or weight_r, body perception (three categories).

Model C: age and height_r or weight_r, body perception (five categories).

Model D: age and height_r or weight_r, body perception (three categories).

Model E: age and height_r or weight_r.

Fig. 1.

ROC curves for Model B (including fractional polynomials of age and height_r or weight_r, body perception (three categories)) for overweight and obesity separately for boys and girls.

Discussion

Through the correction procedure, the mean differences between reported and measured height and weight become remarkably smaller and thus the estimated prevalence rates are more accurate. The corrected proportions for overweight and obesity are less under-reported, while the corrected proportions for underweight are less over-reported. This holds except for the proportion of normal weight, which showed the smallest deviation before getting corrected and which is overestimated after the correction procedure. For girls, the formula estimated with Model B (including linear terms and/or fractional polynomials of age and height_r, or weight_r as well as body perception (in three categories)) seems to result in the smallest discrepancies in weight status prevalence rates after correction, whereas for boys all models showed similar results. ROC curves and AUCs confirmed that. Thus, to simplify the correction procedure, the use of the formulas of Model B for girls and for boys is recommended. The formula of Kurth and Ellert [14], which directly corrects the prevalence rates of weight status showed the smallest difference. However, for further analyses, e.g., analyses of association of weight status with socioeconomic status, correction on an individual level with a formula developed in this study is necessary.

The KiGGS study is part of the health monitoring system of the RKI. Recently, a follow-up – KiGGS Wave 1 (2009-2012) – was carried out as a telephone-based survey. The collected self-reports of height and weight need to be corrected in order to evaluate if the reported plateau of prevalence rates for overweight and obesity in children and adolescents [25,26,27,28] will be reflected by the current, nationwide KiGGS Wave 1 data.

In the validation process the formulas were tested out of sample. This resulted in more accurate prevalence rates and shows that the formulas can also be used for other studies which have only collected self-reported height and weight from adolescents in the same age range.

In a previous comparison of adolescents’ self-reported height and weight with measured data, the authors had identified age, gender, and body perception as main predictors of the difference [3], and thus these factors were used for estimating the equations. However, for studies in which no information about the body perception of the adolescents is collected, the equation which does not include body perception (Model E) could be used. Furthermore, for estimation of the correction equations the exact age was used. If only 1-year age groups are available in other studies, the authors recommend to use the mid-year value, for example for the age group of 11-year olds, 11.5 years should be entered into the correction formula.

The comparison of different correction formulas and statistical indices are focused on overweight and obesity and thus on high BMI values. The correction is more accurate in girls even if they over-report their height and under-report their weight to a greater extent than boys. An explanation might be that a satisfied body image leads to less under-reporting of weight [29]. Due to the social desirability of thinness, which is a burden especially in girls [2,11,30], girls are more often unsatisfied with their body (‘too fat’: 55% of the girls vs. 36% of the boys; ‘right weight’: 36% of the girls vs. 45% of the boys) [3]. This might lead to a systematic misreporting of girls. A systematic misreporting is easier to correct than random misreporting, which is more often the case in boys. Boys in this age group grow at a faster pace and thus tend to report outdated height values without a systematic misreporting.

The cross-validation with a random selection of 90% of the study population as training sample and with the remaining 10% as a test sample showed that the equations can be applied to other studies which have collected self-reported height and weight only and which focus on the same age group of 11- to 17-year-olds. After correction, the mean sensitivity for overweight and obesity increased. Before the correction, one fourth of the overweight and one third of the obese adolescents were not classified as such. After correction, less than one fifth of the overweight and obese girls were not identified as such, while in obese boys, still one fourth were not recognized as obese. Specificity did not alter or slightly decreased. These results are in line with previous studies. Jansen et al. [9] also saw an increase of the sensitivity (from 49% to 70%) and decrease of the specificity (from 96% to 89%) for overweight. Landsberg et al. [10] described after correction a higher sensitivity (e.g. for overweight boys from 39% to 67% and for overweight girls from 44% to 50%), specificity which was already between 97 and 100% remained high. In the present study, sensitivity showed remarkably higher values compared to the studies of Jansen et al. [9] and Landsberg et al. [10]. In the study of Jansen et al. [9], the measurement of the adolescents took place around 3 months after they filled in the questionnaire. The participants comprised were in the age of 12-13 years [9], a period in which the growth spurt is very intense [31]. This might be a reason for the strong misreporting. For the study of Landsberg et al. [10], no exact information is given whether the self-reported height and weight was asked before the measurement or if it was asked in the questionnaire completed after the measurement [10,32]. However, in the present study the self-reported values were collected face-to-face, so that gross over- or underestimation was more difficult than in a written questionnaire. Furthermore, many adolescents in this study may have been aware that height and weight would be measured following the self-reports, because a description of the study procedures had been available to the participants on the internet beforehand.

The explained variances of the regression models to estimate the correction formulas ranged between 91 and 95% for height and amounted to 97% for weight. The study of Landsberg et al. [10] showed similar results with an explained variance for height ranging between 89and 91% and for weight ranging between 91 and 93%. The models of Jansen et al. [9], which correct BMI directly instead of correcting self-reported height and weight separately, reached a remarkably lower explained variance (62-68%). In the present study it was also checked whether the correction of BMI directly might lead to more accurate results instead of correcting height and weight separately. However, residual plots showed a more random, non-systematic pattern than for the models which correct height and weight separately, so the latter were considered superior. Additionally, in order to validate the model correcting BMI directly, cross-validation was calculated. The explained variance of the regression model was smaller (88% for boys and 92% for girls). Sensitivity, specificity, and the difference in prevalence rates of weight status between corrected and measured data show no improvement (data not shown). In conclusion, the procedure presented leads to more accurate estimates.

Conclusion

The correction formulas are an appropriate tool to correct self-reported height and weight on an individual level in order to estimate corrected prevalence rates of overweight and obesity in adolescents. For girls, the correction is more accurate than for boys. The developed formulas based on the KiGGS population can be applied to KiGGS follow-up waves as well as to other studies which have collected self-reported height and weight only. The corrected proportions of weight status categories estimated with the formula of Kurth and Ellert showed the smallest deviations from the prevalence rates derived from measured height and weight. However, since this procedure directly corrects the prevalence rates and does not operate on individual level, for analyses of association the correction formulas developed in this study, which work on an individual level, are necessary.

Disclosure Statement

The authors declare that they have no competing interests.