Formal reduction potentials of difluorotyrosine and trifluorotyrosine protein residues: Defining the thermodynamics of multistep radical transfer

Abstract

Redox-active tyrosines (Ys) play essential roles in enzymes involved in primary metabolism including energy transduction and deoxynucleotide production catalyzed by ribonucleotide reductases (RNRs). Thermodynamic characterization of Ys in solution and in proteins remains a challenge due to the high reduction potentials involved and the reactive nature of the radical state. The structurally characterized α₃Y model protein has allowed the first determination of formal reduction potentials (E°′) for a Y residing within a protein (Berry, B. W.; Martínez-Rivera, M. C.; Tommos, C. Proc. Natl. Acad. Sci. U.S.A. 2012, 109, 9739–9743). Using Schultz’s technology, a series of fluorotyrosines (F_nY, n = 2 or 3) was site-specifically incorporated into α₃Y. The global protein properties of the resulting α₃(3,5)F₂Y, α₃(2,3,5)F₃Y, α₃(2,3)F₂Y and α₃(2,3,6)F₃Y variants are essentially identical to those of α₃Y. A protein film square-wave voltammetry approach was developed to successfully obtain reversible voltammograms and E°’s of the very high-potential α₃F_nY proteins. E°′(pH 5.5; α₃F_nY(O•/OH)) spans a range of 1040 ± 3 mV to 1200 ± 3 mV versus the normal hydrogen electrode. This is comparable to the potentials of the most oxidizing redox cofactors in Nature. The F_nY analogs, and the ability to site-specifically incorporate them into any protein of interest, provide new tools for mechanistic studies on redox-active Ys in proteins and on functional and aberrant hole-transfer reactions in metallo-enzymes. The former application is illustrated here by using the determined α₃F_nY ΔE°’s to model the thermodynamics of radical-transfer reactions in F_nY-RNRs and to experimentally test and support the key prediction made.

TOC Image

INTRODUCTION

The basic principles of electron tunneling (ET) in biological oxidation-reduction processes, within and between proteins, have been studied in great detail because of its centrality to so many important reactions.^1–3 These reactions usually involve metallo-prosthetic groups (e.g. hemes, iron-sulfur clusters, non-heme iron and copper cofactors) placed ~ 14 Å apart within the protein environment. The rate constants for electron transfer (k_ET) depend on the electronic coupling of the electron donor and acceptor wave functions (H_AD), the energy required for the reorganization of their nuclear coordinates (λ), and the driving force (ΔG) for the reaction. k_ET is in general very fast and much faster than substrate redox chemistry (k_cat).

Also at the heart of biology are processes that involve the coupling of electron and proton movements, proton-coupled electron transfer (PCET).^4–8 These processes include among others, light-driven H₂O oxidation in oxygenic photosynthesis,⁹ O₂ reduction in cellular respiration,¹⁰ and deoxynucleotide synthesis required to make the building blocks for DNA replication and repair.¹¹ The basic principles of PCET reactions are actively being investigated and draw heavily on our understanding of ET. In PCET, however, the differences in mass between the electron and proton require much more constrained placement of the proton, typically between a H-bonded donor–acceptor pair, and minimization of charge build up in the low dielectric protein medium. The biological examples cited above all involve stable and/or transient tyrosyl radicals (Y–O•) as redox cofactors, which is the focus of this paper. Methods to study the involvement of these species have been limited since the biochemical systems typically have turnover numbers that are governed by conformational gating (i.e. k_cat ≪ k_PCET). Site-specific incorporation of unnatural amino acids (UAA) presents an approach to address this experimental barrier and disclose the underlying chemistry.^11–13 Recently, a tRNA/aminoacyl-tRNA synthetase (RS) pair was evolved to introduce 2,3,5-trifluorotyrosine into any protein of interest.¹⁴ This RS turned out to be polyspecific and to incorporate both di- and tri-substituted fluorotyrosines (F_nY, n = 2, 3; Figure 1). These analogs are of interest for mechanistic studies of aromatic amino-acid PCET or “hole” hopping reactions since the ring substitutions alter the phenol pK_a and reduction potential significantly. Studies on the zwitterionic¹⁵ and blocked forms (N-acetyl-F_n-L-tyrosinamides)¹⁶ have shown that the pK_a is lowered from 10 for Y to the 6.4 – 7.8 range for the F_nYs. Anodic peak potentials (E_peak) obtained from irreversible voltammograms fall both below and above the corresponding E_peak of Y.¹⁶ ΔE_peak spans ~ 150 mV for the F_nY(O•/OH) redox pair and ~ 165 mV for the F_nY(O•/O⁻) couple. These variations offer the opportunity to perturb the biochemical systems such that individual PCET and chemical steps become experimentally accessible. This is illustrated by recent mechanistic studies on E. coli RNR.^17–20

Figure 1.

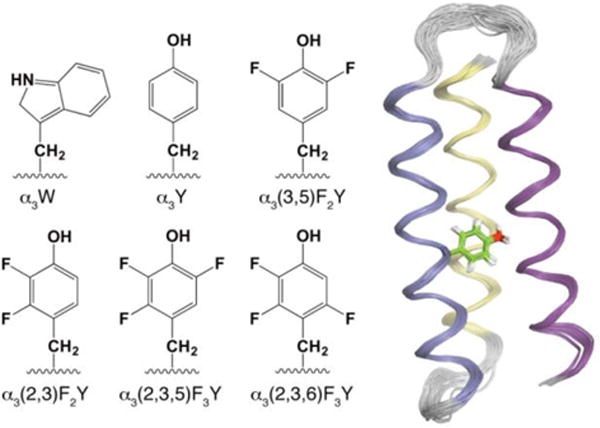
Six members of the α₃X family of de novo designed proteins. The 65-residue sequence common to these proteins: GSR(1)V KALEEKVKALEEKVKA–LGGGGR–IEELKKKX(32)EELKKKIEE–LGGGGE–VKKVEEEVKKLEEEIKK–L(65), forms a three-helix bundle (α₃) with an aromatic residue at a central position (site 32, labeled X).^25,26 The chemical structures display the side chain of residue 32 in α₃W, α₃Y and four α₃F_nY proteins. Solution NMR structures have been obtained for α₃W (PDB ID 1LQ7),²⁷ 2-mercaptophenol-α₃C (2MP-α₃C, 2LXY),²⁸ and α₃Y (2MI7; displayed as a ribbon diagram with the Y₃₂ side chain in stick format).²⁹

The broad utility of the F_nYs is also apparent from recent work on other biological systems. In studies on the photosystem II (PSII) reaction center, for example, 3-FY was globally incorporated to probe changes in the PCET kinetics of the active-site Y_Z residue using time-resolved absorption spectroscopy.²¹ The active site of cytochrome c oxidase (CcO) also contains a redox-active Y species.¹⁰ Lu and coworkers used a series of unnatural Y derivatives, including 3,5-F₂Y and 2,3,5-F₃Y, to study their influence on the reaction mechanism of a CcO model engineered into myoglobin.^22–24 Mechanistic studies revealed that pK_a values of Y and the Y analogs were correlated to O₂ reduction and H₂O production in this model system. Thus, F_nYs are emerging as an important tool to study radical-based protein PCET/hole hopping and to probe and alter active-site chemistry. An essential component currently missing with this approach is the knowledge of the formal reduction potentials (E°′) of the F_nYs measured under reversible conditions and in a protein environment. The main reason the aqueous F_nYs give rise to irreversible voltammograms is because the Y–O• state is unstable on the time scale of the electrochemical measurements. E_peak values reflect the thermodynamics of the redox-active species, but are also influenced by the kinetics of the Y–O• side reactions. Here we use the well-structured α₃X model protein system to stabilize the oxidized state of the F_nYs and render their electrochemical response reversible.

Position 32 is the dedicated radical site in the α₃X proteins (Figure 1).^25,26 This site is occupied by W₃₂, Y₃₂, (3,5)F₂Y₃₂,³⁰ or by a 2-, 3- or 4-mercaptophenol-C₃₂ residue.³¹ Nuclear magnetic resonance (NMR) and circular dichroism (CD) studies have shown that the α₃X proteins are highly helical, stable and well structured across a broad pH range.^25,29–32 Several of the α₃X proteins have been structurally characterized and residue 32 was confirmed to be solvent sequestered in each system.^27–29 The α₃ scaffold does not give rise to a Faradaic current in the absence of a redox-active residue at position 32.^28,31,32 Using square-wave voltammetry (SWV), Tommos and coworkers showed that Y₃₂, 2MP-C₃₂ and (3,5)F₂Y₃₂ can be reversibly oxidized and reduced.^28,30,33 These studies demonstrate that pure thermodynamic potentials, uncompromised by Y–O• side reactions, can be obtained from the α₃X proteins. A photochemical study of α₃Y showed that Y₃₂–O• is formed in a PCET process and slowly (t_1/2 2–10 s) decays via intermolecular radical–radical dimerization. The protein scaffold was shown to stabilize the Y₃₂–O• state by > 10⁴ relative to aqueous Y–O•.²⁹

In this report we refine the SWV approach used in earlier α₃X studies to involve protein film voltammetry (PFV).³⁴ Protein film square-wave voltammograms were collected from α₃Y, α₃(3,5)F₂Y, α₃(2,3)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y (Figure 1) at conditions where the global properties of the five proteins are close to identical. The obtained E°’s of the α₃X proteins are compared to potentials reported for aqueous Y and F_nYs and for one of Nature’s most oxidizing redox cofactor. We find that, remarkably, the 7.5 kDa α₃X model protein can control a redox-active Y as oxidizing as the unique P₆₈₀⁺/P₆₈₀ redox pair, which drives the water-splitting process catalyzed by PSII. The α₃X potentials are also used to construct a thermodynamic model for RNR site-specifically labeled with the F_nYs shown in Figure 1. This model provides important insight into the first step in the multistep radical transfer (RT) process associated with nucleotide reduction in the class Ia RNRs.

MATERIALS AND METHODS

Protein production and spectroscopic characterization

α₃Y was expressed and purified as described earlier.²⁹ 2,3,5-trifluorophenol was synthesized from 2,3,5-trifluorophenylboronic acid using a published protocol, with typical yields of ~ 80%.^20,35 Tyrosine phenol lyase (TPL) was purified as previously reported.³⁶ All F_nYs (n = 2, 3) were enzymatically synthesized from the corresponding phenol using TPL as the catalyst following the published protocol.³⁷ SUMO protease was expressed from pTB145-ulp1 as a His₆-tagged construct as previously reported.³⁸ Construction of the pE-SUMO-α₃TAG₃₂ plasmid, optimization of the protein expression conditions, and the α₃F_nY protein purification protocol are described in the Supporting Information (SI). His₆-Y₇₃₁F-α2 was expressed and purified following the published protocol for wild type (wt) α2.¹⁴ 2,3-F₂Y₁₂₂•-β2 was expressed, purified and reconstituted as previously described.¹⁸ Experimental conditions for all spectroscopic studies are described in detail in the SI.

PFV

SWV was performed using an Autolab PGSTAT12 potentiostat (Metrohm/Eco Chemie) equipped with a temperature-controlled, Faraday-cage protected three-electrode micro-cell (Princeton Applied Research). The Ag/AgCl reference electrode and the platinum wire counter electrode (Advanced Measurements Inc.) were prepared by filling the former with a 3M KCl/saturated AgCl solution and the latter with 20 mM APB, 40 mM KCl. All measurements were carried out using a 3 mm diameter pyrolytic graphite edge (PGE) working electrode (Bio-Logic, USA). CD spectroscopy was used to determine the protein concentration in the α₃F_nY samples used to prepare the protein films. Samples were prepared by dissolving freeze-dried protein in buffer, measuring the 222 nm ellipticity (1 mm path) and calculating the protein concentration using the [Θ]₂₂₂ values listed in Table S1. The final protein concentration was 45 – 80 μM. The electrode surface was prepared for protein adsorption by manually polishing its surface for 60 s in a 1.0 μm diamond/water slurry on a diamond polishing pad (Bio-Logic, USA) followed by 60 s in a 0.05 μm alumina/water slurry on a microcloth pad (Bioanalytical systems Inc.). The electrode surface was rinsed with methanol followed by an excess of milli-Q water (18 MΩ). The pH in the protein samples and in the electrolyte buffers were matched and measured prior to and after data collection. Solution resistance was compensated for by using the Autolab positive feed-back iR compensation function. Potentials are given versus the normal hydrogen electrode (NHE). Buffers and protein samples were prepared using ultra pure chemicals (Sigma Aldrich) and data recorded under an argon atmosphere. Voltammograms were collected using the GPES software (Metrohm/Eco Chemie) and analyzed using PeakFit (Systat Software Inc.).

RESULTS

α₃F_nY protein expression and purification

Construction and optimization (Figure S1) of the α₃F_nY protein expression system are described in the SI. The α₃F_nY yield was typically a few mg per L culture, except for α₃(2,3)F₂Y where it was significantly lower. Figure S2 shows analytical C18 chromatograms and MALDI-TOF traces of purified α₃(2,3)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y. The protein preparations are homogenous and display the correct α₃F_nY molecular weights. Equivalent data for purified α₃(3,5)F₂Y were presented in an earlier study.³⁰

Protein characterization

A key objective for the α₃X approach is to obtain a homogenous set of E°′(radical/amino acid) protein potentials, including the potentials for the F_nY₃₂ residues. Practically this translates into: (i) determining the experimental conditions where the active redox couple is well defined and the α₃ scaffold structurally unperturbed, and (ii) obtaining reversible protein voltammograms at these conditions. The phenol pK_a’s of aqueous N-acetyl-F_n-L-tyrosinamides (n = 2, 3) range from 6.4 to 7.8.¹⁶ α₃F_nY oxidation-reduction may thus involve Y₃₂(O•/OH), Y₃₂(O•/O⁻) or a pH-weighted mixture of these two redox pairs. The UV-Vis and CD studies described here were conducted to determine a common pH range where F_nY₃₂(O•/OH) is the dominant redox couple and the structural properties of the four α₃F_nYs (Figure 1) are minimally perturbed relative to α₃Y.

The apparent pK_a (pK_app) of X₃₂ was determined to be 8.0 ± 0.1 for α₃(3,5)F₂Y,³⁰ 7.2 ± 0.1 for α₃(2,3,5)F₃Y and 7.9 ± 0.1 for α₃(2,3,6)F₃Y (Figure 2; Table 1). The F_nY₃₂ residues absorb poorly in the UV region¹⁶ making the absorption-monitored pH titrations very material demanding. For α₃(2,3)F₂Y, the amount of material precluded this measurement. The protein-induced increase in the phenol pK_a was very similar for α₃(3,5)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y, with an average ΔpK_a of 0.83 ± 0.06 (Table 1). We assume that a shift of similar magnitude occurs for α₃(2,3)F₂Y and a pK_app of 8.6 (7.8 + 0.8) was predicted for this protein (Table 1).

Figure 2.

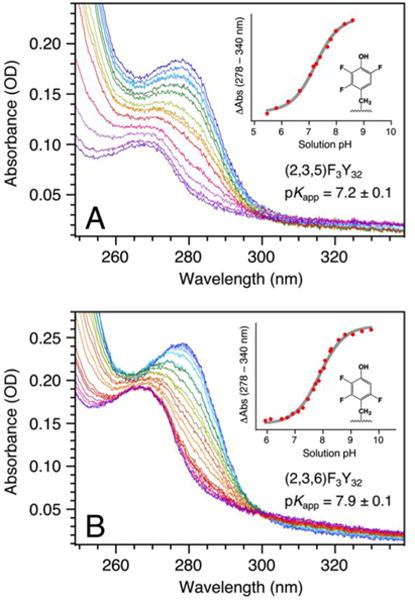
UV-Vis spectra and corresponding pH-titration curves for (A) α₃(2,3,5)F₃Y and (B) α₃(2,3,6)F₃Y. The α₃(2,3,5)F₃Y spectra were incrementally collected from pH 8.59 (dark blue) to pH 5.46 (dark purple) and back to pH 7.04 (red); while the α₃(2,3,6)F₃Y spectra were collected from pH 9.72 (dark blue)to pH 5.94 (dark purple) and back to pH 7.11 (red). The titration plots were fit to a single pK_a.

Table 1.

pK_app and E_net values for α₃Y and α₃F_nY (n = 2, 3)

System	pKapp	Enet (mV) (pH)d	ΔEnet (mV)d
α3(3,5)F2Y	8.0a	1040 ± 3 (5.49 ± 0.03)	− 25
α3Y	11.b	1065 ± 2 (5.53 ± 0.05)	0
α3(2,3,5)F3Y	7.2	1104 ± 2 (5.54 ± 0.05)	+ 39
α3(2,3)F2Y	8.6c	1136 ± 2 (5.57 ± 0.09)	+ 70
α3(2,3,6)F3Y	7.9	1200 ± 3 (5.54 ± 0.05)	+135
System	pKae	DPV ΔEpeak (mV)f	CV ΔEpeak (mV)g
3,5-F2Y	7.2	− 51	− 171h
Y	10	0	0h	0i
2,3,5-F3Y	6.4	+ 5	− 122h	−111i
2,3-F2Y	7.8	+ 44		− 39i
2,3,6-F3Y	7.0	+ 97

^aRef. 30.

^bRef. 25.

^cPredicted value, see Results.

^dE_net = E°′, see Discussion.

^eAqueous N-acetyl-F_n-L-tyrosinamides.

^fFor the Y(O•/OH) redox couple, Seyedsayamdost et al., Ref. 16.

^gCalculated for the Y(O•/OH) redox couple, see Discussion.

^hLu and coworkers, Ref. 23.

ⁱMahmoudi et al., Ref. 46.

CD spectroscopy was used to determine the α-helical contents and the global stabilities of the α₃F_nYs. Figure 3A compares the CD spectra of α₃(2,3)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y to the reference spectrum of α₃W at pH 5.05 ± 0.01. The CD spectra are plotted in the normalized mean residue molar ellipticity form (see Fig. 3 legend) where the 222 nm amplitude ([Θ]₂₂₂) scales with the helical content. The spectra show that altering the Y₃₂ ring has little impact on the helicity of the α₃ scaffold. The α-helical contents of α₃(2,3)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y were calculated from their CD spectra using the α₃W spectrum as the reference. [Θ]₂₂₂ values and corresponding α-helical contents are listed in Table S1.

Figure 3.

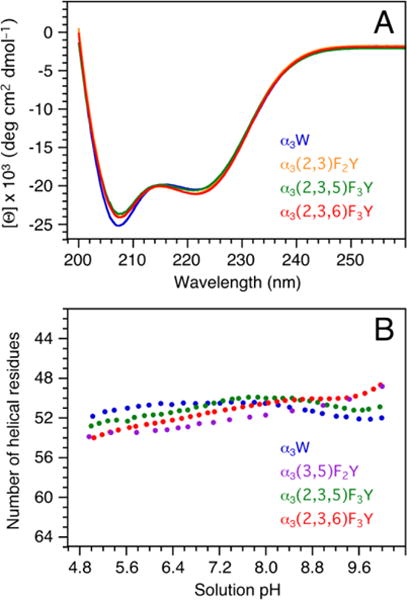
(A) Far-UV CD spectra of α₃(2,3,5)F₃Y (green), α₃(2,3)F₂Y (orange), α₃(2,3,6)F₃Y (red), and α₃W (blue; reference spectrum). The proteins were dissolved in 40 mM sodium acetate and the final sample pH measured to 5.05 ± 0.01. The spectra are displayed in units of mean residue molar ellipticity ([Θ]) obtained by: [Θ] = θ_obs10⁶/Cln where θ_obs is the observed ellipticity in millidegrees, C the protein concentration in μM, l the cuvette path length in mm (2), and n the number of amino-acid residues (65). C was determined using a fluorescence-based assay (SI). (B) pH-induced changes in the α-helical contents of α₃(3,5)F₂Y (purple; reproduced from Ref. 30), α₃(2,3,5)F₃Y (green), α₃(2,3,6)F₃Y (red) and α₃W (blue; reference data^25,27).

Figure 3B displays changes in the total number of α-helical residues in α₃(3,5)F₂Y,³⁰ α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y as a function of pH. A pH plot of α₃W is also shown (51 ± 0.6 α-helical residues, pH 4 – 10).^25,27 The pH-induced structural changes are minor and the α₃F_nYs have the same or somewhat higher α-helical content than α₃W below pH 6.8. The global stability of α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y were determined by urea denaturation at pH 5.0 and 5.5. Representative data are shown in Figure S3 and protein stability values for α₃Y,^29,32 α₃(3,5)F₂Y,³⁰ α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y are summarized in Table S1. For the pH 5.0 – 5.5 range, there is no significant difference in the global stability of α₃Y, α₃(3,5)F₂Y and α₃(2,3,5)F₃Y while α₃(2,3,6)F₃Y is 0.4 kcal mol⁻¹ more stable than α₃Y. The minimal variations in α-helical content and global stabilities of the α₃F_nYs relative to α₃Y are in accordance with previous observations in other proteins.³⁹ Fluorination of amino acids has been shown to result in minimal structural perturbations to the global protein fold.^40,41 Additionally, the increased hydrophobicity resulting from fluorination usually enhance protein stability.^39,42,43

We conclude that the common pH range sought for the α₃F_nY voltammetry studies is limited by the pK_app of α₃(2,3,5)F₃Y (7.2; to drive the system towards Y₃₂(O•/OH) rather than by considerations of the structurally robust α₃ scaffold.

Protein film square-wave voltammetry on the α₃F_nY proteins

SWV is a pulse voltammetric technique supported by well-developed theoretical models for diffusion-controlled and surface-confined electrode mechanisms.^44,45 SWV was used to obtain formal E°′(radical/amino acid) protein potentials for α₃Y,³³ 2MP-α₃C,²⁸ and α₃(3,5)F₂Y.³⁰ The α₃X potentials are pH dependent and measurements performed at low pH are more difficult due to the higher potentials and more pronounced background currents involved.³³ SWV on α₃(2,3,5)F₃Y, α₃(2,3)F₂Y, and α₃(2,3,6)F₃Y was predicted to be challenging since measurements had to be done ~ pH 5.5 (≥ 1.7 pH units below the pK_app’s of the α₃F_nY proteins) and test studies on α₃(2,3,5)F₃Y placed E°′ well above +1 V. Voltammetry data from an on-going parallel project involving an α₃(NH₂)Y variant (Lee, Nocera, Stubbe and Tommos) suggested that a protein film approach, where the protein is introduced to the surface of the working electrode outside the electrochemical cell, improves the Faradaic response of the α₃X system. A protein film protocol was developed for α₃Y and applied to the α₃F_nY proteins. This approach allowed us to obtain high-quality reversible voltammograms in a potential range unprecedented for protein voltammetry and to determine the formal potentials of the highly oxidizing α₃F_nYs.

In SWV the applied potential is stepped progressively in fixed increments (E_step), and at each increment, a forward (here oxidative) potential pulse is applied followed by a reverse (reductive) pulse. The current is sampled at the end of each alternating pulse and plotted as a function of E_step. This generates a forward (I_for), a reverse (I_rev) and a net (I_net = I_for – I_rev) voltammogram. The time period (τ) over which the electrode reaction is driven in the anodic and then cathodic direction is set by the SW frequency (f = 1/τ).^44,45 The apparent redox reversibility of a surface-adsorbed redox pair is described by a kinetic parameter (ω) defined as the ratio of the surface standard ET rate constant (k_sur) and the SW frequency (ω = k_sur/f). Theory predicts that the strongest response from an adsorbed redox pair occurs when f is close to k_sur.⁴⁷ In earlier reports, the Faradaic response of α₃Y and 2MP-α₃C were investigated over a broad frequency range of 30–960 Hz and 30–720 Hz, respectively.^28,33 Figure S4 shows I_net of α₃Y (blue) and 2MP-α₃C (red) divided by f, and the obtained I_net/f amplitudes plotted against the frequency. The parabolic shape of the frequency-normalized data is consistent with the “quasi-reversible maximum” (QRM) feature of surface-confined redox reactions.⁴⁷ The α₃Y and 2MP-α₃C plots are almost identical with a QRM at 440 Hz. The α₃F_nYs were anticipated to behave in a similar fashion and display the strongest Faradaic response around 440 Hz. Test studies on α₃(2,3,5)F₃Y confirmed that the most pronounced response was observed in a rather narrow frequency range of ~ 400 to 450 Hz. The response was poor above and below this range (data not shown). SWV on the α₃F_nY proteins were thus conducted using a SW frequency of 440 Hz.

Background-corrected protein film SW voltammograms of α₃Y are displayed in Figure 4A. The forward, reverse and net currents are colored orange, purple and blue, respectively. The measurement was performed as follows: The surface of the PGE working electrode was polished (see Material and Methods for details) and introduced to a 500 μl 80 μM α₃Y, 20 mM APB, 75 mM KCl, pH 5.5 sample for 30 s. The electrode was transferred to a blank electrochemical cell containing 3 mL 20 mM APB, 40 mM KCl, pH 5.5 and a voltammogram recorded. This procedure was repeated twice to collect data replicas a and b (data panels A and B) and on a separate experimental day using an independently prepared protein sample (panel C). The maximum amplitude of I_net was investigated as a function of the electrode surface/α₃Y sample incubation time and found stable after about 20 s. No increase in I_net was observed at incubation times > 30 s.

Figure 4.

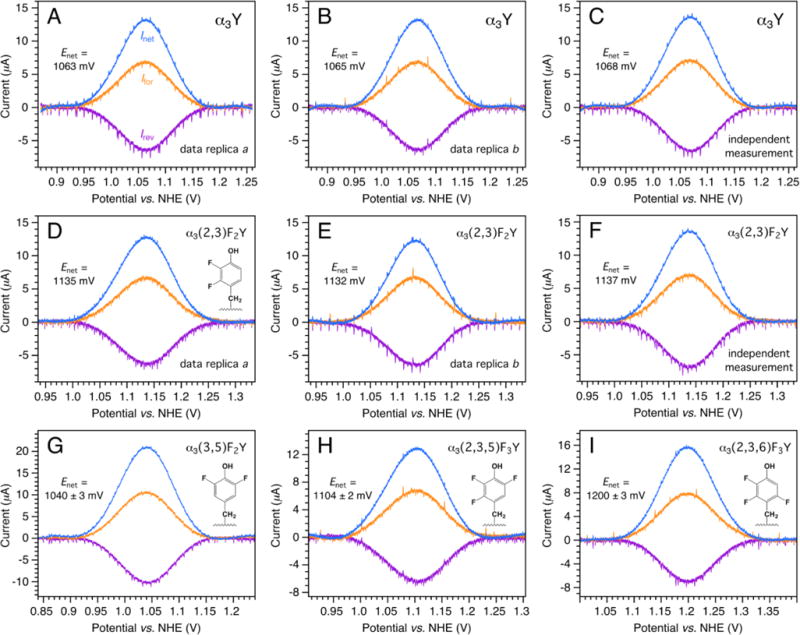
Background-corrected protein film SW voltammograms of (A–C) α₃Y, (D–F) α₃(2,3)F₂Y, (G) α₃(3,5)F₂Y, (H) α₃(2,3,5)F₃Y, and (I) α₃(2,3,6)F₃Y. The proteins were adsorbed onto the surface of a PGE electrode and voltammograms recorded in 20 mM APB, 40 mM KCl, pH 5.54 ± 0.06 buffer at 25 °C. SWV settings: Equilibration time 5 s, step potential 0.15 mV, SW pulse amplitude 25 mV, SW frequency 440 Hz.

The protein film protocol described above was applied to α₃(2,3)F₂Y and the resulting voltammograms are displayed in the middle row of Figure 4. The corresponding uncorrected data for the baseline-subtracted α₃Y (Figures 4A–C) and α₃(2,3)F₂Y (Figures 4D–F) voltammograms are shown in Figures S5 and S6, respectively. Data processing and analysis details are provided in the SI. As shown in Figures 4, S5 and S6, the voltammograms are recorded at very high positive potentials and the background currents are significant. Nonetheless, the I_for, I_rev and I_net waveforms are well defined and their position, lineshape and amplitude are highly reproducible between data replicas and independent measurements. Representative protein film voltammograms collected from α₃(3,5)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y are shown in Figure 4 panels G, H, and I, respectively. The pH in the protein samples and in the electrolyte solutions were measured at the beginning and at the end of each experimental day. The average pH, E_net and ΔE_net values are listed in Table 1.

Summarizing the redox properties of the α₃F_nY proteins as E_net vs. pH diagrams

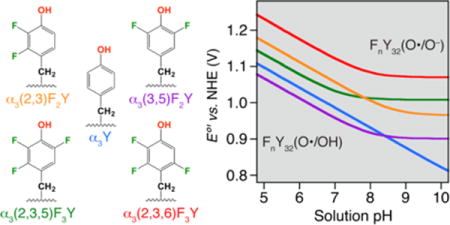
A set of E_net vs. pH diagrams was predicted for the α₃F_nYs based on the results described above combined with the established charge-neutral response of the α₃X redox system.^28,31,33 The plots shown in Figure 5 were constructed from the following data: (i) The four α₃F_nY E_net pH 5.5 values listed in Table 1 (single dots colored purple, green, orange and red). (ii) The α₃Y E_net pH 5.53 ± 0.05 value listed in Table 1 plus three additional PFV E_net values obtained at pH 6.94 ± 0.07, 8.40 ± 0.10 and 9.94 ± 0.08 (blue dots; Figure S7). The pH dependence (55.2 mV/pH unit) of the Y₃₂(O•/OH) potential is consistent with an overall charge-neutral redox cycle where the 1e⁻ oxidation-reduction process driven by the electrode is coupled to the release/uptake of essentially one full H⁺ to/from bulk.³³ (iii) α₃(3,5)F₂Y E_net measured between pH 4.95 ± 0.01 and 6.49 ± 0.02 (grey dots). The pH titration was done on α₃(3,5)F₂Y since it has a higher pK_app than α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y, and expresses better than α₃(2,3)F₂Y. Additionally, the potential is lower than for the other α₃F_nYs making the measurements less challenging. The pH titration was done with α₃(3,5)F₂Y in the electrochemical cell (details provided in the Figure 5 legend). The pH dependence in E_net (55.7 mV/pH unit) was used to predict the E_net vs. pH curves for all of the α₃F_nYs. (iv) The pK_app’s of α₃(3,5)F₂Y, α₃(2,3,5)F₃Y and α₃(2,3,6)F₃Y and (v) the predicted pK_app of α₃(2,3)F₂Y (Table 1). The Nernst equations and parameters used to model the diagrams are listed in Table S2. Table S2 also lists the α₃F_nY potentials at pH 7.0 as a general reference.

Figure 5.

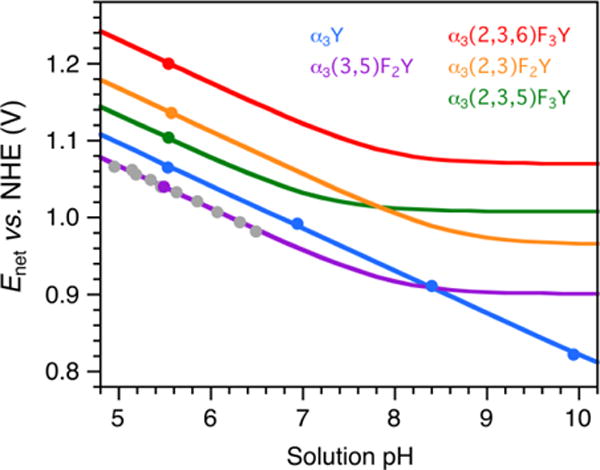
E_net vs. pH diagrams for α₃Y and the α₃F_nY proteins. Solid dots represent E_net values obtained by PFV. Grey dots represent E_net collected from α₃(3,5)F₂Y using the following conditions: 70 μM protein, 20 mM APB, 75 mM KCl; pH adjusted with 1 M NaOH; step potential 0.15 mV, SW pulse amplitude 25 mV, SW frequency 190 Hz, and temperature 25 °C.

DISCUSSION

The α₃X system combined with protein film SWV facilitates studies of “hot” radicals

The experimental advance made here was driven by the very “hot” nature of the F_nY₃₂ radicals and the necessity of recording reversible voltammograms at exceedingly high potentials. This issue was addressed by employing PFV.³⁴

Voltammograms collected at high positive potentials contain capacitive currents, background currents from the oxidation of the solvent, supporting electrolytes and/or chemical groups on the surface of the working electrode (WE), in addition to the Faradaic current of the redox-active species under investigation. The effects of capacitive currents can be minimized by using pulse voltammetric techniques, such as differential pulse voltammetry (DPV) or SWV. Background currents from solvent oxidation can be reduced by using a carbon, rather than a metal-based, WE but they cannot be eliminated. Prominent background currents are unavoidable when doing high-potential voltammetry on aqueous systems and they rapidly increase above ~ 1 V. Thus, an increase in E°′ that would be insignificant when probing a 0.5 V protein oxidant may prove challenging, even prohibiting, when probing a protein oxidant in the +1.0 V range. Berry et al. used SWV and a PGE electrode to optimize the α₃Y response and showed that Y₃₂ can be reversibly oxidized and reduced.³³ Here we show that the α₃Y response improves even further when the protein is adsorbed onto the surface of the PGE electrode outside the electrochemical cell. The protein film samples gave rise to Faradaic currents that are easily identified above the background (Figures S5 and S6). Baseline-corrected traces with well-defined peak positions and lineshapes were reproducibly obtained despite the high potentials involved (Figure 4; Table 1). The protein film SW voltammograms collected from α₃Y and the α₃F_nYs display an I_for/I_rev close or equal to unity and an E_for – E_rev peak separation of − 2 ± 2 mV. This is consistent with a strongly adsorbed redox pair for which the surface standard ET rate constant k_sur is close to the SW frequency (f = 440 s⁻¹) and E_net equals E°′.⁴⁸

The α₃X potentials reflect the local protein environment

Previous DPV and SWV studies were conducted with the α₃X proteins dissolved in the supporting electrolyte solution.^28,30–33 The characteristics of their Faradaic response suggested that diffusion-controlled reactions dominated the electrode process.^28,33 As described above, the I_net amplitude stabilizes in less than 20 s of exposing the surface of the PGE electrode to an α₃Y sample. Data collected with the protein dissolved in the electrolyte solution would typically be started on this time scale after the freshly polished WE was placed in the cell. Previously published α₃X voltammograms thus most likely represent a mixed contribution of surface-adsorbed and diffusional species.

Figure 5 shows a α₃Y E_net vs. pH diagram constructed from PFV data. All previously reported α₃Y potentials recorded over a broad range of conditions (method, SWV or DPV; WE material, pyrolytic graphite or glassy carbon; [protein] 2 – 210 μM; [KCl] 10 – 140 mM; pH 5.5 – 10; SW frequency 190 – 960 Hz)³³ were plotted against values predicted from the α₃Y PFV E_net vs. pH diagram in Figure 5. The plot goes through zero and displays a linear correlation of 1.001 ± 0.002 and a standard error of < ± 7 mV (not shown). Thus, there is no significant change in the observed potential when the system shifts from mixed surface/diffusional to a pure surface mechanism. This is also true for α₃(3,5)F₂Y (E_net(pH 5.70) 1026 ± 4 mV in Ref. 30 and 1028 ± 3 mV in Figure 5). These observation are consistent with the buried position of Y₃₂²⁹ where the Y₃₂(O•/OH) potential is only influenced by local protein interactions and not by external factors such as charges on the surface of the WE.

Interestingly, there is a symmetry to the α₃F_nY ΔE°’s (Table 1). When the common component (2-FY = 6-FY, i.e. F attached to either of the two ring delta carbons) is isolated by pairing the four independently obtained E°’s, the same value is obtained:

$${\mathrm{\alpha }}_3\left(2,3,5\right){\mathrm{F}}_3\mathrm{Y}\left(1104\text{mV}\right)–{\mathrm{\alpha }}_3\left(3,5\right){\mathrm{F}}_2\mathrm{Y}\left(1040\text{mV}\right)=64\text{mV}$$

$${\mathrm{\alpha }}_3\left(2,3,6\right){\mathrm{F}}_3\mathrm{Y}\left(1200\text{mV}\right)–{\mathrm{\alpha }}_3\left(2,3\right){\mathrm{F}}_2\mathrm{Y}\left(1136\text{mV}\right)=64\text{mV}$$

We interpret this to show that the impact of the local protein environment on the F_nY₃₂(O•/OH) E°’s is equal across the four proteins. If the asymmetrically labeled F_nY₃₂ side chains have multiple rotameric states,^18,49 it has little influence on their E°’s.

E⁰′(Y(O•/OH)) increases by about 45–65 mV when placed in a hydrophobic protein environment

Zwitterionic and blocked Y have been studied by pulse radiolysis,^50–52 cyclic voltammetry (CV),^23,46,53,54 DPV^25,54,55 and SWV.⁴⁶ The reported pH 7.0 potentials span a considerable range, 830 – 970 mV, which reflects a number of issues. In pulse radiolysis, equilibrium is established between a reference redox couple and the redox couple of interest. For accurate readings, equilibrium must be established on a time scale that is fast relative to Y–O• decay. Additionally, some of the reference potentials used in the older literature were not as well-determined as they are today.⁵⁶ Aqueous Y gives rise to irreversible voltammograms since Y–O• dimerization is fast (k_dim 2–7 × 10⁸ M⁻¹ s⁻¹)^29,57 relative to the experimental time scale. These voltammograms, which display only an anodic wave, may reflect a reversible, quasi-reversible or irreversible electrode reaction. If the electrode reaction is shown to be reversible,⁵⁸ then the anodic peak potential can be corrected for k_dim and an apparent formal potential derived.^58,59 Harriman⁵³ and DeFelippis et al.⁵⁴ applied this approach but unfortunately used an incorrect equation to compensate for k_dim.⁴⁶ Recently reported (or recommended) pH 7.0 potentials for aqueous Y are 0.93 ± 0.02 V,⁵² 0.91 ± 0.02 V,⁵⁶ and 0.96 V.⁴⁶ We therefore take a consensus value of 920–940 ± 20 mV for the aqueous Y(O•/OH) redox couple at pH 7.0. E°′ of Y₃₂(O•/OH) is 986 ± 3 mV at pH 7.0 (Figure 5; Table S2). Thus, the potential of the Y(O•/OH) couple increases by ~45–65 ± 20 mV when Y is buried inside α₃Y.

Comparing F_nY potentials obtained at reversible and irreversible conditions

Tommos et al.²⁵ and the Stubbe and Nocera groups^11,16,55 have reported raw DPV potentials for Y, W and Y analogs. The focus of these studies was to obtain comparable peak potentials for Y vs. W,²⁵ Y vs. NO₂Y,⁵⁵ and Y vs. F_nYs.¹⁶ Tommos and collaborators are using the unique α₃X system to refine and solidify these ΔEs by comparing the true thermodynamic E°’s of Y₃₂,³³ W₃₂ (Tommos, Hammarström, in preparation), NO₂Y₃₂ (Tommos, on-going) and F_nY₃₂ (this study). Table 1 displays absolute and relative E°’s for α₃Y and the α₃F_nYs proteins and compares the latter to ΔE_peak values obtained by DPV. The absolute potentials differ by ~ 168 mV (α₃Y – Y)^25,55 and ~ 193 mV (α₃F_nY – F_nY).¹⁶ These differences reflect the water vs. protein medium (~ 45–65 ± 20 mV, vide supra), a DPV pulse amplitude factor (25 mV), and peak shifts due to the irreversibilty of the aqueous systems (~ 90 ± 10 mV for Y and ~ 115 ± 10 mV for the F_nYs). Nonetheless, the relative potentials reported in Ref. 16 fit rather well with the α₃X data. The oxidizing power of the Ys increases in the same order (3,5-F₂Y < Y < 2,3,5-F₃Y < 2,3-F₂Y < 2,3,6-F₃Y) and the overall span (E(2,3,6-F₃Y) – E(3,5-F₂Y)) is 160 mV and ~ 150 mV for the α₃F_nYs and F_nYs, respectively. One significant difference between the α₃X ΔE° scale and the DPV ΔE_peak scale in Table 1 is that the four E_peak(F_nY)s are downshifted by ~ 30 mV relative to E_peak(Y). This does not represent a true difference in potential between the protein and aqueous systems but rather reflects the limitations of relying on irreversible voltammograms. Recently, Lu and coworkers^22,23 and Mahmoudi et al.⁴⁶ used CV to measure the difference in the anodic peak potential of irreversible voltammograms obtained from aqueous 3,5-F₂Y, 2,3,5-F₃Y and 2,3-F₂Y relative to Y. Overall, there is little consensus in the values reported for the aqueous systems with ΔE_peak(pH 7.0; 3,5-F₂Y – Y) ranging from −158 to −60 mV^11,23 ΔE_peak(pH 7.0; 2,3,5-F₂Y – Y) from −81 to +30 mV,^11,23,46 and ΔE_peak(pH 7.0; 2,3-F₂Y – Y) from −30 to +30 mV.^11,46 Table 1 lists CV ΔE_peak values for the Y(O•/OH) redox couple (i.e. at pH < 2 units below the phenol pK_a’s) as calculated from the reported pH 7.0 values.^23,46 This is to provide a more direct comparison to the α₃X(O•/OH) ΔE°’s. The large discrepancies observed for the ΔE_peak values highlight the importance of measuring the potentials of reactive radicals under reversible conditions such as those acheived here.

Using the α₃X system to model the initial RT step in F_nY₁₂₂•-β2: PCET or ET?

Below we explore the general use of the α₃X potentials by applying them as an analytical tool to investigate RT in E. coli RNR. This enzyme consists of two homodimeric subunits, α2 and β2, which form the active α2β2 complex.¹¹ β2 contains a stable diferric-Y₁₂₂• cofactor that reversibly generates a transient thiyl radical in α2 (C₄₃₉•; Figure 6). Once formed, C₄₃₉• initiates the catalytic nucleotide reduction process. Each turnover involves multistep RT via Y₁₂₂–O• ⇆ [W₄₈?] ⇆ Y₃₅₆ in β2 and Y₇₃₁ ⇆ Y₇₃₀ ⇆ C₄₃₉ in α2 (Figure 6). Slower conformational gating masks the forward and reverse RT steps and the transient Y₃₅₆•, Y₇₃₀• and Y₇₃₁• species are not observed in wt RNR. Site-specific incorporation of NO₂Y and F_nY residues proved to be a successful approach to perturb the system so that the pathway Y radicals can be observed.^11,61,62 The following ΔE°’s were obtained from the radical distribution patterns at equilibrium: ΔE°′(pH 7.6; 2,3,5-F₃Y₁₂₂• – Y₃₅₆•) = 20 ± 10 mV, ΔE°′(pH 8.2; Y₁₂₂• – 3,5-F₂Y₃₅₆•) = −70 ± 5 mV, and ΔE°′(pH 7.6; Y₃₅₆• – Y₇₃₁•) = ~ −100 mV at 25 °C.^62,63 Taken together these ΔE°’s sketch out the thermodynamic landscape associated with the intersubunit RT pathway in E. coli RNR (Figure S8).⁶³

Figure 6.

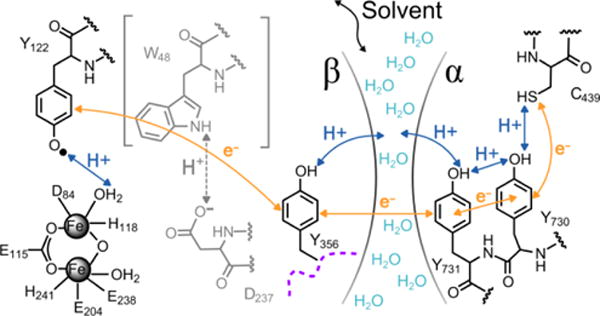
Radical transfer pathway in E. coli RNR. The arrows represent electron (orange) and proton (blue) movements within the α2 and β2 subunits. There is currently no evidence for the involvement W₄₈ (grey) or its proposed proton acceptor D₂₃₇ (grey) in RT. The water interface between α2 and β2 is suggested in Ref. 60.

In the analysis presented below, we combine the α₃X potentials with two of the RNR ΔE°’s listed above to construct a thermodynamic model for sites Y₁₂₂ and Y₃₅₆ in wt and F_nY-RNRs. The modeling addresses the question if the initial RT step in 2,3,5-F₃Y₁₂₂•-β2 is PCET (established for wt RNR; Figure 7A)⁶⁴ or ET (established for NO₂Y₁₂₂•-β2: Figure 7B)⁶¹ and if this process is identical between the different F_nY₁₂₂•-β2 systems.

Figure 7.

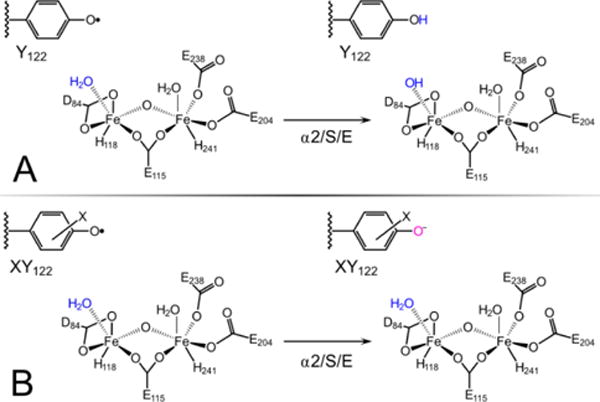
Initiation of RT when (A) wt-β2 or (B) XY-β2 (where X is NO₂ or F_n) reacts with α2, substrate CDP (S) and allosteric effector ATP (E). (A) Reduction of wt Y₁₂₂–O• by Y₃₅₆ is coupled with proton transfer (PT) from Fe1-H₂O.⁶⁴ (B) Reduction of NO₂Y₁₂₂–O• (or F_nY₁₂₂–O•) by Y₃₅₆ is not coupled to PT from the diiron cluster.

Modeling of RNR sites 356 and 122

Figure 8 displays a thermodynamic model for sites 356-β2 (Panel A) and 122-β2 (Panel B) in wt and F_nY-RNRs. The Y₃₅₆ site was modeled by using the absolute α₃X potentials (Figure 5) and phenol pK_a‘s that were upshifted by 0.4 relative to the aqueous F_nYs (Figure 8A). The modeled potentials are pH dependent since the proton released upon Y₃₅₆ oxidation is in rapid exchange with the bulk solvent.⁶³ The pK_a values are based on the 0.4 increase in the pK_a of NO₂Y₃₅₆.⁶⁵ The modeling of site 122 was more involved and is described in a stepwise manner below and in Figure 8B.

Figure 8.

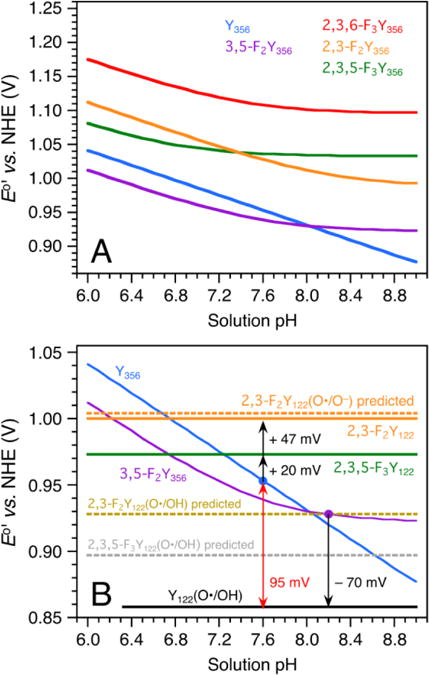
Thermodynamic model for sites Y₁₂₂ and Y₃₅₆ in wt and F_nY-RNR. (A) Predicted E°′ vs. pH diagrams for residue Y₃₅₆ in the wt RNR and in mutants containing F_nY₃₅₆ (n = 2, 3) residues. Y₃₅₆ is modeled with a pK_a of 10.4, which makes Y₃₅₆(O•/OH) the dominant redox couple across the displayed pH range. The F_nY₃₅₆ residues are modeled with lower pK_a’s (3,5-F₂ 7.6; 2,3,5-F₃ 6.8; 2,3-F₂ 8.2; 2,3,6-F₃ 7.4) and their potentials are described by a pH-weighted mixture of the F_nY₃₅₆(O•/OH) and F_nY₃₅₆(O•/O⁻) redox pairs. (B) Modeled E°’s for residue Y₁₂₂ in the wt and F_nY₁₂₂-RNRs. The Y₃₅₆ (blue) and 3,5-F₂Y₃₅₆ (purple) E°′ vs. pH diagrams are superimposed from Panel A. The black arrows represent experimentally obtained ΔE°’s (Figures S8 and S9).⁶³ A +95 mV step is predicted for RT from Y₁₂₂(O•/OH) to Y₃₅₆ (O•/OH) in wt RNR (red double arrow). The E°’s are modeled for 25 °C.

(1) In wt RNR, Y₁₂₂(O•/OH) is the active redox couple and the proton toggles between the phenol oxygen and the Fe1(H₂O/OH) species (Figure 7A).⁶⁴ The site is effectively not in communication with bulk and is therefore modeled with pH-independent potentials. The Y₃₅₆ (blue) and 3,5-F₂Y₃₅₆ (purple) E°′ vs. pH diagrams displayed in Figure 8A are reproduced in Figure 8B. By combining these Y₃₅₆ plots with the RNR ΔE°’s obtained in Ref. 63 (illustrated by black arrows), the potentials of sites 356 and 122 were linked as follows: The potential of 2,3,5-F₃Y₁₂₂ was obtained by taking the predicted E°′(Y₃₅₆(O•/OH)) pH 7.6 value (953 mV, blue dot) and making E°′(2,3,5-F₃Y₁₂₂) 20 ± 10 mV more oxidizing (973 mV; green line). Likewise, the potential of Y₁₂₂(O•/OH) was obtained by taking the predicted E°′(3,5-F₂Y₃₅₆) pH 8.2 value (928 mV, purple dot) and making E°′(Y₁₂₂(O•/OH)) 70 ± 5 mV less oxidizing (858 mV; black line). Importantly, this model predicts a +95 mV uphill step between wt Y₁₂₂ and Y₃₅₆ (at pH 7.6 and 25 °C; red double arrow).

(2) The α₃X ΔE°′(pH 5.5) scale in Table 1 mainly reflects changes in the Y₃₂ fluorination pattern since the other key properties of the redox system (overall charge neutral redox cycle; buried hydrophobic site) are the same between α₃Y and the α₃F_nYs. This, in turn, suggests that the α₃X ΔE°’s can be used as a diagnostic tool. A RNR ΔE°′ that deviates significantly from the corresponding α₃X ΔE°′ is an indicator that some property, in addition to the Y fluorination pattern, has changed. Table 1 predicts a 39 mV difference (ΔE°′(α₃(2,3,5)F₃Y – α₃Y)) between Y₁₂₂(O•/OH) (black) and 2,3,5-F₃Y₁₂₂(O•/OH) (grey dotted line). This is not consistent with the 115 mV difference between Y₁₂₂(O•/OH) and 2,3,5-F₂Y₁₂₂ obtained in step (1) above (black and green lines). Below we examine the possibility that the initial RT step has changed from PCET in wt RNR to ET in 2,3,5-F₃Y₁₂₂-β2.

(3) Y(O•/O⁻) is the experimentally observed redox pair in the single turnover (NO₂)Y₁₂₂-β2 system.⁶¹ It appears likely that Y(O•/O⁻) is the active redox couple also in 2,3,5-F₃Y₁₂₂-β2 since the pK_a of 2,3,5-F₃Y (6.4)¹⁶ is lower than the pK_a of NO₂Y (7.1).⁵⁵ In this scenario, oxidation-reduction of native Y₁₂₂ involves ET between Y₃₅₆ and Y₁₂₂, PT between Fe1(H₂O/OH) and Y₁₂₂(O•/OH), and a net charge change at the diiron cluster (Figure 7A). In contrast, oxidation-reduction of 2,3,5-F₃Y₁₂₂-β2 involves ET between Y₃₅₆ and Y₁₂₂ and a net charge change at site 122 (Figure 7B). The 115 mV difference in potential between Y₁₂₂(O•/OH) and 2,3,5F₃Y₁₂₂(O•/O⁻) is partly due to the ring fluorination and partly due to redox-driven electrostatic changes that are not identical between the wt system and 2,3,5-F₂Y₁₂₂-β2.

(4) 2,3-F₂Y has a higher pK_a (7.8) than the other F_nYs (6.4 –7.2) and 2,3-F₂Y₁₂₂ may thus behave as Y₁₂₂(O•/OH) or as 2,3,5-F₃Y₁₂₂(O•/O⁻). If it is the former, we predict a ΔE°′ of 70 mV (ΔE°′(α₃(2,3)F₂Y – α₃Y); Table 1) between Y₁₂₂(O•/OH) and 2,3-F₂Y₁₂₂(O/OH) (928 mV; tan dotted line). If it is the latter, we predict a ΔE°′ of 31 mV (ΔE°′(α₃(2,3)F₂Y – α₃(2,3,5)F₃Y); Table 1) between 2,3,5-F₃Y₁₂₂(O•/O⁻) and 2,3-F₂Y₁₂₂(O•/O⁻) (1004 mV; orange dotted line). These predictions were tested by performing EPR-monitored radical equilibration studies on 2,3-F₂Y₁₂₂•-β2 (Figure S9). The observed populations of 2,3-F₂Y₁₂₂• and Y₃₅₆• correspond to a ΔE°′(2,3-F₂Y₁₂₂• – Y₃₅₆•) of 47 ± 11 mV (pH 7.6, 25 °C). This results in a 27 ± 11 mV difference between 2,3,5-F₃Y₁₂₂ (green line) and 2,3-F₂Y₁₂₂ (1000 mV; orange solid line). Thus, the α₃X ΔE°′ (31 ± 2 mV) scale is preserved between 2,3,5-F₃Y₁₂₂ and 2,3-F₂Y₁₂₂ (27 ± 11 mV). This suggests that the reactions associated with the 2,3,5-F₃Y₁₂₂ and 2,3-F₂Y₁₂₂ redox cycles are similar and that both occur via Y(O•/O⁻). Furthermore, since pK_a(2,3,5-F₃Y) < pK_a(2,3,6-F₃Y) < pK_a(3,5-F₂Y) < pK_a(2,3-F₂Y), we propose that Y₁₂₂(O•/O⁻) is the operational redox couple in all four F_nY-substituted RNRs.

(5) It appears counterintuitive that ΔE°′(2,3-F₂Y₁₂₂(O•/O⁻) – 2,3,5-F₃Y₁₂₂(O•/O⁻)) is well predicted by the α₃X ΔE°′(pH 5.5) value and not by the ΔE°′ of the corresponding α₃X(O•/O⁻) redox pairs (which equals −43 mV; Table S2). The simplest explanation is that F_nY₁₂₂(O•/O⁻) is not a distinct redox couple but an interacting F_nY₁₂₂(O•/OH)/Fe1(H₂O/OH) redox couple connected by a polarized H-bond or H-bonding network. Mössbauer data from the active (α2β2/substrate/effector) wt system suggest that Y₁₂₂ and Fe1 are H-bonded and that the phenol proton toggles back and forth depending on the redox state of Y₁₂₂.⁶⁴ In the F_nY₁₂₂-RNRs the phenol pK_a’s are lower and we hypothesize that the proton is polarized towards Fe1 at all times. This makes the system behave spectrally as a Y–O⁻ (as observed for reduced NO₂Y₁₂₂) but thermodynamically more as a Y(O•/OH) couple due to the H-bonding interactions between the phenol ring and Fe1.

Concluding remarks

Site-specific incorporation of UAAs provides a powerful tool to study and engineer proteins and enzymes.¹³ As the methodologies for UAA incorporation continue to improve, this approach is expanding in multiple directions in both basic science and applied research. e.g.⁶⁶ This report focuses on the use of F_nYs to perturb and characterize ET/PCET reactions in radical- and metallo-enzymes that use Y–O• in catalytic or long-range radical/hole transfer processes. Figure 1 shows F_nYs that have been used to study these types of reactions in enzymes.^{11,17–23,63} Here we contribute data necessary for F_nY-based approaches to study protein redox chemistry. Until now, true thermodynamic potentials of the F_nYs have not been available for any medium, water or protein. Such data could be obtained by incorporating the F_nYs at site 32 in the well-structured α₃Y model protein (Figure 1). The F_nY₃₂–O• species are powerful oxidants. This issue was addressed by developing a protein film SW voltammetry approach that enabled collection of fully reversible voltammograms at uniquely high potentials. This approach generated α₃F_nY voltammograms of excellent quality and reproducibility. E°’s as high as +1200 mV vs. the NHE could be determined with a precision of 2–3 mV. To provide a relevant comparison, the potential of the transient P₆₈₀⁺/P₆₈₀ redox pair in the PSII reaction center is estimated at ~ 1170–1210 mV.⁶⁷ Thus, E°’s representing the very oxidizing edge of the biological redox scale could be reproduced in the 7.5 kDa α₃X model protein.

It has recently been suggested that aberrant reactive intermediates formed at buried metallo-cofactors may be removed via hole transfer along intramolecular Y/W chains linking the active site to the protein surface.⁶⁸ The F_nY₃₂(O•/OH) and F_nY₃₂(O•/O⁻) redox couples span a considerable ΔE°′ range and, in combination with protein engineering,^13,14 could be used to test this hypothesis by altering the potentials of Ys along the proposed hole-transfer chains. These Y analogs could potentially also be used to map the thermodynamic profiles of radical migration processes observed in enzymes such as CcO, cyclooxygenase I and II and various peroxidases. e.g.⁶⁹ If engineered at active sites, the F_nYs could serve to estimate the potentials of metallo-oxidants too hot or too short-lived to be assessed by any other method. Additionally, and as demonstrated here, the α₃X-derived potentials can be used as a mechanistic tool to investigate the PCET vs. ET nature of Y-based redox reactions.