Glucose-Stimulated Insulin Release: Parallel Perifusion Studies of Free and Hydrogel Encapsulated Human Pancreatic Islets

Abstract

To explore the effects immune-isolating encapsulation has on the insulin secretion of pancreatic islets and to improve our ability to quantitatively describe the glucose-stimulated insulin release (GSIR) of pancreatic islets, we conducted dynamic perifusion experiments with isolated human islets. Free (unencapsulated) and hydrogel encapsulated islets were perifused, in parallel, using an automated multi-channel system that allows sample collection with high temporal resolution. Results indicated that free human islets secrete less insulin per unit mass or islet equivalent (IEQ) than murine islets and with a less pronounced first-phase peak. While small microcapsules (d ≈ 700 μm) caused only a slightly delayed and blunted first-phase insulin response compared to unencapsulated islets, larger capsules (d ≈ 1800 μm) completely blunted the first-phase peak and decreased the total amount of insulin released. Experimentally obtained insulin time-profiles were fitted with our complex insulin secretion computational model. This allowed further fine-tuning of the hormone-release parameters of this model, which was implemented in COMSOL Multiphysics to couple hormone secretion and nutrient consumption kinetics with diffusive and convective transport. The results of these GSIR experiments, which were also supported by computational modeling, indicate that larger capsules unavoidably lead to dampening of the first-phase insulin response and to a sustained-release type insulin secretion that can only slowly respond to changes in glucose concentration. Bioartificial pancreas type devices can provide long-term and physiologically desirable solutions only if immunoisolation and biocompatibility considerations are integrated with optimized nutrient diffusion and insulin release characteristics by design.

Introduction

Patients with type 1 diabetes (T1D) lose their ability to produce insulin and require lifelong administration of exogenous insulin. Technological improvements, such as continuous glucose monitoring systems and continuous subcutaneous insulin infusion (Thabit et al., 2016) have provided improvements, but they are still not successful in all patients. Because such therapies cannot fully reproduce the inherently complex function of the endocrine pancreas, chronic and degenerative complications are still unavoidable in long-term T1D. With improved management and control, some complications have declined (Pambianco et al., 2006; Gregg et al., 2014), but others have not, especially in long-term patients (>30 years) (Pambianco et al., 2006). Transplantation of pancreatic islet cells can provide insulin independence with proven safety and efficacy, and it is likely to become an approved clinical treatment in T1D patients complicated by severe hypoglycemia (Ricordi et al., 2004; Fiorina et al., 2008; Hering et al., 2016; Shapiro et al., 2017). Furthermore, stem cell therapies are becoming increasingly likely to provide novel cell sources (Johnson, 2016; Mahla, 2016) that overcome the problem of limited donors, considerably widening the applicability of such approaches. Nevertheless, to prevent allograft rejection, cellular transplantations require the use of systemic immunosuppression, which imposes a considerable burden on the patient and limits widespread applicability. As a possible approach to circumvent immunorecognition and rejection, islet (micro)encapsulation has been extensively explored since the 1980s (Soon-Shiong, 1999; Silva et al., 2006; Teramura et al., 2010; O’Sullivan et al., 2011; Calafiore et al., 2014; Colton, 2014; Scharp et al., 2014; Steele et al., 2014; Desai et al., 2016; Song et al., 2016). The failure of several clinical and preclinical attempts made it clear that these approaches also have challenges and long-term functionality is not easily achieved (King, 2009; Scharp et al., 2014).

To explore how encapsulation affects insulin secretion and to improve our ability to quantitatively describe the glucose-stimulated insulin release (GSIR) of pancreatic islets, here, we performed dynamic perifusion experiments with free (unencapsulated) and immunoisolated human islets using an automated multi-channel apparatus that allows parallel for perifusion and sample collection with high temporal resolution. Such dynamic GSIR perifusion studies (Figure 1) have been introduced in the early 1970s (Lacy et al., 1972). With improved equipment and analytical techniques, they now allow good quantitative characterization of insulin release kinetics under fully controllable experimental conditions of varying external concentrations of glucose and secretagogues of interest (Dionne et al., 1993; Sweet et al., 2002; Bocca et al., 2008; Cabrera et al., 2008; Buchwald et al., 2015; Garcia-Contreras et al., 2017). Perifusion studies are also emerging as a useful assay of islet quality and function for research and clinical applications (Kayton et al., 2015). Here, we investigated the insulin release kinetics of alginate encapsulated islets (small and large capsules) comparing them to those of free islets perifused in parallel. The corresponding experimentally obtained data also allowed us to perform more detailed theoretical investigations using our recently developed complex computational model of insulin secretion (Buchwald, 2011; Buchwald et al., 2013; Buchwald et al., 2015).

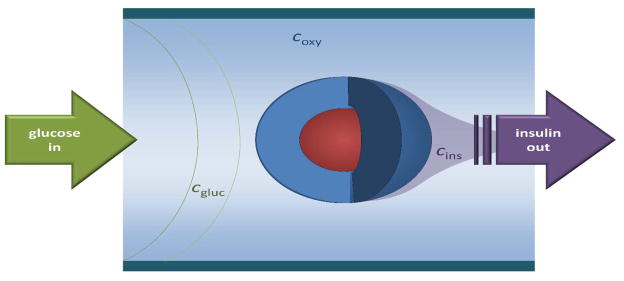
Figure 1. Schematic concept of the dynamic perifusion assay used to assess glucose-stimulated insulin release (GSIR) of free or encapsulated pancreatic islet.

An idealized spherical islet (red) is shown with a hydrogel capsule (dark blue). As indicated, the perifusing solution, which has fully adjustable incoming glucose concentration, flows from left to right, and it is collected at the output to assess its insulin content.

Materials and Methods

Islet isolation and encapsulation

Human islet samples were procured from isolations performed at the Human Islet Cell Processing Facility at the Diabetes Research Institute (University of Miami, Miller School of Medicine, Miami, FL, USA). The islet isolation protocol, as part of the Clinical Pancreatic Islet Transplantation Study, was approved by the institutional review board (IRB) of the University of Miami and the FDA. Human pancreases were isolated from deceased multi-organ donors for whom consent for transplantation was obtained by accredited organ procurement organizations (OPO) from the donor’s families or next of kin. Islets were isolated by using a modification of the automated method (Ricordi et al., 1988) as described previously (Khan et al., 2009; Ricordi et al., 2016). Mouse islets used for some additional perifusion experiments were obtained and processed as described before (Buchwald et al., 2015). All animal studies were reviewed and approved by the University of Miami Institutional Animal Care and Use Committee. All procedures were conducted according to the guidelines of the Committee on Care and Use of Laboratory Animals, Institute of Laboratory Animal Resources (National Research Council, Washington DC). Animals were housed in microisolated cages in Virus Antibody Free rooms with free access to autoclaved food and water at the Department of Veterinary Resources of the University of Miami. Islets were obtained from donor mice (BALB/C or C57BL6/J, Jackson Lab, Bar Harbor, ME) via mechanically enhanced enzymatic digestion followed by density gradient purification using a procedure similar to that previously described (Pileggi et al., 2001; Rengifo et al., 2014). Briefly, animals were sacrificed under general anesthesia, and the pancreas was exposed and injected with Hanks’ balanced salt solution (HBSS; Mediatech, Herndon, VA) containing either 0.8 mg/mL collagenase type V (Sigma-Aldrich, St. Louis, MO) or a mix of 0.2 mg/mL Liberase TL and 0.1 mg/mL DNase (Roche, Indianapolis, IN) via the main bile duct until distension was achieved. Digestion was performed at 37°C for 10–15 min with gentle shaking and terminated by the addition of cold RPMI–10% fetal bovine serum (FBS) with 20×10⁻³ M Hepes buffer, 1% penicillin-streptomycin, and 1% L-glutamine (all from Sigma-Aldrich). Mechanical disruption of the pancreas was achieved by passages through needles of decreasing gauge until release of islets was observed under a microscope; the tissue was filtered through a 450 μm mesh, and islets were purified on Euro-Ficoll (Mediatech) gradients by centrifugation at 400 g for 15–20 min, routinely yielding preparations of 90% purity.

Islet purity was assessed by dithizone (Sigma-Aldrich) staining, and islets were counted and scored using a standard algorithm for the calculation of 150 μm diameter islet equivalent (IEQ) number (Ricordi et al., 1990; Buchwald et al., 2009). Murine islets were cultured in complete CMRL 1066-based medium, which is CMRL 1066 (Mediatech; contains 1 g/L = 5.56 mM glucose) with 10% fetal bovine serum (FBS), 20 mM HEPES buffer, 1% penicillin-streptomycin, and 2 mM L-glutamine added (all from Sigma-Aldrich). Human islets were cultured in CMRL-1066 supplemented medium (Mediatech; contains 5.56 mM glucose, 25 mM HEPES, 1 g/L = 4.6 mM L-alanine–L-glutamine) with 2% human serum albumin (HSA) added, at 37°C in 5% CO₂ humidified incubator for 24 h prior to encapsulation, and perifusions were performed 24–48 h after encapsulations. For encapsulation, standard Ca-alginate microbeads were fabricated using a modification of the protocol originally developed by Lim and Sun (Lim et al., 1980). Briefly, 1.2% (w/v) alginate (UP-MVG, MW 300 kDa, MW/Mn= 1.87, DPn of 28, Batch # FP-504-03, Pronova Novamatrix, FMC) dissolved in HBSS was homogeneously mixed with pelleted islets prior to extrusion through a electrostatic droplet generator (Nisco Engineering, Zurich, Switzerland) into a crosslinking bath of 50 mM calcium chloride, supplemented with 10 mM 3-(N-morpholino)propanesulfonic acid (MOPS), 140 mM D-Mannitol, and 0.025% Tween-20. After crosslinking (10 min), microbeads were washed with HBBS and cultured for 24–48 h prior to study. Resulting beads had average sizes of 700 μm (small capsules) and 1800 μm (large capsules). Small capsules contained an average of 2.9 ± 1.7 islets (corresponding to 4.7 ± 3.9 IEQs), whereas large capsules contained an average of 8.8 ± 2.8 islets (corresponding to 11.4 ± 5.7 IEQs).

Islet perifusion

The perifusion experiments (dynamic GSIR) were performed using a PERI4-02 machine (Biorep Technologies, Miami, FL, USA) that allows parallel perifusion for up to eight independent channels. For each experiment, one hundred free (unencapsulated) or encapsulated islets (all from the same isolation batch) were handpicked and loaded in Perspex microcolumns between two layers of acrylamide-based microbead slurry (Bio-Gel P-4, Bio-Rad Laboratories, Hercules, CA) by the same experienced operator. Perifusing buffer containing 125 mM NaCl, 5.9 mM KCl, 1.28 mM CaCl₂, 1.2 mM MgCl₂, 25 mM HEPES, and 0.1% bovine serum albumin at 37°C with selected glucose (low = 3 mM; high = 11 mM) or KCl (25 mM) concentrations was circulated through the columns at a rate of 100 μL/min. After 45–60 minutes of washing with the low glucose solution for stabilization, islets were stimulated with the following sequence: 5 min of low glucose, 20 min of high glucose, 15 min of low glucose, 10 min of KCl, and 10 min of low glucose. Serial samples (100 μL) were collected every minute from the outflow tubing of the columns in an automatic fraction collector designed for a multi-well plate format. The sample container harboring the islets and the perifusion solutions were kept at 37°C in a built-in temperature controlled chamber. The perifusate in the collecting plate was kept at <4°C to preserve the integrity of the analytes. Insulin concentrations were determined with commercially available ELISA kits (Mercodia Inc., Winston Salem, NC). Values obtained with the human kit are in mU/L; they were converted to μg/L using 1 μg/L = 23 mU/L per the guidelines of the manufacturer. Evaluation of islet mass (IEQ) is challenging and error-prone (Buchwald et al., 2016), and it is especially so for encapsulated islets. Therefore, as before (Buchwald et al., 2015), to account for possible differences in islet mass (IEQ) among islets in different channels, values were rescaled by a normalization factor so as to make the area under the curve (AUC) for the KCl-induced release of insulin in each channel similar to that of the free islets used as a reference. Data used here are averages of at least three samples for each condition.

Computational methods

For computational modeling, our previously developed local concentration-based insulin secretion model has been used. A conceptual schematic of the model is shown in Figure 2; detailed descriptions of its implementation and parameterization have been published (Buchwald, 2011; Buchwald et al., 2015). Briefly, a total of four concentrations are used for (convective and diffusive) mass transport modeling, with their corresponding equations (application modes): glucose, oxygen, and ‘local’ and released insulin (c_gluc, c_oxy, c_insL, c_ins). Diffusion is assumed to be governed by the generic diffusion equation in its nonconservative formulation (incompressible fluid):

$$\frac{\partial c}{\partial t}+\nabla ·(-D\nabla c)=R-\mathit{u}·\nabla c$$ (1)

where c denotes the concentration [mol·m⁻³], D the diffusion coefficient [m²·s⁻¹], R the reaction rate [mol·m⁻³·s⁻¹], u the velocity field [m·s⁻¹], and ∇ the standard del (nabla) operator ( $\nabla \equiv \mathit{i}\frac{\partial }{\partial x}+\mathit{j}\frac{\partial }{\partial y}+\mathit{k}\frac{\partial }{\partial z}$). Diffusion coefficients (D) used are from the original model (Buchwald, 2011); they were selected as consensus estimates of values available from the literature, and are summarized in Table 1. All consumption and release rates are assumed to follow Hill–type dependence (generalized Michaelis-Menten kinetics) on the local concentrations as this provides a convenient and easily parameterizable mathematical function:

$$R=f_H(c)=R_{\mathit{max}}\frac{c^n}{c^n+c_{Hf}^n}$$ (2)

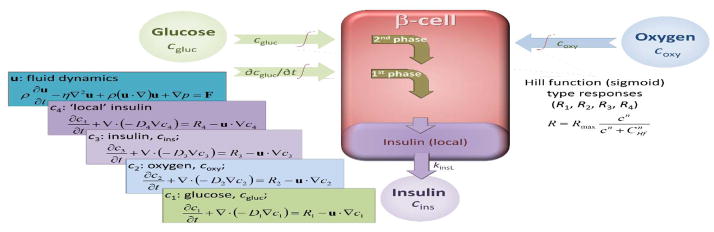
Figure 2. Conceptual representation of the present FEM computational model of insulin release.

Insulin secretion is determined by the local glucose concentration, c_gluc, and its rate of change, ∂c_gluc/∂t, but it is also influenced by the local oxygen concentration, c_oxy. The model uses a total of five coupled modules for glucose, oxygen, and insulin concentrations with incorporated fluid dynamics, as indicated by the corresponding equations on the left side.

Table 1.

Diffusion coefficient used in the present model, D [m²·s⁻¹].

Species\Media	Water	Tissue (islet)	Alginate (capsule)
Oxygen, Doxy	3.0×10−9	2.0×10−9	2.5×10−9
Glucose, Dgluc	0.9×10−9	0.3×10−9	0.6×10−9
Insulin, Dins	0.15×10−9	0.05×10−9	0.1×10−9

In all cases (i.e., insulin, glucose, oxygen), R_max denotes the maximum reaction rate [mol·m⁻³−s⁻¹], C_Hf, the concentration corresponding to half-maximal response [mol·m⁻³], and n, the Hill slope characterizing the shape of the response. The parameter values used are summarized in Table 2; they are generally the same as in the original model (Buchwald, 2011), except for those explicitly discussed in the Results. Total insulin release is obtained as the sum of the first- and second-phase releases (plus an additional modulating function to account for the limiting effect of oxygen availability):

$$R_{\mathit{ins}}=\left(R_{\mathit{ins},ph1}+R_{\mathit{ins},ph2}\right)·{\phi }_{i,o}\left(c_{\mathit{oxy}}\right)$$ (3)

Table 2.

Summary of Hill function parameters used in the present model (Figure 1 and eq. 2; for detailed equations see (Buchwald, 2011)).

Rate	Var.	CHf	n	Rmax	Property and comments
Roxy	coxy	1 μM	1	−0.034 mol/m3/s	Oxygen consumption, basic part. Cut to 0 below critical value, coxy < Ccr,oxy.
Roxy	cgluc	7 mM	2.5	N/A	Oxygen consumption, φo,g metabolic part. Follows increase in metabolic demand; parallels second-phase insulin secretion rate.
Rgluc	cgluc	10 μM	1	−0.028 mol/m3/s	Glucose consumption. Under most conditions, it has no significant influence on insulin secretion.
Rins,ph2	cgluc	7 mM	2.5	H: 1.8×10−5 mol/m3/s M: 3×10−5 mol/m3/s	Insulin secretion rate (second-phase). Total secretion rate is modulated by local oxygen availability (last row). H and M denote values used for human and murine islets, respectively.
Rins,ph1	c′t (∂cgluc/∂t)	0.03 mM/s	2	H: 10×10−5 mol/m3/s M: 21×10−5 mol/m3/s	Insulin secretion rate (first-phase). Modulated to have maximum sensibility around cgluc = 5 mM and be limited at very large or low cgluc. Hill slope of modulating function, σi1,g, changed to 2.5 (previously 4) for a wider range response in phase 1. Release rates kinsL are H: 0.003, M: 0.006 s−1.
Rins, φo,g	coxy	3 μM	3	N/A	Oxygen modulation of insulin secretion rate, φo,g. Limits insulin secretion if coxy becomes critically low.

For human islets, these two R_ins values were now slightly rescaled (decreased, see Table 2) to better fit the experimental data, as well as recent detailed dose-response GSIR experimental data from Henquin and co-workers (Henquin et al., 2015). For a correct time-scale of insulin release, an additional ‘local’ insulin compartment is included in the model (Figure 1) so that insulin is assumed to be first secreted into this compartment and then released following first order kinetics, $\frac{d{c}_{\mathit{insL}}}{dt}=R_{\mathit{ins}}-k_{\mathit{insL}}(c_{\mathit{insL}}-c_{\mathit{ins}})$ (Buchwald, 2011). The original model, which was calibrated for human islets, used a corresponding rate constant of k_insL = 0.003 s⁻¹; for murine islets, this was modified to have a slightly faster release to 0.006 s⁻¹ (Buchwald et al., 2015). Finally, to incorporate media flow, these convection and diffusion models are coupled to a fluid dynamics model. The flowing media is assumed to be an aqueous media at physiological temperature (37°C). Incoming media is assumed to be in equilibrium with atmospheric oxygen and, thus, to have an oxygen concentration of c_oxy,in = 0.200 mol·m⁻³ (mM) corresponding to p_O2 ≈ 140 mmHg. For physiologically relevant conditions, lower values have to be used as tissue oxygen concentrations are likely to be around only c_oxy,tis = 0.060 mol·m⁻³ (mM) (corresponding to p_O2 ≈ 40 mmHg (5%)), as the average oxygen concentrations in arterial and venous blood are ~0.130 and ~0.054 mM (corresponding to ~12% and ~5%, respectively) (Fournier, 2011).

The model is implemented in COMSOL Multiphysics (COMSOL Inc., Burlington, MA) and solved as a time-dependent (transient) problem, allowing intermediate time-steps for the solver, as described previously. Mesh and boundary conditions used are as described before (Buchwald, 2011; Buchwald et al., 2015). Similar to our previous models (Buchwald, 2011; Buchwald et al., 2013; Buchwald et al., 2015), here, we also used two spherical islets of 100 and 150 μm diameter placed in a 2D cross-section of a cylindrical tube with fluid flowing from left to right (see Figure 7 for an illustration of the geometry used). Islet sizes were selected based on our analyses of the size distribution of isolated islets (Buchwald et al., 2009; Buchwald et al., 2016), which found that for isolated human islets, the expected value of diameter is 95 μm while the expected value of volume corresponds to that of an islet with diameter of 133 μm. Islets are considered homogeneous inside; individual cells (e.g., α- or β-cells) are not considered separately.

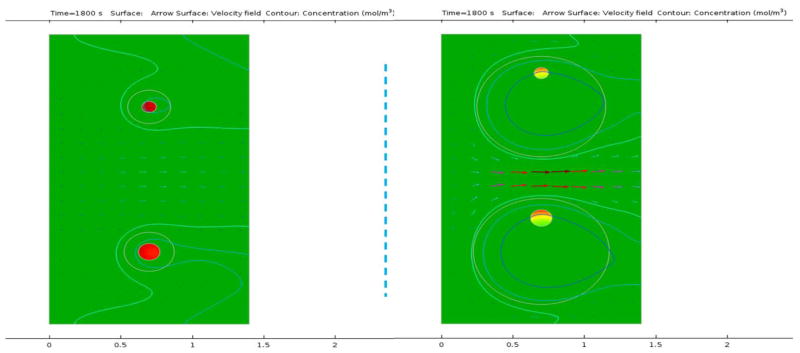
Figure 7. Model-calculated insulin production rates in response to increasing glucose concentrations.

(i.e., during the first-phase response). Calculated insulin production rates for two islets (d = 100 & 150 μm – at the top and bottom, respectively) with small and large capsules (l_caps = 100 and 400 μm; left and right, respectively) under normoxic conditions. Data shown as surface plot are insulin production rates (color-coded from green for low to red for high). Streamlines show the flow of the perifusion fluid (color-coded for velocity; flow from left to right) and colored contour lines show isolevels for the perifusing glucose (from light blue for low to light red for high). Model calculated values are shown during the increase of the glucose concentration from 3 mM to 11 mM; first phase response is noticeably delayed and blunted in the large capsule.

Results

Dynamic perifusions of isolated (avascular) islets were performed using a low (3 mM, G3) → high (11 mM, G11) → low (3 mM, G3) glucose step followed by a KCl step in a fully automated machine that allows parallel perifusion in up to eight channels and frequent sample collection (every minute). As before (Buchwald et al., 2015; Garcia-Contreras et al., 2017), the high glucose step (G11) was maintained for 20 min to allow a clear delineation of the first-phase response.

Human versus murine islets

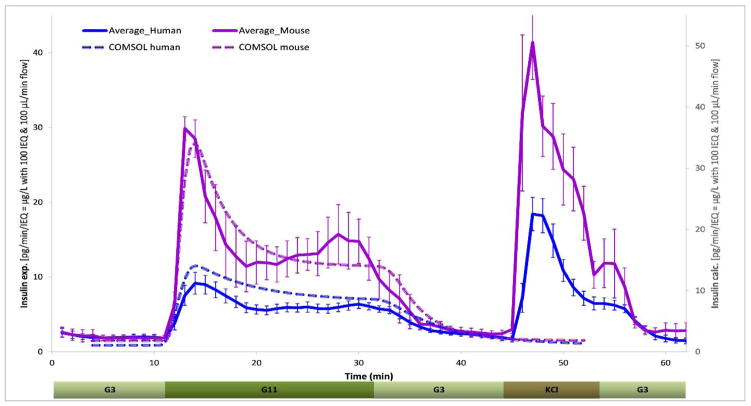
Average insulin responses obtained from perifusion of several islet samples are shown in Figure 3 to compare the insulin secretion of free human and murine islets. Such unencapsulated islets typically show a well-defined first-phase peak, followed by a second-phase plateau as expected from normal functioning islets that are known to release insulin in a biphasic manner in response to a stepwise increase of glucose: a first phase of a relatively quick transient spike is followed by a sustained, but somewhat smaller second phase (Hedeskov, 1980; Henquin, 2000; Rorsman et al., 2000; Henquin, 2009). Our results obtained here with the same perifusion protocol indicate that human islets secrete less insulin per islet equivalent (IEQ) than murine islets and with a less pronounced first phase peak. Comparison of the corresponding average secreted values (Figure 3) indicates an approximate three-fold difference during the peak of the 5–10 min first-phase (24.3 vs. 8.5 pg/IEQ/min) and an approximate two-fold difference during the following second-phase plateau (13.0 vs. 5.8 pg/IEQ/min).

Figure 3. Dynamic GSIR in isolated human and murine islets.

Summary of all experimental data collected for free murine and human islets perifused using a low (3 mM; G3, 5 min) → high (11 mM; G11, 20 min) → low (3 mM; G3, 15 min) incoming glucose stimulation (plus 10 min KCl followed by G3) as shown. Automated PERI4-02 multichannel perifusion apparatus used (samples collected every minute; 0.1 mL/min flow rate, ~100 IEQ per channel). Data are average ± SEM from multiple isolations (single or duplicate channels perifused; n = 12 total samples for both human and murine). Dashed lines are calculated values using the present COMSOL Multiphysics model.

Effect of small and large capsules

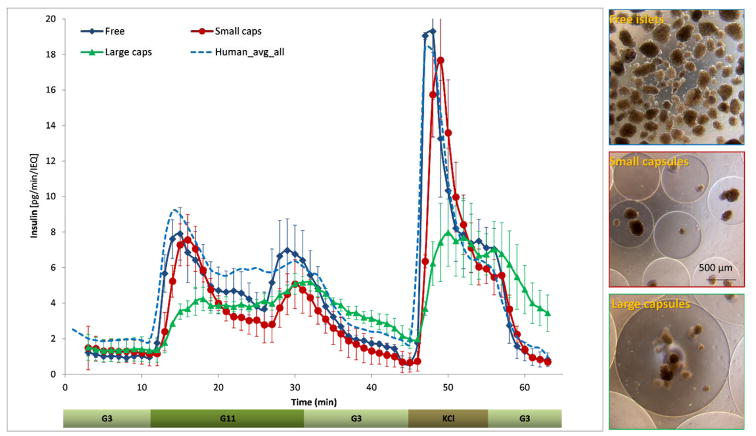
To directly evaluate the effects of hydrogel encapsulation on the insulin release profile of islets, we performed parallel dynamic GSIR perifusion assays with free and alginate encapsulated islets sourced from the same donor (Figure 4). Parallel channels were used for free islets, and the small (d ≈ 700 μm) and large capsules (d ≈ 1800 μm). Whereas the small capsules caused only a slightly delayed and blunted first-phase insulin response compared to free islets, the larger capsules completely blunted this first-phase peak. The same effect could also be seen in the response to the KCl step (Figure 4); clearly indicating a diffusion limitation problem. For the small capsules, the first-phase peak was delayed by about one minute and decreased by ~5% (6.9 vs. 7.2 pg/IEQ/min). With the large capsules, the delay was more than three minutes and the decrease is >40% (4.0 vs. 7.2 pg/IEQ/min). The second-phase plateau decreased somewhat for the small capsules (~30%; 3.6 vs. 5.2 pg/IEQ/min) and a bit less for the large capsules, as the tail of the first-phase peak overlaps due to the diffusional delay (~15%; 4.4 vs. 5.2 pg/IEQ/min).

Figure 4. Glucose-induced insulin secretion in free and encapsulated human islets obtained in parallel perifusion experiments.

Average of experimental data for free, as well as small and large alginate-encapsulated (d = 700 & 1800 μm) human islets perifused using the same protocol as described in Figure 3. Data are average ± SEM from multiple isolations (n = 3 for each condition sourced from the same islet preparation and conducted in parallel). The average of all free human islet perifusions shown in Figure 3 is included for reference (dashed line), and a set of illustrative phase contrast microscope images of free and encapsulated islet samples are shown at right.

Fit with computational model

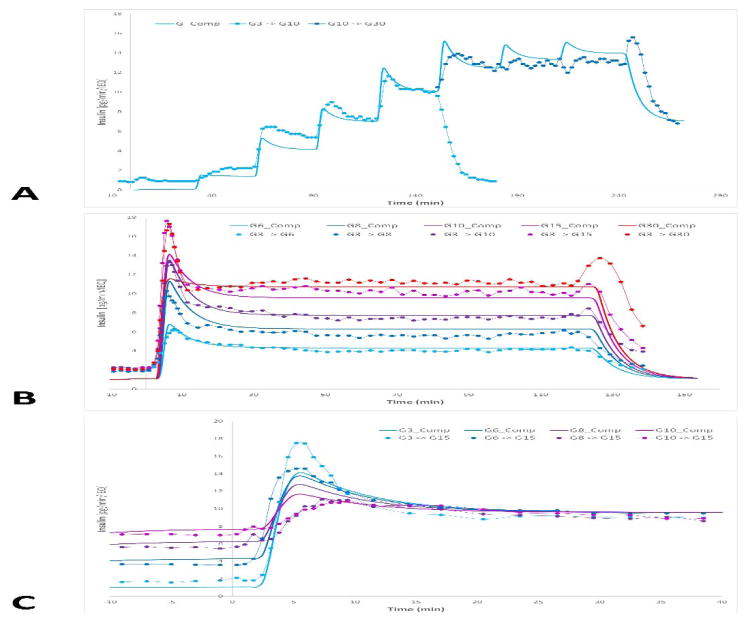
A further goal of the present work was to fine-tune and improve the parametrization of our FEM-based COMSOL Multiphysics model of insulin secretion for avascular islets (Buchwald, 2011) and use it for the computational analysis of these results. The model assumes that the insulin-secreting β-cells act as sensors of both the local glucose concentration, c_gluc, and its change (i.e., its time-gradient), ∂c_gluc/∂t, so that the first-phase response is related to how quickly the (local) glucose concentration changes and the second-phase response is related to the value of the (local) glucose concentration (Figure 2) (Buchwald, 2011). The original model (Buchwald, 2011) was parametrized for human islets and later modified slightly to describe murine islets (Buchwald et al., 2015). To account for the somewhat sharper first-phase response of murine islets, a single parameter was changed (k_insL increased to 0.006 s⁻¹ from the original 0.003 s⁻¹). Since we have now also obtained directly comparable insulin secretion rates in human versus murine islets (Figure 3), and they indicate a lower secretion per unit mass (IEQ) in human islets, the corresponding insulin secretion rates in human islets were recalibrated, as shown in Table 2. The effect of these changes on the predicted insulin secretion can be seen by comparing the calculated profiles for human and murine islets (dashed lines) in Figure 3. To further fine-tune the parameters of the present model, we have also fitted a set of additional GSIR data from three different dose-response experiments, published by Henquin and co-workers (Henquin et al., 2006a; Henquin et al., 2015) (Figure 5). To improve the fit, one additional change was required: the Hill slope of the modulating function for the first-phase response, σ_i1,g, was decreased from 4 to 2.5 to allow a first-phase peak over a wider range of glucose step-up. With these adjustments, the model can fit quite well the overall GSIR profile of human and mouse islets in our standard perifusion experiments (Figure 3), as well as the wider range of different profiles obtained for various incoming glucose step-up challenges (Figure 5). While most first-phase responses are fit well by the model; those for the G3 → G15 and G3 → G30 steps (Figure 5B) are under-predicted, likely because for these large steps, the glucose increase too rapidly making the gradient too large compared to the C_Hf parameter of the first-phase response R_ins,ph1 (0.03 mM/s, Table 2) and the glucose range moves too quickly out of the optimal range of the modulating function σ_i1,g.

Figure 5. Additional dose-response GSIR data used for further calibration of the present computational model.

Dynamic perifusion data from three different dose-response experiments from Henquin and co-workers (Henquin et al., 2006a; Henquin et al., 2015) used for fine-tuning the first- and second-phase insulin response calculated by the present COMSOL Multiphysics model. A variety of incoming glucose step-up challenges were used, as indicated by color-coded symbols: sequential steps of G1 → G3 → G5 → G7 → G10 and then G10 → G15 → G20 → G30 → G7 for the same preparation (A), different single-step challenges of G3 → G6/G8/G10/G15/G30 (B), and different single-step challenges of G3/G6/G8/G10 → G15 (C). Data (symbols) were obtained on a pg/min/IEQ scale here by using the average percent secretion values published with the average insulin content per islet from the same work (17.8 ng/islet; (Henquin et al., 2015)). Continuous thicker lines of corresponding colors are values calculated with the present model.

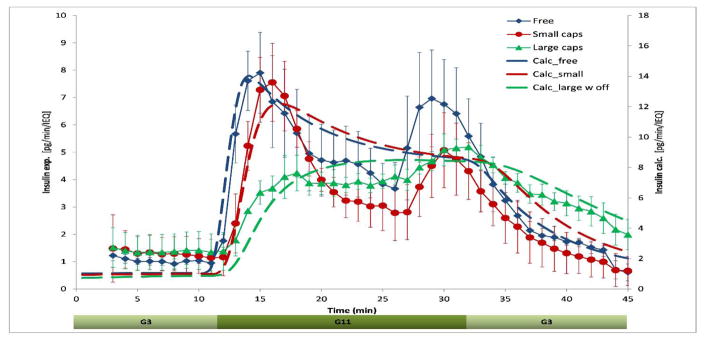
The model was then applied to fit the insulin secretion profile of the hydrogel-encapsulated islets (Figure 6). Previously, we have already shown that because of increasing diffusional distances, the predicted insulin release profiles of encapsulates islets have an increasingly delayed and blunted response as capsule thickness increases (Buchwald, 2011; Buchwald et al., 2015). This is in general agreement with the present experimental results. As before, all calculations shown here were performed with two representative islets with diameters of d = 100 and 150 μm. Predicted responses for the encapsulated islets agree well with the experimental GSIR time-profiles (Figure 6). The fits shown here were obtained with capsule thicknesses of l_caps = 100 μm for the small and l_caps = 400 μm for the large capsules as they gave the best fit (lowest sum of squared errors, SSE) in calculations with a parameter sweep for capsule thickness, l_caps, ranging from 50 to 500 μm in steps of 50. For a ‘standard’ single islet of 150 μm diameter (IEQ), these values correspond to capsules of 350 and 950 μm diameter – somewhat smaller than the alginate beads actually used (d ≈ 700 and 1800 μm). However, in reality, islets are not centrally located in fully symmetric capsules (see illustrative examples in Figure 4), and exchange with the bead surface can take place along shorter diffusional pathways, resulting in a smaller apparent diffusional distance. This accounts for the smaller apparent capsule size that provides the best fit in the computational model. In fact, for the large capsule, the fit could be further improved by placing the islets off-center, and the results shown here were obtained with islets placed 300 μm off-center.

Figure 6. Fit of experimental GSIR data (free and encapsulated islets) with the present computational model.

Experimental data are reproduced from Figure 4, calculated values were obtained as described in the text and converted to pg/min/IEQ for ease in comparison.

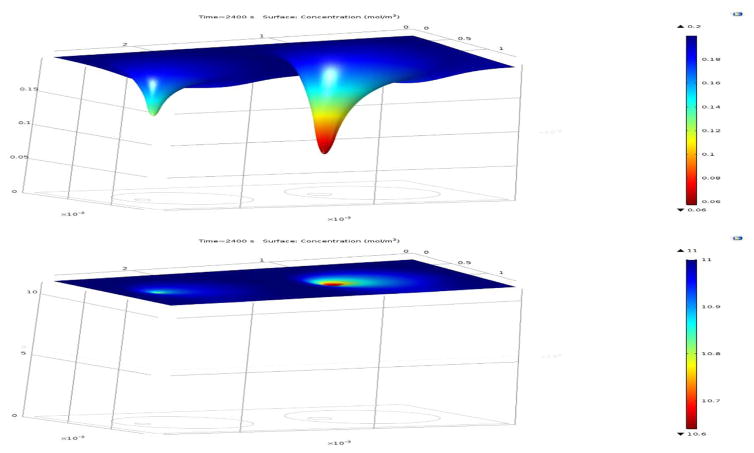
The computational model allowed for detailed calculations of the spatial distribution (concentrations) of all species of interest and their changes, depending on the input conditions. For example, Figure 7 shows calculated insulin production rates for small and large alginate capsules during an increase in the incoming glucose concentration (i.e., during the first-phase response). Figure 8 presents model-calculated oxygen and glucose concentrations during high glucose (G11) exposure under normoxic conditions (atmospheric oxygen). These data are included to show that, in avascular islets, diffusion limitations are more severe for oxygen than for glucose. Thus, oxygen availability is the main issue limiting the functionality and viability of such islets (see Discussion for further details).

Figure 8. Diffusion limitation of oxygen versus glucose in avascular islets.

Model-calculated oxygen (top) and glucose (bottom) concentrations during high glucose (G11) exposure in two encapsulated islets with d = 100 & 150 μm, l_caps = 400 μm (with offset) under normoxic conditions. Data shown as surface plots that are also color-coded from blue for high to red for low to illustrate that oxygen diffusion is the main limiting factor as it decreases more severely inside the islets than glucose. Data are shown at 11 mM glucose exposure under normoxic conditions (atmospheric oxygen; ~20% ≈ 0.2 mM). Oxygen limitations are even more severe for transplanted islets exposed to tissue oxygen concentrations only (~5% ≈ 0.06 mM).

Discussion

Human versus murine islets

According to our dynamic GSIR perifusion experiments, human islets, on average, secrete approximately two- to three-fold less insulin per unit mass (IEQ) than murine islets and with a less pronounced first phase peak (Figure 3). This is even more remarkable when accounting for the fact that the high glucose level used in this study (11 mM) likely provides a stronger stimulus for human than mouse islets, as human islets initiate insulin secretion at lower glucose levels than mouse islets and are likely to have a lower C_Hf,ins (Henquin et al., 2006a; Henquin et al., 2006b; Henquin et al., 2015). Note, however, that there is considerable variability among samples, especially with human islets, and 3–5-fold differences are not uncommon (Kayton et al., 2015). Nevertheless, this observation agrees with previous studies that suggested that murine islets contain more β-cells than human islets. For example, mouse islets were found to contain a higher proportion of insulin-containing cells (77% versus 55%, p < 0.05) and a lower proportion of glucagon-containing cells (18% versus 38%, p < 0.05) than human islets (Cabrera et al., 2006). Similar proportions (averages of ~85% vs. ~60%) were also found in a study that indicated declines in β-cell content in larger human islets (Kilimnik et al., 2012). Lower insulin release from human islets as compared with murine ones has been observed by others as well. For example, Dai and co-workers measured a lower first-phase peak for human versus murine islets, ~ 30 vs. 80 pg/min/IEQ, respectively, when stimulated with 16.7 mM glucose (G16.7) (Dai et al., 2012); however, they also reported a higher insulin output in human islets than in mouse exposed to basal 5.6 mM glucose (G5.6). Here, at 3 mM basal glucose (G3), a clear difference between the baseline insulin secretion of human and mouse islets was not observed, but the baseline secretion varied considerably among preparations. A sharper first-phase peak and a more elevated second-phase plateau in murine islets was also observed by Henquin and co-workers in work that also found the average insulin content of mouse islets to be considerably higher than that of human islets; e.g., (Henquin et al., 2006b) vs. (Henquin et al., 2006a; Henquin et al., 2015).

Effect of increasing capsule size

Results here reaffirm that increasing capsule size imparts an increasingly delayed and blunted first-phase response. Notably, the same effect was present not only during glucose stimulation, but also during KCl depolarization (Figure 3), indicating common diffusion limitations in both the inflow of the secretagogue and the outflow of the secreted insulin. Such delayed and blunted responses due to the impact of the encapsulating hydrogel have been found by others as well (Lim et al., 1980; Chicheportiche et al., 1988; Jesser et al., 1996; de Vos et al., 2003). Consequently, larger capsules unavoidably lead to the dampening of the insulin response and to sustained-release type insulin secretion that can only slowly respond to changes in glucose concentrations. While the delay and blunting may not always be physiologically significant, it still should be an important consideration in the design of improved bioartificial pancreas devices (Korsgren, 2017), particularly because (1) an accelerated loss of the first-phase insulin response has been found in those progressing toward T1D in several studies (Chase et al., 2001; Sosenko et al., 2013; Koskinen et al., 2016; Veijola et al., 2016) and (2) there is evidence that even if a first phase may not exist under physiological conditions following oral administration, the ability of β-cells to generate a rapidly increasing insulin profile is still relevant and particularly effective in restraining hepatic glucose production (Caumo et al., 2004). Hence, lack of an adequate first-phase response might have long-term physiological consequences.

It should also be noted that the perifusion experiments reported herein were performed at atmospheric oxygen (~20% O₂; p_O2 ≈ 150 mmHg; c_oxy = 0.22 mM). Similar experiments are sometimes performed with oxygen-enriched perifusion media (e.g., 95% O₂) to minimize the effects of oxygen limitation (e.g., (Jesser et al., 1996; Garfinkel et al., 1998; de Vos et al., 2003; Henquin et al., 2006a; Barrientos et al., 2009)). More importantly, at lower oxygen concentrations, such as those mimicking the tissue oxygen concentrations that transplanted islets typically encounter (e.g., p_O2 = 35–45 mmHg; c_oxy = 0.05–0.065 mM), the loss in insulin secreting ability is likely to be even more significant due to the more severe hypoxia especially in the core regions of larger islets (Buchwald, 2011). For example, the present model predicts that (i) under low oxygen conditions free islets still secrete insulin at 70–75% of their normal rate, but encapsulated islets decline to 50%; and (ii) the larger the glucose concentration, the more hampered the response (Buchwald, 2011). Various approaches have been explored to overcome this nutrient diffusion problem (Colton, 2014; Scharp et al., 2014), such as the use of smaller (Halle et al., 1994; Hall et al., 2010; Gattás-Asfura et al., 2013; Tomei et al., 2014) or more oxygen-permeable capsules (Chin et al., 2008; Gattás-Asfura et al., 2012), the use of smaller islets/pseudoislets (O’Sullivan et al., 2010; Hilderink et al., 2015), the co-encapsulation of anti-inflammatory drugs (to limit fibrotic overgrowth) (Dang et al., 2013), the use of islet cells that are more hypoxia-resistant (Wright et al., 1998) or are engineered to contain an intracellular oxygen carrier such as myoglobin (Tilakaratne et al., 2002), preimplantation (Sörenby et al., 2008), and the stimulation of blood vessel growth around the implanted devices (Josephs et al., 1999; Lembert et al., 2005). Others focused on local oxygen delivery near the islets, for example, by in situ oxygen generation via an electrochemical process (Wu et al., 1999), local photosynthesis (Bloch et al., 2006), hydrolytically activated oxygen-generating biomaterials (Pedraza et al., 2012; Coronel et al., 2017), or by daily delivery into a dedicated gas chamber integrated into the transplanted device (Ludwig et al., 2012; Ludwig et al., 2013). Macrodevices intended to encapsulate larger tissue amounts within a single immune-isolated volume, such as TheraCyte (Geller et al., 1997; Loudovaris et al., 1999; O’Sullivan et al., 2011) or its Encaptra modification are facing the same oxygen diffusion limitation problem, and, most likely, in an even more challenging form.

Fibrotic overgrowth can further exacerbate the problem of diffusion limitation for nutrient (mostly oxygen) availability, and the efficacy of implanted biomedical devices in general is often compromised by host recognition and subsequent foreign body responses. Depending on the nature of biomaterial as well as that of the graft (allo- vs. xeno-), overgrowth may be limited to a relatively small percent of microencapsulated islets; nevertheless, central necrosis, as well as macrophage-released factors, have still been found to limit long-term graft function (de Vos et al., 1999; de Vos et al., 2004). A more recent investigation suggested that the biocompatibility of (alginate) encapsulated islets is geometry dependent, and can be significantly improved by use of larger capsules, as larger spheres (i.e., d = 1.5 mm) generated less foreign body reaction and fibrosis than smaller spheres (Veiseh et al., 2015). A notable caveat of this approach, however, is the significant increase in diffusional length it imparts. Hence, biocompatibility and diffusion limitation aspects might have to be balanced against each other.

Computational model

Quantitative models of islet function are not only of general interest to characterize the glucose–insulin regulatory system, but are of direct relevance for the design of optimal biohybrid devices. Our general, FEM-based model used here is considerably more complex than any other computational model explored to date for encapsulated islets (Pillarella et al., 1990; Reach et al., 1990; Todisco et al., 1995; Tziampazis et al., 1995; Buladi et al., 1996; Dulong et al., 2002; Dulong et al., 2005) as those do not allow the coupling of both convective and diffusive transport with reactive rates for arbitrary geometries. Most of these models allow transport by diffusion only with only a select number that incorporate convective transport (i.e., fluid dynamics to model flow) (Pillarella et al., 1990; Buladi et al., 1996), and even these are restricted by cylindrical symmetry assumptions. Contrary to these, the present model can be used for arbitrary geometries and flowing fluids to calculate insulin output in response to arbitrary incoming glucose profiles (Buchwald, 2011). It can predict both first- and second-phase insulin responses, and it also incorporates an oxygen-dependence for insulin release (Figure 2). This is important because in avascular islets, the hypoxia resulting from oxygen diffusion limitations is a major limiting factor (Ohta et al., 1990; Dionne et al., 1993; Papas et al., 1999; Sweet et al., 2002; Wang et al., 2005; Buchwald, 2009). Figure 8 highlights this by illustrating the calculated oxygen and glucose concentrations during high glucose (G11) exposure. The drop in oxygen is more significant than the drop in glucose, and most of the decline occurs inside of the islet. Hence, oxygen availability is the main factor limiting viability and functionality, and it is particularly severe in the core of larger islets and capsules.

In general, our calculations are in strong overall agreement, even on a quantitative level, with various experimental observations indicating that when isolated islets are maintained under normoxic conditions, larger islets show central necrosis and this becomes much more severe under hypoxic conditions (Vasir et al., 1998; Giuliani et al., 2005; MacGregor et al., 2006; Komatsu et al., 2016). This is a main reason why islet cultures require very low culture densities to ensure viability in the core of the larger islets: Current standard practice is 100–200 IEQ/cm² corresponding to a surface utilization of only 2–3% (Sander et al., 1996; Murdoch et al., 2004; Papas et al., 2005; Ichii et al., 2007). For the same reason, insulin production rates are more severely limited inside larger islets, as well as in larger capsules (Skiles et al., 2011). This is well illustrated by the color-coding shown in Figure 7. It has to be noted that these predictions should be treated with caution as data available to calibrate the oxygen limitation of insulin secretion was limited. The present model was built using the only data published so far (Dionne et al., 1993). Lacking corresponding experimental data, the effects of different diffusion coefficients within the hydrogel (e.g., due to different alginate concentrations or to incorporation of modifiers) were not modeled; however, exploratory calculations indicate that the impact should be relatively modest.

With the present recalibrations, the fit of the experimental data was not just qualitative, but also quantitative, meaning that, in addition to the overall time-profiles being similar, the calculated insulin amounts were also in good agreement with the measured ones. Note that because islets are spheroid-like cell aggregates of different sizes (<50 to 500 μm) resulting in possible thousand-fold differences between the mass contribution of individual particles, evaluation of islet mass (IEQ) is challenging and error-prone (Buchwald et al., 2016), and it is especially so for encapsulated islets. Therefore, IEQ estimates are uncertain and can vary from lab to lab and from person to person. We and others have previously shown that, for the manual count of islet mass (IEQ), coefficients of variability (CV) can be as high as 30% (Buchwald et al., 2009; Kissler et al., 2010; Friberg et al., 2011; Buchwald et al., 2016). Hence, even with accurate insulin assays, up to two-fold differences in the present insulin release rate per IEQ estimates are feasible depending on laboratory practices and inter-technician variabilities. Nevertheless, the model can provide quantitative estimates, and these can serve as first estimates for future bioengineering or tissue engineering approaches.

Conclusions

In conclusion, dynamic GSIR perifusion experiments can provide a robust quantitative characterization of the insulin release kinetics of encapsulated islets. Here, we found that (i) human islets secrete less insulin per IEQ than murine islets and with a less pronounced first phase peak, (ii) small microcapsules cause only a slightly delayed and blunted first-phase insulin response compared to free islets, (iii) larger capsules completely blunt the first-phase peak and decrease the amount of insulin output in response to a glucose challenge, and (iv) our complex computational insulin secretion model gives adequate qualitative and quantitative description of the insulin secretion profile of free and hydrogel-encapsulated islets. Bioartificial pancreas type devices can provide physiologically desirable insulin release and long-term clinical solutions only if immunoisolation and biocompatibility considerations are also integrated by design with optimized nutrient diffusion and insulin release characteristics.

Abbreviations

FEM

finite element method

GSIR

glucose-stimulated insulin release

IEQ

islet equivalent

SSE

sum of squared errors

T1D

type 1 diabetes mellitus