The numerical abilities of anurans and their neural correlates: insights from neuroethological studies of acoustic communication

Abstract

Acoustic communication is important in the reproductive behaviour of anurans. The acoustic repertoire of most species consists of several call types, but some anurans gradually increase the complexity of their calls during aggressive interactions between males and when approached by females. In these interactions, males may closely match the number of calls or notes in a sequence that a neighbour produces, thereby revealing their numerical abilities. Anurans are also able to discern the number of sequential properly timed pulses (notes). The temporal intervals between successive pulses provide information about species identity and call type. A neural correlate of this numerical ability is evident in the responses of ‘interval-counting’ neurons, which show ‘tuning’ for intermediate to fast pulse rates and respond only after at least a threshold number of pulses have occurred with the correct timing. A single interpulse interval that is two to three times the optimal value can reset this interval-counting process. Whole-cell recordings from midbrain neurons, in vivo, have revealed that complex interplay between activity-dependent excitation and inhibition contributes to this counting process. Single pulses primarily elicit inhibition. As additional pulses are presented with optimal intervals, cells become progressively depolarized and spike after a threshold number of intervals have occurred.

This article is part of a discussion meeting issue ‘The origins of numerical abilities’.

1. Introduction

The general consensus of this Royal Society meeting is that numerical abilities are widespread across taxa, and anurans are no exception. Although not studied explicitly, the numerical abilities of anurans (frogs and toads) are evident in their acoustic communication, which is important in their reproductive biology. Typically, males produce ‘advertisement’ calls to attract females, which choose from many competing males [1,2]. This competition can be particularly intense in ‘prolonged’ breeding species [2], in which matings occur over many nights. Males in many of these species increase the complexity of their calls to match or exceed that of their calling neighbours. It is in the context of this behavioural plasticity that we see evidence of the numerical abilities of anurans.

I first review the behavioural evidence that anurans are able to evaluate the number of calls or sound pulses in calls that they hear. I then show that neurons in the auditory midbrain selectively respond only if a threshold number of sound pulses have occurred with the ‘correct’ timing; these ‘interval-counting’ neurons (ICNs) [3,4] represent a neural correlate of some of the behavioural counting abilities of anurans. Finally, I briefly summarize our current understanding of the mechanisms that underlie these numerical computations.

2. Behaviour

Plasticity of calling behaviour in anurans takes several forms. Males of prolonged breeders establish and defend a calling site from which they broadcast advertisement-type calls [1,2]. In response to the calls of an intruding male, most commonly, the resident produces one or more ‘encounter’ calls, which signal aggressive intent. If the intruding male retreats or does not continue to approach the resident, the latter will resume advertisement calling [5]. In other anurans, however, males compete by adding additional acoustic elements to their advertisement calls. For example, male tungara frogs, Physalaemus pustulosis, produce advertisement calls in which the frequency sweeps downward, i.e. frequency modulated (FM) calls (figure 1). To compete with calling neighbours, males add ‘chucks’ (brief, harmonically rich notes) to the end of their FM sweeps [6,7]. Competing frogs match or exceed (n + 1) the number of chucks produced by their neighbours, and females prefer more complex calls, i.e. calls with the greatest number of chucks [6,7]. To match or ‘one-up’ their competitors, male tungara frogs can add as many as four to six chucks to their advertisement calls, and thereby show evidence of counting to at least this number. Because males produce these ‘chuck’ notes during a single expiration/inspiration cycle, the limit of four to six chucks probably reflects a respiratory constraint.

Figure 1.

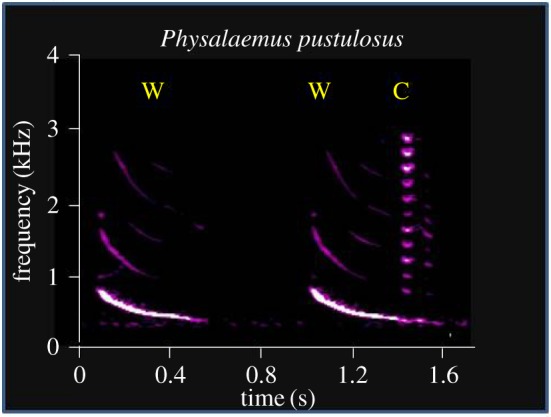
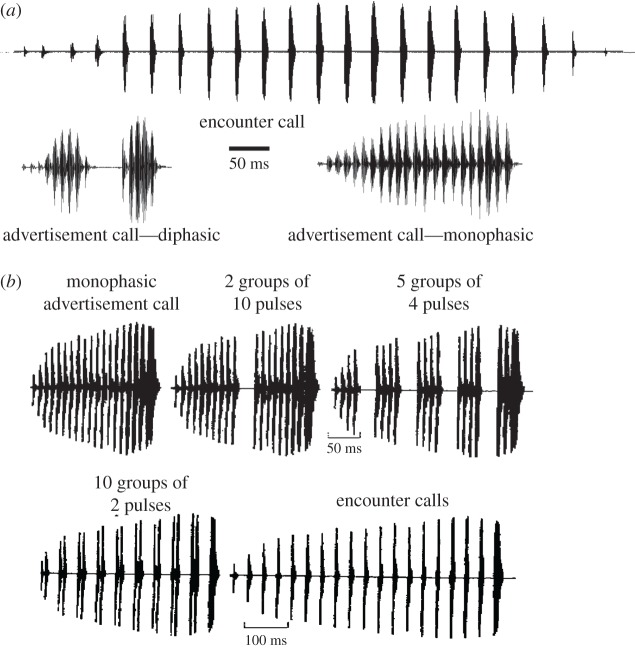
Sound spectrogram (sonogram) of advertisement calls of Physalaemus pustulosis. Males produce a frequency-modulated call, referred to as a ‘whine’ (W), and add ‘chucks’ (C) to the whine in response to the calls of other males.

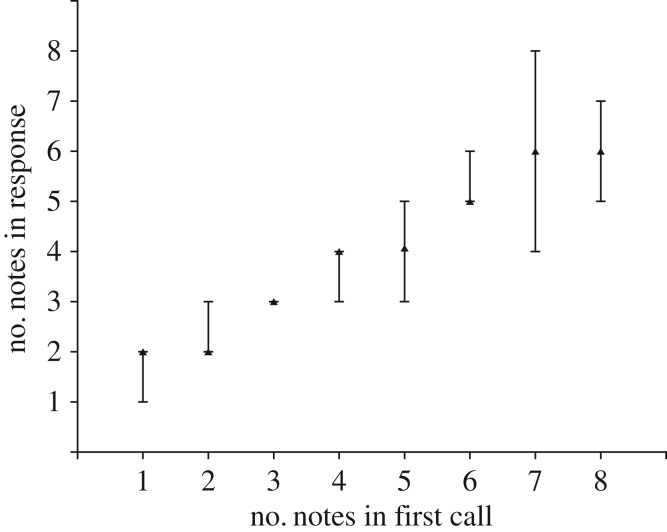
In at least several species, however, male–male acoustic competition involves increasing the number of short calls, sometimes referred to as ‘notes’, in a response sequence; for this review, the terms ‘notes in a call’ and ‘calls in a sequence’ can be considered to be equivalent in these cases. Each call/note results from a discrete respiratory expiration, followed by inspiration during the non-vocalizing period (several hundred milliseconds) between successive calls; thus, respiratory constraints on the number of calls/notes in a sequence are relaxed. In these cases, evidence from natural interactions and playback studies indicate that males attempt to match or exceed the number of calls that a neighbour produces; males appear able to count to at least seven or eight (sequential calls). Arak [8] first documented this matching process in the Srilankan frog, Philautus leucorhinus. He found that males closely matched the number of calls in a sequence to those of a neighbour, and that matching occurred even when the distance between the two frogs varied. It appeared, therefore, that males were not simply integrating and matching the increase in sound energy during these escalations of call number; their call matching reflected an ability to assess the number of calls. Matching or exceeding the number of calls of a neighbour has been hypothesized to function as a graded threat and/or better attract females. Schwartz [9] observed similar call-matching behaviour during interactions between neighbouring males of Dendropsophus microcephalus (formerly Hyla microcephala). In the interactions summarized in figure 2, males produce two-note calls in response to the one-note calls of their neighbours, i.e. add a note to their calls. The neighbours then add a note to their calls, and this matching process and escalation of call complexity continues until plateauing with the neighbours producing seven to eight notes per call motif and the former males producing six-note calls; the accuracy of this matching process remains intact for note sequences up to this value. These data suggest that yellow treefrogs are able to count up to approximately six or seven call notes, but do not experimentally rule out the possibility that males simply increase the number of notes to match the cumulative energy of the calls of their neighbour. Studies of Australian quacking frogs, Crinia georgiana, however, more strongly support the hypothesis that anurans possess true numerical abilities [10]. Calls were broadcast from either of two speakers and responses of a male were recorded. In the experiment shown in figure 3, the focal male accurately matched the number of notes in the playbacks; importantly, this matching accuracy remained intact even when the calls were decreased in amplitude (−6 dB) and broadcast from a different speaker. Together, these studies strongly suggest that at least some species of anurans are able to evaluate the number of notes in the call motifs of a neighbour.

Figure 2.

Call-matching behaviour of male yellow treefrogs, Dendropsophus microcephalus (Hyla microcephala), engaged in natural interactions. Males add notes to their calls to match or exceed the number of notes in the calls of a neighbour. Adapted from Schwartz [9].

Figure 3.

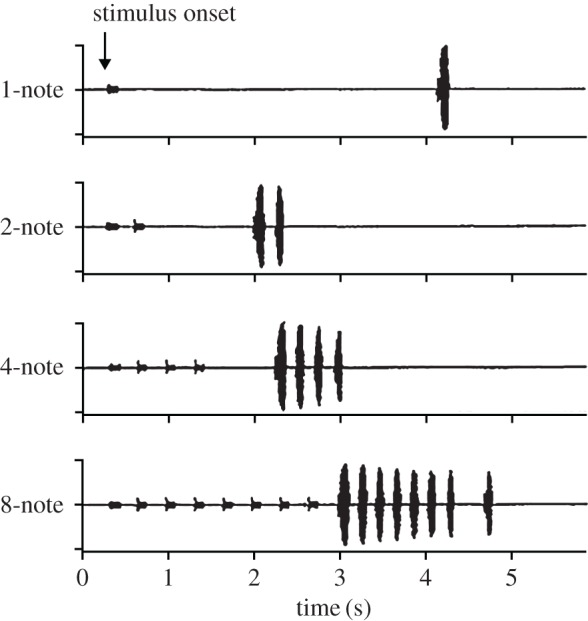
Oscillograms of calls produced by a male quacking frog, Crinia georgiana, in response to playbacks of call stimuli in which the number of calls in sequences varied from 1 to 8. From Gerhardt et al. [10].

Evidence of counting, albeit on a shorter time scale, is evident in anuran species that produce distinct advertisement and encounter (aggressive) calls that differ in their temporal structure. For example, advertisement and encounter calls of Pacific treefrogs, Pseudacris regilla (formerly Hyla regilla), consist of a series of sound pulses that are repeated at rates of approximately 90 pulses s⁻¹ and 25 pulses s⁻¹ (ca 20°C), respectively; that is, intervals between onsets of successive pulses are approximately 11 and 40 ms (figure 4a). Interestingly, males perceived as encounter calls synthetic signals (figure 4b) in which the intervals alternate between these two values [11]; because an equal number of advertisement and encounter call-typical intervals were present, we expected these signals to be ambiguous. Males treated the signals as advertisement calls if the number of consecutive advertisement-like intervals in each pulse group was increased to three, i.e. four pulses per group, or more. That is, males required at least three consecutive advertisement-typical intervals in these repeating patterns of pulses in order to perceptually categorize signals as advertisement-call-like. Similarly, in grey treefrogs, Hyla versicolor, the attractiveness of synthetic calls to females increases nonlinearly as the number of consecutive advertisement-call typical intervals is increased from one to approximately four to six [12]. According to Weber's law, we would expect strongest discrimination between stimuli that had no advertisement-call intervals versus those in which intervals alternated between short (advertisement) and long intervals. Together, these behavioural data suggest that anurans are able to count the number of consecutive pulses that occur with the correct (advertisement-call-typical) timings. I next present an overview of our current understanding of the neural underpinnings of these numerical abilities.

Figure 4.

Oscillograms of advertisement and encounter calls of male Pacific treefrogs, Pseudacris regilla (Hyla regilla) (a), and stimuli used in playback experiments (b), adapted from Rose & Brenowitz [11].

3. Neural correlates of interval counting

Behavioural studies, using a cross-accommodation paradigm, indicated that central filters exist in Pacific treefrogs for discretely processing fast versus slow pulse rates. We, therefore, searched for neurons that showed tuning to fast or intermediate pulse rates, with the objectives of identifying their locations and the computational mechanisms that underlie their temporal selectivity. Previous extracellular, single-unit recordings had revealed neurons in the midbrain (anuran inferior colliculus, IC) that showed band-pass tuning to amplitude-modulation (AM) rate [13–15]; auditory-nerve fibres, in contrast, code the rate of sinusoidal amplitude modulation in the periodicity of their discharges, but do not show AM selectivity. In the anuran IC, however, the firing rate of most neurons is strongly dependent on AM rate [16,17]. Remarkably, neurons that are most sharply tuned to AM rate poorly code the modulation rate in the periodicity of their discharges (quantified by calculating a synchronization coefficient) [17,18]. We, therefore, searched in the anuran IC for neurons that showed selectivity for fast pulse rates, i.e. interval tuning, and interval counting.

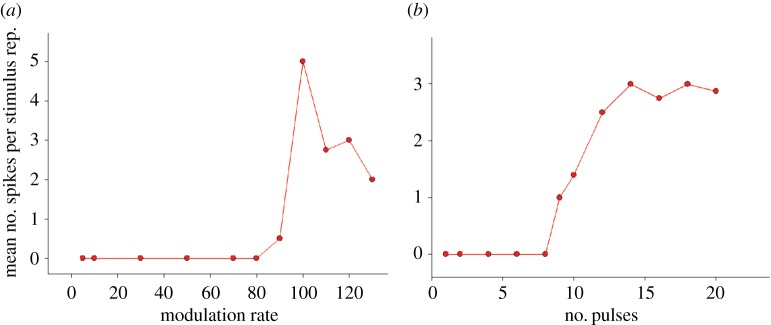
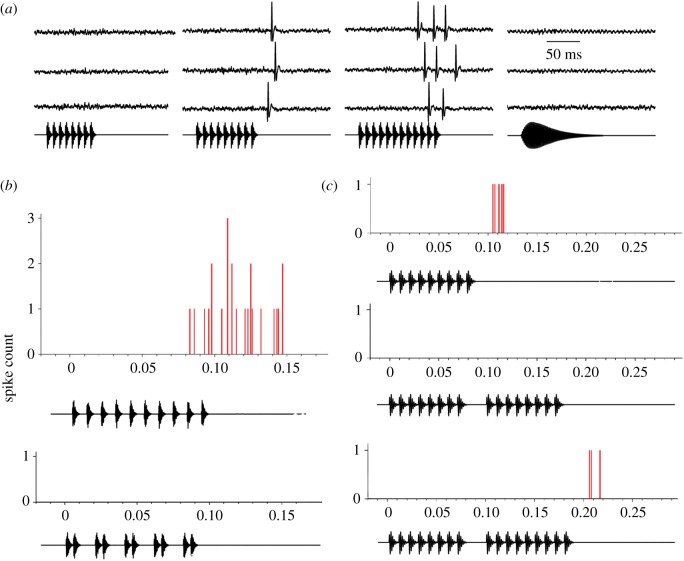
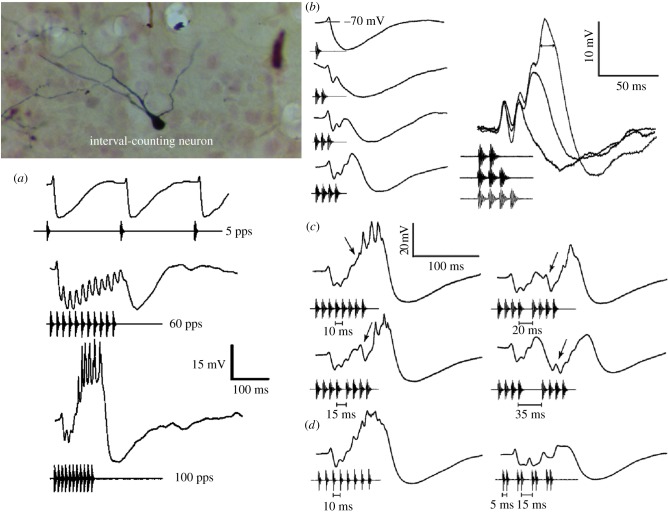
As predicted from behavioural studies, we found neurons in the anuran IC that are selective for fast AM or pulse rates (figure 5). These cells also show selectivity for fast pulse rates when pulse duration, shape and number are held constant, but do not respond to such pulses when presented at slow rates; that is, they show selectivity for pulse rate per se. Most relevant to the topic of numerical processing, these cells respond only after a threshold number of pulses have occurred [3,4,19]. For example, the cell shown in figures 5 and 6 responded to stimuli that had nine or more pulses. This dependence of response on pulse number was not simply related to the increased stimulus energy; a single pulse that had greater energy than the nine-pulse stimulus was ineffective (figure 6a). Further, no spikes were elicited even after a 6 dB increase in the amplitude of a stimulus that had one or two fewer pulses than threshold.

Figure 5.

Response levels of an interval-counting neuron in the anuran inferior colliculus (torus semicircularis) versus rate of amplitude modulation (a), or number of pulses in a stimulus with a pulse rate of 100 pulses s⁻¹ (b). Carrier frequency was 600 Hz.

Figure 6.

(a) Oscillograms of extracellularly recorded responses (spikes) of the cell shown in figure 5 to the stimuli shown below each set of traces. Stimulus pulse rate was 100 pulses s⁻¹, and the numbers of pulses (left to right) were 8, 9, 12; the single-pulse stimulus (right) had the same energy as the 12-pulse stimulus, but did not elicit spikes from this neuron. (b) Histograms of spike responses of the same neuron to stimuli that consisted of 10 pulses with 10 ms (optimal) interpulse onset intervals (top) or that alternated between intervals of 5 and 15 ms (lower). (c) Histograms of spike responses to pulse sequences in which the duration of the middle interpulse-onset interval was either 10 ms or 30 ms; after the gap, nine pulses again were required to elicit spikes (lower histogram).

The responses of these neurons appear to reflect a counting process, but what information is being integrated? These cells might respond after a threshold number of pulses have occurred within a particular integration time window. Alternatively, the salient information might be the number of consecutive pulses that have occurred with the proper timing. To distinguish between these two hypotheses, we tested neurons with stimuli in which pulses were repeated with fixed interpulse intervals versus alternated between intervals that were shorter or longer than the presumed optimal interval (figure 6b). As shown in this figure, the salient temporal feature for eliciting responses was the number of consecutive ‘correct’ intervals, not the mean pulse rate. Remarkably, a single interval of 30 ms reset the interval-counting process (figure 6c); an interval that was two to three times the optimal value rendered calls less attractive in behavioural tests [20,21]. Because a single long interval (time between onsets of successive pulses) just two to three times the optimal value can completely reset the interval-counting process, it could not result purely from classical temporal summation.

4. Mechanisms of interval counting

Extracellular recordings were sufficient for demonstrating that interval-counting neurons (ICNs) are present in the anuran IC, but provided limited understanding of the underlying mechanisms. To further investigate the mechanistic basis of interval counting, we used ‘patch-type’ pipettes to make ‘whole-cell’ recordings from anuran IC neurons, in vivo [22,23]. In these recordings, a high-resistance seal is made between the glass pipette tip and cell membrane. Subsequently, the membrane patch within the pipette is electrophoretically ruptured to gain access to the interior of the cell and measure the trans-membrane potential [23]. In vivo, whole-cell recording has revolutionized our ability to investigate how neurons in the anuran auditory system integrate excitation and inhibition to make computations [24–29].

Whole-cell recordings from an ICN in the anuran IC are shown in figure 7 [29]. This neuron responded best for pulse rates of 100–120 pulses s⁻¹, and did not spike for pulse rates ≤ 60 pulses s⁻¹. As with pulses presented at 5 pulses s⁻¹ (figure 7a), a single pulse elicited a small depolarization followed by a larger and more sustained hyperpolarization (figure 7b); the hyperpolarization was an inhibitory post-synaptic potential because its amplitude decreased when negative current was injected to tonically hyperpolarize the cell (figure 7b, right). Additional pulses, delivered at 10 ms interpulse onset intervals, elicited excitatory post-synaptic potentials (EPSPs) that increased in amplitude and duration; that is, with successive pulses, the synaptic balance shifted towards the excitation. For stimuli with four pulses, excitation overcame inhibition and depolarized the cell approximately 7 mV relative to resting potential; five pulses were required to elicit spikes. To investigate how a single long interval resets the counting process, we progressively increased the gap between the fourth and fifth pulses in a sequence of eight pulses (figure 7c). As this middle interpulse interval was increased, the EPSP to the fifth pulse decreased and the hyperpolarization increased in amplitude. The apparent decrease in excitation and reinstatement of the inhibition following a long interval appeared to contribute to the ineffectiveness of stimuli in which intervals were alternately shorter or longer than optimal (figure 7d).

Figure 7.

Averaged whole-cell recordings of responses from an interval-counting neuron (inset) in the anuran inferior colliculus of Lithobates (Rana) pipiens to stimuli that differed in pulse rate (a), pulse number (b), the duration of the middle interval in a sequence of eight pulses (c), or sequences of 5 ms pulses in which intervals were constant at 10 ms or alternated between 5 and 15 ms (d). In (a), responses to only the first three pulses in the 5 pulses s⁻¹ stimulus are shown. Averaged responses to stimuli in which pulse number was increased from one to four pulses while the cell was hyperpolarized by approximately 12 mV, relative to rest (−70 mV), are shown on the right in (a). Carrier frequency was 800 Hz.

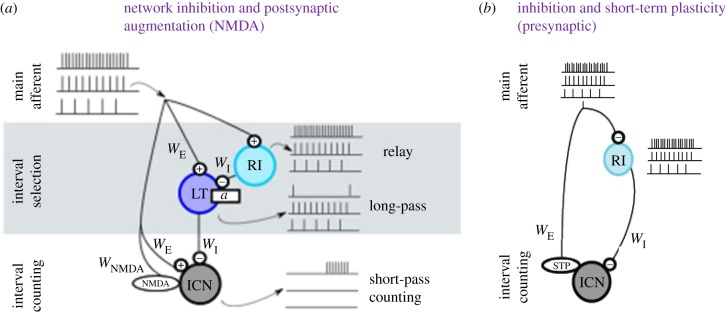
Our current computational model of interval counting and pulse-rate selectivity [30] is presented in figure 8a. Selectivity for interval duration and number appears to result from rate- and time-dependent interplay between excitation and inhibition. In this model, which best fits the experimental data, ICNs receive excitatory inputs via AMPA-type and NMDA-type glutamate receptors and inhibition via GABA_A-type receptors. Initial pulses, delivered at or near the optimal rate, elicit strong inhibition and counteract excitation. However, with additional pulses, cells (LT) that provide inhibition to the ICN are themselves inhibited (feed-forward inhibitory input from RI), thereby temporarily releasing the ICN from inhibition. This ‘disinhibition’ permits excitatory inputs to depolarize the cell, with voltage-dependent augmentation occurring via the progressive recruitment of NMDA-type conductances; depolarization removes Mg²⁺ block of these channels. At the end of the pulse sequence, LT is released from inhibition and provides strong ‘OFF-type’ inhibition to the ICN. This reinstatement of inhibition and reversal of excitatory augmentation is hypothesized to reset the interval-counting process. Alternatively, interval counting may result from integrating non-depressing inhibition and excitation that shows rate-dependent augmentation, which can be reset during a long interval (figure 8b). Simulations indicate that this model requires unrealistic properties of excitation plasticity and, therefore, appears less likely.

Figure 8.

Schematics of neural circuits for short-pass interval selectivity and interval counting. (a) Counting on dis-inhibition circuit motif. From the main afferent, a first layer performs interval selectivity: a relay inhibitory neuron (RI) provides disynaptic inhibition with a weight (W_I) to a low-threshold, long-interval selective cell (LT) with subthreshold adaptation strength a. In the second layer, the interval-counting neuron (ICN) combines afferent excitation (weighting, W_E) with the interval-selective inhibition from LT. ICN receives inhibition at every pulse for long intervals but is dis-inhibited for short intervals. (b) Network diagram for a model based on short-term plasticity. Both excitation and inhibition are triggered at every pulse. The excitatory synapse is dynamic and undergoes substantial short-term facilitation. Adapted from Naud et al. [30].

5. Conclusion and future directions

There is substantial evidence that anurans can evaluate the number of sound pulses that have a particular timing in a sequence. Species that show graded complexity of call structure during aggressive/competitive interactions with conspecifics are able to match the number of notes in a call, or calls in a motif produced by a neighbour. These counting processes take place on time scales of tens of milliseconds in the former cases to seconds in the latter cases. Behavioural studies indicate that anurans are able to count to at least 7 or 8; however, the limits of their counting abilities have not been rigorously tested. Further, nothing is known about how long counting information can persist in memory; in natural interactions, males quickly respond to the calls of a neighbour. The time course of memories of call/note count could possibly be investigated from playback experiments, wherein a calling male interacts with signals that are under experimental control. Interval discrimination and counting in anurans is analogous to recognizing particular temporal arrangements of notes in a musical score by humans.

Neural correlates of the numerical abilities of anurans are seen in the ICNs. These cells respond only after a threshold number of pulses have occurred with the correct timing. Their responses do not simply reflect the number of pulses that occurred in a particular time window; rather, their firing indicates that a threshold number of consecutive intervals have occurred. This property underlies the sharp interval selectivity of these neurons, and appears to function primarily for enabling these animals to discriminate between calls that differ in pulse rate; counting may be a by-product of this temporal selectivity mechanism. These cells are a subset of the neurons in the anuran IC that show selectivity for temporal features of sounds. This paper is focused on the interval-counting cells; however other cells have been found that show selectivity for sound duration [24,28,31], also a numerical property. A primary question for future research is whether this interval-counting process can be rescaled to intervals of several hundred milliseconds. If so, then we might expect to see neurons that respond after several calls, particularly in the quacking frogs and yellow treefrogs. ICNs that respond best for pulse rates of 15–20 pulses s⁻¹ have been recorded from grey treefrogs, which repeat pulses at approximately 20 pulses s⁻¹ (20°C) in their advertisement call [26]. This interval-counting process can be rescaled, therefore, to operate for intervals of at least 50–75 ms. Counting calls, which have longer time intervals, may, however, require temporal integration processes such as those that have been proposed to underlie perceptual decision-making over seconds [32,33]; excitatory feedback to integrators within a network could sustain the progression of depolarization over this time frame. An exciting future direction will be to make recordings from anurans that show evidence of being able to count calls that are repeated at intervals of several hundred milliseconds.

An understanding of the computational mechanisms that underlie interval counting is beginning to emerge from whole-cell recordings in vivo. Experiments using this technique have provided unprecedented views of the subthreshold fluctuations of membrane potential that occur in response to temporal features of sounds, and therefore, how excitation and inhibition are integrated. This work has shown that inhibition dominates if pulses are repeated at slow rates; however, the balance shifts towards excitation as pulses are repeated with correct intervals. Our current model posits that this shift in synaptic balance results from rate-dependent augmentation of excitation and attenuation of inhibition (disinhibition), with inhibition rebounding at the end of a pulse train. Current and future experiments that generate excitatory and inhibitory conductance estimates from recordings under negative current-clamp conditions [24,34], along with pharmacological manipulations of excitatory and inhibitory inputs to these neurons [35], should permit a thorough understanding of the mechanisms that underlie interval counting.

Data accessibility

This article has no additional data.

Competing interests

I declare I have no competing interests.

Funding

This work was supported by grants from NIDCD to G.J.R.