Cognitive access to numbers: the philosophical significance of empirical findings about basic number abilities

Abstract

How can we acquire a grasp of cardinal numbers, even the first very small positive cardinal numbers, given that they are abstract mathematical entities? That problem of cognitive access is the main focus of this paper. All the major rival views about the nature and existence of cardinal numbers face difficulties; and the view most consonant with our normal thought and talk about numbers, the view that cardinal numbers are sizes of sets, runs into the cognitive access problem. The source of the problem is the plausible assumption that cognitive access to something requires causal contact with it. It is argued that this assumption is in fact wrong, and that in this and similar cases, we should accept that a certain recognize-and-distinguish capacity is sufficient for cognitive access. We can then go on to solve the cognitive access problem, and thereby support the set-size view of cardinal numbers, by paying attention to empirical findings about basic number abilities. To this end, some selected studies of infants, pre-school children and a trained chimpanzee are briefly discussed.

This article is part of a discussion meeting issue ‘The origins of numerical abilities'.

1. Introduction

From tiny acorns, mighty oaks do grow. Every professional mathematician was once an infant yet to grasp even small cardinal numbers. How is that initial step even possible, given that cardinal numbers are abstract? That is the main philosophical problem about numbers to be dealt with here, the problem of cognitive access.

In this paper, ‘numbers’ refers exclusively to finite cardinal numbers. These are typically answers to questions starting ‘How many’, followed by a description applicable to individuals, rather than to units of a non-discrete quantity. So, the number of letters in the modern Greek alphabet is a cardinal number, whereas the number of miles between the central stations of Liverpool and Manchester is not a cardinal number but a ratio (of the inter-city distance to the unit distance of a mile).

The paper is organized as follows. The first part is a rough and rapid tour of major rival views of number proposed by mathematicians and philosophers. None of these views escapes objection, and we seem to reach an impasse. To unblock the way, a philosophical error needs to be exposed. That is the second part. The third part presents a case for the claim that, by paying attention to findings of cognitive science about basic number abilities, we can solve the problem of cognitive access to numbers and justifiably settle on one of the views of number as correct.

2. Five major views

(a). The classical view

At the start of Book VII of Euclid's Elements, a number (arithmos) is defined thus:

where a unit is a single individual thing (Book VII, definitions 1 and 2) [1]. On this view, any pair of items is a 2 and so there are many 2s; any trio is a 3 and so there are many 3s. In general, any plurality of k things is a k and there are many ks. We retain a corresponding use of the word ‘number’, as when we say that a number of authors were late with their submissions. While a unit is not a plurality, the number theory of books VII to IX of the Elements includes theorems about units (e.g. VII. 15 is the special case for units of VII. 9). So in effect Euclid had 1s as well as plural numbers, but there was no notion of zero.

The classical view of cardinal numbers has staying power. Its best modern variant, put forward by the mathematical logician John Mayberry, takes arithmetic and number theory to be general truths about sets (including one-membered sets and the empty set), interpreting numerical equations in terms of 1–1 correlations as is done in standard set theory [2]. For example, ‘3 + 2 = 5’ is taken to mean that there is a 1–1 correlation between the union of any triple with any pair not overlapping the triple and any quintet. In fact, the whole of cardinal number theory, including theorems about zero, can be interpreted along these lines in set theory. An attractive feature of the classical view is that the problem of cognitive access seems to melt away: we can have access to some numbers by perception (those three eggs) or by description (Jupiter's moons).

But there is one major disadvantage. The classical approach allows that there are many 1s, many 2s and in general many ks, whereas modern number theory assumes that there is just one number for each numeral. This can be seen from the use of number-counting functions, the definitions of which make no sense unless it is assumed that there is just one number per numeral. A prominent example is Euler's φ-function, to be found in any modern elementary text on number theory [3]: φ(1) = 1; φ(n) = the number of positive integers less than and co-prime to n, for n > 1. Number theory can be applied to many systems and one of those is the system of finite cardinals. So here is a serious problem for the classical view.

(b). Numeralism

‘Numeralism’ here denotes the view that cardinal numbers are the numerals of a numeral system. Numeralism was held by the philosopher Berkeley [4]; closely related views about the natural numbers were proposed by the mathematician Hilbert [5] and the logician Kripke (S. Kripke 1992, unpublished data). Why think that numbers are numerals? Berkeley [4] noted that large numbers within the range of performable calculations defy precise sensory representation; so when we think of 201, what introspective awareness reveals is not an image of 201 items but an image of the numeral. To conclude without further argument that the number itself is the numeral ‘201’ is to confuse the representation with what is represented—a quite common mistake. Berkeley did have further arguments, but they are unsound.

Numeralism has the advantage that it appears to escape the problem of cognitive access: people are satisfied with the fact that we can see written numerals. But a serious disadvantage is that a common core of basic arithmetical information can be expressed using different numeral systems or natural language number words:

• 
• 

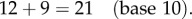
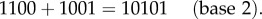
• Twelve plus nine equals twenty-one.

Another disadvantage in the same vein is that many truths about finite cardinals are independent of numeral systems. An example is the truth that every finite cardinal number greater than one is a prime or a product of primes. This is due to the fact that there is no infinite decreasing sequence of finite cardinal numbers [6].

(c). Mentalism

‘Mentalism’ here denotes the view that a cardinal number is a mental representation or a mental (or intellectual) construction. The mathematician and founder of mathematical intuitionism Luitzen Brouwer was the chief proponent of a mentalist view in recent times [7]. The mathematician Dedekind [8] also gave voice to mentalism when he wrote of numbers as free creations of the mind, as did Cantor [9] when he wrote that the cardinal number of a set has existence in our mind as an intellectual image of the set. Mental and intellectual entities are more puzzling to us than physical entities but less so than abstract entities. At least, we might have cognitive access to mental or intellectual entities by inner awareness and reflection.

The big problem for any version of mentalism is that only finitely many brain states have actually been (or could be) realized; hence, there are only finitely many mental representations or intellectual constructions. So the idea that numbers are mental entities conflicts with the fact that for any finite number, there is a yet greater number. Here is one way in which we can know this fact: any cardinal number n is the number of preceding numbers, as we start with zero; so the number of numbers up to and including n is greater than n by one.

(d). The set-size view

This is the view that cardinal numbers are sizes of sets. Set size is a discrete magnitude; in other respects, it is much like length, duration and weight (which we tend to think of as dense and continuous magnitudes). The set-size view takes our pre-theoretical thought and talk literally: ‘class size’ in normal parlance refers to the number of pupils in a class, and ‘family size’ refers to the number of family members.

This view has advantages over each of the previous views. It coheres with mathematical practice in taking each numeral to denote a single cardinal number (unlike the classical view); it is consistent with the fact that there are infinitely many finite cardinal numbers (unlike mentalism); and it allows for facts about numbers which are independent of any and all numeral systems.

However, the set-size view of cardinal numbers runs into the cognitive access problem. I will put this in the form of an argument.

If numbers were set sizes, they would lack space–time location; they could not undergo any change; they could neither emit nor reflect signals; they could leave no traces; they could not affect the behaviour of other things.

So they could have no causal effect on us, even remotely.

So we could have no cognitive access to them.

This turns out to be a persuasive argument. This or some very similar argument has contributed to the emergence over the last 4 decades of a deeply sceptical view about arithmetical truth [10]. This is the next and final view in this ultra brief survey.

(e). Fictionalism

There are no numbers; arithmetic is not literally true, but it is useful to think and act as though it is. This is fictionalism, a view that has been adopted or taken very seriously by some recent philosophers [10]. It is mainly a response to the difficulties faced by the other views, in particular the cognitive access problem. That problem obviously disappears if there are no numbers.

But fictionalism has a serious credibility problem. Opting for one philosophical view over others may be fine if one is denying nothing but a bunch of other philosophical views; but it is not fine if one is denying not only rival philosophical views but also non-philosophical propositions that are generally regarded by rational thinkers as among the most certain things that we know. No philosophical doctrine has greater rational credibility than basic arithmetic.

3. A philosophical error

All five major views of cardinal numbers face serious objections. But the set-size view can be defended. The only objection to it is the argument that if numbers were set sizes, we could have no cognitive access to them, and that argument is unsound. The problem lies with the final inference: set sizes can have no causal effect on us; therefore, we cannot have cognitive access to them. The aim of this short section is to show that this step is invalid.

What underlies this step is a model of cognitive access to something as the outcome of a causal chain which starts with an event involving that thing and ends with an event of sensory perception. This model may be appropriate for cognitive access to physical objects; but it is not appropriate for more abstract kinds of things. Some properties, for example, are cognitively accessible via perception of their instances. Examples are sensory forms. A melody is an aural form; its instances are performances of it. An alphabetic letter type (upper case) is a visual form; its instances are its actual inscriptions. For cognitive access to a melody, it is enough that one can recognize performances of it and distinguish them from performances of other melodies. For cognitive access to a letter type, it is enough that one can recognize inscriptions of it and tell them apart from inscriptions of other letters.

Such a recognize-and-distinguish ability requires one to have an enduring representation of the sensory form. Recognizing something as an instance of the form requires an interaction between (i) a representation produced by current perceptual input and (ii) an enduring representation of the form. We may think of the interaction loosely as a comparison process which, in the case of recognition, has a positive outcome. Distinguishing between instances and non-instances involves the ability, when presented with a non-instance of the form, to perceive that it is not an instance. For this, a necessary condition will be that the ‘comparison’ process between the representation produced by current perceptual input and the enduring representation of the form has a negative outcome.

How does one get an enduring representation of a sensory form? One can get an enduring representation of a melody by attentively hearing performances of it many times; one can get an enduring representation of a letter type by attentively seeing inscriptions of it many times. The subject's attention need not be self-directed. Infants acquire enduring representations of some sensory forms this way, for example, enduring representations of phonemes from hearing verbal output of parents [11]. In this case, the infant can recognize an instance of a phoneme and distinguish it from sounds which are not instances of the phoneme, without being aware of doing so.

These considerations allow us to answer the following question. A sensory form itself can have no causal effect on us; yet we can acquire cognitive access to it. How is this possible? The answer: we have cognitive access to a sensory form if we can recognize instances of the form and distinguish them from non-instances; that ability may be acquired by getting an enduring mental representation of the form, and that can result from repeated attentive perception of instances of the form. So the argument against the set-size view is unsound, as it depends on an inference which rests on the false assumption that we can have cognitive access to only those things which can have a causal effect on us.

4. Cognitive access to numbers

An unsound argument may have a true conclusion; so it remains to be shown that if cardinal numbers are set sizes, cognitive access to some of them is possible. My aim is to show that empirical findings about basic cognitive abilities provide good evidence that some children and some non-humans actually have cognitive access to small set sizes. Only a few illustrative empirical studies are mentioned, due to the space limit.

Although set sizes are not sensory forms, we can use a recognize-and-distinguish ability as a sufficient (but not necessary) condition for cognitive access to small set sizes. A small set is perceptible when it is non-empty and all its members are individually perceptible and together perceptible as a single collection. The chimes of a clock striking three or a pair of dolls are examples. Restricted to perceptible sets, the following is a sufficient condition for cognitive access to a set-size n:

One can recognise non-empty sets as n-membered (when they are) and distinguish them from non-empty sets with fewer or more than n members.

A natural first suggestion is that this kind of access is achievable by means of our well-documented capacity for set-size discrimination, sometimes called ‘the number sense’ [12]. This has features of other magnitude senses: it is rough and fails when, in comparing sets of different sizes (S the smaller, L the larger), the ratio S/(L–S) increases beyond some threshold (which depends on age and training). In number comparison, tasks performance takes longer and is more error-prone with (i) increase in S (the ‘magnitude effect’) and (ii) decrease in L–S (the ‘distance effect’). Empirical studies of infant capacity for set-size discrimination when S > 3 illustrate this table 1.

Table 1.

Infant discrimination capacity [13,14].

age	S/(L–S)	pass/fail	example
6 months	1	pass	8 versus 16
	2	fail	8 versus 12
9 months	2	pass	8 versus 12
	4	fail	8 versus 10

A similar capacity for rough number discrimination has been found even in species which are phylogenetically quite distant from humans, for example, frogs (Bombina orientalis) and angelfish [15,16]. But we cannot rely on this capacity for number discrimination alone, precisely because it is approximate (though the system enabling approximate number discrimination together with links to representations of external number symbols may provide for cognitive grasp of specific numbers) [17]. Typically, however, the approximate system will not enable us to distinguish n-membered sets from sets which are close in size to n. This is the distance effect.

However, for very small numbers, infants have exact discrimination. Again this is not limited to humans. Newborn chicks have a remarkably similar capacity [18]. Here, are some of the findings for human infants. They can distinguish between sets of size n and sets of size n + 1 for n = 1, 2. Starkey & Cooper [19] found this capacity in infants with a mean age of 22 weeks; Antell & Keating [20] found it in infants with the mean age of 53 h. Strauss & Curtis [21] found that some infants approaching 1 year could also distinguish between three-membered and four-membered sets; so for each of one, two and three, they could distinguish between those set sizes and neighbouring set sizes. As discrimination generally becomes easier when the difference increases, one might well expect that for n = 1, two infants can also discriminate between n-membered sets and sets with more than n + 1 members. But there is evidence against this. Feigenson & Carey [22] found that while infants discriminate between one object versus two objects and between one versus three, they failed to discriminate between one versus four. If this is typical, infants do not fully satisfy the proposed condition for cognitive access to cardinal numbers, even for one. It is notable, however, that chicks can discriminate between one versus four (also one versus five and two versus four) [23].

(a). Do infants respond to number?

Did the infants in these experiments respond to the number of members of displayed sets, rather than to some other visually detectable property not controlled for? The infants could have been responding to the total area of the individual items, a not implausible hypothesis if the displays present what look like chocolate buttons. Another suggestion is that they respond to the convex hull (roughly: the area within a rubber band when stretched to go round all the individual items without moving them). There are corresponding aurally detectable features if presented sets are sequences of sounds: the sum of the durations of the sounds, or the duration of the interval from the start of the first sound to the end of the last sound. Why should we conclude that the infants are responding to anything as abstract as number?

While it may be impossible to control for every conceivable alternative hypothesis to explain the infant data, the range of alternatives may be narrowed down so much that the hypothesis that the infants are responding to number stands out as the most plausible. Certain violation-of-expectation experiments involving auditory–visual matching go a long way towards achieving this. In two studies, Koyabashi et al. [24,25] adapted the landmark experiments of Wynn [26]. In the second study, infants saw one or more toy animals like Mickey Mouse dropped from above falling onto a surface, hearing a computer-generated sound at the moment of impact, in the familiarization stage. In each test trial, infants heard either two or three of those sounds, while the falling toy animals were hidden behind an opaque screen. The screen was then removed to reveal either two or three toy animals. The infants looked significantly longer at the toy animals when the number of toy animals and the number of sound bursts were unequal than when they were equal. This study controlled for potential confounds with rate of sound bursts and total duration of the sound sequence. The earlier study, of a similar design, controlled for a combined familiarity and complexity preference postulated by Cohen & Marks [27]. So there is good evidence that infants do at least sometimes respond to number.

(b). Do infants recognize numbers?

Even if infants do respond to numbers, they may not satisfy the sufficient condition for access to a number n that we are focusing on now, namely, the ability to recognize sets as n-membered (when they are) and distinguish them from sets with fewer or more than n members. One problem has already been mentioned: for numbers n = 1, 2, infants distinguish between n and its neighbours, but may fail to distinguish between n and numbers beyond its neighbours. Another problem relates to the ability to recognize numbers. The problem is that relevant infant studies demonstrate mere matching, not recognizing. The difference can be illustrated as follows. Case 1: shown some photos of unfamiliar faces in succession, you notice that the face in the current photo looks very like a face in an earlier photo. That is mere matching. Case 2: shown a photo of a face of someone you know but have not seen (pictured or in life) for a long time, you quickly recall whose face it is. That is recognizing. The relevant infant studies are habituation studies and violation-of-expectation studies. The subjects in the test trials compare something currently perceived with information retained in fairly short-term memory from recent perception. For recognition, there has to be a comparison of input from current perception with an enduring representation. The relevant infant studies do not provide evidence for this.

(c). Pre-schoolers

To summarize, there is good evidence that infants are responsive to the number of items in very small sets and have number-discrimination ability within a very limited range; but we lack evidence that they have the recognize-and-distinguish ability that we are looking for (though they may in fact have it). For evidence of that, studies with pre-schoolers and chimpanzees are more promising, as training and experience make possible the formation of enduring number representations.

The results of two studies of pre-schoolers given several numerical tasks suggest that number words are mapped onto long-term representations of set sizes one to three and sometimes also four. Benoit et al. [28] found that pre-school children of 3–5 years old can name the number in a display of one, two or three items. Sets of dots were presented under two conditions: (i) simultaneous display for 800 ms, and (ii) sequentially, one dot at a time for 800 ms each. Comparing performance under these conditions, the authors concluded that the children would subitize rather than count to get the answers (where ‘counting’ refers to the explicit assignment of number words to objects or events by the child). In a second more extensive study [29], Le Corre & Carey confirmed that children of 3–5 years could quite accurately estimate the size of sets of one, two, three or four circles on a card presented too briefly for counting; but four was found to be the limit for this task, even for those whose competence in the give-a-number task went way beyond four. In these tasks, the children had to name the number of items displayed. This does not involve comparison of one currently or recently perceived set with another; rather, it requires a comparison of input from a current or recent perception with an enduring representation of a set size. So, we may reasonably conclude that the most accurate children could recognize the numbers one, two, three and four. Assuming that these children, unlike infants, can distinguish these numbers not only from each other but also from larger numbers—I know of no evidence to the contrary—they satisfy the recognize-and-distinguish test for cognitive access to the small numbers.

A couple of warnings about the enduring representations of small set sizes involved in these tasks are needed. First, these representations probably do not belong to the system underlying our capacity for quick but rough number discrimination, known as the approximate number system. Secondly, it is possible that these small number representations do not have set-size representation as their prime function. Le Corre & Carey [29,30] argue that these representations are provided by a resource they call ‘the enriched parallel individuation system’.

(d). Number naming by a chimpanzee

Among the non-humans who have been trained to associate visual or aural symbols with numbers of visible things, one of the best known is the female chimpanzee, Ai. At 5 years, Ai was trained to name the number of items in a display (for example, three red pencils) by pressing one of six keys marked with an arabic numeral from 1 to 6. At the same time, she was trained to associate particular visual symbols with types of object (e.g. pencil, bowl, spoon) and colours of objects (e.g. red, blue, yellow). At the start of training, only two objects were displayed and only two numeral keys were available; the number of objects and corresponding numeral keys were increased successively. Training was continued on each number set until accuracy reached over 90% in two consecutive sessions, ending with displays of five objects (varying over object type and colour). Ai achieved over 98% accuracy during the final two sessions of naming numbers from one to five [31].

At 9 years, Ai was trained to name the number of one to seven dots rather than ordinary objects, by pressing keys with arabic numerals. Ai's number naming was tested in four experiments [32]. In the first, semi-random patterns of one to six dots were displayed; then the range was increased to seven dots and specific patterns were mixed in with the semi-random patterns; then the size of dots was changed between and within sessions; finally, red or green objects (blocks, pencils or padlocks) were used in place of dots. Ai's accuracy in the final sessions was again very high. The processes underlying Ai's performance were investigated by obtaining response times (RTs). The RT function was flat for displays of up to three items, then increased but fell for the final number (in each test range). The author of the study took the RT data to suggest that for numbers up to three, Ai was probably subitizing and for numbers beyond three estimating rather using non-verbal counting; and the estimation probably involved a comparison of representations from an analogue magnitude system [32].

Ai was later trained to name the number in computer displays of one to nine items. At 20 years, she was trained with the aim of incorporating ‘0’ into her stock of numerals as a symbol for absence of items [33]. She was then tested using two cardinality tasks and a number ordering task. The cardinality tasks were: (i) presented with two numerals and a square containing zero to nine dots, she had to choose the numeral naming the number of dots; (ii) presented with two squares containing different numbers of dots (from zero to nine) and a single numeral, she had to choose the square containing the number of dots given by the numeral. Accuracy in the cardinality tasks for numbers from zero to nine in the final 10 sessions of testing was over 90%. Accuracy in a numeral ordering task (comparing three numerals, sometimes non-consecutive) was also very high. Persisting confusions of zero with one led the authors to conclude that Ai's grasp of zero was incomplete. But Ai's accuracy with positive numbers confirms the findings of the earlier study. In particular, her performance constitutes good evidence that she could recognize numbers at least within the subitizing range and distinguish them from larger or smaller positive numbers.

Taking numbers to be set sizes, studies with pre-schoolers and chimpanzees provide evidence that they can fulfil the recognize-and-distinguish condition for cognitive access to one, two and three. Moreover, their ability to recognize these numbers is explicit. An infant comes to recognize phonemes of its mother's language, such as ‘’ of UK English. This is tacit recognition, as the infant cannot think or express ‘that's ’. But pre-schoolers and trained chimpanzees can think and express ‘that's 3’.

(e). Cognitive access to larger numbers

The ability to recognize the number of a perceptible set of things and to distinguish it from the number of smaller or larger sets is a sufficient condition for cognitive access to a number, but not a necessary condition: we surely have cognitive access to the cardinal number 53, but most of us, when presented with a set of 53 items in favourable viewing conditions, cannot perceive it to have just that number of members rather than 52 or 54.

A proper account of our cognitive access to larger numbers would exceed my space limit (and my remit, as the account could not be supported by findings about basic number abilities alone). But a brief indication is possible. We have cognitive access to some larger numbers by means of identifying descriptions in terms of smaller numbers. With enough counting experience, we will know the order of the numbers well beyond the subitizing range; once we have acquired a concept for cardinal successor, we can grasp four as the successor of three, and five as the successor of four, and so on. With concepts for cardinal addition and multiplication, other identifying descriptions in terms of smaller numbers become available. For example, we know 28 not only as the successor of 27 but also as 20 + 8 and as 4 × 7. The decimal place system of numerals provides identifying descriptions of much larger numbers, as polynomials in powers of 10: 9605, for example, is 9 × 10³ + 6 × 10² + 5. The cardinal number 0, like the empty set, is more puzzling; but, with a grasp of subtraction, we can know it by an identifying description in terms of an already known number.

5. Conclusion

Taking cardinal numbers to be set sizes, the cognitive access problem for cardinal numbers can be solved by paying attention to empirical findings about basic number abilities. Studies with pre-schoolers, trained chimpanzees and other non-humans [34] provide evidence that they can fulfil a recognize-and-distinguish condition sufficient for cognitive access to numbers one, two and three. We have access to larger numbers by means of identifying descriptions in terms of smaller numbers.

Data accessibility

This article has no additional data.

Competing interests

I declare I have no competing interests.

Funding

I received no funding for this study.