Decomposing the Bragg glass and the peak effect in a Type-II superconductor

Abstract

Adding impurities or defects destroys crystalline order. Occasionally, however, extraordinary behaviour emerges that cannot be explained by perturbing the ordered state. One example is the Kondo effect, where magnetic impurities in metals drastically alter the temperature dependence of resistivity. In Type-II superconductors, disorder generally works to pin vortices, giving zero resistivity below a critical current j_c. However, peaks have been observed in the temperature and field dependences of j_c. This peak effect is difficult to explain in terms of an ordered Abrikosov vortex lattice. Here we test the widespread paradigm that an order-disorder transition of the vortex ensemble drives the peak effect. Using neutron scattering to probe the vortex order in superconducting vanadium, we uncover an order-disorder transition from a quasi-long-range-ordered phase to a vortex glass. The peak effect, however, is found to lie at higher fields and temperatures, in a region where thermal fluctuations of individual vortices become significant.

The disordering of the vortex lattice in a type-II superconductor is widely perceived to underpin unusual peaks in the temperature and field dependence of critical current. By contrast, here Toft-Petersen et al. find an order-disorder transition in a superconducting vanadium sample that is unconnected with peaks observed in critical current.

Introduction

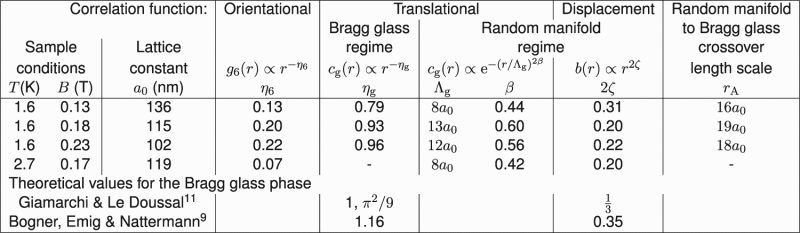
It has taken many decades to unravel the effect of weak disorder on the vortex lattice. In the 1970s, investigations initiated by the famous Russian theorist Anatoly Larkin found that any random disorder, no matter how weak, would destroy long-range order¹. In Larkin’s perturbative approach^1,2, vortex displacements grow linearly with vortex separation r, as measured by the displacement correlation function $b\left(r\right)=⟨{\left({\mathbf{u}}_j-{\mathbf{u}}_l\right)}^2⟩$, where u_j is the displacement of the jth vortex. This leads to an absence of divergent Bragg peaks. Yet resolution-limited Bragg peaks from vortex ensembles are clearly observed in scattering experiments^3–6. It turns out the perturbative approach becomes invalid at in-plane spacings r = r_c, where displacements become larger than ξ, the characteristic scale of the disorder potential. The behaviour at spacings r < r_c is accordingly described as belonging to the Larkin or random-force regime. At larger scales, vortices compete for minima in the disorder potential, slowing the algebraic growth of displacements to b(r) ∝ r^2ζ, where the roughness exponent $\zeta <\frac{1}{2}$. In this so-called random manifold regime, theoretical values for ζ can be derived from the elastic Hamiltonian by scaling estimates^7,8, renormalisation group analysis⁹ or variational replica-symmetry breaking techniques^10,11. A summary of theoretical values for ζ is reproduced in Table 1.

Table 1.

Properties of vortex–vortex correlations in superconducting vanadium

The topologically pristine Bragg glass phase is predicted by elastic theory with weak underlying disorder¹¹. It has quasi-long-range positional order comprising of logarithmically growing displacements b(r) ∝ ln r and algebraically decaying translational correlations $c_{\mathrm{g}}\left(r\right)\propto r^{-{\eta }_g}$ at large length scales r > r_A. At smaller scales r < r_A, correlations show the behaviour of a random manifold with b(r) ∝ r^2ζ and c_g(r) ∝ exp[−(r/Λ_g)^2β]. Our observed exponents in the Bragg glass phase are compared with predicted values from elastic theory^9,11. A slight dependence of these exponents on the elastic moduli is expected⁹ and here we list values appropriate for our vanadium sample. The Bragg glass is expected to become unstable at higher fields or temperatures. As shown by the data at 2.7 K and 0.17 T, the asymptotic regime is the first to be suppressed as the vortex ensemble disorders

At larger scales r = r_A, where vortex displacements become of order $a_0={\left(2{\mathrm{\Phi }}_0\mathrm{∕}\sqrt{3}B\right)}^{\frac{1}{2}}$, the lattice spacing set by flux quantisation, the periodicity of the system becomes crucial¹². Here the random manifold gives way to a Bragg glass regime. This asymptotic regime at r > r_A is characterised by a slower, logarithmic growth¹³ b(r) ∝ ln r and a translational order correlation function $c_{\mathrm{g}}\left(r\right)=⟨{\mathrm{e}}^{\mathrm{i}\mathbf{g}.\left({\mathbf{u}}_j-{\mathbf{u}}_l\right)}⟩$ that decays algebraically with an exponent η_g (Table 1)^9,11. The result is quasi-long-range order with algebraically diverging Bragg peaks and so the resulting vortex phase is referred to as the Bragg glass. Note that this term is used to describe both the asymptotic regime and, synecdochically, all three regimes in length scale. Where clarity is called for, we refer to the former as the Bragg glass regime and the latter as the Bragg glass picture.

The Bragg glass picture is expected to break down when dislocations become important. Upon increasing field or disorder strength, a transition to a short-range ordered vortex glass phase is expected as the pinning energy exceeds the plastic deformation energy^14–16. Notionally, disorder affects translational order more than orientational order, so the vortex glass is likely hexatic¹⁷. The orientational order is characterised by the correlation function $g_6\left(r\right)=⟨{\mathrm{e}}^{\mathrm{i}6\left({\theta }_j-{\theta }_l\right)}⟩$, where θ is the nearest neighbour bond angle^17,18. Experimentally g₆(r) is observed to decay algebraically $\propto r^{-{\eta }_6}$ in both the Bragg glass and vortex glass phases. A previous SANS study¹⁹ of the Bragg glass regime in niobium reported η₆ = 0.07. In the vortex glass, images of disordered vortex ensembles on the surfaces of NbSe₂²⁰ and Bi₂Sr₂CaCu₂O_8+δ^21,22 yield η₆ ≈ 0.06–0.35. These images also show a much faster, exponential decay of translational order, consistent with a hexatic vortex glass.

At temperatures close to the upper critical field B_c2(T) at which bulk superconductivity disappears, thermal fluctuations become increasingly important. They drive a proliferation of dislocations and a thermodynamic melting of the vortex lattice. The relative role of thermal fluctuations is quantified by the Ginzburg number⁸ $\mathrm{Gi}\approx {\left({\mu }_0{k}_{\mathrm{B}}{T}_{\mathrm{c}}{\kappa }^2\mathrm{∕}2{\xi }^3{B}_{\mathrm{c2}}^2\right)}^2$. The thermal melting line B_m(T) of the vortex lattice can be estimated from the phenomenological Lindemann criterion $⟨u^2⟩=c_{\mathrm{L}}^2{a}_0^2$ whereby melting occurs when displacements become a fraction c_L of the lattice spacing a₀. Typically c_L ≈ 0.1–0.2. This gives^23,24

$$\left(B_{\mathrm{c2}}\left(T\right)-B_{\mathrm{m}}\left(T\right)\right)\mathrm{∕}{B}_{\mathrm{c2}}\left(0\right)\approx 0.43c_{\mathrm{L}}^{-\frac{4}{3}}{\mathrm{Gi}}^{\frac{1}{3}}{t}^{\frac{2}{3}}{\left(1-t^2\right)}^{\frac{2}{3}}$$ 1

where the reduced temperature t = T/T_c2. Since Gi ∝ ξ⁻⁶, the position of the melting line is strongly dependent on the coherence length ξ. For cuprate superconductors, ξ is a few nanometres, so Gi ≈ 10⁻²–10⁻³ and the melting line is expected 0.1–10 K (depending on the field) below B_c2(T). The expected position of the melting line is confirmed by experiments on the cuprates^25–27. On the other hand, in low-κ superconductors the melting line is much more difficult to resolve^5,28 due in part to the smallness of Gi. For our vanadium sample κ = 1.3, ξ = 26 nm (see Methods) and Gi ≈ 6 × 10⁻¹⁰, so B_m(T) is anticipated to lie very close to B_c2(T). For example, at 0.3 T and with c_L = 0.2, B_m(T) is within 8 mK of B_c2(T).

Equation (1) is obtained with consideration of thermal fluctuations only. A Lindemann-like approach can also be used to predict the field and temperature dependence of the order-disorder transition line B_dis(T) separating the Bragg glass and the vortex glass phases^23,29,30. To do this, disorder-induced fluctuations must be considered. At B_dis(T) the topologically ordered Bragg glass phase becomes unstable to the formation of dislocations. The dislocation network is anticipated¹⁶ to appear at scales ≈ r_A where displacements are on the order of a₀. This can be shown to be equivalent to a generalised Lindemann criterion of the form $u^2\left(a_0,0\right)=c_{\mathrm{L}}^2{a}_0^2$ where displacements u(r, l) are now parameterised by the separations in and out of the vortex plane^29,30. Note that the order-disorder transition can be driven entirely by disorder-induced displacements. Consequently as thermal fluctuations vanish for T → 0, B_dis(0) remains distinct from and less than B_c2(0) (Fig. 1), in contrast to the fluctuation-induced melting line B_m(T), which curves up to meet B_c2(0).

Fig. 1.

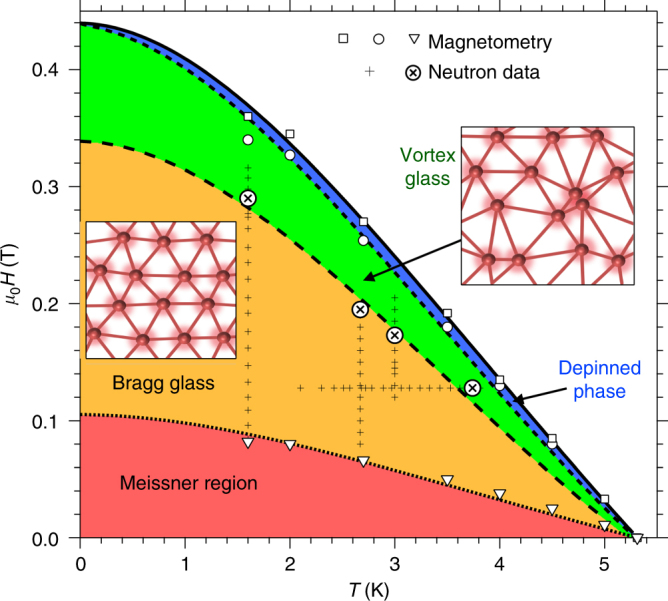
Phase diagram. Vortex phase diagram for our vanadium single crystal from magnetometry and neutron scattering experiments. Superconductivity appears below the upper critical field B_c2(T) (squares and solid line), with vortices forming between B_c2(T) and the lower critical field B_c1(T) (triangles and dotted line). The critical current j_c is observed to collapse in a region close to B_c2(T) (circles and short-dashed line). This collapse indicates that vortices are detaching from their pins. Vortex correlations are probed directly by neutron scattering. Plus signs mark the temperatures and fields where neutron scattering data were collected. The disappearance of neutron diffraction peaks (crosses in circles) marks the vortex order-disorder transition H_dis(T). Short-dashed and long-dashed lines are fits based on different types of Lindemann criteria²³ described in the text. The insets schematically illustrate the vortex phases either side of the order-disorder line. Intriguingly, the peak effect is not located at the order-disorder line. Instead it is observed close to the depinning line

Here we use small-angle neutron scattering (SANS) to probe the long-range correlations of vortex ensembles in a vanadium single crystal. The SANS technique provided the first experimental evidence for the Bragg glass picture: a dependence of the diffracted peak intensity upon magnetic field B that could not be explained by perfect crystalline order³. We also find evidence from the field dependence of the diffracted intensity for a Bragg glass picture at intermediate fields in the phase diagram (Fig. 1). Furthermore we demonstrate the presence of a Bragg glass regime by characterising the shape of the diffraction peak in a high-resolution experimental set-up (Fig. 2) and using reverse Monte Carlo refinement to extract correlation functions from our data¹⁹. Our manuscript proceeds as follows: first we characterise the underlying disorder and the peak effect using magnetometry data on our vanadium single crystal. We subsequently examine our SANS data collected over several experiments and using different experimental setups. These SANS experiments allow the order-disorder transition to be located. Comparing the order-disorder transition line B_dis(T) determined from SANS with the critical current density j_c determined by magnetometry, we discern no jump in j_c around B_dis in our sample. Instead, we observe a nascent peak effect at fields and temperatures close to B_c2(T). This dissimilarity is at odds to the commonly held notion that the peak effect is underpinned by the order-disorder transition from Bragg glass to vortex glass.

Fig. 2.

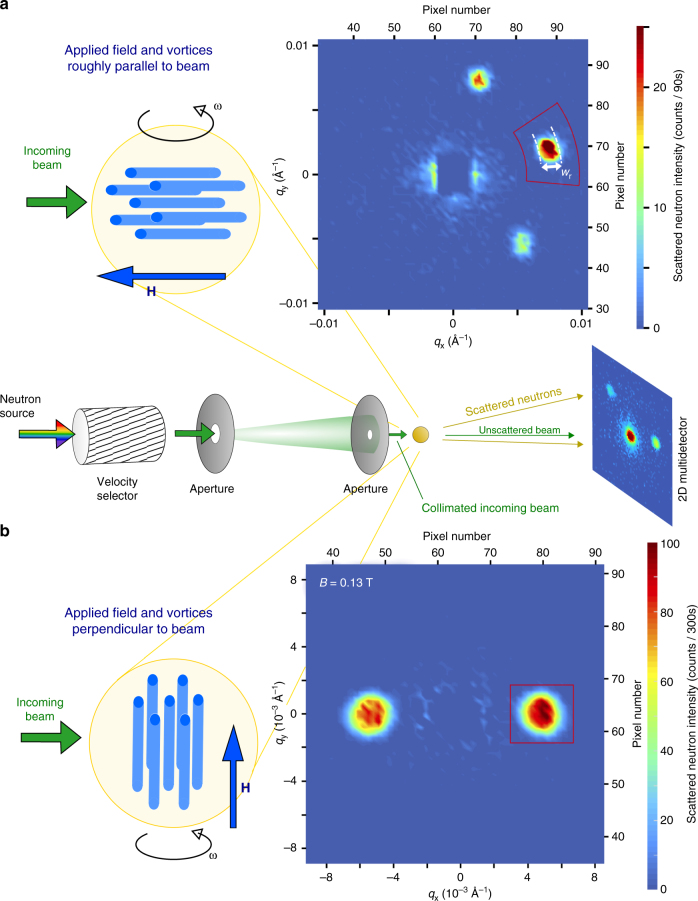
Neutron scattering geometries. Small-angle neutron scattering (SANS) instruments are usually pinhole cameras with small apertures to tightly collimate the incoming neutron beam. The magnetic field profile presented by vortices in the sample diffracts neutrons. Rocking curves are collected by rotating the sample, field and vortices together through the Bragg condition. Two experimental geometries may be used, exploiting the significantly better instrument resolution in the direction probed by the rocking curve: (a) The applied magnetic field and vortices are orientated roughly parallel to the neutron beam. Here the rocking curve is most sensitive to correlations along the vortices. A typical image of the 2D SANS multidetector at the peak of the rocking curve of the right Bragg spot is shown. The rotation axis for this rocking curve is indicated by ω. The unscattered neutron beam in the centre of the image at q = 0 is blocked by a beamstop. (b) The rocking curve probes correlations in the plane of the vortices when the applied magnetic field and vortices are orientated perpendicular to the neutron beam. The detector image shows the peak of the rocking curve of the right spot at 0.13 T

Results

Critical current from magnetometry

Our sample is a vanadium single crystal of cylindrical shape, with length 10 mm and diameter 2R = 5 mm. The [111] cubic crystal direction is coincident with the cylindrical axis. We measured the isothermal magnetisation M as a function of magnetic field H applied parallel to [111], collecting M(H) curves at several temperatures. Figure 3a shows a typical M(H) loop, measured at T = 1.6 K. Extracting the upper critical field from these loops, we find B_c2(T) is well described by the empirical relationship³¹ $B_{\mathrm{c2}}\left(t\right)=B_{\mathrm{c2}}\left(0\right)\left(1-t^2\right)\mathrm{∕}\left(1+\frac{2}{3}{t}^{7∕4}\right)$ with B_c2(0) = 0.440 T. Compared to pure vanadium where T_c = 5.47 K³², the small increase in B_c2(T) and the small suppression of T_c = 5.31 K measured for our sample indicate that the underlying disorder is weak. We obtain a mean free path l = 48 nm (see Methods). From the M(H) loops, the critical current density j_c = 3ΔM/2R is calculated using the critical-state model of Bean³³. j_c is seen to be small <10⁷ Am⁻² (c.f. Fig. 3b) compared to the depairing current density $j_0\approx H_{\mathrm{c}}\mathrm{∕}\lambda \approx B_{\mathrm{c2}}$/$\left(\sqrt{2}\kappa \lambda \right)\approx 4\times 10^{12}$ Am⁻², consistent with weak pinning.

Fig. 3.

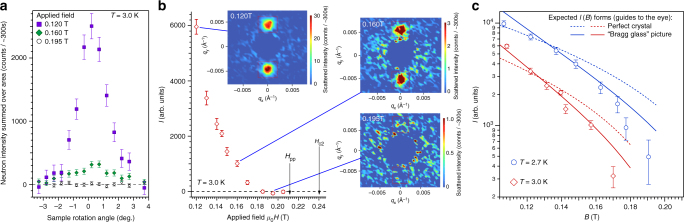
Magnetometry at 1.6 K. a M(H) loop for our vanadium single-crystal with $H\parallel \left[111\right]$. b Critical current density j_c calculated from the width ΔM of the magnetisation loop. j_c(B) decreases monotonically except for a small peak, labelled B_pp, observed just before the sharp downturn where thermal fluctuations start depinning vortices. The dashed line is a fit to an algebraic decay j_c ∝ B^α. Fitted value of α = −1.7. Inset is a magnification of the region around B_pp

Underlying disorder constrains vortex displacements with a pinning force density F_p of magnitude j_cB. Close to B_c2(T), thermal fluctuations enable vortices to ride over the pinning potential and F_p is accordingly expected to decrease at high fields and temperatures. It follows that there must be a maximum in F_p as a function of B. Many models have been proposed to describe the field dependences of F_p and j_c. Motivated by data on alloy superconductors with strong pinning, the first models estimated the average pinning force per vortex, accounting semi-empirically for the type and geometry of the pins^34–37. For isotropic superconductors like vanadium, these models^37,38 place the maximum in F_p(B) at fields ≥ 0.2B_c2. For our vanadium sample we find F_p(B) does scale with B_c2(T), but the maximum is located at 0.13B_c2, i.e. at lower fields than expected and the observed j_c(B) dependence is not accurately reproduced by these semi-empirical models^34–37.

More recent models for j_c(B) consider the contribution to pinning at different length scales⁸ and the dynamic effects of flux creep³⁹. These models give various j_c behaviours depending on the regime of field and temperature. For example, the large bundle pinning regime is entered when pinning is weak and vortex length scales are large compared to the penetration depth λ. Here non-locality of the elastic constants can be neglected. In our low-κ superconductor, λ ≈ 35 nm is short. The minimum vortex lattice spacing a₀ is 74 nm, the value at B_c2(0), so vortex lattice length scales generally exceed λ and we expect to be in the large bundle regime in most of the mixed state. In this regime, j_c is theoretically predicted to follow⁸

$$j_{\mathrm{c}}\approx \frac{j_0}{{\kappa }^2}{\left(\frac{a_0}{l_{\mathrm{c}}}\right)}^6$$ 2

where l_c is the scale delimiting the Larkin regime parallel to the vortices. The $a_0^6$ dependence yields^8,39 an algebraic decay of j_c(B) ∝ B⁻³. As shown in Fig. 3b, we do find j_c(B) can be described by an algebraic decay for 0.1 T < B < 0.33 T. With values of j₀ and j_c for our sample, Eq. (2) gives l_c ≈ 10a₀ over this field range. However the observed algebraic decay is slower than the B⁻³ predicted, with a reduced exponent j_c(B) ∝ B^−1.7.

Perhaps it is not too surprising that no literature model describes our j_c data precisely. All of the theoretical and empirical models described^8,34–39 focus on high-κ superconductors. Our sample has low κ = 1.3 and a large part of the superconducting phase diagram is occupied by a Meissner regime (Fig. 1). In what follows we concentrate our analysis at high B, away from the Meissner regime.

For most of the mixed state, i.e. from B = 0.10 to 0.33 T at 1.6 K (Fig. 3b), the sample supports a finite critical current, indicating that vortices are pinned and frozen. At B = 0.34 T there is a sharp downturn in j_c and it falls rapidly up to B_c2. This indicates vortices are depinning as thermal fluctuations take hold just below B_c2. Just below the depinning region at B = 0.335 T, a nascent peak in j_c can be seen (Fig. 3b inset). This peak effect is less pronounced than in other reports⁴⁰, which is to be expected given the weak underlying disorder in our sample. The location of the peak effect is consistent with previous transport measurements and magnetometry on niobium and vanadium under neutron irradiation^41–43 and with transport measurements on niobium and Nb—Ta alloys under plastic deformation^44,45. These systematic studies all show the same development of the peak effect: with increasing irradiation or deformation, the peak effect emerges first at high fields close to B_c2 before developing into a larger peak that pushes to lower fields as the density of pinning centres increases^41,42. The reverse effect, where the peak effect is reduced and pushed back up to high fields, is also observed when samples are annealed following neutron irradiation⁴³.

Order–disorder line from small-angle neutron scattering

Following the seminal study by Larkin and Ovchinnikov² on the collective pinning of vortex ensembles, we might expect the jump in j_c to be associated with a loss of vortex order. Indeed, peak effects have commonly been linked with order-disorder transitions of vortex lattices in the literature^23,40,46,47 To test this paradigm, we directly probe the vortex lattice order in our sample using SANS (Fig. 2).

Neutron scattering is sensitive to correlations between pairs of vortices via the structure factor $S={\sum }_{j,k}{\mathrm{e}}^{-\mathrm{i}\mathbf{q}.\left({\mathbf{r}}_j-{\mathbf{r}}_k\right)}$ where r_j is the position of the jth vortex and q is the scattering vector. The measured scattered intensity as a function of q is the convolution of ${∣h\left(q\right)∣}^{2}S$ with the instrument resolution function, where h(q) is the form factor of a single vortex. For a perfect vortex lattice, the structure factor consists of δ-function Bragg peaks at reciprocal lattice vectors, with the first such vector appearing at $g=2\pi {\left(2B{\mathrm{∕\Phi }}_0\sqrt{3}\right)}^{\frac{1}{2}}$ in the case of a triangular lattice. Experimentally the Bragg peak intensity is quantified by integrating the measured scattering over three directions in reciprocal space. This is achieved by measuring rocking curves (Fig. 4a), where the sample, field and vortex lattice are rotated together through the Bragg condition. An area on the 2D SANS multidetector encompassing the Bragg spot is then summed and these summed counts, plotted versus rocking angle (Fig. 4a), are fitted to a Gaussian. The resulting integrated intensity I of the first order Bragg peak is shown in Fig. 4b, for T = 1.6 K after field-cooling. In the vortex glass, rapidly decaying translational order c_g is expected that does not support Bragg peaks. Combined with the rapid fall-off of the form factor⁴⁸, this means little, if any, neutron scattering will be discernible from the vortex glass in our SANS experiments.

Fig. 4.

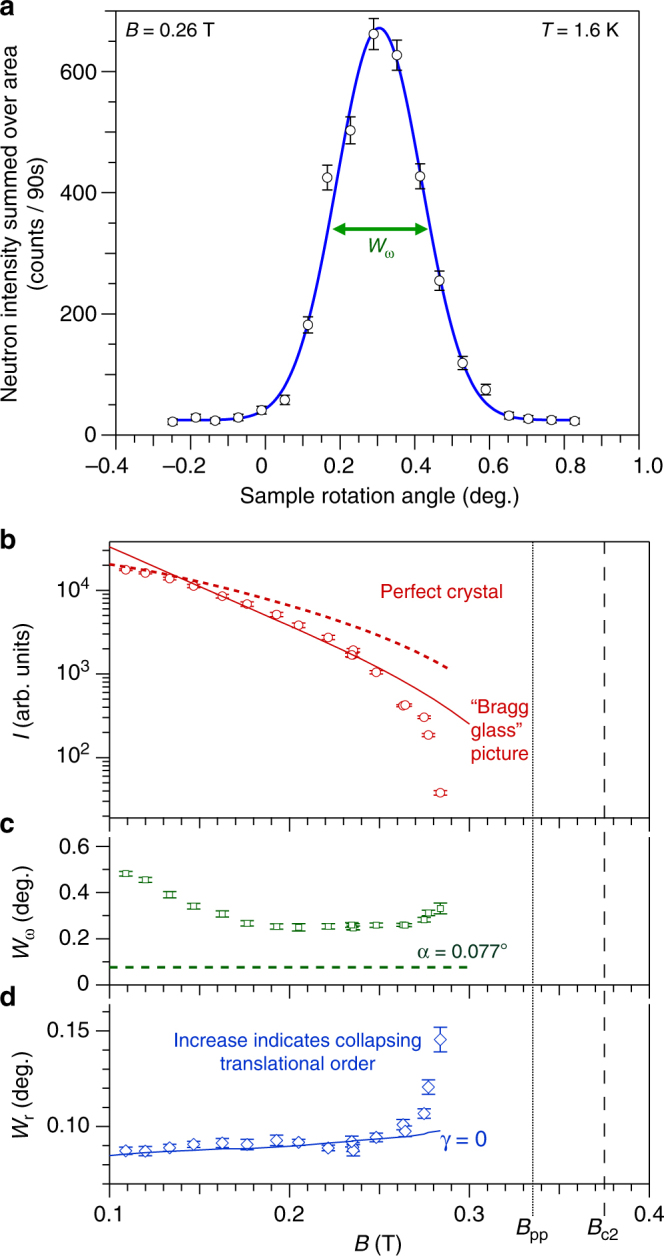
Neutron scattering in parallel field geometry at 1.6 K. a Rocking curves are collected by rotating the sample, field and vortices together through the Bragg condition. Neutron counts are summed over an area on the 2D multidetector encompassing the Bragg spot (red sector in Fig. 2a). Error bars are determined by Poisson statistics. Solid line is a Gaussian fit, with W_ω denoting the measured rocking curve width. b Field dependence, at T = 1.6 K, of the scattered neutron intensity I integrated over the rocking curve. Vortex ensembles are prepared by field-cooling, i.e. by cooling in the desired field from the normal state. Dotted line is a guide to the eye illustrating the form expected if vortices were arranged in a perfect 2D lattice. A steeper descent arises from the quasi-long-range order of the Bragg glass picture and scales to the data well (solid line) up to 0.26 T, whereupon I decays rapidly. Note that I vanishes much before the peak effect B_pp. c W_ω probes correlations along the vortices in the parallel field geometry (Fig. 2a). The dominant contribution to the instrument resolution is the angular spread a of the incoming beam, illustrated by the dashed line. a = 0.077° corresponds to a maximum resolvable correlation length s = 2.35/(ga) = 240a₀. d Radial width W_r of the Bragg spot on the 2D neutron multidetector at the peak of the rocking curve (Fig. 2a) is sensitive to correlations along the vortices and to translational correlations in the vortex plane. Solid line depicts the calculated radial width under the assumption of infinite translational correlational length 1/γ, i.e. it represents the contribution to W_r from instrument resolution and from finite correlations along the vortices. The rapid departure from this line at 0.27 T indicates collapsing translational order as the order-disorder transition is approached

We see in Fig. 4b that at T = 1.6 K the integrated intensity I(B) begins a downturn before quickly becoming indiscernible from the background at 0.29 T. We may therefore locate the vortex order-disorder line B_dis(T) at 1.6 K, 0.29 T. Similarly we locate B_dis(T) from the field dependence of I at two other temperatures (Fig. 5). All three B_dis(T) points identified (crosses in circles in Fig. 1) lie well below B_c2(T). B_dis(T) may also be determined by measuring the scattered intensity as a function of temperature at constant field. In Fig. 6 we show the scattering measured at the peak of the rocking curve upon warming at 0.13 T, starting from a well-ordered vortex ensemble at 2 K. These data also demonstrate the vanishing of neutron intensity well below the upper critical field line and confirm that B_dis(T) lies deep in the mixed state.

Fig. 5.

Field dependence of neutron scattering at higher temperatures. Field-cooled vortex ensembles are probed in perpendicular field geometry (Fig. 2b) with horizontal applied field. Backgrounds measured at zero field are subtracted. a Rocking curves at 3.0 K showing counts summed over an area on the 2D multidetector encompassing the top Bragg spot. Error bars are determined by Poisson statistics. b Top and bottom Bragg spot rocking curves are integrated numerically and the sum I plotted versus applied field. I vanishes much before the peak effect field H_pp anticipated from the phase diagram (Fig. 1). Each 2D image shows the SANS multidetector averaged over the rocking scan. Noise from subtraction at the centre of each image is masked. Red boxes indicate areas summed in the rocking curves. c Semilogarithmic plots at 2.7 and 3.0 K of I versus flux density B calculated from observed Bragg spot position g using the flux quantisation relation $g=2\pi {\left(2B{\mathrm{∕\Phi }}_0\sqrt{3}\right)}^{1∕2}$ for a triangular lattice. Dotted lines are guides to the eye illustrating I(B) forms expected if vortices were arranged in perfect 2D lattices. Solid lines are guides to the eye showing the steeper descents expected in the Bragg glass picture

Fig. 6.

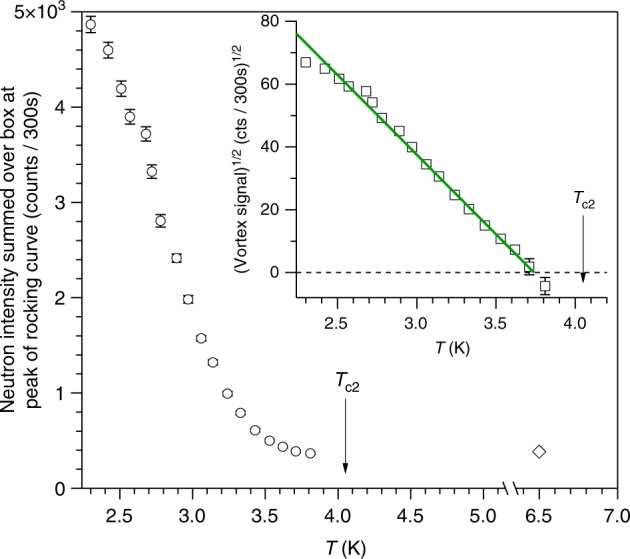
Temperature dependence at 0.13 T. Neutron scattering from the vortex ensemble is measured on warming, after field-cooling to 2 K. Using the perpendicular field geometry (Fig. 2b), neutron counts are collected at the peak of the rocking curve and summed over a box on the 2D multidetector encompassing the Bragg spot (red box in Fig. 2b). The diamond indicates a background measurement at T > T_c characterising the non-vortex scattering that arises predominantly from the walls of the closed-cycle refrigerator used to cool the sample. Error bars are determined by Poisson statistics. Inset shows the square root of the vortex signal I_p, which is proportional to the vortex form factor h when the field is constant. Negative values of vortex signal, arising from the background subtraction, are indicated by plotting $\mathrm{sgn}\left(I_{\mathrm{p}}\right)\sqrt{∣I_{\mathrm{p}}∣}$. Green line is a guide to the eye showing a linear variation, as expected from mean field theory in the vicinity of a phase transition

Bragg glass picture from integrated neutron intensity

For a perfect crystalline lattice, integrating the structure factor S yields a factor 1/g at small scattering angles, so the field dependence of I is described by ${∣h\left(g\right)∣}^2\mathrm{∕}g$. For the Bragg glass picture, on the other hand, the factor³ from integrating S depends on whether the SANS instrument resolution s is larger or smaller than the crossover scale between random manifold and Bragg glass regimes, at which displacements are of the order a₀. For SANS measurements with the field and vortices aligned roughly parallel to the neutron beam, the width of the rocking curve probes correlations along the direction of the vortices (see Methods). Then if s < l_A, where l_A is the crossover scale parallel to the vortices, integrating the structure factor in the Bragg glass picture yields a 1/g, i.e. a B^−1/2 dependence similar to the perfect crystalline case. If s > l_A, the Bragg glass picture produces an additional factor B^−3/2. This hypothesis was borne out by the I(B) measured on (K,Ba)BiO₃ in the first experimental report of the Bragg glass³. Similar I(B) Bragg glass dependences have been reported in the electron-doped cuprate Nd_1.85Ce_0.15CuO₄ and in underdoped La_2−xSr_xCuO₄ (x < 0.15)⁴⁹.

In Fig. 4b we compare the integrated intensity I(B) measured at 1.6 K with the field dependence expected for a perfect crystalline lattice and that expected in the Bragg glass picture. For these measurements, the instrument resolution s ≈ 240a₀ along the direction of the vortices (see Methods). Up to B ≈ 0.26 T, i.e. before I falls-off more sharply as B_dis is approached, it can be seen that the field dependence in the Bragg glass picture describes the data rather better than for the perfect crystalline case. We deduce that l_A < s ≈ 240a₀ from our experiments. In elastic theory⁸, length scales along the vortices and length scales in the vortex plane are coupled through the elastic moduli via $\sqrt{c_{44}\mathrm{∕}{c}_{66}}$. Approximate expressions for the tilt modulus c₄₄ ≈ BH and for the shear modulus are⁵⁰

$$c_{66}\approx \frac{B{\mathrm{\Phi }}_0}{16\pi {\lambda }^2{\mu }_0}\left(1-\frac{1}{2{\kappa }^2}\right){\left(1-\frac{B}{B_{\mathrm{c2}}}\right)}^2$$ 3

The shear modulus c₆₆ softens close to the upper critical field line as vortex cores start to overlap. Deeper in the mixed state, e.g. for fields 0.1 T < B < 0.33 T, we find the calculated aspect ratio $\sqrt{c_{44}\mathrm{∕}{c}_{66}}$ lies in the range from 2 to 30. An upper bound for the crossover scale r_A in the plane of the vortices can thus be determined e.g. at 0.23 T, this upper bound is $s\mathrm{∕}\sqrt{c_{44}\mathrm{∕}{c}_{66}}\approx 30a_0$. In the next section, we see r_A can be precisely quantified using a high-resolution SANS setup.

Bragg glass regime from reverse Monte Carlo refinement

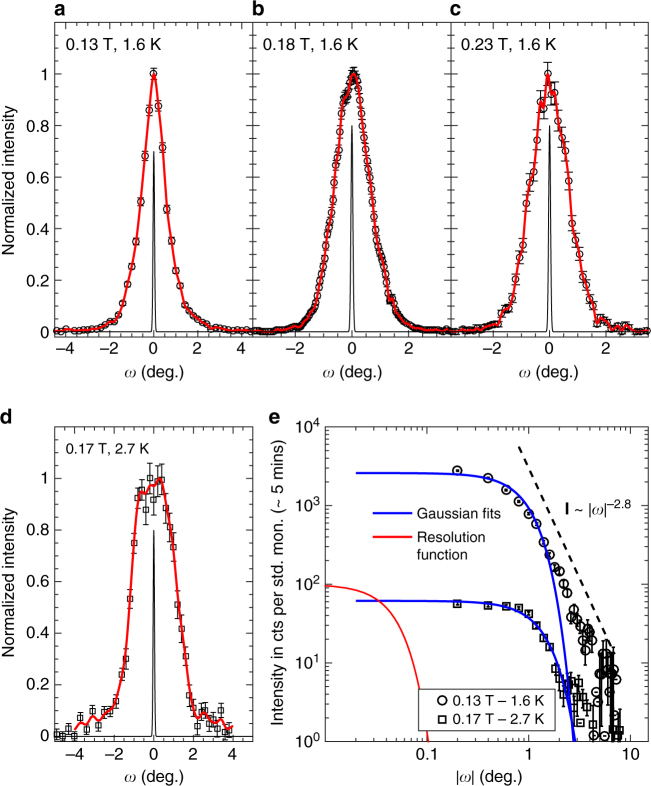
To gain more detailed information about the Bragg glass and vortex glass phases in the sample, we employ a second experiment geometry in our SANS measurements. The SANS data in Fig. 4 are collected in the first experiment geometry where applied field and vortices are orientated roughly parallel to the incoming neutron beam (Fig. 2a). In the second experiment geometry, the applied field and vortices are orientated perpendicular to the beam (Fig. 2b). In this geometry, a high instrument resolution in the plane of the vortices is achieved (see Methods) and rocking curves, collected by rotating the sample, field and vortex ensemble together through the Bragg condition, probe correlations within the vortex plane, transverse to the reciprocal lattice vector (Fig. 7). Such data (Fig. 7e) are seen to exhibit an algebraic decay with rotation angle ω. This algebraic decay is characteristic of the Bragg glass regime^11,19. The observed exponent ≈ −2.8 (Fig. 7e) is roughly consistent with the value ≈ η_g − 3 predicted¹¹ for the Bragg glass regime where c_g(r) decays algebraically $\propto r^{-{\eta }_{\mathrm{g}}}$, with η_g ≈ 1 (Table 1).

Fig. 7.

Neutron scattering in perpendicular field geometry. In the perpendicular field geometry (Fig. 2b), rocking curves probe correlations in the plane of the vortices. a–d Rocking curves of the first order diffraction peak at four selected points in the phase diagram, collected with long counting times to afford high quality data for reverse Monte Carlo refinement. Backgrounds measured at zero field have been subtracted. Ordinate axes are normalised for comparison. In reverse Monte Carlo refinement, simulated vortices are displaced using a Monte Carlo method until the experimental rocking curve (circles) is reproduced (red lines). Black lines indicate instrument resolution. e Rocking curves of a and d on logarithmic axes. Dashed line is a guide showing an algebraic decay. Algebraic tails are expected from the Bragg glass picture. Gaussian fits (blue lines) are drawn for comparison

To uncover more information about the vortex correlations, we use the reverse Monte Carlo (RMC) technique to refine the peak shapes of rocking curves collected in the perpendicular field geometry¹⁹. Up to 220,000 vortices are simulated on a computer, with their positions modified successively via Monte Carlo updates, until the observed peak shape is reproduced (see Methods). Meaningful refinements require experimental data that span several decades of rotation angle ω. Long data collection times are needed for the tails where the scattering is weak. We measured such datasets at four selected fields and temperatures in the phase diagram (Fig. 7).

Once the simulated rocking curve numerically reproduces the experimentally measured rocking curve (Fig. 7), correlation functions may be calculated from the simulated vortex positions. A simulated vortex ensemble is not unique: there are many possible simulated microstates that can reproduce the experimental peak shape. However, similar correlation functions are observed to result from such microstates¹⁹. In Fig. 8 we show typical correlation functions extracted using RMC at each of the four points measured in the phase diagram. Looking first at the three datasets collected at T = 1.6 K and B = 0.13, 0.18 and 0.23 T, we see these show the same qualitative features, namely two distinct regimes in length scale. At small r, the displacement correlation function b(r) is seen to increase algebraically while c_g(r) is seen to decay as a stretched exponential. This clearly represents a random manifold regime^9–11 and accordingly here we fit the data to b(r) ∝ r^2ζ and c_g(r) ∝ exp{−(r/Λ_g)^2β}. The fitted values of roughness exponent ζ, exponential decay exponent β and the effective correlation length Λ_g agree well with theoretical predictions (Table 1). The absence of an observable Larkin regime at small r is consistent with the weak disorder of our sample¹¹. Using the above estimate of l_c ≈ 10a₀ from the critical current, the Larkin regime would be expected at in-plane length scales below $r_{\mathrm{c}}\approx l_{\mathrm{c}}\mathrm{∕}\sqrt{c_{44}\mathrm{∕}{c}_{66}}\approx 2a_0$, a value which is too small to be resolvable from the r-dependence of correlation functions.

Fig. 8.

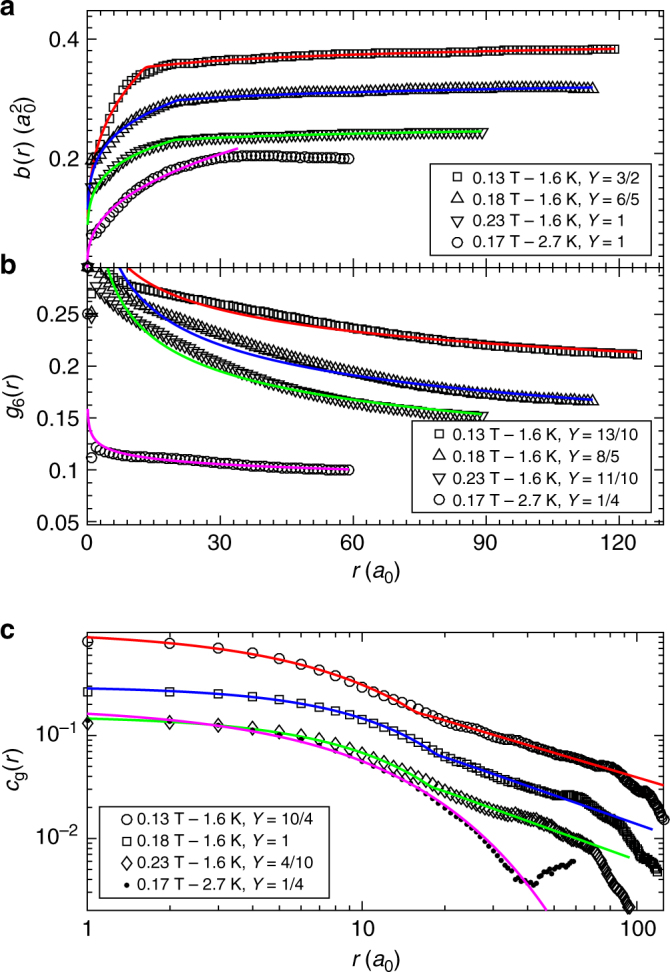
In-plane correlations educed by reverse Monte Carlo refinement. Correlation functions calculated from simulated vortex ensembles (open symbols) that reproduce the neutron scattering data in Fig. 7. For clarity curves are offset with a multiplication factor Y. (a) Displacement correlator $b\left(r\right)=⟨{\left({\mathbf{u}}_j-{\mathbf{u}}_l\right)}^2⟩$. (b) Hexatic orientational order $g_6\left(r\right)=⟨{\mathrm{e}}^{\mathrm{i}6\left({\theta }_j-{\theta }_l\right)}⟩$ is fitted with a single algebraic decay $\propto r^{-{\eta }_6}$. (c) Translational order $c_{\mathrm{g}}\left(r\right)=⟨{\mathrm{e}}^{\mathrm{i}\mathbf{g}.\left({\mathbf{u}}_j-{\mathbf{u}}_l\right)}⟩$ and b(r) comprise three regimes in the Bragg glass picture: the Larkin, random manifold and Bragg glass regimes (see text). Here the Larkin regime lies at indiscernibly small length scales and two-part functions fit b(r) and c_g(r) (solid lines) for the three datasets at 1.6 K. For the 0.17 T, 2.7 K dataset, which lies close to the order-disorder line, a single function fits c_g(r) corresponding to the random manifold regime

At large r, we identify a Bragg glass regime (Fig. 8). In this asymptotic regime, b(r) ∝ ln r and $c_{\mathrm{g}}\left(r\right)\propto r^{-{\eta }_{\mathrm{g}}}$, with the fitted η_g ≈ 1 (Table 1). The orientational order g₆(r) follows an algebraic decay $\propto r^{-{\eta }_6}$ through both the random manifold and the Bragg glass regimes. The fitted exponent η₆ ≈ 0.2 (Table 1) is rather less than the translational exponent η_g, reflecting the longer range of bond orientational order compared to translational order. We conclude that a Bragg glass phase is present at fields and temperatures below the order-disorder transition B_dis(T).

Fracturing of the vortex lattice

We turn now to the fourth dataset collected with long counting times in the perpendicular geometry at 0.17 T and 2.7 K, a point which lies close to the order-disorder transition B_dis(T) (c.f. Fig. 1). Here the rocking curve exhibits a distinctly different shape to the other three RMC datasets measured deeper in the Bragg glass phase. Its brow appears broader (Fig. 7d) and its tails seem less algebraic (Fig. 7e). The correlation functions extracted using RMC show a concomitant suppression of the asymptotic Bragg glass regime: the algebraic decay at large r in c_g(r) is no longer visible (Fig. 8c). Only a stretched exponential decay remains, with fitted exponents indicative of the random manifold regime (Table 1). b(r) also shows the sublinear growth expected in this regime.

There is more intriguing behaviour at large r. Here, instead of the slow logarithmic growth that would signal a Bragg glass, b(r) is seen to saturate at ≈ 30a₀ (Fig. 8a). This suggests the vortex ensemble is fracturing into domains, with reasonable positional order being maintained within each domain and large jumps in displacement, with possible locally amorphous regions, at the domain walls. Indeed, a finite crystalline domain of dimension ≈ 30a₀ would produce a Bragg peak of finite width ≈ 1.6°, which is roughly consistent with the broadening observed in the rocking curve (Fig. 7d). The orientational order g₆(r) for this RMC dataset is reduced compared to the other three RMC datasets, but it continues to decay slowly, with a fitted algebraic exponent η₆ = 0.07. Moreover, g₆(r) persists beyond the domain length scale ≈ 30a₀, indicating that domains share roughly similar nearest neighbour directions.

The fracturing observed of the Bragg glass is evocative of the prediction of a multidomain glass phase, that should separate the Bragg glass and vortex liquid phases⁴⁷. There are differences between prediction and experiment, however. The predicted multidomain glass phase should be confined to a thin sliver in the phase diagram coincident with the peak effect⁴⁷. Here, on the other hand, the fracturing of the ordered phase is observed at much lower fields and temperatures than the peak effect. The predicted multidomain glass also has both orientational and translational order decaying rapidly beyond the domain length scale, but here we see the orientational order g₆(r) survives to larger length scales even in the fractured vortex ensemble. Interestingly, qualitatively similar correlation functions were measured by decoration experiments on the high-T_c layered cuprate Bi₂Sr₂CaCu₂O_8+x (BSCCO)⁵¹. The formation of domains was proposed to be a nonequilibrium effect due to finite cooling rates⁵¹. We have initiated further SANS explorations to clarify this.

Discussion

The peak effect and other features in magnetometry and transport data are frequently assumed to be underpinned by changes in the positional order of the vortex lattice. Under this assumption, conclusions are sometimes drawn as to the nature of vortex phases without directly probing them. Several features can be identified in magnetometry or transport data, including the irreversibility line (H_irr), the peak field (H_pp) and the onset of the peak effect regime (H_po). In the high-T_c cuprates, a broad peak in ΔM may also be present at low temperatures and fields deep in the mixed state and is accordingly designated the fishtail effect or second magnetisation peak⁵². There are reports of both a sharp peak effect close to B_c2 and a broad second magnetisation peak well below B_c2 being observed in the same sample^53,54 indicating that these two effects are distinct. Accordingly, we should add to our list of features the onset field (H_fo), the fishtail peak field (H_fp) and the field at which the magnetisation shows a kink (H_fk).

On the other hand, the route from ordered vortex ensemble to vortex liquid is envisaged to occur in only one or two sharp steps, i.e. a direct melting from Bragg glass to vortex liquid, or an order-disorder transition to a vortex glass followed by melting to the liquid. Recent scanning tunnelling microscope (STM) experiments on Co-doped NbSe₂ indicate that there may be two vortex glass phases—a hexatic vortex glass and an amorphous vortex glass⁵⁵—though it is not clear whether the two phases identified are truly distinct. Nonetheless, there are far fewer disordering transitions than there are features in ΔM and j_c. It is an open question as to which of these features, if any, should be tied to an underlying order-disorder transition in the vortex ensemble. The irreversibility line may reasonably be associated with the melting transition²⁵, since a vortex liquid phase cannot ordinarily support a finite critical current. To which feature, on the other hand, should we associate the Bragg glass to vortex glass transition?

To date only a handful of studies have directly probed the order of the vortex ensemble and simultaneously pinpointed features in magnetisation or critical current. The first such study found the SANS intensity disappeared at the irreversibility line in BSCCO²⁵. This occurred at temperatures consistent with estimates of the melting line using the Ginzburg number and the Lindemann criterion, signalling a link between vortex lattice melting and H_irr. In addition, the diffracted neutron intensity was found to vanish abruptly with increasing fields at temperatures well below the irreversibility line, with no reported corresponding features in the bulk magnetisation²⁵. A subsequent muon spin rotation study indicated that this vanishing was due to a 3D to 2D crossover, where the pancake vortices in BSCCO, which arise due to its highly anisotropic layered structure, become decoupled between the superconducting layers⁵⁶.

Subsequent studies have focussed on the quasi-two-dimensional layered 2H-NbSe₂ system. Here, due to coexisting charge order, the vortex cores are strongly anisotropic in the plane, adopting a sixfold star shape^57,58. Magnetic impurities in 2H-NbSe₂ are adorned by bound states with the same sixfold star shape⁵⁹ which, we suggest, may drive the additional transition from hexatic glass to an amorphous vortex glass observed recently in Co-doped NbSe₂ samples⁵⁵. Interestingly this transition appears to coincide with the peak field H_pp(T) in these samples⁵⁵, indicating that the Bragg glass to hexatic glass transition should lie at lower fields and temperatures, e.g. perhaps at the onset H_po(T). This scenario is consistent with a recent SANS study⁶ on clean NbSe₂ where the SANS intensity all but disappears at H_po(T).

Unfortunately the experimental picture is neither so simple nor general. In Fe-doped NbSe₂, a well-ordered vortex lattice can be observed by SANS at fields much higher than H_pp, leading to the conclusion that the peak effect is unrelated to a bulk order–disorder transition⁶⁰. This conclusion is shared by an earlier decoration study on clean and Fe-doped NbSe₂⁶¹. The picture is muddied further by studies on isotropic conventional superconductors, like niobium, vanadium and V₃Si, where vortices have the usual line structure. In V₃Si⁶², the diffracted SANS intensity disappears at H_pp(T) but in Nb there are conflicting reports: in one SANS study H_pp(T) appears to coincide with the loss of in-plane positional order⁶³ but in another study a clear SANS signal indicating good vortex order is observed above the peak effect⁴. Together with our observation of a Bragg glass to vortex glass transition lying at much lower fields and temperatures than H_pp(T), we are drawn to the inevitable conclusion that the origin of the order–disorder transition and the origin of the peak effect are not a priori the same.

Recent experimental investigations also show that metastability of the vortex configuration and the associated dependences on history affect the vortex order and consequently the perceived position of the order–disorder transition^4,6,55,62. Bulk characteristics from transport^46,62 and magnetic susceptibility⁶ are also similarly affected. Here we note that our data point for B_dis(T) at 0.13 T and 3.74 K (Fig. 6) is obtained by warming the sample at constant field, so it is possible that the vortex configuration is superheated at the perceived order–disorder transition temperature of 3.74 K. If this were the case however, the equilibrium order–disorder transition would lie at even lower temperatures than we report here. Our conclusion, that the order–disorder transition and the peak effect are not necessarily related, would abide.

What are the origins of the order–disorder transition and of the peak effect? One possibility is a change in the nature of the underlying pinning such as a crossover from weak collective to strong pinning⁶⁴. For weak pinning, the pinning force density $F_{\mathrm{p}}=j_{\mathrm{c}}B={\left[f_{\mathrm{p}}^2{n}_{\mathrm{p}}{\left(\xi \mathrm{∕}{a}_0\right)}^2\mathrm{∕}{V}_{\mathrm{c}}\right]}^{1∕2}$, where f_p is the elementary pinning force and n_p = 1/ξ²l is the density of pins, $V_{\mathrm{c}}=l_{\mathrm{c}}{r}_{\mathrm{c}}^2$ is the correlation volume over which displacements reach the superconducting coherence length ξ following the random force model^2,64. Strong and/or single pinning is identified when f_p overcomes the Labusch force⁶⁴ $f_{\mathrm{L}}\approx {\mathrm{\Phi }}_0^2\mathrm{∕}4\pi {\mu }_0{\lambda }^2\approx 10^{-11}$ N for our sample. Since our data do not provide a direct measure of l_c or r_c, we make an overestimate of $V_{\mathrm{c}}\approx \sqrt{c_{44}\mathrm{∕}{c}_{66}}{r}_{\mathrm{A}}^3$ to yield an upper bound for f_p, which is maximal at low inductions, reaching ≈10⁻¹³ N. Thus throughout the mixed state $f_{\mathrm{p}}\ll f_{\mathrm{L}}$ and weak collective pinning is effective. There is no change in pinning regime at B_dis(T) or at the peak effect in our sample.

We return to the effect of thermal fluctuations. These allow the vortices to ride over the pinning potential and result in a collapse of the critical current. The onset of fluctuations in our sample is marked by the sharp downturn in j_c close to the upper critical field. At T = 1.6 K, this depinning of vortices occurs at B = 0.34 T (Fig. 3b). At 0.3 T, the depinning line lies ≈ 140 mK below B_c2(T), substantially below the thermally driven vortex lattice melting line, which we recall is 8 mK below B_c2(T) from Eq. (1). One can quantify how far a (B, T) point in the phase diagram lies from B_c2(T) using the scaled Thouless temperature a_T from the lowest Landau level of the Ginzburg-Landau theory. Thermodynamic melting is expected⁶⁵ at a_T = −9.5. Isotherms of constant a_T have approximately the same form as the melting line B_m(T) from Eq. (1), so we may identify $-a_{\mathrm{T}}\approx 0.43c_{\mathrm{L}}^{-\frac{4}{3}}$, e.g. a_T = −9.5 corresponds to a Lindemann number c_L ≈ 0.1. The depinning line in our sample corresponds to a_T ≈ −60 or c_L ≈ 0.02. The peak effect lies just below this line, i.e. on the edge of the regime where thermal fluctuations dominate. The possibility that thermal fluctuations induce the peak effect has been pointed out previously^66,67, however this view must be reconciled with the reported observations of well-ordered vortex lattices at temperatures and fields above the peak effect^4,60. We suggest that thermal fluctuations sufficiently reduce the order parameter Ψ, such that weak underlying pinning is suddenly accommodated by a rapid but local change in the structure of the vortex cores at the peak effect. This instability, localised to the cores, is not significant at larger scales such as λ or a₀ so lattice order is not necessarily disturbed through the peak effect.

At the order–disorder transition, on the other hand, the role of thermal fluctuations is insignificant. The four data points for B_dis(T) (Fig. 1) lie close to the c_L = 0.006 or a_T = −360 isotherm, i.e. far from the fluctuation dominated regime. Disorder can be incorporated into lowest Landau level theory via random components in the ${∣\mathrm{\Psi }∣}^2$ term (δT_c pinning)⁶⁵ and in the ${∣\mathrm{\Psi }∣}^4$ term⁶⁸, yielding order–disorder lines where the value of a_T varies along the line. We do not find these provide sensible fits to our four data points. We do find a sensible fit is provided by the order–disorder line derived for δT_c pinning using a Lindemann-like approach²³. In the limit of vanishing thermal fluctuations, i.e. Gi → 0, B_dis(T) then takes the form²³

$$1-\frac{B_{\mathrm{dis}}\left(T\right)}{B_{\mathrm{c2}}\left(T\right)}\approx {\left(\frac{2\pi }{c_{\mathrm{L}}^4}\right)}^{\frac{1}{3}}{D}^2{\left(1-t^2\right)}^{-\frac{1}{3}}$$ 4

D measures the strength of the disorder and is equal to ξ/l_c at 0 K in the Larkin model²³. We fit this parameter (Fig. 1), taking c_L = 0.2 and obtaining D = 0.12, which is a reasonable value for weak pinning. This yields l_c ≈ 3 a₀ at 0 K, consistent with our earlier estimate from j_c and with our RMC results. As B_dis(T) is approached upon warming (Fig. 6), the SANS intensity falls continuously to zero, indicating that B_dis(T) is a thermodynamic phase transition from Bragg glass to vortex glass. The radial width of the Bragg diffraction spot on the 2D SANS detector also increases as B_dis is approached (Fig. 4d), signalling a collapsing translational correlation length. We infer that continuous translational symmetry is broken at the Bragg glass to vortex glass transition. It is still a matter of debate, however, as to which symmetry—if any—is broken between the depinned vortex liquid and pinned vortex glass phases. We expect an exciting era in vortex matter physics, where intriguing possibilities such as the vortex glass being no more than a pinned, hexatic liquid¹⁸ are experimentally explored using increasingly available high-quality STM apparatus.

Overall, our data show that the peak effect and similar features in magnetometry or transport data may not a priori be due to a vortex order–disorder transition. SANS provides a direct probe of vortex order and reveals the order–disorder transition B_dis(T) in our sample. It is mediated only by the weak underlying disorder, lying deep in the mixed state, far from the regime dominated by thermal fluctuations. A jump in j_c around B_dis, as might be expected following the theory of Larkin and Ovchinnikov², cannot be detected in our j_c data derived from magnetometry (Fig. 3b). In contrast, these data show a nascent peak effect at high temperatures and fields, where thermal fluctuations become apparent.

Methods

Laboratory characterisation

Bulk magnetic measurements were carried out using a high-field cryogen free measurement system (CFMS) at the DTU Risø Campus. The field was applied parallel to the [111] crystal axis. We used the Goodman-Gor’kov relations⁶⁹ to compare the upper critical field B_c2(T) and superconducting critical temperature T_c of our sample to the values reported by ref.³² for varying sample purity. We calculate a mean free path l = 48 nm, impurity parameter α = 0.84, superconducting coherence length ξ₀ = 26 nm and London penetration depth λ(0) = 35 nm implying κ = 1.3 for our sample.

Neutron scattering

SANS experiments were performed on three instruments: D22 at the Institut Laue-Langevin (Fig. 4), NG7 at the NIST Center for Neutron Research (Fig. 6) and, SANS-II at the Swiss Spallation Neutron Source (Figs. 5, 7 and 8). In a typical setup on D22, neutrons of wavelength λ = 0.9 nm with spread Δλ/λ = 0.1 were collimated over a 18 m distance, providing a beam of angular spread a = 0.077°. Scattered neutrons were detected using a 2D multidetector placed 18 m behind the sample (Fig. 2). NG7 and D22 are at reactor sources where the neutron flux at the sample position is constant over experiment time scales. SANS-II is at a continuous spallation source with varying neutron flux, so in these experiments multidetector count rates are normalised with a monitor detector situated upstream of the sample. Rather than plotting detector counts/monitor counts, in Fig. 5a and in Fig. 7e the ordinates are scaled to a typical monitor value that was used for measurements containing low or zero vortex signal. When the spallation source is stable, this corresponds to a measurement time of 5 min per rocking angle.

The [111] axis of our vanadium crystal was aligned to within 0.2° of the applied magnetic field direction. Unless otherwise noted, vortex ensembles were prepared by cooling in the desired magnetic field from the normal state, i.e. field-cooled. Any misalignment between the applied field and the vortex directions due to crystalline anisotropy would have been much smaller than the observed peak widths. In niobium, which has a larger crystalline anisotropy than vanadium, 0.2° of misalignment from [111] would distort the vortex direction by 0.007°⁷⁰. The edges of the sample were masked from the incident neutrons in view of possible demagnetisation effects.

Two experimental geometries were used in our SANS measurements: (a) the magnetic field was applied roughly parallel to the incident neutron beam (Fig. 2a); (b) the field was applied perpendicular to the beam (Fig. 2b). The parallel geometry was used with a = 0.077° on D22 to survey the field dependence (Fig. 4). This geometry also confirmed the absence of transitions in vortex lattice shape away from the hexagonal symmetry expected for fields along [111]. The perpendicular geometry was used on NG7 with vertical applied field (Fig. 2b) for the temperature dependence (Fig. 6). It was also used on SANS-II with horizontal applied field to further map the phase diagram (Fig. 5) and to collect rocking curves with high resolution (a = 0.035°) (Fig. 7) suitable for educing in-plane correlations by reverse Monte Carlo refinement. To describe the field dependences of the intensity I integrated over the rocking curve (Figs. 4b and 5c), we used the form factor h calculated for a hexagonal vortex lattice⁴⁸.

As an initial approximation, the instrument resolution and mosaic spread of the vortex ensemble may be modelled as Gaussian distributions. All widths quoted in this manuscript are full-width half maxima. In either experiment geometry, the measured rocking curve width W_ω (Fig. 4a) is given by⁷¹

$$W_{\omega }^2=a^2+c^2+{\sigma }^2$$ 5

where c is the combined width parallel to the scattering vector: $c^2\mathrm{∕}{\theta }_{\mathrm{B}}^2={\left(\mathrm{\Delta }\lambda \mathrm{∕}\lambda \right)}^2+{\gamma }^2$ with θ_B the Bragg angle and γ the mosaic spread of the vortex ensemble parallel to the reciprocal lattice vector g. In the parallel geometry, σ probes correlations along the vortices. In the perpendicular geometry, σ probes the direction perpendicular to both the field and to g. The scattering angles are small, e.g. θ_B = 0.2° for 0.9 nm neutrons when B = 0.1 T, so c is small in Eq. (5) and the angular spread a of the incoming beam sets the minimum measurable width of the rocking curve.

The radial width W_r of the spot on the 2D multidetector measured at the peak of the rocking curve (Fig. 2a) is given by

$$W_{\mathrm{r}}^2=\frac{a^2{c}^2+a^2{\sigma }^2+4{\sigma }^2{c}^2}{a^2+c^2+{\sigma }^2}$$ 6

Equations (5) and (6) may be solved simultaneously to find the unknowns σ and c, and therefore γ. However, due to the smallness of θ_B, it is difficult to quantify γ without significant uncertainty. As an alternative, in Fig. 4d we calculate W_r assuming γ = 0 and with σ determined from W_ω. The W_r values calculated reproduce the measurements for fields up to 0.27 T at 1.6 K (Fig. 4d), indicating that $\gamma \ll \mathrm{\Delta }\lambda \mathrm{∕}\lambda =0.1$ in this field range. At higher fields the measured values diverge from the γ = 0 line. This indicates that γ must increase to the order of Δλ/λ = 0.1, i.e. the autocorrelation length parallel to g shrinks to ≈ 3a₀.

Reverse Monte Carlo refinement

In reverse Monte Carlo (RMC) refinement, the in-plane positions of vortices are simulated on a computer. These positions are recursively modified using a Monte Carlo procedure with cost function ${\chi }^2=\sum {\left(R_{\mathrm{sim}}\left(\omega \right)-R_{\exp }\left(\omega \right)\right)}^2\mathrm{∕}{\delta }_{\exp }{\left(\omega \right)}^2$ where R_exp(ω) is the experimentally determined angular dependence of the rocking curve measured in the perpendicular geometry, with corresponding uncertainty δ_exp(ω). Simulated rocking curves R_sim(ω) are calculated by convolving the elastic structure factor with the experimental resolution before scaling by a factor F, chosen to minimise χ². Simulated vortex ensembles are chosen to be sufficiently large that finite size effects are insignificant compared to the experimental R_exp(ω) widths, varying from 40,000 vortices used for the 0.17 T, 2.7 K dataset (intrinsic simulation width w_s = 0.29°) to 220,000 vortices used for the 0.13 T, 1.6 K dataset (w_s = 0.12°). Our implementation here extends that used previously for niobium¹⁹ by incorporating a simulated annealing procedure to assure a global minimum in χ² and to speed up the refinement. Once the minimum in χ² is reached, in-plane vortex-vortex correlation functions are calculated directly from the simulated ensemble. The simulated ensemble is not unique. For example, it is possible that the measured rocking curve at 0.17 T, 2.7 K (Fig. 7d) could also be reproduced by an ensemble containing large ($\gg 30a_0$) domains in which the Bragg glass regime persists, but with average bond angles of neighbouring domains differing to reproduce the observed 3.5° wide distribution in ω. This scenario, for which we note that fracturing of the vortex lattice still occurs, is not accessible in our RMC refinements as the exceptionally large ensemble sizes required lie beyond the computational resources currently available. Similarly, the extra degree of freedom realised by F leads to a gradual decay of correlations with increasing simulation time even after χ² is minimised¹⁹, so many different vortex ensembles are generated in each RMC run that all reproduce the shape of the experimental rocking curve. These ensembles are observed to yield the same form for each correlation function¹⁹, though the ordinate scale should not be taken too seriously. The ensembles obtained here are all found to be essentially free of dislocations: there is no need to impose minimum nearest neighbour distances or planarity constraints¹⁹.

Data availability

The data that support the findings of this study are available from the corresponding author upon request.

Author contributions

M.L. provided the sample. Magnetisation data were measured by A.B. and R.T.-P. and analysed by R.T.-P. and M.L. SANS experiments were conducted by M.L. at NG7, S.B., R.T.-P. and M.L. at SANS-II, and L.P. and M.L. at D22. R.T.-P. analysed the SANS data using reverse Monte Carlo refinement, with remaining SANS analysis performed by M.L. The manuscript was written by M.L. with assistance from R.T.-P. and contributions from all authors.

Competing interests

The authors declare no competing interests.