String model for the dynamics of glass-forming liquids

Abstract

We test the applicability of a living polymerization theory to describe cooperative string-like particle rearrangement clusters (strings) observed in simulations of a coarse-grained polymer melt. The theory quantitatively describes the interrelation between the average string length L, configurational entropy S_conf, and the order parameter for string assembly Φ without free parameters. Combining this theory with the Adam-Gibbs model allows us to predict the relaxation time τ in a lower temperature T range than accessible by current simulations. In particular, the combined theories suggest a return to Arrhenius behavior near T_g and a low T residual entropy, thus avoiding a Kauzmann “entropy crisis.”

I. INTRODUCTION

While an understanding of the fundamental factors controlling the properties of glass-forming (GF) liquids has continually improved, there is still no generally accepted theory of glass formation. In addition to the familiar phenomenology relating to the macroscopic properties of GF liquids, such as the dramatic and nearly universal temperature T dependence of diffusion coefficient and fluid viscosity,¹ experimental and computational studies have shown a tendency for particle clusters of excessively high and low mobility to grow upon cooling toward the glass-transition temperature T_g, a phenomenon termed “dynamical heterogeneity.”^2–5 The related notion of “cooperatively rearranging regions” (CRR) forms the foundation of the Adam and Gibbs (AG) theory of relaxation in glass-forming liquids⁶—a model frequently shown to describe the T dependence of relaxation in both experimental^7,8 and computational studies of GF systems.^9–16 Specifically, AG argued that the growth of the activation free energy for molecular rearrangement is proportional to the CRR size (defined by the number of units in these clusters rather than their length scale). A similar notion of dynamic clusters of cooperatively rearranging particles is central to the Random First-Order Transition (RFOT) theory¹⁷ (where the emphasis is given to the CRR length scale rather than their mass).

Although, the AG and RFOT theories offer no precise molecular definition of these CRR, many authors have speculated on the connection to dynamical heterogeneity, and several recent studies have demonstrated that the growth of the size of cooperative motion in cooled liquids, in the form of string-like particle rearrangements, parallels the growth of the activation free energy ΔG. Consequently, it was proposed that the “strings” may be a concrete realization of the CRR.^18–21 The proportionality between the average string length L and ΔG(T) was found to hold even when the fragility of the GF fluid was varied over a broad range in a model polymer-nanoparticle composite.^19,21 Additionally, this relation between ΔG and L can account for the dynamical changes in supported polymer films having variable thickness, substrate roughness, stiffness, and polymer-substrate interaction strength.²² Given this relation between string length and the activation barrier, in this work we aim to better understand these fluid thermal excitations from a theoretical perspective. While identifying CRR with string-like motion in GF fluids provides a concrete picture of the nature of cooperative motion, it does not explain the origin and geometrical nature of these structures, nor their growth upon approaching T_g, which is their main significance in relation to relaxation. The present work develops a statistical mechanical model for the strings based on the framework of treating them as “initiated equilibrium polymers,” and validates the applicability of the model through comparison to recently published molecular simulations.

II. CHARACTERISTICS OF STRING-LIKE PARTICLE REARRANGEMENT

As a prelude to formal comparisons of molecular simulations with theory, it is valuable to first summarize the key features of cooperatively replacing particles. Most of this information is available in Ref. 20, but it is helpful to review and expand on essential aspects that form the basis of our equilibrium polymerization description. To characterize these “strings,” we use the simulation results from Ref. 20, which studied a dense melt of “bead-spring” polymers where each chain consisted of 20 Lennard-Jones (LJ) monomers connected by anharmonic springs.²³ All values we report are in standard reduced LJ units, which can be mapped to physical units relevant to real polymer materials, where the size of a chain segments is typically about 1 nm to 2 nm, time is measured in ps, and non-bonded bead interactions have a strength of ≈1 kJ/mol for a typical polymer like polystyrene with T_g ≈ 100 °C. Clusters of highly mobile replacing particles (strings) were identified following the procedures originally developed in Refs. 24 and 25.

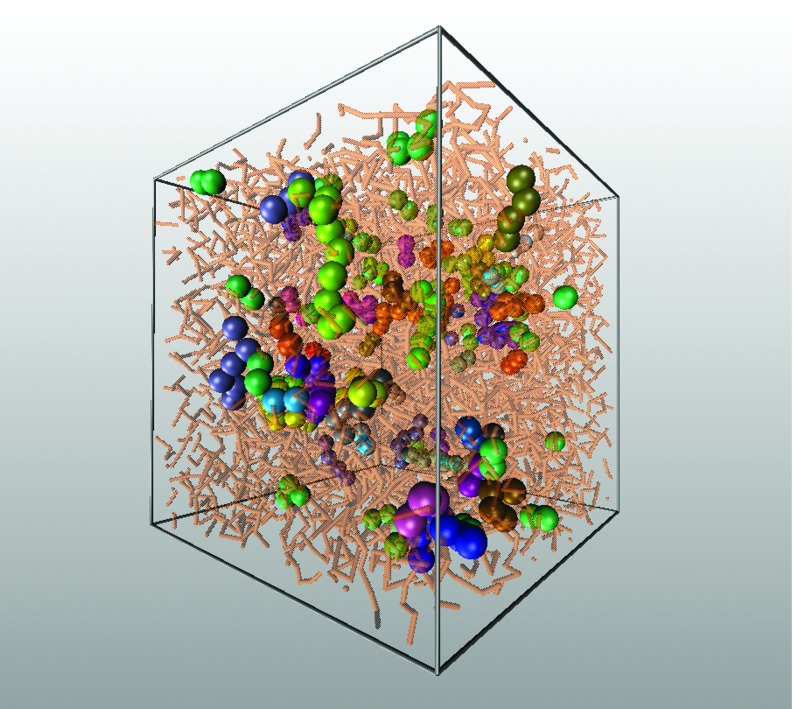
To clarify the physical nature of the problem and motivate the model for string-like correlated motion, it is helpful to visualize the cooperatively replacing monomers. Figure 1 shows a representative “snapshot” of these replacing mobile particles, which clearly indicates their string-like connectivity. The slender segments in this figure correspond to polymer backbone bonds and the spheres indicate the string-like coordinated particle rearrangement events. The strings shown in Fig. 1 are equilibrium structures, and their polymeric form (defined simply as a chain of repeating units) is evident. However, we emphasize that the string-like connectivity refers to the coordinated motion of polymer segments, rather than to the polymer chains having fixed covalent linkages, and thus should not be confused with chain reptation. This type of collective motion is seen in many glass-forming fluids, and so we expect the phenomenon to be a general feature of structural glass-forming liquids.

FIG. 1.

Visualization of string-like segmental cooperative motion in a model glass-forming polymer melt. The monomers that move cooperatively are indicated by the large spheres. Different groups are indicated with different colors. All other polymer segments are shown as translucent thin cylinders.

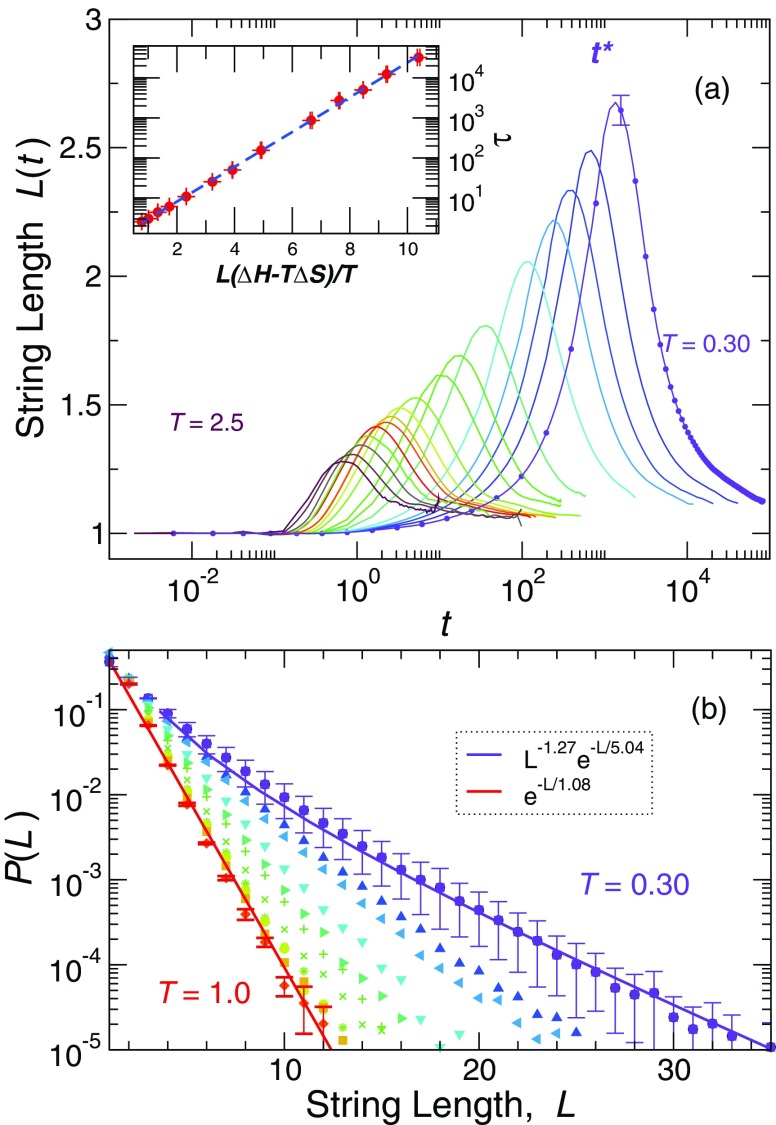
Since the “strings” are comprised of highly mobile particles, the identity of which vary depending on the time interval t considered, the strings are themselves highly dynamic objects. This inherently dynamic nature is illustrated in Fig. 2(a), which shows the average L(t) over the entire T range studied in the simulations. As discussed in earlier works,^{19,20,24–27} L(t) is small for very short (ballistic) and very long (diffusive) time intervals, when mobility correlations are weak; at intermediate time, L(t) has a peak, defining the characteristic size L, at a time that defines the characteristic string “lifetime.” The characteristic L grows on cooling towards T_g, as demanded if these objects are identified with the CRR of the AG model. It should be appreciated that this characteristic size (mass) also has an accompanying characteristic spatial extent, the average radius of gyration of these polymeric structures. This string lifetime is approximately proportional to t*, the peaking time of the much studied non-Gaussian parameter. Previous work has shown that the lifetime of the strings corresponds to a diffusive relaxation time,²⁰ so this relaxation time is intermediate between the high frequency beta relaxation regime and the timescale of alpha structural relaxation. Although the strings are related to a diffusive molecular motion, they are linked to α relaxation at longer times through a power law decoupling relation, which makes the strings relevant to understanding changes of the activation energies governing both diffusion and structural relaxation.¹⁹

FIG. 2.

(a) Average string length L(t) as a function of interval t for many T approaching T_g. The color gradient indicates T, from violet at T = 0.30 to maroon at T = 2.5. The error bars indicate the statistical uncertainty obtained from four independent runs. The inset shows the α-relaxation time τ can be described by the AG relation (Eq. (4)), where the size of the CRR, z, is exactly identified with string length L. (b) String length distribution P(L) at the characteristic peak time t* of string size. At high T, P(L) has a nearly exponential form (red line for T = 1.0), a canonical property of the linear equilibrium polymers in mean field theory. At low T, there is curvature in P(L) that can be approximately captured by a power-law dependence for small L (violet line at T = 0.30), suggesting a possible change in string topology, as discussed in the text.

In addition to mean string size, it is instructive to examine the distribution of string sizes P(L) at the characteristic time where L(t) is largest. If strings can be described as equilibrium polymers, we expect P(L) to exhibit a nearly exponential mass dependence.²⁸ Consistent with this expectation, Fig. 2(b) shows that the mass distribution of the strings follows an approximately exponential variation, as noted in previous studies.^20,24 However, at low T, modest curvature is evident in P(L) in Fig. 2(b). The deviations from exponential behavior at small L can be reasonably described by a power-law variation, so that

$$P\left(L\right)\sim L^{-\theta }{e}^{-L/L_0}.$$ (1)

This type of power law times an exponential scaling for the distribution of replacing particles has recently been seen in simulations of lipid membranes,²⁹ where θ has a similar value. If such a form is used for all T, we find the exponent θ increases on cooling toward T_g. Interestingly, an exponent value of θ = 2.5 is consistent with ideal ring equilibrium polymers. Consequently, the increase of θ may reflect a progressive topological transition between open strings at high T, to predominantly ring-like structures at low T, as seen in crystal melting³⁰ and the polymerization of dipolar fluids.³¹ Regardless of whether such a scenario occurs here, it is apparent that the exponential tail is the predominate feature of our data for P(L), a characteristic of equilibrium polymers.

We can further characterize the structure of these strings by their radius of gyration R_g and its relation to string size,

$$R_g\sim L^{\nu },$$ (2)

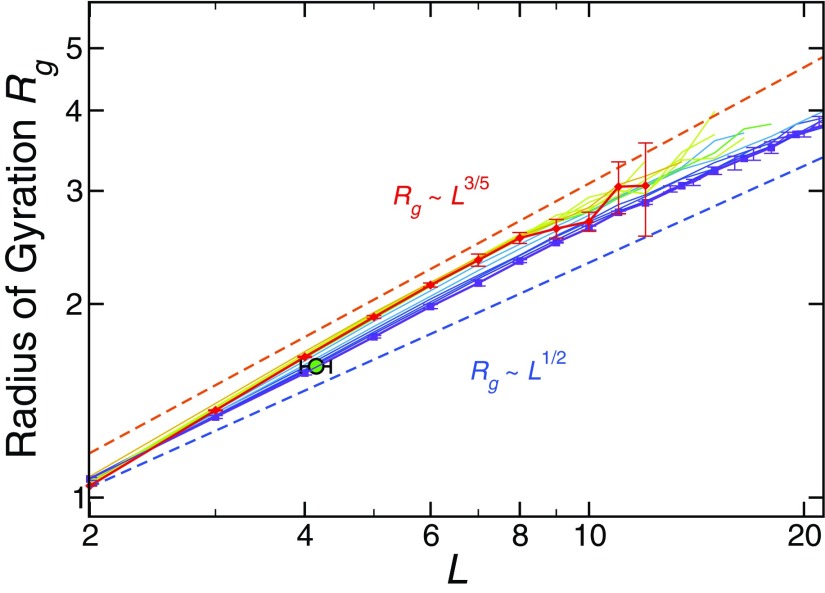
where d_f = 1/ν defines the fractal dimension of the strings. For short strings, excluded volume interactions dominate, so strings can be expected to behave like self-avoiding walks (SAW) with ν = 3/5. Figure 3 shows that, indeed, such behavior holds, but that ν approaches 1/2 for longer strings, which occur primarily at low T. This change can be understood by the proposed analogy to equilibrium polymers. Specifically, the longer the chains grow, the stronger their interchain interactions become, resulting in a greater screening of their excluded volume interactions, just as in ordinary flexible linear equilibrium polymers.²⁸ This screening causes strings to become progressively more like simple random walks (RW), which have ν = 1/2, consistent with the observed behavior.

FIG. 3.

Dependence of the radius of gyration R_g(L) on the string length, defining the mass scaling exponent ν. The color gradient goes from blue at the lowest T = 0.30 to red at T = 1.0. The dashed lines indicate the limiting scaling behaviors of the SAW (ν = 3/5) and RW (ν = 1/2). The error bars indicate the statistical uncertainty obtained from four independent runs. The filled green circle indicates the mean estimated value of ⟨R_g⟩ in the low T limit corresponding to the predicted low T mean L ≈ 4.1 from the string-polymerization model (discussed below).

III. STRINGS AS COOPERATIVELY REARRANGING REGIONS

A convenient way to parameterize the rapid growth of relaxation time τ approaching T_g is through the effective activation free energy ΔG(T). Assuming that relaxation can be described as a thermally activated process, ΔG(T) can be defined by

$$\Delta G\left(T\right)\equiv k_{B}T\ln \left(\tau /{\tau }_0\right),$$ (3)

where τ₀ is a vibrational relaxation time, approximated as τ₀ ≈ 10⁻¹³ s (or τ₀ ≈ 0.1 in our reduced units) for small molecule fluids. Above T_A, τ has simple Arrhenius dependence,³² and the activation free energy ΔG(T > T_A) ≡ Δμ. As T_g is approached, the activation free energy ΔG grows, but the magnitude of this change is modest. Figure 4 illustrates ΔG(T)/Δμ for a number of different GF liquids, where Tri–α–Naphthyl Benzene, TαNB, shows the largest change ΔG(T_g)/Δμ ≈ 4 at T_g in this collection of GF liquids. The data in this figure are well-known in the glass literature, but, surprisingly, we could not find this data presented in a way that directly indicates the variation of activation free energy. It is striking how small the variation in ΔG(T_g)/Δμ needs to be to account the variable relaxation time and fragility of glass-forming liquids.

FIG. 4.

Temperature dependence of the experimental relative activation energy ΔG(T)/Δμ for different glass-forming liquids. The filled symbols with a darker color indicates their values at T_g. Data were obtained from multiple resources: (1) o–terphenyl Fig. 2 of Ref. 33, (2) m–toluidine from Fig. 8 of Ref. 34, (3) salol from Fig. 3 of Ref. 35, (4) glycerol from Fig. 4 of Ref. 36, (5) GeO₂ from Fig. 2 of Ref. 33, (6) toluene from Fig. 4 of Ref. 37, (7) propylene carbonate from Fig. 4 of Ref. 38, (8) $\mathrm{T}\alpha \mathrm{NB}$ Fig. 15 of Ref. 39, (9) B₂O₃ from Table 2 of Ref. 40. Note that, while these data are well known, it is the first time that this form of data reduction has been considered. See original references cited above for a discussion of experimental uncertainties for the data shown in this figure.

The approach developed by Adam and Gibbs⁶ rationalizes the growth of the activation free energy by hypothesizing that ΔG is extensive in the size z of CRR, so that ΔG = zΔμ. Thus, under the assumption of activated dynamics,

$$\begin{array}{c}\hfill \tau ={\tau }_0\exp [z\Delta \mu /k_{B}T].\end{array}$$ (4)

If z approaches unity at high T, the limiting Gibbs free energy of activation ΔG = Δμ. In their original work, AG assumed (without explanation) that the entropic contribution ΔS_a to Δμ ≡ ΔH_a − TΔS_a could be neglected, so that Δμ ≈ ΔH_a. When z is constant (expected at high T), this assumption can be justified by a simple redefinition of τ₀ to incorporate ΔS_a; however, the validity of the assumption is questionable if z varies with T. Note that the Arrhenius activation enthalpy ΔH_a changes with polymer molecular mass by an amount comparable to ΔG(T)/Δμ over the wide temperature range between T_A and T_g,⁴¹ and, likewise, ΔS_a also varies rather strongly with polymer mass.⁴² Indeed, in recent work we found that it is inappropriate to neglect the entropic contribution to the activation free energy.²¹ Similarly, in hard-sphere liquids, where the AG relation has been confirmed computationally, Δμ is purely entropic, so that Eq. (4) takes the limiting form,

$$\begin{array}{c}\hfill \tau ={\tau }_0\exp [z\Delta S_a/k_B],\end{array}$$ (5)

and such relation was observed in early measurements relating fluid excess entropy and τ.^43,44 Truskett and co-workers⁴⁵ also considered the possibility that the entropy term is the primary contributor to dynamics for dense fluids, since repulsive particle interactions dominate in this regime. Likewise, Dyre and co-workers argued that ΔG is entropic for fluids composed of soft particles having a predominantly repulsive interactions.^46,47 However, an entropy dominated scenario is necessarily limited in validity, since the Arrhenius T dependence of transport properties demonstrates a role for enthalpic contributions. Evidently, an understanding of both ΔH_a and ΔS_a has significance for understanding the dynamics of fluids.

A basic shortcoming of the AG approach is that it provides no molecular definition for CRR size z, but recent works^19–21 provide evidence that the average string length is proportional to z. In parallel with the growth of ΔG(T), the scale of the collective string-like collective motion grows upon cooling with comparable magnitude. More specifically, for the polymer melt simulation data we analyze here, the inset of Fig. 2(a) shows that the α relaxation time τ, the decay time of the coherent density-density correlation function, is precisely described by the AG expression, Eq. (4), where z is replaced by L. A subtle but important consequence is that, at high T, L approaches a value L_A that is slightly larger than one, a point we shall expand on later.

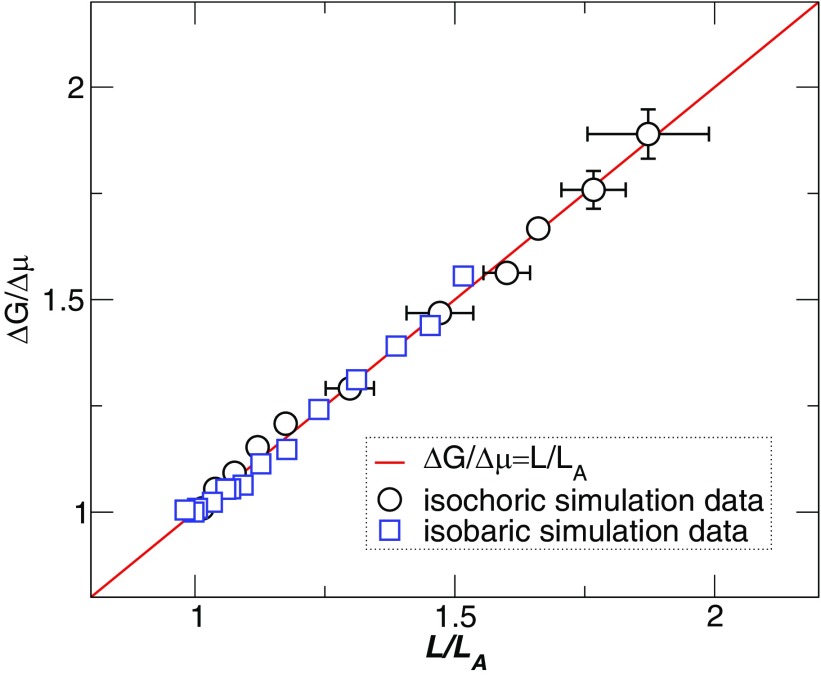
The string-like clusters undergo cooperative particle rearrangement (in contrast to, e.g., mobile particle clusters, where motion need not be cooperative), and so these are natural objects to identify with the CRR of AG.^19,21 We can further test the consistency of this identification without any free parameters, by directly comparing the computed ΔG/Δμ (using τ from Refs. 20 and 21 in Eq. (3)) with the independently observed L values. If our identification of L/L_A and z is correct, then these quantitates must be identical. For generality, we make this comparison in Fig. 5 for simulation data along a constant density path,²⁰ as well as constant pressure path,²¹ where the temperature dependence of τ is quite different, as discussed below. The agreement between L/L_A and ΔG/Δμ is striking, and supports the direct identification of the strings with the CRR. Within AG, the reduced activation energy ΔG/Δμ exactly quantifies the extent of cooperative motion L/L_A = z and ∂L/∂T quantifies the fragility of glass formation in systems for which Δμ ∝ T_g¹⁹ (This relation between Δμ and T_g is observed in the current model to a good approximation,^19,21 and is commonly observed as an approximation in real glass-forming liquids).

FIG. 5.

Parameter free comparison between L/L_A and the expected size of CRR from AG. The circles represent simulation data along a constant density path (using relaxation time data from Ref. 20 in Eq. (3)), and squares are data along constant pressure path (data from Ref. 21). The error bars indicate the statistical uncertainty obtained from four independent runs.

IV. THE STRING MODEL FOR GLASS FORMATION

A. Equilibrium polymerization models

Given the qualitative resemblance of the strings (illustrated in Fig. 1) to the supramolecular assembly of synthetic and biological molecules,⁴⁸ we wish to consider if the behavior strings can be quantitatively described by an equilibrium polymerization model. Since the strings appear to describe the CRR of the AG theory, such a polymerization description would provide a theoretical basis for the variation of CRR size, and thus the relaxation time approaching the glass transition.

Equilibrium polymerization, the formation of particle and molecular clusters that form and disintegrate in a dynamic equilibrium, is often accompanied by constraints on the association dynamics. For example, there may be initiation and inhibition by distinct chemical species, as in living polymerization of poly-alpha methyl styrene with sodium napthalide as the initiating chemical species, or thermal activation, as in the equilibrium polymerization of sulfur, where the breaking open of the S₈ ring monomer plays the role of initiator.⁴⁸ There can also be topological constraints limiting the polymerization,⁴⁹ such as ring formation, branching and geometrical confinement that can alter the thermodynamics of the polymerizing fluids in significant ways. In short, one must be careful to select the equilibrium polymerization model most appropriate for the problem at hand.

One of the simplest models of equilibrium polymerization is termed the “isodesmic model” or “freely-associating (FA) model,” where no constraints on the polymerization process are operative—hence the term “freely-associating.”⁵⁰ In this case, we have the simple association scheme that particle or atom A associates and dissociates with a well-defined equilibrium constant, dimers can associate with another A to form a trimer with the same equilibrium constant, and so on. In the FA model, there is no limit on the size of the associating group, or on the breakup of these clusters from the interior of the clusters to form smaller chains. Although this model is rather idealized, it has been popular in modeling the formation of worm-like micelles,⁵¹ magnetic chain formation of dipolar particles,³¹ protein fiber formation,^52–55 DNA origami tiles,⁵⁶ etc.

A well-known result of the FA model is that the average length L of the dynamic polymer chains at fixed initial concentration of the associating species grows on cooling in a simple Arrhenius fashion,

$$L\simeq L_0\exp \left(\frac{\Delta H_p}{2k_{B}T}\right),$$ (6)

where L₀ is a constant determined by molecular parameters of the polymerization model and ΔH_p is the enthalpy of polymerization. In the FA model, exact calculations dictate L = 1 at high temperatures, corresponding to non-existence of polymeric structures.^57,58 If we formally identify the string size L with the CRR of AG and adopt the FA model to describe the string formation thermodynamics, Eqs. (4) and (6) imply a “double-exponential” T dependence of relaxation,

$$\tau ={\tau }_0\exp \left[\Delta G_o\exp \left(\frac{\Delta H_p}{2k_{B}T}\right)\right].$$ (7)

Indeed, such a relation qualitatively captures the very rapid change of relaxation on cooling toward T_g. In fact, it has been recently noted that Eq. (7) describes the temperature dependence of relaxation times of diverse glass-forming liquids,⁵⁹ which we can take as an encouraging sign for modeling strings as equilibrium polymers.

However, our goal is to go beyond curve-fitting relaxation data, and a closer examination of the string data (Fig. 2) reveals a significant problem with applying the FA model (and consequently Eq. (7)); namely, the average polymerization index L does not approach 1 at high T, which is demanded in the FA model. Instead, L takes a somewhat larger value, L(T_A) ≡ L_A ≈ 1.4 (see Fig. 2). The intuitive assumption that cooperative motion is completely absent at high T would lead us to expect, as suggested by AG, that the extent L → 1 at high T, but apparently, some cooperativity remains at elevated temperatures.⁶⁰ Since the string sizes are exponentially distributed (Fig. 2(b)), a mean size of 1.4 implies that there much larger strings in the system. This may seem like a small effect, but a change in the mean string length by 0.4 implies an appreciable change in the dynamics of the fluid. Of the many models of equilibrium polymerization, we know only one analytically tractable model that has the property that the average degree of polymerization L does not approach unity upon heating^28,61 In this “living polymerization model,” a distinct species having a fixed concentration serves to initiate the polymerization process. The thermally activated polymerization theory is a plausible model for glass-forming liquids, and there is precedent for inferring thermally activated excitations that influence molecular transport through initiation of collective molecular motion. For example, unstable five-coordinated molecules in water have been shown to “catalyze” molecular motion in water.^62,63 Granato and co-workers have similarly argued that relatively high density interstitial excitations exist in liquids and glasses,⁶⁴ and they rationalized many of the low temperature properties of condensed disordered materials based on this model.⁶⁵ Keys et al.⁶⁶ have identified thermally activated elementary excitations that seem to be associated with string-like collective motion. In future work, we hope to find a quantitative relation between these high frequency excitations and those studied in the present work. The physical nature of activating molecules also requires an in depth investigation.

Based on these considerations, we next test the extent to which the strings in our glass-forming liquid can be quantitatively described by living polymerization. In the comparison of the model to our molecular simulations, we consider multiple thermodynamic properties to ensure self-consistently. After establishing this consistency, we then examine the implications of our polymerization model for predicting the properties of our glass-forming liquid outside the temperature range in which equilibrium simulation is possible. We find that this model makes valuable predictions about the nature of the glass state and the general nature of the glass transition.

B. Living polymerization model of strings

In order to explain the T dependence of the strings, and thus of ΔG, we now consider the theoretical implications of the hypothesis that these strings can be considered as dynamic polymers that form and disintegrate in equilibrium.

We consider a model for initiated equilibrium polymerization by Dudowicz et al.,²⁸ which assumes that chain formation is governed by two simple reactions:

$$\begin{array}{cc}& 2M+2I\to M_2{I}_2,\hfill \end{array}$$ (8)

$$\begin{array}{cc}& M_2{I}_2+M\rightleftharpoons M_{2+i}{I}_{2}i=2,3,...,\infty ,\hfill \end{array}$$ (9)

where reaction of the monomer species M, mobile particles in the context of glass-forming liquids, requires an energetically excited initiating species I. Such energetically activated particles are exemplified in water by the occurrence of local high density configurations which catalyze molecular movement.^62,63,67 The strings are dynamic polymers of these highly mobile monomers that have a temperature dependent average length, or polymerization index, L ≡ ⟨L⟩. The dynamics polymer should not be confused with the covalently bonded polymer chains. The fraction of linked mobile particles Φ serves as the order parameter for the string self-assembly process, and L and Φ of living polymer solutions are related by

$$L=\frac{1}{1-\Phi +r/2},$$ (10)

where r is the ratio of the initiator to the monomer volume fraction ϕ₀. Φ is limited to the range r ⩽ Φ ⩽ 1. Consequently, L has an upper bound determined by r, a fact that has important implications for glass formation at low T. Note that the high temperature limit of L (where Φ ≈ r) from Eq. (10) is larger than 1, consistent with our MD simulations observations on GF liquids.

To test the applicability of this model of string formation for GF fluids, we need to map observable quantities from the simulation to the input variables of the theory. For initiated, or “living” polymerization, r ≈ Φ for T above the “onset” temperature in the theory (see Fig. 7 of Ref. 28). Similarly, we know that string-like motion in GF liquids is only prevalent below an onset temperature T_A where relaxation becomes non-Arrhenius and caging becomes conspicuous.^32,68 Thus, we restrict the application of the polymerization model to T ≲ T_A. Identifying the onset condition in the simulation and in living polymers, we have L(T_A) ≡ L_A. The approximations r ≈ Φ_A and Eq. (10) give rise to the useful closed analytic form,

$$L\approx \frac{L_A\left(1-\frac{{\Phi }_A}{2}\right)}{1-\Phi +\frac{{\Phi }_A}{2}},T\le T_A,$$ (11)

expressed in terms of observable properties, L_A, Φ_A, Φ. In the living polymerization model, L_A and Φ_A are not independent, but we take these as independent observables in our application of Eq. (11) because of the approximation Φ_A ≈ r. Equations (10) and (11) imply that L saturates to a constant value at low T, a point extensively discussed below. In living polymer solutions, the magnitude of r (and thus Φ_A by analogy) links the string mass at high and low T and also governs the cooperativity of the polymerization transition,⁶⁹ as measured by the rate of change of L(T), and the magnitude of the change in the specific heat; similar definitions of cooperativity have been applied to GF liquids.^19,70

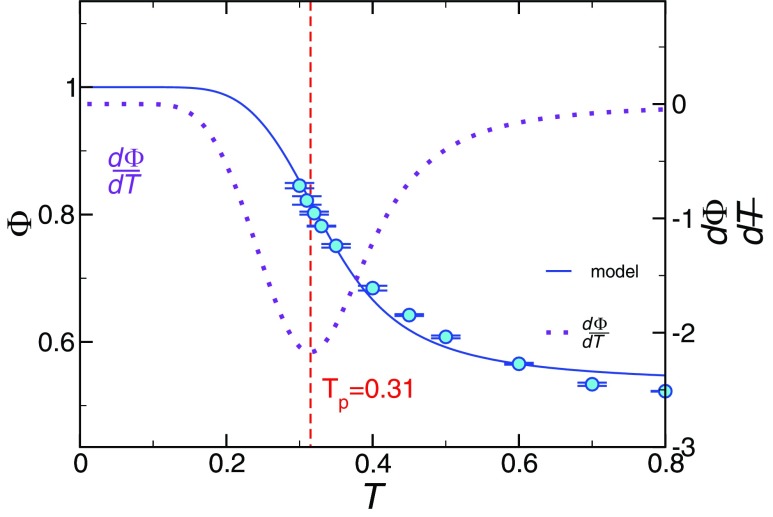
In the living polymerization model, the order parameter Φ is the extent of polymerization, defined by the fraction of monomers forming polymeric structures. In our case, this is the fraction of those highly mobile monomers participating in strings, and Fig. 6 shows the temperature dependance of Φ(T). We find that the variation of the behavior of Φ(T) is well described by the polymerization model prediction,²⁸

$$\Phi =1-\varphi \left(T\right)/{\varphi }_0,$$ (12)

where ϕ(T) is the fraction of monomers, given by²⁸

$$\begin{array}{ccc}\hfill \varphi \left(T\right)& =& \frac{1}{2}{e}^{\frac{\Delta G_p}{k_{B}T}}\left[1+{\varphi }_0\left(1-\frac{{\Phi }_A}{2}\right)e^{-\frac{\Delta G_p}{k_{B}T}}\right.\hfill \\ & & \left.-\sqrt{{\left(1+{\varphi }_0\left(1-\frac{{\Phi }_A}{2}\right)e^{-\frac{\Delta G_p}{k_{B}T}}\right)}^2-4{\varphi }_0(1-{\Phi }_A)e^{-\frac{\Delta G_p}{k_{B}T}}}\right].\hfill \end{array}$$ (13)

The free energy ΔG_p = H_p − TΔS_p describes the thermodynamics of polymerization (string formation) where ΔH_p and ΔS_p are the enthalpy and entropy of chain assembly, respectively. Note that the free energy of polymerization ΔG_p should not be confused with the system activation free energy Δμ (and similarly for enthalpy and entropy). Accordingly, the association into a strings is governed by an equilibrium constant k = exp [ΔG_p/k_BT]. The volume fraction of mobile particles ϕ₀ = f₀(π/6) = 0.034, using a mobile particle fraction f₀ = 0.065, determined in earlier work.⁷¹

FIG. 6.

Temperature dependance of the extent of polymerization Φ(T). The symbols represent the simulation data. The solid curve represents the model prediction for Φ. The dashed curve illustrates the numerical derivative of Φ(T). The minimum of $\frac{\partial \Phi }{\partial T}$ defines the polymerization transition location T_p, indicated by the vertical line. The error bars indicate the statistical uncertainty obtained from four independent runs.

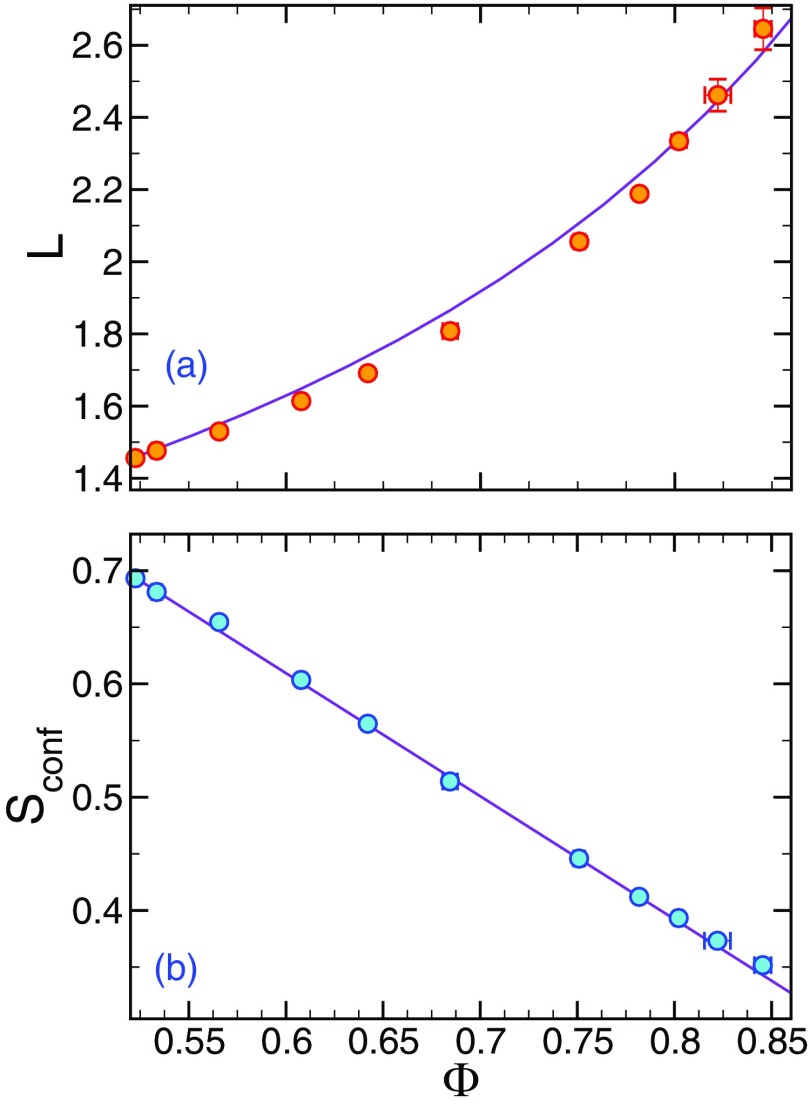
We next test the validity of the relation between L and Φ (Eq. (11)) in Fig. 7(a) using no fitting parameters, since L_A, Φ_A are taken directly from the numerical results. The figure shows agreement over the entire range of temperature investigated. We note that the Φ_A = 0.52, which may seem large at first glance. However, recall that this is the fraction of the relatively small subset of mobile particles so that the initiator volume fraction ϕ_I = 0.018 is quite small. Moreover, Ref. 30 found that the mobile particles associated with the “activated” species have a concentration that is comparable to the coordination number of the mobile particles. This rough argument makes the activated particles on the order of 50%, as we find here. In both the AG and living polymerization models, the activation energy is expected to vary inversely proportional to the configurational entropy S_conf.^28,70 Since L also varies inversely to Φ, we then expect S_conf ∝ (1 − Φ). Figure 4(b) confirms this expectation, providing a possible alternative means of estimating S_conf, a quantity highly cumbersome to compute in molecular simulations. Notably, there are also fundamental experimental difficulties in estimating S_conf. It is not possible to reliably experimentally estimate from specific heat measurements, as AG originally suggested, because S_conf can be quite small in comparison with the vibrational entropy in polymer fluids.²⁰

FIG. 7.

(a) Model prediction of the string mass L as a function of the fraction Φ of mobile-particles in the strings. Simulation results are given by the solid symbols, and the solid curve represents Eq. (11) with no free parameters. (b) Test of the living polymerization model relationship between the configurational entropy S_conf and Φ. We find excellent agreement with the predicted linear relation, S_conf ∝ (1 − ϕ). Note that, in the free association model of self-assembly,⁷⁰ S_conf ∝ (1 − Φ)^1/2, providing further evidence of the inadequacy of the FA model in describing glass formation.

Given the success of this initial parameter-free test on the string model for glass-formation, we next consider the implications of this theoretical description for the T dependence of L(T), and thus τ(T), both in the region where we have simulation results, and also at lower T, approaching T_g and below.

Using the living polymerization model prediction for the T dependence of Φ(T), we obtain an analytic expression for L(T) using Eq. (13) in Eq. (11) (which we do not list, for brevity). In addition, we may obtain a simpler expression by combining Eq. (11) and the high T expansion of Φ from Ref. 28, which yields the approximate high T expansion,

$$\begin{array}{c}\hfill L\approx L_A\left(1-\frac{{\Phi }_A}{2}\right)\left[1+\frac{{\Phi }_A}{2}\left(1+{\varphi }_0\exp \left[-\frac{\Delta G_p}{k_{B}T}\right]\right)\right],\end{array}$$ (14)

which exhibits the expected Arrhenius T dependence of L for equilibrium polymerization models at high T. We expect Eq. (14) to be valid in the range T_c≲ T ≲ T_A, where T_c is the crossover temperature frequently associated with the mode-coupling theory of supercooled liquid dynamics.⁷² In practice, T_c is obtained by empirically fitting τ to a power-law in T − T_c over a restricted T range and, in the present work, T_c = 0.35.²⁰ The polymerization temperature T_p indicated in Fig. 6 is defined by the temperature at which ∂Φ/∂T reaches its minimum, T_p = 0.31. Since T_p is an intermediate temperature, it is natural to consider its possible relation to T_c of glass formation. Ref. 70 argued that T_c should be identified with T_p in the polymerization model, and our T_c value is in reasonable accord with our estimate of T_p.

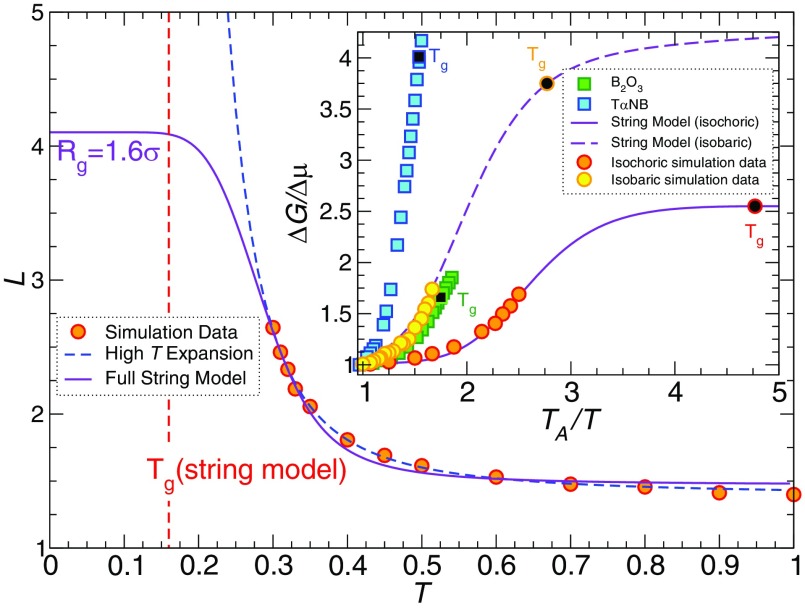
The T dependence of L predicted by the full theory, as well as the high T approximation are shown in Fig. 8, where ΔH_p = −1.55 and ΔS_p/k_B = −0.46. Both the complete expression and high T expansion can account for the T dependence of the available data for L, but show dramatically different behavior when we extend to lower T. The high T expansion predicts unbounded string growth (L → ∞), but the nature of the high T approximations make this estimate unreliable at low T. In contrast, the complete model (without approximation) predicts that L(T) reaches a plateau at low $T,L(T\to 0)=L_A(\frac{2}{{\Phi }_A}-1)\approx 4.1$, corresponding to a radius of gyration R_g = 1.6 σ ≈ 1.6 nm to 3.2 nm (see Fig. 3). This length scale is consistent with empirical CRR size estimates obtained from specific heat measurements in small-molecule liquids and estimates of the change in the activation energy in GF liquids.^40,73,74 This range of length scales also accords with the typical “cooperative motion scale” from neutron and Raman scattering, Boson peak, and NMR measurements.⁷⁵ In addition, the inset of Fig. 8 shows that the predicted low T limit of ΔG/Δμ = L/L_A from our model is compatible with the experimentally observed behavior of several glass-forming liquids.

FIG. 8.

Temperature dependance of the string length L(T). The lines in main panel represent the model predictions from the full theory (solid line) and the high T expansion (dashed line). The full theory reaches a plateau at low T, while the high T approximation yields a divergence. (Inset) The comparison of ΔG/Δμ for simulation data and ΔG/Δμ for the experimental values (which should equal L/L_A to our simulation data of our glass former model). The experimental data emphasize the similarity to our simulation data. We compare the equilibrium polymer model data both isobaric and isochoric simulation data to show that change does not alter the qualitative trend. Note the somewhat stronger T variation of the reduced activation energy ΔG/Δμ, and thus, fragility in the constant pressure simulations. McKenna and co-workers⁷⁶ have also found the T dependence of the structural relaxation measurements on polymeric glass-forming liquids to be weaker under constant density conditions.

Using the AG-inspired relation, ΔG(T) = (ΔH_a − TΔS_a)L(T)/L_A, we obtain our string model for τ(T),

$$\tau \left(T\right)={\tau }_0\exp [(\Delta H_a-T\Delta S_a)(L\left(T\right)/L_A)/k_{B}T],$$ (15)

to predict the the structural relaxation time at T lower than those accessible by equilibrium simulation (Fig. 9(a)). First, we note that using either the high T approximation or the full model for L accounts well for τ in the T range where simulation data are available. However, the behavior of the full and approximate model differ significantly at lower T. The predicted behavior for τ(T) can be compared with that obtained from the commonly used Volgel-Fulcher-Tammann (VFT) equation,^77–79

$${\tau }_{\alpha }={\tau }_0\exp \left[\frac{D{T}_0}{T-T_0}\right],$$ (16)

where, for the present data, T₀ = 0.20²⁰ is the extrapolated divergence temperature of τ. Interestingly, the fit using the VFT form is nearly indistinguishable from the extrapolation of the high T approximation to the string model. Both show a more rapid predicted growth of τ at low T than that of the full string model. One way to quantify this difference is in terms of T_g. Using the common laboratory definition of T_g as τ(T_g) ≈ 100s, and taking our reduced time unit as 1 ps, we find from the string model T_g = 0.16. Due to the more rapid variation of the high T approximation and the VFT fit, the estimated T_g(VFT) = 0.23 is larger than predicted from the full string model described in the present paper. This large discrepancy in T_g estimates is primarily a consequence of the fact that L approaches a constant at low T in the string model.

FIG. 9.

(a) Temperature dependance of τ(T) and the prediction for τ from combining the string model with the AG expression (Eq. (15)). We also show the fits to the VFT equation, as well as the high-T expansion of the string model. Note that for the high-T expansion is nearly coincident with the VFT extrapolation. The horizontal dashed blue line illustrates the value where τ ≈ 100 s, which typically defines the glass transition temperature. The inset shows τ as a function of T_g/T in order to highlight the predicted return to Arrhenius (strong) behavior in the vicinity of T_g. (b) Configurational entropy S_conf(T) from numerical simulations²⁰ in comparison with the predicted variation from the string model.

The plateau of L(T) at low T has important consequences for the low T behavior of τ. Specifically, such a plateau implies a return to an Arrhenius temperature dependence, as illustrated in the inset of Fig. 9(a). Consequently, the fragility, based on the string model, is smaller than values estimated from a VFT fit. This “fragile-to-strong” crossover in the string model occurs rather close to the expected T_g for the system. Given the extrapolation required to assign a precise value for T_g, it is plausible that this crossover behavior occurs roughly on entering the glass state. Such an Arrhenius temperature dependence near T_g has been observed in a variety of GF fluids,¹ and recent experimental aging results in a 20 million-year-old amber suggests this Arrhenius behavior continues significantly below T_g,⁸⁰ as the our polymerization model predicts.

Based on the inverse scaling relation between L and S_conf, we can also use L from the polymerization model to anticipate the low T behavior of S_conf, shown in Fig. 9(b). Configurational entropy is taken from Ref. 28, and the T dependence of S_conf is similar to that of many fluids.^9–16,81

The low T plateau of L corresponds to a prediction for the saturation of S_conf to a residual low T residual value that is about one-quarter of its value near T_A. Consequently, the Kauzmann entropy “catastrophe,”¹ in which S_conf of would be negative at low T, is naturally avoided in the string model of glass formation. The plateau in S_conf does not violate any thermodynamic relationship, and there is experimental evidence⁸² that the predicted plateau in S_conf at low T, accompanying the plateau in L, can occur as an equilibrium phenomena. For example, the X-Y spin model can be described by equilibrium polymerization transition involving directed closed strings (rings). S_conf remains constant in this model for a substantial T range at low T, as in our string model of glass-forming liquids.⁸³

The residual entropy as T approaches zero is determined by Φ_A and L_A in the living polymerization model, and these parameters control the sharpness or “cooperativity” of the polymerization transition. If we consider the extrapolation of the high T expansion to estimate S_conf at low T (Fig. 9(b)), we see the extrapolation predicts a Kauzmann temperature T_k = 0.2 (i.e., where S_conf = 0). The vanishing is an artifact of the high-T expansion, and the data are entirely consistent with an earlier extrapolation of S_conf based on a simple polynomial fit,²⁰ as well as the VFT extrapolated divergence temperature T₀. A similar vanishing of S_conf based on the use of a high T expansion outside its range of validity is also found in a recent entropy theory for polymer glass formation.⁸⁴ Although the vanishing of S_conf here is a result of an improper extrapolation, the VFT and Kauzmann temperatures remain useful characterization temperatures of glass formation. The temperature is at least better defined than T_g, whose value varies appreciably with cooling rate.

V. CONCLUSIONS

Our identification of the living polymerization model with clusters of coherent string-like displacements in GF liquids implies that glass formation can be interpreted as a kind order-disorder transition in which particles reversibly associate into string-like clusters at equilibrium, but where the “polymerized” ordered state that does not have long-ranged translational order. This type of order-disorder, or “rounded” transition,⁸⁵ is accompanied by progressive, but continuous, changes in enthalpy and configurational entropy and is characteristic of numerous self-assembly process in nature. Since rounded transitions are not phase transitions having a well-defined critical temperature, it is necessary to characterize these relatively broad transitions with an onset temperature T_A, and end-point temperature, here T_g (or perhaps better, T₀). In living polymerization, the approximate midpoint T is termed the polymerization temperature, T_p.

Dynamical heterogeneity in the form of strings seems to be ubiquitous in strongly-interacting, condensed-phase systems, ranging from simple GF fluids, to the interfacial dynamics of nanoparticles, lipid membranes, crystal melting, and grain boundaries,^{24,29,30,86–88} and may be relevant to describe physical aging in glass-forming liquids.⁸⁹ Strings have been observed directly experimentally in the amorphous interfacial region of crystals and in particle tracking measurements of granular and colloidal particles.^90,91 Such string-like excitations are natural expressions of thermal fluctuations associated with the progressive emergence of rigidity in cooled liquids, and equilibrium polymerization models have been introduced to describe emergent collective macroscopic response in other contexts.⁹² For example, polymerization models have been used to describe the disorder transition in type II superconductors⁹³ and the melting transition of crystals.⁹⁴ In modeling the heterogenous nature of turbulent fluids, the vortex fluid excitations have been modeled as equilibrium polymers.⁹⁵ Zwanzig⁹⁶ developed a theory of elementary excitations in classical liquids that parallels the treatment of collective excitations in liquid ⁴He, and this framework offers a potential starting point for understanding the strings at a more fundamental level.

Keys et al.⁶⁶ have also introduced a thermal excitation model of glass-forming liquids that might have some relation to those in our work, but there are qualitative differences in the character of these excitations from those studied in the present work. While their excitations are likewise associated with persistent changes in particle displacement, their size exhibits essentially no temperature dependence and their concentration changes with temperature, features different from strings whose average length L is directly related to the activation energy of structural relaxation and diffusion. There is no real discrepancy. Since the collective excitations of Keys et al.⁶⁶ occur on a picosecond timescale rather than the string lifetime, which relates to relaxation time related to molecular diffusion. As Keys et al. note, their excitations are more closely related to the microstrings⁶⁶ (or stringlets^97,98) defined in previous work on glass-forming liquids, and future work should investigate the relation between these fundamentally different thermal excitations. The facilitation approach to glass formation⁶⁶ is also predicated on a different physical view of glass formation. This approach is built around a purely dynamical description of vitrification, while our approach, like that of the Adam-Gibbs theory and the RFOT, are founded on an intimate linkage between thermodynamics and dynamics.

Finally, we make some general observations about our modeling of structural relaxation in relation to the classical Adam-Gibbs model.⁶ The string model of glass-formation involves a number of extensions and revisions of the AG model:

• •AG do not specify the hypothetical forms of their clusters. Our theory indicates these CRR have the form of self-avoiding polymers with progressively screened excluded volume interactions upon cooling. The string model takes the activation free energy to be proportional to the average string length, L, a quantity that can be readily identified and computed. We also note that L_A is larger than unity, a finding having ramifications for our model of relaxation.
• •AG assume that Δμ is a purely temperature independent enthalpic parameter; we include the sometimes appreciable entropy of activation, so that Δμ = ΔH_a − TΔS_a.
• •AG argued that the hypothetical CRR should be weakly interacting to rationalize an inverse scaling between z* and the fluid configurational entropy S_conf. Instead, the inverse relation between L and S_conf naturally derives from the theory of living polymerization. Our simulations show that the string excitations actually exhibit rather strong interactions, leading to a screening of their excluded volume interactions as they grow and interpenetrate.

Our integration of the AG and living polymerization theories evidently involves features that are distinct from AG, even if it is built on the same qualitative picture that the activation free energy varies with the growth of fluid excitations whose mass grows upon cooling. In contrast, the string theory of relaxation offers a quantitative and physically verifiable route to describe the structural nature of cooperative motion in condensed materials, and also provides direct insight into how these structures influence the relaxation and diffusion of the fluid. Of course, this model requires further tests for other glass-forming systems to check its general validity and to better understand the energetic parameters of the theory. An important aspect of this theory is that it provides a metrology for characterizing essential activation parameters and measures of collective motion and fragility that can be widely used to characterize diverse glass-forming materials in materials science and biology.