Abstract
Lanthanum hexaboride (LaB6) has become a material of intense interest in recent years due to its low work function, thermal stability and intriguing optical properties. LaB6 is also a semiconductor plasmonic material with the ability to support strong plasmon modes. Some of these modes uniquely stretch into the infrared, allowing the material to absorb around 1000 nm, which is of great interest to the window industry. It is well known that the plasmon of LaB6 can be tuned by controlling particle size and shape. In this work, we explore the options available to further tune the optical properties by describing how metal vacancies and Eu doping concentrations are additional knobs for tuning the absorbance from the near-IR to far-IR in La1−xEuxB6 (x = 0, 0.2, 0.5, 0.8, and 1.0). We also report that there is a direct correlation between Eu concentration and metal vacancies within the Eu1−xLaxB6.
Keywords: plasmon, hexaboride, doping, lanthanum hexaboride, LaB6
1. Introduction
Plasmonic nanoparticles are well known for their intriguing properties [1], and are being explored in a variety of fields such as photovoltaics [2], nanosensors [3], drug delivery devices [4], and quantum optics [5]. The physical properties of plasmonic materials are typically easy to tune because of their high carrier concentration and small size, where seemingly minor adjustments such as altering the particle shape or size have a substantial influence on the absorbance spectrum [1]. Vacancies also play a large role in tuning the optical properties of such materials, having a significant influence on free carrier density and doping constraints [6,7]. It′s even possible to fully tune the plasmon independent of dopant concentration in core-shell indium-tin-oxide nanoparticles [8] and by reducing holes in the valence band in copper sulfide [9].
Plasmonic materials are highly sought after in the windows industry. The ability to design a material to selectively transmit in the visible region while absorbing the most intense radiative heat in the IR (about 750 nm–1250 nm) is important for smarter window design, especially in hot climates. [10,11,12] Metal hexaborides (MB6) are being sought after for these applications, and with lanthanum hexaboride (LaB6) absorbing in the middle of this range (~1000 nm) [13,14] we focus our efforts here on the tuning of LaB6. It has already been shown that changing the particle size of LaB6 nanoparticles offers a means of controlling the plasmon [15,16] and that these particles may be incorporated into polymers to make films [17,18]. Though some work has been done on LaB6 to study how La vacancies influence vibrational energies [19] and how doping impacts the thermionic power [20,21], there is a potential link between doping content and vacancies in LaB6 that has gone unexplored. Given the ability of doping levels and metal vacancies to alter free electron concentrations and thus the optical properties in Eu1-xLaxB6, we wished to explore the possible connection between doping concentration and metal vacancies as an additional means of controlling the plasmon.
In this work we demonstrate the possibility of alloying LaB6 nanoparticles with Eu using, for the first time, a low temperature solid state technique with varying ratios of Eu to La. Interestingly, we report there is a direct correlation between Eu concentration and metal (M) vacancies within the Eu1-xLaxB6 system. Furthermore, this method allows the plasmon to be tuned across an incredibly large absorbance range from 1100 nm to 2050 nm, which may open doors to new optoelectronic applications.
2. Experimental Procedures
Anhydrous lanthanum (III) chloride (99.9% pure, Strem Chemical), anhydrous europium (III) chloride (99.99% pure, Strem Chemical) and sodium borohydride (EMD) were used as received and stored in an argon atmosphere glove box until use. Reactant powders were a stoichiometric 6:1 ratio of NaBH4 to metal chloride, where the metal chloride content was a mixture of EuCl3 and LaCl3 with varying ratios of (Eu:La). The mixtures were transferred to alumina boats approximately two inches long and 1 cm wide and the reactions run in a one-inch diameter quartz tube in a Lindberg tube furnace. The reaction was purged with argon at 200 cc/min for 20 min prior to heating. Gas flow was reduced to 100 cc/min and the furnace heated to 450 °C at a rate of 10 °C/min. The reaction was held at 450 °C for 60 min and then cooled to room temperature under argon. The black solid was cleaned in air using methanol to react excess NaBH4, HCl to convert residual sodium into sodium chloride and, finally, water to remove the sodium chloride. With each washing step, the solution was centrifuged at 10,000 rpm for ten minutes and the solvent removed. Severe aggregation of these ligand-free particles rendered electron-microscopy imaging infeasible. However, diffraction data suggest that the particles were approximately 17 nm, with the Scherrer equation giving calculated sizes of 17.46, 16.84, 17.62 and 17.21 nm, respectively, for x = 0.2, 0.5, 0.8 and 1.0.
Samples were analyzed by powder X-ray diffraction on a D8 Discover diffractometer (Bruker AXS Inc., Madison, WI, USA) operated at 35 kV and 40 mA using CoKα radiation. Samples were prepared for optical measurements by drop casting onto quartz slides. Raman spectra were collected on a LabRAM ARAMIS (HORIBA Jobin Yvon, Edison, NJ, USA) automated scanning confocal Raman microscope using a 532-nm excitation laser. Elemental analysis was performed by EDX spectroscopy on a Gemini Ultra-55 scanning electron microscope (Zeiss, Thornwood, NY, USA), and FTIR spectroscopy was performed on a Spectrum One equipped with an HATR assembly (PerkinElmer, Santa Clara, CA, USA). The absorbance was collected on a Cary-5000 UV-Vis-NIR (Agilent Technologies, Santa Clara, CA, USA). Samples were prepared for optical measurements by drop casting from water onto quartz slides, and the films were allowed to dry naturally in air.
3. Results and Discussion
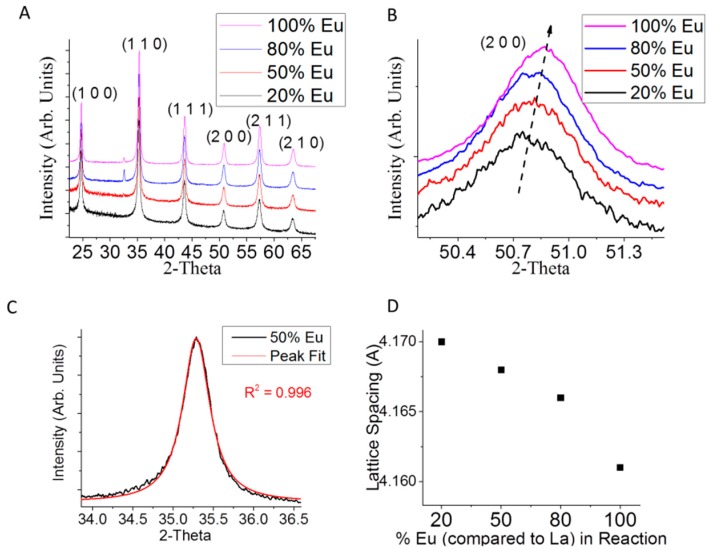
The success of the incorporation of a Eu into LaB6 was evident in changes to the XRD pattern of LaxEu1-xB6 (Figure 1A). Note that the small peak at ~33° is from an unidentified impurity in the EuCl3. Increasing the concentration of Eu in the LaxEu1−xB6 synthesis caused a shift of the diffraction pattern to higher 2-Theta (Figure 1B), which is indicative of increased compressive lattice strain. This seems counterintuitive since incorporating larger atoms typically expands a crystal lattice. For instance, in Eu1-xCaxB6 the larger Eu atom replaces Ca and the lattice expands [21]. There is a possibility that increasing the amount of Eu in LaxEu1-xB6 may produce two phases, as reported for the (BaxCa1−x)B6 system which has a mixture of both Ba-rich and Ca-rich particles in the final product [22]. Though this could account for the unexpected change to the lattice strain in our system, the diffraction peaks of LaxEu1−xB6 are symmetric, which is indicative of a single phase (Figure 1C). In LaxEu1-xB6, there appears to be a decrease in lattice spacing with increasing Eu content (Figure 1D), even though Eu is larger than La. The B6 network, like all boron lattices, is electron-deficient and is only stable because of electron transfer from the metals [23]. Though Eu2+ and Ca2+ in Eu1−xCaxB6 are different sizes they are also both divalent, so the free electron density does not change when increasing the Ca content. By contrast, in LaxEu1−xB6 there is a mix of trivalent La3+ and divalent Eu2+. This and the metal (M) vacancies within the system are likely responsible for the increasing lattice strain with increasing Eu concentration.
Figure 1.
X-ray diffraction of (A) LaxEu1−xB6; (B) a magnified image of the (2 0 0) diffraction plane with LaxEu1−xB6 where x = 0.0, 0.2, 0.5 and 0.8; (C) Pearson VII peak fit of LaxEu1−xB6 with x = 0.5; and (D) lattice spacing versus atomic % Eu in the LaxEu1−xB6 reaction (calculated using Bragg′s law).
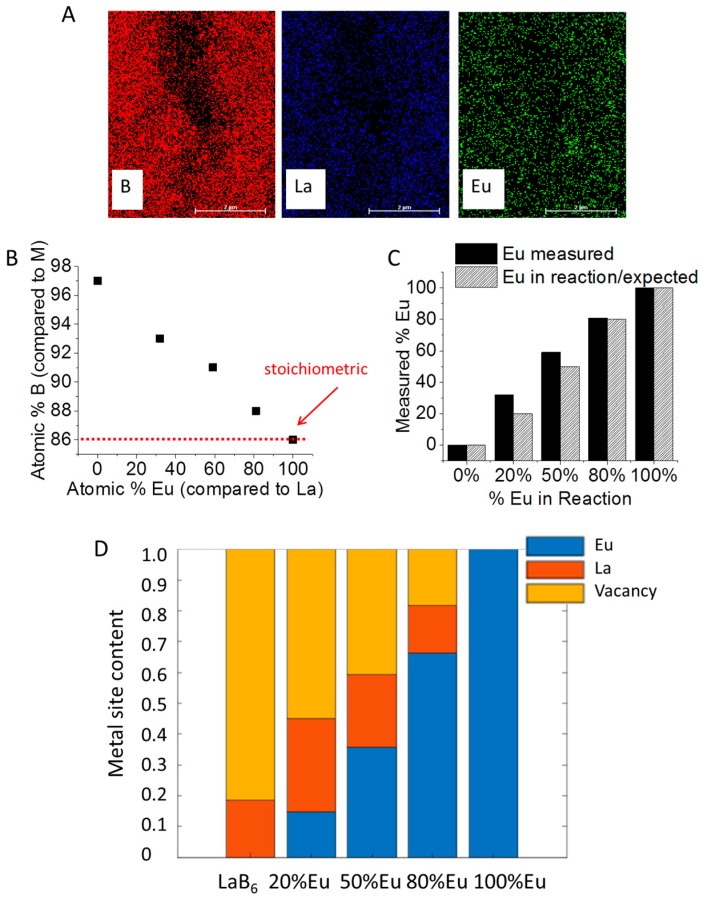
EDS confirmed the presence of all three elements (La, B, and Eu) in LaxEu1−xB6 samples (Figure 2A; LaxEu1−xB6 with x = 0.2). Intriguingly, LaB6 synthesized under this method contained about 97% B, which indicates a huge amount of M vacancies with x = 0.19 (equivalent to about 80% M vacancies). M vacancies are common in LaB6, but it is understood that the lattice constant is unaffected by these voids [19,24,25]. he stability of the crystal structure is dictated by the bonds in the boron framework and not by the metal content so long as the electronic requirements of the structure are met [26]. However, if there is too much void space then MB6 becomes unstable. Though there is a lot of disagreement surrounding La-B phase diagrams, a B content above 90% [24,27] is expected to contain both LaB6 and an additional B phase [25,26,28,29], which suggests that any excess boron in our system may not lie within the MB6 structure. However, we see no indication of a separate B phase beyond LaxEu1−xB6 by XRD. The phase diagrams of La-B were developed under the assumption that high temperatures (≥1500 °C) are required to make LaB6, which was disproved only recently [15,30]. With low temperature reactions we recently reported the existence of bridging halogens between La atoms which are involved in the lattice formation of LaB6 [31,32,33], so even though a sample containing 97% B may potentially have a massive amount of vacancies, it′s possible that the structure was stable during formation because these halogens fulfilled the electronic requirements necessary to stabilize the material without the need of an additional B phase. Unfortunately, the amount of Cl in the materials reported here were either too low in concentration to be detected by EDS or the 450 °C reaction temperature was high enough to remove the bridging-Cl atoms as the final product formed. Work is ongoing understand exactly how halogen atoms enter into the reaction mechanism.
Figure 2.
(A) View of the EDS map of LaxEu1−xB6 (x = 0.2) including B, La and Eu; (B) atomic % B versus atomic % Eu (the red dashed line is stoichiometric with 1M:6B); (C) measured versus expected % Eu (comparing Eu to La) in LaxEu1−xB6; and (D) metal content (Eu and La) and M void in LaxEu1−xB6.
As the concentration of Eu in the LaxEu1−xB6 reaction is increased there is a clear trend of increasing amounts of B relative to M until the system becomes stoichiometric with EuB6 (86% B or x = 1; Figure 2B), with a slightly higher Eu content in LaxEu1−xB6 than was expected with x < 1 (Figure 2C). It′s possible that EuB6 is more energetically favored than LaB6 or that there are so many vacancies that at low concentrations the divalent Eu2+ has an easier time filling holes in addition to replacing La atoms. Regardless, there is a clear trend of decreasing vacancies with increasing Eu in the reaction (Figure 2D). Unfortunately, the ligand-free nature of these particles results in an aggregated product, rendering single-particle analysis on individual LaB6 particles infeasible.
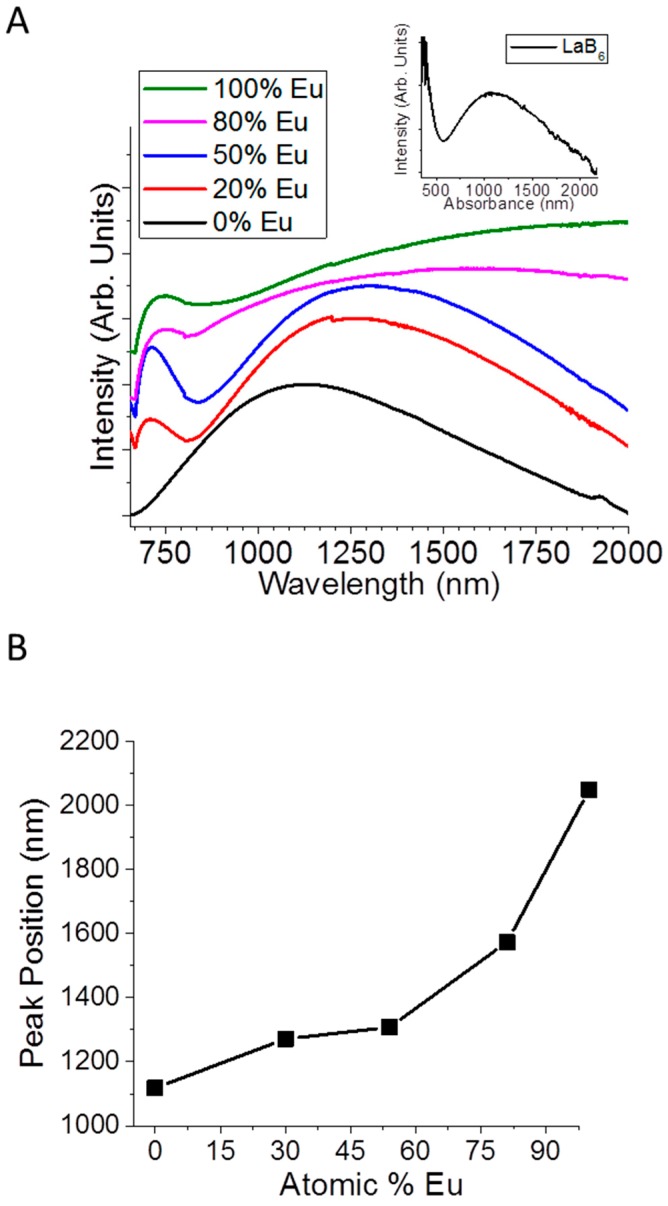
There have been several publications studying the ability to tune the plasmon of LaB6 to achieve desired optical properties [13,14,15], but little is yet known about how vacancies influence these properties. Research discussing vacancies related to optical and vibrational properties in LaB6 are very recent [19,31], and though much has been done to study the magnetic and thermoelectric properties of LaxEu1−xB6 [34,35], no one until now has synthesized doped hexaborides at low temperatures. Furthermore, only in very recent years have the optical properties of doped MB6 been explored [36,37,38]. In this work, we used absorbance spectroscopy to determine how the Eu concentration and M vacancies in LaxEu1−xB6 nanocrystals can be used to tune the plasmonic properties (Figure 3A). When increasing the concentration of Eu the small absorbance peak in the visible region that is indicative of metal hexaborides shifts from ~380 nm in pure LaB6 to 730 nm in pure EuB6, while the larger absorbance peak red shifts from 1100 nm in pure LaB6 to 2050 nm in pure EuB6 (Figure 3B). Introducing Eu as a dopant causes a constant red shift of the absorbance peak from 1100 nm in pure LaB6 to 2050 nm in pure EuB6 (Figure 3B). This shift is a result of changes to the number of electrons in the conduction band as divalent Eu2+ replaces trivalent La3+. The sudden broadening of the absorbance at 80% Eu is most likely due to the changing carrier concentration which results from Eu incorporation as well as from changing metal vacancies within the lattice.
Figure 3.
(A) Absorbance of LaxEu1−xB6 changing with Eu content (normalized) and (B) absorbance peak position versus atomic % Eu in LaxEu1−xB6.
The electron deficiency is calculated as vacancy content minus lanthanum content. Whatever the mechanism causing the change in lattice spacing (vacancies or changing Eu content), the shifting absorption peak is indicative of an increase in carrier density with lanthanum content, and is impacted by vacancies within the system. Equation 1 gives the most basic model for the wavelength of the plasmon resonance [39],
| (1) |
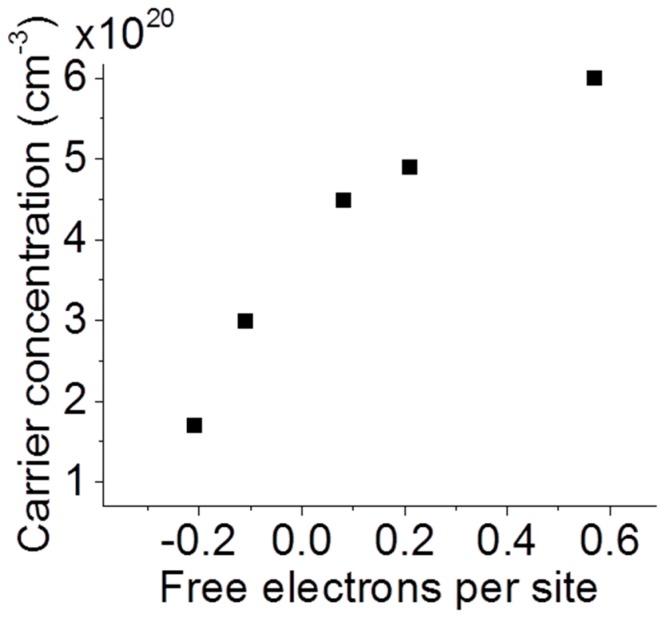
where N is the number of charge carriers per unit volume, e is the charge of each carrier, m* is the effective mass of the charge carriers, ε0 is the permittivity of free space, εm is the dielectric function of the surrounding medium, ε∞ is the dielectric limit for the material at high frequencies (accounting for bound charge), and k is a geometrical factor. The absorbance spectroscopy was performed in air, so εm is nearly unity. We treat the particles as spherical [15,19], which is associated with a constant of k = 2 and an effective electron mass of 0.225 m0 in EuB6 as reported based on optical measurements [40]. Finally, taking ε∞ as unity, our absorption peaks translate to the charge concentrations in Figure 4. In short, Figure 4 illustrates qualitative agreement between increasing carrier concentration as inferred from plasmonic resonance and increasing carrier concentration as inferred from composition measurements. As the Eu content is increased the samples lose free electrons and the absorbance peak expands and broadens.
Figure 4.
Localized surface plasmon resonance inferred carrier concentration versus number of free electrons per metal site in LaxEu1−xB6.
4. Conclusions
We have found that systematically increasing the amount of divalent Eu2+ compared to trivalent La3+ within Eu1−xLaxB6 not only decreases the lattice spacing but drastically changes the vacancies within the system. These vacancies have a large influence on the optical properties and allow the plasmon to be tuned across an incredibly large range from 1100 nm to 2050 nm. The true nature of these particles on the nanoscale is not fully understood (i.e., the influence of Cl bridging atoms), but we are making great strides to improve our knowledge of this system. It is our hope that this work will not only help to further our understanding of the MB6 crystal structure, but may open new doors for developing new devices, optoelectronics, and more. Research is ongoing to study how this synthetic method may be used to alter the nanoparticle surface, bringing to light new properties which may become a vital aspect for biosensing applications.
Acknowledgments
This work was supported by the Molecular Foundry and the Advanced Light Source at Lawrence Berkeley National Laboratory, both user facilities supported by the Office of Science, Office of Basic Energy Sciences, of the U.S. Department of Energy (DOE) under Contract No. DE-AC02-05CH11231. Work was also supported in part by the DOE Office of Science, Office of Workforce Development for Teachers and Scientists (WDTS) under the Science Undergraduate Laboratory Internship (SULI) program.
Author Contributions
T.M.M. and J.J.U. conceived the idea; T.M.M. designed the experiments and wrote the paper; D.K.C., I.R., and C.S. performed experiments and analysis.
Conflicts of Interest
The authors declare no competing financial interests.
References
- 1.Mattox T.M., Ye X., Manthiram K., Schuck P.J., Alivisatos A.P., Urban J.J. Chemical Control of Plasmons in Metal Chalcogenide and Metal Oxide Nanostructures. Adv. Mater. 2015;27:5830–5837. doi: 10.1002/adma.201502218. [DOI] [PubMed] [Google Scholar]
- 2.Stockman M.I. Nanoplasmonics: The physics behind the applications. Phys. Today. 2011;64:39–44. doi: 10.1063/1.3554315. [DOI] [Google Scholar]
- 3.Anker J.N., Hall W.P., Lyandres O., Shah N.C., Zhao J., Duyne R.P.V. Biosensing with plasmonic nanosensors. Nat. Mater. 2008;7:442. doi: 10.1038/nmat2162. [DOI] [PubMed] [Google Scholar]
- 4.Lopatynskyi A.M., Lytvyn V.K., Mogylnyi I.V., Rachkov O.E., Soldatkin O.P., Chegel V.I. Smart nanocarriers for drug delivery: Controllable LSPR tuning. Semicond. Phys. Quantum Electron. Optoelectron. 2016;19:358–365. doi: 10.15407/spqeo19.04.358. [DOI] [Google Scholar]
- 5.Tame M.S., McEnery K.R., Özdemir S.K., Lee J., Maier S.A., Kim M.S. Quantum plasmonics. Nat. Phys. 2013;9:329–340. doi: 10.1038/nphys2615. [DOI] [Google Scholar]
- 6.Elimelech O., Liu J., Plonka A.M., Frenkel A.I., Banin U. Size Dependence of Doping by a Vacancy Formation Reaction in Copper Sulfide Nanocrystals. Angew. Chem. 2017;129:10471–10476. doi: 10.1002/ange.201702673. [DOI] [PubMed] [Google Scholar]
- 7.Luther J.M., Jain P.K., Ewers T., Alivisatos A.P. Localized surface plasmon resonances arising from free carriers in doped quantum dots. Nat. Mater. 2011;10:361. doi: 10.1038/nmat3004. [DOI] [PubMed] [Google Scholar]
- 8.Crockett B.M., Jansons A.W., Koskela K.M., Johnson D.W., Hutchison J.E. Radial Dopant Placement for Tuning Plasmonic Properties in Metal Oxide Nanocrystals. ACS Nano. 2017;11:7719–7728. doi: 10.1021/acsnano.7b01053. [DOI] [PubMed] [Google Scholar]
- 9.Kalanur S.S., Seo H. Tuning plasmonic properties of CuS thin films via valence band filling. RSC Adv. 2017;7:11118–11122. doi: 10.1039/C6RA27076J. [DOI] [Google Scholar]
- 10.Granqvist C.G. Electrochromics for smart windows: Oxide-based thin films and devices. Thin Solid Films. 2014;564:1–38. doi: 10.1016/j.tsf.2014.02.002. [DOI] [Google Scholar]
- 11.Runnerstrom E.L., Llordés A., Lounis S.D., Milliron D.J. Nanostructured electrochromic smart windows: Traditional materials and NIR-selective plasmonic nanocrystals. Chem. Commun. 2014;50:10555–10572. doi: 10.1039/C4CC03109A. [DOI] [PubMed] [Google Scholar]
- 12.Zhou Y., Huang A., Li Y., Ji S., Gao Y., Jin P. Surface plasmon resonance induced excellent solar control for VO2 @SiO2 nanorods -based thermochromic foils. Nanoscale. 2013;5:9208–9213. doi: 10.1039/c3nr02221h. [DOI] [PubMed] [Google Scholar]
- 13.Adachi K., Miratsu M., Asahi T. Absorption and scattering of near-infrared light by dispersed lanthanum hexaboride nanoparticles for solar control filters. J. Mater. Res. 2010;25:510–521. doi: 10.1557/JMR.2010.0075. [DOI] [Google Scholar]
- 14.Takeda H., Kuno H., Adachi K. Solar Control Dispersions and Coatings with Rare-Earth Hexaboride Nanoparticles. J. Am. Ceram. Soc. 2008;91:2897–2902. doi: 10.1111/j.1551-2916.2008.02512.x. [DOI] [Google Scholar]
- 15.Mattox T.M., Agrawal A., Milliron D.J. Low Temperature Synthesis and Surface Plasmon Resonance of Colloidal Lanthanum Hexaboride (LaB6) Nanocrystals. Chem. Mater. 2015;27:6620–6624. doi: 10.1021/acs.chemmater.5b02297. [DOI] [Google Scholar]
- 16.Machida K., Adachi K. Particle shape inhomogeneity and plasmon-band broadening of solar-control LaB6 nanoparticles. J. Appl. Phys. 2015;118:013013. doi: 10.1063/1.4923049. [DOI] [Google Scholar]
- 17.Jiang F., Leong Y.K., Martyniuk M., Keating A., Dell J.M. Dispersion of lanthanum hexaboride nanoparticles in water and in sol-gel silica arrays; Proceedings of the 2010 Conference on Optoelectronic and Microelectronic Materials and Devices; Canberra, Australia. 12–15 December 2010; pp. 163–164. [Google Scholar]
- 18.Schelm S., Smith G.B. Dilute LaB6 nanoparticles in polymer as optimized clear solar control glazing. Appl. Phys. Lett. 2003;82:4346–4348. doi: 10.1063/1.1584092. [DOI] [Google Scholar]
- 19.Mattox T.M., Chockkalingam S., Roh I., Urban J.J. Evolution of Vibrational Properties in Lanthanum Hexaboride Nanocrystals. J. Phys. Chem. C. 2016;120:5188–5195. doi: 10.1021/acs.jpcc.5b12680. [DOI] [Google Scholar]
- 20.Zhou S., Zhang J., Liu D., Hu Q., Huang Q. The effect of samarium doping on structure and enhanced thermionic emission properties of lanthanum hexaboride fabricated by spark plasma sintering. Phys. Status Solid A. 2014;211:555–564. doi: 10.1002/pssa.201330152. [DOI] [Google Scholar]
- 21.Jong-Soo Rhyee B.K.C., Kim H.C. Possible adiabatic paloronic hopping in Ca1-xEuxB6 (x = 0.005, 0.01, and 0.05) Phys. Rev. B. 2005;71:073104. doi: 10.1103/PhysRevB.71.073104. [DOI] [Google Scholar]
- 22.Cahill J.T., Alberga M., Bahena J., Pisano C., Borja-Urby R., Vasquez V.R., Edwards D., Misture S.T., Graeve O.A. Phase Stability of Mixed-Cation Alkaline-Earth Hexaborides. Cryst. Growth Des. 2017;17:3450–3461. doi: 10.1021/acs.cgd.7b00391. [DOI] [Google Scholar]
- 23.Etourneau J. Critical survey of rare-earth borides: Occurence, crystal chemistry and physical properties. J. Less-Common Met. 1985;110:267–281. doi: 10.1016/0022-5088(85)90333-9. [DOI] [Google Scholar]
- 24.Otani S., Honma S., Ishizawa Y. Preparation of LaB6 single crystals by the floating zone method. J. Alloys Compd. 1993;193:286–288. doi: 10.1016/0925-8388(93)90373-U. [DOI] [Google Scholar]
- 25.Korsukova M.M., Gurin V.N. Physicochemical Problems in the Preparation of Defect-free Monocrystals of Lanthanum Hexaboride. Russ. Chem. Rev. 1987;56:1. doi: 10.1070/RC1987v056n01ABEH003252. [DOI] [Google Scholar]
- 26.Johnson R.W., Daane A.H. The lanthanum-boron system. J. Phys. Chem. 1961;65:909–915. doi: 10.1021/j100824a004. [DOI] [Google Scholar]
- 27.Otani S., Nakagawa H., Nishi Y., Kieda N. Floating Zone Growth and High Temperature Hardness of Rare-Earth Hexaboride Crystals: LaB6, CeB6, PrB6, NdB6, and SmB6. J. Solid State Chem. 2000;154:238–241. doi: 10.1006/jssc.2000.8842. [DOI] [Google Scholar]
- 28.Schlesinger M.E., Liao P.K., Spear K.E. The B-La (Boron-Lanthanum) System. J. Phase Equilib. 1999;20:73–78. doi: 10.1361/105497199770335974. [DOI] [Google Scholar]
- 29.Lundstrom T. The homogeneity range of LaB6—An instructive example of phase analytical techniques. Z. Anorg. Allg. Chem. 1986;540:163–168. doi: 10.1002/zaac.19865400917. [DOI] [Google Scholar]
- 30.Zhang M., Wang X., Zhang X., Wang P., Xiong S., Shi L., Qian Y. Direct low-temperature synthesis of RB6 (R = Ce, Pr, Nd) nanocubes and nanoparticles. J. Solid State Chem. 2009;182:3098–3104. doi: 10.1016/j.jssc.2009.08.032. [DOI] [Google Scholar]
- 31.Groome C., Roh I., Mattox T.M., Urban J.J. Effects of size and structural defects on the vibrational perperties of lanthanum hexaboride nanocrystals. ACS Omega. 2017;2:2248–2254. doi: 10.1021/acsomega.7b00263. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Mattox T.M., Croome G., Doran A., Beavers C.M., Urban J.J. Anion-mediated negative thermal expansion in lanthanum hexaboride. Solid State Commun. 2017 doi: 10.1016/j.ssc.2017.07.012. accepted. [DOI] [Google Scholar]
- 33.Mattox T.M., Groome C., Doran A., Beavers C.M., Urban J.J. Chloride Influence on the Formation of Lanthanum Hexaboride: An In-Situ Diffraction Study. J. Cryst. Growth. 2018 doi: 10.1016/j.jcrysgro.2018.01.013. in press. [DOI] [Google Scholar]
- 34.Song M., Yang I.-S., Seo C.W., Cheong H., Kim J.Y., Cho B.K. Local symmetry breaking in Eu1−xLaxB6. J. Magn. Magn. Mater. 2007;310:1019–1020. doi: 10.1016/j.jmmm.2006.10.221. [DOI] [Google Scholar]
- 35.Zhitomirsky M.E., Rice T.M., Anisimov V.I. Ferromagnetism in the hexaborides. Nature. 1999;402:251–253. doi: 10.1038/46207. [DOI] [Google Scholar]
- 36.Chao L., Bao L., Shi J., Wei W., Tegus O., Zhang Z. The effect of Sm-doping on optical properties of LaB6 nanoparticles. J. Alloys Compd. 2015;622:618–621. doi: 10.1016/j.jallcom.2014.10.141. [DOI] [Google Scholar]
- 37.Chao L., Bao L., Wei W., Tegus O. Optical properties of Yb-doped LaB6 from first-principles calculation. Mod. Phys. Lett. B. 2016;30:1650091. doi: 10.1142/S0217984916500913. [DOI] [Google Scholar]
- 38.Li Q., Zhao Y., Fan Q., Han W. Synthesis of one-dimensional rare earth hexaborides nanostructures and their optical absorption properties. Ceram. Int. 2017;43:10715–10719. doi: 10.1016/j.ceramint.2017.05.035. [DOI] [Google Scholar]
- 39.Willets K.A., Duyne R.P.V. Localized Surface Plasmon Resonance Spectroscopy and Sensing. Annu. Rev. Phys. Chem. 2007;58:267–297. doi: 10.1146/annurev.physchem.58.032806.104607. [DOI] [PubMed] [Google Scholar]
- 40.Gurin V.N., Korsukova M.M., Karin M.G., Sidorin K.K., Smirnov I.A., Shelikh A.I. Optical Constants of EuB sub (6) and LaB sub (6) Sov. Phys. Solid State Commun. 1980;22:418–421. [Google Scholar]