Abstract
Purpose
Current clinical perimetric test paradigms present stimuli randomly to various locations across the visual field (VF), inherently introducing spatial uncertainty, which reduces contrast sensitivity. In the present study, we determined the extent to which spatial uncertainty affects contrast sensitivity in glaucoma patients by minimizing spatial uncertainty through attentional cueing.
Methods
Six patients with open-angle glaucoma and six healthy subjects underwent laboratory-based psychophysical testing to measure contrast sensitivity at preselected locations at two eccentricities (9.5° and 17.5°) with two stimulus sizes (Goldmann sizes III and V) under different cueing conditions: 1, 2, 4, or 8 points verbally cued. Method of Constant Stimuli and a single-interval forced-choice procedure were used to generate frequency of seeing (FOS) curves at locations with and without VF defects.
Results
At locations with VF defects, cueing minimizes spatial uncertainty and improves sensitivity under all conditions. The effect of cueing was maximal when one point was cued, and rapidly diminished when more points were cued (no change to baseline with 8 points cued). The slope of the FOS curve steepened with reduced spatial uncertainty. Locations with normal sensitivity in glaucomatous eyes had similar performance to that of healthy subjects. There was a systematic increase in uncertainty with the depth of VF loss.
Conclusions
Sensitivity measurements across the VF are negatively affected by spatial uncertainty, which increases with greater VF loss. Minimizing uncertainty can improve sensitivity at locations of deficit.
Translational Relevance
Current perimetric techniques introduce spatial uncertainty and may therefore underestimate sensitivity in regions of VF loss.
Keywords: standard automated perimetry, glaucoma, eccentricity, retina, psychophysics
Introduction
Standard automated perimetry (SAP) is a commonly used method of assessing contrast sensitivity across the visual field (VF).1–3 In SAP, contrast sensitivity thresholds are measured using achromatic stimuli of fixed size (Goldmann size III [GIII]) presented briefly (∼100–200 ms) upon a uniformly illuminated achromatic background.3 Patients are instructed to respond when they see a stimulus appear in the VF. Stimuli appear in pseudorandom order: typically, four ‘seeding' points are tested to determine the patient's initial Hill of Vision, after which the contrast levels for testing adjacent points are modulated. Modern test algorithms also incorporate a Bayesian approach to determine the most likely threshold level representing the contrast sensitivity at each location.4
There are a number of inherent limitations to clinical SAP testing.5 While adaptive algorithms provide a means for efficiently determining thresholds, errors arising from individual patient variability and subjective criterion may be present, particularly in regions of reduced sensitivity in diseases, such as in glaucoma.6,7 Use of a large stimulus size (e.g., Goldmann V [GV]), may reduce variability in such cases.8–10 Although smaller stimuli have been shown to be better able to detect VF defects,11,12 variability of thresholds obtained using GV is relatively lower.13,14 GV offers a wider dynamic range of testing, which may make it more useful with increased VF defect depth.15 It is also more resistant to blur and defocus, at the cost of disease detection ability.16
More recently, spatial uncertainty in perimetric testing has been suggested to play a significant role in contrast sensitivity measurement across the VF.17,18 Following the initial ‘seeding' points in SAP, the number of possible test locations increases dramatically, and so the observer is unable to predict the location of the next presentation.19 Although the initial ‘seeding' points may generate some level of uncertainty (four possible test locations), more spatial uncertainty is introduced following these points, as the observer must now attend to a greater number of test regions within the VF. This affects the detectability of stimuli, especially at contrast levels close to threshold, because there is an increase in the possibility that a target is missed due to inattention.20,21 Naturally, contrast sensitivity is lower due to spatial uncertainty, as the stimulus must be higher in contrast to overcome this limitation. Spatial uncertainty in SAP may be reduced through the use of retest options on certain perimeters (e.g., the Medmont Automated Perimeter) or in the design of custom test paradigms (e.g., the Custom Test function of the Humphrey Field Analyzer or the Octopus Open Perimetry Interface).
In healthy observers, verbal17 and visual attentional cueing18 in contrast sensitivity testing across the VF results in improved contrast sensitivity by reducing spatial uncertainty. Spatial attention endogenously focused onto one or a small subset of locations avoids low-contrast stimuli being ‘missed' due to inattention during the brief presentation of the stimulus.22 Uncertainty has been shown to be heightened when testing with small stimuli (e.g., Goldmann size I [GI] equivalent) and at peripheral test locations as both factors reduce the visibility of the test stimulus.17,18 Conversely, thresholds measured using large stimuli (e.g., GV) and at test locations close to fixation do not exhibit the same improvement with cueing. However, in patients with impaired VFs, the loss of detector and neural elements leads to sparseness in sampling, leading to poorer sensitivity and greater threshold variability.23–25 Elevated thresholds are therefore a product of not only the underlying deficit, but may also be conflated due to spatial uncertainty inherent to SAP. No study to date has examined how spatial uncertainty might conflate contrast sensitivity thresholds in disease, and whether the effect is dependent on the depth of deficit. This has clinical significance as the thresholds returned by SAP instruments may not accurately reflect the threshold elevation as a result of disease, but may be confounded by the presence of spatial uncertainty.
Glaucoma, the most common primary optic neuropathy, is a slowly progressive ocular disease in which the gradual loss of retinal ganglion cells is accompanied by retinotopically concordant loss of contrast sensitivity in the VF.11,12 Due to its structure–function concordance and typically slowly progressive loss of contrast sensitivity, it offers a model for examining the effect of spatial uncertainty in regions of the VF with impaired contrast sensitivity. The loss of detector elements, in combination with other potential pathologic changes23–25 may increase stimulus uncertainty, and an improvement in contrast sensitivity may be expected if uncertainty were reduced.26
In the present study, we investigated the role of spatial uncertainty in contrast sensitivity in patients with glaucoma. Using contrast sensitivity thresholds obtained using SAP as a basis for testing, we determined the individual frequency of seeing (FOS) curves for patients with glaucoma in multiple regions of VF impairment under different cueing conditions (1, 2, 4, or 8 points cued), with two stimulus sizes (GIII and GV) and at two eccentric locations (9.5° and 17.5° from fixation). The use of laboratory-based psychophysical testing differs from that of SAP testing, but the chosen stimulus parameters, baseline thresholds, and stimulus arrangements to maximize spatial uncertainty mimicking common SAP parameters provide a means with which to compare performance in the presence of attentional cueing. For example, eight points cued has been previously shown to result in the same contrast sensitivity threshold when spatial uncertainty is maximal (i.e., cueing more points does not result in significant changes in sensitivity), such as that expected when all 75 points are testing in the 30-2 test grid in SAP.17 The stimulus sizes and eccentric locations were chosen as perimetric defects typically appear in these locations in early to moderate stages of glaucoma, and the level of defects at these locations are expected to be conducive to showing changes in contrast sensitivity across a range of sensitivity values. Although smaller stimuli may reveal greater defects, the low thresholds may further conflate variability and extend beyond the dynamic range of testing.11,12 The results of patients with glaucoma were compared with a cohort of healthy subjects.
We hypothesize that the loss of detector and neural elements in glaucoma patients results in greater spatial uncertainty, and a greater improvement of contrast sensitivity arises from attentional cueing. One potential explanation for this is perceptual ‘filling in', which results in fallacious perceptions within areas of the VF with impairment.27,28 We also determined the effect of attentional cueing when testing areas exhibiting no apparent functional deficits on SAP. If the effect were the same as if testing a healthy subject free of disease, then it suggests that uncertainty is an additional localized variability factor induced by loss of detector elements. However, a greater magnitude of loss even in regions without deficits suggests that there may be some global effect of glaucomatous defects upon visual perception, which appears to be characteristic of the disease for some visual functions.29 Determining the effect of uncertainty on sensitivity measurements has relevance for understanding how disease impacts the way visual function is measured.
Methods
Participants
Six subjects with healthy vision and ocular health (5 males, 1 female; mean and SD age: 47.5 ± 14.8 years) and six patients (4 males, 2 females; mean and SD age: 65.7 ± 4.8 years) with mild-moderate primary open-angle glaucoma (POAG) acted as participants in the present study. All participants had normal or corrected-to-normal visual acuity, with refractive errors within ±6.00 Diopters Sphere (DS) and ±3.00 Diopters Cyl (DC). All healthy subjects had no evidence of ocular or systemic disease that would affect the VF or visual pathway. Patients with POAG had a mild to moderate stage of glaucoma, classified according to their Humphrey Field Analyzer (HFA; Carl Zeiss Meditec, Dublin, CA) 24-2 SITA-standard SAP results (mean deviation [MD] range: −1.88 to −8.07 dB), with a combination of central and peripheral VF loss.30 The diagnosis of POAG was made on the basis of stereoscopic optic nerve head examination and VF test results when the patients were seen at the Glaucoma Management Clinic at the Centre for Eye Health, University of New South Wales. In short, optic nerve head criteria included: enlarged cup-disk ratio (>0.7), intereye cup-disk ratio asymmetry (>0.2), and focal or diffuse loss or thinning of neuroretinal rim tissue following consideration of optic nerve head size, notching, and/or excavation. VF criteria for a diagnosis of glaucoma and for inclusion in the present study (preperimetric glaucoma patients were not tested) constituted at least one of the following on 24-2 SAP using the HFA: the presence of three or more contiguous nonedge points with a probability (P) of being normal of P < 5%, of which at least one had a P < 1% (‘event' fail), a pattern standard deviation (PSD) score of P < 5%, or a Glaucoma Hemifield Test (GHT) result that was ‘outside normal limits'.12,30 Note that these VF defects were required to match the structural losses seen on optic nerve head examination. Only mild to moderate glaucoma (better than −12.00 dB) subjects were included, that is, none of the subjects had MD values exceeding −12.00 dB on the HFA, which would place them at the severe classification level.30 All participants gave their informed written consent prior to testing. The relevant University of New South Wales Committee gave ethical approval, and the experiment followed the tenets of the Declaration of Helsinki.
Stimulus and Procedures
All observers firstly underwent testing on the HFA to approximate baseline contrast sensitivity across the VF and to determine locations of VF loss. The HFA is a commonly used instrument for testing the VFs in patients with glaucoma, and hence we used these as the baseline measurements of contrast sensitivity, which were then adjusted for each specific patient and test location for the laboratory testing phase.1 SAP testing was conducted using the 24-2 test grid and the SITA-standard algorithm, as per typical clinical practice.31 The achromatic stimulus was used, presented upon an achromatic background of 10 candelas (cd)/m2, which rendered the adaptive state of the eye to be within the low photopic range. Stimuli were presented for 200 ms, and both GIII and GV targets were used for testing. Full threshold was used as adaptive algorithms such as SITA-standard are known to modify thresholds during and at the conclusion of the test.32 Output thresholds on the HFA (in dB) were converted into equivalent Weber contrast units for use in psychophysical testing on the computer-based system (equations 1 and 2 from Khuu and Kalloniatis,13 and also see Phu et al.17 for similar uses).
Then, all subjects underwent psychophysical testing in a laboratory-based set up on custom written software presented on a computer screen. Testing conditions in the laboratory-based testing phase were matched as closely as possible with the clinical testing conditions. Stimuli were also white circular spots of light of constant size (GIII 0.43° in diameter, which is the standard stimulus size used in SAP; and GV 1.72° in diameter, which is a stimulus that may be clinically useful in end-stage disease15,33) presented upon a white-gray background of constant luminance (10 cd/m2) for 200 ms. Test locations lay within specific regions of interest within the 9.5° (corresponding to HFA Cartesian coordinates: [+3, +9], [+3, −9], [−3, +9], [−3, −9], [+9, +3], [+9, −3], [−9, +3], and [−9, −3], which we refer to as “paracentral” in the present study) and 17.5° (corresponding to HFA Cartesian coordinates: [+9, +15], [+9, −15], [−9, +15], [−9, −15], [+15, +9], [+15, −9], [−15, +9], and [−15, −9], which we refer to as “midperipheral”) ‘rings' away from fixation (Fig. 1A). A black fixation mark (0.42° × 0.42°, Weber contrast −1) was placed at the center of the screen, upon which the participant was instructed to fixate during the trial. Stimuli were generated on a gamma-corrected iMac computer (Apple Inc, Cupertino, CA) using custom written software in MATLAB (version 7; MathWorks, Natick, MA), and were presented on the linearized iMac 27-inch (59.6 × 33.6 cm, or 23.5 × 13.2 in) monitor driven at a frame rate of 60 Hz. Linearization was performed using a photometer (Pritchard Photo Research PR-880; Photo Research, Syracuse, NY) and three colored channels presented using custom written software in MATLAB, whereby input linear values were remapped into the screen's output values. With respect to the limits of the screen size, the closest a stimulus came to the edge of the screen was 8.2° (as the largest y-axis coordinate was 21°). A head and chin rest were used to ensure a constant viewing distance of 30 cm. A trial frame with wide aperture trial lenses (38 mm diameter) was used to correct for refractive error and working distance for each participant.
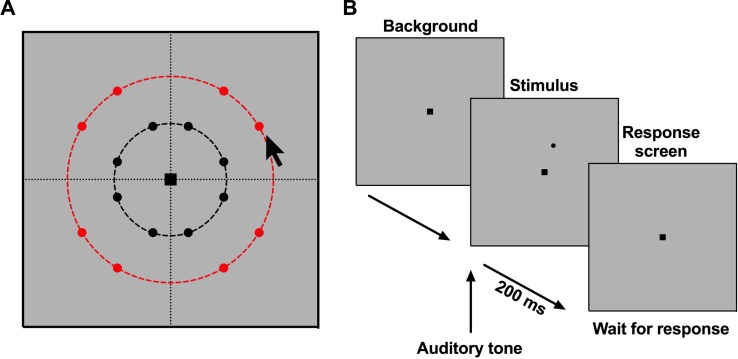
Figure 1.
Schematic of the psychophysical procedure used in the present study. (A) Eccentric locations at which points were located for testing. The black dashed line indicates the 9.5° eccentricity and the red dashed line indicates the 17.5° eccentricity, with the eight points representing locations coincident with test locations within the 30-2 HFA test grid. The black arrow pointer in (A) refers to the pointer used to indicate to the subject the location of the forthcoming stimuli. The arrow was moved around to the different locations matching all of the possible points cued under each condition. The arrow was used prior to the test only, and not during the trial. (B) The sequence of screens during each test of contrast level within the trial.
The number and location of stimuli for each trial was verbally cued to the participant prior to the commencement of the trial, whereby each trial consisted of a set number of points cued (1, 2, 4, or 8) at a particular test location and using one stimulus size. Thus, such cueing arises from endogenous “top-down” processes in which voluntary attention is allocated to certain regions of the VF, as during the course of the trial, participants were not reminded of the cued locations. The locations of the stimuli were cued by their approximate clock-hour location, alongside the total number of points for each individual trial (examples include: “there will be one point appearing at the 1:00 o'clock position,” or “there will be four points appearing: one at the 2:00 o'clock position, one at 5:00 o'clock, 8:00 o'clock, and 11:00 o'clock”). This was accompanied by the corresponding visual indication with a pointer or finger on the screen. Participants were required to maintain central fixation, but to pay attention to the cued locations. After 3 seconds, the presentations began (Fig. 1B). Stimuli were presented for 200 ms, coinciding with an auditory tone, after which the background was shown again while awaiting a response. A single-interval forced-choice procedure was used: the participant indicated through button presses on a keyboard whether the stimulus was seen or not seen. The forced-choice paradigm was also the reason for the auditory tone, which is different to SAP procedures, as it signaled to the subject to provide a ‘yes' or ‘no' response.
Reliability indices were measured for each participant. The Heijl-Krakau method was used to monitor fixation: 5% of trials within each trial were stimuli presented at locations corresponding to the physiologic blind spot.34 Cueing was not used for fixation checks, but the expected location of the blind spot was determined using the patient's HFA result. The patient knew that the blind spot would be tested, and was still instructed to press the button if they saw a stimulus, as this would provide further information regarding fixation losses. Thus, the proportion of ‘yes' responses to stimuli presented at that location was the number of fixation losses. False-positive and false-negative responses were gauged using the sub- and suprathreshold stimuli, respectively. Subthreshold stimuli were equivalent to the background luminance (i.e., Weber contrast of 0) but were still signaled by an auditory tone: responses of ‘yes' to these stimuli were regarded as ‘false-positives'. Suprathreshold stimuli were equivalent to the maximum output luminance of the screen: responses of ‘no' to these stimuli were regarded as ‘false-negatives'. In summary, trials in which the proportion of fixation losses was greater than 0.2, false negatives and false positives greater than 0.15 were excluded for analysis, similar to existing cut-offs used by the HFA.
Method of Constant Stimuli (MoCS) was used to randomly present stimuli at nine possible contrast levels for each combination of stimulus size, eccentricity, and number of points cued. The nine contrast levels were tailored specifically for each patient according to their sensitivities measured at the same location on the HFA. The step sizes between each contrast level were also modulated by stimulus size (i.e., GV had a smaller step size compared with GIII). Note that because of the differences in dynamic range of the stimulus, step sizes between the instrument and the laboratory set up, there were subtle differences in the contrast of the targets used in the laboratory-based phase of testing. Nonetheless, the patients with glaucoma had significantly reduced sensitivities at the locations of interest compared with the healthy subjects.
Due to the length of time required to measure FOS curves at each location, we only measured the responses and FOS curve obtained at one ‘test' location, which was specific to the participant (see below). FOS curves were constructed using at least 20 responses at each contrast level for each subject. Nine contrast levels were used at all other locations: two of these contrast levels were subthreshold, and the others were all suprathreshold. In particular, the greater instances of suprathreshold stimuli at test locations aside from the ‘test' location helps to maintain attention at other locations (distractor locations); dim or subthreshold contrast levels would not be able to play the same role. Each trial concluded once the ‘test' location had 10 responses per contrast level, irrespective of the number of presentations at all other distractor locations. The conditions were tested in random order, and were each conducted at least twice to obtain at least 20 total responses at each contrast level. Participants were given three practice trials before results were recorded. Rest breaks were given between every three trials to reduce fatigue.
As we have previously reported on the FOS curves of healthy subjects,17 we only tested two cueing conditions for the healthy subjects: one and eight points cued. This is because we wished only to obtain a basis for comparing the magnitude of sensitivity change following attentional cueing. As one and eight points represent the peak of the cueing effect and no cueing effect for healthy subjects, respectively, the difference in sensitivity obtained from these two conditions in our healthy group was used for comparison with the glaucoma patients. We otherwise tested the healthy subjects using the same conditions and methods as the glaucoma patients.
Test Location Selection
All healthy and glaucoma subjects were experienced psychophysical observers and had a long history of automated perimetry results. Using these results, we preselected a range of test locations for each participant. For the glaucoma patients, there were two categories of locations of interest.
First, we tested locations at which there was a statistically significant VF defect identified by the HFA. A statistically significant VF defect was defined as a point flagged at a level of at least P < 0.05 on the pattern deviation map on the HFA for GIII, the standard size used in clinical practice.
Second, we tested locations at which there was no statistically significant VF defect (i.e., a location with normal sensitivity [P > 0.05 on the HFA]). This was tested to determine whether the effects of cueing were specifically localized to a region of VF loss or if the effects had a more global effect upon visual perception even in areas of apparently normal vision. Additionally, locations with normal sensitivity were required to have the four immediately adjacent test locations (6° apart) to have normal sensitivity as well, as the junction of the scotoma in glaucoma may be poorly sampled in glaucoma, and we wished to rule out a potentially small region of defect.35 Following test point selection, other test locations for when two, four, or eight points cued were determined as per 180°, 90°, and 45° away from the test point, respectively, in order to maximize the amount of spatial uncertainty (Fig. 1A).
Although there may be slight variations in sensitivities measured at discrete test locations, previous studies have suggested the presence of ‘rings' of locations of identical contrast sensitivity (“isocontrast”) across the VF when using static perimetry techniques, such as the HFA, resembling that of kinetic perimetry isopters.36–38 Therefore, for the healthy group of subjects, we tested locations, which were within the same isocontrast ‘ring' as those examined in the glaucoma patients. Thus, comparisons of sensitivity change between glaucoma and healthy (healthy subjects and nondefective test locations in glaucoma) were performed at roughly the same eccentric locations.
Statistical Analysis
For the glaucoma patients, we plotted proportion seen as a function of contrast (log Weber contrast, ΔL/L) for each of the four combinations of test size (GIII or GV) and approximate test location (9.5° or 17.5°). We fitted FOS curves—the proportion of stimuli reported as seen (y-axis) as a function of log Weber contrast levels (x-axis)—using a sigmoidal nonlinear regression curve with variable slope (Version 7; GraphPad Prism, La Jolla, CA). To allow for a degree of false-negatives and false-positives (∼10% each) at each end of the FOS curve, we allowed the top and bottom to float between 0.9 and 1.0 and 0 and 0.1, respectively.39 From this, we extracted the point of subjective equivalence (PSE) because the present paradigm used a threshold frequency at 50% seen. The PSE was then averaged across all observers, as these represented magnitudes of values relative to each individual contrast level, and was compared across conditions using two-way ANOVA to determine the effect of number of points cued and stimulus size.
The slope parameter of the FOS curve was determined using two methods. In the first method, the x-axis was conveyed in terms of the modulated contrast steps used in the study (−6, −3, −2, −1, 0, +1, +2, +3, and +6) and the slope parameter was extracted directly from GraphPad Prism, representing the slope of the full function width (i.e., from lowest to highest contrast). The x-axis was converted into modulated contrast steps for two main reasons. First, the lower asymptote of the function was only defined by a small number of points under conditions of low uncertainty (e.g., 1 point cued) and is asymmetric about the threshold when log units were used. Second, the baseline contrast used across the subjects was different and thus affected the step size between data points along the x-axis. Comparisons between slopes obtained with differently scaled x-axes would not be informative. A higher slope value is indicative of a steeper slope (i.e., a shorter transition distance between seen and nonseen). A one-phase decay function (y = (y0 – l) × e−k*x + l) was fitted to each individual to provide a measure of the change in contrast sensitivity improvement with increasing number of points cued.
In addition to the use of the full function width, we also determined the slope parameter using the interquartile range (IQR) of the function. The IQR is defined as the contrast interval along the x-axis corresponding to 0.25 and 0.75 proportion seen, as calculated by the resultant sigmoidal nonlinear regression. The slope was then calculated by dividing the IQR by 0.5.
For the healthy subjects, the same FOS curves were plotted for the one and eight points cued conditions. The difference in sensitivity at the PSE between these two conditions was determined, and this difference (i.e., between least and maximum spatial uncertainty) served as a control comparison with the glaucoma patients.
Results
The Effect of Cueing on the Frequency-of-Seeing Curves in Glaucoma Patients
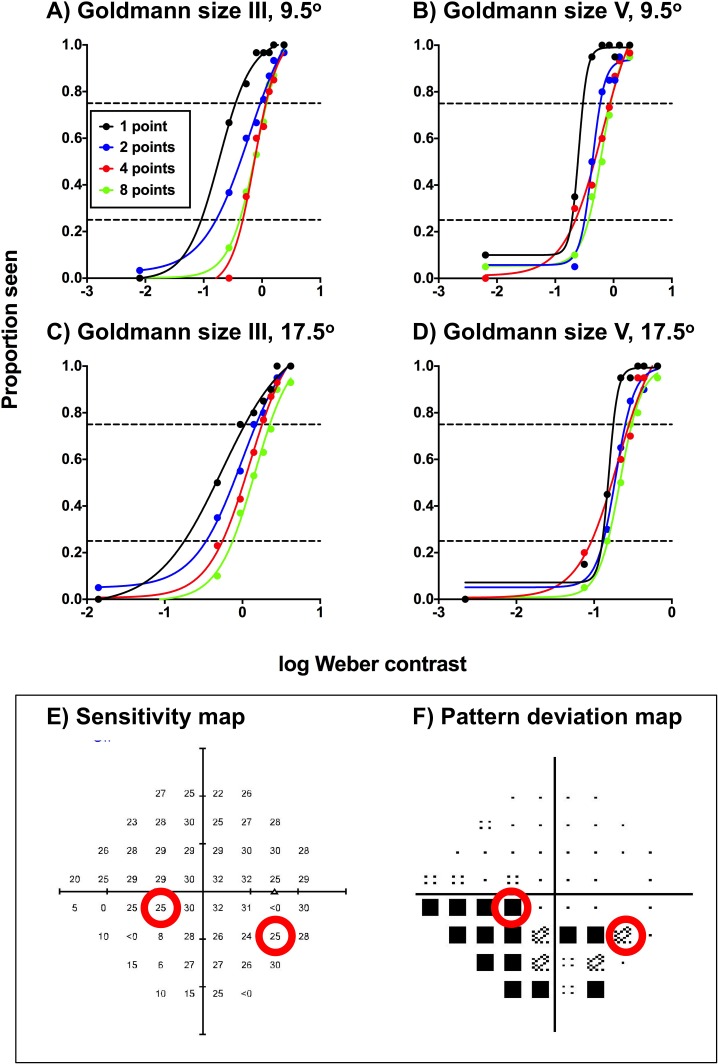
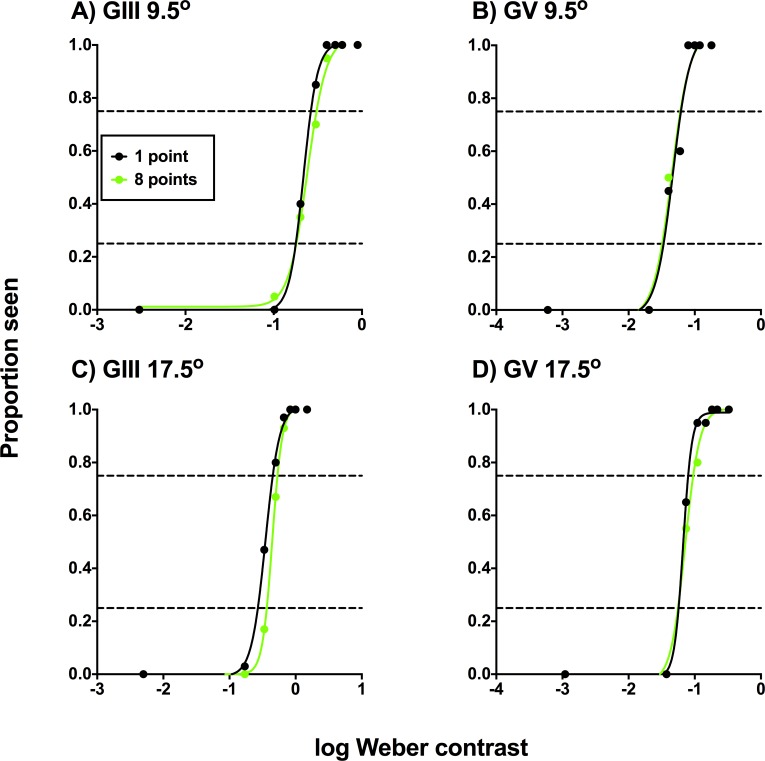
The FOS curves of a representative glaucoma patient are shown in Figure 2 for the four combinations of stimulus size (GIII and GV) and test eccentricity (9.5° and 17.5°). Although FOS curves are typically conveyed with a zone indicating the IQR, Figure 2 only shows the points of intersections with the functions at 0.25 and 0.75 proportion seen (i.e., the IQR) for clarity, due to the number of functions conveyed on the one panel. All other patients displayed similar effects at different magnitudes, depending on their individual contrast levels. When eight points were cued, the PSE was no different to baseline HFA sensitivity. Cueing a small number of points resulted in two main changes in the FOS curve in comparison to when eight points were cued: there was a steepening of the overall function (i.e., a reduction in uncertainty); and a leftward shift of the PSE (i.e., an improvement in sensitivity).
Figure 2.
Frequency-of-seeing curves for one of the glaucoma patients for the four test conditions (test size: GIII or GV; test location: 9.5° or 17.5° [A–D]) at locations with glaucomatous VF defects. The results for each cueing condition (1, 2, 4, or 8 points cued) are denoted by different colors. Each proportion seen value was generated using at least 20 presentations. Note that although nine contrast levels were tested, only eight are reported here, as the dimmest condition was equal to a Weber contrast of zero (hence, not shown on a logarithmic x-axis). The black dashed horizontal line indicates a proportion seen of 0.5, which was taken to be the threshold in the present study. The HFA sensitivity map (E) and pattern deviation map (F) are shown in the inset box, with the red circles corresponding to the test locations. Note that there were slight differences in the absolute sensitivities measured on the HFA and on the computer-based set up due to the adaptive thresholding algorithm used on the HFA (SITA-standard).
Cueing Improves Contrast Sensitivity at Locations With Glaucomatous Defects
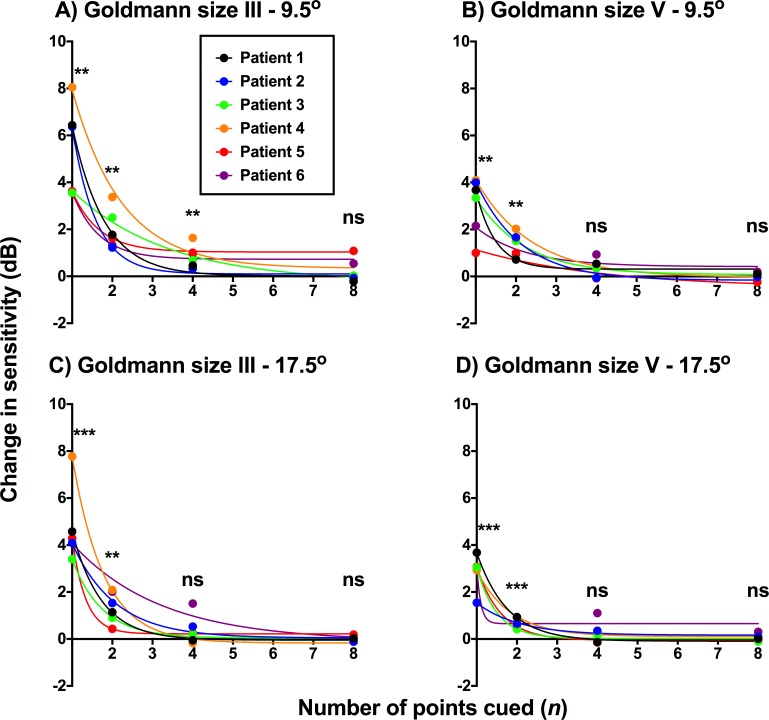
We plotted the difference between sensitivity level under cueing conditions (1, 2, 4, and 8 points cued) and baseline contrast sensitivity for each stimulus size and location condition for all six glaucoma patients (Fig. 3). There was an exponential decay in sensitivity with increasing number of points cued: a maximal effect was seen when only one point was cued to the subject, and no effect when eight points were cued. One-sample t-test showed sensitivity improvement to be significant when one or two points were cued for all conditions. The four points cued condition showed significant sensitivity improvement in the GIII 9.5° condition (Fig. 3A), but these improvements were much smaller than that found when only one point was cued. One-way ANOVA showed a significant effect of number of points cued (P < 0.0004 for all conditions). Multiple comparisons showed no significant difference between four and eight points cued conditions (average P value = 0.3174).
Figure 3.
Change in sensitivity from baseline (in dB) as a function of number of points cued (1–8) for the glaucoma patients. Each value along the y-axis was generated using at least 20 stimulus presentations for each patient under each condition. Note that zero is not shown on the x-axis, but rather, the x-axis begins at 1, as one point cued was the smallest condition. Each patient is depicted using a different colored point and decay function. The four test conditions are represented in separate panels. The asterisks indicate the level of significance of the one-sample t-test (difference from 0 dB; **P < 0.01; ***P < 0.001) and ns indicates no significant difference to 0 dB.
Although the test locations for all patients were within the same eccentricity ‘ring' from fixation, the baseline sensitivity differed significantly across subjects, and thus the amount of change in sensitivity would be individual to the patient (see below section on defect depth). For this reason, a two-way, rather than three-way, ANOVA was performed, examining the effect of the number of points cued and stimulus size. At the 9.5° test location, there were significant effects of the number of points cued (F3,30 = 56.98, P < 0.0001) and of test size (F1,10 = 10.18, P = 0.0096), as well as interaction effects (F3,30 = 4.062, P = 0.0155); a similar trend was found for the 17.5° test location.
Change in Slope Value With Attentional Cueing
Slope values of the FOS curves were determined first when the x-axis was converted to the modulating steps used in the present study, as described in the Methods section, and extracted from the full function width (Figs. 4A, 4B) and when calculated from the IQR (Figs. 4C, 4D). This allowed for pooling across all glaucoma subjects, as the baseline contrast levels, and hence the x-axis steps (in Weber contrast units) would be substantially different.
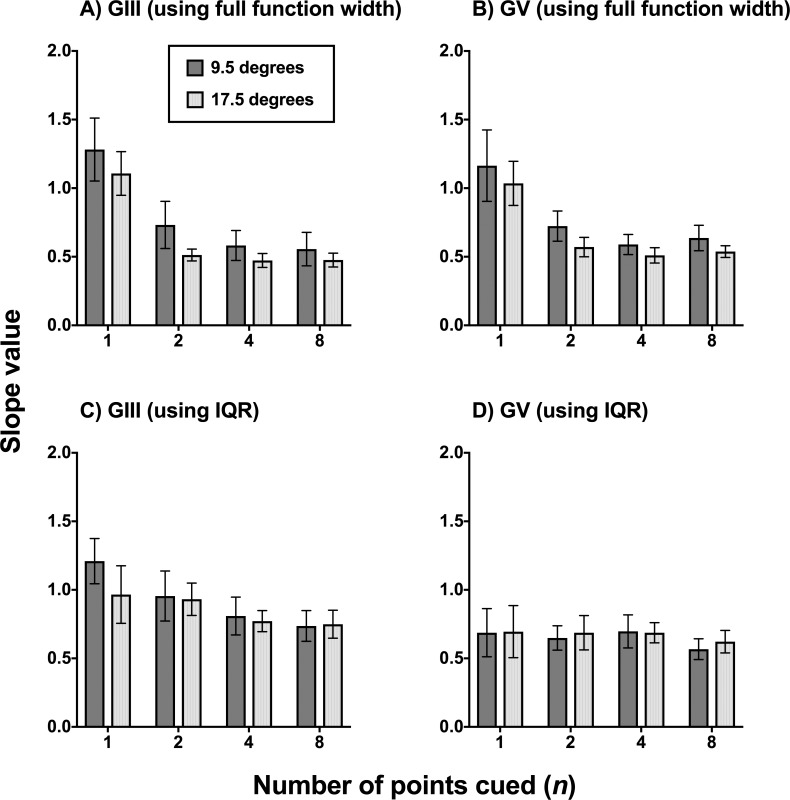
Figure 4.
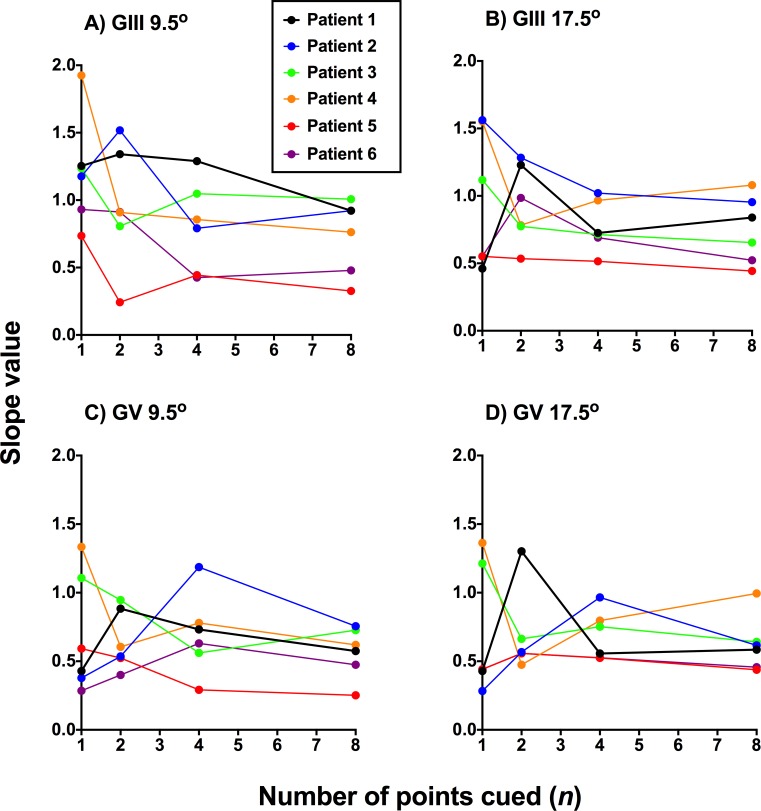
Slope value as a function of number of points cued when plotting the FOS curves of the glaucoma patients (n = 6) in proportion seen as a function of the contrast modulating steps used in the present study (−6, −3, −2, −1, 0, +1, +2, +3, and +6), equated for pooling and comparison across all six participants. A higher slope value is indicative of a steeper slope (i.e., a better defined junction between seen and nonseen). A smaller slope value indicates a flatter slope, which was taken to mean relatively greater uncertainty. Slopes values are expressed in mean and error bars indicate 1 SD. In (A, B), the slope value was extracted directly from GraphPad Prism using the full width of the nonlinear regression function. In (C, D), the slope was calculated using the IQR determined for each patient at the 0.25 and 0.75 proportion seen levels.
First, the effect of cueing was analyzed for GIII and GV separately when using the full function width (Figs. 4A, 4B). Two-way ANOVA showed a significant effect of number of points cued (GIII: F3,20 = 8.903, P = 0.0006; GV: F3,20 = 8.068, P = 0.0003) but no effect of test location (GIII: F1,20 = 3.568, P = 0.0735; GV: F1,20 = 1.637, P = 0.2081). Tukey's multiple comparison tests showed that the one point cued condition resulted in a significantly higher slope value than all other cued conditions (GIII and GV conditions average P value = 0.0028). All other pairwise comparisons showed no significant difference (GIII and GV conditions average P value = 0.9413). There was also no effect of stimulus size. These results suggest that the one point cued condition results in the greatest reduction in uncertainty.
However, the calculated slope using the IQR showed no significant effect of number of points cued (Figs. 4C, 4D). Although there was a tendency for one point cued to have a steeper slope when using GIII, this did not reach statistical significance (P = 0.2651). The slope values also appeared similar across all conditions when using GV.
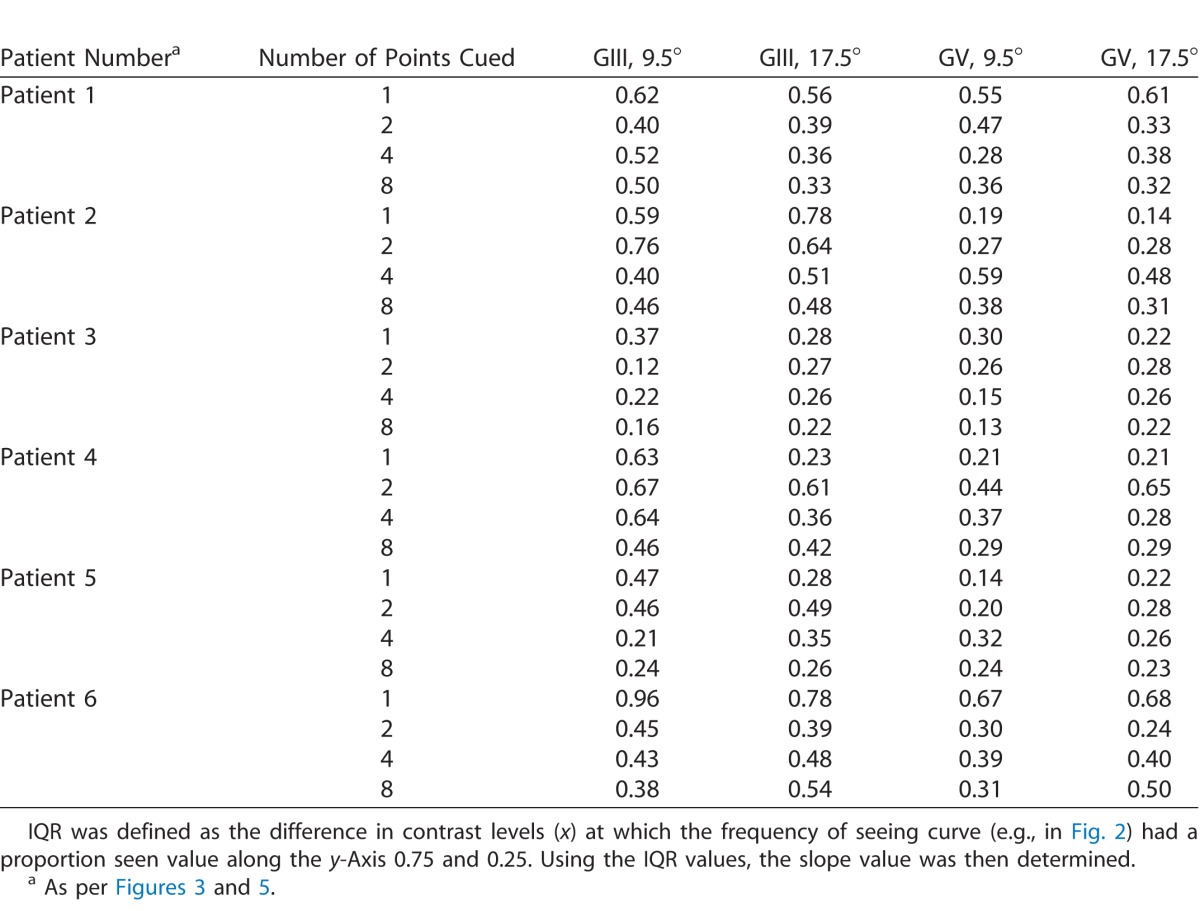
The individual slope values estimated using the IQR are shown in Figure 5, with the IQR values for each subject listed in the Table. Again, although there was a tendency for the one point cued condition to show the steepest slope, there were subjects which had different patterns of function steepness change at two and four points cued, which likely contributed to the overall lack of statistically significant effect.
Figure 5.
Slope values for individual glaucoma patients (n = 6) as a function of number of points cued (1–8) for each stimulus size and eccentricity condition. A higher slope value is indicative of a steeper slope (i.e., a better-defined junction between seen and nonseen). A smaller slope value indicates a flatter slope, which was taken to mean relatively greater uncertainty. The IQR values used to derive these individual slope values are shown in the Table.
Table.
Interquartile Ranges for Each Glaucoma Patient for 1, 2, 4, or 8 Points Cued When Tested With Each Size and Eccentricity Condition.
Sensitivity Improvement With Maximum Reduction in Spatial Uncertainty: Glaucoma Compared With Healthy Subjects
Here, the maximum reduction of uncertainty was defined as the difference in sensitivity between one and eight points cued condition. The results of the glaucoma patients and healthy subjects were compared under the four test conditions. As mentioned in the Methods, the test locations were specific to the individual subject, but were located at the same eccentric distance from fixation (i.e., representing locations with roughly similar sensitivity values).37
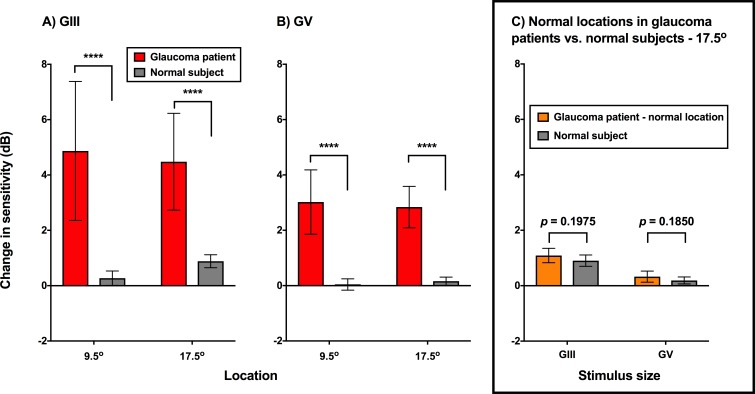
As expected, there were significant differences in the change in sensitivity between glaucoma patients and healthy subjects (Fig. 6). Under all test stimulus and location conditions, sensitivity increased significantly in patients with glaucoma compared with healthy subjects (P < 0.0001). The change in sensitivity in the healthy subjects was similar to that reported by Phu et al.,17 with a greater increase in sensitivity at the relatively more peripheral test location of 17.5° compared with 9.5° (a representative subject is shown in Fig. 7).
Figure 6.
Change in sensitivity (dB) when comparing the PSE of the FOS curves for one and eight points cued conditions for glaucoma patients (red) and healthy subjects (gray). GIII (A) and GV (B) are shown separately, with the test locations shown separately (9.5° and 17.5°). The asterisks indicate a difference at the level of P < 0.0001. In the inset (C), the normal locations of the glaucoma patients (orange) are compared with the healthy subjects (gray) at the same eccentricity (17.5°) for GIII and GV. Columns represent mean and the error bars indicate 1 SD.
Figure 7.
FOS curves for one of the healthy subjects for the four test conditions (test size: GIII or GV; test location: 9.5° or 17.5° [A–D]) as per Figure 2. The results for each cueing condition (1 or 8 points cued) are denoted by different colors. Each proportion seen value was generated using at least 20 presentations.
As the effect of cueing on sensitivity was greater at the 17.5° test location compared with 9.5°, we also tested locations at the 17.5° eccentricity with statistically normal sensitivity (P > 0.05 on the HFA pattern deviation map) in patients with glaucoma (Fig. 6C). These were compared with the results of the healthy subjects. There was no significant difference between the normal locations of glaucoma patients and the healthy subjects when using GIII (P = 0.1975) or GV (P = 0.1850) at the 17.5° test location. This suggests that the effect of cueing on the magnitude of sensitivity improvement is local to the region of defect, rather than being a global effect at locations of normal sensitivity in glaucoma patients.
Improvement in Contrast Sensitivity Correlates With Defect Depth
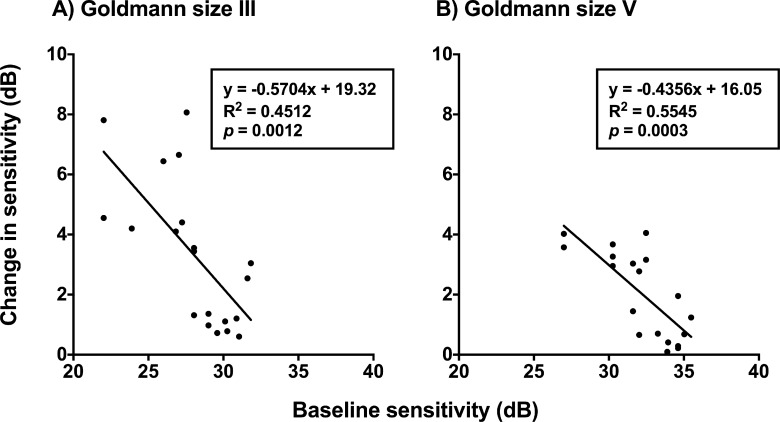
The improvement in sensitivity was plotted as a function of defect depth (in HFA dB) across all glaucoma patients when one point was cued (the least amount of spatial uncertainty; Fig. 8). The linear regression slope for GIII was significantly different to zero (R2 = 0.4445, P = 0.0013) and for GV, though the slope was slightly flatter (R2 = 0.5545, P = 0.0003), indicating less improvement with worsening baseline sensitivity. There was a modest relationship found when two points were cued for GIII (R2 = 0.2290, P = 0.0328), and there was no relationship when GV was used (R2 = 0.1418, P = 0.1236). As expected from the decaying effect of cueing seen in Figure 3, there was also no relationship found when four points were cued for GIII (P = 0.6811) or GV (P = 0.9690). Overall, these results indicated that as sensitivity reduced, there was a greater improvement in sensitivity when spatial uncertainty was reduced by cueing just one point.
Figure 8.
Change in sensitivity (difference between 1 and 8 points cued conditions, in dB) as a function of baseline sensitivity (dB). Goldmann size III (A) and Goldmann size V (B) are shown separately. Each datum point represents the result from one patient at one location. Linear regression slopes are shown with the analysis results shown in the insets.
Could the Improvement in Sensitivity be due to Errors in Fixation?
Aside from the cueing effect to reduce uncertainty, it was also possible that for conditions in which one point was cued, patients might alter their fixation to look toward that target, and thereby possibly using a different part of the retina that is normal with higher sensitivity. As the test locations are spaced 6° apart on the 24-2, it would mean that a fixation loss of at least 6° would be required to result an increase in sensitivity attributable to a different spatial location (e.g., that of a healthier part of the retina). Given that the size of the physiologic blind spot is approximately 6°, a fixation loss resulting in testing a different spatial location within the 24-2 test grid would likely manifest using the Heijl-Krakau method.
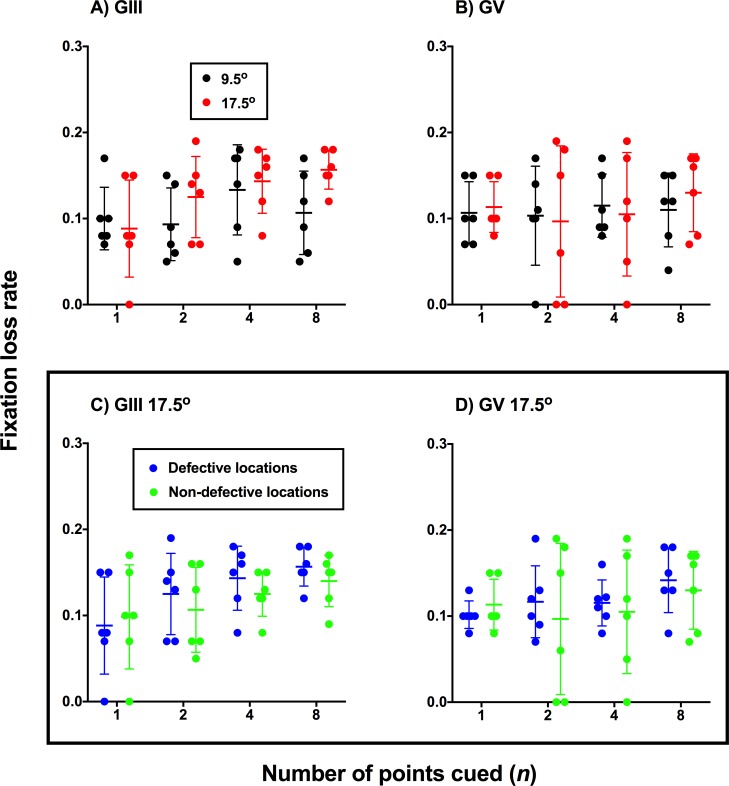
In the present study, we directly monitored fixation using methods similar to conventional fixation monitoring techniques in SAP: presentation of a stimulus at the physiologic blind spot, and whereby patient responses to those stimuli are indicative of a fixation loss. Here, if fixation loss rate was significantly elevated when one point was cued, and if a similar decaying trend was seen as a function of number of points cued, then this would suggest that the change in sensitivity may be due to a combination of fixation drift and attention. The results for all glaucoma patients are shown in Figure 9 where the fixation loss rate is plotted against different numbers of points cued. As shown in Figure 9, there was no significant effect of number of points cued (GIII: F3,20 = 2.005, P = 0.1457; GV: F3,20 = 2.165, P = 0.8838) or eccentricity (GIII: F1,20 = 3.455, P = 0.0778; GV: F1,20 = 0.034, P = 0.8553). Additionally, there was also no effect of whether the test location was defective or nondefective at the 17.5° eccentricity upon fixation loss rate using GIII (Fig. 9C, F1,20 = 1.672, P = 0.2108) or GV (Figure 8D, F1,20 = 0.3781, P = 0.5456).
Figure 9.
Fixation loss rate as a function of number of points cued for defective locations tested. In (A, B) the fixation loss rate was compared at the two test eccentricities (black, 9.5°; red, 17.5°) for GIII and GV. In (C, D), defective test points (blue) were compared with nondefective test points (green) at the same eccentricity (17.5°) for GIII and GV. Each point represents the result from one patient for each stimulus size, eccentricity, and number of points cued conditions. The line and error bars represent the mean and SD of the fixation loss rate for each condition.
Similarly, false-positives could be an index for apparent increase in sensitivity, as the trigger happy patient may indicate a ‘yes' response proportionally more times, even if the stimulus is below their sensitivity threshold. This could then potentially manifest as abnormally high sensitivity in the results, rather than the improvement being attributable to attentional factors. However, like with fixation loss measurements, we found no significant difference in false-positive rates when comparing defective (mean rates: GIII, 0.05 ± 0.04; GV, 0.03 ± 0.02) and nondefective locations (mean rates: 0.04 ± 0.04 vs. 0.06 ± 0.04; F1,10 = 0.1281, P = 0.7278) at the 17.5° eccentricity condition and when comparing different stimulus sizes (F1,10 = 0.8471, P = 0.3791). An alternative method for analyzing would be comparing sensitivities at cued glaucoma locations with directly adjacent values on the HFA test grid. However, this analysis was not performed due to differences in the thresholding algorithms (SITA used on the HFA, which modulates thresholds based on individual responses and in-built error functions and thus does not represent a true sensitivity result32) that may confound the comparison.
Discussion
The use of SAP devices for measuring sensitivity introduces spatial uncertainty through presentation of stimuli in pseudorandom order, such that the location of the stimulus appearance cannot be predicted.19,40 Other potential confounders such as mental load and divided attention are overcome by conducting the test in a controlled environment.41 Previous studies have highlighted the importance of maintaining attention throughout perimetric testing with a resultant improvement in reliability42 and performance17,18,43–45 by reducing spatial uncertainty. Conversely, divided attention worsens performance.41 As expected with one point cued being the smallest possible number of points to attend to within the VF, we found the greatest improvement in sensitivity using this condition. The exponential decay in performance and the number of points to the plateau of cueing effect in the present study is consistent with previous work in working memory, attentional load, and attentional allocation.45–49
In glaucoma, progressive loss of neural tissue leads to corresponding gradual functional impairment at localized regions of the VF, from defects that are detectable only with small stimuli, to end-stage disease where measurable thresholds are beyond that of the dynamic range of the instrument.11,12,33,50 Glaucoma therefore offers a model to measure contrast sensitivity at locations with different levels of VF defects. Specifically, locations with greater VF loss tend to also have increased variability as a result of the sparseness of the detector elements.6,23–25,51,52 In the present study, attentional cueing was able to improve sensitivity at regions with VF loss in glaucoma patients, not only under conditions of relatively high spatial uncertainty (a relatively smaller stimulus size, GIII, and in more peripheral test locations), but was also seen under conditions of low uncertainty (e.g., GV and more central test locations). The improvement did not appear to be eccentricity dependent, but was location dependent, specific to the depth of the VF defect at the test location.
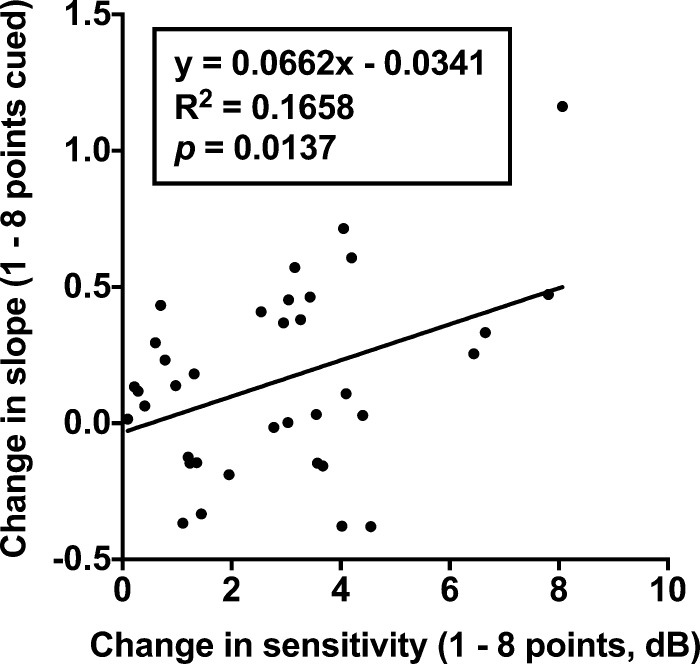
Interestingly, the slight steepening of the slope when cueing a GIII target appeared inconsistent with the work of Pelli53 and Cohn54 who found that the slope of the FOS curve flattened with increasing uncertainty (i.e., >1 element, which was the smallest number in our study). Instead, our results were more consistent with the findings of Henson et al.25 and Chauhan et al.51 who showed relative steepening of the FOS curve in glaucoma patients compared with normal subjects. One of the possible reasons for this is the method used to determine the FOS curve. Depending on the level of defect in glaucoma, the lower left side of the FOS curve, representing low-contrast targets, may remain flat or unchanged with cueing, especially if it is a severe VF defect. We did find that an increasing amount of sensitivity increase was correlated with a greater steepening of the slope when comparing one and eight points cued in glaucoma patients, suggesting that the change in slope may be dependent on the improvement in sensitivity (Fig. 10). Thus, instead of both a leftward and upward shift in the FOS curve with reduction of uncertainty, which manifests as flattening of the slope, we found a steepening of the curve due to a much larger improvement in the proportion seen at higher contrast levels compared with lower contrast levels (e.g., Fig. 2). A more extensive range of stimulus contrast levels would be further informative to characterize the slope.
Figure 10.
Change in slope as a function of change in sensitivity when comparing one and eight points cued conditions. Each datum point represents one glaucoma patient's result. All stimulus size and eccentricity conditions were pooled together as the x-axis represents change in sensitivity, irrespective of condition.
Changes in Sensitivity as a Function of Depth of Visual Field Defect
If the effect of uncertainty were uniform regardless of the depth of the VF defect, then it would be a matter of applying a uniform correction factor to thresholds to account for uncertainty. However, we found that the improvement in sensitivity systematically varied according to both the number of points cued and the depth of defect, suggesting a compounding effect of uncertainty with different levels of disease. Locations with only a mild defect on the HFA (e.g., 2–3 dB) had a small improvement in contrast sensitivity. This could still have a number of clinical implications. Threshold variability within the central VF tends to be smaller compared with more peripheral test locations,3,13 and thus, the normative distribution limits tend to be similarly narrow.12,37 Small improvements in sensitivity due to shifts in attention could mean the difference between the point being flagged as a statistically significant VF defect or not. As the defect progresses, there is an increase in the effect of cueing, with a concurrent increase in threshold variability. With a large improvement in contrast sensitivity with cueing, it is possible that the magnitude of defect can be overestimated. Errors in VF defect depth estimation may also confound progression analysis over time.55,56
Reduction of variability is desirable to measure progression of functional loss in disease over time.5,55,56 Sources of variability in threshold measurements may be multifactorial; however, the procedures and subjects in the present study were aimed at minimizing the effects of factors, such as reaction time, anticipation and habituation, procedural learning, internal noisiness, and criterion bias.5,23,57 Thus, elimination of spatial uncertainty, such as when one point was cued, could represent a more accurate level of contrast sensitivity as a result of neural loss, after discounting these other sources of variability. Further studies correlating sensitivity with underlying structural measurements would be informative. Correlations with structure could also test the effect of small eye movements, as different retinotopic locations may be tested with each stimulus presentation, potentially increasing test–retest variability.58,59
Although test–retest variability in sensitivity measurements is known to be elevated in patients with glaucoma,23,52 the present results are unlikely to be due to simple test–retest variability. Criterion bias was minimized in the present study using a forced-choice procedure and MoCS. The goodness-of-fit results of the FOS curves also suggest low variability in the responses of the subjects (the SD of the residuals were similar between locations with a VF defect and locations that had normal HFA sensitivity), suggesting no contamination by variability induced by disease. Finally, all glaucoma patients were highly experienced psychophysical observers (using both HFA- and iMac-based testing systems) and underwent four practice trials (each of 1 and 8 points cued using two different stimulus sizes) before recording results, and so factors such as procedural learning were unlikely to have played a role.57 An inexperienced or more variable cohort would provide different results for cueing. However, as the purpose of the study was to determine the contribution of spatial uncertainty in contrast sensitivity, we attempted to minimize other causes of variability.
The Effect of Attentional Cueing at Locations With Normal Sensitivities in Glaucoma Patients
The similarities in the magnitude of effect under the same conditions between locations without a VF defect in the present cohort of glaucoma patients and the healthy subjects support a localized, rather than global, effect of cueing. Instead, cueing effects were limited to areas of VF deficit in the glaucoma patients. Although the age of the healthy subjects was slightly lower than that of the glaucoma patients, there was no difference in the effect of attentional cueing in similar regions of normal sensitivity, suggesting that it was the local disease process, rather than any other cognitive impairment, which was the main driving force behind the effect of cueing on sensitivity in the present study.60,61
Attentional Cueing and Change in Sensitivity in Other Visual Tasks
Aside from having implications on the validity and accuracy of sensitivity measurement in glaucoma, the effect of cueing may be examined in visual search tasks. Glaucoma patients have worse performance in visual search tasks, which has been explained by impaired eye movements, fixation, and poor contrast sensitivity.62–64 It is possible that spatial uncertainty may also confound visual search, as the typical arrangement of targets and distractor elements introduces uncertainty.65 Therefore, adaptive strategies, such as changing visual scanning behavior and redirection of attention, could be useful in overcoming peripheral VF defects.66,67 These results may also have translational significance into activities of daily living. Areas with VF defects may benefit from cueing or covert attention to improve sensitivity,68 with the potential for improving certain task performance, such as driving, in which attention is routinely divided into many areas. Patients in whom central vision loss is present, such as in age-related macular degeneration, have also been shown to benefit from strategies incorporating directed attention and altered eye movement behavior to overcome deficits in visual function.69
Interestingly, there were smaller changes seen with GV compared with GIII in locations with VF defects. Similarly, there were also small and almost negligible changes in sensitivity in normal locations, especially under conditions of low spatial uncertainty (e.g., GV at a relatively central test location [9.5°]). This can be contrasted with recent work showing that cueing could improve performance even at locations close to the fovea,70 despite the normal area of attention focused upon the region of fixation.71 One reason for the discrepancy with our results is the paradigm used: we used a detection task that did not account for reaction time.70 Importantly, experiments measuring reaction time or signal detection may result in different attentional strategies, and hence results may not reflect exactly the same process.72 Given the role of response/reaction time in conventional SAP31,32 and the interest in reaction-time based perimetric tests,73 it may be useful to study different cueing paradigms and experimental procedures for their implications in perimetric testing of patients with disease.
Limitations
The glaucoma cohort in the present study consisted of patients with predominantly early stages of glaucomatous functional loss. Patients with a more severe stage of disease, a longer time since diagnosis, and more central VF loss may have further alterations in uncertainty, as they may have had more time to adapt to worse levels of visual function. A more diverse range of patients may be helpful in further characterizing this effect. This consecutive series of six patients, all of whom showed a depth-dependent effect of cueing on sensitivity, provides a basis for future testing.
The limited stimulus parameters in the present study were selected to resemble those used in SAP. In particular, a duration of 200 ms means that stimuli are operating outside of complete temporal summation74 but appear faster than that of a voluntary saccade. It is possible that longer stimulus durations may provide another cue. Deployment of an eye tracker could assist in monitoring eye movements, particularly for more prolonged stimulus exposures. Furthermore, although we tested whether fixation drifts were potentially responsible for the increases in sensitivity at regions of deficit compared with nondefective regions in the glaucoma patients, it remains to be seen whether fixation steadiness differ between different numbers of cued points and their relative positions. Recent studies have also suggested that the Heijl-Krakau method for fixation monitoring may be enhanced when used in conjunction with gaze tracking methods to provide better estimates of test reliability.75,76
We also used verbal cueing only, which is a form of endogenous cueing, wherein attention is voluntarily deployed and maintained without change for the duration of the task (a ‘top-down' effect).22 In contrast, visual cueing, especially during the course of the test, is an exogenous cue (also known as an involuntary cue), and may provide further reminders for the locus of attention.77 False exogenous cues may even impair performance if presented at locations that differ to the site of stimulus appearance.46 Preattentive vision and visual cues, such as alterations in orientation, motion, and color may operate in a similar manner to exogenous cueing, as such features or enhancements may increase the salience of the target.78 Specifically for glaucoma and other states of disease, preattentive visual cues and features require intact detector elements at the site of presentation in order to direct overt attention, and hence it may exhibit different contrast sensitivity changes to that of endogenous cueing.78 A ‘no cue' condition with the same number of points may provide information regarding the effect of learned anticipation by the subject, but this may additionally result in further confounding components of variability, such as criterion bias. Also, it remains to be seen whether covert attention to discrete areas suppress or impair performance at uncued locations, similar to the phenomenon of change blindness.79,80 Finally, it would be informative to also test the useful area or total region of cueing, as the appearance of the test stimuli did not vary or jitter within the cued regions.
Conclusions
In conclusion, spatial uncertainty in current SAP paradigms may be induced by not only stimulus parameters, but also disease. Although the present study used a computer-based system instead of a commercially available SAP instrument, these results are still likely to be relevant to other forms of threshold determination, such as SAP. Cues at locations within the VF using standard perimeters could be achieved verbally using custom test paradigms minimizing the number of possible test locations to obtain sensitivity values less contaminated by uncertainty. Implementation of visual cues may require further changes to instrument software design. A system for cueing points with suspicious or unreliable sensitivities in regions of deficit could also highlight the contribution of uncertainty over pathology in certain patients. Information about the effect of cueing and its interactions with subtle eye movements could inform developments in measurement of the VF, such as in children.81 The depth of defect appears to correlate with an improvement in sensitivity once uncertainty is reduced through attentional cueing, and is greater in the effect seen in healthy subjects. Thus, uncertainty may confound accurate determination of contrast sensitivity in SAP, especially in patients with disease.
Acknowledgments
Supported by grants through a PhD scholarship provided by Guide Dogs NSW/ACT and an Australian Government Research Training Program Scholarship (JP). This work was supported by the National Health and Medical Research Council of Australia (NHMRC #1033224). Guide Dogs NSW/ACT are partners in the NHMRC grant.
M. Kalloniatis and S. K. Khuu are named inventors on a patent involving the use of different Goldmann target sizes at different visual field locations for contrast sensitivity testing (International Publication Number WO 2014/094035 A1 (USA) and European Patent Number: 13865419.9).
Disclosure: J. Phu, None; M. Kalloniatis, P; S.K. Khuu, P
References
- 1. Jampel HD, Singh K, Lin SC, Chen TC, Francis BA, Hodapp E, Samples JR, Smith SD. . Assessment of visual function in glaucoma: a report by the American Academy of Ophthalmology. Ophthalmology. 2011; 118: 986– 1002. [DOI] [PubMed] [Google Scholar]
- 2. Phu J, Khuu SK, Yapp M, Assaad N, Hennessy MP, Kalloniatis M. . The value of visual field testing in the era of advanced imaging: clinical and psychophysical perspectives. Clin Exp Optom. 2017; 100: 313– 332. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 3. Heijl A, Lindgren G, Olsson J. . Normal variability of static perimetric threshold values across the central visual field. Arch Ophthalmol. 1987; 105: 1544– 1549. [DOI] [PubMed] [Google Scholar]
- 4. McKendrick AM. . Recent developments in perimetry: test stimuli and procedures. Clin Exp Optom. 2005; 88: 73– 80. [DOI] [PubMed] [Google Scholar]
- 5. Stewart WC, Hunt HH. . Threshold variation in automated perimetry. Surv Ophthalmol. 1993; 37: 353– 361. [DOI] [PubMed] [Google Scholar]
- 6. Gardiner SK, Swanson WH, Goren D, Mansberger SL, Demirel S. . Assessment of the reliability of standard automated perimetry in regions of glaucomatous damage. Ophthalmology. 2014; 121: 1359– 1369. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 7. Gardiner SK, Mansberger SL. . Effect of restricting perimetry testing algorithms to reliable sensitivities on test-retest variability. Invest Ophthalmol Vis Sci. 2016; 57: 5631– 5636. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 8. Wall M, Doyle CK, Zamba KD, Artes P, Johnson CA. . The repeatability of mean defect with size III and size V standard automated perimetry. Invest Ophthalmol Vis Sci. 2013; 54: 1345– 1351. [DOI] [PubMed] [Google Scholar]
- 9. Wall M, Brito CF, Woodward KR, Doyle CK, Kardon RH, Johnson CA. . Total deviation probability plots for stimulus size V perimetry: a comparison with size III stimuli. Arch Ophthalmol. 2008; 126: 473– 479. [DOI] [PubMed] [Google Scholar]
- 10. Wall M, Doyle CK, Eden T, Zamba KD, Johnson CA. . Size threshold perimetry performs as well as conventional automated perimetry with stimulus sizes III, V, and VI for glaucomatous loss. Invest Ophthalmol Vis Sci. 2013; 54: 3975– 3983. [DOI] [PubMed] [Google Scholar]
- 11. Kalloniatis M, Khuu SK. . Equating spatial summation in visual field testing reveals greater loss in optic nerve disease. Ophthalmic Physiol Opt. 2016; 36: 439– 452. [DOI] [PubMed] [Google Scholar]
- 12. Phu J, Khuu SK, Zangerl B, Kalloniatis M. . A comparison of Goldmann III, V and spatially equated test stimuli in visual field testing: the importance of complete and partial spatial summation. Ophthalmic Physiol Opt. 2017; 37: 160– 176. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13. Khuu SK, Kalloniatis M. . Standard automated perimetry: determining spatial summation and its effect on contrast sensitivity across the visual field. Invest Ophthalmol Vis Sci. 2015; 56: 3565– 3576. [DOI] [PubMed] [Google Scholar]
- 14. Gilpin LB, Stewart WC, Hunt HH, Broom CD. . Threshold variability using different Goldmann stimulus sizes. Acta Ophthalmol (Copenh). 1990; 68: 674– 676. [DOI] [PubMed] [Google Scholar]
- 15. Wall M, Woodward KR, Doyle CK, Zamba G. . The effective dynamic ranges of standard automated perimetry sizes III and V and motion and matrix perimetry. Arch Ophthalmol. 2010; 128: 570– 576. [DOI] [PubMed] [Google Scholar]
- 16. Anderson RS, McDowell DR, Ennis FA. . Effect of localized defocus on detection thresholds for different sized targets in the fovea and periphery. Acta Ophthalmol Scand. 2001; 79: 60– 63. [DOI] [PubMed] [Google Scholar]
- 17. Phu J, Kalloniatis M, Khuu SK. . The effect of attentional cueing and spatial uncertainty in visual field testing. PLoS One. 2016; 11: e0150922. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18. Khuu SK, Kalloniatis M. . Spatial summation across the central visual field: implications for visual field testing. J Vis. 2015; 15 1: 15.1.6. [DOI] [PubMed] [Google Scholar]
- 19. Fankhauser F. . Problems related to the design of automatic perimeters. Doc Ophthalmol. 1979; 47: 89– 139. [DOI] [PubMed] [Google Scholar]
- 20. Rose A. . The sensitivity performance of the human eye on an absolute scale. J Opt Soc Am. 1948; 38: 196– 208. [DOI] [PubMed] [Google Scholar]
- 21. Tanner WP., Jr. Physiological implications of psychophysical data. Ann N Y Acad Sci. 1961; 89: 752– 765. [DOI] [PubMed] [Google Scholar]
- 22. Carrasco M. . Visual attention: the past 25 years. Vision Res. 2011; 51: 1484– 1525. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 23. Bengtsson B, Heijl A. . False-negative responses in glaucoma perimetry: indicators of patient performance or test reliability? Invest Ophthalmol Vis Sci. 2000; 41: 2201– 2204. [PubMed] [Google Scholar]
- 24. Gardiner SK, Swanson WH, Demirel S. . The effect of limiting the range of perimetric sensitivities on pointwise assessment of visual field progression in glaucoma. Invest Ophthalmol Vis Sci. 2016; 57: 288– 294. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 25. Henson DB, Chaudry S, Artes PH, Faragher EB, Ansons A. . Response variability in the visual field: comparison of optic neuritis, glaucoma, ocular hypertension, and normal eyes. Invest Ophthalmol Vis Sci. 2000; 41: 417– 421. [PubMed] [Google Scholar]
- 26. Pan F, Swanson WH, Dul MW. . Evaluation of a two-stage neural model of glaucomatous defect: an approach to reduce test-retest variability. Optom Vis Sci. 2006; 83: 499– 511. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 27. Crabb DP, Smith ND, Glen FC, Burton R, Garway-Heath DF. . How does glaucoma look?: patient perception of visual field loss. Ophthalmology. 2013; 120: 1120– 1126. [DOI] [PubMed] [Google Scholar]
- 28. Hoste AM. . New insights into the subjective perception of visual field defects. Bull Soc Belge Ophtalmol. 2003: 65– 71. [PubMed]
- 29. Redmond T, Garway-Heath DF, Zlatkova MB, Anderson RS. . Sensitivity loss in early glaucoma can be mapped to an enlargement of the area of complete spatial summation. Invest Ophthalmol Vis Sci. 2010; 51: 6540– 6548. [DOI] [PubMed] [Google Scholar]
- 30. Mills RP, Budenz DL, Lee PP,et al. . Categorizing the stage of glaucoma from pre-diagnosis to end-stage disease. Am J Ophthalmol. 2006; 141: 24– 30. [DOI] [PubMed] [Google Scholar]
- 31. Bengtsson B, Olsson J, Heijl A, Rootzen H. . A new generation of algorithms for computerized threshold perimetry, SITA. Acta Ophthalmol Scand. 1997; 75: 368– 375. [DOI] [PubMed] [Google Scholar]
- 32. Bengtsson B, Heijl A. . Evaluation of a new perimetric threshold strategy, SITA, in patients with manifest and suspect glaucoma. Acta Ophthalmol Scand. 1998; 76: 268– 272. [DOI] [PubMed] [Google Scholar]
- 33. Zalta AH. . Use of a central 10 degrees field and size V stimulus to evaluate and monitor small central islands of vision in end stage glaucoma. Br J Ophthalmol. 1991; 75: 151– 154. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 34. Asman P, Fingeret M, Robin A,et al. . Kinetic and static fixation methods in automated threshold perimetry. J Glaucoma. 1999; 8: 290– 296. [PubMed] [Google Scholar]
- 35. Haefliger IO, Flammer J. . Fluctuation of the differential light threshold at the border of absolute scotomas. Comparison between glaucomatous visual field defects and blind spots. Ophthalmology. 1991; 98: 1529– 1532. [DOI] [PubMed] [Google Scholar]
- 36. Lachenmayr BJ, Kiermeir U, Kojetinsky S. . Points of a normal visual field are not statistically independent. Ger J Ophthalmol. 1995; 4: 175– 181. [PubMed] [Google Scholar]
- 37. Phu J, Khuu SK, Nivison-Smith L,et al. . Pattern recognition analysis reveals unique contrast sensitivity isocontours using static perimetry thresholds across the visual field. Invest Ophthalmol Vis Sci. 2017; 58: 4863– 4876. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38. Phu J, Al-Saleem N, Kalloniatis M, Khuu SK. . Physiologic statokinetic dissociation is eliminated by equating static and kinetic perimetry testing procedures. J Vis. 2016; 16 14: 5. [DOI] [PubMed] [Google Scholar]
- 39. Wichmann FA, Hill NJ. . The psychometric function: I. Fitting, sampling, and goodness of fit. Percept Psychophys. 2001; 63: 1293– 1313. [DOI] [PubMed] [Google Scholar]
- 40. Fankhauser F, Koch P, Roulier A. . On automation of perimetry. Albrecht Von Graefes Arch Klin Exp Ophthalmol. 1972; 184: 126– 150. [DOI] [PubMed] [Google Scholar]
- 41. Wall M, Woodward KR, Brito CF. . The effect of attention on conventional automated perimetry and luminance size threshold perimetry. Invest Ophthalmol Vis Sci. 2004; 45: 342– 350. [DOI] [PubMed] [Google Scholar]
- 42. Miranda MA, Henson DB. . Perimetric sensitivity and response variability in glaucoma with single-stimulus automated perimetry and multiple-stimulus perimetry with verbal feedback. Acta Ophthalmol. 2008; 86: 202– 206. [DOI] [PubMed] [Google Scholar]
- 43. Gould IC, Wolfgang BJ, Smith PL. . Spatial uncertainty explains exogenous and endogenous attentional cuing effects in visual signal detection. J Vis. 2007; 7 13: 4.1– 17. [DOI] [PubMed] [Google Scholar]
- 44. Posner MI, Snyder CR, Davidson BJ. . Attention and the detection of signals. J Exp Psychol. 1980; 109: 160– 174. [PubMed] [Google Scholar]
- 45. Close A, Sapir A, Burnett K, d'Avossa G. . Attention to multiple locations is limited by spatial working memory capacity. J Vis. 2014; 14 9. [DOI] [PubMed] [Google Scholar]
- 46. Pestilli F, Carrasco M. . Attention enhances contrast sensitivity at cued and impairs it at uncued locations. Vision Res. 2005; 45: 1867– 1875. [DOI] [PubMed] [Google Scholar]
- 47. Luck SJ, Vogel EK. . The capacity of visual working memory for features and conjunctions. Nature. 1997; 390: 279– 281. [DOI] [PubMed] [Google Scholar]
- 48. Zhang W, Luck SJ. . Discrete fixed-resolution representations in visual working memory. Nature. 2008; 453: 233– 235. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 49. Verghese P. . Visual search and attention: a signal detection theory approach. Neuron. 2001; 31: 523– 535. [DOI] [PubMed] [Google Scholar]
- 50. Zalta AH, Burchfield JC. . Detecting early glaucomatous field defects with the size I stimulus and Statpac. Br J Ophthalmol. 1990; 74: 289– 293. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 51. Chauhan BC, Tompkins JD, LeBlanc RP, McCormick TA. . Characteristics of frequency-of-seeing curves in normal subjects, patients with suspected glaucoma, and patients with glaucoma. Invest Ophthalmol Vis Sci. 1993; 34: 3534– 540. [PubMed] [Google Scholar]
- 52. Gardiner SK, Demirel S, Johnson CA. . Modeling the sensitivity to variability relationship in perimetry. Vision Res. 2006; 46: 1732– 1745. [DOI] [PubMed] [Google Scholar]
- 53. Pelli DG. . Uncertainty explains many aspects of visual contrast detection and discrimination. J Opt Soc Am. 1985; 2: 1508– 1532. [DOI] [PubMed] [Google Scholar]
- 54. Cohn TE. . Absolute threshold: analysis in terms of uncertainty. J Opt Soc Am. 1981; 71: 783– 785. [DOI] [PubMed] [Google Scholar]
- 55. Spry PG, Johnson CA. . Identification of progressive glaucomatous visual field loss. Surv Ophthalmol. 2002; 47: 158– 173. [DOI] [PubMed] [Google Scholar]
- 56. Turpin A, McKendrick AM. . What reduction in standard automated perimetry variability would improve the detection of visual field progression? Invest Ophthalmol Vis Sci. 2011; 52: 3237– 3245. [DOI] [PubMed] [Google Scholar]
- 57. Werner EB, Adelson A, Krupin T. . Effect of patient experience on the results of automated perimetry in clinically stable glaucoma patients. Ophthalmology. 1988; 95: 764– 767. [DOI] [PubMed] [Google Scholar]
- 58. Henson DB, Evans J, Chauhan BC, Lane C. . Influence of fixation accuracy on threshold variability in patients with open angle glaucoma. Invest Ophthalmol Vis Sci. 1996; 37: 444– 450. [PubMed] [Google Scholar]
- 59. Wyatt HJ, Dul MW, Swanson WH. . Variability of visual field measurements is correlated with the gradient of visual sensitivity. Vision Res. 2007; 47: 925– 936. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 60. Harrabi H, Kergoat MJ, Rousseau J,et al. . Age-related eye disease and cognitive function. Invest Ophthalmol Vis Sci. 2015; 56: 1217– 1221. [DOI] [PubMed] [Google Scholar]
- 61. Yochim BP, Mueller AE, Kane KD, Kahook MY. . Prevalence of cognitive impairment, depression, and anxiety symptoms among older adults with glaucoma. J Glaucoma. 2012; 21: 250– 254. [DOI] [PubMed] [Google Scholar]
- 62. Smith ND, Glen FC, Crabb DP. . Eye movements during visual search in patients with glaucoma. BMC Ophthalmol. 2012; 12: 45. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 63. Smith ND, Crabb DP, Glen FC, Burton R, Garway-Heath DF. . Eye movements in patients with glaucoma when viewing images of everyday scenes. Seeing Perceiving. 2012; 25: 471– 492. [DOI] [PubMed] [Google Scholar]
- 64. Burton R, Crabb DP, Smith ND, Glen FC, Garway-Heath DF. . Glaucoma and reading: exploring the effects of contrast lowering of text. Optom Vis Sci. 2012; 89: 1282– 1287. [DOI] [PubMed] [Google Scholar]
- 65. Cosman JD, Vecera SP. . The contents of visual working memory reduce uncertainty during visual search. Atten Percept Psychophys. 2011; 73: 996– 1002. [DOI] [PubMed] [Google Scholar]
- 66. Kubler TC, Kasneci E, Rosenstiel W,et al. . Driving with glaucoma: task performance and gaze movements. Optom Vis Sci. 2015; 92: 1037– 1046. [DOI] [PubMed] [Google Scholar]
- 67. Glen FC, Crabb DP. . Living with glaucoma: a qualitative study of functional implications and patients' coping behaviours. BMC Ophthalmol. 2015; 15: 128. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 68. Alberti CF, Horowitz T, Bronstad PM, Bowers AR. . Visual attention measures predict pedestrian detection in central field loss: a pilot study. PLoS One. 2014; 9: e89381. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 69. Lingnau A, Schwarzbach J, Vorberg D. . Adaptive strategies for reading with a forced retinal location. J Vis. 2008; 8 5: 6.1– 18. [DOI] [PubMed] [Google Scholar]
- 70. Yang T, Zhang J, Bao Y. . Spatial orienting around the fovea: exogenous and endogenous cueing effects. Cogn Process. 2015; 16 Suppl 1: 137– 141. [DOI] [PubMed] [Google Scholar]
- 71. Lavidor M, Walsh V. . The nature of foveal representation. Nat Rev Neurosci. 2004; 5: 729– 735. [DOI] [PubMed] [Google Scholar]
- 72. Handy TC, Kingstone A, Mangun GR. . Spatial distribution of visual attention: perceptual sensitivity and response latency. Percept Psychophys. 1996; 58: 613– 627. [DOI] [PubMed] [Google Scholar]
- 73. Murray IC, Perperidis A, Cameron LA,et al. . Comparison of saccadic vector optokinetic perimetry and standard automated perimetry in glaucoma. Part I: threshold values and repeatability. Transl Vis Sci Technol. 2017; 6 5: 3. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 74. Mulholland PJ, Redmond T, Garway-Heath DF, Zlatkova MB, Anderson RS. . Spatiotemporal summation of perimetric stimuli in early glaucoma. Invest Ophthalmol Vis Sci. 2015; 56: 6473– 6482. [DOI] [PubMed] [Google Scholar]
- 75. Ishiyama Y, Murata H, Asaoka R. . The usefulness of gaze tracking as an index of visual field reliability in glaucoma patients. Invest Ophthalmol Vis Sci. 2015; 56: 6233– 6236. [DOI] [PubMed] [Google Scholar]
- 76. Ishiyama Y, Murata H, Mayama C, Asaoka R. . An objective evaluation of gaze tracking in Humphrey perimetry and the relation with the reproducibility of visual fields: a pilot study in glaucoma. Invest Ophthalmol Vis Sci. 2014; 55: 8149– 8152. [DOI] [PubMed] [Google Scholar]
- 77. Coull JT, Nobre AC. . Where and when to pay attention: the neural systems for directing attention to spatial locations and to time intervals as revealed by both PET and fMRI. J Neurosci. 1998; 18: 7426– 7435. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 78. Loughman J, Davison P, Flitcroft I. . Open angle glaucoma effects on preattentive visual search efficiency for flicker, motion displacement and orientation pop-out tasks. Br J Ophthalmol. 2007; 91: 1493– 1498. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 79. Rensink RA. . Change detection. Annu Rev Psychol. 2002; 53: 245– 277. [DOI] [PubMed] [Google Scholar]
- 80. Simons DJ. . Attentional capture and inattentional blindness. Trends Cogn Sci. 2000; 4: 147– 155. [DOI] [PubMed] [Google Scholar]
- 81. Murray I, Perperidis A, Brash H,et al. . Saccadic Vector Optokinetic Perimetry (SVOP): a novel technique for automated static perimetry in children using eye tracking. Conf Proc IEEE Eng Med Biol Soc. 2013; 2013: 3186– 3189. [DOI] [PubMed] [Google Scholar]