Lithography-Free Fabrication of Reconfigurable Substrate Topography For Contact Guidance

Abstract

Mammalian cells detect and respond to topographical cues presented in natural and synthetic biomaterials both in vivo and in vitro. Micro- and nano-structures influence the adhesion, morphology, proliferation, migration, and differentiation of many phenotypes. Although the mechanisms that underpin cell-topography interactions remain elusive, synthetic substrates with well-defined micro- and nano-structures are important tools to elucidate the origin of these responses. Substrates with reconfigurable topography are desirable because programmable cues can be harmonized with dynamic cellular responses. Here we present a lithography-free fabrication technique that can reversibly present topographical cues using an actuation mechanism that minimizes the confounding effects of applied stimuli. This method utilizes strain-induced buckling instabilities in bi-layer substrate materials with rigid uniform silicon oxide membranes that are thermally deposited on elastomeric substrates. The resulting surfaces are capable of reversible of substrates between three distinct states: flat substrates (A = 1.53 ± 0.55 nm, Rms = 0.317 ± 0.048 nm); parallel wavy grating arrays (A_|| = 483.6 ± 7.8 nm and λ_|| = 4.78 ± 0.16 μm); perpendicular wavy grating arrays (A_⊥ = 429.3 ± 5.8 nm; λ_⊥ = 4.95 ± 0.36 μm). The cytoskeleton dynamics of 3T3 fibroblasts in response to these surfaces was measured using optical microscopy. Fibroblasts cultured on dynamic substrates that are switched from flat to topographic features (FLAT-WAVY) exhibit a robust and rapid change in gross morphology as measured by a reduction in circularity from 0.30 ± 0.13 to 0.15 ± 0.08 after 5 min. Conversely, dynamic substrate sequences of FLAT-WAVY-FLAT do not significantly alter the gross steady-state morphology. Taken together, substrates that present topographic structures reversibly can elucidate dynamic aspects of cell-topography interactions.

Introduction

Mammalian cells can detect the topography of biomaterials in both natural and synthetic microenvironments [1–5]. Topography plays an important role in determining the collective cell behavior in many complex biological processes in development, wound healing, and tissue regeneration [1,4,6–11]. Topographical cues control fundamental cellular functions including adhesion, migration, proliferation, and differentiation [12–16]. Most phenomenological studies that correlate cell function with feature geometry and size employ substrates with static structures [7,17–25]. Static surfaces can extract many complex mechanisms that underpin cell-materials interactions such as contact guidance. However, there is a limit to the insight that can be gained by interrogating dynamic systems with static cues. Substrates that present topography with spatiotemporal control are advantageous in studying cell-materials interactions including contact guidance. They can potentially decouple contact guidance responses from other confounding processes such as cell attachment and spreading [5,26].

Controlled presentation of topographical cues has improved through recent advances in stimuli-responsive materials [27,28] precise delivery of stimuli such as temperature changes, light, or mechanical strain [1,4,10,16,20,28,29]. This strategy has been used for dynamic microstructure presentation to study cell-materials interactions in numerous contexts [1,3,5,30]. Programmable topography can also be engineered using stimuli-responsive polymers including those that respond to cues such as electric fields, temperature changes, and enzymes [3,5,27,31,32].

The introduction of external stimuli may affect baseline metabolism, viability, or proliferation of cell populations [33]. For example, changes in temperature, the presence of enzymes, or irradiated light can impact basal cell function [3,33]. Mechanical stimuli via direct application of strain is advantageous for topographic feature formation because it is rapid, robust, and facile [1,5]. Most currently available methods that use strain-induced topography require uniaxial strains of greater than 10% [1,26,34]. Mammalian cells can detect strains of the underlying substrate as small as 3.5% and respond to these stains by altering their morphology [35,36]. Therefore, inducing topographic features using mechanical strains >3.5% may convolve contact guidance with responses to substrate deformation. Strain-induced topography will ideally utilize mechanical stimuli that are below the lower limit of detection for mammalian cells. Furthermore, mechanical stimuli can be coupled with materials that can reversibly present homogeneous topographical cues in various orientations. Herein we report a lithography-free fabrication technique that is capable of producing strain-induced topography using sub-threshold uniaxial strains. Cytoskeleton morphodynamics in fibroblasts are measured using these model surfaces.

Materials & Methods

Fabrication and Characterization of Programmable Dynamic Topography

Elastomeric substrates were prepared using polydimethylsiloxane (PDMS, Sylgard 184, Dow Corning, Midland, MI USA) cured in a 10:1 ratio at 75 °C. Rectangular PDMS coupons (W x L x H = 1.5 cm x 3.5 cm x 600 μm) were mounted in a custom fixation device and strained to the desired amount. Pre-strained substrates were coated with 100 nm of silicon oxide (SiO_x) deposited by thermal evaporation. Briefly, silicon monoxide (SiO) was thermally deposited at a rate of 1 Å/sec at 10⁻⁶ Torr (NexDep, Angstrom Engineering, Kitchener, ON Canada). All substrates were processed in an identical manner with the exception that the thickness of the bi-layer membrane was set at either 10 or 100 nm. Dynamic topography sequences were switched between states in less than 3 sec. Microstructures of bilayer membranes were characterized using optical microscopy (Olympus BH2 microscope, Olympus America Inc., Center Valley, PA USA) and atomic force microscopy (AFM, Dimension 3100 SPM, Veeco, Plainville, NY USA). Fourier transforms of optical micrographs were prepared using ImageJ (National Institute of Health, USA, available at http://rsb.info.nih.gov/ij).

Fibroblast Culture and Imaging

All cell culture supplies were purchased from Invitrogen (Carlsbad, CA USA) unless otherwise stated. Bi-layer membranes were sterilized in ethanol (70% v/v) and irradiated with UV for 30 min. Bi-layer membranes were incubated with RGD solution (50 μg/cm²) for 40 min and rinsed with 3x PBS. NIH 3T3 fibroblasts (ATCC, Monassas, VA USA) were seeded at densities of 25,000 cells/cm² and incubated in DMEM medium supplemented with 10% fetal bovine serum (FBS) and 1% penicillin/streptomycin (P/S) at 37 °C. Live cell images were performed by culturing cells for 24 hr prior to dynamic topography sequences. Cells were cultured in en environmentally controlled chamber set at 37 °C with >90% relative humidity and 5% CO₂ (BioImager, Mississauga, ON Canada). FB were imaged in phase contrast and analyzed using NIH ImageJ to measure morphology and relative orientation. Cell circularity was calculated using the following expression.

$$C_{\mathit{cell}}=\frac{4\pi A_{\mathit{proj}}}{P^2}$$ Eqn. 1

where A_proj and P are the projected surface area and perimeter of the cell, respectively. The axial ratio R_axis was calculated from the length of the major axis divided by the length of the minor axis (Figure S1) [37].

Cells were fixed in 4% formaldehyde for 20 min, stained using 20 μL of Alexa Fluor® 488 Phalloidin (200 U/mL), and counterstained with SlowFade® Gold Antifade Reagent with DAPI. Phase contrast and fluorescent images were recorded using an EvosFL microscope (Advanced Microscopy Group, Bothell, WA USA). FB on a static substrate were also imaged using confocal fluorescence microscopy (LSM 510 META DuoScan, Carl Zeiss, Heidelberg Germany) and scanning electron microscopy (SEM) (PhilipsXL-30 FEG, FEI, Hillsboro, OR, USA). Cells dedicated for SEM imaging were fixed in 4% formaldehyde for 20 min, washed with distilled water for 3 times, and dehydrated in a series of mixtures containing ethanol and hexamethyldisilazane (HDMS), as previously described [38]. Dehydrated samples were coated with 4 nm of platinum prior to imaging (Emtech K575X, Quorum Technologies, Guelph, ON, Canada).

Statistical Methods

Cell morphodynamic measurements were based on at least 100 cells per each data point and all experiments were repeated in triplicate. All data is shown as mean ± s. e. m. unless otherwise stated. A student’s t-test with p-value of 0.05 was performed to consider significant difference of two groups with statistical significance set at p-values of < 0.05. One-way ANOVA with Tukey post-hoc criterion was used to assess the significance across more than 2 groups.

Results and Discussion

Microstructural Characterization of Dynamic Topography

Strain-induced feature formation is a convenient strategy for rapid programmable presentation of topographic cues. Ordered buckling is an energy-relief mechanism that occurs when a thin rigid membrane on an elastomeric substrate is compressed [39,40]. Releasing the pre-strain of bi-layer substrates produces grating arrays composed of ridge-groove features with short-range order (Figure 3) [41]. This phenomenon has been used as a non-conventional microfabrication technique [42]. Rigid silicon oxide (SiO₂) membranes on polydimethylsiloxane (PDMS) substrates are commonly fabricated by exposing PDMS to O₂ plasma [43,44]. PDMS-SiO₂ bilayers fabricated in this manner typically require large uniaxial strains (ε > 10%) to create grating arrays [43,45]. One possible explanation is that the minimum thickness of SiO₂ membranes processed using O₂ plasma is large because a significant depth of the PDMS must be converted into SiO₂ before a strain-sensitive percolating network of oxide structures is formed within the substrate. The alternative method described herein uses thermal evaporation of SiO to deposit homogeneous SiO_x (with 1.5 < x < 2) film apical to PDMS substrates [46]. This approach reduces the effective critical strain required for feature formation via compression. Although the exact composition of SiO_x rigid membranes depends upon the deposition procedure, the composition will hereby be referred to as SiO₂ for simplification.

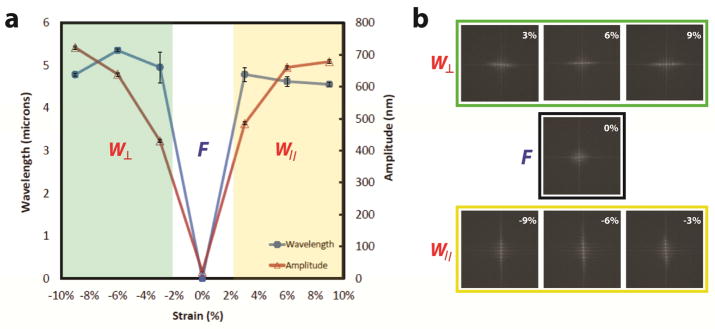
Figure 3.

PDMS-SiO₂ bilayer substrates produce reconfigurable programmable topography. PDMS-SiO₂ bilayer substrates are pre-strained to ε_pre = 9% and coated with SiO₂ layers of h_f = 100 nm. a) Wavelengths and amplitudes of the surface features are measured as a function of Δε = ε_app – ε_re. Values of Δε < 0%, Δε = 0% and Δε > 0% generate perpendicular wavy gratings (W_⊥), featureless flat substrates (F), and parallel wavy gratings (W_||)features, respectively. b) Fast Fourier Transforms (FFT) of PDMS-SiO₂ bilayer substrates with the for −9% < Δε < +9%. The intensity and relative orientation of FFT signals confirm the presence (absence) of distinguishable features and the orientation of wavy grating arrays in the following discrete states: W_⊥, F, and W_||.

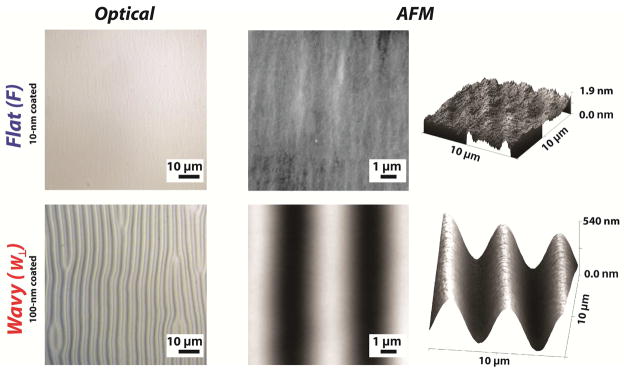
The morphologies of PDMS-SiO₂ bilayers in flat (F) and wavy (W_⊥) configurations are shown in Figure 2. Cycling the strain forms microstructures reversibly as indicated by optical and scanning probe microscopy. AFM images of bilayers in flat configuration (ε = 0%) contain features with characteristic peak-to-trough amplitudes of approximately 2 nm. This feature height is an order of magnitude smaller than the minimum detection limit for mammalian cells [20,47]. The size of the microstructure of the grating is a strong function of the intensive mechanical properties of the two materials: the thickness of the membrane h_f, and the amount of pre-strain ε_pre [48,49]. The anticipated values for average peak-to-through amplitude A₀ and wavelength of the features λ₀ in grating arrays with net compressive strains can be predicted using the following relationships:

$${\lambda }_0=2\pi h_f{\left(\frac{E_f}{3E_s}\right)}^{⅓}$$ Eqn. 2

$$A_0=h_f\sqrt{\frac{{\epsilon }_{\mathit{pre}}}{{\epsilon }_c}-1}$$ Eqn. 3

Figure 2.

PDMS coupons are pre-strained to ε_pre = +3% and SiO₂ membranes of thickness h_f are deposited on elastomeric substrates. The pre-strain is released such that Δε = -3% prior to characterization. SiO₂ membranes with h_f = 100 nm generate flat (F) surfaces, while SiO₂ membranes with h_f = 100 nm generate substrates with perpendicular gratings (W_⊥).

The parameters in each equation are as follows: h_f is the thickness of the rigid membrane; E_f and E_s are Young’s moduli of substrate and rigid membrane, respectively; ε_pre is amount of uniaxial pre-strain; ε_c is critical buckling strain given by [49]:

$${\epsilon }_c=\frac{1}{4}{\left(\frac{3E_s}{E_f}\right)}^{⅔}$$ Eqn. 4

A buckling pattern is established only when the applied strain exceeds ε_c. Values for λ₀ are predicted to be largely independent of the amount of pre-strain. However, experimentally observed values of λ₀ are reduced for uniaxial strains greater than 10% [49–51]. Relationships that describe feature sized formed via spontaneous buckling are valid if certain criteria are satisfied. The intensive properties and extensive geometry of the film must be such that E_f ≫ E_f and h_f ≪ t_s where t_s is the substrate thickness. The net strain Δε is defined as the difference between the resulting applied strain ε_app and the pre-strain via Δε = ε_app − ε_pre. Feature formation either by compression or tension (|ε_app|> ε_pre) yields grating features with peak-to-trough amplitudes of A > 400 nm. Topographic features produced from pre-strained PDMS-SiO₂ bilayer substrates in this study were consistent with constitutive relationships in Eqns. 2—4. Releasing the pre-strain produces a net compressive strain ε_app < ε_pre and a uniaxial grating array that is oriented orthogonally to the axis of the applied strain. Orthogonal features have an average amplitude of A_⊥ = 429.3 ± 5.8 nm and λ_⊥ = 4.95 ± 0.36 μm (Figure 2). Applying a strain ε_app such that Δε = 0% abolishes the grating. Tensile strains of Δε = ε_app − ε_pre > 3% produce grating arrays parallel to the axis of applied strain. Parallel features have an average amplitude of A_|| = 483.6 ± 7.8 nm and λ_|| = 4.78 ± 0.16 μm. Features emerge for strains as small as Δε = |ε_app − ε_pre|= 3%. Fast Fourier Transforms (FFT) of optical micrographs are shown in Figure 3b. The intensity and orientation of these data confirm the presence (absence) and orientation of grating arrays under compression, tension, and zero-strain states. The feature amplitude A is also a strong function of the SiO₂ membrane thickness h_f. Optical and scanning probe micrographs of PDMS-SiO₂ bilayer substrates with h_f = 10 nm, pre-strain of ε_pre = +3%, and an applied strain of Δε = −3% produce random isotropic features with no short-range order and an Rms roughness of 0.317 ± 0.048 nm (Figure 2). Strain-dependent topography can be cycled without any observed hysteresis in feature size. “Strain cycles could likely be repeated dozens of times without any fatigue or fracture of the SiO₂ membrane or mechanical failure of the PDMS substrate.” PDMS-SiO₂ bilayer substrates formed by thermal evaporation of SiO₂ membranes offer additional advantages for use in dynamic presentation of substrate topography. First, the minimum critical strain ε_c is reduced, which minimizes crosstalk from mechanical stimuli during quantification of cytoskeleton remodeling [52]. Second, small strains can alter the surface topography between three discrete states: flat (F), orthogonal wavy (W_⊥), and parallel wavy (W_||). The precise selection of the SiO₂ membrane permits facile fabrication of control substrates that are identical to dynamic topography substrates in terms of surface chemistry and applied strain. Lastly, topography can be presented both rapidly (~5 sec) and reversibly without indirectly impacting feature geometry.

Morphological Responses of Fibroblasts to Static Topography

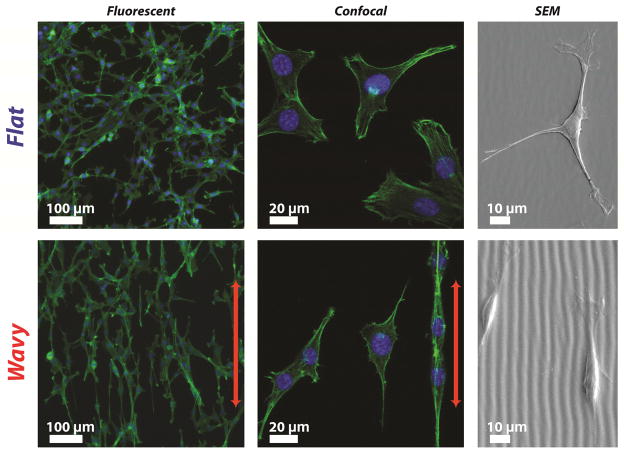
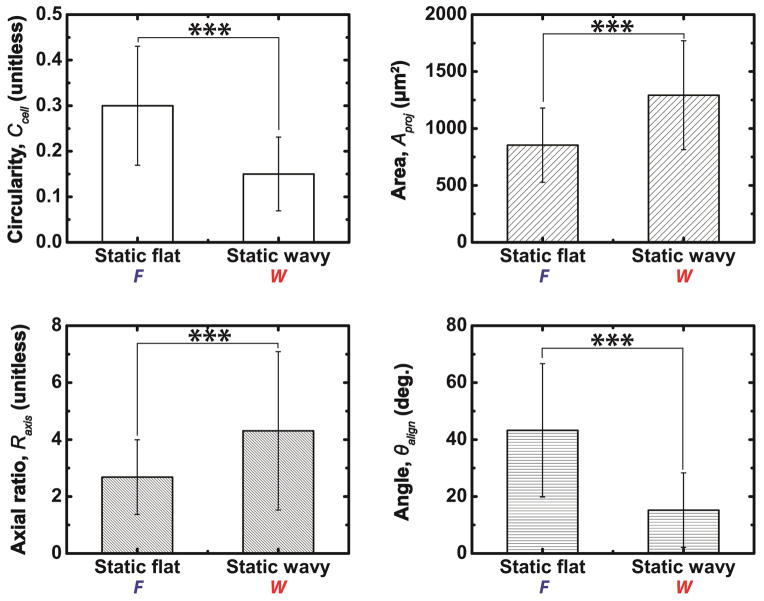
FB cultured on static flat (Static F) exhibit a more rounded morphology and random spreading compared to the aligned morphologies of fibroblasts cultured on static grating arrays (Wavy W) as assessed by fluorescent microscopy (Figure 4). Four parameters were used to quantify FB morphology: circularity C_cell, ratio of major-to-minor axes R_axis, projected area A_proj, and angle of major axis to grating direction θ_align [37,53]. FB cultured on flat substrates exhibit a larger average cell circularity (0.30 ± 0.13 versus 0.15 ± 0.08; p<0.001) and a higher average angle between the major axis of the cell and the features compared to topographic substrates (43.25° ± 23.40° versus 15.22° ± 13.10°; *** p <0.001) (Figure 5). These results suggest that static surfaces with grating topography can recapitulate the highly conserved morphological response of mammalian cells to grating features that is associated with contact guidance [54]. These measurements produce a baseline to compare the evolution of FB cytoskeleton morphology on substrates with dynamic topography.

Figure 4.

Fibroblast morphology and orientation on static flat (F) and static wavy gratings (W). Fibroblasts are fixed on PDMS-SiO₂ bilayers for 24 hr prior to characterization. Micrographs suggest that fibroblasts were randomly oriented on static flat (F) substrates and aligned to wavy gratings (W). The direction of the grating is indicated by the red arrows. Actin and nuclei are shown in green and blue, respectively.

Figure 5.

Quantification of fibroblast morphology and orientation on substrates with static flat (F) and static perpendicular gratings (W_⊥). Values of circularity C_cell, projected area A_proj, axial ratio R_axis, and alignment angle θ_align confirm that fibroblasts exhibit reduced spreading and increased alignment and orientation when cultured on W_⊥ substrates compared to F substrates (*** p<0.001).

Cell Culture on Substrates with Programmable Topography

Many mammalian cell phenotypes respond to dynamic topographical features through cytoskeleton reorganization [26]. The strain detection threshold for mammalian cells in response to uniaxial stretching is ε_cycle = 3.5% as inferred by gross morphological characterization [35,36]. This threshold provides a benchmark to limit the maximum applies strains to |Δε| < 3.5%. Nevertheless, applying strain in sub-threshold regimes may still impact other as of yet unknown aspects of cell-materials interactions. Therefore, several substrate topography sequences were utilized to assess the potential impact of strains in the sub-threshold regime. Static substrates that are either Flat (F) or Wavy (W) are mapped to corresponding dynamic substrates with the following sequences: FW_⊥F and FW_⊥. Substrates with FW_⊥F sequences are critical control conditions to measure the potential impact of the transient strain on the downstream cytoskeleton morphodynamics. Furthermore, a direct comparison between sequences of FW_⊥F and FW_⊥ can isolate the direct impact of dynamic topography presentation on cytoskeleton morphology. FB morphology was largely preserved immediately after applied strains of Δε = ±3% (Figure 6). The orthogonal and parallel axes refer to the relative orientation of the grating features to the direction of applied strain. The morphology of FB cultured on PDMS substrates coated with 10 nm SiO₂ membranes was measured for t ≤ 6 hr (Figure 7). PDMS substrates with SiO₂ membranes of h_f = 10 nm, ε_pre = +3%, and Δε = −3% exhibit random gratings with feature sizes of A = 1.53 ± 0.55 nm and λ = 1.8 ± 0.1 μm. These features do not significantly alter FB morphology via contact guidance mechanisms since the feature heights are smaller than 35 nm, the previously reported detection limit for contact guidance [55]. PDMS substrates with 10 nm SiO₂ membranes serve as valuable control materials since the strain and chemistry of the substrate are identical to PDMS-SiO₂ bilayers that with thicker SiO₂ membranes. Maintaining constant material properties in dynamic substrates can be challenging [5]. Thin film deposition and precise strains achieve dynamic programmable topography while preserving many of the other physicochemical aspects of the cellular microenvironment.

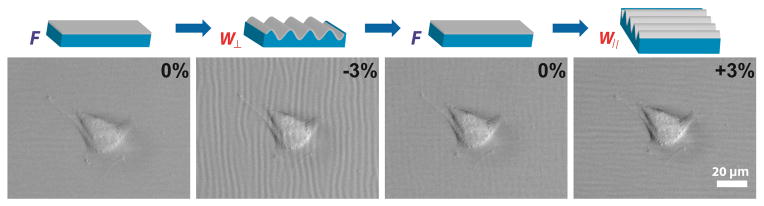
Figure 6.

Transient response of isolated fibroblast in response to PDMS-SiO₂ bilayers cycled through discrete states of substrate topography. The applied strain is applied rapidly to achieve the following configurations: The strain is sequentially and quickly applied across the following values: Δε = 0% generates flat substrates (F) with no grating features; Δε = +3% produces perpendicular wavy grating arrays (W_⊥); Δε = -3% produces wavy grating arrays that are parallel to the axis of applied strain (W_||). The rapid switching of substrate topographies does not induce observable morphological changes in FB over the span of 30 sec.

Figure 7.

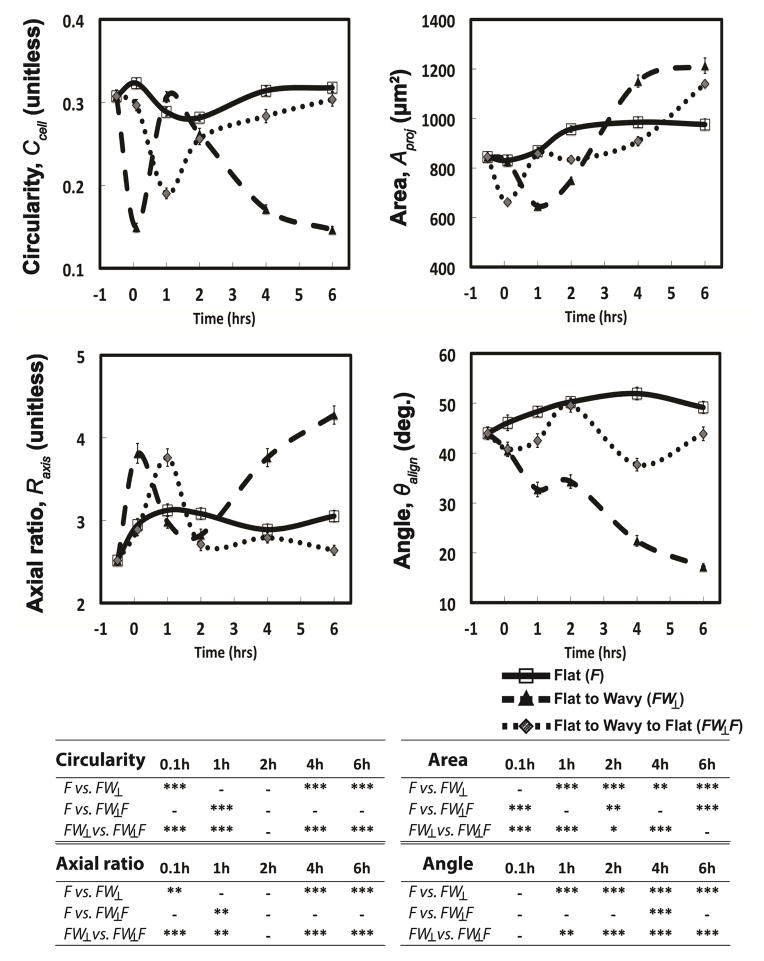
Cytoskeleton morphodynamics for FB cultured on substrates over the course of 6 hr with the following sequences are shown: Static flat (F), dynamic flat to wavy grating (FW_⊥), and dynamic flat to wavy grating back to flat (FW_⊥F). Results of one-way ANOVA with Tukey post hoc tests are summarized in the table.

After 24 hr of cell seeding (t = 0 hr), the surface topography is switched either from flat to wavy (FW_⊥) or flat to wavy to flat (FW_⊥F) in rapid succession. The dynamic presentation of substrate topography induces alterations in FB morphology that initiate within 6 min and persist for t > 6 hr (Figure 7). The circularity and ratio of major/minor axes of the (FW_⊥) both exhibit a significant change within 5 min of substrate actuation (** p < 0.01), while the average projected area A and major axis angle exhibit significant differences within 1 hour (** p < 0.001). Substrates with FW_⊥F programs present gratings briefly (5 ± 3 sec). FB cultured on FW_⊥F programs exhibit cytoskeleton dynamics that are substantially different compared to Static F substrates. FW_⊥F sequences induce transient alterations in the following parameters that are associated with elongated morphologies: circularity C_cell, projected area A_proj, and axial ratio R_axis. Values for C_cell and R_axis in FB populations cultured on substrates with FW_⊥F sequences converge to values observed in cells on Static F substrates for t ≤ 2 hr. These results suggest that sub-threshold strains induce transient fluctuations in morphological response. Temporary presentation of topographical cues may engage memory mechanisms in mammalian cells that have been previously reported in the context of other types of dynamic cell-materials interactions [56].

Substrates with FW_⊥ sequences yield morphological changes in FB that are internally consistent with other observations within this study. Substrate actuation via compressive strains is comparable between FW_⊥ and FW_⊥F sequences. FB cultured on substrates with FW_⊥ sequences adopt a temporary rounded morphology that is manifested by a transient decrease in A_proj for t = 1 hr (*** p < 0.001), a decrease in C_cell at t = 0.1 hr (*** p < 0.001), and an increase in R_axis at t = 0.1 hr (** p < 0.01) compared to Static F substrates (Figure 7). The morphology of FB cultured on substrates with FW_⊥ sequences for t ≥ 6 hr compared to Static F substrates approximate that of Static W compared to Static F. These results suggest that steady state FB morphology is achieved within 6 hr.

Single Cell Sensing on Programmable Substrates

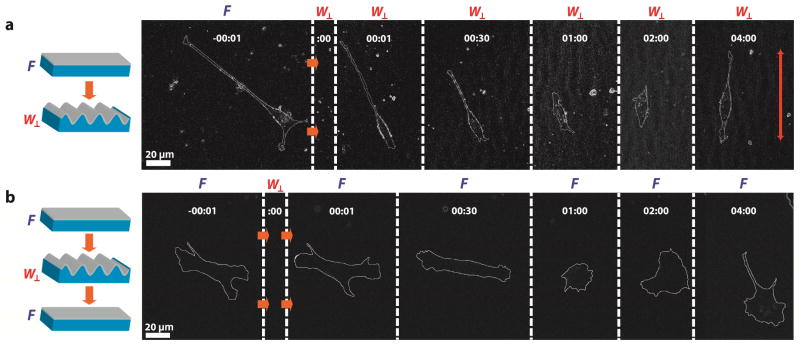
Programmable substrates can measure the cytoskeleton morphology of individual FB to transiently altered strain-induced topography. Isolated FB alter their shape and orientation for t ≤ 1 hr. Single cell morphological responses observed in FB populations cultured on substrates with FW_⊥ and FW_⊥F sequences are shown in Figure 8. FB alignment and elongation occurs for t < 2 hr for FW_⊥ sequences. FB cultured on substrates with FW_⊥F sequences extend lamellipodia in seemingly random directions within t < 2 hr. Single-cell morphodynamics are consistent with morphological observations made in FB populations. A notable observation is that both FW_⊥ and FW_⊥F sequences induce an initial temporary phase of cell rounding immediately after substrate actuation. This observation is curious because the minimum apparent strain detection threshold during cyclic stretching is ε_cycle = 3.5%. Furthermore, the strains applied in this system are approximately 1 order of magnitude smaller than other experiments that employ dynamic strain. Typical cyclic strain experiments require uniaxial substrate strains of ε_cycle > 10% [57]. Furthermore, the impact of mechanical stress on cytoskeleton dynamics typically results in strains of γ ~ 20% [58,59]. It is unlikely that the application of transient strains will directly impact binding between integrins and adhesion-promoting peptides because of the strength of these bonds [60]. Typical dissociation constants for this specific kind of receptor-ligand interaction is on the order of K_D = 10⁻⁹ M, which corresponds to a characteristic binding distance of r₀ = 3 nm [60]. Considering the characteristic dimension of integrins is ~10 nm [61], it is likely that 3% strains will not dissociate integrin-peptide coupling. Dynamic topography presentation may, however, alter other downstream effectors of cell-matrix interactions. Transient substrate strains may disrupt focal adhesions or cytoskeleton proteins that induce an altered state in which the cell rapidly interrogates the local chemical and topographical microenvironments by forming nascent integrins [62]. This topic is the subject of ongoing research.

Figure 8.

Morphodynamics of a single FB in response to substrate perturbations. Single cells are captured before and after topographical switching, indicated at zero time point (:00). Phase contrast micrographs are processed using ImageJ to depict changes of cellular shape and orientation. Substrates are programmed with the following sequences: (a) flat to perpendicular wavy grating (FW_⊥); (b) flat to perpendicular wavy grating back to flat substrates (FW_⊥F).

Conclusions

We describe a lithography-free approach to fabricate dynamic substrate topography for measuring morphodynamics of cells during contact guidance. This substrate is advantageous because it presents ordered topographical cues in a manner that decouples potential contributions from other stimuli including substrate chemistry and large mechanical strains. Homogeneous surface chemistry (SiO₂) and small required extensive strains (~3%) are key features of the approach described herein. Although the substrate cannot completely eliminate the effect of external mechanical stimuli on in vitro cell-topography interactions, using small strains minimizes the impact of the applied stimulus. This work represents a strategy to better isolate the effect of dynamic programmable topography on living cells. Furthermore, this technique permits rapid and reversible presentation of topographic cues with precise temporal precision. Dynamic substrate topography afforded by the strategy described herein could be further applied to studying signal transduction pathways that original from transient cell-matrix interactions and propagate to downstream pathways that control cytoskeleton reorganization. Thus, programmable substrate topography is a promising strategy to elucidate cytoskeleton remodeling dynamics in many cell phenotypes in the context of cell-biomaterials interactions.

Supplementary Material

1. Figure S1.

Measurement of cell components on a substrate with gratings. The cell components include cell perimeter (P), projected area (A_proj), length of major and minor axes, and alignment angle (θ_align). P and A_proj are indicated in red dotted line and green area, respectively. Major axis is the longest straight line located within a cell. Minor axis is the longest straight line that is perpendicular with the major axis. θ_align is an angle between substrate and major axes. SI-2

2. Figure S2.

Dynamic change in the average alignment angle θ_align of fibroblasts over 6 hr on substrates with the following topographic sequences: dynamic flat to wavy grating (FW_⊥); dynamic flat to wavy grating back to flat (FW_⊥F). These data demonstrate the gradual shift in alignment for FW_⊥ sequences compared to unperturbed distributions in FW_⊥F sequences.

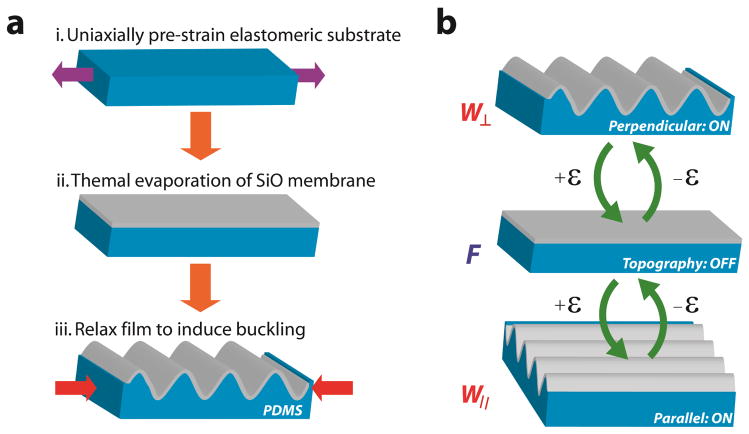
Figure 1.

Fabrication scheme and actuation of reconfigurable dynamic topography. a) The fabrication flow is shown: (i) PDMS substrates are strained to a prescribed amount and (ii) SiO₂ membranes are deposited on the surface using thermal evaporation. (iii) The pre-strain is released to create wavy grating arrays via spontaneous buckling. b) Programmable topography consists of three discrete strain-dependent states that depend on the difference between the pre-strain and applied strain Δε: Δε < 0% produces perpendicular wavy gratings (W_⊥); Δε = 0% produces flat substrates (F); Δε > 0% produces parallel wavy gratings (W_||). These states are shown sequentially from top to bottom as the value of Δε becomes more positive.