Abstract
Purpose
to establish reference values for ultrasound shear-wave elastography for pericranial muscles in healthy individuals (m. trapezius, m. splenius capitis, m. semispinalis capitis, m. sternocleidomastoideus and m. masseter). Also to evaluate day-to-day variations in the shear-wave speeds and evaluate the effect of the pennation of the muscle fibers, ie scanning parallel or perpendicularly to the fibers.
Materials and Methods
10 healthy individuals (5 males and 5 females) had their pericranial muscles examined with shear-wave elastography in two orthogonal planes on two different days for their dominant and non-dominant side. Mean shear wave speeds from 5 ROI’s in each muscle, for each scan plane for the dominant and non-dominant side for the two days were calculated. The effect of the different parameters – muscle pennation, gender, dominant vs non-dominant side and day was evaluated.
Results
The effect of scan plane in relation to muscle pennation was statistically significant (p<0.0001). The mean shear-wave speed when scanning parallel to the muscle fibers was significantly higher than the mean shear-wave speed when scanning perpendicularly to the fibers. The day-to-day variation was statistically significant (p=0.0258), but not clinically relevant. Shear-wave speeds differed significantly between muscles. Mean shear wave speeds (m/s) for the muscles in the parallel plane were: for masseter 2.45 (SD:+/−0.25), semispinal 3.36 (SD:+/−0.75), splenius 3.04 (SD:+/−0.65), sternocleidomastoid 2.75 (SD:+/−0.23), trapezius 3.20 (SD:+/−0.27) and trapezius lateral 3.87 (SD:+/−3.87).
Conclusion
The shear wave speed variation depended on the direction of scanning. Shear wave elastography may be a method to evaluate muscle stiffness in patients suffering from chronic neck pain.
Key words: muscular, ultrasound, elastography
Introduction
Ultrasound (US) elastography is a method for evaluating tissue stiffness. Mainly two different methods are commercially available – strain and shear wave elastography. Shear wave elastography can be subdivided into shear wave speed measurements and shear wave speed imaging according to the EFSUMB guidelines 1 .
Strain elastography has mainly been evaluated for focal lesions in the breast and in the thyroid 2 3 and is based on mechanical stress applied by the physician performing the examination. The elasticity is shown visually on a color scale from blue to red and semiquantitative values may be calculated. Shear wave elastography evaluates tissue stiffness by applying an acoustic push pulse, which deforms the tissue. This deformation causes shear waves, which occur perpendicularly to the US push pulse. The speed of these shear waves is proportional to tissue stiffness and a quantitative value of stiffness is shown. The method has mainly been evaluated for assessment of liver stiffness but also recently in skeletal muscle 4 5 .
Tension-type headache (TTH) is subdivided into tension-type headache associated with or without pericranial muscle tenderness 6 and the one-year prevalence of neck pain is 88% in TTH 7 . Experimentally, pericranial muscles are significantly more tender in patients with chronic tension-type headache than in healthy controls 8 and the degree of tenderness is strongly correlated to the frequency and intensity of tension-type headaches 9 . In addition, pericranial muscles are significantly harder in patients with chronic tension-type headache than in healthy controls 10 , which correlate with muscle tenderness of the trapezius muscle and relate to the headache state 11 . To date, there are no studies using elastography in primary headaches, but US elastography may be an ideal noninvasive quantitative tool to uncover muscle tension in these patients.
The aim of the study was to establish reference values for shear wave elastography for the pericranial muscles in healthy individuals, more specifically the m. trapezius, m. splenius capitis, m. semispinalis capitis, m. sternocleidomastoideus and m. masseter, and to evaluate day-to-day variations in shear wave speeds and evaluate the effect of the pennation of the muscle fibers, i. e., scanning parallel or perpendicular to the fibers.
Materials and Methods
Subjects
All subjects gave informed oral consent to participate in the study. The study was approved by the Regional Committee on Health Research Ethics (ID: H-15014237). 10 healthy volunteers were included in the study – 5 males and 5 females, median age: 31 years (range: 22–46 years). All subjects were physically active, but were asked not to do any sports within 48 h before the study. The median weight, height and BMI were 70.5 kg (range: 48–105 kg), 177 cm (range: 157–195 cm) and 22.4 (range: 19.5–27.6), respectively. All volunteers were scanned twice with one week separating the two sessions in order to determine possible day-to-day variation.
The volunteers had the trapezius muscle, the semispinalis capitis muscle, the splenius muscle, the sternocleidoid muscle and the masseter muscle scanned on both sides ( Fig. 1 ). The dominant hand and possible sport activity were registered.
Fig. 1.
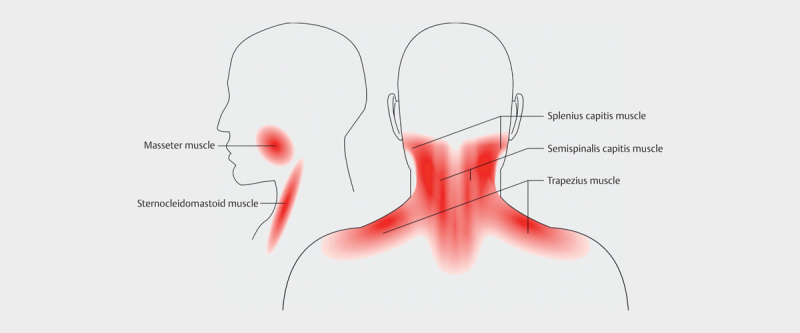
Drawing of the muscles examined in two planes (Thien Phu Do is acknowledged for drawing the figure)
Ultrasound
A GE Logic E9 system with a 9 MHz linear array transducer and MSK preset (GE, Chalfont St. Giles) was used for all measurements. All muscles were scanned parallel and perpendicular to the direction of the muscle fibers. For the trapezius, two measurements, one medial measurement and one more lateral measurement, were performed. In the masseter muscle two scan planes perpendicular to each other were obtained, but due to the pennation of the muscle it was impossible to obtain images with only one direction of the pennation.
Elastography
The system shows real-time color coded shear-wave elastography images in a pre-defined ROI of the B-mode image with a frame rate of approximately 1 Hz. Cine loops of ten frames were stored in the system for later post-processing. All recordings were performed by the same experienced physician with light pre-compression on the skin. The subjects were examined sitting on a chair with their arms resting in their lap. All recordings were done from the back of the patient. An example of an elastogram is shown in Fig. 2 3 4 .
Fig. 2.
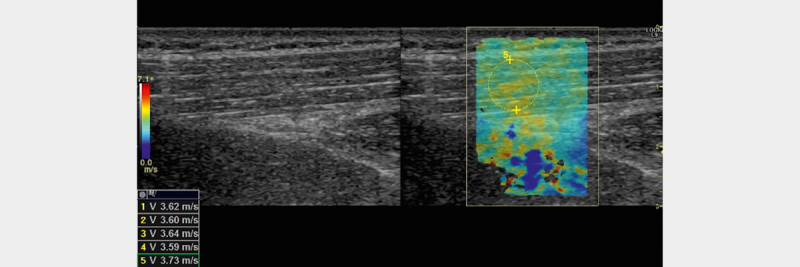
B-mode image of the trapezius muscle (left) and corresponding image with elastogram (right). Shear wave speed is shown in the lower left corner for 5 different ROIs (only number 5 shown).
Fig. 3.
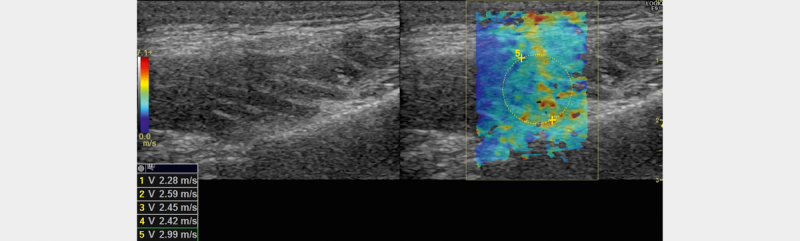
B-mode image of the masseter muscle (left) and corresponding image with elastogram (right)
Fig. 4.
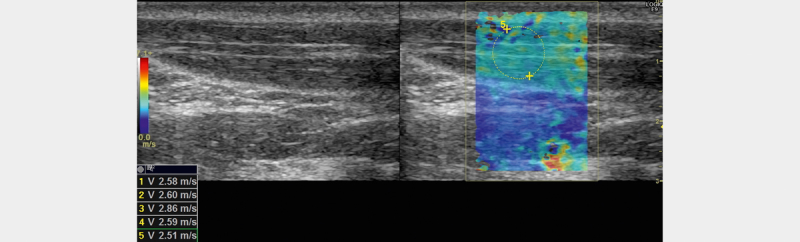
B-mode image of the sternocleidomastoid muscle (left) and corresponding image with elastogram (right)
After all recordings were performed, the shear wave speeds of the different muscles in m/s were registered. ROIs were placed in each muscle in agreement by two observers. Circular ROIs were placed to cover as much muscle as possible. Five measurements were performed in each cine loop from each measuring point in each muscle – one for each recorded frame. The size and depth of the ROI were registered for all measurements.
Statistics
The mean shear wave speeds for all muscle groups in the two orthogonal planes were calculated.
The repeated measurements were analyzed in a mixed effects linear statistical model including sequence, side, dominant side, muscle group, gender, muscle group-scanning direction interaction, dominant side-scanning direction interaction, sequence-scanning direction, and sequence-muscle group interaction as fixed effects and subject-dominant side-muscle group-scanning direction as random effect.
The effects of sequence, dominant side and scanning direction were tested in the statistical model using SAS 9.4 (SAS Institute). The fixed effects were tested in the full model using two-sided type 3 tests of effects. A paired t-test was performed to analyze the variation between days.
Results
A total of 20 repeated samples were measured for each of the 6 groups of muscles for each subject for right and left sides, adding up to 240 measurements per subject. The mean shear wave speeds (m/s) in the parallel plane were: 2.45 (SD:+/−0.25) for masseter, 3.36 (SD:+/−0.75) for semispinalis, 3.04 (SD:+/−0.65) for splenius, 2.75 (SD:+/−0.23) for sternocleidomastoid, 3.20 (SD:+/−0.27) for trapezius and 3.87 (SD:+/−3.87) for trapezius lateral. Fig. 5 presents histograms for the combination of muscle groups and scanning direction using subject mean values within each category.
Fig. 5.
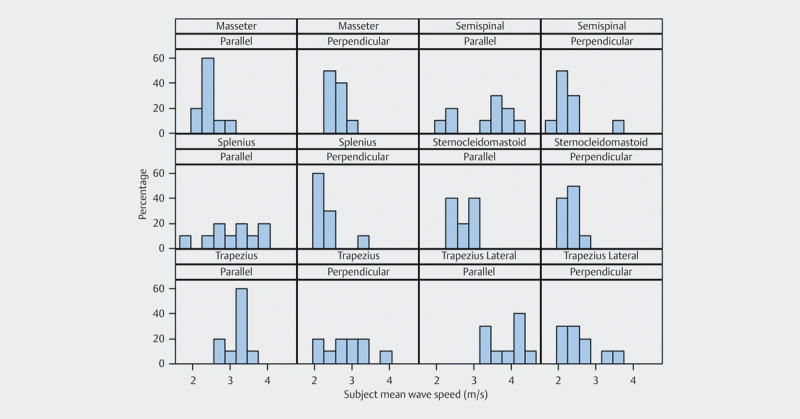
Probability histogram panel plot. X-axis shows mean shear wave speed and y-axis shows proportion of subjects for all examined muscles in two planes.
The effect of sequence (day-to-day variation) was statistically significant (p=0.0258, test for sequence-muscle group interaction). Fig. 6 shows that even though the effect is statistically significant, the effect is not in the same direction for all muscle groups. Further analysis showed no statistically significant difference between the two days. The mean shear wave speed for each muscle, each fiber direction, dominant and non-dominant hand for the two different days is shown in Table 1 .
Fig. 6.
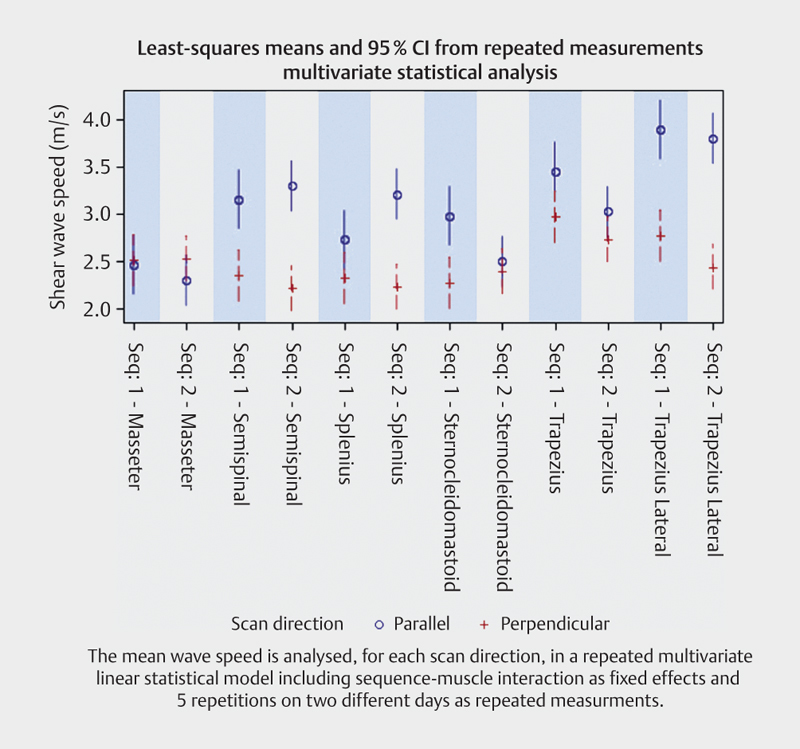
Mean shear wave speed for all examined muscles for each scan plane
Table 1 Mean shear wave speed for each muscle, each fiber direction, dominant and non-dominant hand for each of the two days including SD.
| Dominant side | Muscle | Fiber direction | Day | Mean (m/s) | SD |
|---|---|---|---|---|---|
| Yes | Masseter | Parallel | 1 | 2.38 | 0.31 |
| Yes | 2 | 2.38 | 0.26 | ||
| Yes | Perpendicular | 1 | 2.49 | 0.35 | |
| Yes | 2 | 2.48 | 0.41 | ||
| Yes | Semispinalis | Parallel | 1 | 3.50 | 1.15 |
| Yes | 2 | 3.67 | 1.15 | ||
| Yes | Perpendicular | 1 | 2.36 | 0.47 | |
| Yes | 2 | 2.09 | 0.53 | ||
| Yes | Splenius | Parallel | 1 | 2.78 | 0.57 |
| Yes | 2 | 3.30 | 1.08 | ||
| Yes | Perpendicular | 1 | 2.44 | 0.51 | |
| Yes | 2 | 2.18 | 0.53 | ||
| Yes | Sternocleidomastoid | Parallel | 1 | 3.14 | 0.39 |
| Yes | 2 | 2.53 | 0.30 | ||
| Yes | Perpendicular | 1 | 2.29 | 0.54 | |
| Yes | 2 | 2.37 | 0.30 | ||
| Yes | Trapezius | Parallel | 1 | 3.32 | 0.60 |
| Yes | 2 | 2.80 | 0.37 | ||
| Yes | Perpendicular | 1 | 3.04 | 0.73 | |
| Yes | 2 | 3.03 | 0.72 | ||
| Yes | Trapezius lateral | Parallel | 1 | 3.93 | 0.65 |
| Yes | 2 | 3.71 | 0.41 | ||
| Yes | Perpendicular | 1 | 2.82 | 1.03 | |
| Yes | 2 | 2.54 | 0.29 | ||
| No | Masseter | Parallel | 1 | 2.62 | 0.55 |
| No | 2 | 2.43 | 0.37 | ||
| No | Perpendicular | 1 | 2.70 | 0.53 | |
| No | 2 | 2.51 | 0.31 | ||
| No | Semispinalis | Parallel | 1 | 3.07 | 0.84 |
| No | 2 | 3.22 | 0.58 | ||
| No | Perpendicular | 1 | 2.24 | 0.58 | |
| No | 2 | 2.33 | 0.63 | ||
| No | Splenius | Parallel | 1 | 2.93 | 1.02 |
| No | 2 | 3.15 | 0.48 | ||
| No | Perpendicular | 1 | 2.13 | 0.43 | |
| No | 2 | 2.40 | 0.69 | ||
| No | Sternocleidomastoid | Parallel | 1 | 2.82 | 0.47 |
| No | 2 | 2.50 | 0.30 | ||
| No | Perpendicular | 1 | 2.24 | 0.38 | |
| No | 2 | 2.39 | 0.63 | ||
| No | Trapezius | Parallel | 1 | 3.42 | 0.78 |
| No | 2 | 3.25 | 0.49 | ||
| No | Perpendicular | 1 | 2.88 | 0.80 | |
| No | 2 | 2.43 | 0.31 | ||
| No | Trapezius lateral | Parallel | 1 | 3.91 | 0.65 |
| No | 2 | 3.93 | 0.62 | ||
| No | Perpendicular | 1 | 2.75 | 0.68 | |
| No | 2 | 2.33 | 0.60 |
The effect of dominant side was not statistically significant (p=0.69, test for dominant side-scanning direction interaction and p=0.60 main effect of dominant side).
The effect of scan plane in relation to muscle pennation was statistically significant (p<0.0001, test for muscle-scanning direction interaction). The mean shear-wave speed when scanning parallel to the muscle fibers was significantly higher than the mean shear-wave speed when scanning perpendicular to the fibers. The results are shown as model estimate LSMeans in Fig. 7 . Values differed significantly between muscles.
Fig. 7.
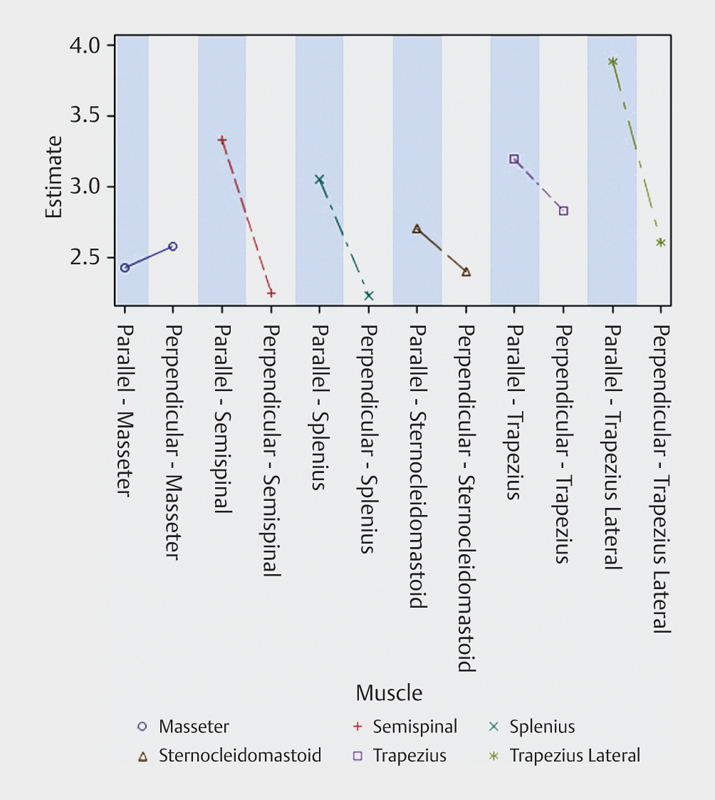
Mean shear wave speed estimates when scanning parallel and perpendicular to the muscle fibers
Discussion
We have examined factors influencing the elasticity of head and neck muscles in healthy individuals and found that the pennation of the muscle fibers had an influence on the shear wave speeds measured.
This has been confirmed in other studies. Gennisson et al. examined beef muscle fibers and found that the shear waves propagated more easily parallel to the muscle fibers that perpendicular to them and Eby et al. examined shear moduli with increasing strain with different transducer positions - parallel, perpendicular and 45 degrees – and shear waves propagated better in the parallel position 12 13 .
The shear modulus of tissue may be calculated from the shear-wave speed using the equation: μ=c 2 ρ, where μ is the shear modulus, c is the shear wave speed and ρ is the density which may be assumed to be 1000 kg/m 3 . This formula assumes isotropy, and homogeneous materials, and it may only be accurate to report shear modulus when the waves are propagating parallel to the underlying muscle fibers. In other transducer orientations it may be more correct only to report shear wave speeds 1 13 . We only reported the shear wave speeds in order to be able to compare the values from scanning parallel and perpendicular to the muscle fibers. Also in the masseter muscle fibers were interleaved in different directions. In musculoskeletal US, anisotropy is a well-known artifact especially when scanning tendons. Skeletal muscle may be considered transversely isotropic but in this transducer orientation anisotropy from the examined tissue may be a challenge 14 .
There was a statistically significant effect from the day the volunteers were scanned, but we do not find it clinically relevant and it may be due to coincidence in this relatively small population, also because the effect was not in the same direction for all muscles. Niitsu et al. found a post-exercise hardness of the biceps muscle, which lasted for two to four days 15 . All volunteers were asked not to perform vigorous exercise two days before our measurements. This may also explain why we did not find a statistically significant effect of dominant vs. non-dominant hand. Furthermore, the muscles were examined when relaxed and not during exercise.
Another group has examined US elastography for evaluation of trigger points 16 . Although they only examined three persons with chronic neck pain and compared them with 17 healthy volunteers, they found significantly stiffer trapezius muscles in the persons suffering from chronic neck pain. They examined the muscle in the transverse plane and the median shear wave speed was 2.02+/−0.42 m/s in healthy volunteers and 2.49+/−0.39 in persons with chronic neck pain. Our mean values in the trapezius medial and lateral were: 2.84 +/−0.55 m/s and 2.61 +/−0.49 m/s, respectively, where the medial position was comparable to the position in the paper. This difference may be due to the elastography method not being exactly the same. We measured shear wave speeds from an area sampled in real time and the authors of the other study used point shear wave elastography from another vendor.
We chose the ROI to cover as much as possible of the muscle thickness to obtain representative values. We noticed that the signal was dampened in persons with thick muscles and in the muscles that were lying deeper (semispinalis muscle compared to splenius muscle). Other studies have shown a dependency on depth in a phantom and in the liver for shear wave elastography 17 and also in muscles 18 . Kot et al. found an effect of ROI size on the maximum value of the shear wave speed, but not on the mean shear wave speed when scanning the thigh muscles 19 .
Limitations
Our study had some limitations. Although the same radiologist examined the volunteers, it was impossible to ensure that the measurement was performed at exactly the same point in the two sessions. It was difficult to distinguish between the splenius and the semispinalis muscle, which were both rather thin muscles. Also the scan planes in the masseter were not only parallel or perpendicular to the fibers as the two heads of the masseter muscles are interleaved. All persons were examined in the sitting position resting, but even minor tension in the muscles may have influenced the measurements.
Conclusion
The shear wave speed variation depended on the direction of scanning. No difference was seen between measurements between dominant and non-dominant side. The day-to-day variation was statistically significant, but the differences were not clinically relevant.
Shear wave elastography may be a method to evaluate muscle stiffness in patients suffering from chronic neck pain.
References
- 1.Bamber J, Cosgrove D, Dietrich C F, Fromageau J, Bojunga J, Calliada F et al. EFSUMB guidelines and recommendations on the clinical use of ultrasound elastography. Part 1: Basic principles and technology. Ultraschall in Med. 2013;34:169–184. doi: 10.1055/s-0033-1335205. [DOI] [PubMed] [Google Scholar]
- 2.Cantisani V, D'Andrea V, Biancari F, Medvedyeva O, Di Segni M, Olive M et al. Prospective evaluation of multiparametric ultrasound and quantitative elastosonography in the differential diagnosis of benign and malignant thyroid nodules: Preliminary experience. Eur J Radiol. 2012;81:2678–2683. doi: 10.1016/j.ejrad.2011.11.056. [DOI] [PubMed] [Google Scholar]
- 3.Itoh A, Ueno E, Tohno E, Kamma H, Takahashi H, Shiina T et al. Breast disease: Clinical application of US elastography for diagnosis. Radiology. 2006;239:341–350. doi: 10.1148/radiol.2391041676. [DOI] [PubMed] [Google Scholar]
- 4.Cassinotto C, Lapuyade B, Ait-Ali A, Vergniol J, Gaye D, Foucher J et al. Liver fibrosis: Noninvasive assessment with acoustic radiation force impulse elastography–comparison with FibroScan M and XL probes and FibroTest in patients with chronic liver disease. Radiology. 2013;269:283–292. doi: 10.1148/radiol.13122208. [DOI] [PubMed] [Google Scholar]
- 5.Chino K, Akagi R, Dohi M, Fukashiro S, Takahashi H. Reliability and validity of quantifying absolute muscle hardness using ultrasound elastography. PloS One. 2012;7:e45764. doi: 10.1371/journal.pone.0045764. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Headache Classification Committee of the International Headache S . The international classification of headache disorders, 3rd ed. (beta version) Cephalalgia. 2013;33:629–808. doi: 10.1177/0333102413485658. [DOI] [PubMed] [Google Scholar]
- 7.Ashina S, Bendtsen L, Lyngberg A C, Lipton R B, Hajiyeva N, Jensen R. Prevalence of neck pain in migraine and tension-type headache: A population study. Cephalalgia. 2015;35:211–219. doi: 10.1177/0333102414535110. [DOI] [PubMed] [Google Scholar]
- 8.Langemark M, Jensen K, Jensen T S, Olesen J. Pressure pain thresholds and thermal nociceptive thresholds in chronic tension-type headache. Pain. 1989;38:203–210. doi: 10.1016/0304-3959(89)90239-x. [DOI] [PubMed] [Google Scholar]
- 9.Jensen R, Rasmussen B K, Pedersen B, Olesen J. Muscle tenderness and pressure pain thresholds in headache. A population study. Pain. 1993;52:193–199. doi: 10.1016/0304-3959(93)90131-8. [DOI] [PubMed] [Google Scholar]
- 10.Sakai F, Ebihara S, Akiyama M, Horikawa M. Pericranial muscle hardness in tension-type headache. A non-invasive measurement method and its clinical application. Brain. 1995;118:523–531. doi: 10.1093/brain/118.2.523. [DOI] [PubMed] [Google Scholar]
- 11.Ashina M, Bendtsen L, Jensen R, Sakai F, Olesen J. Muscle hardness in patients with chronic tension-type headache: Relation to actual headache state. Pain. 1999;79:201–205. doi: 10.1016/s0304-3959(98)00167-5. [DOI] [PubMed] [Google Scholar]
- 12.Gennisson J L, Catheline S, Chaffai S, Fink M. Transient elastography in anisotropic medium: Application to the measurement of slow and fast shear wave speeds in muscles. J Acoust Soc Am. 2003;114:536–541. doi: 10.1121/1.1579008. [DOI] [PubMed] [Google Scholar]
- 13.Eby S F, Song P, Chen S, Chen Q, Greenleaf J F, An K N. Validation of shear wave elastography in skeletal muscle. J biomech. 2013;46:2381–2387. doi: 10.1016/j.jbiomech.2013.07.033. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Morrow D A, Haut Donahue T L, Odegard G M, Kaufman K R. Transversely isotropic tensile material properties of skeletal muscle tissue. J Mech Behav Biomed Mater. 2010;3:124–129. doi: 10.1016/j.jmbbm.2009.03.004. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Niitsu M, Michizaki A, Endo A, Takei H, Yanagisawa O. Muscle hardness measurement by using ultrasound elastography: A feasibility study. Acta Radiol. 2011;52:99–105. doi: 10.1258/ar.2010.100190. [DOI] [PubMed] [Google Scholar]
- 16.Kuo W H, Jian D W, Wang T G, Wang Y C. Neck muscle stiffness quantified by sonoelastography is correlated with body mass index and chronic neck pain symptoms. UMB. 2013;39:1356–1361. doi: 10.1016/j.ultrasmedbio.2012.11.015. [DOI] [PubMed] [Google Scholar]
- 17.Chang S, Kim M J, Kim J, Lee M J. Variability of shear wave velocity using different frequencies in acoustic radiation force impulse (ARFI) elastography: A phantom and normal liver study. Ultraschall in Med. 2013;34:260–265. doi: 10.1055/s-0032-1313008. [DOI] [PubMed] [Google Scholar]
- 18.Ewertsen C, Carlsen J F, Christiansen I R, Jensen J A, Nielsen M B. Evaluation of healthy muscle tissue by strain and shear wave elastography – Dependency on depth and ROI position in relation to underlying bone. Ultrasonics. 2016;71:127–133. doi: 10.1016/j.ultras.2016.06.007. [DOI] [PubMed] [Google Scholar]
- 19.Kot B C, Zhang Z J, Lee A W, Leung V Y, Fu S N. Elastic modulus of muscle and tendon with shear wave ultrasound elastography: Variations with different technical settings. PloS One. 2012;7:e44348. doi: 10.1371/journal.pone.0044348. [DOI] [PMC free article] [PubMed] [Google Scholar]


