Substitutability of nicotine alone and an electronic cigarette liquid using a concurrent choice assay in rats: A behavioral economic analysis

Abstract

Background

For the Food and Drug Administration to effectively regulate tobacco products, the contribution of non-nicotine tobacco constituents to the abuse liability of tobacco must be well understood. Our previous work compared the abuse liability of electronic cigarette refill liquids (EC liquids) and nicotine (Nic) alone when each was available in isolation and found no difference in abuse liability (i.e., demand elasticity). Another, and potentially more sensitive measure, would be to examine abuse liability in a choice context, which also provides a better model of the tobacco marketplace.

Methods

Demand elasticity for Nic alone and an EC liquid were measured when only one formulation was available (alone-price demand) and when both formulations were concurrently available (own-price demand), allowing an assessment of the degree to which each formulation served as a substitute (cross-price demand) when available at a low fixed-price.

Results

Own-price demand for both formulations were more elastic compared to alone-price demand, indicating that availability of a substitute increased demand elasticity. During concurrent access, consumption of the fixed-price formulation increased as the unit-price of the other formulation increased. The rate of increase was similar between formulations, indicating that they served as symmetrical substitutes.

Conclusion

The cross-price model reliably quantified the substitutability of both nicotine formulations and indicated that the direct CNS effects of non-nicotine constituents in EC liquid did not alter its abuse liability compared to Nic. These data highlight the sensitivity of this model and its potential utility for examining the relative abuse liability and substitutability of tobacco products.

1. Introduction

The 2009 Family Smoking Prevention and Tobacco Control Act charges the Food and Drug Administration (FDA) to regulate tobacco products, including regulating the levels of nicotine and other non-nicotine constituents in tobacco products (Hatsukami et al., 2013). Specifically, it requires the FDA Center for Tobacco Products (CTP) to evaluate new tobacco products that claim to have reduced abuse potential or, at most, an abuse potential that is substantially equivalent to existing products (Berman et al., 2015; Brennan et al., 2014). Animal models are vital for this purpose because they allow studies (e.g., those controlling for the sensory effects of constituents) that are difficult to accomplish in humans (Donny et al., 2012). Those that utilize state-of-the-art methods for assessing abuse liability in animal models may be the most useful to inform regulatory policy on tobacco products.

There are several methods to determine the relative abuse liability of drugs in rats. Most often researchers use low fixed-ratio (FR) schedules to compare rates of acquisition and/or the amount of responding maintained by intravenous self-administration across a range of doses (Ator and Griffiths, 2003; Banks and Negus, 2012). A more robust method is to examine the reinforcing efficacy of a drug by measuring responding on a progressive ratio schedule (Hodos, 1961), where the response requirement increases after each reinforcer delivery to determine a breakpoint or the highest response requirement the drug will maintain across an effective dose range (Stafford et al., 1998). Collectively, these approaches have been used to compare the relative abuse liability of a drug; drugs that engender quicker and/or more reliable acquisition of self-administration, maintain responding across a broader range of schedule requirements and produce higher breakpoints are considered to have greater abuse liability (Ator and Griffiths, 2003).

Behavioral economics (Hursh, 1984) provides an alternative model to assess abuse liability that combines several of these aforementioned measures under a unified theoretical construct (see a review Bickel et al., 2000). In the behavioral economic model, drug intake is measured across a range of FR values (e.g., FR 1, 3, 6, 9, 15, etc.) to produce a demand curve whereby drug consumption (mg/kg) is plotted as a function of unit price (FR/mg/kg). The demand curve allows several abuse liability factors to be collectively assessed, including demand intensity (i.e., the amount of consumption with relatively free access [e.g., an FR 1]), breakpoint (i.e., the unit price where zero consumption occurs) and demand elasticity (i.e., the rate at which drug consumption decreases with increases in its response requirement or unit price [FR/unit dose]). Of these measures, demand elasticity provides an overarching metric for the abuse liability of a drug since it captures how sensitive drug consumption is to an increase in unit price (Hursh et al., 2013; Hursh and Roma, 2016). Demand is considered inelastic if consumption of a drug decreases slowly in proportion to increases in unit price. If demand for one drug is more inelastic compared to another drug, it indicates that it has higher abuse liability or essential value (Hursh and Silberberg, 2008).

A primary concern in evaluating the relative abuse potential of products is the possible role of addiction-relevant non-nicotine constituents (Brennan et al., 2014). Several studies have recently examined the potential contribution of non-nicotine tobacco constituents to the abuse liability of tobacco products. Some non-nicotine constituents (i.e., nornicotine and acetaldehyde) have been shown to maintain self-administration in isolation or to enhance the reinforcing effects of nicotine, suggesting they might contribute to the abuse liability of tobacco products via their direct reinforcing effects (Bardo et al., 1999; Belluzzi et al., 2005; Hoffman and Evans, 2012). Consequently, some of these constituents (e.g., nornicotine, anabasine) have been added to the FDA CTP's list of Harmful or Potentially Harmful Constituents (HPHCs) in tobacco products, which are chemicals or chemical compounds in tobacco products or tobacco smoke that cause or could cause harm to users or nonusers (CTP, 2014). HPHCs must be measured and reported for all tobacco products by industry to provide a basis for determining whether new products are substantially equivalent to or pose a reduced health risk compared to currently marketed products.

To determine if the abuse liability of products is enhanced by an interaction between nicotine non-nicotine constituents (both known and unknown), researchers have compared responding for Nic to extracts from smokeless tobacco, cigarette smoke, and electronic cigarettes refill liquids (EC liquids). In general, there have been mixed findings using traditional and behavioral economic models of abuse liability with some studies showing no difference between formulations (Brennan et al., 2015; LeSage et al., 2016a, b) and others showing extracts have an increased abuse liability compared to Nic under some conditions (Brennan et al., 2013, 2015; Costello et al., 2014; Gellner et al., 2016). Several factors have been proposed to explain these discrepant findings, such as the relative differences in non-nicotine tobacco constituents present across different classes of products (combustible versus non-combustible) and the various methods used to prepare extracts from the tobacco products (Brennan et al., 2015). Another factor that may have played a role in these inconsistent results was that they were all examined in isolation and not under concurrent access, which more closely mimics the human tobacco marketplace. Previous animal research has shown that the reinforcing efficacy of drugs can appear similar under isolated conditions, but differ under concurrent access conditions (Wang et al., 2001; Ward et al., 2005). Indeed, the demand elasticity of drugs (e.g., cocaine, ethanol, PCP, remifentanil) are not static and depend upon the availability of other reinforcers (e.g., Wade-Galuska et al., 2007, 2011; Campbell and Carroll, 2000; Carroll et al., 1995).

In humans, previous behavioral economic research has also assessed how the availability of alternative reinforcers alters the abuse liability of regular nicotine-containing cigarettes (e.g., Shahan et al. 1999; Johnson and Bickel 2003; Johnson et al. 2004). Shahan et al. (1999) compared self-administration of regular and denicotinized cigarettes across increasing unit prices (i.e., the response cost/puff). When self-administered individually, both cigarette types had similar demand elasticity, suggesting that they had equivalent reinforcing efficacy. However, when self-administered concurrently across equivalent prices, regular cigarettes were strongly preferred to denicotinized ones. In a follow-up study, Johnson et al. (2004) examined substitutability of these different cigarettes by providing denicotinized cigarettes at a consistently low price while the price of regular cigarettes was increased. They found that demand for regular cigarettes was more elastic when denicotinized cigarettes were concurrently available compared to when only regular cigarettes were available (i.e., own- vs. alone-price elasticity, respectively; see Hursh and Roma 2016 for a review), and those denicotinized cigarettes fully substituted for regular ones (i.e., their intake increased as consumption of regular cigarettes decreased)(see also Quisenberry et al., 2016). Collectively, these findings indicate that while nicotine is a primary determinant of preference between cigarettes in a choice context, other aspects (e.g., sensory or central nervous system (CNS) effects of non-nicotine tobacco constituents) may contribute to the reinforcing efficacy of cigarettes that is not apparent when only one cigarette type is available.

The present study is an initial attempt to isolate the effect of non-nicotine constituents on the reinforcing efficacy of an EC liquid within a concurrent choice situation. We expanded on our prior work (LeSage et al. 2016b) that assessed, in isolation, demand for nicotine alone and an EC liquid in rats. While no statistical differences in demand elasticity were found in that study, a trend toward greater demand elasticity for EC liquid was apparent. We hypothesized that concurrent access to these alternatives might provide a more sensitive measure to detect differences in reinforcer efficacy, as has been shown previously (see Wade-Galuska et al., 2007). The present study examined the alone-, own- and cross-price elasticity of nicotine and an EC liquid by assessing initial preference between the alternatives and then increasing the unit price of the preferred alternative by escalating the FR value. Differences between demand elasticity when the preferred alternative was the sole commodity (alone-price elasticity) and when the other alternative was concurrently available (own-price elasticity) at a low fixed-price were compared to determine the substitutability of these commodities.

2. Method

2.1. Animals

Male adult Holtzman rats (Harlan, Indianapolis, IN) weighing 300-350 g at arrival were individually housed with free access to water in a temperature- (22° C) and humidity-controlled colony room. Upon arrival, rats were provided free-access to show for one week and then were food restricted to 18 g/day. Protocols were approved by the Institutional Animal Care and Use Committee of the Minneapolis Medical Research Foundation and were in accordance with NIH guidelines set forth in the Guide for the Care and Use of Laboratory Animals (National Research Council, 2011).

2.2. Apparatus

Drug self-administration chambers (Med-Associates, St. Albans, VT) were composed of aluminum and polycarbonate walls and a stainless-steel grid floor. The chamber had three response levers, each with a white stimulus light located directly above, and a house light mounted centrally at the top of the back panel to provide general illumination. The front panel contained two response levers, separated by a food dispenser (not used in this study). The back wall contained one response lever on the left side. Chambers were contained in sound-attenuating boxes equipped with ventilation fans to provide masking noise. Infusion pumps (Model RHSY, Fluid Metering, Syosset, NY) were connected to Tygon tubing that attached to a dual-channel swivel (Instech Inc., Plymouth Meeting, PA) affixed to a counter-balanced arm centered over the experimental chamber. Tubing from the swivel ran through a spring leash that attached to a dual channel harness (VAHD115AB, Instech) worn by the rat. A computer running MED-PC IV orchestrated experimental sessions and recorded data.

2.3. Drugs

A (-) nicotine liquid was obtained from Sigma Chemical Co. (St. Louis, MO) and dissolved in saline (doses expressed as the base). The EC liquid was DK PORT (obtained from Janty USA, http://usa.janty.com), which was selected because of the relatively higher concentration, relative to nicotine, of some minor alkaloids (i.e., nornicotine [0.03%], anabasine [0.16%], anatabine [2.09%]) compared to the previously employed EC liquid (Aroma E-Juice -Dark Honey: nornicotine [0.03%], anabasine [0.18%], anatabine [0.93%]; LeSage et al., 2016b). Per the packaging, the EC liquid was 66% propylene glycol and had a nicotine concentration of 24 mg/ml (vegetable glycerin concentration not reported by manufacturer). The nicotine content of the Nic and EC liquid solutions (range 0.542-0.655 mg/ml mean 0.59 mg/ml) used for self-administration were determined using gas chromatography with nitrogen phosphorous detection, which is standard protocol for our laboratory (LeSage et al., 2016b). Subsequently, the solutions were diluted in saline, and the PH of the solution was adjusted to ∼7.4 with NaOH or HCL. Heparin was added (30 units/ml) to aid catheter patency.

2.4. Procedure

2.4.1. Jugular and femoral catheterization surgery

Rats were implanted with a chronic indwelling catheter into the right jugular and left femoral veins according to our standard procedures (LeSage et al., 2003, 2002). The catheters exited the body between the scapulae and attached to the vascular-access harness. Rats were anesthetized with i.m. ketamine (75-90 mg/kg) and dexmedetomidine (0.25 mg/kg) and then recovered for four days. During recovery, rats were given daily i.v. catheter flushes of heparinized saline (30 units/ml) and ceftriaxone (5.25 mg), and an s.c. injection of buprenorphine (0.05 mg/kg; first two days only) for analgesia. Infusions of methohexital (50 mg/ml, i.v.) were periodically provided to determine catheter patency following each FR progression (see demand assessment procedures below). If a catheter became occluded or lost patency, another catheter was implanted in the other femoral or jugular vein.

2.4.2. Alone-price demand elasticity assessment

Rats (N = 8) were initially trained to self-administer i.v. Nic (0.06 mg/kg/inf; n = 4) or EC liquid (0.06 mg Nic/kg/inf; n = 4) in daily23-h sessions. Drug availability was signaled by illumination of the house light. The location of the active lever on the chamber panels (front right or back left lever) was counterbalanced acrossrats. Responses on the remaining two inactive levers were recorded but had no programmed consequence. During the initial training, drug (100 ul/kg) was delivered (50 ul/s) following completion of a Fixed-Ratio 1 (FR 1) schedule on the active lever. Drug delivery was accompanied by illumination of the stimulus light above the active lever, followed by a 7-secondpost-infusion timeout. After the timeout, the stimulus light was darkened, indicating the return of drug availability. At the start of the first session, chow dust was placed on the active lever to facilitate exposure to the reinforcement contingency. Rats self-administered drug for a minimum of 10 sessions on a FR 1 schedule until drug intake was stable, defined as a 2:1 active to inactive lever pressing (mean of two inactive levers) and no apparent visual trend across the last five sessions (one rat was inadvertently deemed stable after seven sessions on FR 1). Once stable, the FR requirement was increased each session until 0 infusions were earned, per the following progression: 1, 2, 3, 6, 9, 15, 30, 60, 120, 240, 480 (see LeSage et al., 2016). Following the FR progression, the FR was reduced back to 1, and the type of drug was switched (e.g., Nic was switched to EC liquid). Once self-administration stabilized following the drug switch, the FR progression was repeated.

2.4.3. Own-price demand and cross-price elasticity assessment

A separate group of rats (n = 16) was trained to self-administer Nic and EC liquid (0.06 mg/kg/inf nicotine dose for both formulations) in daily 23-h sessions. Each drug was concurrently available on independent FR 1 schedules of reinforcement across two active levers (front right and back left lever), with the location of the Nic and EC liquid counterbalanced across subjects. Drug delivery and the accompanying stimulus conditions were identical to those described previously. Chow dust was placed on both active levers for the first session. Following at least 10 sessions, self-administration of the two alternatives was assessed for stability, which required at least a 2:1 ratio between active (mean of the two alternatives) to inactive lever pressing and a lack of a visually apparent trend over the last five sessions. Once stable, the FR requirement on the alternative with greater self-administration (i.e., the primary adjusted-price commodity) was increased until 0 infusions were earned per the same progression as the alone price condition; the other alternative (i.e., the alternative fixed-price commodity) remained at a FR 1 throughout the progression.

2.5 Data Analysis

2.5.1 Dependent Measures

The main dependent measures were the mean lever presses on the active and inactive lever(s) and the mg/kg intake of nicotine for Nic and EC liquid across each session of the FR progression. Differences in responding across condition type (alone- vs. own-price), formulation (Nic vs. EC liquid) and response operandum (active vs. inactive) were assessed using an analysis-of-variance (ANOVA). To accommodate missing data at higher prices (not all rats were exposed to all prices), active and inactive responding was analyzed up to unit prices (Alone: 1000; Own: 500) where at least 6 of 8 subjects were responding; row means were inserted to replace missing values for the other 2 rats.

2.5.2 Exponential Demand Quantification

To quantify the elasticity for alone- and own-price demand functions, exponential demand curves were fit to nicotine consumption in mg/kg at each FR value for both individual subjects and group means using the Hursh and Silberberg (2008) demand equation:

$$logQ=log{Q}_0+k(e^{-\alpha Q_{0}C}-1)$$ (1)

In this equation, Q is the quantity of a commodity consumed (mg/kg of Nic), C is unit-price cost of the commodity (FR/infusion), and Q₀ and α are free parameters resulting from the best-fit function and refer to maximal consumption at zero price (i.e., demand intensity) and rate of change in consumption across price (i.e., demand elasticity), respectively. The scaling parameter, k, is a constant that is fit globally across groups to normalize consumption. Such normalization allows for comparisons of free parameter estimates (i.e., α and Q₀) of individual subjects between the different demand functions. Specifically, differences in demand elasticity were quantified using α, which is inversely related to reinforcer strength or essential value and characterizes how rapidly consumption decreases in response to increased price. Commodities that have larger α values have more elastic demand (i.e., rapid decrease in consumption) and less essential value, whereas those with smaller α values have more inelastic demand (i.e., a slower decrease in consumption) and essential value. An Excel template (Kaplan & Reed, 2014) was used to calculate Pmax and Omax values (Hursh, 2014) for each subject using the group fit k (2.407) and the individually fit Q₀ and α values. The value of individually fit Q₀, α, P_max and O_max values were employed as the primary analysis to assess differences both within and between Nic and EC liquid during alone- and own-price demand functions using independent-samples t-tests (log transforms were used to normalize α values). A secondary analysis on the demand functions was conducted using an Extra Sum-of-squares (ESS) F-test to determine if a shared or different α values provided a better curve fit to the nicotine alone and EC liquid group data; this analysis is common and was included to highlight how this approach impacts interpretation of the present dataset. To provide a complete demand function, unit prices where consumption was 0 were replaced with 0.01 since 0 is undefined on a log scale and the log of 0.01 (i.e., log 0.01 = -2) is the lowest log-unit value below the log of 1 infusion (i.e., log 0.06 = -1.22). Additionally, to make group fits of demand functions more representative, 0 infusions (i.e., 0.01) were interpolated for each subject from the point where 0 infusions were earned to the highest unit price achieved within the group. These interpolated data were not used to determine the individual-subject fits of demand curves, but were used to conduct the ESS F-tests.

2.5.3. Cross-price Demand Quantification

To quantify cross-price elasticity, the consumption of the fixed-price alternative commodity across unit prices were fit to group mean and individual data using the Hursh and Roma (2013) cross-price demand equation:

$$Q=log{Q}_{\text{alone}}+I{e}^{-\beta \mathrm{C}}$$ (2)

where Q is consumption of the alternative commodity, Q_alone is the maximum level of consumption of that commodity when the price for the adjusted-price primary commodity approaches infinity, I is the interaction constant, β is sensitivity of consumption of the alternative to the change in unit price of the primary commodity and C is the cost of the primary commodity. The value of I quantifies the range, in log units, of consumption observed in the alternative from the lowest unit price to Q_alone. The value of I serves to quantify the relationship between the primary and alternative commodities, with positive values indicating the alternative functions as a complement and negative values indicating it functions as a substitute. To compare individually fit I and β values between Nic and EC liquid, independent-samples t-tests were conducted. Values more than two standard deviations from the mean were considered outliers. For 3 rats best-fit Q_alone values were high (e.g., ∼10 fold higher than observed) due to near linear fits. To correct for this issue, Q_alone values were fixed at the level of consumption at the highest unit price prior to fitting cross-price functions (note: conducting additional sessions at unit prices beyond when primary consumption reaches 0 infusions would avoid this issue).

2.5.4. Commodity Relation Index (CRI)

The nature of the economic relationship between the primary and alternative commodity is not entirely captured by I since it only captures the absolute log unit change in consumption of the alternative. Additionally, since I is expressed in log units, values close to 0 will produce a skewed distribution toward large negative values. A solution is to calculate a Commodity Relation Index (CRI) that quantifies the proportional change in consumption of the alternative commodity relative to the change in the primary. Such an index expresses the degree to which the alternative functioned as a complement or substitute as a relative proportion. To calculate the CRI, the demand intensity (y-intercept) or Q_{0 cross} of the alternative needs to be calculated using Q_alone and I:

$$Q_{0\mathit{\text{cross}}}={10}^{\mathit{\text{Log}}(Q_{\mathit{\text{alone}}})+I}$$ (3)

and once calculated, it is incorporated with Q_alone and Q_{0 own} of the own-price (primary commodity) demand function to determine the CRI:

$$\text{CRI}=\frac{Q_{\mathit{\text{alone}}}-Q_{0\mathit{\text{cross}}}}{Q_{0\mathit{\text{own}}}}$$ (4)

where CRI values closer to 1 indicate the alternative functions as a full substitute (i.e. the increase in consumption of the alternative is proportional to the decrease in the primary), values of -1 would indicate it functions as a complete complement (i.e. the decrease in consumption of the alternative is proportional to the decrease in the primary), and values close to 0 indicate it functions as an independent relative to the primary commodity.

2.5.5. Relationship Among Price Sensitivity Parameters

The relationship between the values of α and β were assessed with a Pearson correlation coefficient. Theoretically, these price sensitivity parameters should be positivity correlated between these two demand functions if they functioned as substitutes and changes in consumption mirror each other across unit price.

3. Results

3.1. Alone-price Demand

The upper panel of Figure 1 shows mean active (circles) and inactive (triangles) responses for Nic (filled symbols) and EC liquid during the alone-price condition. Active lever responding was significantly higher than inactive, which was confirmed by a main effect of lever type for both Nic (F _{1, 7} = 18.88, p < .001) and EC liquid (F _{1, 7} = 53.45, p < .001). In general, active lever pressing produced an inverted-U shaped function across unit price during the alone-price demand functions for both formulations. There was no effect of nicotine formulation, but there was an effect of Unit Price (F _7,49 = 9.07, p < 0.001) and an interaction between Unit Price and formulation (F _7,49 = 2.85, p < 0.05), indicating that responding for EC liquid increased to a greater degree as a function of unit price than Nic. Responding for EC liquid was significantly higher than for nicotine alone at the 100 (t ₄₉ = 3.43, p < 0.05) and 150 (t ₄₉ = 2.88, p < 0.05) response/mg Nic unit prices per a Sidak post-hoc comparison. The lower panel of Figure 1 plots the consumption of Nic and EC liquid during the alone-price demand condition. Consumption of Nic and EC liquid were similar at the lowest unit price and exhibited a similarly decreasing exponential demand function. According to a dependent-samples t-test, there were no significant differences in the value of Q₀ (demand intensity) or α (demand elasticity) from the exponential demand equation (see Table 1 for parameters).

Figure 1.

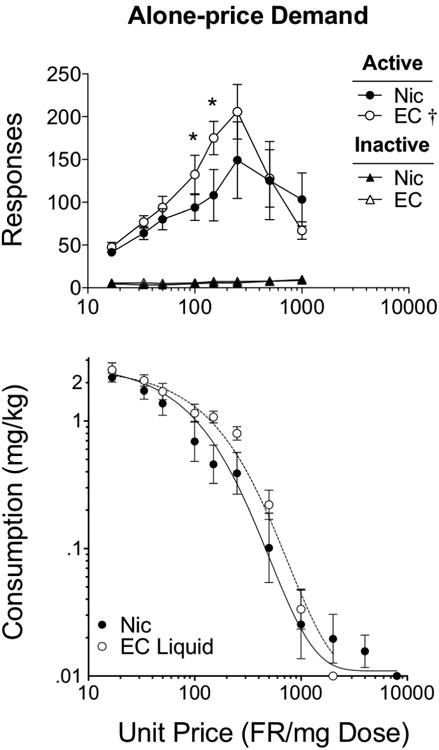
Group mean active (circles) and inactive (triangles) responses for Nic (filled symbols) and EC liquid (open symbols) across unit price during the alone-price condition (upper panel). The resulting consumption (lower panel) is fit with the exponential demand equation (see Table 1 for parameter fits). † = significant (p < 0.05) difference in active responding between Nic and EC liquid. * Significant post-hoc difference (p < 0.05) between Nic and EC Liquid. (Note: response output data is presented up to unit prices where 6 of 8 rats produced responding sufficient to earn reinforcement).

Table 1.

Exponential Demand Curve Parameters for Individual Rats (k = 2.407).

Alone-Price Demand						Own-Price Demand
Nicotine
ID #	α	Q0	Pmax	Omax	r2	ID #	α	Q0	Pmax	Omax	r2
1	0.00019	1.56	780.64	393.67	0.97	9	0.00236	2.97	32.42	31.18	0.9
2	0.00013	2.30	781.97	580.89	0.96	10	0.00549	3.66	11.32	13.41	0.88
3	0.00085	2.75	96.95	86.33	0.98	11	0.00144	2.76	57.46	51.26	0.94
4	0.00257	4.72	18.76	28.64	0.92	12	0.00115	4.48	44.15	63.99	0.94
5	0.00062	3.04	120.25	118.40	0.94	13	0.00078	4.74	61.54	94.46	0.87
6	0.00116	3.30	59.31	63.33	0.92	14	0.00353	3.32	19.43	20.89	0.86
7	0.00114	1.79	111.41	64.44	0.99	15	0.00294	1.24	62.43	25.1	0.82
8	0.00063	2.49	144.64	116.34	0.91	16	0.00261	2.18	40.06	28.25	0.85
							0.00254
Mean	0.00091	* 2.74	264.24	181.51	0.95	Mean	*	3.17	41.1	41.07	0.88
SEM	0.00027	0.35	113.65	69.80	0.01	SEM	0.00054	0.41	6.79	9.61	0.02
EC Liquid
1	0.00036	3.12	204.06	206.15	0.96	17	0.00079	1.95	148.26	93.58	0.91
2	0.00031	3.37	214.45	234.13	0.96	18	0.00110	3.60	57.52	67.02	0.93
3	0.00054	2.66	157.05	135.32	0.98	19	0.00149	3.68	41.54	49.47	0.96
4	0.00052	1.70	257.81	142.03	0.94	20	0.00202	0.70	160.15	36.50	0.68
5	0.00058	2.97	131.47	126.34	0.90	21	0.00112	3.74	54.35	65.76	0.93
6	0.00053	3.94	108.18	137.93	0.94	22	0.00136	2.34	71.49	54.08	0.87
7	0.00058	1.41	280.54	127.76	0.97	23	0.00232	2.95	33.23	31.69	0.84
8	0.00070	2.99	108.82	105.31	0.94	24	0.00160	3.57	39.90	46.12	0.97
				151.87			0.00147			55.53
Mean	0.00052	* 2.77	182.80	*	0.95	Mean	*	2.82	75.81	*	0.89
SEM	0.00004	0.30	23.52	15.63	0.01	SEM	0.00018	0.38	17.65	7.00	0.03

^*- Significant difference between alone- and own-price demand assessments

Note: Non-normally distributed data were log-transformed prior to statistical analysis. Non-transformed data are presented in the tables.

3.2. Own-price Demand

Figure 2 plots infusions for Nic and EC liquid in the own-price demand rats that self-administered more Nic (left panel) or EC liquid (right panel) across the 10 baseline sessions preceding own- and cross-price demand assessment. Self-administration of the alternative with higher intake did not significantly differ between groups. However, baseline self-administration of the alternative with lower intake was significantly higher for the EC liquid compared to Nic, F _1,142 = 12.61, p < 0.05.

Figure 2.

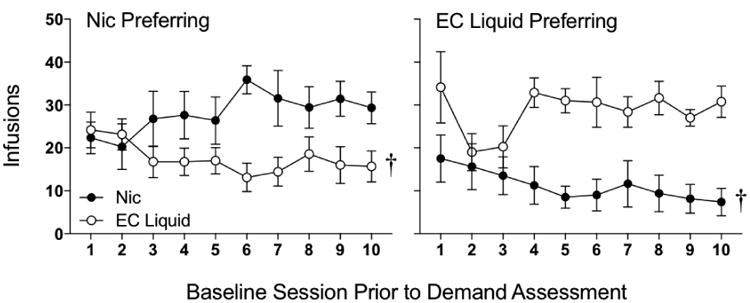
Mean (± S.E.M.) group baseline infusions of Nic and EC liquid in rats that had concurrent access to both alternatives during the 10 baseline sessions preceding the own- and cross-price demand assessment. Rats with higher self-administration of Nic (n = 8) and EC liquid (n = 8) are plotted in the left and right panels, respectively. † = significant (p < 0.05) difference between non-preferred alternatives between groups.

The upper panel of Figure 3 shows mean active (circles) and inactive (triangles) response for Nic (filled symbols) and EC liquid during the own-price condition. Active lever responding was significantly higher than inactive, which was confirmed by a main effect of lever type for both Nic (F _{1, 7} = 21.33, p < .001) and EC liquid (F _{1, 7} = 16.76, p < .001). In general, active lever pressing exhibited a much flatter inverted-U shaped function across unit price in the own-price condition compared to the alone-price functions (Figure 1 - top panel). An examination of own-price active lever responding revealed a main effect of unit price (F _8,84 = 4.14, p < 0.001), but no effect of the formulation or an interaction between these factors. Active lever responding for Nic and EC liquid were higher under the alone-price condition than the own-price one, which was confirmed by the main effect of condition in both Nic (F _1,14 = 7.60, p < 0.05) and EC (F _1,14 = 21.48, p < 0.001). The lower panel of Figure 3 plots the consumption of Nic and EC liquid during the own-price demand condition. Consumption of Nic and EC liquid were similar at the lowest unit price, and both decreased exponentially with increases in unit price. There were no significant differences in the individual fits of the Q₀ or α parameters from the exponential demand equation (see Table 1).

Figure 3.

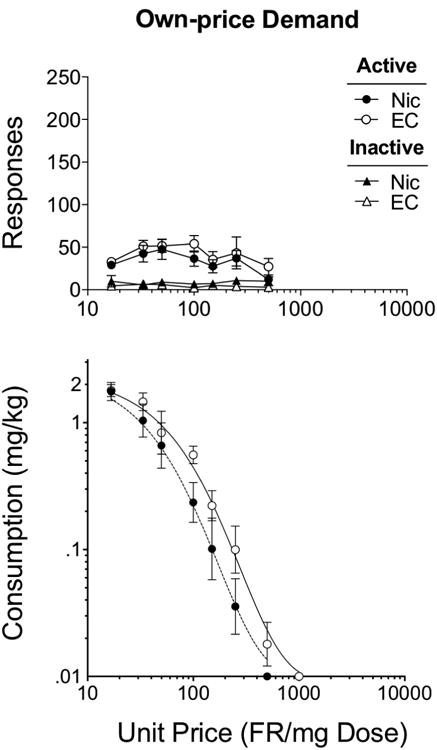
Group mean active (circles) and inactive (triangles) responses for Nic (filled symbols) and EC liquid (open symbols) across unit price during the own-price condition (upper panel). The resulting consumption (lower panel) is fit with the exponential demand equation (see Table 1 for parameter fits). (Note: response output data is presented up to unit prices where 6 of 8 rats produced responding sufficient to earn reinforcement).

3.3. Assessment of Cross-price Demand and Comparison of Alone- and Own-price Demand

Figure 4 replots the consumption of Nic and EC liquid from the alone-, own- and cross-price demand conditions shown in Figures 1 and 3 to allow comparison across demand conditions. In general, Nic and EC alone-price demand functions were more inelastic than the own-price functions. The best-fit parameters for these functions (alone-price in Fig 1. – Lower Panel and own-price in Fig. 3 – Lower Panel) are provided in Table 1 (k set to 2.407 to allow comparison of parameters between groups and conditions). Demand was significantly more elastic (larger α) in the own-price condition group compared to the alone-price condition group for both the Nic (t₁₄ = 2.92, p < 0.05) and EC (t ₁₄ = _6.62, p < 0.001) formulations. Additionally, within the EC formulation there were a significantly lower Pmax (t ₁₄ = 3.64, p < 0.01) and Omax (t ₁₄ = 5.63, p < 0.001) values in the own-price group compared to the alone-price one.

Figure 4.

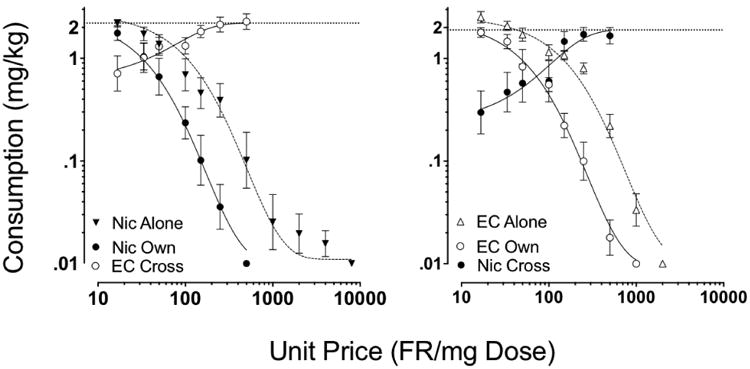
A summary comparison of alone-, own- and cross-price demand functions for Nic and EC liquid. Data points are fit with predicted curves generated from the exponential demand (alone- and own-price) and cross-price demand equations. Parameter fits of exponential and cross-price demand equations are provided in Tables 1 and 2.

Both Nic and EC served as substitutes for the other formulation. Table 2 presents the best-fit parameters and the CRI for the cross-price demand functions for Nic and EC liquid. Values of Q_alone, Q_{0 cross}, I, β, and CRI were not significantly different between nicotine formulations. CRI values were significantly greater than 0 for both the EC liquid (t ₇ = 3.24, p < 0.05) and Nic (t ₇ = 3.71, p < 0.01), suggesting both formulations served as substitutes for each other. Values of I were significantly less than 0 for the EC liquid (t ₇ = 3.08, p < 0.05), but not for Nic. However, with the outlier removed, values of I for Nic were significantly less than 0 (t ₆ = 3.34, p < 0.05). Overall, both Nic and EC served as symmetrical substitutes for one another.

Table 2. Cross-price Demand Curve Parameters for Individual Rats.

EC Liquid Cross-Price Demand							Nicotine Cross-Price Demand
ID #	Qalone	I	β	Q0 cross	CRI	r2	ID #	Qalone	I	β	Q0 cross	CRI	r2
9	1.49	-1.74	0.103	0.03	0.49	0.81	17	1.32	-1.06	0.002	0.11	0.62	0.69
10	1.79	-0.89	0.055	0.23	0.43	0.90	18	2.53	-3.05	0.032	0.00	0.70	0.80
11	3.05	-0.70	0.008	0.61	0.89	0.88	19	2.80	-0.65	0.011	0.63	0.59	0.90
12	4.18	-0.58	0.019	1.11	0.69	0.96	20	1.38	-19.5	1 0.160	0.00	1.97	0.96
13	1.57	0.16	0.028	2.28	-0.15	0.17	21	2.18	-0.35	0.010	0.97	0.32	0.42
14	1.32	-2.30	0.030	0.01	0.39	0.95	22	1.98	-1.04	0.004	0.18	0.77	0.64
15	1.86	0.02	0.012	1.95	-0.07	0.01	23	1.23	-1.58	0.011	0.03	0.41	0.72
16	1.80	-0.63	0.007	0.42	0.63	0.78	24	1.49	-0.47	0.037	0.50	0.28	0.88
Mean		-0.83					Mean					0.71
	2.13	†	0.033	0.83	0.41	† 0.68		1.86	-3.47	0.033	0.30	†	0.75
SEM	0.35	0.29	0.011	0.31	0.13	0.13	SEM	0.21	2.31	0.019	0.13	0.19	0.06

^†- significantly (p < 0.05) different than 0

Note: Non-normally distributed data were log-transformed prior to statistical analysis. Non-transformed data are presented in the tables.

3.4. Assessment of Differences in Demand Elasticity Based on Group Data

The ESS F-test, which determines if the same or different parameter values produce better fits for the different data sets, was also employed to examine differences in demand elasticity between demand conditions and nicotine formulations. Unlike the t-tests from the previously reported individual subject fits, a comparison of formulations within demand conditions revealed that different α values produced significantly better fits both within the alone- (F_1,156 = 5.37, p <0.05) and the own-price demand conditions (F_1,116 = 5.39, p < 0.05). Like the t-tests from the previously reported individual subject fits, a comparison of alone- and own-price demand conditions within formulations revealed that different α values produced significantly better fits both for the Nic (F_1,140 = 37.22, p < 0.001) and the EC liquid (F_1,132 = 88.67, p < 0.001)formulations.

3.5. Relationship Between Price Sensitivity Parameters

Figure 5 illustrates the relationship between the log values of α and β, which are the price sensitivity parameters from own- and cross-price demand equations, respectively. The present set of data only tended toward a positive relationship (r = 0.39, p = 0.14).

Figure 5.

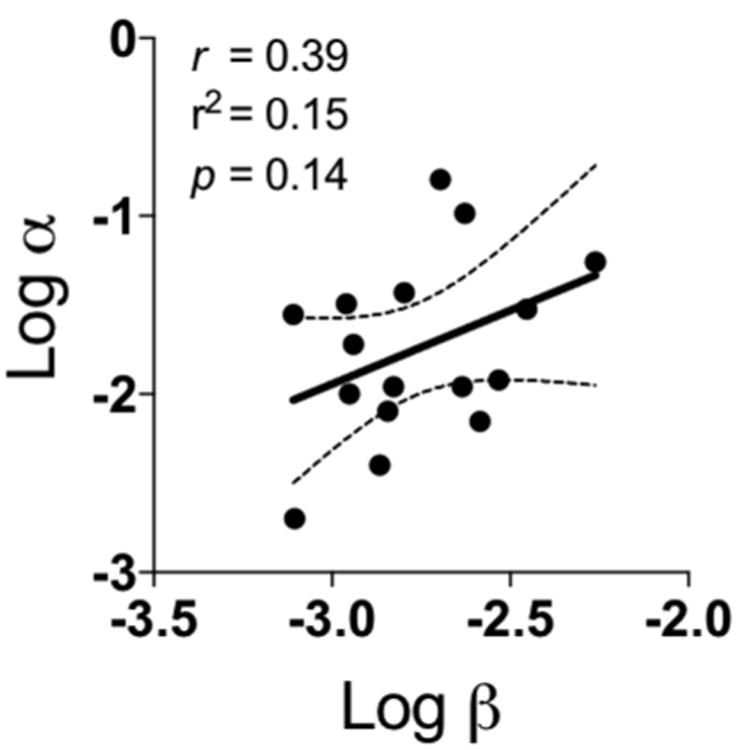
A within-subject correlation of Log α and Log β derived from the own and cross-price demand functions, respectively; both parameters quantify price sensitivity in their respective demand equations.

4. Discussion

The present study is the first to examine concurrent choice between two different nicotine formulations in nonhumans and suggests an approach that future studies could use to model the choices smokers have between tobacco products. Demand elasticity of Nic and an EC liquid were assessed when each was available alone (alone-price demand; see Figure 1) and under conditions where the other was concurrently available at a low fixed-price (own-price demand; see Figure 3). Similar to previous research with other drug types (Spiga et al. 2005; Wade-Galuska et al. 2007, 2011), demand for both formulations was more inelastic (lower α) when each was available alone compared to when the other was available as a substitute (See Table 1). Within each condition (alone- and own-price), however, there were no differences in demand elasticity between formulations, suggesting that the abuse liability of EC liquid was not altered by the non-nicotine constituents present in the EC liquid. These findings are consistent with the LeSage et al. (2016b) finding from our lab that found little evidence that non-nicotine tobacco constituents alter the abuse liability of another EC liquid, suggesting that demand for these formulations under single reinforcer conditions was predictive of demand under concurrent access conditions.

Differences in cross-price demand elasticity (Figure 4) were visually apparent, but were not statistically significantly different. Traditionally, the change in consumption of the fixed-price alternative is fit with a linear regression (e.g., Johnson and Bickel 2003) or visually analyzed (e.g., Wade-Galuska et al. 2007). In the present study, we fit these functions using the cross-price demand equation (Hursh and Roma, 2013), which provided better fits than a linear regression (although note that in Table 2 a few subjects had poor fits due to variability in consumption, an issue that issue that could be dampened in the future by conducting additional sessions at each unit price). The enhancement in fits with the cross-price demand equation should not be surprising given the nonlinear pattern of the data and the addition of two parameters in the model. The cross-price demand equation also provides additional parameters that quantify the relationship between commodities, including unit price sensitivity (β) and the log change in consumption of the fixed-price alternative (I). Both parameters provide useful information to understand the nature of the relationship between the primary and alternative commodities.

The sensitivity parameter may prove useful in future studies to determine how tightly related price sensitivity is between commodities. Specifically, the values of α and β should be correlated since both measure price sensitivity within the own- and cross-price demand functions, respectively. Although not statistically significant, there was a trend toward a positive relationship between these sensitivity measures (Figure 5). In future work, one could use the correlation between the values of α and β as an index of the strength of interdependence between reinforcers. Presumably, the higher the correlation and its goodness-of-fit, the stronger the interdependence between reinforcers. A stronger correlation might have been observed in the present study if assessments at each unit price were conducted over several sessions to allow consumption to become more stable.

Hursh and Roma (2013) propose that I can be used to determine if the fixed-price alternative functions as a substitute (- I) or complement (+ I) since it quantifies the log change in consumption of the alternative. The value of I, however, provides no indication for the degree of substitutability or complementarity (e.g., full or partial), which would be captured by the change in consumption of the primary commodity relative to that of the alternative. Therefore, we propose the use of the CRI as a novel metric that quantifies the degree to which the alternative functions as a complement, substitute or an independent (see Equation 4). The CRI provides advantages over I since it not only quantifies the change in consumption of the alternative, but it is also an index that is relative to the change in consumption of the primary commodity. Another advantage of using CRI is that values of I are skewed toward large negative values when functions are fit to near 0 levels of consumption at the lowest unit price (see Subject 20 in Table 2). Thus, the CRI is useful in that it provides an index of the degree and the direction of the economic relationship between two reinforcers that is not entirely captured by I.

In the present study, differences in demand elasticity across nicotine formulations and demand conditions were conducted using exponential (α) and cross-price (β) demand curves fit to individual-subject data (see Tables 1 and 2). An alternative method often used in behavioral economic studies is the ESS F-test to determine if curve fits are significantly improved between conditions or groups when the same or different parameter values are used (e.g., do different α values reduce total variance?). We found that the ESS F-test produced less conservative statistical findings, with the conclusion that alpha was significantly different between formulations, both within the alone- and own-price demand conditions. One factor that made this test less conservative is that individual variability in the demand functions are dampened because the ESS F-test ignores the range between each demand function by only using the mean and SD at each unit price. Moreover, it ignores the fact that the data are correlated across unit prices, violating the assumption of independence of observation. We suggest that caution is exercised when interpreting treatment effects on demand parameters using the ESS F-test and instead, recommend that researchers use parameters from individual-subject demand curve fits to make comparisons between conditions. This point is especially pertinent when data is extrapolated to inform public policy where individual differences may have a substantial impact on policy decisions (e.g., setting product standards to protect vulnerable populations).

The main implications of the present study for tobacco regulatory policy are that non-nicotine tobacco constituents present in the EC liquid tested are not contributing significantly to the CNS-mediated reinforcing effects of this product. However, the non-nicotine constituents may contribute to abuse liability via their peripheral sensory effects (e.g., taste). These findings mirror our previous work (LeSage et al., 2016b) and suggest that, at least for the EC refill liquids tested thus far, nicotine may be the primary determinant of the abuse liability of these products. Any FDA efforts to reduce their addictiveness could involve setting nicotine standards if deemed appropriate for the protection of public health. As discussed previously, the levels of non-nicotine constituents are relatively low in e-cigarettes compared to those measured in smokeless tobacco or conventional cigarette smoke (e.g., Brennan et al., 2015), which leaves open the possibility that non-nicotine constituents could contribute to demand elasticity for other types of tobacco products.

There are a few issues that could limit the interpretation of the present study. First, there were no forced-choice periods in the choice assay to ensure exposure to both reinforcers and minimize the development of a position preference (other than baiting the levers during the first session). However, position bias was controlled by design (counterbalancing) and all rats responded on both levers to experience the drug alternatives during baseline. Moreover, there was no relationship between drug preference and the location of the lever in the operant chamber. Second, preference between the two nicotine formulations was not directly assessed via a preference reversal, which would have controlled for any side-bias that may have confounded preference for one formulation over the other. However, since differences in demand elasticity were not observed between formulations across different demand assessments, it appears to be a minor issue for the interpretation of the present findings. Third, exponential demand functions are typically only conducted up to a unit price that produces 0 infusions. With own-price demand assessments, however, it would be advisable to examine at least two additional unit prices beyond where 0 infusions are earned to ensure that the cross-price demand equation can properly estimate Q_alone when consumption of the alternative commodity hits an asymptote. Fourth, because this was an initial attempt to study choice between nicotine formulations, the sample size was based on that used in our previous studies using single-reinforcer procedures (e.g., LeSage et al. 2016b). Given the trends apparent in the present study, higher sample sizes may be needed to confirm whether these represent meaningful effects. Finally, because saline was not offered as a control option (i.e., the same cues used, but saline is delivered), it is not possible to ascertain to what extent cues contributed responding for the drug options versus the drugs per se. The influence of cues was beyond the scope of this initial choice study but is an important future focus.

In summary, the present study found that availability of an alternative nicotine formulation produced a similar increase in elasticity of demand for Nic and EC liquid. This indicates that the abuse liability of both nicotine formulations decreased when a low-priced substitute was concurrently available. Within the alone- and own-price conditions, there was no difference between formulations, which suggests that the non-nicotine constituents present in the EC liquid did not contribute substantially to its CNS-mediated reinforcing effects. There were also no differences in cross-price demand, which indicates that both Nic and EC liquid served as symmetrical economic substitutes for one another. The present findings demonstrate the sensitivity and potential utility of concurrent choice procedures in animal models for assessing the relative abuse liability and substitutability of tobacco products.

Highlights.

• Preference for nicotine alone and E-cigarette liquid formulations in rats.

• Assessed impact of non-nicotine constituents on abuse liability of nicotine.

• Abuse liability assessed using behavioral economic demand functions.

• A novel cross-price demand function to quantified formulation substitutability.