Microtubule Dynamics: Moving Towards a Multi-Scale Approach

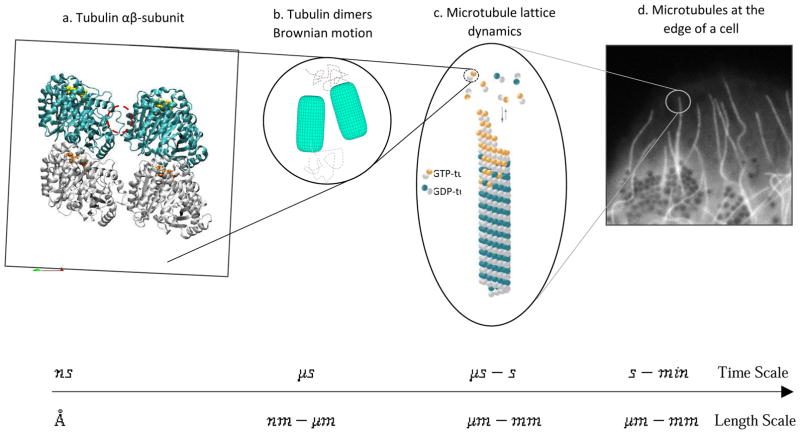
Abstract

Microtubule self-assembly dynamics serve to facilitate many vital cellular functions, such as chromosome segregation during mitosis and synaptic plasticity. However, the detailed atomistic basis of assembly dynamics has remained an unresolved puzzle. A key challenge is connecting together the vast range of relevant length-time scales, events happening at time scales ranging from nanoseconds, such as tubulin molecular interactions (Å-nm), to minutes-hours, such as the cellular response to microtubule dynamics during mitotic progression (μm). At the same time, microtubule interactions with associated proteins and binding agents, such as anti-cancer drugs, can strongly affect this dynamic process through atomic-level mechanisms that remain to be elucidated. New high-resolution technologies for investigating these interactions, including cryo-electron microscopy (EM) techniques and total internal reflection fluorescence (TIRF) microscopy, are yielding important new insights. Here, we focus on recent studies of microtubule dynamics, both theoretical and experimental, and how these findings shed new light on this complex phenomenon across length-time scales, from Å to μm and from nanoseconds to minutes.

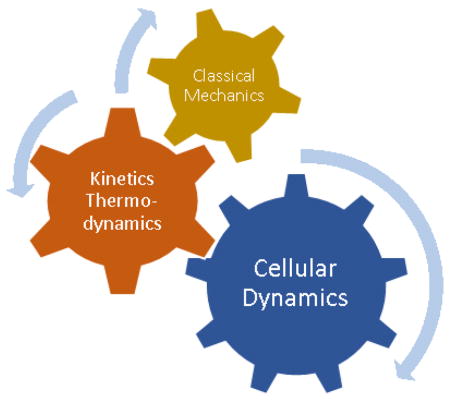
Graphical Abstract

Schematic representation of how classical mechanics of molecular interactions working in harmony with kinetics and thermodynamic fundamentals of a biological system will explain their complicated dynamic behavior at cellular level like a mechanical gear system meshing together to transmit information

Introduction

Microtubules (MTs), filamentous structures self-assembled from αβ-tubulin heterodimers, constitute an essential part of the cytoskeleton of eukaryotic cells. Microtubules perform diverse cellular functions such as cell division, mitosis, and motor-mediated organelle transport, and in general, help establish cellular structure and enable motility. They accomplish subcellular reorganization through their “dynamic instability” behavior, defined as stochastic switching from growth to shortening and back again (referred to as “catastrophe” and “rescue,” respectively) [1, 2]. The guanosine nucleotide state in the β-tubulin subunit has proven to be an important mediator of microtubule assembly dynamics. Whereas guanosine triphosphate (GTP)-tubulin is believed to establish a protective cap at the growing ends of microtubules by being more stable in the microtubule lattice, guanosine diphosphate (GDP)-tubulin is believed to act as a destabilizing factor [3–6]. However, some conflicting views exist regarding the GTP-cap being mainly responsible for stabilization [7,8]. In addition, microtubule associated proteins (MAPs), and microtubule-targeting agents (MTAs) interact with polymerized and un-polymerized tubulin, and consequently influence the dynamic cycle, making them potential pathways for treating cancer and neurodegenerative diseases [3, 9–12]. Considering the fundamental role of microtubules in the cell, recent studies have aimed at understanding how their atomistic-level structure impacts dynamic instability and function. This review focuses on novel computational and experimental approaches that probe the dynamic behavior of microtubules and their interactions with various MAPs and MTAs.

Computational Modeling from Angstroms to Micrometers, and Nanoseconds to Minutes

Microtubules have been a significant subject for computational modeling at different length-time scales. Their dynamic behavior is dependent on hydrolysis of the GTP nucleotide in β-tubulin. This atomic-scale change is believed to be responsible for the ultimate dynamic instability of microtubules [6]. Considering this small-scale event, microtubules and their building blocks, αβ-tubulin dimers, are prominent targets to be modeled at different scales to understand the underlying mechanism of their behavior and the effect of their interactions with MAPs and MTAs. Starting from the atomic-scale, experimental techniques have been notably improved to obtain high-resolution X-ray crystal and, more recently, cryo-electron microscopy (cryo-EM) structures of tubulin, making atomic-resolution modeling possible. These studies provided tubulin structure both when polymerized in a microtubule lattice [13], and when un-polymerized in solution bound to MAPs [14–16]. Of particular note, Zhang et al. (2015) [17**], focused on the structural transitions that occur upon hydrolysis. In contrast to previous studies that noted lateral contacts as the difference between polymerized GDP- and GTP-tubulin [18], they concluded that GDP- and GTPγS-MT lattices are more compact around the longitudinal interface compared to the extended GMPCPP-lattice, confirming the results of their previous study [19]. The end-binding protein EB3 was also shown to have a binding preference for the compacted GTPγS-MT, as the intermediate state of the two other nucleotides. The surge of recent cryo-EM structures has helped the field to improve our understanding of the microtubule interaction with several MAPs that regulate the dynamic behavior such as EB proteins, molecular motors kinesin and dynein, and tubulin tyrosine ligase-like (TTLL7) enzyme [20*].

These three-dimensional structural investigations have provided insight into binding mechanisms in addition to establishing a firm basis for molecular-scale modeling, which can be employed to study the dynamic behavior of tubulin movement, deformations and energetics in an aqueous solution with salts and other interacting molecules. All-atom molecular dynamics (AA-MD) simulations, now extending out to microseconds with the help of parallel graphical processing unit (GPU) computing, have been useful in obtaining an accurate estimate of free energies of binding, solvation, and conformational energies, although they are still computationally expensive [21]. On the other hand, protein-ligand docking studies, trading off speed vs. accuracy, have grown noticeably, especially in drug discovery and mutation probing [22–24]. Tripathi et al. (2016) [25**] investigated leucine point mutations (L215H, L217R, and L225M), known for paclitaxel resistance in cells, using molecular docking, MD simulations and approximate binding energy calculations [26]. They concluded that effects of mutations on M-loop flexibility play a key role in mediating paclitaxel binding strength to tubulin. In another docking modeling and MD simulation study [27**], differential binding affinity of tubulin isotypes αβ_I, αβ_III and αβ_IV for a colchicine analogue, DAMA-colchicine, was monitored. It was revealed that αβ_IV has the highest binding energy for the drug among the three isotypes, although it should be noted that the binding free energy was estimated without taking into account the entropic cost of binding. One of the drawbacks of free energy estimates that neglect full entropy costs is the inability to directly relate these computed energy landscapes to larger-scale coarse-grained simulations, which can capture a wider range of dynamic time- and length-scales. It remains to be demonstrated that these relative docking calculations are sufficiently accurate to predict the interaction differences that influence large-scale events such as tubulin-tubulin bond formation.

In parallel with structural studies and MD simulations, Brownian dynamics and thermo-kinetic simulations have grown rapidly, capturing the kinetics, thermodynamics and mechanics of tubulin addition and loss at microtubule ends at nano- and micro-scales. Different pioneering models vary in their assumptions and parameter space, all trying to provide a framework for explaining microtubule catastrophe, rescue and other related features of dynamic instability. Zakharov et al. (2015) [28**] employed a coarse-grained Brownian dynamics simulation, incorporating thermal fluctuations as well as fluctuations in protofilament tip shapes, beyond those contained in previous simpler thermo-kinetic models [29]. Variations in the extent of protofilament curling at the microtubule tip were identified as the dominant factor causing catastrophe, rather than the loss of GTP-tubulin cap. Moreover, they reported that microtubule aging is traced back to slow evolution of molecular events at the microtubule tip rather than an accumulation of specific permanent defects in microtubule tip or wall. Although emphasizing the critical role of bond energies and elastic deformation, GDP- and GTP-state tubulin were assumed to have similar energetics in their model, both having an energy barrier in their potential of interaction, the justification of which has not been confirmed by molecular simulations. In addition, the GTP-hydrolysis rate was increased 6–18 fold to be able to observe microtubule catastrophe within the model, which deviates from a physiologically-relevant value of ~1 s⁻¹. In another study, Piedra et al. (2016) [30**], favoring the role of a GTP-cap in microtubule stabilization and using a simplified thermo-kinetic model similar to [18], found that adding GDP-to-GTP exchange on terminal subunits results in less frequent catastrophe, an observation that was verified using in vitro assays. In their model, they assumed a trans-acting nucleotide effect, where the nucleotide state of the tubulin subunit underneath the terminal subunit determines the terminal subunit’s energetics, rather than the nucleotide state of the terminal subunit itself (cis-acting nucleotide). This model originates from the finding that previous studies failed to find a structural change in soluble tubulin as a function of nucleotide-state [31–33]. Additionally, a trans-acting nucleotide effect is consistent with the previously noted observation of lattice compaction upon hydrolysis [17]. Still, the question of how nucleotide-state induced conformational changes in tubulin and upon its neighbors translate to changes in kinetics and thermodynamics remains to be clarified.

Recently, by integrating updated thermo-kinetic modeling, previously successful in recapitulating experimental observations of dynamics [29, 34], and fluorescent microscopy, the mechanisms of action of two important MTAs, paclitaxel and vinblastine, were described in the context of kinetic and thermodynamic fundamentals [35**]. Consequently, the employed methodology relates micrometer-scale behavior to nanometer-scale computational modeling parameters, such as hydrolysis rate and bond energy weakening or strengthening. Although, the model could be improved with a more precise knowledge of the tubulin-tubulin interaction energies and the energetic penalty upon hydrolysis. In this study, two distinct mechanisms of kinetic stabilization were identified for the drugs. The first mechanism, associated with paclitaxel, is achieved by eliminating the energetic difference (ΔΔG⁰) between GDP- and GTP-tubulin states without suppressing the kinetic rates. In contrast, the second mechanism, which is consistent with vinblastine, is achieved by reducing the kinetic rates of both association and dissociation of tubulin subunits at the microtubule end. Together, all of these recent coarse-grained studies have moved forward to find a way to translate molecular information to physiological behavior of microtubules both in vitro and in vivo. At the same time, they have failed to take full advantage of molecular all-atom models to further constrain the parameter choices for the model. Thus, to build a truly multi-scale model that explains the dynamic behavior of microtubules starting with atomic level information, it is still necessary to make a direct connection between structural studies and atomic-scale dynamic simulations, ideally extending all the way up to cellular scales (Figure 1).

Figure 1.

Multi-scale approach required for a comprehensive description of the dynamic behavior of microtubules. This multiscale approach requires spatio-temporal bridging of the atomic-scale ensemble to cellular-scale behavior a) structural and molecular dynamics studies, b) coarse-grained Brownian dynamics simulations, c) thermo-kinetic modeling, and d) experimental studies in vitro/vivo to inform whole-cell models.

Experimental Studies on Microtubule Dynamic Behavior Regulation

Microtubule-associated Proteins

For the purpose of controlling the dynamic cycle of assembly and disassembly and successfully accomplishing cellular functions, several proteins, collectively known as MAPs, bind to microtubules. A major group of MAPs that have gained significant attention for their role in reporting on the microtubule protective cap are the end-binding proteins (EBs) [36]. Duellberg et al. 2016 [36 37**] used a microfluidic channel with TIRF microscopy as a tool to directly visualize microtubule stability and EB cap size with improved spatio-temporal resolution. The direct link between the EB binding region and the protective cap causing microtubule stability was detected within their experimental setup. Further, the observation of a transient slow shrinkage phase (~25 tubulin layers) after washout confirmed that a small or single-layer cap model can be ruled out. Their simple threshold model for catastrophe, however, failed to explain the observed fluctuations of the individual delay times after the washout experiments. In a subsequent study, again using their microfluidic assay, microtubule aging and its molecular causes were investigated in the context of a simple kinetic threshold model [38**]. They found that the delay time to catastrophe after tubulin washout depended on the age of the growing microtubule, thus confirming the phenomenon of microtubule “aging,” and challenging recent modeling predictions [28]. While the authors considered a microtubule defect accumulation model [39, 40], they found that experiments failed to reproduce the delay times predicted from the model. Rather, a gradually increasing tip-taper model [41] best described the reduced stability with respect to growth time. In the future, this high resolution experimental method could be integrated with more detailed molecular models, as well as real-time observation of the microtubule tip taper or microtubule defects by structural studies, to explain the precise molecular interaction of EB proteins at the microtubule end.

Another class of noteworthy MAPs are kinesins, motor-proteins that move directionally on the microtubule surface toward the plus-end of microtubules (with a few exceptions), mediating intracellular transport. Since kinesin binds to the tubulin surface, remote from the nucleotide-binding region, it is unclear how any change in this binding pocket will affect the polymerization dynamics. In a recent study, structural and light microscopy data were used to show that disease-related point mutations close to the kinesin binding region in β-tubulin isotype III caused reduced affinity for motor- and non-motor MAPs [42**]. As a result, microtubule polymerization and catastrophe dynamics were altered at both the plus- and minus- end. Several other recent studies have investigated the role of different kinesins in controlling microtubule dynamics and the regulation of their activity by phosphorylation, G proteins, and ion levels [43–46], all ultimately influencing net microtubule assembly dynamics.

Microtubule-targeting Agents

Another important class of molecules that interact with microtubules are so-called MTAs, such as the chemotherapy drugs paclitaxel and vinblastine, which affect cancer cell mitosis and migration as well as tumor angiogenesis. However, cancer cells are capable of developing resistance to these drug compounds, even though they were effective during initial treatment [47]. This has raised the need for a better understanding of how various drugs stabilize microtubule dynamics and how we can rationally identify combination therapies to limit the resistance problem. In one such study [48*], eribulin, a vinca-binding drug used to treat patients with metastatic breast cancer resistant to taxanes [49], was explored for its effects on microtubule stabilization. By the combination of structural data with fluorescence microscopy, the authors predicted that the drug has either a tubulin-sequestering effect or an end-blocking mechanism at the microtubule end that inhibits growth overall, similar to vinblastine’s mechanism at the microtubule end as predicted by thermo-kinetic modeling [35].

Although knowing the actual molecular mechanism of a potent drug has the potential to improve its therapeutic effects, one cannot circumvent the systematic side-effects of anti-cancer drugs traveling through a patient’s bloodstream [50]. Recently, Borowiak et al [51**] introduced a method for controlling microtubule dynamics in space and time by the use of photoswitchable microtubule inhibitors, photostatins (PSTs), which are analogs of the colchicine site binding drug, combretastatin. Strikingly, turning these molecules “on” by ultraviolet (UV) light resulted in a 250 times more cytotoxic effect compared to the “off” molecules kept in the dark. Importantly, even the active-PSTs that diffused out of the targeted space were deactivated spontaneously within 0.8–120 min, limiting their spatial toxicity. However, because active-PSTs were shown to be only effective at micromolar concentrations, it is questionable whether these agents can succeed clinically, and further studies will be required. Furthermore, the side-effects of extended UV exposure required for PST activation in patients has yet to be determined [52]. Collectively, cellular dynamics monitored by these recently developed experimental techniques will be better understood using kinetic and thermodynamic basics and further advanced by linking individual atom’s motions and interactions using classical mechanics and precise force fields.

Future Directions

Considering that microtubule dynamics are essential to cell division and cell migration, we have come a long way in understanding the major causes of this process and its regulation by interacting proteins and agents. With the help of advances in computational resources, such as GPU-accelerated simulations, and high resolution imaging techniques, including TIRF and cryo-electron microscopy, several studies have shed new light on how the dynamic behavior of microtubules is modulated. One potential discrepancy between different modeling assumptions and outcomes is the underlying theoretical information on which the model is based. Questions such as how conformational transitions in tubulin or the microtubule lattice structure will affect the kinetics of assembly and dynamic behavior remain to be answered. In most in vitro/vivo studies, mathematical/physical computational modeling, with a well-established connection to experiments, needs to be integrated to fully explain the observed results. Building fundamentally-sound connections across different length-time scales will require new insights, moving from smaller scales with more degrees of freedom to larger-scale coarse-grained models. In other words, microtubule dynamics is a multi-scale process with various elements affecting the system’s overall behavior, standing in need of a multi-scale approach for studying each small-scale element as part of the big picture. In that perspective, questions such as how tubulin mutations or isoforms change microtubule dynamics in cells or how a specific MAP induces conformational changes within tubulin that will result in a change in assembly dynamics can be thoroughly addressed. In conclusion, while our knowledge of the mechanisms of microtubule dynamic behavior has been increasing, there is plenty of space for improvement in the stitching together of our current information at various scales with a physically-grounded multiscale modeling approach.