Low loss Plasmon-assisted electro-optic modulator

Abstract

For nearly two decades, the field of plasmonics¹ - which studies the coupling of electromagnetic waves to the motion of free electrons in a metal² - has sought to realize subwavelength optical devices for information technology^3–6, sensing^7,8, nonlinear optics^9,10, optical nanotweezers¹¹ and biomedical applications¹². Although the heat generated by ohmic losses is desired for some applications (e.g. photo-thermal therapy), plasmonic devices for sensing and information technology have largely suffered from these losses inherent to metals¹³. This has led to a widespread stereotype that plasmonics is simply too lossy to be practical. Here, we demonstrate that these losses can be bypassed by employing “resonant switching”. In the proposed approach, light is only coupled to the lossy surface plasmon polaritons in the device’s off-state (in resonance) where attenuation is desired to ensure large extinction ratios and facilitate sub-ps switching. In the on state (out of resonance), light is prevented from coupling to the lossy plasmonic section by destructive interference. To validate the approach, we fabricated a plasmonic electro-optic ring modulator. The experiments confirm that low on-chip optical losses (2.5 dB), high-speed operation (>>100 GHz), good energy efficiency (12 fJ/bit), low thermal drift (4‰ K^-1), and a compact footprint (sub-λ radius of 1 μm) can be realized within a single device. Our result illustrates the potential of plasmonics to render fast and compact on-chip sensing and communications technologies.

Telecommunication devices, such as electro-optic (EO) modulators must feature low insertion loss (IL) while providing a large phase (Δn) or amplitude (Δα) change accumulated over a short device length¹⁴. Beyond that, modulators should offer low driving voltages and high-speed operation.

In recent years, silicon photonics has emerged which benefits from low propagation losses (dB/cm) but have struggled to achieve large modulation depth for sub-mm devices (Δn and Δα)¹⁵. State-of-the-art devices maximize their modulation through the use of resonant structures¹⁶, enabling compact (μm²-sized) and energy efficient components^17,18. And while large Q-factors (on the order of a several 1000) enable a large reduction of the driving voltage, they limit the speed and increase the sensitivity of devices to temperature and fabrication fluctuations¹⁹. More recently, surface plasmonic polariton (SPP) devices have exploited the extreme confinement of light to achieve exceptional modulation within a few μm (Δn and Δα)^3,20. However, the metals that bring such promise to plasmonics are also the largest hindrance, as such devices suffer from large on-state loss (~dB/μm). To combat plasmonic losses, some devices employ hybrid-plasmonic-photonic modes^4,6,21 while others minimize the length of the active section^5,22. Still, typical IL of 10 dB due to the plasmonic propagation loss and photonic-to-plasmonic mode conversion loss remain a concern for high-speed state-of-the-art devices^5,6. Thus, plasmonic on-chip technologies have been unable to replace the existing photonic or electronic solutions, placing the field of plasmonics at a crossroad to either abandon development or explore additional solutions.

We propose a novel approach, in which losses in plasmonic waveguides can be selectively used or bypassed to achieve low IL, strong modulation, compact footprint and high speed, simultaneously. This approach relaxes the prior goal to reduce the ohmic loss as much as possible to minimize the device’s on-state loss. Instead, we show that plasmonic losses can be tolerated by designing the device geometry such that light passes through the lossy section when required (in the off-state). To achieve this, we utilize a plasmonic ring resonator coupled to a buried low-loss silicon photonic waveguide, see Figure 1. Unlike prior approaches, which aim to reduce the resonator loss to realize high Q cavities or lasing^7,23–26, our resonator exhibits typical propagation losses within the plasmonic cavity and mostly bypasses this lossy section in the on-state through destructive interference. Using this design, we demonstrate for the first time, a plasmonic modulator that is able to meet the key performance metrics of modern optical communications links.

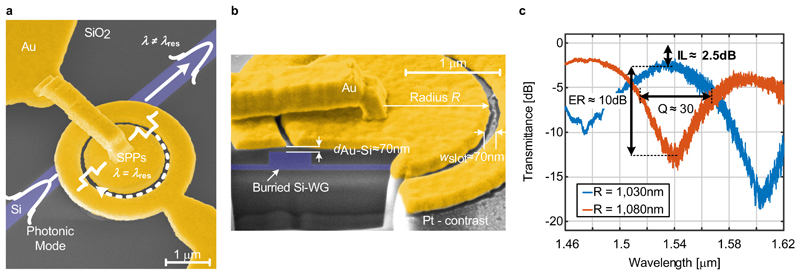
Figure 1.

False-colored SEM image of a plasmonic ring resonator and the corresponding transmittance over wavelength. (a) Top view and (b) cross section of the resonator. Photonic modes propagating in the buried silicon waveguide resonator couple partially to the SPPs in the metal-insulator-metal-ring when the resonance condition is fulfilled. While out of resonance operation results in a low loss light transmission. (c) Passive measurements of two identical ring resonators that only differ in radii (blue - 1030 nm; red - 1080 nm). Due to the resonant approach, insertion losses of 2.5 dB are measured with extinction ratios (ER) above 10 dB.

Figure 1 shows the proposed device geometry, which comprises a gold metal-insulator-metal (MIM) slot waveguide ring coupled to a buried silicon bus waveguide, forming a notch filter with a resonant wavelength of (λ_res) (supplementary information: chapter II). The slot waveguide is filled with an organic electro-optic (OEO) material which alters the device’s resonance condition through the Pockels effect (Δn_SPP)²⁷. This enables a fast and selective use of the resonator’s Ohmic loss to attenuate the signal (Δα) in the bus waveguide by applying a voltage.

Figure 1(c) shows the measured transmittance over the wavelength of two representative devices that differ in radius. For these structures, we have observed a distinct off-resonance (λ₀ ≠ λ_res) and on-resonance (λ₀ = λ_res) condition at the telecommunication wavelength of 1.54 μm with an IL of 2.5 dB, an extinction ratio (ER) of 10 dB, and a Q-factor of ~30. Alternatively, we note that non-resonant devices based on MIM waveguides with a similar length feature an IL ranging from 8-10 dB^5,6,22.

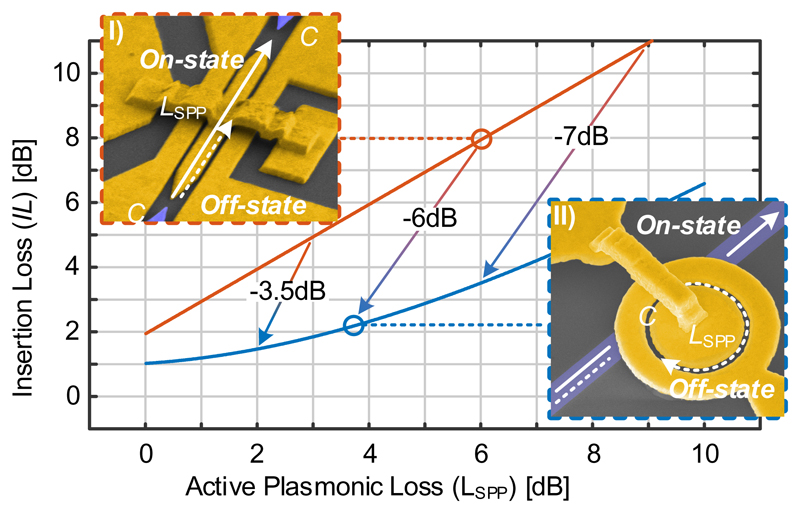
The reduced IL can be understood by comparing the exemplary operating principles of a non-resonant Mach-Zehnder (MZ)⁵, Figure 2 (I) and a resonant ring, Figure 2 (II). The IL of the device is a function of its coupling efficiency, geometry and accumulated ohmic loss. In both concepts, light couples to and from the plasmonic structure with a coupling efficiency (C). A transmission modulation is then induced by the Pockels effect over an active plasmonic section of length or circumference (l). The modulation depth (ΔT) – and also the loss (L_SPP) – scale with the length of the active plasmonic section⁶. Figure 2 shows the overall IL over the L_SPP in the active plasmonic area for a MZ and a critically coupled resonator. The arrows indicate the performance of devices with an equal ΔT.

Figure 2.

Theoretical loss advantage of critical coupled resonant over non-resonant push-pull Mach-Zehnder devices. The IL are plotted over the active plasmonic loss (L_SPP) in the slot waveguide of the MZ (left inset, red) and resonator (right inset, blue). Losses can be reduced by more than 6 dB. This is due to following reasons: I) bypassing mechanism – only a fraction of light experiences plasmonic losses; II) resonant enhancement – resonators achieve the same modulation depth for shorter devices than their non-resonant counterpart (indicated by the arrows); and III) coupling scheme – non-resonant approaches require two photonic/SPP converters while resonant approaches require only one.

The following points arise from Figure 2. First, the resonator’s total loss is always smaller (blue curve < red curve) due to the bypassing mechanism²⁸ . The IL increases with L_SPP (i.e. inverse to the cavity’s Q-factor) as the critical coupling condition requires that more light couples to the plasmonic section. Second, the resonator has a 1 dB lower IL at L_SPP ≈ 0 dB. This is because the non-resonant device requires two photonic-SPP converters as both the on- and the off-state propagate through the plasmonic section. Ohmic losses in the converters limit the conversion efficiency (C) to ~1 dB^22,29. Contrarily, the selection mechanism of the resonator (on-state→bus waveguide; off-state→ ring) requires a converter which couples only a fraction of the light to the ring. Third, in the ring we take advantage of the resonantly enhanced ΔT to reduce the device length (supplementary information: chapter VII). For our resonant structures, we have calculated an enhancement of ~1.5. Consequently, our ring with a circumference of l = 6 μm (L_SPP ≈ 4 dB) offers the same transmittance change as a MZ of l = 9 μm (L_SPP ≈ 6 dB). In total, the ring device offers a 6 dB IL advantage over the MZ modulator. Additionally, losses can be further reduced by under-coupling the resonator as limited ERs of 10 dB are sufficient for many practical applications³⁰.

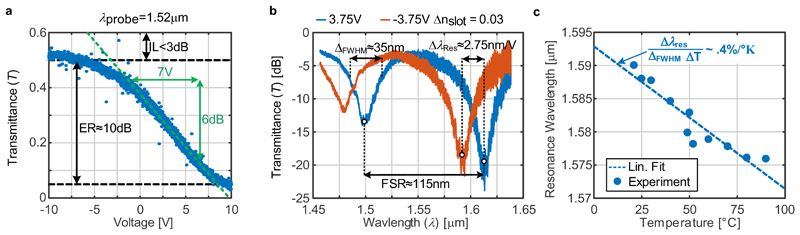
To illustrate the modulation performance (ΔT) of the plasmonic resonator, the SPPs’ effective refractive index (Δn_SPP) is altered by applying a bias between the inner and outer rings²⁷. Figure 3 (a) shows the transmitted power versus applied voltage for a wavelength of ~1.52 μm. We observed an IL < 3 dB, an ER of ~10 dB, and a linear response (dashed green line) for a peak voltage of 3.5 V with an ER of ~6 dB. This performance in terms of IL and ER is similar to well-developed CMOS photonic resonators¹⁹. We estimate that operation under a digital driving voltage (1 V_pp)¹⁹ is achievable in the near future by utilizing other plasmonic materials like silver or copper, using the newest OEO-materials and improving fabrication (methods: future improvements). To highlight the mechanism of the modulation the transmission of the device has been measured under a positive and negative bias of ±3.75 V as a function of wavelength, see Figure 3(b). This results in a normalized sensitivity (S_λ/Δ_FWHM = Δλ_res/(Δ_FWHM·Δn)) of ~17 RIU^-1, assuming a relative change in OEO’s refractive index of ~0.03. This can be compared to commercial SPR sensors that achieve values of 50 RIU^-1 using a free-space Kretschmann configuration³¹. So, currently our approach is already close to the non-integrated free space approaches.

Figure 3.

Sensitivity and stability of the plasmonic resonator. (a) Voltage sensitivity of the resonator’s transmittance. (b) Sensitivity of the ring as a function of the wavelength. A change (Δn_slot≈0.03) in the refractive index of the slot-filling material causes a large change of the resonance wavelength (blue/red). (c) The resonator shows stable operation across a large thermal variation. These characteristics make the plasmonic MIM-ring resonator a promising candidate in the field of optical modulators and sensors.

The moderate Q-factor guarantees a high operational speed and provides good thermal stability. For example, the measured resonance frequency is plotted in Figure 3(c) over a temperature range from 20°C up to 90°C. In this case, the resonant frequency is found to follow a linear trend line with a slope of ~0.4% K^-1. The insensitivity of the low-Q plasmonic resonator to temperature fluctuations is in strong contrast to high-Q photonic resonators, which are two orders of magnitude more sensitive to temperature fluctuations (~100% K^-1)¹⁸. This is extremely beneficial for applications where strong temperature fluctuations occur. For example, a low-Q plasmonic resonant sensor or modulator would be immune to thermal fluctuations of ±5 °C which normally occur in CPUs while high-Q photonic resonators require power-consuming temperature controls to maintain operation¹⁹. Furthermore, the moderate Q factors are also beneficial for high-speed operation as desired in electro-optic modulators. As a result, we are able to push the bandwidth of a resonant electro-optic modulator well beyond 100 GHz (methods: speed limitations). In comparison, photonic resonators are more likely limited to bandwidths of ~20 GHz and below^18,19.

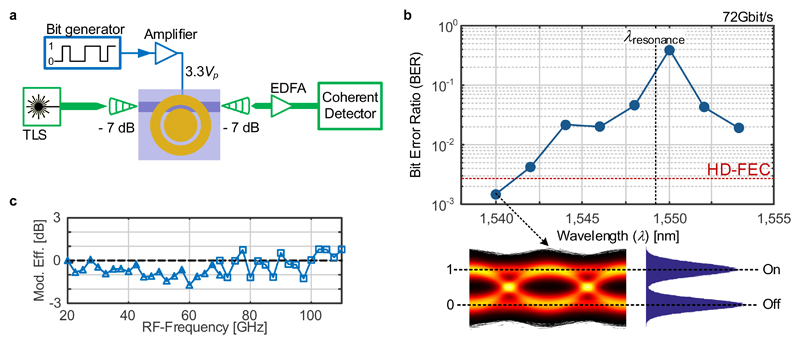
Subsequently, we performed high-speed data experiments to demonstrate the robustness, high speed and low power switching capability. Although, the “resonant switching” principle can also be used to optimize sensors, we focus on high speed applications because their sensitivity and stability requirements are stricter.

In the experiment of Figure. 4(a) the peak driving voltage was ~3.3 V_peak and the laser wavelength was varied to capture the response of the modulator. Low device losses of 2.5 dB and fiber-to-silicon waveguide losses of ~7 dB resulted in fiber-to-fiber coupling losses of 16.5 dB enabling successful operation with low laser powers of 4 dBm and below. The resulting bit-error-ratio (BER) versus wavelength for a 72 Gbit/s signal is shown in Figure. 4(b) where a peak in the BER is observed at λ_res. A high BER is observed at λ_res since applying the same voltage with an opposing sign results in the same optical amplitude but a different phase. Consequently, we confirmed that the operating mechanism relies on amplitude modulation. Off-resonance, the BER quickly dropped to ~ 1×10^-3 which is below the hard-decision forward error correction (HD-FEC) limit and allows for successful data modulation and detection³². No thermal heater was required for stabilization. Additionally, we reduced the data-rate to 36 Gbit/s and 18 Gbit/s and found BERs of ~ 2×10^-6 and < 1×10^-6, respectively, indicating that the BER at 72 Gbit/s is mainly limited by the electrical equipment (methods: data experiments). We estimate the energy consumption of the modulator to be ~ 12 fJ/bit at 72 Gbit/s¹⁴ for a device capacitance of 1.1fF.

Figure 4.

High-speed data experiments with a plasmonic ring resonator used as an EO-modulator. (a) Depicts the experimental setup. (b) Bit-error-ratio (BER) vs. wavelength for a resonator with λ_resonance = 1549nm. BERs below the hard-decision forward error correction (HD-FEC) limit show successful data modulation and detection without the use of a temperature control. The BER increases at the resonance wavelength as expected from the notch-filter response of the resonator. (c) Shows the bandwidth of the plasmonic resonator in the bottom, which is beyond 110 GHz.

We demonstrate that low-Q resonant designs can enable low-loss active plasmonic devices with a good modulation depth by utilizing highly confined SPPs. We believe that our approach – unlike conventional resonant photonics – breaks the trade-off between sensitivity (high-Q) on the one hand and speed and temperature stability (low-Q) on the other. Our work can be seen as a step towards practical plasmonics that ultimately serves as a compact and fast gateway between electronics (local signal processing) and photonics (broad bandwidth and low-loss data stream). The proposed slot waveguide approach could be of interest in sensing because the resonant response can be exploited for many other material systems ranging from low index materials like aqueous solutions to high index materials such as silicon.

Methods

Future Improvements

The plasmonic material strongly influences the performance of the ring resonator in terms of losses and resonant enhancement. In this section, we discuss the influence of various plasmonic materials and we show that the modulator performance improves when replacing gold with copper (CMOS compatible) or silver (lowest loss).

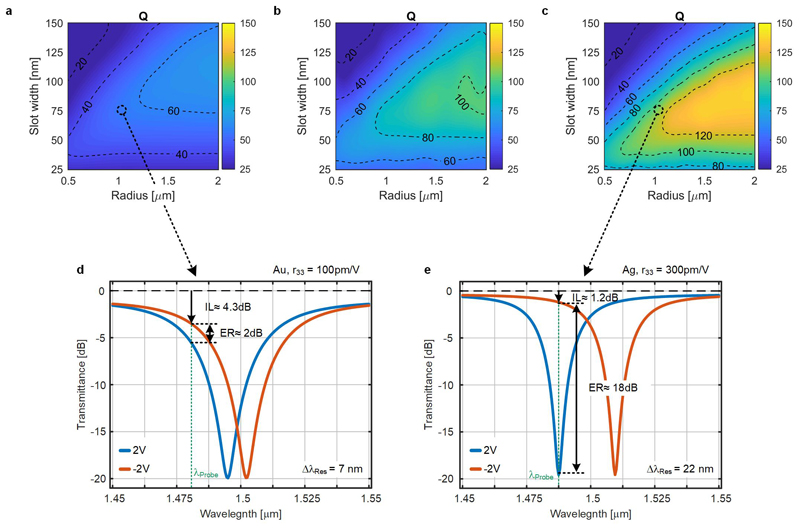
In a first step, we investigate the unloaded Q-factor for different plasmonic metals. Extended Data Figure 1shows the unloaded quality factor Q as a function of the slot width and the radius for Au (a), Cu (b), and Ag (c).

Both copper and silver feature higher Q-factors. When changing the plasmonic metal from Au to Ag the quality factor is increased twofold for geometries dominated by propagation loss (Ag: 140/ Au: 60). Each material shows the trade-off between propagation loss and bending loss resulting in the characteristic triangular contour lines, see chapter III in supplementary information. Furthermore, using low loss materials such as silver will not only increase the intrinsic Q-factor, but also the coupling efficiency C. 3-D simulations indicate that C increases from 0.8 to 0.9.

Besides the plasmonic material, the OEO material provides an additional path for further improvement. Recent, experiments have shown that electro-optic (EO) coefficients over 300 pm/V are achievable by operating in close vicinity of the OEO material resonance (λ ≈ 1250 nm)³³. Additionally, other OEO materials such as JRD1³⁴ show EO-coefficients >300 pm/V JRD1^35,36.

To evaluate the influence of these improvements on the modulator performance, Extended Data Figure 1 shows the wavelength-dependent transmittance of a MIM ring resonator obtained by the analytic model (equation (2) in supplementary information). Extended Data Figure 1(d) shows the transmittance for gold while Extended Data Figure 1(e) includes the possible improvements with Ag and JRD1 (EO coefficient of 300 pm/V). The radius is 1 μm, the slot width is 80 nm and the outer electrode height is 350 nm. The solid lines correspond to positive (blue) and negative (red) biasing of 2 V.

Such improved configurations offer through port IL as small as 0.5 dB (Ag) compared to 1.5 dB (Au). Furthermore, the switching sensitivity is significantly increased due to the higher nonlinearity (3 x) and the larger Q-factor (2 x). Thus, the absolute IL reduce form 4.3 dB to 1.2 dB and the ER increases from 2 dB to 18 dB when driven with ±2 V at the chosen probing wavelength (vertical green line). Thus, low-power CMOS transistors may drive the plasmonic resonators without the help of electrical amplification. This would reduce the total energy consumption of the modulators to below 1 fJ/Bit.

Alternative Active Materials for Modulation and Sensing

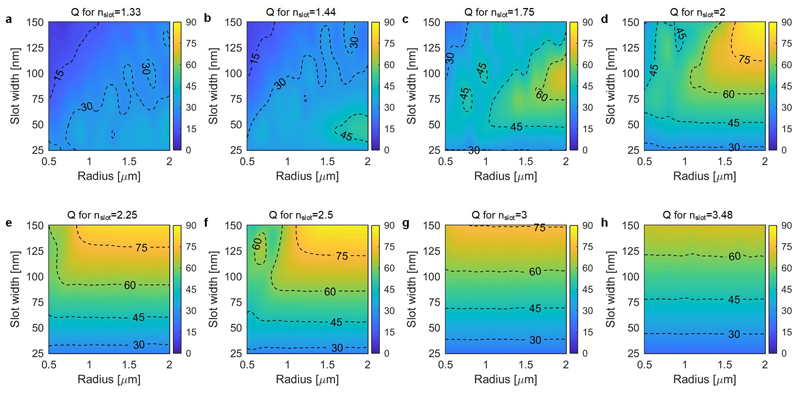
Here, we discuss the influence of the refractive index of the slot material on the unloaded Q-factor of plasmonic ring resonators. The active material could be either an OEO material for modulation or a bio-sensitive material for sensing purposes. Extended Data Figure 2 shows the simulated Q-factors for different slot widths and radii. The simulated refractive indices range from 1.33 up to 3.48 as shown in subplots (a) to (h).

All material systems achieve Q-factors above 30. The refractive index of the active material strongly influences the bending and propagation losses. In the case of low n_slot, for (a) and (b), the Q-factor is mainly limited by bending losses for all slot widths as indicated by the diagonal contour lines (dashed lines). This behaviour changes when n_slot increases to 1.75 (c) or 2 (d) and typical triangular contour lines can be observed highlighting the trade-off between bending losses and propagation losses, see section IV in supplementary information. A further increase of the refractive index results in dominating propagation losses. The triangular shape of the contour line changes to contour lines parallel to the R-axis. In this region, see subplot (e) to (h), the Q-factor drops for the same geometrical parameters as propagation loss increases with the refractive index³⁷.

Subplots (a), (c),(e) and (h) are of most interest as they represent materials that can be used for sensing: (a) n_slot =1.33(H2O); or for EO-modulation: (c) n_slot =1.75 (OEO)³⁵; (e) n_slot =2.25 (BTO)³⁸; (h) n_slot =3.48(Si)²².

Sample Fabrication

All reported devices were processed on SOI wafers with a 220 nm thick silicon device layer and 3 μm thick buried oxide. Electron beam lithography (Vistec EBPG5200) in combination with ICP-RIE (Oxford Plasmalab System 100) were applied to pattern the silicon photonic components. A positive tone resist (PMMA) and a negative tone resist (HSQ) were used as masks to define the partially (70 nm) and fully (220 nm) etched silicon structures, respectively³⁹. Planarization of the patterned silicon structures was realized by spin-coating the samples with a 1 μm thick HSQ layer followed by rapid thermal annealing to convert HSQ to SiO₂⁴⁰. We observed thickness variations of ±10 nm with a periodicity of 50 μm throughout the chip. This does not affect device performance due to the large discrepancy in size between the footprint of the resonators and the periodicity. We controlled the coupling between the ring and bus waveguide by using the following protocol, which enabled us to adjust the average vertical spacing (d_Au,Si) within 5nm of the target value. First, we use an e-beam patterned PMMA resist mask to open Silicon etch marks placed next to the device to measure the HSQ thickness during the following wet etching process. Afterwards, we used a 2^nd e-beam patterned PMMA resist to locally etch down the SiO₂ over the bus waveguide to the desired spacing d_Au,Si = 70±10 nm by using a buffered HF (7:1) solution. The slow etch-rate of 1.2 nm/s enables good process control. Please note, that the spin-coating process rather than the wet etching process introduce the uncertainty of ±10 nm. Contact pads, outer rings (h = 350 nm), inner rings (h = 150 nm) and bridges (h = 350 nm) were produced with a sequence of e-beam lithography, electron-beam evaporation of gold and lift-off processes as reported in Ref. 5. Extended Data Figure 3 shows a tilted top view of the fabricated ring resonator prior to the bridge fabrication step. The height of the outer electrode is 350 nm, while the inner electrode is only 150 nm enabling a reduction of the bending losses, see chapter IV in supplementary information.

Afterwards, we characterized the geometry of all devices using scanning-electron microscopy (SEM) for better comparisons to simulations. This, allows us to estimate important parameters such as the electro-optic coefficient, r₃₃, later on. Finally, the binary OEO material (75%HD-BB-OH/25%YLD124)⁴¹ was spin-coated then poled at its glass transition temperature (115 °C) by applying electric fields of ~130 V/μm. It has been shown that this OEO material allows operation up to 80 °C³³.

We choose the following design parameter sets: slot widths of 80 nm and 100 nm with radii ranging from 900 nm to 1200 m and 1400 nm to 1600 nm.

Passive Characterization

We determined the transmittance of the resonators by subtracting back-to-back measurements of structures that comprise the same grating couplers and photonic waveguides but lack the plasmonic section. The standard deviation of the back-to-back measurements was below ±0.5 dB over the whole wavelength range of the tunable laser (1465 nm to 1635 nm). We measured the passive spectrum for various input power up to 10 dBm and didn’t observe a drift of the resonance wavelength due to self-heating. To prevent any self-heating, we set the laser power to 0 dBm for the passive characterization.

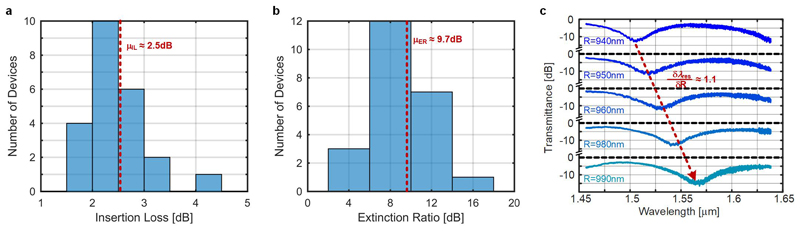
Extended Data Figure 4 shows two histograms of the measured insertion loss (a) and extinction ratio (b) of 23 ring resonators with targeted slot widths of 80 nm. The average insertion loss is ~2.5 dB and the mean extinction ratio is ~9.7 dB.

We designed rings with varying radii to account for fabrication variations. Extended Data Figure 4(c) shows the passive spectrum of ring resonators with radii ranging from 940 nm up to 990 nm. The resonance wavelength shifts with increasing radius by (δλ_res/δR ≈ 1.1)

Sensitivity Experiments

We used the same optical setup as the passive characterization measurements to estimate the DC/ low frequency electro-optic response of six devices. In a first experiment, we applied a 100 Hz triangular shaped electrical signal with an amplitude of 10 V_peak. We measured the modulation of the optical signal when operating the resonator around its 3 dB point. In a second experiment, we applied a DC-bias and measured the resonators’ wavelength shift as a function of the voltage. Extended Data Table 1 provides an overview of the insertion loss, extinction ratio and sensitivity of the resonance wavelength (Δλ_res/V) as extracted from these two experiments. The ER (6-10 dB) and IL (2-3 dB) during active switching match the values obtained from passive characterization. This shows that the modulators can be driven from minimum to maximum output power before dielectric breakdown occurs. 3D simulation of device 79 revealed an Δλ_res/V ≈ 3.1nm/V for an electro-optic coefficient of 100 pm/V. Thus, we estimate an in-device electro-optic coefficient of ~90 pm/V and a Δn ≈ 0.03 when biasing with ±3.75 V based on the Pockels effect. Device 79 (last row) is shown in the manuscript Fig. 3(a) and (b).

Speed Limitations

The electro-optic bandwidth of resonant EO modulators is often determined by the photon lifetime of the resonant cavity. In case of a ring-based EO modulator with a Q-factor of 6000, this calculates to an electro-optic bandwidth of a few tens of GHz⁴². In the case of resonant plasmonic structures, the photon lifetime does not limit the electro-optic bandwidth since these structures feature Q-factors that are two orders of magnitude smaller than the Q-factors of photonic approaches.

In general, the slowest of the following effects limits the electro-optic bandwidth of an EO-modulator: 1) RC-time constant; 2) timescale of the nonlinear effect (here Pockels effect); and 3) cavity lifetime. In the case of the plasmonic MIM ring modulator presented in this work these contributions can be approximated to:

• τ_RC: The calculated capacitance of the MIM ring is ~1.1fF (electro-static 3D simulation), while its resistance is a few Ω with the nm-scaled bridge being the main contributor⁵. This results in a RC-bandwidth of >>1 THz.

• τ_Pockels: The Pockels effect is based on the reorganization of π-electrons within a nm-long molecule and is estimated to be on the time scale of femto- or attoseconds²⁷. This results in a Pockels-bandwidth of >>1 THz.

• τ_cavity: The SPP lifetime is given by the quality factor divided by the angular frequency (τ_res≈Q/ω_photon). The resonant structures presented in this work feature Q-factors of 50, while future implementations may reach values of up to 150. Thus, the cavity lifetime of SPPs is ~100 fs or less, resulting in a cavity lifetime bandwidth limit of > 1 THz.

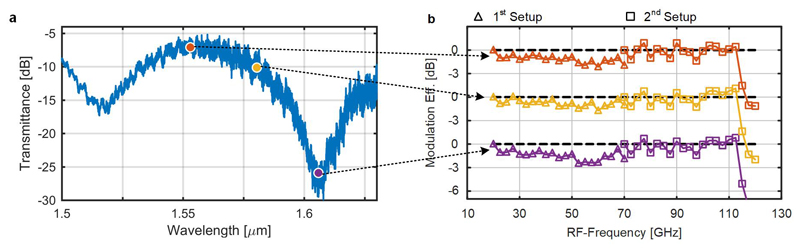
Based on these approximations, we expect, at worst, a THz bandwidth for a plasmonic MIM resonator utilizing the Pockels effect. To confirm that bandwidth limitations are not an issue for resonant plasmonic modulators we performed electro-optic bandwidth measurements with a plasmonic resonator of radius 1.5 μm and a designed slot width of 100 nm. These design parameters are chosen because they feature largest Q-factors and therefore the longest cavity lifetimes. Extended Data Figure 5(a) shows the wavelength dependent transmittance of the plasmonic resonators and (b) shows the normalized modulation efficiency as a function of the modulation frequency. The ratio between the unmodulated carrier and the modulated sidebands is the modulation efficiency which was measured with an optical spectrum analyzer and normalized with respect to the lowest applied frequency⁴³.

The different colors correspond to three characteristic probing wavelengths. Red, yellow and purple are the off-resonance, 3 dB point and on-resonance probing wavelengths, respectively. For all three curves a similar behavior is observed and electro-optic bandwidths beyond 100 GHz are found. This indicates that the cavity lifetime is not yet limiting the bandwidth. Otherwise, a discrepancy in bandwidth between on-resonance (purple) and off-resonance (red) operation should be observed. The kink at ~110 GHz is attributed to limitations in the electrical test setup.

Two different measurement setups were required to cover the broad electrical spectrum ranging from 20 GHz up to 115 GHz in steps of 2.5 GHz. The 1^st setup (20 GHz up to 70 GHz) utilized a tunable RF signal generator (100 kHz – 70 GHz) to generate a sinusoidal RF signal. The electrical output power of the source was adjusted for each frequency such that a constant power of 0 dBm was applied to the ring resonator via GSG probes. The 2^nd setup was used to cover the frequency range from 70 GHz up to 115 GHz with a step size of 2.5 GHz. We used the 6^th harmonic of a Schottky diode to generate these frequencies. The electrical input power to the Schottky diode was kept constant. We compensated the variations in the diode’s output power by measuring the power with a broadband power meter. The measured power values were used in a post-processing step to subtract the influence of the varying power. The power levels of the harmonic terms other than the 6^th harmonic were negligible.

Please note, the signal was applied via and 100 μm x 100 μm sized contact pads The resonator itself (~1.1fF) does not determine the total capacitance as the large contact pads interact with the substrate resulting in a capacitance of several 10s of fF⁴⁴. The modulator behaves like an open circuit element due to the small capacitance.

Data Experiments

This section provides details about the experimental setup shown in Fig. 4 of the manuscript. A tunable, external cavity laser (1465 nm to 1575 nm) was used as a light source with output powers ranging from 6 dBm up to 13 dBm. The laser light was fed to an attenuator to enable a flexible adjustment of the optical power. Light was coupled to and from the chip via grating couplers, which have an efficiency (C_GC) of -7 dB each at 1550 nm. All non-return-to-zero (NRZ) electrical data streams were random binary sequences of length 2¹⁸ generated by a 72 GSa/s, 6-bit digital to analog converter (f_3dB ≈ 20 GHz). Afterwards, an RF amplifier (f_3dB ≈ 50-55 GHz) amplified the electrical signal to a certain peak-to-peak driving voltage (V_pp,50Ω measured over a 50 Ω resistor. Finally, the electrical signal was applied by GS RF-probes (f_3dB > 67 GHz) to the device via 100 μm x 100 μm sized contact pads. Two 25 cm-long RF-cables (1.85 mm connectors) connected the generator, amplifier and RF probe. The modulated light was detected coherently. We used a second laser for heterodyne detection. The 3dB bandwidth of the coherent detector’s photodiode is 70 GHz. An EDFA (Erbium Doped Fiber Amplifier) amplified the optical signal after the modulator to power levels of ~ 8 dBm to enable best performance. The electrical oscilloscope (Electrical bandwidth: 63 GHz and sampling rate: 160 GSa/s) sampled and digitized the waveform. Offline digital-signal-processing was performed to account for timing errors⁴⁵, carrier frequency and phase offsets and the linear frequency response of the system by feedforward equalization with adaptive least mean square (LMS)-based filter updates. Extended Data Table 2 shows the results in terms of bit-error-ratio (BER) obtained from six different devices under various driving voltages (V_peak), data rates (R), and optical input powers at the grating coupler. Similar BERs are obtained for different devices under equal conditions. For instance, low BERs are obtained at 18 Gbit/s for small driving voltages of ~3.3 V_peak and low input powers. At 72Gbit/s the BER drops to 10^-3 under similar conditions. We increased the driving voltages twofold and the input power fourfold and measured only a moderate reduction of the BER to 10^-4. We attribute this due to the limited bandwidth of the electrical components. Device 34 is shown in the manuscript Fig. 4.

Comparison State-of-the-Art EO Modulators

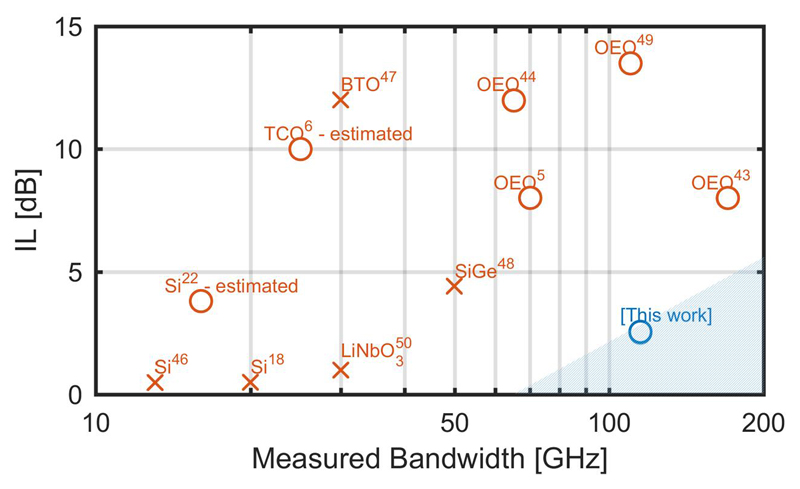
In the following we compare the plasmonic ring resonators with prior art. EO-modulators are expected to help replace electrical communication links with their optical counterparts for short-range applications. Potential candidates have to be compact (μm² footprint) to enable a dense co-integration of electronics and photonics. Furthermore, EO-modulators must be optimized for high speed (> 100GHz) and low insertion loss (<3 dB) to enable multi-gigabit operation. Extended Data Figure 6 shows the characteristics of state-of-the-art devices (photonic – crosses; plasmonic – circles) in terms of insertion loss and EO-bandwidth^{5,6,18,22,43,44,46–50}. The different abbreviations represent various EO materials. Ideally, modulators should occupy the bluish area at the bottom right of the figure. However, large bandwidths correlate with large insertion loss. This is because the plasmonic waveguides also serve as highly conductive electrodes enabling a low RC-time constant, but, ohmic losses in the metals prevent low insertion losses. Contrarily, the resonant switching of photonic modulators enable low losses but suffer from limited bandwidths. Combining plasmonics and the resonant switching concept breaks the trade-off and enables high-speed and low losses modulators.

For the convenience of the reader, we provide a detailed summary of key metrics in Extended Data Table 3 (demonstrated plasmonic modulators) and in Extended Data Table 4 (photonic EO-modulators). The performance of the plasmonic ring resonator (last column) serves as reference in both tables. Extended Data Table 3 shows four non-resonant modulators based on various nonlinear effects and material systems such as χ⁽²⁾ in OEO-materials, free-carrier-dispersion (FCD) in silicon, FCD in transparent conductive oxides (TCO) and χ⁽²⁾ in barium titanate (BTO).

• The resonant approach (last column) outperforms its direct non-resonant counterpart (first column).

• Plasmonic resonators requires moderate driving voltages. And only the TCO based modulator is driven with 1V_peak. However, different data rates of 72 Gbit/s to 2.5 Gbit/s make a comparison challenging.

• Finally, we would like to note that applying the resonant plasmonic approach to other material systems would enable a further boost in performance for each individual technology. For instance, one can imagine TCO-based plasmonic resonators operating with ~mV driving voltages.

Extended Data Table 4 shows 6 photonic modulators based on FCD in silicon^18,46, Franz-Keldysh Effect (FKE) in SiGe⁴⁸, χ⁽²⁾ in barium titanate (BTO)⁴⁷, χ⁽²⁾ in lithium niobate (LiNbO₃)⁵⁰ and Pauli blocking in Graphene⁵¹.

Data availability statement

The data that support the findings of this study are available form the corresponding author upon reasonable request.

Extended Data

Extended Data Figure 1.

Resonator performance for various plasmonic materials. (a) Gold (Au) which is interesting for research due to its chemical stability. (b) Copper (Cu) is of interest as it is a CMOS-compatible material. (c) Silver (Ag) feature the best plasmonic properties and could be of interest for high performance applications. Switching capability of an (d) gold and (e) silver ring resonator for a 2V bias The latter utilizes the newest OEO material, which has a three-times-larger electro-optic coefficient r₃₃. The performance improvement enables a significant reduction in terms of the driving voltage. The number at the bottom right indicates the shift in the resonance wavelength.

Extended Data Figure 2.

Q-factors of various materials filling the slot. The materials differ in their refractive index and one can observe that low n-materials are limited by bending loss (diagonal lines) while high n-materials are limited by propagation loss (parallel lines). Please note these simulations were performed with 150 nm height of the outer and inner electrode to account for limitations in fabrication processes different to ours.

Extended Data Figure 3.

Tilted SEM image of a processed ring resonator. The different height of the outer and inner electrodes reduces the bending losses.

Extended Data Figure 4.

Reproducibility of plasmonic ring resonators. Insertion loss (a) and extinction ratio (b) histograms. Data are obtained from passive measurements of 23 devices with a designed slot with of 80 nm and radii ranging from 900 nm to 1100 nm. (c) Dependence of the resonance wavelength versus ring radius.

Extended Data Figure 5.

Transmission spectrum (a) and the measured bandwidth (b) at the off-resonance, 3dB and on-resonance operating point. No bandwidth limitation can be observed up to 110 GHz. The drop at 115 Ghz frequencies is due to a limited measurement setup. Recent studies show that the modulation efficiency at lower RF-frequency is not limited⁴⁴

Extended Data Figure 6.

Technology overview in terms of insertion loss and bandwidth of electro-optic modulators. Ideal Candidates should feature low insertion loss with high electro-optic (EO) bandwidths found.

Extended Data Table 1.

Measured DC-Sensitivity. The values of the radius and the slot width are the design parameters.

Radius [nm]/Slot width [nm], (Device Id)	IL [dB]	ER [dB]	Δλres/Bias [nm/V]
1200/80, (22)	~3	7	3
1260/80, (28)	~3	8	N.A.
1220/100, (36)	~2	8	1.8
1250/100, (39)	~2	6	2.1
1280/100, (42)	~2.5	7.5	2.33
1080/80, (79)	~2.75	10	2.75

Extended Data Table 2.

Overview of the results obtained from data experiments with various devices

Radius [nm]/Slot width [nm], Device Id	Vpeak [V]	Data rate (R) [Gbit/s]	Bit-error-ratio (BER)	Popitcal[dBm]
1230/80, (25)	~3.3	18	~9x10-6	0
1250/80, (27)	~3.3	18	<1x10-6	0
1200/100, (34)	~3.3	18	<1x10-6	0
“	~3.3	36	~2x10-5	4
“	~3.3	72	~1x10-3	4
960/80, (88)	~5	72	~2x10-3	6
“	~7.8	72	~8x10-4	6
940/80, (86)	~7.8	72	~4x10-4	12
930/80, (85)	~7.8	72	~1x10-3	12

Extended Data Table 3.

Comparison State-of-the art Plasmonic EO Modulator -*estimated values.

Reference	43	22	6	38	this work
Modulation Mech.	χ(2)	FCD	FCD	χ(2)	χ(2)
	OEO	Si	TCO	BTO	OEO
IL [dB]	~8	~3.8	~10	25	~2.5
Fiber-2-fiber loss[dB]	34	N.A.	N.A.	N.A.	16
ER [dB]	N.A.	6-10	6	>15	10
LActive [µm]	20	1	4	10	6
Bandwidth [GHz]	>170	16°	>2.5	N.A.	>110
Data Rate [Gbit/s]	100	<<1	2.5	72	72
BER
Driving Voltage [Vpeak]	4	6	1	2.8	3.3
Energy Consumption	48°	~100	N.A.	N.A.	12
[fJ/Bit]

Extended Data Table 4.

Comparison State-of-the art Photonic EO – Modulator

Reference	18	46	47	50	51	48	this work
Modulation Mech.	FCD Si	FCD Si	χ(2)BTO	χ(2)LiNbO3	Pauli Blocking Graphene	FKE Si Ge	χ(2)OEO
IL [dB]	∼0.5	∼0.5	>12	1	N.A.	4.4	∼2.5
ER [dB]	12	10	N.A.	~7*	30	4.2	10
Lactive [μm]	15	30	37	∼600*	∼250	40	6
Bandwidth [GHz]	∼17	13	30*	30	30	>50	>110
Data Rate [Gbit/s]	44	12	N.A.	40	22	50	72
Driving Voltage [Vpeak]	0.5	2.46	16	2.88	3.75	1	3.3
Energy Consumption [fJ/Bit]	<1	N.A.	N.A.	240	800	13.8	12
Q	6000	15000	N.A.	8000	<1200	N.A	50
Temp. Stability [Δλres K−1 FWHM−1]	100%	N.A.	N.A.	N.A.	N.A.	N.A.	0.4%
Sensitivity [Δλres V−1 FWHM−1]	100%	11%	N.A.	N.A.	N.A.	N.A.	5%

Supplementary Material

Supplementary Information is linked to the online version of the paper at www.nature.com/nature.