Abstract
Determining what factors affect species occurrence is vital to the study of primate biogeography. We investigated the metapopulation dynamics of a lemur community consisting of eight species (Avahi occidentalis, Propithecus coquereli, Microcebus murinus, Microcebus ravelobensis, Lepilemur edwardsi, Cheirogaleus medius, Eulemur mongoz, and Eulemur fulvus) within fragmented tropical dry deciduous forest habitat in Ankarafantsika National Park, Madagascar. We measured fragment size and isolation of 42 fragments of forest ranging in size from 0.23 to 117.7 ha adjacent to continuous forest. Between June and November 2011, we conducted 1218 surveys and observed six of eight lemur species (M. murinus, M. ravelobensis, C. medius, E. fulvus, P. coquereli, and L. edwardsi) in the 42 fragments. We applied among patch incidence function models (IFMs) with various measures of dispersal and a mainland-island IFM to lemur species occurrence, with the aim of answering the following questions: 1) Do lemur species in dry deciduous forest fragments form metapopulations? 2) What are the separate effects of area (extinction risk) and connectivity/isolation (colonization potential) within a lemur metapopulation? 3) Within simulated metapopulations over time, how do area and connectivity/isolation affect occurrence? and 4) What are the conservation implications of our findings? We found that M. murinus formed either a mainland-island or an among patch metapopulation, M. ravelobensis formed a mainland-island metapopulation, C. medius and E. fulvus formed among patch metapopulations, and neither P. coquereli or L. edwardsi formed a metapopulation. Metapopulation dynamics and simulations suggest that area was a more consistent positive factor determining lemur species occurrence than fragment isolation and is crucial to the maintenance of lemur populations within this fragmented landscape. Using a metapopulation approach to lemur biogeography is critical for understanding how lemur species respond to forest loss and fragmentation.
Introduction
Endemic to Madagascar, lemurs are the most endangered mammal group in the world, 94% of lemur species are threatened with extinction [1] largely due to habitat loss and fragmentation of the forests in Madagascar [2]. While there is some disagreement on precisely how much forest has been lost in Madagascar [3], researchers estimate that between 40 and 52 percent of the forest cover has been converted to non-forested habitat between the 1950s and 2010 [2–4]. The processes of habitat loss and fragmentation create landscapes with discrete fragments of habitat [5]. In western Madagascar, the forest is mostly rare tropical dry deciduous forest, and Ankarafantsika National Park contains one of the largest remaining intact portions of continuous dry forest. Dry forest is extremely sensitive to fire [6], which has resulted in a high degree of forest loss and increased habitat fragmentation in this area [4]. Indeed, satellite imagery shows that habitat loss and fragmentation continue in these tracks of continuous forest [7]. Therefore, even in protected areas such as Ankarafantsika, lemurs could be subject to increased habitat fragmentation.
The effects of forest loss and fragmentation on primate species occurrence are well studied [8–14]. Habitat loss is simply the removal of habitat from a landscape and habitat fragmentation is the separation of habitat into smaller less connected portions [5,15]. Typically, primate species occurrence decreases with increased forest loss [10,13,16–19]. Conversely, landscape connectivity/isolation appears to have little to no effect on individual primate occurrence when compared to fragment area [13,20–23]. The effect of habitat fragmentation separate from habitat loss on primate occurrence is not well understood [24–26]. To better understand how fragmentation impacts primate species, researchers need to further assess how connectivity, independent of habitat loss, affects primate occurrence [26].
Metapopulation dynamics offers multiple models for determining the population viability of lemur species in remnant forest patches. There are different types of single species metapopulation models with variable characteristics, including but not limited to the mainland-island metapopulation [27] and an among patch metapopulation model where colonization is not influenced by a mainland. A mainland-island metapopulation occurs where a patch or population within a fragmented landscape is particularly large (mainland) and is surrounded by smaller patches. The mainland has a large population of individuals that is unlikely to become extinct [28]. However, extinction risk is confounded by patch size [29], with smaller patches having a relatively higher extinction risk than larger patches. Because of its large size the mainland produces an unlimited supply of migrants called propagule rain (Hanski, 1994a). The mainland’s unlimited supply of migrants is independent of the number of patches occupied within the system [27]. The colonization potential or isolation of island patches is related to their distance from the mainland [27]. A mainland-island metapopulation may help explain the source-sink dynamics observed in some metapopulations [30]. Similar to a mainland-island model, in an among patch model extinction probability of a patch is a function of the area of that patch [29]. However, colonization is a negative exponential function of the distance to the nearest occupied patch plus a species-specific dispersal parameter [29].
Researchers have used metapopulation theory as a conservation tool to predict species persistence in a fragmented landscape under varying conservation strategies [8,31], to assess extinction risk [32], to determine factors impacting species occurrence [22], to determine species minimal critical forest patch size [22], and for population viability analyses [33]. Other studies have investigated the impact of fragment size and isolation within suspected metapopulations but they did not use metapopulation dynamics per se [9]. Despite metapopulation dynamics being widely recognized as a useful approach to determine how individual species respond to habitat loss and fragmentation [27,34,35], there is no research on metapopulation dynamics in lemurs and few studies on primates [8,22,33]. In one example of a study on primates, Chapman et al. [12] examined forest fragments along the periphery of Kibale National Park, Uganda and fitted a mainland-island incidence function model to occurrence data on four primate species. The metapopulation models accounted for a substantial amount of variation in each species occurrence. However, the authors found low confidence in the estimated coefficients for the models. For both Procolobus badius (red colobus) and Colobus guereza (black and white colobus), Chapman et al. [8] found a strong area effect on occurrence but little influence of connectivity on each species occurrence. For Cercopithecus ascanius (red-tailed monkey) fragment size or distance did not affect occurrence while Chapman et al. [8] found Pan troglodytes (chimpanzees) were an unsuitable species for the application of metapopulation dynamics because of their highly mobile nature. In another example of a metapopulation study on primates, Lawes et al. [11] examined a fragmented portion of Podocarpus forest in KwaZulu-Natal Province, South Africa, and applied a mainland-island incidence function model to Cercopithecus mitis labiatus occurrence and additional land use and environmental factors. Lawes et al. [22] found that the best-fit model incorporated only area as a factor determining C. m. labiatus occurrence. Model fit was not improved by the inclusion of isolation, land use, or other environmental factors.
Preliminary biogeography research on lemur species indicates considerable differences in lemur responses between continuous and fragmented forests [10,36–38]. For example, Steffens and Lehman [37] found that contrary to a previous study of two species of mouse lemurs (Microcebus murinus and M. ravelobensis) in continuous forest [39], there were significant, positive correlations between density and abundance for both species in forest fragments. Knowing that there are differences in biogeographic patterns in some species in continuous versus fragmented habitat raises the question: How will other species will respond to increased habitat fragmentation? Thus, understanding spatial variations in lemur responses to forest fragmentation is critical to a more informed understanding of their conservation biogeography.
The goal of this study is to investigate the vulnerability of eight lemur species to habitat loss and fragmentation in a fragmented landscape in Ankarafantsika National Park. Vulnerability was determined using different stochastic patch occupancy models (incidence function models (IFM)). Following to previous research on mammal patch occupancy in tropical environments [40], we do not employ standard hypothesis testing. Rather, we compare IFMs to answer the following questions: 1) Do lemur species in dry deciduous forest fragments form metapopulations? 2) What are the relative effects of area (extinction risk) and connectivity/isolation (colonization potential) within a lemur metapopulation? 3) Within simulated metapopulations over time, how do area and connectivity/isolation affect occurrence? and 4) What are the conservation implications of our findings?
Methods
Study site and study species
We conducted this study in an approximately 3000 ha fragmented landscape consisting of 42 relatively homogeneous forest fragments surrounded by a relatively homogeneous grassland matrix within the western boundary of Ankarafantsika National Park (ANP), Madagascar (Fig 1). To the north, east, and south of the fragments there is continuous forest (Fig 1). We received permission to conduct our study from the Ministère de l'Environnement, de l'Ecologie et des Forêts, and Madagascar National Parks (Permit Number: 089/11/MEF/SG/DGF/DCB.SAP/SCB). ANP is approximately 135,800 ha, and consists of a mosaic of approximately 72,670 ha of dry deciduous forest and grassland [41,42]. The climate is mostly dry with mean yearly rainfall of 1,000–1,500 mm occurring mostly in the rainy season between November and April [41]. There are eight species of lemurs in ANP (Table 1). We conducted this study in the dry season and early part of the wet season (June–November) of 2011 to facilitate access, and because there is increased visibility due to reduced foliage. All species were active during the entire study except C. medius, which is in torpor between April and October [43].
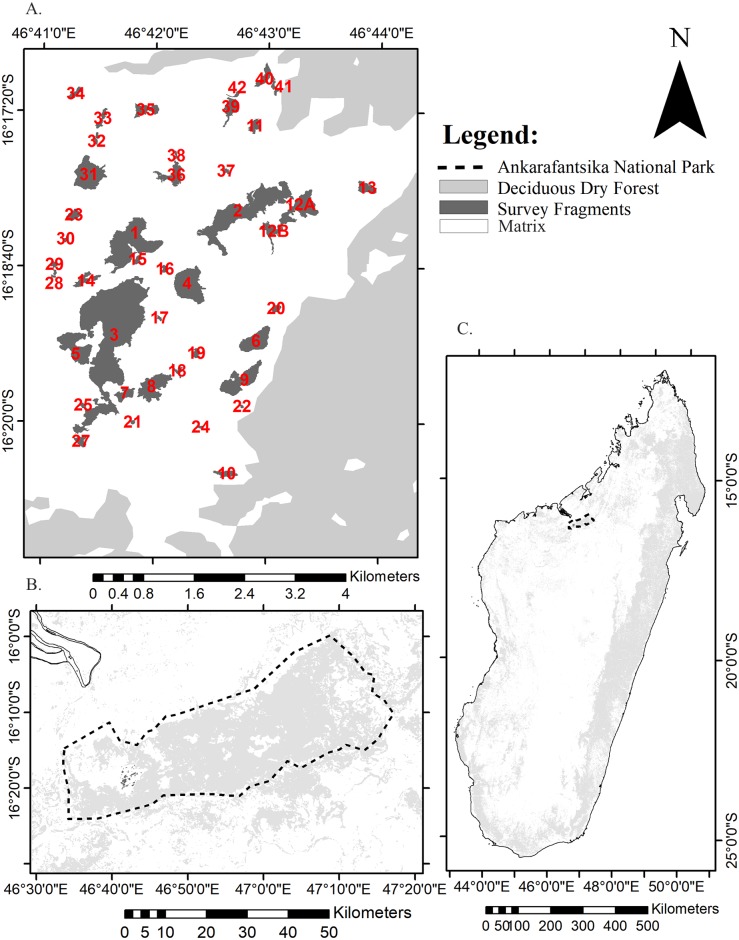
Fig 1. Study site and distribution of forest within Madagascar.
a) Location of study site within Madagascar. b) Location of the study site within Ankarafantsika National park. c) Close up of study site showing the fragmented landscape, consisting of 42 fragments of dry deciduous forest separated by a mainly homogeneous matrix of grassland. Survey fragments are represented in dark grey and continuous forest in light grey and grassland in white.
Table 1. Primate species characteristics and patch occupancy in Ankarafantsika National Park found within study site.
| Species | Body mass (g) | Activity pattern | Diet | Mean home range (ha) | Median dispersal distance (m) based on home range* | Median/Mean dispersal distance reported in literature (m) | IUCN status | # of occupied patches |
|---|---|---|---|---|---|---|---|---|
| Cheirogaleus medius | 120–270 | Nocturnal | Frugivore | 1.55 ±0.42 [45] | 873 | N/A | Least Concern | 12 |
| Microcebus murinus | 58–67 | Nocturnal | Omnivore | 2.83 ±1.44 [46] | 1177 | Median = 251 [44] | Least Concern | 35 |
| Microcebus ravelobensis | 56–87 | Nocturnal | Omnivore | 0.59 ±0.11 [47] | 538 | Mean = 54 [45] | Endangered | 34 |
| Propithecus coquereli | 3700–4300 | Diurnal | Folivore | 19.36 [48] | 3080 | N/A | Endangered | 3 |
| Eulemur fulvus | 1700–2100 | Cathemeral | Frugivore | 13.5 [49] | 2572 | N/A | Near Threatened | 7 |
| Eulemur mongoz | 1100–1600 | Cathemeral | Frugivore | 2.85 [50] | 1182 | N/A | Critically Endangered | 0 |
| Lepilemur edwardsi | 1100 | Nocturnal | Folivore | 1.09 [51] | 731 | N/A | Endangered | 2 |
| Avahi occidentalis | 800–1100 | Nocturnal | Folivore | 1.64 [51] | 896 | N/A | Endangered | 0 |
*Median-dispersal distance was calculated as seven times the square root of the mean reported home range from each study.
To determine patch occupancy we used a single season visual survey along a single line transect within each of the 42 habitat fragments. We placed one survey transect along the longest axis of each fragment while going through the center of the fragment except in Fragment 12A where we placed the transect along the longest axis of the largest portion of the fragment, and fragment three where we had two transects. During survey walks each researcher walked slowly (approximately one km/hour), scanning and listening for all lemur species. During diurnal surveys one or two researchers scanned both sides of the transect simultaneously. Two researchers walked together during all nocturnal surveys and each researcher focused on one side of the transect for the entire duration of the survey. Each team member used high-powered flashlights and headlamps during nocturnal surveys to observe eye shine. Each team member carried binoculars to facilitate species identification and a laser range finder to measure distance metrics (below). We conducted diurnal and nocturnal surveys as follows: early diurnal surveys between 06:19 and 09:07 hours, late diurnal surveys between 14:39 and17:18 hours, early nocturnal surveys between 18:00 and 21:27 hours, and late nocturnal surveys between 02:17 and 5:55 hours. To ensure temporal independence for each survey, we only conducted one of each survey type (diurnal and nocturnal) per 24-hour period in each transect. To ensure spatial independence we alternated the direction of each transect walk. We surveyed all fragments at least twice during early June and between October and November to ensure an accurate assessment of the occurrence of C. medius, who can be in torpor between April and October [43,44]. In total, we conducted between 11 and 18 diurnal, and 11 and 21 nocturnal surveys in each fragment (S1 Table). Prior to the surveys, we trained the core team members on identifying each species within Ampijoroa field station. When we conducted surveys we ensured that there was at least one core team member experienced in identifying each of the eight different species on the survey. When team members observed a group or individual lemur, the team spent up to 15 minutes measuring and recording the following information: observer to animal distance, perpendicular distance of animal to transect, GPS location of the observer, angle of animal from transect, time, date, researchers names, which side the animal was detected, transect number, walk number, height animal was found, tree height animal was found, animal activity, group size, group spread, and species identity. Each of the species was easily identified by size, except for the two Microcebus species. These cryptic species are difficult to visually identify. Therefore, we used a suite of characteristics to determine the species identity of the two Microcebus species. We determined a positive identification of M. murinus only when the team observed all of the following characteristics: grey/brown fur, small body size, and a short tail that was thick at the base. We determined a positive identification of M. ravelobensis when the team observed all of the following characteristics: rufus fur, and a long tail that was thin at the base. We found it difficult to identify 41% of Microcebus sightings to the species level during surveys. Because of the cryptic nature of the Microcebus spp., in this study we conducted analysis on the sightings for M. murinus and M. ravelobensis when we were confident of their identification and second analysis combining all Microcebus spp. sightings. If we did not observe a species during any survey of a fragment, we considered it absent for that fragment.
Question 1: Do lemur species in dry deciduous forest fragments form metapopulations?
In an incidence function model (IFM), incidence is a measure of the probability of species occurrence within a patch and is a function of both patch extinction probability and colonization potential [34]. It is difficult to measure patch extinction probability and colonization potential directly [34]. To determine a species extinction probability within a patch or patch network, it is necessary to acquire long-term data on mortality of individual primates, who are long lived, within patches of varying sizes. To determine colonization potential of a patch or patch network, it is necessary to know a species dispersal abilities between patches over more than one year. For primates this requires difficult to acquire long-term data on species dispersal patterns.
Using an IFM researchers can determine incidence in a metapopulation model without data on species extinction or colonization rates. From an IFM, we can infer the extinction probability of a patch and its colonization potential using simple occurrence data (presence/absence) gathered from a single-survey period among patches within a fragmented landscape [27,29]. An IFM uses area as a proxy for extinction risk and isolation as a proxy for colonization potential. Thus, it describes the probability that a species occurs (incidence) within a patch as a function of both the area (extinction risk) and isolation (colonization potential) of that patch [27,29]. The only additional data required are the sizes and locations of each patch and knowledge of the median-dispersal range of a species within the landscape. The benefit of an IFM is that it is more realistic because it incorporates patch area and isolation directly measured from the landscape and it can be easily parameterized based on occurrence data of species within a fragmented landscape at one particular point in time.
The incidence function models we used are spatially explicit models that have some simplifying assumptions including the following: that the patch has a size but no shape, the quality of the patch is constant, and the matrix is relatively uniform. The data needed for a metapopulation IFM include at least a single survey of patch occupancy within a network of patches, the x and y coordinates of each patch to determine the distance between each patch, patch area, and the species-specific dispersal ability within the landscape [27]. We selected our study site because it suits many assumptions of the model including having mostly homogeneous forest fragments of varying size separated by mostly homogeneous matrix of grassland. It is relatively easy to gather occurrence data for primates and to measure patch area and distances using current GPS and GIS technology. However, it is very difficult to know the dispersal ability of primates within a landscape.
We chose to investigate three different models including: 1. an among-patch incidence function model with rescue effect (including four associated sub-models: IFM, IFMproxy, IFMproxy2, IFMlit), 2. a mainland-island incidence function model without rescue effect (MI-IFM), and 3. a null model where lemur species occurrence varies randomly with respect to patch area and isolation (Null). The four among-patch sub-models are similar but differ in the way the dispersal parameter (α) was defined (see below).
For each model, we input data on patch occupancy for each species and Microcebus spp. combined, a measure of species-specific dispersal distance (α), patch area, and isolation (see below). We then took a linearized version of the IFM sub-models (see Eq (9) below) and the MI-IFM model (see Eq (15) below) and ran binomial generalized linear models (GLM) with a logit link function using the glm function in R [52], for each species and Microcebus spp. combined (adapted from [53]). For each GLM we ran we input species occurrence as the response variable and species-specific dispersal distance (α), patch area, and isolation as the predictor variables.
The IFM sub-models with rescue effect takes the following form [29,53]:
| (1) |
| (2) |
| (3) |
| (4) |
| (5) |
where Ji in Eq (1) is patch incidence in patch i defined in terms of extinction and colonization rates, Ei is the extinction probability, Ci is the colonization probability, Si is a measure of connectivity for patch i, and Ai is the area of patch i. Mi is a measure of connectivity within a landscape. Ji in Eq (5) is patch incidence in patch i defined in terms of patch size Ai and patch connectivity Si. It is difficult to estimate extinction and colonization directly [27,29], however, it is possible to calculate e and y (parameters estimated from the data that relate to extinction and colonization probabilities respectively; see Question 2: What are the Separate Effects of Area (Extinction Risk) and Connectivity/Isolation (Colonization Potential) within a Lemur Metapopulation? for more explanation) with data on patch occupancy pj, patch size A, and connectivity S collected during a single time period survey [53]. Connectivity is estimated using the following:
| (6) |
where is α inverse of the median species-specific dispersal distance and dij is a distance matrix among patches. It is possible to change Eq (5) by applying a linear model for the log-odds of incidence:
| (7) |
| (8) |
A logit transformation results in:
| (9) |
The mainland-island incidence function model without rescue effect takes the following form (MI-IFM; [29]):
| (10) |
| (11) |
where q and ß are two parameters and Di is the distance from the mainland to each island. Assuming that all the species are common on the mainland, where Ci approaches one when Di approaches zero than q = 1 and Eq (11) can be simplified further [29]:
| (12) |
| (13) |
It is possible to linearize Eq (13) by applying the log-odds of incidence:
| (14) |
A logit transformation then results in:
| (15) |
Patch occupancy
We determined patch occupancy of each fragment using the methods described above. We considered a species as present if we visually or acoustically (one instance) recorded their presence within a fragment. We considered a species as absent if we did not visually observe or acoustically confirm their presence within a fragment.
Dispersal distance
To determine connectivity within each IFM sub-model, we needed to determine the dispersal parameter (α). However, there is limited data on dispersal ability in most primates, especially lemurs. For the species in this study, dispersal distance has only been estimated only in M. murinus [54,55] and to a lesser degree of accuracy in M. ravelobensis [56]. Therefore, we ran multiple IFMs incorporating different dispersal parameters (α). For each species, we ran three IFM sub-models using different measures for dispersal, except for M. murinus and M. ravelobensis for which we ran four IFM sub-models with different measures for dispersal. For the first IFM sub-model, we determined which α fit the survey data by running all possible values for α and selecting the one that provided the lowest deviance (IFM; [53]). For the second IFM sub-model, we used a proxy for median-dispersal distance based on a function of the home range size of each species (IFMproxy) [57]. Bowman et al. [57] argues that median-dispersal distance could be estimated as the linear dimension (square root) of the mean home range multiplied by a factor of 7. Therefore, we took the mean reported home range for each species and determined its dispersal ability with the following formula:
| (16) |
Alpha (α) is calculated as:
| (17) |
The proxy for dispersal using the formula from Bowman et al. [57] overestimated the median-dispersal of the two known species (M. murinus and M. ravelobensis; Table 1). Therefore, for the third IFM sub-model (IFMproxy2), we created a second proxy where we took the linear dimension of the mean reported home range:
| (18) |
Because lemurs like many arboreal primates may be dispersal limited [58] the value derived from formula (16) may overestimate median-dispersal distance. The dispersal distance values derived using formula (18) better fit the known dispersal distances for M. murinus and M. ravelobensis (Table 1). For the final IFM sub-model (IFMlit: M. murinus and M. ravelobensis only), we used the largest median-dispersal distance reported for each species regardless of sex (M. murinus = 251 m [54]; M. ravelobensis = 54 m [56]. For Microcebus spp. combined we included the all the sub-models as above but used both proxy dispersal estimates for both species (i.e. IFMproxyMM, IFMproxyMR, IFMproxy2MM, and IFMproxy2MR).
Patch area and isolation
To measure the area (ha) of each fragment we first walked the perimeter of each fragment recording the track with a handheld global positioning device (Garmin GPS map 60csx). We input the track into QGIS (2012; n = 38). If obstructions prevented a complete walk of the fragments perimeter (n = 4; Fragments 37, 39, 40, 42), we traced the fragments perimeter from a high resolution DigitalGlobe™ satellite image, via Google Earth™ taken during the study (10/8/2011). We input each polygon in QGIS and used the field calculator tool to determine the area of these fragments. Because an IFM does not incorporate shape of a fragment we estimated the edge-to-edge distance between each fragment by first calculating the center-to-center distance between each fragment using the ArcGIS Spatial Join tool and subtracting that by the radius of each fragment pairing assuming a circular shape for each fragment.
Model comparison
To determine which model was the most likely among the models/sub-models we tested we calculated corrected Akaike's information criterion with a correction for finite sample sizes (AICc) and then calculated AIC weights (wi; [59]). We considered the model with the highest wi as the model with the highest likelihood of being selected among the models/sub-models we tested [59]. We considered models with AICc values within two of the model with the lowest AICc as potential candidate models.
Question 2: What are the separate effects of area (extinction risk) and connectivity/isolation (colonization potential) within a lemur metapopulation?
To determine if the incidence probability was positively related to area and connectivity (Si) we ran univariate GLM analysis on each species incidence probability against area and connectivity for the candidate model with the lowest AICc selected in question 1. We determined the incidence probability for each patch (Ji) based on among patch models (Eq 1) or the mainland-island model (Eq 10). However, to calculate incidence probability (Ji), we needed to determine the extinction probability (Ei) and colonization probability (Ci) for each patch. For the among patch models, with known patch sizes, patch occupancies, and connectivity, we used a generalized linear binomial model with logit link function (Eq (9)) to determine the coefficients ß0 and ß1 = x and to separate e (a parameter related to extinction probabilities) from y (a parameter related to colonization probabilities) to calculate the extinction and colonization probabilities for each patch using the following steps:
| (19) |
| (20) |
| (21) |
It is not possible to calculate e and y from the GLM directly (Eq 9) because any possible combinations of pairs of e and y giving the estimated are equally good [53]. Therefore following [53], we separated e and y from by fixing e as the smallest patch where a species was present as the area that extinction probability equals 1 (Eq 20) and dividing to determine y (Eq 21). We calculated the extinction probability (Ei) and colonization probability (Ci) and subsequently incidence probability (Ji)of each patch using Eqs (2) and (4) respectively.
For the mainland-island model, we also used a generalized linear binomial model from a single survey of patch occupancy on Eq (15). Using this equation, we could determine the coefficients ß0 = μ, ß1 = ß and ß2 = x to calculate the colonization and extinction probabilities with Eqs (11) and (12) respectively. We then input the values from the colonization and extinction probabilities into Eq (10) to determine the incidence probability for each patch.
Using a Shapiro Wilk’s test we assessed normality for the following independent variables: area, species specific connectivity measures for the among patch models (Si), and the edge of fragment to continuous forest distance for the mainland-island models (DCF) [60]). We found that some variables needed transformation to meet the assumption of normality (e.g. log 10 for area, log 10 for Si estimations for E. fulvus, and square root for the edge of fragment to continuous forest distance (DCF).
Question 3: Within simulated metapopulations over time, how do area and connectivity/isolation affect occurrence? What are the conservation implications?
To see if there was a difference in how area affected occurrence compared to connectivity, we simulated metapopulation dynamics for each species over time based on the extinction and colonization probabilities derived from the IFM selected in question 1 in R following Oksanen [53]. We ran two sets of simulations. The first set represents a worst-case scenario where we ran a simulation separating out the five largest fragments and a second simulation where we separated out the five most connected/closest fragments. The second set represents the opposite scenario where fragments that are smaller and least connected/furthest were removed from the simulation. For each species and Microcebus spp. combined, we then ran the two sets of two simulations for 200 time steps (equivalent of 200 years). In the first half of the simulation all 42 fragments contribute to the metapopulation. At time step 101, we continue simulations on the five removed fragments (i.e. five largest, five smallest, five most connected, five least connected) and on the remaining 37 fragments for 99 more time steps. This method allows the ability to model what happens to lemur species occupancy in the five removed and remaining 37 fragments independently of each other. We used the number five because this represented a realistic conservation scenario where in a single event five of the largest, smallest, least/furthest, or most connected/closest fragments could be lost.
Results
We sampled 42 fragments within the study landscape with a median size of 2.16 and range of 0.23–117.70 ha. The mean distance between centroids of each fragment was 2.82 ± 1.36 km with a range of 0.15 km to 6.48 km. The proxy for median-dispersal distance using Eq (16) ranged from 538 m (M. ravelobensis) to 3080 m (P. coquereli) and using Eq (18) ranged from 77 m (M. ravelobensis) and 400 m (P. coquereli: Table 1). Patch occupancy differed among species. Smaller-bodied Cheirogaleids occurred in the largest number of fragments while the remaining three larger species occurred in the fewest (Table 1). However, a linear regression of frequency of occupancy versus body size yielded no relationships (adjusted R2 = 0.33, P = 0.14). Transect and survey data are summarized in S1 Table. We did not observe any A. occidentalis or E. mongoz individuals during our study. Therefore, we did not include these species in the analysis.
Question 1: Do lemur species in dry deciduous forest fragments form metapopulations?
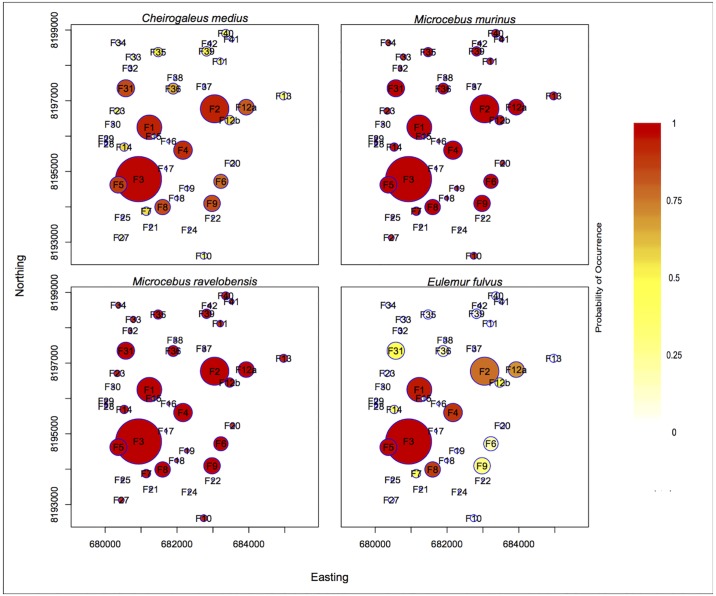
The probability of occurrence differed among species (Fig 2). Both Microcebus species had the highest probability of occurrence in the landscape followed by C. medius. E. fulvus had the lowest probability of occurrence within the landscape.
Fig 2. Probability of occurrence among patches for four lemur species in a fragmented landscape.
Colors represent the probability of occurrence: red reflects the highest probability of occurrence for a species within a fragment and white the lowest. The probability of occurrence is based on the fitted incidence function model with α parameterized from the data (IFM) for C. medius, M. murinus, and E. fulvus and the IFM with α determined from the literature (IFMlit) for M. ravelobensis. The size of each circle represents the size of each fragment relative to one another. The position of fragments is based on Universal Transverse Mercator (UTM) coordinate system. Northing is equivalent to latitude and easting is equivalent to longitude.
We found differences in model selection results between species (Table 2). For C. medius, the among patch sub-model where dispersal was determined based on the IFM had the lowest AICc (IFM: Table 2). No other models had AICc values within two of the lowest model for C. medius. For both M. murinus and M. ravelobensis separately and combined the mainland-island model had the lowest AICc (Table 2). However, for M. murinus and both Microcebus spp. combined we found additional candidate models within two AICc values of the lowest model including the among patch (IFM) model and the null model (Table 2). These results suggest that when these species data are combined it is likely that they do not form either a mainland island or an among patch metapopulation (Table 2). For both P. coquereli and L. edwardsi, there were no models with values lower than or within two of the null model suggesting these species do not form either type of metapopulation that we tested for. However, for P. coquereli the among patch model was close at 2.31 AICc away from the null model (Table 2). For E. fulvus, the model with the lowest AICc value was IFM followed by IFMproxy as the only other model within two AICc values (Table 2). Therefore, we found support for among patch metapopulations in C. medius and E. fulvus and mainland-island metapopulations in M. murinus and M. ravelobensis. However, we found no support for the formation of metapopulations for P. coquereli or L. edwardsi. We found limited support for a mainland-island metapopulation when the Microcebus data was combined at the genus level.
Table 2. Metapopulation models of six lemur species in 42 fragments in a fragmented landscape.
| Species | Modela | K | AICc | ΔAICc | Wi | Log Likelihood |
|---|---|---|---|---|---|---|
| Cheirogaleus medius | IFM | 2 | 30.83 | 0 | 0.66 | -13.26 |
| MI-IFM | 3 | 33.12 | 2.29 | 0.21 | -13.24 | |
| IFMproxy | 2 | 34.07 | 3.24 | 0.13 | -14.88 | |
| NULL | 2 | 56.75 | 25.92 | 0 | -26.22 | |
| IFMproxy2 | 2 | 83.73 | 52.9 | 0 | -39.71 | |
| Microcebus murinus | MI-IFM | 3 | 31.88 | 0 | 0.72 | -12.62 |
| IFM | 2 | 33.81 | 1.93 | 0.27 | -14.75 | |
| NULL | 2 | 40.59 | 8.71 | 0.01 | -18.14 | |
| IFMproxy | 2 | 44.61 | 12.73 | 0 | -20.15 | |
| IFMlit | 2 | 64.05 | 32.17 | 0 | -29.87 | |
| IFMproxy2 | 2 | 70 | 38.12 | 0 | -32.85 | |
| Microcebus ravelobensis | MI-IFM | 3 | 33.28 | 0 | 0.98 | -13.32 |
| IFM | 2 | 42.73 | 9.45 | 0.01 | -19.21 | |
| IFMproxy | 2 | 43.59 | 10.32 | 0.01 | -19.64 | |
| NULL | 2 | 44.98 | 11.7 | 0 | -20.34 | |
| IFMproxy2 | 2 | 76.35 | 43.07 | 0 | -36.02 | |
| IFMlit | 2 | 111.51 | 78.23 | 0 | -53.6 | |
| Microcebus spp. Combined | MI-IFM | 3 | 20.19 | 0 | 0.33 | -6.78 |
| NULL | 2 | 20.54 | 0.35 | 0.28 | -8.11 | |
| IFM | 2 | 20.69 | 0.51 | 0.26 | -8.19 | |
| IFMproxyMM | 2 | 23.22 | 3.03 | 0.07 | -9.45 | |
| IFMproxy2M | 2 | 23.43 | 3.24 | 0.07 | -9.56 | |
| IFMproxy2M | 2 | 31.38 | 11.2 | 0 | -13.54 | |
| IFMproxyMR | 2 | 31.38 | 11.2 | 0 | -13.54 | |
| Propithecus coquereli | NULL | 2 | 9.55 | 0 | 0.56 | -2.62 |
| IFM | 2 | 11.85 | 2.31 | 0.18 | -3.77 | |
| IFMproxy | 2 | 11.98 | 2.43 | 0.17 | -3.83 | |
| MI-IFM | 3 | 12.95 | 3.41 | 0.1 | -3.16 | |
| IFMproxy2 | 2 | 148.48 | 138.94 | 0 | -72.09 | |
| Eulemur fulvus | IFM | 2 | 9.64 | 0 | 0.5 | -2.67 |
| IFMproxy2 | 2 | 9.7 | 0.06 | 0.49 | -2.7 | |
| IFMproxy | 2 | 17.57 | 7.93 | 0.01 | -6.63 | |
| MI-IFM | 3 | 19.53 | 9.89 | 0 | -6.45 | |
| NULL | 2 | 40.59 | 30.95 | 0 | -18.14 | |
| Lepilemur edwardsi | NULL | 2 | -6.42 | 0 | 1 | 5.36 |
| MI-IFM | 3 | 12.8 | 19.22 | 0 | -3.08 | |
| IFM | 2 | 19.09 | 25.51 | 0 | -7.39 | |
| IFMproxy | 2 | 22.85 | 29.27 | 0 | -9.27 | |
| IFMproxy2 | 2 | 148.48 | 154.9 | 0 | -72.09 |
a IFM = incidence function model where α was parameterized based on occupancy data from one survey period; MI-IFM = mainland-island incidence function model; IFMproxy, IFMproxyMM, and IFMproxyMR = IFM where α was calculated as a proxy for dispersal ability based on the square root of the mean home range multiplied by seven reported for each species in the literature (MM represents M. murinus and MR represents M. ravelobensis); IFMproxy2, IFMproxy2MM, and IFMproxy2MR = IFM where α was calculated as a proxy for dispersal ability based on the square root of the mean home range reported for each species in the literature; IFMlit = IFM where α was based on the literature for species where data has been reported on dispersal ability. K = number of parameters in the model.
Question 2: What are the separate effects of area (extinction risk) and connectivity/isolation (colonization potential) on a lemur metapopulation?
We found that log10 area was a significant positive contributor to incidence probability for all species for all models (Table 3). However, the results for connectivity (Si) were more complicated. For the among patch models, connectivity (Si) was not a significant contributor to incidence probability for C. medius (Table 3). The square root of connectivity (sqrt(Si)) was a significant positive contributor to incidence probability for sub-model IFM for E. fulvus (Table 3). For the mainland-island models, the square root of the distance to continuous forest (sqrt(DCF)) was a significant negative contributor to incidence for M. murinus, M. ravelobensis, and Microcebus spp. combined (Table 3).
Table 3. Univariate GLM results for the probability of occurrence (Ji) for lemur species against area and connectivity.
| Species | Metapopulation Modela | GLM Model | Coefficient Value | Estimate | Standard Error | t-value | p-value |
|---|---|---|---|---|---|---|---|
| Cheirogaleus medius | IFM | (Ji) = B+Blog10Area | Intercept | 0.9903 | 0.0578 | 17.1 | <0.01 |
| log10 Area | 0.4295 | 0.0339 | 12.5 | <0.01 | |||
| (Ji) = B+B*(Si) | Intercept | 0.32088 | 0.27173 | 1.18 | 0.24 | ||
| Si | -0.0467 | 0.16724 | -0.03 | 0.98 | |||
| Microcebus murinus | MI-IFM | (Ji) = B+Blog10Area | Intercept | 1.2737 | 0.0682 | 18.69 | <0.01 |
| log10 Area | 0.3341 | 0.04 | 8.35 | <0.01 | |||
| (Ji) = B+B*sqrtDCF | Intercept | 1.0755 | 0.0912 | 11.8 | <0.01 | ||
| sqrt(DCF) | -0.3635 | 0.0925 | -3.93 | <0.01 | |||
| Microcebus ravelobensis | IFMlit | (Ji) = B+Blog10Area | Intercept | 1.2583 | 0.0585 | 21.49 | <0.01 |
| log10 Area | 0.2884 | 0.0344 | 8.39 | <0.01 | |||
| (Ji) = B+B*sqrtDCF | Intercept | 1.0474 | 0.0824 | 12.71 | <0.01 | ||
| sqrt(DCF) | -0.2697 | 0.0863 | -3.23 | <0.01 | |||
| Microcebus spp. | MI-IFM | (Ji) = B+Blog10Area | Intercept | 1.2394 | 0.0679 | 18.26 | <0.01 |
| log10 Area | 0.2307 | 0.0398 | 5.79 | <0.01 | |||
| (Ji) = B+B*sqrtDCF | Intercept | 1.059 | 0.0816 | 12.98 | <0.01 | ||
| sqrt(DCF) | -0.2028 | 0.0828 | -2.45 | 0.02 | |||
| Eulemur fulvus | IFM | (Ji) = B+Blog10Area | Intercept | 0.7493 | 0.0928 | 8.08 | <0.01 |
| log10 Area | 0.3694 | 0.0544 | 6.79 | <0.01 | |||
| (Ji) = B+B*sqrt(Si) | Intercept | 0.4095 | 0.1166 | 3.51 | <0.01 | ||
| sqrt(Si) | 0.1206 | 0.0527 | 2.29 | 0.03 |
a See Table 2 for definitions.
Question 3: Within simulated metapopulations over time, how do area and connectivity/isolation affect occurrence?
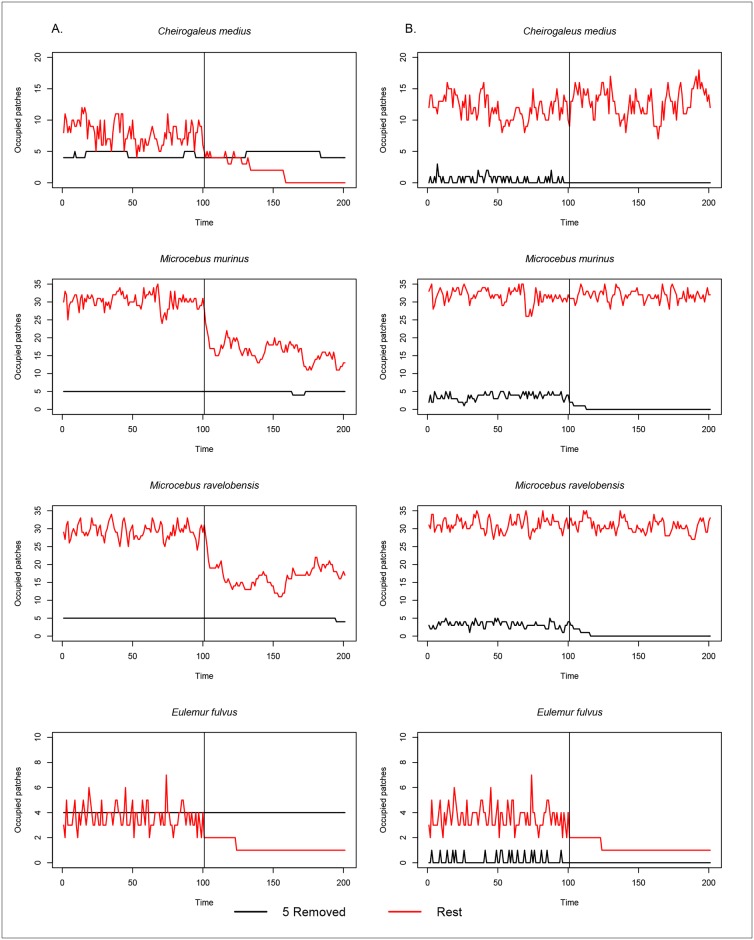
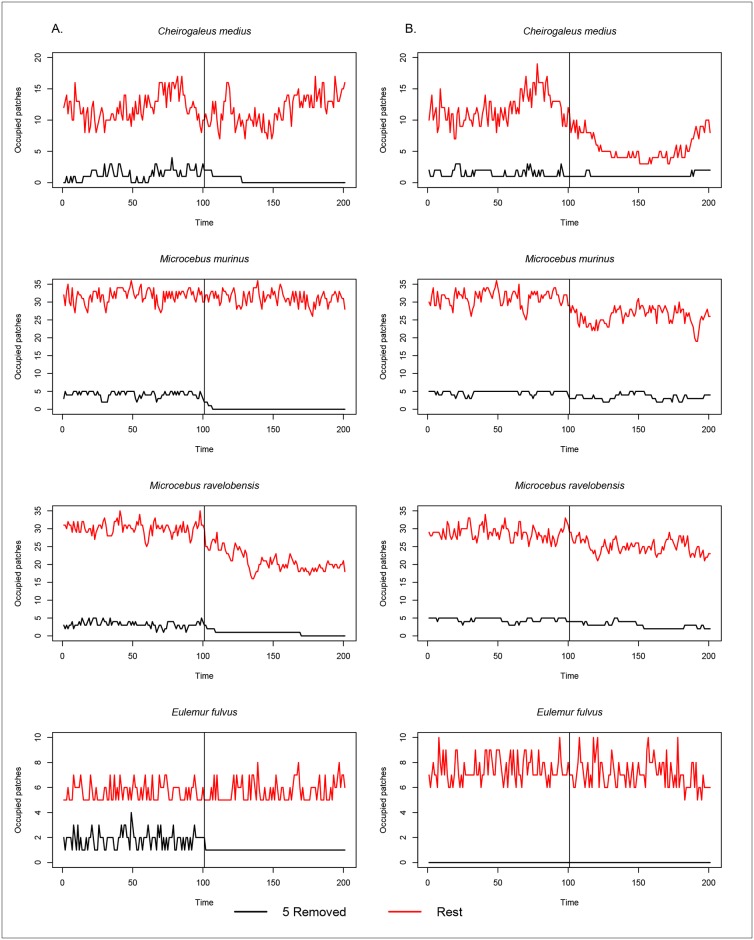
Fragment area has a greater influence than fragment isolation on overall species occurrence. Removal of the five largest fragments via simulation caused all species to decline in occurrence (Fig 3A). The most extreme example was C. medius, which became extinct in the remaining fragments. None of the species showed any appreciable change in occurrence among the five largest fragments when they were separated from the remaining 37, although occurrence of C. medius did vary between three and five in the five largest fragments. Removing the five smallest fragments (Fig 3B) caused no noticeable decline in species occurrence in the 37 remaining fragments. However, separation of the five smallest fragments from the remaining 37 caused a decline in occurrence of all species in the five smallest fragments. Removal of the five most connected/closest fragments via simulation resulted in no obvious changes in occurrence for any species except M. ravelobensis which declined following the removal of the five closest fragments to the continuous forest (Fig 4A). Occurrence for all four species declined within the five most connected fragments following separation from the remaining 37 patches. Removal of the five least connected/furthest fragments in no change in occurrence for E. fulvus but caused a declining trend in occurrence for C. medius and to a lesser degree M. murinus followed by M. ravelobensis following removal of the five least connected fragments (Fig 4B). In the five least connected/furthest fragments there was little difference before and after separation from the remaining 37.
Fig 3. Simulations of metapopulation dynamics for four lemur species over 200 time steps in a fragmented landscape when the five largest and five smallest fragments are removed.
Simulated species occurrence over time using a Markov chain process. The five largest (A) and five smallest (B) fragments (black lines), respectively are removed from the rest of the fragments (n = 37; red lines) at time period 101(vertical line). After this point we ran simulations, to time period 200, separately to demonstrate the impact of either removing the largest (A) or smallest (B) fragments (black). We ran simulations using the IFM with α parameterized from the data (IFM) for C. medius, M. murinus, and E. fulvus and the IFM with α determined from the literature (IFMlit) for M. ravelobensis.
Fig 4. Simulations of metapopulation dynamics for four lemur species over 200 time steps in a fragmented landscape when the five most connected and five least connected fragments are removed.
Simulated species occurrence over time using a Markov chain process. We removed the five most connected/closest (A) and five least connected/furthest (B) fragments, respectively (black lines) from the rest of the fragments (n = 37; red lines) at time period 101(vertical line). After this point we ran simulations, to time period 200, separately to demonstrate the impact of either removing the most/closest (A) or least/furthest (B) connected fragments (black). We ran simulations using the IFM with α parameterized from the data (IFM) for C. medius, M. murinus, and E. fulvus and the IFM with α determined from the literature (IFMlit) for M. ravelobensis.
Discussion
Using a single-season survey of patch occupancy, we found that six lemur species respond differently to area and connectivity/isolation in a fragmented landscape. Simulations of metapopulation dynamics provide support that lemur species occurrence was consistently positively related to area but had mixed results for connectivity. For both Microcebus species, occurrence was negatively related to connectivity, while E. fulvus occurrence was positively related to connectivity. Based on the simulation results, we suggest that the most connected fragments are not as important to the maintenance of the metapopulation as would be predicted by metapopulation theory [34]. For example, when we separated the most connected/closest fragments from the remaining fragments in the system only the simulated occurrence for M. ravelobensis declined within the most connected fragments. However, when we separated the least connected/furthest fragments our simulations showed declines in species occurrence for C. medius, M. murinus, and M. ravelobensis.
We would like to identify some potential caveats that could impact the interpretation of our results. The first is the known difficulty in visually assessing Microcebus spp. in our study area. We acknowledge this difficulty and warn readers to consider the implications of misidentification when interpreting the results. Our research teams are currently using mark-recapture methods combined with genetic analysis to determine if visual surveys provide an accurate representation of species determination in mouse lemurs. It is not only difficult to visually determine species identity but there is also the potential for hybridization between the two Microcebus spp. We observed numerous occurrences of individuals that had what appeared to be mixed characteristics. In total we were unsure in the identification of 41% of our Microcebus spp. sightings. Further genetic research is needed to determine if hybridization is occurring within the landscape. The second caveat is the fact that C. medius goes into torpor between April and October. In an attempt to determine the occurrence of C. medius we re-surveyed each fragment (two more times) where C. medius had not been observed when C. medius was coming out of torpor. We found that C. medius are extremely active and very conspicuous when coming out of torpor. However, it is important to note that the limited number of surveys we conducted when C. medius was potentially active may impact our results for this species.
Question 1: Do lemur species in dry deciduous forest fragments form metapopulations?
Metapopulation dynamics represents an ideal approach for conservation biogeography under the following circumstances: when the habitat is in discrete patches, when ecological processes occur at the local and metapopulation scale, when habitat within the discrete spatial unit is large enough for local breeding populations, and when the patches are relatively permanent [34]. The level of habitat loss and fragmentation in Madagascar provides an ideal situation in which to apply a metapopulation approach to studying lemur populations: there is only a fraction of habitat remaining, forest patches (fragments) are discrete units separated by a non-habitat matrix, many patches are large enough to maintain local breeding populations, and local populations are connected to one another through dispersal, thus creating metapopulations.
We found support for only the null model (where occurrence was randomly related to patch area and connectivity) for P. coquereli and L. edwardsi, suggesting these species did not form metapopulations within our study site. Both of these species only occurred within a small number of large fragments within the landscape (three for P. coquereli and two for L. edwardsi) and appear to be more impacted by habitat loss and fragmentation than the other species we studied.
It is possible that the existing populations of the two species within the fragments declined and became locally extinct over time because fragment size was too small to support local populations. The majority of the fragments are smaller than home ranges reported for P. coquereli [48]. Warren and Crompton [51] found that for L. edwardsi, yearly home range size was 0.81–1.70 ha and Rasoloharijaona et al. [61] found median home range size for L. edwardsi ranged between 0.98 ha (females) and 1.0 ha (males). These home range sizes suggests that L. edwardsi should be able to tolerate smaller fragments, however their mean horizontal distance travelled per day was quite large. Warren and Crompton [51] found that L. edwardsi could travel as much as 463 m (horizontal travel distance) per day. Few fragments in our study site had linear dimensions greater than 463 m. L. edwardsi occurrence appears to differ in continuous versus fragmented habitats. For example, Craul et al. [10] found L. edwardsi to occur in 13 of 17 continuous forest sites surveyed but only two of six habitat fragments, a finding that supports the hypothesis that this species is not tolerant to habitat loss and fragmentation. We found both P. coquereli and L. edwardsi to occur only in larger fragments (the three largest for P. coquereli and the largest and fourth largest for L. edwardsi), and both were absent in all fragments smaller than 11.58 ha. Therefore, the explanation of fragments being too small for survival is plausible for both P. coquereli and L. edwardsi. L. edwardsi may also be particularly sensitive to anthropogenic disturbance. Rabesandratana et al. [62] undertook a survey of L. edwardsi at 10 sites in Ankarafantsika National Park, but not in the vicinity of our study. Although this lemur species was present at 9 of the 10 sites, density estimates were low for sites subject to anthropogenic disturbance (e.g., near villages and areas for palm exploitation). Thus, the proximity of our research site to local villages may indicate the deleterious effects of anthropogenics on L. edwardsi.
Minimum area requirements, limited numbers of large trees, and hunting pressure may also relate to the absence of A. occidentalis in the forest fragments. In eastern littoral forests, Norscia [63] only found Avahi meridionalis in forest fragments larger than 75 ha and no correlation between fragment area and Avahi density. Although A. occidentalis have small home ranges (median range between 1.57 ha– 1.79 ha, [64]) and rely on low quality abundant leaves, suggesting that they would be able to inhabit relatively small fragments, it is possible that the number and distribution of large trees impacts Avahi occurrence [63]. Norscia [63] found that the percentage of large trees above 3.2 cm DBH was significantly positively related to Avahi density. Illegal hunting does occur within the park, and the although the most commonly hunted species are the larger-bodied and more common P. coquereli and E. fulvus, García and Goodman [65] did identify remains of A. occidentalis from a hunt within the park. This lemur species is also thought to be particularly sensitive to seasonal burning of grasslands adjacent to forest fragments, which is undertaken by local people to promote fresh browse for domestic cattle.
Both Microcebus spp. appear to form mainland-island metapopulations. Dispersal ability differs between M. murinus and M. ravelobensis in continuous forest [39,54–56] with M. ravelobensis possibly more dispersal limited than M. murinus with estimated 0.05 km [56] and 0.25 km [54] median-dispersal distances respectively. We found that our estimates of dispersal ability determined from the metapopulation models were vastly higher than those reported for each species (10 km for M. murinus and 0.27 km for M. ravelobensis). However, the trend of M. murinus having a higher dispersal ability remained. It should be noted that dispersal derived from incidence function models can be inaccurate [53]. It appears that M. ravelobensis is more dispersal limited than M. murinus in both continuous and fragmented forest. If M. ravelobensis is more dispersal limited then why do both species exhibit similar patterns of occurrence within the landscape? M. murinus prefers higher elevation and drier forests than M. ravelobensis [39]. However, our study site was chosen for its relative homogeneity. In a study in the same landscape Steffens and Lehman [37] found that abundance in both M. murinus and M. ravelobensis were related to similar factors such as dendrometrics, fragment area, and isolation. In a continuous forest, Burke and Lehman [66] found differences between M. murinus and M. ravelobensis in the capture rates of each species and the body mass of female M. ravelobensis between the edge and interior habitat. They captured more M. ravelobensis and fewer M. murinus along the edge than in the interior habitat. They found female M. ravelobensis along the edge had greater body mass than those in the interior habitat. Therefore, both species may form mainland-island metapopulations but for different reasons. We suggest M. murinus is more capable of dispersing to further fragments than M. ravelobensis, but due to greater edge tolerance M. ravelobensis is more capable of surviving in fragments, at least in the short term. This would imply that M. murinus may be forming a source-sink metapopulation.
For both C. medius and E. fulvus, the most likely among patch incidence function sub-model were where we estimated the dispersal parameter (α) using the survey data (IFM). For E. fulvus the sub-model where dispersal was based on the linear dimension of their mean home range (IFMproxy2) was nearly as likely as the IFM model. Based on the support for these models and lack of support for the mainland-island model for both lemur species it appears they are sufficiently capable of moving between fragments to colonize extinct fragments without needing to rely on the mainland for more migrants. C. medius and E. fulvus were the only two frugivores in the study site. Larger-bodied species tend to have greater dispersal ability than smaller species [67] and frugivorous primates tend to have larger home ranges than folivorous primates [68,69]. Thus, home range size may predict dispersal ability [57] and frugivores have greater dispersal ability than folivores. However, E. fulvus occurred in fewer fragments than C. medius. C. medius with its small body mass and hibernation patterns, may be better suited to survive in more fragments than E. fulvus. For example, we found C. medius in fragments as small as 1.69 ha. Within this landscape there were likely few fragments large enough for E. fulvus to live in, which required E. fulvus to move between fragments. E. fulvus may be transient within patches that are smaller than they would normally need to be able to survive. For example, we found E. fulvus in one fragment that was smaller (4.16 ha) than their reported home range yet absent in four fragments that were within their reported home range. E. fulvus is smaller in body mass but occurs in larger groups than P. coquereli which is why they both have similar home range sizes (Table 1; [48,49]). However, E. fulvus occurred in seven fragments and P. coquereli only two fragments. P. coquereli may be limited by edge effects that reduce habitat suitability, hunting avoidance, or predator avoidance [48,70,71] where E. fulvus may be more edge tolerant. A study on P. coquereli distribution found that they are a capable of living in degraded habitat [72]. Further study is needed to determine why P. coquereli appears capable of living in degraded habitats but also appears edge avoidant. Regarding E. fulvus Lehman et al. [73,74] found that contrary to predictions Eulemur rubriventer was edge tolerant. Lehman [73] suggests that another species of Eulemur, E. rubriventer, behaved more like a folivore/frugivore than a strict folivore. It is possible that E. fulvus behaved the same way. However, we need further study to determine if E. fulvus is more folivorous or frugivorous within the fragments and to determine what is the availability of fruiting trees within the fragments.
Unlike any of the other species observed within the fragments, C. medius is capable of extended hibernation [43]. Like other Cheirogaleus species, C. medius consumes large amounts of high-sugar fruits prior to hibernation in order to build up fat reserves [44]. Therefore, C. medius may be able to survive only in fragments that have a high availability of fruit during this crucial period. Tree holes used by C. medius must be carefully selected in order to allow sufficient maintenance of body temperature during the months that they hibernate [43]. Like fruit availability, tree holes may be a limiting resource for C. medius in the fragments.
Question 2: What are the separate effects of area (extinction risk) and connectivity/isolation (colonization potential) on a lemur metapopulation?
Area
We found that fragment area was positively related to each species probability of occurrence, regardless of the selected candidate model. In metapopulation dynamics as local populations grow and reach their carrying capacity, individuals are forced to leave the patch to find a new suitable patch [75]. An empty patch is considered colonized when an immigrant arrives and subsequently survives in that patch. The quality and size of the habitat determines survival of an individual in a previously unoccupied patch [75]. However, assessing habitat quality is more difficult than measuring area of a patch. Hanski [76] argued that the ratio of habitat quality versus area contributions to species occurrence is dependent on certain species-specific factors. For primates, many biogeographic studies found that area was the largest predictor of primate species occurrence [9,10,22,77,78]. For example, Lawes et al. [22] found that area, rather than isolation and habitat disturbance, was the only factor that impacted occurrence in C. m. labiatus in a fragmented landscape. We need future research to evaluate the relative contribution of habitat quality versus area to lemur species occurrence.
Connectivity/Isolation
The metapopulation model predicts that connectivity should have a significant positive effect on lemur species occurrence. E. fulvus was the only species to show a positive relationship in occurrence probability and connectivity. Contrary to model predictions, connectivity had no significant effect on occurrence probability for C. medius and a negative effect on occurrence probability for M. murinus and M. ravelobensis. Migration between fragments is risky for arboreal lemurs. For example, species travelling through the matrix have increased predation risk [71] and there is the possibility of arriving at an unsuitable fragment requiring further migration. In our study, E. fulvus, although capable of migrating to any fragment and using matrix elements between fragments, appears to stay within the largest and most connected fragments. Although occurring in multiple fragments, C. medius tended to occur near the largest fragment (Fragment 3 and S2 Table) or the continuous forest. Therefore, they are either not limited by dispersal in a fragmented landscape or they form an intermediate metapopulation (combination of different metapopulations e.g. among patch and mainland-island metapopulations). If C. medius do represent an intermediate metapopulation, then they are likely to be able to move between fragments but one or more of the larger fragments would act as a mainland source of more colonists [76]. For example, if Fragment 3 acted as a second mainland source, this pattern would explain a lack of difference in occurrence probability between more and less connected fragments. For both Microcebus species, the differences in occurrence probability were negative, meaning that the probability of occurrence was lower in closer than in further fragments. One explanation for the negative relationship with connectivity is that the two species of Microcebus have higher occurrence probability in more isolated fragments because they are not area limited and are able to survive, possibly in the long-term, within the smallest fragments regardless of isolation. Other studies have recorded Microcebus species in all but the smallest (<1 ha) fragments [79–81]. However, these studies did not include as many small (<1 ha) fragments as our study. We found Microcebus to occur in fragments as small as 0.23 ha. It is possible that there are source-sink dynamics occurring within the study landscape, where large patches and possibly the nearby continuous forest provide a constant source of potential immigrants for smaller patches [30]. For example, we only observed one Microcebus individual in the smallest fragment as well as numerous sightings of mouse lemurs within matrix elements between fragments (Steffens unpublished data), suggesting that occupancy in this patch is ephemeral and thus maintained through colonization via the matrix. Ganzhorn and Schmid [82] also found a potential source-sink relationship occurring for M. murinus in secondary forests within a fragmented landscape. They observed poorer conditions (smaller, fewer trees and warmer temperatures) within the secondary versus primary forest and within the secondary forest they never re-captured any individuals but were able to recapture seven within the continuous forest.
Question 3: Within simulated metapopulations over time, how do area and connectivity/isolation affect occurrence?
One of the advantages of a metapopulation approach is that it is possible to model extinction (area) and colonization (connectivity/isolation) probabilities, which allows for the simulation of their effects on occurrence over time. Our simulation results confirm that area is consistently positively related to lemur species occurrence. The simulations on removing the most connected/closest or least connected/furthest may help us understand Microcebus spp. metapopulation dynamics within the landscape. M. ravelobensis occurrence declined when the closest fragments were removed but M. murinus occurrence did not. This result supports our previous suggestion that M. ravelobensis is more dispersal limited. They appear to need the closer fragments as stepping-stones to the other fragments whereas M. murinus isn’t impacted by the proximity of the closest fragments due to their greater dispersal ability.
Question 4: What are the conservation implications of our findings?
Visual inspection of satellite imagery from 1984 to 2017 [7] shows that human activity has maintained levels of habitat fragmentation in our study site with little to no forest recovery. However, in the same time period forest along the periphery of Ankarafantsika National Park has declined from human activity. The results of our study suggest that maintaining or increasing fragment area within the study site will have the greatest positive benefit to the most lemur species. All analyses suggest that large fragments are crucial to maintaining lemur species metapopulations. Landscape connectivity should be an important variable for each lemur species but our results show that only E. fulvus occurrence was positively related to connectivity. Connectivity was negatively related to occurrence probability of M. murinus and M. ravelobensis and was not significantly related to occurrence probability of C. medius. To have the maximum benefit for the most species in the short-term we recommend that conservation efforts focus on maintaining or increasing fragment size rather than increasing fragment connectivity. Additionally, increasing fragment size will have the secondary benefit of improving fragment connectivity as the distance between fragments will decrease as fragment size increases. However, we do recommend improving fragment connectivity for conservation measures focusing on E. fulvus as it is an important seed disperser in the landscape [83] and we found their occurrence to be positively related to fragment connectivity. If source-sink dynamics are occurring within the landscape for M. murinus and M. ravelobensis, then we also specifically recommend increasing the area of potential sink fragments to reduce the likely mortality of individuals moving from source populations in large fragments.
Conclusion
A metapopulation approach is useful for determining the combined and separate effects of area and connectivity/isolation on lemur species occurrence in a fragmented landscape. This study shows that four lemur species (C. medius, M. ravelobensis, M. murinus, and E. fulvus) form metapopulations in fragmented landscapes. Within their metapopulations, lemurs are impacted by both habitat area and isolation but our study shows that area strongly affects species occurrence and that isolation has species-specific neutral, negative, and positive impacts on lemur species occurrence. We identified dispersal ability and edge tolerance as potentially explaining differences in metapopulation dynamics. It is possible that source-sink dynamics at the studied scale are impacting populations of M. murinus and M. ravelobensis within the landscape. The two most frugivorous lemurs, E. fulvus and C. medius, may be able to maintain stable metapopulations likely through dispersal and their ability to survive within the largest fragments. To maintain metapopulations for each species, we recommend strategies that reduce further habitat loss and isolation among fragments in the landscape. We should pay special attention to connecting the largest fragments allowing seed dispersers, such as E. fulvus and C. medius to increase the area of potential seed deposition.
Supporting information
The number of surveys conducted uring the day (diurnal), night (nocturnal), and total. And the number of associated sightings of all lemur species.
(DOCX)
DCF represents distance to continuous forests; CM represents Cheirogaleus medius occurrence; MM represents Microcebus murinus occurrence; MR represents Microcebus ravelobensis occurrence; PC represents Propithecus coquereli occurrence; EF represents Eulemur fulvus occurrence; LE represents Lepilemur edwardsi occurrence.
(DOCX)
Acknowledgments
The authors would like to thank the following: The Department of Anthropology at the University of Toronto, Jarred Heinrich, Vincent Dorie, Fernando Mercado Malabet, the Department of Paleontology at the University of Antananarivo, Madagascar Institut pour la Conservation des Ecosystèmes Tropicaux (MICET/ICTE), Madagascar National Parks, the residents of Andranohobaka and Maevatanimbary, the field assistants Mamy Razafitsalama, Rindra Rakotoarvony, Jean Paul, Velontsara, Nada, Rollin, and Alpha, and the reviewers who provided valuable feedback that greatly improved our manuscript.
Data Availability
All relevant data are within the paper and its Supporting Information files.
Funding Statement
Financial support for this work was provided by the following intuitions and organizations: Sigma Xi Grants in Aid of Research (https://www.sigmaxi.org), American Society of Primatology (Conservation Small Grant; https://www.asp.org), Calgary and Edmonton Valley Zoos (https://www.calgaryzoo.com and https://www.edmonton.ca/attractions_events/edmonton-valley-zoo.aspx), Primate Conservation, Inc. (http://www.primate.org), The Explorers Club (Exploration Fund; https://explorers.org), and the University of Toronto School of Graduate Studies Travel Grant to TS, and the Natural Sciences and Engineering Research Council of Canada (Discovery Grant; http://www.nserc-crsng.gc.ca/index_eng.asp) to SL.
References
- 1.Schwitzer C, Mittermeier RA, Davies N, Johnson S, Ratsimbazafy J, Razafindramanana J, Louis EE Jr, Rajaobelina S. Lemurs of Madagascar: A strategy for their conservation 2013–2016. Bristol, UK: IUCN SSC Primate Specialist Group, Bristol Conservation and Science Foundation, and Conservation International; 2013;185. [Google Scholar]
- 2.Schwitzer C, Mittermeier RA, Johnson SE, Donati G, Irwin M, Peacock H, Ratsimbazafy J, Razafindramanana J, Louis EE, Chikhi L, Colquhoun IC. Averting lemur extinctions amid Madagascar's political crisis. Science. 2014;343(6173):842–3. doi: 10.1126/science.1245783 [DOI] [PubMed] [Google Scholar]
- 3.Schwitzer C, Chikhi L, Donati G, Irwin M, Johnson SE, Mittermeier RA, Peacock H, Ratsimbazafy J, Razafindramanana J, Louis EE, Colquhoun IC. Protecting lemurs—response. Science. 2014;344(6182):358–60. [DOI] [PubMed] [Google Scholar]
- 4.Harper GJ, Steininger MK, Tucker CJ, Juhn D, Hawkins F. Fifty years of deforestation and forest fragmentation in Madagascar. Env Conserv. 2007;34(4):325–33. [Google Scholar]
- 5.Fahrig L. Effects of habitat fragmentation on biodiversity. Annu Rev Ecol Evol Syst. 2003;34(1):487–515. [Google Scholar]
- 6.Bloesch U. Fire as a tool in the management of a savanna/dry forest reserve in Madagascar. Appl Veg Sci. 1999;2(1):117–24. [Google Scholar]
- 7.Gorelick N, Hancher M, Dixon M, Ilyushchenko S, Thau D, Moore R. Google Earth Engine: Planetary-scale geospatial analysis for everyone. Remote Sens Environ. Elsevier; 2017;202:18–27. [Google Scholar]
- 8.Chapman CA, Lawes MJ, Naughton-Treves L, Gillespie TR. Primate survival in community-owned forest fragments: Are metapopulation models useful amidst intensive use In: Marsh LK, editor. Primates in fragments: Ecology and conservation. Boster: Springer; 2003. p. 63–78. [Google Scholar]
- 9.Rodriguez-Toledo EM, Mandujano S, García-Orduña F. Relationships between forest fragments and howler monkeys (Alouatta palliata mexicana) in southern Veracruz, Mexico In: Marsh LK, editor. Primates in Fragments: Ecology and conservation. Boston: Springer; 2003. p. 79–97. [Google Scholar]
- 10.Craul M, Chikhi L, Sousa V, Olivieri GL, Rabesandratana A, Zimmermann E, Radespiel U. Influence of forest fragmentation on an endangered large-bodied lemur in northwestern Madagascar. Biol Conserv. 2009. December 1;142(12):2862–71. [Google Scholar]
- 11.Irwin MT. Diademed sifaka (Propithecus diadema) ranging and habitat use in continuous and fragmented forest: higher density but lower viability in fragments?. Biotropica. 2008. March 1;40(2):231–40. [Google Scholar]
- 12.Bodin Ö, Norberg J. A network approach for analyzing spatially structured populations in fragmented landscape. Landscape Ecol. 2007. January 1;22(1):31–44. [Google Scholar]
- 13.Raboy BE, Neves LG, Zeigler S, Saraiva NA, Cardoso N, dos Santos GR, Ballou JD, Leimgruber P. Strength of habitat and landscape metrics in predicting golden-headed lion tamarin presence or absence in forest patches in southern Bahia, Brazil. Biotropica. 2010. May 1;42(3):388–97. [Google Scholar]
- 14.Arroyo-Rodríguez V, González-Perez IM, Garmendia A, Solà M, Estrada A. The relative impact of forest patch and landscape attributes on black howler monkey populations in the fragmented Lacandona rainforest, Mexico. Landscape Ecol. 2013. November 1;28(9):1717–27. [Google Scholar]
- 15.McGarigal K, Cushman SA. Comparative evaluation of experimental approaches to the study of habitat fragmentation effects. Ecol Appl. 2002;12(2):335–45. [Google Scholar]
- 16.Anzures-Dadda A, Manson RH. Patch- and landscape-scale effects on howler monkey distribution and abundance in rainforest fragments. Anim Conserv. 2007;10(1):69–76. [Google Scholar]
- 17.Arroyo-Rodríguez V, Mandujano S, Benítez-Malvido J. Landscape attributes affecting patch occupancy by howler monkeys (Alouatta palliata mexicana) at Los Tuxtlas, Mexico. Am J Primatol. 2008. January 1;70(1):69–77. doi: 10.1002/ajp.20458 [DOI] [PubMed] [Google Scholar]
- 18.Boyle SA, Smith AT. Can landscape and species characteristics predict primate presence in forest fragments in the Brazilian Amazon? Biol Conserv. 2010;143(5):1134–43. [Google Scholar]
- 19.Irwin MT, Wright PC, Birkinshaw C, Fisher BL, Gardner CJ, Glos J, Goodman SM, Loiselle P, Rabeson P, Raharison JL, Raherilalao MJ. Patterns of species change in anthropogenically disturbed forests of Madagascar. Biol Conserv. 2010;143(10):2351–62. [Google Scholar]
- 20.Quéméré E, CROUAU-ROY BR, Rabarivola C, Louis EE, Chikhi L. Landscape genetics of an endangered lemur (Propithecus tattersalli) within its entire fragmented range. Mol Ecol. 2010;19(8):1606–21. doi: 10.1111/j.1365-294X.2010.04581.x [DOI] [PubMed] [Google Scholar]
- 21.Radespiel U, Rakotondravony R, Chikhi L. Natural and anthropogenic determinants of genetic structure in the largest remaining population of the endangered golden-brown mouse lemur, Microcebus ravelobensis. Am J Primatol. 2008;70(9):860–70. doi: 10.1002/ajp.20574 [DOI] [PubMed] [Google Scholar]
- 22.Lawes MJ, Mealin PE, Piper SE. Patch occupancy and potential metapopulation dynamics of three forest mammals in fragmented afromontane forest in South Africa. Conserv Biol. 2000;14(4):1088–98. [Google Scholar]
- 23.Cristóbal-Azkarate J, Veà JJ, Asensio N, Rodríguez-Luna E. Biogeographical and floristic predictors of the presence and abundance of mantled howlers (Alouatta palliata mexicana) in rainforest fragments at Los Tuxtlas, Mexico. Am J Primatol. 2005;67(2):209–22. doi: 10.1002/ajp.20178 [DOI] [PubMed] [Google Scholar]
- 24.Marsh LK. The nature of fragmentation In: Marsh LK, editor. Primates in fragments: Ecology and conservation. Boston: Springer; 2003. p. 1–10. [Google Scholar]
- 25.Cardillo M, Grace GM, Gittleman JL, Jones KE, Bielby J, Purvis A. The predictability of extinction: biological and external correlates of decline in mammals. Proc R Soc B Biol Sci. 2008;275(1641):1441–8. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Arroyo-Rodríguez V, Cuesta-del Moral E, Mandujano S, Chapman CA, Reyna-Hurtado R, Fahrig L. Assessing habitat fragmentation effects on primates: the importance of evaluating questions at the correct scale In: Marsh LK, editor. Primates in fragments: Ecology and conservation. Boston: Springer; 2013. p. 13–28. [Google Scholar]
- 27.Hanski I. Patch-occupancy dynamics in fragmented landscapes. Trends Ecol Evol. 1994;9(4):131–5. doi: 10.1016/0169-5347(94)90177-5 [DOI] [PubMed] [Google Scholar]
- 28.Wilson EO, MacArthur RH. The theory of island biogeography. Princeton: Princeton University Press; 1967 [Google Scholar]
- 29.Hanski I. A practical model of metapopulation dynamics. J Anim Ecol. 1994;63(1):151–62. [Google Scholar]
- 30.Harrison S. Local extinction in a metapopulation context: an empirical evaluation. Biol J Linn Soc. 1991;42(1–2):73–88 [Google Scholar]
- 31.Swart J, Lawes MJ. The effect of habitat patch connectivity on samango monkey (Cercopithecus mitis) metapopulation persistence. Ecol Modell. 1996;93:57–74. [Google Scholar]
- 32.Zeigler SL, De Vleeschouwer KM, Raboy BE. Assessing Extinction Risk in Small Metapopulations of Golden-headed Lion Tamarins (Leontopithecus chrysomelas) in Bahia State, Brazil. Biotropica. 2013;45(4):528–35. [Google Scholar]
- 33.Mandujano S, Escobedo-Morales LA. Population viability analysis of howler monkeys (Alouatta palliata mexicana) in a highly fragmented landscape in Los Tuxtlas, Mexico. Trop Conserv Sci. 2008;1(1):43–62. [Google Scholar]
- 34.Hanski I. Metapopulation ecology. Oxford University Press; 1999. [Google Scholar]
- 35.Hanski I, Ovaskainen O. Metapopulation theory for fragmented landscapes. Theor Popul Biol. 2003;64(1):119–27. [DOI] [PubMed] [Google Scholar]
- 36.Crowley BE, Blanco MB, Arrigo-Nelson SJ, Irwin MT. Stable isotopes document resource partitioning and effects of forest disturbance on sympatric cheirogaleid lemurs. Naturwissenschaften. 2013;100(10):943–56. doi: 10.1007/s00114-013-1094-6 [DOI] [PubMed] [Google Scholar]
- 37.Steffens TS, Lehman SM. Factors determining Microcebus abundance in a fragmented landscape in Ankarafantsika National Park, Madagascar In: Lehman SM, Radespiel U, Zimmermann E, editors. Dwarf Mouse Lemurs Madagascar: Biology, Behavior, and Conservation Biogeography of the Cheirogaleidae. Cambridge Univesity Press; 2016;73:477. [Google Scholar]
- 38.Farris ZJ, Karpanty SM, Ratelolahy F, Kelly MJ. Predator–primate distribution, activity, and co-occurrence in relation to habitat and human activity across fragmented and contiguous forests in northeastern Madagascar. Int J Primatol. Springer; 2014;35(5):859–80. [Google Scholar]
- 39.Rakotondravony R, Radespiel U. Varying patterns of coexistence of two mouse lemur species (Microcebus ravelobensis and M. murinus) in a heterogeneous landscape. Am J Primatol. 2009;71(11):928–38. doi: 10.1002/ajp.20732 [DOI] [PubMed] [Google Scholar]
- 40.Dolrenry S, Stenglein J, Hazzah L, Lutz RS, Frank L. A metapopulation approach to African lion (Panthera leo) conservation. PLoS ONE. 2014;9(2):e88081 doi: 10.1371/journal.pone.0088081 [DOI] [PMC free article] [PubMed] [Google Scholar]
- 41.Alonso LE, Thomas SS, Radilofe S, Missa O. A biological assessment of the Réserve Naturelle Intégrale d’Ankarafantsika, Madagascar. RAP Bull Biol Assess. 2002;23. [Google Scholar]
- 42.Razafy Fara L. Rapport sur l’actualisation de la carte de végétation du parc national à Ankarafantsika. ANGAP, GFA, Antananarivo, Madagascar p. 2003;26.
- 43.Dausmann KH, Glos J, Ganzhorn JU, Heldmaier G. Hibernation in the tropics: lessons from a primate. J Comp Physiol B. 2005;175(3):147–55. doi: 10.1007/s00360-004-0470-0 [DOI] [PubMed] [Google Scholar]
- 44.Fietz J, Ganzhorn JU. Feeding ecology of the hibernating primate Cheirogaleus medius: how does it get so fat? Oecologia. 1999;121(2):157–64. doi: 10.1007/s004420050917 [DOI] [PubMed] [Google Scholar]
- 45.Müller AE. A preliminary report on the social organisation of Cheirogaleus medius (Cheirogaleidae; Primates) in north-west Madagascar. Folia Primatol. 2000;69(3):160–6. [Google Scholar]
- 46.Radespiel U. Sociality in the gray mouse lemur (Microcebus murinus) in northwestern Madagascar. Am J Primatol. 2000;51(1):21–40. doi: 10.1002/(SICI)1098-2345(200005)51:1<21::AID-AJP3>3.0.CO;2-C [DOI] [PubMed] [Google Scholar]
- 47.Weidt A, Hagenah N, Randrianambinina B, Radespiel U, Zimmermann E. Social organization of the golden brown mouse lemur (Microcebus ravelobensis). Am J Phys Anthropol. 2004;123(1):40–51. doi: 10.1002/ajpa.10296 [DOI] [PubMed] [Google Scholar]
- 48.McGoogan KC. Edge effects on the behaviour and ecology of Propithecus coquereli in Northwest Madagascar [dissertation]. Toronto(ON): University of Toronto; 2011.
- 49.Mittermeier RA, Louis EE Jr, Richardson M, Schwitzer C, Langrand O, Rylands AB, et al. Lemurs of Madagascar, 3rd edn, Tropical Field Guide Series. Conserv Int; Arlington, VA: 2010 [Google Scholar]
- 50.Curtis DJ, Zaramody A. Group size, home range use, and seasonal variation in the ecology of Eulemur mongoz. Int J Primatol. 1998;19(5):811–35. [Google Scholar]
- 51.Warren RD, Crompton RH. A comparative study of the ranging behaviour, activity rhythms and sociality of Lepilemur edwardsi (Primates, Lepilemuridae) and Avahi occidentalis (Primates, Indriidae) at Ampijoroa, Madagascar. J Zool. 1997;243(2):397–415. [Google Scholar]
- 52.Team R. Development Core (2007) R: a language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria: ISBN 3-900051-07-0, URL http://www.R-project.org/; 2005. [Google Scholar]
- 53.Oksanen J. Incidence function model in R. URL: http://cc.oulu.fi/~jarioksa/opetus/openmeta/metafit.pdf. 2004 May 11.
- 54.Radespiel U, Lutermann H, Schmelting B, Bruford MW, Zimmermann E. Patterns and dynamics of sex-biased dispersal in a nocturnal primate, the grey mouse lemur, Microcebus murinus. Anim Behav. Elsevier; 2003;65(4):709–19. [Google Scholar]
- 55.Schliehe-Diecks S, Eberle M, Kappeler PM. Walk the line—dispersal movements of gray mouse lemurs (Microcebus murinus). Behav Ecol Sociobiol. 2012;66(8):1175–85. doi: 10.1007/s00265-012-1371-y [DOI] [PMC free article] [PubMed] [Google Scholar]
- 56.Radespiel U, Jurić M, Zimmermann E. Sociogenetic structures, dispersal and the risk of inbreeding in a small nocturnal lemur, the golden–brown mouse lemur (Microcebus ravelobensis). Behaviour. 2009;146(4):607–28. [Google Scholar]
- 57.Bowman J, Jaeger JAG, Fahrig L. Dispersal distance of mammals is proportional to home range size. Ecology. 2002;83(7):2049–55. [Google Scholar]
- 58.Bannar-Martin KH. Primate and nonprimate mammal community assembly: The influence of biogeographic barriers and spatial scale. Int J Primatol. 2014;35(6):1122–42. [Google Scholar]
- 59.Burnham KP, Anderson DR, Huyvaert KP. AIC model selection and multimodel inference in behavioral ecology: some background, observations, and comparisons. Behav Ecol Sociobiol. 2011;65(1):23–35. [Google Scholar]
- 60.Shapiro SS, Wilk MB. An analysis of variance test for normality (complete samples). Biometrika. 1965;52(3–4):591. [Google Scholar]
- 61.Rasoloharijaona S, Randrianambinina B, Braune P, Zimmermann E. Loud calling, spacing, and cohesiveness in a nocturnal primate, the Milne Edwards’ sportive lemur (Lepilemur edwardsi). Am J Phys Anthropol. 2006;129(4):591–600. doi: 10.1002/ajpa.20342 [DOI] [PubMed] [Google Scholar]
- 62.Rabesandrantana AZ T, Rakotondravony R, Zimmermann E. Distribution and abundance of Milne Edwards’ sportive lemur Lepilemur edwardsi in the Ankarafantsika National Park, northwestern Madagascar. Lemur News. 2011/12;16:57–60. [Google Scholar]
- 63.Norscia I. Pilot survey of Avahi population (woolly lemurs) in littoral forest fragments of southeast Madagascar. Primates. 2008;49:85–8. doi: 10.1007/s10329-007-0061-2 [DOI] [PubMed] [Google Scholar]
- 64.Ramanankirahina R, Joly M, Scheumann M, Zimmermann E. The role of acoustic signaling for spacing and group coordination in a nocturnal, pair-living primate, the western woolly lemur (Avahi occidentalis). Am J Phys Anthropol. 2016;159(3):466–77. doi: 10.1002/ajpa.22898 [DOI] [PubMed] [Google Scholar]
- 65.García G, Goodman SM. Hunting of protected animals in the Parc National d'Ankarafantsika, north-western Madagascar. Oryx. 2003;37(1):1–4. [Google Scholar]
- 66.Burke RJ, Lehman SM. Edge effects on morphometrics and body mass in two sympatric species of mouse lemurs in Madagascar. Folia Primatol. 2014;85(5):277–91. doi: 10.1159/000360082 [DOI] [PubMed] [Google Scholar]
- 67.Sutherland GD, Harestad AS, Price K, Lertzman KP. Scaling of natal dispersal distances in terrestrial birds and mammals. Conserv Ecol. 2000;4(1):16. [Google Scholar]
- 68.Clutton-Brock TH, Harvey PH. Primate ecology and social organization. J Zool. 1977;183(1):1–39. [Google Scholar]
- 69.Richard AF. Primates in nature. WH Freeman and Co; New York; 1985. [Google Scholar]
- 70.Kun-Rodrigues C, Salmona J, Besolo A, Rasolondraibe E, Rabarivola C, Marques TA, et al. New density estimates of a threatened sifaka species (Propithecus coquereli) in Ankarafantsika National Park. Am J Primatol. 2014;76(6):515–28. doi: 10.1002/ajp.22243 [DOI] [PubMed] [Google Scholar]
- 71.Irwin MT, Raharison J-L, Wright PC. Spatial and temporal variability in predation on rainforest primates: do forest fragmentation and predation act synergistically? Anim Conserv. 2009;12(3):220–30. [Google Scholar]
- 72.Salmona J, Jan F, Rasolondraibe E, Besolo A, Ousseni DS, Beck A, et al. Extensive survey of the endangered coquerel’s sifaka Propithecus coquereli. Endanger Species Res. 2014;25(2):175–83. [Google Scholar]
- 73.Lehman SM, Rajaonson A, Day S. Lemur responses to edge effects in the Vohibola III classified forest, Madagascar. Am J Primatol. 2006;68(3):293–9. doi: 10.1002/ajp.20224 [DOI] [PubMed] [Google Scholar]
- 74.Lehman SM, Rajaonson A, Day S. Edge effects and their influence on lemur density and distribution in Southeast Madagascar. Am J Phys Anthropol. 2006;129(2):232–41. doi: 10.1002/ajpa.20241 [DOI] [PubMed] [Google Scholar]
- 75.Hanski I. Single-species metapopulation dynamics: concepts, models and observations. Biol J Linn Soc. 1991;42(1–2):17–38. [Google Scholar]
- 76.Hanski I. The theories of island biogeography and metapopulation dynamics In: Losos JB, Ricklefs RE, editors. The theory of island biogeography revisited. Princeton University Press; 2010. p. 186–213. [Google Scholar]
- 77.Marshall AR, Jørgensbye HIO, Rovero F, Platts PJ, White PCL, Lovett JC. The species-area relationship and confounding variables in a threatened monkey community. Am J Primatol. 2010;72(4):325–36. doi: 10.1002/ajp.20787 [DOI] [PubMed] [Google Scholar]
- 78.Arroyo-Rodríguez V, Dias PA. Effects of habitat fragmentation and disturbance on howler monkeys: a review. Am J Primatol. 2010;72(1):1–16. doi: 10.1002/ajp.20753 [DOI] [PubMed] [Google Scholar]
- 79.Ganzhorn JU. Effects of introduced Rattus rattus on endemic small mammals in dry deciduous forest fragments of western Madagascar. Anim Conserv. 2003;6(2):147–57. [Google Scholar]
- 80.Schad J, Sommer S, Ganzhorn JU. MHC variability of a small lemur in the littoral forest fragments of southeastern Madagascar. Conserv Genet. 2004;5(3):299–309. [Google Scholar]
- 81.Olivieri GL, Sousa V, Chikhi L, Radespiel U. From genetic diversity and structure to conservation: genetic signature of recent population declines in three mouse lemur species (Microcebus spp.). Biol Conserv. 2008;141(5):1257–71. [Google Scholar]
- 82.Ganzhorn JU, Schmid J. Different population dynamics of Microcebus murinus in primary and secondary deciduous dry forests of Madagascar. Int J Primatol. 1998;19(5):785–96. [Google Scholar]
- 83.Valenta K, Miller CN, Monckton SK, Melin AD, Lehman SM, Styler SA, et al. Fruit Ripening Signals and Cues in a Madagascan Dry Forest: Haptic Indicators Reliably Indicate Fruit Ripeness to Dichromatic Lemurs. Evol Biol. 2016;43(3):344–55. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
The number of surveys conducted uring the day (diurnal), night (nocturnal), and total. And the number of associated sightings of all lemur species.
(DOCX)
DCF represents distance to continuous forests; CM represents Cheirogaleus medius occurrence; MM represents Microcebus murinus occurrence; MR represents Microcebus ravelobensis occurrence; PC represents Propithecus coquereli occurrence; EF represents Eulemur fulvus occurrence; LE represents Lepilemur edwardsi occurrence.
(DOCX)
Data Availability Statement
All relevant data are within the paper and its Supporting Information files.