Skip to main content
PMC home page
Journal of Sports Science & Medicine logoLink to Journal of Sports Science & Medicine
. 2018 May 14;17(2):223–228.

Relationship between Achilles Tendon Stiffness and Ground Contact Time during Drop Jumps

Mohamed Abdelsattar 1, Andreas Konrad 2,✉, Markus Tilp 2
PMCID: PMC5950739  PMID: 29769823

Abstract

The purpose of this study was to investigate the relationship between Achilles tendon stiffness and ground contact time (GCT) during drop jumps. The property of “springiness” and a short GCT during the movement is required in several types of sports. Therefore, a stiff tendon might be advantageous due to the quick force transmission from the muscle to the bone. Hence, a secondary aim was to relate Achilles tendon stiffness with squat jump (SJ) and counter movement jump (CMJ) performance, respectively. Nineteen physically active healthy males (mean ± SD: 26.7 ± 3.9 years, 1.77 ± 0.07 m, 76.5 ± 6.7 kg) participated in this study. Subjects were asked to perform squat jumps and counter movement jumps to determine jump height, and drop jumps were undertaken on a force plate to determine GCT. We then simultaneously measured isometric maximum voluntary contraction (MVIC) of the plantar flexors with a dynamometer and recorded the elongation of the tendon with ultrasound; hence, we could calculate tendon stiffness. The results show a correlation between GCT and Achilles tendon stiffness (r = -0.50) and MVIC (r = -0.48), respectively. Achilles tendon stiffness was not significantly correlated with squat jump and counter movement jump height, respectively. According to the results, we can confirm the main hypothesis that a stiff Achilles tendon tends to result in a shorter GCT during drop jumps. However, Achilles tendon stiffness does not appear to be a key determinant in jumping performance.

Key points.

  • Significant correlation between Achilles tendon stiffness and ground contact time.

  • Significant correlation between isometric maximum voluntary contraction and ground contact time.

  • No correlation between Achilles tendon stiffness and other jumping tests (squat jump and counter movement jump).

Key words: Isometric maximum voluntary contraction, ultrasound, dynamometer, squat jump, counter movement jump

Introduction

Human locomotion during daily activities and athletic performance is accompanied by the repetitive lengthening and subsequent shortening of the muscle-tendon unit (MTU) (Biewener et al. 2009). During such stretch-shortening cycle movements, energy is stored in the elastic parts of the MTU during the lengthening phase and then regained during the shortening phase (Biewener et al. 2009; Obst et al. 2013). The interaction of the muscle and tendon during such stretch-shortening cycles is complex and could be better understood by separately monitoring the relative length changes of the contractile and elastic parts of the muscle (Lichtwark et al. 2007). In addition to the properties of the muscle (e.g., force-velocity relation (Hill, 1938) and force-length relation (Gordon et al., 1966)), the properties and behavior of the tendons in human movement are of great importance for locomotion. The mechanical property that describes the relation between the force exerted on the tendon and its change in the length is the tendon stiffness (Kubo et al., 1999), which greatly affects the kinematics of the tendon during movement. The mechanical characteristics of tendons and their response to exercise have been examined in previous studies. An increase of elastic modulus, the material property of the tendon, was reported by several authors as a response to repeated stress (Buchanan and Marsh, 2001) or exercise at high strain magnitude (Arampatzis et al., 2007b; 2010). Several authors (Buchanan and Marsh, 2001; Michna and Hartmann, 1989; Woo et al., 1980; Vilarta and Vidal, 1989) have reported an increase in ultimate tensile force and hypertrophy of tendons as an adaptation to exercise, while immobilization has been reported to reduce these properties (Kubo et al., 2004; Woo et al., 1982; Yamamoto et al., 1999).

Such properties are also closely related to performance. Kim (2013) and Alexander and Vernon (2009) reported that the recoil of elastic energy caused by stretching of the muscle-tendon complex is reutilized to enhance the performance for the following shortening during a counter movement jump (CMJ) or hopping.

Lichtwark et al. (2007) showed that Achilles tendon compliance is optimized for muscle efficiency during prolonged running. Similarly, Arampatzis et al. (2007a) and Fletcher et al. (2010) reported that stiffer Achilles tendons are positively related to running economy. Besides efficiency, peak performance is also affected by tendon properties. Lai et al. (2014) found that during human ankle plantar flexion, storage and recovery of the tendon’s elastic strain energy supports muscle performance and increases maximum sprinting speed. While efficiency is important for prolonged running, a short GCT is important in sprint running and jumping, and could be realized by direct transfer of force via a stiff tendon.

Bobbert and Casius (2011) and Farley et al. (1999) reported that the stiffness of the leg spring, including both tendon and muscle properties, is regulated according to the hopping height or frequency, and therefore affects GCT.

The ability to move with a short GCT and achieve a high jump height is needed in various sports (Phillips and Flanagan, 2015). During jumping and other types of sport movements with short GCT, muscle force should be transmitted directly to the bone and subsequently to the movement surface. Hence, Proske and Morgan (1987) suggested that a stiff tendon, which directly transmits force, is advantageous. This was confirmed by Bojsen-Møller et al. (2005), who reported a correlation between tendon (aponeurosis) stiffness and jumping height in SJ, CMJ, respectively. Regarding the whole MTU, previous studies have already shown a negative correlation between leg/ankle stiffness and GCT (Arampatzis et al., 2001; 2004; Morin et al., 2007). This phenomenon is related to both the muscle (by its activation status) and the tendon properties. However, to the best of our knowledge, no studies to date have investigated the relationship between tendon stiffness and GCT. Understanding the tendon-specific mechanisms may explain the negative relationship between ankle/leg stiffness and GCT.

Therefore, in this study, we investigated the relationship between Achilles tendon stiffness (ATS) and GCT. A secondary aim was to relate ATS with jumping ability during squat jumps (SJs) and CMJs, respectively. We hypothesized that ATS would be negatively related to GCT (i.e., that stiffer tendons would be related to shorter GCT). We further hypothesized that ATS will be positively related to the jump height of SJs and CMJs.

Methods

Participants

Nineteen physically active healthy males (mean ± SD: 26.7 ± 3.9 years, 1.77 ± 0.07 m, 76.5 ± 6.7 kg, 7.2 ± 2.6 h training per week) participated in this study. The subjects were informed about the test procedure, and they each gave written consent to participate in the study. Participants with a history of lower-leg injuries were excluded. The study was approved by the Ethical Committee of the University of Graz.

Experimental design

We performed an empirical experimental cross-sectional study in laboratory conditions. After a warm-up of 5 min cycling at a moderate intensity, subjects performed CMJs and SJs, from which we determined jump height. In addition, drop jumps were performed on a force plate, from which we determined GCT. Subsequently, we asked the volunteers to perform isometric maximal voluntary plantar flexions on a dynamometer, while ultrasound measurements were also taken, from which we determined isometric maximum voluntary contraction (MVIC) torque and ATS.

Jump performance

Participants were asked to perform SJs and CMJs (Kistler Quattro Jump Bosco Protocol, version 1.1.0.3). During SJ tests subjects initiated the jump from a squatted position with a knee angle of 90°. During CMJ tests, subjects started from an erect position and jumped by flexing and subsequently extending their joints of the lower limbs. Both types of jump were executed with the hands on the hips with the aim to reach maximum height. Each subject performed three trials of both SJs and CMJs, respectively. The highest jump height was used for the further analysis. The participants were then instructed to perform three drop jump trials from a 40-cm box on a force plate (Kistler® force platform (1000 Hz)), with rest periods of at least 1 min between the measurements to avoid any fatigue. Participants were asked to perform with maximum effort, i.e. keep the GCT as short as possible and jump as high as possible (Taube et al., 2012). Jumps that did not appear to be maximal had to be repeated until maximum effort was achieved. Jumps were recorded on video to control the measurement protocol. GCT was determined using the ground reaction force curve in vertical direction, which was recorded using Dewesoft® (X2 SP5 2015). The force-time trace of the drop jump illustrating GCT was recorded when the force was greater than 5 N until it was below 5 N (Figure 1).

Figure 1.

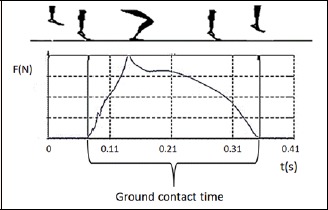
Figure 1.

Open in a new tab

GCT determination from a drop jump.

Isometric maximum voluntary contraction (MVIC) measurement

MVIC measurements were made with a dynamometer (CON-TREX MJ, CMV AG, Duebendorf, Switzerland), with the participant in a seated position with a hip joint angle of 110° and a neutral ankle position (90°) (see Figure 2). The ankle joint was carefully aligned with the center of rotation of the dynamometer, and the foot was strapped to the foot adapter to avoid any loss of heel contact during contractions. Participants were instructed to perform three MVICs of the plantar flexors for 5 s, with rest periods of at least 1 min between the measurements to avoid any fatigue. The attempt with the highest MVIC torque value was taken for further analysis.

Figure 2.

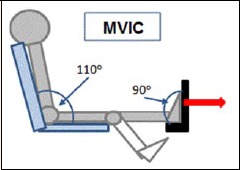
Figure 2.

Open in a new tab

MVIC measurement position in the dynamometer

Measurement of elongation of the muscle-tendon structures

A real-time ultrasound apparatus (MyLab 60, Esaote S.p.A., Genova, Italy) with a 10-cm B-mode linear-array probe (LA 923, Esaote S.p.A., Genova, Italy) was used to obtain Achilles tendon length changes.

During the MVIC measurements, the ultrasound probe was placed on the distal end of the GM, where the muscle merges into the Achilles tendon, i.e., the muscle-tendon junction (MTJ, Kato et al., 2010). The ultrasound probe was covered by a custom-built Styrofoam block and secured with elastic bands to prevent any displacement of the probe. The validity of this method was tested in previous projects (Konrad et al., 2017a). To determine the muscle displacement, the echoes of the MTJ in the ultrasound videos were manually tracked (see Figure 3; Kato et al., 2010). The ultrasound images were recorded at 25 Hz, with an image depth resolution of 74 mm. During MVIC measurements, the videos were synchronized with the other data with a custom-built manual trigger. The videos were cut and digitized in VirtualDub open-source software (version 1.6.19, www.virtual dub.org) and analyzed in the Tracker video analysis and modeling tool (Douglas Brown Open-Source Physics software, version 4.92). During the analysis, every frame was measured by the investigator, corresponding to a time resolution of 0.04 s.

Figure 3.

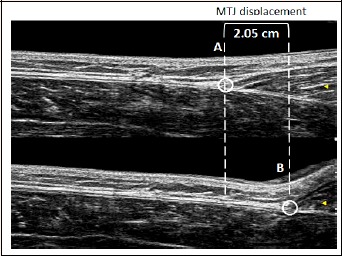
Figure 3.

Open in a new tab

MTJ in resting position (A) and during MVIC (B). The distance between the vertical dashed lines represents the overall movement of the MTJ during the contraction, and hence the elongation of the Achilles tendon.

Calculation of muscle-tendon force and tendon stiffness

The muscle force of the GM was estimated by multiplying the measured torque by the relative contribution of the physiological cross-sectional area of the GM (18%) within the plantar flexor muscles (Kubo et al., 2002; Mahieu et al., 2009), and dividing by the moment arm (MA) of the triceps surae muscle, which was individually measured by tape measure as the distance between the malleolus lateralis and the Achilles tendon at rest at neutral ankle position (90°) (Konrad and Tilp, 2014a; Konrad and Tilp, 2014b; Konrad et al., 2015). Active tendon stiffness was calculated as the change in the active force divided by the change of the related tendon length during the MVIC measurements over a range of force of 50–100% at neutral ankle position (Mahieu et al., 2007; 2009; Kubo et al., 2002).

Statistical analyses

SPSS (version 16.0, SPSS) was used for the statistical analyses. For the verification of the normal distribution of all the parameters, a Shapiro-Wilk test was used. If the data were normally distributed, a Pearson’s correlation analysis was used and correlation coefficients rP reported. Otherwise, a Spearman’s correlation analysis was used to calculate the correlation (reported as rS) between the parameters. An alpha level of p = 0.05 was defined for statistical significance.

Results

The first two rows of Table 1 show the mean and standard deviation of all the measured parameters. The ATS data were not normally distributed. Data from all the other variables were normally distributed.

Table 1.

Mean and standard deviation (SD) of all the measured parameters (n=19) and correlation coefficients between the assessed parameters.

Variables Mean SD ATS GCT SJ height CMJ height
ATS (N/mm) 28.17 11.44 1 - - -
GCT (ms.) 252.98 56.78 -.50* 1 - -
SJ height (cm.) 42.67 6.48 -.02 -.02 1 -
CMJ height (cm) 44.34 6.79 .18 -.23 .92* 1
IMVC (torque (Nm)) 150.11 39.04 .39 -.48* .03 .21
Open in a new tab

*P < 0.05. ATS=Achilles tendon stiffness; GCT=ground contact time; SJ=squat jump; CMJ=counter movement jump; MVIC= maximum voluntary isometric contraction.

There was a negative correlation between ATS and GCT (rS = -0.50; p = 0.03; see Figure 4 and Table 1). Furthermore, there was a correlation between MVIC and GCT (rP = -0.48; p = 0.04) and between CMJ height and SJ height (rP = 0.92; p = 0.00). We did not find any significant relationships between GCT and CMJ and SJ, respectively (see Table 1).

Figure 4.

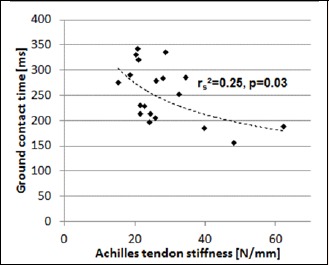
Figure 4.

Open in a new tab

Relationship between ATS and GCT during drop jumps from 40 cm (n=19). RS indicates Spearman correlation coefficient.

Discussion

In this study, we hypothesized that greater ATS would be related to shorter GCT during drop jumps. The results confirm this hypothesis, as subjects with stiffer Achilles tendons demonstrated shorter GCT following drop jumps from 40 cm. Hence, we conclude that a stiffer Achilles tendon might be advantageous in sports which involve short GCTs, such as gymnastics, handball, volleyball, soccer, and several jump and sprinting disciplines in track and field.

These results are similar to the findings of Arampatzis et al. (2001), Morin et al. (2007), who all found a negative correlation between leg or ankle stiffness and GCT during different jump movements (e.g., hopping). Leg and ankle stiffness, as studied by these authors, are affected both by the material structure of the passive system (e.g., stiffness of the tendons), as well as the amplitude of the muscle activation. In contrast to these studies, we focused on the material properties (i.e., ATS) only. It is notable that Morin et al. (2007) reported that a 20% increase in leg stiffness, achieved by greater muscle activation, leads to a 10 % decrease in GCT, highlighting the substantial effect of muscle activation on GCT, as also shown by others (e.g., Bobbert and Cassius, 2011). In our study, the MVIC results were also significantly correlated with GCT (-0.48). Subjects with stronger plantar flexor muscles demonstrated shorter GCT (Table 1). Lately, shear wave ultrasonography analyses revealed that the muscle shear elastic modulus is linearly related to muscle torque during isometric contractions (Ates et al, 2015). This implies that a muscle gets stiffer when it produces more force. Therefore, one could speculate that stronger muscles could increase their stiffness more than weaker muscles. A stronger muscle would then resist the muscle lengthening during the landing phase of the drop jump better by increasing its stiffness. Hence, less energy would be absorbed and ground contact time would be reduced, as observed in our results. However, future studies are needed to support this hypothesis.

Although it appears that muscle activation is the major source of ankle or leg stiffness, our results underline that the properties of the passive structures also substantially affect the movement. The reasons for this could be mechanical or physiological. A mechanical reason would be that muscles and tendons are in series and, therefore, the stiffness of the overall system is affected by the stiffness of both the active and the passive subsystem. Another physiological reason could be the tendon adaptation (i.e. the increase in tendon stiffness; Kubo et al. 2012) that goes along with the increase of muscular strength. This was suggested by Arampatzis et al. (2007a), who reported that tendon and aponeurosis stiffness of the triceps surae was correlated to the maximal tendon force produced during MVIC. Similarly, Kubo et al. (2012) showed that increases of MVIC following two months of a three-month isometric training program were accompanied with significant increases in ATS. Therefore, individuals with strong plantar flexors would reach high ankle/leg stiffness by activating their muscles and would also have stiff Achilles tendons. Both properties would favor short GCT.

Furthermore, stiff tendons allow faster tension changes and joint motion responses. Witvrouw et al. (2004) speculated that stiff tendons provide more feedback to the central nervous system with regard to muscle length and tension. Moreover, the same research group (Witvrouw et al., 2007) pointed out that a stiff tendon is able to transfer forces faster to the bones compared to a compliant tendon especially in concentric contractions. Therefore, they assume that less energy must be provided by the muscle during a contraction because less energy is absorbed by a stiff tendon. However, there is a performance-injury risk trade off in tendon stiffness because the absorption of energy also reduces injury risk of the muscle-tendon system. Witvrouw et al. (2007) concluded that the key point of the prevention and treatment of tendon injuries is to increase the capacity to store elastic energy in the tendon by decreasing its stiffness.

The relationship between ATS and MVIC did not reach statistical significance (p = 0.10) in our study, which is in accordance with the results of Kubo et al. (1999), but is in contrast to reports from Arampatzis et al. (2007a). These differences could be explained by the high number of subjects and the heterogeneous sample (non-active, endurance runners, and sprinters; n = 66) in the study by Arampatzis and co-workers (2007a), which favor significant correlations. Furthermore, the stiffness in their study included the Achilles tendon and aponeurosis structure, while we calculated the stiffness of the Achilles tendon only.

Concerning the comparability to other studies tendon stiffness values of the current study (28.17 N/mm) were similar with (27.7 N/mm to 28.1 N/mm) to the study of Kubo et al. (2002) but lower compared to studies from Mahieu et al. (2007; 2009) with 46.06 N/mm to 66.27 N/mm with the same technique to calculate Achilles tendon stiffness. One possible explanation for this could be the moment arm of the Achilles tendon, which was individually measured in the present study, while Mahieu and co-workers used the same moment arm for all subjects. Further differences are the warm-up routine and shoed subjects in the Mahieu et al. (2007; 2009) studies.

Against our second hypothesis, jump performance for both SJs and CMJs was not related to ATS in the present study. This result is in accordance with the results of Kubo et al. (1999), who also observed that neither stiffness nor MVIC were significantly related to jump height, with or without counter movement. However, this was in contrast to the study of Bojsen-Møller et al. (2005) who reported a correlation between the tendon (and aponeurosis) stiffness of the vastus lateralis and SJs, CMJs, respectively. Whilst during drop jump the triceps surae is the major muscle group contributing to the movement, the knee extensors are the major muscle group involved in SJs and CMJs. Therefore, we think that the low and not significant correlations between ATS to both SJs (-0.02) and CMJs (0.18) in our study are a result of the fact that the muscle-tendon unit of the lower limb is not the major source in these jumping movements.

There are some limitations to this study. Firstly, we did not include both sexes in our study. Since we know from previous studies (Konrad et al., 2017b) that there are significant differences between females and males in MVIC torque and ATS, we refrained from including female subjects to retain data homogeneity. Hence, the outcome of our study is restricted to males only. Secondly, the method of measuring the moment arm of the ankle joint in vivo was quite simple. However, the values obtained in this study were very similar to others using magnetic resonance imaging data (Rugg et al., 1990) or ultrasound (Lee and Piazza 2009) indicating the validity of the method. Thirdly, we did not relate ATS with the jumping height of the drop jump and are therefore not able to estimate the influence of ATS on performance.

Conclusion

The present results suggest increasing ATS in order to decrease GCT for enhanced performance, e.g., in sprint running. However, a randomized controlled training study would be necessary to confirm this assumption. According to previous studies, an appropriate intervention to induce increased tendon stiffness could be isometric (Kubo et al., 2012), plyometric (Kubo et al., 2017), or eccentric training with high strain magnitude protocols at low frequency (Arampatzis et al., 2010).

Acknowledgements

The authors acknowledge the financial support by the University of Graz. The authors have no conflicts of interest to declare. All experiments comply with the current laws of the country.

Biographies

graphic file with name jssm-17-223-g005.gif

Mohamed ABDELSATTAR

Employment

Univ. Ass. Prof. Faculty of Physical Education, Mansoura University, Egypt

Degree

Mag, PhD

Research interests

Training science, Biomechanics, muscle-tendon-unit, soccer science

E-mail: dr_mabdalsatar@mans.edu.eg

graphic file with name jssm-17-223-g006.gif

Andreas KONRAD

Employment

Institute of Sports Science, University of Graz, Austria

Degree

Mag, PhD

Research interests

Biomechanics, training science, muscle-tendon-unit, soccer science

E-mail: andreas.konrad@uni-graz.at

graphic file with name jssm-17-223-g007.gif

Markus TILP

Employment

Univ. Prof. Institute of Sports Science, University of Graz, Austria

Degree

Mag, PhD

Research interests

Biomechanics, training science, muscle-tendon-unit, sports game analysis

E-mail: markus.tilp@uni-graz.at

References

  1. Alexander R.M., Vernon A. (2009) The mechanics of hopping by kangaroos (Macropodidae). Journal of Zoology 177(2), 265-303. [Google Scholar]
  2. Arampatzis A., Schade F., Walsh M., Brüggemann G. (2001) Influence of leg stiffness and its effect on myodynamic jumping performance. Journal of Electromyography and Kinesiology 11(5), 355-364. [DOI] [PubMed] [Google Scholar]
  3. Arampatzis A., Brüggemann G., Morey-Klapsing G. (2004) Interaction of the human body and surfaces of different stiffness during drop jumps. Medicine and Science in Sports and Exercise 36(3), 451-459. [DOI] [PubMed] [Google Scholar]
  4. Arampatzis A., Karamanidis K., Morey-Klapsing G. (2007a) Mechanical properties of the triceps surae tendon and aponeurosis in relation to intensity of sport activity. Journal of Biomechanics 40(9), 1946-1952. [DOI] [PubMed] [Google Scholar]
  5. Arampatzis A., Peper A., Bierbaum S., Albracht K. (2010) Plasticity of human Achilles tendon mechanical and morphological properties in response to cyclic strain. Journal of Biomechanics 43(16), 3073-3079. [DOI] [PubMed] [Google Scholar]
  6. Arampatzis A., Karamanidis K., Albracht K. (2007b) Adaptational responses of the human Achilles tendon by modulation of the applied cyclic strain magnitude. Journal of Experimental Biology 210(15), 2743-2753. [DOI] [PubMed] [Google Scholar]
  7. Ates F., Hug F., Bouillard K., Jubeau M., Frappart T., Couade M., Bercoff J., Nordez A. (2015) Muscle shear elastic modulus is linearly related to muscle torque over the entire range of isometric contraction intensity. Journal of Electromyography and Kinesiology 25(4), 703-708. [DOI] [PubMed] [Google Scholar]
  8. Biewener A., Alexander R.M., Heglund N.C. (2009) Elastic energy storage in the hopping of kangaroo rats (Dipodomys spectabilis). Journal of Zoology 195(3), 369-383. [Google Scholar]
  9. Bobbert M.F., Richard Casius L.J. (2011) Spring-like leg behaviour, musculoskeletal mechanics and control in maximum and submaximum height human hopping. Philosophical Transactions of the Royal Society of London B: Biological Sciences 366(1570), 1516-1529. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Bojsen-Møller J., Magnusson S.P., Rasmussen L. R., Kjaer M., Aagaard P. (2005) Muscle performance during maximal isometric and dynamic contractions is influenced by the stiffness of the tendinous structures. Journal of Applied Physiology 99(3), 986-994. [DOI] [PubMed] [Google Scholar]
  11. Buchanan C.I., Marsh R.L. (2001) Effects of long-term exercise on the biomechanical properties of the Achilles tendon of guinea fowl. Journal of applied physiology (Bethesda, Md.: 1985) 90(1), 164-171. [DOI] [PubMed] [Google Scholar]
  12. Farley C.T., Morgenroth D.C. (1999) Leg stiffness primarily depends on ankle stiffness during human hopping. Journal of Biomechanics 32(3), 267-273. [DOI] [PubMed] [Google Scholar]
  13. Fletcher J.R., Esau S.P., MacIntosh B.R. (2010) Changes in tendon stiffness and running economy in highly trained distance runners. European Journal of Applied Physiology 110(5), 1037-1046. [DOI] [PubMed] [Google Scholar]
  14. Hill A.V. (1938). The heat of shortening and the dynamic constants of muscle. Proceedings of the Royal Society of London 126(843), 136-195. [Google Scholar]
  15. Kato E., Kanehisa H., Fukunaga T., Kawakami Y. (2010) Changes in ankle joint stiffness due to stretching: The role of tendon elongation of the gastrocnemius muscle. European Journal of Sport Science 10(2), 111-119. [Google Scholar]
  16. Kim S. (2013) An Effect of the Elastic Energy Stored in the Muscle-Tendon Complex at Two Different Coupling-Time Conditions during Vertical Jump. Advances in Physical Education 3(1), 10-14. [Google Scholar]
  17. Konrad A., Budini F., Tilp M. (2017a) Acute effects of constant torque and constant angle stretching on the muscle and tendon tissue properties. European Journal of Applied Physiology, 117(8), 1649-1656. [DOI] [PMC free article] [PubMed] [Google Scholar]
  18. Konrad A., Stafilidis S., Tilp M. (2017b) Effects of acute static, ballistic, and PNF stretching exercise on the muscle and tendon tissue properties. Scandinavian Journal of Medicine & Science in sports 27(10), 1070-1080. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Konrad A., Gad M., Tilp M. (2015) Effect of PNF stretching training on the properties of human muscle and tendon structures. Scandinavian Journal of Medicine & Science in Sports 25(3), 346-355. [DOI] [PubMed] [Google Scholar]
  20. Konrad A., Tilp M. (2014a) Effects of ballistic stretching training on the properties of human muscle and tendon structures. Journal of Applied Physiology 117(1), 29-35. [DOI] [PubMed] [Google Scholar]
  21. Konrad A., Tilp M. (2014b) Increased range of motion after static stretching is not due to changes in muscle and tendon structures. Clinical Biomechanics 29(6), 636-642. [DOI] [PubMed] [Google Scholar]
  22. Kubo K., Kanehisa H., Fukunaga T. (2002) Effect of stretching training on the viscoelastic properties of human tendon structures in vivo. Journal of Applied Physiology 92(2), 595-601. [DOI] [PubMed] [Google Scholar]
  23. Kubo K., Akima H., Ushiyama J., Tabata I., Fukuoka H., Kanehisa H., Fukunaga T. (2004) Effects of resistance training during bed rest on the viscoelastic properties of tendon structures in the lower limb. Scandinavian Journal of Medicine and Science in Sports 14(5), 296-302. [DOI] [PubMed] [Google Scholar]
  24. Kubo K., Ikebukuro T., Maki A., Yata H., Tsunoda N. (2012) Time course of changes in the human Achilles tendon properties and metabolism during training and detraining in vivo. European Journal of Applied Physiology 112(7), 2679-2691. [DOI] [PubMed] [Google Scholar]
  25. Kubo K., Ishigaki T., Ikebukuro T. (2017) Effects of plyometric and isometric training on muscle and tendon stiffness in vivo. Physiological Reports 5(15), e13374. [DOI] [PMC free article] [PubMed] [Google Scholar]
  26. Kubo K., Kawakami Y., Fukunaga T. (1999) Influence of elastic properties of tendon structures on jump performance in humans. Journal of Applied Physiology 87(6), 2090-2096. [DOI] [PubMed] [Google Scholar]
  27. Lai A., Anthony G. S., Yi-Chung L., Marcus G.P. (2014) Tendon elastic strain energy in the human ankle plantar-flexors and its role with increased running speed. Journal of Experimental Biology 217(17), 3159-3168. [DOI] [PubMed] [Google Scholar]
  28. Lee S. S., Piazza S. J. (2009) Built for speed: musculoskeletal structure and sprinting ability. Journal of Experimental Biology 212(22), 3700-3707. [DOI] [PubMed] [Google Scholar]
  29. Lichtwark G.A., Bougoulias K., Wilson A.M. (2007) Muscle fascicle and series elastic element length changes along the length of the human gastrocnemius during walking and running. Journal of Biomechanics 40(1), 157-164. [DOI] [PubMed] [Google Scholar]
  30. Mahieu NN., Cools A., De Wilde B., Boon M., Witvrouw E. (2009) Effect of proprioceptive neuromuscular facilitation stretching on the plantar flexor muscle-tendon tissue properties. Scandinavian Journal of Medicine & Science in Sports 19(4), 553-560. [DOI] [PubMed] [Google Scholar]
  31. Mahieu N.N., Mcnair P., Muynck M., Stevens V., Blanckaert I., Smits N., Witvrouw E. (2007) Effect of Static and Ballistic Stretching on the Muscle-tendon Tissue Properties. Medicine and Science in Sports and Exercise 39(3), 494-501. [DOI] [PubMed] [Google Scholar]
  32. Michna H., Hartmann G. (1989) Adaptation of tendon collagen to exercise. International Orthopaedics 13(3), 161-165. [DOI] [PubMed] [Google Scholar]
  33. Morin J.B., Samozino P., Zameziati K., Belli A. (2007) Effects of altered stride frequency and contact time on leg-spring behavior in human running. Journal of biomechanics 40(15), 3341-3348. [DOI] [PubMed] [Google Scholar]
  34. Morrissey D., Roskilly A., Twycross-Lewis R., Isinkaye T., Screen H., Woledge R., Bader D. (2010) The effect of eccentric and concentric calf muscle training on Achilles tendon stiffness. Clinical Rehabilitation 25(3), 238-247. [DOI] [PubMed] [Google Scholar]
  35. Obst S.J., Barrett R.S., Newsham-West R. (2013) Immediate effect of exercise on achilles tendon properties: systematic review. Medicine and science in sports and exercise, 45(8), 1534-1544. [DOI] [PubMed] [Google Scholar]
  36. Phillips J.H., Flanagan S.P. (2015) Effect of Ankle Joint Contact Angle and Ground Contact Time on Depth Jump Performance. Journal of Strength and Conditioning Research 29(11), 3143-3148. [DOI] [PubMed] [Google Scholar]
  37. Proske U., Morgan D.L. (1987) Tendon stiffness: methods of measurement and significance for the control of movement. A review. Journal of Biomechanics 20(1), 75-82. [DOI] [PubMed] [Google Scholar]
  38. Rugg S.G., Gregor R.J., Mandelbaum B.R., Chiu L. (1990) In vivo moment arm calculations at the ankle using magnetic resonance imaging (MRI). Journal of Biomechanics 23(5), 495-501. [DOI] [PubMed] [Google Scholar]
  39. Taube W., Leukel C., Lauber B., Gollhofer A. (2012) The drop height determines neuromuscular adaptations and changes in jump performance in stretch shortening cycle training. Scandinavian Journal of Medicine & Science in Sports 22(5), 671-683. [DOI] [PubMed] [Google Scholar]
  40. Vilarta R., Vidal B. de C. (1989) Anisotropic and biomechanical properties of tendons modified by exercise and denervation: aggregation and macromolecular order in collagen bundles. Matrix (Stuttgart, Germany) 9(1), 55-61. [DOI] [PubMed] [Google Scholar]
  41. Witvrouw E., Mahieu N., Danneels L., McNair P. (2004) Stretching and injury prevention. Sports Medicine 34(7), 443-449. [DOI] [PubMed] [Google Scholar]
  42. Witvrouw E., Mahieu N., Roosen P., McNair P. (2007) The role of stretching in tendon injuries. British Journal of Sports Medicine 41(4), 224-226. [DOI] [PMC free article] [PubMed] [Google Scholar]
  43. Woo S.L., Gomez M.A., Woo Y.K., Akeson W.H. (1982) Mechanical properties of tendons and ligaments. II. The relationships of immobilization and exercise on tissue remodeling. Biorheology 19(3), 397-408. [DOI] [PubMed] [Google Scholar]
  44. Woo S.L.-Y., Ritter M.A., Amiel D., Sanders T.M., Gomez M.A., Kuei S.C., Garfin S.R., Akeson W.H. (1980) The Biomechanical and biochemical properties of swine tendons — long term effects of exercise on the digital extensors. Connective Tissue Research 7(3), 177-183. [DOI] [PubMed] [Google Scholar]
  45. Yamamoto E., Hayashi K., Yamamoto N. (1999) Mechanical properties of collagen fascicles from stress-shielded patellar tendons in the rabbit. Clinical biomechanics (Bristol, Avon) 14(6), 418-425. [DOI] [PubMed] [Google Scholar]

Articles from Journal of Sports Science & Medicine are provided here courtesy of Dept. of Sports Medicine, Medical Faculty of Uludag University

RESOURCES