Calculation of Forward Scatter Dose Distribution at the skin entrance from the patient table for fluoroscopically guided interventions using a pencil beam convolution kernel

Abstract

The forward-scatter dose distribution generated by the patient table during fluoroscopic interventions and its contribution to the skin dose is studied. The forward-scatter dose distribution to skin generated by a water table-equivalent phantom and the patient table are calculated using EGSnrc Monte-Carlo and Gafchromic film as a function of x-ray field size and beam penetrability. Forward scatter point spread function’s (PSFn) were generated with EGSnrc from a 1×1 mm simulated primary pencil beam incident on the water model and patient table. The forward-scatter point spread function normalized to the primary is convolved over the primary-dose distribution to generate scatter-dose distributions. The utility of PSFn to calculate the entrance skin dose distribution using DTS (dose tracking system) software is investigated. The forward-scatter distribution calculations were performed for 2.32 mm, 3.10 mm, 3.84 mm and 4.24 mm Al HVL x-ray beams for 5×5 cm, 9×9 cm, 13.5×13.5 cm sized x-ray fields for water and 3.1 mm Al HVL x-ray beam for 16.5×16.5 cm field for the patient table. The skin dose is determined with DTS by convolution of the scatter dose PSFn’s and with Gafchromic film under PMMA “patient-simulating” blocks for uniform and for shaped x-ray fields. The normalized forward-scatter distribution determined using the convolution method for water table-equivalent phantom agreed with that calculated for the full field using EGSnrc within ±6%. The normalized forward-scatter dose distribution calculated for the patient table for a 16.5×16.5 cm FOV, agreed with that determined using film within ±2.4%. For the homogenous PMMA phantom, the skin dose using DTS was calculated within ±2 % of that measured with the film for both uniform and non-uniform x-ray fields. The convolution method provides improved accuracy over using a single forward-scatter value over the entire field and is a faster alternative to performing full-field Monte-Carlo calculations.

1. INTRODUCTION

Fluoroscopically guided interventions are increasingly used to administer surgically inaccessible anomalies. The x-ray guided procedures can be prolonged and could result in significant entrance skin dose.¹ The primary x-ray field interacts with the patient table generating forward scatter radiation which mostly impinges on the patient skin and serves as an additional source of skin dose.² The present study investigates the forward scatter distribution from the patient table of a Toshiba Biplane Infinix angio machine using EGSnrc Monte Carlo simulations and a convolution technique using a pencil beam forward scatter model to calculate the forward scatter dose distribution.³ Initial investigations are performed on a simulated water phantom block equivalent to the patient table transmissions using EGSnrc, followed by the forward scatter dose measurement of the patient table with Gafchromic film for uniform x-ray fields. The variation of dose distribution inside the field of view (FOV) and beyond the collimation edge is studied as a function x-ray beam penetrability and x-ray field size. Furthermore, the feasibility of using convolution of the scatter dose kernels for skin dose calculations are explored by incorporating the convolution in the skin Dose Tracking System (DTS) Software.⁴ Validation of the DTS is performed for homogenous and non-homogenous x-ray fields using Gafchromic film.

2. MATERIALS AND METHODS

2.1 Forward Scatter Point Spread Function

2.1.1 Water Phantom

The x-ray transmission of the patient table using a normally incident narrow beam was measured using a 0.6 cc Farmer type ionization chamber for a range of beam qualities. The x-ray beam quality was varied by changing the x-ray tube potential (60 kVp, 80 kVp, 100 kVp, 110 kVp) with an added filtration of 1.8 mm Al in the tube assembly. The water thickness equivalent to the table transmission at each kVp is determined using beam spectra generated using EGSnrc Monte Carlo and mass attenuation coefficients for water obtained from NIST reference data.

EGSnrc MC was used to simulate the water phantom with calculated equivalent table thickness, composed of voxels sized 1×1×1 mm. The voxel dimensions of the exit surface layer of the water phantom was 1 × 1 × 0.5 mm thick. A 1×1 mm sized pencil beam was simulated for varying beam qualities and incident over the simulated water block of the corresponding thickness. The simulated water phantom had a thickness equivalent to the measured table transmission for the appropriate beam quality. The entrance surface of the water table phantom was positioned at the interventional reference point, 15 cm tube-side of the iso-center resulting in an SSD of 54 cm. The transmitted dose distribution was obtained from the exit surface of the simulated water phantom which included the transmitted primary and forward scatter dose. The primary dose intensity was obtained using the same simulation set up but with the phantom model placed close to the x-ray tube and a large air gap between the phantom and the planar surface where the dose distribution is determined. The primary dose was subtracted from the total dose distribution to obtain the forward scatter spread function. The forward scatter distribution was normalized with the primary dose intensity to obtain a normalized forward scatter point spread function (PSFn). Successive PSFn’s are generated for varying beam penetrability by changing the x-ray tube voltage.

2.1.2. Patient Table

The patient table of the Toshiba Biplane Infinix C-Arm was simulated for the current study. The table has a uniform thickness on the cranial side and this side was chosen for the scatter measurements. The table is heterogeneous in composition and composed of a central layer of hard foam, enclosed by layers of carbon fiber on either side. The multilayer elements of the table were simulated in EGSnrc with their thickness and composition equivalent to the patient table. The voxel dimensions of the simulated table was 1 × 1 × 1 mm, except for the table beam exit surface which had a thickness of 0.5 mm. The table top was positioned at the interventional reference point, and the PSF was obtained with the same technique as described in section 2.1.1 for the table-equivalent water phantom.

2.2 Full Field Forward Scatter Dose Distribution

2.2.1 Water Phantom

Three different size x-ray beams (5.5 cm, 9 cm & 13.5 cm at the exit surface of the table-equivalent water) were generated using EGSnrc for 80 kVp (3.1 mm Al HVL), and the forward scatter dose distributions were generated using the procedure as explained in section 1.2.1. The simulated water models are composed of 5×5×5 mm voxels, except the exit layer which was 5×5×0.1 mm. Full field forward scatter dose distributions for 13.5 cm FOV were calculated for various beam energies (60 kVp, 80 kVp, 100 kVp and 110 kVp).

In order to perform the convolution, a 2D matrix of primary dose intensities was analytically calculated using the transmission factor obtained for the patient table for a normally incident narrow x-ray beam. The transmission corrections for the divergent angulated x-rays in the beam periphery was performed, and the heel effect non-uniformity in the anode-cathode direction, determined using the EGSnrc MC, were superimposed over the primary dose matrix. The dimension of the 2D primary dose distribution matrix element was 1×1 mm, compatible with the size of the pencil beam used for the PSFn generated. The primary dose distributions for the water were obtained for different field sizes (5×5 cm, 9×9 cm, 13.5×13.5 cm) and for 4 different beam energies. The corresponding forward scatter PSFn kernels are convolved over the primary dose distribution profiles to generate the forward scatter dose distribution. The resulting full-field dose distributions were compared with those generated using the EGSnrc Monte Carlo.

2.2.2 Patient Table

XR-QA2 Gafchromic film was used to obtain the dose distribution transmitted by the patient table which includes the attenuated primary and forward scatter dose distribution. The table surface was positioned 64 cm from the x-ray source, with an SID of 120 cm, and FOV of 16.5 × 16.5 cm at the table surface. The primary dose distribution was obtained with the table placed close to the x-ray tube, thereby resulting in an air gap between the film and the table to minimize forward scatter. The Gafchromic film was calibrated by exposure to known dose values determined using a 0.6 cc Farmer type ionization chamber. Eight 2.5 cm square pieces of Gafchromic film were exposed to a range of x-ray dose values extending to the peak dose received by the Gafchromic film when placed on the table surface. The film calibration comprised the dose intensities ranging from 4 mGy to184 mGy which was within the XR-QA2 Gafchromic film sensitivity range of 1 mGy-200 mGy as per the manufacturer specifications. A dose-response calibration curve, fitted with a second order polynomial was used to convert the scanned film intensity to dose in mGy.

The film was digitized with an effective pixel size of 1 mm. A 2D 5 × 5 median filter was applied across the scanned film to reduce the inherent noise and statistical fluctuations.⁵ The scanned 2D film intensity distribution was converted to the equivalent dose distribution by using the calibration film-dose response curve. The primary dose distribution was deducted from the transmitted dose distribution which includes the forward scatter, to obtain a forward scatter dose distribution. The forward scatter dose distribution was obtained alternatively by convolving the PSFn across the 2D primary dose distribution and its accuracy was determined by comparison with that measured using Gafchromic film.

2.3 Skin Dose Mapping with Dose Tracking System

Previously we have reported the calculation of “patient” backscatter dose distributions and skin dose distributions using a backscatter convolution kernel, with validations performed using DTS and XR-QA2 Gafchromic film on PMMA and SK-150 head phantoms.^{6, 7, 8} In that case, the exposure was delivered cross-table to avoid the forward scatter dose contribution from the patient table, and hence included only the primary dose and backscatter dose distribution. For the current study, the skin dose distribution is determined for a posterior-anterior vertical x-ray beam incident on a 20 cm thick PMMA block placed over the patient table using the DTS and XR-QA2 Gafchromic film. For the current investigation, we extend the convolution technique using the DTS to include convolution of the forward scatter kernel for the dose distribution determination for x-ray beams intersected by the patient table. The accuracy of the dose calculated by DTS using the scatter dose convolution kernels are validated for uniform x-ray fields as well as non-uniform x-ray fields generated using beam-shaping devices like region of interest (ROI) attenuation filters.

2.3.1 Uniform X-Ray Fields for PMMA Phantom

A 20 cm thick PMMA block was placed on the patient table with the Gafchromic film positioned between the phantom entrance surface and table. The phantom entrance was placed 54 cm from the x-ray source and was irradiated with a beam energy of 80 kVp and a field of view (FOV) of nearly 16 cm. The DTS was attached to the angio machine through a digital CAN (Control Area Network) bus interface and was used to calculate the skin dose distribution. The dose at the PMMA entrance surface was comprised of primary radiation, backscattered radiation from the phantom and the forward scattered radiation from the table. The normalized backscatter and the forward scatter PSFn convolution was incorporated in DTS and has been used for the skin dose determination for the validation procedure. A line dose profile was obtained from the Gafchromic film along the midline perpendicular to the anode-cathode direction using the film-dose response curve. This dose profile was compared to the corresponding dose profile obtained from the DTS phantom model.

2.3.2 Non-Uniform X-Ray Fields for PMMA Phantom

The entrance dose distribution over the PMMA phantom was modified using an ROI beam attenuator, an inherent beam shaping device installed in the tube collimator assembly of the Toshiba Infinix Angio machine.^{9, 10} The ROI attenuator was inserted at the center of the x-ray field, and the phantom was irradiated with the x-rays using the identical setup as described in section 2.3.1. The resultant dose distribution was comprised of a high dose circular region of interest and a low dose periphery due to the dose intensity attenuation by the ROI beam attenuator. The dose comparison and the validation procedure was performed as indicated in section 2.3.1.

3. RESULTS & DISCUSSIONS

3.1 Forward Scatter PSFn’s and Dose Distributions for Water

Table 1 indicates the patient table transmission factors measured for the beam qualities obtained by changing the beam kVp and the corresponding calculated water thickness with equivalent transmission. The transmission factors range from 76 % for a 2.32 mm Al HVL (60 kVp) narrow x-ray beam up to nearly 80 % for the 4.24 mm Al HVL (110 kVp) x-ray beam. The equivalent calculated water thickness for the corresponding x-ray beam qualities ranged from 7.76 mm to 8.77 mm, respectively.

Table 1.

Table transmission as a function of beam energy and the equivalent water thickness.

Beam Energy (kVp)	Half Value Layer (mm Al)	Table Transmission	Water Thickness (mm)
60	2.32	0.764	7.76
80	3.10	0.775	8.60
100	3.84	0.785	9.04
110	4.24	0.797	8.77

Fig 1 shows the normalized forward scatter PSFn’s determined for the beam qualities listed in Table 1. The scatter dose spreading out to a radial distance of 10 mm with respect to the central voxel is shown in the image. The normalized scatter dose intensity at the central voxel indicates an averaged dose across 1×1×0.5 mm³. The peak scatter to primary intensity is 0.0212, 0.0207, 0.0204, and 0.0216 for 60 kVp, 80 kVp, 100 kVp and 110 kVp pencil beams, respectively. The integral of the PSFn over the entrance skin indicates the cumulative normalized forward scatter dose intensity received by the skin for the pencil beam, which was 0.171, 0.186, 0.190, 0.183 for 60 kVp, 80 kVp, 100 kVp and 110 kVp pencil beams, respectively. The pencil beam forward scatter radial spread up to 25 mm relative to the central voxel which constitutes a 50×50 PSFn kernel is used for the convolution to improve the computational performance.

Fig.1.

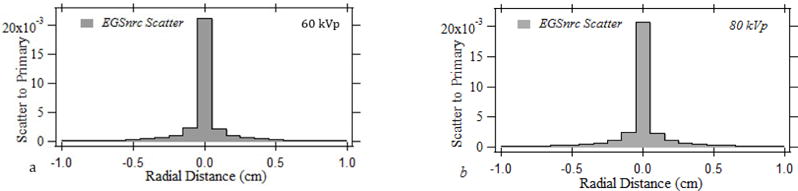
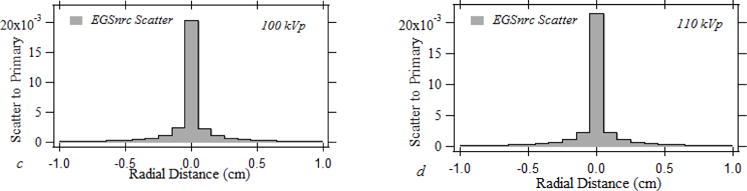
The normalized forward scatter distribution for a simulated 1×1 mm pencil beam for (a) 60 kVp, (b) 80 kVp, (c) 100 kVp, (d) 110 kVp.

Fig. 2. shows the primary normalized forward scatter dose distribution obtained for 80 kVp (3.1 mm of Al HVL) for x-ray field sizes of 13.5 × 13.5 cm, 9 × 9 cm and 5.5 × 5.5 cm. The beam quality was chosen due to it’s close proximity to the x-ray beams used during neuro interventions. Shown in the figure are the forward scatter distributions obtained through convolution of the PSFn over the primary dose distribution and through the scatter to primary distributions calculated using full-field EGSnrc Monte Carlo simulations. The primary dose distribution for the convolution is calculated using transmission measurements by the 0.6 cc Farmer ion chamber and the heel effect profile measured by Gafchromic film as indicated in section 1.2.1. The forward scatter to primary distributions for a 5.5×5.5 cm FOV, 9×9 cm FOV and 13.5×13.5 cm FOV are shown in fig 2 (a), (b), and (c) respectively. The dose distribution is calculated beyond the collimation as shown in fig 2. The peak scatter is observed to increase from 14.7 % for the 5.5×5.5 cm FOV to 17.1 % for the 9×9 cm and 18.2 % for the 13.5×13.5 cm field. The convolution-calculated peak forward scatter dose was within ± 1 % for the 5×5 cm field, ± 4 % for the 9×9 cm field and ± 6 % for the 13.5×13.5 cm field compared to the dose determined with EGSnrc Monte Carlo. The relative increment in the scatter dose difference with the increase in FOV could be attributed to the limited 50 × 50 dimension of the PSFn as indicated in section 3.1. The forward scatter tail of the 1×1 mm pencil beam spreads beyond a radius of 25 mm from the central voxel. The dose distribution calculated using PSFn convolution is obtained at an interval of 1 mm, comparable with the size of the 1 × 1 mm convolution kernel elements, whereas the EGSnrc dose distribution is obtained every 5 mm, which is consistent with the MC voxel size.

Fig.2.

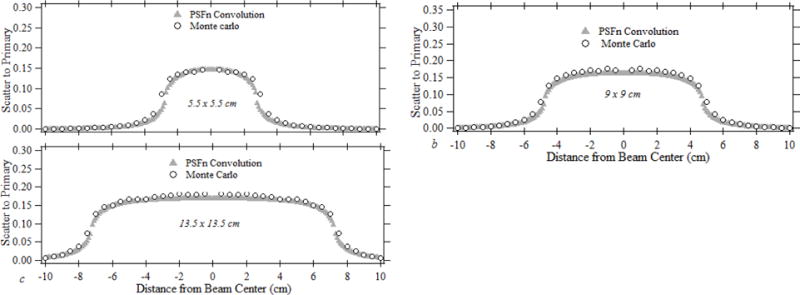
The normalized forward scatter distribution for (a) 5.5×5.5 cm, (b) 9×9 cm and (c) 13.5×13.5 cm fields for an 80 kVp x-ray beam.

Fig. 3 illustrates the forward scatter dose distribution obtained for a changing beam quality with a fixed 13.5×13.5 cm field size. Fig 3 (a), 3 (b), and 3 (c) present the normalized forward scatter dose distribution obtained for 60 kVp (2.32 mm Al HVL), 100 kVp (3.84 mm Al HVL) and 110 kVp (4.24 mm Al HVL) beams with an additional 1.8 mm Al beam filtration. The dose distributions obtained using the forward scatter PSFn convolution and the EGSnrc Monte Carlo are shown in each graph. The peak scatter dose intensity is 15.4 % for 60 kVp, 18.4 % for 100 kVp and 17.3 % for 110 kVp with respect to the primary dose. The convolution-calculated peak scatter was within ±2.2 % for 60 kVp, ±6 % for 80 kVp, ± 4.2% for 100 kVp and ± 2 % for 110 kVp compared to the dose determined with full-field EGSnrc Monte Carlo.

Fig. 3.

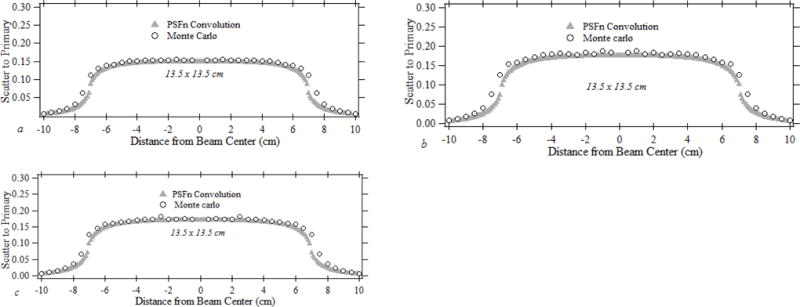
The normalized forward scatter distribution for (a) 60 kVp, (b) 100 kVp and (c) 110 kVp x-ray beams with 13.5 cm square FOV

3.2 Forward Scatter PSFn’S and Dose Distributions for Patient Table

Fig. 4 illustrates the normalized forward scatter PSF obtained for the patient table. The peak scatter intensity normalized to the primary dose for the 1×1 mm pencil beam was 1.8 %. The integral of the PSFn was observed to be 0.199 that is within 7 % of that measured for the table-equivalent water for 80 kVp tube potential. The scatter drops rapidly off the central voxel and the dose distribution up to a radial extend of 10 mm from the central voxel is shown.

Fig. 4.

Forward scatter PSFn for the patient table.

Fig. 5 shows the normalized forward scatter dose line profile obtained along the mid axis of the x-ray field. The line profile was obtained in a direction perpendicular to the anode-cathode direction and, hence, the dose profile is symmetric on either side relative to the center. The transmitted primary dose distribution and the primary dose with forward scatter dose distribution was obtained with the patient table and the Gafchromic film as described in section 2.2.2. The film was irradiated at 80 kVp (300 mAs) with an inherent beam filtration of 1.8 mm Al for nearly 25 seconds. The exposure resulted in a peak absorbed dose of nearly 30 mGy to the film with primary and scattered dose, and roughly 24 mGy to the film with the primary dose. The dose distributions measured with the Gafchromic film is plotted along with the line dose profile calculated using PSFn convolution. The scatter to primary ratio at the center of the FOV is observed to be nearly 21.3 %, which gradually decreases to approximately 12 % at the edge of the field. Previous studies has shown the relative calibration uncertainty of the film is less than 5 % for the skin dose range used for the current study.¹¹ The local variations in the film dose is due to the statistical fluctuations between scan elements due to variables like film layer defects, dust, electronic noise from scanner etc.

Fig. 5.

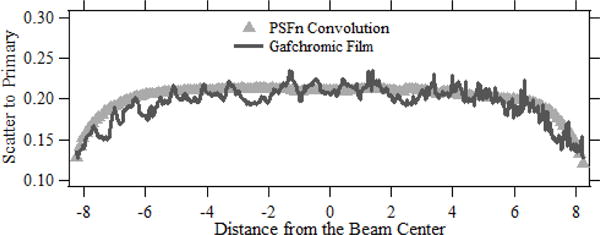
The normalized forward scatter dose profiles from the patient table for a 16.5 cm field with dose distributions obtained using convolution and Gafchromic film.

3.3 Entrance Dose Calculation for PMMA Phantom

3.3.1. For Uniform X-Ray Fields

The PMMA phantom and the Gafchromic film placed on the patient table was irradiated at 80 kVp (840 mAs) with digital angiography (DA) for nearly 70 seconds resulting in a peak dose of 174 mGy at the center of the field. The table transmitted primary dose measured using a Farmer ion chamber for the similar setup was nearly 105 mGy. Fig 6 (a) illustrates the DTS color dose map over the PMMA Model for the validation procedure with the dose-color scale and Fig. 6 (b) shows the scatter to primary line dose profile obtained from the DTS and film. The dose-color scale ranges from 0–225 mGy. The peak normalized scatter which includes the backscattered and forward scattered radiation measured with film was determined to be 1.39. The noisy spikes in the film line dose profile is caused by the local statistical fluctuations. The film dose profile coincides with the DTS dose profile predominantly in the peak dose regions. The small difference of the dose profiles towards the field edge could be due to the limited 50 × 50 sized PSFn as discussed in section 1.

Fig. 6.

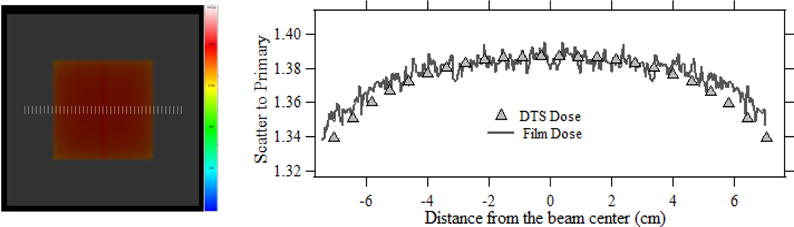
(a) The PMMA graphic model with color coded dose distribution generated by DTS for a plain field. (b) Comparison of forward scatter dose measured with the film to that calculated using DTS by convolution.

3.3.2. For Non-Uniform X-Ray Fields

Fig. 7(a) shows the DTS dose map for a PMMA block phantom exposed to a non-uniform x-ray field generated using an ROI beam attenuator, and fig. 7 (b) shows the mid-axis line dose profiles of scatter to primary ratio acquired from the DTS PMMA graphic and Gafchromic film. The scatter dose included both forward scatter radiation originating from the table and backscattered radiation from the phantom. The dose profiles were obtained along the scale bars in the DTS graphic as shown in fig 7(a). The exposure was delivered with the set up indicated in section 3.3.1 for nearly 60 seconds, that resulted in a peak skin dose of nearly 119 mGy’s which was within the sensitivity range of the XR-QA2 film. The peak total scatter to primary ratio at the center of the ROI calculated with the DTS was 1.10, within ± 2 % of that measured using the film.

Fig. 7.

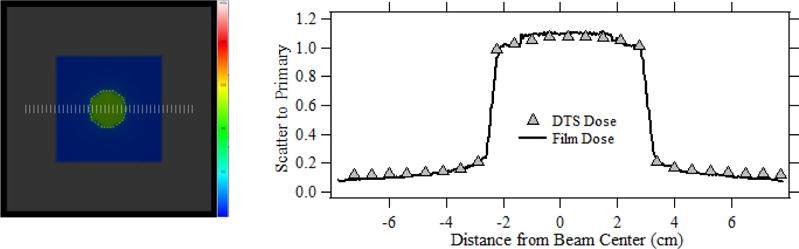
(a) The DTS color dose map showing the x-ray field with an ROI attenuator inserted at the center of field. (b) comparison of DTS and film normalized scatter dose profiles for the x-ray field shown in fig. (a).

4. CONCLUSIONS

An accurate method for the estimation of the forward scatter dose distribution from the patient table is devised. Calculations were performed for different x-ray field sizes and varying beam quality for a table-equivalent water phantom with full-field EGSnrc MC and compared with the dose calculated using PSFn convolutions, with good agreement. The convolution method to estimate the forward scatter dose is validated for the patient table using Gafchromic film for a uniform x-ray field. The suitability of the PSFn convolution incorporated in the DTS to determine the skin dose distribution was also evaluated for homogenous phantoms, for both uniform and non-uniform x-ray fields. The DTS convolution-calculated skin dose agreed well with that measured using Gafchromic film.